2. Details of the Effect
“In the Hamiltonian of the relative (internal) motion for the hydrogen atom in a magnetic field
B, the potential energy has the form (see, e.g., Schmelcher–Cederbaum paper [
11], Equation (6))
where
K is the pseudomomentum, M is the mass of the hydrogen atom, c is the speed of light, and e is the electron charge. We follow paper [
11] in choosing e < 0; we also note that in paper [
11] it was set at c = 1. In Equation (1), (
B ×
K)
r stands for the scalar product (also known as the dot product) of vector
r and vector (
B ×
K)”.
The first term in Equation (1) is the diamagnetic one; the second term is due to the motional Stark effect; the third term represents the Coulomb interaction.
In paper [
11], its authors chose the following configuration:
B = (0, 0, B),
K = (0, K, 0), where K > 0. Here, we use the same choice. Then, Equation (1) can be written as
It is important to emphasize the following. The general expression for the pseudomomentum is (see, e.g., paper [
2]):
where
re and
rp are the radius vectors of the electron and proton, respectively. For the values of K~10
2 a.u. (a.u. standing for atomic units) or greater, the last term in the right side of Equation (3) predominates, so that the pseudomomentum characterizes electron–proton separation rather than velocity—see, e.g., papers [
1,
2]. We are interested in the values of K~10
2 a.u. or greater, so that in view of the predominance of the last term in the right side of Equation (3), it is clear that vector
K is practically perpendicular to the magnetic field
B. Therefore, the choice of
B = (0, 0, B),
K = (0, K, 0) is appropriate. (In any case, it is the component of
K perpendicular to
B that causes the appearance of the second potential well and is responsible for the rich physics of the system.)
Physically, the quantity V
s is the scaled potential energy. Then, Equation (2) takes the form:
With respect to the y- and z-coordinates, the minimum of the potential occurs at y = z = 0 [
9]. Then, we calculate
Along the line of y = z = 0, Equation (6) simplifies to:
where, as usual, sign x = −1 for x < 0 or sign x = 1 for x > 0.
The right side of Equation (7) vanishes if
For x > 0, Equation (8) reads as
It is easy to see that there are no positive roots of this equation.
For x < 0, Equation (8) reads as
which is mathematically equivalent to Equation (8b) from paper [
9] (where their authors set e = −1 and c = 1.
“The polynomial in Equation (10) has either two or zero real roots. Thus, the total number of roots of Equation (8) is also either two or zero—since there are no positive roots.
We note in passing that the authors of paper [
9] erroneously stated that ∂V/∂x, calculated at y = z = 0, can have three real roots. Their error originates from the fact that they missed the factor (sign x) in the corresponding equation.
We introduce the scaled magnetic field b and the scaled pseudomomentum k, as follows
where b has the dimension of cm
−3/2 and k has the dimension of cm
−1/2. Below, while using particular numerical values of b and k, we omit the dimensions for brevity”.
In the scaled notations, Equation (10) reads as
This cubic equation has the following discriminant:
Then, Equation (12) has two real negative roots different from each other.
We remind that the atomic unit, in which K
min is measured in the left side of Equation (15), is the atomic unit of any linear momentum—it is equal to
Below is the Cardano’s solution for the two real roots x
1 and x
2 of the cubic Equation (12):
The above expressions yield real values for x
1 and x
2 under the condition (14)—regardless of the formal appearance of the imaginary unit i in these expressions. For example, for the root x
2, this is analytically proven in
Appendix A.
For sufficiently large values of the pseudomomentum K, such that
the expressions for the roots of Equation (12) simplify to:
Under condition (14), the scaled potential energy V
s reaches the maximum at x = x
2 and exhibits the minimum at x = x
1. At y = z = 0, the scaled potential energy V
s has the form:
Figure 1 depicts the dependence of the scaled potential energy V
s from Equation (21) on the coordinate x (in cm) for b = 1.04 × 10
9 (corresponding to B = 40,000 Tesla, such as, e.g., in the DA white dwarfs) and k = 19,200 corresponding to K = 60 a.u. (solid line). The dashed line corresponds to the energy E
top at the top of the potential barrier.
Here, we come to the central point. For hydrogen energy levels below the top of the potential barrier (E < Etop), the atomic electron is confined in a relatively narrow potential well. However, for the energy levels at or above the top of the potential barrier, the width of the potential well increases by several orders of magnitude (for sufficiently large values of the pseudomomentum K). According to the uncertainty relation, this means that, in the latter case, the spacing of the energy levels decreases by several orders of magnitude. Since these energy levels have a finite width (e.g., due to the collisional and natural broadenings), the dramatic decrease of the spacing between these energy levels creates a quasi-continuum out of them and thus causes the atomic electron to be practically free from the proton. This is equivalent to the ionization on the atom.
Moreover, the above reasoning related only to the x-coordinate. However, in reality, the location of the B-well on the x-axis corresponds in three dimensions to the saddle point. Therefore, the atomic electron, excited to the top or above the top of the potential barrier, would get far away from the proton through the saddle point—even regardless of the one-dimensional considerations of the sudden decrease of the spacing of the energy levels and the formation of the quasi-continuum. In other words, in the three-dimensional picture, our main conclusion of the ionization of the atom (when the atomic electron is excited at or above the top of the potential barrier) would be actually reinforced.
Thus, this mechanism (the lowering of the top of the potential barrier) limits the number of the discrete energy levels E
n by the condition:
At the top of the potential barrier (i.e., at x = x
2), under the condition (19) the scaled potential energy is
The corresponding value of the non-scaled potential energy is
From Formulas (22) and (24), it is easy to obtain the following expression for the maximum principal quantum number n
max,B (caused by the presence of the B-well):
where a
0 is the Bohr radius. The practical formula for n
max,B is:
where B is in Tesla and K is in atomic units (a.u.).
Hydrogen atoms moving across the magnetic field experience the Lorentz field
ELT, whose average value is E
LT = Bv
T/c, where v
T = (2T/M)
1/2 is the atomic thermal velocity. In papers [
12,
13], it was shown that, under the Lorentz field, the principal quantum number n
max,L of the last observable hydrogen line (while disregarding the effect of the B-well) is:
(the letter L in the subscript stands for Lorentz field).
By the way, in papers [
12,
13], it was shown that the average Lorentz field exceeds the most probable ion microfield E
i when the magnetic field B exceeds the following critical value: B
c(Tesla) = 4.69 × 10
−7N
e(cm
−3)
2/3/[T(K)]
1/2. Thus, under this condition, the effect of the ion microfield on the number of observable hydrogen lines can be neglected.
It is worth mentioning that the simultaneous usage of the average velocity v
T and a certain value of the pseudomomentum K is legitimate. In paper [
14], it was stated that the dependence of the energy E on the pseudomomentum (the latter being denoted as
P in paper [
14]) allows one to determine the
mean velocity of the atom for a given (i.e., fixed)
P:
By comparing Equations (25) and (27), it is easy to find out that the presence of the B-well controls the number of the observable hydrogen lines (i.e., n
max,B < n
max,L) for sufficiently large values of the pseudomomentum
The practical formula for the critical pseudomomentum value is:
where K
crit is in a.u., T is in eV, and B is in Tesla.
Now, let us consider some examples. For B = 5 Tesla, Equation (25) yields nmax,B = 15 for K = 100 a.u. or nmax,B = 11 for K = 300 a.u. We note that B = 5 Tesla can be created in many labs—such magnets are used, e.g., in contemporary tokamaks.
Superconductive magnets, such as, e.g., the MIT fusion magnet, create B = 20 Tesla [
15]. For B = 20 Tesla, Equation (25) yields n
max,B = 10 for K = 100 a.u. or n
max,B = 8 for K = 300 a.u.
The atmospheres of DA white dwarfs (i.e., of the white dwarfs emitting hydrogen lines) are characterized by magnetic fields B from 100 to 100,000 Tesla. For B = 2000 Tesla, Equation (25) yields nmax,B = 4 for K = 50 a.u.
In all of the above examples, the principal quantum number of the last observable hydrogen line is controlled by nmax,B < nmax,L.
Thus, the primary effect of the diamagnetic term in the Hamiltonian is the creation of the B-well causing the decrease of the number of observable hydrogen lines. Compared to this primary effect, other effects of the diamagnetic term—those discussed in paper [
16]—are just minor, secondary outcomes.
Our results open up an avenue for the experimental determination of the pseudomomentum K. Indeed, from Equation (25) one gets:
Thus, from the experimental values of the magnetic field B and the number nmax,B of the last observable hydrogen line, it is possible to deduce the value of the pseudomomentum by using Equation (29). This is the first proposed method for the experimental determination of the pseudomomentum—to the best of our knowledge.
We remind again that for K~102 a.u. or greater, i.e., for the values of K considered in the present paper, from measuring the atomic velocity it is impossible to deduce the value of the pseudomomentum. At this range of values, K is controlled by the vector product of the magnetic field B and the electron proton separation (re − rp)—see Equation (3) and the paragraph after Equation (3). Therefore, for this range of K, there is no other method to measure K, except the method we suggest.
Now, we analyze the accuracy of Equations (25) and (29) obtained using the asymptotic result for the root x
2 from Equation (20) valid for relatively large values of the pseudomomentum (such that k >> b
1/3). For this purpose, we use the following exact expression for the root x
2 in the trigonometric form, which is more compact than the corresponding exact result (18) and does not contain the imaginary unit i (see, e.g., handbooks [
17,
18])
where
Then, the scaled energy at the top of the potential barrier becomes:
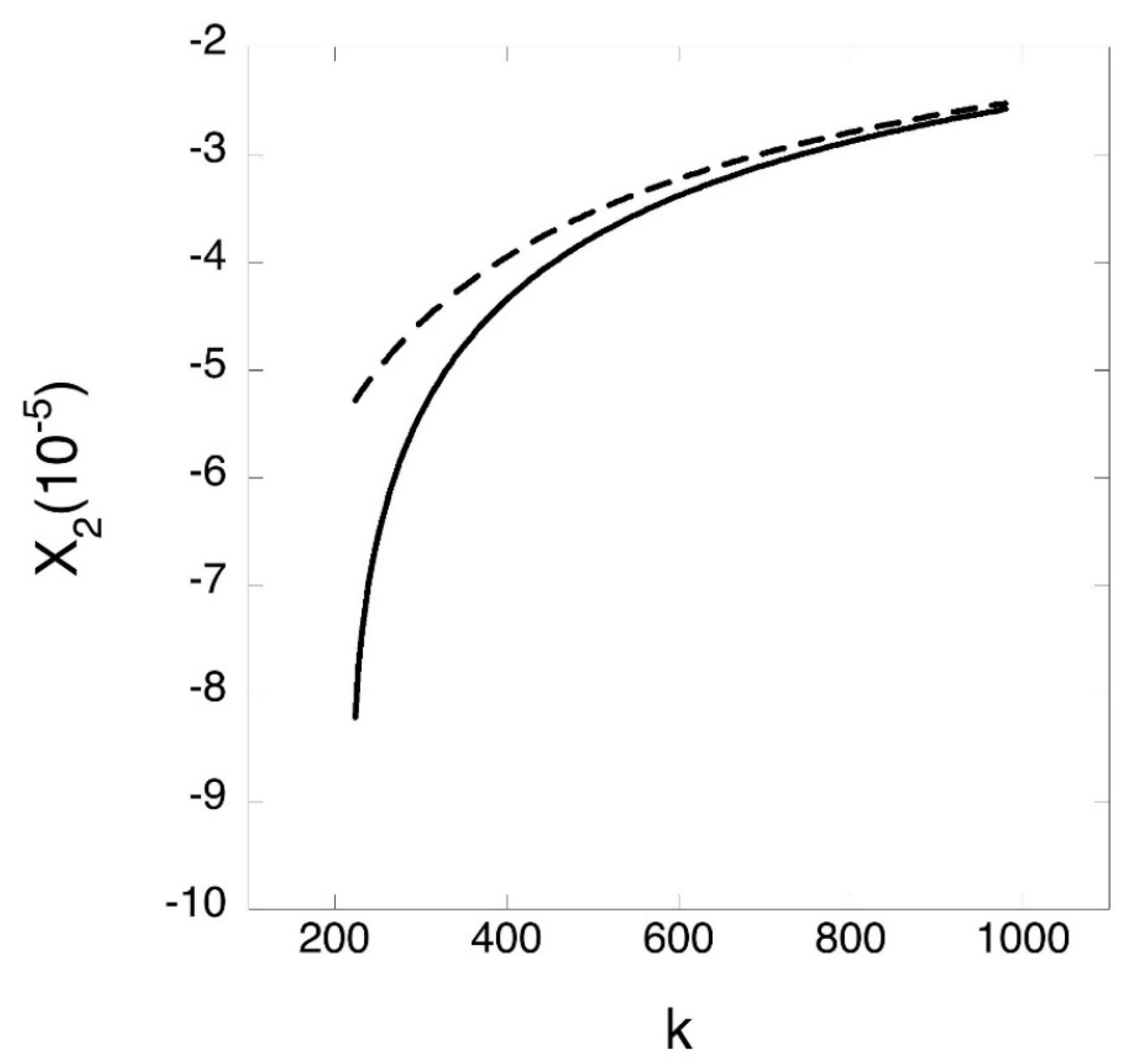
Figure 2 presents the coordinate x
2 of the top of the potential barrier versus the scaled pseudomomentum k for the scaled magnetic field b = 1.3 × 10
6 (corresponding to B = 5 Tesla). The solid line is the exact result from Equation (32), the dashed line—the asymptotic result from Equation (20). It is seen that for values of k close to k
min given by Equation (14), k
min being equal to 206 for b = 1.3 × 10
6, there is a significant difference between the exact and asymptotic results, but the difference becomes practically negligible for values of k just a few times greater than k
min.
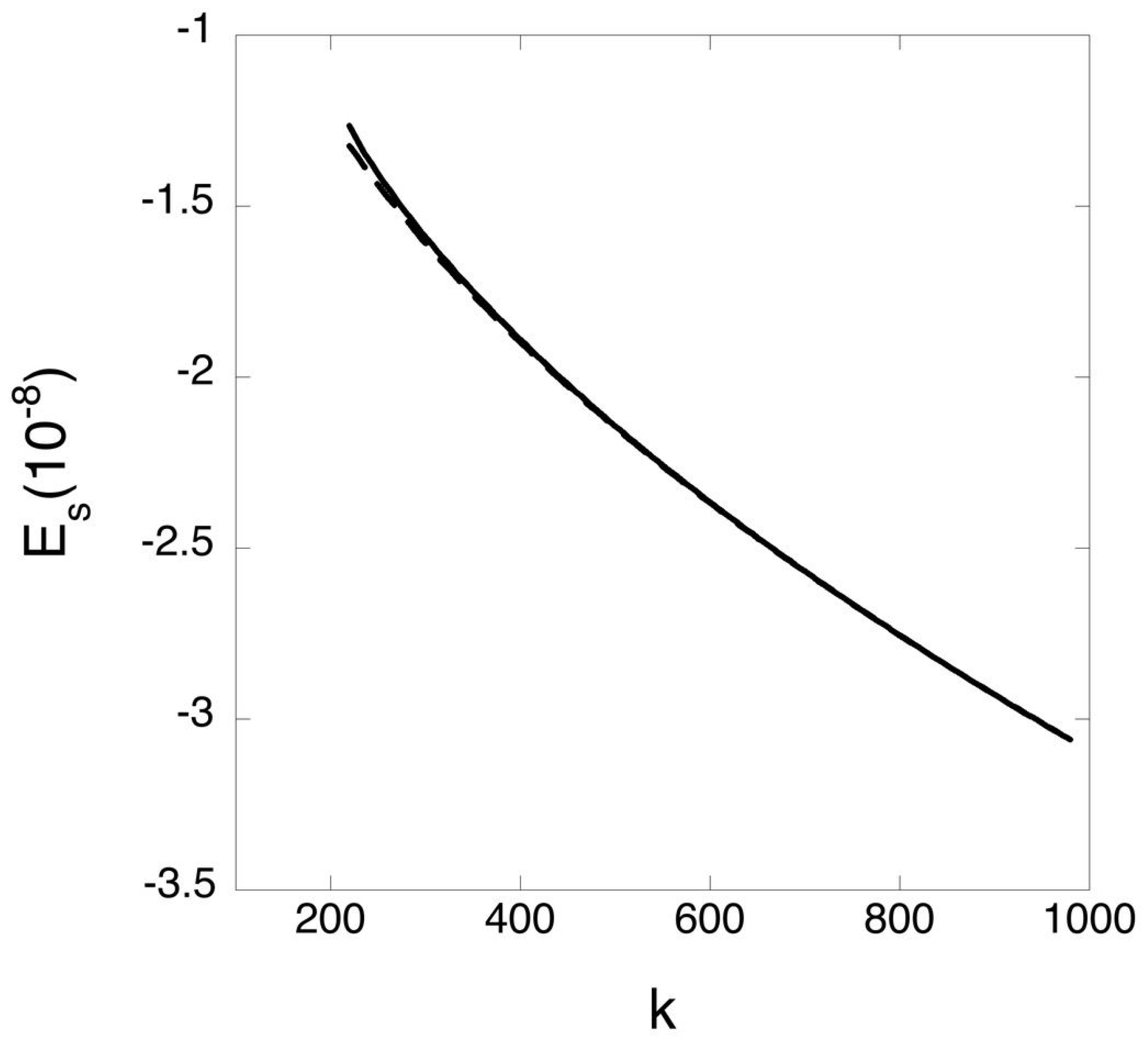
Figure 3 shows the scaled energy E
s = V
s,top at the top of the potential barrier versus the scaled pseudomomentum k for the scaled magnetic field b = 1.3 × 10
6 (corresponding to B = 5 Tesla). The solid line is the exact result from Equation (34), the dashed line—the asymptotic result from Equation (23). It is seen that even for values of k close to k
min, the difference is practically negligible. Thus, Equations (25) and (29), obtained by using the asymptotic value of V
s,top from Equation (23), yield the sufficient accuracy.