Adaptive DC-Link Voltage Control for 22 kW, 40 kHz LLC Resonant Converter Considering Low-Frequency Voltage Ripple
Abstract
1. Introduction
2. Design Specifications
2.1. PEM Electrolyzer Specifications
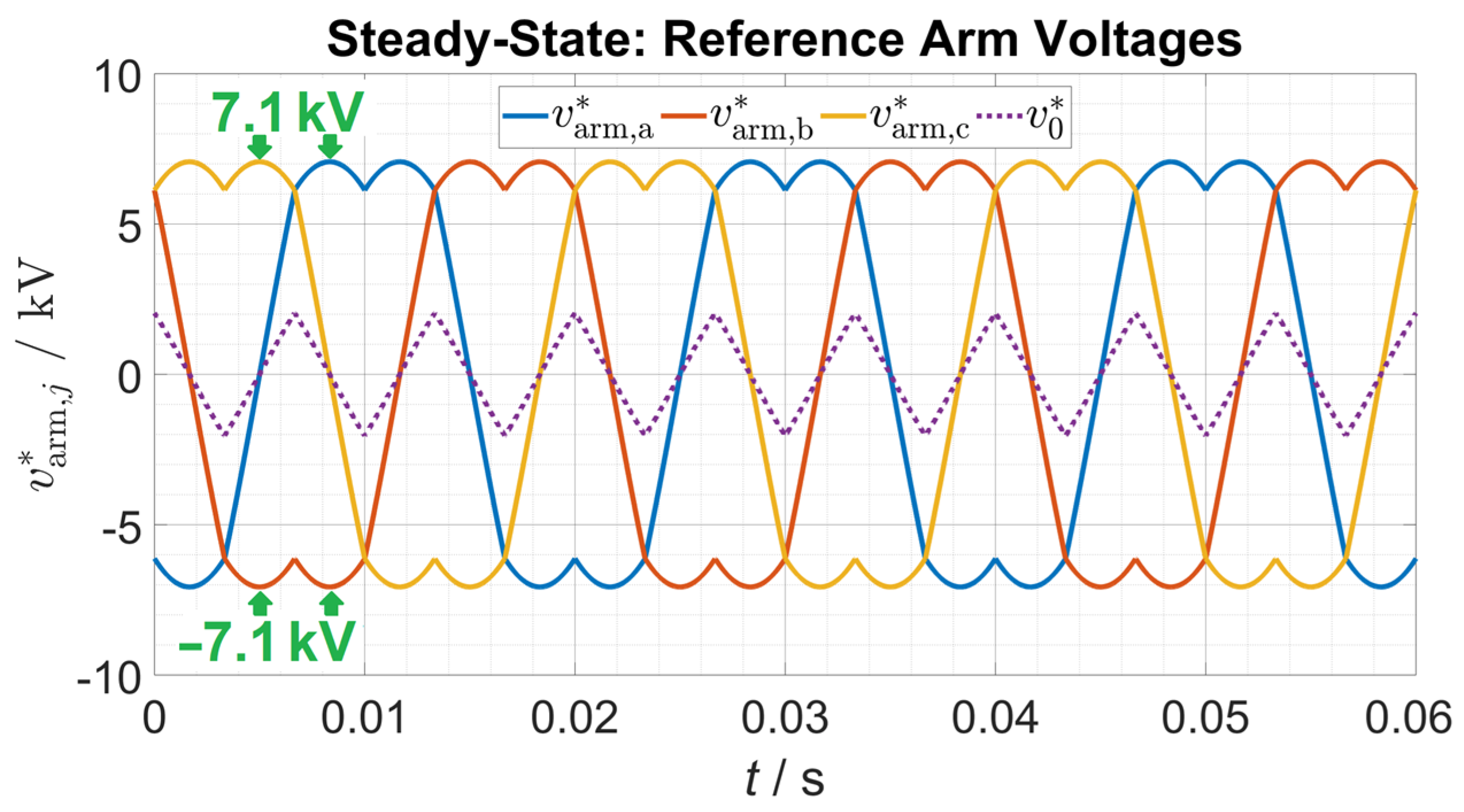
2.2. DC-Link Voltage Specifications
2.3. Module Power Specifications
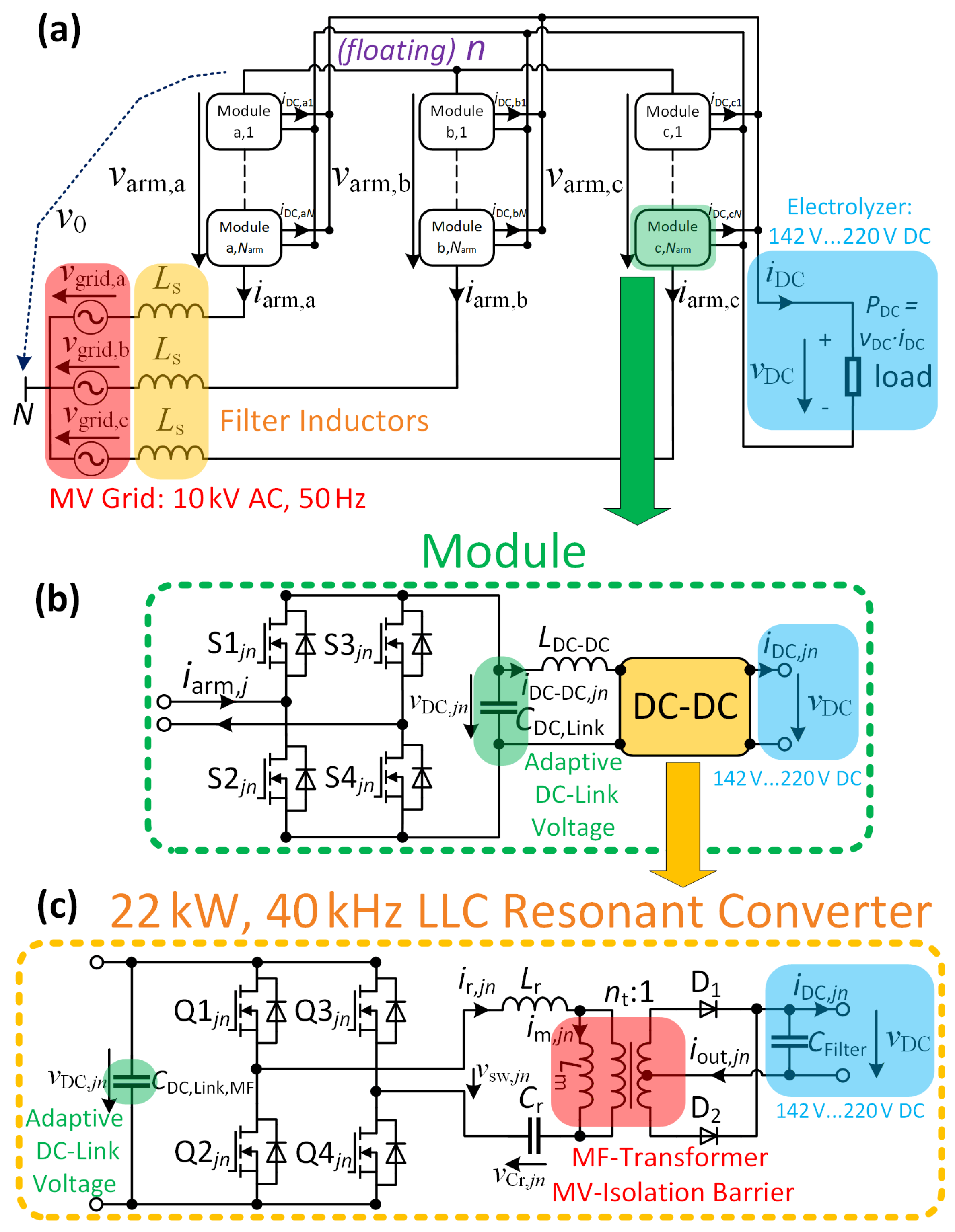
3. Design of the 22 kW LLC Resonant Converter
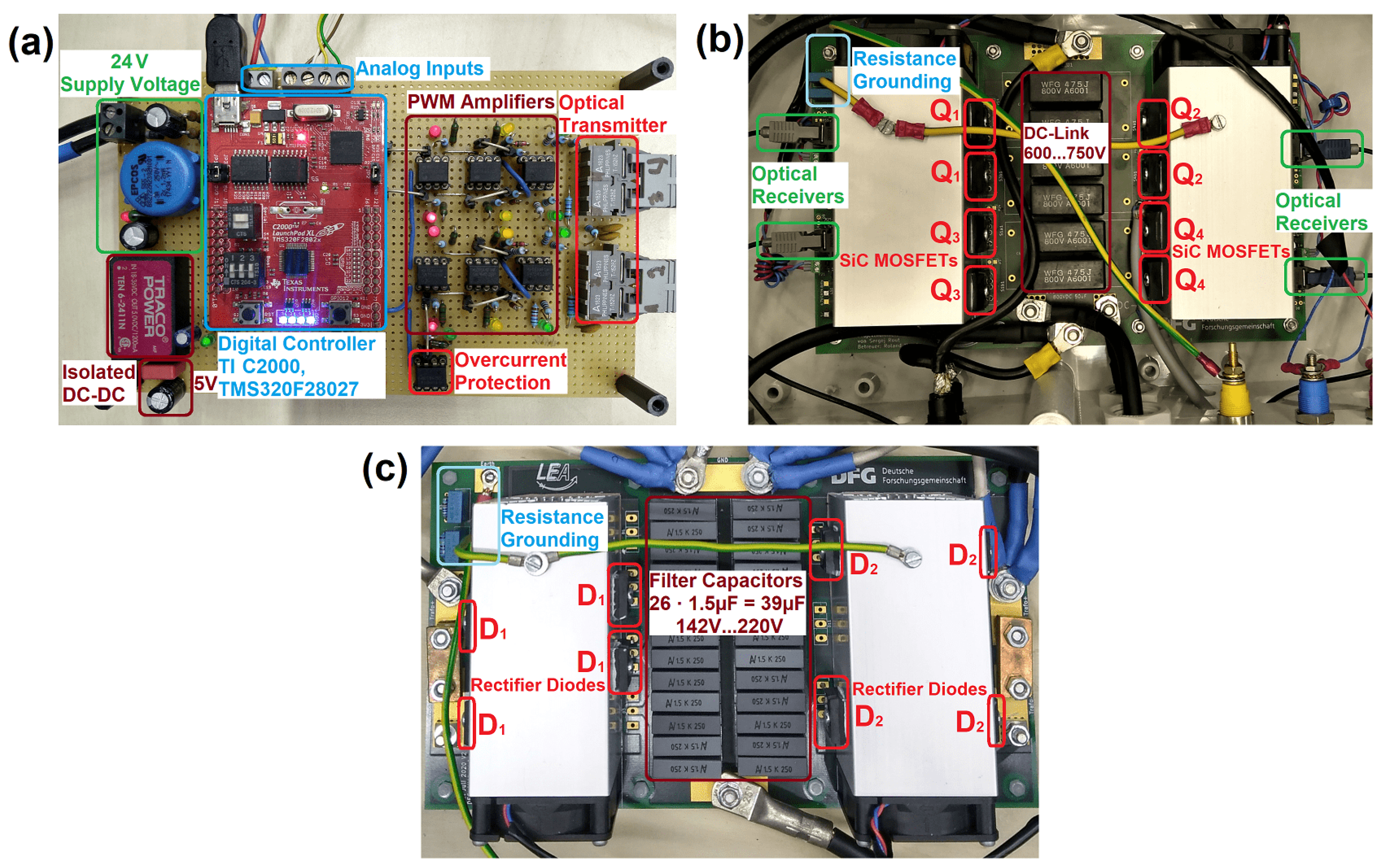
3.1. Components of the SiC Inverter and Rectifier Stage
3.2. Components of the Resonant Tank
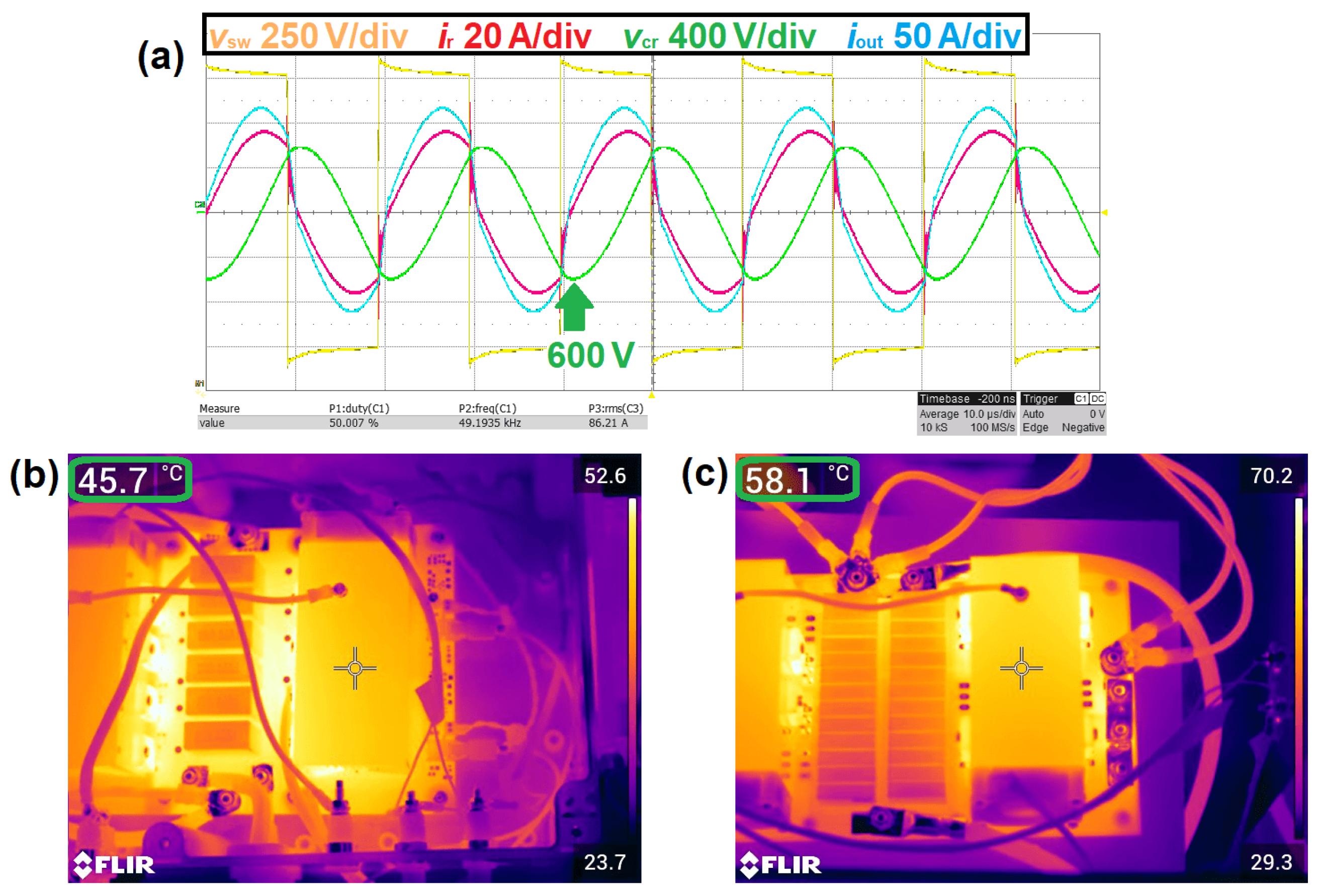
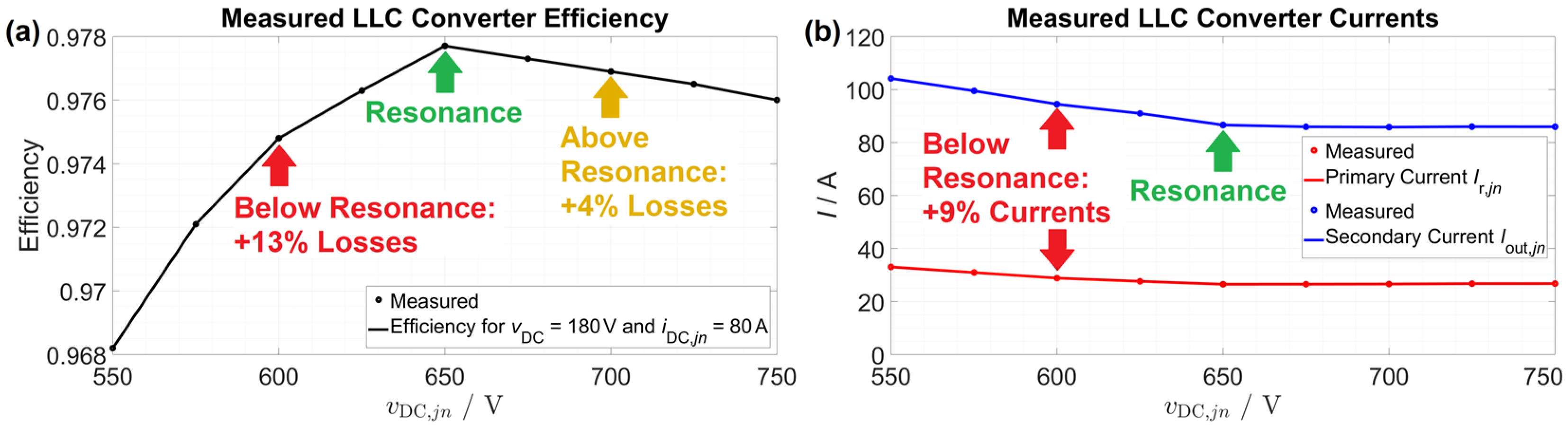
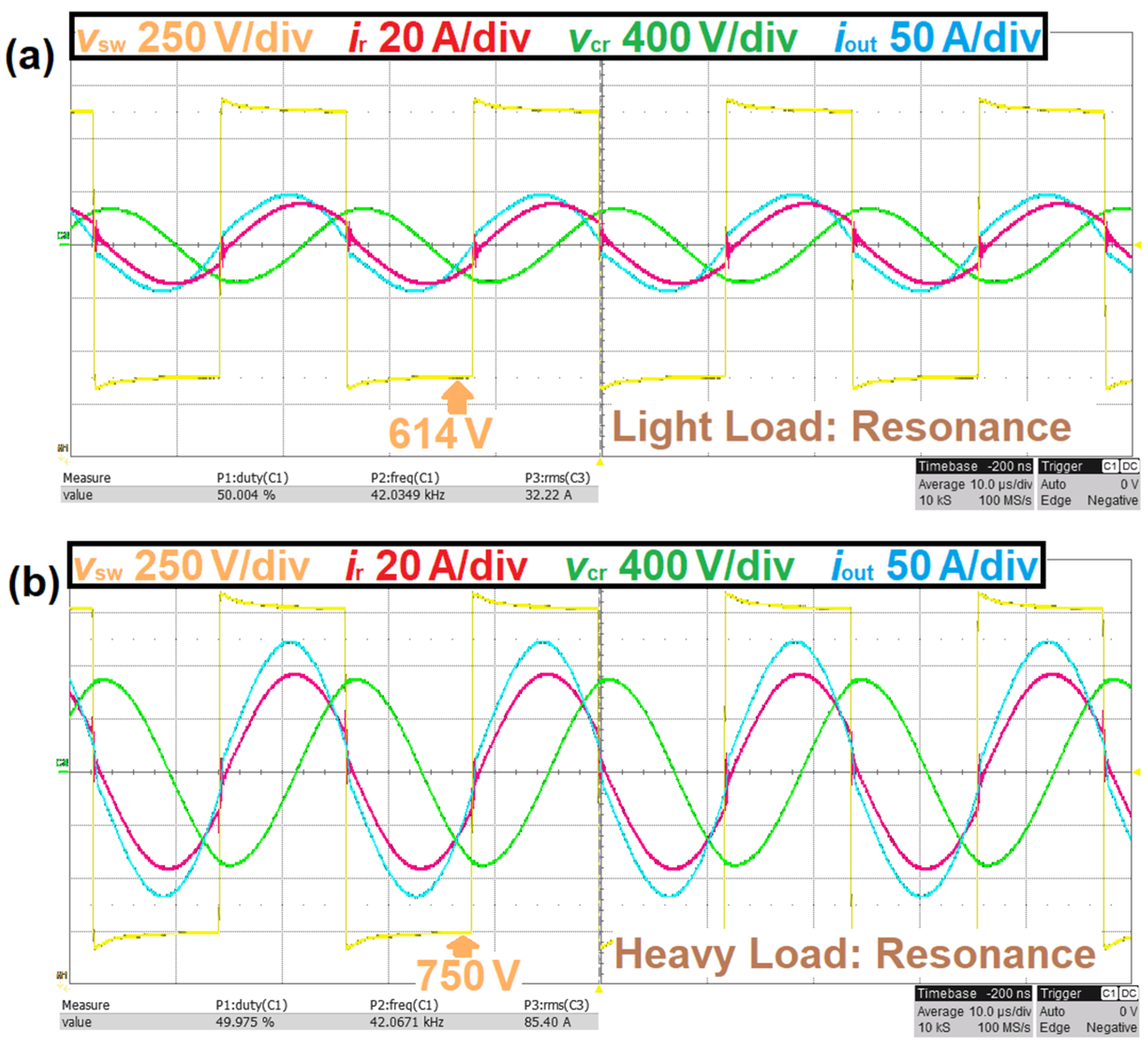
4. Experimental Results
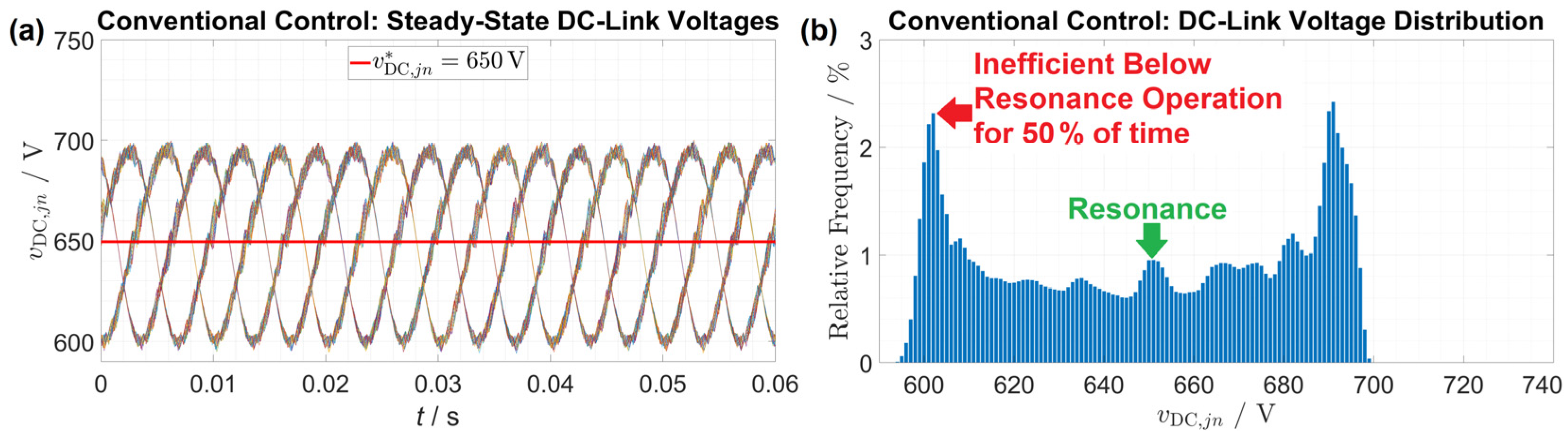
Conventional Method to Determine DC-Link Voltage
5. Simulation Results
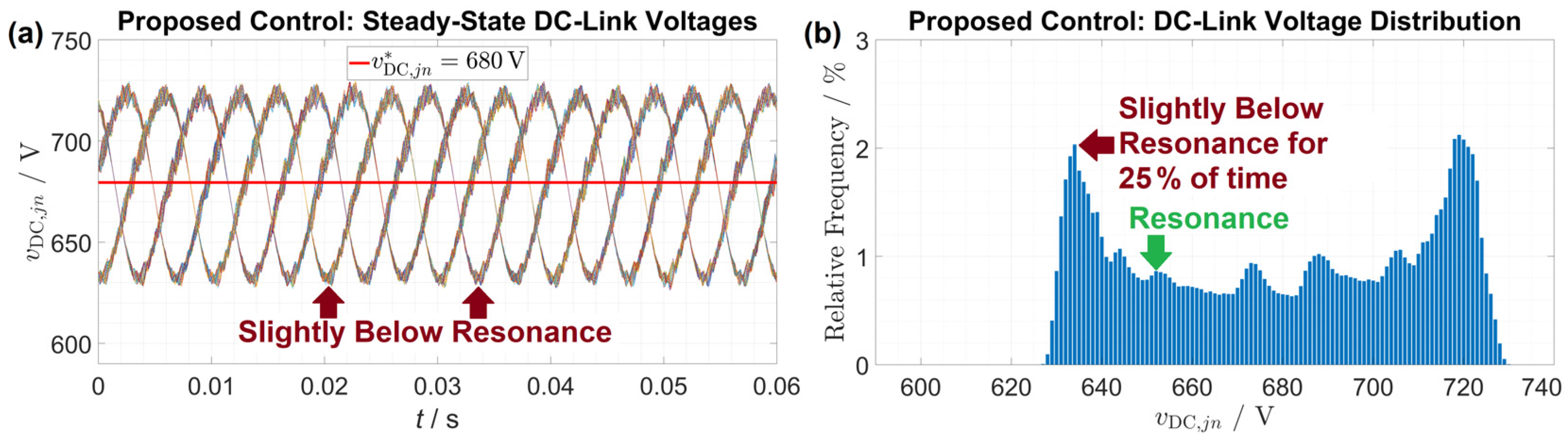
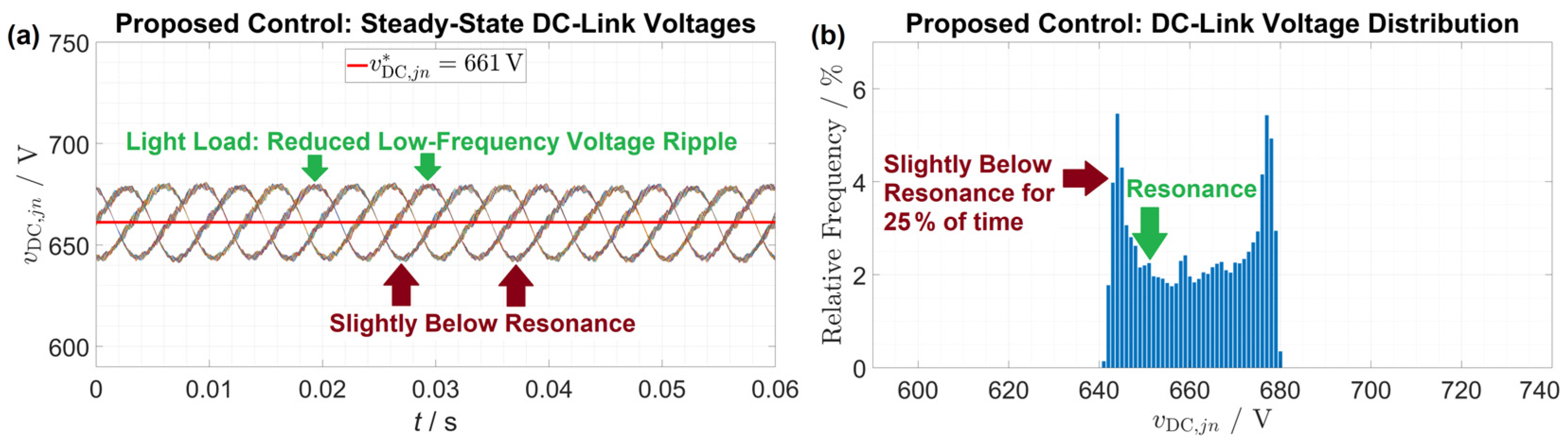
Proposed Method to Determine DC-Link Voltage
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chandesris, M.; Médeau, V.; Guillet, N.; Chelghoum, S.; Thoby, D.; Fouda-Onana, F. Membrane degradation in PEM water electrolyzer: Numerical modeling and experimental evidence of the influence of temperature and current density. Int. J. Hydrogen Energy 2015, 40, 1353–1366. [Google Scholar] [CrossRef]
- Vargas-Ferrer, P.; Álvarez-Miranda, E.; Tenreiro, C.; Jalil-Vega, F. Integration of high levels of electrolytic hydrogen production: Impact on power systems planning. J. Clean. Prod. 2023, 409, 137110. [Google Scholar] [CrossRef]
- Yodwong, B.; Guilbert, D.; Phattanasak, M.; Kaewmanee, W.; Hinaje, M.; Vitale, G. AC-DC Converters for Electrolyzer Applications: State of the Art and Future Challenges. Electronics 2020, 9, 912. [Google Scholar] [CrossRef]
- Solanki, J. High Power Factor High-Current Variable-Voltage Rectifiers. Ph.D. Thesis, Paderborn University, Paderborn, Germany, 2015. [Google Scholar]
- Chen, M.; Chou, S.-F.; Blaabjerg, F.; Davari, P. Overview of Power Electronic Converter Topologies Enabling Large-Scale Hydrogen Production via Water Electrolysis. Appl. Sci. 2022, 12, 1906. [Google Scholar] [CrossRef]
- Rothmund, D.; Guillod, T.; Bortis, D.; Kolar, J.W. 99% Efficient 10 kV SiC-Based 7 kV/400 V DC Transformer for Future Data Centers. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 753–767. [Google Scholar] [CrossRef]
- Li, Z.; Hsieh, Y.-H.; Li, Q.; Lee, F.C.; Ahmed, M.H. High-Frequency Transformer Design with High-Voltage Insulation for Modular Power Conversion from Medium-Voltage AC to 400-V DC. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 5053–5060. [Google Scholar]
- Aghoy, A.R.; Ebrahimi, J.; Pahlevani, M.; Bakhshai, A. Feasibility Review of Multilevel Converters in Electric Vehicle Chargers. In Proceedings of the 2024 IEEE Canadian Conf. on Electrical and Computer Engineering (CCECE), Kingston, ON, Canada, 6–9 August 2024; pp. 495–500. [Google Scholar]
- Koponen, J.; Ruuskanen, V.; Kosonen, A.; Niemelä, M.; Ahola, J. Effect of Converter Topology on the Specific Energy Consumption of Alkaline Water Electrolyzers. IEEE Trans. Power Electron. 2019, 34, 6171–6182. [Google Scholar] [CrossRef]
- Ruuskanen, V.; Koponen, J.; Kosonen, A.; Niemelä, M.; Ahola, J.; Hämäläinen, A. Power quality and reactive power of water electrolyzers supplied with thyristor converters. J. Power Sources 2020, 459, 228075. [Google Scholar] [CrossRef]
- Unruh, R.; Böcker, J.; Schafmeister, F. Experimentally Verified 22 kW, 40 kHz LLC Resonant Converter Design with new Control for a 1 MW Cascaded H-Bridge Converter. In Proceedings of the 2024 Energy Conversion Congress & Expo Europe (ECCE Europe), Darmstadt, Germany, 2–6 September 2024. [Google Scholar]
- Kurokawa, F.; Murata, K. A new fast digital P-I-D control LLC resonant converter. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011. [Google Scholar]
- Kim, J.; Kim, C.; Kim, J.; Lee, J.; Moon, G. Analysis on Load-Adaptive Phase-Shift Control for High Efficiency Full-Bridge LLC Resonant Converter Under Light-Load Conditions. IEEE Trans. Pow. Electr. 2016, 31, 4942–4955. [Google Scholar] [CrossRef]
- Lumbreras, D.; Gálvez, E.; Collado, A.; Zaragoza, J. Trends in Power Quality, Harmonic Mitigation and Standards for Light and Heavy Industries: A Review. Energies 2020, 13, 5792. [Google Scholar] [CrossRef]
- Lu, B.; Liu, W.; Liang, Y.; Lee, F.C.; van Wyk, J.D. Optimal design methodology for LLC resonant converter. In Proceedings of the 21th Annual IEEE Applied Power Electronics Conference and Exposition, APEC ’06, Dallas, TX, USA, 19–23 March 2006. [Google Scholar]
- Rivera, S.; Wu, B.; Kouro, S.; Wang, H.; Zhang, D. Cascaded H-bridge multilevel converter topology and three-phase balance control for large scale photovoltaic systems. In Proceedings of the 2012 3rd IEEE International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Aalborg, Denmark, 25–28 June 2012. [Google Scholar]
- Unruh, R.; Schafmeister, F.; Böcker, J. Zero-Sequence Voltage Reduces DC-Link Capacitor Demand in Cascaded H-Bridge Converters for Large-Scale Electrolyzers by 40%. In Proceedings of the 2022 24th European Conference on Power Electronics and Applications (EPE’22 ECCE Europe), Hanover, Germany, 5–9 September 2022. [Google Scholar]
- Langbauer, T.; Menzi, D.; Marugg, V.; Vollmaier, F.; Azurza, J.; Kasper, M.; Kolar, J.W. Third-Harmonic-Type Modulation Minimizing the DC-Link Energy Storage Requirement of Isolated Phase-Modular Three-Phase PFC Rectifier Systems. IEEE Access 2023, 11, 34359–34371. [Google Scholar] [CrossRef]
- Xie, D.; Lin, C.; Zhang, Y.; Sangwongwanich, A.; Ge, X.; Feng, X.; Wang, H. Diagnosis and Resilient Control for Multiple Sensor Faults in Cascaded H-Bridge Multilevel Converters. IEEE Trans. Power Electron. 2023, 38, 11435–11450. [Google Scholar] [CrossRef]
- Mogorovic, M.; Dujic, D. 100 kW, 10 kHz Medium-Frequency Transformer Design Optimization and Experimental Verification. IEEE Trans. Power Electron. 2019, 34, 1696–1708. [Google Scholar] [CrossRef]
- Jung, J. Bifilar Winding of a Center-Tapped Transformer Including Integrated Resonant Inductance for LLC Resonant Converters. IEEE Trans. Power Electron. 2013, 28, 615–620. [Google Scholar] [CrossRef]
- Choi, H. Analysis and Design of LLC Resonant Converter with Integrated Transformer. In Proceedings of the APEC 07—22th Annual IEEE Applied Power Electronics Conference and Exposition, Anaheim, CA, USA, 25 February–1 March 2007; pp. 1630–1635. [Google Scholar]
- Kim, B.-C.; Park, K.-B.; Kim, C.-E.; Lee, B.-H.; Moon, G.-W. LLC Resonant Converter with Adaptive Link-Voltage Variation for a High-Power-Density Adapter. IEEE Trans. Power Electron. 2010, 25, 2248–2252. [Google Scholar] [CrossRef]
- Su, Z.-J.; Lai, Y.-S. On-line DC-link voltage control of LLC resonant converter for server power applications. In Proceedings of the 2014 IEEE Energy Conversion Congress and Exposition (ECCE), Pittsburgh, PA, USA, 14–18 September 2014. [Google Scholar]
- Wang, H.; Dusmez, S.; Khaligh, A. Maximum Efficiency Point Tracking Technique for LLC-Based PEV Chargers Through Variable DC Link Control. IEEE Trans. Ind. Electron. 2014, 61, 6041–6049. [Google Scholar] [CrossRef]
- Wang, X.; Jiang, C.; Lei, B.; Teng, H.; Bai, H.K.; Kirtley, J.L. Power-Loss Analysis and Efficiency Maximization of a Silicon-Carbide MOSFET-Based Three-Phase 10-kW Bidirectional EV Charger Using Variable-DC-Bus Control. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 880–892. [Google Scholar] [CrossRef]
- Liu, Z.; Li, B.; Lee, F.C.; Li, Q. High-Efficiency High-Density Critical Mode Rectifier/Inverter for WBG-Device-Based On-Board Charger. IEEE Trans. Ind. Electron. 2017, 64, 9114–9123. [Google Scholar] [CrossRef]
- Shih, L.-C.; Liu, Y.-H.; Luo, Y.-F. Adaptive DC-link voltage control of LLC resonant converter. CPSS Trans. Power Electron. Appl. 2018, 3, 187–196. [Google Scholar] [CrossRef]
- Li, B.; Li, Q.; Lee, F.C.; Liu, Z.; Yang, Y. A High-Efficiency High-Density Wide-Bandgap Device-Based Bidirectional On-Board Charger. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 1627–1636. [Google Scholar] [CrossRef]
- Lai, Y.-S.; Yu, M.-H. Online Autotuning Technique of Switching Frequency for Resonant Converter Considering Resonant Components Tolerance and Variation. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 2315–2324. [Google Scholar] [CrossRef]
- Pandey, R.; Singh, B. PFC-SEPIC converter-fed half-bridge LLC resonant converter for e-bike charging applications. IET Electr. Syst. Transp. 2020, 10, 225–233. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Z.; Wang, S.; Tang, J.; Ren, X.; Chen, Q. A 300-kHz 6.6-kW SiC Bidirectional LLC Onboard Charger. IEEE Trans. Ind. Electron. 2020, 67, 1435–1445. [Google Scholar] [CrossRef]
- Unruh, R.; Schafmeister, F.; Böcker, J. 11kW, 70kHz LLC Converter Design with Adaptive Input Voltage for 98% Efficiency in an MMC. In Proceedings of the 2020 IEEE 21st Workshop on Control and Modeling for Power Electronics (COMPEL), Aalborg, Denmark, 9–12 November 2020. [Google Scholar]
- Yu, G.; Choi, S. An Effective Integration of APM and OBC with Simultaneous Operation and Entire ZVS Range for Electric Vehicle. IEEE Trans. Power Electron. 2021, 36, 10343–10354. [Google Scholar] [CrossRef]
- Zhao, S.; Kempitiya, A.; Chou, W.T.; Palija, V.; Bonfiglio, C. Variable DC-Link Voltage LLC Resonant DC/DC Converter with Wide Bandgap Power Devices. IEEE Trans. Ind. Appl. 2022, 58, 2965–2977. [Google Scholar] [CrossRef]
- Unruh, R.; Böcker, J.; Schafmeister, F. An Optimized Third-Harmonic Injection Reduces DC-Link Voltage Ripple in Cascaded H-Bridge Converters up to 50% for all Power Factors. In Proceedings of the 2023 25th European Conference on Power Electronics and Applications (EPE’23 ECCE Europe), Aalborg, Denmark, 4–8 September 2023. [Google Scholar]
- Moosavi, M.; Farivar, G.; Iman-Eini, H.; Shekarabi, S.M. A voltage balancing strategy with extended operating region for cascaded H-bridge converters. IEEE Trans. Power Electron. 2014, 29, 5044–5053. [Google Scholar] [CrossRef]
- Miranbeigi, M.; Iman-Eini, H. Hybrid Modulation Technique for Grid-Connected Cascaded Photovoltaic Systems. IEEE Trans. Ind. Electron. 2016, 63, 7843–7853. [Google Scholar] [CrossRef]
- Wang, S.; Jiao, N.; Ma, J.; Liu, T.; Liu, X. Analysis and Optimization of Voltage Balancing Control Limits for Cascaded H-Bridge Rectifiers. IEEE Trans. Ind. Electron. 2021, 68, 10677–10687. [Google Scholar] [CrossRef]
- Ko, Y.; Tcai, A.; Liserre, M. DC-Link Voltage Balancing Modulation for Cascaded H-Bridge Converters. IEEE Access 2021, 9, 103524–103532. [Google Scholar] [CrossRef]
- Li, J.; Chen, J.; Gong, C.; He, Q. Improved Square Wave Injection Strategy for the Voltage Balance Control of Cascaded H-Bridge Rectifier. IEEE Trans. Transp. Electrif. 2023, 9, 3711–3722. [Google Scholar] [CrossRef]



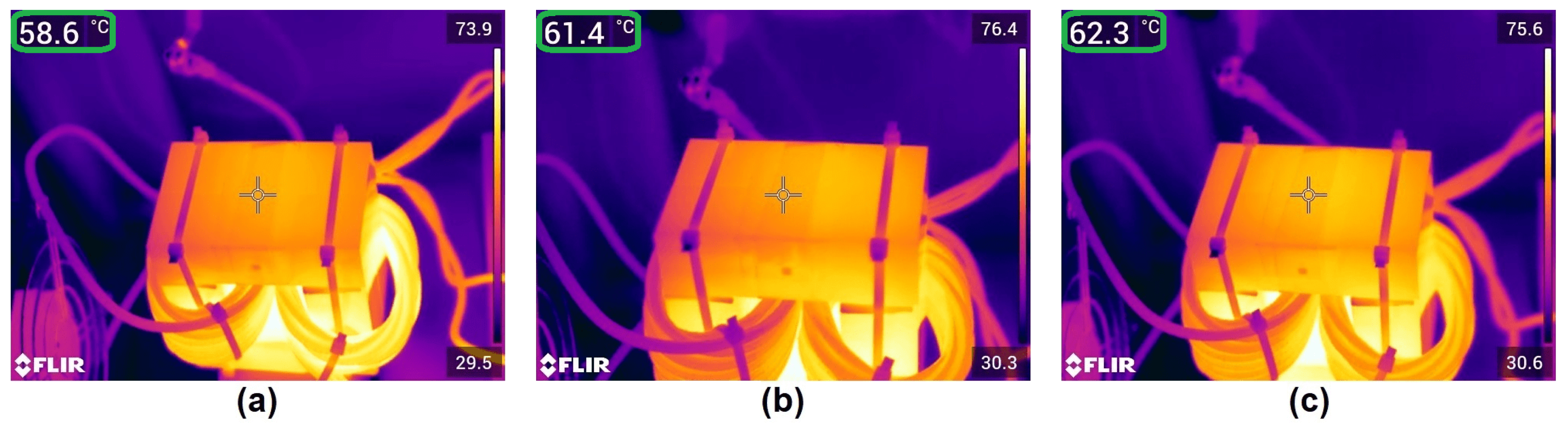
| Parameter | Symbol | Value |
|---|---|---|
| Rated Peak Power | ||
| Nominal Output Voltage | ||
| Nominal Output Current | ||
| Nominal DC-Link Voltage | ||
| Resonant Frequency | ||
| Resonant Capacitance | ||
| Resonant Inductance | ||
| Magnetizing Inductance | ||
| Transformer Transfer Ratio | ||
| Transformer Turns Ratio |
| Parameter | Conventional Method | Proposed Method |
|---|---|---|
| LLC Converter Efficiency | (+0.04%) | |
| Peak Resonant Capacitor Voltage | (−13%) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Unruh, R.; Böcker, J.; Schafmeister, F. Adaptive DC-Link Voltage Control for 22 kW, 40 kHz LLC Resonant Converter Considering Low-Frequency Voltage Ripple. Electronics 2025, 14, 1517. https://doi.org/10.3390/electronics14081517
Unruh R, Böcker J, Schafmeister F. Adaptive DC-Link Voltage Control for 22 kW, 40 kHz LLC Resonant Converter Considering Low-Frequency Voltage Ripple. Electronics. 2025; 14(8):1517. https://doi.org/10.3390/electronics14081517
Chicago/Turabian StyleUnruh, Roland, Joachim Böcker, and Frank Schafmeister. 2025. "Adaptive DC-Link Voltage Control for 22 kW, 40 kHz LLC Resonant Converter Considering Low-Frequency Voltage Ripple" Electronics 14, no. 8: 1517. https://doi.org/10.3390/electronics14081517
APA StyleUnruh, R., Böcker, J., & Schafmeister, F. (2025). Adaptive DC-Link Voltage Control for 22 kW, 40 kHz LLC Resonant Converter Considering Low-Frequency Voltage Ripple. Electronics, 14(8), 1517. https://doi.org/10.3390/electronics14081517






