A Cryo-CMOS, Low-Power, Low-Noise, Phase-Locked Loop Design for Quantum Computers
Abstract
1. Introduction
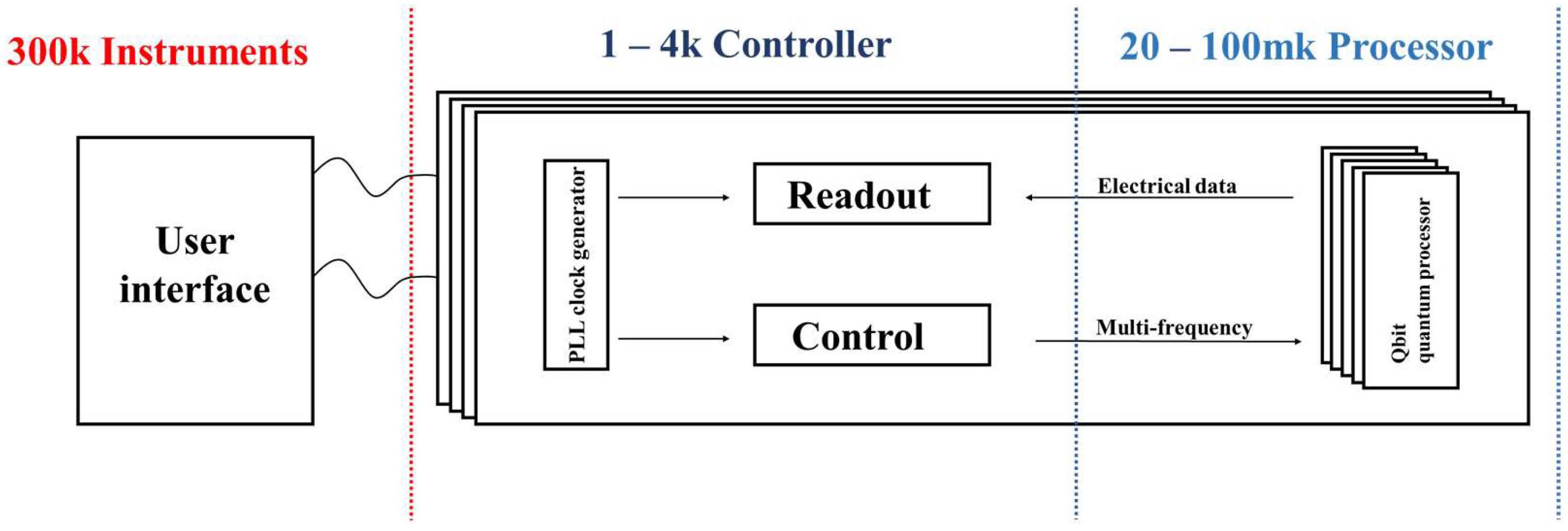
2. Performance Requirements for Clock Generators Imposed by Quantum Computers
3. Negative Effects of Circuits at Low Temperatures
3.1. Mismatch of Devices
3.2. Noise at Low Temperature
4. Circuit Design and Noise Analysis
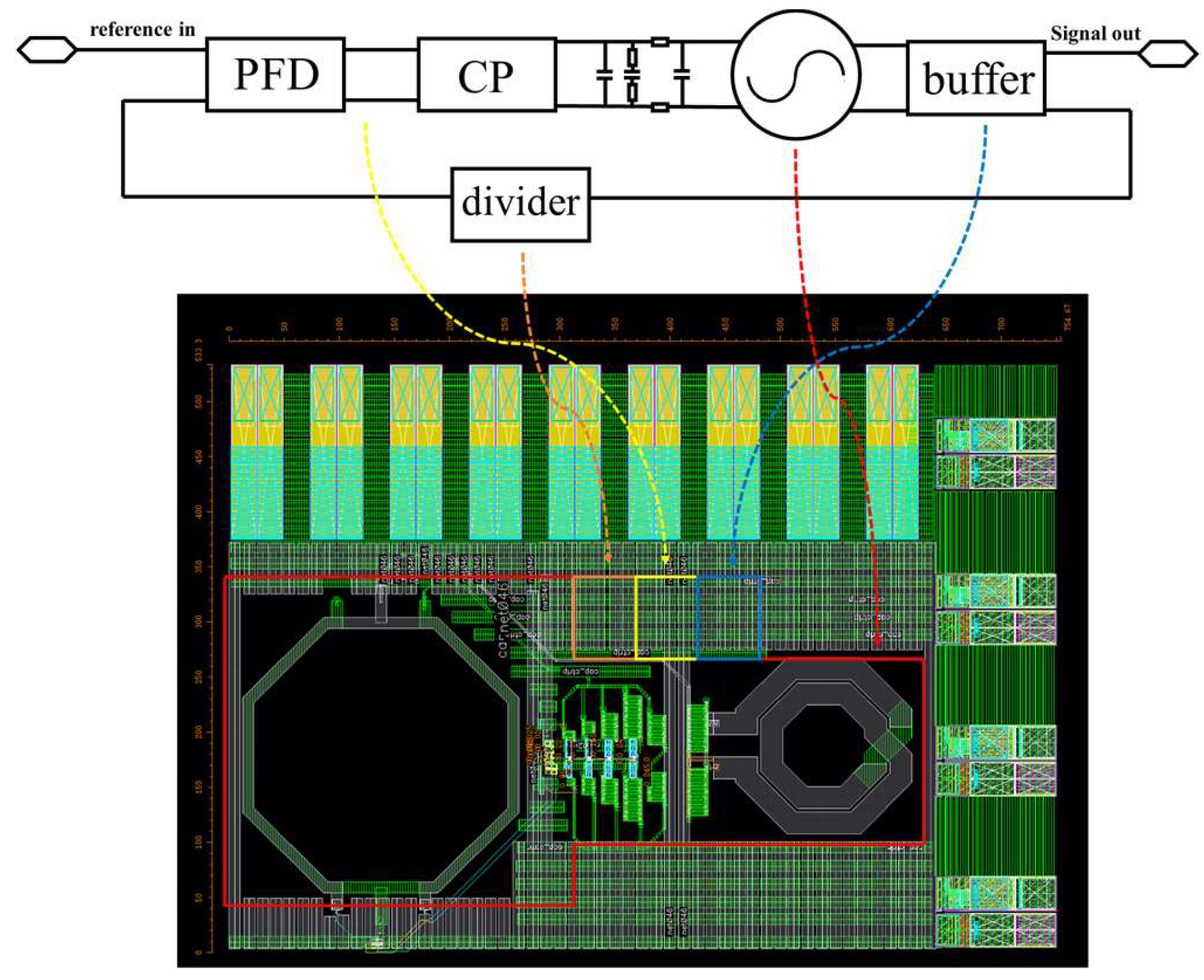
4.1. F-Class VCO
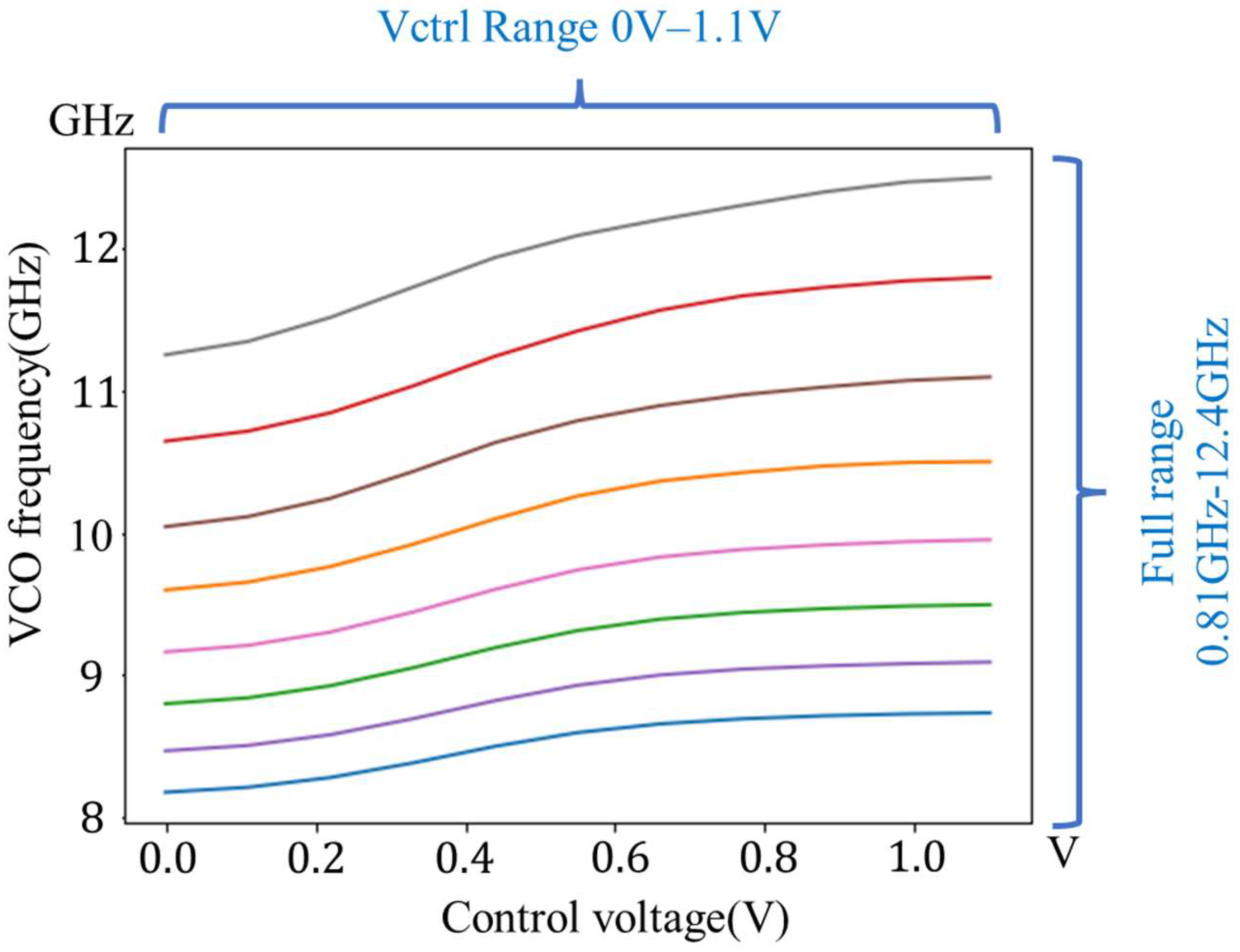
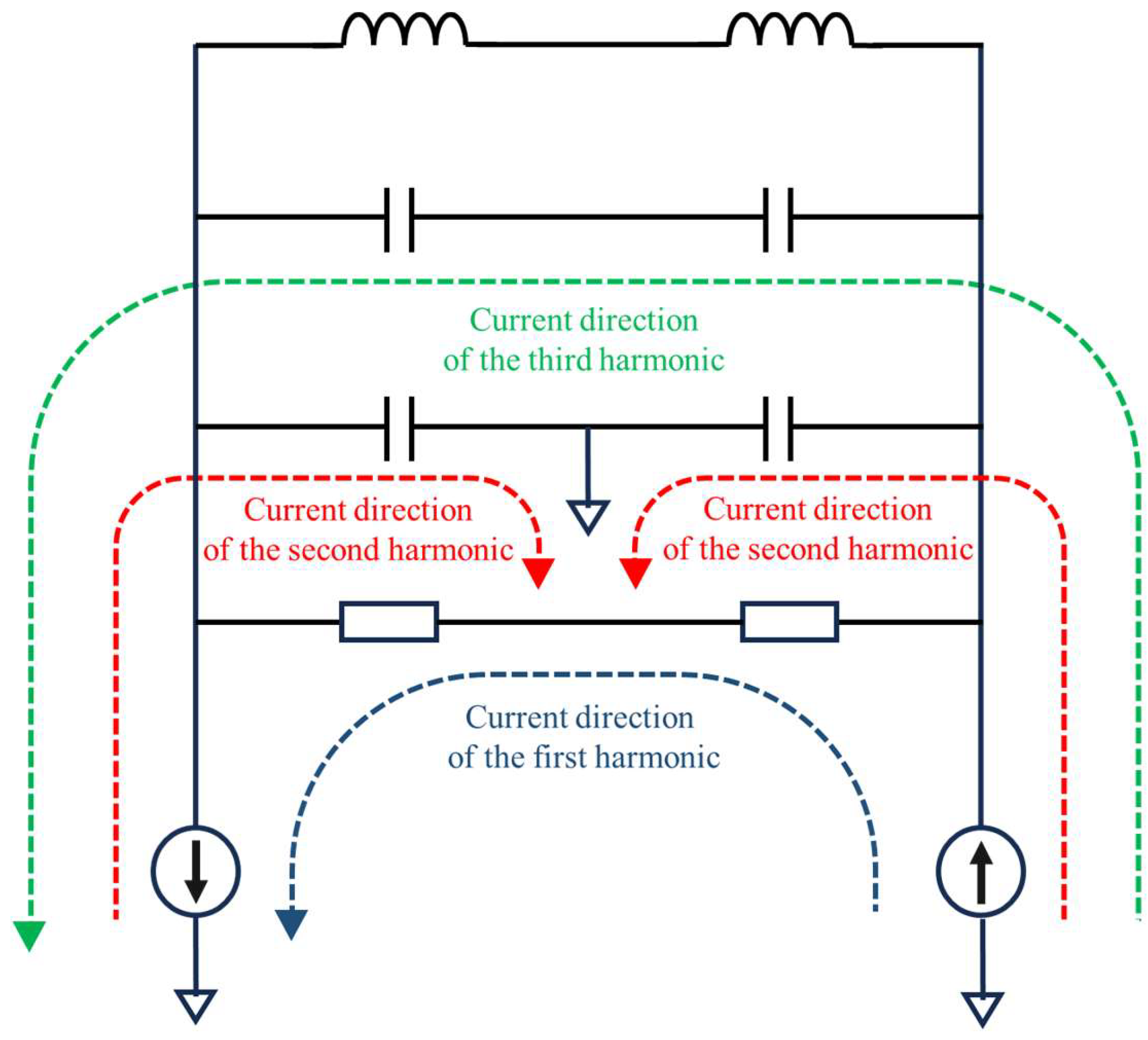
4.1.1. Design of the Circuit
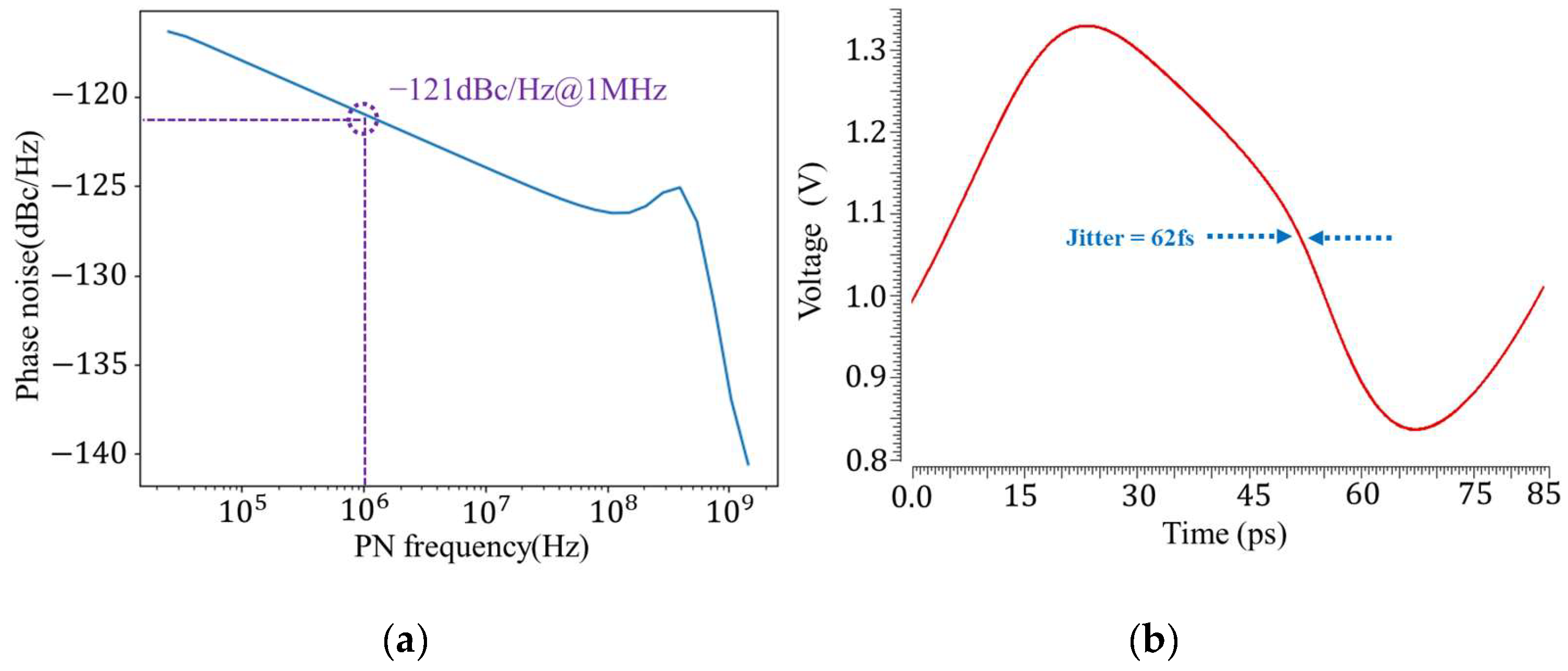
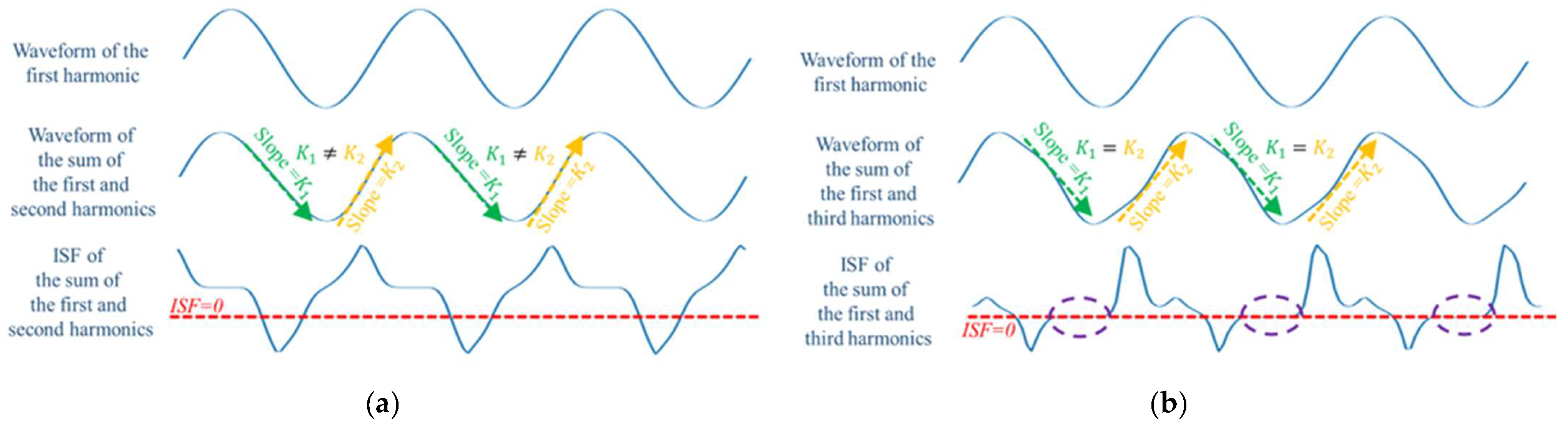
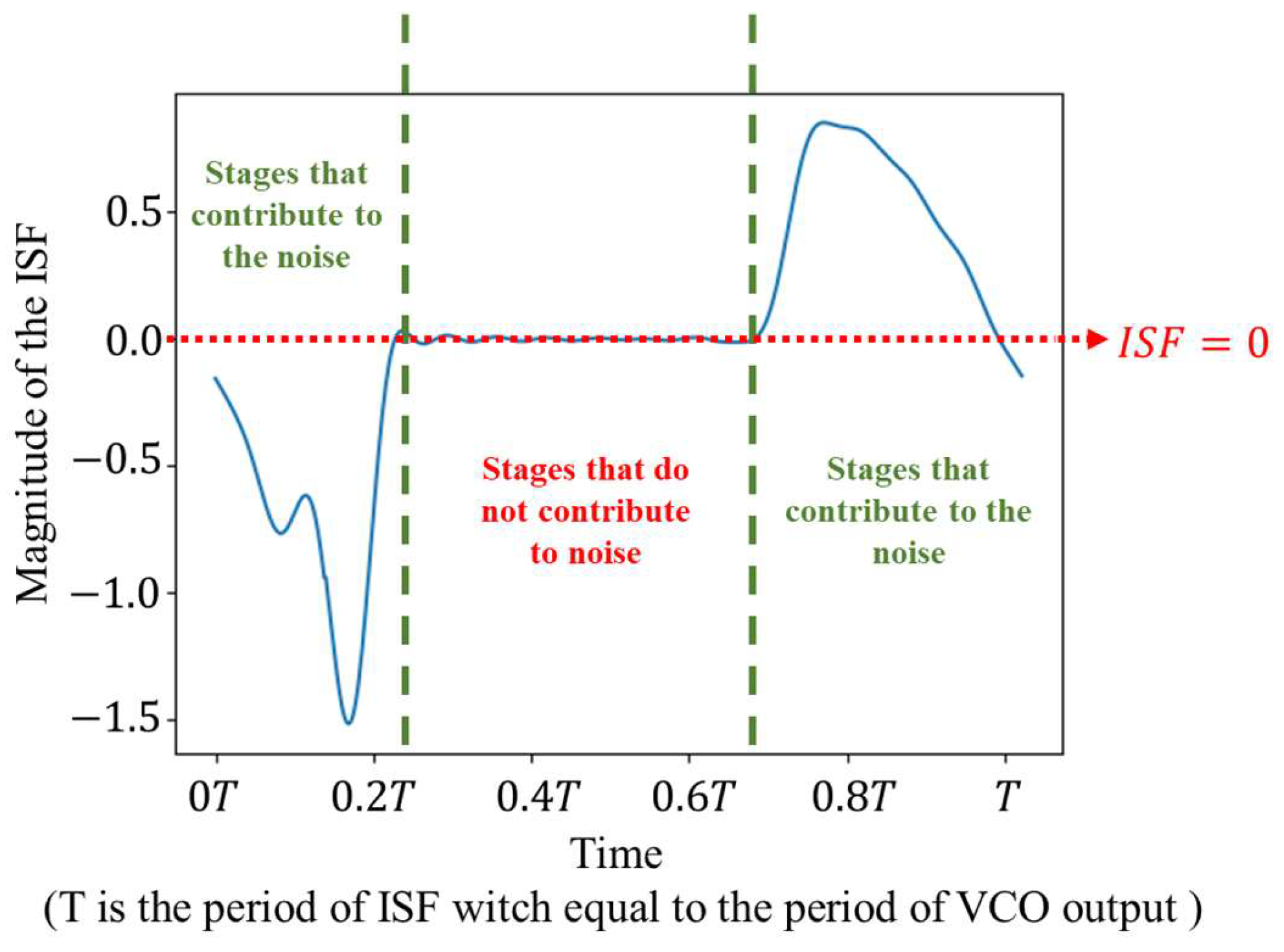
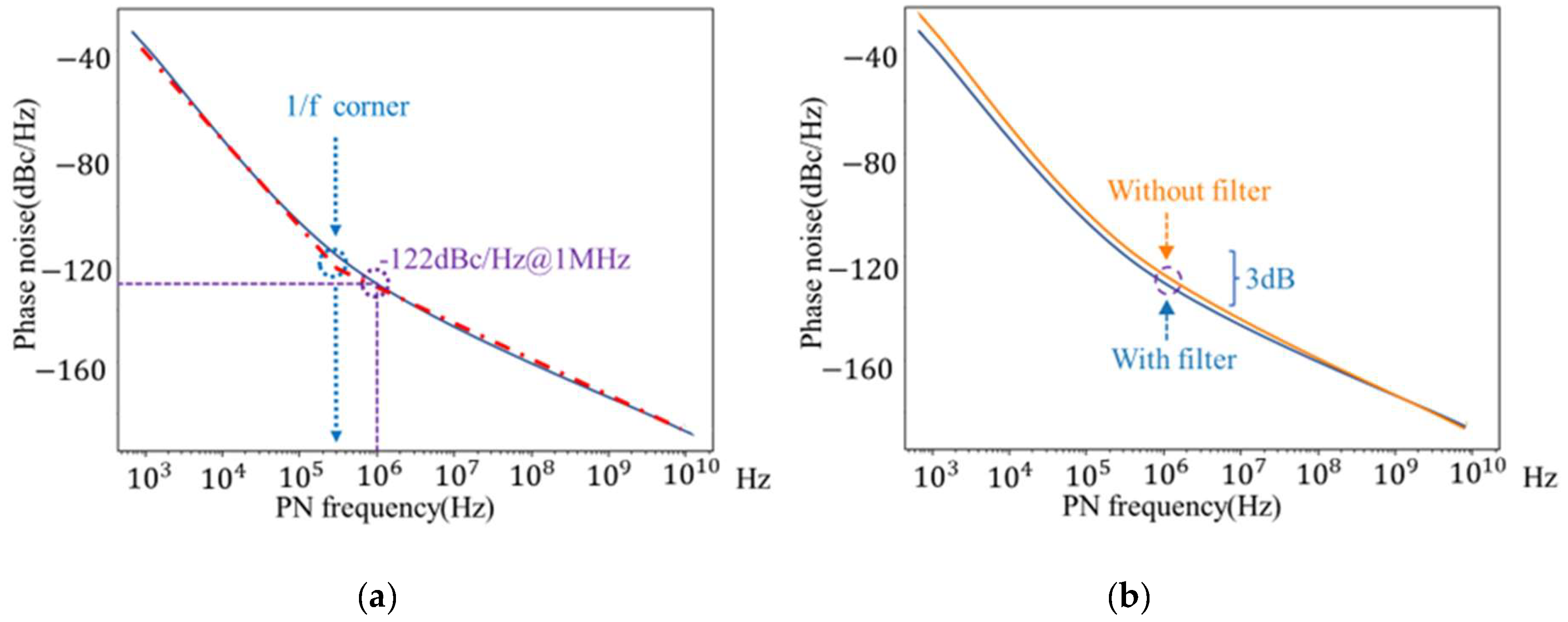
4.1.2. Analysis of the Reasons Why This Circuit Can Reduce Noise
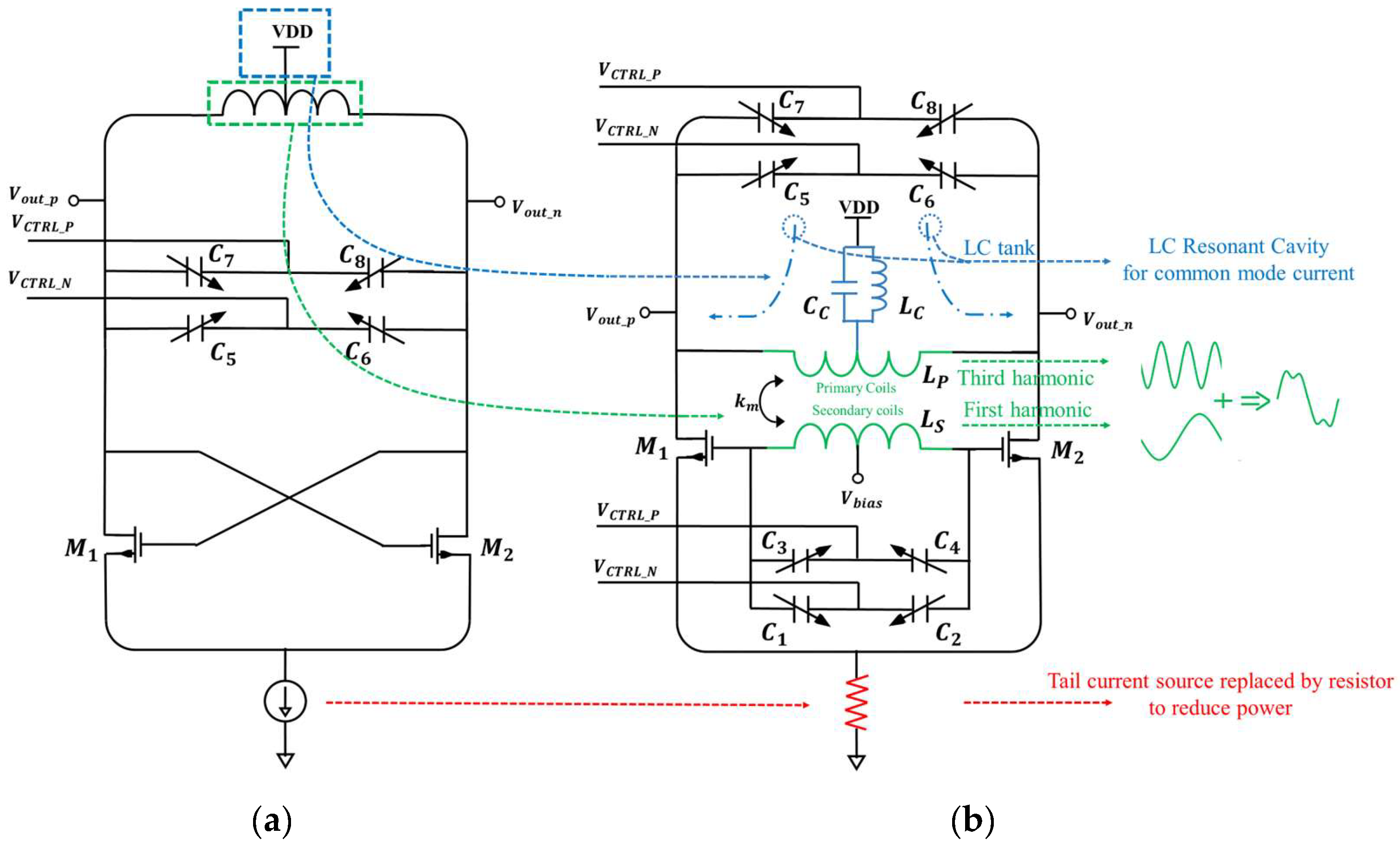
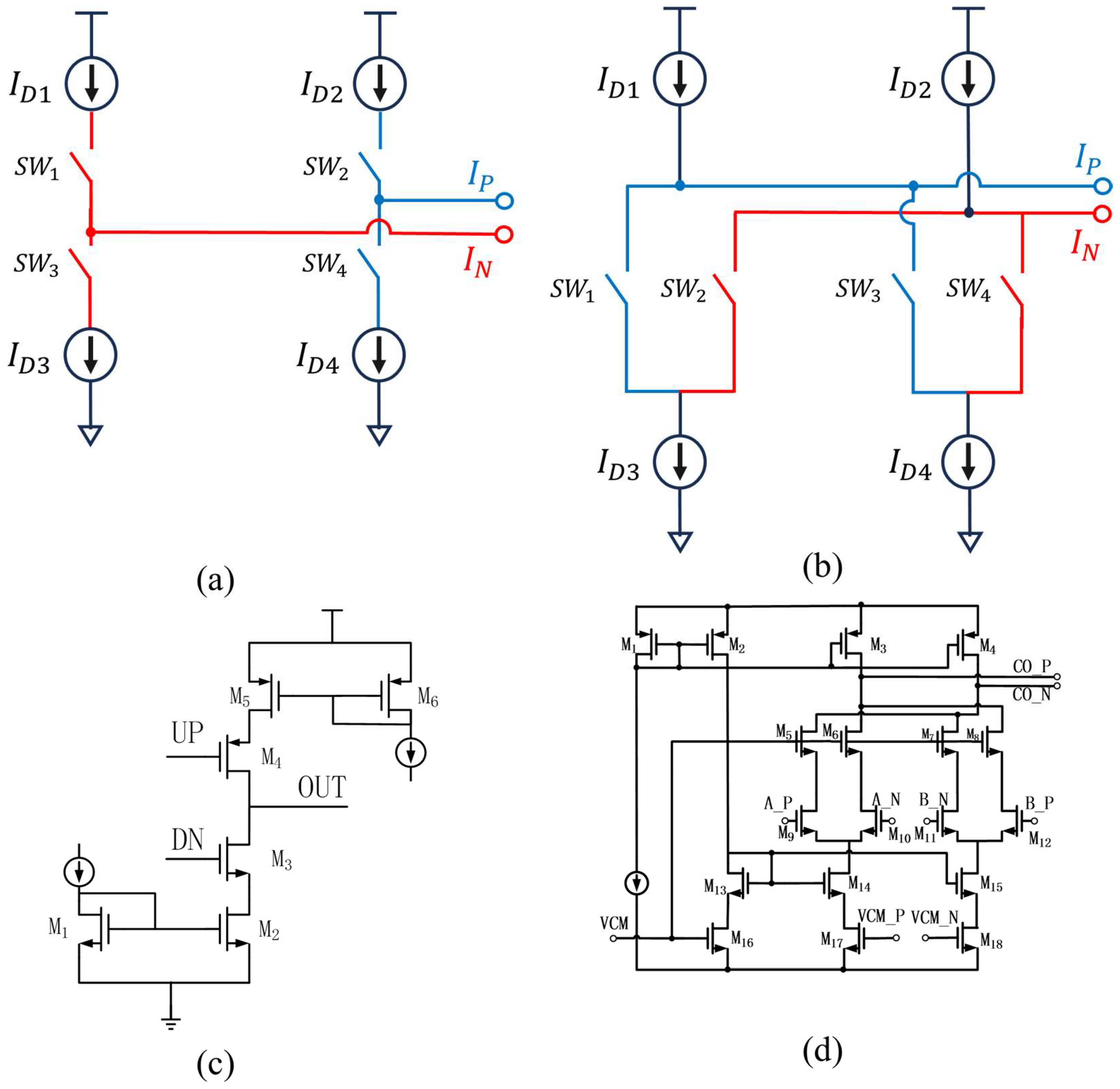
4.2. Fully Differential Charge Pump
4.2.1. Design of the Fully Differential CP
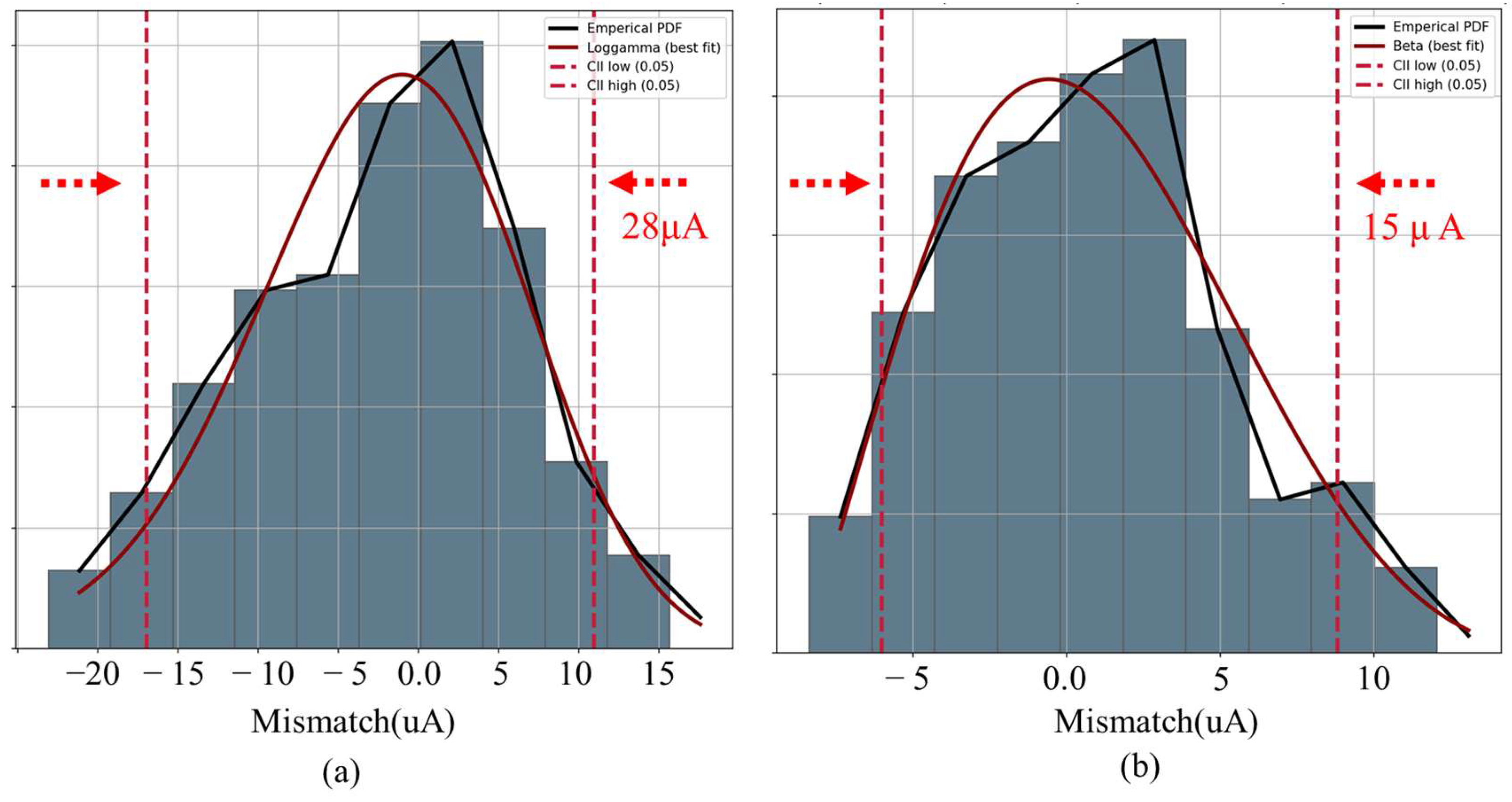
4.2.2. Analysis of Device Mismatch
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Montanaro, A. Quantum algorithms: An overview. NPJ Quantum Inf. 2016, 2, 1–8. [Google Scholar] [CrossRef]
- Shor, P.W. Algorithms for quantum computation: Discrete logarithms and factoring. In Proceedings of the 35th Annual Symposium on Foundations of Computer Science, Santa Fe, NM, USA, 20–22 November 1994. [Google Scholar] [CrossRef]
- Grover, L.K. A fast quantum mechanical algorithm for database search. STOC ′96. In Proceedings of the Twenty-Eighth Annual ACM Symposium on Theory of Computing, Philadelphia, PA, USA, 22–24 May 1996; pp. 212–219. [Google Scholar] [CrossRef]
- Feynman, R.P. Simulating physics with computers. Int. J. Theor. Phys. 1982, 21, 467–488. [Google Scholar] [CrossRef]
- Vu, C.; Fay, M. IBM Builds Its Most Powerful Universal Quantum Computing Processors; IBM Research: Armonk, NY, USA, 2017. [Google Scholar]
- Kelly, J.; Barends, R.; Fowler, A.G.; Megrant, A.; Jeffrey, E.; White, T.C.; Sank, D.; Mutus, J.Y.; Campbell, B.; Chen, Y. State preservation by repetitive error detection in a superconducting quantum circuit. Nature 2015, 519, 66–69. [Google Scholar] [CrossRef] [PubMed]
- Monz, T.; Schindler, P.; Barreiro, J.T.; Chwalla, M.; Nigg, D.; Coish, W.A.; Blatt, R. 14-Qubit Entanglement: Creation and Coherence. Phys. Rev. Lett. 2011, 106, 130506. [Google Scholar] [CrossRef]
- Patra, B.; Incandela, R.M.; Van Dijk, J.P.; Homulle, H.A.; Song, L.; Shahmohammadi, M.; Charbon, E. Cryo-CMOS Circuits and Systems for Quantum Computing Applications. IEEE J. Solid-State Circuits 2018, 53, 309–321. [Google Scholar] [CrossRef]
- Van Meter, R.; Horsman, D. A blueprint for building a quantum computer. Commun. ACM 2013, 56, 84–93. [Google Scholar] [CrossRef]
- Vandersypen LM, K.; Bluhm, H.; Clarke, J.S.; Dzurak, A.S.; Ishihara, R.; Morello, A.; Reilly, D.J.; Schreiber, L.R.; Veldhorst, M. Interfacing spin qubits in quantum dots and donors—Hot, dense, and coherent. NPJ Quantum Inf. 2017, 3, 34. [Google Scholar] [CrossRef]
- Mehrpoo, M.; Sebastiano, F.; Charbon, E.; Babaie, M. A Cryogenic CMOS Parametric Amplifier. IEEE Solid-State Circuits Lett. 2019, 3, 5–8. [Google Scholar] [CrossRef]
- Le Guevel, L.; Billiot, G.; Jehl, X.; De Franceschi, S.; Zurita, M.; Thonnart, Y.; Pillonnet, G. 19.2 A 110 mK 295 µW 28 nm FDSOI CMOS Quantum Integrated Circuit with a 2.8 GHz Excitation and nA Current Sensing of an On-Chip Double Quantum Dot. In Proceedings of the 2020 IEEE International Solid- State Circuits Conference—(ISSCC), San Francisco, CA, USA, 16–20 February 2020. [Google Scholar] [CrossRef]
- Vliex, P.; Degenhardt, C.; Grewing, C.; Kruth, A.; Nielinger, D.; Van Waasen, S.; Heinen, S. Bias Voltage DAC Operating at Cryogenic Temperatures for Solid-State Qubit Applications. IEEE Solid-State Circuits Lett. 2020, 3, 218–221. [Google Scholar] [CrossRef]
- Ruffino, A.; Peng, Y.; Sebastiano, F.; Babaie, M.; Charbon, E. A Wideband Low-Power Cryogenic CMOS Circulator for Quantum Applications. IEEE J. Solid-State Circuits 2020, 55, 1224–1238. [Google Scholar] [CrossRef]
- Ball, H.; Oliver, W.D.; Biercuk, M.J. The role of master clock stability in quantum information processing. npj Quantum Inf. 2016, 2, 16033. [Google Scholar] [CrossRef]
- Raftery, J.; Vrajitoarea, A.; Zhang, G.; Leng, Z.; Srinivasan, S.; Houck, A. Direct Synthesis of Microwave Waveforms for Quantum Computing. APS March Meet. Abstr. 2017, 2017, C46-003. [Google Scholar] [CrossRef]
- Green, T.; Uys, H.; Biercuk, M.J. High-Order Noise Filtering in Nontrivial Quantum Logic Gates. Phys. Rev. Lett. 2012, 109, 1224–1238. [Google Scholar] [CrossRef]
- Green, T.J.; Sastrawan, J.; Uys, H.; Biercuk, M.J. Arbitrary quantum control of qubits in the presence of universal noise. New J. Phys. 2013, 15, 095004. [Google Scholar] [CrossRef]
- Reed, M.D.; Maune, B.M.; Andrews, R.W.; Borselli, M.G.; Eng, K.; Jura, M.P.; Kiselev, A.A.; Hunter, A.T. Reduced Sensitivity to Charge Noise in Semiconductor Spin Qubits via Symmetric Operation. Phys. Rev. Lett. 2016, 116, 110402. [Google Scholar] [CrossRef]
- Martins, F.; Malinowski, F.K.; Nissen, P.D.; Barnes, E.; Fallahi, S.; Gardner, G.C.; Manfra, M.J.; Marcus, C.M.; Kuemmeth, F. Noise Suppression Using Symmetric Exchange Gates in Spin Qubits. Phys. Rev. Lett. 2016, 116, 116801. [Google Scholar] [CrossRef]
- Didier, N.; Sete, E.A.; Combes, J.; da Silva, M.P. ac Flux Sweet Spots in Parametrically Modulated Superconducting Qubits. Phys. Rev. Appl. 2019, 12, 054015. [Google Scholar] [CrossRef]
- Anton, S.M.; Müller, C.; Birenbaum, J.S.; O’Kelley, S.R.; Fefferman, A.D.; Golubev, D.S.; Clarke, J. Pure dephasing in flux qubits due to flux noise with spectral density scaling as 1/f α. Phys. Rev. B 2012, 85, 224505. [Google Scholar] [CrossRef]
- Rebentrost, P.; Serban, I.; Schulte-Herbrüggen, T.; Wilhelm, F.K. Optimal Control of a Qubit Coupled to a NonMarkovian Environment. Phys. Rev. Lett. 2009, 102, 090401. [Google Scholar] [CrossRef]
- Nazarov, Y.V. Quantum Noise in Mesoscopic Physics; Springer Science & Business Media: Delft, The Netherlands, 2012; Volume 97. [Google Scholar]
- Gong, J.; Charbon, E.; Sebastiano, F.; Babaie, M. A Cryo-CMOS PLL for Quantum Computing Applications. IEEE J. Solid-State Circuits 2022, 58, 1362–1375. [Google Scholar] [CrossRef]
- Van Dijk, J.P.-G.; Kawakami, E.; Schouten, R.N.; Veldhorts, M.; Vandersypen, L.M.K.; Babaie, M.; Charbon, E.; Sebastiano, F. Impact of Classical Control Electronics on Qubit Fidelity. Phys. Rev. Appl. 2019, 12, 044054. [Google Scholar] [CrossRef]
- Nielsen, M.A. A simple formula for the average gate fidelity of a quantum dynamical operation. Phys. Lett. A 2002, 303, 249–252. [Google Scholar] [CrossRef]
- Pedersen, L.H.; Møller, N.M.; Mølmer, K. Fidelity of quantum operations. Phys. Lett. A 2007, 367, 47–51. [Google Scholar] [CrossRef]
- Croon, J.; Rosmeulen, M.; Decoutere, S.; Sansen, W.; Maes, H. An easy-to-use mismatch model for the MOS transistor. IEEE J. Solid-State Circuits 2002, 37, 1056–1064. [Google Scholar] [CrossRef]
- Pelgrom, M.; Duinmaijer, A.; Welbers, A. Matching properties of MOS transistors. IEEE J. Solid-State Circuits 1989, 24, 1433–1439. [Google Scholar] [CrossRef]
- Shahmohammadi, M.; Babaie, M.; Staszewski, R.B. A 1/f Noise Upconversion Reduction Technique for Voltage-Biased RF CMOS Oscillators. IEEE J. Solid-State Circuits 2016, 51, 2610–2624. [Google Scholar] [CrossRef]
- Hong, F.; Ding, T.; Zhao, D. A 196.5 dBc/Hz FOMT 16.8–21.6-GHz Class-F23 CMOS VCO With Transformer-Based Optimal Q-Factor Tank. IEEE Solid-State Circuits Lett. 2022, 5, 62–65. [Google Scholar] [CrossRef]
- Ming, S.; Zhou, J. A 19 GHz Circular-Geometry Quad-Core Tail-Filtering Class-F VCO with −115 dBc/Hz Phase Noise at 1 MHz Offset in 65-nm CMOS. In Proceedings of the ESSCIRC 2021—IEEE 47th European Solid State Circuits Conference (ESSCIRC), Virtual, 13–22 September 2021. [Google Scholar] [CrossRef]
- Hajimiri, A.; Lee, T. A general theory of phase noise in electrical oscillators. IEEE J. Solid-State Circuits 1998, 33, 179–194. [Google Scholar] [CrossRef]
- Hu, Y.; Siriburanon, T.; Staszewski, R.B. A Low-Flicker-Noise 30-GHz Class-F23 Oscillator in 28-nm CMOS Using Implicit Resonance and Explicit Common-Mode Return Path. IEEE J. Solid-State Circuits 2018, 53, 1977–1987. [Google Scholar] [CrossRef]
- Hu, Y.; Siriburanon, T.; Staszewski, R.B. Oscillator Flicker Phase Noise: A Tutorial. IEEE Trans. Circuits Syst.—II Express Briefs 2021, 68, 538–544. [Google Scholar] [CrossRef]
- Ruffino, A.; Peng, Y.; Yang, T.-Y.; Michniewicz, J.; Gonzalez-Zalba, M.F.; Charbon, E. A fully-integrated 40-nm 5–6.5 GHz cryo-CMOS system-on-chip with I/Q receiver and frequency synthesizer for scalable multiplexed readout of quantum dots. In Proceedings of the 2021 IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 13–22 February 2021; pp. 210–212. [Google Scholar] [CrossRef]
- Peng, Y.; Ruffino, A.; Benserhir, J.; Charbon, E. A cryogenic SiGe BiCMOS hybrid class B/C mode-switching VCO achieving 201 dBc/Hz figure-of-merit and 4.2 GHz frequency tuning range. In Proceedings of the 2022 IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 20–24 February 2022. [Google Scholar] [CrossRef]


| VCO in This Paper | VCO in [36] | VCO in [36] | |
|---|---|---|---|
| Structure | F CLASS VCO | nMOS only | complementary |
| Corner frequency | 200 KHz | 3 MHz | 3 MHz |
| Mismatch of classical charge pumps | ||
| Mismatch of fully differential charge pumps | or | or |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, K.; Lai, M.; Lv, F.; Guo, K.; Pang, Z.; Xu, C.; Zhang, G.; Wang, W.; Li, M. A Cryo-CMOS, Low-Power, Low-Noise, Phase-Locked Loop Design for Quantum Computers. Electronics 2023, 12, 3237. https://doi.org/10.3390/electronics12153237
Xin K, Lai M, Lv F, Guo K, Pang Z, Xu C, Zhang G, Wang W, Li M. A Cryo-CMOS, Low-Power, Low-Noise, Phase-Locked Loop Design for Quantum Computers. Electronics. 2023; 12(15):3237. https://doi.org/10.3390/electronics12153237
Chicago/Turabian StyleXin, Kewei, Mingche Lai, Fangxu Lv, Kaile Guo, Zhengbin Pang, Chaolong Xu, Geng Zhang, Wenchen Wang, and Meng Li. 2023. "A Cryo-CMOS, Low-Power, Low-Noise, Phase-Locked Loop Design for Quantum Computers" Electronics 12, no. 15: 3237. https://doi.org/10.3390/electronics12153237
APA StyleXin, K., Lai, M., Lv, F., Guo, K., Pang, Z., Xu, C., Zhang, G., Wang, W., & Li, M. (2023). A Cryo-CMOS, Low-Power, Low-Noise, Phase-Locked Loop Design for Quantum Computers. Electronics, 12(15), 3237. https://doi.org/10.3390/electronics12153237






