High-Level Renewable Energy Integrated System Frequency Control with SMES-Based Optimized Fractional Order Controller
Abstract
1. Introduction
1.1. Issues with Frequency Deviation
1.2. Mitigation Schemes
1.3. Research Gaps and Contributions
- The inertia of the system is supported virtually, which makes the system stable over a wide range of load-generation mismatch.
- The system frequency deviation is greatly improved with the proposed approach.
- The proposed optimized FOC-based SMES approach is robust against system parameter variations.
- The overshoot, undershoot, and settling time of the response are improved compared to the conventional approach.
- The proposed approach endorses the green effort to augment sustainability.
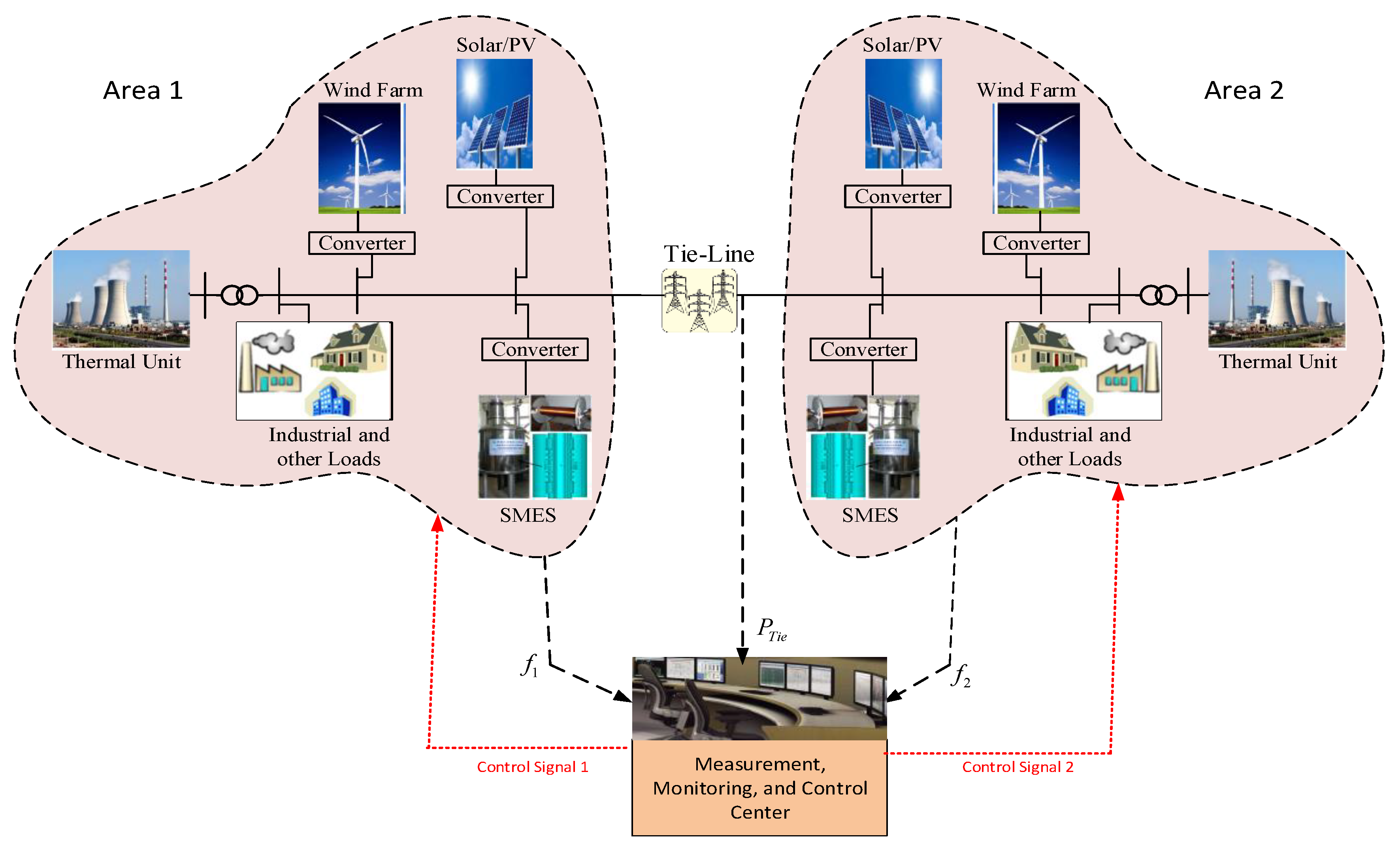
2. Renewable Energy Integrated System Modeling
2.1. System Configuration
2.2. System Dynamic Modeling
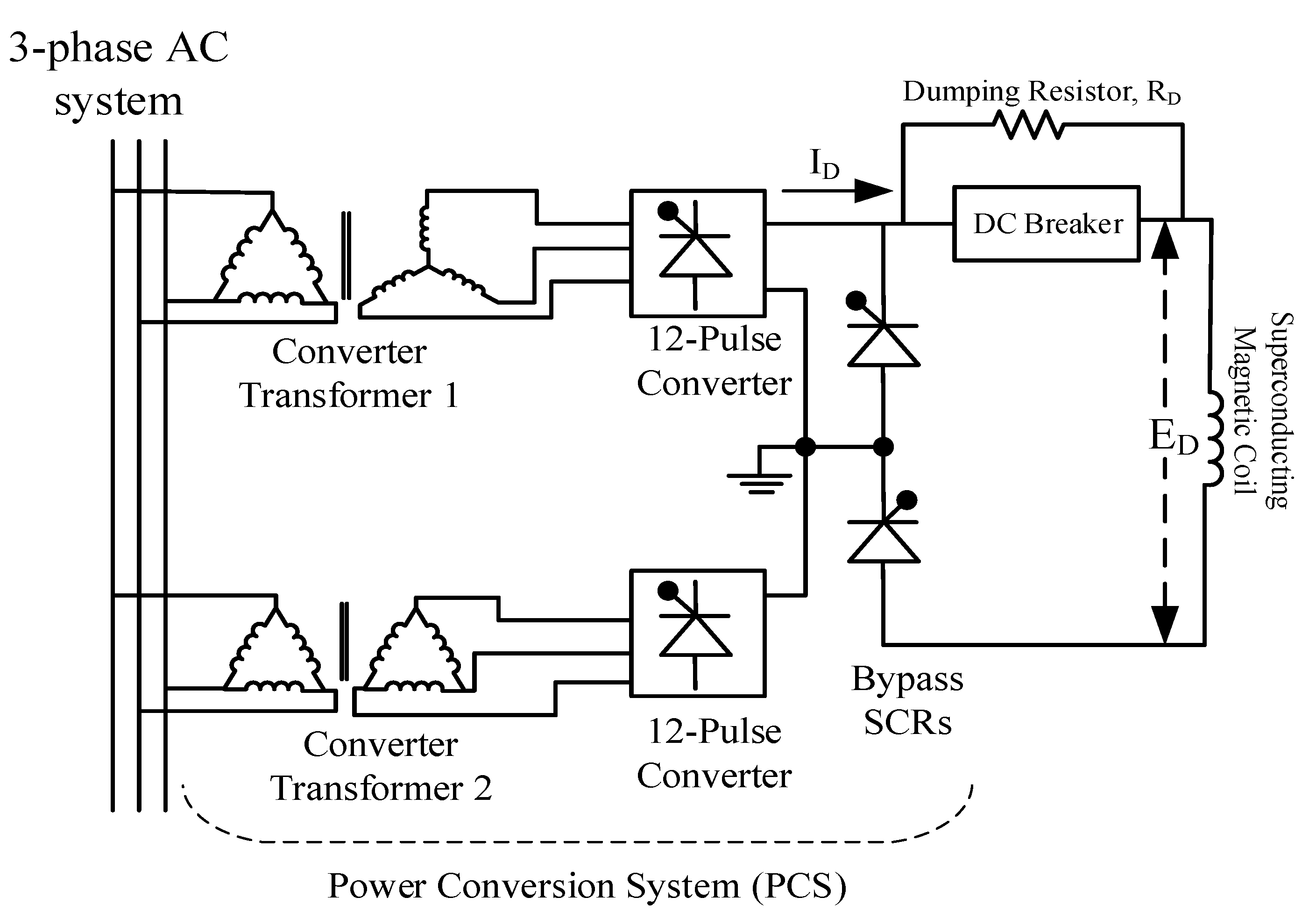
3. SMES Model with FOC
3.1. Fractional Order PI-Based SMES Controller Design
3.2. Description of the Cost Function
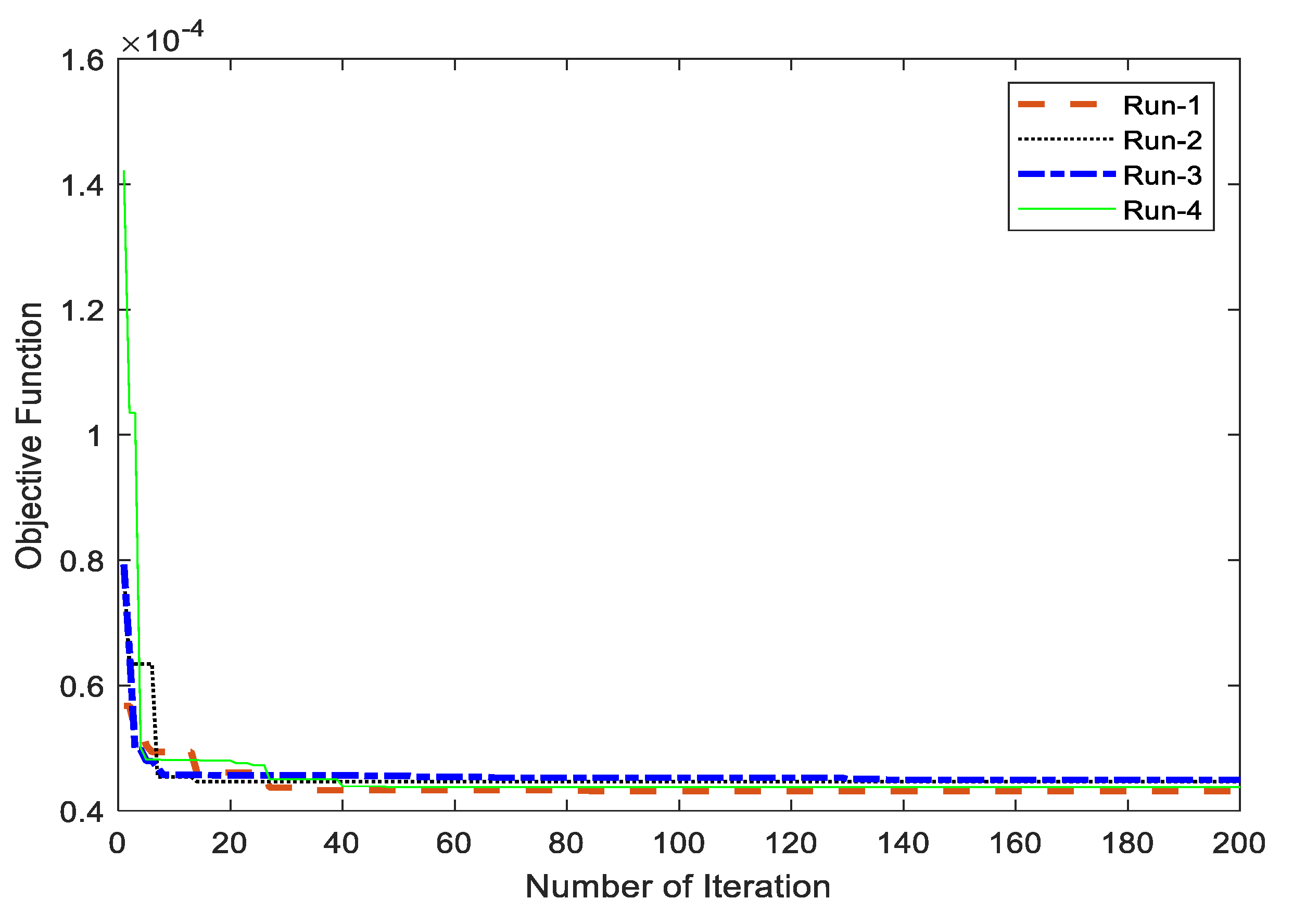
3.3. Controller Design with WOA
- a.
- Encircling prey
- b.
- Bubble-net attacking mechanism
- c.
- Search for prey
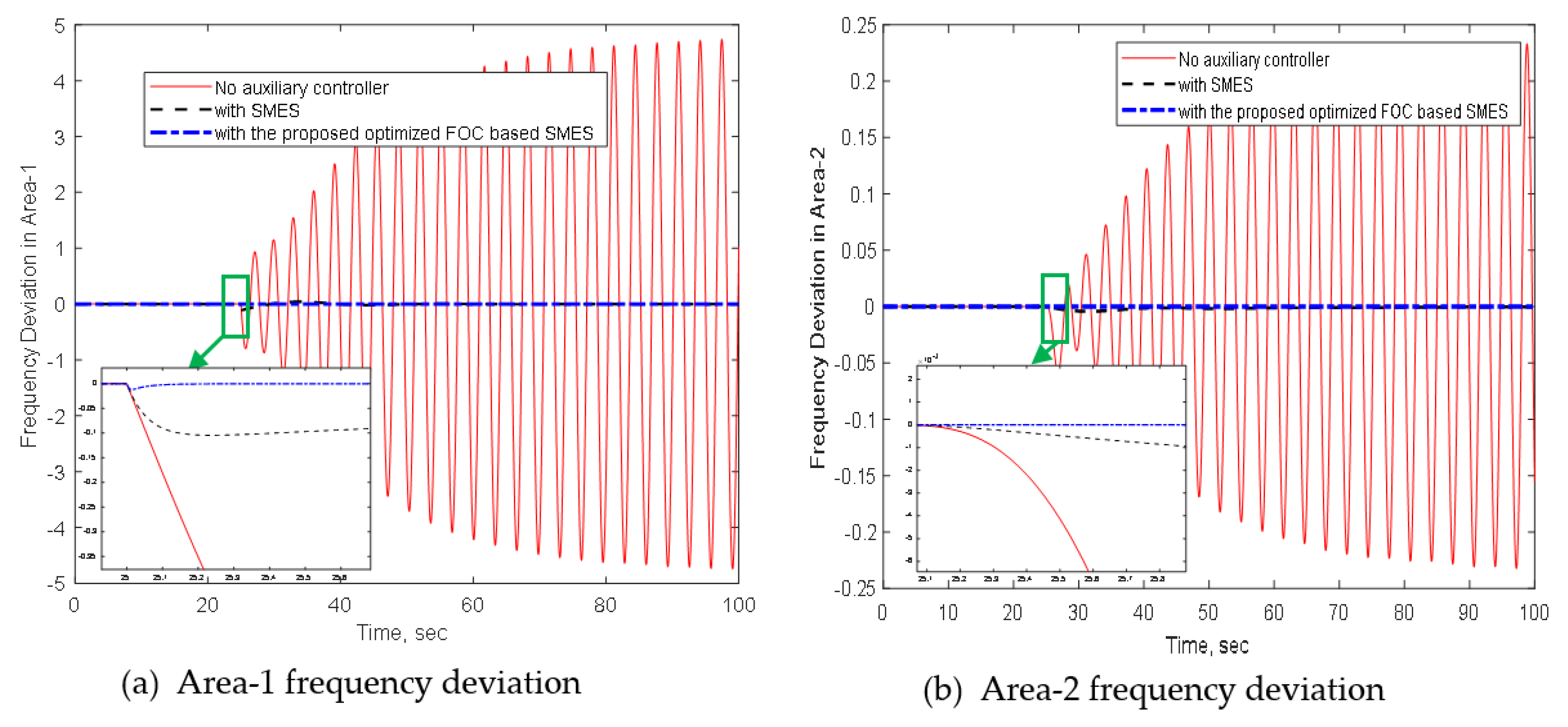
4. Simulation Results and Discussion
4.1. Simulation Results
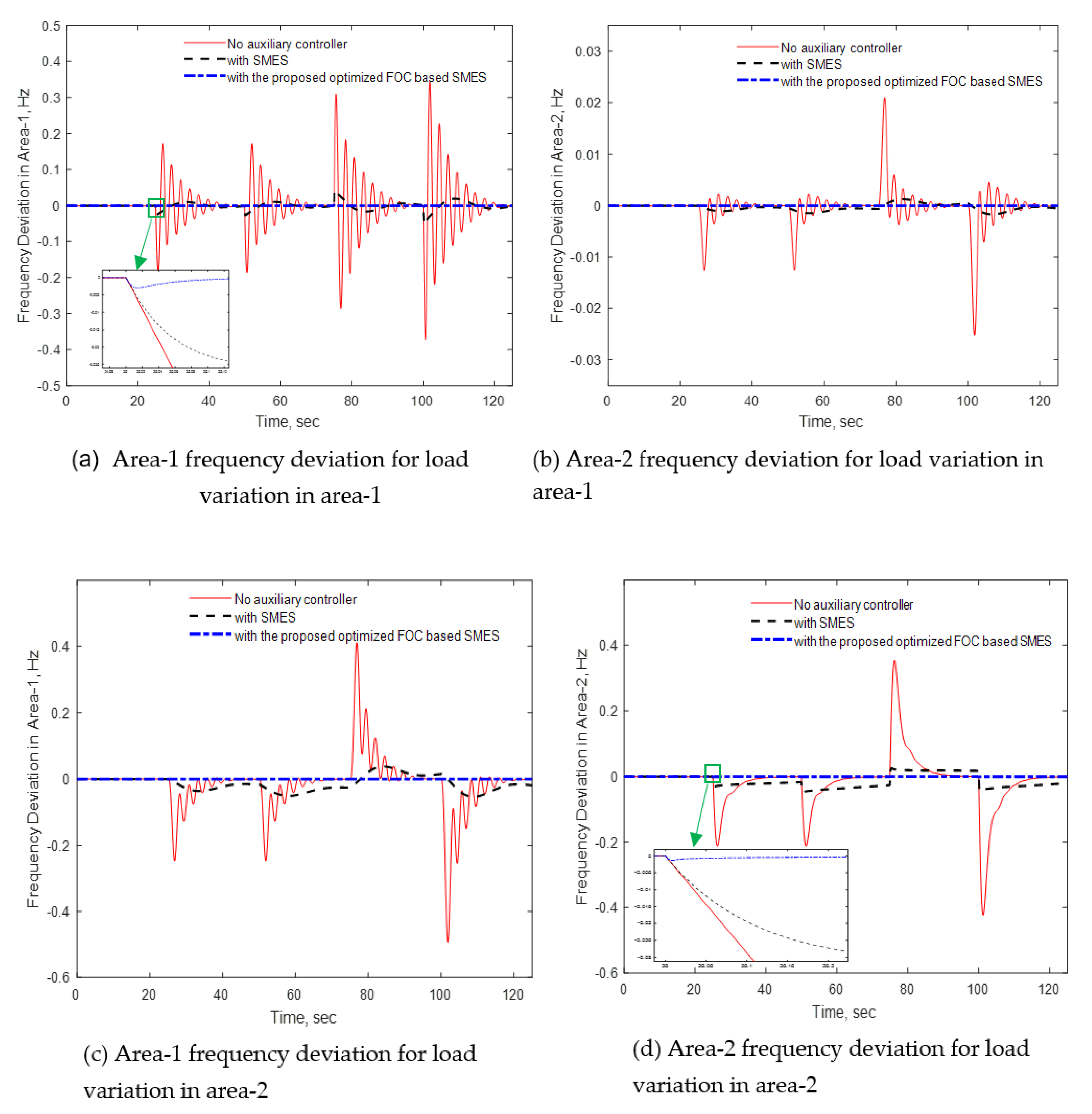
4.1.1. Load Profile Variation in Area-1
4.1.2. Load Profile Variation in Area-2
4.1.3. Multiple Profile Variations in Area-1 and Area-2
4.1.4. Frequency Response Analysis for Solar and Wind Power Variations
4.1.5. Frequency Response Analysis for Reduced System Inertia
4.2. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations and Symbols
| RESs | Renewable energy sources | TA | Tabu search |
| FOC | Fractional order controller | GDB | Generator dead band |
| SMES | Superconducting magnetic energy storage | GRC | Generation rate constraint |
| WOA | Whale optimization algorithm | H | Inertia constant |
| RoCoF | Rate of change of frequency | D | Damping constant |
| SG | Synchronous generator | PCS | Power conversion system |
| PV | Photovoltaic | Thyristor firing angle | |
| LFC | Load frequency control | KSMES | SMES proportional gain |
| PID | Proportional integral derivative | KID | SMES feedback gain |
| MPC | Model predictive controller | Kp | Proportional gain |
| BESS | Battery energy storage system | Ki | Integral gain |
| DFIG | Doubly fed induction generator | λ | Fractional order |
| CCFC | Capability coordinated frequency control | ∆f | Frequency deviation |
| PSO | Particle swarm optimization | Converter delay time | |
| MPSO | Modified PSO | Incremental current change | |
| NSGA | Non-dominated sorting genetic algorithm Genetic algorithm | Incremental voltage change | |
| GA | Genetic algorithm | RD | Damping resistor |
| ABC | Ant bee colony |
References
- Ekkert, M. Global Energy Transformation: A Roadmap to 2050; The International Renewable Energy Agency (IRENA): Abu Dhabi, United Arab Emirates, 2018. [Google Scholar]
- Alam, M.S.; Al-Ismail, F.S.; Salem, A.; Abido, M.A. High-Level Penetration of Renewable Energy Sources into Grid Utility: Challenges and Solutions. IEEE Access 2020, 8, 190277–190299. [Google Scholar] [CrossRef]
- Alam, S.; Ali, M.; Abido, Y.; Hussein, A.E. Fault Ride through Capability Augmentation of a DFIG-Based Wind Integrated VSC-HVDC System with Non-Superconducting Fault Current Limiter. Sustainability 2019, 11, 1232. [Google Scholar] [CrossRef]
- Milano, F.; Dörfler, F.; Hug, G.; Hill, D.J.; Verbič, G. Foundations and Challenges of Low-Inertia Systems (Invited Paper). In Proceedings of the 2018 Power Systems Computation Conference (PSCC), Dublin, Ireland, 11–15 June 2018; pp. 1–25. [Google Scholar]
- Sarojini, K.; Palanisamy, K.; Yang, G. Future low-inertia power systems: Requirements, issues, and solutions—A review. Renew. Sustain. Energy Rev. 2020, 124, 109773. [Google Scholar]
- Hajiakbari Fini, M.; Hamedani Golshan, M.E. Frequency control using loads and generators capacity in power systems with a high penetration of renewables. Electr. Power Syst. Res. 2019, 166, 43–51. [Google Scholar] [CrossRef]
- Ulbig, A.; Borsche, T.S.; Andersson, G. Impact of Low Rotational Inertia on Power System Stability and Operation; IFAC: Cape Town, South Africa, 2014; Volume 19, ISBN 9783902823625. [Google Scholar]
- Alam, M.S.; Abido, M.A.Y.; El-Amin, I. Fault Current Limiters in Power Systems: A Comprehensive Review. Energies 2018, 11, 1025. [Google Scholar] [CrossRef]
- Yu, M.; Roscoe, A.J.; Dyśko, A.; Booth, C.D.; Ierna, R.; Zhu, J.; Urdal, H. Instantaneous penetration level limits of nonsynchronous devices in the British power system. IET Renew. Power Gener. 2017, 11, 1211–1217. [Google Scholar] [CrossRef]
- Saxena, P.; Singh, N.; Pandey, A.K. Enhancing the dynamic performance of microgrid using derivative controlled solar and energy storage based virtual inertia system. J. Energy Storage 2020, 31, 101613. [Google Scholar] [CrossRef]
- Pradhan, C.; Bhende, C. Enhancement in Primary Frequency Contribution using Dynamic Deloading of Wind Turbines. IFAC-PapersOnLine 2015, 48, 13–18. [Google Scholar] [CrossRef]
- Ma, H.T.; Chowdhury, B.H. Working towards frequency regulation with wind plants Combined control approaches. IET Renew. Power Gener. 2010, 4, 308–316. [Google Scholar] [CrossRef]
- Haes Alhelou, H.; Hamedani-Golshan, M.E.; Zamani, R.; Heydarian-Forushani, E.; Siano, P. Challenges and Opportunities of Load Frequency Control in Conventional, Modern and Future Smart Power Systems: A Comprehensive Review. Energies 2018, 11, 2497. [Google Scholar] [CrossRef]
- Kerdphol, T.; Watanabe, M.; Mitani, Y.; Phunpeng, V. Applying virtual inertia control topology to SMES system for frequency stability improvement of low-inertia microgrids driven by high renewables. Energies 2019, 12, 3902. [Google Scholar] [CrossRef]
- Cheema, K.M. A comprehensive review of virtual synchronous generator. Int. J. Electr. Power Energy Syst. 2020, 120, 106006. [Google Scholar] [CrossRef]
- Bevrani, H.; Ise, T.; Miura, Y. Virtual synchronous generators: A survey and new perspectives. Int. J. Electr. Power Energy Syst. 2014, 54, 244–254. [Google Scholar] [CrossRef]
- Tamrakar, U.; Shrestha, D.; Maharjan, M.; Bhattarai, B.P.; Hansen, T.M.; Tonkoski, R. Virtual inertia: Current trends and future directions. Appl. Sci. 2017, 7, 654. [Google Scholar] [CrossRef]
- Ali, M.H.; Wu, B.; Dougal, R.A. An Overview of SMES Applications in Power and Energy Systems. IEEE Trans. Sustain. Energy 2010, 1, 38–47. [Google Scholar] [CrossRef]
- Muttaqi, K.M.; Islam, M.R.; Sutanto, D. Future Power Distribution Grids: Integration of Renewable Energy, Energy Storage, Electric Vehicles, Superconductor, and Magnetic Bus. IEEE Trans. Appl. Supercond. 2019, 29, 1–5. [Google Scholar] [CrossRef]
- Thiesen, H.; Jauch, C.; Gloe, A. Design of a system substituting today’s inherent inertia in the European continental synchronous area. Energies 2016, 9, 582. [Google Scholar] [CrossRef]
- Hasanien, H.M.; El-Fergany, A.A. Symbiotic organisms search algorithm for automatic generation control of interconnected power systems including wind farms. IET Gener. Transm. Distrib. 2017, 11, 1692–1700. [Google Scholar] [CrossRef]
- WU, Q.; Bao, W.; Ding, L.; Huang, S.; Teng, F. Synthetic Inertial Control of Wind Farm with BESS Based on Model Predictive Control. IET Renew. Power Gener. 2020, 14, 2447–2455. [Google Scholar] [CrossRef]
- Gomez, L.A.G.; Grilo, A.P.; Salles, M.B.C.; Filho, A.J.S. Combined control of DFIG-based wind turbine and battery energy storage system for frequency response in microgrids. Energies 2020, 13, 894. [Google Scholar] [CrossRef]
- Yang, L.; Hu, Z.; Xie, S.; Kong, S.; Lin, W. Adjustable virtual inertia control of supercapacitors in PV-based AC microgrid cluster. Electr. Power Syst. Res. 2019, 173, 71–85. [Google Scholar] [CrossRef]
- Magdy, G.; Mohamed, E.A.; Shabib, G.; Elbaset, A.A.; Mitani, Y. SMES based a new PID controller for frequency stability of a real hybrid power system considering high wind power penetration. IET Renew. Power Gener. 2018, 12, 1304–1313. [Google Scholar] [CrossRef]
- Magdy, G.; Shabib, G.; Elbaset, A.A.; Mitani, Y. A novel coordination scheme of virtual inertia control and digital protection for microgrid dynamic security considering high renewable energy penetration. IET Renew. Power Gener. 2019, 13, 462–474. [Google Scholar] [CrossRef]
- Kerdphol, T.; Watanabe, M.; Hongesombut, K.; Mitani, Y. Self-Adaptive Virtual Inertia Control-Based Fuzzy Logic to Improve Frequency Stability of Microgrid with High Renewable Penetration. IEEE Access 2019, 7, 76071–76083. [Google Scholar] [CrossRef]
- Kim, J.; Muljadi, E.; Gevorgian, V.; Mohanpurkar, M.; Luo, Y.; Hovsapian, R.; Koritarov, V. Capability-coordinated frequency control scheme of a virtual power plant with renewable energy sources. IET Gener. Transm. Distrib. 2019, 13, 3642–3648. [Google Scholar] [CrossRef]
- Abo-Elyousr, F.K.; Abdelaziz, A.Y. A Novel Modified Robust Load Frequency Control for Mass-Less Inertia Photovoltaics Penetrations via Hybrid PSO-WOA Approach. Electr. Power Compon. Syst. 2019, 47, 1744–1758. [Google Scholar] [CrossRef]
- Yap, K.Y.; Sarimuthu, C.R.; Lim, J.M.Y. Grid Integration of Solar Photovoltaic System Using Machine Learning-Based Virtual Inertia Synthetization in Synchronverter. IEEE Access 2020, 8, 49961–49976. [Google Scholar] [CrossRef]
- Zhang, X.; Zhu, Z.; Fu, Y.; Li, L. Optimized virtual inertia of wind turbine for rotor angle stability in interconnected power systems. Electr. Power Syst. Res. 2020, 180, 106157. [Google Scholar] [CrossRef]
- Alam, M.S.; Razzak, M.A.; Shafiullah, M.; Chowdhury, A.H. Application of TCSC and SVC in damping oscillations in Bangladesh Power System. In Proceedings of the 7th International Conference on Electrical and Computer Engineering, Dhaka, Bangladesh, 20–22 December 2012; IEEE: New York, NY, USA, 2012; pp. 571–574. [Google Scholar] [CrossRef]
- Pappachen, A.; Fathima, A.P. Load frequency control in deregulated power system integrated with SMES–TCPS combination using ANFIS controller. Int. J. Electr. Power Energy Syst. 2016, 82, 519–534. [Google Scholar] [CrossRef]
- Birs, I.; Muresan, C.; Nascu, I.; Ionescu, C. A Survey of Recent Advances in Fractional Order Control for Time Delay Systems. IEEE Access 2019, 7, 30951–30965. [Google Scholar] [CrossRef]
- Padula, F.; Visioli, A. Tuning rules for optimal PID and fractional-order PID controllers. J. Process Control 2011, 21, 69–81. [Google Scholar] [CrossRef]
- Muresan, C.I.; Dutta, A.; Dulf, E.H.; Pinar, Z.; Maxim, A.; Ionescu, C.M. Tuning algorithms for fractional order internal model controllers for time delay processes. Int. J. Control 2016, 89, 579–593. [Google Scholar] [CrossRef]
- Shah, P.; Agashe, S. Review of fractional PID controller. Mechatronics 2016, 38, 29–41. [Google Scholar] [CrossRef]
- Cao, J.Y.; Liang, J.; Cao, B.G. Optimization of Fractional Order PID controllers based on genetic algorithms. In Proceedings of the International Conference Machine and Learning Cybernetics ICMLC, Guangzhou, China, 18–21 August 2005; Springer: Berlin/Heidelberg, Germany, 2005; pp. 5686–5689. [Google Scholar] [CrossRef]
- Chang, L.Y.; Chen, H.C. Tuning of fractional PID controllers using adaptive genetic algorithm for active magnetic bearing system. WSEAS Trans. Syst. 2009, 8, 158–167. [Google Scholar]
- Aghababa, M.P. Optimal design of fractional-order PID controller for five bar linkage robot using a new particle swarm optimization algorithm. Soft Comput. 2016, 20, 4055–4067. [Google Scholar] [CrossRef]
- Biswas, A.; Das, S.; Abraham, A.; Dasgupta, S. Design of fractional-order PIλDμ controllers with an improved differential evolution. Eng. Appl. Artif. Intell. 2009, 22, 343–350. [Google Scholar] [CrossRef]
- Pan, I.; Das, S. Fractional-order load-frequency control of interconnected power systems using chaotic multi-objective optimization. Appl. Soft Comput. J. 2015, 29, 328–344. [Google Scholar] [CrossRef]
- Kesarkar, A.A.; Selvaganesan, N. Tuning of optimal fractional-order PID controller using an artificial bee colony algorithm. Syst. Sci. Control Eng. 2015, 3, 99–105. [Google Scholar] [CrossRef]
- Kumar, M.R.; Deepak, V.; Ghosh, S. Fractional-order controller design in frequency domain using an improved nonlinear adaptive seeker optimization algorithm. Turkish J. Electr. Eng. Comput. Sci. 2017, 25, 4299–4310. [Google Scholar] [CrossRef]
- Mosaad, M.I. Direct power control of SRG-based WECSs using optimised fractional-order PI controller. IET Electr. Power Appl. 2020, 14, 409–417. [Google Scholar] [CrossRef]
- Ateş, A.; Yeroglu, C. Optimal fractional order PID design via Tabu Search based algorithm. ISA Trans. 2016, 60, 109–118. [Google Scholar] [CrossRef]
- Pham, Q.; Mirjalili, S.; Member, S.; Kumar, N.; Member, S. Whale Optimization Algorithm With Applications to Resource Allocation in Wireless Networks. IEEE Trans. Veh. Technol. 2020, 69, 4285–4297. [Google Scholar] [CrossRef]
- Lee, D.J.; Wang, L. Small-signal stability analysis of an autonomous hybrid renewable energy power generation/energy storage system part I: Time-domain simulations. IEEE Trans. Energy Convers. 2008, 23, 311–320. [Google Scholar] [CrossRef]
- Morsali, J.; Zare, K.; Tarafdar Hagh, M. Appropriate generation rate constraint (GRC) modeling method for reheat thermal units to obtain optimal load frequency controller (LFC). In Proceedings of the 5th Conference on Thermal Power Plants (CTPP), Tehran, Iran, 10–11 June 2014; IEEE: New York, NY, USA, 2015; pp. 29–34. [Google Scholar] [CrossRef]
- Alam, M.S.; Abido, M.A.Y. Fault ride-through capability enhancement of voltage source converter-high voltage direct current systems with bridge type fault current limiters. Energies 2017, 10, 1898. [Google Scholar] [CrossRef]
- Li, H.; Luo, Y.; Chen, Y. A fractional order proportional and derivative (FOPD) motion controller: Tuning rule and experiments. IEEE Trans. Control Syst. Technol. 2010, 18, 516–520. [Google Scholar] [CrossRef]
- Luo, Q.; Ling, Y.; Zhou, Y. Modified Whale Optimization Algorithm for Infinitive Impulse Response System Identification. Arab. J. Sci. Eng. 2020, 45, 2163–2176. [Google Scholar] [CrossRef]
- Mirjalili, S.; Lewis, A. The Whale Optimization Algorithm. Adv. Eng. Softw. 2016, 95, 51–67. [Google Scholar] [CrossRef]










| Parameters | Value | |
|---|---|---|
| Area-1 | Area-2 | |
| Inertia | 0.082 | 0.13 |
| Damping constant | 0.0165 | 0.0195 |
| Solar system time constant | 1.2 | 1.2 |
| Wind system time constant | 1.4 | 1.4 |
| Frequency bias factor | 0.3674 | 0.4103 |
| Maximum limit of valve gate | 0.5 | 0.5 |
| Minimum limit of valve gate | −0.5 | −0.5 |
| Synchronizing coefficient | 0.09 | 0.09 |
| Area capacity ratio | −0.055 | 0.055 |
| GRC | 0.3 | 0.3 |
| Optimized Parameters | |||
|---|---|---|---|
| Parameters Name | Value | Parameters Name | Value |
| 13.0182 | 0.1595 | ||
| 110.428 | 0.2360 | ||
| 0.88310 | 0.0053 | ||
| 35.1420 | 3.0000 | ||
| 130.815 | 0.0053 | ||
| 0.51580 | 2.8051 | ||
| Indices | No Auxiliary Controller | With SMES | Optimized FOC-Based SMES |
|---|---|---|---|
| Maximum overshoot, Hz | 0.650 | 0.100 | 0.004 |
| Maximum undershoot, Hz | 0.250 | 0.025 | 0.000 |
| Settling time, sec | inf | 0075 | 0.900 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alam, M.S.; Alotaibi, M.A.; Alam, M.A.; Hossain, M.A.; Shafiullah, M.; Al-Ismail, F.S.; Rashid, M.M.U.; Abido, M.A. High-Level Renewable Energy Integrated System Frequency Control with SMES-Based Optimized Fractional Order Controller. Electronics 2021, 10, 511. https://doi.org/10.3390/electronics10040511
Alam MS, Alotaibi MA, Alam MA, Hossain MA, Shafiullah M, Al-Ismail FS, Rashid MMU, Abido MA. High-Level Renewable Energy Integrated System Frequency Control with SMES-Based Optimized Fractional Order Controller. Electronics. 2021; 10(4):511. https://doi.org/10.3390/electronics10040511
Chicago/Turabian StyleAlam, Md. Shafiul, Majed A. Alotaibi, Md Ahsanul Alam, Md. Alamgir Hossain, Md Shafiullah, Fahad Saleh Al-Ismail, Md. Mamun Ur Rashid, and Mohammad A. Abido. 2021. "High-Level Renewable Energy Integrated System Frequency Control with SMES-Based Optimized Fractional Order Controller" Electronics 10, no. 4: 511. https://doi.org/10.3390/electronics10040511
APA StyleAlam, M. S., Alotaibi, M. A., Alam, M. A., Hossain, M. A., Shafiullah, M., Al-Ismail, F. S., Rashid, M. M. U., & Abido, M. A. (2021). High-Level Renewable Energy Integrated System Frequency Control with SMES-Based Optimized Fractional Order Controller. Electronics, 10(4), 511. https://doi.org/10.3390/electronics10040511











