A Survey of Candidate Waveforms for beyond 5G Systems
Abstract
1. Introduction
- More flexible and efficient use of the current spectrum available in sub-6 Ghz bands, which may include the aggregation of non-contiguous and fragmented under-utilized spectrum bands for different network deployment scenarios [11];
- Expand the operation of 5G and beyond mobile network to consider also carrier frequencies above 6 GHz, enabling high capacity and high throughput services with low latency [12];
- Enhanced higher-order modulations, frame structure, multiple access and coding schemes;
- The concept of network slicing, which uses resources when and where needed, that are after released [15].
- To provide a detailed overview of some promising multi-carrier waveforms.
- To perform an analysis between these waveforms regarding the peak-to-average power ratio (PAPR), power spectral density (PSD) and computation complexity.
- To derive a performance comparison between the waveforms in typical channel models.
2. Waveform Key Performance Indicators for 5G and beyond Communications
- High Spectral Efficiency: Spectral efficiency is an important parameter since it indicates the achievable amount of bits that can be transmitted per second and per unit of bandwidth (bits/s/Hz), thus defining the maximum attainable bit rate given the available bandwidth. It is crucial to transmit the maximum amount data, using the minimum bandwidth that is possible, due to both licensing requirements and the spectrum scarcity resulting from the increasing transmission bandwidth requirement with demand for any time. Low spectral efficiency waveform formats can lead to high spectral amplitude outside the allocated bandwidth. This is known as out of band (OOB) radiation, which causes multiplexed services being transmitted on adjacent frequency channels to interfere with each other, a phenomena known as inter-channel interference (ICI) [6].
- Peak-to-average-power-ratio: The PAPR indicates the ratio between the maximum peak and the average transmitted power of the signal. A high PAPR results from the large fluctuations of the signal’s envelope and it is associated to a high power consumption at the base station’s terminals front-end, decreasing transmission energy efficiency. This is mainly due to the need of using linear power amplifiers, that are poorly efficient and is even lower when they are operated with some amount of back-off in order to avoid amplifier’s saturation and signal distortion (which can lead to spectral regrowth and higher bit error rates (BERs)) [24,25].
- Processing delay: Directly related to the URLLC 5G requirement, a waveform format with high complexity and large block processing delays increases the overall latency. The processing delay can be controlled by reducing the symbol temporal duration or period or increasing the sub-carrier spacing, which can be performed by efficient algorithms and signal processing techniques [4,6].
- Robustness to frequency-selective channels: When the transmitted signal travels through a wireless channel, it travels trough several paths with varied length, with multiple echos of the signal reaching the receiver. This causes an effect denoted as multipath fading [26]. The several copies of the waves that carry the transmitted signal arrive at the receiver with random amplitudes, frequencies and phases and can be combined constructively and destructively, interfering with one another. This leads to a temporal dispersion of the signal which can induce inter-symbol interference (ISI), impacting severely the transmission. Therefore, waveforms must be designed in order to be robust to this impairment.
- Robustness to time-selective channels: In wireless environments, transmitter and receiver mobility and, consequently, time-varying channels are still an open issue. The waveform transceiver system must be design in order to be robust to time-selective channels, by taking into account the channel coherence time, related to changes in the amplitudes, delays and the number of multipath components are observed. In fact, larger transmitted blocks can lead to higher sensibility to both carrier frequency offset (CFO) and Doppler effects [6].
- Massive Asynchronous Transmission: In 5G and beyond systems, a high number of communicating nodes will be communicating at a given time. In order to efficiently utilize network resources, asynchronous multiple access is essential. Thus, waveforms designs that are well localized in a multiplexed domain by allowing asymmetric and dynamic allocation of both time and frequency resources, as in frequency division duplex (FDD) and time division duplex (TDD), can achieve higher throughput through more efficient channel utilization [6].
- Complexity: The hardware and computation complexity represent a critical metric. It mainly depends on the the number of operations required at the transmitter or receiver, which may include windowing, filtering operations, as well as interference cancellation algorithms. The overall system complexity will influence the cost and the energy efficiency of the system and can represent a bottleneck upon selecting and determining the most suitable waveform candidate to be implemented for a certain type of applications [4].
- High flexibility, reliability and MIMO friendless: The ideal waveform should also be able to support the coexistence of different numerologies and multi-numerology to enable several services, while allowing dynamic allocation of bandwidth for these (numerologies/services) [9]. An extremely high reliability is also necessary. This means that the evaluated BER performances for the chosen waveform should be better, or at least, similar to previous standard waveforms. The new waveform should support and be extended to MIMO (especially massive-MIMO), without requiring much additional effort.
- Filtering/Windowing: The waveform should allow a filtering and/or windowing operation to be performed in both the transmission and reception stages, in order to manage the OOB emissions and latency. On the one hand, a wide filter bandwidth, which results in shorter filter length (in time domain), can control the system latency. However they are not very efficient at lowering the OOB emissions. On the other hand, a narrow filter implies very low OOB emissions but results in a long filter length (in time domain) which increases the system latency. Hence, there must be a trade-off between low OOB emissions and low latency [6].
3. Candidate Waveforms
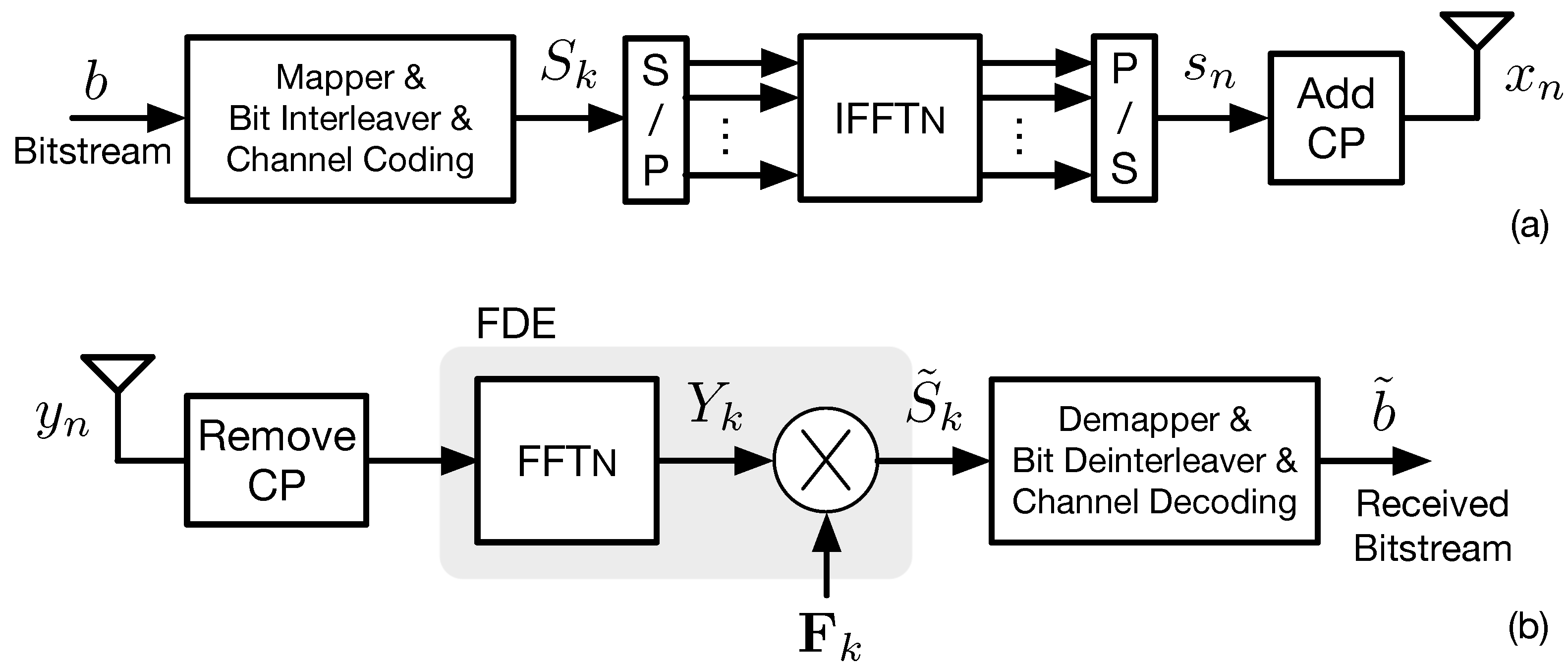
3.1. Orthogonal Frequency Division Multiplexing
3.2. Filtered Orthogonal Frequency Division Multiplexing
3.2.1. F-OFDM Transmitter and Filter Design
3.2.2. F-OFDM Receiver
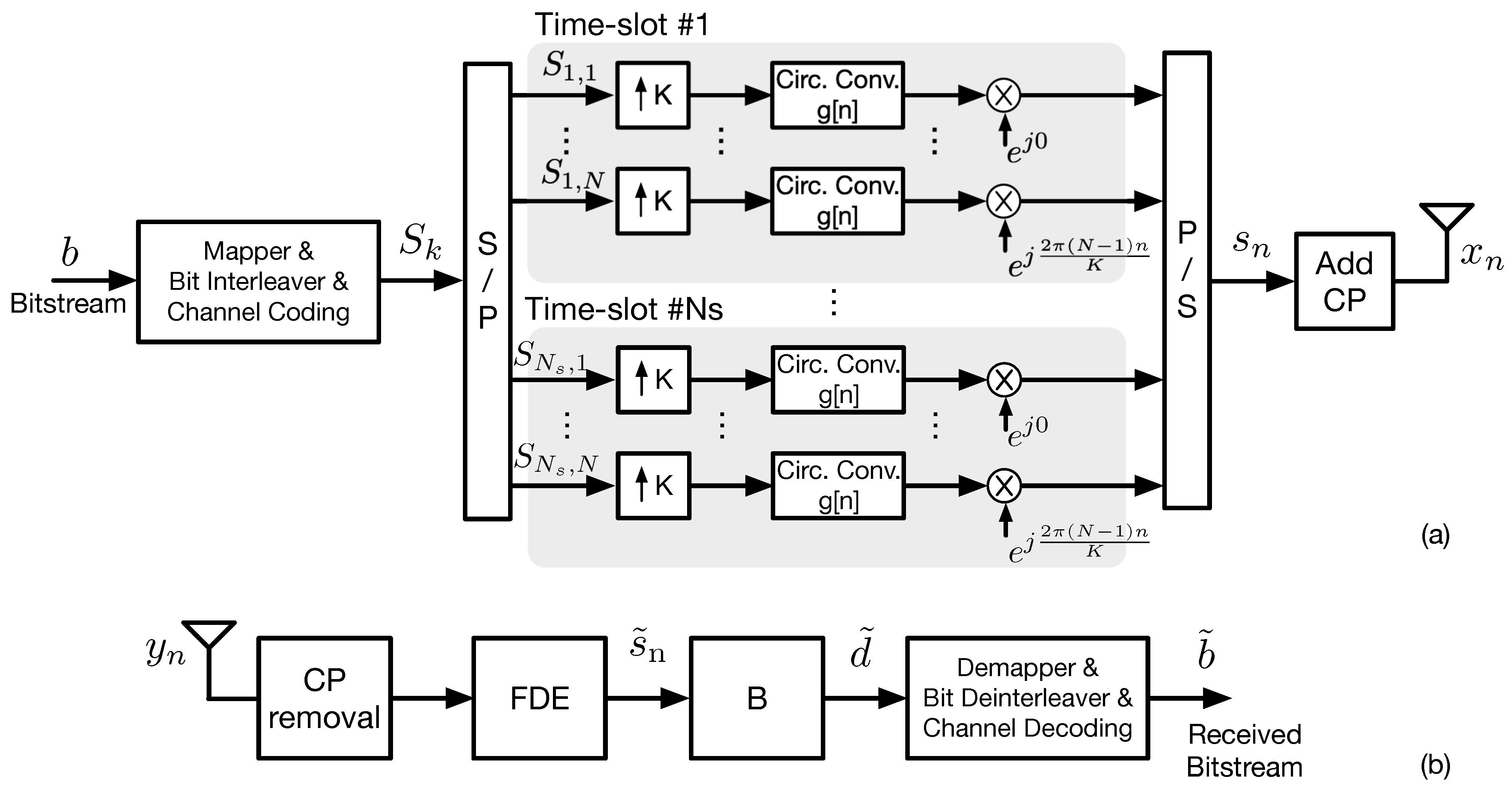
3.3. Generalized Frequency Division Multiplexing
3.3.1. GFDM Transmitter
- The possibility of adjusting the sub-carrier spacing allows a reduction on the OOB emissions.
- In the GFDM block construction, the overhead needed to avoid IBI is relatively small. Instead of adding a CP to every symbol like CP-OFDM schemes, the GFDM transmitter includes the addition of a single CP for an entire block that includes multiple sub-symbols [43], resulting in . Windowing techniques can also be employed in the GFDM multi-symbols in order to avoid discontinuities due to tail-biting.
3.3.2. GFDM Receiver
- Match Filtering (MF), where the same filter included in the transmitter is now applied to each received block, i.e., , where H is the Hermitian operator (conjugate and transpose). This maximizes the SNR ratio per sub-carrier, but introduces self-interference when a non-orthogonal transmit pulse is employed [43].
- Zero Forcing (ZF), where the inverse of matrix , presented in (7), is applied to recover the data symbols, i.e., . This approach completely removes any ICI at the cost of enhancing the influence of the noise in the detected symbols.
- SIC, which is similar to DSSIC but only the interference from the th sub-carrier is compensated.
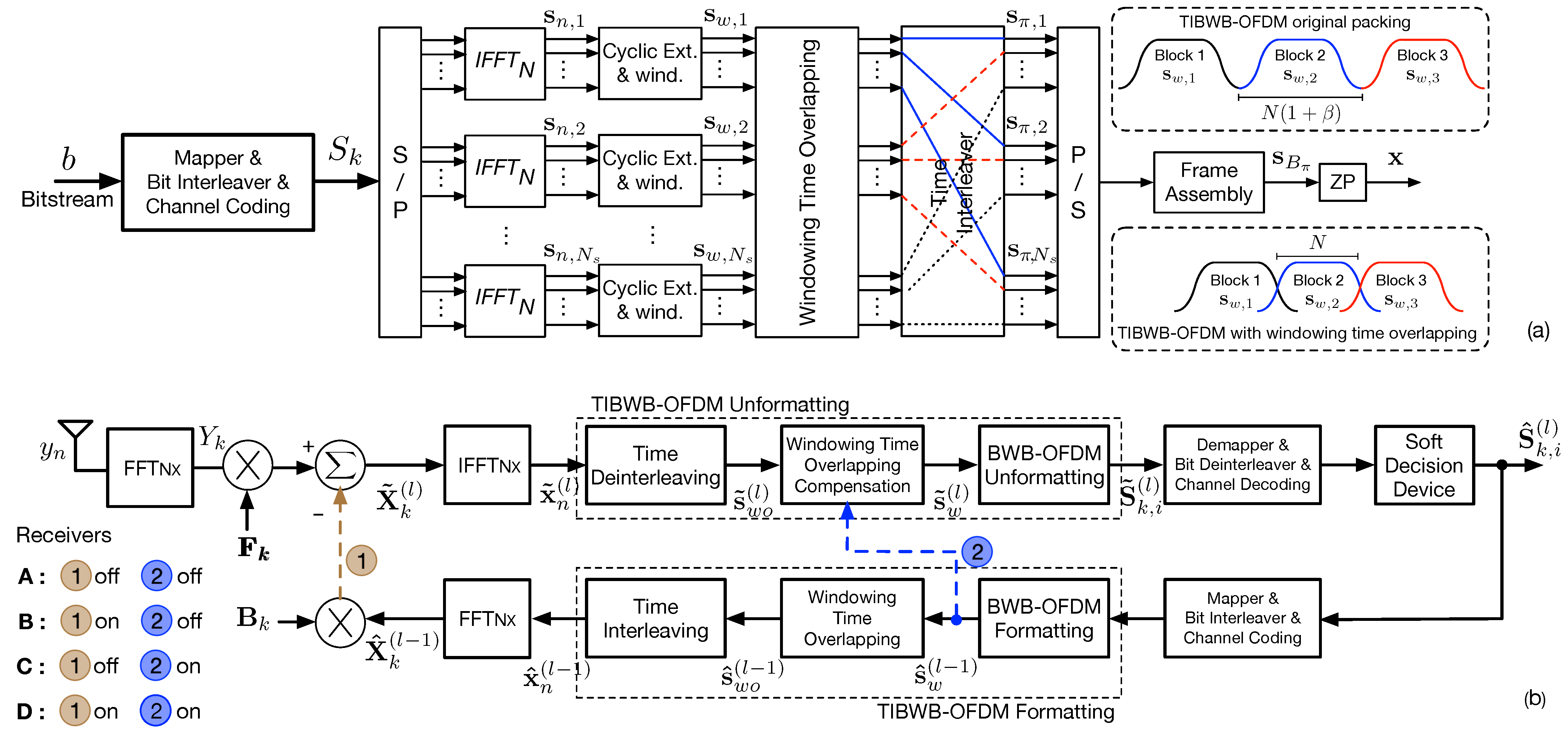
3.4. Time Interleaved Block Windowed Burst Orthogonal Frequency Division Multiplexing
3.4.1. TIBWB-OFDM
3.4.2. TIBWB-OFDM Packing with WTO
- improving spectral confinement by reducing OOB emission when using a larger roll-off. This however, results in greater multi-symbol length, which increases the required bandwidth, in order to keep transmission rate [49].
- by improving symbol rate when conventional rectangular window is used since a sole ZP is used per group of packed OFDM-based blocks. This results in very high OOB emissions, just like typical OFDM schemes [49].
3.4.3. Receivers for TIBWB-OFDM with WTO
- Receiver A (Link 1—off; Link 2—off): It consists on the linear MMSE FDE technique, presented in (10) to deal with channel impairments and an MMSE ISC algorithm to cancel WTO interference.
- Receiver B (Link 1—on; Link 2—off): It consists on the non-linear IB-DFE FDE [47] and MMSE ISC algorithms. Each iteration allows an improvement in BER performance since the ISC algorithm is applied iteratively.
- Receiver C (Link 1—off; Link 2—on): This receiver is similar to A since it includes the linear MMSE FDE scheme. However, instead of applying the MMSE ISC algorithm per iteration, an iterative IBIC algorithm is employed, assuming perfect reconstruction.
- Receiver D (Link 1—on; Link 2—on): This receiver is a combination of both receivers B and C, wherein the IB-DFE FDE technique and the iterative IBIC algorithm are employed.
4. Performance Results and Discussion
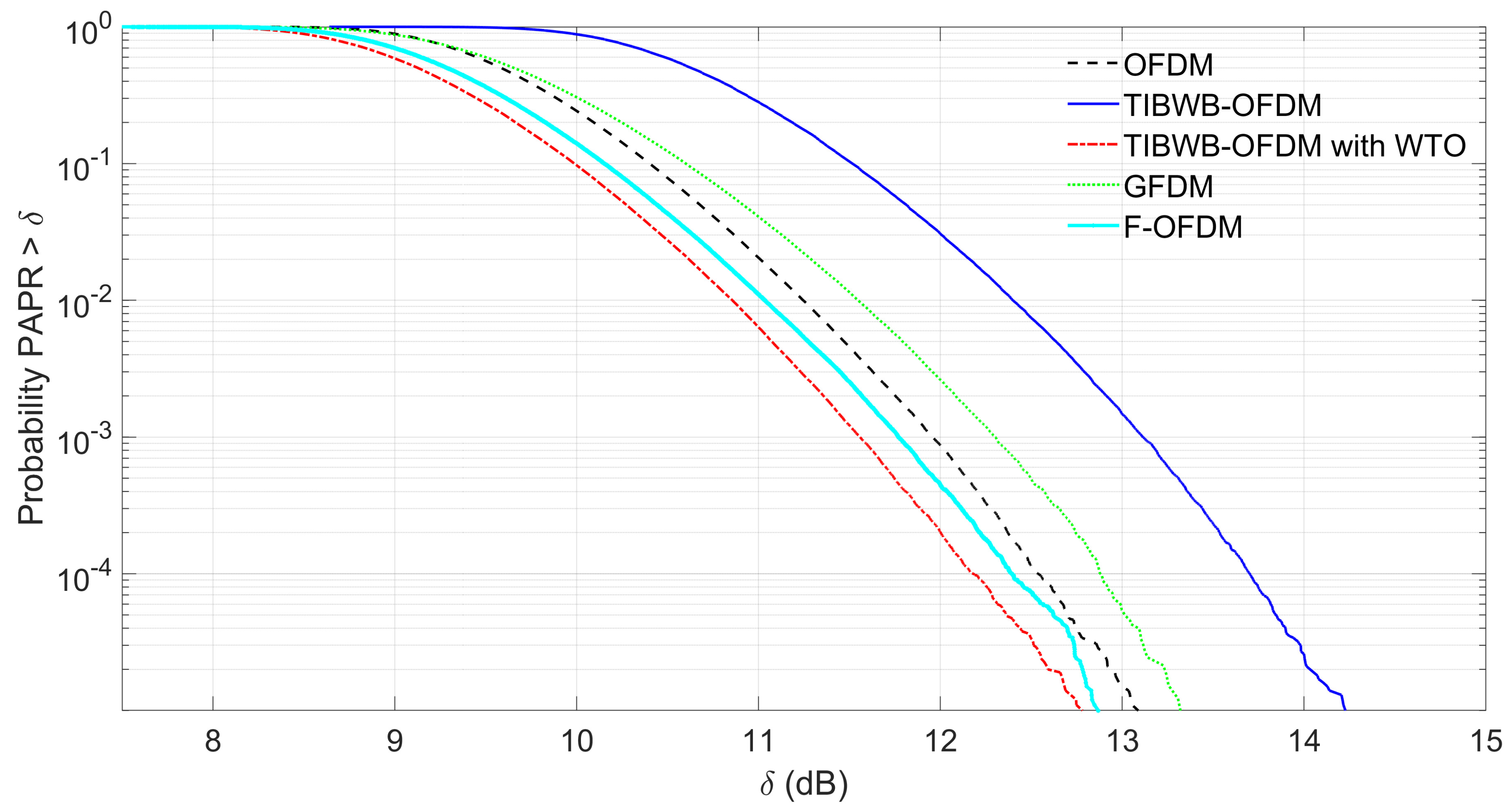
4.1. PAPR
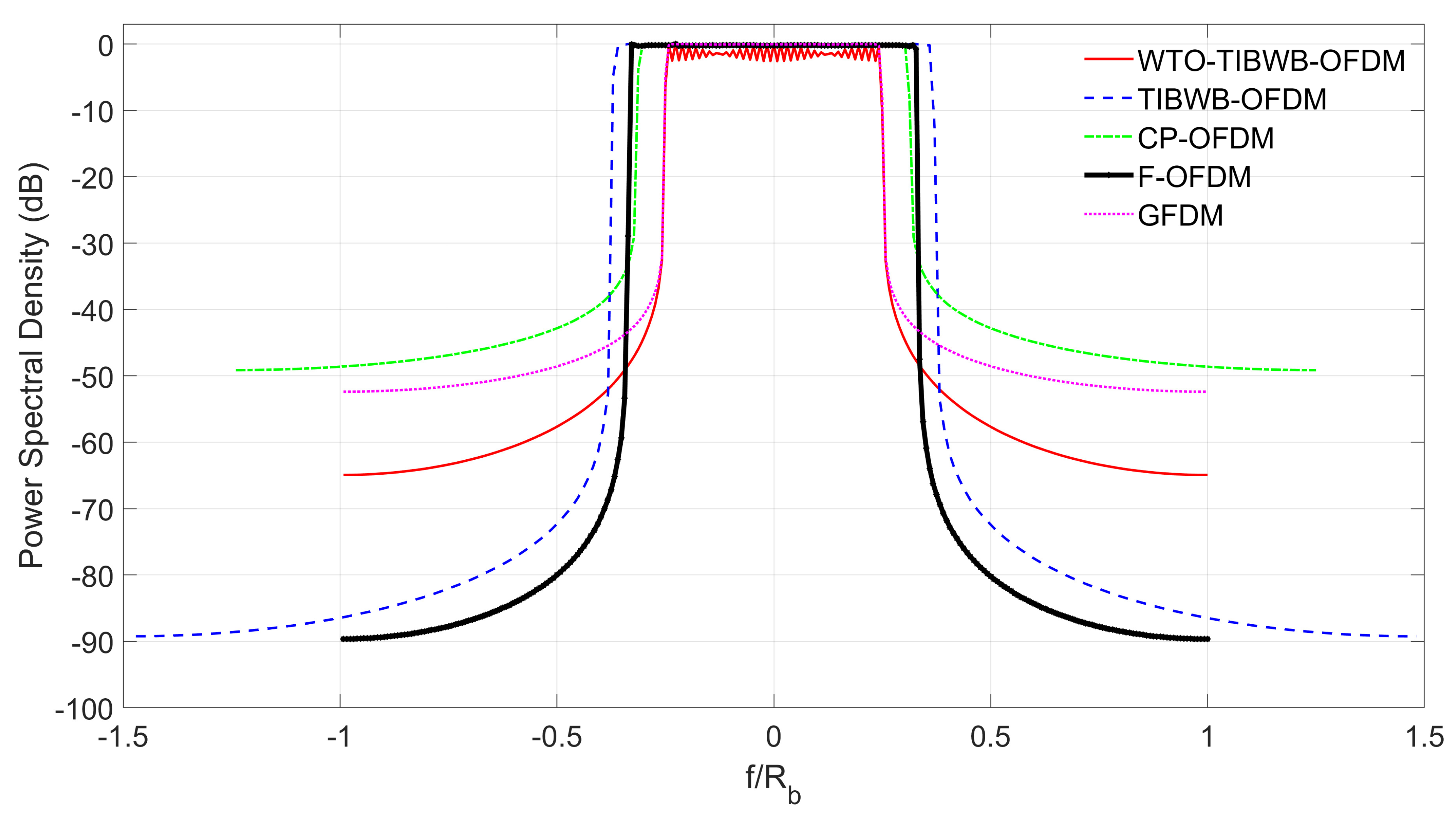
4.2. PSD and Spectral Efficiency
4.3. BER Performance
4.4. Computational Complexity
- Receiver A includes only a direct path, where includes a -sized FFT upon signal reception, a MMSE equalization algorithm and BWB-OFDM unformatting with WTO compensation.
- Receivers B and D include the direct path but instead of MMSE equalization, the IB-DFE algorithm is employed. The feedback path wherein the BWB-OFDM block formatting is performed is also included. For L iterations, the number of multiplications will increase proportionally by L in the direct path and in the feedback path.
- Receiver C is similar to receiver A in the first iteration, performing all the operation in the direct path, while including the BWB-OFDM formatting operation in the feedback path. For , the direct path only includes the BWB unformatting with WTO compensation and the feedback path includes the BWB-OFDM formatting.
4.5. Further KPI Discussion
- Processing delay and filtering/windowing: These KPI are directly related. GFDM relies on a CP insertion and performs the filtering operation by sub-carrier, after an upsample operation, requiring a long filter length (narrow bandwidth). Thus, the overall block processing delay will be high [4,6]. Both TIBWB-OFDM with and without WTO perform the filtering operating per sub-band, and thus, they use shorter filter length (wide bandwidth). However, the overall system delay is still high because the systems require that each one of the OFDM-based sub-symbol go through several operations. Besides, FFT modulation formats involving a relatively high duration multi-symbols are employed. Therefore, the overall block processing delay will be high for all these waveforms and they are not suitable for low latency applications. Additionally, F-OFDM schemes use shorter filter lengths and although including large CP lengths, the overall symbol duration remains low, compared to the previous waveforms. Thus, from the point of view of this KPI, the most suitable waveforms are OFDM and F-OFDM.
- Robustness to frequency-selective channels: Overall, all the MC waveforms are robust to the frequency selectivity of the wireless channel. The OFDM principle is to divide the transmission channel’s bandwidth into narrowband sub-carriers, by transforming a broadband frequency selective channel into multiple narrowband flat-fading sub-channels. Therefore, deep fadings will affect only a few sub-carriers. F-OFDM is based on OFDM schemes and GFDM can be seen as a generalization of OFDM [68], with both presenting the same robustness as OFDM, regarding multipath propagation. Both TIBWB-OFDM with and without WTO go beyond that and allow a deeper level of robustness against deep fading [46], due to the inclusion of the time interleave/deinterleave operations in their transceiver design, granting a higher degree of diversity in the frequency domain and robustness upon transmission under deep inband channel fades. Thus, from the point of view of this KPI, the most suitable waveforms are TIBWB-OFDM with and without WTO.
- Robustness to time-selective channels: When the user mobility is taken into account, the changes of the transmission channel can cause ICI, affecting all the MC waveforms. F-OFDM is as robust as OFDM regarding this KPI. However, in [38,47] it is shown, respectively, that both GFDM and TIBWB-OFDM waveforms are MC schemes that are relatively robust regarding this impairment. In GFDM the use of very well localised pulse shapes in the frequency domain allows a certain degree of CFO resilience [38]. Additionally, in TIBWB-OFDM, the large multi-symbol length can also allow a more accurate estimation of the CFO or Doppler drift based on the IB-DFE principle [47]. This way, GFDM and TIBWB-OFDM with and without WTO are the most suitable waveforms to be considered in a mobile transmission/reception environment.
- High flexibility and efficient MIMO implementation: All of the waveform contenders presented in this paper are flexible with the possibility of employing multiple numerology parameters since they are based on OFDM scheme. A friendly MIMO adaptation is directly related to the implementation complexity regarding the channel equalization techniques that are employed in the system [6]. In general, OFDM-based waveforms (F-OFDM and TIBWB-OFDM) allow an efficient MIMO implementation since the transceiver architecture allows a simplification in the FDE with only one equalization iteration per sub-carrier with simple channel estimation techniques. Also, in [63], it is shown that the TIBWB-OFDM waveform is also easily integrated in MIMO systems. GFDM is an exception since the sub-carrier superposition is performed in frequency domain causing ICI that must be dealt in the receiver, requiring a channel estimation in each sub-symbol [6]. However, in TIBWB-OFDM with WTO the interference is added locally between adjacent sub-symbols in time domain. Hence, concerning this KPI, the only waveform that is not recommended is GFDM.
4.6. Final Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DFT | Discrete Fourier Transform |
| KPI | Key Performance Indicator |
| eMBB | Enhanced Mobile Broadband |
| mMTC | Massive Machine-Type Communication |
| CCDF | Complementary Cumulative Distribution Function |
| URLLC | Ultra-Reliable and Low-Latency Communication |
| GFDM | Generalized Frequency Division Multiplexing |
| FDE | Frequency Domain Equalization |
| TIBWB-OFDM | Time Interleaved Block Windowed Burst OFDM |
| MIMO | Multiple-Input-Multiple-Output |
| MMSE | Minimum Mean Square Error |
| IBIC | Inter-Block Interference Cancellation |
| ISC | Interference Successive Cancellation |
| AWGN | Additive White Gaussian Noise |
| ICI | Inter-Channel Interference |
| SRRC | Square Root Raised Cosine |
| IB-DFE | Iterative Block Decision Feedback Equalizer |
| DSSIC | Double Sided Interference Cancellation |
| PAPR | Peak-to-Average-Power-Ratio |
| IFFT | Inverse Fast Fourier Transform |
| WTO | Windowing Time Overlapping |
| FDD | Frequency Division Duplex |
| ITSBI | Inter Sub-Band Interference |
| OFDM | Orthogonal Frequency Division Multiplexing |
| 3GPP | 3rd Generation Partnership Project |
| S/P | Serial to Parallel |
| DL | Downlink |
| ISI | Inter-Symbol Interference |
| PSD | Power Spectral Density |
| IoT | Internet of Things |
| 5G | Fifth Generation |
| UL | Uplink |
| F-OFDM | Filtered-OFDM |
| UE | User Equipment |
| CFO | Carrier Frequency Offset |
| OOB | Out of Band |
| AP | Access Point |
| mm | Milli-Meter |
| PHY | Physical Layer |
| BS | Base Station |
| SNR | Signal-to-Noise Ratio |
| LTE | Long Term Evolution |
| BER | Bit Error Rate |
| MF | Match Filtering |
| ZF | Zero-Forcing |
| SC | Single-Carrier |
| TDD | Time Division Duplex |
| MC | Multi-Carrier |
| CP | Cyclic Prefix |
| INSBI | Inner Sub-Band Interference |
References
- Strinati, E.C.; Barbarossa, S.; Gonzalez-Jimenez, J.L.; Kténas, D.; Cassiau, N.; Dehos, C. 6G: The Next Frontier. arXiv 2019, arXiv:1901.03239. [Google Scholar]
- Chin, W.H.; Fan, Z.; Haines, R. Emerging technologies and research challenges for 5G wireless networks. IEEE Wirel. Commun. 2014, 21, 106–112. [Google Scholar] [CrossRef]
- Norp, T. 5G Requirements and Key Performance Indicators. J. ICT 2018, 6, 15–30. [Google Scholar] [CrossRef]
- Demir, A.F.; Elkourdi, M.; Ibrahim, M.; Arslan, H. Waveform Design for 5G and Beyond. In 5G Networks: Fundamental Requirements, Enabling Technologies, and Operations Management; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2018; pp. 51–76. [Google Scholar]
- Andrews, J.G.; Buzzi, S.; Choi, W.; Hanly, S.; Lozano, A.; Soong, A.C.K.; Zhang, J.C. What Will 5G Be? IEEE J. Sel. Areas Commun. 2014, 32, 1065–1082. [Google Scholar] [CrossRef]
- Santacruz, J.P.; Rommel, S.; Johannsen, U.; Jurado-Navas, A.; Monroy, I.T. Candidate Waveforms for ARoF in Beyond 5G. Appl. Sci. 2020, 10, 3891. [Google Scholar] [CrossRef]
- International Telecommunications Union-Radiocommunications Sector (ITU-R). IMT Vision—Framework and Overall Objectives of the Future Development of IMT for 2020 and Beyond; Technical Report M.2083-0; ITU: Geneva, Switzerland, 2015. [Google Scholar]
- Wunder, G.; Kasparick, M.; ten Brink, S.; Schaich, F.; Wild, T.; Gaspar, I.; Ohlmer, E.; Krone, S.; Michailow, N.; Navarro, A.; et al. 5GNOW: Challenging the LTE Design Paradigms of Orthogonality and Synchronicity. In Proceedings of the 2013 IEEE VTC Spring, Dresden, Germany, 2–5 June 2013; pp. 1–5. [Google Scholar]
- Zhang, X.; Chen, L.; Qiu, J.; Abdoli, J. On the Waveform for 5G. IEEE Commun. Mag. 2016, 54, 74–80. [Google Scholar] [CrossRef]
- Balint, C.; Budura, G. OFDM-Based Multi-Carrier Waveforms Performances in 5G. In Proceedings of the 2018 International Symposium on Electronics and Telecommunications (ISETC), Timisoara, Romania, 8–9 November 2018; pp. 1–4. [Google Scholar]
- Gerzaguet, R.; Bartzoudis, N.; Baltar, L.G.; Berg, V.; Doré, J.B.; Kténas, D.; Font-Bach, O.; Mestre, X.; Payaró, M.; Färber, M.; et al. The 5G candidate waveform race: A comparison of complexity and performance. EURASIP J. Wirel. Commun. Netw. 2017, 2017, 13. [Google Scholar] [CrossRef]
- Zaidi, A.A.; Luo, J.; Gerzaguet, R.; Wolfgang, A.; Zaidi, A.A.; Weiler, R.J.; Vihriäla, J.; Svensson, T.; Qi, Y.; Halbauer, H.; et al. A Preliminary Study on Waveform Candidates for 5G Mobile Radio Communications above 6 GHz. In Proceedings of the 2016 IEEE 83rd Vehicular Technology Conference (VTC Spring), Nanjing, China, 15–18 May 2016; pp. 1–6. [Google Scholar]
- Dehos, C.; Gonzalez, J.; Domenico, A.; Ktenas, D.; Dussopt, L. Millimeter-Wave Access and Backhauling: The Solution to the Exponential Data Traffic Increase in 5G Mobile Communications Systems. IEEE Commun. Mag. 2014, 52, 88–95. [Google Scholar] [CrossRef]
- Tesanovic, M.; Nekovee, M. mmWave-Based Mobile Access for 5G: Key Challenges and Projected Standards and Regulatory Roadmap. In Proceedings of the 2015 IEEE Global Communications Conference (GLOBECOM), San Diego, CA, USA, 6–10 December 2015; pp. 1–6. [Google Scholar]
- Kukliński, S.; Tomaszewski, L. Key Performance Indicators for 5G network slicing. In Proceedings of the 2019 IEEE Conference on Network Softwarization (NetSoft), Paris, France, 24–28 June 2019; pp. 464–471. [Google Scholar]
- Marzetta, T.; Larsson, E.; Yang, H.; Ngo, H. Fundamentals of Massive MIMO; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar]
- Björnson, E.; Larsson, E.G.; Marzetta, T.L. Massive MIMO: Ten myths and one critical question. IEEE Commun. Mag. 2016, 54, 114–123. [Google Scholar] [CrossRef]
- Interdonato, G.; Björnson, E.; Ngo, H.; Frenger, P.; Larsson, E. Ubiquitous Cell-Free Massive MIMO Communications. EURASIP J. Wirel. Commun. Netw. 2019, 2019, 197. [Google Scholar] [CrossRef]
- Zhang, J.; Chen, S.; Lin, Y.; Zheng, J.; Ai, B.; Hanzo, L. Cell-Free Massive MIMO: A New Next-Generation Paradigm. IEEE Access 2019, 7, 99878–99888. [Google Scholar] [CrossRef]
- Ngo, H.Q.; Ashikhmin, A.; Yang, H.; Larsson, E.G.; Marzetta, T.L. Cell-Free Massive MIMO Versus Small Cells. IEEE Trans. Wirel. Commun. 2017, 16, 1834–1850. [Google Scholar] [CrossRef]
- Boccardi, F.; Heath, R.W.; Lozano, A.; Marzetta, T.L.; Popovski, P. Five disruptive technology directions for 5G. IEEE Commun. Mag. 2014, 52, 74–80. [Google Scholar] [CrossRef]
- Zaidi, A.A.; Baldemair, R.; Tullberg, H.; Bjorkegren, H.; Sundstrom, L.; Medbo, J.; Kilinc, C.; Da Silva, I. Waveform and Numerology to Support 5G Services and Requirements. IEEE Commun. Mag. 2016, 54, 90–98. [Google Scholar] [CrossRef]
- Wunder, G.; Jung, P.; Kasparick, M.; Wild, T.; Schaich, F.; Chen, Y.; Brink, S.T.; Gaspar, I.; Michailow, N.; Festag, A.; et al. 5GNOW: Non-orthogonal, asynchronous waveforms for future mobile applications. IEEE Commun. Mag. 2014, 52, 97–105. [Google Scholar] [CrossRef]
- Rohde & Schwarz. 5G Waveform Candidates. Tech. Rep. June 2016. Available online: https://www.rohde-schwarz.com/nl/applications/5g-waveform-candidates-application-note56280-267585.html (accessed on 7 December 2020).
- Rahmatallah, Y.; Mohan, S. Peak-To-Average Power Ratio Reduction in OFDM Systems: A Survey and Taxonomy. IEEE Commun. Surv. Tutor. 2013, 15, 1567–1592. [Google Scholar] [CrossRef]
- Rappaport, T.S. Wireless Communications: Principles and Practice; Dorling Kindersley: Upper Saddle River, NJ, USA, 2009. [Google Scholar]
- Chiueh, T.D.; Tsai, P.Y. OFDM Baseband Receiver Design for Wireless Communications; Wiley Publishing: Singapore, 2007. [Google Scholar]
- Maziar, N.; Yue, W.; Milos, T.; Shangbin, W.; Yinan, Q.; Mohammed, A. Overview of 5G Modulation and Waveforms Candidates. J. Commun. Inf. Netw. 2016, 1, 44–60. [Google Scholar] [CrossRef]
- Study on Scenarios and Requirements for Next Generation Access Technologies; 3rd Generation Partnership Project (3GPP), Tech. Rep. 38 913; ETSI: Sophia Antipolis, France, 2017.
- Poornima, T.; Dhinesh, K.; Sudhakar, R. Waveform candidates for 5G mobile communications. In Proceedings of the 2017 2nd IEEE International Conference on Recent Trends in Electronics, Information & Communication Technology (RTEICT), Bangalore, India, 19–20 May 2017; pp. 856–860. [Google Scholar]
- Nee, R.V.; Prasad, R. OFDM for Wireless Multimedia Communications, 1st ed.; Artech House, Inc.: Norwood, MA, USA, 2000. [Google Scholar]
- Hazareena, A.; Mustafa, B.A. A Survey: On the Waveforms for 5G. In Proceedings of the 2018 Second International Conference on Electronics, Communication and Aerospace Technology (ICECA), Coimbatore, India, 29–31 March 2018; pp. 64–67. [Google Scholar]
- Banelli, P.; Buzzi, S.; Colavolpe, G.; Modenini, A.; Rusek, F.; Ugolini, A. Modulation Formats and Waveforms for 5G Networks: Who Will Be the Heir of OFDM?: An overview of alternative modulation schemes for improved spectral efficiency. IEEE Signal Process. Mag. 2014, 31, 80–93. [Google Scholar] [CrossRef]
- Doré, J.; Gerzaguet, R.; Cassiau, N.; Ktenas, D. Waveform contenders for 5G: Description, analysis and comparison. Phys. Commun. 2017, 24, 46–61. [Google Scholar] [CrossRef]
- Schaich, F.; Wild, T. Waveform contenders for 5G—OFDM vs. FBMC vs. UFMC. In Proceedings of the 2014 6th International Symposium on Communications, Control and Signal Processing (ISCCSP), Athens, Greece, 21–23 May 2014; pp. 457–460. [Google Scholar]
- Jebbar, H.; Hassani, S.E.; Abbassi, A.E. Performance study of 5G multicarrier waveforms. In Proceedings of the 2017 International Conference on Wireless Networks and Mobile Communications (WINCOM), Rabat, Morocco, 1–4 November 2017; pp. 1–6. [Google Scholar]
- Liu, Y.; Chen, X.; Zhong, Z.; Ai, B.; Miao, D.; Zhao, Z.; Sun, J.; Teng, Y.; Guan, H. Waveform Design for 5G Networks: Analysis and Comparison. IEEE Access 2017, 5, 19282–19292. [Google Scholar] [CrossRef]
- Tiwari, S.; Chatterjee, S.; Das, S.S. Comparative analysis of waveforms for fifth generation mobile networks. In Proceedings of the 2016 IEEE International Conference on Advanced Networks and Telecommunications Systems (ANTS), Bangalore, India, 6–9 November 2016; pp. 1–6. [Google Scholar]
- Kaur, R.; Kaur, N.; Kansal, L. Comprehensive study of future waveforms for 5G. Int. J. Pure Appl. Math. 2018, 118, 4663–4672. [Google Scholar]
- Khan, B.; Velez, F.J. Multicarrier Waveform Candidates for Beyond 5G. In Proceedings of the 2020 12th International Symposium on Communication Systems, Networks and Digital Signal Processing (CSNDSP), Porto, Portugal, 20–22 July 2020; pp. 1–6. [Google Scholar]
- Fettweis, G.; Krondorf, M.; Bittner, S. GFDM—Generalized Frequency Division Multiplexing. In Proceedings of the VTC Spring 2009—IEEE 69th Vehicular Technology Conference, Barcelona, Spain, 26–29 April 2009; pp. 1–4. [Google Scholar]
- Michailow, N.; Gaspar, I.; Krone, S.; Lentmaier, M.; Fettweis, G. Generalized frequency division multiplexing: Analysis of an alternative multi-carrier technique for next generation cellular systems. In Proceedings of the 2012 International Symposium on Wireless Communication Systems (ISWCS), Paris, France, 28–31 August 2012; pp. 171–175. [Google Scholar]
- Michailow, N.; Matthé, M.; Gaspar, I.S.; Caldevilla, A.N.; Mendes, L.L.; Festag, A.; Fettweis, G. Generalized Frequency Division Multiplexing for 5th Generation Cellular Networks. IEEE Trans. Commun. 2014, 62, 3045–3061. [Google Scholar] [CrossRef]
- Abdoli, J.; Jia, M.; Ma, J. Filtered OFDM: A new waveform for future wireless systems. In Proceedings of the 2015 IEEE 16th International Workshop on Signal Processing Advances in Wireless Communications (SPAWC), Stockholm, Sweden, 28 June–1 July 2015; pp. 66–70. [Google Scholar]
- Zhang, X.; Jia, M.; Chen, L.; Ma, J.; Qiu, J. Filtered-OFDM—Enabler for Flexible Waveform in the 5th Generation Cellular Networks. In Proceedings of the 2015 IEEE Global Communications Conference (GLOBECOM), San Diego, CA, USA, 6–10 December 2015; pp. 1–6. [Google Scholar]
- Fernandes, T.; Gomes, M.; Silva, V.; Dinis, R. Time-Interleaved Block Windowed Burst OFDM. In Proceedings of the 2016 IEEE 84th Vehicular Technology Conference (VTC-Fall), Montreal, QC, Canada, 18–21 September 2016; pp. 1–5. [Google Scholar]
- Fernandes, T.; Pereira, A.; Gomes, M.; Silva, V.; Dinis, R. A new hybrid multicarrier transmission technique with iterative frequency domain detection. Phys. Commun. 2018, 27, 7–16. [Google Scholar] [CrossRef]
- Pereira, A.; Bento, P.; Gomes, M.; Dinis, R.; Silva, V. TIBWB-OFDM: A Promising Modulation Technique for MIMO 5G Transmissions. In Proceedings of the 2018 IEEE 88th Vehicular Technology Conference (VTC-Fall), Chicago, IL, USA, 27–30 August 2018; pp. 1–5. [Google Scholar]
- Conceição, F.; Gomes, M.; Silva, V.; Dinis, R. Highly efficient TIBWB-OFDM waveform for broadband wireless communications. In Proceedings of the 2020 IEEE 91st Vehicular Technology Conference (VTC2020-Spring), Antwerp, Belgium, 25–28 May 2020; pp. 1–5. [Google Scholar]
- Conceição, F.; Gomes, M.; Silva, V.; Dinis, R. Time Overlapping TIBWB-OFDM Symbols for Peak-To-Average Power Ratio Reduction. In Proceedings of the 11th Conference on Telecommunications (ConfTele 2019), Lisbon, Portugal, 26–28 June 2019. [Google Scholar]
- Chen, H.; Hua, J.; Li, F.; Chen, F.; Wang, D. Interference Analysis in the Asynchronous f-OFDM Systems. IEEE Trans. Commun. 2019, 67, 3580–3596. [Google Scholar] [CrossRef]
- Huawei, HiSilicon. f-OFDM Scheme and Filter Design. In Proceedings of the 3GPP TSG RAN WG1 Meeting 85, R1-165425, Nanjing, China, 23–27 May 2016. [Google Scholar]
- Alves, B.; Mendes, L.; Guimarães, D.; Gaspar, I. Performance of GFDM over Frequency-Selective Channels—Invited Paper. Telecomunicações 2013, 15, 1–9. [Google Scholar]
- Gaspar, I.; Michailow, N.; Navarro, A.; Ohlmer, E.; Krone, S.; Fettweis, G. Low Complexity GFDM Receiver Based on Sparse Frequency Domain Processing. In Proceedings of the 2013 IEEE 77th Vehicular Technology Conference (VTC Spring), Dresden, Germany, 2–5 June 2013; pp. 1–6. [Google Scholar]
- Barba-Maza, L.M.; Dolecek, G.J. PAPR reduction of GFDM system using Xia pulse and OPTS scheme. In Proceedings of the 2020 IEEE 63rd International Midwest Symposium on Circuits and Systems (MWSCAS), Springfield, MA, USA, 9–12 August 2020; pp. 774–777. [Google Scholar]
- Michailow, N.; Fettweis, G. Low peak-to-average power ratio for next generation cellular systems with generalized frequency division multiplexing. In Proceedings of the 2013 International Symposium on Intelligent Signal Processing and Communication Systems, Naha, Japan, 11–12 November 2013; pp. 651–655. [Google Scholar]
- Kassam, J.; Miri, M.; Magueta, R.; Castanheira, D.; Pedrosa, P.; Silva, A.; Dinis, R.; Gameiro, A. Two-Step Multiuser Equalization for Hybrid mmWave Massive MIMO GFDM Systems. Electronics 2020, 9, 1220. [Google Scholar] [CrossRef]
- Dias, W.D.; Mendes, L.L.; Rodrigues, J.J.P.C. Low Complexity GFDM Receiver for Frequency-Selective Channels. IEEE Commun. Lett. 2019, 23, 1166–1169. [Google Scholar] [CrossRef]
- Lim, B.; Ko, Y. Multiuser Interference Cancellation for GFDM With Timing and Frequency Offsets. IEEE Trans. Commun. 2019, 67, 4337–4349. [Google Scholar] [CrossRef]
- Mohammadian, A.; Tellambura, C.; Valkama, M. Analysis of Self-Interference Cancellation Under Phase Noise, CFO, and IQ Imbalance in GFDM Full-Duplex Transceivers. IEEE Trans. Veh. Technol. 2020, 69, 700–713. [Google Scholar] [CrossRef]
- Datta, R.; Michailow, N.; Lentmaier, M.; Fettweis, G. GFDM Interference Cancellation for Flexible Cognitive Radio PHY Design. In Proceedings of the 2012 IEEE Vehicular Technology Conference (VTC Fall), Quebec City, QC, Canada, 3–6 September 2012; pp. 1–5. [Google Scholar]
- Nunes, J.; Bento, P.; Gomes, M.; Dinis, R.; Silva, V. Block-Windowed Burst OFDM: A High-Efficiency Multicarrier Technique. Electron. Lett. 2014, 50, 1757–1759. [Google Scholar] [CrossRef]
- Pereira, A.; Bento, P.; Gomes, M.; Dinis, R.; Silva, V. MIMO Time Interleaved Block Windowed Burst OFDM with Iterative Frequency Domain Equalization. In Proceedings of the 2018 15th International Symposium on Wireless Communication Systems (ISWCS), Lisbon, Portugal, 28–31 August 2018; pp. 1–6. [Google Scholar]
- Conceição, F.; Gomes, M.; Silva, V.; Dinis, R. An OFDM-Based Waveform with High Spectral Efficiency. IEEE Commun. Lett. 2020, 24, 2614–2618. [Google Scholar] [CrossRef]
- Souto, N.; Dinis, R.; Correia, A.; Reis, C. Interference-Aware Iterative Block Decision Feedback Equalizer for Single-Carrier Transmission. IEEE Trans. Veh. Technol. 2015, 64, 3316–3321. [Google Scholar] [CrossRef][Green Version]
- Dinis, R.; Montezuma, P.; Souto, N.; Silva, J. Iterative Frequency-Domain Equalization for general constellations. In Proceedings of the 2010 IEEE Sarnoff Symposium, Princeton, NJ, USA, 12–14 April 2010; pp. 1–5. [Google Scholar]
- Ijaz, A.; Zhang, L.; Xiao, P.; Tafazolli, R. Analysis of Candidate Waveforms for 5G Cellular Systems; InTech: Rijeka, Croatia, 2016. [Google Scholar]
- Michailow, N. Integration of a GFDM Secondary System in an Existing OFDM System. Ph.D. Thesis, Technische Universitat Dresden, Dresden, Germany, 2010. [Google Scholar]


| Waveform | Bandwidth |
|---|---|
| OFDM | |
| F-OFDM | |
| GFDM | |
| TIBWB-OFDM | |
| TIBWB-OFDM with WTO |
| Waveform | Complex Multiplications |
|---|---|
| OFDM | |
| F-OFDM | |
| GFDM | |
| TIBWB-OFDM with WTO | |
| A | |
| C | |
| B and D |
| KPI | OFDM | F-OFDM | GFDM | TIBWB-OFDM | TIBWB-OFDM with WTO |
|---|---|---|---|---|---|
| PAPR | High | High | High | Very High | High |
| Spectral efficiency | Low | Low | Very High | High | Very High |
| OOB emissions | High | Very Low | Moderate/Low | Very low | Low |
| Reliability (BER performance) | Moderate | Moderate | Low | Low/Moderate | Moderate |
| Computation complexity | Low | Moderate | High | Moderate/High | High/Very High |
| Processing delay | Low | Low | High | High | High |
| Robustness to frequency selectivity | High | High | High | Very High | Very High |
| Robustness to time selectivity | Low | Low | High | High | High |
| Efficient MIMO implementation | High | High | Moderate | High | High |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Conceição, F.; Gomes, M.; Silva, V.; Dinis, R.; Silva, A.; Castanheira, D. A Survey of Candidate Waveforms for beyond 5G Systems. Electronics 2021, 10, 21. https://doi.org/10.3390/electronics10010021
Conceição F, Gomes M, Silva V, Dinis R, Silva A, Castanheira D. A Survey of Candidate Waveforms for beyond 5G Systems. Electronics. 2021; 10(1):21. https://doi.org/10.3390/electronics10010021
Chicago/Turabian StyleConceição, Filipe, Marco Gomes, Vitor Silva, Rui Dinis, Adão Silva, and Daniel Castanheira. 2021. "A Survey of Candidate Waveforms for beyond 5G Systems" Electronics 10, no. 1: 21. https://doi.org/10.3390/electronics10010021
APA StyleConceição, F., Gomes, M., Silva, V., Dinis, R., Silva, A., & Castanheira, D. (2021). A Survey of Candidate Waveforms for beyond 5G Systems. Electronics, 10(1), 21. https://doi.org/10.3390/electronics10010021









