Age-at-Death Estimation of Fetuses and Infants in Forensic Anthropology: A New “Coupling” Method to Detect Biases Due to Altered Growth Trajectories
Abstract
:Simple Summary
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample
2.1.1. Normal and Pathological Development
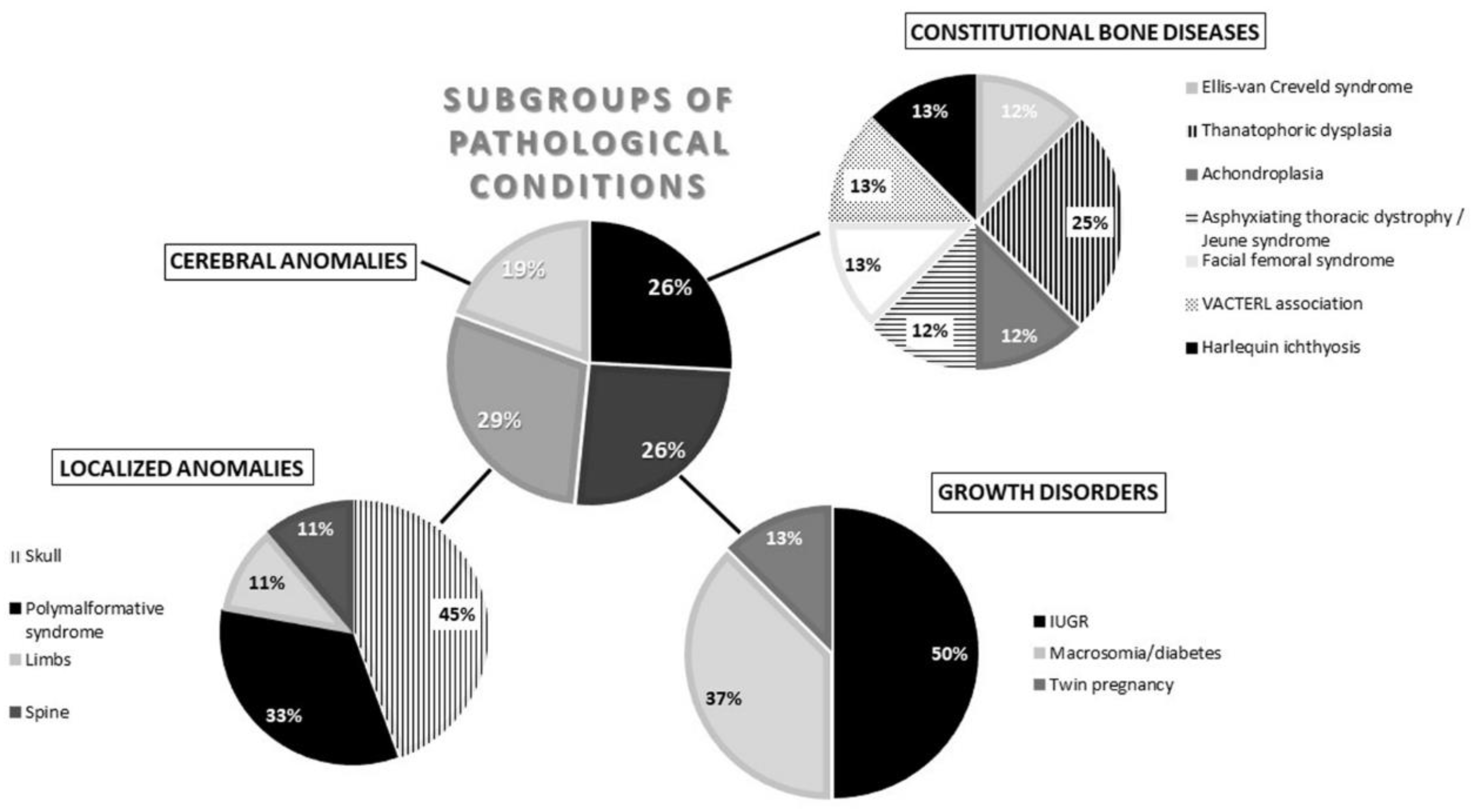
2.1.2. Pathologies Groups
- -
- Constitutional bone diseases or CBD (Ellis–van Creveld syndrome, thanatophoric dysplasia, achondroplasia, Jeune syndrome, facial femoral syndrome, VACTERL association, and harlequin ichthyosis = 14%);
- -
- Growth disorders or conditions justifying differentiated growth or GD (intrauterine growth retardation, macrosomia/diabetes, and twin pregnancy = 39%);
- -
- Localized anomalies or LA (skull, polymalformative syndrome, limbs, and spine = 23%);
- -
- Cerebral anomalies or CA (21%);
- -
- Chromosomal anomalies or CHRA (trisomy 21 and trisomy 18 = 3%).
2.2. Data Acquisition
2.3. Bone Reconstruction
2.4. Maturation Criterion: Elliptic Fourier Analysis of the Pars Basilaris
2.4.1. Outline Process
2.4.2. Measurement Error
2.4.3. Harmonics Number
2.5. Coupling between Maturation and Growth Process
2.5.1. Maturation Criterion: Shape Stages
2.5.2. Growth Criterion: Femoral Lengths
2.6. Statistical and Morphometric Analyses
2.6.1. Bilateral Femoral Asymmetry and Sex Effect on the Variables
2.6.2. Application of the Coupling Method in Samples B and C
3. Results
3.1. Quantification of Pars Basilaris Shapes
3.1.1. Number of Harmonics
3.1.2. Measurement Error
3.2. Between-Sex Differences and Femoral Length
3.3. Coupling between Maturation and Growth
Method Application
4. Discussion
4.1. The Fetus and Infant Sample
4.2. Quantification of Shape
4.3. Interest in the Pars Basilaris
4.4. Morphology of the Pars Basilaris
4.5. Maturation and Growth Criterion
4.6. The Two Main Advantages of This Coupling Method
4.7. Pathological Uncoupling
- -
- (1–2) Two microcephaly cases (one was associated with craniosynostosis);
- -
- (3) One ossification defect of the vault with the enlargement of the fontanelles and the presence of Wormian bones in the parietal and occipital region;
- -
- (4) One severe hydrocephalus;
- -
- Anomaly of the femurs with shortening and curving;
- -
- A spina bifida;
- -
- One case with arthrogryposis, club feet, clenched hands, 11 pairs of slender ribs and platyspondyly;
- -
- One case with abnormalities of the spine and ribs, as well as retrognathism;
- -
- One case with anomalies of the spine, a short thorax, and a malposition of the four limbs (clenched hands, knees in extension, and club feet).
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CA | Cerebral Anomalies |
| CBD | Constitutional Bone Diseases |
| CHRA | Chromosomal Anomalies |
| CT scan | Computerized Tomography Scan |
| DICOM | Digital Imaging and Communications in Medicine |
| EFA | Elliptical Fourier Analysis |
| GD | Growth Disorders |
| GPA | Generalized Procrustes Analysis |
| IUGR | Intrauterine Growth Retardation |
| LA | Localized Anomalies |
| MANOVA | Multivariate Analysis Of Variance |
| MRI | Magnetic Resonance Imaging |
| VACTERL | Vertebral, Anal, Cardiac, Tracheal, Esophageal, Renal, and Limb |
| WHO | World Health Organization |
References
- Ritz-Timme, S.; Cattaneo, C.; Collins, M.J.; Waite, E.R.; Schutz, H.W.; Kaatsch, H.J.; Borrman, H.I.M. Age Estimation: The State of the Art in Relation to the Specific Demands of Forensic Practise. Int. J. Legal Med. 2000, 113, 129–136. [Google Scholar] [CrossRef]
- Adalian, P. Evaluation Multiparamétrique de la Croissance Foetale: Application à la Détermination de L’âge et du Sexe; Université de la Méditerranée-Aix-Marseille 2: Marseille, France, 2001; Available online: http://www.theses.fr/2001AIX20692 (accessed on 5 April 2018).
- Adalian, P.; Piercecchi-Marti, M.D.; Bourliere-Najean, B.; Panuel, M.; Leonetti, G.; Dutour, O. New foetal age determination formula. Comptes Rendus Biol. 2002, 325, 261–269. [Google Scholar] [CrossRef]
- AlQahtani, S.J.; Hector, M.P.; Liversidge, H.M. Brief Communication: The London Atlas of Human Tooth Development and Eruption. Am. J. Phys. Anthropol. 2010, 142, 481–490. [Google Scholar] [CrossRef]
- Balthazard, V.; Dervieux, X. Etudes Anthropométriques Sur Le Foetus Humain. Ann. Med. Leg. 1921, 1, 37–42. [Google Scholar]
- Cardoso, H.F.V.; Gomes, J.; Campanacho, V.; Marinho, L. Age Estimation of Immature Human Skeletal Remains Using the Post-Natal Development of the Occipital Bone. Int. J. Legal Med. 2013, 127, 997–1004. [Google Scholar] [CrossRef]
- Cardoso, H.F.V.; Abrantes, J.; Humphrey, L.T. Age Estimation of Immature Human Skeletal Remains from the Diaphyseal Length of the Long Bones in the Postnatal Period. Int. J. Legal Med. 2014, 128, 809–824. [Google Scholar] [CrossRef]
- Carneiro, C.; Curate, F.; Borralho, P.; Cunha, E. Radiographic Fetal Osteometry: Approach on Age Estimation for the Portuguese Population. Forensic Sci. Int. 2013, 231, 397.e1. [Google Scholar] [CrossRef] [Green Version]
- Carneiro, C.; Curate, F.; Cunha, E. A Method for Estimating Gestational Age of Fetal Remains Based on Long Bone Lengths. Int. J. Legal Med. 2016, 130, 1333–1341. [Google Scholar] [CrossRef] [Green Version]
- Chavez-Martinez, P.; Ortega-Palma, A.; Castrejón-Caballero, J.L.; Arteaga-Martínez, M. Equations to Estimate Fetal Age at the Moment of Death in the Mexican Population. Forensic Sci. Int. 2016, 266, 587.e1–587.e10. [Google Scholar] [CrossRef]
- Fazekas, I.G.; Kósa, F. Forensic Fetal Osteology; Akadémiai Kiadó: Budapest, Hongrie, 1978; ISBN 978-963-05-1491-0. [Google Scholar]
- Hadlock, F.; Harrist, R.; Deter, R.; Park, S. Fetal Femur Length as a Predictor of Menstrual Age: Sonographically Measured. Am. J. Roentgenol. 1982, 138, 875–878. [Google Scholar] [CrossRef] [Green Version]
- Kurmanavicius, J.; Wright, E.M.; Royston, P.; Zimmermann, R.; Huch, R.; Huch, A.; Wisser, J. Fetal Ultrasound Biometry: 2. Abdomen and Femur Length Reference Values. BJOG Int. J. Obstet. Gynaecol. 1999, 106, 136–143. [Google Scholar] [CrossRef]
- Maresh, M.M. Linear Growth of Long Bones of Extremities from Infancy through Adolescence: Continuing Studies. AMA Am. J. Dis. Child. 1955, 89, 725–742. [Google Scholar] [CrossRef]
- Olivares, J.I.; Aguilera, I.A. Proposal of New Regression Formulae for the Estimation of Age in Infant Skeletal Remains from the Metric Study of the Pars Basilaris. Int. J. Legal Med. 2017, 131, 781–788. [Google Scholar] [CrossRef]
- Olivier, G.; Pineau, H. Nouvelle Détermination de La Taille Foetale d’après Les Longueurs Diaphysaires Des Os Longs. Ann. Med. Leg. 1960, 40, 141–144. [Google Scholar]
- Scheuer, L.; Musgrave, J.; Evans, S. The Estimation of Late Fetal and Perinatal Age from Limb Bone Length by Linear and Logarithmic Regression. Ann. Hum. Biol. 1980, 7, 257–265. [Google Scholar] [CrossRef]
- Scheuer, L.; MacLaughlin-Black, S. Age Estimation from the Pars Basilaris of the Fetal and Juvenile Occipital Bone. Int. J. Osteoarchaeol. 1994, 4, 377–380. [Google Scholar] [CrossRef]
- Simon, D.; Bhowmick, B.; Simon, M.; Varghese, D.; Varghese, A. Fetal Growth and Age Estimation Based on Osteometry on the Diaphysis of Human Fetal Fibula. Int. J. Innov. Res. Dev. 2020, 9, 49–57. [Google Scholar] [CrossRef]
- Simon, D.; Varghese, A.; Bhowmick, B.; Simon, M.; Varghese, D. Human Fetal Growth and Age Estimation Based on Osteometry on the Diaphysis of Fetal Tibia. Int. J. Innov. Res. Dev. 2020, 9, 40–48. [Google Scholar] [CrossRef]
- Feess-Higgins, A.; Larroche, J.-C. Development of the Human Fetal Brain: Anatomical Atlas; Editions Inserm: Paris, France, 1987; ISBN 978-2-85598-337-0. [Google Scholar]
- Piercecchi-Marti, M.D.; Adalian, P.; Pelissier-Alicot, A.-L.; Cianfarani, F.; Leonetti, G. Estimation de l’âge Foetal à Partir de l’examen Macroscopique et Histologique Des Viscères. J. Médecine Légale Droit Médical 2000, 43, 545–552. Available online: http://cat.inist.fr/?aModele=afficheN&cpsidt=972452 (accessed on 18 May 2015).
- Scheuer, L.; Black, S.; Cunningham, C. Developmental Juvenile Osteology; Academic Press: Cambridge, MA, USA, 2000; ISBN 978-0-08-053038-3. [Google Scholar]
- Captier, G.; Boë, L.-J.; Badin, P.; Guihard-Costa, A.-M.; Canovas, F.; Larroche, J.-C. Modèles Géométriques de Croissance Du Cerveau, Cervelet, Tronc Cérébral et Modification Des Angles de La Base Du Crâne Au Cours de La Période Fœtale. Morphologie 2013, 97, 38–47. [Google Scholar] [CrossRef]
- Ford, E.H. The Growth of the Foetal Skull. J. Anat. 1956, 90, 63–72. [Google Scholar] [PubMed]
- Grossman, J.W.; Zuckerman, S. An X-ray Study of Growth Changes in the Base of the Skull. Am. J. Phys. Anthropol. 1955, 13, 515–519. [Google Scholar] [CrossRef] [PubMed]
- Jeffery, N.; Spoor, F. Brain Size and the Human Cranial Base: A Prenatal Perspective. Am. J. Phys. Anthropol. 2002, 118, 324–340. [Google Scholar] [CrossRef] [PubMed]
- Lieberman, D.E.; Pearson, O.M.; Mowbray, K.M. Basicranial Influence on Overall Cranial Shape. J. Hum. Evol. 2000, 38, 291–315. [Google Scholar] [CrossRef] [Green Version]
- Mandarim de lacerda, C.; Alves, M. Growth of the Cranial Bones in Human Fetuses (2nd and 3rd Trimesters). Surg. Radiol. Anat. 1992, 14, 125–129. [Google Scholar] [CrossRef]
- Morimoto, N.; Ogihara, N.; Katayama, K.; Shiota, K. Three-Dimensional Ontogenetic Shape Changes in the Human Cranium during the Fetal Period. J. Anat. 2008, 212, 627–635. [Google Scholar] [CrossRef]
- Trenouth, M.J. Shape Changes during Human Fetal Craniofacial Growth. J. Anat. 1984, 139, 639–651. [Google Scholar]
- Sherwood, R.; Meindl, R.S.; Robinson, H.B.; May, R.L. Fetal Age: Methods of Estimation and Effects of Pathology. Am. J. Phys. Anthropol. 2000, 113, 305–315. [Google Scholar] [CrossRef]
- Delteil, C.; Lesieur, E.; Tuchtan, L.; Álvarez, A.C.; Chaumoitre, K.; Saliba, B.; Adalian, P.; Piercecchi-Marti, M. Study of the Growth and Shape of the Brain and Cranial Base during the First Two Years of Life. Morphol. Bull. Assoc. Anat. 2020, 105, 45–53. [Google Scholar] [CrossRef]
- Delteil, C.; Mopin, C.; Tuchtan, L.; Alvarez, A.; Piercecchi, M.-D.; Adalian, P. Ontogeny of Cranial Base during the First Two Years of Life. Morphologie 2021, in press. [Google Scholar] [CrossRef] [PubMed]
- Sastre, J.; Tardivo, D.; Khemira, A.; Chaumoitre, K.; Panuel, M.; Piercecchi-Marti, M.D.; Leonetti, G.; Adalian, P. 3D CT Scan Study of Fetal Cranial Base: Interests of Occipital Bone Measurements in Age Estimation. Odonto-Stomatol. Trop. Trop. Dent. J. 2011, 34, 17–25. [Google Scholar]
- Sastre, J. Etude Tridimensionnelle de la Partie Postérieure de la Base du Crâne chez le Fœtus: Applications à la Détermination de L’âge en Anthropologie Médico-Légale; Université de la Méditerranée-Aix Marseille 2: Marseille, France, 2011. [Google Scholar]
- Lewis, M.E. The Bioarchaeology of Children: Perspectives from Biological and Forensic Anthropology; Cambridge University Press: New York, NY, USA, 2006; ISBN 978-1-139-45968-6. [Google Scholar]
- Coleman, M.N.; Colbert, M.W. Technical Note: CT Thresholding Protocols for Taking Measurements on Three-Dimensional Models. Am. J. Phys. Anthropol. 2007, 133, 723–725. [Google Scholar] [CrossRef] [PubMed]
- Spoor, F.; Zonneveld, F.W.; Macho, G.A. Linear Measurements of Cortical Bone and Dental Enamel by Computed Tomography: Applications and Problems. Am. J. Phys. Anthropol. 1993, 91, 469–484. [Google Scholar] [CrossRef] [PubMed]
- Adalian, P.; Piercecchi-Marti, M.D.; Bourliere-Najean, B.; Panuel, M.; Fredouille, C.; Dutour, O.; Leonetti, G. Postmortem Assessment of Fetal Diaphyseal Femoral Length: Validation of a Radiographic Methodology. J. Forensic Sci. 2001, 46, 215–219. [Google Scholar] [CrossRef]
- Bareggi, R.; Grill, V.; Zweyer, M.; Sandrucci, M.A.; Martelli, A.M.; Narducci, P.; Forabosco, A. On the Assessment of the Growth Patterns in Human Fetal Limbs: Longitudinal Measurements and Allometric Analysis. Early Hum. Dev. 1996, 45, 11–25. [Google Scholar] [CrossRef]
- Haj Salem, N.; Dedouit, F.; Maret, D.; Adalian, P.; Rougé, D.; Piercecchi, M.-D.; Assaoui, A.; Chadly, A.; Norbert, T. Estimation de l’âge foetal par étude scanographique des longueurs fémorales et tibiales: Comparaison entre les reconstructions en deux et en trois dimensions. Rev. Médecine Légale 2010, 1, 71–76. [Google Scholar] [CrossRef]
- Niel, M.; Chaumoître, K.; Corny, J.; Lalys, L.; Adalian, P. Maturation of the Human Foetal Basioccipital: Quantifying Shape Changes in Second and Third Trimesters Using Elliptic Fourier Analysis. J. Anat. 2019, 235, 34–44. [Google Scholar] [CrossRef]
- Bookstein, F.L. Morphometric Tools for Landmark Data: Geometry and Biology; Cambridge University Press: New York, NY, USA, 1991. [Google Scholar]
- Rohlf, F.J. TpsDig2: Digitize Landmarks and Outlines; Department of Ecology, Evolution and Anthropology, State University of New York at Stony Brook: New York, NY, USA, 2013; Available online: http://life.bio.sunysb.edu/morph/soft-dataacq.html (accessed on 13 April 2021).
- Friess, M.; Baylac, M. Exploring Artificial Cranial Deformation Using Elliptic Fourier Analysis of Procrustes Aligned Outlines. Am. J. Phys. Anthropol. 2003, 122, 11–22. [Google Scholar] [CrossRef]
- Gower, J.C. Generalized Procrustes Analysis. Psychometrika 1975, 40, 33–51. [Google Scholar] [CrossRef]
- Rohlf, F.J. Statistical Power Comparisons among Alternative Morphometric Methods. Am. J. Phys. Anthropol. 2000, 111, 463–478. [Google Scholar] [CrossRef]
- Rohlf, F.J.; Slice, D. Extensions of the Procrustes Method for the Optimal Superimposition of Landmarks. Syst. Biol. 1990, 39, 40–59. [Google Scholar] [CrossRef] [Green Version]
- Shannon, C.E. Communication in the Presence of Noise. Proc. IRE 1949, 37, 10–21. [Google Scholar] [CrossRef]
- Claude, J. Log-Shape Ratios, Procrustes Superimposition, Elliptic Fourier Analysis: Three Worked Examples in R. Hystrix-Ital. J. Mammal. 2013, 24, 94–102. [Google Scholar] [CrossRef]
- Claude, J.; Paradis, E.; Tong, H.; Auffray, J.C. A Geometric Morphometric Assessment of the Effects of Environment and Cladogenesis on the Evolution of the Turtle Shell. Biol. J. Linn. Soc. 2003, 79, 485–501. [Google Scholar] [CrossRef] [Green Version]
- Debat, V.; Cornette, R.; Korol, A.B.; Nevo, E.; Soulet, D.; David, J.R. Multidimensional Analysis of Drosophila Wing Variation in Evolution Canyon. J. Genet. 2008, 87, 407–419. [Google Scholar] [CrossRef]
- Goodall, C. Procrustes Methods in the Statistical Analysis of Shape. J. R. Stat. Soc. Ser. B Methodol. 1991, 53, 285–339. [Google Scholar] [CrossRef]
- Klingenberg, C.P.; McIntyre, G.S. Geometric Morphometrics of Developmental Instability: Analyzing Patterns of Fluctuating Asymmetry with Procrustes Methods. Evolution 1998, 52, 1363–1375. [Google Scholar] [CrossRef]
- Claude, J. Morphometrics with R; Springer Science & Business Media: New York, NY, USA, 2008; ISBN 978-0-387-77790-0. [Google Scholar]
- Monti, L.; Baylac, M.; Lalanne-Cassou, B. Elliptic Fourier Analysis of the Form of Genitalia in Two Spodoptera Species and Their Hybrids (Lepidoptera: Noctuidae). Biol. J. Linn. Soc. 2001, 72, 391–400. [Google Scholar] [CrossRef]
- Rohlf, F.J.; Archie, J.W. A Comparison of Fourier Methods for the Description of Wing Shape in Mosquitoes (Diptera: Culicidae). Syst. Biol. 1984, 33, 302–317. [Google Scholar] [CrossRef]
- Anderson, M. A New Method for Non-Parametric Multivariate Analysis of Variance. Austral Ecol. 2001, 26, 32–46. [Google Scholar] [CrossRef]
- Mitteroecker, P.; Gunz, P. Advances in Geometric Morphometrics. Evol. Biol. 2009, 36, 235–247. [Google Scholar] [CrossRef] [Green Version]
- Zelditch, M.L.; Swiderski, D.L.; Sheets, H.D. Geometric Morphometrics for Biologists, Second Edition: A Primer, 2nd ed.; Academic Press: Amsterdam, The Netherlands, 2012; ISBN 978-0-12-386903-6. [Google Scholar]
- Bonhomme, V.; Claude, J. Momocs: Morphometrics Using R; 2017. Available online: https://CRAN.R-project.org/package=Momocs (accessed on 1 March 2018).
- Schlager, S.; Jefferis, G. Morpho: Calculations and Visualisations Related to Geometric Morphometrics; 2017; Available online: https://CRAN.R-project.org/package=Morpho (accessed on 1 March 2018).
- Adams, D.; Collyer, M.; Kaliontzopoulou, A.; Sherratt, E. Geomorph: Geometric Morphometric Analyses of 2D/3D Landmark Data; 2017; Available online: https://cran.r-project.org/package=geomorph (accessed on 1 March 2018).
- Fox, J.; Weisberg, S.; Price, B.; Adler, D.; Bates, D.; Baud-Bovy, G.; Bolker, B.; Ellison, S.; Firth, D.; Friendly, M.; et al. Car: Companion to Applied Regression; 2018; Available online: https://CRAN.R-project.org/package=car (accessed on 10 April 2018).
- Zhao, J.H.; Hornik, K.; Ripley, B. Gap: Genetic Analysis Package; 2018; Available online: https://CRAN.R-project.org/package=gap (accessed on 10 April 2018).
- Cordoliani, Y.-S.; Foehrenbach, H.; Dion, A.-M.; Lahutte, M. Risques liés à l’exposition d’une grossesse aux rayonnements ionisants. J. Radiol. 2005, 86, 601–606. [Google Scholar] [CrossRef]
- Chen, S.Y.; Lestrel, P.E.; Kerr, W.J.; McColl, J.H. Describing Shape Changes in the Human Mandible Using Elliptical Fourier Functions. Eur. J. Orthod. 2000, 22, 205–216. [Google Scholar] [CrossRef] [PubMed]
- Corina Vera, M.; Laura Ponssa, M.; Abdala, V. Further Data on Sesamoid Identity from Two Anuran Species. Anat. Rec.-Adv. Integr. Anat. Evol. Biol. 2015, 298, 1376–1394. [Google Scholar] [CrossRef] [PubMed]
- Corny, J.; Detroit, F. Technical Note: Anatomic Identification of Isolated Modern Human Molars: Testing Procrustes Aligned Outlines as a Standardization Procedure for Elliptic Fourier Analysis. Am. J. Phys. Anthropol. 2014, 153, 314–322. [Google Scholar] [CrossRef] [PubMed]
- Daegling, D.J.; Jungers, W.L. Elliptical Fourier Analysis of Symphyseal Shape in Great Ape Mandibles. J. Hum. Evol. 2000, 39, 107–122. [Google Scholar] [CrossRef]
- Ferson, S.; Rohlf, F.J.; Koehn, R.K. Measuring Shape Variation of Two-Dimensional Outlines. Syst. Biol. 1985, 34, 59–68. [Google Scholar] [CrossRef]
- Lestrel, P.E. Fourier Descriptors and Their Applications in Biology; Cambridge University Press: New York, NY, USA, 2008; ISBN 978-0-521-05573-4. [Google Scholar]
- Lestrel, P.E.; Bodt, A.; Swindler, D.R. Longitudinal Study of Cranial Base Shape Changes in Macaca Nemestrina. Am. J. Phys. Anthropol. 1993, 91, 117–129. [Google Scholar] [CrossRef]
- Mokrane, F.-Z.; Dedouit, F.; Gellee, S.; Sans, N.; Rousseau, H.; Rouge, D.; Telmon, N. Sexual Dimorphism of the Fetal Ilium: A 3D Geometric Morphometric Approach with Multislice Computed Tomography. J. Forensic Sci. 2013, 58, 851–858. [Google Scholar] [CrossRef]
- Caple, J.; Byrd, J.; Stephan, C.N. Elliptical Fourier Analysis: Fundamentals, Applications, and Value for Forensic Anthropology. Int. J. Legal Med. 2017, 131, 1675–1690. [Google Scholar] [CrossRef]
- Kuhl, F.P.; Giardina, C.R. Elliptic Fourier Features of a Closed Contour. Comput. Graph. Image Process. 1982, 18, 236–258. [Google Scholar] [CrossRef]
- Jeffery, N.; Spoor, F. Ossification and Midline Shape Changes of the Human Fetal Cranial Base. Am. J. Phys. Anthropol. 2004, 123, 78–90. [Google Scholar] [CrossRef]
- Macklin, C.C. Preliminary Note on the Skull of a Human Fetus of 43 MM. Greatest Length. Anat. Rec. 1921, 22, 251–265. [Google Scholar] [CrossRef] [Green Version]
- Nemzek, W.R.; Brodie, H.A.; Hecht, S.T.; Chong, B.W.; Babcook, C.J.; Seibert, J.A. MR, CT, and Plain Film Imaging of the Developing Skull Base in Fetal Specimens. Am. J. Neuroradiol. 2000, 21, 1699–1706. [Google Scholar] [PubMed]
- Noback, C.R. The Developmental Anatomy of the Human Osseous Skeleton during the Embryonic, Fetal and Circumnatal Periods. Anat. Rec. 1944, 88, 91–125. [Google Scholar] [CrossRef]
- Noback, C.R.; Robertson, G.G. Sequences of Appearance of Ossification Centers in the Human Skeleton during the First Five Prenatal Months. Am. J. Anat. 1951, 89, 1–28. [Google Scholar] [CrossRef]
- Redfield, A. A New Aid to Aging Immature Skeletons: Development of the Occipital Bone. Am. J. Phys. Anthropol. 1970, 33, 207–220. [Google Scholar] [CrossRef] [PubMed]
- Schaefer, M.; Black, S.; Scheuer, L. Juvenile Osteology: A Laboratory and Field Manual; Elsevier: Amsterdam, The Netherlands; Academic Press: Burlington, MA, USA, 2009; ISBN 978-0-12-374635-1. [Google Scholar]
- Scheuer, L.; Black, S. The Juvenile Skeleton; Elsevier Academic Press: London, UK, 2004; ISBN 978-0-08-047459-5. [Google Scholar]
- Slice, D.E. Geometric Morphometrics. Annu. Rev. Anthropol. 2007, 36, 261–281. [Google Scholar] [CrossRef]
- Needham, A.E. The Form-Transformation of the Abdomen of the Female Peacrab, Pinnotheres Pisum Leach. Proc. R. Soc. Lond. B Biol. Sci. 1950, 137, 115–136. [Google Scholar]
- Herlin, C.; Largey, A.; deMattei, C.; Daures, J.P.; Bigorre, M.; Captier, G. Modeling of the Human Fetal Skull Base Growth: Interest in New Volumetrics Morphometric Tools. Early Hum. Dev. 2011, 87, 239–245. [Google Scholar] [CrossRef]
- Jeffery, N. A High-Resolution MRI Study of Linear Growth of the Human Fetal Skull Base. Neuroradiology 2002, 44, 358–366. [Google Scholar] [CrossRef]
- Schmeling, A.; Geserick, G.; Reisinger, W.; Olze, A. Age Estimation. Forensic Sci. Int. 2007, 165, 178–181. [Google Scholar] [CrossRef] [PubMed]
- Tocheri, M.W.; Molto, J.E. Aging Fetal and Juvenile Skeletons from Roman Period Egypt Using Basiocciput Osteometrics. Int. J. Osteoarchaeol. 2002, 12, 356–363. [Google Scholar] [CrossRef]
- Hill, L.M.; Guzick, D.; Hixson, J.; Peterson, C.S.; Rivello, D.M. Composite Assessment of Gestational Age: A Comparison of Institutionally Derived and Published Regression Equations. Am. J. Obstet. Gynecol. 1992, 166, 551–555. [Google Scholar] [CrossRef]
- Piercecchi-Marti, M.D.; Adalian, P.; Liprandi, A.; Figarella-Branger, D.; Dutour, O.; Leonetti, G. Fetal Visceral Maturation: A Useful Contribution to Gestational Age Estimation in Human Fetuses. J. Forensic Sci. 2004, 49, 912–917. [Google Scholar] [CrossRef] [PubMed]
- Kyrkanides, S.; Kjaer, I.; Fischerhansen, B. Development of the Basilar Part of the Occipital Bone in Normal Human Fetuses. J. Craniofac. Genet. Dev. Biol. 1993, 13, 184–192. [Google Scholar]
- Gaillard, R.; Rurangirwa, A.; Williams, M.A.; Hofman, A.; Mackenbach, J.P.; Franco, O.H.; Steegers, E.A.P.; Jaddoe, V.W.V. Maternal Parity, Fetal and Childhood Growth, and Cardiometabolic Risk Factors. Hypertension 2014, 64, 266–274. [Google Scholar] [CrossRef] [Green Version]
- Hinkle, S.N.; Albert, P.S.; Mendola, P.; Sjaarda, L.A.; Yeung, E.; Boghossian, N.S.; Laughon, S.K. The Association between Parity and Birthweight in a Longitudinal Consecutive Pregnancy Cohort. Paediatr. Perinat. Epidemiol. 2014, 28, 106–115. [Google Scholar] [CrossRef] [Green Version]
- Ong, K.K.L.; Preece, M.A.; Emmett, P.M.; Ahmed, M.L.; Dunger, D.B.; ALSPAC Study Team. Size at Birth and Early Childhood Growth in Relation to Maternal Smoking, Parity and Infant Breast-Feeding: Longitudinal Birth Cohort Study and Analysis. Pediatr. Res. 2002, 52, 863–867. [Google Scholar] [CrossRef]
- Prentice, A.; Cole, T.; Whitehead, R.G. Impaired Growth in Infants Born to Mothers of Very High Parity. Hum. Nutr. Clin. Nutr. 1987, 41, 319–325. [Google Scholar]
- Wilcox, M.A.; Chang, A.M.; Johnson, I.R. The Effects of Parity on Birthweight Using Successive Pregnancies. Acta Obstet. Gynecol. Scand. 1996, 75, 459–463. [Google Scholar] [CrossRef] [PubMed]
- Lejarraga, H. Growth in Infancy and Childhood: A Pediatric Approach. In Human Growth and Development; Academic Press: Cambridge, MA, USA, 2012; pp. 21–44. [Google Scholar]
- Smith, D.W.; Truog, W.; Rogers, J.E.; Greitzer, L.J.; Skinner, A.L.; McCann, J.J.; Harvey, M.A. Shifting Linear Growth during Infancy: Illustration of Genetic Factors in Growth from Fetal Life through Infancy. J. Pediatr. 1976, 89, 225–230. [Google Scholar] [CrossRef]
- Cameron, N.; Bogin, B. Human Growth and Development; Academic Press: Cambridge, MA, USA, 2012; ISBN 978-0-12-383882-7. [Google Scholar]
- Kawakita, A.; Sato, K.; Makino, H.; Ikegami, H.; Takayama, S.; Toyama, Y.; Umezawa, A. Nicotine Acts on Growth Plate Chondrocytes to Delay Skeletal Growth through the A7 Neuronal Nicotinic Acetylcholine Receptor. PLoS ONE 2008, 3. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Koren, G. Measurement of Drugs in Neonatal Hair; a Window to Fetal Exposure. Forensic Sci. Int. 1995, 70, 77–82. [Google Scholar] [CrossRef]
- Lejeune, C. Syndrome d’alcoolisation fœtale. Devenir 2001, 13, 77–94. [Google Scholar] [CrossRef]
- Merialdi, M.; Caulfield, L.E.; Zavaleta, N.; Figueroa, A.; Costigan, K.A.; Dominici, F.; Dipietro, J.A. Randomized Controlled Trial of Prenatal Zinc Supplementation and Fetal Bone Growth. Am. J. Clin. Nutr. 2004, 79, 826–830. [Google Scholar] [CrossRef] [Green Version]
- Samper, M.P.; Jiménez-Muro, A.; Nerín, I.; Marqueta, A.; Ventura, P.; Rodríguez, G. Maternal Active Smoking and Newborn Body Composition. Early Hum. Dev. 2012, 88, 141–145. [Google Scholar] [CrossRef]
- Simpson, M.E.; Duggal, S.; Keiver, K. Prenatal Ethanol Exposure Has Differential Effects on Fetal Growth and Skeletal Ossification. Bone 2005, 36, 521–532. [Google Scholar] [CrossRef]
- Sobrian, S.K.; Vaughn, V.T.; Ashe, W.K.; Markovic, B.; Djuric, V.; Jankovic, B.D. Gestational Exposure to Loud Noise Alters the Development and Postnatal Responsiveness of Humoral and Cellular Components of the Immune System in Offspring. Environ. Res. 1997, 73, 227–241. [Google Scholar] [CrossRef]
- Tabet, C.; Dupuis-Gauthier, C.; Schmidt, P.; Maerten-Lesot, B.; Porez, S.; Delion, P.; Soulé, M. Maltraitance à fœtus: Comment comprendre pour prévenir. Devenir 2009, 21, 205–244. [Google Scholar] [CrossRef]
- Fetal and Neonatal Pathology, 4th ed; Keeling, J.W.; Khong, T.Y. (Eds.) Springer: London, UK, 2007; ISBN 978-1-84628-743-5. [Google Scholar]
- Benacerraf, B.; Gelman, R.; Frigoletto, F. Sonographic Identification of 2nd-Trimester Fetuses with Downs-Syndrome. N. Engl. J. Med. 1987, 317, 1371–1376. [Google Scholar] [CrossRef] [PubMed]
- Fitzsimmons, J.; Droste, S.; Shepard, T.; Pascoemason, J.; Chinn, A.; Mack, L. Long-Bone Growth in Fetuses with down Syndrome. Am. J. Obstet. Gynecol. 1989, 161, 1174–1177. [Google Scholar] [CrossRef]
- Ortner, D.J. Identification of Pathological Conditions in Human Skeletal Remains, 2nd ed.; Academic Press: San Diego, CA, USA, 2003; 664p, ISBN 978-0-12-528628-2. [Google Scholar]
- Le Merrer, M.; Lambot, K. Maladies Osseuses Constitutionnelles. Radiol. Imag. MÉDICALE Musculosquelettique-Neurol.-Maxillofac. 2012, 7, 1–19. [Google Scholar] [CrossRef]
- Goncalves, L.; Jeanty, P.J. Fetal Biometry of Skeletal Dysplasias. J Ultrasound Med 1994, 13, 977–985. [Google Scholar] [CrossRef] [PubMed]
- Hauguel-de Mouzon, S.; Lepercq, J. Physiopathologie de La Croissance Du Fœtus de Mère Diabétique. Médecine Thérapeutique Endocrinol. 2001, 3, 49–52. [Google Scholar]
- Touhami Elouazzani, F.; Kabiri, M.; Karboubi, L.; Keswati, J.; Mrabet, M.; Barkat, A. La Macrosomie: À Propos de 255 °C as. J. Pédiatrie Puériculture 2012, 25, 97–101. [Google Scholar] [CrossRef]
- Fournié, A.; Kessler, S.; Biquard, F.; Parant, O.; Connan, L. Hypotrophie, Retard de Croissance Intra-Utérin, Souffrance Fœtale Chronique. EMC-Gynécologie-Obs. 2004, 1, 97–126. [Google Scholar] [CrossRef]
- Egounlety, F. Suivi Entre 1 et 3 ans D’enfants nés Petits Pour L’âge Gestationnel (”RCIU”): Étude Descriptive Multicentrique de 2 Populations Issues du Réseau Pédiatrique Sud et Ouest Francilien, les Grands Prématurés RICU et les Nouveau-nés de Plus de 33 SA RICU A.; Université de Lorraine: Nancy, France, 2016. [Google Scholar]
- Lee, P.A.; Chernausek, S.D.; Hokken-Koelega, A.C.S.; Czernichow, P. International Small for Gestational Age Advisory Board Consensus Development Conference Statement: Management of Short Children Born Small for Gestational Age, April 24–October 1, 2001. Pediatrics 2003, 111, 1253–1261. [Google Scholar] [CrossRef]
- Urs Zumsteg, B. RCIU et retard de croissance-indication pour l’hormone de croissance. Paediatrica 2011, 22, 12–13. [Google Scholar]
- Gascoin, G.; Flamant, C. Conséquences à long terme des enfants nés dans un contexte de retard de croissance intra-utérin et/ou petits pour l’âge gestationnel. J. Gynécologie Obs. Biol. Reprod. 2013, 42, 911–920. [Google Scholar] [CrossRef]
- Léger, J. L’enfant Né Petit Pour l’âge Gestationnel: Sa Croissance, Son Devenir. Médecine Thérapeutique Pédiatrie 2006, 9, 242–250. [Google Scholar]
- Larroche, J.C. Developmental Pathology of the Neonate; Excerpta Medica: Paris, France, 1977; ISBN 978-90-219-2107-5. [Google Scholar]
- Singer, D.; Sung, C.; Wigglesworth, J. Fetal Growth and Maturation: With Standards for Body and Organ Development. In Textbook of Fetal and Perinatal Pathology; Blackwell Scientific: Cambridge, MA, USA, 1991; pp. 11–47. [Google Scholar]
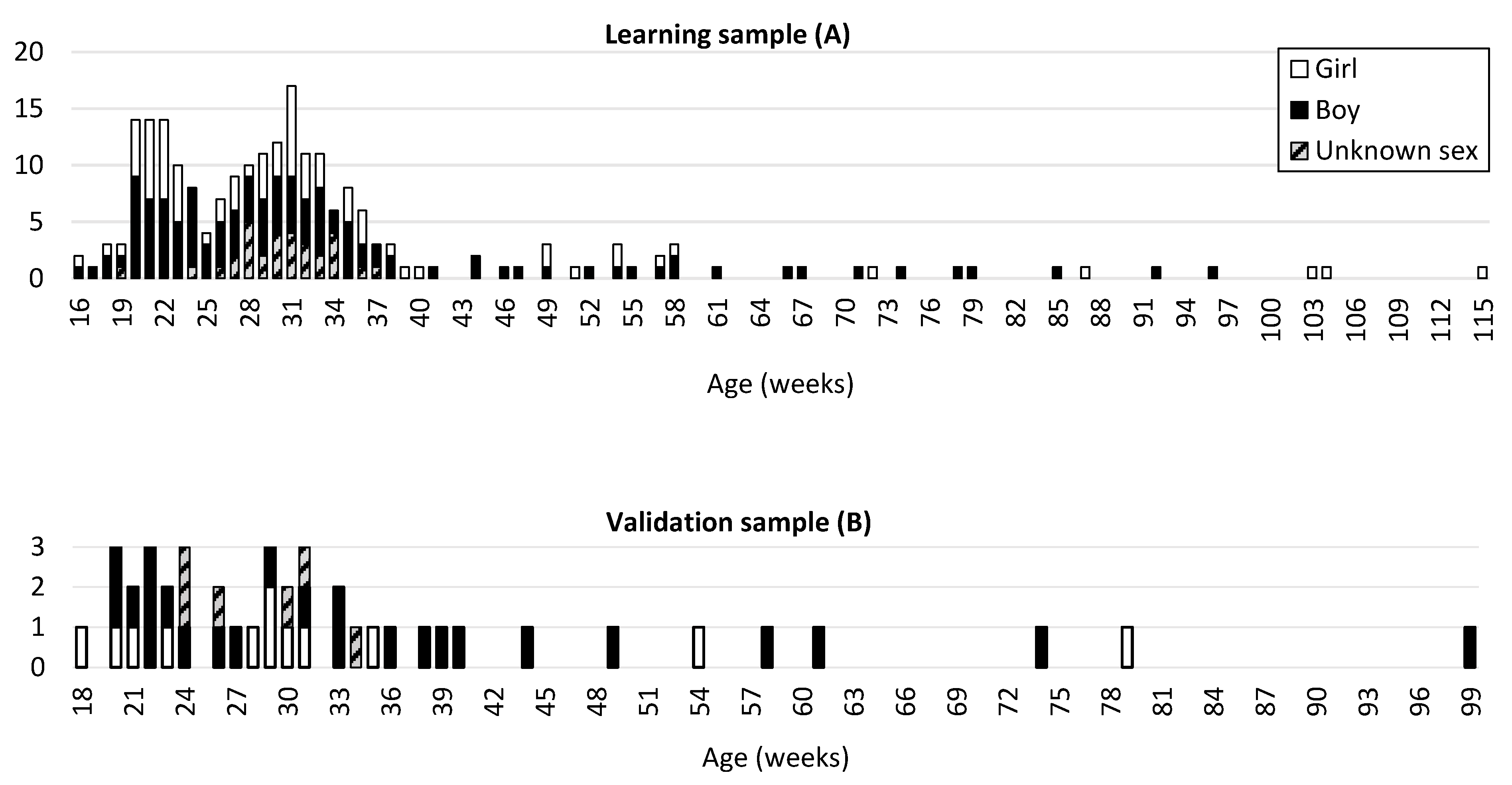



| Stage | Age Group (Weeks) | Number of Weeks | Number of Individuals | Percentiles | ||||
|---|---|---|---|---|---|---|---|---|
| 0–10 | 10 | 50 | 90 | 100 + 10 | ||||
| 1 | 16–19 | 4 | 9 | 22.11 | 25.57 | 30.09 | 35.33 | 40.08 |
| 2 | 18–21 | 4 | 34 | 23.41 | 31.58 | 37.93 | 41.12 | 46.34 |
| 3 | 20–23 | 4 | 52 | 27.71 | 34.73 | 39.42 | 44.36 | 51.21 |
| 4 | 22–25 | 4 | 36 | 32.25 | 36.88 | 43.19 | 47.18 | 54.30 |
| 5 | 24–27 | 4 | 28 | 33.41 | 43.17 | 49.34 | 54.97 | 58.60 |
| 6 | 26–29 | 4 | 37 | 38.74 | 48.04 | 52.20 | 56.72 | 63.89 |
| 7 | 28–31 | 4 | 50 | 43.60 | 51.15 | 56.70 | 60.42 | 65.57 |
| 8 | 30–33 | 4 | 51 | 46.62 | 56.22 | 59.28 | 62.54 | 72.43 |
| 9 | 32–35 | 4 | 36 | 51.58 | 58.09 | 62.53 | 66.44 | 81.15 |
| 10 | 34–37 | 4 | 23 | 61.72 | 63.59 | 65.56 | 71.68 | 75.91 |
| 11 | 36–40 | 5 | 14 | 61.55 | 65.66 | 71.14 | 76.48 | 79.70 |
| 12 | 38–44 | 7 | 8 | 71.60 | 73.41 | 77.71 | 88.29 | 102.87 |
| 13 | 41–51 | 11 | 9 | 79.18 | 82.26 | 90.68 | 94.95 | 96.20 |
| 14 | 44–57 | 14 | 15 | 75.98 | 85.46 | 94.79 | 104.14 | 105.43 |
| 15 | 51–66 | 16 | 13 | 87.78 | 94.42 | 103.51 | 111.75 | 122.13 |
| 16 | 58–78 | 21 | 10 | 97.49 | 107.55 | 112.40 | 117.30 | 118.52 |
| 17 | 67–92 | 26 | 9 | 104.94 | 111.99 | 117.91 | 135.63 | 164.87 |
| 18 | 79–104 | 26 | 7 | 121.72 | 123.99 | 132.02 | 151.45 | 155.06 |
| 19 | 92–115 | 24 | 5 | 131.92 | 132.13 | 149.79 | 152.05 | 154.46 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niel, M.; Chaumoître, K.; Adalian, P. Age-at-Death Estimation of Fetuses and Infants in Forensic Anthropology: A New “Coupling” Method to Detect Biases Due to Altered Growth Trajectories. Biology 2022, 11, 200. https://doi.org/10.3390/biology11020200
Niel M, Chaumoître K, Adalian P. Age-at-Death Estimation of Fetuses and Infants in Forensic Anthropology: A New “Coupling” Method to Detect Biases Due to Altered Growth Trajectories. Biology. 2022; 11(2):200. https://doi.org/10.3390/biology11020200
Chicago/Turabian StyleNiel, Mélissa, Kathia Chaumoître, and Pascal Adalian. 2022. "Age-at-Death Estimation of Fetuses and Infants in Forensic Anthropology: A New “Coupling” Method to Detect Biases Due to Altered Growth Trajectories" Biology 11, no. 2: 200. https://doi.org/10.3390/biology11020200
APA StyleNiel, M., Chaumoître, K., & Adalian, P. (2022). Age-at-Death Estimation of Fetuses and Infants in Forensic Anthropology: A New “Coupling” Method to Detect Biases Due to Altered Growth Trajectories. Biology, 11(2), 200. https://doi.org/10.3390/biology11020200






