Effect of Intermediate Principal Stress on the Bearing Capacity of Footings in Soft Rock

Abstract

The bearing capacity for footings is a fundamental scientific problem in civil engineering. The evaluation of the bearing capacity of footings usually does not take into account the effect of the intermediate principal stress. In practice, the intermediate principal stress has certain influences on the strength of geomaterials (e.g., rock and soil) or concrete. In this paper, a series of numerical solutions are presented to evaluate the bearing capacity of footings in a soft rock foundation via a two-dimensional finite difference code (FLAC) with a strain hardening/softening constitutive model based on the unified strength theory (UST). The values of the bearing capacity factor N_c and N_γ for strip, circular and square footings in a soft rock foundation were evaluated using the strain hardening/softening constitutive model. The effect of the intermediate principal stress on the bearing capacity of strip, circular and square footings in a soft rock foundation was analyzed. The results of the numerical computation show that the intermediate principal stress has a significant influence on the bearing capacity and failure mechanisms of a soft rock medium. The influence of the intermediate principal stress on the peak and residual values of the bearing capacity for a strip footing is much greater than for circular and square footings. Research works for the reasonable estimation of the bearing capacity of footings in soft rock are facilitated by this study.

1. Introduction

A rock material can be classified as soft rock if the uniaxial compressive strength (USC) is below 30 MPa [1,2]. The deformation process of soft rock often has a remarkable strain-softening characteristic with a peak and residual strength [3,4,5,6]. Many researchers investigate the problems of soft rock in geotechnical engineering. For example, the stability of tunnels constructed in soft rock was analyzed by Zhu and Tokiwa [7,8]. The evaluation of the bearing capacity for footings is an essential problem in geotechnical engineering. In recent years, the bearing capacity of footings in clay or sand is often researched by many investigators using analytical or numerical methods with a linearly elastic-perfectly plastic constitutive model [9,10,11,12,13,14,15]. However, the bearing capacity of footings in strain-softening materials (e.g., soft rock) is seldom studied [16]. Using the linearly elastic, perfectly plastic constitutive model, Ma investigated the influence of the intermediate principal stress on the bearing capacity of strip or circular footings [17]. The intermediate principal stress has more influence on the bearing capacity of a strip footing than that of circular footing, and the intermediate principal stress has much more influence on the bearing capacity factor N_γ than that of factor N_c [17].

Many complex stress tests (e.g., true-triaxial tests, plane strain tests, or torsion shear tests) have verified that the intermediate principal stress has specific influences on the mechanical behavior of geomaterials [18,19,20,21,22,23,24,25,26]. The polyaxial test data of sand obtained by Sutherland and Mesdary suggest that the intermediate principal stress has a marked effect on the strength of sand and that the Mohr–Coulomb theory will therefore underestimate the strength over most of the stress state range [20]. Mogi found that in true triaxial testing of many rock samples, the effect of the intermediate principal stress must be considered for rock [21]. The results of complex stress tests for concrete indicate that the intermediate principal stress also has a marked effect on the strength of concrete [27,28]. We can conclude that the intermediate principal stress effect is an inherent mechanical behavior of many materials. Several strength criteria that take the effect of the intermediate principal stress and nonlinear yield surface into account were proposed for geomaterials [29]. These criteria include the Zienkiewicz–Pande criterion, the Lade–Duncan criterion, and the Matsuoka–Nakai criterion [30,31].

In this study, a numerical solution of the bearing capacity of footings in a soft rock foundation was proposed taking the strain hardening/softening material behavior and the intermediate principal stress effect into account. The influence of the intermediate principal stress on the bearing capacity of strip, circular and square footings in a soft rock foundation will be analyzed.

2. Materials, Theories and Methods

The unified strength theory (UST), which takes the influence of the intermediate principal shear stress (or intermediate principal stress) into account, was proposed by Yu [32]. The expressions of the UST can be written in terms of the principal stresses as follows:

$$\{\begin{array}{l}f=\frac{b{\sigma }_2+{\sigma }_3}{\left(1+b\right)\left(1+\sin \phi \right)/\left(1-\sin \phi \right)}-{\sigma }_1+\frac{2c}{\sqrt{\left(1+\sin \phi \right)/\left(1-\sin \phi \right)}},\mathrm{when}{\sigma }_2\le \frac{1+\sin \phi }{2}{\sigma }_1+\frac{1-\sin \phi }{2}{\sigma }_3\\ f^{\prime }=\frac{{\sigma }_3}{\left(1+\sin \phi \right)/\left(1-\sin \phi \right)}-\frac{{\sigma }_1+b{\sigma }_2}{1+b}+\frac{2c}{\sqrt{\left(1+\sin \phi \right)/\left(1-\sin \phi \right)}},\mathrm{when}{\sigma }_2\ge \frac{1+\sin \phi }{2}{\sigma }_1+\frac{1-\sin \phi }{2}{\sigma }_3\end{array}$$

(1)

where b is a coefficient reflecting the effect of the intermediate principal stress on the strength of geomaterials, c and φ are the cohesion and friction angle of geomaterials, respectively. The order of the three principal stresses follows σ₁ ≥ σ₂ ≥ σ₃. For the non-associated flow rule, the plastic potential function g can be written as follows:

$$\{\begin{array}{l}g=\frac{b{\sigma }_2+{\sigma }_3}{(1+b)\left(1+\sin \psi \right)/\left(1-\sin \psi \right)}-{\sigma }_1,\mathrm{when}{\sigma }_2\le \frac{1+\sin \phi }{2}{\sigma }_1+\frac{1-\sin \phi }{2}{\sigma }_3\\ g^{\prime }=\frac{{\sigma }_3}{\left(1+\sin \psi \right)/\left(1-\sin \psi \right)}-\frac{{\sigma }_1+b{\sigma }_2}{1+b},\mathrm{when}{\sigma }_2\ge \frac{1+\sin \phi }{2}{\sigma }_1+\frac{1-\sin \phi }{2}{\sigma }_3\end{array}$$

(2)

where ψ is the dilation angle for geomaterials (the plastic flow rule is non-associated if ψ < φ, and the plastic flow rule is associated if ψ = φ). The limit loci of UST on the deviatoric plane or principal stress space are shown in Figure 1a,b, where θ_b is the stress angle for the junction of two yield surfaces and depends only on the friction angle of the material. Comparisons between the yield surfaces of the UST and test data for granite, marble, and sand under a complex stress state are plotted in Figure 2, the intermediate principal stress has a substantial influence on the strengths of geomaterials under complex stress states. UST can predict the potential strengths of different geomaterials with various values of the parameter b. The yield surfaces of the UST cover the entire region of convex theory from the lower bound (UST b = 0.0) to the upper bound (UST b = 1.0). The Mohr–Coulomb strength criterion is the special case of the UST when the coefficient b equals zero.

In this study, the vertical bearing capacity solutions for strip, circular and square footings in a soft rock foundation were evaluated using a finite difference code FLAC/FLAC3D and strain hardening/softening constitutive model based on the UST. FLAC/FLAC3D (fast Lagrangian analysis of continua) is a two/three-dimensional (2D/3D) finite-difference code that uses an explicit Lagrangian computation scheme. The behavior of structures built of soil, rock, or other materials that may undergo plastic flow when their yield limits are reached can be simulated easily by FLAC/FLAC3D [33,34]. The derivation and verification of the UST elastoplastic constitutive model in FLAC/FLAC3D were discussed by Ma [35,36]. In this study, the strain hardening/softening constitutive model based on the UST is written in C++ as a user-written constitutive model and compiled as a DLL file (dynamic link library), and it can be loaded into the FLAC/FLAC3D code. The elastic relations between the elastic principal strain increments and principal stress increments in FLAC/FLAC3D are as follows:

$$\Delta {\sigma }_i=S_i\left(\Delta {\epsilon }_n^e\right)i=1,n\mathrm{and}n=1,3$$

(3)

where S_i is a linear function of the elastic principal strain increments $\Delta {\epsilon }_n^e$. The plastic principal strain increments can be written as follows:

$$\Delta {\epsilon }_i^p=\lambda \partial g/\partial {\sigma }_i$$

(4)

where λ is a non-negative multiplier if plastic loading occurs. The expression of the elastoplastic constitutive model for FLAC/FLAC3D can be formulated as follows:

$${\sigma }_i^N={\sigma }_i^I-\lambda \cdot S_i\left(\frac{\partial g}{\partial {\sigma }_n}\right)$$

(5)

where S_i(∂g/∂σ_n) is the matrix of the constitutive model composed by the plastic principal strain component, g is the plastic potential function, ${\sigma }_i^I$ are the stress components obtained from the elastic Hooke’s law (elastic trial stress), and ${\sigma }_i^N$ are the new stress components obtained from the plastic flow rule (if the elastic trial stress exceed the Equation (1)). The expression of the plastic multiplier λ can be written as follows:

$$\lambda =f\left({\sigma }_n^I\right)/\left[f\left(S_n\left(\frac{\partial g}{\partial {\sigma }_n}\right)\right)-f\left(0\right)\right]$$

(6)

Equation (6) is accurate only for an elastic perfectly plastic material, for which the strength parameters are constant. Theoretically, Equation (6) is non-rigorous for hardening/softening material because it lacks the hardening modulus. In this study, the hardening parameter e^ps is used to characterize the hardening/softening behavior via the plastic shear strain [37], and e^ps is defined as follows:

$$e^{ps}={\left\{\frac{1}{2}\left[{\left({\epsilon }_1^{ps}-{\epsilon }_m^{ps}\right)}^2+{\left({\epsilon }_2^{ps}-{\epsilon }_m^{ps}\right)}^2+{\left({\epsilon }_3^{ps}-{\epsilon }_m^{ps}\right)}^2\right]\right\}}^{1/2}$$

(7)

where ${\epsilon }_1^{ps}$, ${\epsilon }_2^{ps}$ and ${\epsilon }_3^{ps}$ are the three plastic principal strains of the shear strength envelope and ${\epsilon }_m^{ps}=\left({\epsilon }_1^{ps}+{\epsilon }_2^{ps}+{\epsilon }_3^{ps}\right)/3$. It is observed from Equation (7) that the hardening parameter e^ps equals the square root of the second invariant of the strain. For the plastic hardening/softening stage, the plastic potential function g can be written as follows:

$$g\left[{\sigma }_n,c(e^{ps}),\phi (e^{ps})\right]=0n=1,3$$

(8)

Liao et al. and Li conducted consolidated-undrained (CU) triaxial shear tests on saturated diatomaceous soft rock and mudstone, respectively [38,39]. The effective stress is used to calculate the shear strength parameters using the test data of the CU test for diatomaceous rock and mudstone. The triaxial test results on the diatomaceous soft rock and mudstone show that the effective strength parameter φ for the two types of soft rock has peak and residual values with the value of axial strain ε₁ increased (shown in Figure 3a). The effective strength parameter c (cohesion) of the two types of soft rock remains constant during the deformation progress of the soft rock sample. In this study, the friction angle φ in the plastic potential function g varies with the values of the hardening parameter e^ps. In contrast, cohesion c does not depend on the hardening parameter e^ps. The hump curve function is used as the hardening/softening function to simulate the strain-softening behavior of soft rock in this study. The relationship between the friction angle φ and the hardening parameter e^ps predicted by the hump curve function is shown in Figure 4, and the hump curve function is described as follows:

$$\phi (e^{ps})=\frac{e^{ps}\left(H+R{e}^{ps}\right)}{{\left(H+P{e}^{ps}\right)}^2}$$

(9)

where the parameter H controls the slope of the hump curve, and the parameters P and R control the peak and residual value of the hump curve. The values of the parameters H, P, and R in Equation (9) can be determined by the variation in the strength parameter φ yielded by a triaxial test of the soft rock. The UST elastoplastic constitutive model can be established via the substitution of Equations (4), (6), and (9) into Equation (5). The plastic shear strain e^ps are updated by each time step (time increment Δt) in the numerical computation, and the values of friction angle φ will be changed with the variation of the plastic shear strain e^ps after each time step. Thus, the type of hardening rule is isotropic. The loading surface of the strain hardening/softening model is expanded with the variation of the values of friction angle φ, and the hardening/softening behavior of the soft rock can be predicted.

A 2D axisymmetric element was used to simulate a test sample of soft rock, and the consolidated-undrained triaxial test was simulated using FLAC code. The confining pressure σ₃ was applied by the stress boundary condition on all sides of the test sample element to generate the consolidated stress. For the conventional triaxial test situation (simple stress state), the three principal stresses follow σ₁ ≥ σ₂ = σ₃, a vertical velocity load (1 × 10⁻⁶ m/step) was applied to the top of the test sample element to simulate the maximum principal stress σ₁. The bottom of the test sample element was fixed in the vertical direction. The values of the material property parameters for numerical analysis are presented in

Table 1. Parameters and values for numerical analysis.

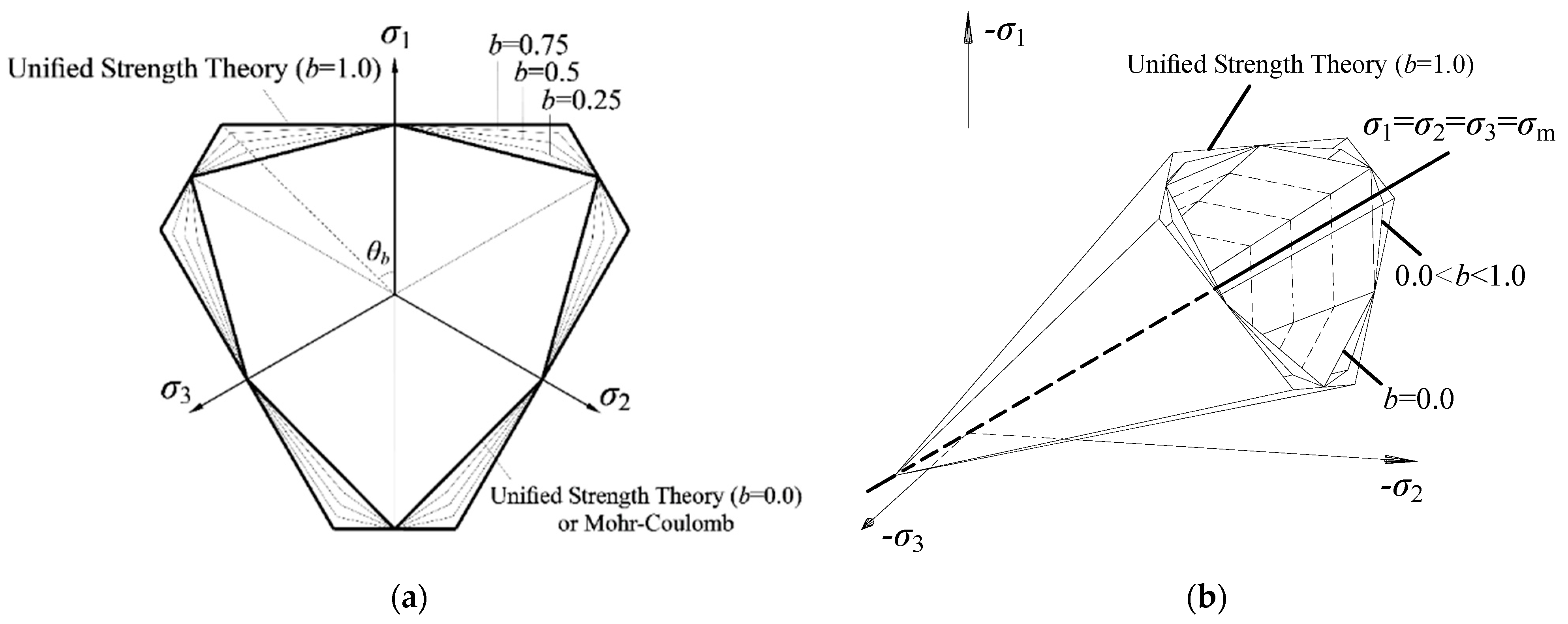
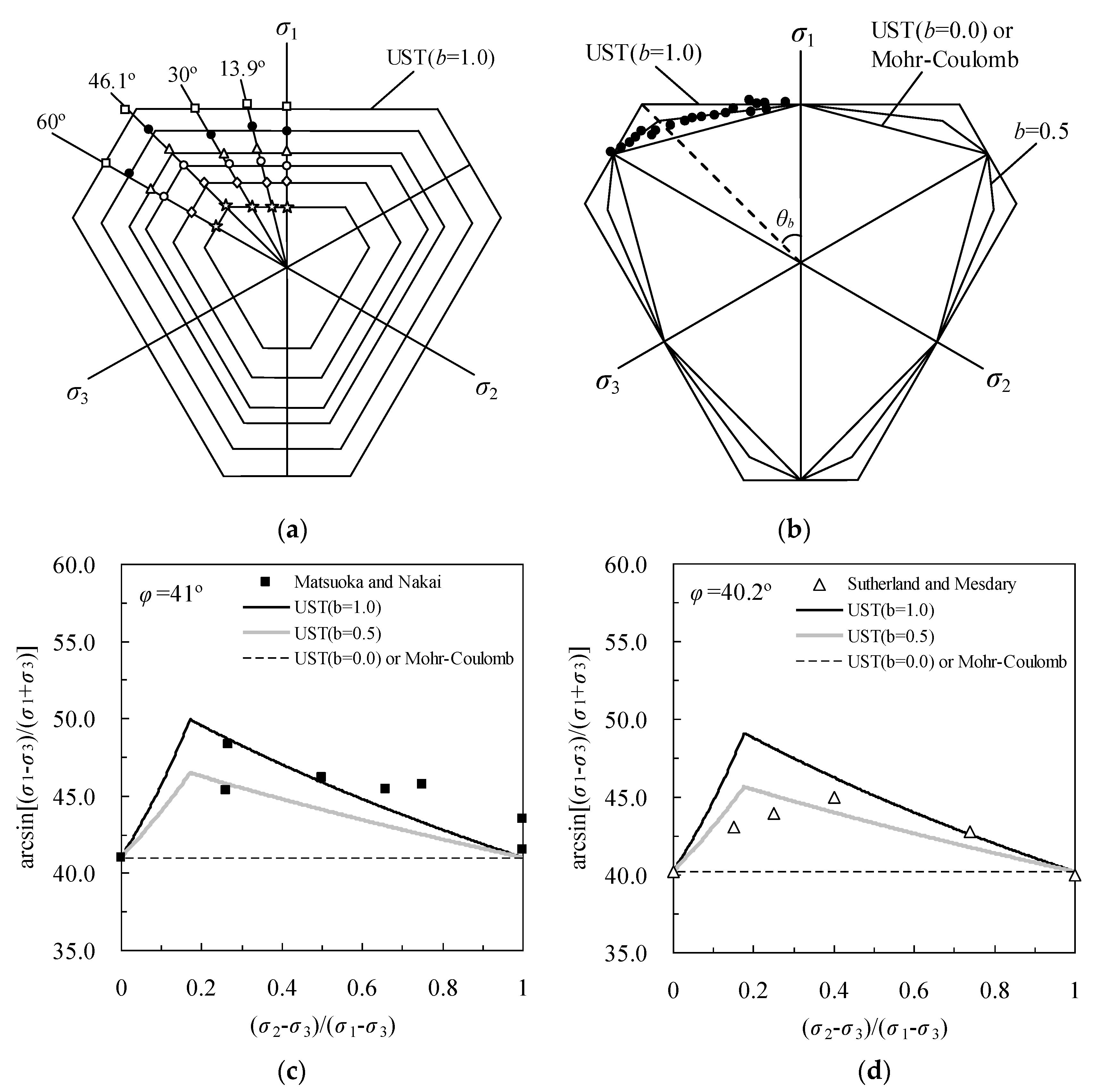
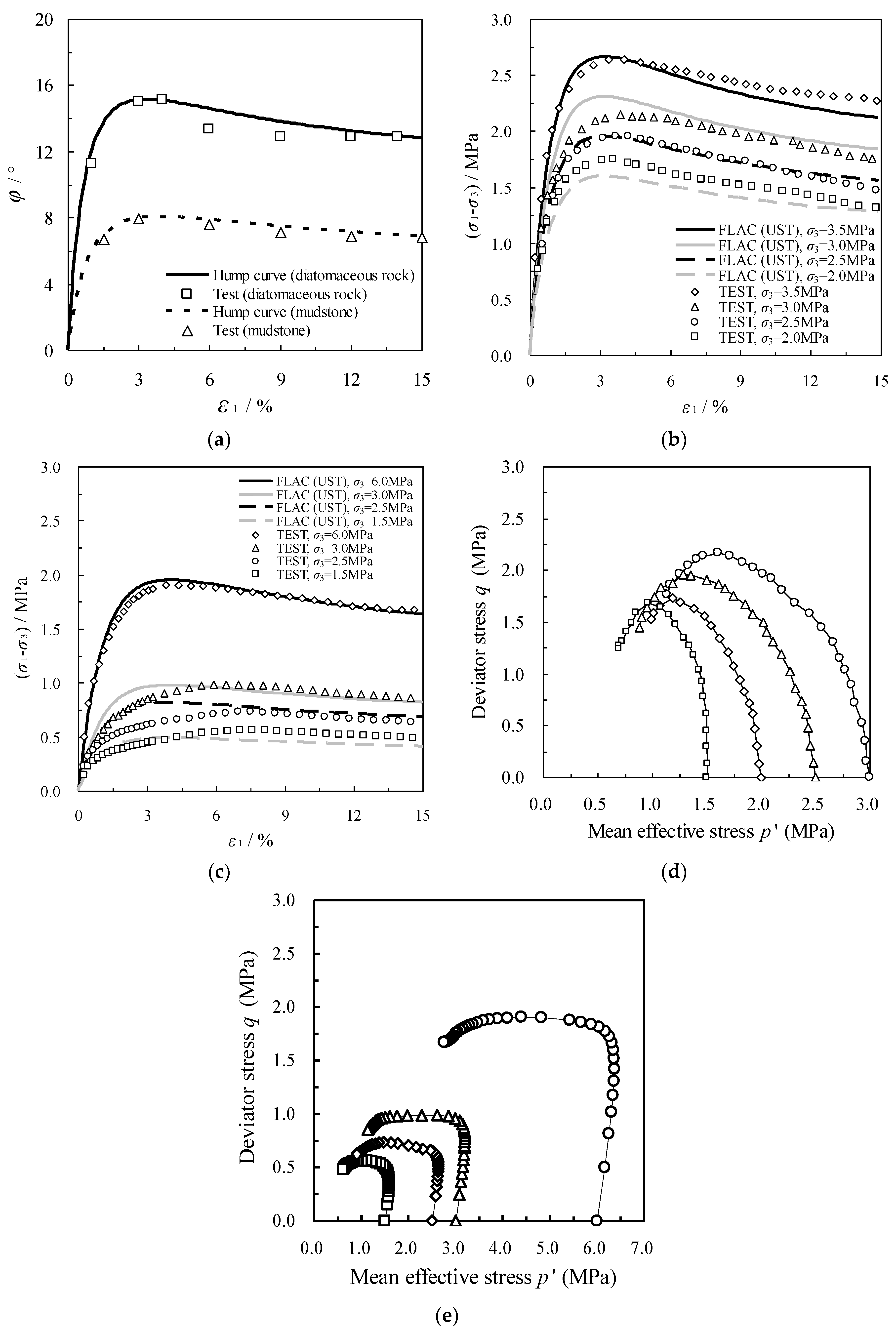
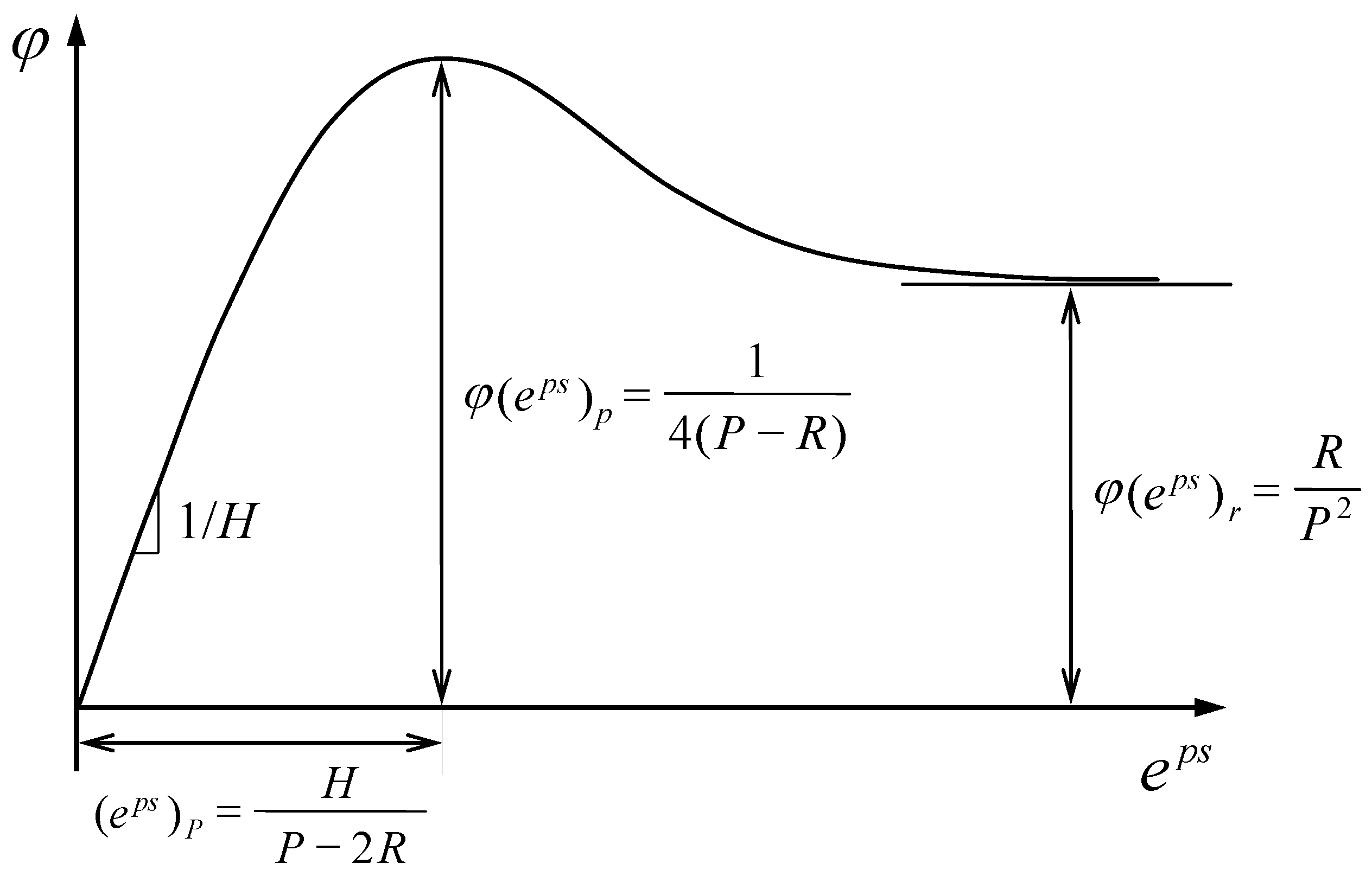
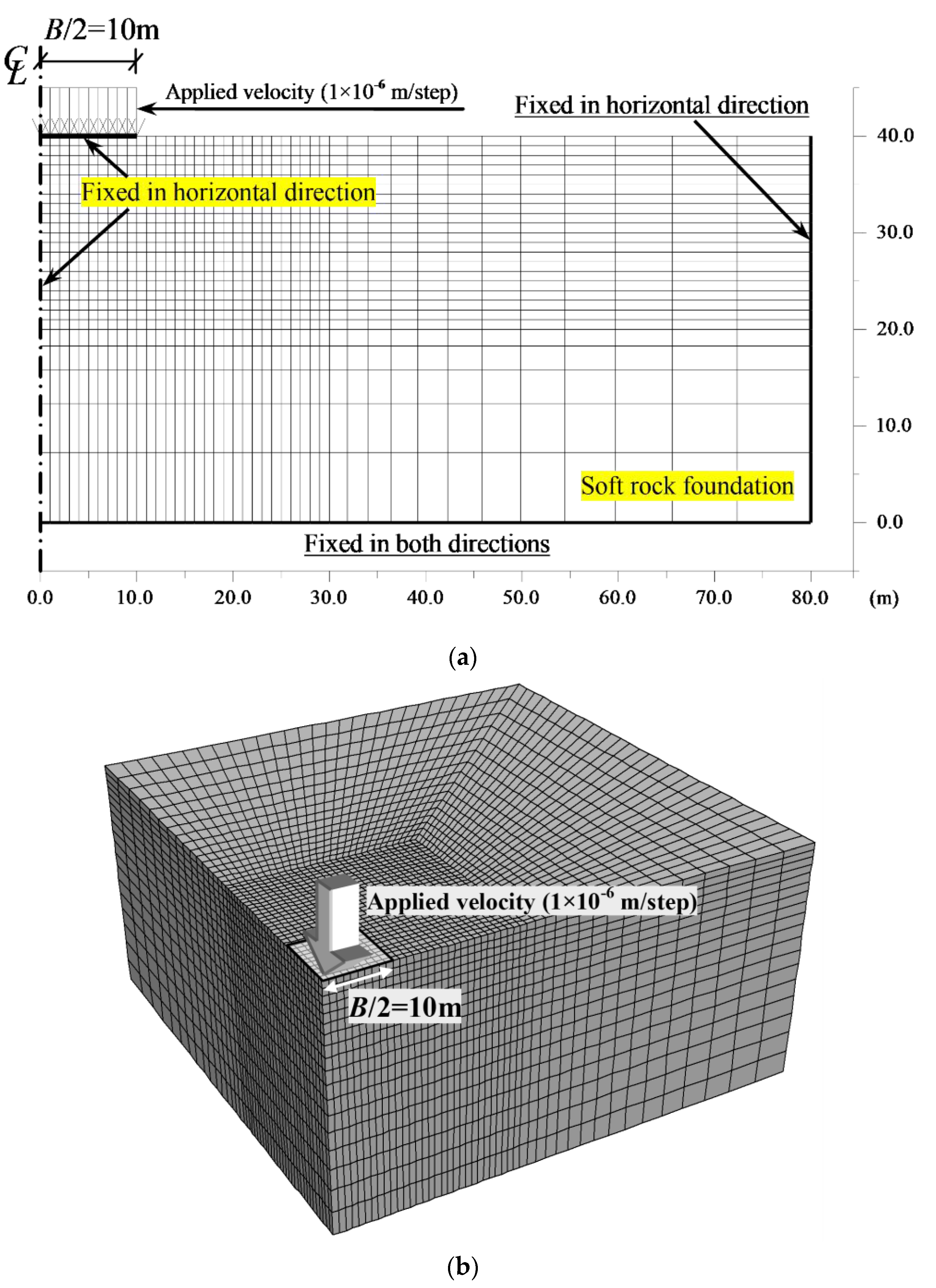
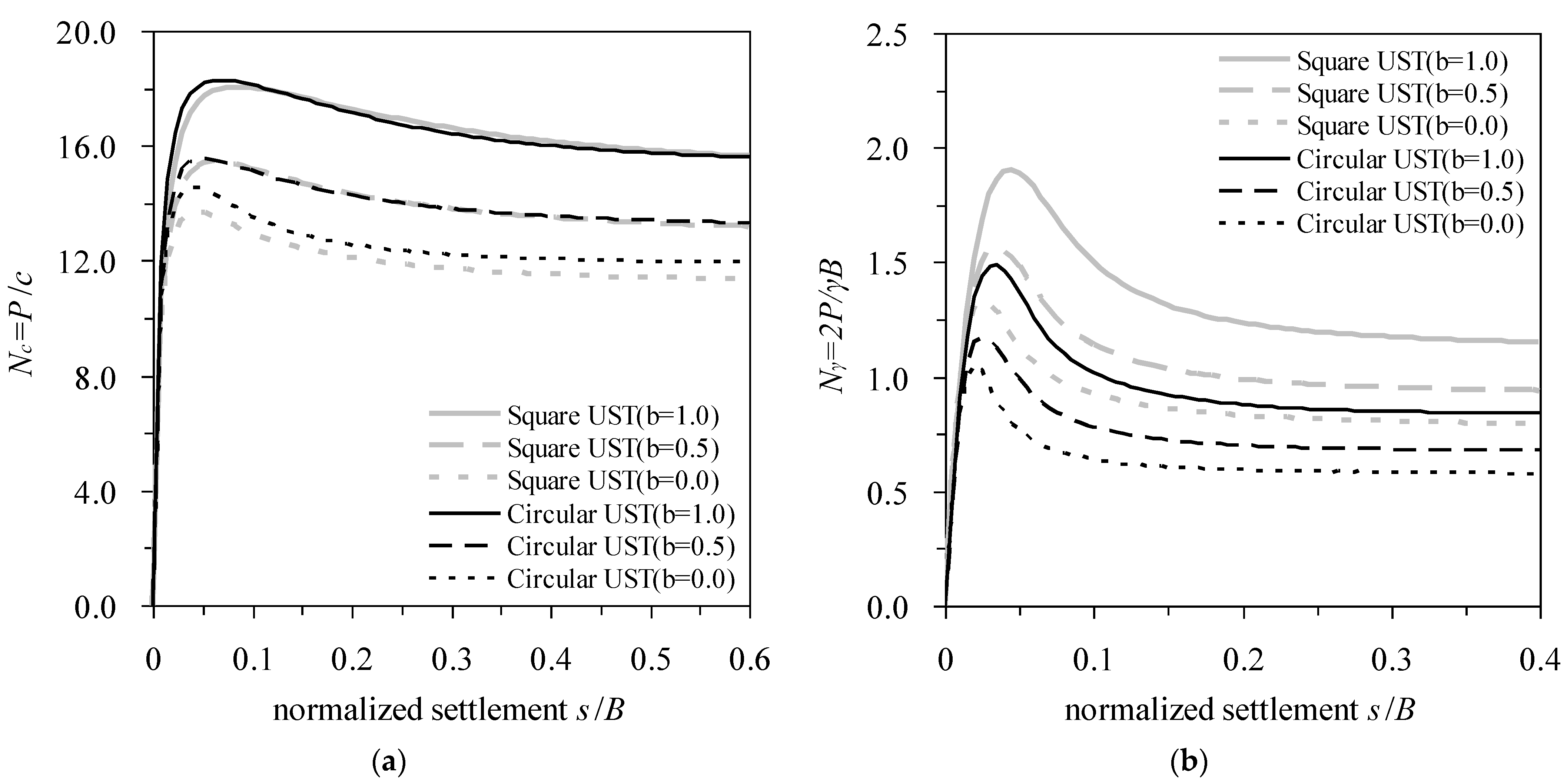
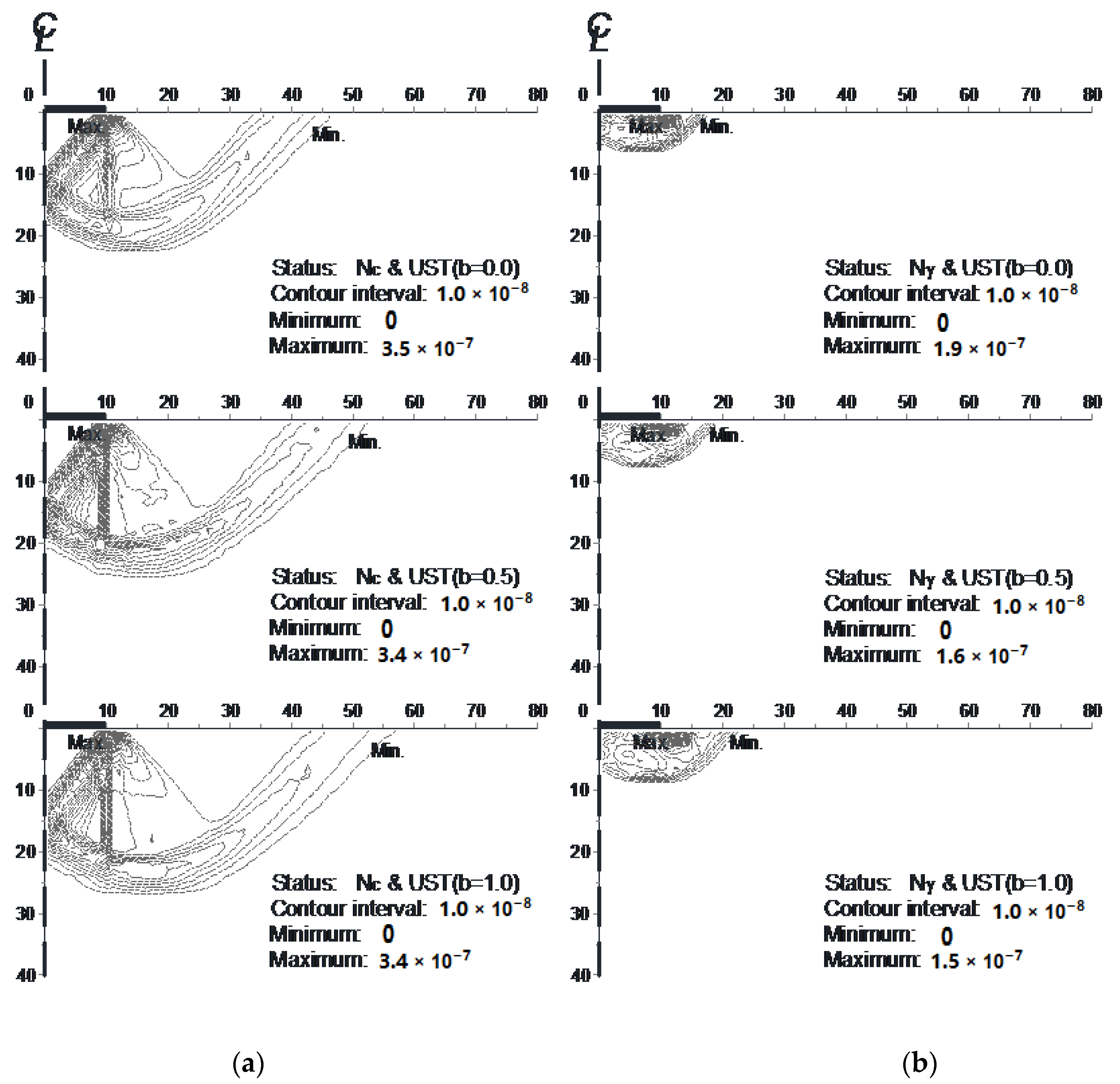
Name	Density ρ (kg/m3)	Elastic Modulus E (MPa)	Poisson’s Ratio ν	Cohesion c (kPa)	Peak Friction Angle (°)	Residual Friction Angle (°)	P	H	R
Diatomaceous soft rock	2183	500	0.25	40	15.1	12.8	0.021	0.04	0.0045
Mudstone	2032	500	0.25	1.2	8.0	6.8	0.039	0.09	0.0079

. Because the test sample of soft rock had slight volume dilation after the consolidated-undrained triaxial test, the dilation angle ψ was set to zero (non-associated flow rule) throughout the whole calculation process of the triaxial test simulation. Figure 3a,b show the relationship of stress (σ₁–σ₃) versus axial strain (ε₁) for diatomaceous soft rock and mudstone, respectively, measured by the consolidated-undrained triaxial test or simulated by FLAC using the hump curve function (Equation (9)). The comparison between the model prediction and measured results shows that the stress–strain relationship of soft rock under the triaxial test state (σ₁ ≥ σ₂ = σ₃) can be simulated by the elastoplastic model with the hump curve function. Then, the values of the bearing capacity factor N_c and N_γ for strip, circular and square footings in diatomaceous soft rock were evaluated by FLAC/FLAC3D using the strain hardening/softening constitutive model following the UST.

3. Calculations and Results

The 2D problem of the plane strain and axisymmetry is also under the complex stress state; three principal stress are independent and not equals to each other. Thus, the intermediate principal stress will have a specific influence on the 2D problem. Strip and circular footings can be considered as plane strain and axisymmetric problems, respectively. The square footing must be analyzed as a 3D problem. Vesić suggested an equation of the ultimate bearing capacity for a strip footing on a soil foundation. The formula is expressed as follows [40]:

$$P_u=c\cdot N_c+\frac{\gamma \cdot B}{2}{N}_{\gamma }$$

(10)

$$N_c=\cot \phi \cdot \left[\frac{1+\sin \phi }{1-\sin \phi }\exp (\pi \cdot \tan \phi )-1\right]$$

(11)

$$N_{\gamma }=2\left[\frac{1+\sin \phi }{1-\sin \phi }\exp (\pi \cdot \tan \phi )+1\right]\tan \phi$$

(12)

$$P_u=c\cdot N_c+q{N}_q+0.4B{N}_{\gamma }\left(\mathrm{for}\mathrm{square}\mathrm{foundation}\right)$$

(13)

where P_u is the ultimate bearing capacity of strip footing, N_c is the bearing capacity factor of cohesion c, γ is the soil unit weight, B is the footing width, and N_γ is the soil self-weight bearing capacity. Terzaghi also proposed the formulas of factors N_c and N_γ for rough rigid and strip footings [41]. Circular footings, which belong to the class of axisymmetric problems, were considered by Eason and Shield and Cox et al. using the characteristic method [42,43]. The bearing capacity of a square footing has been investigated by some researchers using numerical and testing methods [44,45,46,47].

In this study, the foundation was considered a strain-softening and homogeneous material (soft rock) and discretized into several finite-difference element meshes. The footings are considered to be perfectly rigid and rough. The influence of the intermediate principal stress on the bearing capacity of strip, circular and square footings was analyzed, taking the strain hardening/softening behavior of soft rock into account. The residual strength of soft rock is practically irrelevant, and it is only of the theoretical value. In this study, the peak and residual bearing capacity of soft rock foundations are both considered for analysis. The values of the factors N_c and N_γ for strip, circular and square footings in diatomaceous soft rock were evaluated by FLAC and FLAC3D code with strain hardening/softening constitutive model following the UST and dilation angle ψ = 0. Because the problem domain is symmetric, only half of the problem domain was considered, and the footing width B was held constant at 20 m. Because the left vertical boundary was the symmetry plane, the horizontal displacement was fixed, and the vertical displacement was free. The right vertical boundary was only constrained in the horizontal direction. The displacement of the bottom boundary was fixed in both the vertical and horizontal directions. The boundary condition and mesh for numerical analysis are shown in Figure 5. The analyses were performed by applying a vertical velocity (1 × 10⁻⁶ m/step) to simulate the load from the rigid footing base. The contact stress P beneath the strip footing was calculated as the sum of the vertical nodal forces beneath the footing divided by the half-width of the footing [10]. The contact stress P beneath the circular footing was calculated by dividing the sum of the vertical footing nodal forces by the footing area, the radius of which is equal to the distance to the center of the first element outside the footing [9]. The horizontal velocity at the surface nodes beneath the footing was set to zero to simulate the rough interface between the footing and soft rock foundation. Previous studies observed that the value of Poisson’s ratio ν and elastic modulus E do not influence the value of the bearing capacity of footings [11]. The tensile failure at the free surface near the footing base has little influence on the ultimate bearing capacity of footings [9,10]. Shear failure is placed in most elements beneath the footing under the limit state, and the ultimate bearing capacity of footing mainly depends on the shear strength parameters (c and φ) of soft rock.

The two bearing capacity factors (N_c and N_γ) can be obtained individually using the numerical method [10,11] and can be expressed by the following equations:

$$\{\begin{array}{l}{N}_c=P/c,\mathrm{when}\gamma =0\\ N_{\gamma }=2P/\gamma B,\mathrm{when}c=0\end{array}$$

(14)

where, P is the contact stress beneath the footing base, B is the width of the footing. The initial soil stress state must be established before the numerical computation of the bearing capacity factor N_γ. The initial geostress state for foundation soil is calculated with gravity, and a load of footing base is not applied to the surface of the foundation. Secondly, the vertical load is applied to the nodes underneath the footing base after the initial geostress of the foundation is established. The peak and residual values of bearing capacity factor N_c and N_γ yield by FLAC and UST are shown in

Table 2. Peak and residual values of bearing capacity factor N_c yield by FLAC and UST.

Geometry	Strip					Circular					Square
UST	b = 0.0	b = 0.25	b = 0.5	b = 0.75	b = 1.0	b = 0.75	b = 1.0	b = 0.5	b = 0.75	b = 1.0	b = 0.0	b = 0.25	b = 0.5	b = 0.75	b = 1.0
Peak values	10.27	12.27	14.22	15.77	17.20	14.56	14.88	15.57	16.69	18.28	13.68	14.45	15.43	16.55	18.05
Residual values	8.72	10.30	11.90	13.10	14.16	11.94	12.46	13.32	14.17	15.60	11.34	12.21	13.17	14.31	15.65

and

Table 3. Peak and residual values of bearing capacity factor N_γ yield by FLAC and UST.

Geometry	Strip					Circular					Square
UST	b = 0.0	b = 0.25	b = 0.5	b = 0.75	b = 1.0	b = 0.0	b = 0.25	b = 0.5	b = 0.75	b = 1.0	b = 0.0	b = 0.25	b = 0.5	b = 0.75	b = 1.0
Peak values	1.17	1.51	1.83	2.09	2.35	1.05	1.11	1.18	1.30	1.49	1.33	1.44	1.57	1.73	1.90
Residual values	0.67	0.86	0.99	1.14	1.28	0.58	0.63	0.68	0.75	0.84	0.79	0.85	0.94	1.03	1.15

, respectively. Two parameters ξ_c and ξ_γ are given to estimate the influence of the intermediate principal stress and are described as follows:

$${\xi }_c=\frac{N_c(\mathrm{UST},b\ge 0)}{N_c(\mathrm{Mohr}-\mathrm{Coulomb})},{\xi }_{\gamma }=\frac{N_{\gamma }(\mathrm{UST},b\ge 0)}{N_{\gamma }(\mathrm{Mohr}-\mathrm{Coulomb})}$$

(15)

where ξ_c and ξ_γ are the efficiency factors of the intermediate principal stress effect for the bearing capacity factors N_c and N_γ, respectively. Figure 6 shows the relationship of N_c = P/c and N_γ = 2P/γB versus vertical displacement s/w for strip, circular and square footings in diatomaceous soft rock as calculated by the finite-difference code FLAC. The relationship of the peak and residual values of the bearing capacity factors N_c and N_γ for strip, circular and square footings versus the parameter b of UST are shown in Figure 7. The results from Figure 6 indicate that the strain-softening characteristic for N_γ is more remarkable than that of N_c. The results from Figure 7 suggest that the peak and residual values of the factors N_c and N_γ for strip, circular and square footings are increased with increasing values of the parameter b in UST. Figure 8 shows the relationship of the efficiency factors ξ_c and ξ_γ versus the values of parameter b of UST. The results from Figure 8 indicate that the values of the efficiency factor ξ_γ for N_γ are generally higher than the efficiency factor ξ_c with the values of the parameter b increased. The influence of the intermediate principal stress on the peak and residual values of N_γ is more apparent than that of N_c. The influence of the intermediate principal stress on the peak and residual values of the bearing capacity for a strip footing is much more significant than for circular and square footings. The intermediate principal stress has a similar influence on the peak and residual values of the bearing capacity of circular and square footings. The difference between the influence of the intermediate principal stress on the peak and residual values of the bearing capacity of a soft rock foundation appears relatively small. Figure 9 shows the maximum shear strain rate contours for a rough strip footing when the soft rock foundation’s peak or residual bearing capacity has been reached.

4. Discussion

The results of numerical computation show that the influence of the intermediate principal stress on the peak and residual values of N_γ is more apparent than on the bearing capacity factor N_c. The influence of the intermediate principal stress on the peak and residual values of the bearing capacity for a strip footing is much greater than for circular and square footings. The strain-softening behavior of soft rock foundations beneath strip, circular and square footings for factor N_γ evaluations are more apparent than that of the N_c evaluations. The influence of the intermediate principal stress on the peak and residual values of N_γ is more apparent than on the bearing capacity factor N_c. The influence of the intermediate principal stress on the peak and residual values of the bearing capacity for a strip footing is much greater than for circular and square footings. The difference between the influence of the intermediate principal stress on the peak and residual values of the bearing capacity of a soft rock foundation is relatively small. The size of the shear zone in the soft rock foundation increases as the value of the UST parameter b increases. The shear zone of the soft rock foundation under peak values of the bearing capacity is larger than that of the residual values of the bearing capacity.

The results obtained from FLAC/FLAC3D code using the UST indicate that a clear difference exists between the failure mechanisms of the foundation when the influence of the intermediate principal stress is taken into account versus when it is not. The size of the shear zone increases as the value of parameter b increases, and more soft rock beneath the footing contributes to the bearing capacity of the foundation. The size of the shear zone in the peak bearing capacity of soft rock foundations is larger than that of the residual bearing capacity, and the size of the shear zone for N_c evaluation is larger than that of the N_γ.

5. Conclusions

This paper presents a series of numerical solutions for the bearing capacity of strip, circular, and square footings in a soft rock foundation. Based on the results of this study, the following conclusions can be drawn:

(1)

A strain hardening/softening constitutive model which takes the influence of the intermediate principal stress into account was established in this study. The mechanical behavior of the strain-softening material under the complex stress state can be analyzed using this model.

(2)

The intermediate principal stress significantly influences a soft rock foundation’s bearing capacity and failure mechanisms. The intermediate principal stress has less influence on the bearing capacity of footing when gravity is neglected. The influence of the intermediate principal stress on the bearing capacity for strip footing is much more significant than that of circular and square footings. The size of the failure area in the soft rock foundation increases with the increased effect of the intermediate principal stress.

(3)

The research works of our study would benefit the reasonable estimation of the bearing capacity of footings in a soft rock medium. In our future research, taking the nonlinear failure envelope on the meridian plane into account, the influence of the intermediate principal stress effect on soft rock material will be investigated.