Fluid-Induced Vibration of a Hydraulic Pipeline with Piezoelectric Active Constrained Layer-Damping Materials
Abstract
:1. Introduction
2. The Finite Element Model of ACLD Pipeline
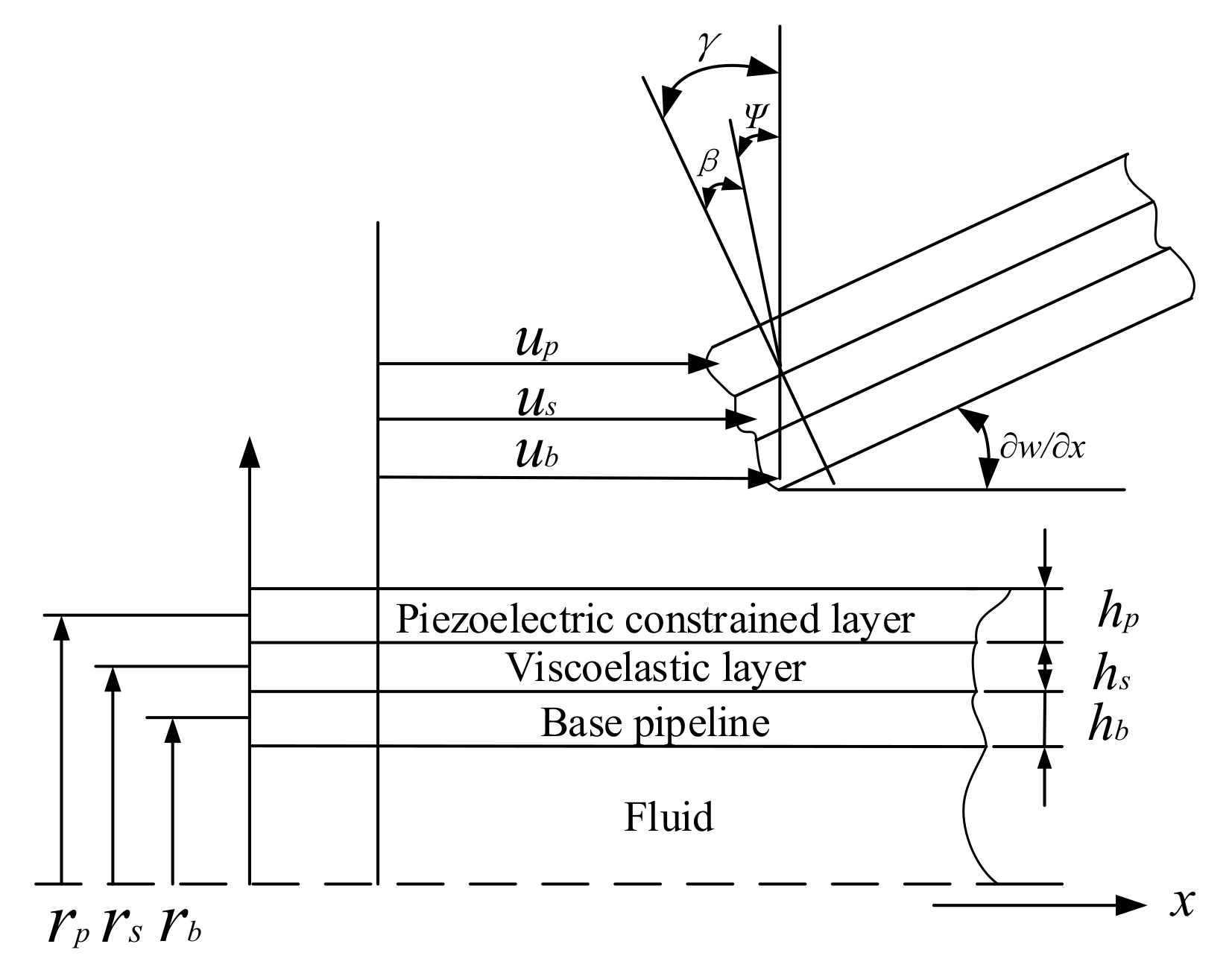
2.1. Displacement Fields
2.2. Subsection
2.3. Energy Expressions
2.3.1. The Energy Expressions of Potential Energy
2.3.2. The Energy Expressions of Kinetic Energy
2.3.3. The Virtual of Kinetic Energy
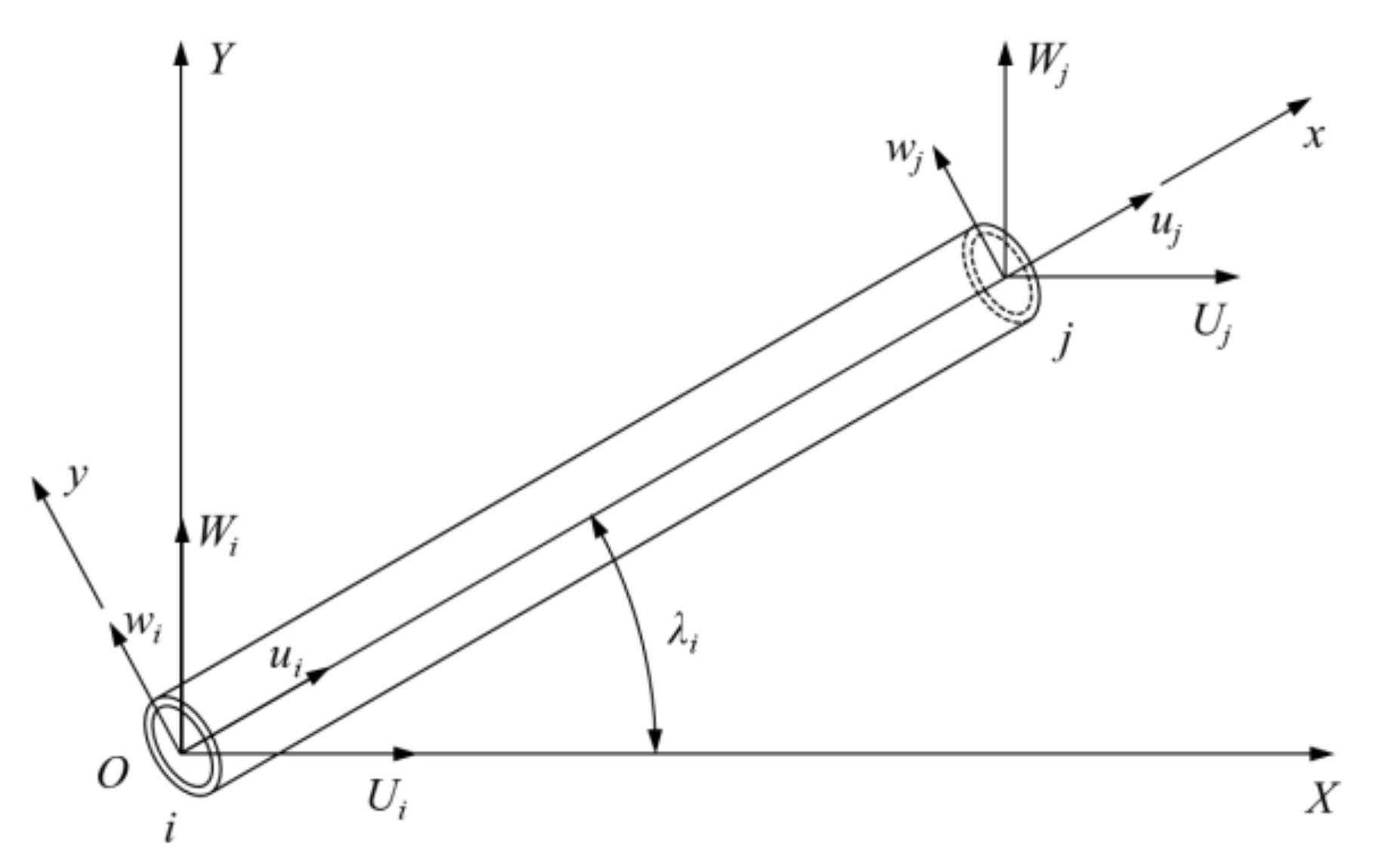
2.4. Fluid Element
2.5. Load Vector
2.6. Dynamic Equation of the ACLD Pipeline
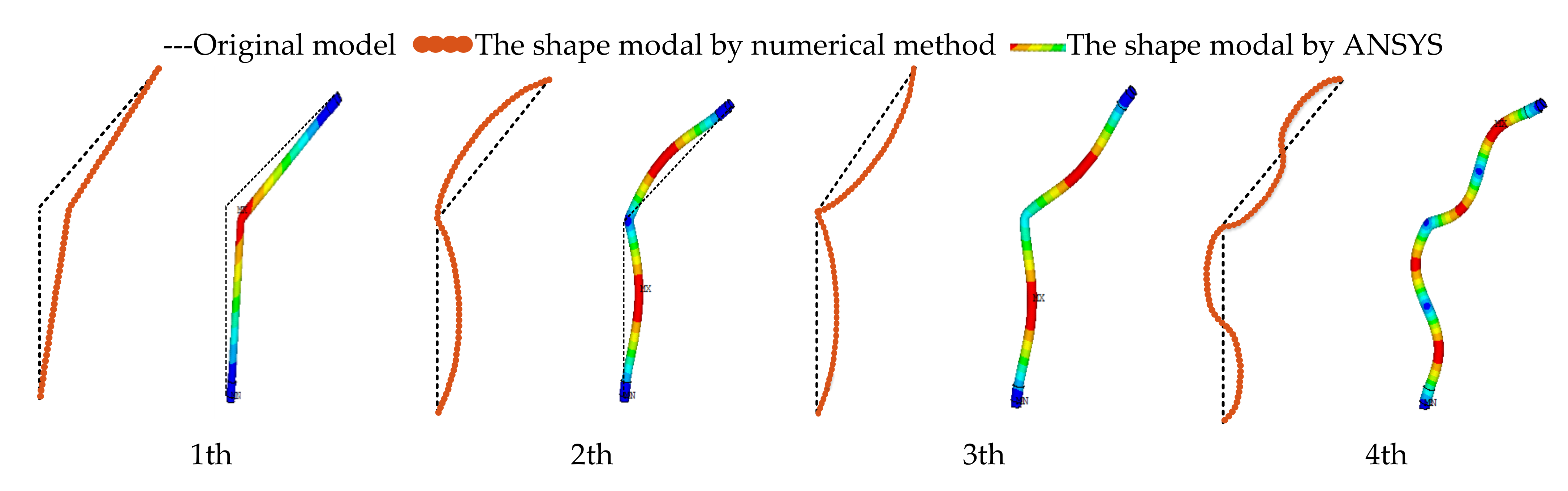
3. Validation
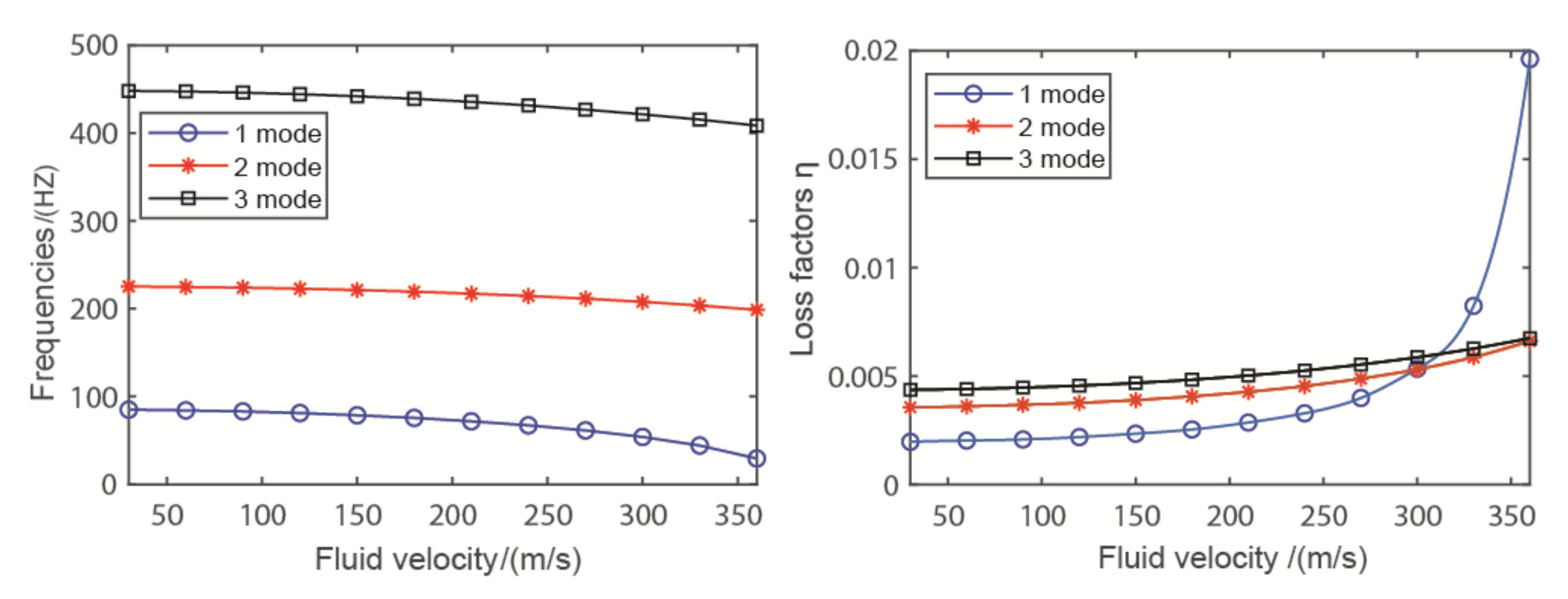
4. Parametric Study and Discussion
4.1. Subsection
4.2. The Influence of the Viscoelastic Layer Parameters
4.3. The Influence of the Piezoelectric Confinement Parameters
4.4. Influence of the Length of ACLD Patch (x2)
4.5. Influence of Position of the ACLD Patch (x1)
4.6. Influence of the Angle (θ) of Pipeline
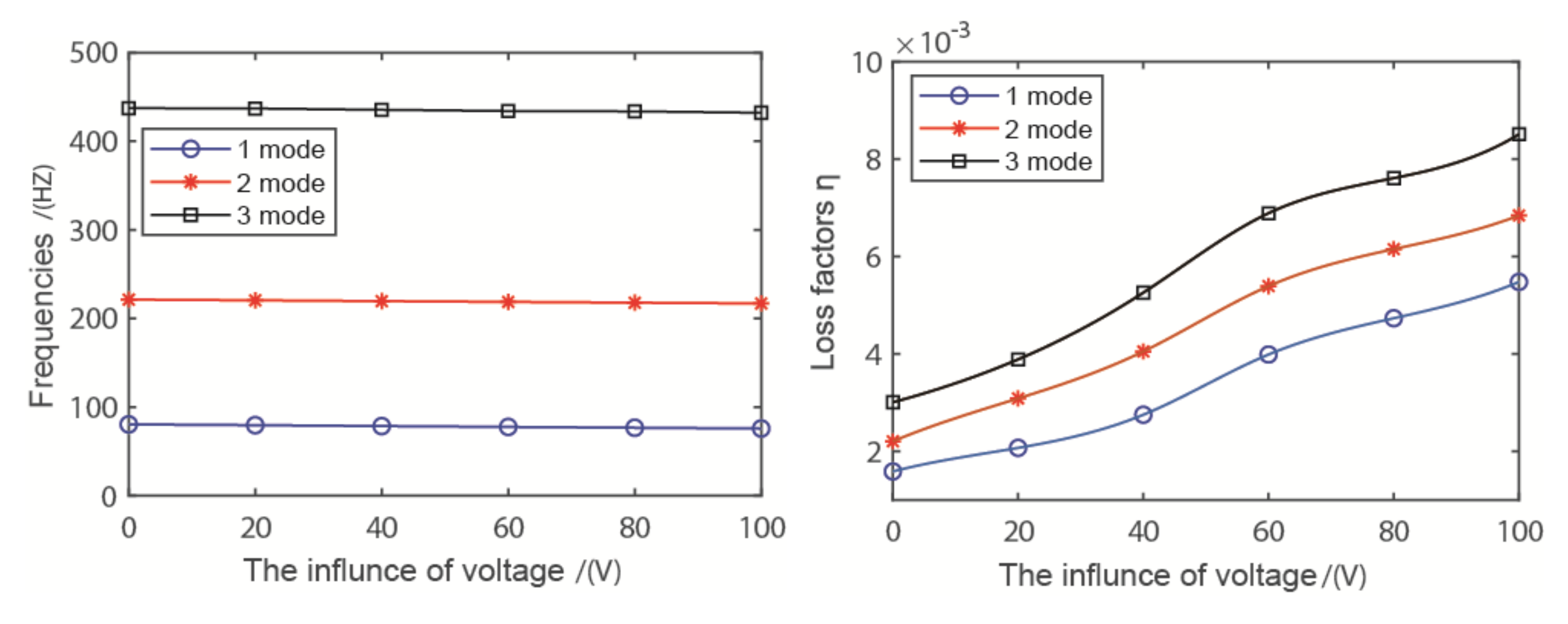
4.7. The Influence of the Voltage
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, P.; Yu, T.; Zhang, Y.; Wang, J.; Zhai, J. Vibration analysis and control technologies of hydraulic pipeline system in aircraft: A review. Chin. J. Aeronaut. 2020, 34, 83–114. [Google Scholar] [CrossRef]
- Gao, P.; Li, J.; Zhai, J.; Tao, Y.; Han, Q. A novel optimization layout method for clamps in a pipeline system. Appl. Sci. 2020, 10, 390. [Google Scholar] [CrossRef] [Green Version]
- Zhai, H.B.; Li, B.H.; Jiang, Z.F.; Yue, Z.F. Supports’ dynamical optimized design for the external pipeline of aircraft engine. Adv. Mater. Res. 2010, 139–141, 2456–2459. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, W. Maximization of structural natural frequency with optimal support layout. Struct. Multidiscip. Optim. 2006, 31, 462–469. [Google Scholar] [CrossRef]
- Xin, L.; Wang, S.; Pan, Z.; Ran, L. Simulation and optimized layout analysis of hydraulic power system in aircraft. In Proceedings of the International Conference on Fluid Power & Mechatronics, Beijing, China, 17–20 August 2011; pp. 93–97. [Google Scholar]
- Gao, P.X.; Zhai, J.Y.; Qu, F.Z.; Han, Q.K. Vibration and damping analysis of aerospace pipeline conveying fluid with constrained layer damping treatment. Proc. Inst. Mech. Eng. 2018, 232, 1529–1541. [Google Scholar] [CrossRef]
- Yano, D.; Ishikawa, S.; Tanaka, K.; Kijimoto, S. Vibration analysis of viscoelastic damping material attached to a cylindrical pipe by added mass and added damping. J. Sound Vib. 2019, 454, 14–31. [Google Scholar] [CrossRef]
- Ishikawa, S.; Tanaka, K.; Yano, D.; Kijimoto, S. Design of a disc-shaped viscoelastic damping material attached to a cylindrical pipe as a dynamic absorber or Houde damper. J. Sound Vib. 2020, 475, 115272. [Google Scholar] [CrossRef]
- Ulanov, A.; Bezborodov, S. Wearing of MR Wire Vibration Insulation Material Under Random Load. In Proceedings of the International Conference on Industrial Engineering, Moscow, Russia, May 2018; pp. 845–852. [Google Scholar]
- Ulanov, A.M.; Bezborodov, S.A. Life-time of vibration insulators made of metal rubber material under random load. Res. J. Appl. Ences 2014, 9, 664–668. [Google Scholar] [CrossRef]
- Panda, S.; Kumar, A. A design of active constrained layer damping treatment for vibration control of circular cylindrical shell structure. J Vib Control 2018, 24, 5811–5841. [Google Scholar] [CrossRef]
- Guo, Y.; Li, L.; Zhang, D. Dynamic modeling and vibration analysis of rotating beams with active constrained layer damping treatment in temperature field. Compos. Struct. 2019, 226, 111217. [Google Scholar] [CrossRef]
- Li, L.; Liao, W.H.; Zhang, D.; Guo, Y. Vibration analysis of a free moving thin plate with fully covered active constrained layer damping treatment. Compos. Struct. 2019, 235, 111742. [Google Scholar] [CrossRef]
- Lee, J.T. Active structural acoustics control of beams using active constrained layer damping through loss factor maximization. J. Sound Vib. 2005, 287, 481–503. [Google Scholar] [CrossRef]
- Zheng, H.; Pau, G.; Wang, Y.Y. A comparative study on optimization of constrained layer damping treatment for structural vibration control. Thin-Walled Struct. 2006, 44, 886–896. [Google Scholar] [CrossRef]
- Lee, D.-H. Optimal placement of constrained-layer damping for reduction of interior noise. AIAA J. 2008, 46, 75–83. [Google Scholar] [CrossRef]
- Trindade, M.A.; Benjeddou, A.; Ohayon, R. Modeling of frequency-dependent viscoelastic materials for active-passive vibration damping. J. Vib. Acoust. 2000, 122, 169–174. [Google Scholar] [CrossRef]
- Chen, L.H.; Huang, S.C. Vibrations of a cylindrical shell with partially constrained layer damping (CLD) treatment. Int. J. Mech. Sci. 1999, 41, 1485–1498. [Google Scholar] [CrossRef]
- Zhai, J.; Li, J.; Wei, D.; Gao, P.; Han, Q. Vibration control of an aero pipeline system with active constraint layer damping treatment. Appl. Sci. 2019, 9, 2094. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Liu, X.; Rong, W.; Gao, P.; Yu, T.; Han, H.; Xu, L. Vibration and damping analysis of pipeline system based on partially piezoelectric active constrained layer damping treatment. Materials 2021, 14, 1209. [Google Scholar] [CrossRef]
- Lin, Y.H.; Tsai, Y.K. Non-linear active vibration control of a cantilever pipe conveying fluid. J. Sound Vib. 1997, 202, 477–490. [Google Scholar] [CrossRef]
- Fong, K.S.; Yassin, A.M.; Abdulbari, H.A. Fluid-Structure Interaction (FSI) of damped oil conveying pipeline system by finite relement method. MATEC Web Conf. 2017, 111, 01005. [Google Scholar] [CrossRef] [Green Version]
- Cravero, C.; Marsano, D. Numerical prediction of tonal noise in centrifugal blowers. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Oslo, Norway, 11–15 June 2018; p. V001T009A001. [Google Scholar]
- Velarde, S.; Tajadura, R. Numerical simulation of the aerodynamic tonal noise generation in a backward-curved blades centrifugal fan. J. Sound Vib 2006, 295, 781–786. [Google Scholar]
- Gao, P.-x.; Zhai, J.-y.; Han, Q.-k. Dynamic response analysis of aero hydraulic pipeline system under pump fluid pressure fluctuation. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 1585–1595. [Google Scholar] [CrossRef]
- Lee, S.H.; Jeong, W.B. An efficient method to predict steady-state vibration of three-dimensional piping system conveying a pulsating fluid. J. Mech. Sci. Technol. 2012, 26, 2659–2667. [Google Scholar] [CrossRef]
- Sreejith, B.; Jayaraj, K.; Ganesan, N.; Padmanabhan, C.; Chellapandi, P.; Selvaraj, P. Finite element analysis of fluid–structure interaction in pipeline systems—ScienceDirect. Nucl. Eng. Des. 2004, 227, 313–322. [Google Scholar] [CrossRef]
- Ulanov, A.M.; Bezborodov, S.A. Research of stress-strained state of pipelines bundle with damping support made of MR material. Procedia Eng. 2017, 206, 3–8. [Google Scholar] [CrossRef]
- Chai, Q.; Zeng, J.; Ma, H.; Li, K.; Han, Q. A dynamic modeling approach for nonlinear vibration analysis of the L-type pipeline system with clamps. Chin. J. Aeronaut. 2020, 33, 3253–3265. [Google Scholar] [CrossRef]
- Gao, P.-x.; Zhai, J.-y.; Yan, Y.-y.; Han, Q.-k.; Qu, F.-z.; Chen, X.-h. A model reduction approach for the vibration analysis of hydraulic pipeline system in aircraft. Aerosp. Sci. Technol. 2016, 49, 144–153. [Google Scholar] [CrossRef]



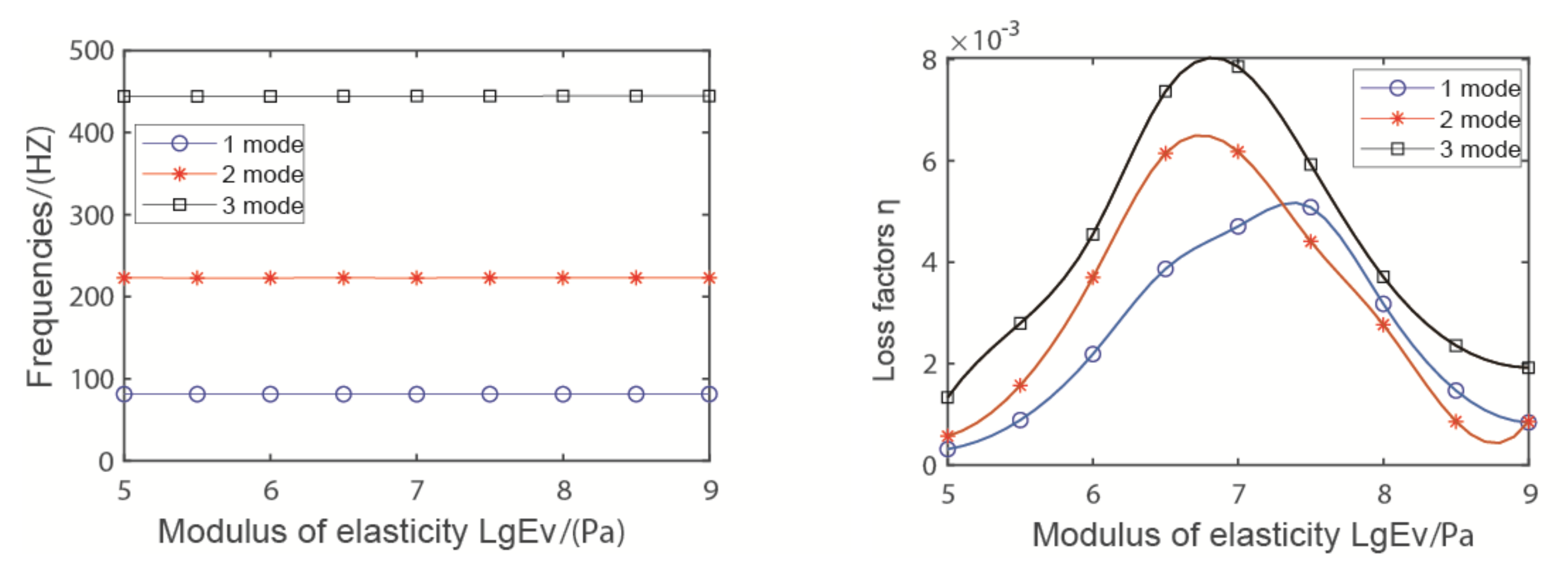

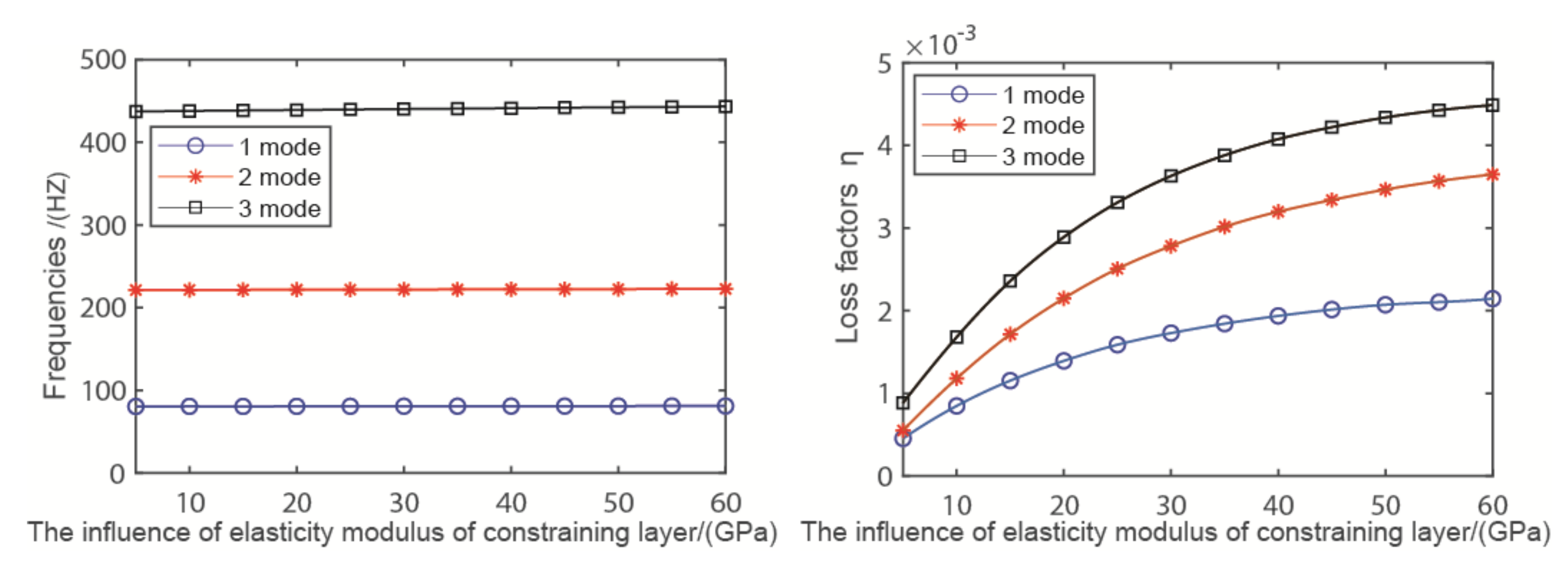
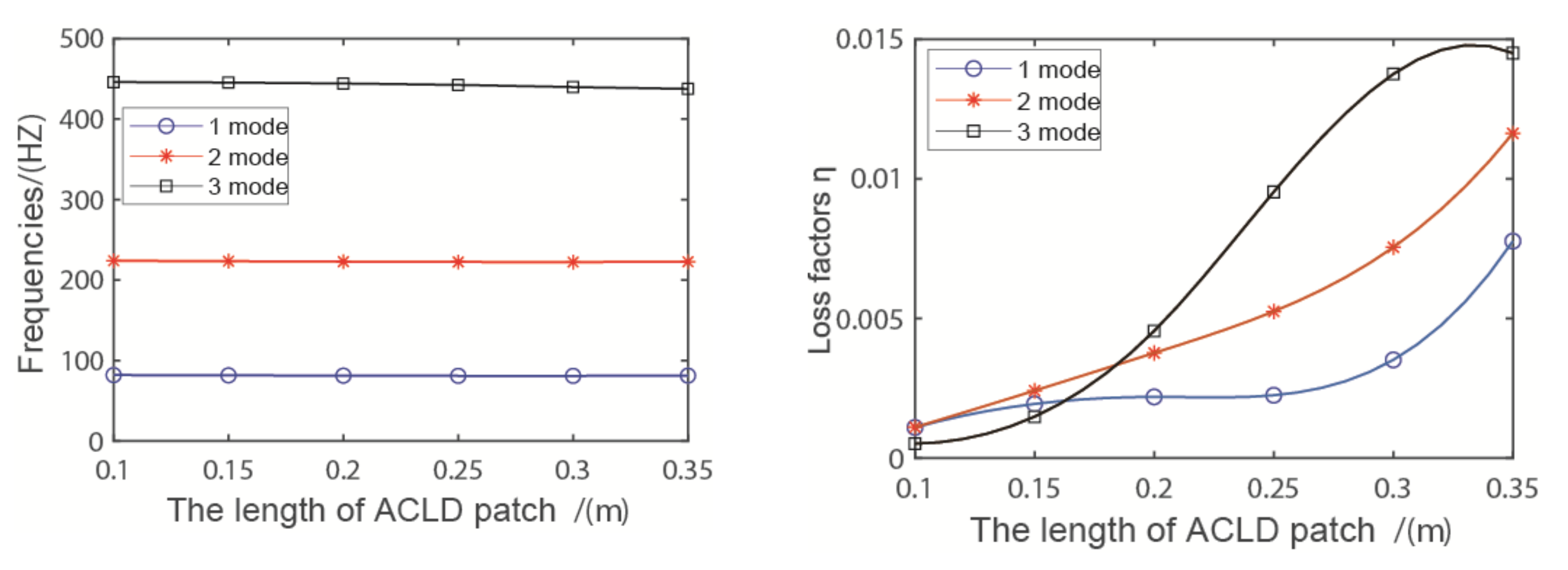

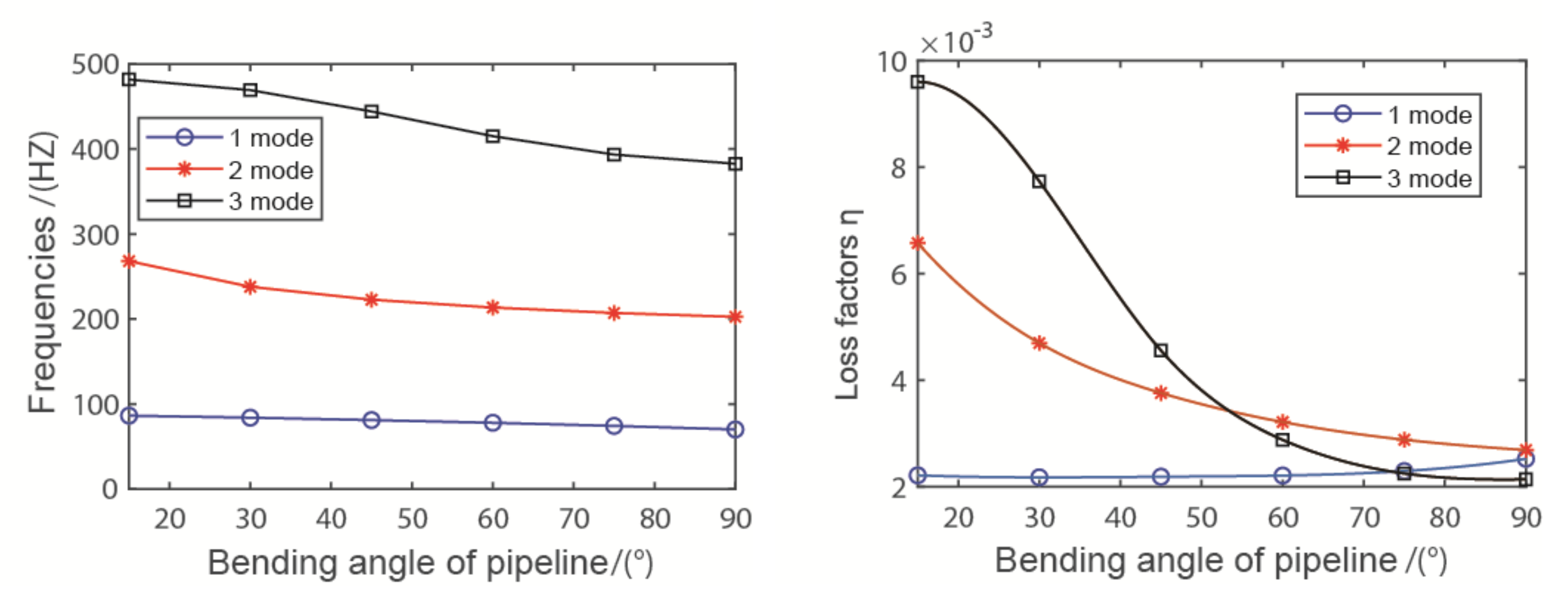
| Quantities | Base Pipeline | Viscoelastic Layer | Constraining Layer |
|---|---|---|---|
| Elastic modulus (GPa) | 201 | – | 70 |
| Shear modulus (MPa) | – | 1 | – |
| Density (kg/m3) | 7850 | 1580 | 2800 |
| Thickness (mm) | 2 | 0.5 | 0.5 |
| Poisson ratio | 0.3 | 0.498 | 0.3 |
| Loss factor | – | 0.29 | – |
| Pipeline outer diameter = 18 mm; the length of Part1 = 500 mm; the length of Part2 = 500 mm | |||
| Mode | The Results by Numerical Method | The Results by ANSYS | ||
|---|---|---|---|---|
| Modal Frequency (HZ) | Loss Factor | Modal Frequency (HZ) | Loss Factor | |
| Mode 1 | 81.1697 | 0.0022 | 82.3188 | 0.0021 |
| Mode 2 | 222.8073 | 0.0038 | 222.5072 | 0.0036 |
| Mode 3 | 444.2120 | 0.0046 | 443.9939 | 0.0046 |
| Mode 4 | 896.3123 | 0.0109 | 897.1242 | 0.0108 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Gao, P.; Liu, X.; Yu, T.; Huang, Z. Fluid-Induced Vibration of a Hydraulic Pipeline with Piezoelectric Active Constrained Layer-Damping Materials. Coatings 2021, 11, 757. https://doi.org/10.3390/coatings11070757
Zhang Y, Gao P, Liu X, Yu T, Huang Z. Fluid-Induced Vibration of a Hydraulic Pipeline with Piezoelectric Active Constrained Layer-Damping Materials. Coatings. 2021; 11(7):757. https://doi.org/10.3390/coatings11070757
Chicago/Turabian StyleZhang, Yuanlin, Peixin Gao, Xuefeng Liu, Tao Yu, and Zhaohua Huang. 2021. "Fluid-Induced Vibration of a Hydraulic Pipeline with Piezoelectric Active Constrained Layer-Damping Materials" Coatings 11, no. 7: 757. https://doi.org/10.3390/coatings11070757
APA StyleZhang, Y., Gao, P., Liu, X., Yu, T., & Huang, Z. (2021). Fluid-Induced Vibration of a Hydraulic Pipeline with Piezoelectric Active Constrained Layer-Damping Materials. Coatings, 11(7), 757. https://doi.org/10.3390/coatings11070757






