Modeling of Paper-Based Bi-Material Cantilever Actuator for Microfluidic Biosensors
Abstract
1. Introduction
2. Materials and Methods
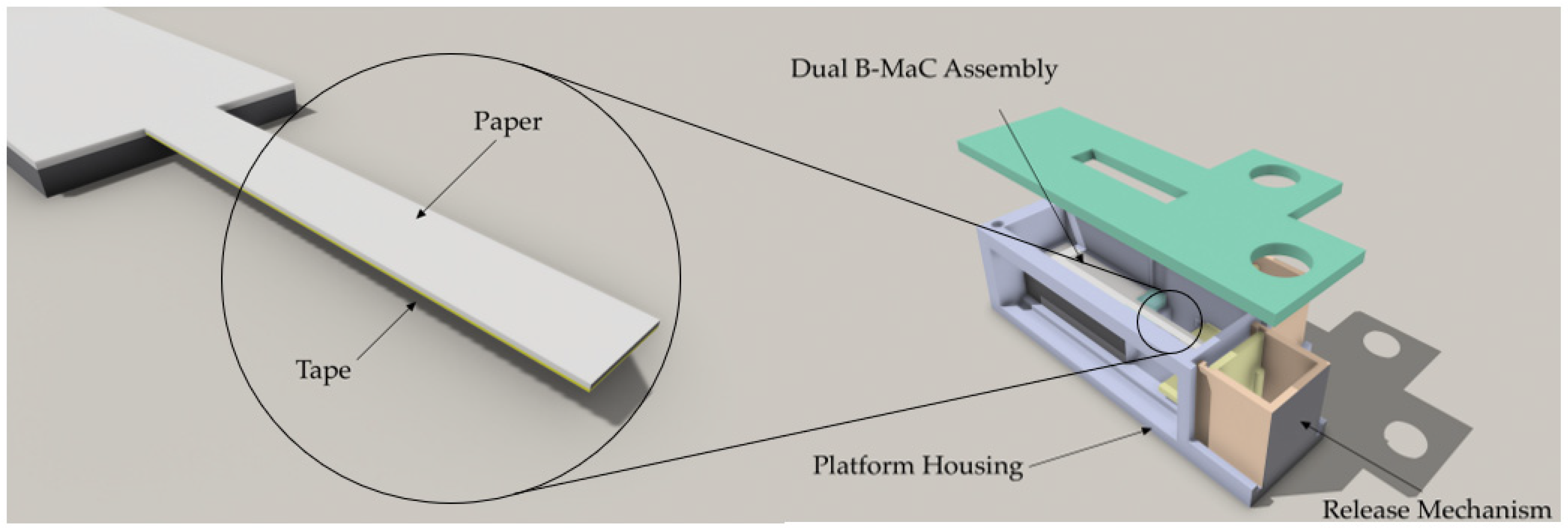
2.1. Materials
Experiment Flow
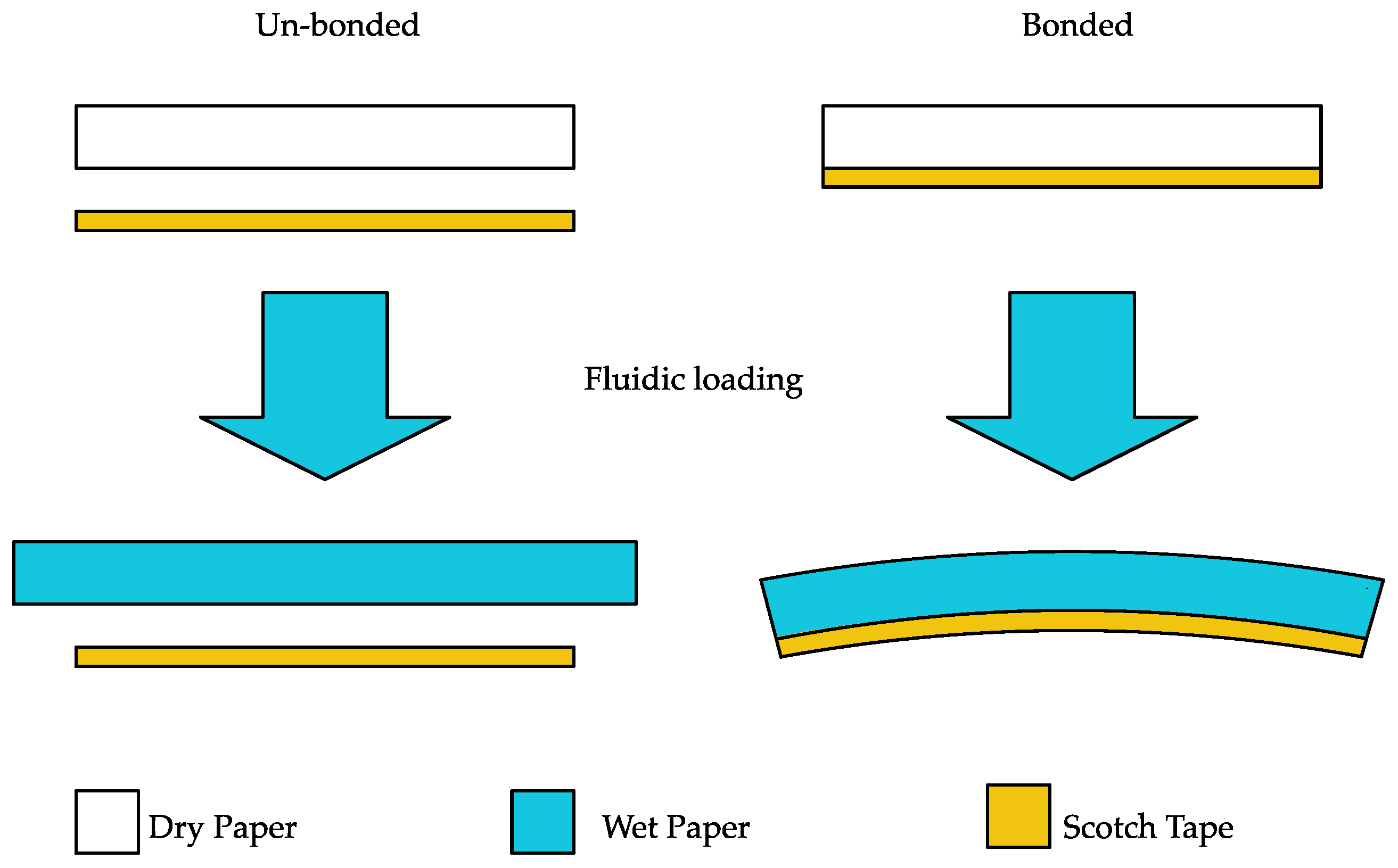
2.2. Modeling—Bimaterial Cantilever (B-MaC)
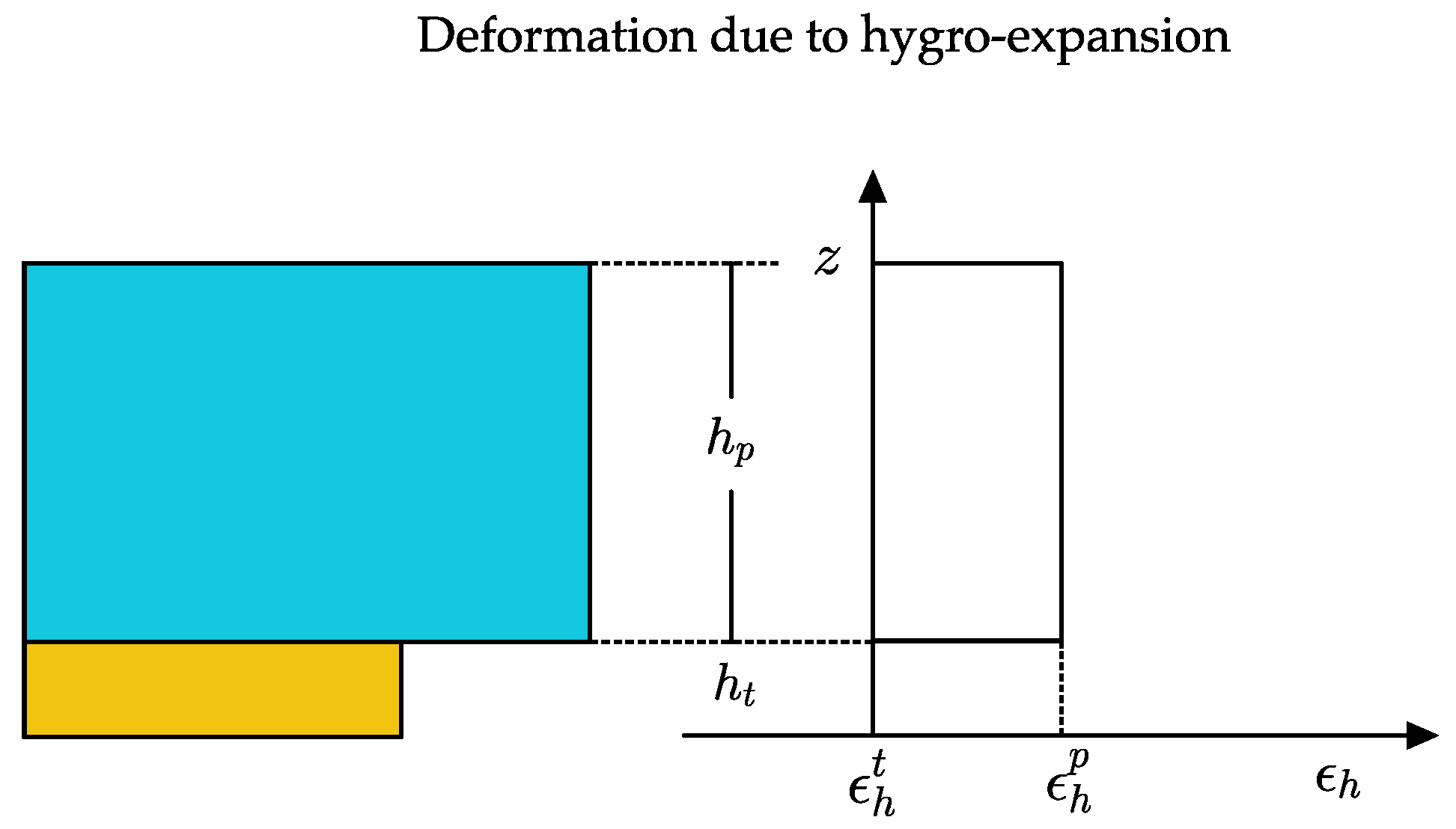
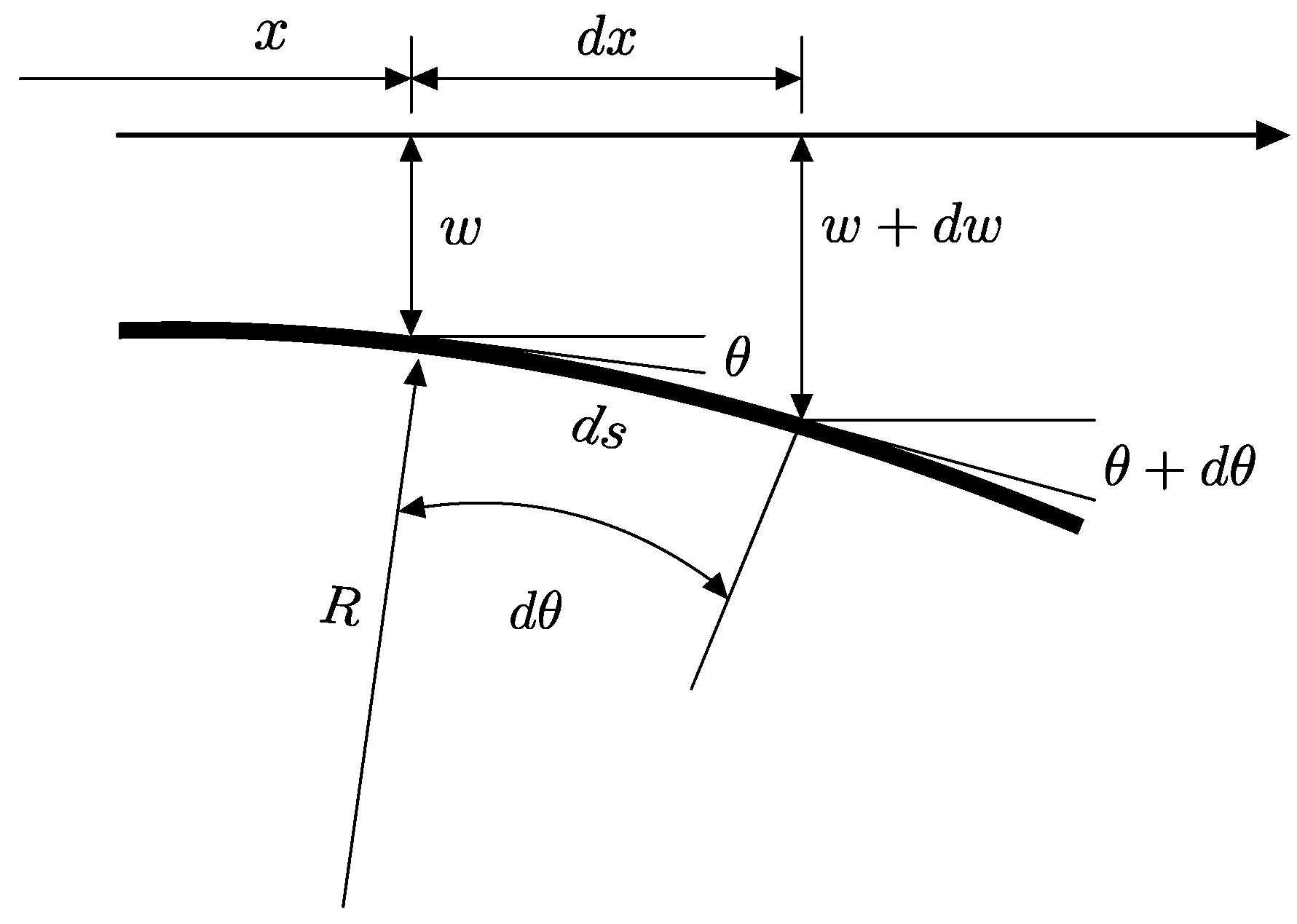
2.2.1. Strain in B-MaC
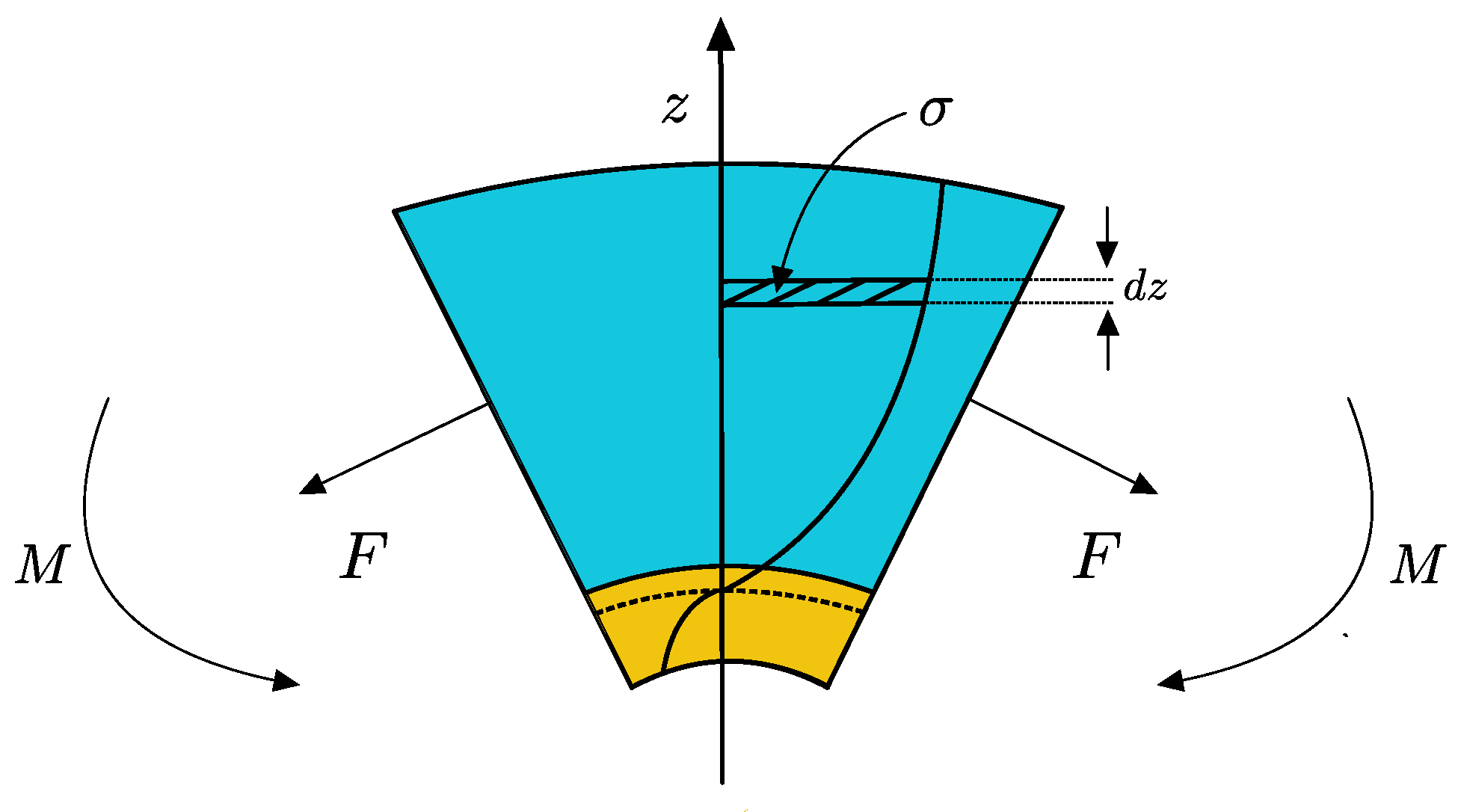
2.2.2. Stress of B-MaC
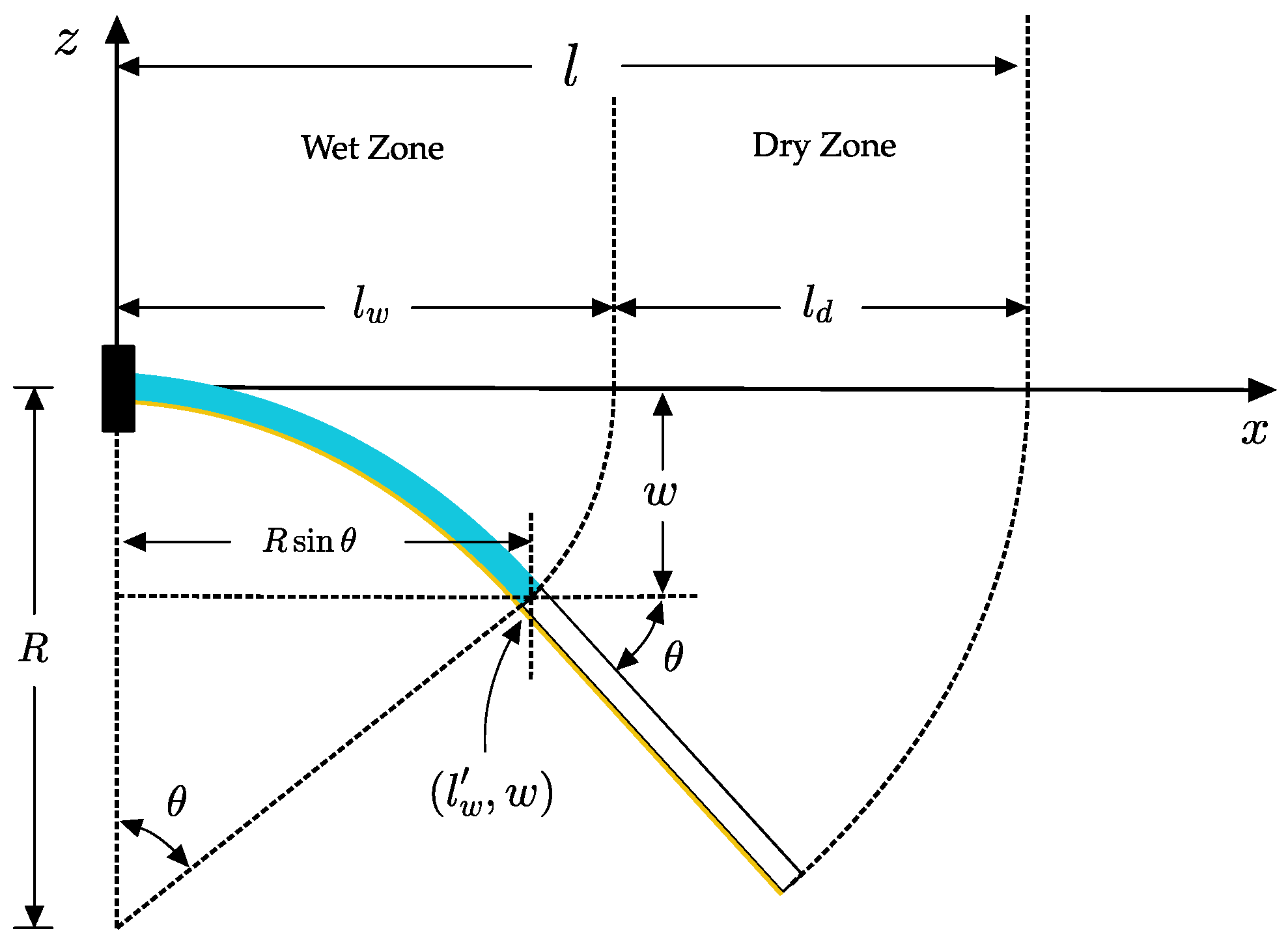
2.2.3. Fluid Flow in B-MaC
2.2.4. Material Properties of B-MaC
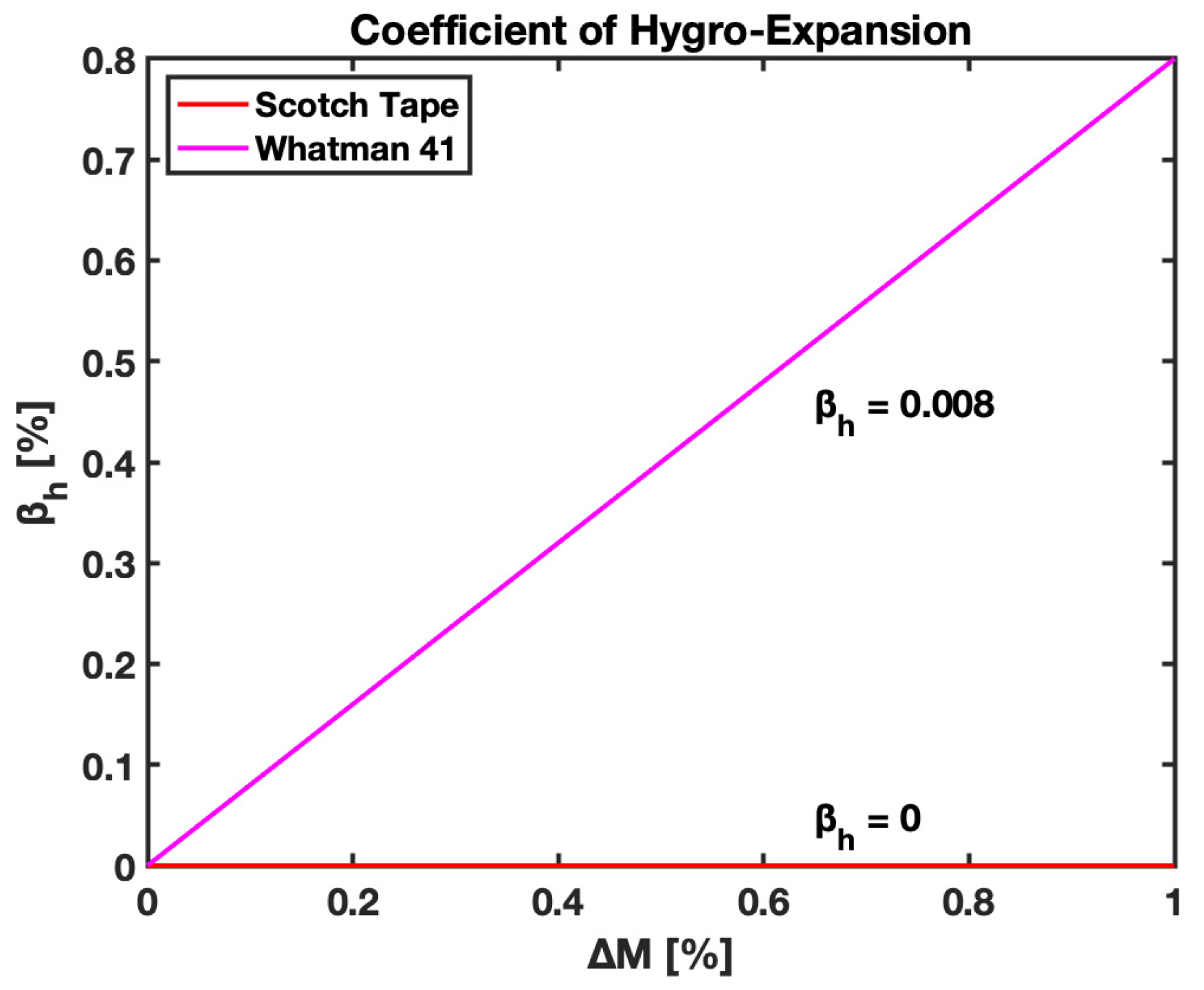
2.2.5. Linear Coefficient of Hygroexpansion
2.3. Modeling of B-MaC
- Boundary conditions:
3. Results
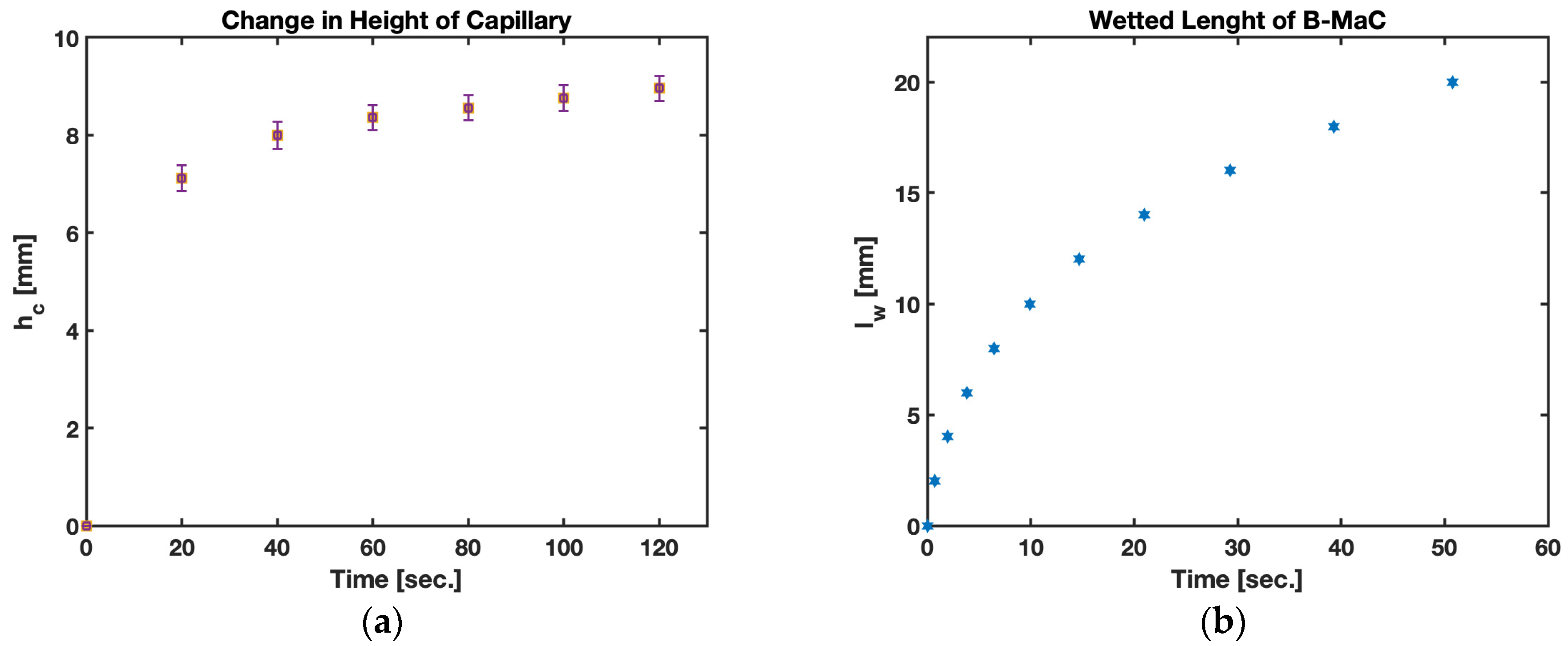
3.1. Fluid Flow in B-MaC
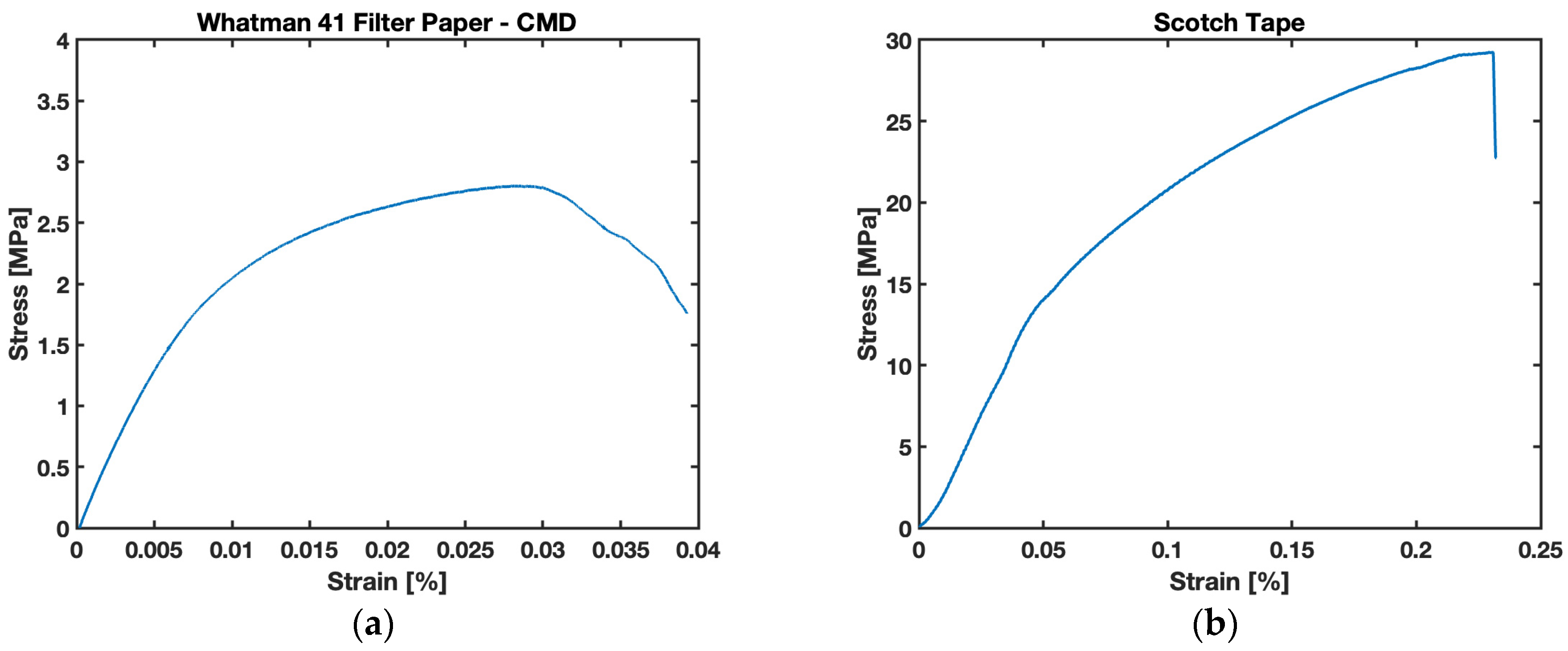
3.2. Young’s Modulus of B-MaC
3.3. Stress and Strain in B-MaC
3.4. Linear Coefficient of Hygroexpansion Paper
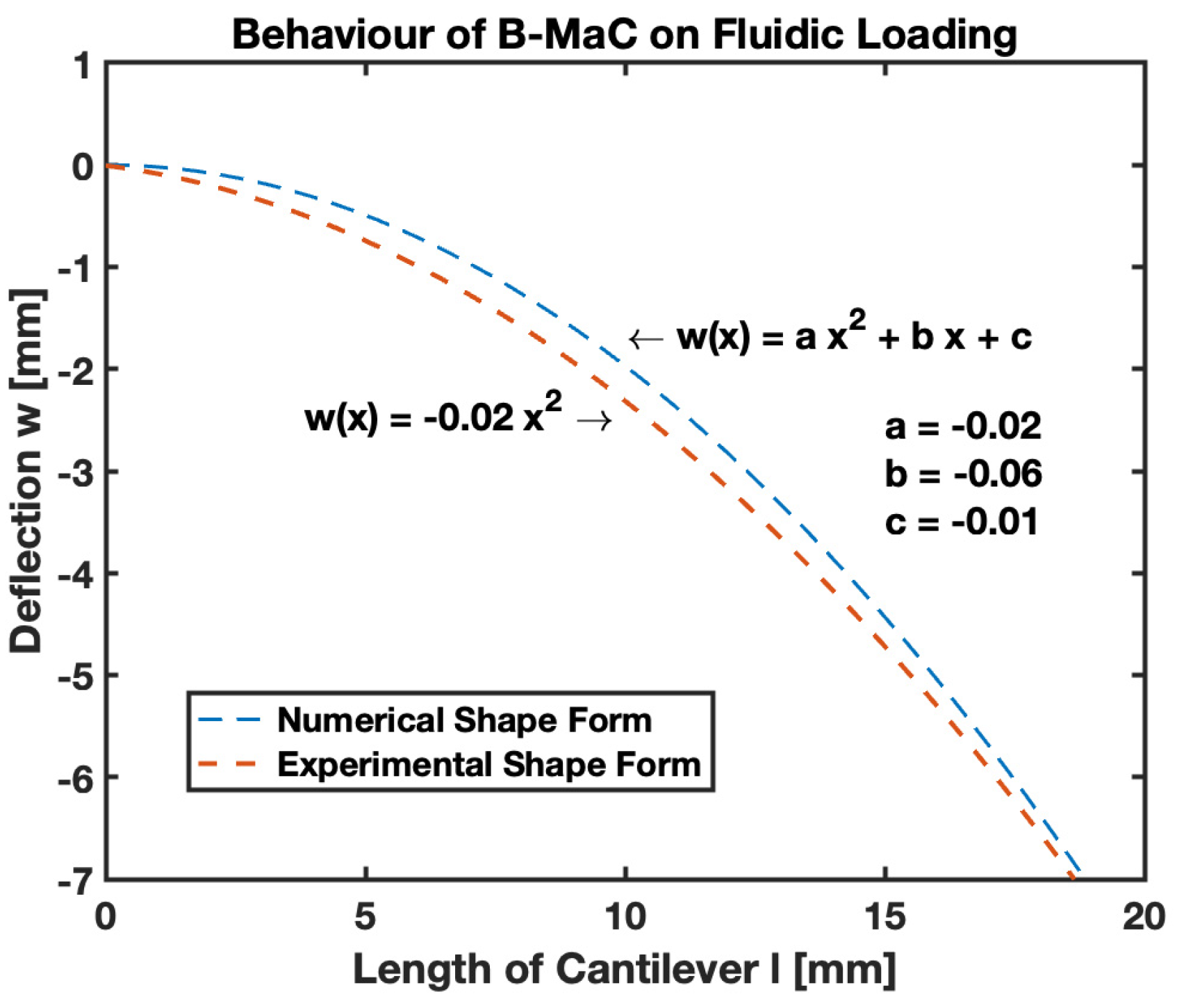
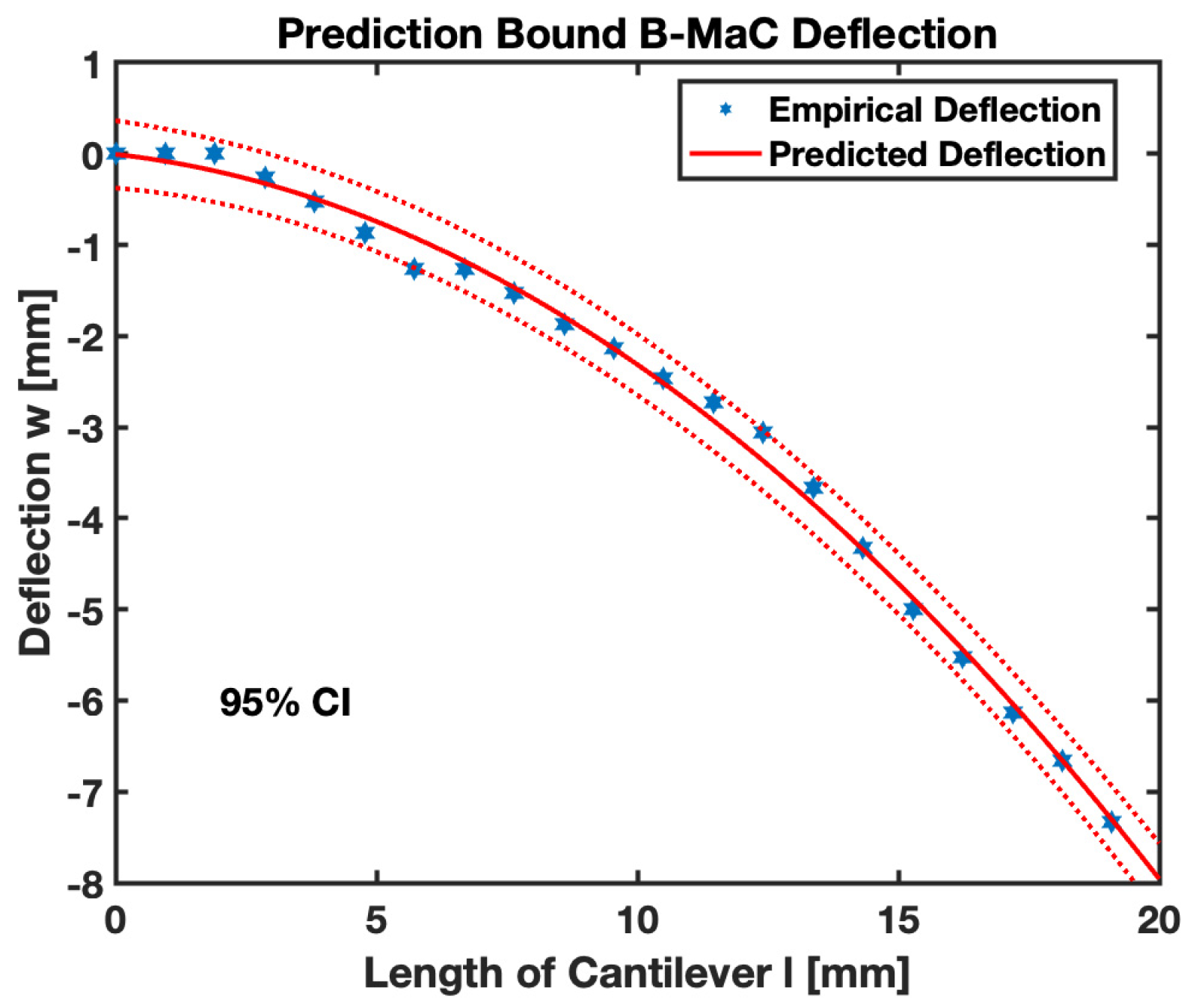
3.5. Deflection of B-MaC
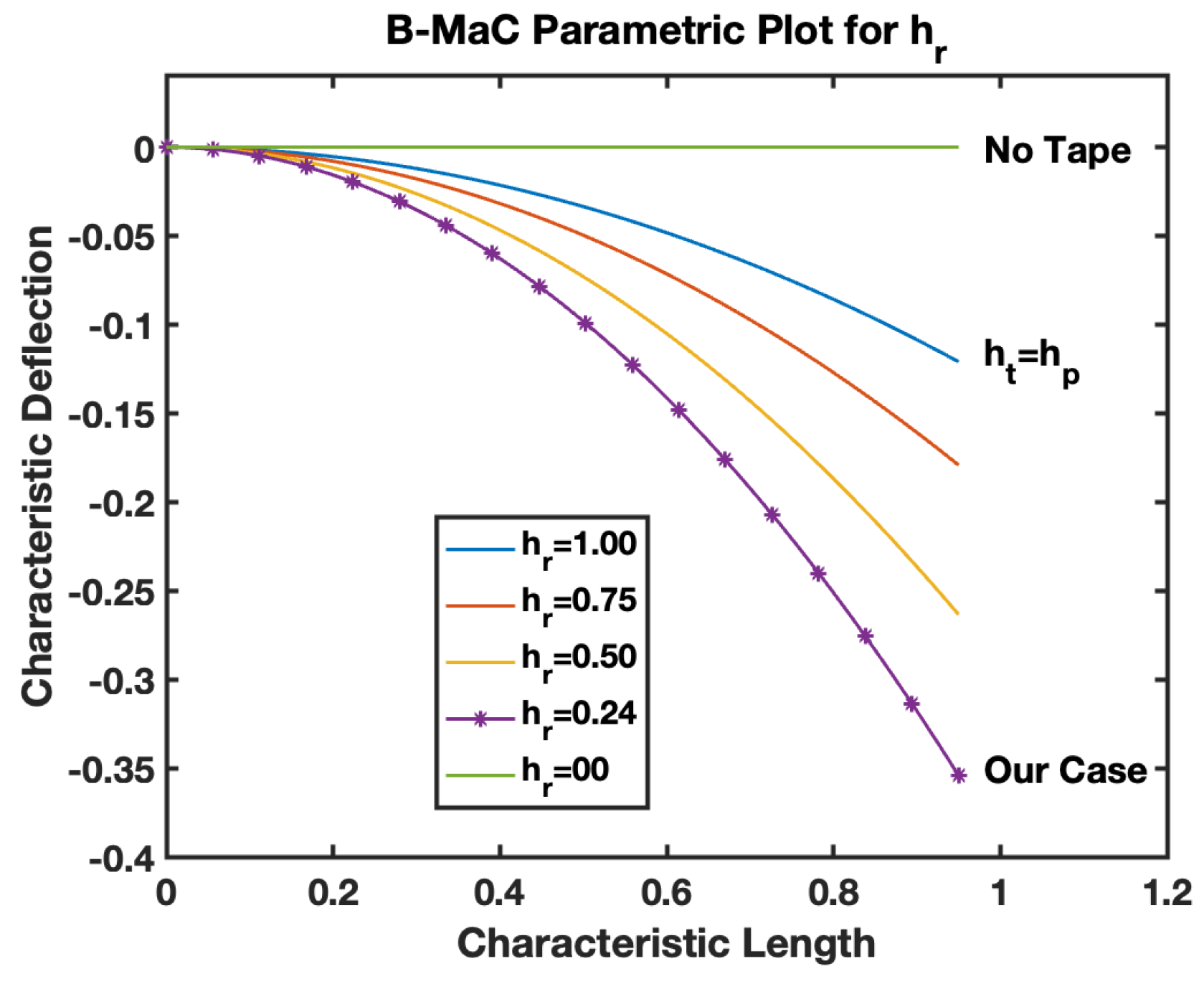
3.6. Parametric Models
4. Discussion
4.1. Fluid Flow in B-MaC
4.2. Young’s Modulus of B-MaC
4.3. Stress and Strain in B-MaC
4.4. Linear Coefficient of Hygroexpansion
4.5. Deflection of B-MaC
4.6. Parametric Models
4.7. Limitation of Model
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- From Figure 10:
- Radius of curvature:
- Substituting Equation (A3) in (A2), we obtain
- For small , :
References
- Kumar, A.; Heidari-Bafroui, H.; Charbaji, A.; Rahmani, N.; Anagnostopoulos, C.; Faghri, M. Numerical and Experimental Modeling of Paper-Based Actuators. Chem. Proc. 2021, 5, 15. [Google Scholar] [CrossRef]
- Binnig, G.; Quate, C.F.; Gerber, C. Atomic force Microscope. Phys. Rev. Lett. 1986, 56, 930–933. [Google Scholar] [CrossRef] [PubMed]
- Heidari-Bafroui, H.; Kumar, A.; Charbaji, A.; Smith, W.; Rahmani, N.; Anagnostopoulos, C.; Faghri, M. A Parametric Study on a Paper-Based Bi-Material Cantilever Valve. Micromachines 2022, 13, 1502. [Google Scholar] [CrossRef] [PubMed]
- Calleja, M.; Kosaka, P.M.; Paulo, A.S.; Tamayo, J. Challenges for nanomechanical sensors in biological detection. Nanoscale 2012, 4, 4925–4938. [Google Scholar] [CrossRef] [PubMed]
- Xu, R.; Lee, J.W.; Pan, T.; Ma, S.; Wang, J.; Han, J.H.; Ma, Y.; Rogers, J.A.; Huang, Y. Designing Thin, Ultrastretchable Electronics with Stacked Circuits and Elastomeric Encapsulation Materials. Adv. Funct. Mater. 2017, 27, 1604545. [Google Scholar] [CrossRef]
- Quirion, D.; Manna, M.; Hidalgo, S.; Pellegrini, G. Manufacturability and Stress Issues in 3D Silicon Detector Technology at IMB-CNM. Micromachines 2020, 11, 1126. [Google Scholar] [CrossRef]
- Zhang, L.; Pan, J.; Liu, Y.; Xu, Y.; Zhang, A. NIR–UV Responsive Actuator with Graphene Oxide/Microchannel-Induced Liquid Crystal Bilayer Structure for Biomimetic Devices. ACS Appl. Mater. Interfaces 2020, 12, 6727–6735. [Google Scholar] [CrossRef]
- Wang, L.; Wang, D.; Huang, S.; Guo, X.; Wan, G.; Fan, J.; Chen, Z. Controllable Shape Changing and Tristability of Bilayer Composite. ACS Appl. Mater. Interfaces 2019, 11, 16881–16887. [Google Scholar] [CrossRef]
- Morteza, A. Functional Nanomaterial Composites for Soft Sensing and Actuation. Ph.D. Thesis, ETH Zurich, Zurich, Switzerland, 2019. [Google Scholar] [CrossRef]
- Liu, F.; Alici, G.; Zhang, B.; Beirne, S.; Li, W. Fabrication and characterization of a magnetic micro-actuator based on deformable Fe-doped PDMS artificial cilium using 3D printing. Smart Mater. Struct. 2015, 24, 035015. [Google Scholar] [CrossRef]
- Makino, E.; Mineta, T.; Mitsunaga, T.; Kawashima, T.; Shibata, T. Sphincter actuator fabricated with PDMS/SMA bimorph cantilevers. Microelectron. Eng. 2011, 88, 2662–2665. [Google Scholar] [CrossRef]
- Maleki, T.; Chitnis, G.; Ziaie, B. A batch-fabricated laser-micromachined PDMS actuator with stamped carbon grease electrodes. J. Micromech. Microeng. 2011, 21, 027002. [Google Scholar] [CrossRef]
- Bhattacharjee, N.; Urrios, A.; Kanga, S.; Folch, A. The upcoming 3D- printing revolution in microfluidics. Lab Chip 2016, 16, 1720–1742. [Google Scholar] [CrossRef] [PubMed]
- Mirzaee, I.; Song, M.; Charmchi, M.; Sun, H. A microfluidics-based on-chip impinger for airborne particle collection. Lab Chip 2016, 16, 2254–2264. [Google Scholar] [CrossRef] [PubMed]
- Pandya, H.J.; Park, K.; Desai, J.P. Design, and fabrication of a flexible MEMS-based electro-mechanical sensor array for breast cancer diagnosis. J. Micromech. Microeng. 2015, 25, 13. [Google Scholar] [CrossRef]
- Gaitas, A.; Malhotra, R.; Pienta, K. A method to measure cellular adhesion utilizing a polymer micro-cantilever. Appl. Phys. Lett. 2013, 103, 4. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.Y.H.; Nandra, M.; Tai, Y.C. IEEE, Cantilever Actuated by Piezoelectric Parylene-C. In Proceedings of the 25th IEEE International Conference on Micro Electromechanical Systems (MEMS), Paris, France, 29 January–2 February 2012. [Google Scholar] [CrossRef]
- Shang, Y.F.; Ye, X.Y.; Feng, J.Y.; Zhou, H.Y.; Wang, Y. Fabrication and Characterization of a Polymer/Metal Bimorph Microcantilever for Ultrasensitive Thermal Sensing. IEEE Sens. J. 2014, 14, 1304–1312. [Google Scholar] [CrossRef]
- Li, X.; Tian, J.F.; Nguyen, T.; Shen, W. Paper-Based Microfluidic Devices by Plasma Treatment. Anal. Chem. 2008, 80, 9131–9134. [Google Scholar] [CrossRef]
- Jahanshahi-Anbuhi, S.; Chavan, P.; Sicard, C.; Leung, V.; Hossain, S.M.Z.; Pelton, R.; Brennan, J.D.; Filipe, C.D. Creating fast flow channels in paper fluidic devices to control the timing of sequential reactions. Lab Chip 2012, 12, 5079–5085. [Google Scholar] [CrossRef]
- Han, K.N.; Choi, J.-S.; Kwon, J. Three-dimensional paper-based slip device for one-step point-of-care testing. Sci. Rep. 2016, 6, 25710. [Google Scholar] [CrossRef]
- Martinez, A.W.; Phillips, S.T.; Nie, Z.; Cheng, C.-M.; Carrilho, E.; Wiley, B.; Whitesides, G.M. Programmable diagnostic devices made from paper and tape. Lab Chip 2010, 10, 2499–2504. [Google Scholar] [CrossRef]
- Rodriguez, N.M.; Wong, W.S.; Liu, L.; Dewar, R.; Klapperich, C.M. A fully integrated paper fluidic molecular diagnostic chip for the extraction, amplification, and detection of nucleic acids from clinical samples. Lab Chip 2016, 16, 753–763. [Google Scholar] [CrossRef] [PubMed]
- Jayawardane, B.M.; Wei, S.; McKelvie, I.D.; Kolev, S. Microfluidic Paper-Based Analytical Device for the Determination of Nitrite and Nitrate. Anal. Chem. 2014, 86, 7274–7279. [Google Scholar] [CrossRef] [PubMed]
- Fu, H.; Song, P.; Wu, Q.; Zhao, C.; Pan, P.; Li, X.; Li-Jessen, N.Y.K.; Liu, X. A paper-based microfluidic platform with shape-memory-polymer-actuated fluid valves for automated multi-step immunoassays. Microsyst. Nanoeng. 2019, 5, 50. [Google Scholar] [CrossRef] [PubMed]
- Bowden, N.; Brittain, S.; Evans, A.G.; Hutchinson, J.W.; Whitesides, G.M. Spontaneous formation of ordered structures in thin films of metals supported on an elastomeric polymer. Nature 1998, 393, 146–149. [Google Scholar] [CrossRef]
- Efimenko, K.; Rackaitis, M.; Manias, E.; Vaziri, A.; Mahadevan, L.; Genzer, J. Nested self-similar wrinkling patterns in skins. Nat. Mater. 2005, 4, 293–297. [Google Scholar] [CrossRef]
- Huang, J.; Juszkiewicz, M.; de Jeu, W.H.; Cerda, E.; Emrick, T.; Menon, N.; Russell, T.P. Capillary wrinkling of floating thin polymer films. Science 2007, 317, 650–653. [Google Scholar] [CrossRef]
- Holmes, D.P.; Roche, M.; Sinha, T.; Stone, H.A. Bending and twisting of soft materials by non-homogenous swelling. Soft Matter 2011, 7, 5188–5193. [Google Scholar] [CrossRef]
- Siddique, J.I.; Anderson, D.M.; Bondarev, A. Capillary rise of a liquid into a deformable porous material. Phys. Fluids 2009, 21, 013106. [Google Scholar] [CrossRef]
- Ma, J.; Zhang, C.; Xi, F.; Chen, W.; Jiao, K.; Du, Q.; Bai, F.; Liu, Z. Experimental Study on the Influence of Environment Conditions on the Performance of Paper-Based Microfluidic Fuel Cell. SSRN Electron. J. 2022, 219, 119487. [Google Scholar] [CrossRef]
- Liu, Z.; Hu, J.; Zhao, Y.; Qu, Z.; Xu, F. Experimental and numerical studies on liquid wicking into filter papers for paper-based diagnostics. Appl. Therm. Eng. 2015, 88, 280–287. [Google Scholar] [CrossRef]
- Cheng, H.; Liu, J.; Zhao, Y.; Hu, C.; Zhang, Z.; Chen, N.; Jiang, L.; Qu, L. Graphene Fibers with Predetermined Deformation as Moisture-Triggered Actuators and Robots. Angew. Chem. Int. Ed. 2013, 52, 10482–10486. [Google Scholar] [CrossRef] [PubMed]
- Leppa, T.; Sorvari, J.; Erkkila, A.; Ha, J. Mathematical modeling of moisture induced out-of-plane deformation of a paper sheet. Model. Simul. Mater. Sci. Eng. 2005, 13, 841. [Google Scholar] [CrossRef]
- Madsen, B.; Hoffmeyer, P.; Lilholt, H. Hemp yarn reinforced composites-III. Moisture content and dimensional changes. Compos. Part A-Appl. Sci. Manuf. 2012, 43, 2151–2160. [Google Scholar] [CrossRef]
- Yao, Y.; Chen, X.; Guo, H.; Wu, Z.; Li, X. Humidity sensing behaviors of graphene oxide-silicon bi-layer flexible structure. Sens. Actuators B Chem. 2012, 161, 1053–1058. [Google Scholar] [CrossRef]
- Duigou, L.; Castro, M. Moisture-induced self-shaping flax-reinforced polypropylene biocomposite actuator. Ind. Crops Prod. 2015, 71, 1–6. [Google Scholar] [CrossRef]
- Mao, Z.; Yoshida, K.; Kim, J.W. A micro vertically-allocated SU-8 check valve and its characteristics. Microsyst. Technol. 2019, 25, 245–255. [Google Scholar] [CrossRef]
- Chen, G.; Kumar, A.; Heidari-Bafroui, H.; Smith, W.; Charbaji, A.; Rahmani, N.; Anagnostopoulos, C.; Faghri, M. Paper-Based Bi-Material Cantilever Actuator Bending Behavior and Modeling. Micromachines 2023, 14, 924. [Google Scholar] [CrossRef]
- Gendron, G.; Dano, M.L.; Cloutier, A. A numerical study of the hygro- mechanical deformation of two cardboard layups. Compos. Sci. Technol. 2004, 64, 619–627. [Google Scholar] [CrossRef]
- Dano, M.-L.; Bourque, J.-P. Deformation behavior of paper and board subjected to moisture diffusion. Int. J. Solids Struct. 2009, 46, 1305–1316. [Google Scholar] [CrossRef]
- Heidari-Bafroui, H.; Kumar, A.; Hahn, C.; Scholz, N.; Charbaji, A.; Rahmani, N.; Anagnostopoulos, C.; Faghri, M. Development of a New Lab-on-Paper Microfluidics Platform Using Bi-Material Cantilever Actuators for ELISA on Paper. Biosensors 2023, 13, 310. [Google Scholar] [CrossRef]
- Mäkelä, P.; Östlund, S. Orthotropic elastic-plastic material model for paper materials. Int. J. Solids Struct. 2003, 40, 5599–5620. [Google Scholar] [CrossRef]
- Washburn, E.W. The dynamics of capillary flow. Phys. Rev. 1921, 17, 273. [Google Scholar] [CrossRef]
- Lee, M.; Kim, S.; Kim, H.-Y.; Mahadevan, L. Bending and buckling of wet paper. Phys. Fluids 2016, 28, 042101. [Google Scholar] [CrossRef]















| Variables | Description | Dimension |
|---|---|---|
| l | Length of B-MaC | L |
| w | Deflection in y | L |
| u | Deformation in x | L |
| R | Bending radius | L |
| lw | Wetted length | L |
| b | B-MaC width | L |
| ht | Tape layer thickness | L |
| hp | Paper layer thickness | L |
| ts | Time required for saturation | T |
| Stress per unit length | ML−2T−2 | |
| Fh | Hygroexpansion force | MLT−2 |
| Mh | Bending moment due to actuation | ML2T−2 |
| Et | Young’s modulus of tape | ML−1T−2 |
| Ep | Young’s modulus of saturated paper | ML−1T−2 |
| Variables | Expression | Description |
|---|---|---|
| Lw | Characteristic Wetted Length | |
| W | Characteristic Deflection | |
| H | Characteristic Thickness | |
| T | Characteristic Time |
| Variables | Description | Value |
|---|---|---|
| Length of B-MaC | ||
| b | B-MaC width | |
| Tape layer height | ||
| Paper layer wetted height | ||
| Young’s modulus of tape | ||
| Young’s modulus of saturated paper | ||
| Hygroexpansion strain in paper |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kumar, A.; Heidari-Bafroui, H.; Rahmani, N.; Anagnostopoulos, C.; Faghri, M. Modeling of Paper-Based Bi-Material Cantilever Actuator for Microfluidic Biosensors. Biosensors 2023, 13, 580. https://doi.org/10.3390/bios13060580
Kumar A, Heidari-Bafroui H, Rahmani N, Anagnostopoulos C, Faghri M. Modeling of Paper-Based Bi-Material Cantilever Actuator for Microfluidic Biosensors. Biosensors. 2023; 13(6):580. https://doi.org/10.3390/bios13060580
Chicago/Turabian StyleKumar, Ashutosh, Hojat Heidari-Bafroui, Nassim Rahmani, Constantine Anagnostopoulos, and Mohammad Faghri. 2023. "Modeling of Paper-Based Bi-Material Cantilever Actuator for Microfluidic Biosensors" Biosensors 13, no. 6: 580. https://doi.org/10.3390/bios13060580
APA StyleKumar, A., Heidari-Bafroui, H., Rahmani, N., Anagnostopoulos, C., & Faghri, M. (2023). Modeling of Paper-Based Bi-Material Cantilever Actuator for Microfluidic Biosensors. Biosensors, 13(6), 580. https://doi.org/10.3390/bios13060580







