Flat-Band AC Transport in Nanowires
Abstract
1. Introduction
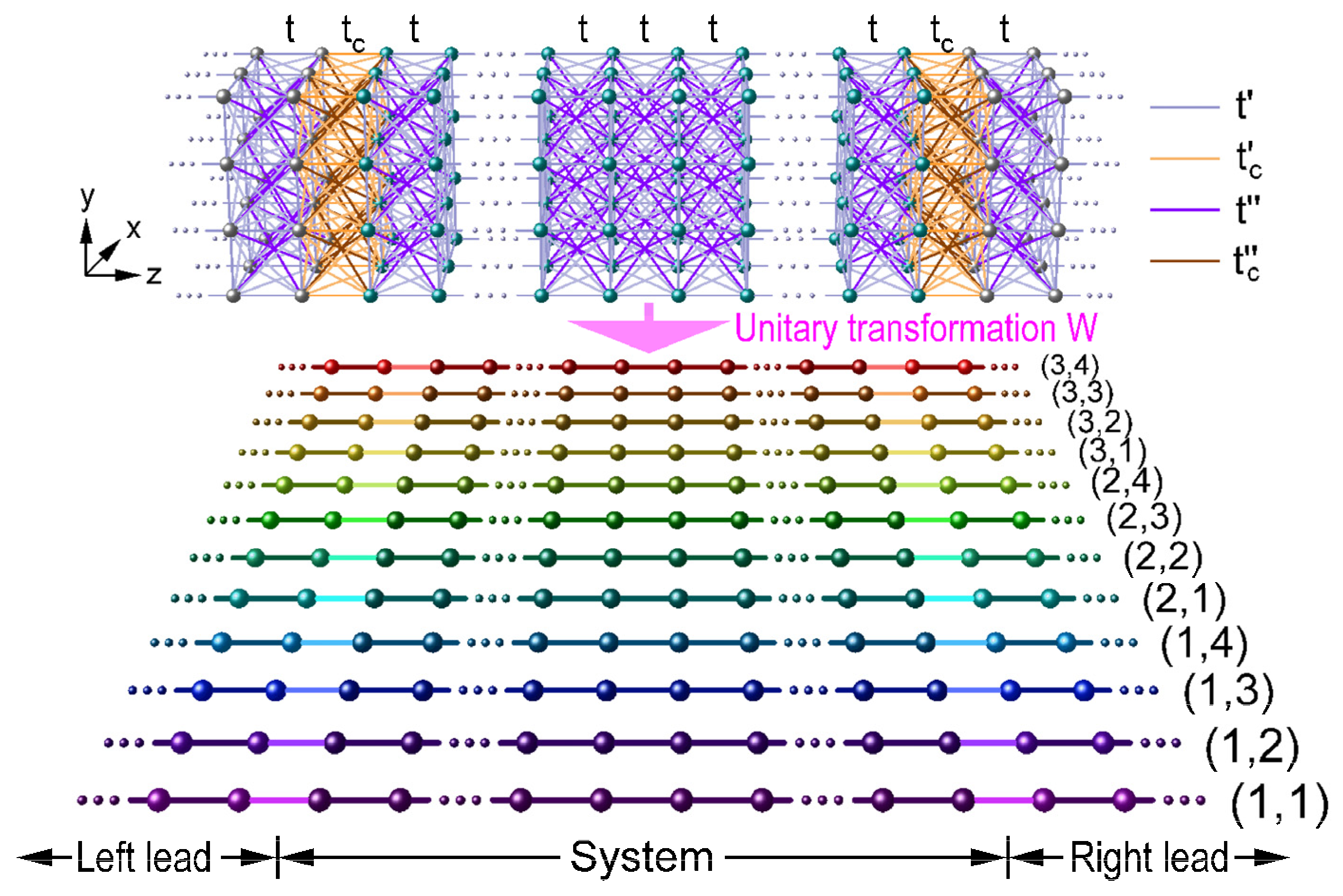
2. Real Space Modeling
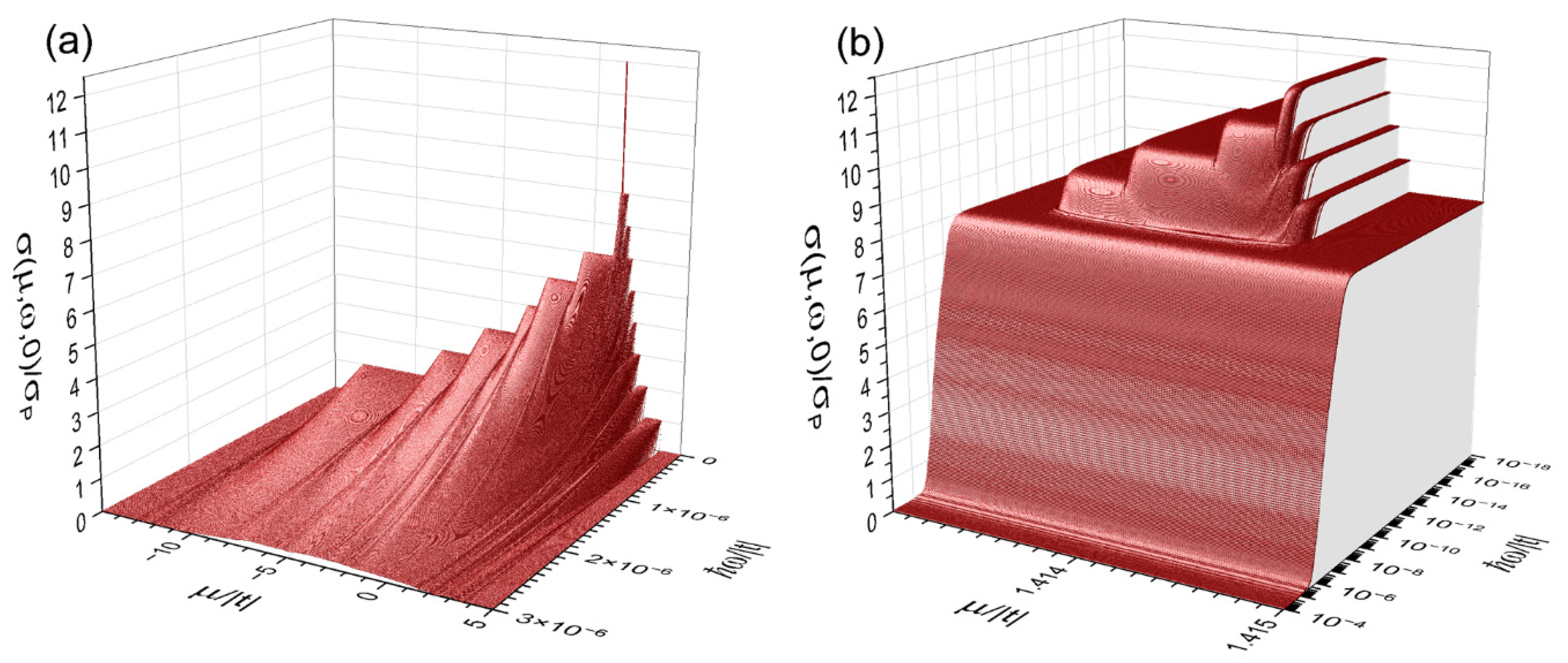
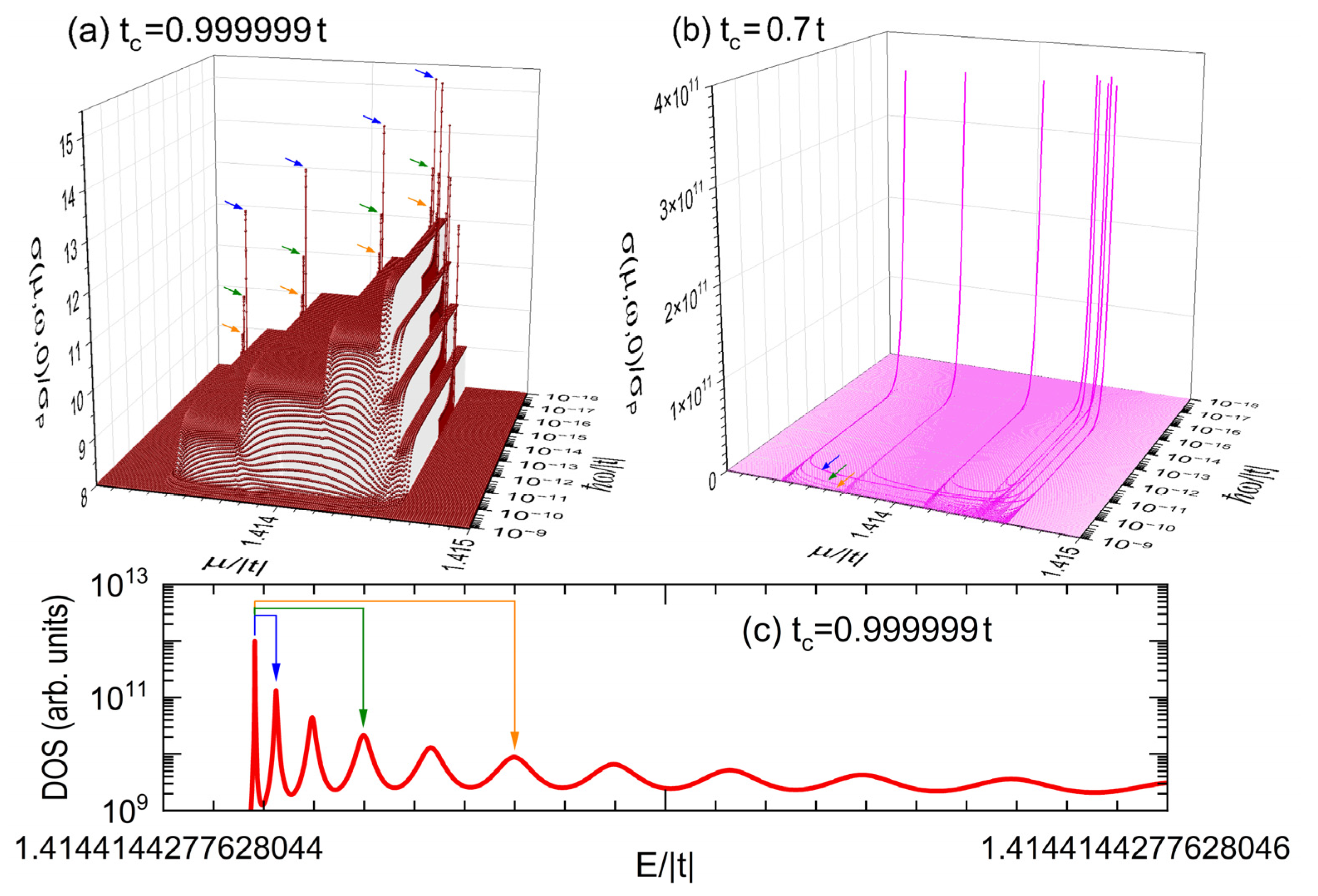
3. AC Conductivity
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Independent Channel Method for the Kubo–Greenwood Formula
References
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional superconductivity in magic-angle graphene superlattices. Nature 2018, 556, 43–50. [Google Scholar] [CrossRef] [PubMed]
- Tang, L.; Song, D.; Xia, S.; Xia, S.; Ma, J.; Yan, W.; Hu, Y.; Xu, J.; Leykam, D.; Chen, Z. Photonic flat-band lattices and unconventional light localization. Nanophotonics 2020, 9, 1161–1176. [Google Scholar] [CrossRef]
- Kumar, A.; Tan, Y.J.; Navaratna, N.; Gupta, M.; Pitchappa, P.; Singh, R. Slow light topological photonics with counter-propagating waves and its active control on a chip. Nat. Commun. 2024, 15, 926. [Google Scholar] [CrossRef]
- Yang, J.; Li, Y.; Yang, Y.; Xie, X.; Zhang, Z.; Yuan, J.; Cai, H.; Wang, D.-W.; Gao, F. Realization of all-band-flat photonic lattices. Nat. Commun. 2024, 15, 1484. [Google Scholar] [CrossRef] [PubMed]
- Dyre, J.C.; Schrøder, T.B. Universality of ac conduction in disordered solids. Rev. Mod. Phys. 2000, 72, 873–892. [Google Scholar] [CrossRef]
- Huhtinen, K.-E.; Törmä, P. Conductivity in flat bands from the Kubo-Greenwood formula. Phys. Rev. B 2023, 108, 155108. [Google Scholar] [CrossRef]
- Danieli, C.; Andreanov, A.; Leykam, D.; Flach, S. Flat band fine-tuning and its photonic applications. Nanophotonics 2024, 13, 3925–3944. [Google Scholar] [CrossRef]
- Jia, C.; Lin, Z.; Huang, Y.; Duan, X. Nanowire electronics: From nanoscale to macroscale. Chem. Rev. 2019, 119, 9074–9135. [Google Scholar] [CrossRef] [PubMed]
- Sánchez, V.; Wang, C. Application of renormalization and convolution methods to the Kubo-Greenwood formula in multidimensional Fibonacci systems. Phys. Rev. B 2004, 70, 144207. [Google Scholar] [CrossRef]
- Forman, R.A.; Piermarini, G.J.; Barnett, J.D.; Block, S. Pressure measurement made by the utilization of ruby sharp-line luminescense. Science 1972, 176, 284–285. [Google Scholar] [CrossRef] [PubMed]
- Economou, E.N. Green’s Functions in Quantum Physics, 3rd ed.; Springer: Berlin, Germany, 2006; pp. 14–16, 183–184. [Google Scholar]
- Oviedo-Roa, R.; Pérez, L.A.; Wang, C. AC conductivity of the transparent states in Fibonacci chains. Phys. Rev. B 2000, 62, 13805–13808. [Google Scholar] [CrossRef]
- Sánchez, V.; Wang, C. Resonant AC conducting spectra in quasiperiodic systems. Int. J. Comput. Mater. Sci. Eng. 2012, 1, 1250003. [Google Scholar] [CrossRef]
- Sánchez, V.; Wang, C. Improving the ballistic AC conductivity through quantum resonance in branched nanowires. Philos. Mag. 2015, 95, 326–333. [Google Scholar] [CrossRef]
- Griffiths, D.J.; Schroeter, D.F. Introduction to Quantum Mechanics, 3rd ed.; Cambridge University Press: Cambridge, UK, 2018; pp. 246–247. [Google Scholar]
- Sutton, A.P. Electronic Structure of Materials; Oxford University Press: New York, NY, USA, 1994; p. 41. [Google Scholar]
- Sánchez, V.; Wang, C. Real space theory for electron and phonon transport in aperiodic lattices via renormalization. Symmetry 2020, 12, 430. [Google Scholar] [CrossRef]
- Sánchez, F.; Sánchez, V.; Wang, C. Independent dual-channel approach to mesoscopic graphene transistors. Nanomaterials 2022, 12, 3223. [Google Scholar] [CrossRef] [PubMed]
- Palavicini, A.; Wang, C. Ab initio design and experimental confirmation of Fabry-Perot cavities based on freestanding porous silicon multilayers. J. Mater. Sci. Mater. Electron. 2020, 31, 60–64. [Google Scholar] [CrossRef]
- Sánchez, V.; Wang, C. A real-space study of flat bands in nanowires. Nanomaterials 2023, 13, 2864. [Google Scholar] [CrossRef] [PubMed]
- Bruus, H.; Flensberg, K. Many-Body Quantum Theory in Condensed Matter Physics, an Introduction; Oxford University Press: Oxford, UK, 2016; pp. 3–9. [Google Scholar]
- Sire, C. Electronic spectrum of a 2D quasi-crystal related to the octagonal quasi-periodic tiling. Europhys. Lett. 1989, 10, 483–488. [Google Scholar] [CrossRef]
- Sánchez, F.; Sánchez, V.; Wang, C. Ballistic transport in aperiodic Labyrinth tiling proven through a new convolution theorem. Eur. Phys. J. B 2018, 91, 132. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sánchez, V.; Wang, C. Flat-Band AC Transport in Nanowires. Nanomaterials 2025, 15, 38. https://doi.org/10.3390/nano15010038
Sánchez V, Wang C. Flat-Band AC Transport in Nanowires. Nanomaterials. 2025; 15(1):38. https://doi.org/10.3390/nano15010038
Chicago/Turabian StyleSánchez, Vicenta, and Chumin Wang. 2025. "Flat-Band AC Transport in Nanowires" Nanomaterials 15, no. 1: 38. https://doi.org/10.3390/nano15010038
APA StyleSánchez, V., & Wang, C. (2025). Flat-Band AC Transport in Nanowires. Nanomaterials, 15(1), 38. https://doi.org/10.3390/nano15010038




