Application of a Dynamic Step Size Iterative Method for Parameter Inversion in the Unified Hardening Models
Abstract
1. Introduction
2. Elastoplastic Constitutive Relationship Theory
2.1. General Elastoplastic Constitutive Relationship
2.2. Elastoplastic Stress–Strain Relationship
2.3. Iterative Formula of Stress and Strain Increment Under Different Boundary Conditions
2.3.1. Isotropic Compression (ISO) Stress Path
2.3.2. Oedometer Compression (K0) Stress Path
2.3.3. Constant σ3 Drained Shear Stress Path
2.3.4. Undrained Shear Stress Path with Constant Confining Pressure
2.3.5. Constant Mean Effective Stress (p) Drained Shear (p0) Stress Path
3. CSUH Model: Framework and Theory
3.1. Three-Dimensional Constitutive Model Framework
3.2. Third-Order Flexibility Matrix of the Model
4. Dynamic Iteration Method
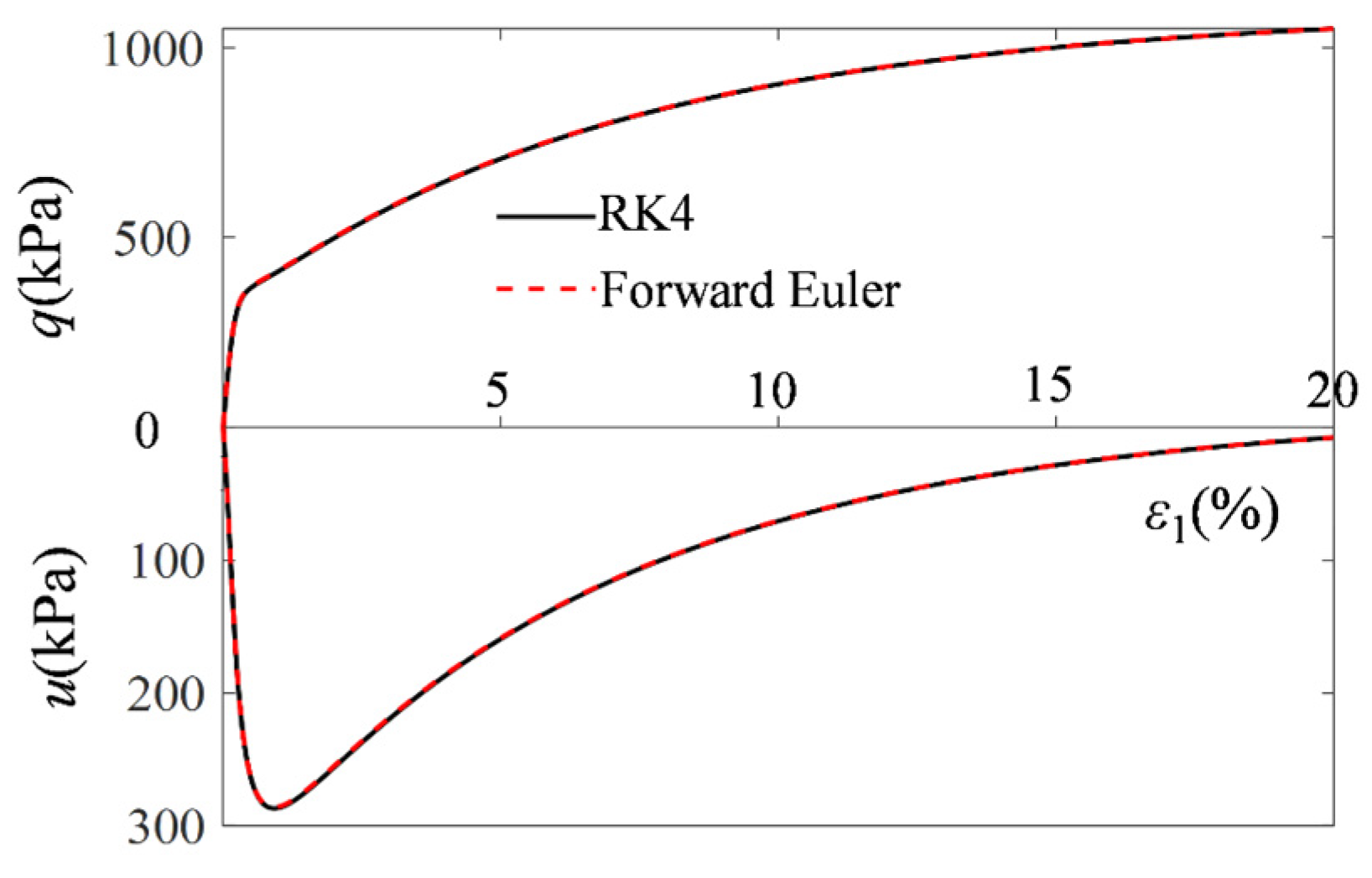
4.1. Common Iteration Methods
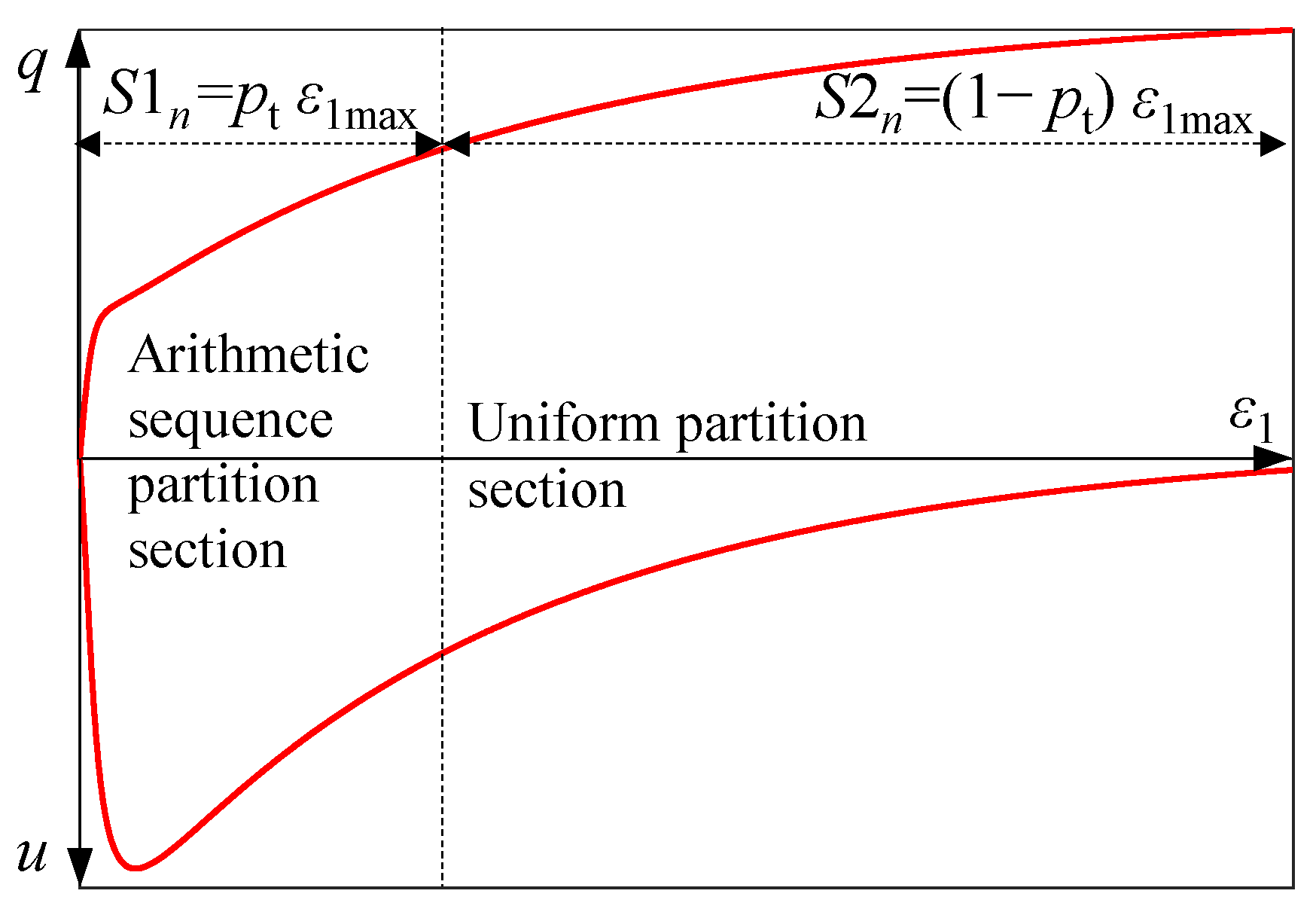
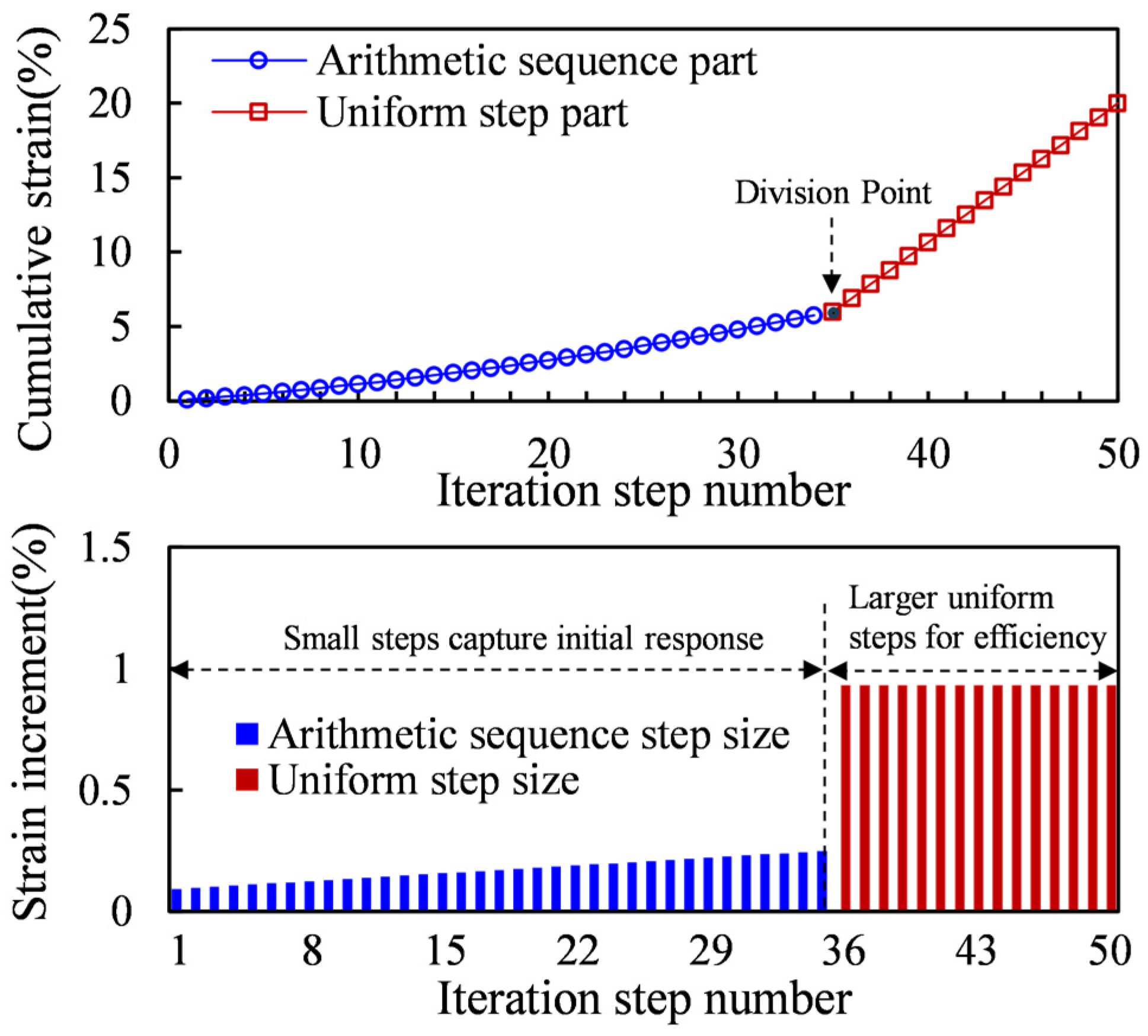
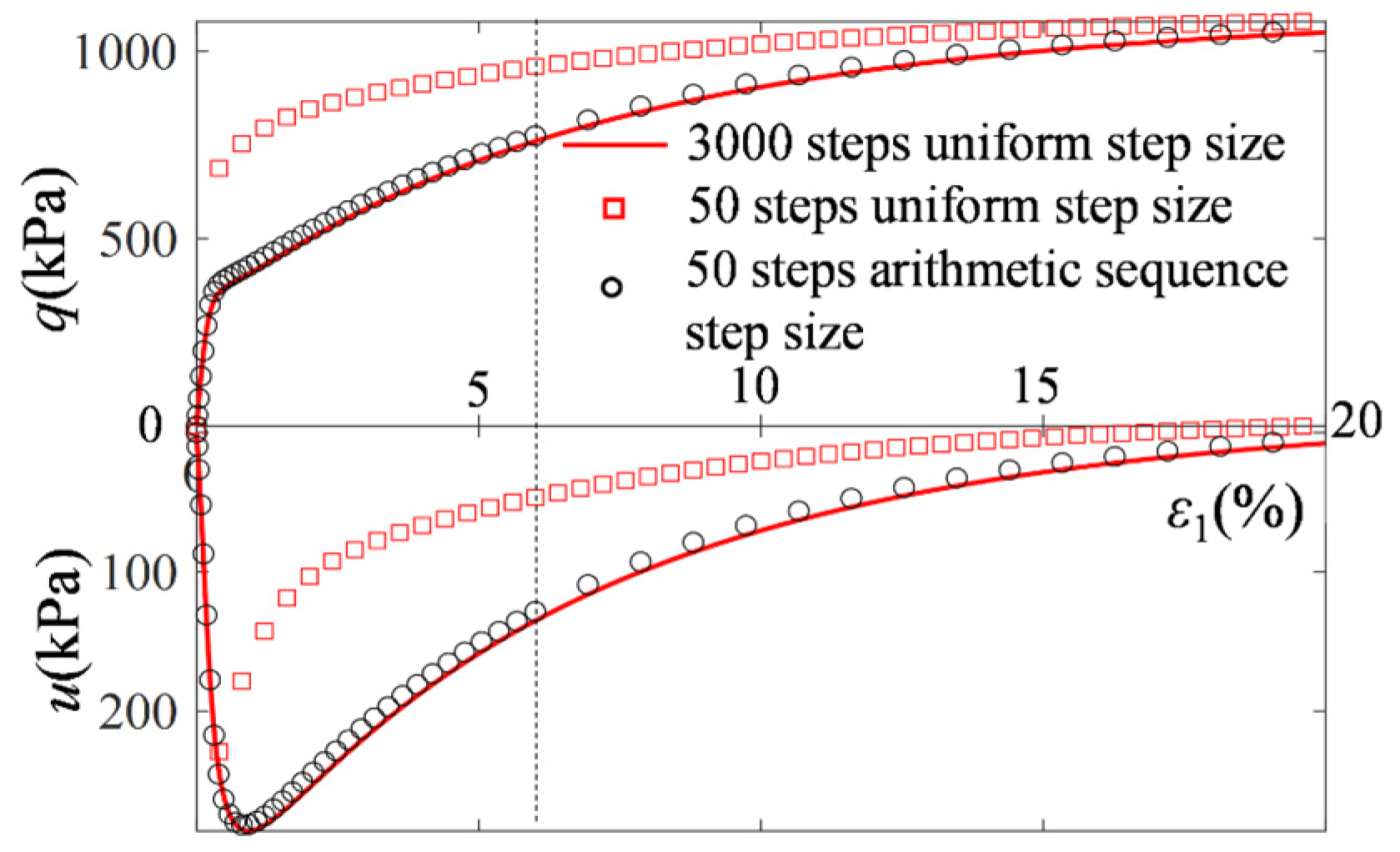
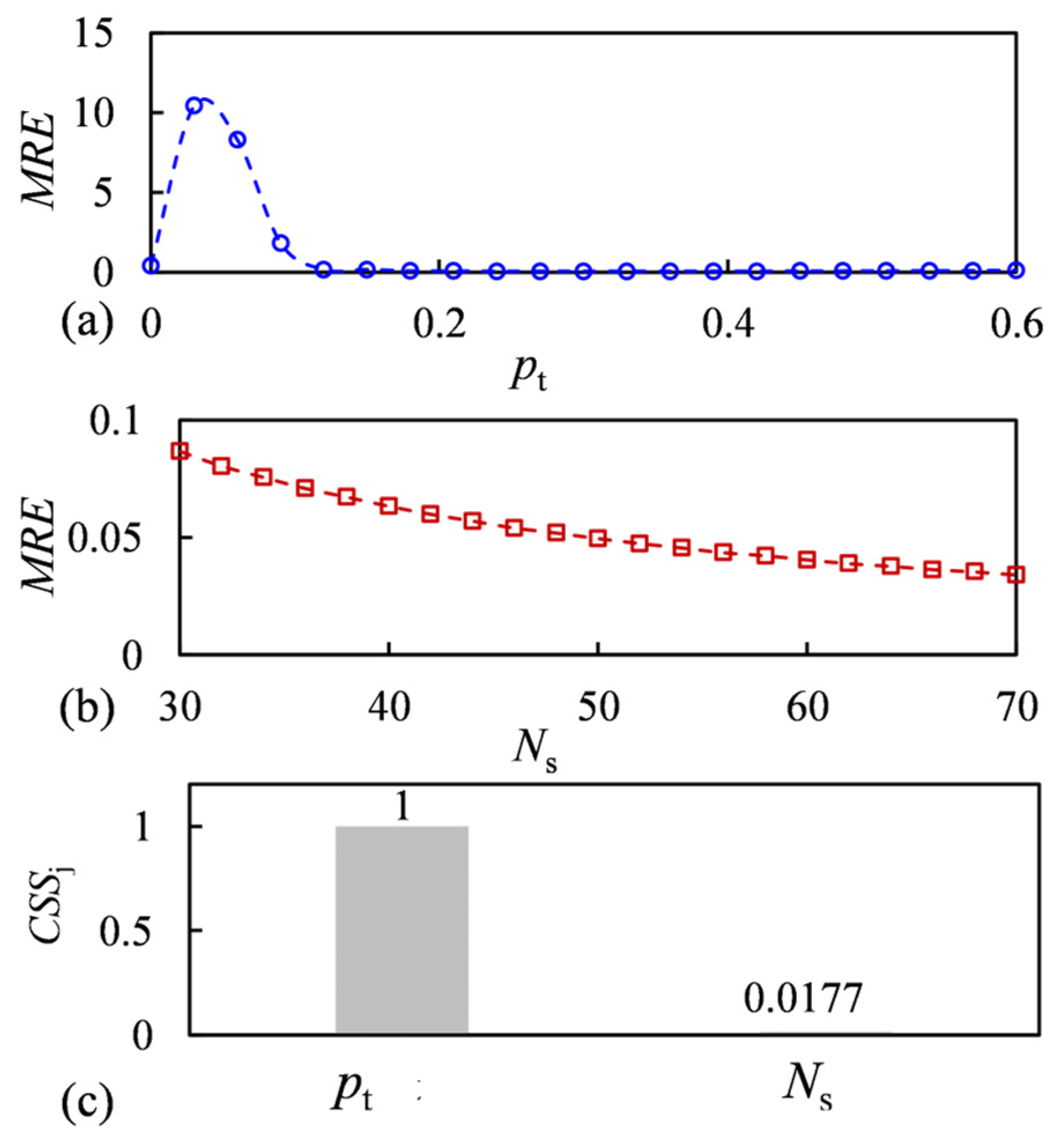
4.2. Proposed Dynamic Iterative Method
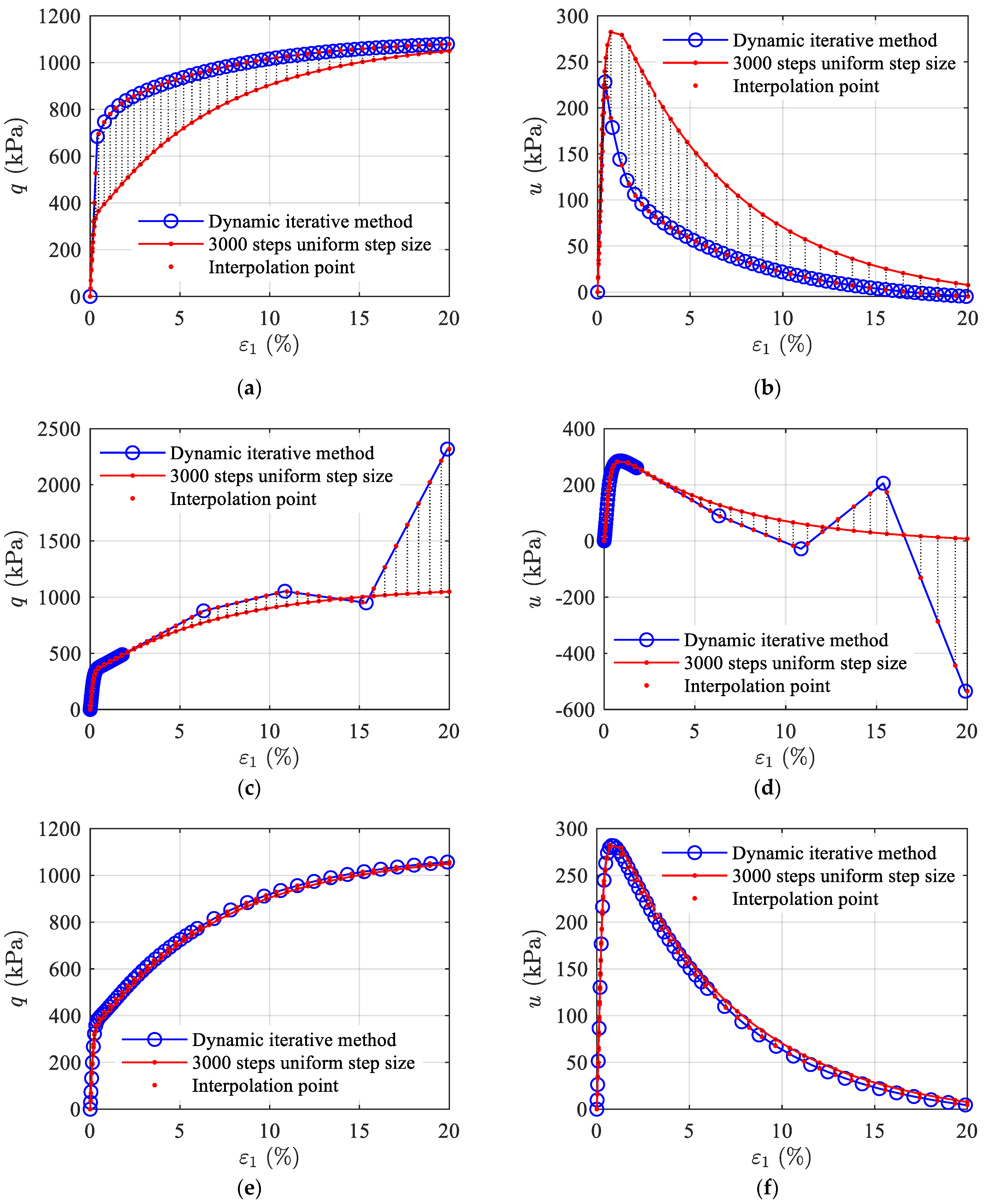
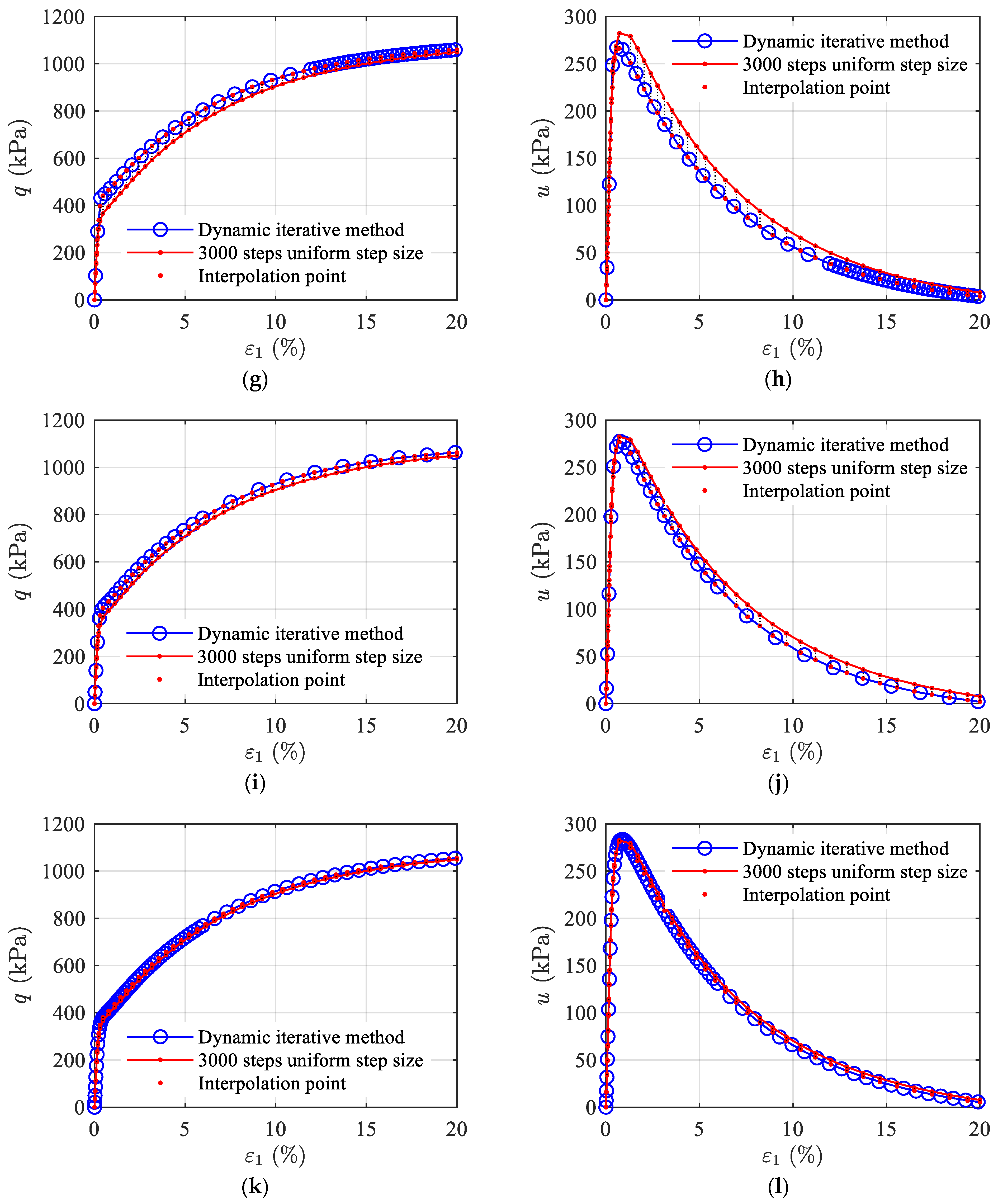
5. CSUH Model Iterative Inversion Parameters
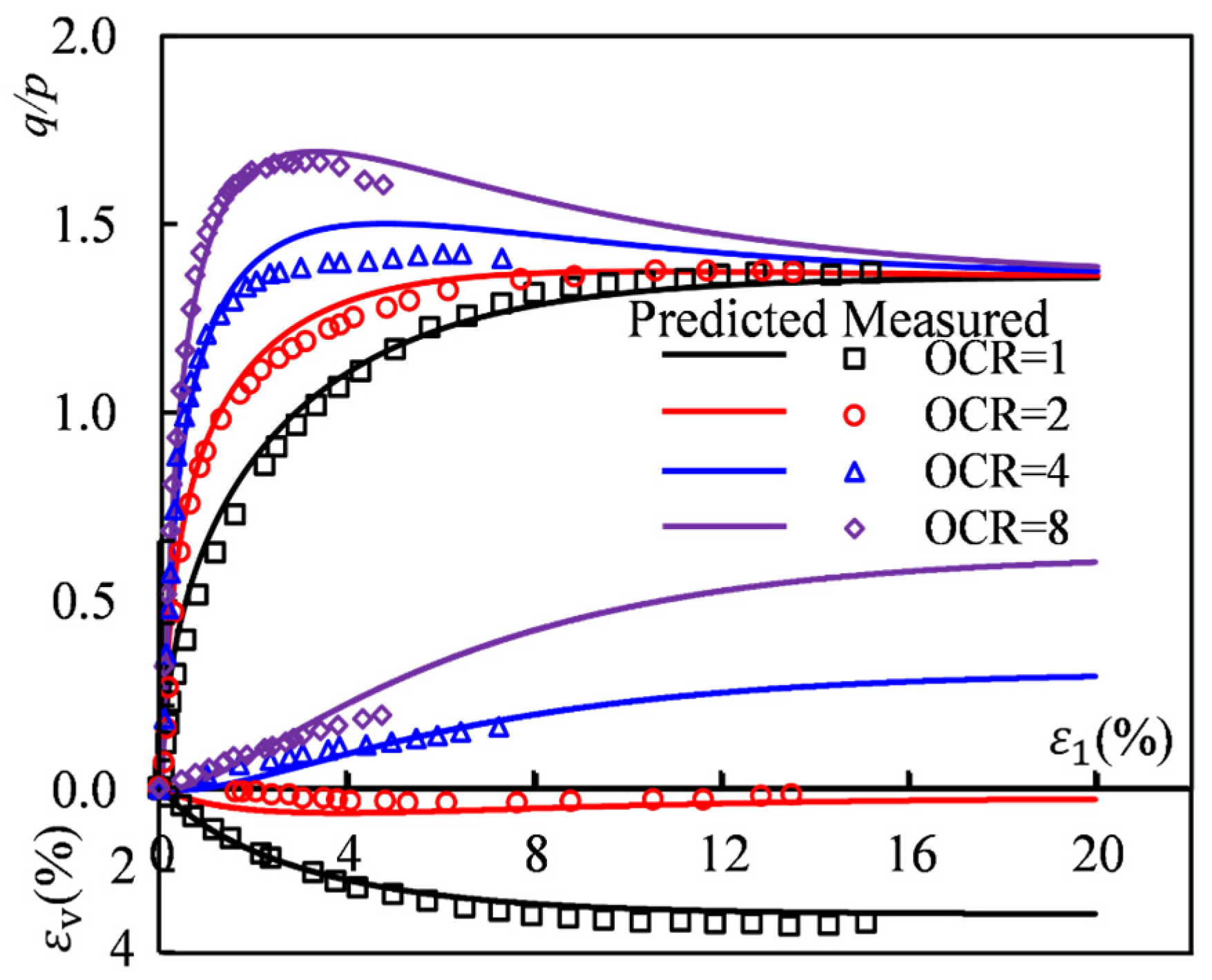
5.1. Fujinomori Clay
5.1.1. Constant p Compression Test
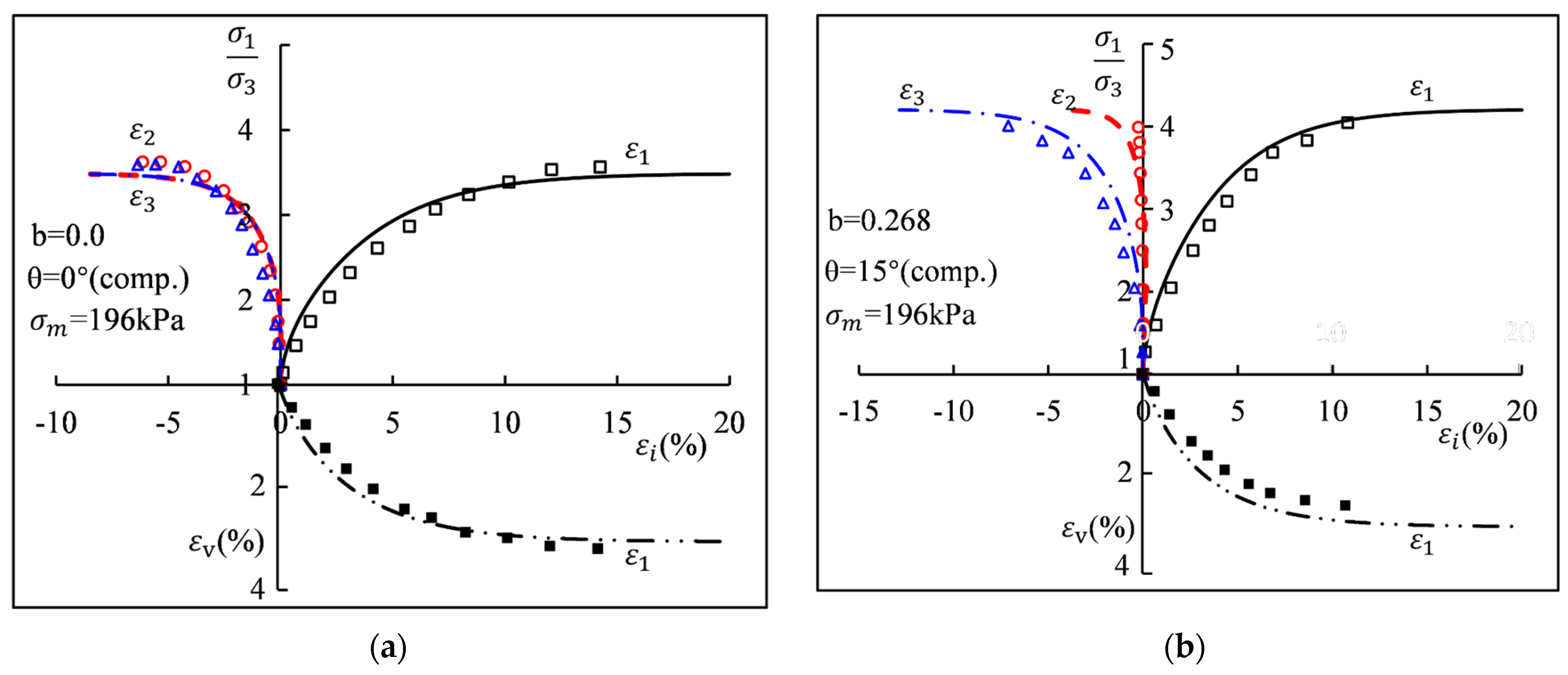
5.1.2. True Triaxial Test
5.2. Calcareous Sand
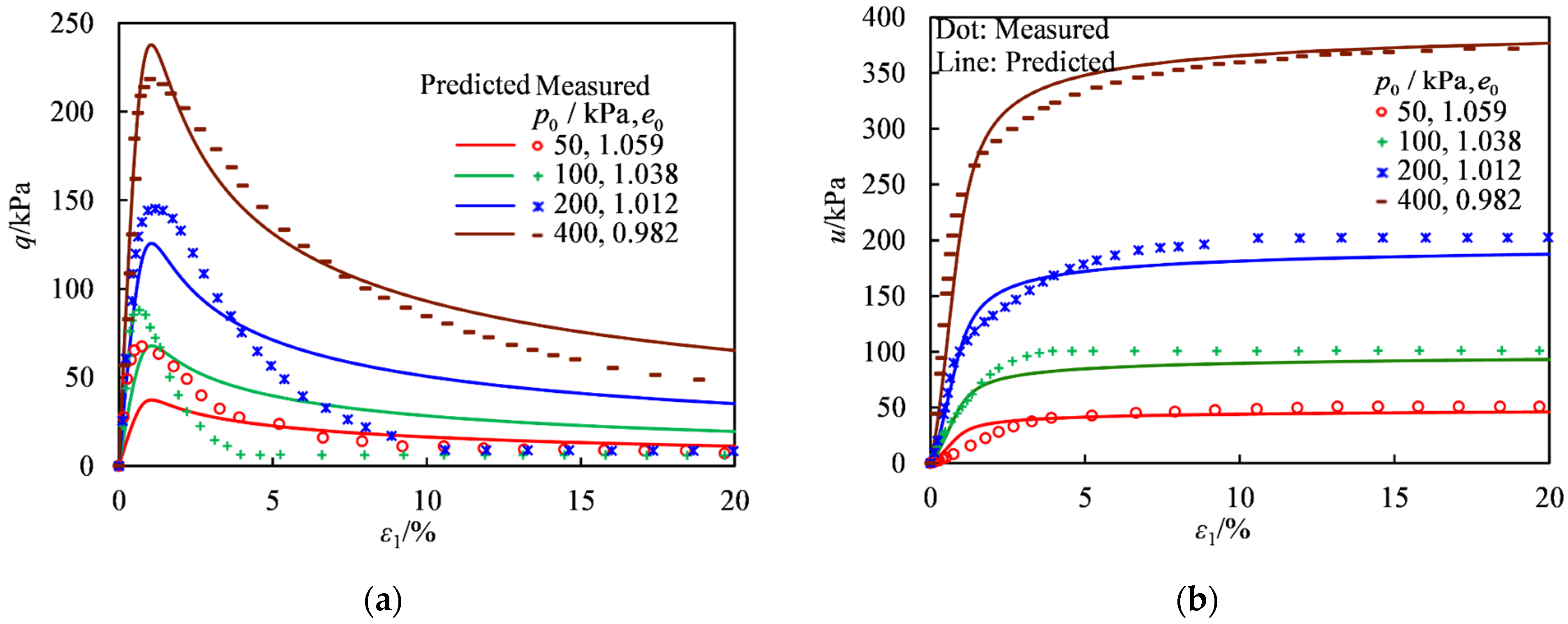
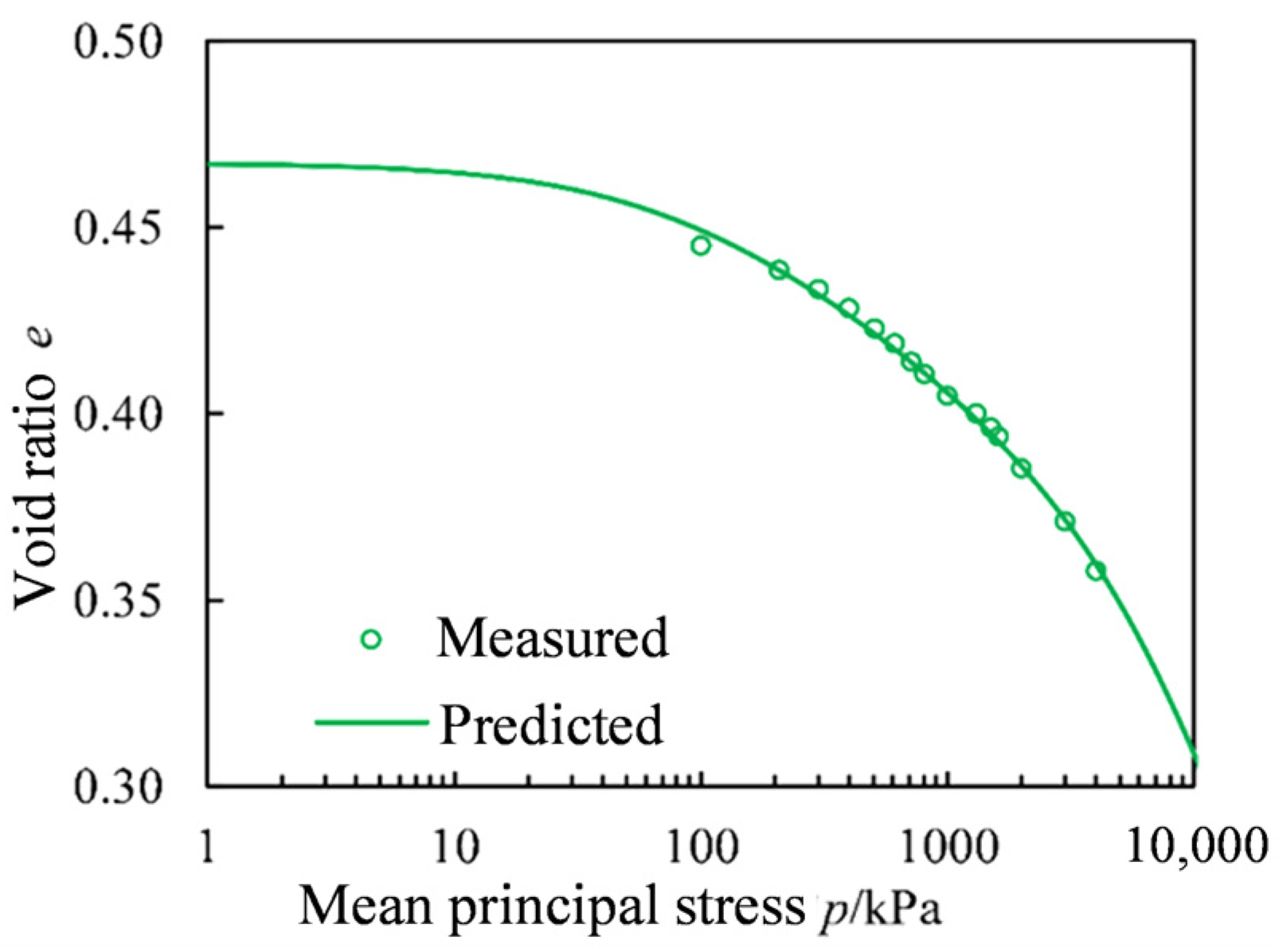
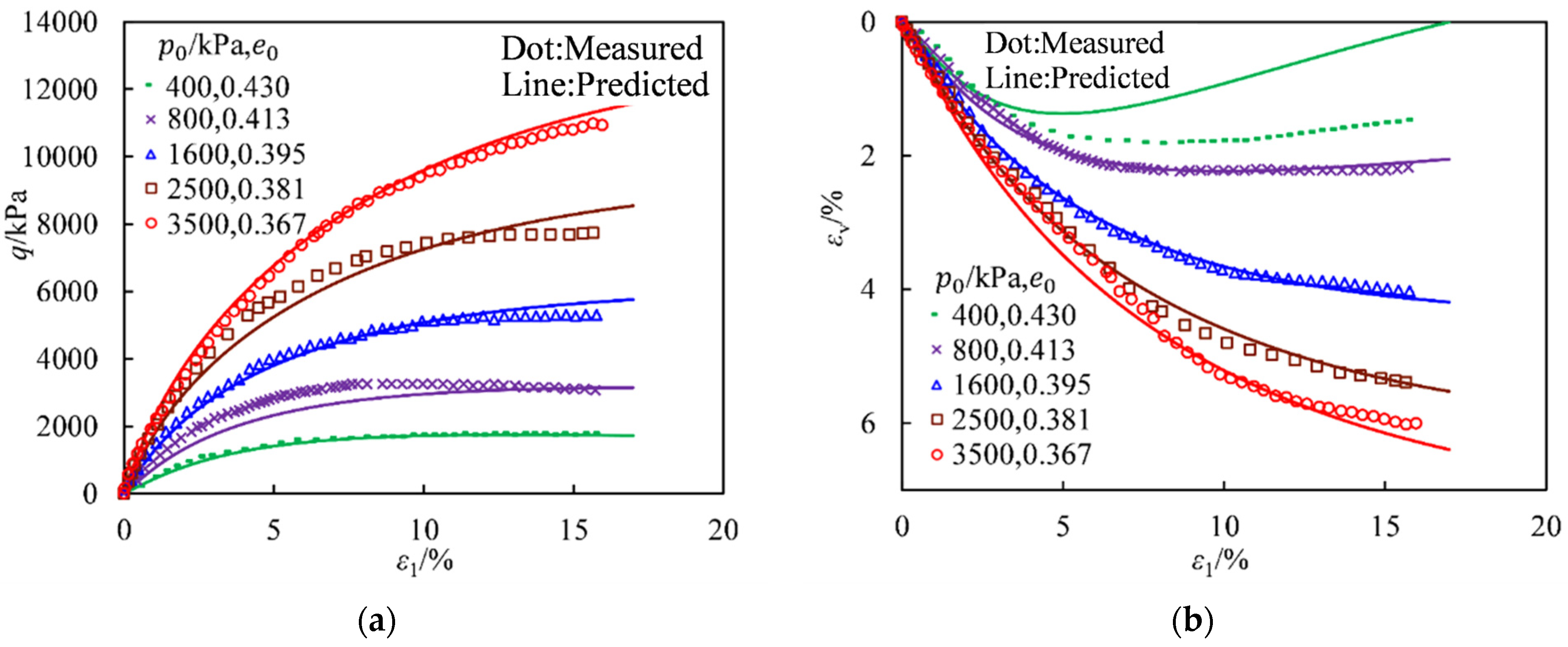
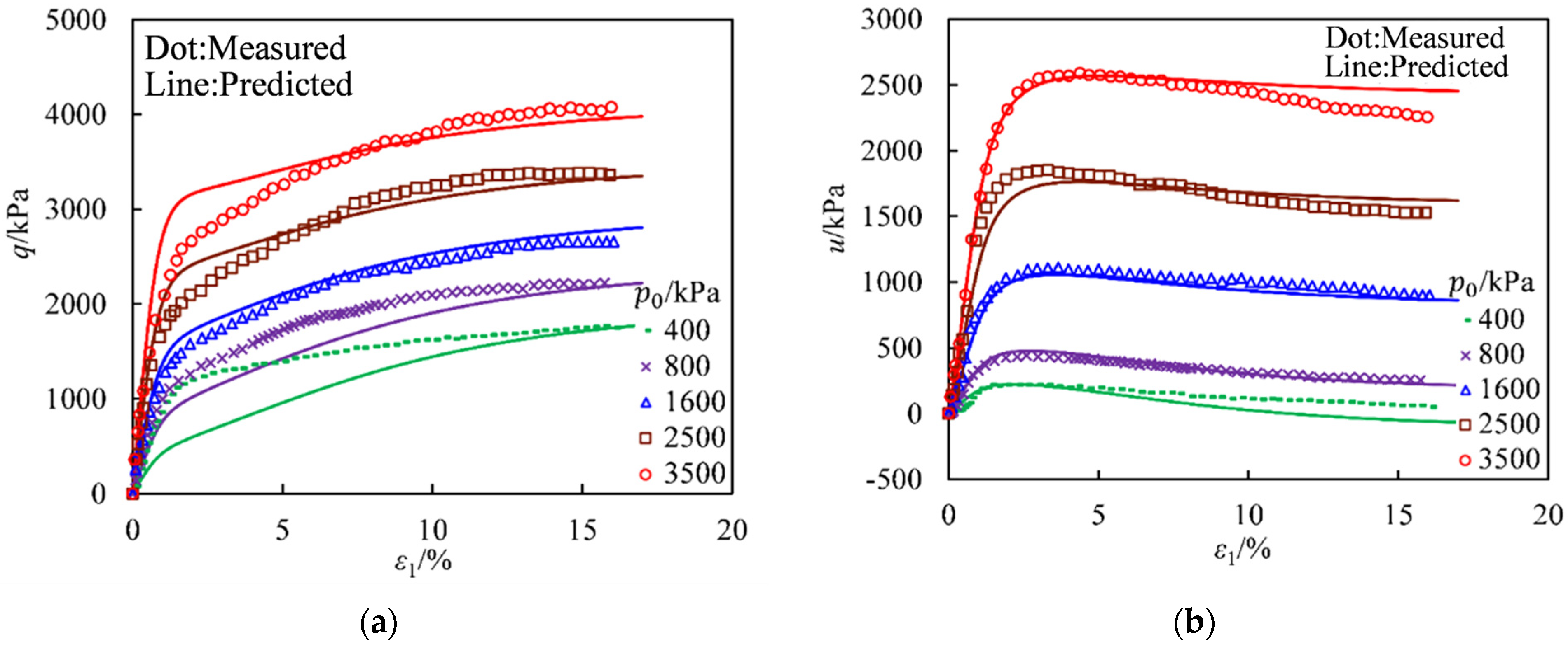
5.3. Rockfill Material
5.3.1. Isotropic Compression Test
5.3.2. CD Triaxial Compression Test
5.3.3. CU Triaxial Compression Test
6. Conclusions
- (1)
- The elastoplastic flexibility matrix of the elastoplastic constitutive model and the incremental stress–strain relationships under different boundary conditions are derived. This eliminates the need to solve equation systems when calculating theoretical model curves, thereby accelerating computation speed.
- (2)
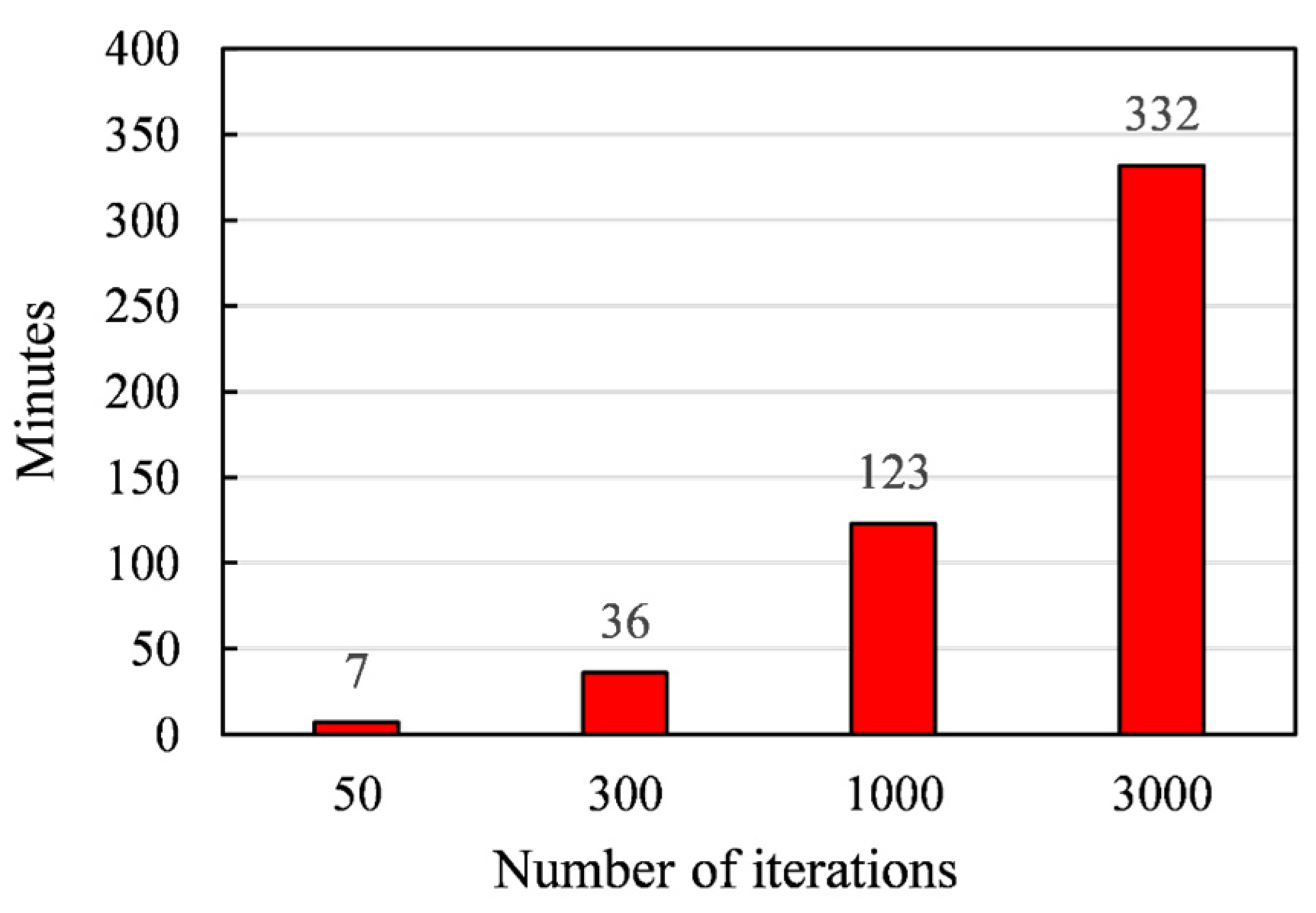
- Based on the variation characteristics of the stress–strain curve slope, the iterative step size is distributed using an arithmetic sequence over 30% of the total axial strain range, while a uniform distribution is applied over the remaining 70%. This effectively balances computational accuracy and efficiency. The study shows that this method reduces the number of iterations from the traditional 3000 steps to just 50 steps, decreasing computation time by approximately 47 times while maintaining accuracy comparable to the fourth-order Runge–Kutta method.
- (3)
- The CSUH model accurately describes the stress–strain characteristics of different soil types (such as clay, sand, and rockfill) under various stress paths, including isotropic compression, constant p drained conditions with different intermediate principal stress ratios, CD, and CU tests.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yao, Y.; Luo, T.; Hou, W. Soil Constitutive Models; People’s Transportation Publishing House Co., Ltd.: Beijing, China, 2018. [Google Scholar]
- Zhang, Y.; Chen, Y. A constitutive relationship for gravelly soil considering fine particle suffusion. Materials 2017, 10, 1217. [Google Scholar] [CrossRef] [PubMed]
- Cong, S.; Ling, X.; Li, X.; Geng, L.; Xing, W.; Li, G. Elastoplastic model framework for saturated soils subjected to a freeze–thaw cycle based on generalized plasticity theory. Materials 2021, 14, 6485. [Google Scholar] [CrossRef] [PubMed]
- Dong, L.; Tian, S.; Yao, C.; Han, X.; Wang, K. A nonlinear constitutive model for remoulded fine-grained materials used under the Qinghai–Tibet railway line. Materials 2022, 15, 5119. [Google Scholar] [CrossRef] [PubMed]
- Roscoe, K.H.; Schofield, A.N.; Thurairajah, A. Yielding of clays in states wetter than critical. Géotechnique 1963, 13, 211–240. [Google Scholar] [CrossRef]
- Roscoe, K.H.; Burland, J.B. On the generalized stress–strain behaviour of ‘wet clay’. In Engineering Plasticity; Cambridge University Press: Cambridge, UK, 1968. [Google Scholar]
- Hou, W.; Yao, Y. Analysis in behaviors of over-consolidated clays described by cam-clay model and unified hardening model. Ind. Constr. 2011, 41, 18–23+140. [Google Scholar] [CrossRef]
- Yao, Y.P.; Gao, Z.W.; Zhao, J.D.; Wan, Z. Modified UH model: Constitutive modeling of overconsolidated clays based on a parabolic Hvorslev envelope. J. Geotech. Geoenviron. Eng. 2012, 138, 860–868. [Google Scholar] [CrossRef]
- Yao, Y.P.; Hou, W.; Zhou, A. Constitutive model for overconsolidated clays. Sci. China Ser. E-Technol. Sci. 2008, 51, 179–191. [Google Scholar] [CrossRef]
- Yao, Y.P.; Hou, W.; Zhou, A. UH model: Three-dimensional unified hardening model for overconsolidated clays. Géotechnique 2009, 59, 451–469. [Google Scholar] [CrossRef]
- Yao, Y.P.; Liu, L.; Luo, T.; Tian, Y.; Zhang, J.M. Unified hardening (UH) model for clays and sands. Comput. Geotech. 2019, 110, 326–343. [Google Scholar] [CrossRef]
- Zhu, B.; Chen, Z. Calibrating and validating a soil constitutive model through conventional triaxial tests: An in-depth study on CSUH model. Acta Geotech. 2022, 17, 3407–3420. [Google Scholar] [CrossRef]
- Ilie-Octavian, P.; Mihai-Octavian, P.; Mihaela, O.; Gabriela-Petruța, R.; Lucia, C.A. Calibrating DC01 material properties for finite element analysis with Abaqus and Isight. Methodology 2023, 7, 8. [Google Scholar] [CrossRef]
- Sloan, S.W.; Abbo, A.J.; Sheng, D. Refined explicit integration of elastoplastic models with automatic error control. Eng. Comput. 2001, 18, 121–194. [Google Scholar] [CrossRef]
- Sloan, S.W. Substepping schemes for the numerical integration of elastoplastic stress–strain relations. Int. J. Numer. Methods Eng. 1987, 24, 893–911. [Google Scholar] [CrossRef]
- Zhu, B.; Su, X.; Cao, Y.; Yan, F. Determining parameters of the CSUH constitutive model by genetic algorithm. Jpn. Geotech. Soc. Spec. Publ. 2020, 8, 188–193. [Google Scholar] [CrossRef]
- Yin, Z.; Jin, Y. Development of Geotechnical Optimization Platform EROSOPT. In Practice of Optimisation Theory in Geotechnical Engineering; Springer: Singapore, 2019; pp. 243–292. [Google Scholar] [CrossRef]
- Yao, Y.P.; Wang, N. Transformed stress method for generalizing soil constitutive models. J. Eng. Mech. 2014, 140, 614–629. [Google Scholar] [CrossRef]
- Kadlíček, T.; Janda, T.; Šejnoha, M.; Najser, J.; Beneš, Š. Automated calibration of advanced soil constitutive models. Part I: Hypoplastic sand. Acta Geotech. 2022, 17, 3421–3438. [Google Scholar] [CrossRef]
- Nakai, T.; Hinokio, M. A simple elastoplastic model for normally and over consolidated soils with unified material parameters. Soils Found. 2004, 44, 53–70. [Google Scholar] [CrossRef] [PubMed]
- Nakai, T.; Matsuoka, H.; Okuno, N.; Tsuzuki, K. True triaxial tests on normally consolidated clay and analysis of the observed shear behavior using elastoplastic constitutive models. Soils Found. 1986, 26, 67–78. [Google Scholar] [CrossRef] [PubMed]
- Weng, Y. Study on the Shear Strength of Calcareous Soil and Its Influencing Mechanism. Ph.D. Thesis, Guangxi University, Nanning, China, 2017. [Google Scholar]
- Liu, E.; Chen, S.; Li, G.; Zhong, Q.M. Critical state of rockfill materials and a constitutive model considering grain crushing. Rock Soil Mech. 2011, 32, 148–154. [Google Scholar] [CrossRef]
- Yao, Y.; Zhang, B.; Zhu, J. Behaviors, constitutive models and numerical simulation of soils. China Civ. Eng. J. 2012, 45, 127–150. [Google Scholar] [CrossRef]



| Parameters | M | ν | κ | λ | N | Z | χ | m |
|---|---|---|---|---|---|---|---|---|
| Values | 1.25 | 0.3 | 0.04 | 0.135 | 1.973 | 0.934 | 0.4 | 1.8 |
| OCR | 1 | 2 | 4 | 8 |
|---|---|---|---|---|
| p0 | 196 | 196 | 196 | 98 |
| e0 | 0.769 | 0.719 | 0.668 | 0.684 |
| Parameters | M | ν | κ | λ | N | Z | χ | m |
|---|---|---|---|---|---|---|---|---|
| Values | 1.36 | 0.0 | 0.02 | 0.093 | 1.26 | 1.26 | 0.05 | 5 |
| Parameters | M | ν | κ | λ | N | Z | χ | m |
|---|---|---|---|---|---|---|---|---|
| Values | 1.473 | 0.337 | 0.023 | 0.057 | 1.332 | 1.21 | 0.99 | 0.145 |
| Parameters | M | ν | κ | λ | N | Z | χ | m |
|---|---|---|---|---|---|---|---|---|
| Values | 1.678 | 0.272 | 0.021 | 0.087 | 1.125 | 0.742 | 0.385 | 1.716 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, B.; Cai, D.; Yan, H.; Bi, Z.; Ouyang, M.; Yao, J. Application of a Dynamic Step Size Iterative Method for Parameter Inversion in the Unified Hardening Models. Appl. Sci. 2025, 15, 5147. https://doi.org/10.3390/app15095147
Zhu B, Cai D, Yan H, Bi Z, Ouyang M, Yao J. Application of a Dynamic Step Size Iterative Method for Parameter Inversion in the Unified Hardening Models. Applied Sciences. 2025; 15(9):5147. https://doi.org/10.3390/app15095147
Chicago/Turabian StyleZhu, Binglong, Degou Cai, Hongye Yan, Zongqi Bi, Mingzhe Ouyang, and Junkai Yao. 2025. "Application of a Dynamic Step Size Iterative Method for Parameter Inversion in the Unified Hardening Models" Applied Sciences 15, no. 9: 5147. https://doi.org/10.3390/app15095147
APA StyleZhu, B., Cai, D., Yan, H., Bi, Z., Ouyang, M., & Yao, J. (2025). Application of a Dynamic Step Size Iterative Method for Parameter Inversion in the Unified Hardening Models. Applied Sciences, 15(9), 5147. https://doi.org/10.3390/app15095147







