Stability Evaluation Method for Rock Slope-Anchorage Systems Based on Genetic Algorithms and Discrete Element Analysis
Abstract
1. Introduction
2. Evaluation Model
3. Case Study
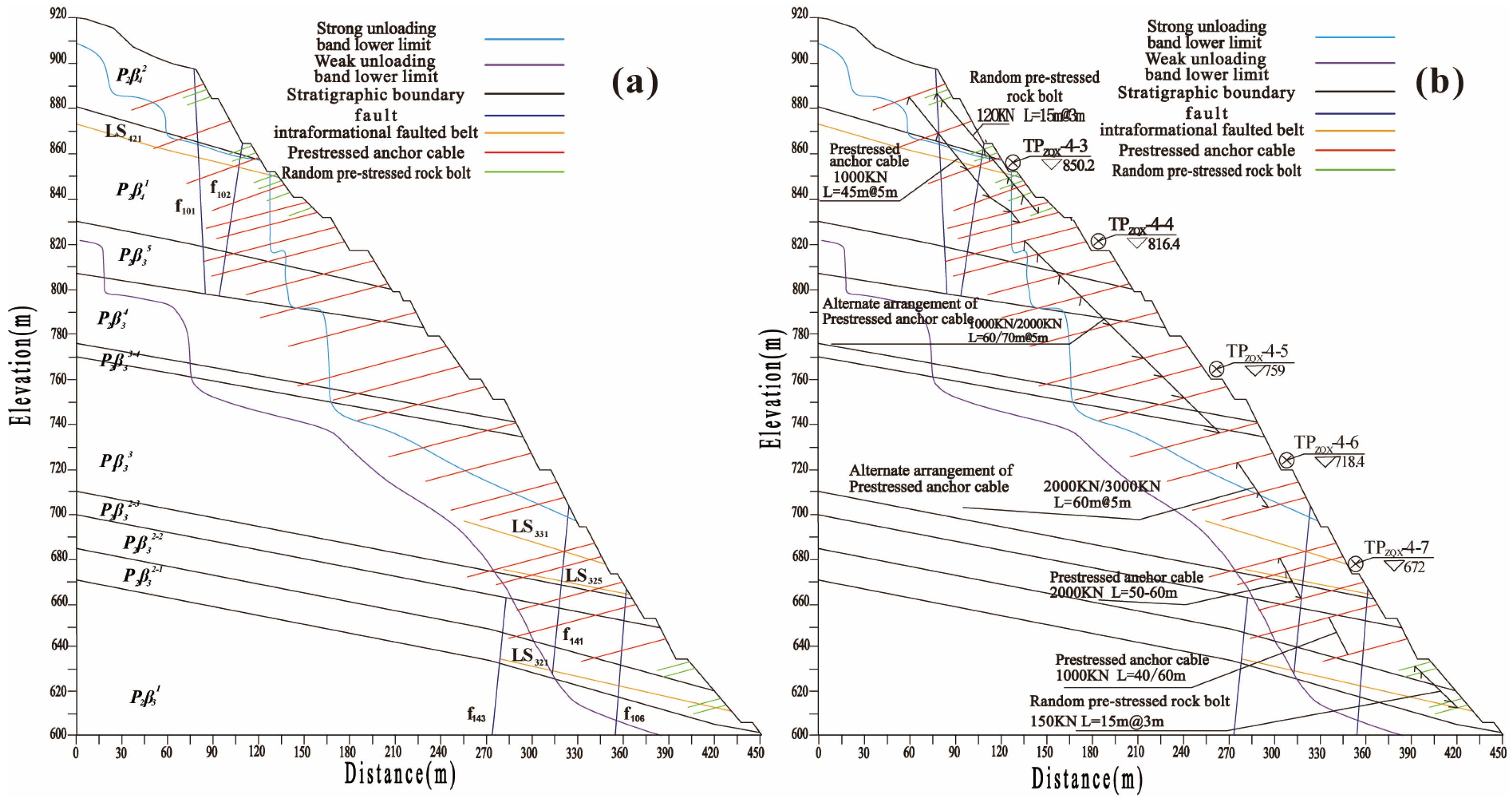
3.1. Engineering Situation
3.2. Characteristics of Rock Slope Anchoring System
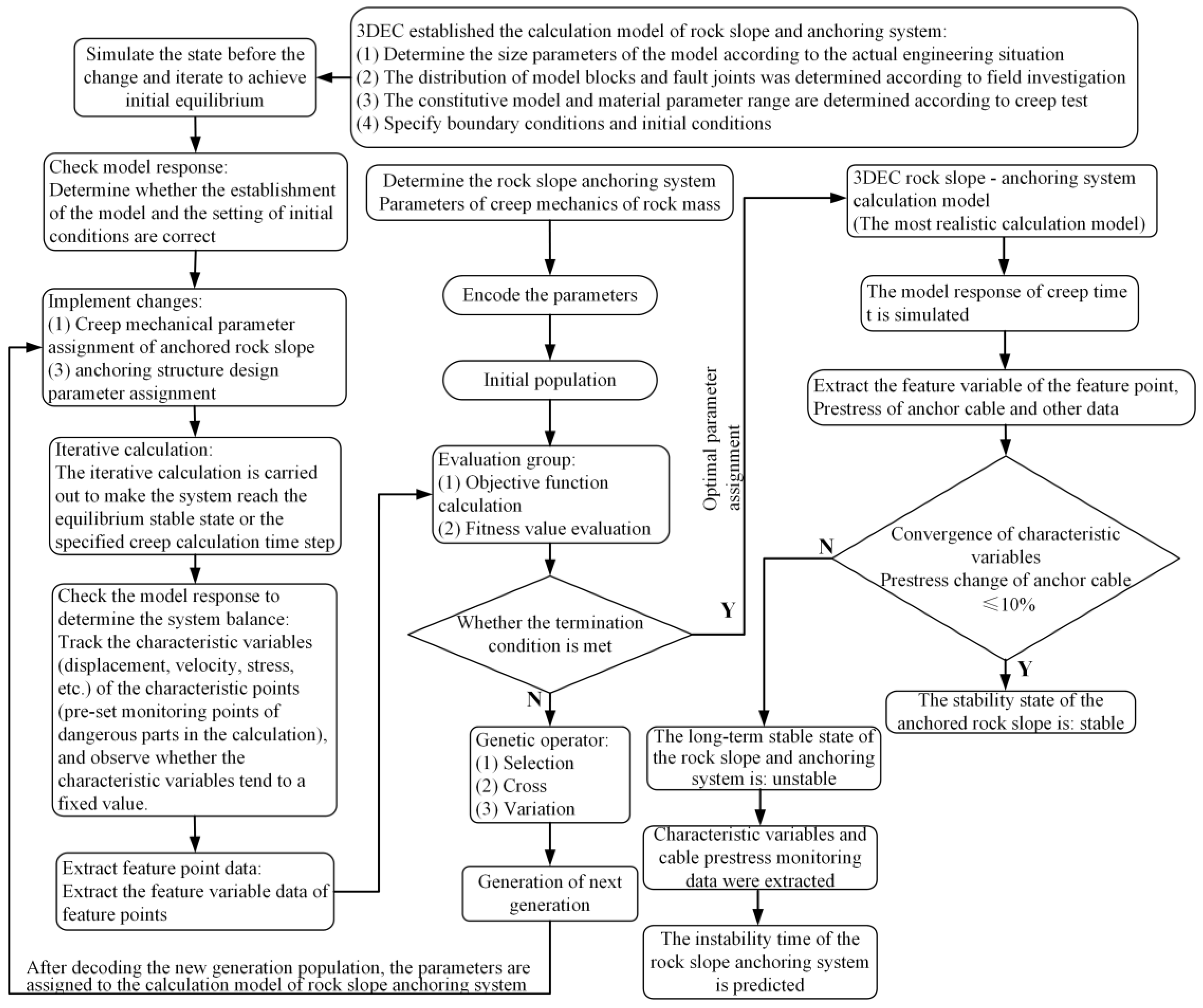
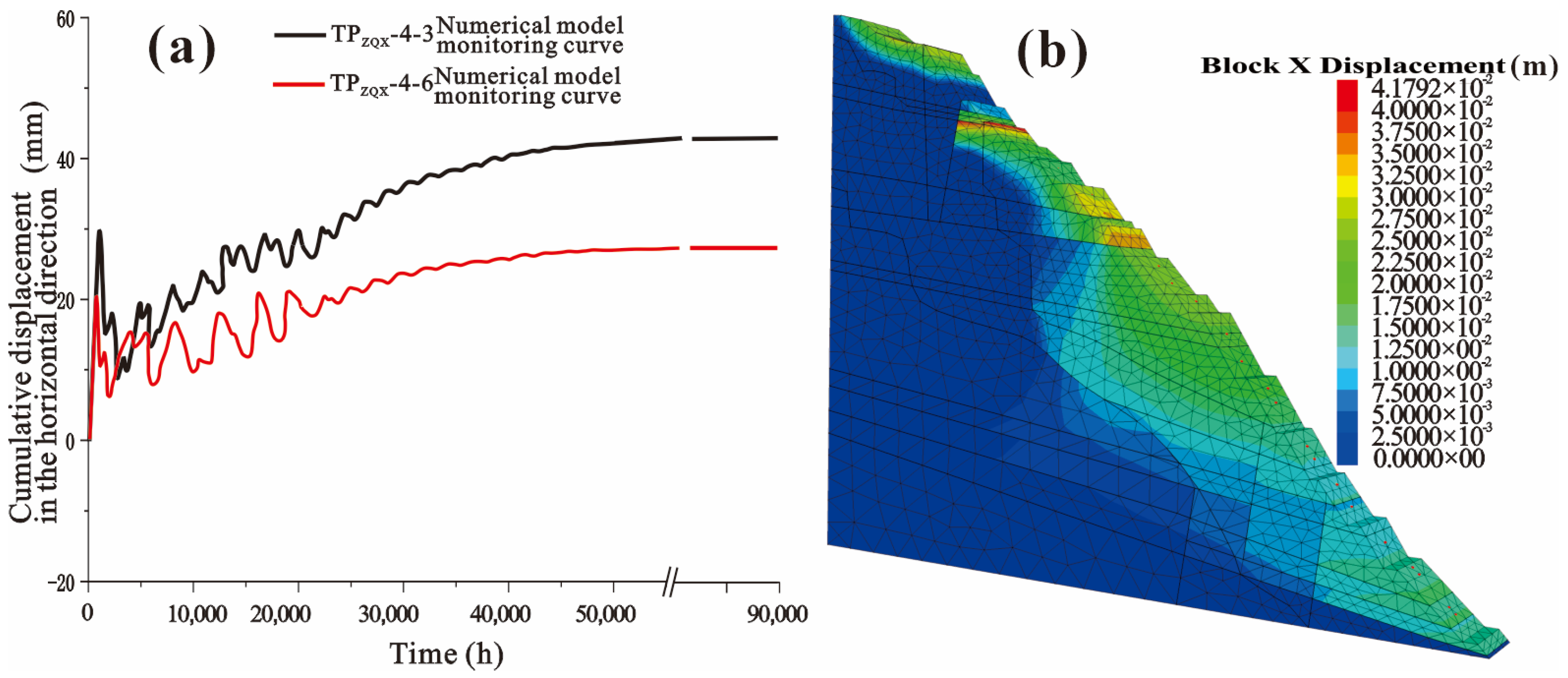
3.3. Stability Evaluation Based on Genetic Algorithm and Discrete Element Analysis
- (1)
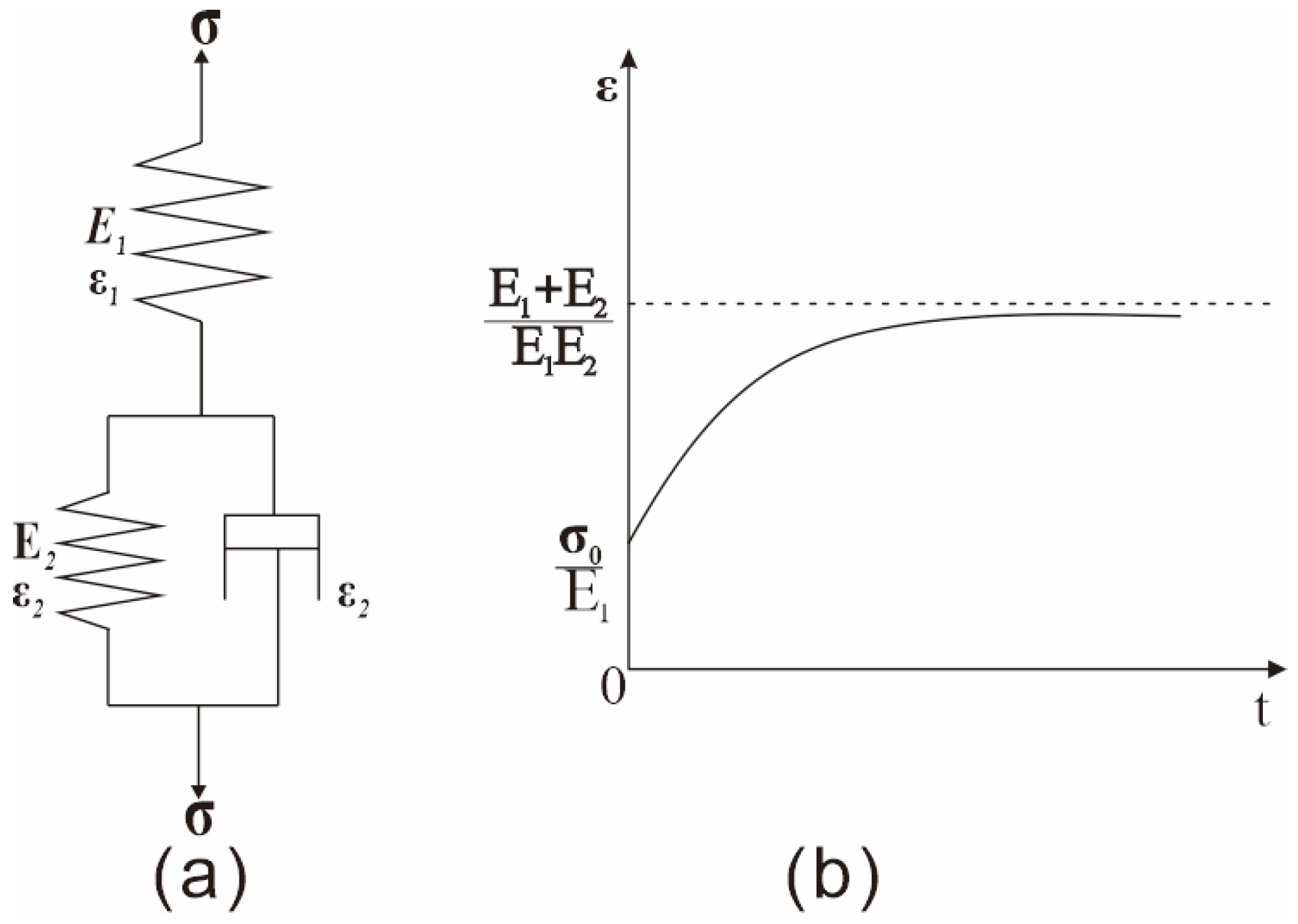
- Rock Mass Constitutive Model and Parameter Range
- (2)
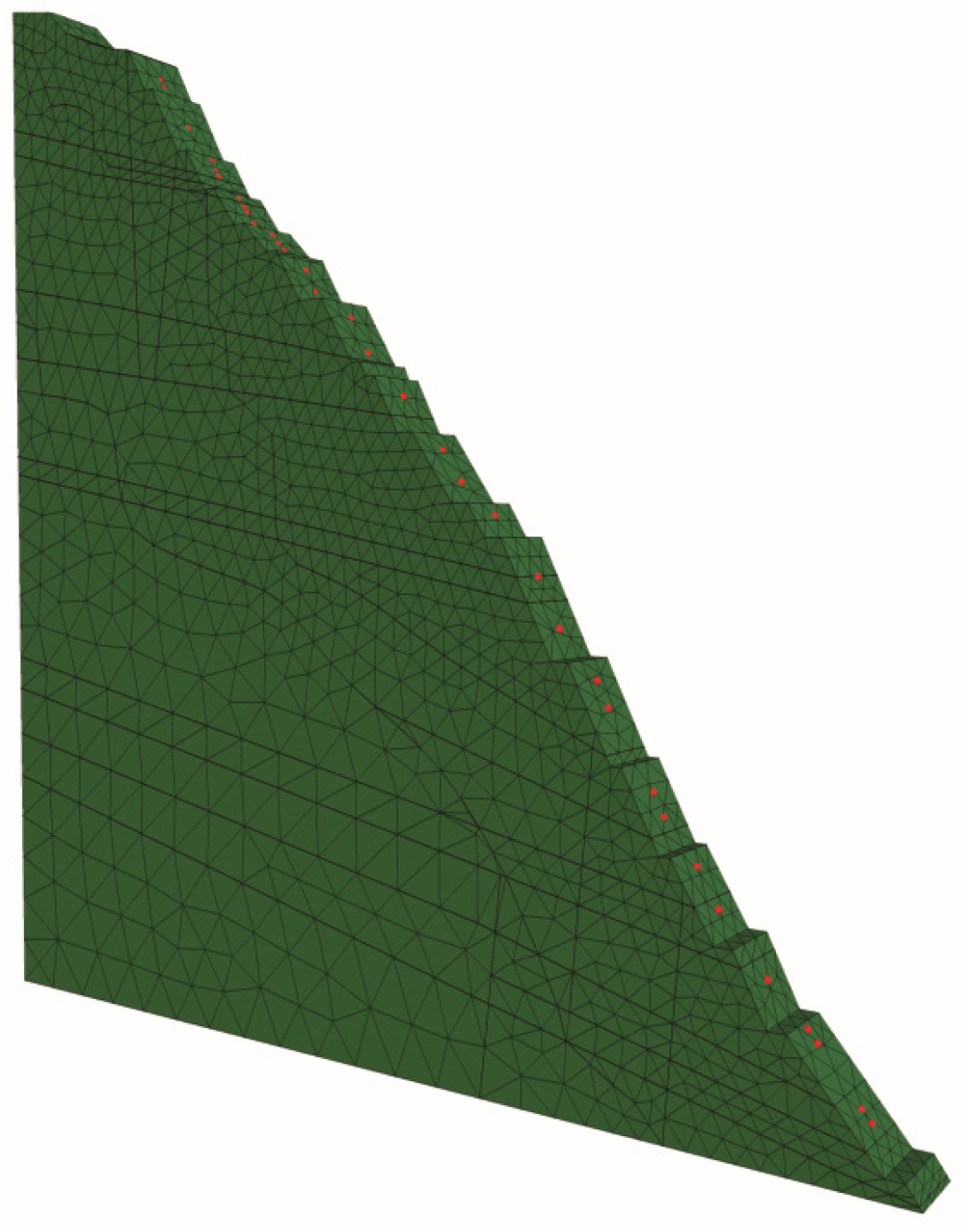
- Development of the Numerical Model Using the Discrete Element Method
- (3)
- Optimization Using Genetic Algorithm
- (4)
- Stability Evaluation of the Left Bank Water Cushion Rock Slope Anchoring System
4. Discussion
5. Conclusions
- (1)
- The stability evaluation method for the rock slope anchoring system proposed in this paper considers the interaction between the anchoring structure and the rock slope and accurately reflects the displacement, deformation, and stress distribution of the rock mass slope anchoring system.
- (2)
- The stability evaluation method for the rock slope anchoring system is based on the discrete element method, which can fully account for large displacements in discontinuous rock masses and phenomena such as contact surface slip and separation and can more accurately reflect the internal deformation and stress distribution of structural surfaces and jointed rock masses. Simultaneously, a genetic algorithm is introduced to optimize the parameters of the numerical model, effectively improving the evaluation efficiency. Furthermore, the most representative numerical analysis model for the engineering conditions is obtained, combining the practical engineering situation with the numerical model, thereby improving the accuracy of the stability evaluation.
- (3)
- This evaluation method clearly presents the stability evolution process of the rock slope anchoring system, providing intuitive evaluation results.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- He, M.; Ren, S.; Guo, L.; Lin, W.; Zhang, T.; Tao, Z. Experimental study on influence of host rock strength on shear performance of Micro-NPR steel bolted rock joints. Int. J. Rock Mech. Min. Sci. 2022, 159, 105236. [Google Scholar] [CrossRef]
- Li, C.C. Field observations of rock bolts in high stress rock masses. Rock Mech. Rock Eng. 2010, 43, 491–496. [Google Scholar] [CrossRef]
- Ma, S.; Zhao, Z.; Shang, J. An analytical model for shear behaviour of bolted rock joints. Int. J. Rock Mech. Min. Sci. 2019, 121, 104019. [Google Scholar] [CrossRef]
- Pei, S.F.; Hao, W.F.; Fan, Y.L.; Chen, H.; Li, W.T. Operation condition of anchoring system in large-scale water conservancy and hydropower projects. J. Chang. Jiang River Sci. Res. Institute 2024, 41, 142–150. (In Chinese) [Google Scholar]
- Wen, L.; Huang, L.; Xiang, X.; Yang, X.Z.; Zhang, G.C. Research status and development trend of geotechnical anchorage technology in hydropower projects. Saf. Environ. Eng. 2023, 30, 35–45. (In Chinese) [Google Scholar]
- Deng, D.P.; Li, L.; Zhao, L.H. Stability analysis of slopes reinforced with anchor cables and optimal design of anchor cable parameters. Eur. J. Environ. Civ. Eng. 2021, 25, 2425–2440. [Google Scholar] [CrossRef]
- Bian, X.; Liu, J.; Nie, L.; Ma, S.; Tao, W.; Qiu, C.; Xu, G. Reliability evaluation of a high slope reinforced by anchor based on the stochastic finite-element method: Case study. Case Stud. Constr. Mater. 2023, 19, e02650. [Google Scholar] [CrossRef]
- Chen, J.F.; Du, C.C.; Peng, M.; Sun, R.; Zhao, F.; Shi, Z.M. System reliability analysis of a slope stabilized with anchor cables and piles under seismic loading. Acta Geotech. 2023, 18, 4493–4514. [Google Scholar] [CrossRef]
- Park, H.; West, T.R. Development of a probabilistic approach for rock wedge failure. Eng. Geol. 2001, 59, 233–251. [Google Scholar] [CrossRef]
- Ahmadabadi, M.; Poisel, R. Probabilistic analysis of rock slopes involving correlated non-normal variables using point estimate methods. Rock Mech. Rock Eng. 2016, 49, 909–925. [Google Scholar] [CrossRef]
- Duzgun, H.S.B.; Bhasin, R.K. Probabilistic stability evaluation of Oppstadhornet rock slope, Norway. Rock Mech. Rock Eng. 2009, 42, 729–749. [Google Scholar] [CrossRef]
- Li, N.; Li, G.; He, M. Key techniques for the analysis and evaluation of high rock slopes. Arab. J. Geosci. 2021, 14, 1171. [Google Scholar] [CrossRef]
- Chen, S.; Yang, Z.; Zhang, W.; Li, L.; Zheng, Y.; Yuan, Y. Numerical simulation of the stability of a cutting slope and study on its reinforcement scheme. Adv. Civ. Eng. 2022, 2022, 5306923. [Google Scholar] [CrossRef]
- Liu, Y.; Lai, J.; Xu, J. Study on slope monitoring and stability based on bolt–cable combined support. Buildings 2024, 14, 886. [Google Scholar] [CrossRef]
- Bi, J.; Luo, X.; Zhang, H.; Shen, H. Stability analysis of complex rock slopes reinforced with prestressed anchor cables and anti-shear cavities. Bull. Eng. Geol. Environ. 2019, 78, 2027–2039. [Google Scholar] [CrossRef]
- Yan, M.; Xia, Y.; Liu, T.; Bowa, V.M. Limit analysis under seismic conditions of a slope reinforced with prestressed anchor cables. Comput. Geotech. 2019, 108, 226–233. [Google Scholar] [CrossRef]
- Sun, J.; Yu, T.; Dong, P. Pseudo-dynamic analysis of reinforced slope with anchor cables. Soil Dyn. Earthq. Eng. 2022, 162, 10751. [Google Scholar] [CrossRef]
- Hara, T.; Tatta, N.; Yashima, A. Assessment of ground-anchored slope stability based on variation in residual tensile forces. Soils Found. 2023, 63, 101353. [Google Scholar] [CrossRef]
- Jiang, L.; Zhang, Q.; Jia, C.; Yu, J.; Huang, Q. Investigating on deformation characteristics and failure mechanism of bedding rock slope: Field study, long-term monitoring, and reinforcement measures. Environ. Earth Sci. 2024, 83, 45. [Google Scholar] [CrossRef]
- Lin, C.; Li, T.; Zhao, L.; Zhang, Z.; Lin, C.; Liu, X.; Niu, Z. Reinforcement effects and safety monitoring index for high steep slopes: A case study in China. Eng. Geol. 2020, 279, 105861. [Google Scholar] [CrossRef]
- Tao, Z.; Wang, Y.; Zhu, C.; Xu, H.; Li, G.; He, M. Mechanical evolution of constant resistance and large deformation anchor cables and their application in landslide monitoring. Bull. Eng. Geol. Environ. 2019, 78, 4787–4803. [Google Scholar] [CrossRef]
- Sun, L.; Grasselli, G.; Liu, Q.; Tang, X.; Abdelaziz, A. The role of discontinuities in rock slope stability: Insights from a combined finite-discrete element simulation. Comput. Geotech. 2022, 147, 104788. [Google Scholar] [CrossRef]
- Tao, Z.; Zhang, T.; Zhu, D.; Gong, W.; He, M. Physical modeling test on deformation and failure of rock slope with new support system. Adv. Civ. Eng. 2020, 2020, 8825220. [Google Scholar] [CrossRef]
- Liu, S.Y.; Shao, L.T.; Li, H.J. Slope stability analysis using the limit equilibrium method and two finite element methods. Comput. Geotech. 2015, 63, 291–298. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Fenton, G.A. Probabilistic slope stability analysis by finite elements. J. Geotech. Geoenviron. Eng. 2004, 130, 507–518. [Google Scholar] [CrossRef]
- Bao, Y.; Li, Y.; Zhang, Y.; Yan, J.; Zhou, X.; Zhang, X. Investigation of the role of crown crack in cohesive soil slope and its effect on slope stability based on the extended finite element method. Nat. Hazards 2022, 110, 295–314. [Google Scholar] [CrossRef]
- Jing, L. A review of techniques, advances and outstanding issues in numerical modelling for rock mechanics and rock engineering. Int. J. Rock Mech. Min. Sci. 2003, 40, 283–353. [Google Scholar] [CrossRef]
- Nguyen, P.M.V.; Marciniak, M. Stochastic rock slope stability analysis: Open pit case study with adjacent block caving. Geotech. Geol. Eng. 2024, 42, 5827–5845. [Google Scholar] [CrossRef]
- Yang, H.; Zhang, L. Bayesian back analysis of unsaturated hydraulic parameters for rainfall-induced slope failure: A review. Earth-Sci. Rev. 2024, 251, 104714. [Google Scholar] [CrossRef]
- Li, T.; Liu, G.; Wang, C.; Wang, X.; Li, Y. The probability and sensitivity analysis of slope stability under seepage based on reliability theory. Geotech. Geol. Eng. 2020, 38, 3469–3479. [Google Scholar] [CrossRef]
- Kumar, A.; Pandit, B.; Tiwari, G. Reliability-based stability analysis of large rock slopes with different failure mechanisms using response surface methodology. Environ. Earth Sci. 2022, 81, 511. [Google Scholar] [CrossRef]
- Yu, J.; Zhang, Q.; Wu, C.; Jia, C. Investigation on stability of soil–rock mixture slope with discrete element method. Environ. Earth Sci. 2023, 82, 449. [Google Scholar] [CrossRef]
- Lu, Y.; Tan, Y.; Li, X. Stability analyses on slopes of clay-rock mixtures using discrete element method. Eng. Geol. 2018, 244, 116–124. [Google Scholar] [CrossRef]
- An, H.; Fan, Y.; Liu, H.; Cheng, Y.; Song, Y. The state of the art and new insight into combined finite–discrete element modelling of the entire rock slope failure process. Sustainability 2022, 14, 4896. [Google Scholar] [CrossRef]
- Holland, J.H. Adaptation in natural and artificial systems. Q. Rev. Biol. 1975, 6, 126–137. [Google Scholar]
- Goldberg, D.E.; Holland, J.H. Genetic algorithms and machine learning. Mach. Learn. 1988, 3, 95–99. [Google Scholar] [CrossRef]
- Fatty, A.; Li, A.J.; Qian, Z.G. An interpretable evolutionary extreme gradient boosting algorithm for rock slope stability assessment. Multimed. Tools Appl. 2024, 83, 46851–46874. [Google Scholar] [CrossRef]
- Katoch, S.; Chauhan, S.S.; Kumar, V. A review on genetic algorithm: Past, present, and future. Multimed. Tools Appl. 2021, 80, 8091–8126. [Google Scholar] [CrossRef]
- Soumaya, Z.; Taoufiq, B.D.; Benayad, N.; Yunus, K.; Abdelkrim, A. The detection of Parkinson disease using the genetic algorithm and SVM classifier. Appl. Acoust. 2021, 171, 107528. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, C.; Meng, F.; Liu, T.; Xia, K. Assessing the stability of rock slopes with respect to flexural toppling failure using a limit equilibrium model and genetic algorithm. Comput. Geotech. 2020, 124, 103619. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, C.; Meng, F.; Zhang, H.; Xia, K.; Chen, X. Assessing the stability of rock slopes with respect to block-flexure toppling failure using a force-transfer model and genetic algorithm. Rock Mech. Rock Eng. 2020, 53, 3433–3445. [Google Scholar] [CrossRef]
- Xu, J.; Du, X. Seismic stability of 3D rock slopes based on a multi-cone failure mechanism. Rock Mech. Rock Eng. 2023, 56, 1595–1605. [Google Scholar] [CrossRef]
- He, B.; Du, X.; Bai, M.; Yang, J.; Ma, D. Inverse analysis of geotechnical parameters using an improved version of non-dominated sorting genetic algorithm II. Comput. Geotech. 2024, 171, 106416. [Google Scholar] [CrossRef]
- SL/T 212-2020; Technical Specification for Hydraulic Prestressed Anchorage. China Water & Power Press: Beijing, China, 2020.

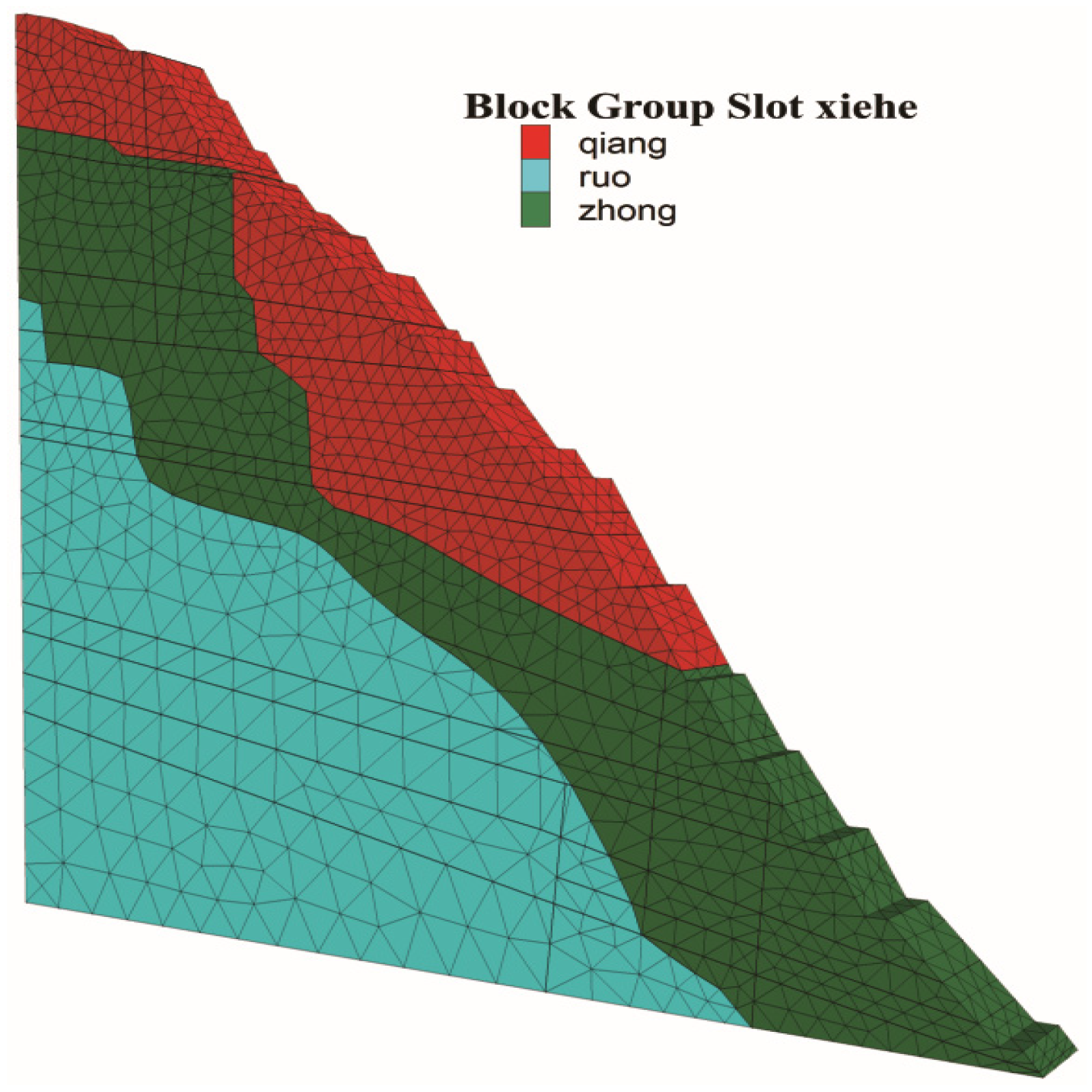
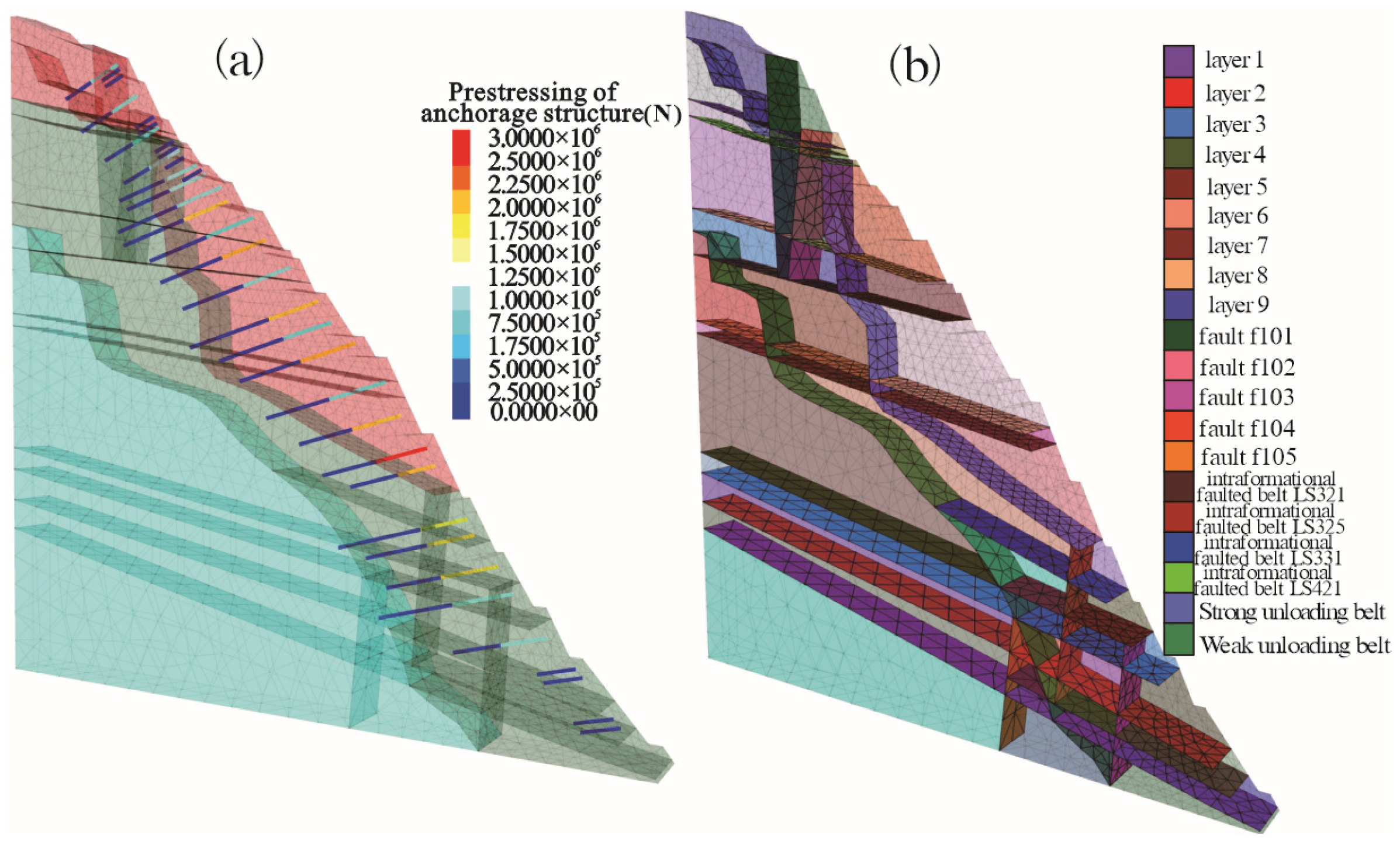
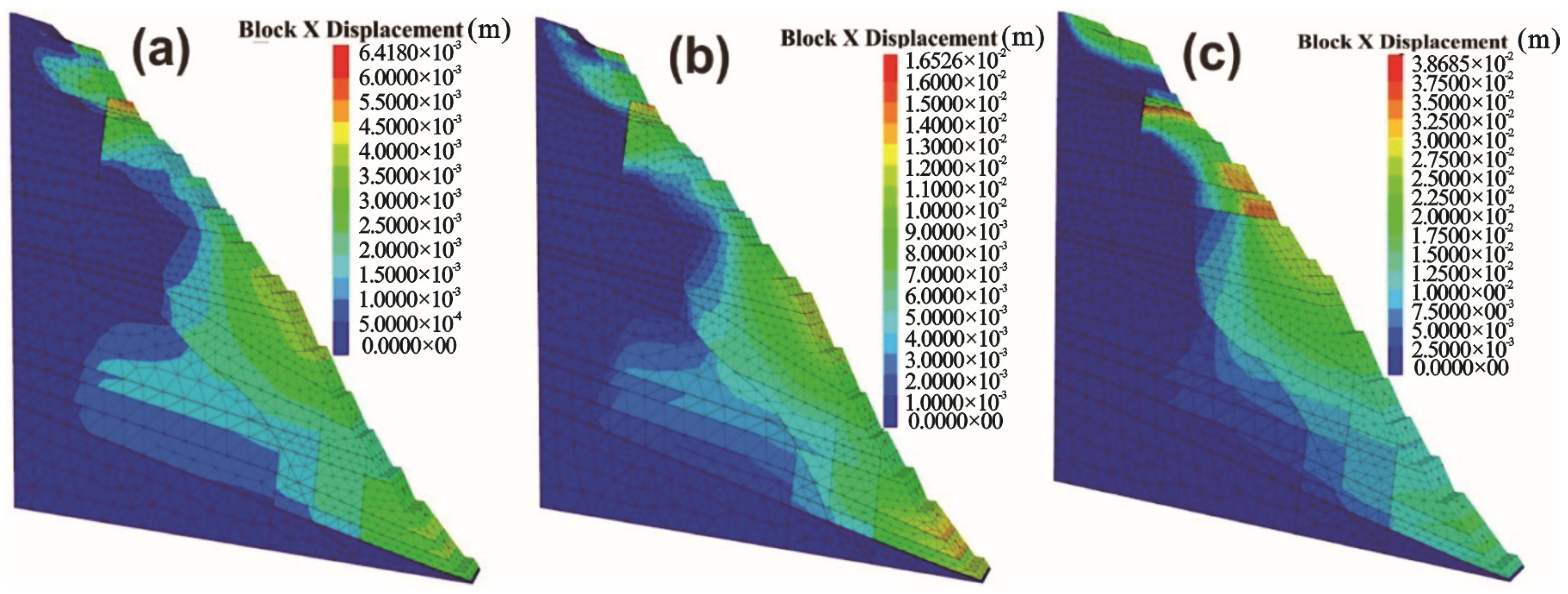
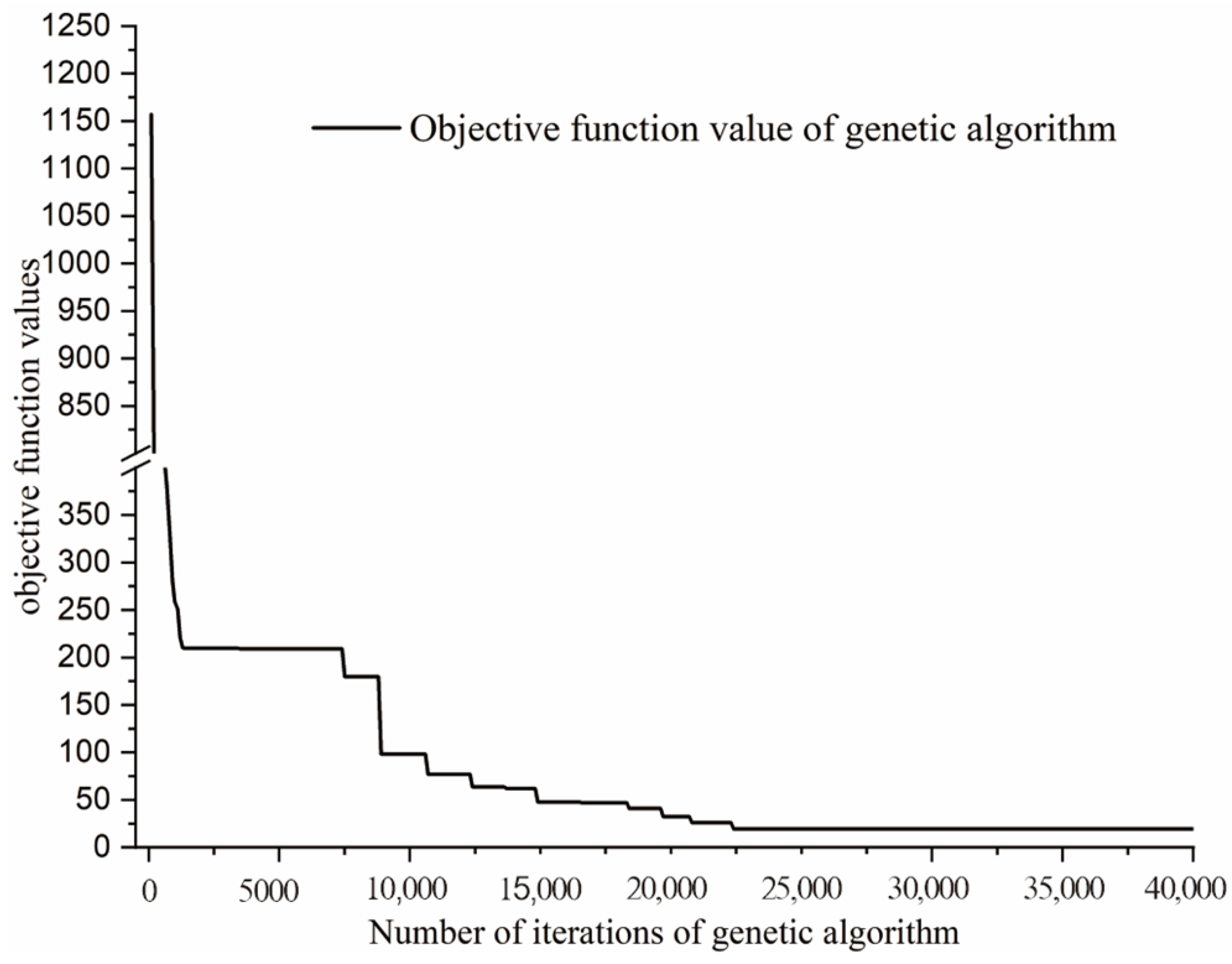


| Evaluation Method of Rock Slope Anchorage Systems Stability | Research Content | Reference |
|---|---|---|
| Reliability Theory | Applied reliability theory to calculate the probability of rock slope failure and assessed the stability of high slopes reinforced with anchorage. | Bian [7] |
| Proposed a stability evaluation method for anchored slopes based on system reliability analysis (SRA), considering the effects of local failures in anchors and piles on overall system performance. | Chen [8] | |
| Demonstrated the accuracy and effectiveness of PEMS, FORM, SORM, and MCS in addressing various reliability issues in rock slope stability assessment. | Park [9] Ahmadabadi [10] Duzgun [11] | |
| Finite Element Analysis | Developed a frictional contact interface element for potential sliding surfaces and proposed a novel numerical model for prestressed anchors to compute the slope stability factor using a direct finite element method. | Li [12] |
| Utilized the strength reduction method (SRM) in conjunction with finite element software to analyze the stability of slopes under reinforcement conditions. | Chen [13] | |
| Employed FLAC 3D numerical simulation software to evaluate the reliability of support design and slope stability based on variations in shear strain and displacement. | Liu [14] | |
| Analytical Method | Applied an improved limit equilibrium method to assess the stability of anchored slopes. | Bi [15] Deng [16] |
| Combined limit analysis, the principle of minimum potential energy, and the pseudo-dynamic method to propose a seismic stability evaluation approach that accounts for dynamic variations in anchor axial forces. | Yan [16] Sun [17] | |
| Monitoring and Detection | Proposed a comprehensive stability assessment method for anchored slopes based on residual tension variations, validated through pull-out testing. | Hara [18] |
| Conducted continuous deformation monitoring of slopes after reinforcement and analyzed the data to evaluate the stability of a layered rock slope with anchorage. | Jiang [19], Lin [20] | |
| Developed a remote monitoring and forecasting system for landslides using constant resistance to large deformation (CRLD) anchors, aimed at predicting the stability of rock slope anchoring systems. | Tao [21] | |
| Analyzed slope stability by monitoring structural force variations in anchor rods and cables during and after excavation processes. | Liu [14] | |
| Model Testing | Conducted laboratory physical model experiments on collapse control and monitoring, investigating failure characteristics and the effectiveness of energy-absorbing reinforcement measures. | Tao [23] |
| Test Point No | Pressure (MPa) | Generalized Kelvin Model Parameters | |||
|---|---|---|---|---|---|
| E1 (GPa) | E2 (GPa) | H (GPa·h) | r2 | ||
| C36-101 | 11.23 | 13 | 98 | 1380 | 95% |
| C36-102 | 12.06 | 18 | 173 | 8951 | 96% |
| C36-103 | 11.38 | 7 | 56 | 550 | 94.2% |
| C36-201 | 11.38 | 15 | 79 | 5000 | 96.2% |
| C36-202 | 12.06 | 10 | 94 | 3840 | 90% |
| C36-203 | 12.14 | 8 | 49 | 6900 | 97.4% |
| E1 | E2 | η |
|---|---|---|
| 7 GPa~18 GPa | 49 GPa~173 GPa | 550 GPa·h ~8951 GPa·h |
| Cross-sectional Area (mm2) | 140 |
| Elastic Modulus (GPa) | 190 |
| Strength Grade (MPa) | 1860 |
| Ultimate Load of Anchor Cable (kN) | 265 |
| Strain Limit of Anchor Cable | 0.035 |
| Grout Strength (MPa) | 30 |
| Lithology | Rock Mass Category | Lithological Layer | Weathering and Unloading State | Deformation Modulus (GPa) | Poisson’s Ratio | Density (kN/m3) | Shear Strength Parameters | |
|---|---|---|---|---|---|---|---|---|
| f | c (MPa) | |||||||
| Basalt | IV1 | P2β2~P2β6 | slight weathering, strong unloading. | 3 | 0.32 | 25.8 | 0.55 | 0.40 |
| III2 | columnar-jointed basalt, agglomerate lava | slight weathering, slight unloading. | 7 | 0.27 | 27.3 | 0.90 | 0.75 | |
| P2β2~P2β6 (excluding columnar-jointed basalt and agglomerate lava) | slight weathering, slight unloading | |||||||
| III1 | columnar-jointed basalt, agglomerate lava | slightly fresh, no unloading | 9 | 0.24 | 27.2 | 1.1 | 1.1 | |
| P2β2~P2β6 (excluding columnar-jointed basalt and agglomerate lava) | slight weathering, slight unloading | |||||||
| II | 2β2~P2β6 (excluding columnar-jointed basalt and agglomerate lava) | slightly fresh, no unloading | 17 | 0.22 | 28.7 | 1.3 | 1.4 | |
| Type | Identification Number | Deformation Modulus (GPa) | Shear Strength Parameters | |
|---|---|---|---|---|
| f | c (MPa) | |||
| Shear Zone | LS321 | 3.75 | 0.40 | 0.06 |
| LS331 | 3.85 | 0.38 | 0.07 | |
| LS325 | 3.8 | 0.38 | 0.06 | |
| LS421 | 4.14 | 0.45 | 0.07 | |
| Fault | f101 | 2.45 | 0.43 | 0.08 |
| f102 | 2.46 | 0.43 | 0.08 | |
| f143 | 3.65 | 0.35 | 0.05 | |
| f141 | 3.64 | 0.35 | 0.05 | |
| f106 | 4.35 | 0.35 | 0.05 | |
| Objective Function | Objective Dimension | Population Size | Maximum Number of Iterations | Mutation Probability |
|---|---|---|---|---|
| Difference between the numerical model slope monitoring data and actual monitoring data | 3 | 30 | 100 | 0.01 |
| Upper Limit of Creep Parameters | Lower Limit of Creep Parameters | Precision |
|---|---|---|
| 7 | 18 | 0.1 |
| 49 | 173 | 1 |
| 550 | 8951 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, P.; Zeng, B.; Pan, Y. Stability Evaluation Method for Rock Slope-Anchorage Systems Based on Genetic Algorithms and Discrete Element Analysis. Appl. Sci. 2025, 15, 5057. https://doi.org/10.3390/app15095057
Xia P, Zeng B, Pan Y. Stability Evaluation Method for Rock Slope-Anchorage Systems Based on Genetic Algorithms and Discrete Element Analysis. Applied Sciences. 2025; 15(9):5057. https://doi.org/10.3390/app15095057
Chicago/Turabian StyleXia, Peng, Bowen Zeng, and Yiheng Pan. 2025. "Stability Evaluation Method for Rock Slope-Anchorage Systems Based on Genetic Algorithms and Discrete Element Analysis" Applied Sciences 15, no. 9: 5057. https://doi.org/10.3390/app15095057
APA StyleXia, P., Zeng, B., & Pan, Y. (2025). Stability Evaluation Method for Rock Slope-Anchorage Systems Based on Genetic Algorithms and Discrete Element Analysis. Applied Sciences, 15(9), 5057. https://doi.org/10.3390/app15095057






