Analysis and Design of a Transient-State Resonant Converter Used as a Frequency Multiplier
Abstract
Featured Application
Abstract
1. Introduction
2. Resonant Converter Transient
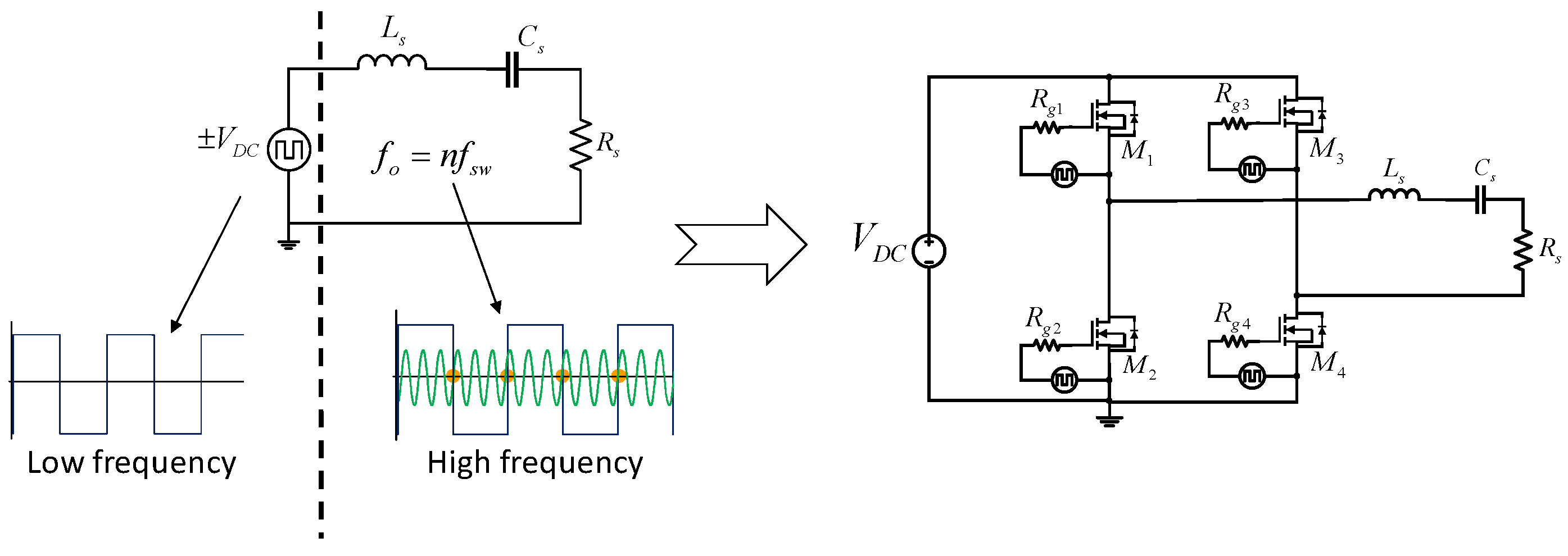
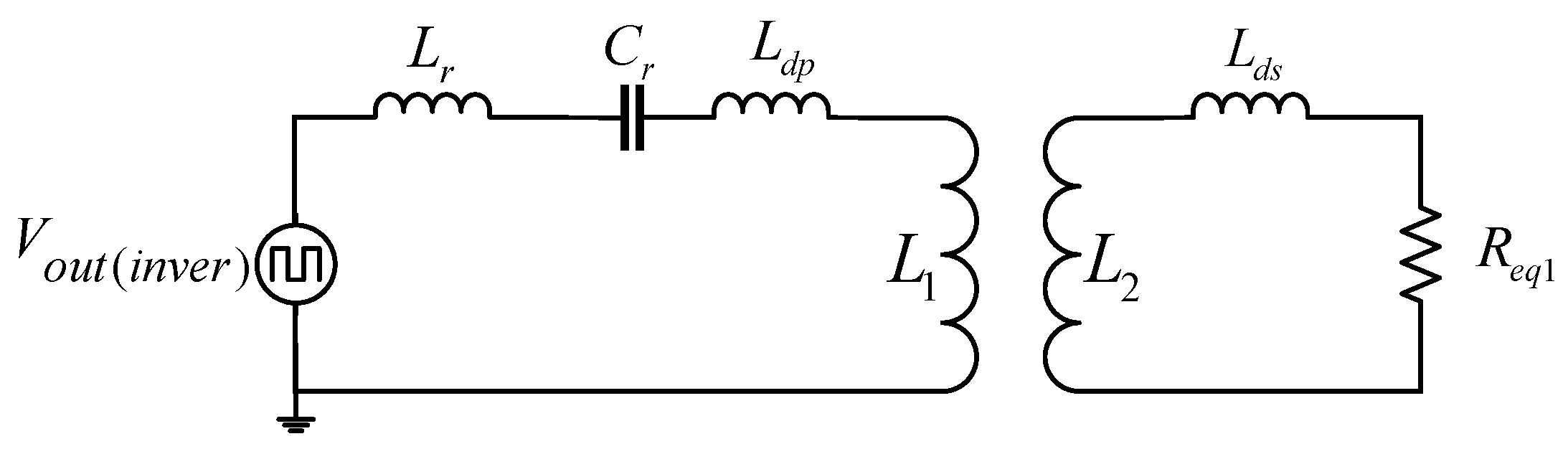
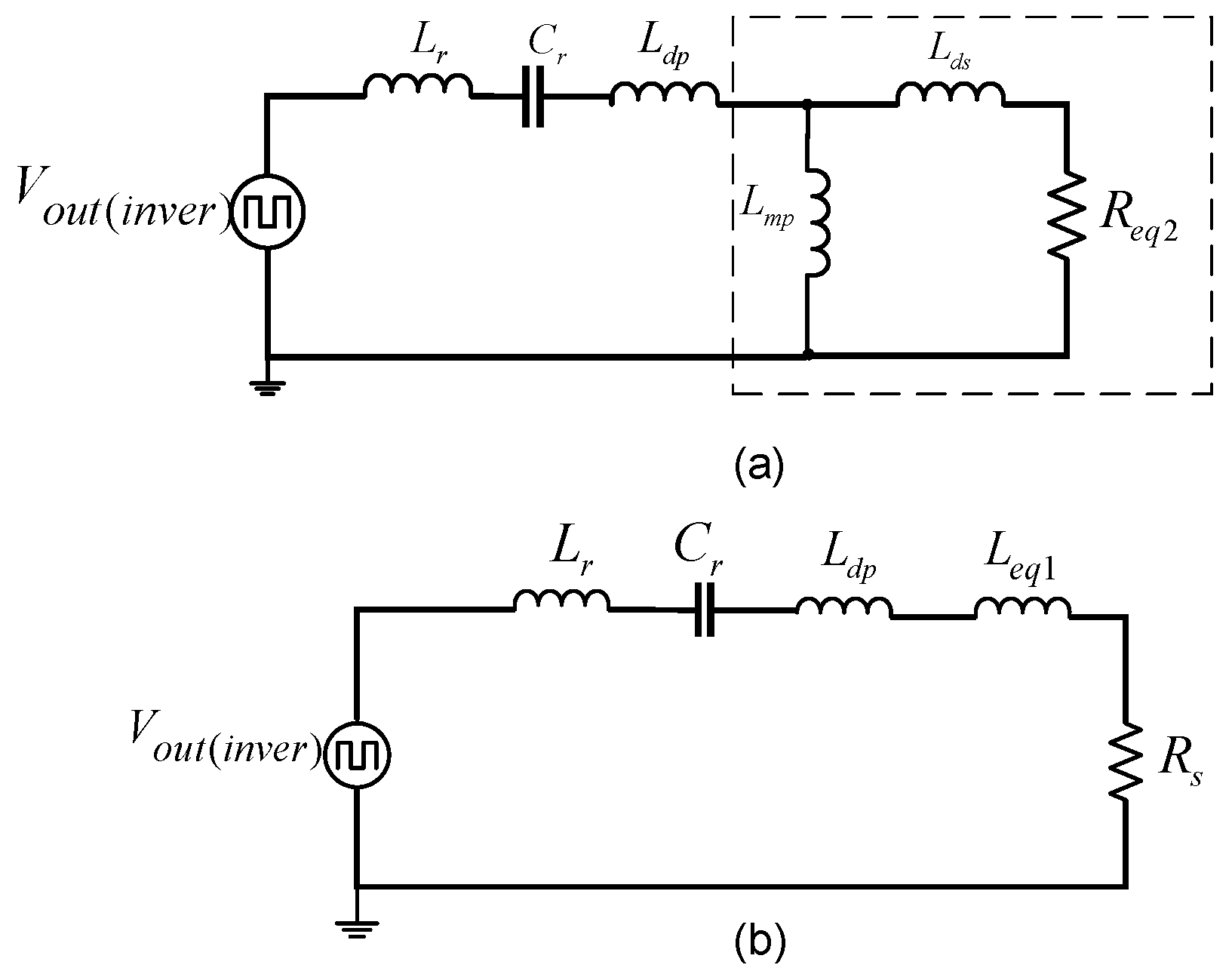
2.1. Determination of Equivalent Circuits for Transient Design of the Resonant Converter
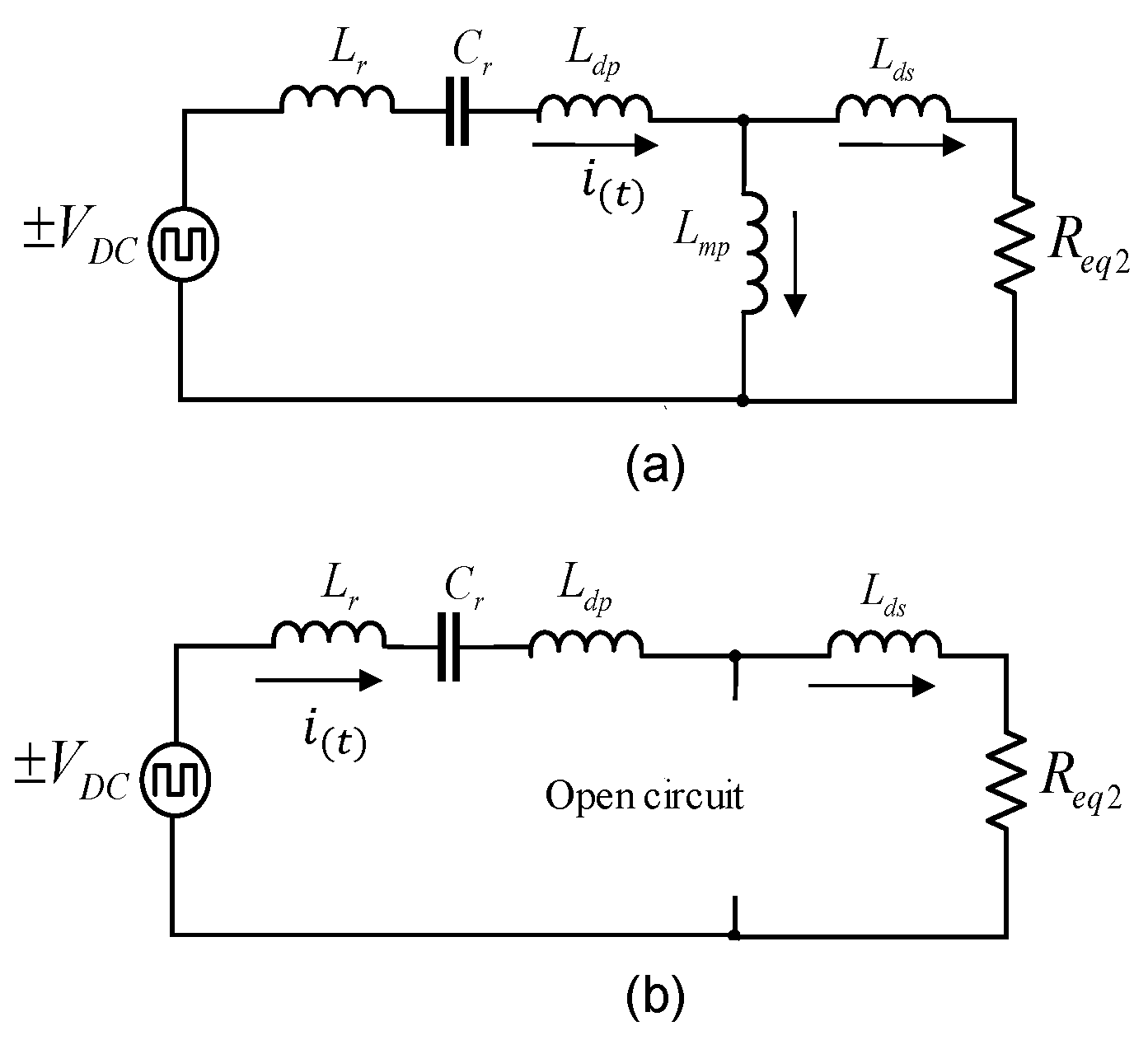
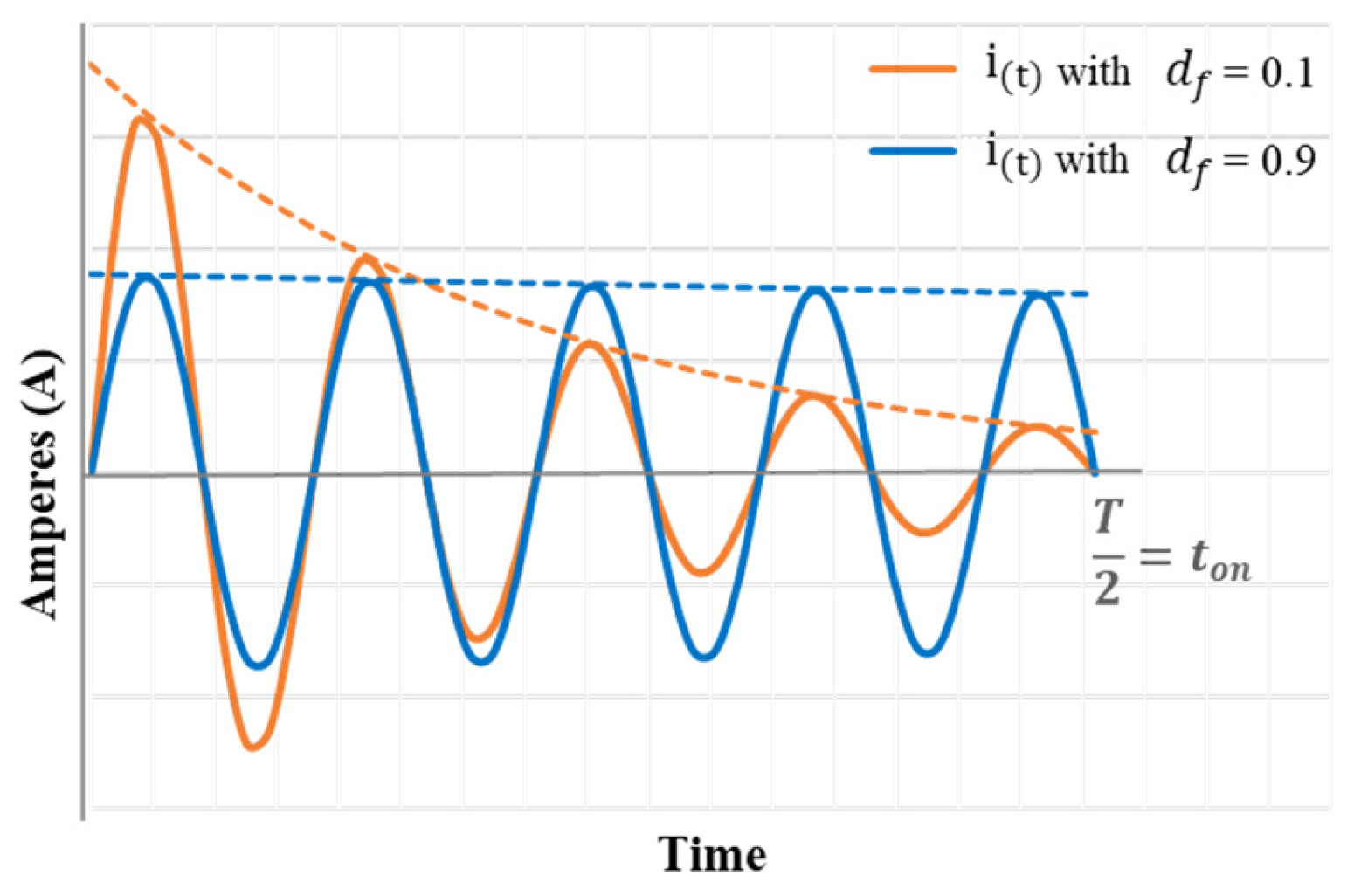
2.2. Optimization Constant “g” for Satisfying the Series Condition of the RLC Equivalent Circuit
2.3. Transient Operation of the RLC Equivalent Circuit in Series of the Resonant Converter
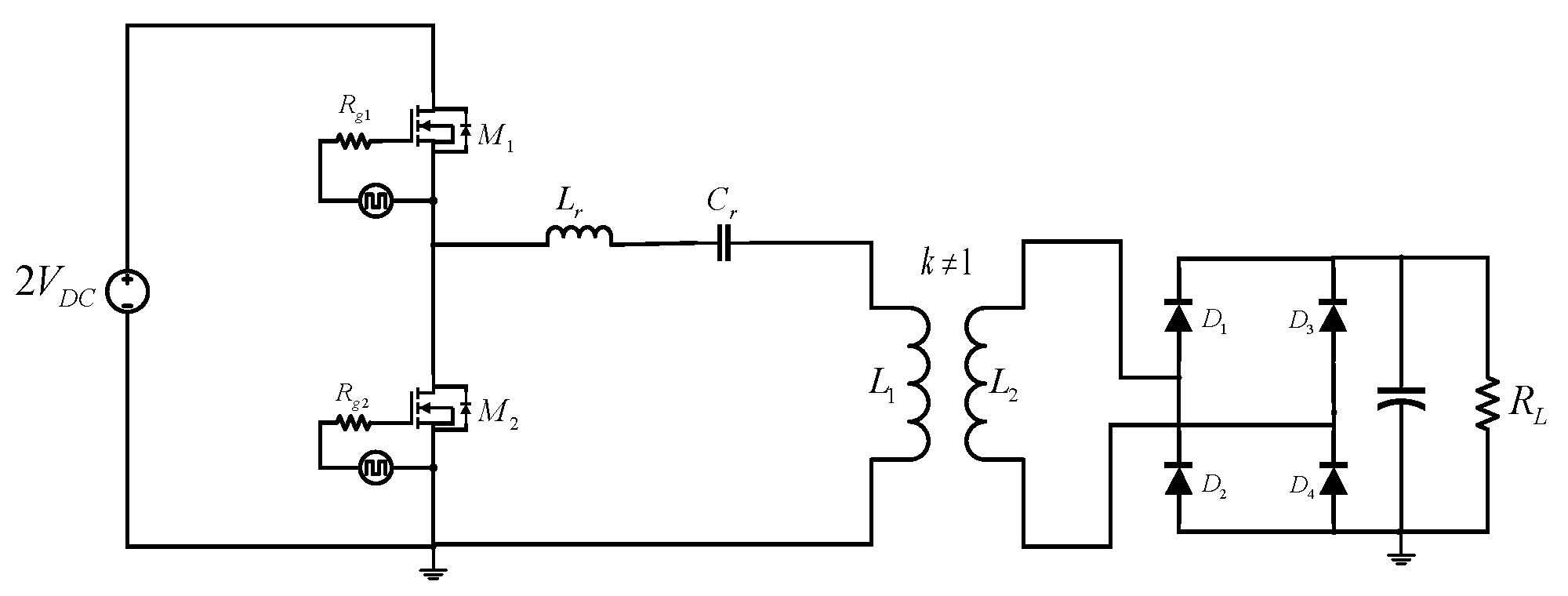
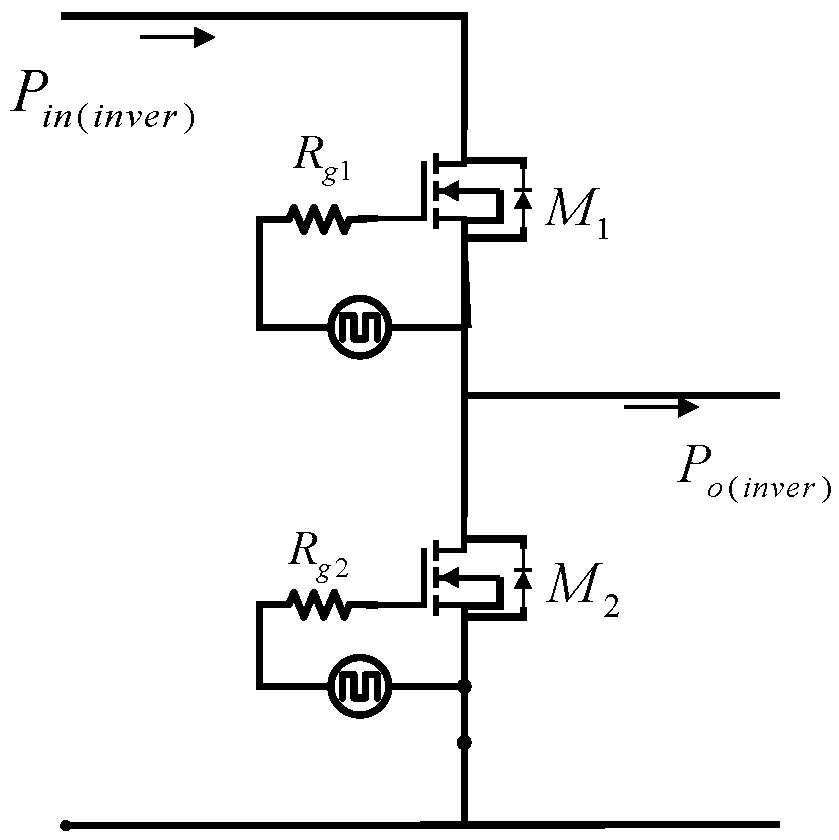
2.4. Reduction of Components in the Switching Stage
3. Design Methodology and Experimental Testing
3.1. Design Methodology and Establishment of Operating Parameters
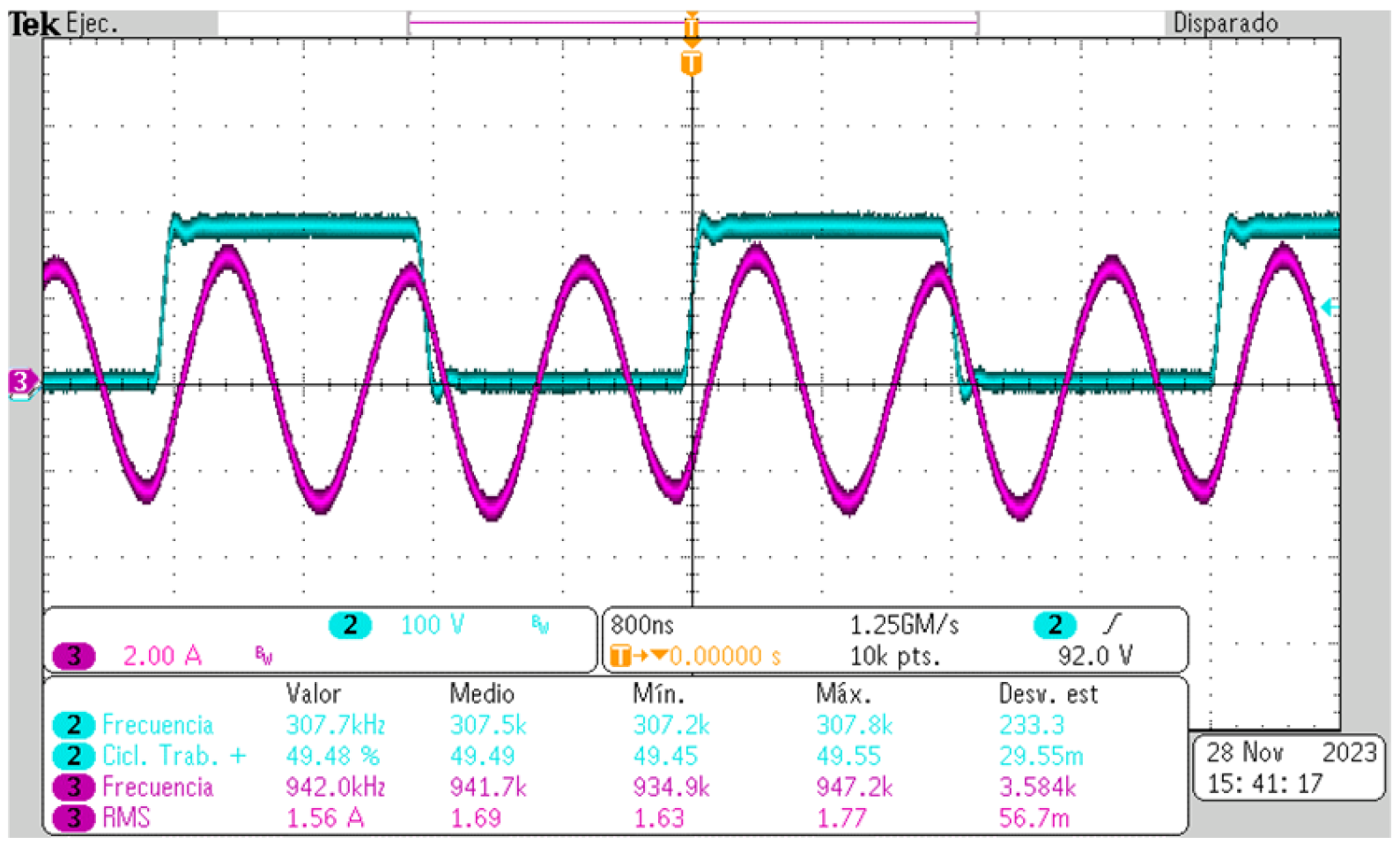
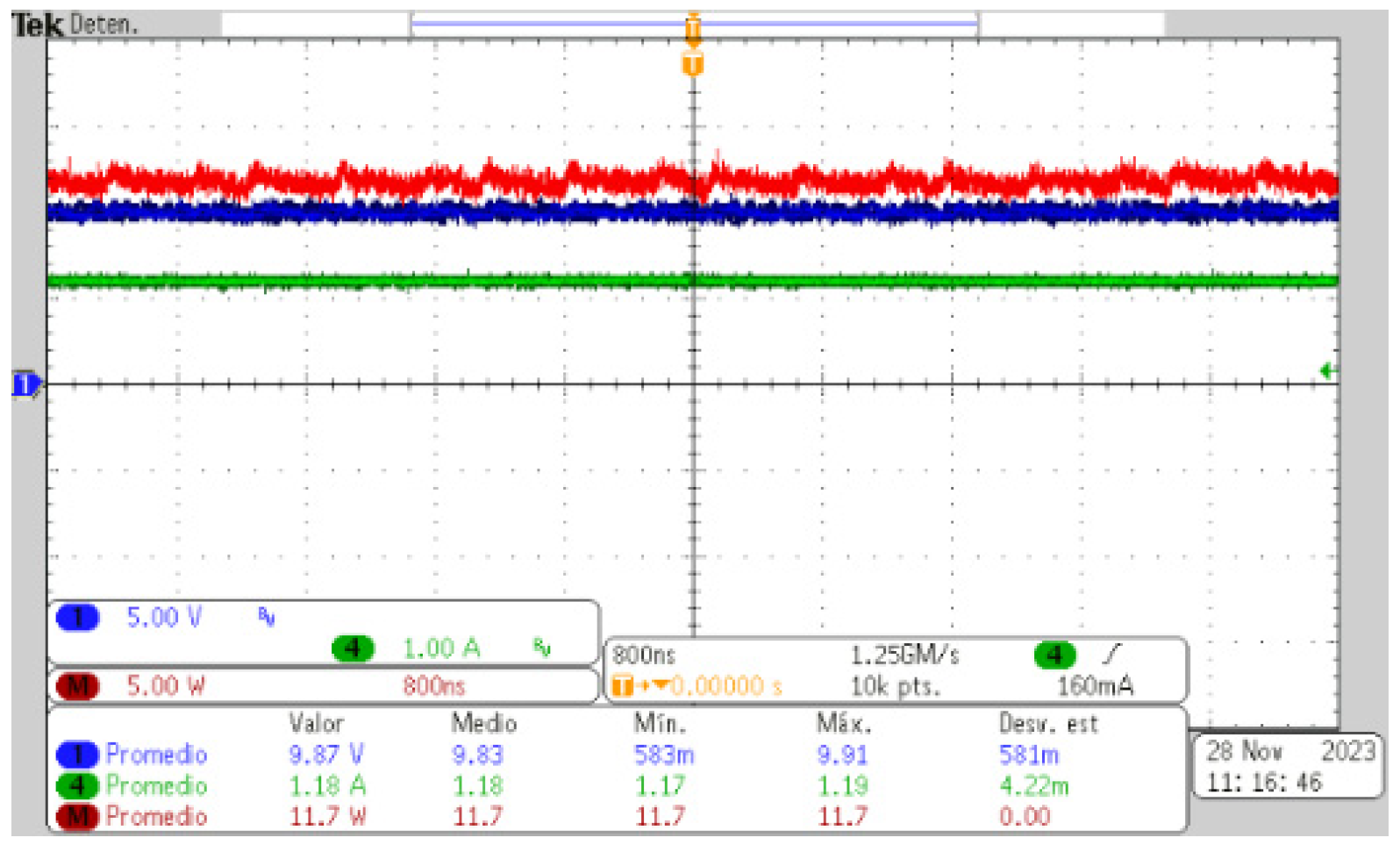
3.2. Development of Experimental Tests
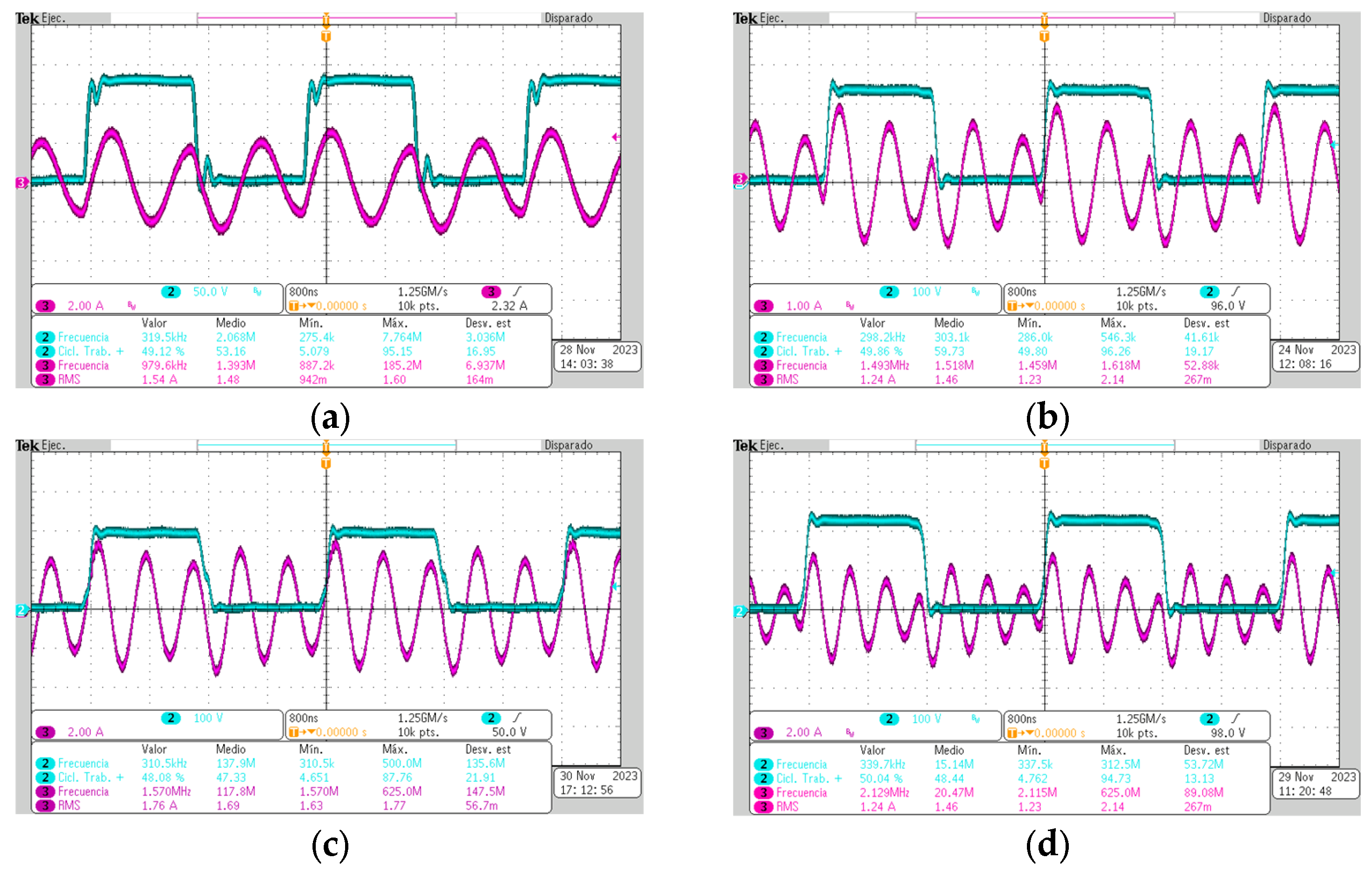
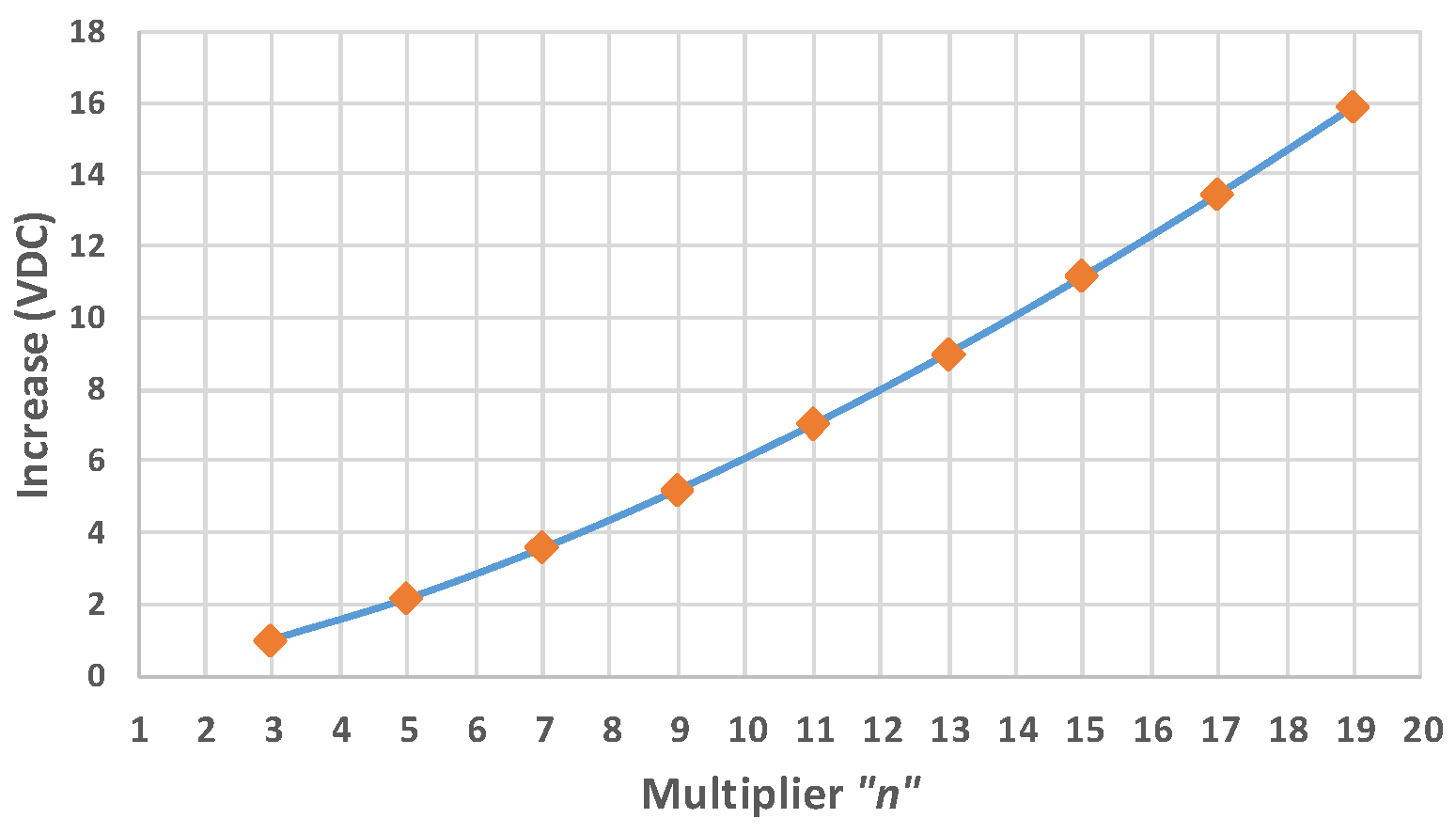
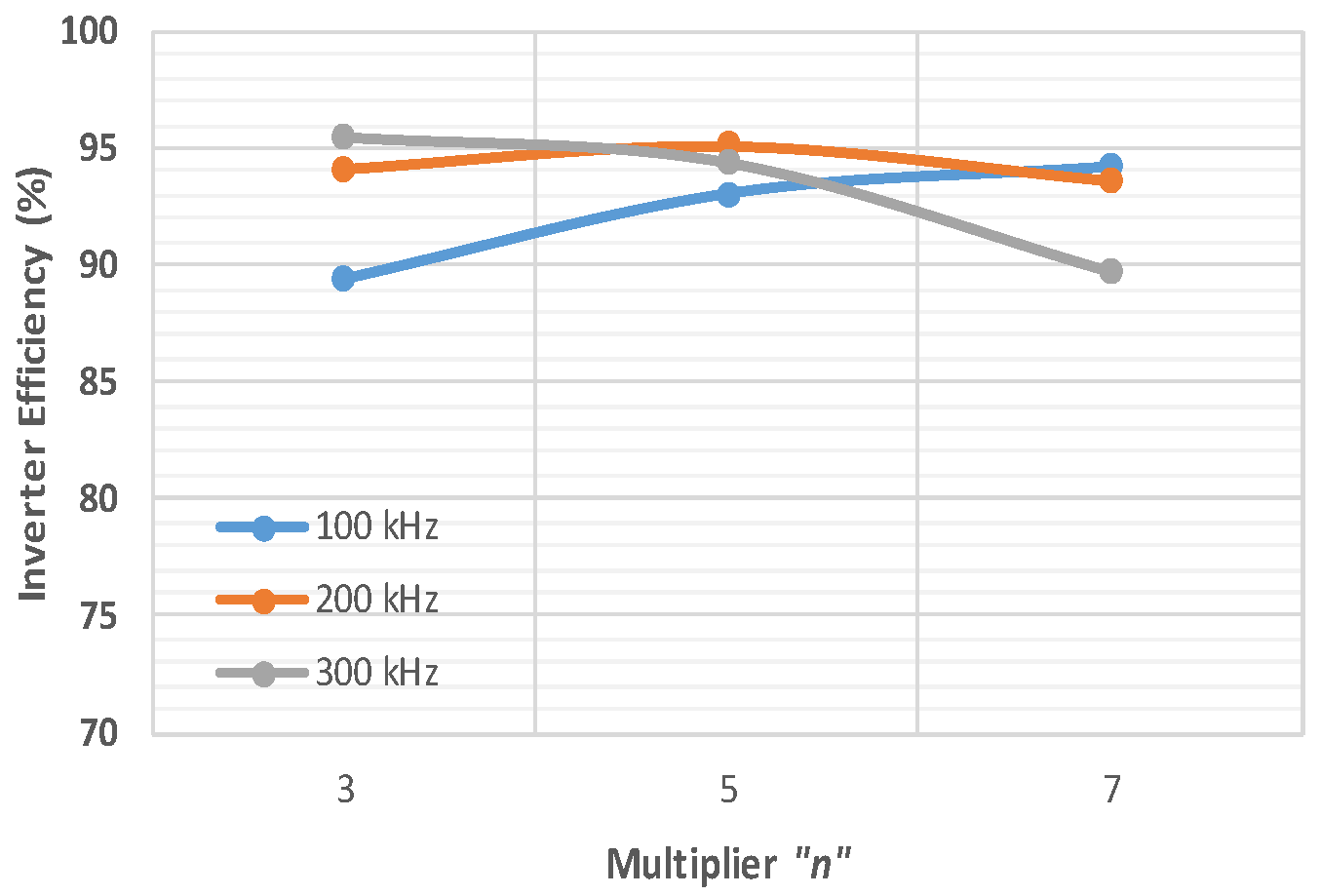
3.3. Implications on the Efficiency of the Resonant Converter Acting as a Frequency Multiplier
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Blaabjerg, F.; Yang, Y.; Kim, K.A.; Rodriguez, J. Power electronics technology for large-scale renewable energy generation. Proc. IEEE 2023, 111, 335–355. [Google Scholar] [CrossRef]
- Erickson, R.W.; Maksimovic, D. Fundamentals of Power Electronics; Springer Science & Business Media: Berlin, Germany, 2007. [Google Scholar]
- Ponce-Silva, M.; García-García, A.R.; Arau, J.; Lara-Reyes, J.; Cortés-García, C. Inductive Compensation of an Open-Loop IPT Circuit: Analysis and Design. Inventions 2023, 8, 104. [Google Scholar] [CrossRef]
- Elezab, A.; Zayed, O.; Abuelnaga, A.; Narimani, M. High efficiency LLC resonant converter with wide output range of 200–1000 V for DC-connected EVS ultra-fast charging stations. IEEE Access 2023, 11, 33037–33048. [Google Scholar] [CrossRef]
- Zhou, Y.; Choi, J. Design of high-frequency, load-independent resonant inverter using phase-shift control method. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), Orlando, FL, USA, 19–23 March 2023; pp. 1070–1076. [Google Scholar]
- Kazimierczuk, M.K.; Czarkowski, D. Resonant Power Converters; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Lara-Reyes, J.; Ponce-Silva, M.; Cortés-García, C.; Lozoya-Ponce, R.E.; Parrilla-Rubio, S.M.; García-García, A.R. High-Power-Factor LC Series Resonant Converter Operating Off-Resonance with Inductors Elaborated with a Composed Material of Resin/Iron Powder. Electronics 2022, 11, 3761. [Google Scholar] [CrossRef]
- Lopušina, I.; Bouvier, Y.E.; Grbović, P.J. Steady-State and Transient Modeling of the Series Resonant Balancing Converter. IEEE Open J. Power Electron. 2023, 4, 900–912. [Google Scholar] [CrossRef]
- Maji, S.; Hou, Y.; Etta, D.; Afridi, K.K. A Frequency Multiplier Architecture for High-Power High-Frequency Capacitive Wireless Charging Systems. In Proceedings of the Energy Conversion Congress and Exposition (ECCE), Nashville, TN, USA, 29 October–2 November 2023; pp. 1672–1677. [Google Scholar]
- Yao, J.; Li, M.; Yu, X.; Li, T.; Dai, Z. Analysis and Design of Class E Frequency Multiplier With Shunt Linear and Nonlinear Capacitances at Any Duty Cycle. IEEE Trans. Circuits Syst. II Express Briefs 2021, 69, 1827–1831. [Google Scholar] [CrossRef]
- Kim, T.; Jang, M.; Agelidis, V.G. Ultra-fast MHz range driving circuit for SiC MOSFET using frequency multiplier with eGaN FET. IET Power Electron. 2016, 9, 2085–2094. [Google Scholar] [CrossRef]
- Shinoda, K.; Fujii, M.; Matsuo, M.; Suetsuga, T.; Mori, S. Idealized operation of Class DE frequency multipliers. In Proceedings of the IEEE International Symposium on Circuits and Systems (ISCAS), Atlanta, Georgia, 12–15 May 1996; Volume 1, pp. 581–584. [Google Scholar]
- Zaheri, R.; Moghani, J.S. A Modular Two-Stage High Step-Down DC-DC Converter Using Frequency Multiplier Circuit for Datacenter Applications. In Proceedings of the IEEE 12th Power Electronics, Drive Systems, and Technologies Conference (PEDSTC), Tabriz, Iran, 2–4 February 2021; pp. 1–5. [Google Scholar]
- Oh, K.-I.; Ko, G.-H.; Kim, G.-S.; Kim, J.-G.; Baek, D. A 17.8–34.8 GHz (64.6%) Locking Range Current-Reuse Injection-Locked Frequency Multiplier with Dual Injection Technique. Electronics 2021, 10, 1122. [Google Scholar] [CrossRef]
- Kwiatkowski, P.; Knioła, M.; Szczepaniak, Z. Microwave Frequency Doubler with Improved Stabilization of Output Power. Sensors 2023, 23, 3598. [Google Scholar] [CrossRef] [PubMed]
- Li, T.; Li, M.; Dai, Z.; Pang, J.; Shi, W.; Cao, H. Analysis and Design of the Subnominal Operation of Voltage-Source Parallel Resonant Class E Frequency Multiplier. In Proceedings of the IEEE 6th International Conference on Energy Conservation and Efficiency (ICECE), Lahore, Pakistan, 15–16 March 2023; pp. 1–5. [Google Scholar]
- Cai, H.; Shi, L.; Li, Y. Harmonic-based phase-shifted control of inductively coupled power transfer. IEEE Trans. Power Electron. 2013, 29, 594–602. [Google Scholar] [CrossRef]
- Moradi, A.; Tahami, F.; GhaziMoghadam, M.A. Wireless power transfer using selected harmonic resonance mode. IEEE Trans. Transp. Electrif. 2017, 3, 508–519. [Google Scholar] [CrossRef]
- Zeng, H.; Yang, S.; Peng, F.Z. Design consideration and comparison of wireless power transfer via harmonic current for PHEV and EV wireless charging. IEEE Trans. Power Electron. 2016, 32, 5943–5952. [Google Scholar] [CrossRef]
- Lara-Reyes, J.; Ponce-Silva, M.; Hernández-González, L.; DeLeón-Aldaco, S.E.; Cortés-García, C.; Ramirez-Hernandez, J. Series RLC Resonant Circuit Used as Frequency Multiplier. Energies 2022, 15, 9334. [Google Scholar] [CrossRef]



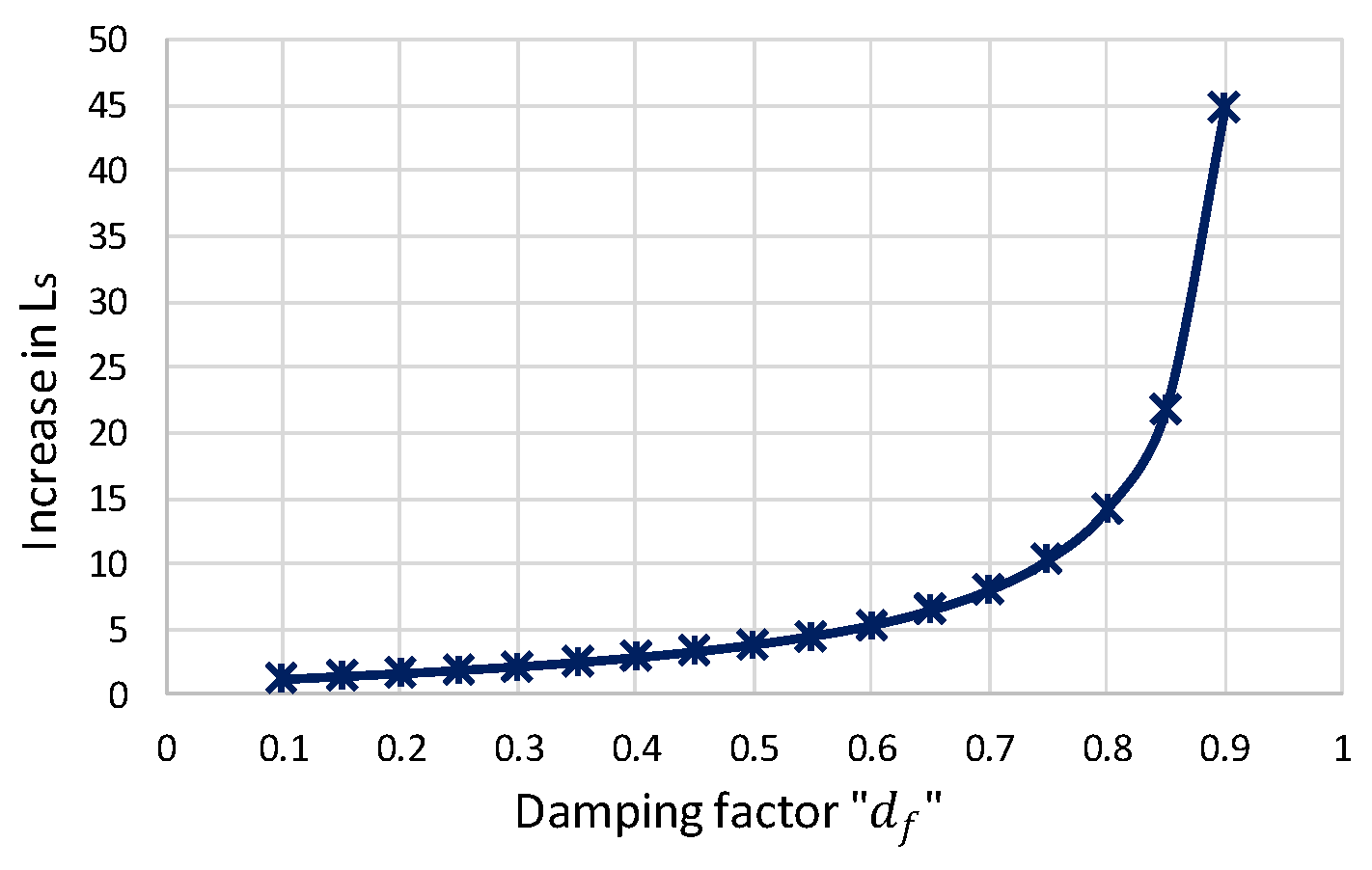

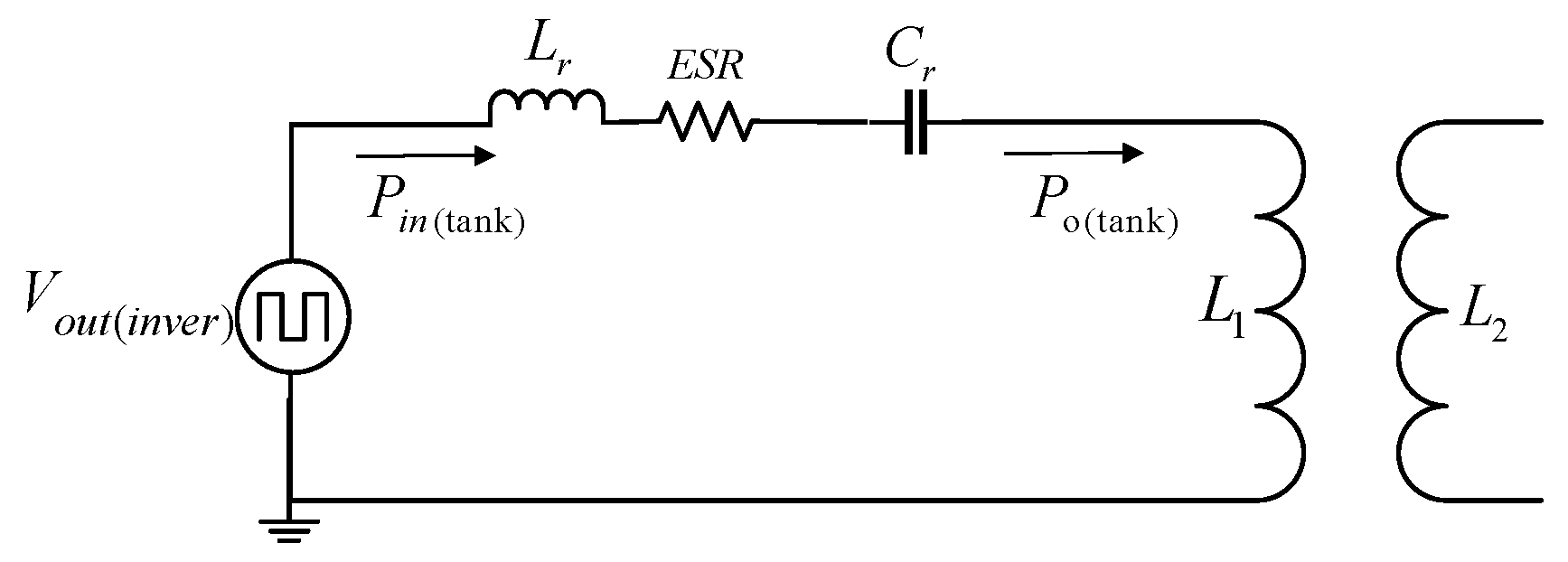
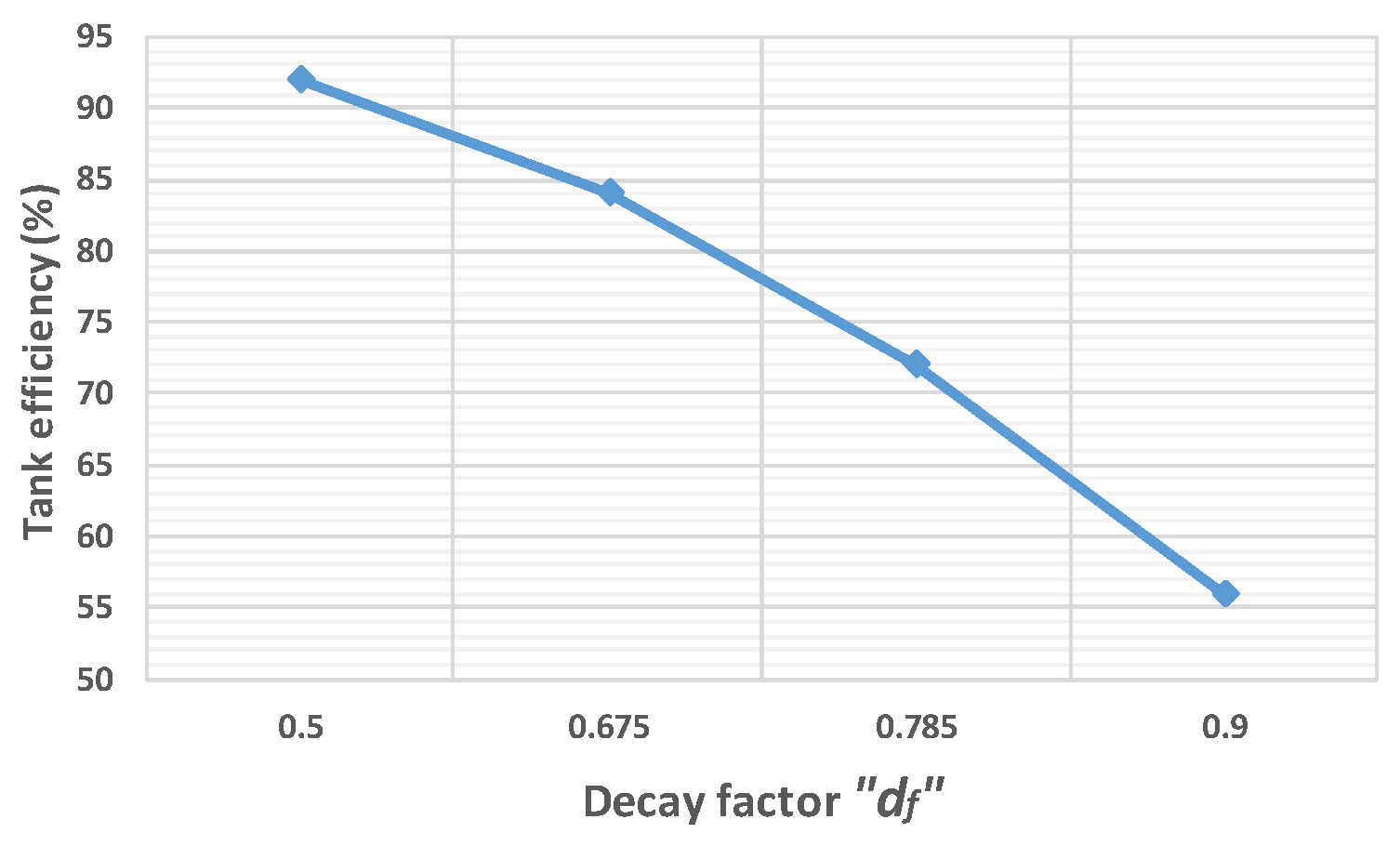
| Parameter | Description | Value |
|---|---|---|
| Po | Average output power | 12 W |
| Vout | RMS voltage at the load resistor | 10 V |
| fsw | Inverter switching frequency | 300 kHz |
| D | Duty cycle | 50% |
| n | Frequency multiplier | 3 |
| df | Damping factor | 0.9 |
| Parameter | Description | Equation | Value |
|---|---|---|---|
| fo | Frequency of current in the resonant tank. | 900 kHz | |
| ωo | Angular frequency in the resonant tank. | 5.654106 rad/s | |
| ɳrec | Rectifier efficiency. | 0.88 | |
| RL | Load at the output. | 8 Ω | |
| Req1 | Rectifier equivalent resistance. | 6.11 Ω | |
| XLds | Secondary stray reactance. | 9.52 Ω | |
| XLmp | Reactance of magnetizing inductance. | 61.18 Ω | |
| Rs | Series resistance of the equivalent circuit. | 4.54 Ω | |
| XLeq1 | Equivalent inductance reactance. | 8.63 Ω | |
| a | Constant which defines the level of underdamping of the current in the resonant tank. | 58 | |
| Ls | Series inductance of the equivalent circuit. | 23.31 µH | |
| Lr | Inductor in the resonant tank of the converter. | 20.26 µH | |
| Cr | Capacitor in the resonant tank. | 1.34 nF | |
| b | Constant that determines the RMS current in the resonant tank. | 0.426 | |
| XC | Capacitive reactance. | 131 Ω | |
| codd | Constant that determines the voltage level in the resonant tank capacitor. | 12.28 | |
| Q | Quality factor. | 29 | |
| VDC | Input voltage. | 50 V | |
| M | Gain in the resonant tank. | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lara Reyes, J.; Ponce-Silva, M.; Hernandez-Gonzalez, L.; Cortés-García, C.; Ramirez-Hernandez, J.; DeLeon-Aldaco, S.E.; Juarez-Sandoval, O.U.; Lozoya-Ponce, R.E. Analysis and Design of a Transient-State Resonant Converter Used as a Frequency Multiplier. Appl. Sci. 2025, 15, 3346. https://doi.org/10.3390/app15063346
Lara Reyes J, Ponce-Silva M, Hernandez-Gonzalez L, Cortés-García C, Ramirez-Hernandez J, DeLeon-Aldaco SE, Juarez-Sandoval OU, Lozoya-Ponce RE. Analysis and Design of a Transient-State Resonant Converter Used as a Frequency Multiplier. Applied Sciences. 2025; 15(6):3346. https://doi.org/10.3390/app15063346
Chicago/Turabian StyleLara Reyes, Josué, Mario Ponce-Silva, Leobardo Hernandez-Gonzalez, Claudia Cortés-García, Jazmin Ramirez-Hernandez, Susana E. DeLeon-Aldaco, Oswaldo Ulises Juarez-Sandoval, and Ricardo E. Lozoya-Ponce. 2025. "Analysis and Design of a Transient-State Resonant Converter Used as a Frequency Multiplier" Applied Sciences 15, no. 6: 3346. https://doi.org/10.3390/app15063346
APA StyleLara Reyes, J., Ponce-Silva, M., Hernandez-Gonzalez, L., Cortés-García, C., Ramirez-Hernandez, J., DeLeon-Aldaco, S. E., Juarez-Sandoval, O. U., & Lozoya-Ponce, R. E. (2025). Analysis and Design of a Transient-State Resonant Converter Used as a Frequency Multiplier. Applied Sciences, 15(6), 3346. https://doi.org/10.3390/app15063346











