Biomechanical Evaluation of Attachment and Trimline Modifications in Maxillary Molar Distalization Using Clear Aligners
Abstract
Featured Application
Abstract
1. Introduction
2. Materials and Methods
2.1. Simulation Model Development
2.2. Meshing and Analysis Settings
2.3. Loading and Boundary Conditions
- X-axis: Bucco-palatal direction (+palatal, −buccal);
- Y-axis: Mesio-distal direction (+distal, −mesial);
- Z-axis: Occluso-gingival direction (+gingival, −occlusal).
- Crown landmarks: Mesiobuccal cusp tip (MBCT), distobuccal cusp tip (DBCT), mesiopalatal cusp tip (MPCT), and distopalatal cusp tip (DPCT).
- Root landmarks: Mesiobuccal root apex (MBRA), distobuccal root apex (DBRA), and palatal root apex (PRA).
2.4. Ethical Approval
3. Results
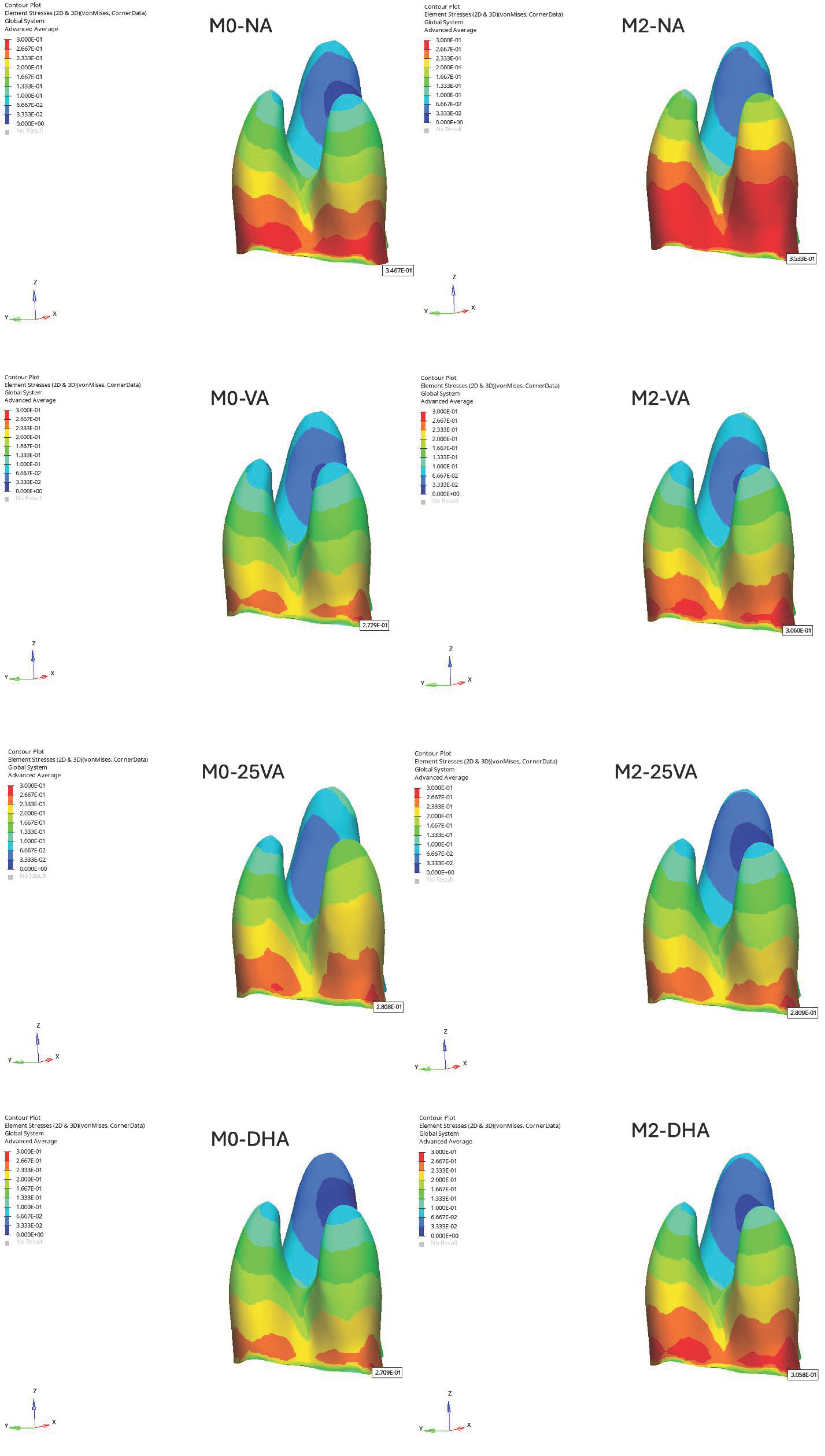
3.1. Stress Distributions in the Periodontal Ligament
3.2. Displacement Along the X-Axis (Bucco-Palatal Direction)
3.3. Displacement Along the Y-Axis (Mesio-Distal Direction)
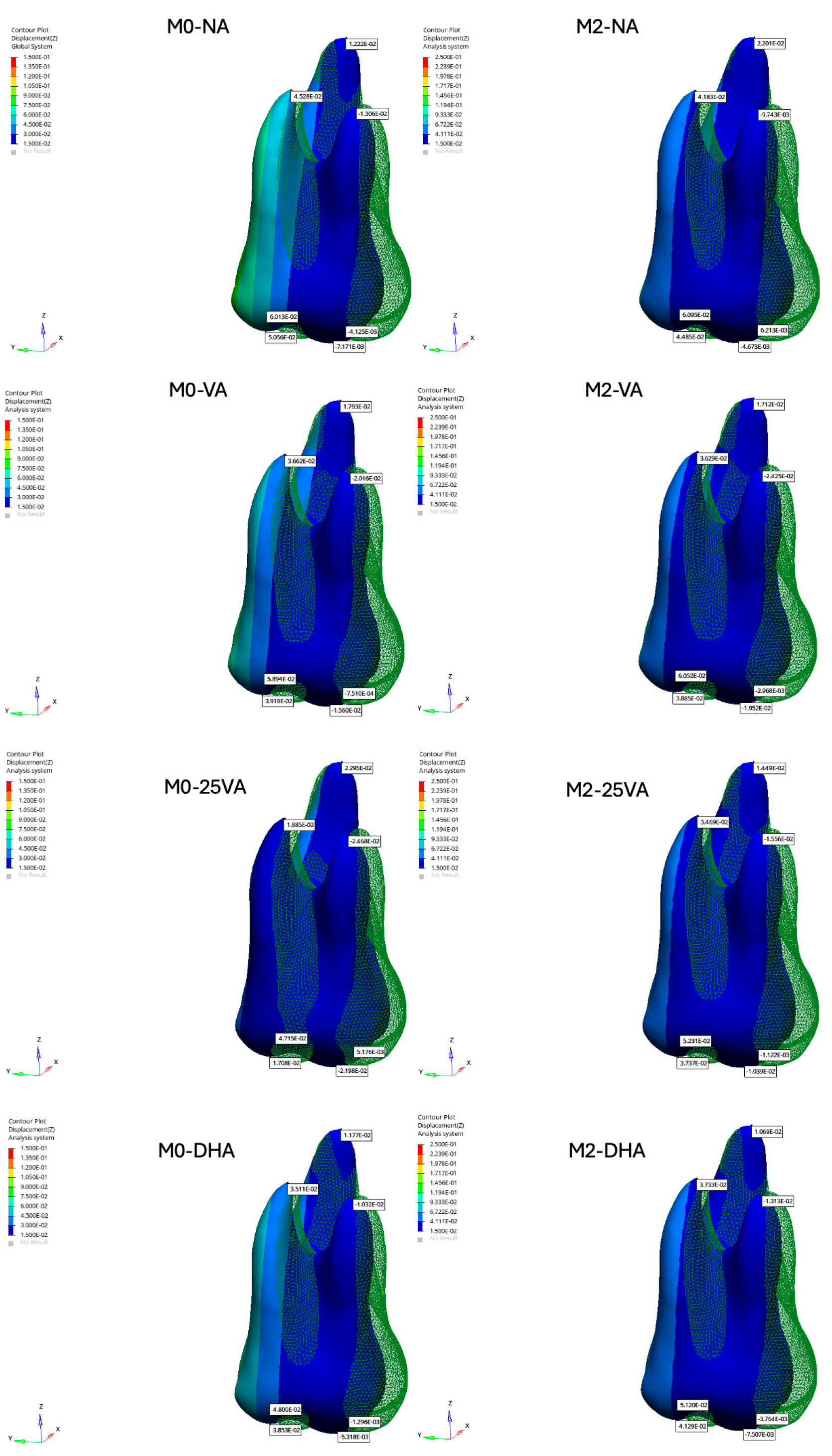
3.4. Displacement Along the Z-Axis (Occluso-Gingival Direction)
3.5. Rotational Displacement
4. Discussion
4.1. Stress Distribution and Attachment Effects
4.2. Displacement Patterns
4.3. Clinical Implications
4.4. Limitations and Future Directions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FEA | Finite element analysis |
| NA | No attachment |
| VA | Vertical attachment |
| 25VA | 25° angled vertical attachment |
| DHA | Double horizontal attachment |
| M0 | Model with scalloped trimline (0 mm) |
| M2 | Model with extended trimline (2 mm) |
| MBCT | Mesiobuccal cusp tip |
| DBCT | Distobuccal cusp tip |
| MPCT | Mesiopalatal cusp tip |
| DPCT | Distopalatal cusp tip |
| MBRA | Mesiobuccal root apex |
| DBRA | Distobuccal root apex |
| PRA | Palatal root apex |
Appendix A
| Total # of Nodes | Total # of Elements | |
|---|---|---|
| Model 1 | 164,435 | 598,335 |
| Model 2 | 198,100 | 722,605 |
| Model 3 | 200,102 | 728,318 |
| Model 4 | 204,528 | 741,683 |
| Model 5 | 199,771 | 726,917 |
| Model 6 | 200,656 | 727,333 |
| Model 7 | 201,307 | 732,212 |
| Model 8 | 205,092 | 743,064 |
References
- Moyers, R.E.; Riolo, M.L.; Guire, K.E.; Wainright, R.L.; Bookstein, F.L. Differential diagnosis of Class II malocclusions: Part 1. Facial types associated with Class II malocclusions. Am. J. Orthod. 1980, 78, 477–494. [Google Scholar] [CrossRef] [PubMed]
- Bolla, E.; Muratore, F.; Carano, A.; Bowman, S.J. Evaluation of maxillary molar distalization with the distal jet: A comparison with other contemporary methods. Angle Orthod. 2002, 72, 481–494. [Google Scholar]
- Basdra, E.; Huber, H.; Komposch, G. A clinical report for distalizing maxillary molars by using super-elastic wires. J. Orofac. Orthop. 1996, 57, 118–123. [Google Scholar] [CrossRef]
- Cangialosi, T.J.; Melstrell, M.E., Jr.; Leung, M.A.; Ko, J.Y. A cephalometric appraisal of edgewise Class II nonextraction treatment with extraoral force. Am. J. Orthod. Dentofac. Orthop. 1988, 93, 315–324. [Google Scholar] [CrossRef] [PubMed]
- Enacar, A.; Ozgen, M. Asymmetric maxillary expansion appliance (ABHE). Cleft Palate Craniofac. J. 1993, 30, 416–417. [Google Scholar] [CrossRef]
- Luppanapornlarp, S.; Johnston, L.E., Jr. The effects of premolar-extraction: A long-term comparison of outcomes in “clear-cut” extraction and nonextraction Class II patients. Angle Orthod. 1993, 63, 257–272. [Google Scholar]
- Simon, M.; Keilig, L.; Schwarze, J.; Jung, B.A.; Bourauel, C. Treatment outcome and efficacy of an aligner technique—Regarding incisor torque, premolar derotation and molar distalization. BMC Oral Health 2014, 14, 68. [Google Scholar] [CrossRef]
- Galan-Lopez, L.; Barcia-Gonzalez, J.; Plasencia, E. A systematic review of the accuracy and efficiency of dental movements with Invisalign®. Korean J. Orthod. 2019, 49, 140–149. [Google Scholar] [CrossRef]
- Garino, F.; Castroflorio, T.; Daher, S.; Ravera, S.; Rossini, G.; Cugliari, G.; Deregibus, A. Effectiveness of composite attachments in controlling upper-molar movement with aligners. J. Clin. Orthod. 2016, 50, 341–347. [Google Scholar] [PubMed]
- Jia, L.; Wang, C.; Li, L.; He, Y.; Wang, C.; Song, J.; Wang, L.; Fan, Y. The effects of lingual buttons, precision cuts, and patient-specific attachments during maxillary molar distalization with clear aligners: Comparison of finite element analysis. Am. J. Orthod. Dentofac. Orthop. 2023, 163, e1–e12. [Google Scholar] [CrossRef]
- Kinzinger, G.S.; Eren, M.; Diedrich, P.R. Treatment effects of intraoral appliances with conventional anchorage designs for non-compliance maxillary molar distalization: A literature review. Eur. J. Orthod. 2008, 30, 558–571. [Google Scholar] [CrossRef]
- Liu, F.; Liu, J.; Guo, M.; Li, Z.; Shu, G.; Dai, F. Miniscrew anchorage versus Class II elastics for maxillary arch distalization using clear aligners. Angle Orthod. 2024, 94, 45–52. [Google Scholar] [CrossRef] [PubMed]
- Barone, S.; Paoli, A.; Razionale, A.V.; Savignano, R. Computational design and engineering of polymeric orthodontic aligners. Int. J. Numer. Methods Biomed. Eng. 2017, 33, e2839. [Google Scholar] [CrossRef] [PubMed]
- Cortona, A.; Rossini, G.; Parrini, S.; Deregibus, A.; Castroflorio, T. Clear aligner orthodontic therapy of rotated mandibular round-shaped teeth: A finite element study. Angle Orthod. 2020, 90, 247–254. [Google Scholar] [CrossRef]
- Gomez, J.P.; Peña, F.M.; Martínez, V.; Giraldo, D.C.; Cardona, C.I. Initial force systems during bodily tooth movement with plastic aligners and composite attachments: A three-dimensional finite element analysis. Angle Orthod. 2015, 85, 454–460. [Google Scholar] [CrossRef]
- Ayidağa, C.; Kamiloğlu, B. Effects of variable composite attachment shapes in controlling upper molar distalization with aligners: A nonlinear finite element study. J. Healthc. Eng. 2021, 2021, 5557483. [Google Scholar] [CrossRef] [PubMed]
- Rossini, G.; Schiaffino, M.; Parrini, S.; Sedran, A.; Deregibus, A.; Castroflorio, T. Upper second molar distalization with clear aligners: A finite element study. Appl. Sci. 2020, 10, 7739. [Google Scholar] [CrossRef]
- Gao, L.; Wichelhaus, A. Forces and moments delivered by the PET-G aligner to a maxillary central incisor for palatal tipping and intrusion. Angle Orthod. 2017, 87, 534–541. [Google Scholar] [CrossRef]
- Cowley, D.P. Effect of Gingival Margin Design on Retention of Thermoformed Orthodontic Aligners. Master’s Thesis, University of Nevada, Las Vegas, NV, USA, 2012. [Google Scholar] [PubMed]
- Elshazly, T.M.; Salvatori, D.; Elattar, H.; Bourauel, C.; Keilig, L. Effect of trimming line design and edge extension of orthodontic aligners on force transmission: A 3D finite element study. J. Mech. Behav. Biomed. Mater. 2023, 140, 105741. [Google Scholar] [CrossRef]
- Kim, W.H.; Hong, K.; Lim, D.; Lee, J.H.; Jung, Y.J.; Kim, B. Optimal position of attachment for removable thermoplastic aligner on the lower canine using finite element analysis. Materials 2020, 13, 3369. [Google Scholar] [CrossRef]
- Wu, J.-L.; Liu, Y.-F.; Peng, W.; Dong, H.-Y.; Zhang, J.-X. A biomechanical case study on the optimal orthodontic force on the maxillary canine tooth based on finite element analysis. J. Zhejiang Univ. Sci. B 2018, 19, 535–546. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Akbari, A.; Jiang, F.; Liu, Y.; Chen, J. The effects of different types of periodontal ligament material models on stresses computed using finite element models. Am. J. Orthod. Dentofac. Orthop. 2022, 162, e328–e336. [Google Scholar] [CrossRef] [PubMed]
- Wei, Z.; Yu, X.; Xu, X.; Chen, X. Experiment and hydro-mechanical coupling simulation study on the human periodontal ligament. Comput. Methods Programs Biomed. 2014, 113, 749–756. [Google Scholar] [CrossRef]
- Cattaneo, P.M.; Dalstra, M.; Melsen, B. The finite element method: A tool to study orthodontic tooth movement. J. Dent. Res. 2005, 84, 428–433. [Google Scholar] [CrossRef] [PubMed]
- Kwak, K.-H.; Oh, S.; Choi, Y.-K.; Kim, S.-H.; Kim, S.-S.; Park, S.-B.; Kim, Y.-I. Effects of different distalization directions and methods on maxillary total distalization with clear aligners: A finite element study. Angle Orthod. 2023, 93, 348–356. [Google Scholar] [CrossRef]
- Fan, D.; Liu, H.; Yuan, C.-Y.; Wang, S.-Y.; Wang, P.-L. Effectiveness of the attachment position in molar intrusion with clear aligners: A finite element study. BMC Oral Health 2022, 22, 474. [Google Scholar] [CrossRef] [PubMed]
- Eliades, T.; Eliades, G. Intraoral ageing of aligners and attachments: Adverse effects on clinical efficiency and release of biologically-active compounds. Korean J. Orthod. 2024, 54, 199–209. [Google Scholar] [CrossRef]
- Traversa, F.; Chavanne, P.; Mah, J. Biomechanics of clear aligner therapy: Assessing the influence of tooth position and flat trimline height in translational movements. Orthod. Craniofac. Res. 2024, 28, 1–11. [Google Scholar] [CrossRef]
- Taffarel, I.A.; Gasparello, G.G.; Mota-Júnior, S.L.; Pithon, M.M.; Taffarel, I.P.; Meira, T.M.; Tanaka, O.M. Distalization of maxillary molars with Invisalign aligners in nonextraction patients with Class II malocclusion. Am. J. Orthod. Dentofac. Orthop. 2022, 162, e176–e182. [Google Scholar] [CrossRef]
- Drake, C.T.; McGorray, S.P.; Dolce, C.; Nair, M.; Wheeler, T.T. Orthodontic tooth movement with clear aligners. Int. Sch. Res. Notices 2012, 2012, 657973. [Google Scholar] [CrossRef]
- Henry, R. Relationship of the maxillary first permanent molar in normal occlusion and malocclusion: An intraoral study. Am. J. Orthod. 1956, 42, 288–306. [Google Scholar] [CrossRef]
- Lamons, F.F.; Holmes, C.W., III. The problem of the rotated maxillary first permanent molar. Am. J. Orthod. 1961, 47, 246–272. [Google Scholar] [CrossRef]
- Nucera, R.; Dolci, C.; Bellocchio, A.M.; Costa, S.; Barbera, S.; Rustico, L.; Farronato, M.; Militi, A.; Portelli, M. Effects of composite attachments on orthodontic clear aligners therapy: A systematic review. Materials 2022, 15, 533. [Google Scholar] [CrossRef]
- Kravitz, N.D.; Kusnoto, B.; Agran, B.; Viana, G. Influence of attachments and interproximal reduction on the accuracy of canine rotation with Invisalign: A prospective clinical study. Angle Orthod. 2008, 78, 682–687. [Google Scholar] [CrossRef]
- Saif, B.S.; Pan, F.; Mou, Q.; Han, M.; Bu, W.; Zhao, J.; Guan, L.; Wang, F.; Zou, R.; Zhou, H.; et al. Efficiency evaluation of maxillary molar distalization using Invisalign based on palatal rugae registration. Am. J. Orthod. Dentofac. Orthop. 2022, 161, e372–e379. [Google Scholar] [CrossRef]
- Mamani, J.; Sessirisombat, C.; Hotokezaka, H.; Yoshida, N.; Sirisoontorn, I. Effectiveness of Clear Aligners on Sequential Maxillary Molar Distalization: Discrepancy between Treatment Goal and Outcome. J. Clin. Med. 2024, 13, 4216. [Google Scholar] [CrossRef]
- Caruso, S.; Nota, A.; Ehsani, S.; Maddalone, E.; Ojima, K.; Tecco, S. Impact of molar teeth distalization with clear aligners on occlusal vertical dimension: A retrospective study. BMC Oral Health 2019, 19, 182. [Google Scholar] [CrossRef]
- Park, T.H.; Shen, C.; Chung, C.-H.; Li, C. Vertical Control in Molar Distalization by Clear Aligners: A Systematic Review and Meta-Analysis. J. Clin. Med. 2024, 13, 2845. [Google Scholar] [CrossRef]
- Andrews, L.E. The Six Keys to Normal Occlusion. Am. J. Orthod. Dentofac. Orthop. 1972, 62, 296–309. [Google Scholar] [CrossRef]
- Stoller, W. The Significance of the First Molar in Occlusion. Am. J. Orthod. 1954, 40, 315–324. [Google Scholar]
- Savignano, R.; Valentino, R.; Razionale, A.V.; Michelotti, A.; Barone, S.; D’Antò, V. Biomechanical Effects of Different Auxiliary-Aligner Designs for the Extrusion of an Upper Central Incisor: A Finite Element Analysis. J. Healthc. Eng. 2019, 2019, 9687127. [Google Scholar] [CrossRef] [PubMed]
- Ye, N.; Brown, B.E.; Mantell, S.C.; Heo, Y.C.; Larson, B.E.; Fok, A.S.L. Validation of Finite Element Models for Orthodontic Aligners. J. Mech. Behav. Biomed. Mater. 2022, 134, 105404. [Google Scholar] [CrossRef] [PubMed]
- Loberto, L.; Perrotti, G.; Testa, M.; Di Carlo, S.; Marzo, G.; Pilloni, A.; D’Addona, A. Anchorage Loss Evaluation during Maxillary Molars Distalization Performed by Clear Aligners: A Retrospective Study on 3D Digital Casts. Appl. Sci. 2023, 13, 3646. [Google Scholar] [CrossRef]
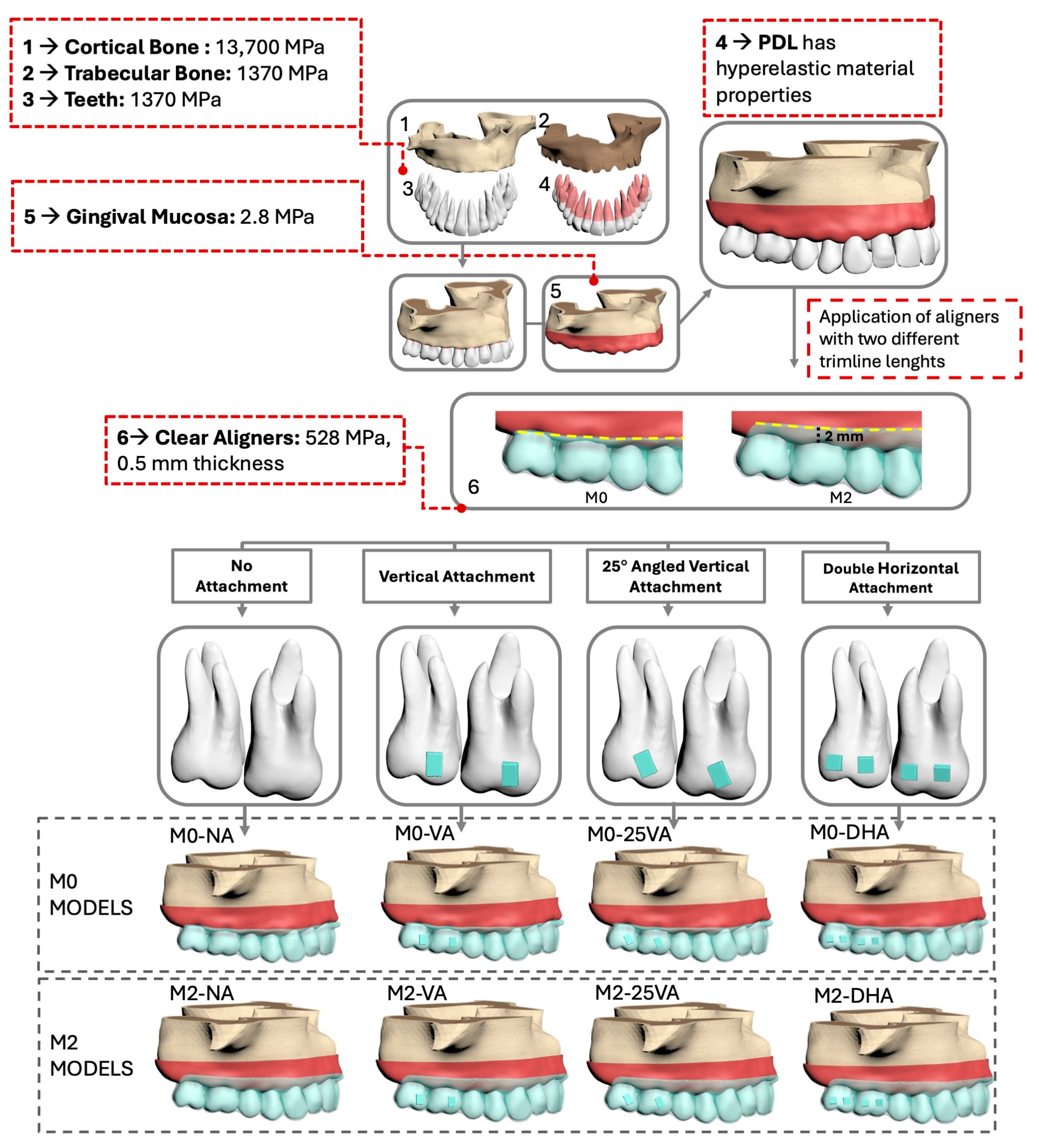


| Component | Elastic Modulus (MPa) | Poisson Rate |
|---|---|---|
| Cortical Bone | 13,700 | 0.3 |
| Cancellous Bone | 1370 | 0.3 |
| Gingiva | 3.45 | 0.45 |
| Teeth | 19,600 | 0.3 |
| Aligner | 528 | 0.36 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Büyükpatır Türk, T.; Küçükkaraca, E. Biomechanical Evaluation of Attachment and Trimline Modifications in Maxillary Molar Distalization Using Clear Aligners. Appl. Sci. 2025, 15, 5873. https://doi.org/10.3390/app15115873
Büyükpatır Türk T, Küçükkaraca E. Biomechanical Evaluation of Attachment and Trimline Modifications in Maxillary Molar Distalization Using Clear Aligners. Applied Sciences. 2025; 15(11):5873. https://doi.org/10.3390/app15115873
Chicago/Turabian StyleBüyükpatır Türk, Tulca, and Ebru Küçükkaraca. 2025. "Biomechanical Evaluation of Attachment and Trimline Modifications in Maxillary Molar Distalization Using Clear Aligners" Applied Sciences 15, no. 11: 5873. https://doi.org/10.3390/app15115873
APA StyleBüyükpatır Türk, T., & Küçükkaraca, E. (2025). Biomechanical Evaluation of Attachment and Trimline Modifications in Maxillary Molar Distalization Using Clear Aligners. Applied Sciences, 15(11), 5873. https://doi.org/10.3390/app15115873








