Asymmetry Propagation in a Pipe Flow Downstream of a 90° Sharp Elbow Bend
Abstract
Featured Application
Abstract
1. Introduction
2. Numerical Setup
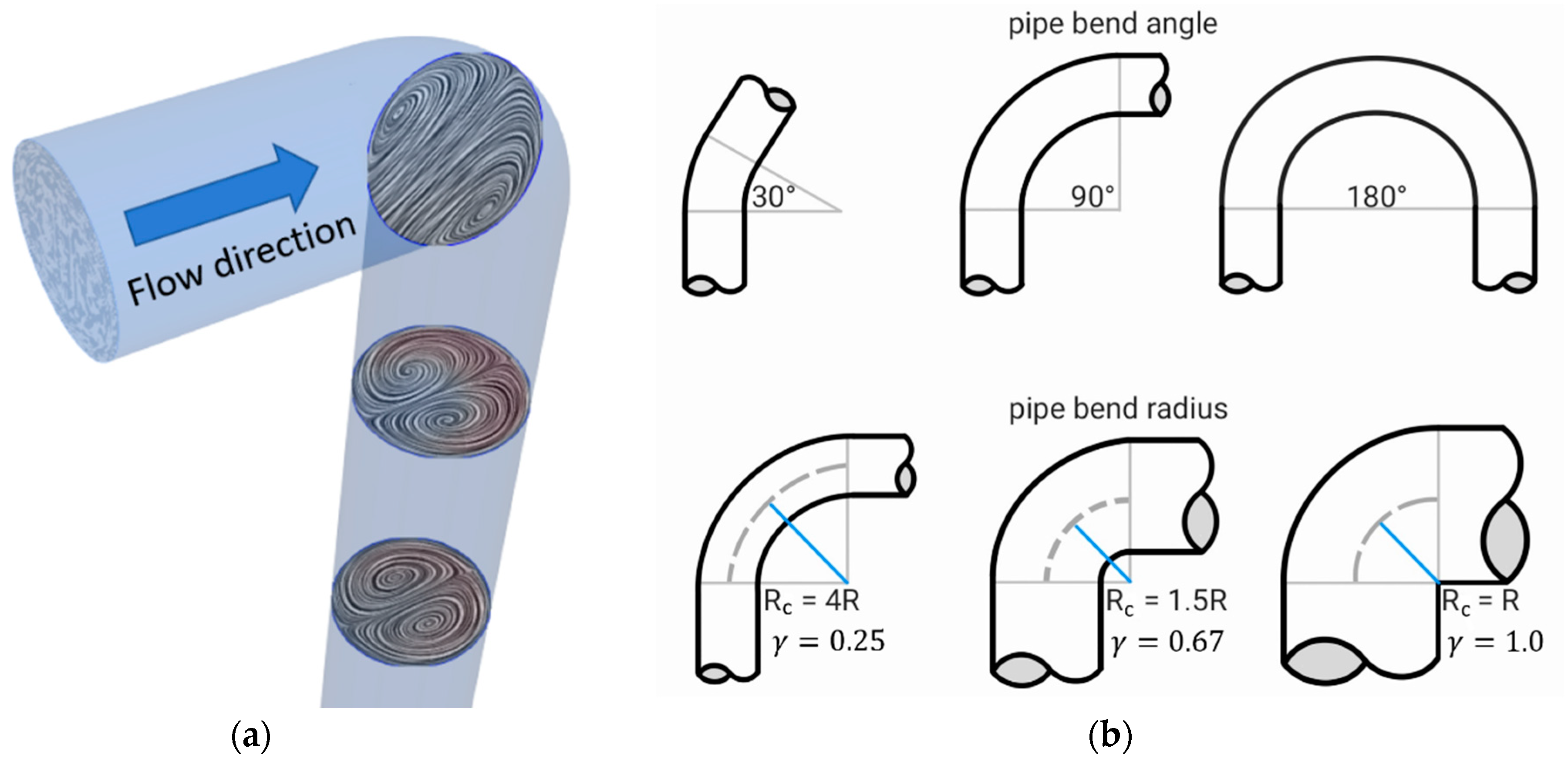
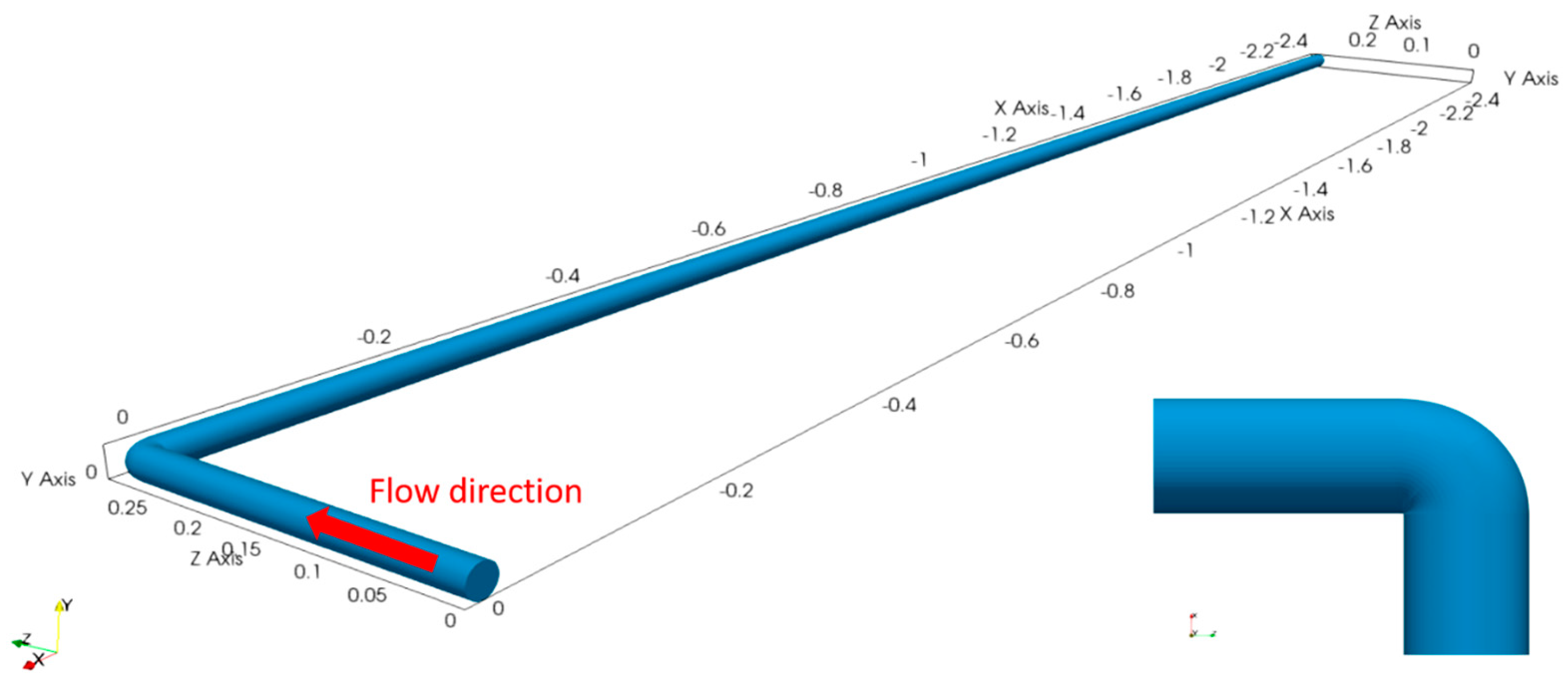
2.1. Geometry and Computational Domain
2.2. Governing Equations and Numerical Schemes
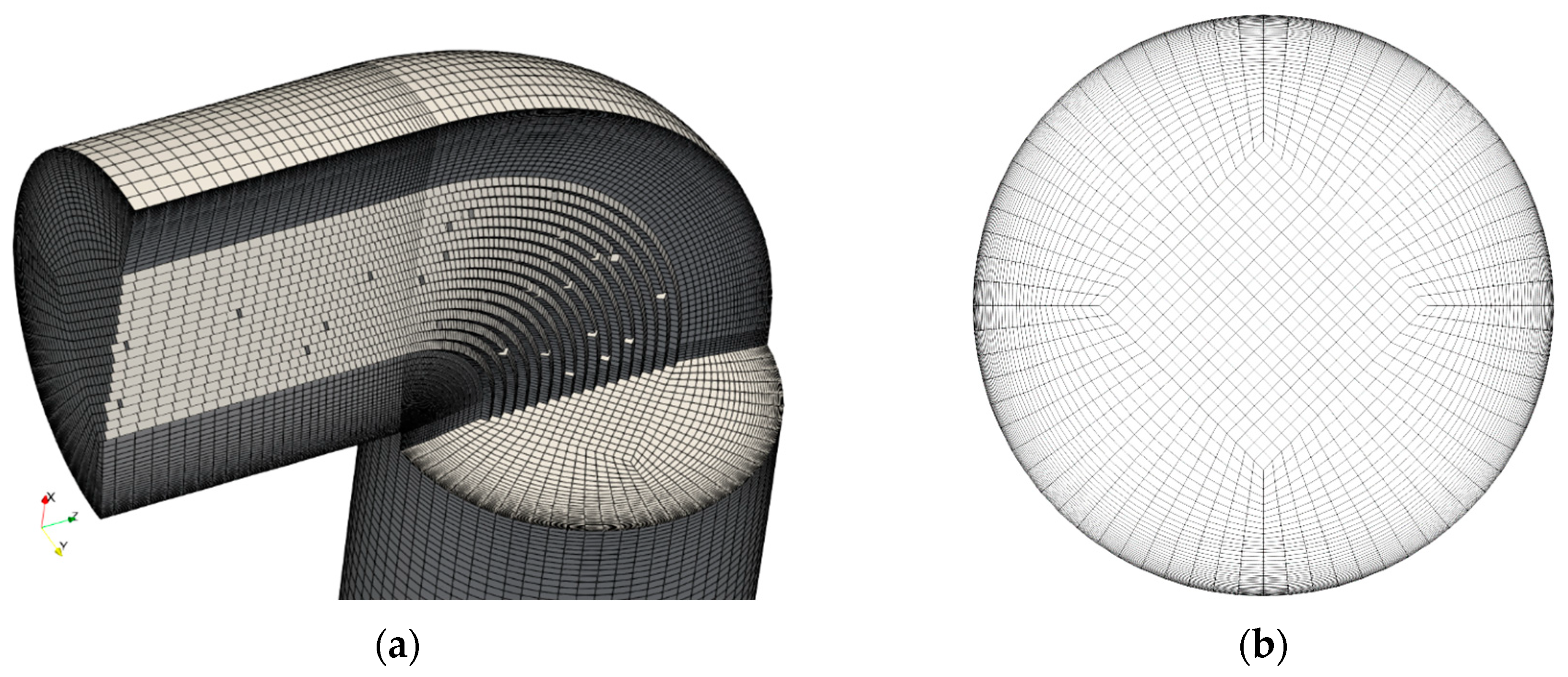
2.3. Computational Mesh
2.4. Boundary Conditions and Statistics Accumulation
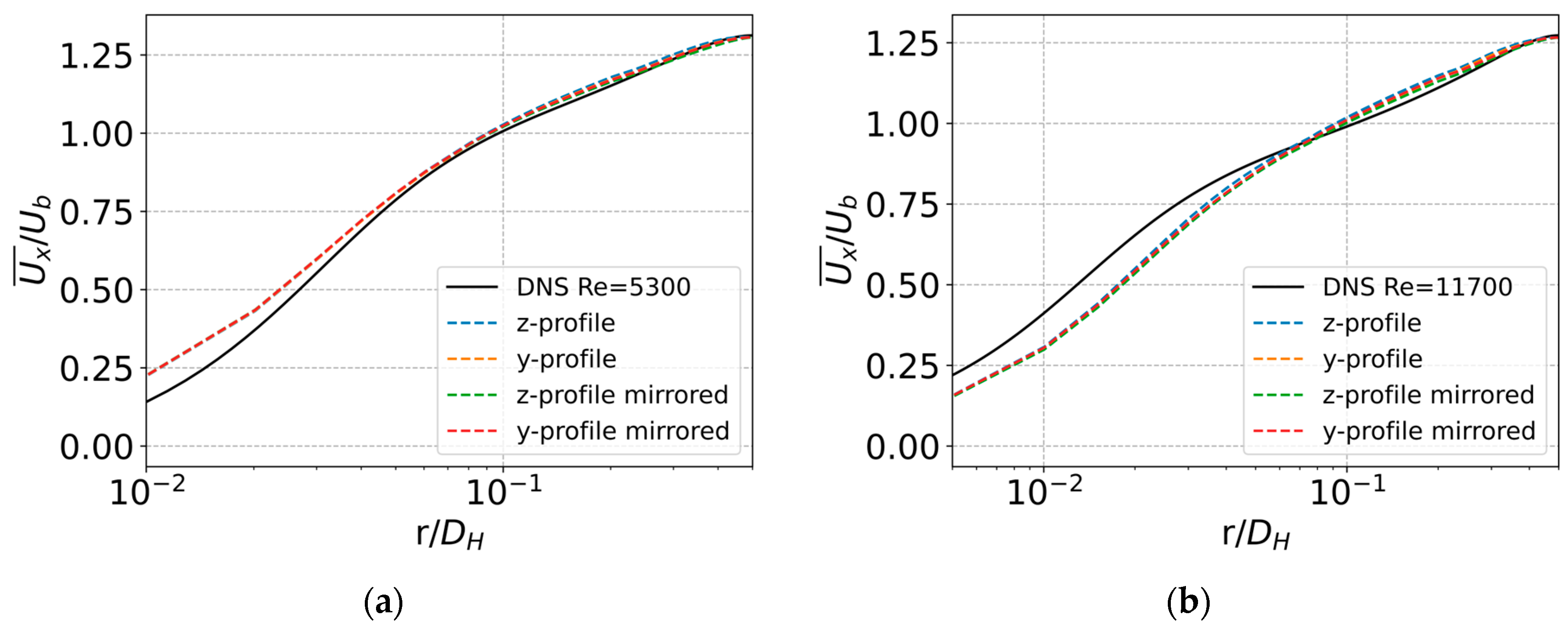
2.5. Validation
3. Results
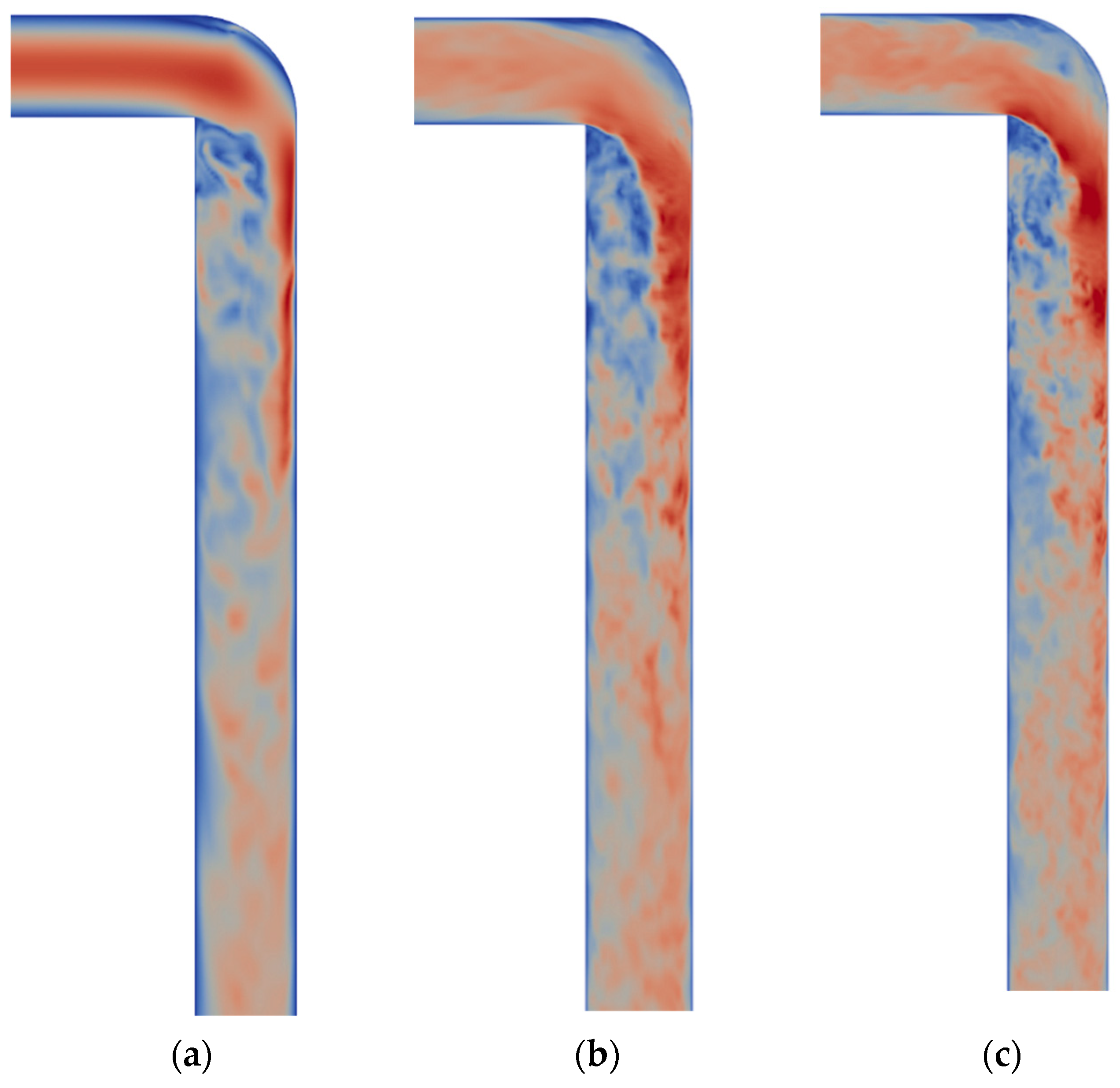
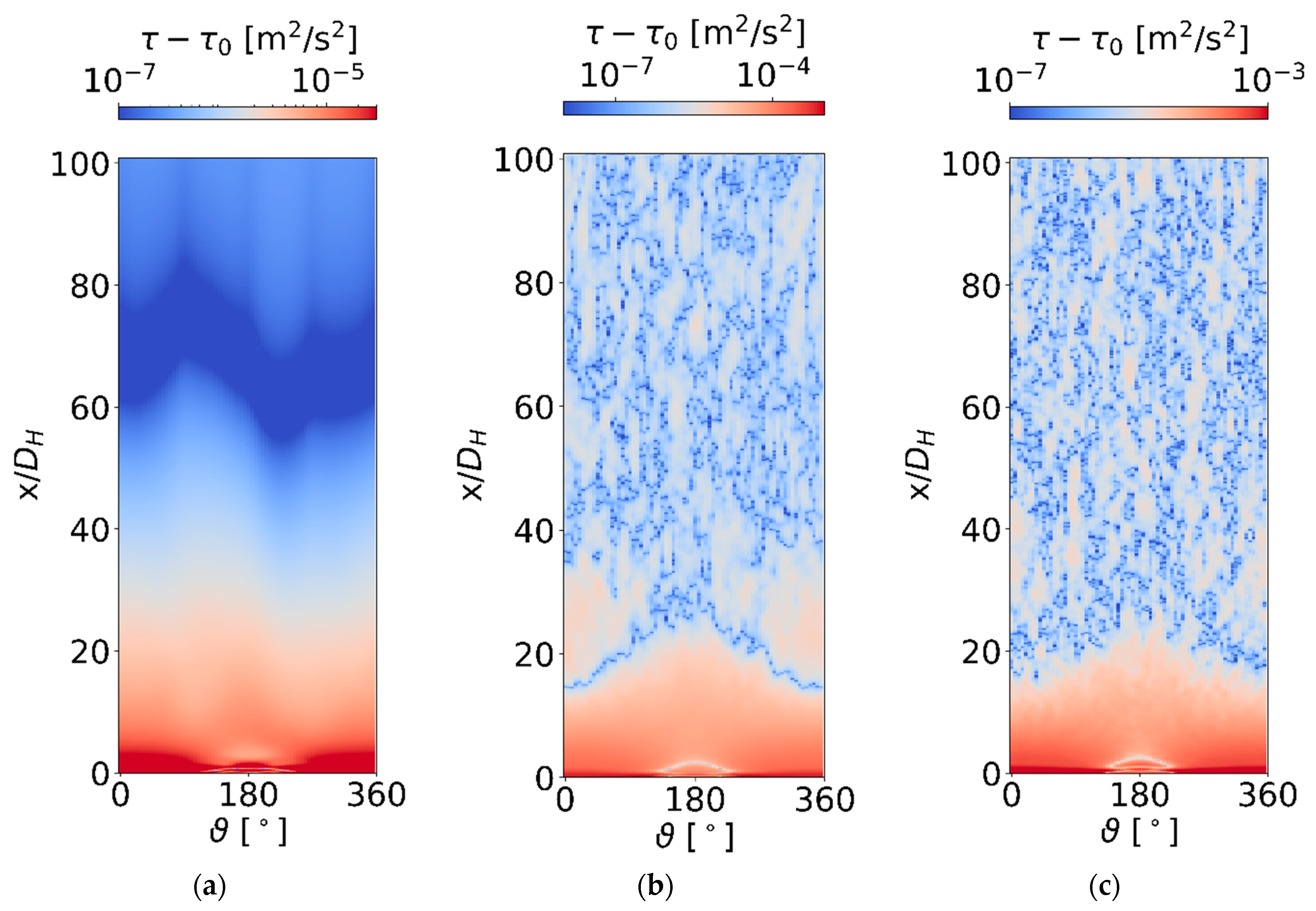
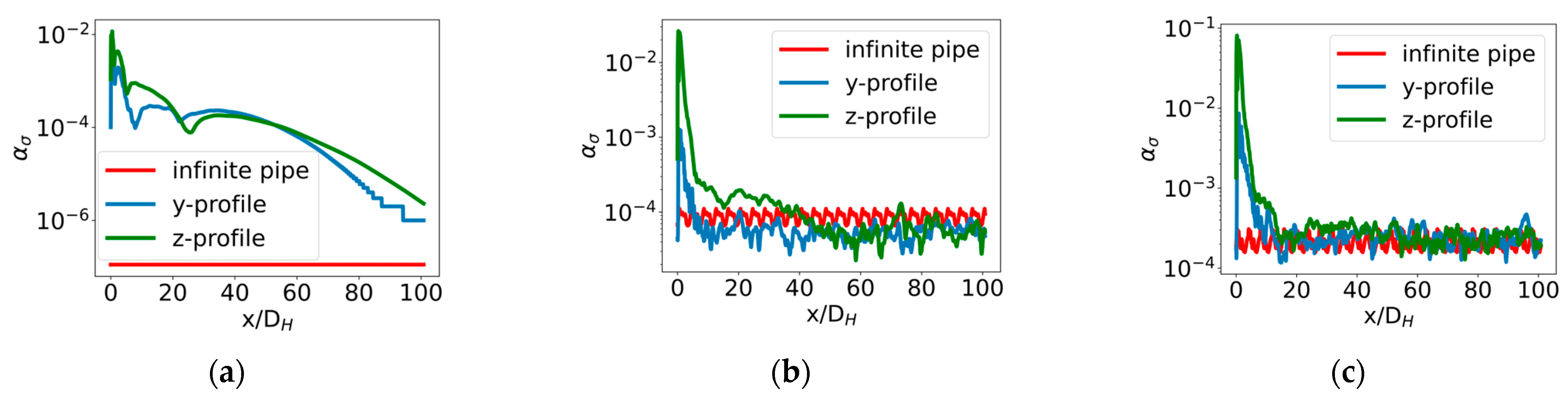
3.1. Asymmetry in Wall Shear Stress
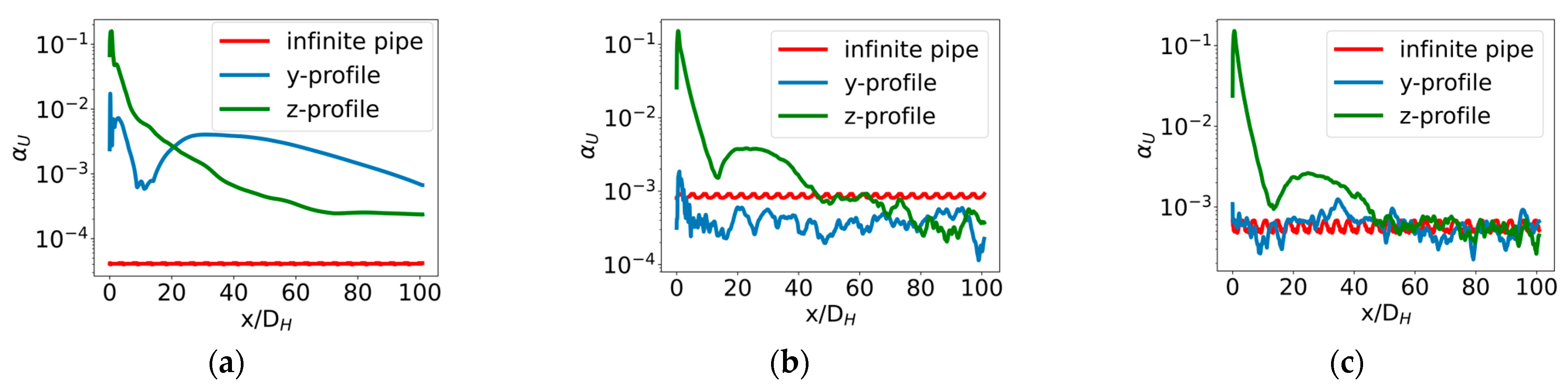
3.2. Asymmetry in Mean Velocity Profiles
3.3. Asymmetry in Velocity Fluctuation Profiles
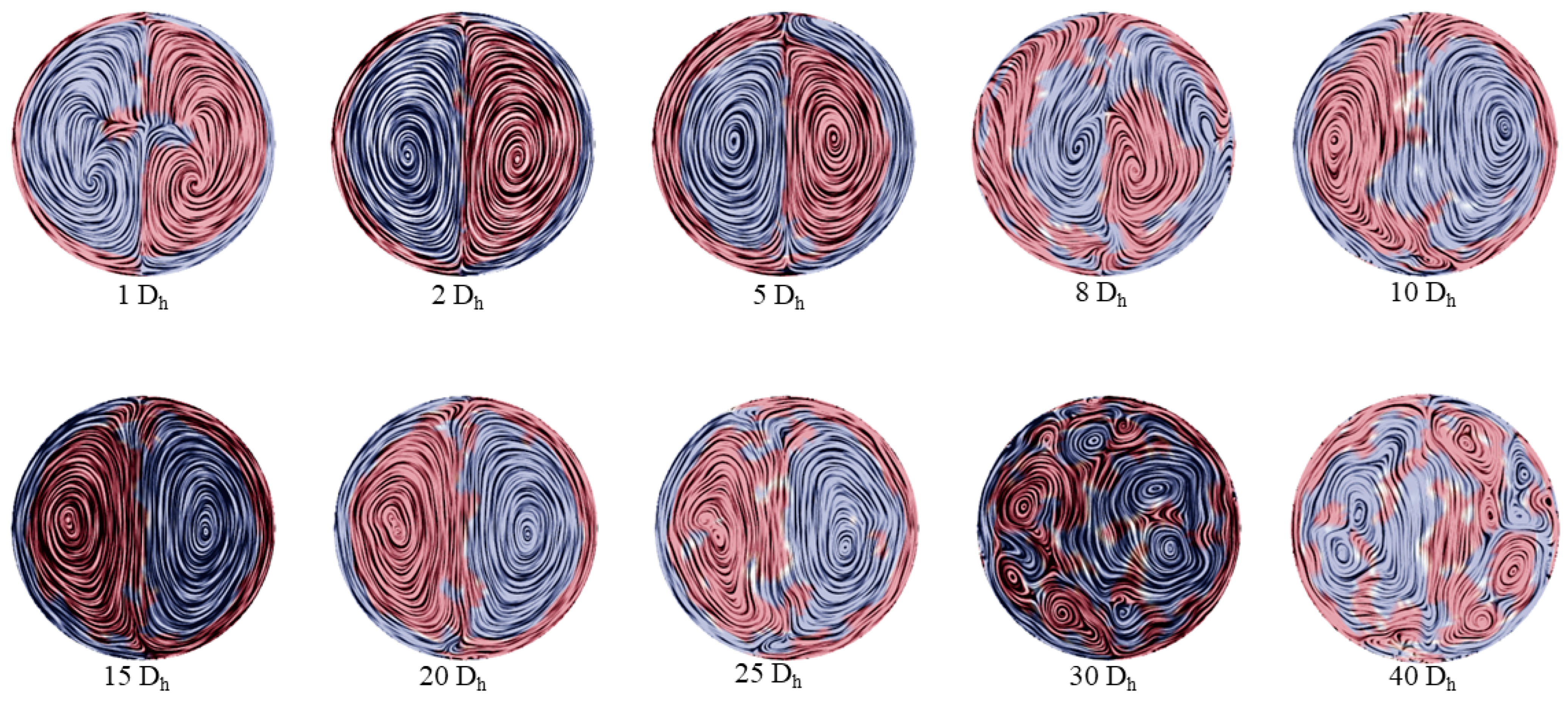
3.4. Asymmetry in Vorticity Profiles
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dean, W.R. LXXII. The Stream-Line Motion of Fluid in a Curved Pipe (Second Paper). Lond. Edinb. Dublin Philos. Mag. J. Sci. 1928, 5, 673–695. [Google Scholar] [CrossRef]
- Dean, W.R.; Hurst, J.M. Note on the Motion of Fluid in a Curved Pipe. Mathematika 1959, 6, 77–85. [Google Scholar] [CrossRef]
- Prandtl, L. Essentials of Fluid Dynamics: With Applications to Hydraulics, Aeronautics, Meteorology and Other Subjects; Blackie & Son: Glasgow, UK, 1952. [Google Scholar]
- Kalpakli Vester, A.; Örlü, R.; Alfredsson, P.H. Turbulent Flows in Curved Pipes: Recent Advances in Experiments and Simulations. Appl. Mech. Rev. 2016, 68, 050802. [Google Scholar] [CrossRef]
- Düz, H. Numerical and Experimental Study to Predict the Entrance Length in Pipe Flows. J. Appl. Fluid Mech. 2019, 12, 155–164. [Google Scholar] [CrossRef]
- Doherty, J.; Ngan, P.; Monty, J.; Chong, M. The Development of Turbulent Pipe Flow. In Proceedings of the 16th Australasian Fluid Mechanics Conference, Gold Coast, Australia, 3–7 December 2007; pp. 266–270. [Google Scholar]
- Avila, M.; Barkley, D.; Hof, B. Transition to Turbulence in Pipe Flow. Annu. Rev. Fluid Mech. 2023, 55, 575–602. [Google Scholar] [CrossRef]
- Mullin, T. Experimental Studies of Transition to Turbulence in a Pipe. Annu. Rev. Fluid Mech. 2011, 43, 1–24. [Google Scholar] [CrossRef]
- Sudo, K.; Sumida, M.; Hibara, H. Experimental Investigation on Turbulent Flow in a Circular-Sectioned 90-Degree Bend. Exp. Fluids 1998, 25, 42–49. [Google Scholar] [CrossRef]
- Sudo, K.; Sumida, M.; Hibarra, H. Experimental Investigation on Turbulent Flow through a Circular-Sectioned 180° Bend. Exp. Fluids 2000, 28, 51–57. [Google Scholar] [CrossRef]
- Hellström, L.H.O.; Zlatinov, M.B.; Cao, G.; Smits, A.J. Turbulent Pipe Flow Downstream of a Bend. J. Fluid Mech. 2013, 735, R7. [Google Scholar] [CrossRef]
- Tunstall, M.; Harvey, J. On the Effect of a Sharp Bend in a Fully Developed Turbulent Pipe-Flow. J. Fluid Mech. 1968, 34, 595–608. [Google Scholar] [CrossRef]
- Sattarzadeh, S.S. Experimental Study of Complex Pipe Flow. Master’s Thesis, Royal Institute of Technology, Stockholm, Sweden, 2011. [Google Scholar]
- Kalpakli, A.; Örlü, R. Turbulent Pipe Flow Downstream a 90° Pipe Bend with and without Superimposed Swirl. Int. J. Heat Fluid Flow 2013, 41, 103–111. [Google Scholar] [CrossRef]
- Kalpakli Vester, A.; Sattarzadeh, S.S.; Örlü, R. Combined Hot-Wire and PIV Measurements of a Swirling Turbulent Flow at the Exit of a 90° Pipe Bend. J. Vis. 2016, 19, 261–273. [Google Scholar] [CrossRef]
- Canton, J.; Schlatter, P.; Örlü, R. Modal Instability of the Flow in a Toroidal Pipe. J. Fluid Mech. 2016, 792, 894–909. [Google Scholar] [CrossRef]
- Lupi, V.; Canton, J.; Schlatter, P. Global Stability Analysis of a 90°-Bend Pipe Flow. Int. J. Heat Fluid Flow 2020, 86, 108742. [Google Scholar] [CrossRef]
- Massaro, D.; Lupi, V.; Peplinski, A.; Schlatter, P. Global Stability of 180°-Bend Pipe Flow with Mesh Adaptivity. Phys. Rev. Fluids 2023, 8, 113903. [Google Scholar] [CrossRef]
- Di Liberto, M.; Di Piazza, I.; Ciofalo, M. Turbulence Structure and Budgets in Curved Pipes. Comput. Fluids 2013, 88, 452–472. [Google Scholar] [CrossRef]
- Noorani, A.; Schlatter, P. Swirl-Switching Phenomenon in Turbulent Flow through Toroidal Pipes. Int. J. Heat Fluid Flow 2016, 61, 108–116. [Google Scholar] [CrossRef]
- Hufnagel, L.; Canton, J.; Örlü, R.; Marin, O.; Merzari, E.; Schlatter, P. The Three-Dimensional Structure of Swirl-Switching in Bent Pipe Flow. J. Fluid Mech. 2018, 835, 86–101. [Google Scholar] [CrossRef]
- Röhrig, R.; Jakirlić, S.; Tropea, C. Comparative Computational Study of Turbulent Flow in a 90° Pipe Elbow. Int. J. Heat Fluid Flow 2015, 55, 120–131. [Google Scholar] [CrossRef]
- He, X.; Apte, S.V.; Karra, S.K.; Doğan, Ö.N. An LES Study of Secondary Motion and Wall Shear Stresses in a Pipe Bend. Phys. Fluids 2021, 33, 115102. [Google Scholar] [CrossRef]
- Al-Baghdadi, M.A.; Resan, K.K.; Al-Waily, M. CFD Investigation of the Erosion Severity in 3D Flow Elbow during Crude Oil Contaminated Sand Transportation. Eng. Technol. J. 2017, 35, 930–935. [Google Scholar] [CrossRef]
- Ma, R.; Tang, R.; Gao, Z.; Yu, T. Optimized Design of Pipe Elbows for Erosion Wear. NATO Adv. Sci. Inst. Ser. E Appl. Sci. 2024, 14, 984. [Google Scholar] [CrossRef]
- Ma, G.; Ma, H.; Sun, Z. Simulation of Two-Phase Flow of Shotcrete in a Bent Pipe Based on a CFD–DEM Coupling Model. NATO Adv. Sci. Inst. Ser. E Appl. Sci. 2022, 12, 3530. [Google Scholar] [CrossRef]
- Henríquez Lira, S.; Torres, M.J.; Guerra Silva, R.; Zahr Viñuela, J. Numerical Characterization of the Solid Particle Accumulation in a Turbulent Flow through Curved Pipes by Means of Stokes Numbers. NATO Adv. Sci. Inst. Ser. E Appl. Sci. 2021, 11, 7381. [Google Scholar] [CrossRef]
- Khalifa, A.; Gollwitzer, J.; Breuer, M. LES of Particle-Laden Flow in Sharp Pipe Bends with Data-Driven Predictions of Agglomerate Breakage by Wall Impacts. Fluids 2021, 6, 424. [Google Scholar] [CrossRef]
- Gotfredsen, E.; Kunoy, J.D.; Mayer, S.; Meyer, K.E. Experimental Validation of RANS and DES Modelling of Pipe Flow Mixing. Heat Mass Transf. 2020, 56, 2211–2224. [Google Scholar] [CrossRef]
- Wojewodka, M.M.; White, C.; Shahpar, S.; Kontis, K. Numerical Study of Complex Flow Physics and Coherent Structures of the Flow through a Convoluted Duct. Aerosp. Sci. Technol. 2022, 121, 107191. [Google Scholar] [CrossRef]
- Rütten, F.; Schröder, W.; Meinke, M. Large-Eddy Simulation of Low Frequency Oscillations of the Dean Vortices in Turbulent Pipe Bend Flows. Phys. Fluids 2005, 17, 035107. [Google Scholar] [CrossRef]
- Alabdalah, A.; Wnos, L. Numerical Simulation and Flow Analysis of a 90-Degree Elbow. Int. J. Civ. Mech. Energy Sci. 2020, 6, 10–13. [Google Scholar] [CrossRef]
- Bilde, K.G.; Sørensen, K.; Hærvig, J. Decay of Secondary Motion Downstream Bends in Turbulent Pipe Flows. Phys. Fluids 2023, 35, 015102. [Google Scholar] [CrossRef]
- Tunstall, R.; Laurence, D.; Prosser, R.; Skillen, A. Large Eddy Simulation of a T-Junction with Upstream Elbow: The Role of Dean Vortices in Thermal Fatigue. Appl. Therm. Eng. 2016, 107, 672–680. [Google Scholar] [CrossRef]
- Zhou, M.; Costa Garrido, O.; Ma, S.; Zhang, N. Numerical Investigation of Turbulent Thermal Stratification at a Horizontally Oriented 90° Pipe-Elbow with Varied Elbow Radiuses. Int. J. Therm. Sci. 2023, 185, 108092. [Google Scholar] [CrossRef]
- Kren, J.; Frederix, E.M.A.; Tiselj, I.; Mikuž, B. Numerical Study of Taylor Bubble Breakup in Counter-Current Flow Using Large Eddy Simulation. Phys. Fluids 2024, 36, 023311. [Google Scholar] [CrossRef]
- Kren, J.; Zajec, B.; Tiselj, I.; Shawish, S.E.; Perne, Ž.; Tekavčič, M.; Mikuž, B. Dynamics of Taylor Bubble Interface in Vertical Turbulent Counter-Current Flow. Int. J. Multiph. Flow 2023, 165, 104482. [Google Scholar] [CrossRef]
- OpenFOAM V10. Available online: https://openfoam.org/version/10/ (accessed on 17 February 2024).
- HPC Vega. Available online: https://en-vegadocs.vega.izum.si/ (accessed on 17 February 2024).
- Cerkovnik, K. Simulacija Turbuletnega Toka z Metodo Velikih Vrtincev v Cevi s Kolenom. Master’s Thesis, Faculty of Mathematics and Physics, University of Ljubljana, Ljubljana, Slovenia, 2023. [Google Scholar]
- Nicoud, F.; Ducros, F. Subgrid-Scale Stress Modelling Based on the Square of the Velocity Gradient Tensor. Flow Turbul. Combust. 1999, 62, 183–200. [Google Scholar] [CrossRef]
- Komen, E.; Shams, A.; Camilo, L.; Koren, B. Quasi-DNS Capabilities of OpenFOAM for Different Mesh Types. Comput. Fluids 2014, 96, 87–104. [Google Scholar] [CrossRef]
- Mikuž, B.; Tiselj, I. Wall-Resolved Large Eddy Simulation in Grid-Free 5×5 Rod Bundle of MATiS-H Experiment. Nucl. Eng. Des. 2016, 298, 64–77. [Google Scholar] [CrossRef]
- Frederix, E.; Tajfirooz, S.; Hopman, J.; Fang, J.; Merzari, E.; Komen, E. Two-Phase Turbulent Kinetic Energy Budget Computation in Co-Current Taylor Bubble Flow. Nucl. Sci. Eng. 2023, 197, 2585–2601. [Google Scholar] [CrossRef]
- Fukagata, K.; Kasagi, N. Highly Energy-Conservative Finite Difference Method for the Cylindrical Coordinate System. J. Comput. Phys. 2002, 181, 478–498. [Google Scholar] [CrossRef]
- El Khoury, G.K.; Schlatter, P.; Noorani, A.; Fischer, P.F.; Brethouwer, G.; Johansson, A.V. Direct Numerical Simulation of Turbulent Pipe Flow at Moderately High Reynolds Numbers. Flow Turbul. Combust. 2013, 91, 475–495. [Google Scholar] [CrossRef]
- Komen, E.M.J.; Camilo, L.H.; Shams, A.; Geurts, B.J.; Koren, B. A Quantification Method for Numerical Dissipation in Quasi-DNS and under-Resolved DNS, and Effects of Numerical Dissipation in Quasi-DNS and under-Resolved DNS of Turbulent Channel Flows. J. Comput. Phys. 2017, 345, 565–595. [Google Scholar] [CrossRef]



| Mesh | Coarse | Middle | Fine |
|---|---|---|---|
| Number of cells [in millions] | 0.305 | 5.95 | 13.7 |
| Number of near-wall prism layers | 31 | 31 | 37 |
| Stretching ratio (SR) | 1.0 | 1.1 | 1.1 |
| Max. aspect ratio AR | 52.6 | 108 | 127 |
| Non-orthogonality (mean/max) [°] | 5.6/35 | 4.1/45 | 3.9/46 |
| Maximum skewness | 1.22 | 0.86 | 0.88 |
| First cell height at walls [mm] | 0.3–0.6 | 0.04–0.56 | 0.02–0.56 |
| Streamwise cell size [mm] | 11.6 | 1.2 | 0.6 |
| First cell height at walls [wall units] | 2.7–5.4 | 0.36–4.8 | 0.21–4.8 |
| Streamwise cell size [wall units] | 100 | 10 | 5 |
| Reynolds Number (Re) | 1400 | 5600 | 10,000 |
|---|---|---|---|
| Mean (bulk) velocity [m/s] | 0.04469 | 0.1792 | 0.32 |
| Time-averaging duration [s] | 800 | 400 | 55 |
| Convective time units | 1880 | 3750 | 950 |
| Number of time steps | 10 × 106 | 26 × 106 | 15 × 106 |
| Asymmetry Parameter | |||
|---|---|---|---|
| Variable | |||
| Normalization N |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mikuž, B.; Cerkovnik, K.; Tiselj, I. Asymmetry Propagation in a Pipe Flow Downstream of a 90° Sharp Elbow Bend. Appl. Sci. 2024, 14, 7895. https://doi.org/10.3390/app14177895
Mikuž B, Cerkovnik K, Tiselj I. Asymmetry Propagation in a Pipe Flow Downstream of a 90° Sharp Elbow Bend. Applied Sciences. 2024; 14(17):7895. https://doi.org/10.3390/app14177895
Chicago/Turabian StyleMikuž, Blaž, Klemen Cerkovnik, and Iztok Tiselj. 2024. "Asymmetry Propagation in a Pipe Flow Downstream of a 90° Sharp Elbow Bend" Applied Sciences 14, no. 17: 7895. https://doi.org/10.3390/app14177895
APA StyleMikuž, B., Cerkovnik, K., & Tiselj, I. (2024). Asymmetry Propagation in a Pipe Flow Downstream of a 90° Sharp Elbow Bend. Applied Sciences, 14(17), 7895. https://doi.org/10.3390/app14177895







