Influence of Wood and Thickness of Back Wall of Wooden Organ Pipe and Air Pressure in Windchest on Sound

Abstract

In the past, organ builders used many tree species for the production of wooden organ pipes, and the thicknesses of the pipe walls were also different. From the point of view of the sound of wooden organ pipes, it appears that the type of wood, as well as the thickness of the pipe wall, affect the resulting organ sound. Therefore, this research focused on how the wood species and the thickness of the pipe’s wall affect the sound of the organ. Two wood species were selected for our experiment: maple wood, representing hardwood; and spruce wood, representing softwood. The physical and acoustic characteristics (PACHs)—density (ρ), dynamic modulus of elasticity along the wood grain (E_L), specific modulus (E_sp), speed of sound along the grain (c_L), resonant frequency (f_r), acoustic constant (A), logarithmic decrement (ϑ), loss coefficient (η), acoustic conversion efficiency (ACE) and sound quality factor (Q)—were determined. Subsequently, the boards were used for making the back wall of the experimental wooden organ pipe with a replaceable back wall. The boards used for the back wall had an initial thickness of 7 mm. The boards were gradually thinned in 1 mm decrements to a final thickness of 1 mm. For each board thickness, the frequency spectrum was recorded at four different air pressures in the windchest, namely, 588 Pa, 716 Pa, 814 Pa and 941 Pa. The results of the experiment showed that at a given back wall thickness, the fundamental tone frequency increases with increasing air pressure. The decrease in the back wall thickness was manifested by a decrease in the fundamental frequency. With increasing air pressure, the intensity of higher harmonic frequencies also increased.

1. Introduction

To produce wooden musical instruments, it is necessary to use wood that meets certain predetermined quality parameters. The wood quality parameters, based on which it is possible to determine the quality of logs depending on the purpose, include wood density, the absence of defects and rot, the density of annual rings and wood grain. Nowadays, industrial computer tomography of wood is also used. With its help, it is possible to observe the internal features of the scanned wood, which makes it possible to certify the quality of logs for musical instruments [1]. When making different types of musical instruments from wood, it is necessary to consider the characteristics of wood because they greatly affect the sound characteristics of the musical instrument. Therefore, it is important to study the characteristics of wood and their influence on the sound of the musical instruments.

One of the oldest and most complicated musical instruments is the organ. It can be classified as a wind instrument. The organ pipes are mainly made of wood and metal. In the case of cylindrical metal organ pipes, the material has a smooth surface, and it has a little effect on the sound of a cylindrical pipe [2]. The organ pipes made of wood are square in cross section. Unlike metal pipes, the walls of wooden organ pipes are rougher and can vibrate due to internal acoustic pressures, which eventually affects the tone quality [3,4,5,6,7]. This is especially true when the playing frequency or one of its harmonics coincides with the vibration frequency of the wall boards. the effect of wall vibration is not significant if wooden pipes are sufficiently thick, but the influence can be detected by acoustic measurements [2,8]. In addition, the properties of the wood depend on the relative humidity of the air, which leads to a potential change in the sound properties of the pipes [9,10,11]. Due to the above, wooden pipes will have a different tonal character than metal pipes.

Organ builders use various wood species. It is possible to see, for example, organ pipes made only of spruce, but in another instrument, the pipes can be made of spruce in combination with a front wall of oak or beech [12]. Wood, as a material for wind instruments, has no direct influence on sound creation. The body wall material is not primarily important from the acoustic viewpoint because air vibrating down the tube of the instrument produces sound [13]. However, wind instruments made from different wood species generate dissimilar sounds. The sound quality depends on physical and mechanical properties (density, hardness, wood structure) [14]. Higher or lower hardness and brittleness have a very great effect upon the quality of tone [15]. In addition, the sound of a wooden organ pipe is influenced by the thickness of the wall, as well as by the strength of the wall pull, and by the properties of the flowing medium [16,17,18]. The pipe width influences the tone quality, as well. The wider the pipe, the larger its scale, and the louder the sound it can be made to produce. Narrow-scale pipes can be made to produce more overtones in relation to the fundamental, thus giving a brighter sound [19].

Nowadays, the sounding of the organ pipes is not a problem because it is controlled by mechanical, pneumatic or electric links that supply air to the pipes. In the present, the matter of interest is the materials from which the wooden organ pipes are made. There are two reasons for this: the lack of high-quality wood and its optimal use regarding the required sound properties of wooden pipes.

The aim of this study was to investigate the influence of two wood species (softwood—Norway spruce (Picea abies (L.) Karst.) and hardwood—sycamore maple (Acer pseudoplatanus L.)), the thickness of the back wall of the pipe and the pressure in the windchest on the resulting sound of the wooden organ pipe. The measurement of the physical and acoustic characteristics of wood was performed by the method of modal analysis with harmonic excitation using Chladni patterns. The sound of the organ pipe when the thickness of the back wall was gradually reduced (7 mm, 6 mm, 5 mm, 4 mm, 3 mm, 2 mm, and 1 mm—spruce wood; 6 mm, 5 mm, 4 mm, 3 mm, 2 mm, 1 mm and 0.5 mm—maple wood) and when the pressure in the air system was gradually increased (588 Pa, 716 Pa, 814 Pa and 941 Pa—at each thickness) was evaluated using the frequency analysis.

2. Materials and Methods

In the experiment, boards of Norway spruce wood (Picea abies (L.) Karts.) and sycamore maple wood (Acer pseudoplatanus L.) were used. The boards prepared of spruce wood (marked SW) had from 6 to 8 annual rings per centimeter and were free of cracks, knots, and biological and other defects. Almost all boards of maple wood (marked MW) had a unique wavy pattern in the grain. The boards were sawn radially into the shape of a thin right-angle board (thickness h = 7 mm, width b = 30 mm and length (along the wood grain) a = 293 mm). The dimensions of the boards were chosen in such a way that they met the requirements of the measurement method, and they could be used in the second step of the experiment without dimensional adjustments. The prepared boards were stored for four weeks in the laboratory (where the relative air humidity was φ = 55% and the air temperature was t = 20 °C). The final moisture content of the wood, which was determined by the gravimetric method, reached w ≈ 10%.

The experiment consisted of two steps. In the first step of our experiment, the physical and acoustic characteristics of boards were determined, i.e., density ρ, resonant frequency f_r, modulus of elasticity along the grain E_L, specific modulus (E_sp), acoustic constant A, speed of sound along the grain c_L, logarithmic decrement ϑ, loss coefficient η, acoustic conversion efficiency (ACE) and sound quality factor Q.

The density of each board was determined by the direct method, i.e., dimensions and mass were measured, and then the density was calculated as the ratio of mass to volume.

The method of Chladni patterns was used to obtain the resonant frequency of the 4th vibration mode (2.0) of a rectangular board with free edges [20]. The VIBROVIZER device was used for the measurements (Figure 1).

The measuring device consists of a tone generator producing a sinusoidal audio signal, which is amplified by a power amplifier. A loudspeaker is connected to the amplifier, which vibrates the tested board. The board is supported on four small pieces of soft foam in a horizontal position above the loudspeaker mounted under a hole in a box. The board is sprinkled with tea leaves, which will begin to collect along the nodal lines of a particular vibration mode upon resonance. Knowing the resonant frequency of vibration mode (2.0), it is possible to determine the modulus of elasticity E_L (Pa).

The formulas for calculating the modulus of elasticity E_L (Pa) are

$$E_L=D_1·12·\eta ;\hspace{1em}\eta =1-{\mu }_{LR}·{\mu }_{RL};D_1\approx 0.0789·{\displaystyle \frac{f_4^2·\rho ·a^4}{h^2}}$$

(1)

where D₁ is the elastic constant, f₄ (Hz) is the resonant frequency of the 4th mode (2.0), ρ (kg·m⁻³) is the density, a (m) is the length of wooden plate, h (m) is the thickness and μ_LR, μ_RL are Poisson’s ratios. The calculation of other PACHs (E_sp, A, c_L, ϑ, η, ACE and Q) was performed according to the following Equations (2)–(9) [8,21,22,23].

The specific modulus of elasticity E_sp (m²·s⁻²):

$$E_{sp}={\displaystyle \frac{E_L}{\rho }}$$

(2)

The acoustic constant A (m⁴·kg⁻¹·s⁻¹), also well known as sound radiation coefficient—R:

$$A=\sqrt{{\displaystyle \frac{E_L}{{\rho }^3}}}$$

(3)

The speed of sound c_L (m·s⁻¹):

$$c_{\mathrm{L}}=\sqrt{{\displaystyle \frac{E_L}{\rho }}}$$

(4)

The logarithmic decrement $\vartheta$(-):

$$\vartheta ={\displaystyle \frac{\mathsf{\pi }}{\sqrt{3}}}.{\displaystyle \frac{f_2-f_1}{f_r}}$$

(5)

The loss coefficient η (-):

$$\eta ={\displaystyle \frac{\vartheta }{\pi }}$$

(6)

The acoustical converting efficiency—ACE (m⁴·kg⁻¹·s⁻¹):

$$ACE={\displaystyle \frac{A}{\eta }}$$

(7)

The sound quality factor Q (-):

$$Q={\displaystyle \frac{1}{\eta }}$$

(8)

The characteristic acoustic impedance z (kg∙s⁻¹∙m⁻²):

$$z=\rho ·c_L$$

(9)

In the second step, an experimental wooden organ pipe (Figure 2) was made upon a scale corresponding to a medium-sized organ pipe (

Table 1. Scaling data of the experimental organ pipe.

Scaling Data	Experimental Pipe
Body length of pipe	293 mm
Pipe width	20 mm
Pipe depth	29 mm
Thickness of wall A	5 mm
Thickness of wall B	5 mm
Thickness of wall C	7 mm
Thickness of wall D	5 mm
Height of cut up	7 mm
Foot diameter	4.2 mm

).

The pipe was made of Norway spruce (Picea abies (L.) Karts.), while the core of the pipe was made of sessile oak (Quercus petraea), and the overlay was made of wild pear (Pyrus pyraster (L.) Burgsd.). The wood used to make the experimental wooden organ pipe was chosen so that the pipe could be used over a wide range of air pressures. The height of the experimental pipe cutout was also adjusted to the range of air pressures. The boards of maple and spruce wood were used for the back wall of the experimental wooden organ pipe. The back wall does not interfere directly with the sound-producing elements of the pipe; therefore, it does not have an immediate effect on the process of tone creation, but it can influence the resulting sound of the pipe.

The air pressure in the windchest was gradually increased to 588 Pa, 716 Pa, 814 Pa and 941 Pa. The stated range of air pressures was chosen with respect to the real pressures occurring within organ positives.

The experimental organ pipe was made so that the back wall could be replaced. The board was glued to the body of the pipe with a hide glue (also used in the production and repair of violins). After recording the sound frequency spectrum at a certain back wall thickness and pressure, the joint was non-abrasively removed using a biopreparation [24]. The wooden board used for the back wall of organ pipe had an initial thickness of 7 mm (resp. 6 mm for maple wood). The board was gradually thinned in steps by 1 mm to a thickness of 1 mm (resp. 0.5 mm for maple wood). At each back wall thickness, the frequency spectrum of the sound at each of the above air pressure values were recorded.

The sounds of the experimental wooden organ pipe were measured in the semi-anechoic room of the Faculty of Civil Engineering of the Slovak University of Technology (STU). The methodology and measurement system (Figure 3) developed by our research team were used [25].

The measuring set consists of two parts. In the first part, the pipe tone is excited and recorded by a microphone. The pipe tone excitation ensures an experimental windchest connected to the bellows, an air pressure regulator, and an air pump (Laukhuff brand).

The second part of the measuring set is used for evaluating the sound frequency spectra in real time using the acoustic software ARTA version 1.9.3 (Audio Measurement and Analysis). This software contains the program ARTA.EXE for the real-time spectrum analysis and frequency response measurements as well as the program STEPS.EXE for frequency response measurements. This program, simultaneously with a frequency response measurement, estimates the levels of the 2nd-, 3rd-, 4th-, 5th-, and higher-order harmonic frequencies. The DAW (Digital Audio Workstation) Samplitude 11.0 software, which allows working with several audio files simultaneously, was used to record and edit the digitized sound.

The sound of the organ pipe was recorded by a Shure SM81 microphone, was amplified in a microphone preamplifier, and from its output went to the distributor, where it was divided into two branches. The first led into the A/D converter, where the sound was digitized. The digitized sound was then evaluated in the ARTA acoustic software. At the same time, the signal was recorded in the audio DAW program and a sound file was created corresponding to the analyzed sound spectrum. The second branch from the distributor led to the Brüel & Kjær type 2270 sound level meter (SPL), which was used to measure and evaluate the sound pressure level. This configuration allowed an assignment of a corresponding audio signal to each evaluated spectrum.

During the measurements, the parameters were set for the RTSA (Real Time Spectrum Analyzer): sample frequency 44.1 kHz; the number of samples in the measured window 8192; weighting window “Hanning” and linear averaging from 100 samples.

3. Results

3.1. Physical and Acoustic Characteristic Spuce and Maple Wood Boards

The mean values (MV), standard deviation (SD) and coefficient of variation (CV) of the physical and acoustic characteristics (density ρ, dynamic modulus of elasticity E_L, specific modulus (E_sp), acoustic constant A, speed of sound along the grain c_L, logarithmic decrement ϑ, characteristic acoustic impedance z, loss coefficient η, acoustic conversion efficiency (ACE) and sound quality factor Q) of spruce and maple boards are given in

Table 2. PACHs (density ρ, dynamic modulus of elasticity E_L, acoustic constant A, speed of sound c_L, resonant frequency f_r, damping decrement ϑ, loss coefficient η, characteristic acoustic impedance z, acoustic conversion efficiency (ACE), sound quality factor Q of spruce wood—SW and maple wood—MW.

PACH		Norway Spruce (Picea abies (L.) Karst.)	Sycamore Maple (Acer pseudoplatanus, L.)
	n	12	12
ρ (kg∙m−3)	MV	452	669
	SD	42.8	22.5
	CV (%)	9.0	3.0
EL (GPa)	MV	13.38	11.69
	SD	1.08	1.18
	CV (%)	8.1	9.3
Esp (106 m2∙s−2)	MV	29.60	17.43
	SD	0.031	0.033
	CV (%)	0.1	0.2
cL (m·s−1)	MV	5440	4180
	SD	355.5	289.5
	CV (%)	6.5	6.9
A (m4∙kg−1·s−1)	MV	12.04	6.25
	SD	1.15	0.82
	CV (%)	9.6	13.1
$\vartheta$ (-)	MV	0.034	0.053
	SD	0.00329	0.00417
	CV (%)	9.7	7.9
η (-)	MV	0.0108	0.0169
	SD	0.0009	0.0017
	CV (%)	8.3	10.1
z (105∙kg∙s−1∙m−2)	MV	24.59	27.96
	SD	2.34	2.91
	CV (%)	9.5	10.4
Q	MV	92.59	59.17
	SD	5.8	5.2
	CV (%)	6.3	8.1
ACE (m4∙kg−1·s−1)	MV	1115	399
	SD	92	42
	CV (%)	8.3	10.5

Note: n—number of boards; MV—mean value; SD—standard deviation; CV—coefficient of variation.

).

The influence of the wood species (maple and spruce) on the PACHs (ρ, E_L, E_sp, c_L, A, ϑ, η, z, ACE) was evaluated in Statistica software (Statsoft Inc., version 7, Prague, Czech Republic). The one-way analysis of variance (ANOVA) was used. According to the ANOVA test, there is statistically highly significant difference between the density ρ (Fρ = 0.62, p << 0.05), acoustic constant A (F_A = 0.03, p_A << 0.05), speed of sound c_L (F_cL = 0.08, p_cL << 0.05), logarithmic decrement $\vartheta \left(\mathrm{F}\upsilon =1.08,\mathrm{p}\mathsf{\upsilon }<<0.05\right), \mathrm{l}\mathrm{o}\mathrm{s}\mathrm{s} \mathrm{c}\mathrm{o}\mathrm{e}\mathrm{f}\mathrm{f}\mathrm{i}\mathrm{c}\mathrm{i}\mathrm{e}\mathrm{n}\mathrm{t}$ η (F_η = 1.03, p_η << 0.05), characteristic acoustic impedance z (F_z = 0.81, p_η << 0.05; F_ACE = 0.08) and acoustic conversion efficiency ACE (F_ACE = 0.08, p_ACE << 0.05) of spruce wood and maple wood.

3.2. FFT Analysis—Harmonic Frequencies of the Sound of an Experimental Wooden Organ Pipe

3.2.1. Influence of the Thickness of Back Wall—Spruce Wood on the Frequency Spectrum

The fundamental frequencies of tone for individual thicknesses of the back wall of the experimental wooden organ pipe made of spruce wood can be found in the

Table 3. Fundamental frequencies of wooden organ pipe tone (back wall of spruce wood—SW).

Plate Thickness	7 mm	6 mm	5 mm	4 mm	3 mm	2 mm	1 mm
Air Pressure in Windchest	Fundamental Frequency f (Hz)
588 Pa	516.80	516.80	511.41	511.41	516.80	511.41	506.03
716 Pa	518.80	516.80	516.80	516.80	516.18	515.80	511.41
814 Pa	522.18	522.18	522.18	522.18	522.18	521.18	511.41
941 Pa	522.18	522.18	522.18	522.18	522.18	521.18	511.41

at pressures of 588 Pa, 716 Pa, 814 Pa and 941 Pa.

Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9 and Figure 10 show the harmonic frequencies of the frequency spectra of the sound of the wooden pipes for each thickness of the spruce wood board which was used for the back wall of the wooden organ pipe (i.e., 7, 6, 5, 4, 3, 2 and 1 mm) at individual air pressures (i.e., 588 Pa, 716 Pa, 814 Pa and 941 Pa).

From Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9 and Figure 10, it is evident that when the thickness of the back wall of pipe made of spruce wood is reduced, there is no significant change in the sound pressure level of the fundamental frequency. The difference was only 2–5 dB.

3.2.2. Influence of the Thickness of Back Wall—Maple Wood on the Frequency Spectrum

The fundamental frequencies of tone for individual thicknesses of the back wall of the experimental wooden organ pipe made of maple wood are presented in the

Table 4. Fundamental frequencies of wooden organ pipe tone (back wall of maple wood—MW).

Plate Thickness	6 mm	5 mm	4 mm	3 mm	2 mm	1 mm	0.5 mm
Air Pressure in Windchest	Fundamental Frequency f (Hz)
588 Pa	522.18	522.18	522.18	522.18	522.18	522.18	516.80
716 Pa	527.56	527.56	527.56	527.56	527.56	523.18	522.18
814 Pa	527.56	527.56	527.56	527.56	527.56	527.56	522.18
941 Pa	527.56	527.56	527.56	527.56	527.56	527.56	527.56

at pressures of 588 Pa, 716 Pa, 814 Pa and 941 Pa.

Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16 and Figure 17 show the harmonic frequencies of the frequency spectra of the sound of the wooden pipes for each thickness of the spruce wood board which was used for the back wall of the wooden organ pipe (i.e., 6, 5, 4, 3, 2, 1 and 0.5 mm) at individual air pressures (i.e., 588 Pa, 716 Pa, 814 Pa and 941 Pa).

Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16 and Figure 17 show that when reducing the thickness of the back wall of the pipe made of maple wood, there is no significant change in the sound pressure level of the fundamental frequency or higher harmonics.

4. Discussion

4.1. Physical and Acoustic Characteristic of Wood

The quality of the sound of woodwind instruments is primarily determined by the interaction of the material with the column of vibrating air, but the characteristics of the wood (PACHs) also have a certain share in the timbre of sound of the organ pipe.

The density of spruce wood boards was in the range 410–480 kg∙m⁻³ and the density of maple wood boards was in range 640–690 kg∙m⁻³. According to the density, we can say that maple wood is a more suitable material for wind instruments. Maple wood is smoother and has less porosity than spruce wood. The walls of the organ pipe should be heavy and rigid so that there is no audible vibration of the wall due to the acoustic pressure of the standing wave in the air column. The surface of denser maple wood is smoother and has less porosity than spruce wood, and therefore the vibrational damping is lower in the organ pipes. The characteristic acoustic impedance z of maple wood is also higher than that of spruce wood, i.e., the sound absorption by the walls of a wooden organ pipe is lower.

The dynamic modulus of elasticity E_L of SW was within the interval 12 to 14 GPa, and that of MW was in the range 10.5 to 12.9 GPa. A statistically slightly significant difference was found between the E_L of maple and spruce wood (F_EL = 0.98, p_EL < 0.05). Despite the statistically significant difference, we can state that both woods are suitable for wooden organ pipes, because wood for wind instruments should have a modulus of elasticity higher than 8 GPa. However, the modulus of elasticity (as well as the density) is highly dependent on the moisture content of the wood, which varies with relative humidity and temperature. The changing moisture content of the wood is the cause of changes in the modulus of elasticity and changes in the dimensions of the wooden organ pipe, which results in the instrument being out of tune. In high-density wood, the diffusivity is lower than in low-density wood, so high-density wood absorbs moisture from the air more slowly, i.e., the rate of change of the modulus of elasticity of maple wood is smaller.

The acoustic constant A (i.e., the sound radiation coefficient) of SW was within the interval 11–13 m⁴∙kg⁻¹∙s⁻¹ and that of MW was in the interval 6.4–7.0 m⁴∙kg⁻¹∙s⁻¹. The large difference between the values of the acoustic constant A of spruce and maple wood indicates large differences in the vibroacoustic properties of these wood species. The acoustic constant A of wood for woodwind instruments can be lower, in the range 4 to 8 m⁴∙kg⁻¹∙s⁻¹, so that less acoustic energy is radiated through the pipe wall into the surroundings. For this reason, maple wood is more suitable for wooden organ pipes.

The speed of sound c_L of SW was above 5500 m·s⁻¹, while the c_L of MW was lower (from 4000 m·s⁻¹ to 4300 m·s⁻¹). The speed of sound propagation in wood for woodwind instruments should be lower than 5000 m·s⁻¹ [26]. The characteristic acoustic impedance z of MW is higher than that of SW, i.e., the reflection of sound within the pipe is higher and only a little energy transmitted.

The specific modulus of elasticity and internal friction (the loss coefficient η) are very important characteristics in the selection of wood for musical instruments. In general, wood with a low specific modulus of elasticity E_sp combined with a high loss coefficient η is suitable for woodwind instruments, i.e., wooden organ pipes. The high density and a relatively small modulus of elasticity of maple wood represents wood with a low specific modulus E_sp. In the case of spruce wood with a low density and a high E_sp, the air jet can excite vibrations of the pipe walls and it is possible that the walls will radiate sound.

The loss coefficient η is important because it shows the amount of absorbed acoustic energy. The preferred wood for wind instruments is wood in the range from 0.004 (-) to 0.008 (-). The loss coefficient η of both wood species was higher. This means the attenuation of the acoustic energy by the pipe walls will be higher. Our measurements confirmed the results of [26] that the loss coefficient η and E_L are not at all correlated. The loss coefficient η is more influenced by the surface smoothness and the wood porosity.

The quality factor Q is a descriptor of the wood quality which characterizes the time needed for attenuation of vibrations after the excitation has stopped. The resonators with high quality factors Q have low damping, so that they vibrate longer, and vice versa. The values of the wood quality factor Q for wooden organ pipes should be low. From this point of view, maple wood seems more suitable (Q_MW ≈ 60 and Q_SW ≈ 93).

The acoustic conversion efficiency (ACE) is the ratio of the radiated acoustic energy to the pipe vibration energy. Low sound radiation (acoustic constant A) combined with high internal friction (loss coefficient η) means that more sound energy is consumed by internal friction and less is radiated into the environment, which is desirable for woodwind instruments. Maple wood has a significantly lower ACE, so it is more suitable than spruce wood for wooden organ pipes.

4.2. Influence of the Thickness of the Back Wall on the Frequency Spectrum

When reducing the thickness of the back wall of the pipe, the sound pressure level is preserved, when using both spruce and maple wood. The number of higher harmonic components within one wood species does not change. A similar result was obtained by [2], who also found that the wood species does not seem to have any significant effect on the sound quality of the organ pipe. However, the sound quality differs with the thickness of the pipe wall. The walls of the pipe can vibrate under the influence of internal acoustic air pressures, and thus can affect the quality of the tone. This will happen when the playback frequency or one of its harmonics approaches the vibration frequency of the pipe wall. Backus and Hundley [6] demonstrated that the vibrating back wall reduce the fundamental frequency, which was also confirmed by our measurements in the case of back wall thicknesses of 2 mm, 1 mm and 0.5 mm for the species of wood investigated.

The decrease in the fundamental frequency with the thinning of the back wall of the pipe can also be explained by the end correction. This means that the distance where the sound pressure of the pipe equalizes with the surrounding barometric pressure shifts outward from the pipe, increasing the wavelength of the oscillating air column and lowering the fundamental frequency. If the walls of the pipe are thick enough or made of wood with a higher density, the influence of the vibrations of the pipe walls on the sound is minimal; it can only be detected by acoustic measurements, not subjectively.

The results of this study are in line with the results of similar research which was carried out on an experimental pipe with a different scale [13].

4.3. The Influence of Pressure in the Windchest on the Frequency Spectrum

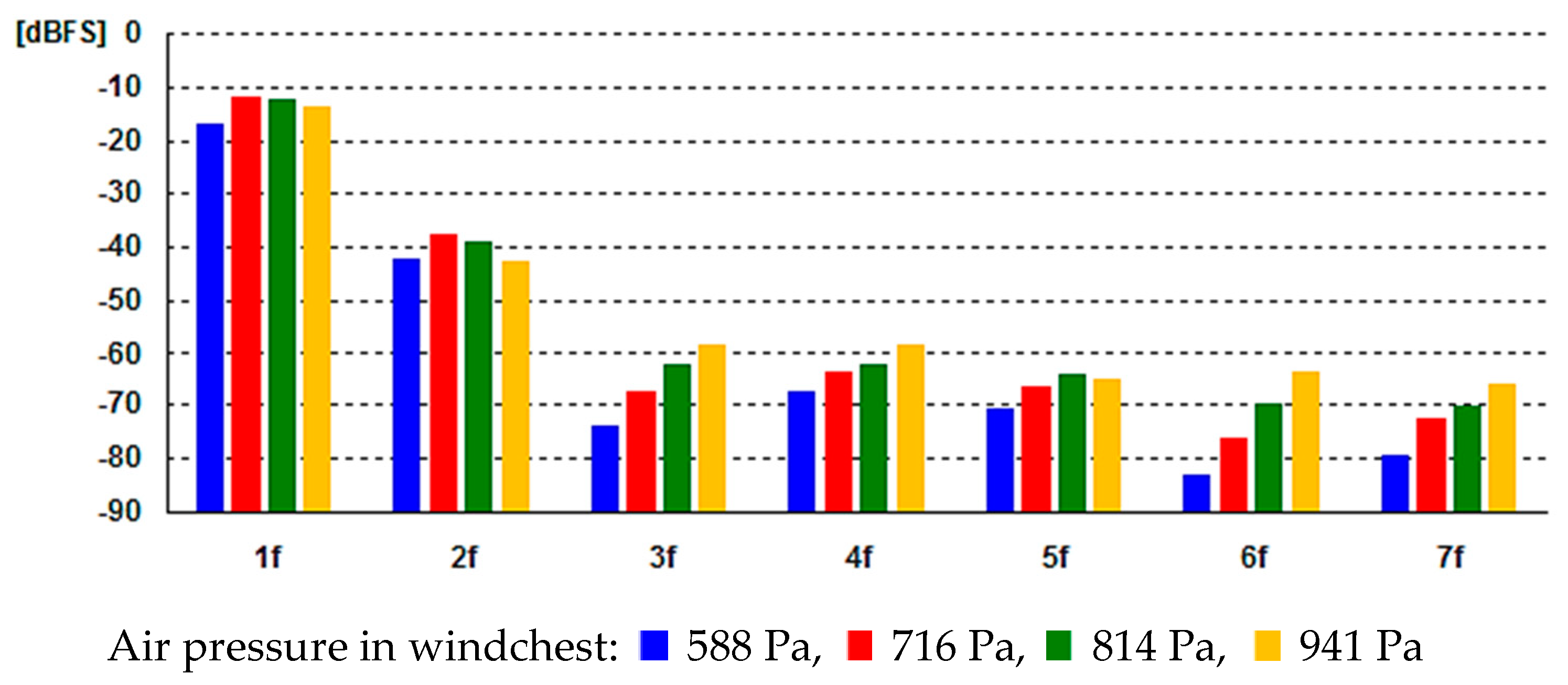
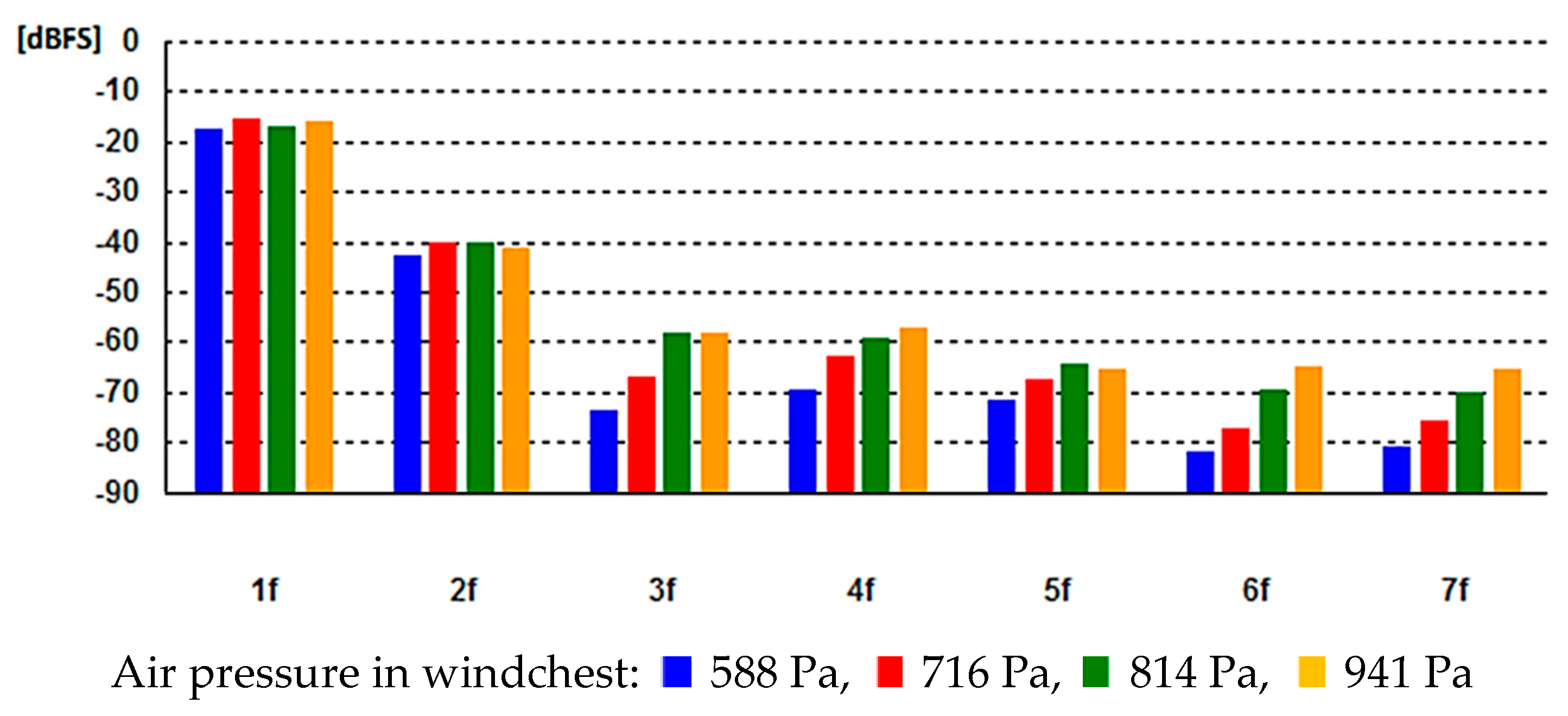
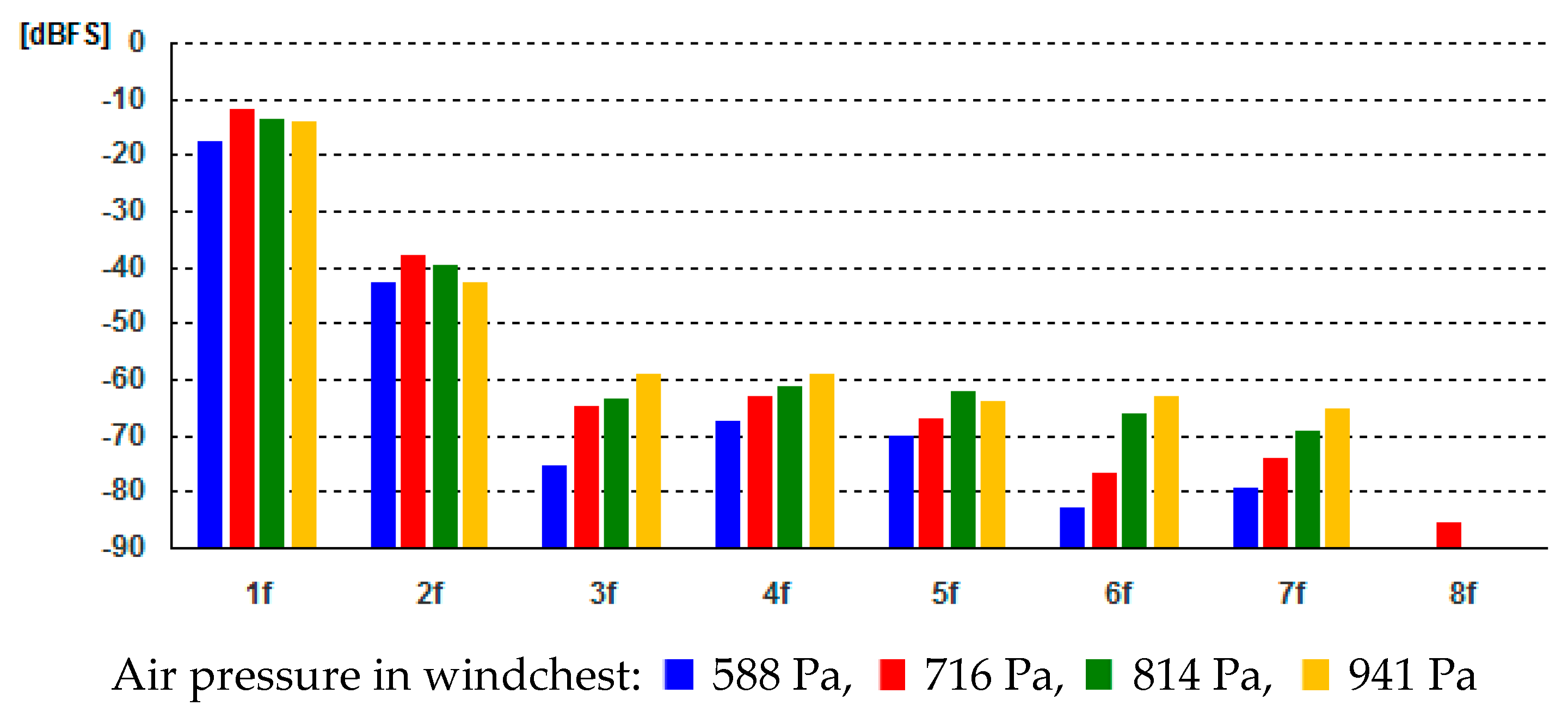
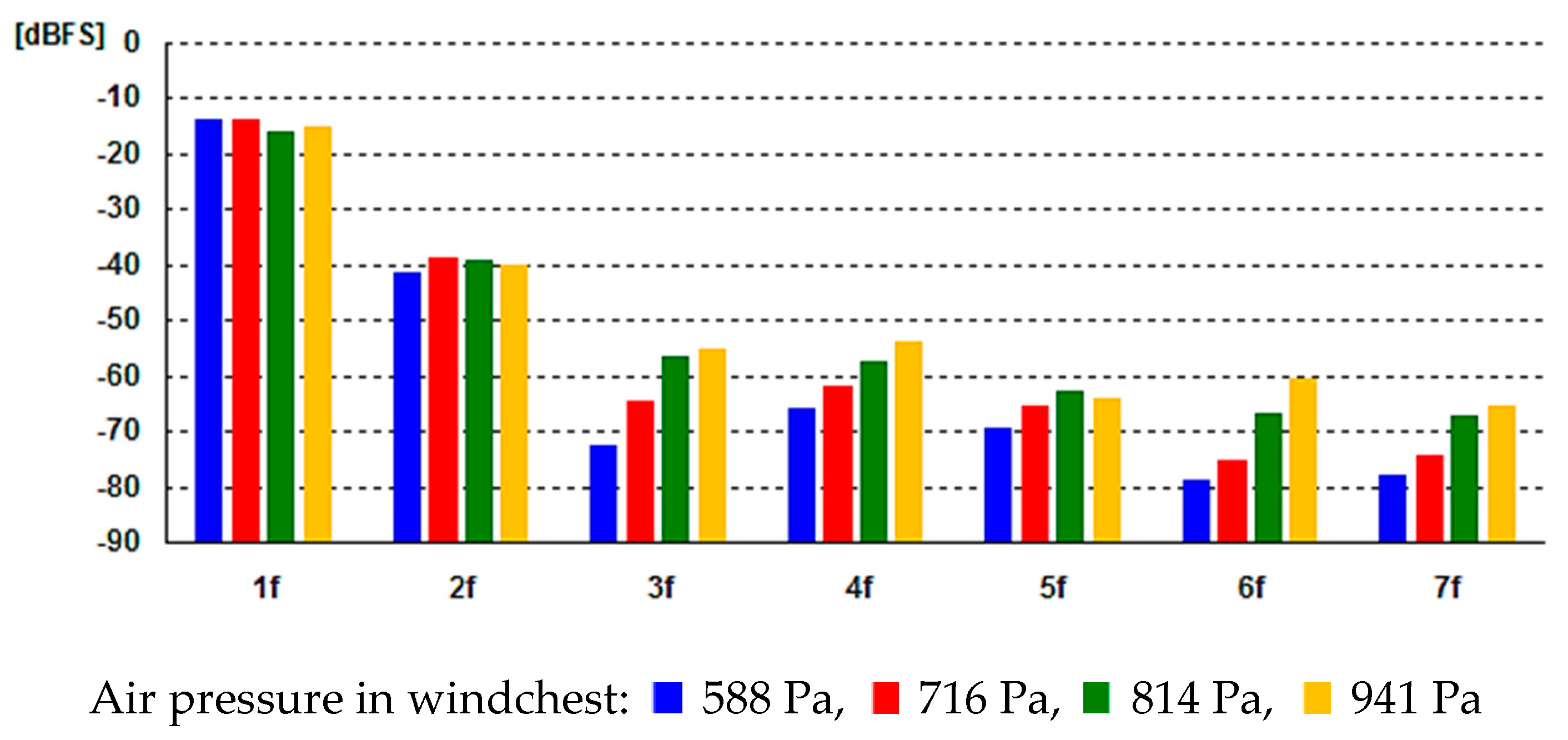
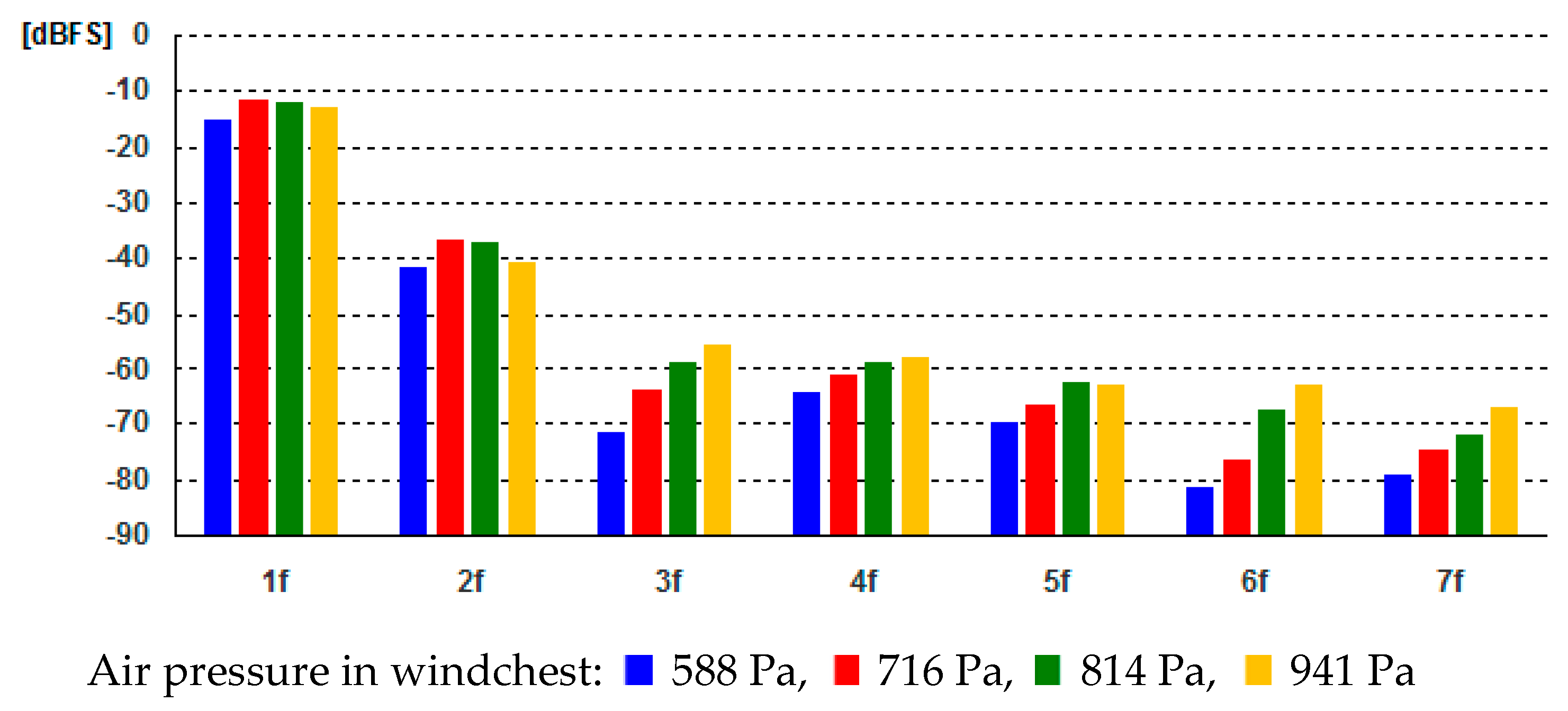
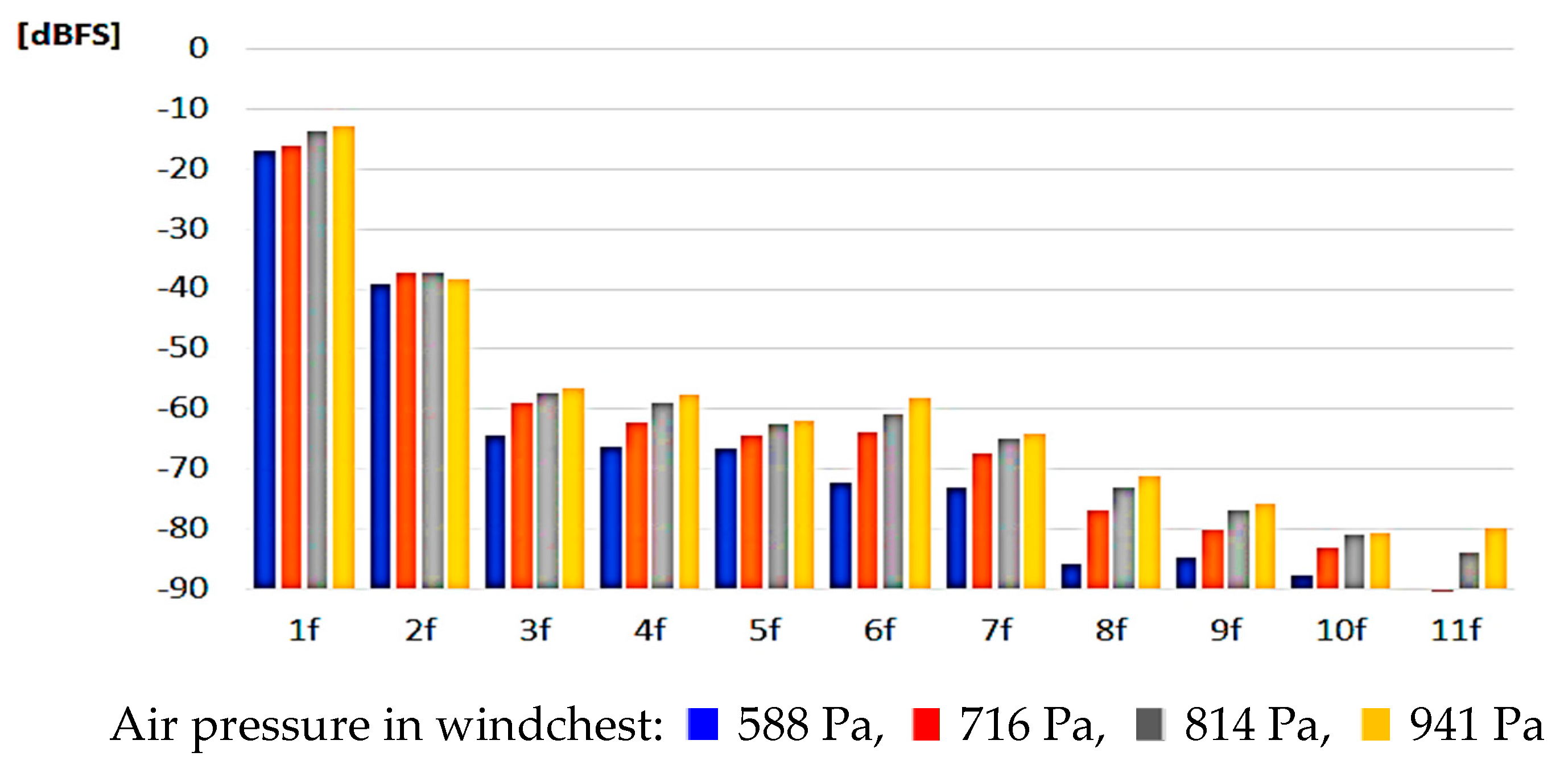
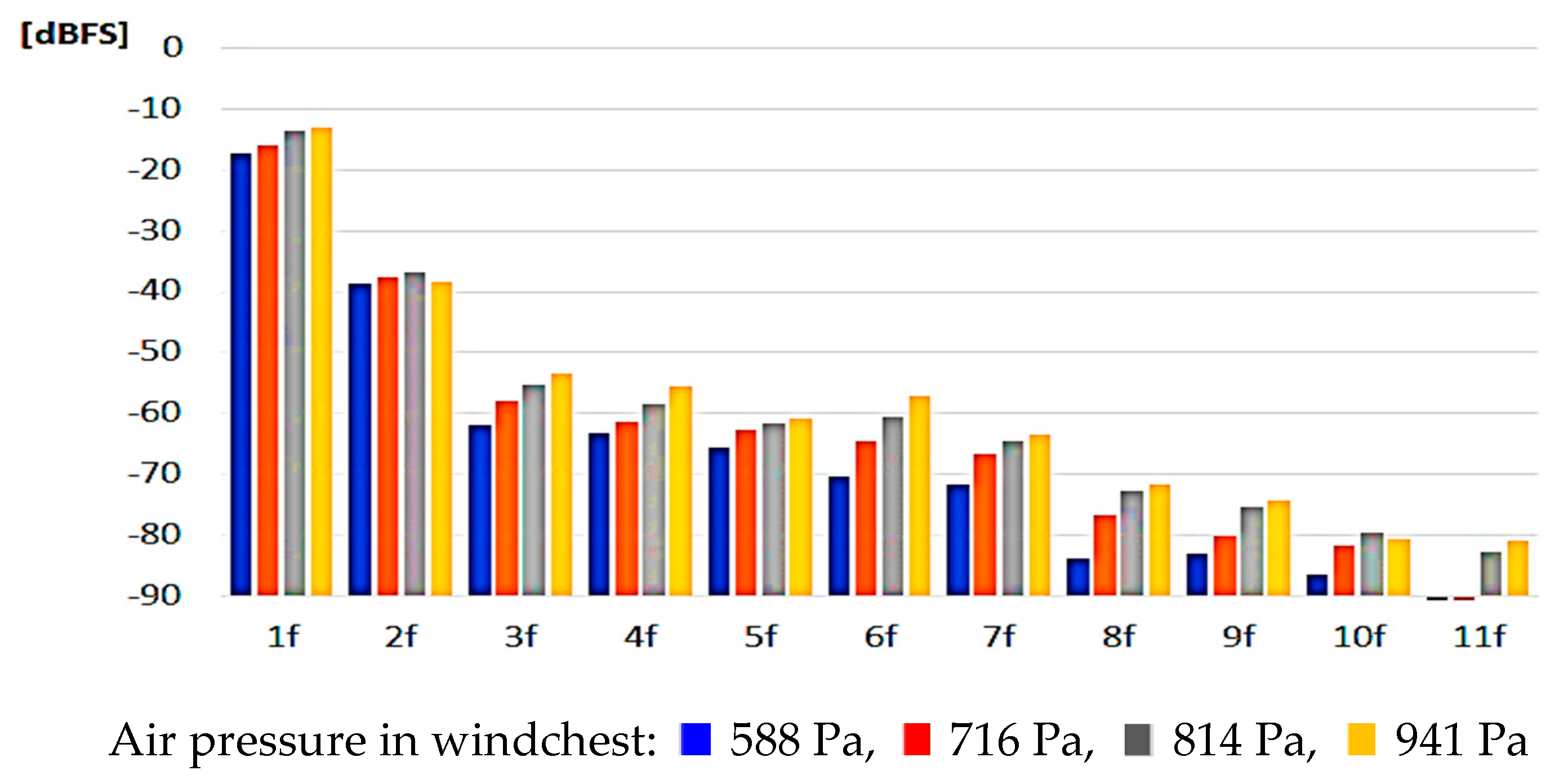
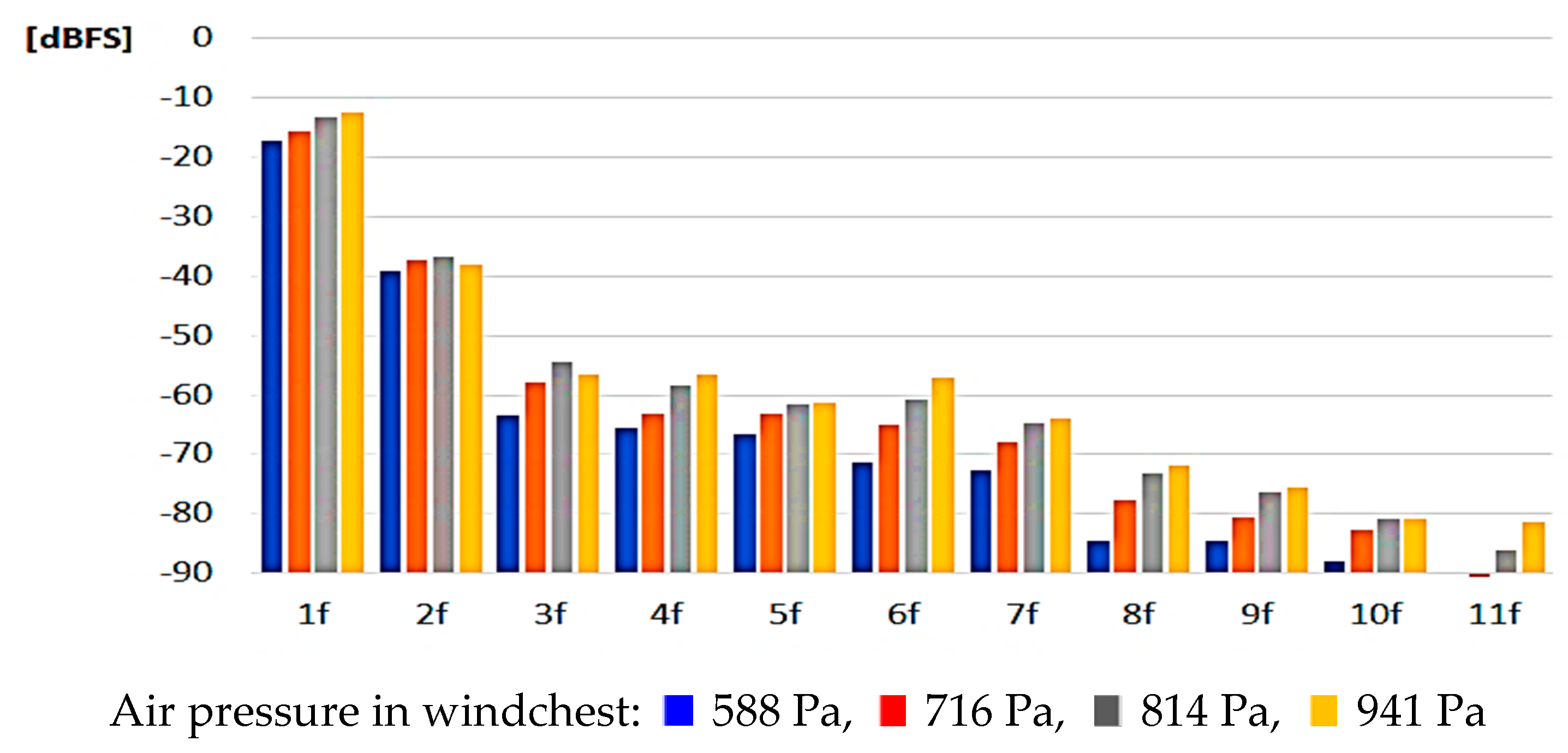
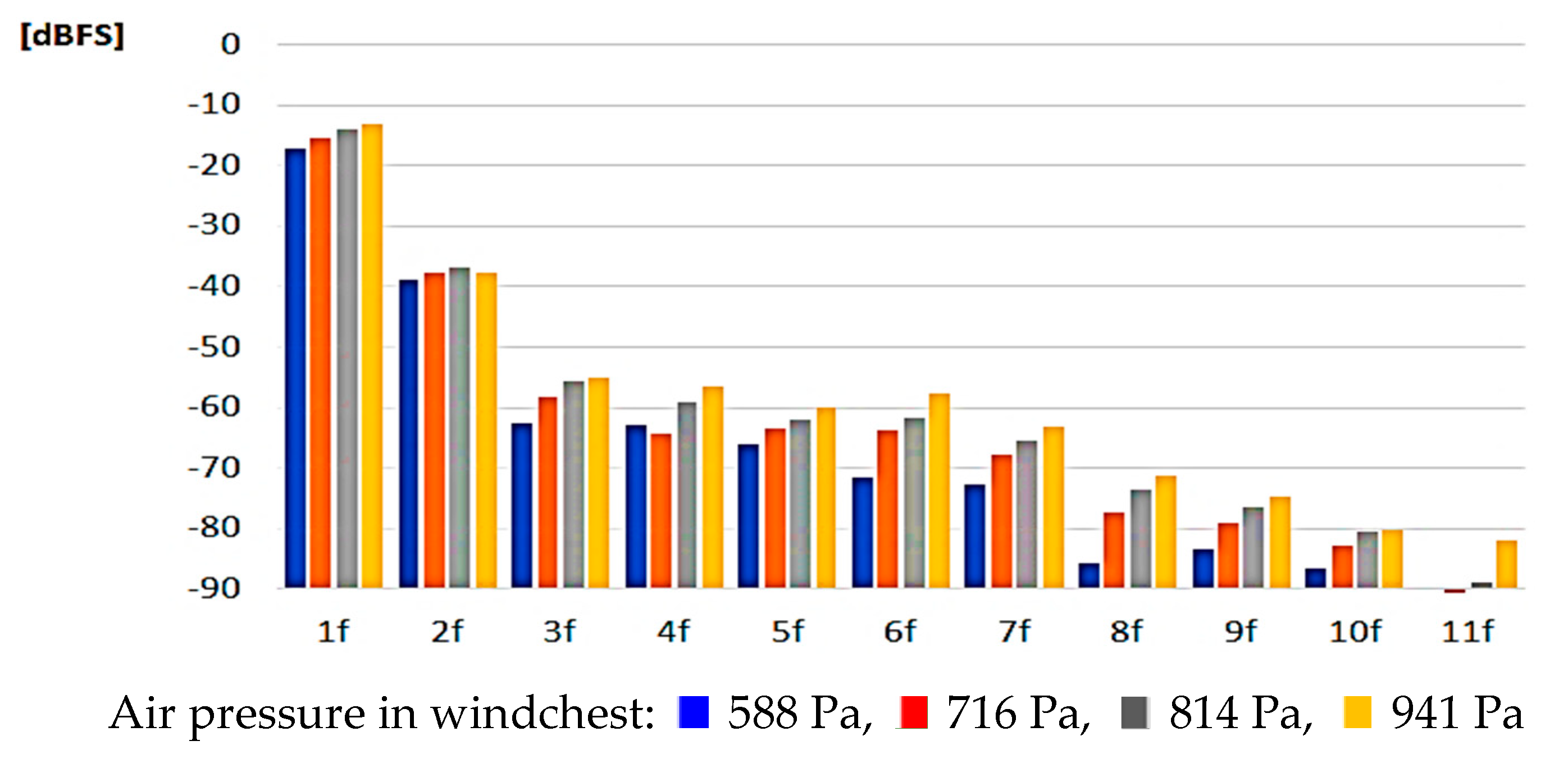
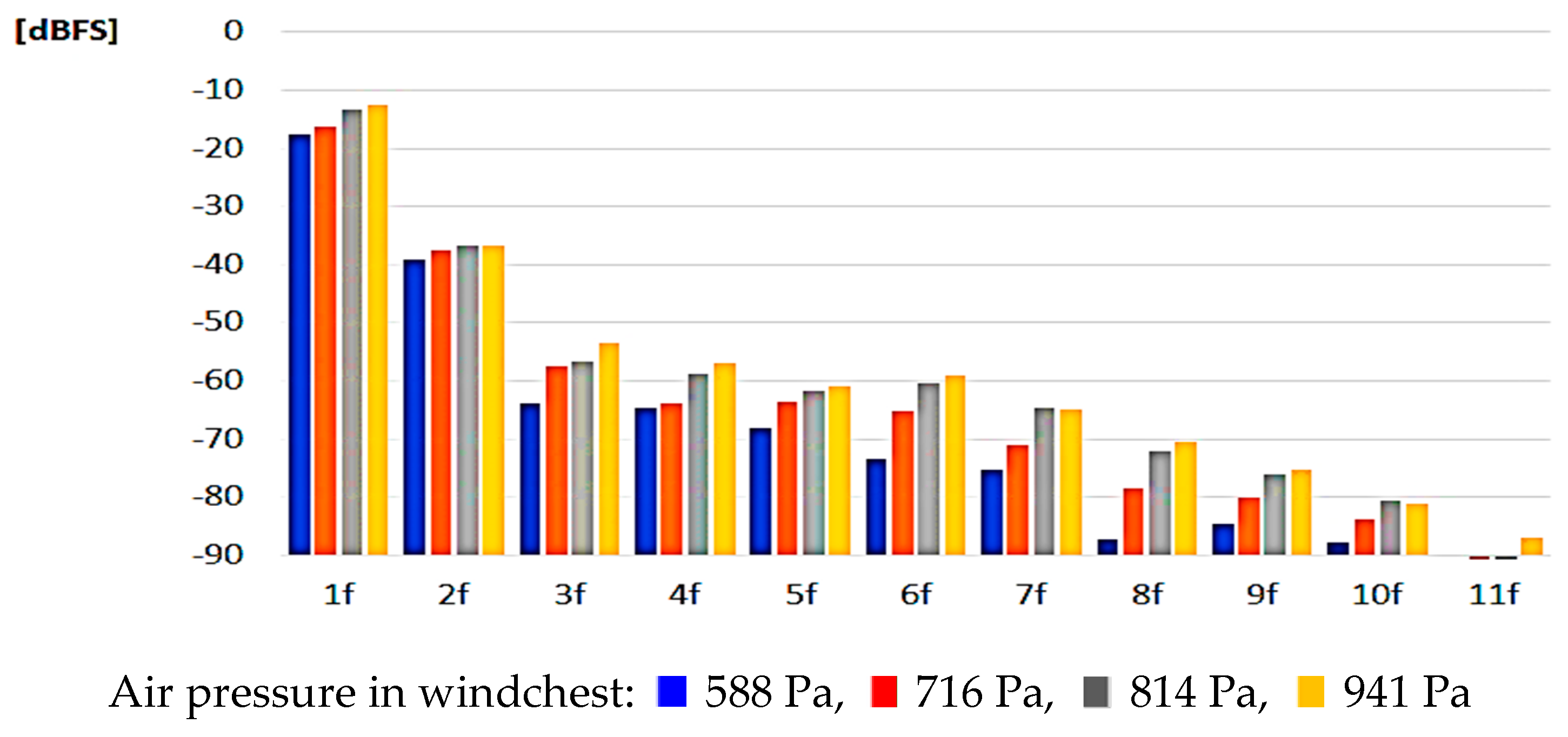
The slight decrease in the fundamental frequency of the pipe back wall was recorded at thicknesses 2 mm, 1 mm, and 0.5 mm. So, the question was whether it is possible and appropriate to compensate for this decrease by increasing the pressure in the windchest. The measurements showed that the fundamental frequency of the tone increases slightly with increasing air pressure for a given thickness of the back wall made of spruce. The fundamental frequency increased by 4–5 Hz when the pressure increased from 588 Pa to 716 Pa. The same tendency was noted when maple wood was used for the back wall of the pipe. When the pressure was further increased, the fundamental frequency remained the same for almost all monitored thicknesses of the back wall of the pipe.

The sound pressure level of the higher harmonics of the sound spectrum of the pipe (with both spruce and maple back wall) gradually increased with increasing air pressure, except for the second harmonic, when the sound pressure level was almost the same for each back wall thickness and air pressure in the windchest.

A slight decrease in intensity was found only at the fifth harmonic and an air pressure of 941 Pa, independent of the thickness of the back wall of the pipe from spruce wood. A wider representation of the higher harmonic frequencies of the wooden organ pipe was recorded when the back wall of the pipe was made from maple wood at all wall thicknesses.

It can therefore be concluded that a change in the sound pressure level of the individual higher harmonics of the sound spectrum leads to a change in the timbre of the sound.

5. Conclusions

The measurements of selected physical and acoustic characteristic of spruce and maple wood were carried out to investigate the effect of the wood species, the thickness, and the air pressure in windchest on sound quality of wooden organ pipe. The following results were obtained:

The PACHs of spruce and maple wood,

• The density ρ and the characteristic acoustic impedance z of MW is significantly higher than those of SW;
• The dynamic moduli of elasticity E_L of SW and MW are above 8 GPa;
• The acoustic constant A, the speed of sound c_L, the specific modulus E_sp and the quality factor Q of MW are significantly lower than those of SW.

These characteristics are important indicators of the suitability of wood for musical instruments. Wood with low elasticity, sound radiation and movement, but high resistance of wood to sound wave propagation and high structural strength, are suitable for wind musical instruments. Based on the above, it is clear that maple wood is more suitable for wooden organ pipes.

When reducing the thickness of back wall of organ pipe from spruce wood,

• There is no significant change in the sound pressure level of the fundamental frequency or higher harmonics;
• The sound pressure level of the fundamental frequency as well as the higher harmonics increases slightly with increasing air pressure;
• The thickness does not change the number of higher harmonic components;
• The fundamental frequency increases slightly with increasing air pressure for a given thickness.
• When reducing the thickness of back wall of organ pipe from maple wood,
• There is no significant change in the sound pressure level of the fundamental frequency or higher harmonics;
• The sound pressure level of the fundamental frequency as well as the higher harmonics increases slightly with increasing air pressure;
• The thickness does not change the number of higher harmonic components.

The fundamental frequency over the entire range of pressures and thicknesses is almost constant.

Higher harmonic frequencies were more widely represented in maple wood than in spruce wood. The results showed that spruce and maple with a smaller thickness can also be used at higher air pressures within the wooden organ pipe without a significant change in sound quality. The results will also need to be verified with different pipe scales and different intonation designs.