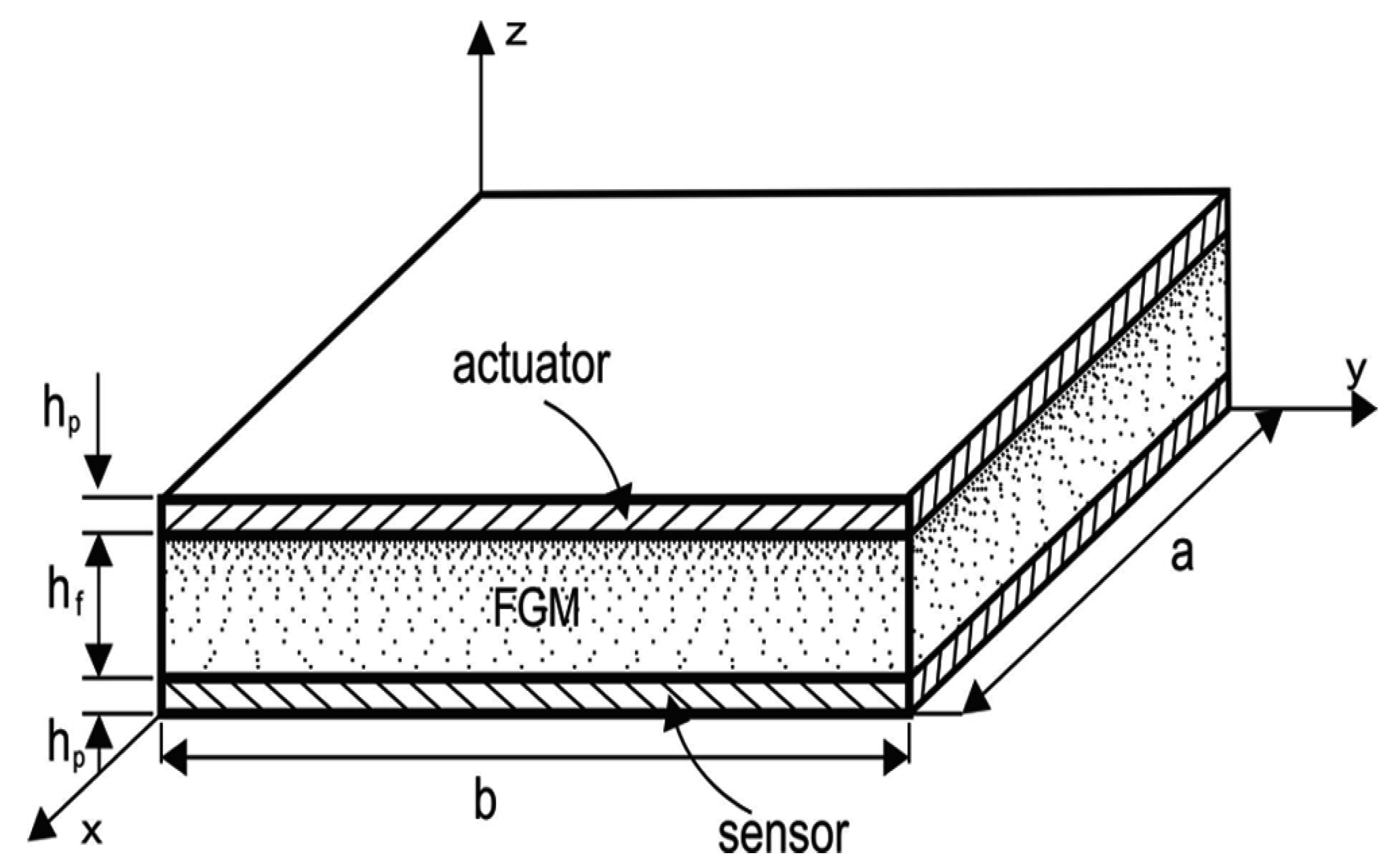
Three-Dimensional Thermoelasticity Analysis of Viscoelastic FGM Plate Embedded in Piezoelectric Layers under Thermal Load
Abstract
1. Introduction
2. Governing Equations
2.1. Temperature Field
2.2. FGM Layer
3. Solution Procedure
3.1. Temperature Gradient
3.2. FGM Layer
3.3. Piezoelectric Layer
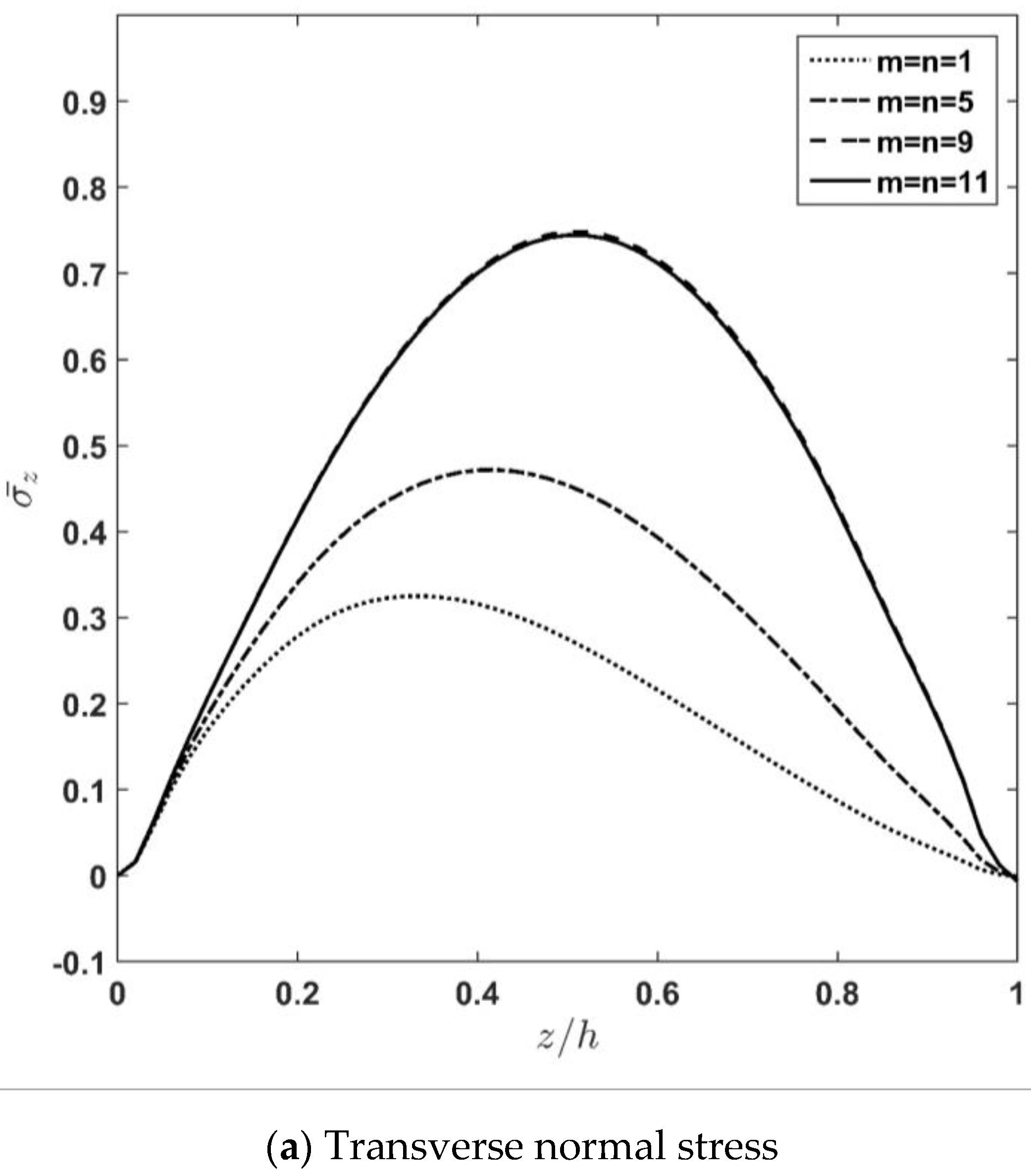
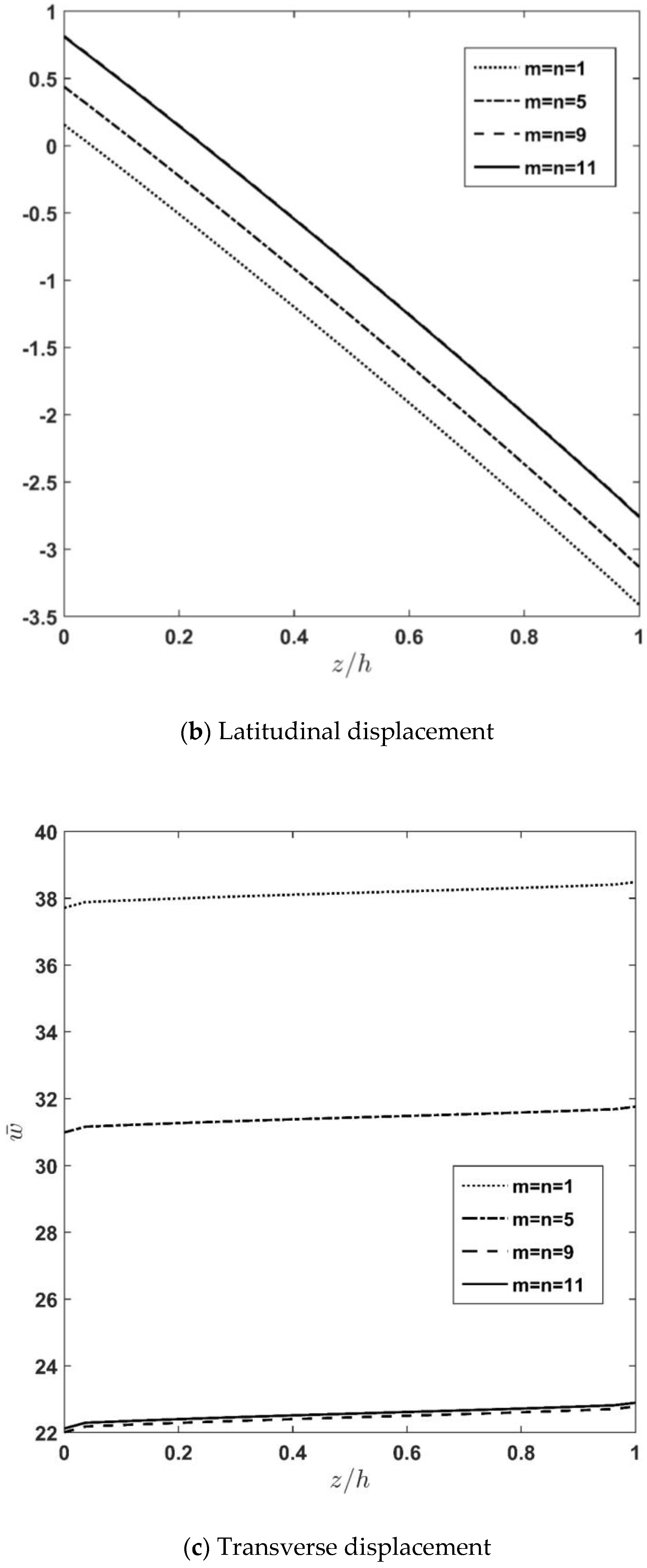
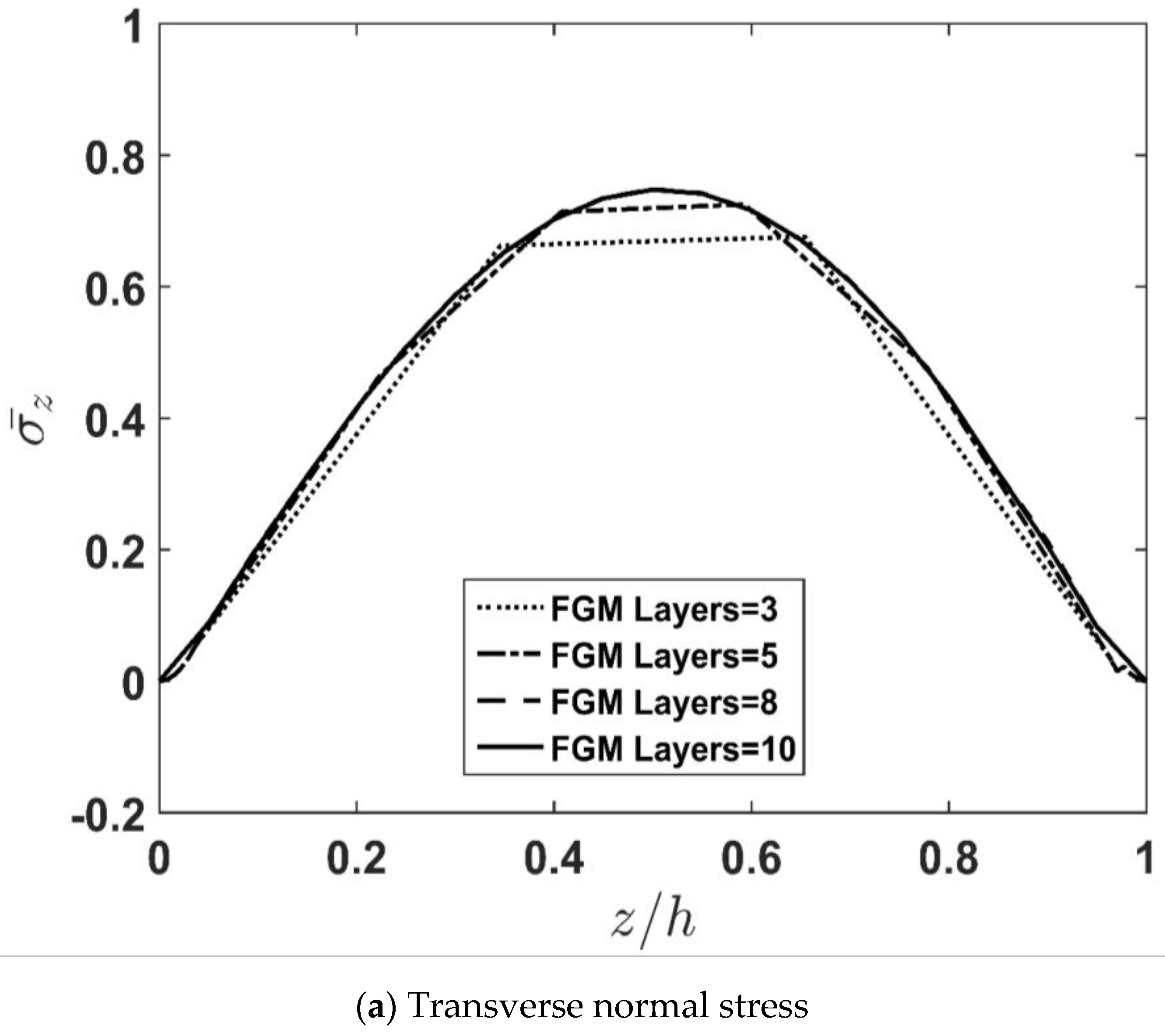
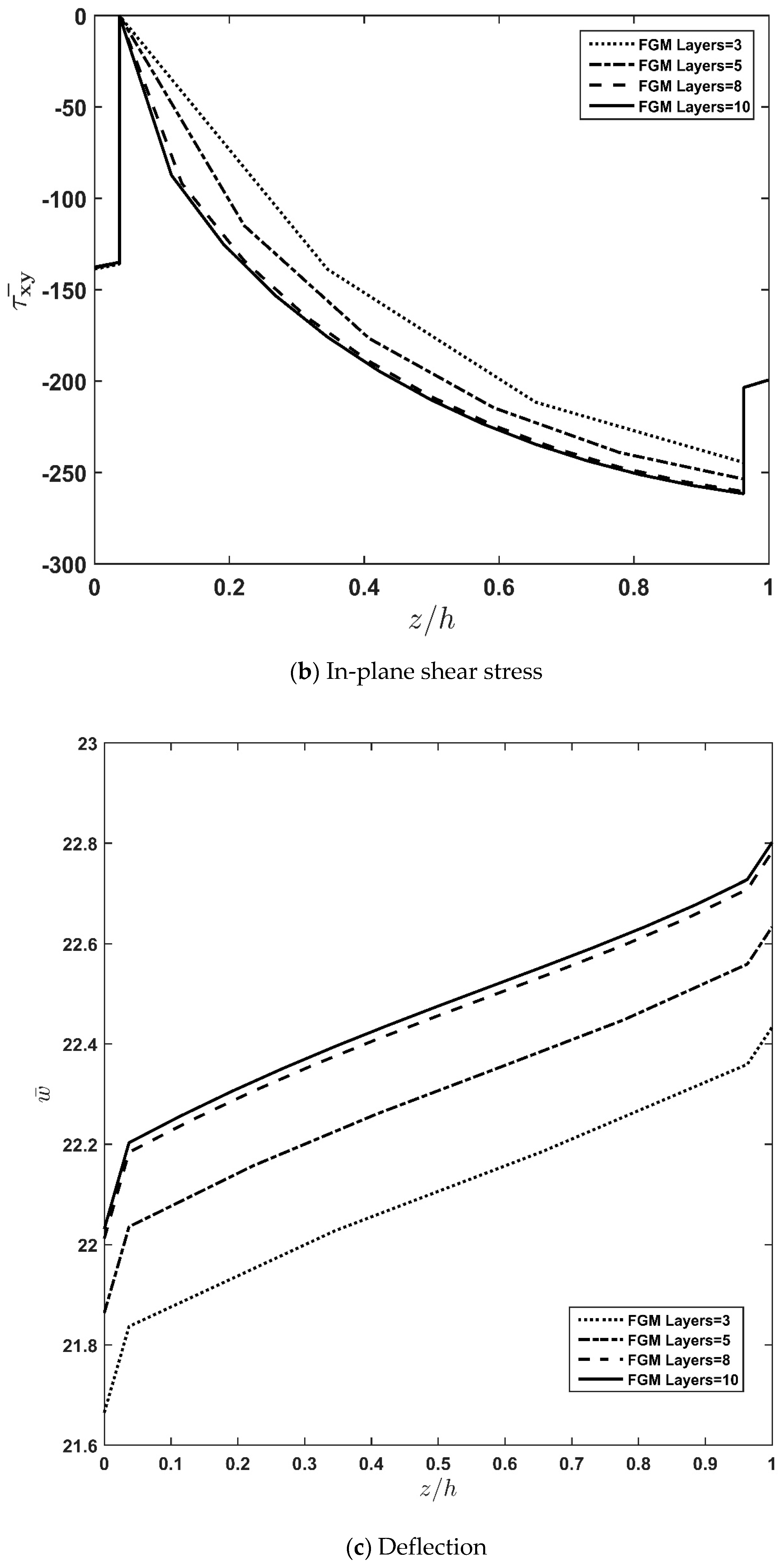
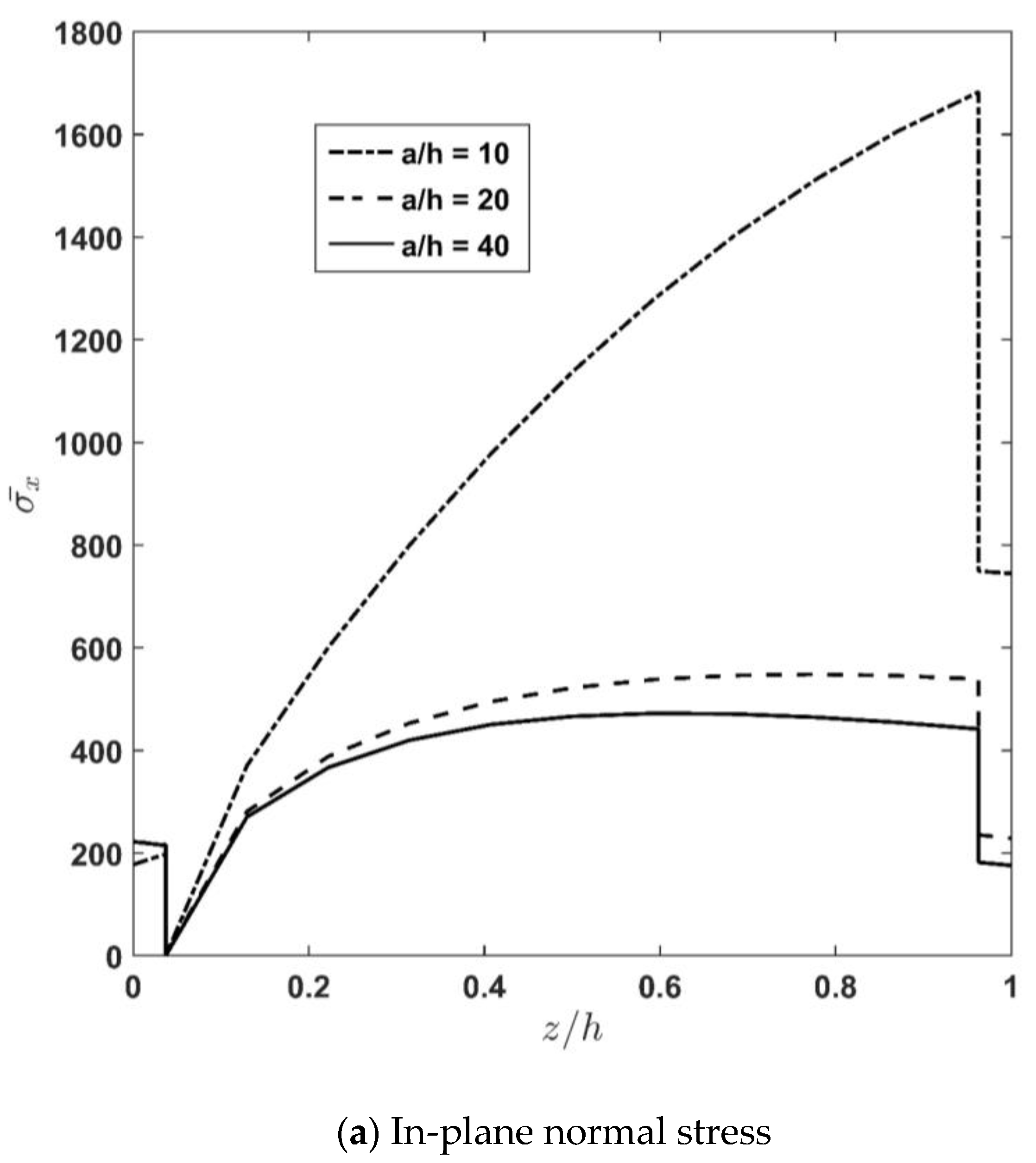
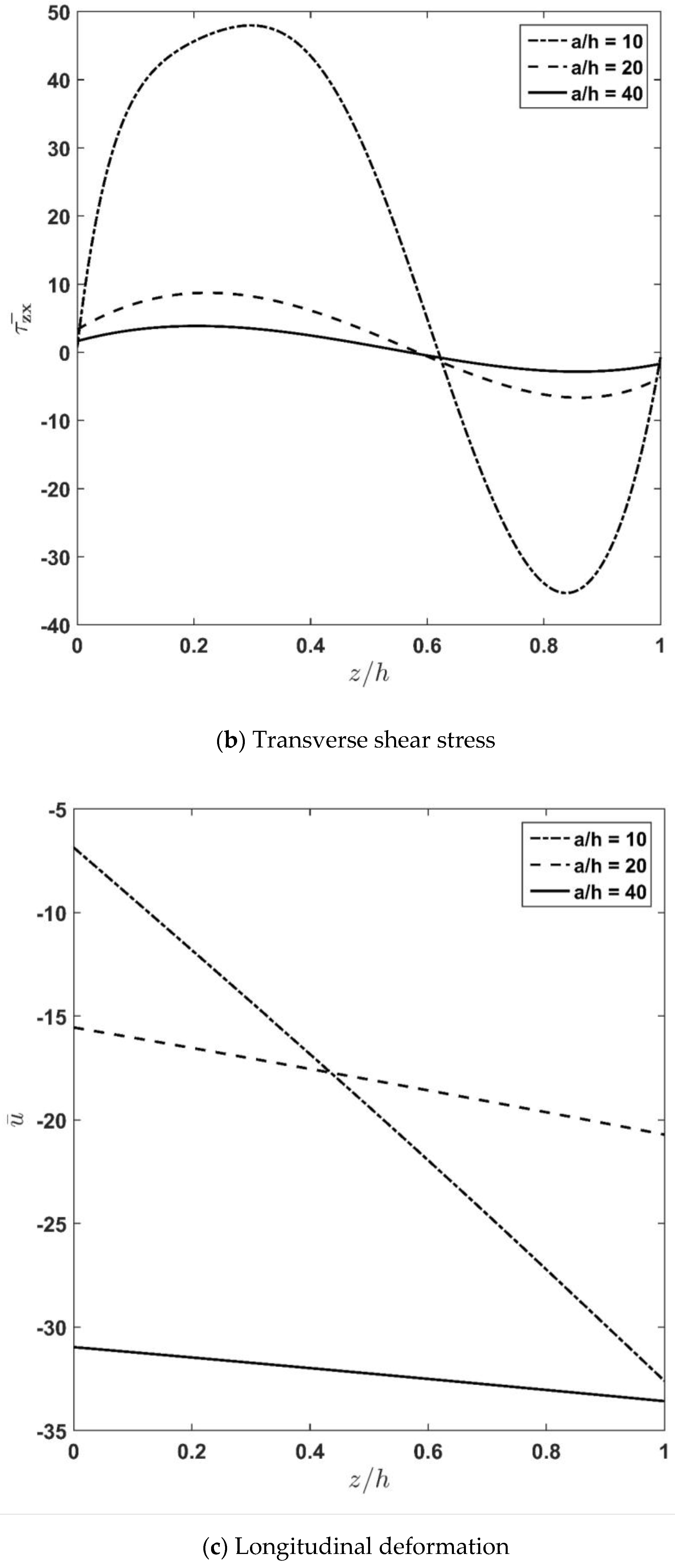
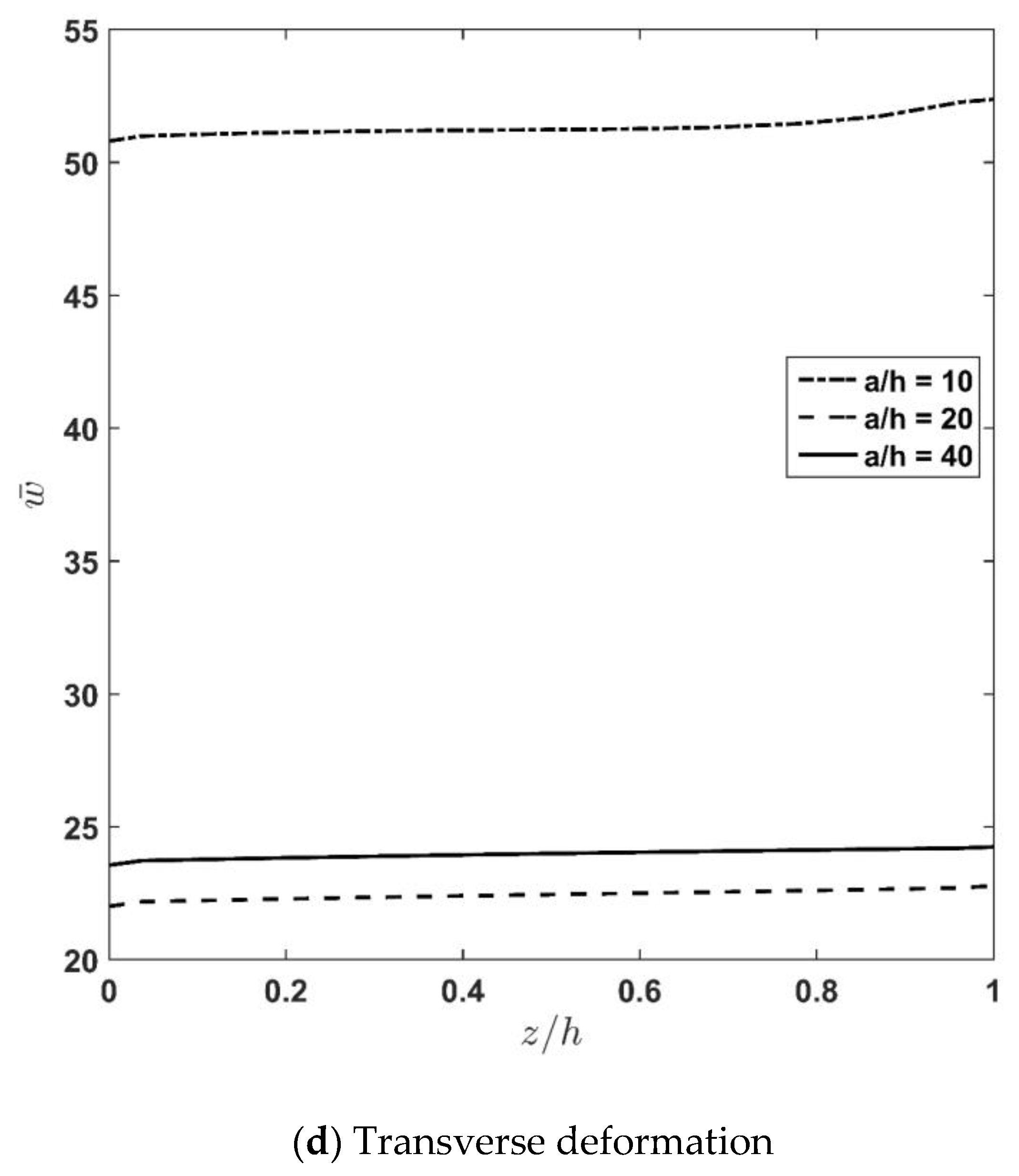
4. Numerical Results and Discussion
5. Conclusions
- Increasing the length-to-thickness ratio leads to decrease in deflections and increase in stresses.
- In the absence of an applied voltage when , the effect of the piezoelectric layer thickness on the thermo-elastic behavior becomes negligible.
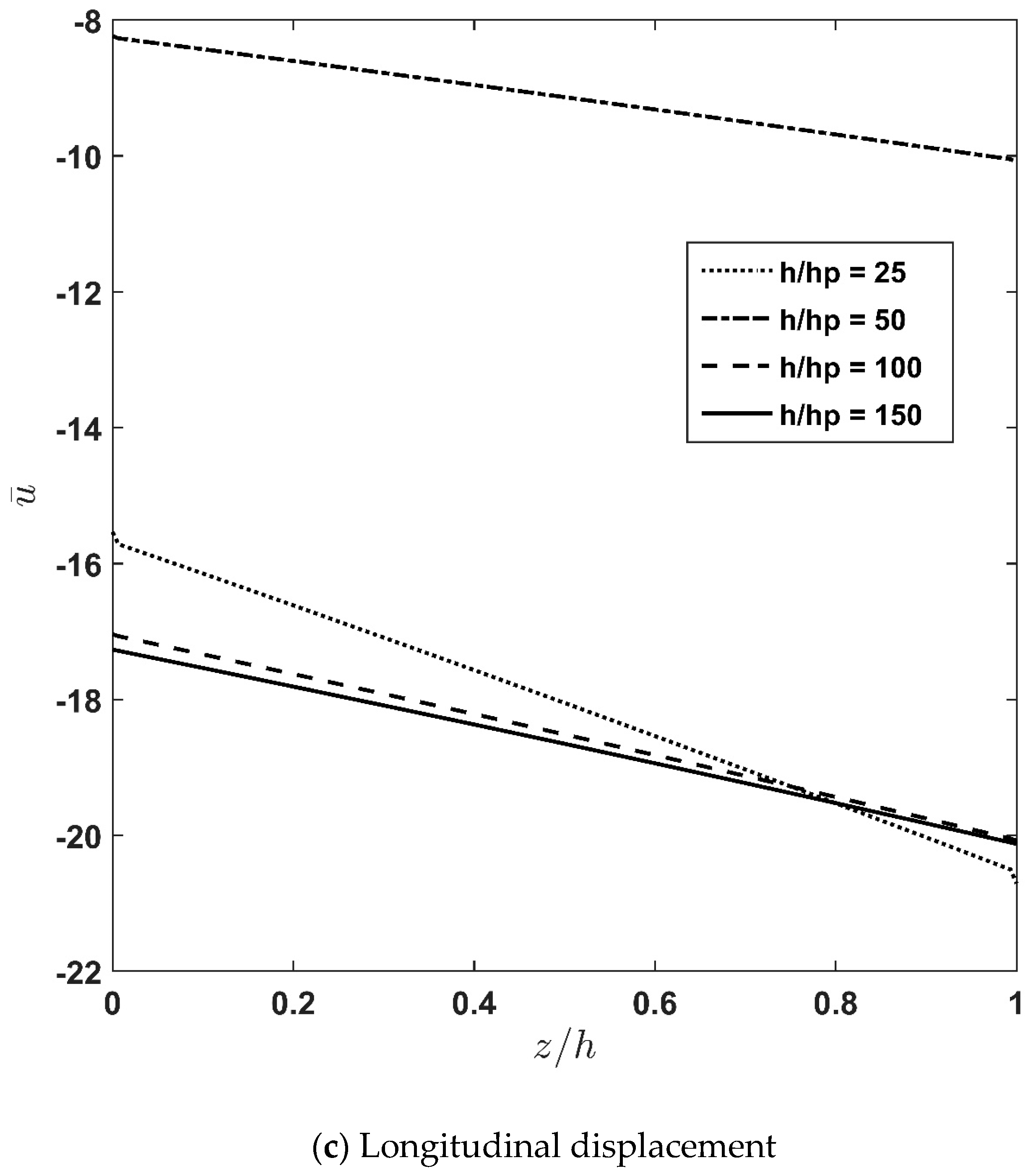
- Stiffness of the plate decreases by increasing and, accordingly, stresses and the deflection decrease.
- The effect of a temperature difference in the lower region is more significant than in the upper region due to the thermal barrier behavior of the FGM core at the upper surface.
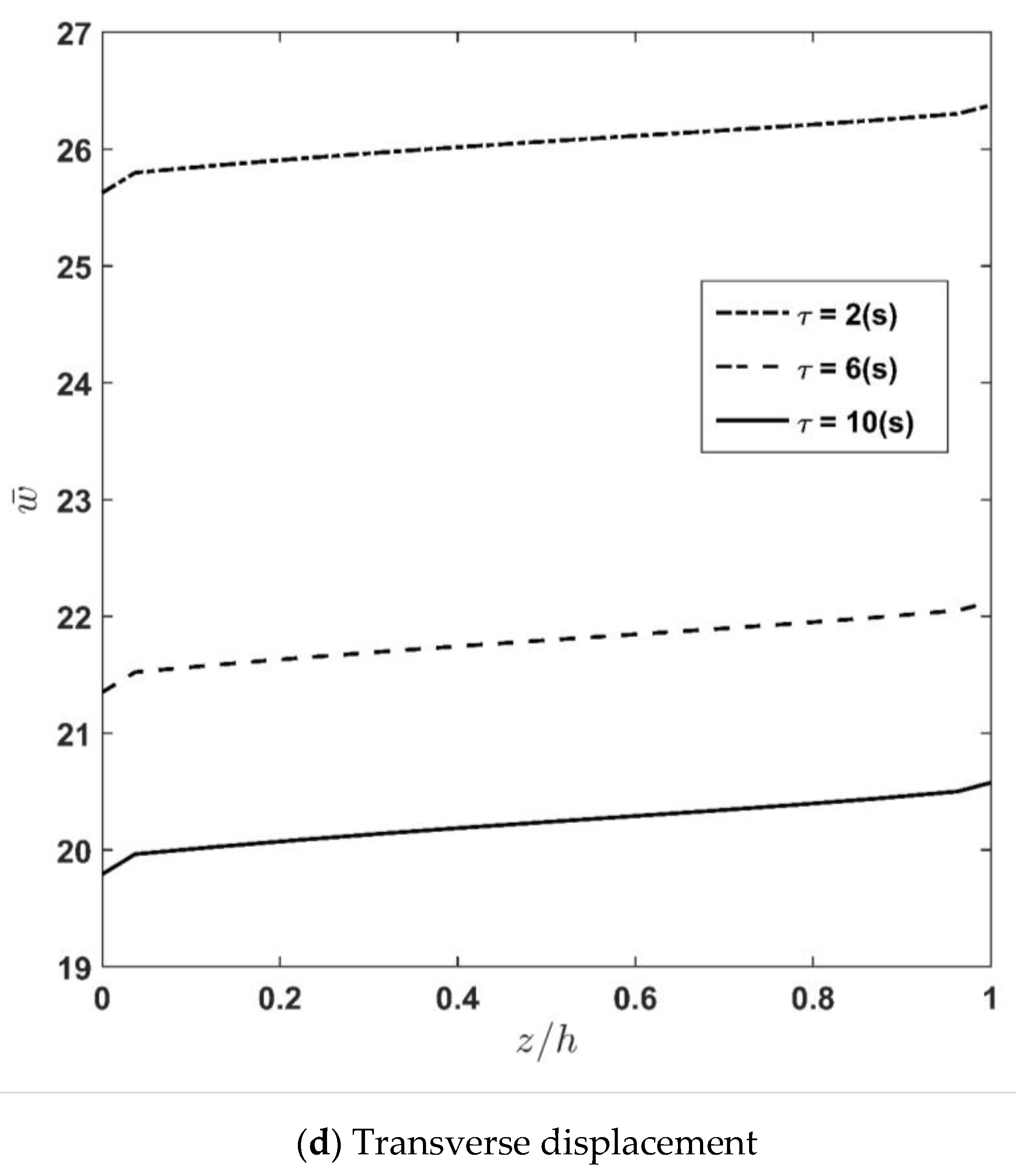
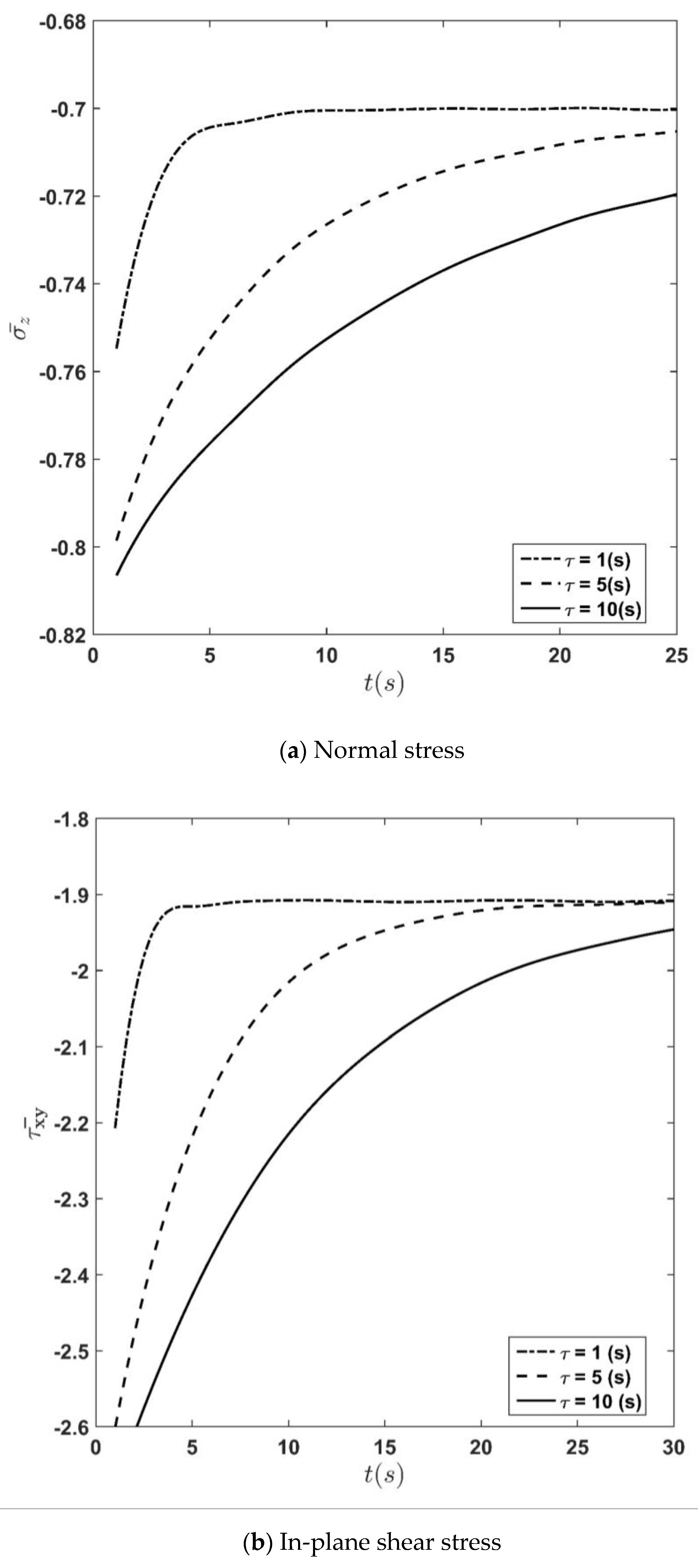
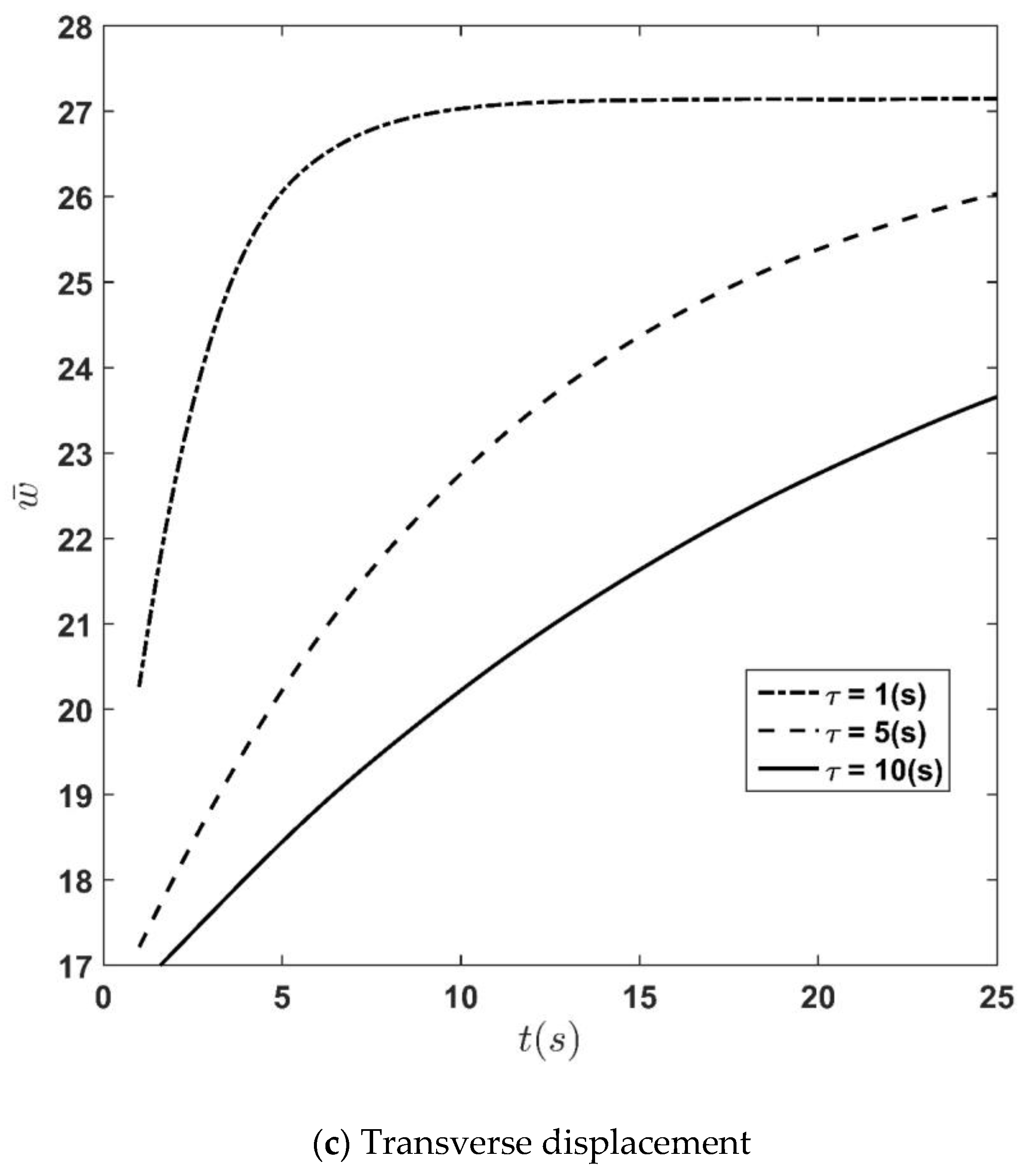
- Increasing the relaxation time constant causes the stiffness of the viscoelastic plate and, accordingly, stress components to increase and the displacement to decrease.
- Increasing the relaxation time constant causes the rate of convergence to the elastic behaviour to decrease.
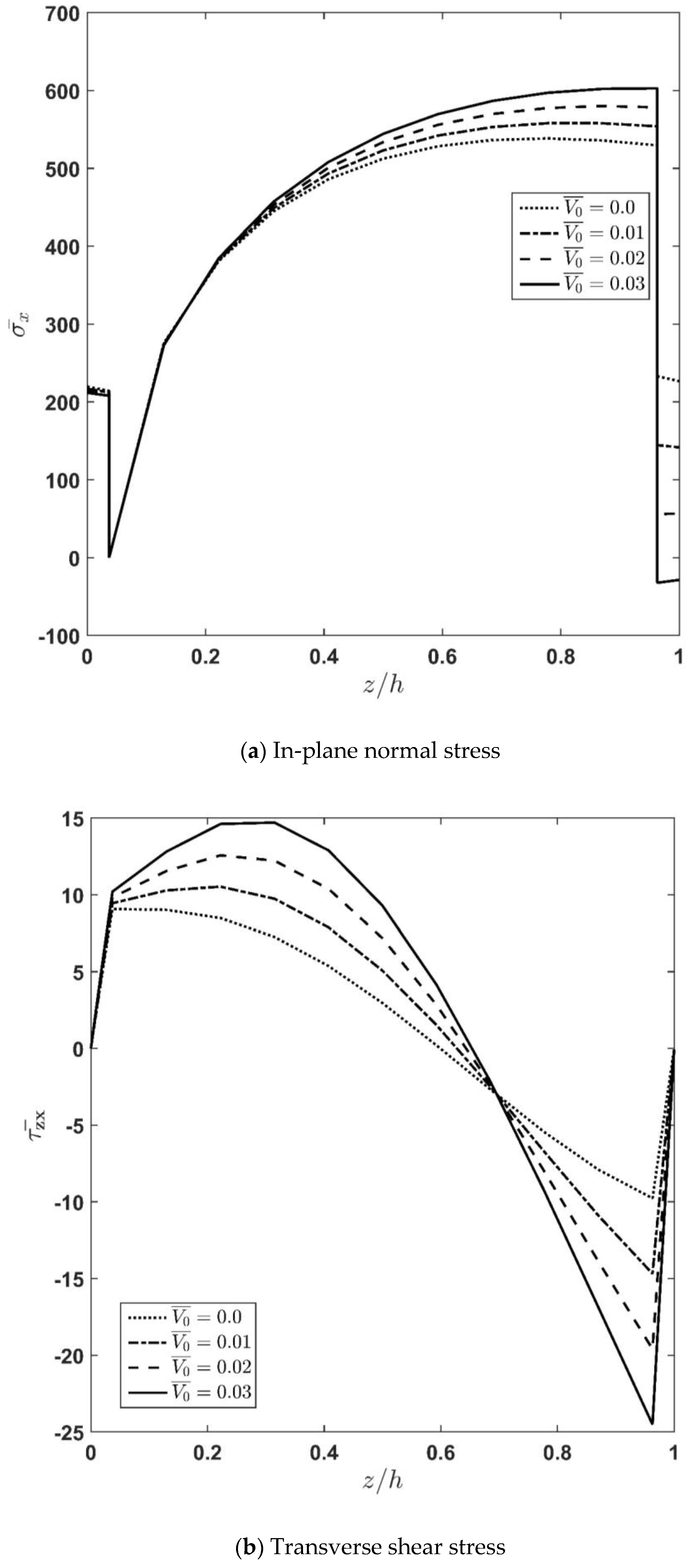
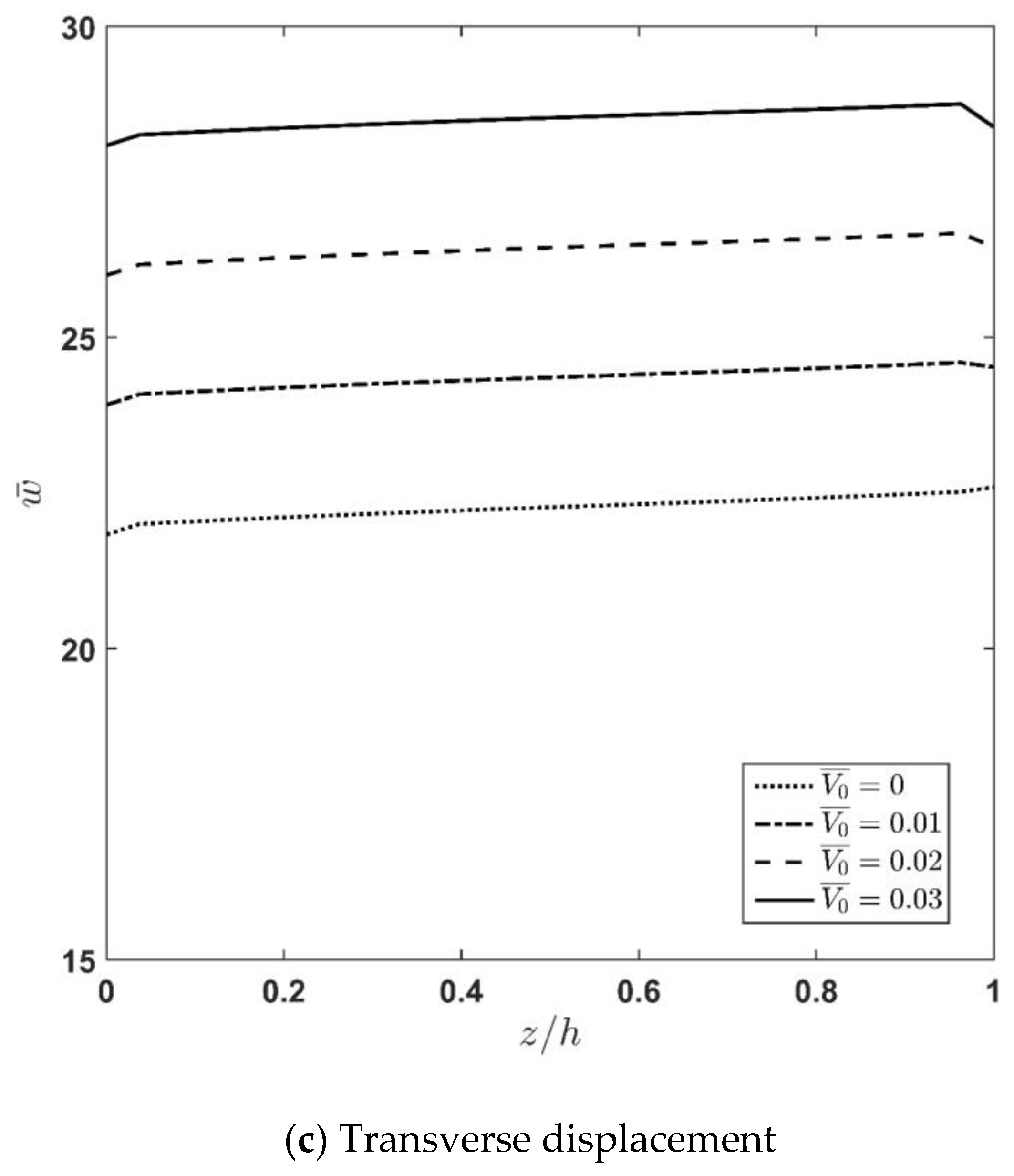
- The effect of the applied voltage near the outer region of the FGM layer is more significant due to the actuator layer’s effect.
- Deflection of the plate increases by increasing the applied voltage.
- Through-thickness distribution of deflection is linear in piezoelectric and FGM layers with different slope.
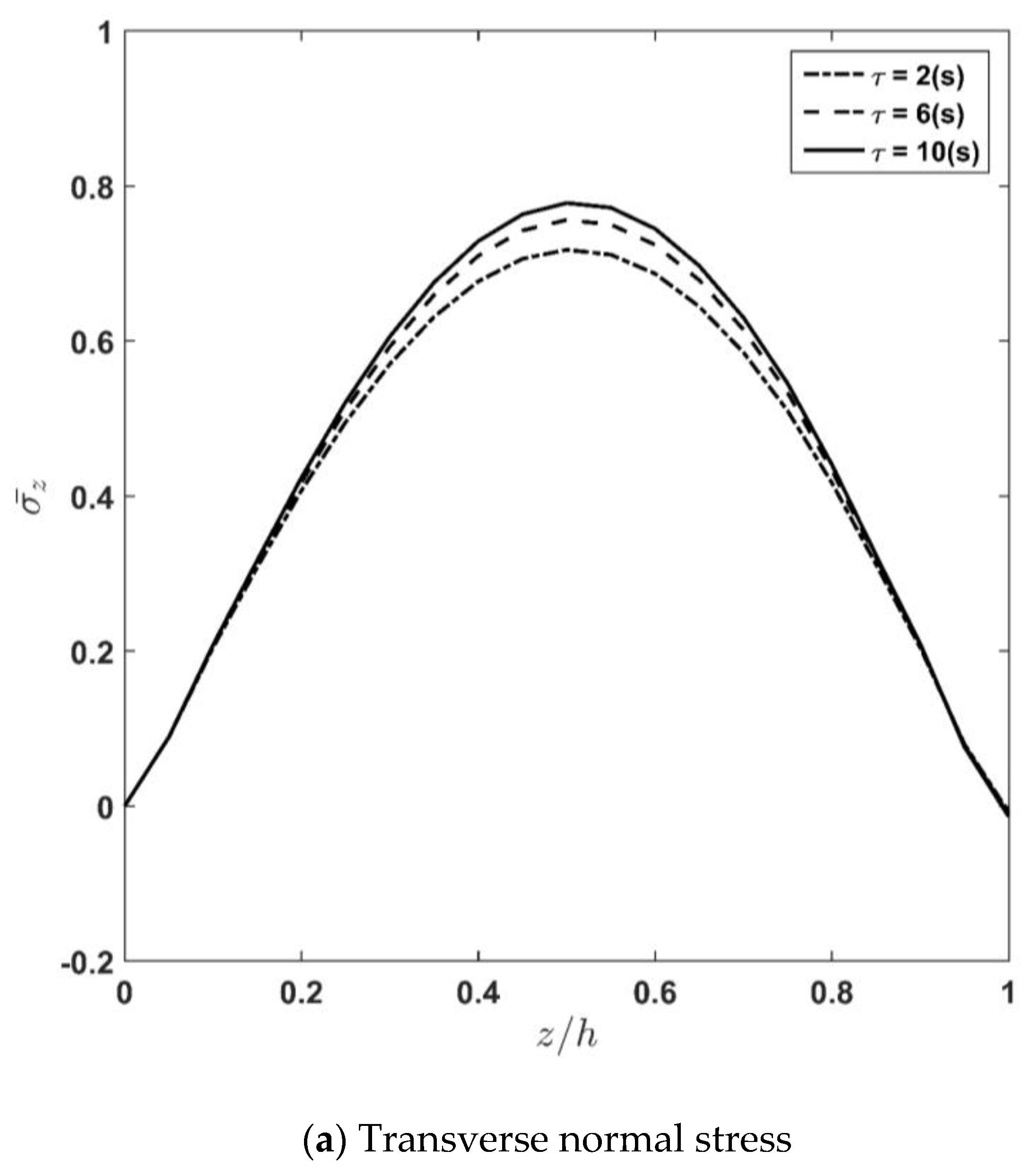
- Increasing the time constant causes delay in the steady state condition for stresses and displacement.
- Increasing the time constant causes a decrease in the transverse displacement .
- The maximum values of transverse normal stress are not at the mid-thickness of the plate, which is due to the FGM property.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| a, b, h | Plate dimensions in x-, y-, and z-directions |
| Subscripts designating FGM, actuator, and sensor layers, respectively | |
| Temperature distribution | |
| , | Temperature at the bottom and top surfaces, respectively |
| , | Temperature at the bottom and top surface of FGM layer, respectively |
| Thermal conductivity coefficient for FGM layer | |
| Thermal expansion coefficient | |
| Stress–temperature coefficients in x-, y-, and z-directions | |
| Pyroelectric constant | |
| Relaxation moduli coefficients | |
| Electric displacement | |
| Elasticity constant | |
| Electric field in x-, y-, and z-directions | |
| Young’s modulus | |
| Piezoelectric coefficient | |
| Dielectric constants | |
| d1 | Piezoelectric modulus |
| Thermal conductivity coefficient for piezoelectric layer in the x-, y-, and z-directions | |
| Thicknesses of the FGM and piezoelectric layers | |
| Half-wave numbers in the x- and y-directions | |
| Displacement components in the x-, y- and z-directions | |
| Normal stresses | |
| Shear stresses | |
| Normal strains | |
| Shear strains | |
| Relaxation time constant | |
| State vectors of the FGM and piezoelectric layers | |
| Electric voltage | |
| Poisson’s ratio |
Appendix A
References
- Jagtap, K.R.; Lal, A.; Singh, B.N. Stochastic nonlinear bending response of functionally graded material plate with random system properties in thermal environment. Int. J. Mech. Mater. Des. 2012, 8, 149–167. [Google Scholar] [CrossRef]
- Alibeigloo, A.; Emtehani, A. Static and free vibration analyses of carbon nanotube-reinforced composite plate using differential quadrature method. Meccanica 2015, 50, 61–76. [Google Scholar] [CrossRef]
- Alibeigloo, A. Three-dimensional thermoelasticity analysis of graphene platelets reinforced cylindrical panel. Eur. J. Mech. A/Solids 2020, 81, 103941. [Google Scholar] [CrossRef]
- Phung-Van, P.; Thai, C.H.; Abdel-Wahab, M.; Nguyen-Xuan, H. Optimal design of FG sandwich nanoplates using size-dependent isogeometric analysis. Mech. Mater. 2019, 142, 103277. [Google Scholar] [CrossRef]
- Beg, M.S.; Yasin, M.Y. Bending, free and forced vibration of functionally graded deep curved beams in thermal environment using an efficient layerwise theory. Mech. Mater. 2021, 159, 103919. [Google Scholar] [CrossRef]
- Wang, Y.; Feng, C.; Yang, J.; Zhou, D.; Liu, W. Static response of functionally graded graphene platelet–reinforced composite plate with dielectric property. J. Intell. Mater. Syst. Struct. 2020, 31, 2211–2228. [Google Scholar] [CrossRef]
- Brischetto, S.; Torre, R. 3D Stress Analysis of Multilayered Functionally Graded Plates and Shells under Moisture Conditions. Appl. Sci. 2022, 12, 512. [Google Scholar] [CrossRef]
- Amiri Delouei, A.; Emamian, A.; Karimnejad, S.; Sajjadi, H.; Jing, D. Two-dimensional analytical solution for temperature distribution in FG hollow spheres: General thermal boundary conditions. Int. Commun. Heat Mass Transf. 2020, 113, 104531. [Google Scholar] [CrossRef]
- Amiri Delouei, A.; Emamian, A.; Karimnejad, S.; Sajjadi, H. A closed-form solution for axisymmetric conduction in a finite functionally graded cylinder. Int. Commun. Heat Mass Transf. 2019, 108, 104280. [Google Scholar] [CrossRef]
- Khan, Y.; Akram, S.; Athar, M.; Saeed, K.; Muhammad, T.; Hussain, A.; Imran, M.; Alsulaimani, H.A. The Role of Double-Diffusion Convection and Induced Magnetic Field on Peristaltic Pumping of a Johnson–Segalman Nanofluid in a Non-Uniform Channel. Nanomaterials 2022, 12, 1051. [Google Scholar] [CrossRef]
- Saeed, K.; Akram, S.; Ahmad, A.; Athar, M.; Imran, M.; Muhammad, T. Impact of partial slip on double diffusion convection and inclined magnetic field on peristaltic wave of six-constant Jeffreys nanofluid along asymmetric channel. Eur. Phys. J. Plus 2022, 137, 364. [Google Scholar] [CrossRef]
- Akram, S.; Razia, A.; Umair, M.Y.; Abdulrazzaq, T.; Homod, R.Z. Double-diffusive convection on peristaltic flow of hyperbolic tangent nanofluid in non-uniform channel with induced magnetic field. Math. Methods Appl. Sci. 2022. [Google Scholar] [CrossRef]
- Akram, S.; Athar, M.; Saeed, K.; Imran, M.; Muhammad, T. Slip impact on double-diffusion convection of magneto-fourth-grade nanofluids with peristaltic propulsion through inclined asymmetric channel. J. Therm. Anal. Calorim. 2022, 147, 8933–8946. [Google Scholar] [CrossRef]
- Akram, S.; Athar, M.; Saeed, K.; Umair, M.Y. Nanomaterials effects on induced magnetic field and double-diffusivity convection on peristaltic transport of Prandtl nanofluids in inclined asymmetric channel. Nanomater. Nanotechnol. 2022, 12, 18479804211048630. [Google Scholar] [CrossRef]
- Akram, S.; Athar, M.; Saeed, K.; Razia, A. Impact of slip on nanomaterial peristaltic pumping of magneto-Williamson nanofluid in an asymmetric channel under double-diffusivity convection. Pramana 2022, 96, 1–13. [Google Scholar] [CrossRef]
- Alibeigloo, A. Thermoelasticity analysis of functionally graded beam with integrated surface piezoelectric layers. Compos. Struct. 2010, 92, 1535–1543. [Google Scholar] [CrossRef]
- Alibeigloo, A.; Chen, W.Q. Elasticity solution for an FGM cylindrical panel integrated with piezoelectric layers. Eur. J. Mech. A/Solids 2010, 29, 714–723. [Google Scholar] [CrossRef]
- Kiani, Y.; Rezaei, M.; Taheri, S.; Eslami, M.R. Thermo-electrical buckling of piezoelectric functionally graded material Timoshenko beams. Int. J. Mech. Mater. Des. 2011, 7, 185–197. [Google Scholar] [CrossRef]
- Brischetto, S.; Carrera, E. Static analysis of multilayered smart shells subjected to mechanical, thermal and electrical loads. Meccanica 2013, 48, 1263–1287. [Google Scholar] [CrossRef]
- Alibeigloo, A. Three-dimensional thermoelasticity solution of functionally graded carbon nanotube reinforced composite plate embedded in piezoelectric sensor and actuator layers. Compos. Struct. 2014, 118, 482–495. [Google Scholar] [CrossRef]
- Alibeigloo, A. Thermoelastic solution for static deformations of functionally graded cylindrical shell bonded to thin piezoelectric layers. Compos. Struct. 2011, 93, 961–972. [Google Scholar] [CrossRef]
- Feri, M.; Alibeigloo, A.; Zanoosi, A.A.P. Three dimensional static and free vibration analysis of cross-ply laminated plate bonded with piezoelectric layers using differential quadrature method. Meccanica 2016, 51, 921–937. [Google Scholar] [CrossRef]
- Kulikov, G.M.; Plotnikova, S.V. An analytical approach to three-dimensional coupled thermoelectroelastic analysis of functionally graded piezoelectric plates. J. Intell. Mater. Syst. Struct. 2017, 28, 435–450. [Google Scholar] [CrossRef]
- Heydarpour, Y.; Malekzadeh, P.; Dimitri, R.; Tornabene, F. Thermoelastic analysis of functionally graded cylindrical panels with piezoelectric layers. Appl. Sci. 2020, 10, 1397. [Google Scholar] [CrossRef]
- Moradi-Dastjerdi, R.; Behdinan, K. Thermo-electro-mechanical behavior of an advanced smart lightweight sandwich plate. Aerosp. Sci. Technol. 2020, 106, 106142. [Google Scholar] [CrossRef]
- Zeng, S.; Peng, Z.; Wang, K.; Wang, B.; Wu, J.; Luo, T. Nonlinear Analyses of Porous Functionally Graded Sandwich Piezoelectric Nano-Energy Harvesters under Compressive Axial Loading. Appl. Sci. 2021, 11, 11787. [Google Scholar] [CrossRef]
- Xiang, H.J.; Shi, Z.F. Static analysis for functionally graded piezoelectric actuators or sensors under a combined electro-thermal load. Eur. J. Mech. -A/Solids 2009, 28, 338–346. [Google Scholar] [CrossRef]
- Koutsawa, Y.; Haberman, M.R.; Daya, E.M.; Cherkaoui, M. Multiscale design of a rectangular sandwich plate with viscoelastic core and supported at extents by viscoelastic materials. Int. J. Mech. Mater. Des. 2009, 5, 29–44. [Google Scholar] [CrossRef]
- Cai, Y.; Sun, H. Thermo-viscoelastic analysis of three-dimensionally braided composites. Compos. Struct. 2013, 98, 47–52. [Google Scholar] [CrossRef]
- Norouzi, H.; Alibeigloo, A. Three dimensional static analysis of viscoelastic FGM cylindrical panel using state space differential quadrature method. Eur. J. Mech.-A/Solids 2017, 61, 254–266. [Google Scholar] [CrossRef]
- Malikan, M.; Dimitri, R.; Tornabene, F. Effect of sinusoidal corrugated geometries on the vibrational response of viscoelastic nanoplates. Appl. Sci. 2018, 8, 1432. [Google Scholar] [CrossRef]
- Yang, Z.; Wu, P.; Liu, W.; Fang, H. Analytical Solutions for Functionally Graded Sandwich Plates Bonded by Viscoelastic Interlayer Based on Kirchhoff Plate Theory. Int. J. Appl. Mech. 2020, 12, 2050062. [Google Scholar] [CrossRef]
- Liu, C.; Shi, Y. A thermo-viscoelastic analytical model for residual stresses and spring-in angles of multilayered thin-walled curved composite parts. Thin-Walled Struct. 2020, 152, 106758. [Google Scholar] [CrossRef]
- Chen, J.; Han, R.; Liu, D.; Zhang, W. Active Flutter Suppression and Aeroelastic Response of Functionally Graded Multilayer Graphene Nanoplatelet Reinforced Plates with Piezoelectric Patch. Appl. Sci. 2022, 12, 1244. [Google Scholar] [CrossRef]
- Hetnarski, R.B.; Eslami, M.R.; Gladwell, G. Thermal Stresses: Advanced Theory and Applications; Springer: Berlin/Heidelberg, Germany, 2009; Volume 41. [Google Scholar]
- Sadd, M.H. Elasticity: Theory, Applications, and Numerics; Academic Press: Cambridge, MA, USA, 2009; ISBN 0-08-092241-4. [Google Scholar]
- Li, C.; Guo, H.; Tian, X.; He, T. Generalized thermoviscoelastic analysis with fractional order strain in a thick viscoelastic plate of infinite extent. J. Therm. Stress. 2019, 42, 1051–1070. [Google Scholar] [CrossRef]
- Alibeigloo, A. Thermo-elasticity solution of functionally graded plates integrated with piezoelectric sensor and actuator layers. J. Therm. Stress. 2010, 33, 754–774. [Google Scholar] [CrossRef]
- Abate, J.; Whitt, W. Numerical inversion of Laplace transforms of probability distributions. ORSA J. Comput. 1995, 7, 36–43. [Google Scholar] [CrossRef]
- Norouzi, H.; Alibeigloo, A. Three-dimensional thermoviscoelastic analysis of a FGM cylindrical panel using state space differential quadrature method. J. Therm. Stress. 2018, 41, 383–398. [Google Scholar] [CrossRef]
- Brischetto, S.; Leetsch, R.; Carrera, E.; Wallmersperger, T.; Kröplin, B. Thermo-mechanical bending of functionally graded plates. J. Therm. Stress. 2008, 31, 286–308. [Google Scholar] [CrossRef]
- Reddy, J.; Cheng, Z.-Q. Three-dimensional thermomechanical deformations of functionally graded rectangular plates. Eur. J. Mech. -A/Solids 2001, 20, 841–855. [Google Scholar] [CrossRef]


| Elasticity Constant [109 Nm−2] | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Sensor (PZT-4) | 139 | 78 | 74 | 139 | 74 | 115 | 25.6 | 25.6 | 30.5 |
| Actuator (Ba2 NaNb5 O15) | 239 | 104 | 50 | 274 | 52 | 135 | 65 | 66 | 76 |
| Piezoelectric coefficients [coul·m−2] | |||||||||
| Sensor | −5.2 | −5.2 | 15.1 | 12.7 | 12.7 | ||||
| Actuator | −0.4 | −0.3 | 4.3 | 3.4 | 2.8 | ||||
| Dielectric constants [10−9 farads·m−1] | η1 | η2 | η3 | ||||||
| Sensor | 6.5 | 6.5 | 5.6 | ||||||
| Actuator | 1.96 | 2.01 | 0.28 | ||||||
| Thermal conductivity | |||||||||
| Sensor | 2.1 | 3.15 | |||||||
| Actuator | 8.6 | 12.9 | |||||||
| Thermal expansion [] | |||||||||
| Sensor | 1.97 | 2.62 | |||||||
| Actuator | 4.39 | 2.45 | |||||||
| Piezoelectric modulus pyroelectric constant: | |||||||||
| Sensor | −3.92 | 5.4 | |||||||
| Actuator | −3.92 | 5.4 | |||||||
| [41] | [42] | [38] | Present | [41] | [42] | [38] | Present | |
|---|---|---|---|---|---|---|---|---|
| 3.043 | 3.043 | 3.0431 | 3.043 | 28.54 | 28.53 | 28.53 | 28.53 | |
| 2.144 | 2.143 | 2.1443 | 2.143 | 28.46 | 28.45 | 28.448 | 28.45 | |
| 1.901 | 1.901 | 1.9012 | 1.900 | 28.44 | 28.43 | 28.432 | 28.43 | |
| −1.681 | −1.681 | −1.681 | −1.681 | −1.703 | −1.703 | −1.7027 | −1.703 | |
| −0.6822 | −0.6822 | −0.6823 | −0.6860 | −0.8080 | −0.8081 | −0.8081 | −0.808 | |
| 0.08266 | 0.08240 | 0.08242 | 0.08241 | 0.08553 | 0.08528 | 0.08527 | −0.08552 | |
| −1018 | −1018 | −1018 | −1018 | −1003 | −1003 | −1003 | −1003 | |
| −204.7 | −204.8 | −204.82 | −204.821 | −251.2 | −251.2 | −251.208 | −251.2084 | |
| −74.03 | −73.53 | −73.525 | −73.525 | −76.59 | −76.10 | −76.12 | −76.1239 | |
| 4.203 | 4.186 | 4.1875 | 4.1875 | 0.3135 | 0.3122 | 0.3123 | 0.3123 | |
| 6.300 | 6.217 | 6.23 | 6.2342 | 0.1178 | 0.04067 | 0.04051 | 0.4051 | |
| 0 | 0.2 | 0.4 | 0.6 | 0.8 | 1 | ||
|---|---|---|---|---|---|---|---|
| 600 | −20.620 | −20.850 | −21.100 | −21.392 | −21.706 | −22.040 | |
| 800 | −15.547 | −16.507 | −17.509 | −18.574 | −19.576 | −20.704 | |
| 900 | −13.856 | −15.088 | −16.403 | −17.635 | −18.929 | −20.265 | |
| 600 | 5.6785 | 6.0438 | 6.1482 | 6.3048 | 6.4092 | 6.5658 | |
| 800 | 22.015 | 22.276 | 22.380 | 22.484 | 22.589 | 22.797 | |
| 900 | 27.443 | 27.704 | 27.756 | 27.860 | 28.017 | 28.173 | |
| 600 | 0 | 0.4945 | 0.7732 | 0.7438 | 0.4281 | 0 | |
| 800 | 0 | 0.4155 | 0.7031 | 0.7167 | 0.4301 | 0 | |
| 900 | 0 | 0.3892 | 0.6798 | 0.7076 | 0.4307 | 0 | |
| 600 | −199.79 | −181.63 | −239.87 | −257.41 | −258.66 | −202.92 | |
| 800 | −137.79 | −124.63 | −187.27 | −225.47 | −248.64 | −199.16 | |
| 900 | −117.12 | −105.22 | −169.73 | −214.82 | −246.14 | −197.91 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feri, M.; Krommer, M.; Alibeigloo, A. Three-Dimensional Thermoelasticity Analysis of Viscoelastic FGM Plate Embedded in Piezoelectric Layers under Thermal Load. Appl. Sci. 2023, 13, 353. https://doi.org/10.3390/app13010353
Feri M, Krommer M, Alibeigloo A. Three-Dimensional Thermoelasticity Analysis of Viscoelastic FGM Plate Embedded in Piezoelectric Layers under Thermal Load. Applied Sciences. 2023; 13(1):353. https://doi.org/10.3390/app13010353
Chicago/Turabian StyleFeri, Maziyar, Michael Krommer, and Akbar Alibeigloo. 2023. "Three-Dimensional Thermoelasticity Analysis of Viscoelastic FGM Plate Embedded in Piezoelectric Layers under Thermal Load" Applied Sciences 13, no. 1: 353. https://doi.org/10.3390/app13010353
APA StyleFeri, M., Krommer, M., & Alibeigloo, A. (2023). Three-Dimensional Thermoelasticity Analysis of Viscoelastic FGM Plate Embedded in Piezoelectric Layers under Thermal Load. Applied Sciences, 13(1), 353. https://doi.org/10.3390/app13010353









