On the Effects of Disc Deformation on the Tilting-Induced Vibration of a Spline-Guided Spinning Disc with an Axial-Fixed Boundary
Abstract
1. Introduction
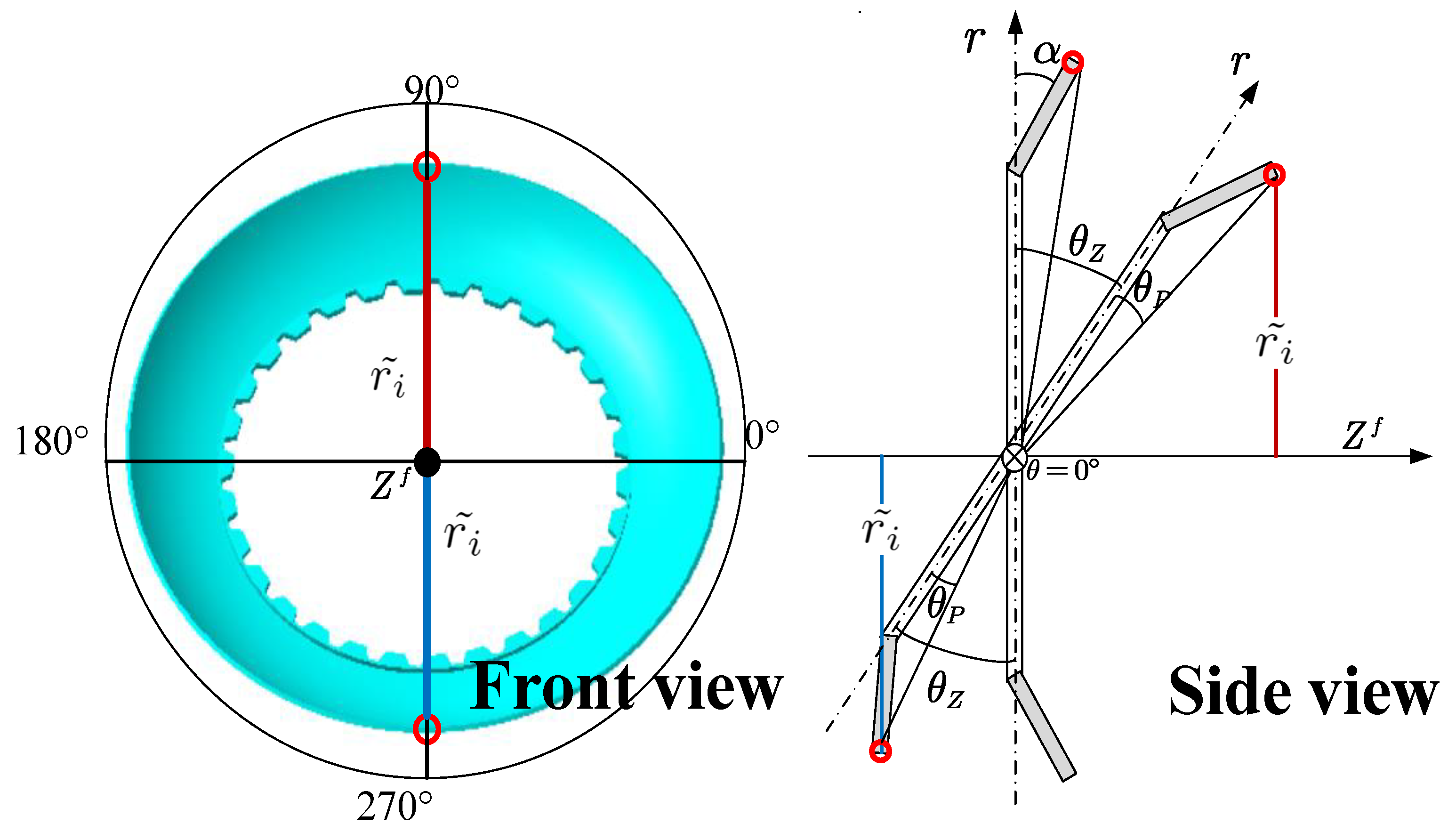
2. Model and Formulation
2.1. Flat Spinning Disc
2.1.1. Rotation-Induced Alignment Moment
2.1.2. Moment of Inertia
2.2. Formulation of the Deformed Plate
2.2.1. Centrifugal Moment of the Deformed Disc
2.2.2. Moment of Inertia of the Deformed Disc
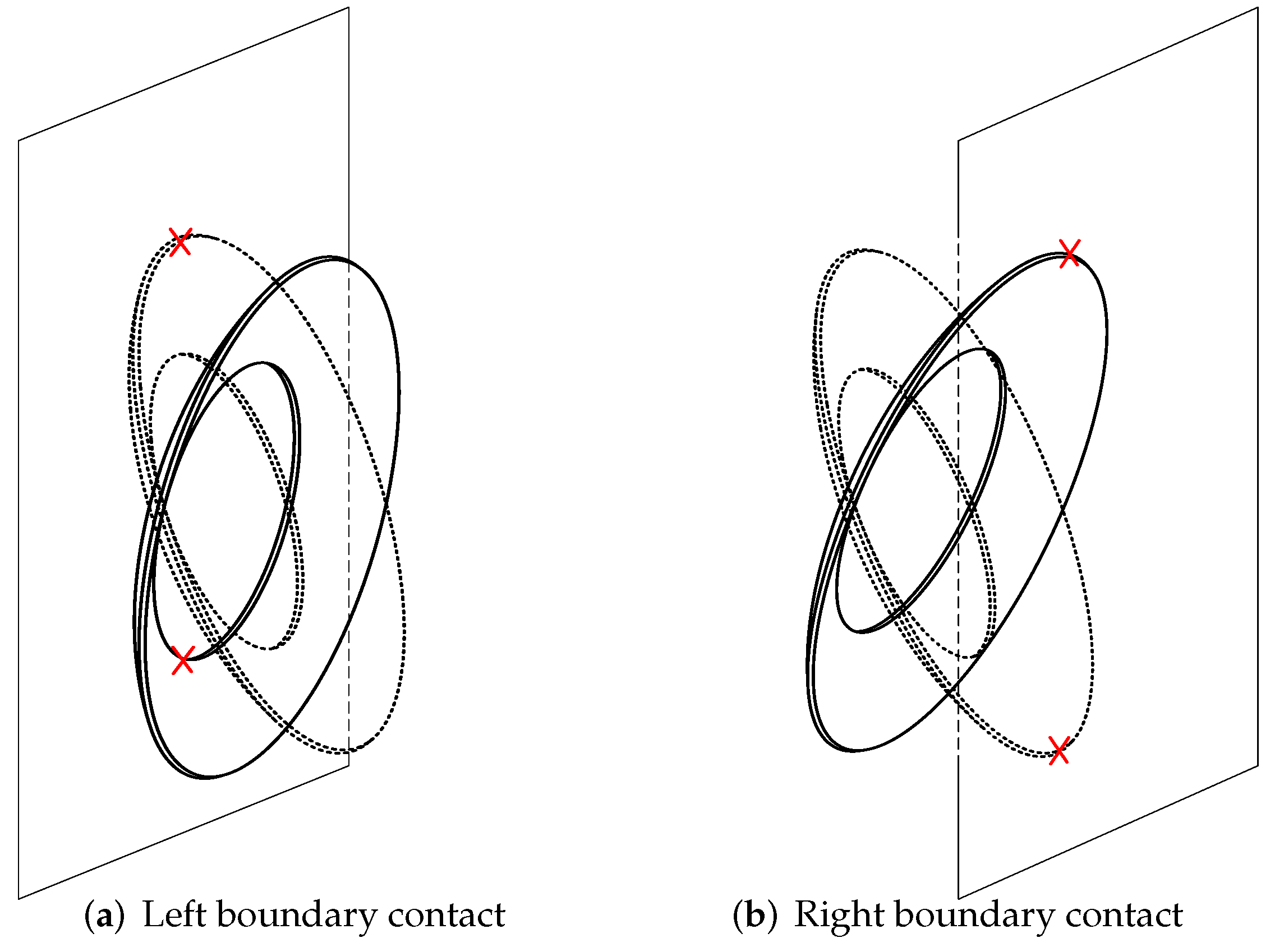
2.3. Boundary Impact Model of the Flat Disc
2.4. Spline Force Characteristics
3. Numerical Analysis
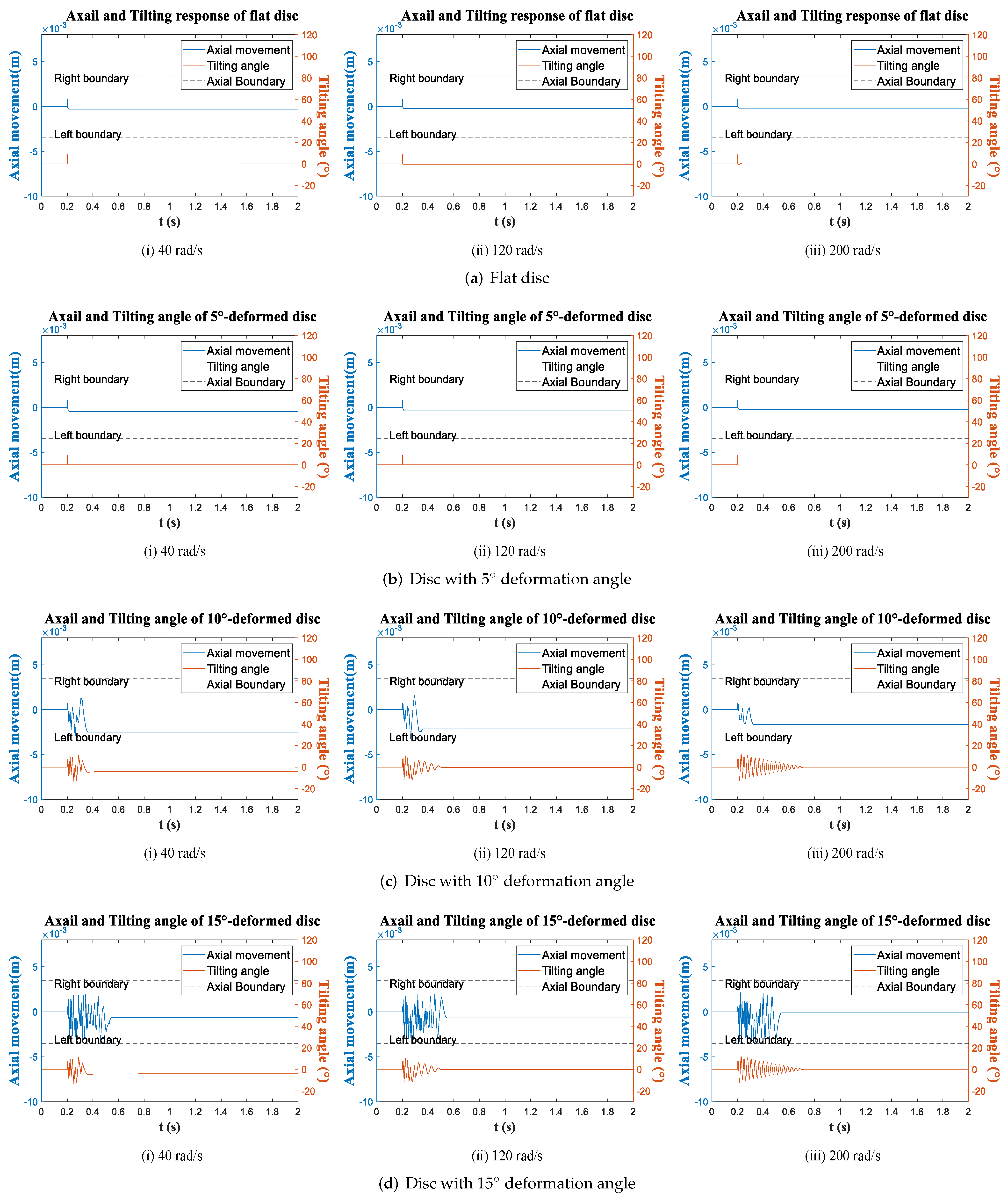
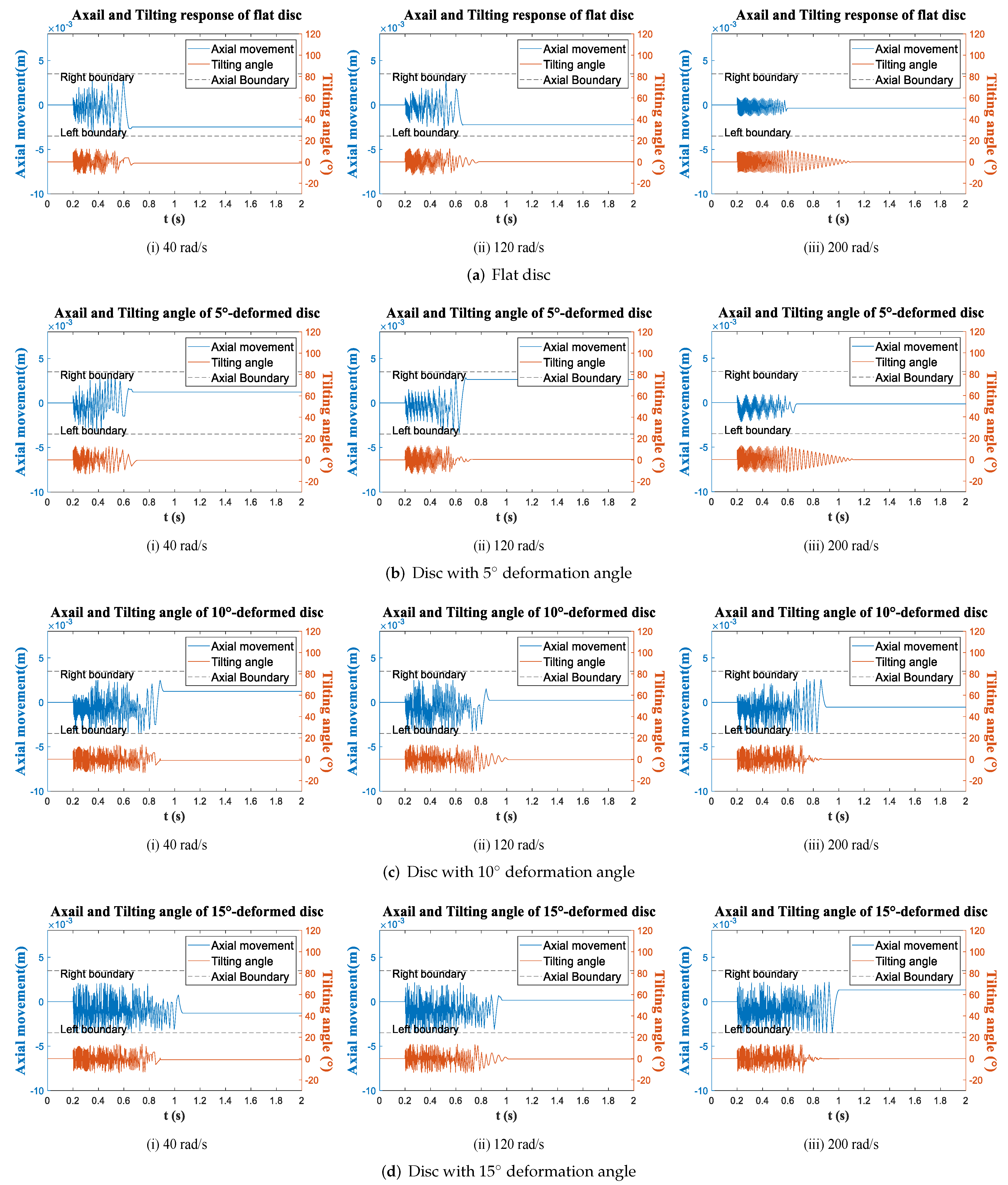
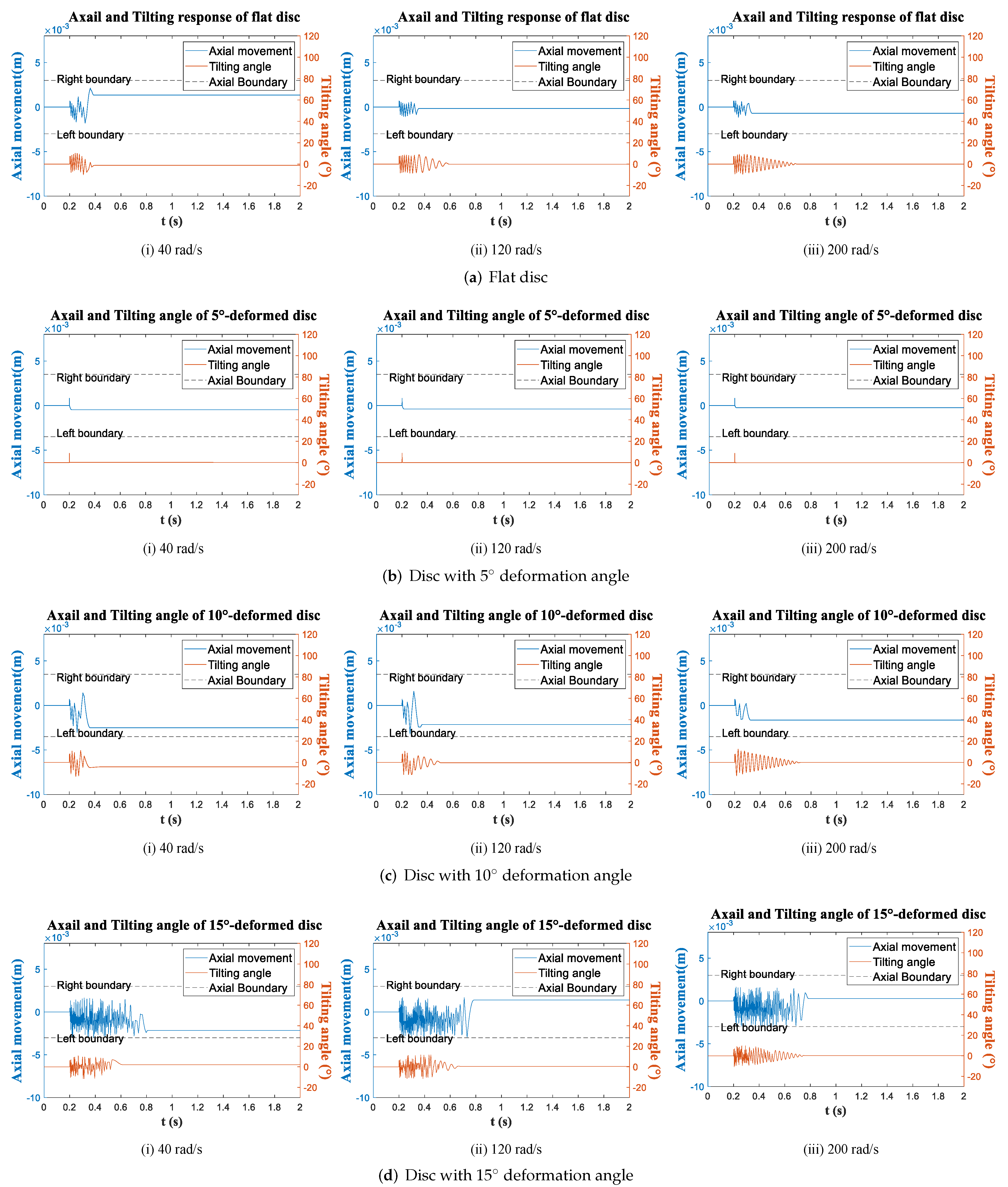
3.1. Time Response Analysis
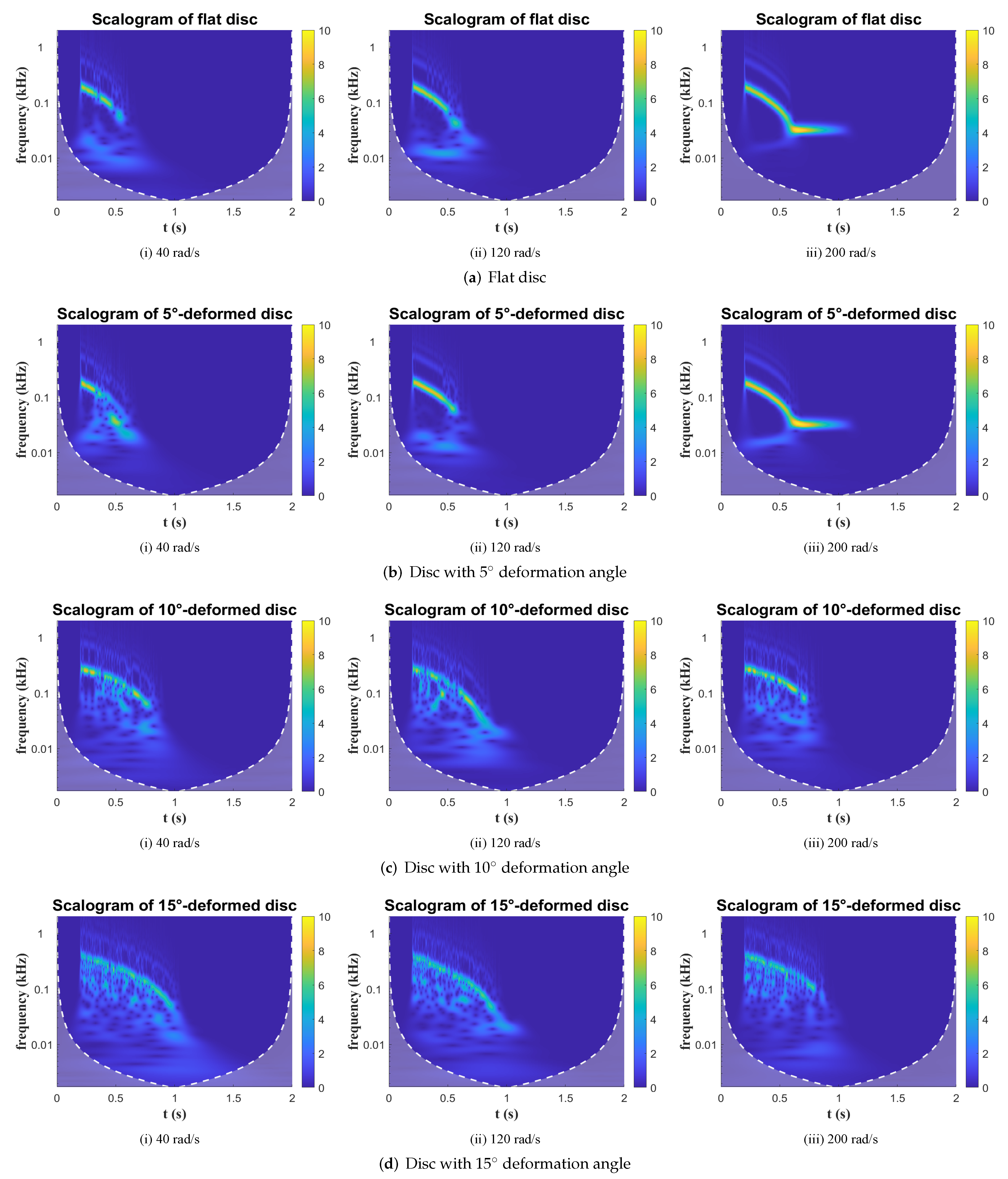
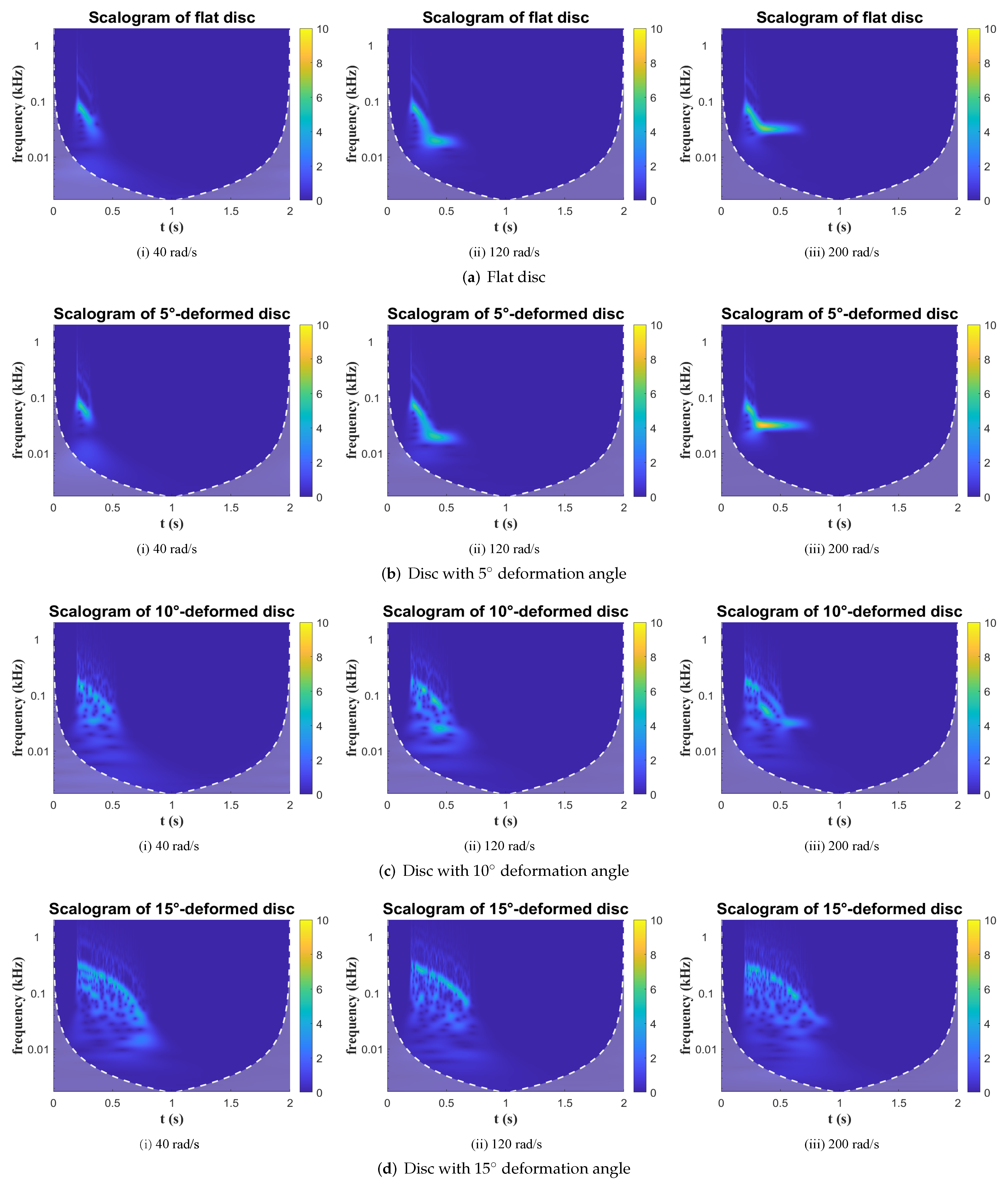
3.2. Frequency Analysis of Tilting Response
4. Conclusions
- Regardless of the deformation, the disc tilting and axial motion are highly correlated. Both motions either show strong nonlinearity or stop immediately after the impulse, and when one motion stops the other stops soon. Also the final position of axial displacement is hard to predict, it varies as long as the condition changes.
- The deformation of the disc increases the complexity of the tilting and axial motion of spinning discs. The dynamic responses of discs with larger deformation angles are more dramatic and longer than those of less-deformed discs, because the deformation of the disc increases the possibility of impact between the disc and boundary.
- The effect of increasing the impulse force is similar to reducing the boundary distance; they both increase the intensity of the motions. The difference is that, narrowing the boundary distance could restrict the maximum tilting angle, and larger impulsion increase the tilting frequency. In addition, the rotation speed can also extend the time of tilting.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khorasany, R.M.H.; Hutton, S.G. Large displacement analysis of elastically constrained rotating disks with rigid body degrees of freedom. Int. J. Mech. Sci. 2012, 54, 1–11. [Google Scholar] [CrossRef]
- Yu, L.; Ma, B.; Chen, M.; Li, H.; Zhang, H.; Liu, J. Thermodynamic Differences of Different Friction Pairs in a Multidisc Clutch Caused by Spline Friction: Numerical Simulation and Experimental Verification. Tribol. Trans. 2019, 62, 724–736. [Google Scholar] [CrossRef]
- Zhao, E.H.; Ma, B.; Li, H.Y. The Tribological Characteristics of Cu-Based Friction Pairs in a Wet Multidisk Clutch under Nonuniform Contact. J. Tribol. 2018, 140, 011401. [Google Scholar] [CrossRef]
- Cui, J.; Hou, P.; Zhang, B.; Zhao, X. Investigation of flow between deformed disks in hydro-viscous drive. Tribol. Int. 2018, 121, 287–301. [Google Scholar] [CrossRef]
- Hou, S.; Hu, J.; Peng, Z. Experimental investigation on unstable vibration characteristics of plates and drag torque in open multiplate wet clutch at high circumferential speed. J. Fluids Eng. Trans. ASME 2017, 139, 111103. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, M.; Xue, J.; Ma, B.; Li, H. The influence of friction component buckling on the vibration characteristic of the wet clutch. J. Phys. Conf. Ser. 2020, 1449, 012112. [Google Scholar] [CrossRef]
- Xue, J.; Ma, B.; Chen, M.; Zhang, Q.; Zheng, L. Experimental Investigation and Fault Diagnosis for Buckled Wet Clutch Based on Multi-Speed Hilbert Spectrum Entropy. Entropy 2021, 23, 1704. [Google Scholar] [CrossRef] [PubMed]
- Jeong, T.G.; Bogy, D.B. Measurements of Slider-Disk Contacts During Dynamic Load-Unload. IEEE Trans. Magn. 1991, 27, 5073–5075. [Google Scholar] [CrossRef]
- Chen, J.S.; Bogy, D.B. Effects of Load Parameters on the Natural Frequencies and Stability of a Flexible Spinning Disk with a Stationary Load System. J. Appl. Mech. 1992, 59, S230–S235. [Google Scholar] [CrossRef]
- Chen, J.S.; Bogy, D.B. Natural Frequencies and Stability of a Flexible Spinning Disk-Stationary Load System with Rigid-Body Tilting. J. Appl. Mech. 1993, 60, 470. [Google Scholar] [CrossRef]
- Pei, Y.C.; Tan, Q.C.; Zheng, F.S.; Zhang, Y.Q. Dynamic stability of rotating flexible disk perturbed by the reciprocating angular movement of suspensionslider system. J. Sound Vib. 2010, 329, 5520–5531. [Google Scholar] [CrossRef]
- Pei, Y.C.; Tan, Q.C.; Yang, X.; Chatwin, C. Vibrational response of a moving suspension-slider loading system exciting a rotating flexible disk. J. Sound Vib. 2012, 331, 3762–3773. [Google Scholar] [CrossRef]
- Li, C.; Wang, C.; Xia, W.; Zhang, C. Vortex-induced vibrations of an elastically mounted disk: The characteristics of wake and trajectory. Eur. J. Mech. B/Fluids 2021, 86, 113–122. [Google Scholar] [CrossRef]
- Fidlin, A.; Drozdetskaya, O.; Waltersberger, B. On the minimal model for the low frequency wobbling instability of friction discs. Eur. J. Mech. A Solids 2011, 30, 665–672. [Google Scholar] [CrossRef][Green Version]
- Yang, L.; Mao, Z.; Chen, X.; Yan, R.; Xie, J.; Hu, H. Dynamic coupling vibration of rotating shaft–disc–blade system—Modeling, mechanism analysis and numerical study. Mech. Mach. Theory 2022, 167, 104542. [Google Scholar] [CrossRef]
- Miyasato, H.H.; Segala Simionatto, V.G.; Junior, M.D. On the interaction between rigid discs and rotating damped contact elements. Mech. Res. Commun. 2021, 111, 103644. [Google Scholar] [CrossRef]
- Cheng, L.; Li, Y.Y.; Yam, L.H. Vibration analysis of annular-like plates. J. Sound Vib. 2003, 262, 1153–1170. [Google Scholar] [CrossRef]
- Maretic, R. Transverse vibration and stability of an eccentric rotating circular plate. J. Sound Vib. 2005, 280, 467–478. [Google Scholar] [CrossRef]
- Carpino, M. The effect of initial curvature in a flexible disk rotating near a flat plate. J. Tribol. 1991, 113, 355–360. [Google Scholar] [CrossRef]
- Khorasany, R.M.; Hutton, S.G. The effect of axisymmetric nonflatness on the oscillation frequencies of a rotating disk. J. Vib. Acoust. Trans. ASME 2010, 132, 051012. [Google Scholar] [CrossRef]
- Khorasany, R.M.; Hutton, S.G. On the effects of a general form of the initial runout on the oscillation frequencies and critical speeds of a spinning disk. J. Sound Vib. 2011, 330, 6435–6455. [Google Scholar] [CrossRef]
- Olver, A.V. Regimes of Contact in Spline Couplings. J. Tribol. 2002, 124, 351–357. [Google Scholar] [CrossRef]
- Mohammadpanah, A.; Hutton, S.G. Theoretical and Experimental Verification of Dynamic Behaviour of a Guided Spline Arbor Circular Saw. Shock Vib. 2017, 2017, 6213791. [Google Scholar] [CrossRef]
- Mohammadpanah, A.; Hutton, S.G. Dynamics Behavior of a Guided Spline Spinning Disk, Subjected to Conservative In-Plane Edge Loads, Analytical and Experimental Investigation. J. Vib. Acoust. 2017, 138, 041005. [Google Scholar] [CrossRef]
- Zhang, L.; Wei, C.; Hu, J.; Hu, Q. Influences of lubrication flow rates on critical speed of rub-impact at high circumferential velocities in No-Load multi-plate wet clutch. Tribol. Int. 2019, 140, 105847. [Google Scholar] [CrossRef]
- Safaeifar, H.; Farshidianfar, A. A new model of the contact force for the collision between two solid bodies. Multibody Syst. Dyn. 2020, 50, 233–257. [Google Scholar] [CrossRef]
- Zhang, J.; Li, W.; Zhao, L.; He, G. A continuous contact force model for impact analysis in multibody dynamics. Mech. Mach. Theory 2020, 153, 103946. [Google Scholar] [CrossRef]
- Hu, J.; Hou, S.; Wei, C. Drag torque modeling at high circumferential speed in open wet clutches considering plate wobble and mechanical contact. Tribol. Int. 2018, 124, 102–116. [Google Scholar] [CrossRef]
- Yu, L.; Ma, B.; Chen, M.; Li, H.; Liu, J.; Li, M. Investigation on the failure mechanism and safety mechanical- thermal boundary of a multi-disc clutch. Eng. Fail. Anal. 2019, 103, 319–334. [Google Scholar] [CrossRef]
- Zheng, L.; Ma, B.; Chen, M.; Yu, L.; Wang, Q. Influence of the lubrication oil temperature on the disengaging dynamic characteristics of a cu-based wet multi-disc clutch. Appl. Sci. 2021, 11, 1299. [Google Scholar] [CrossRef]
- Cura, F.; Mura, A. Theoretical and numerical evaluation of tilting moment in crowned teeth splined couplings. Meccanica 2018, 53, 413–424. [Google Scholar] [CrossRef]
- Narin, A. Detection of Focal and Non-focal Epileptic Seizure Using Continuous Wavelet Transform-Based Scalogram Images and Pre-trained Deep Neural Networks. Irbm 2022, 43, 22–31. [Google Scholar] [CrossRef]

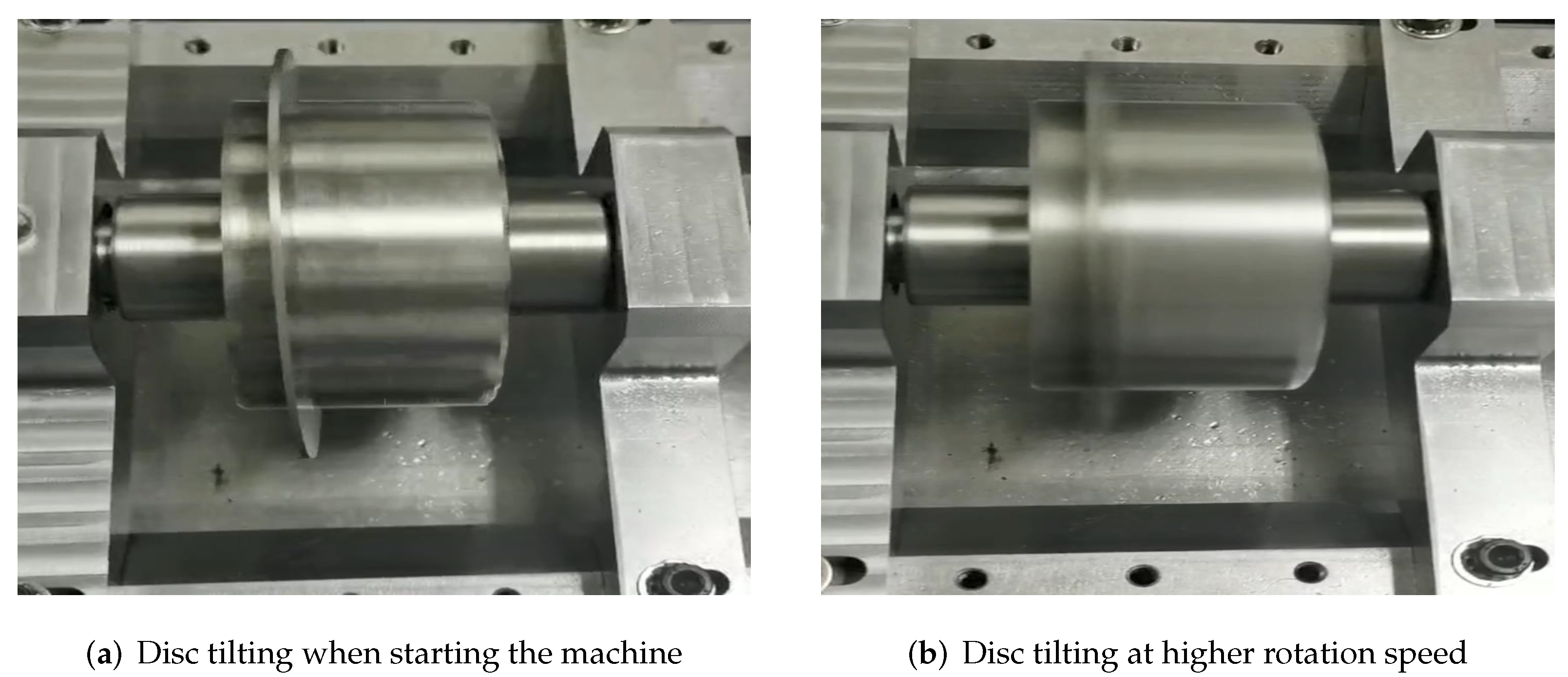
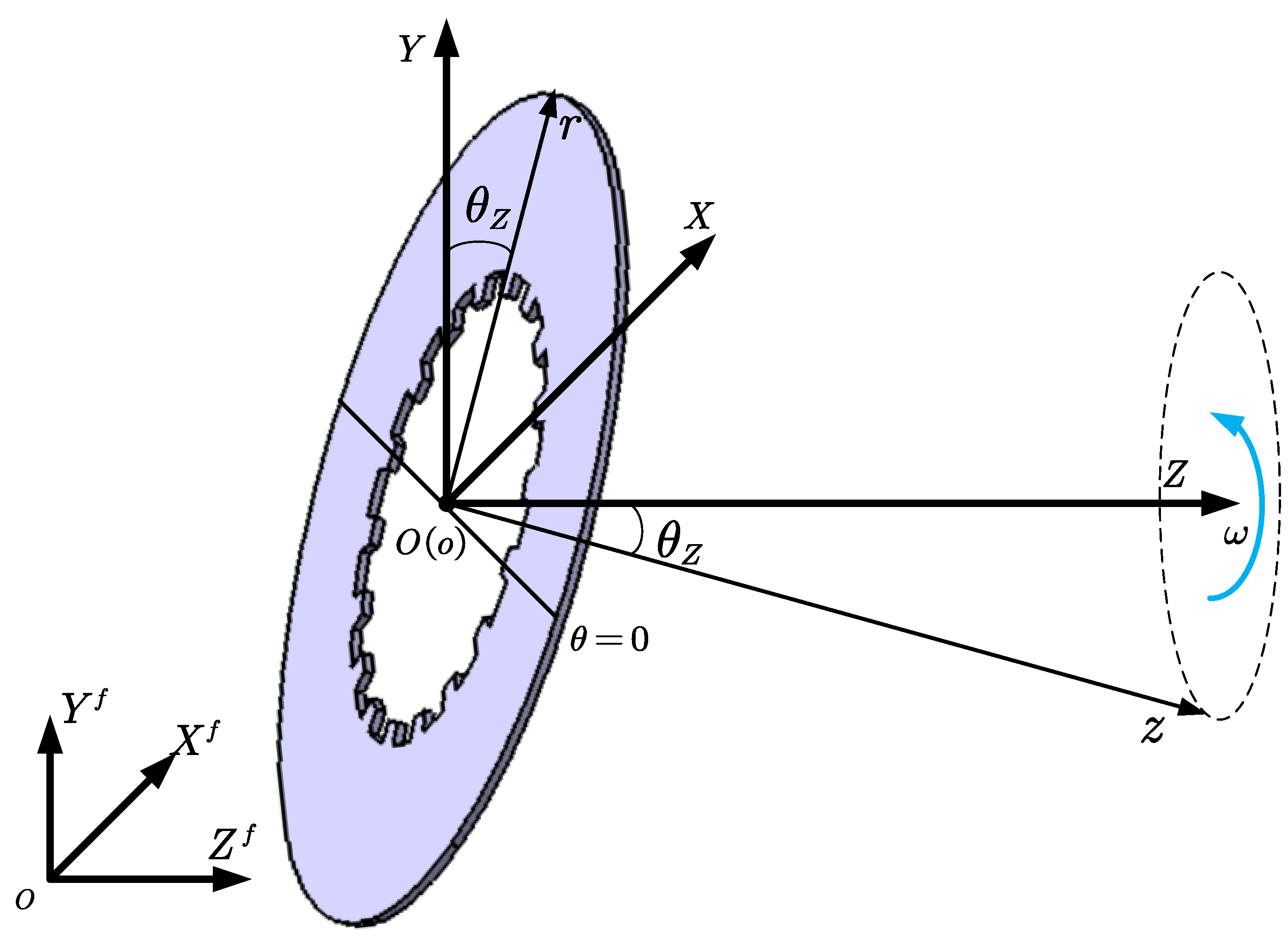
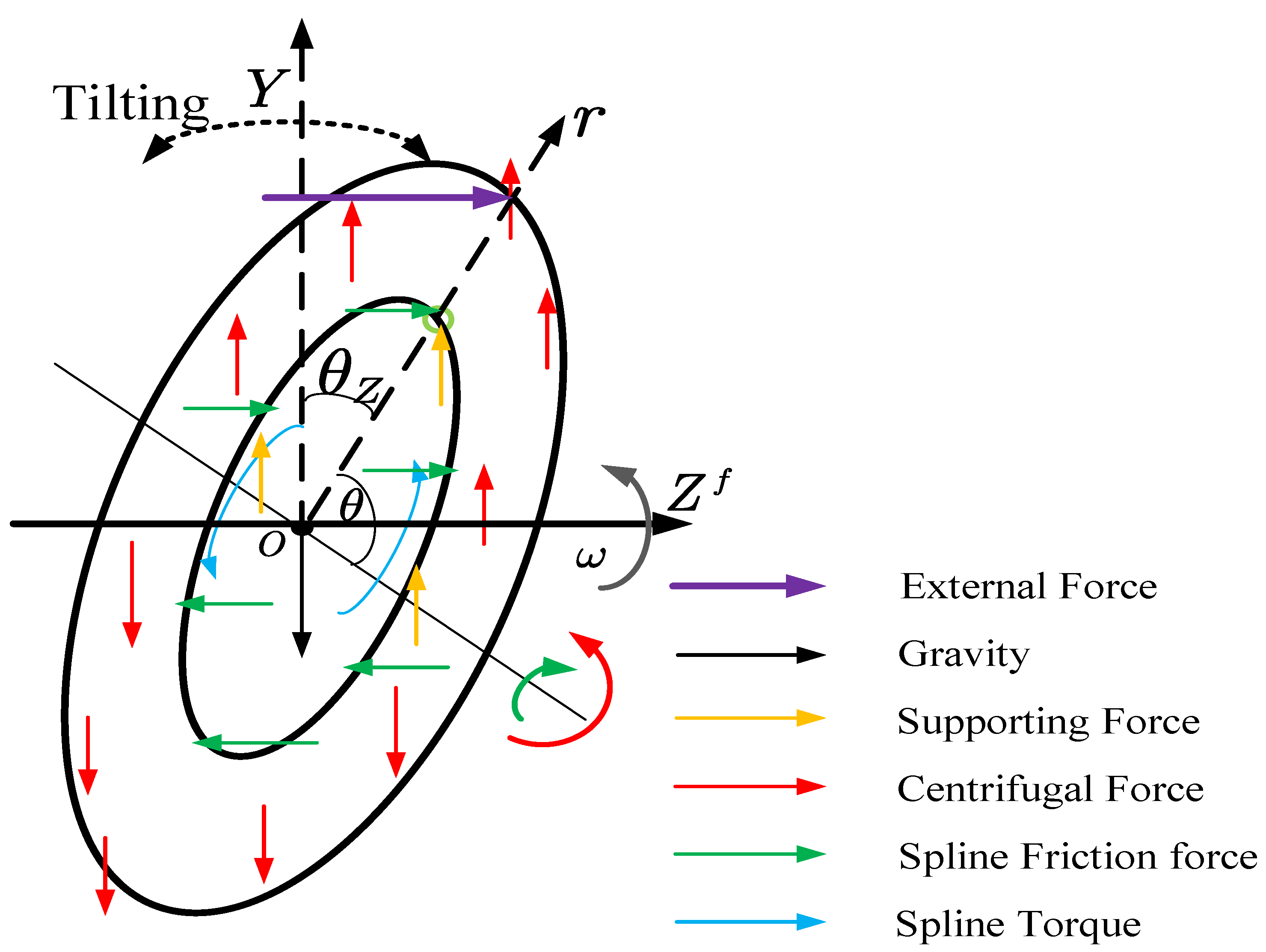
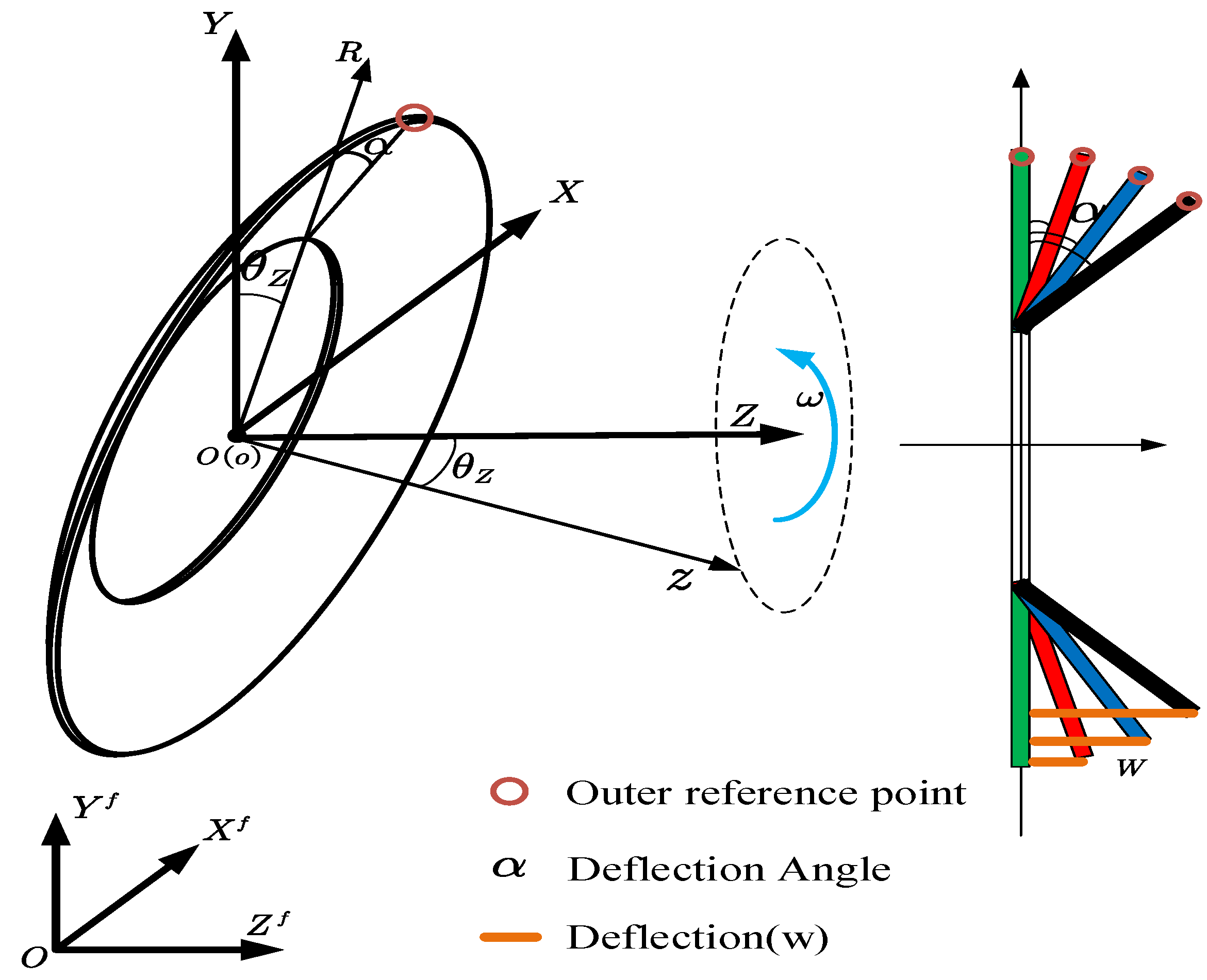


| Group | Impulse Force | Boundary Distance |
|---|---|---|
| Condition 1 (reference group) | 5 N | 7 mm |
| Condition 2 | 7 N | 7 mm |
| Condition 3 | 5 N | 6 mm |
| Deformation angle [] | 0 (flat), 5, 10, 15 | |
| Angular velocity [rad/s] | 40, 120, 200 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, J.; Ma, B.; Chen, M.; Yu, L.; Zheng, L. On the Effects of Disc Deformation on the Tilting-Induced Vibration of a Spline-Guided Spinning Disc with an Axial-Fixed Boundary. Appl. Sci. 2022, 12, 3637. https://doi.org/10.3390/app12073637
Xue J, Ma B, Chen M, Yu L, Zheng L. On the Effects of Disc Deformation on the Tilting-Induced Vibration of a Spline-Guided Spinning Disc with an Axial-Fixed Boundary. Applied Sciences. 2022; 12(7):3637. https://doi.org/10.3390/app12073637
Chicago/Turabian StyleXue, Jiaqi, Biao Ma, Man Chen, Liang Yu, and Liangjie Zheng. 2022. "On the Effects of Disc Deformation on the Tilting-Induced Vibration of a Spline-Guided Spinning Disc with an Axial-Fixed Boundary" Applied Sciences 12, no. 7: 3637. https://doi.org/10.3390/app12073637
APA StyleXue, J., Ma, B., Chen, M., Yu, L., & Zheng, L. (2022). On the Effects of Disc Deformation on the Tilting-Induced Vibration of a Spline-Guided Spinning Disc with an Axial-Fixed Boundary. Applied Sciences, 12(7), 3637. https://doi.org/10.3390/app12073637








