On the Physics of Kayaking
Abstract
:1. Introduction
2. General Model and Experimental Set-Up
2.1. Theoretical Motivation
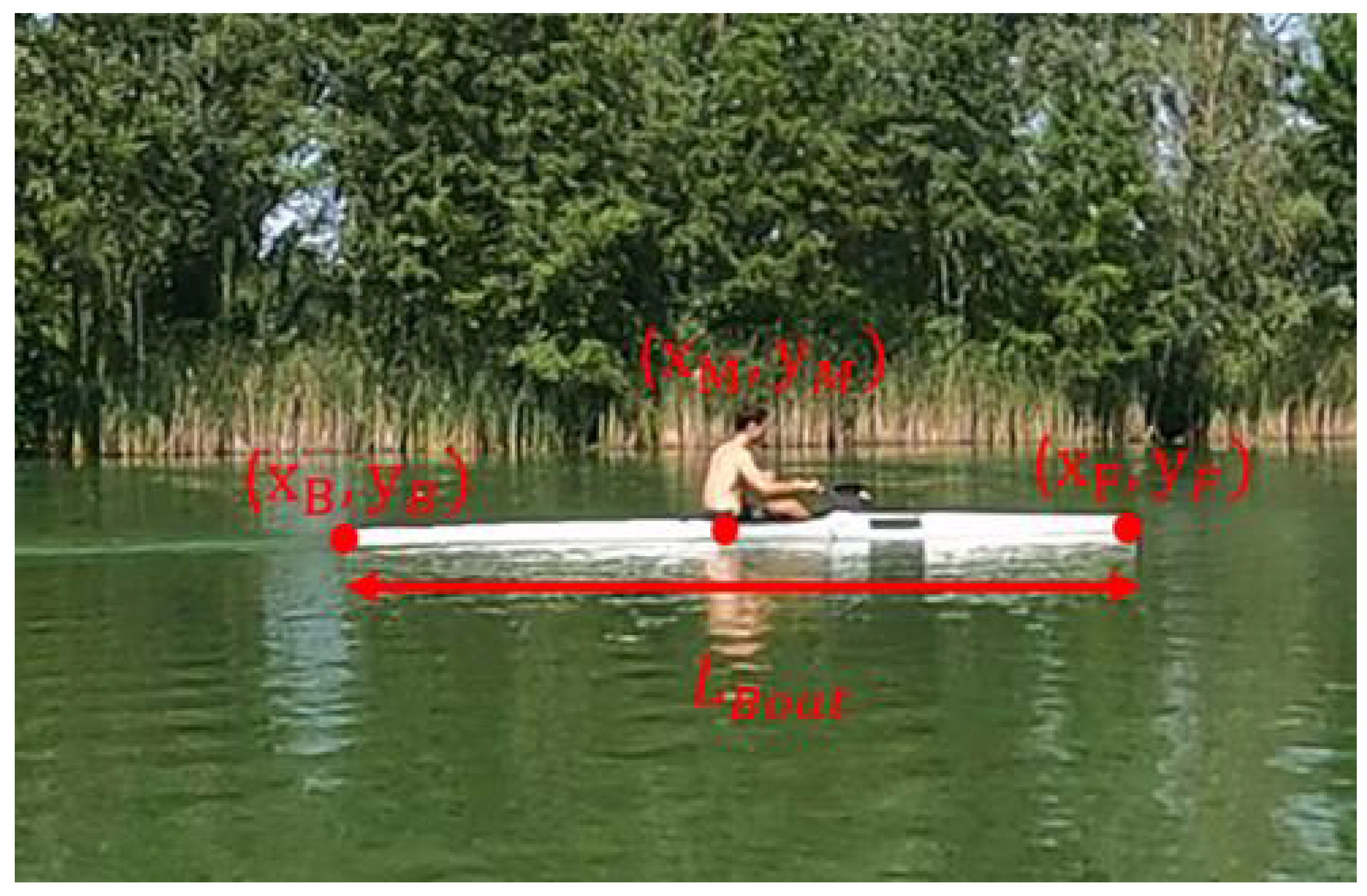
2.2. Experimental Set-Up
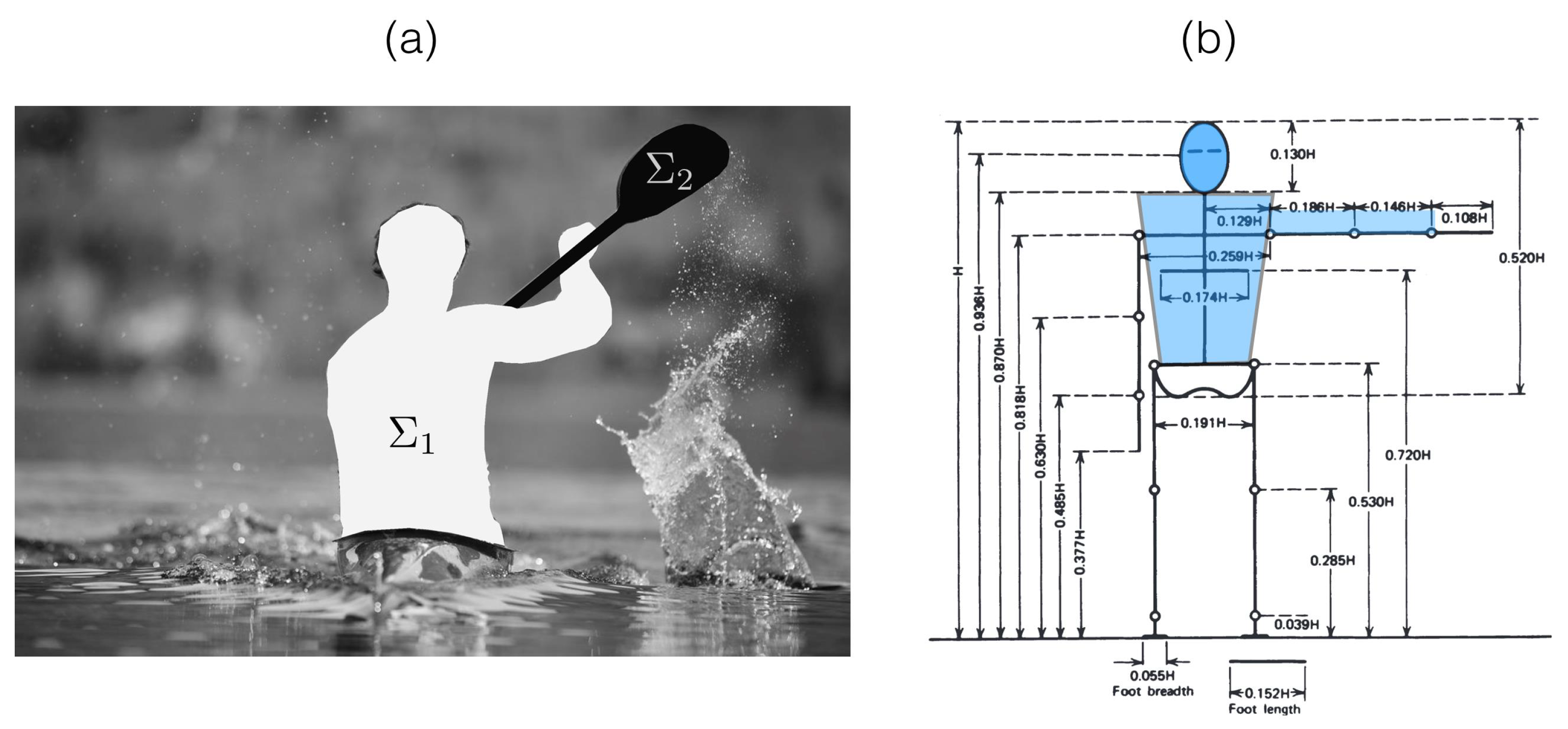
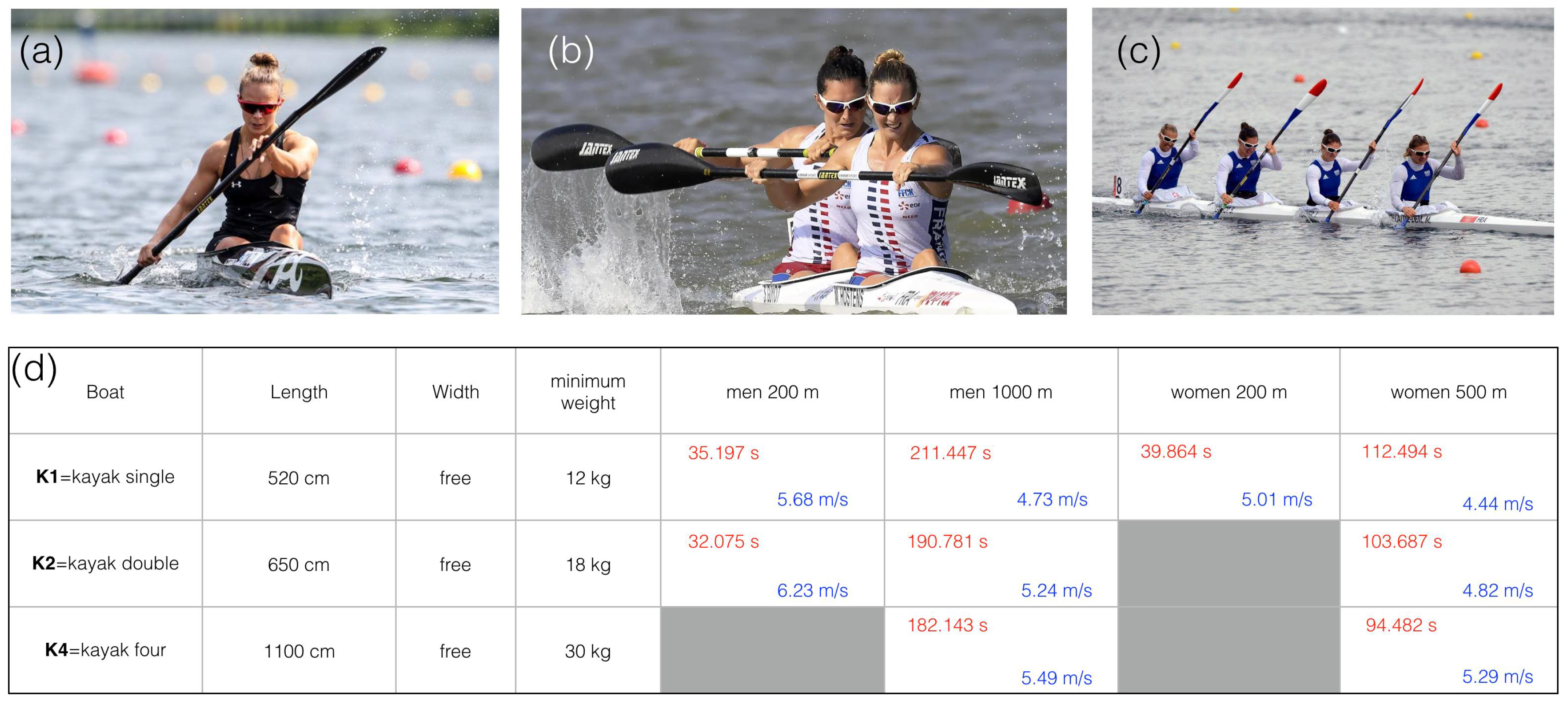
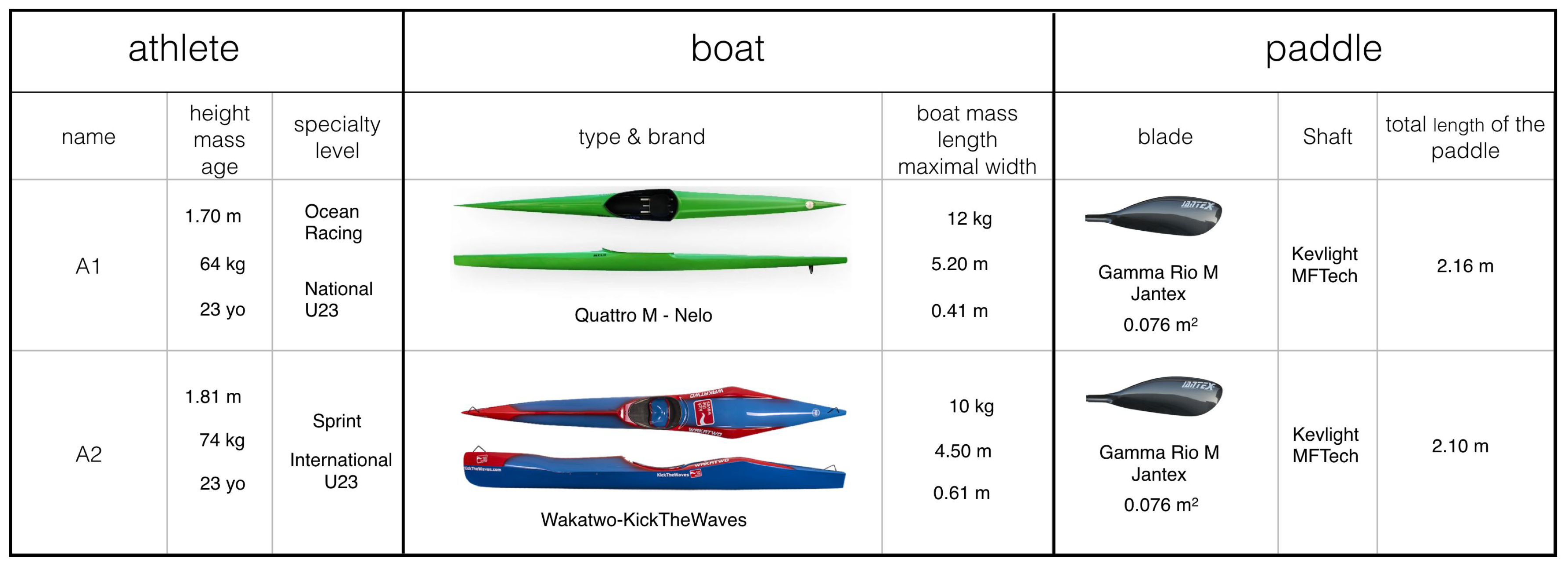
2.2.1. Athletes and Boats
2.2.2. Force Measurement
2.2.3. Velocity Measurement
3. Pure Deceleration: The Zero Propulsion Limit,
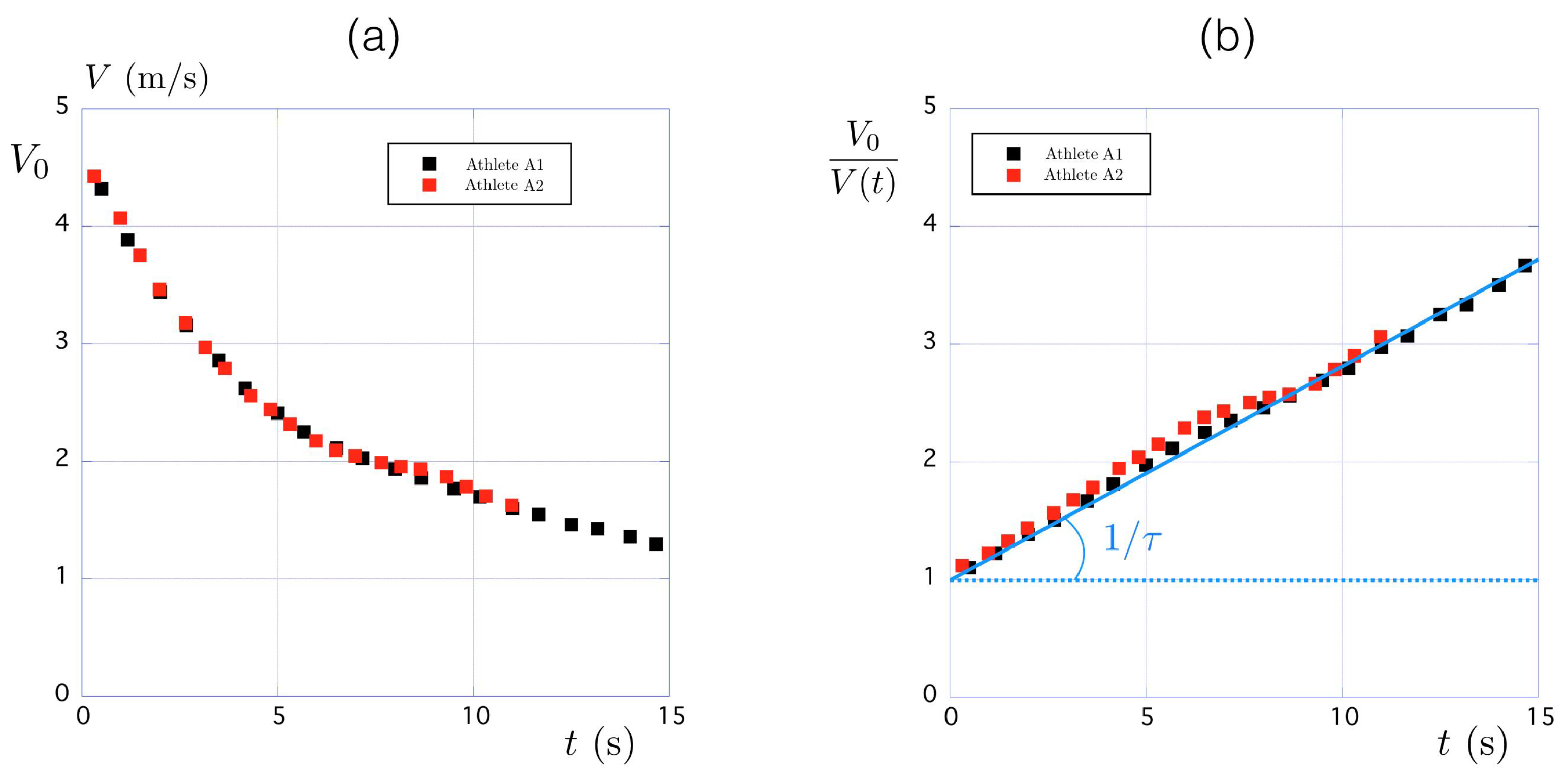
3.1. Observations and Velocity Evolution
3.2. The Effective Mass
3.3. The Total Drag,
4. Standing Start
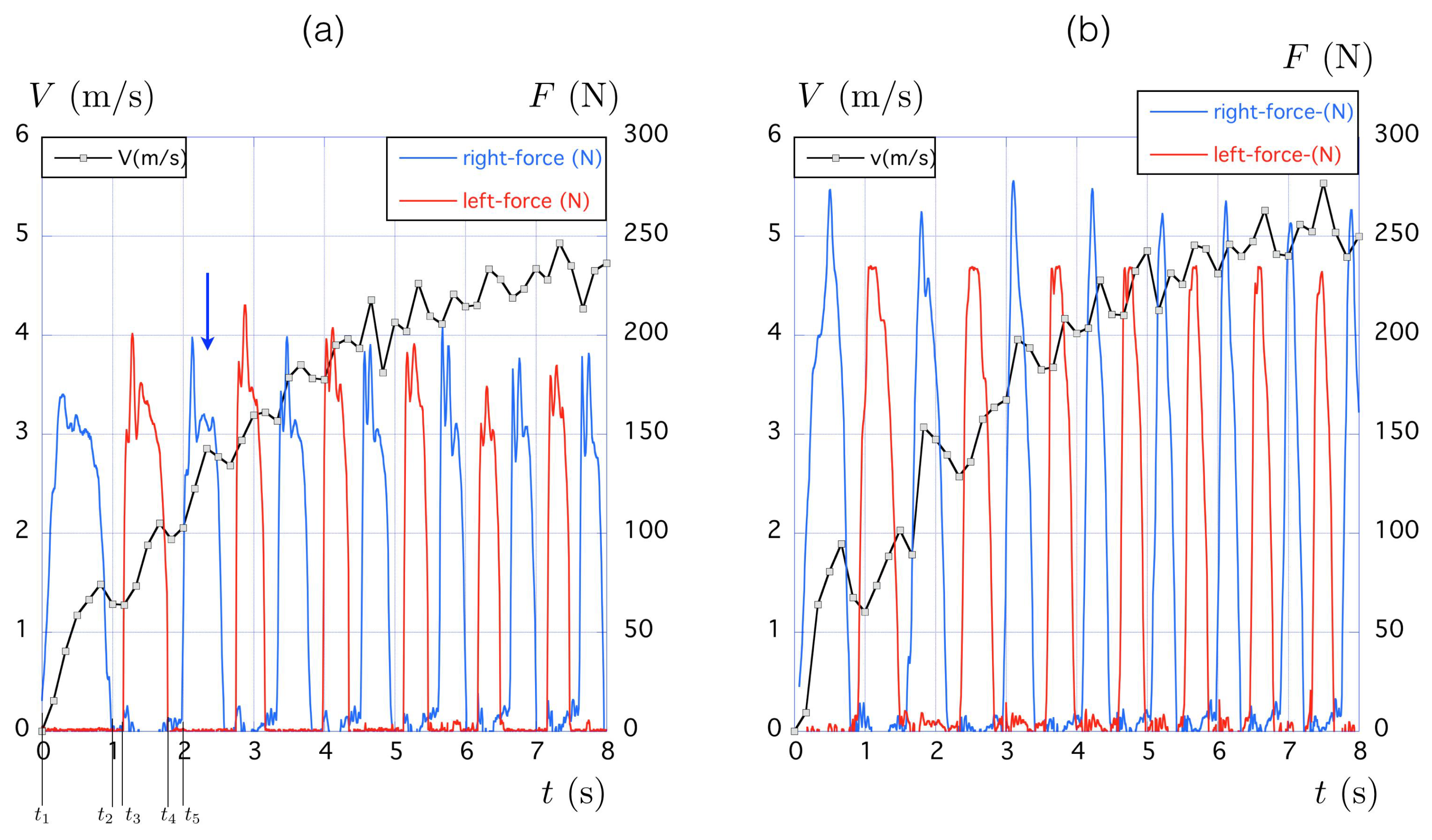
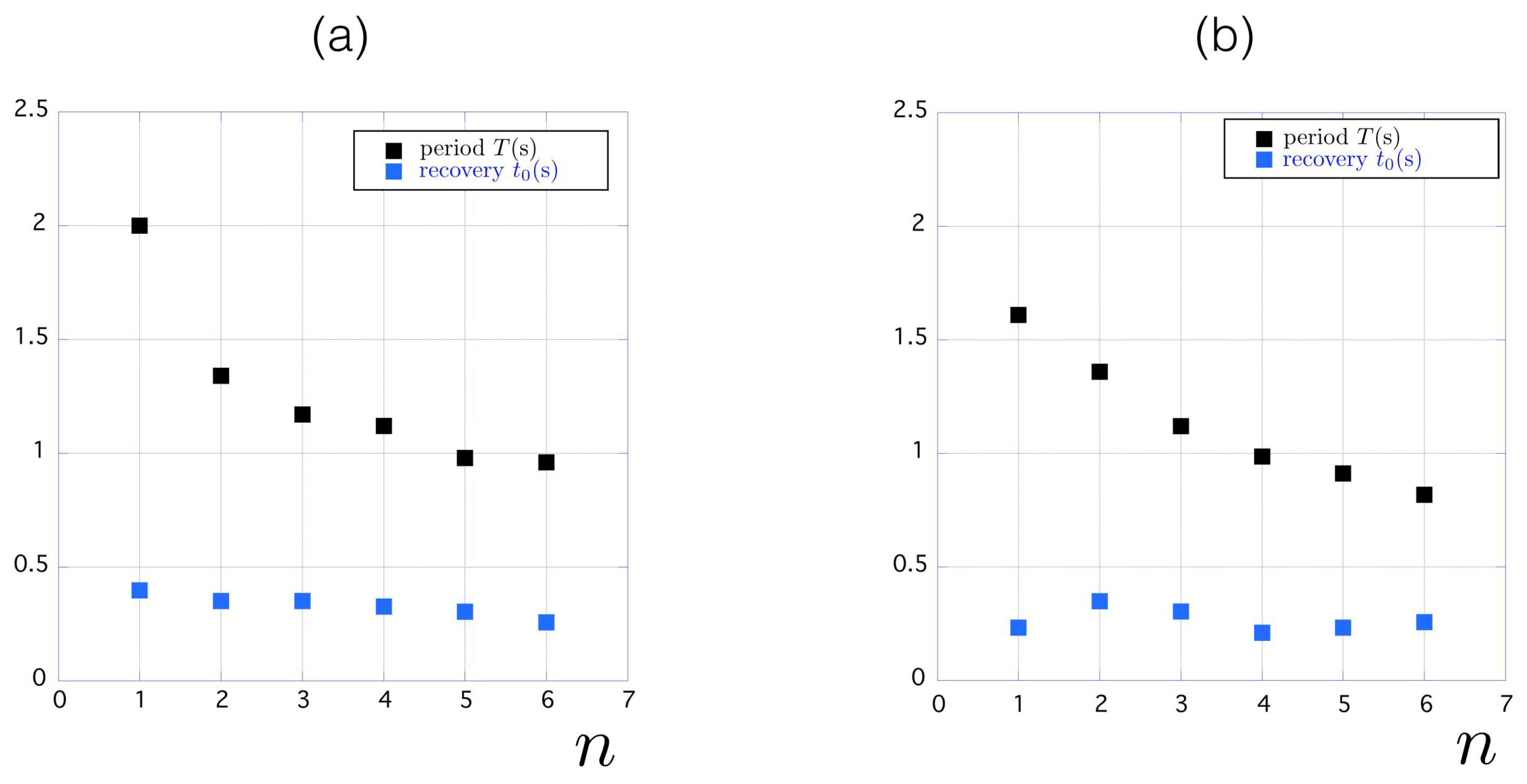
4.1. Single Paddling Stroke
4.2. Experimental Data of the Standing Start
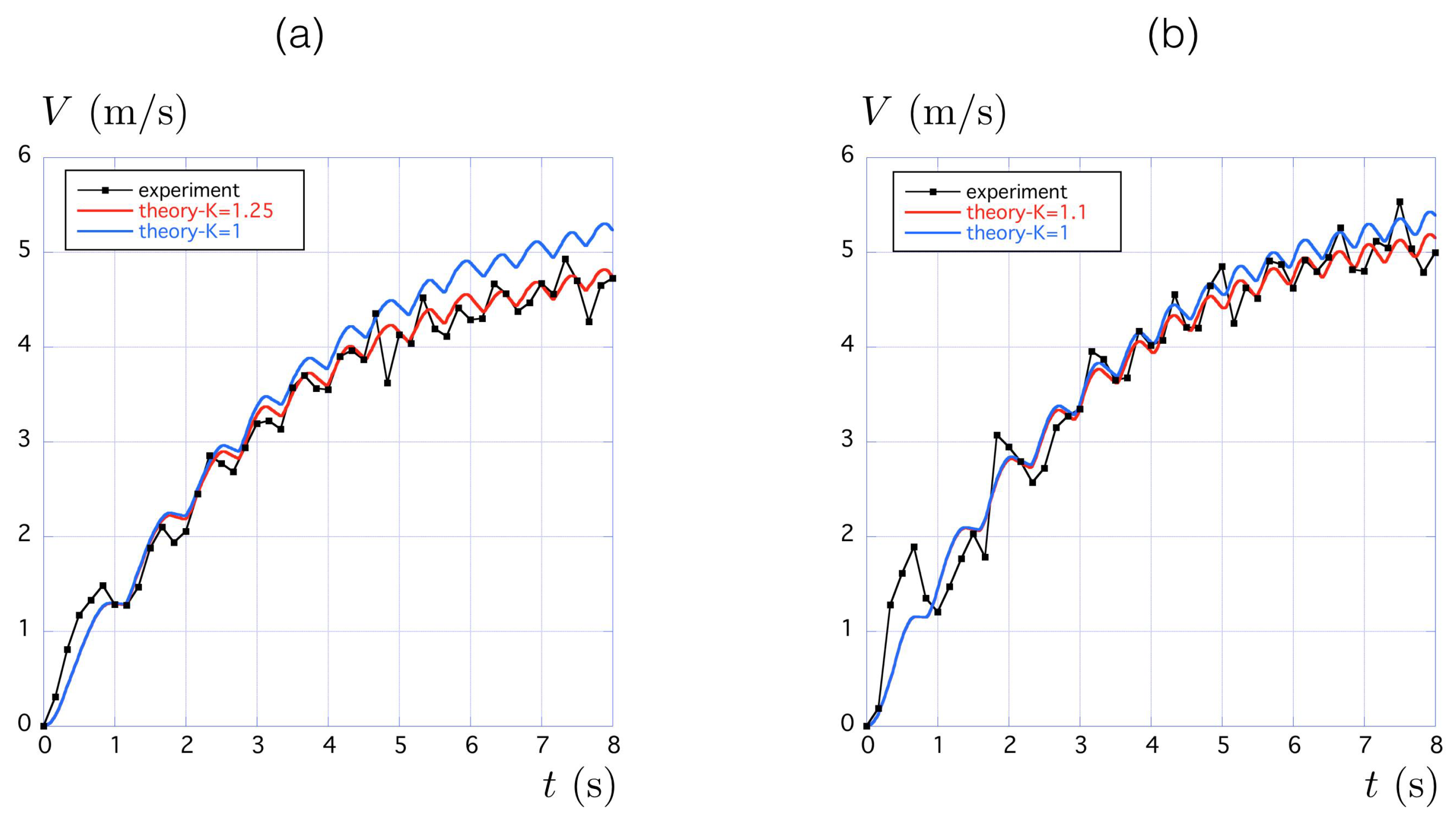
4.3. Theoretical vs. Experimental Velocity
4.4. An Algebraic Approximate Solution for the Mean Velocity
5. The 10 × 50 Meter Trial: Kayaking at Constant Velocity
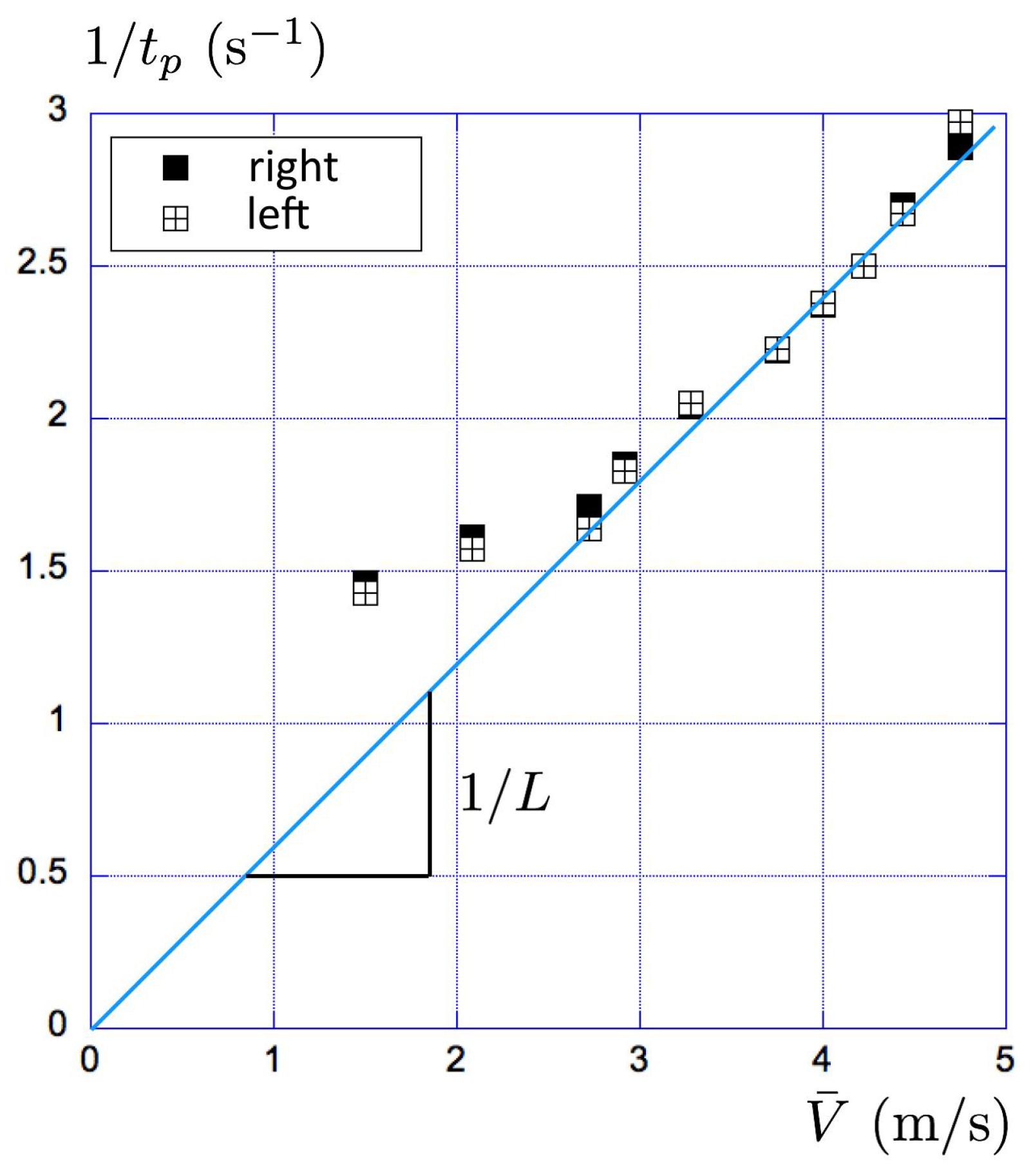
5.1. Experimental Data
5.2. Velocity–Stroke Rate Relationship Model
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Skin Friction Fs
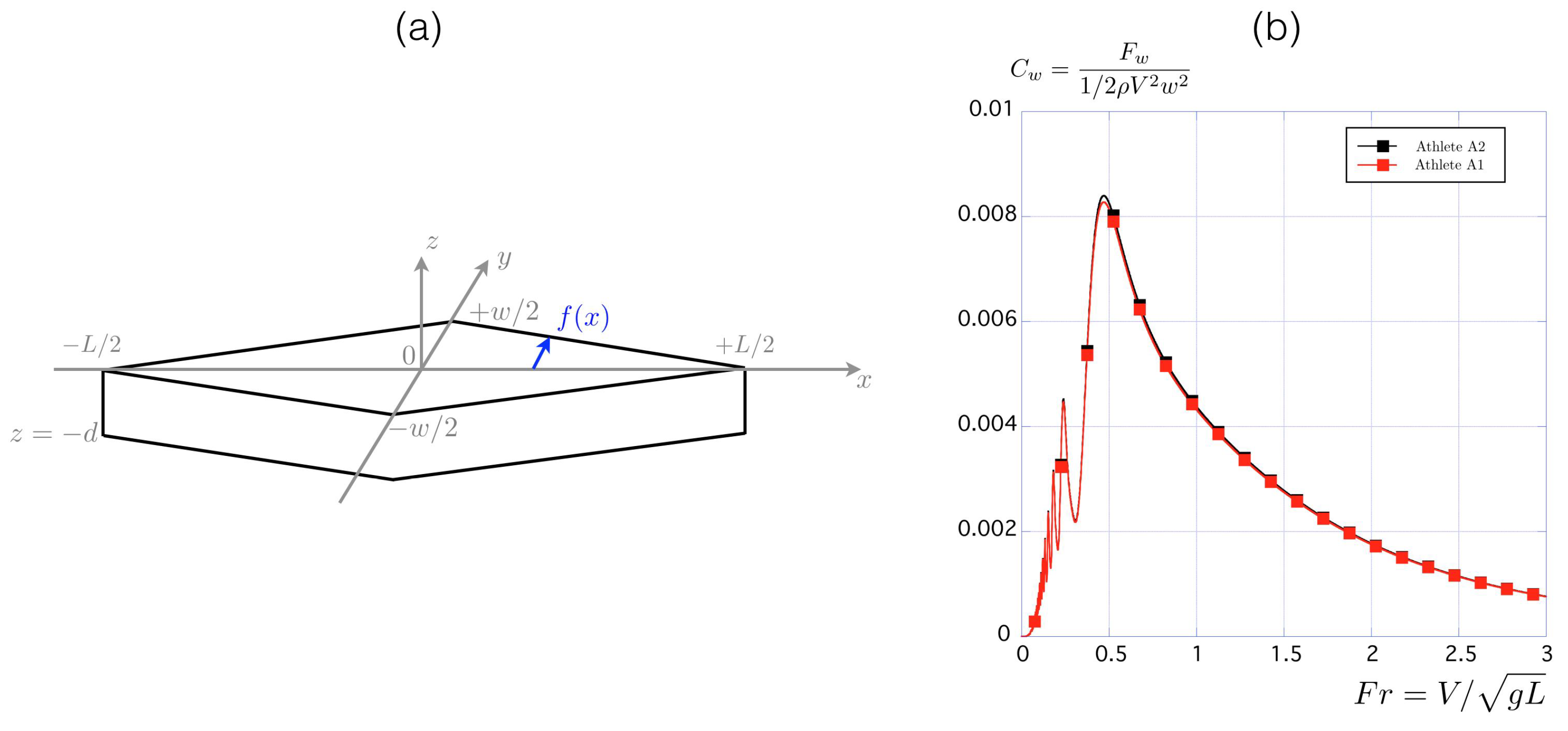
Appendix A.2. The Wave Drag Fw
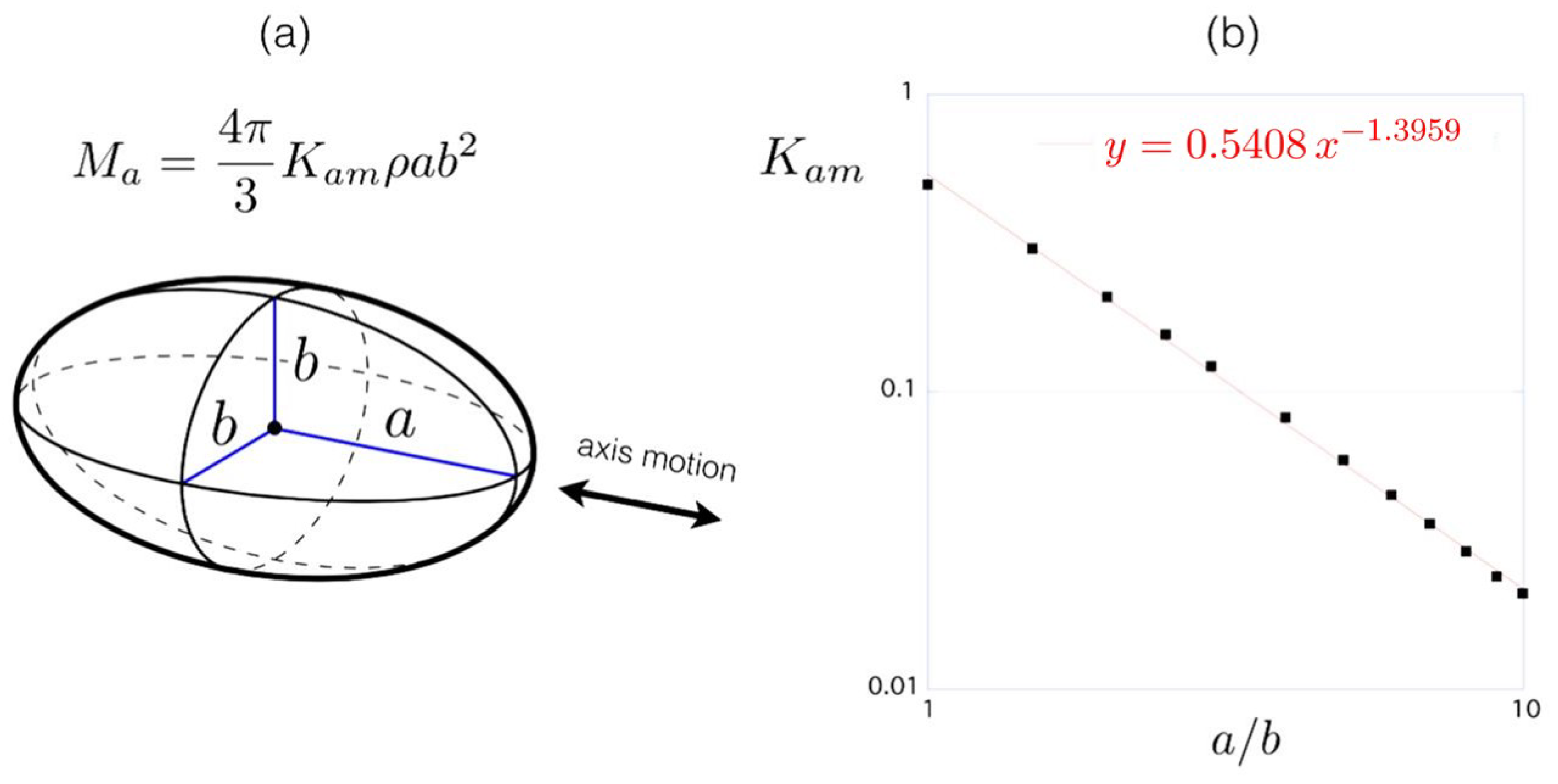
Appendix A.3. The Aerodynamic Force Fa
Appendix A.4. Comparison between the Theoretical Drag and the One Measured by Deceleration
| V | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 1 | |||||||||
| 2 | |||||||||
| 3 | |||||||||
| 4 | |||||||||
| 5 |
References
- Berglund, B.; McKenzie, D. Handbook of Sports Medicine and Science, Canoeing; Olympic Handbook of Sports Medicine Series; John Wiley & Sons: Hoboken, NJ, USA, 2018. [Google Scholar]
- Jackson, P. Performance prediction for Olympic kayaks. J. Sport Sci. 1995, 13, 239–245. [Google Scholar] [CrossRef] [PubMed]
- Gomes, B.B.; Conceição, F.; Pendergast, D.; Sanders, R.; Vaz, M.; Vilas-Boas, J. Is passive drag dependent on the interaction of kayak design and paddler weight in flat-water kayaking ? Sport Biomech. 2015, 14, 394–403. [Google Scholar] [CrossRef] [PubMed]
- Gomes, B.; Machado, L.; Ramos, N.; Conceição, F.; Sanders, R.; Vaz, M.; Vilas-Boas, J.; Pendergast, D. Effect of wetted surface area on friction, pressure, wave and total drag of a kayak. Sport Biomech. 2018, 17, 453–461. [Google Scholar] [CrossRef]
- Sumner, D.; Sprigings, E.J.; Bugg, J.D.; Heseltine, J.L. Fluid forces on kayak paddle blades of different design. Sport Eng. 2003, 6, 11–19. [Google Scholar] [CrossRef]
- Hémon, P. Hydrodynamic characteristics of sea kayak traditional paddles. Sport Eng. 2017, 21, 189–197. [Google Scholar] [CrossRef]
- Bjerkefors, A.; Tarassova, O.; Rosén, J.S.; Zakaria, P.; Arndt, A. Three-dimensional kinematic analysis and power output of elite flat-water kayakers. Sport Biomech. 2017, 17, 414–427. [Google Scholar] [CrossRef] [PubMed]
- Klitgaard, K.K.; Hauge, C.; Oliveira, A.S.C.; Heinen, F. A kinematic comparison of on-ergometer and on-water kayaking. Eur. J. Sport Sci. 2020, 21, 1375–1384. [Google Scholar] [CrossRef] [PubMed]
- Pickett, C.W.; Abbiss, C.; Zois, J.; Blazevich, A.J. Pacing and stroke kinematics in 200-m kayak racing. J. Sport Sci. 2020, 39, 1096–1104. [Google Scholar] [CrossRef]
- Goreham, J.A.; Miller, K.B.; Frayne, R.J.; Ladouceur, M. Pacing strategies and relationships between speed and stroke parameters for elite sprint kayakers in single boats. J. Sport Sci. 2021, 39, 2211–2218. [Google Scholar] [CrossRef]
- Helmer, R.; Farouil, A.; Baker, J.; Blanchonette, I. Instrumentation of a kayak paddle to investigate blade/water interactions. Procedia Eng. 2011, 13, 501–506. [Google Scholar] [CrossRef] [Green Version]
- Gomes, B.; Viriato, N.; Sanders, R.; Conceição, F.; Vilas-Boas, J.P.; Vaz, M. Analysis of the on-water paddling force profile of an elite kayaker. In Proceedings of the ISBS-Conference Proceedings Archive, Porto, Portugal, 27 June–1 July 2011. [Google Scholar]
- Gomes, B.B.; Ramos, N.; Conceição, F.; Sanders, R.; Vaz, M.; Vilas-Boas, J. Paddling force profiles at different stroke rates in elite sprint kayaking. J. Appl. Biomech. 2015, 31, 258–263. [Google Scholar] [CrossRef] [PubMed]
- Gomes, B.B.; Ramos, N.; Conceição, F.; Sanders, R.; Vaz, M.; Vilas-Boas, J. Paddling time parameters and paddling efficiency with the increase in stroke rate in kayaking. Sport Biomech. 2020. [Google Scholar] [CrossRef] [PubMed]
- Tullis, S.; Galipeau, C.; Morgoch, D. Detailed on-water measurements of blade forces and stroke efficiencies in sprint canoe. Multidiscip. Digit. Publ. Inst. Proc. 2018, 2, 306. [Google Scholar]
- Delgado, D.; Ruiz, C. One-Dimensional Mathematical Model for Kayak Propulsion. Appl. Sci. 2021, 11, 10393. [Google Scholar] [CrossRef]
- Patton, K. An Experimental Determination of Hydrodynamic Masses and Mechanical Impedances; Technical Report; Navy Underwater Sound Laboratory: New London, CT, USA, 1965. [Google Scholar]
- Fossati, F. Aero-Hydrodynamics and the Performance of Sailing Yachts: The Science Behind Sailing Yachts and Their Design; A & C Black: Valencia, CA, USA, 2009. [Google Scholar]
- Boucher, J.; Labbé, R.; Clanet, C.; Benzaquen, M. Thin or bulky: Optimal aspect ratios for ship hulls. Phys. Rev. Fluids 2018, 3, 074802. [Google Scholar] [CrossRef]
- Pendergast, D.; Bushnell, D.; Wilson, D.; Cerretelli, P. Energetics of kayaking. Eur. J. Appl. Physiol. Occup. Physiol. 1989, 59, 342–350. [Google Scholar] [CrossRef]
- Keller, J.B. A theory of competitive running. Phys. Today 1973, 26, 43. [Google Scholar] [CrossRef]
- Hadler, J. Coefficients for International Towing Tank Conference 1957 Model-Ship Correlation Line; Technical Report; David Taylor Model Basin: Washington, DC, USA, 1958. [Google Scholar]
- Molland, A.F.; Turnock, S.; Hudson, D. Ship Resistance and Propulsion: Practical Estimation of Propulsive Power, 2nd ed.; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Michell, J. The wave resistance of a ship. Philos. Mag. 1898, 45, 106–123. [Google Scholar] [CrossRef]
- Wehausen, J.V. The wave resistance of ships. Adv. Appl. Mech. 1973, 13, 93–245. [Google Scholar]
- Tuck, E. The wave resistance formula of JH Michell (1898) and its significance to recent research in ship hydrodynamics. ANZIAM J. 1989, 30, 365–377. [Google Scholar]
- Drillis, R.; Contini, R.; Bluestein, M. Body segment parameters. Artif. Limbs 1964, 8, 44–66. [Google Scholar] [PubMed]
- Barber, H.L. Effect of Wind in the Field of Play for Elite Sprint Kayakers. Master’s Thesis, Ottawa-Carleton Institute for Mechanical & Aerospace Engineering, Ottawa, ON, Canada, 2018. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prétot, C.; Carmigniani, R.; Hasbroucq, L.; Labbé, R.; Boucher, J.-P.; Clanet, C. On the Physics of Kayaking. Appl. Sci. 2022, 12, 8925. https://doi.org/10.3390/app12188925
Prétot C, Carmigniani R, Hasbroucq L, Labbé R, Boucher J-P, Clanet C. On the Physics of Kayaking. Applied Sciences. 2022; 12(18):8925. https://doi.org/10.3390/app12188925
Chicago/Turabian StylePrétot, Charlie, Rémi Carmigniani, Loup Hasbroucq, Romain Labbé, Jean-Philippe Boucher, and Christophe Clanet. 2022. "On the Physics of Kayaking" Applied Sciences 12, no. 18: 8925. https://doi.org/10.3390/app12188925
APA StylePrétot, C., Carmigniani, R., Hasbroucq, L., Labbé, R., Boucher, J.-P., & Clanet, C. (2022). On the Physics of Kayaking. Applied Sciences, 12(18), 8925. https://doi.org/10.3390/app12188925








