Recent Developments in Sonic Crystals as Barriers for Road Traffic Noise Mitigation
Abstract
1. Introduction
2. Sonic Crystals as Acoustic Barriers
- the density ratio M, that is, the ratio between the densities of the scatterers’ material and that of the medium in which they are immersed;
- the filling factor ff, expressing the ratio between the volume occupied by the scatterers and the total volume of the crystal;
- the lattice designs.
3. Parameters Influencing Insertion Loss and Band Gap
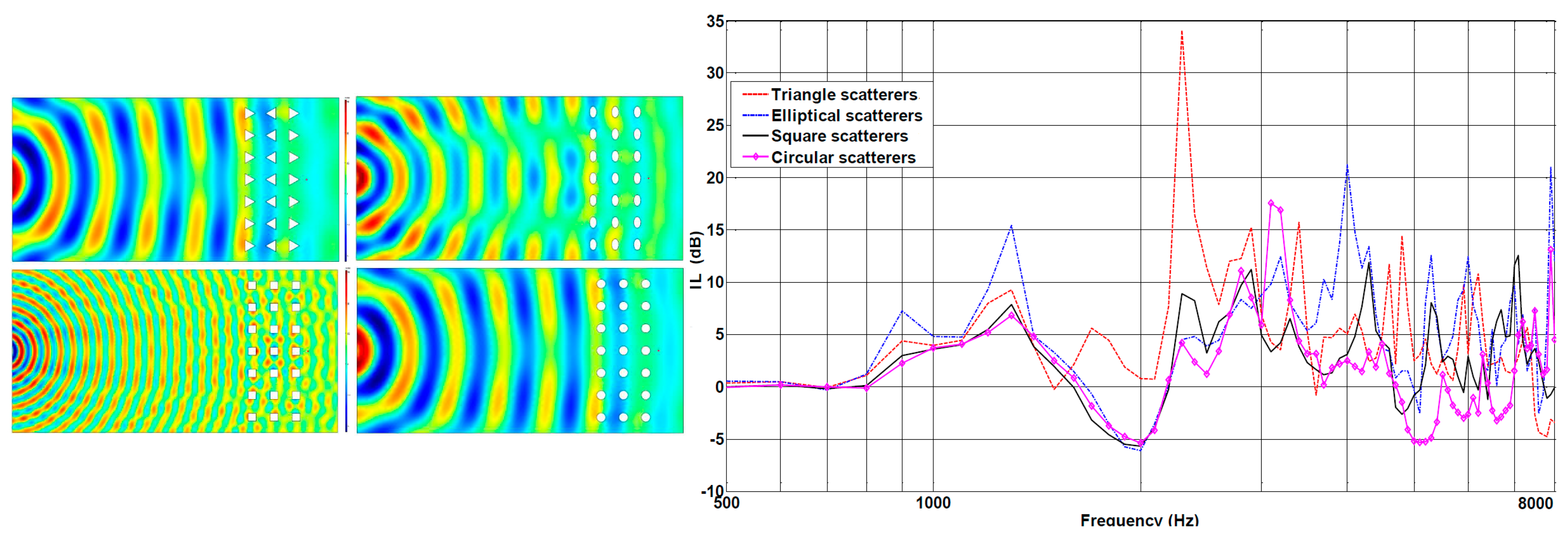
3.1. Shape of Scatterers
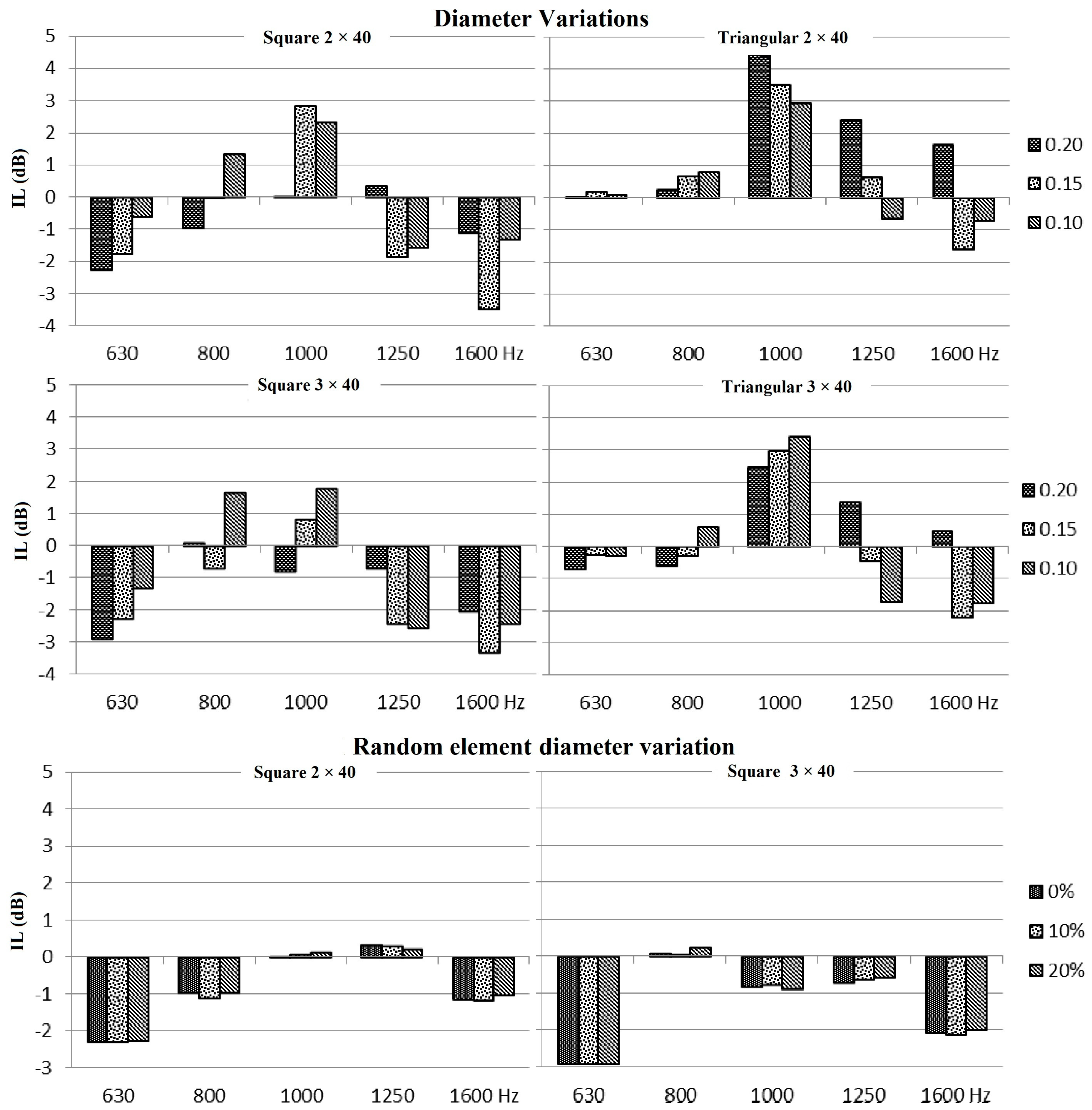
3.2. Diameter of Scatterers
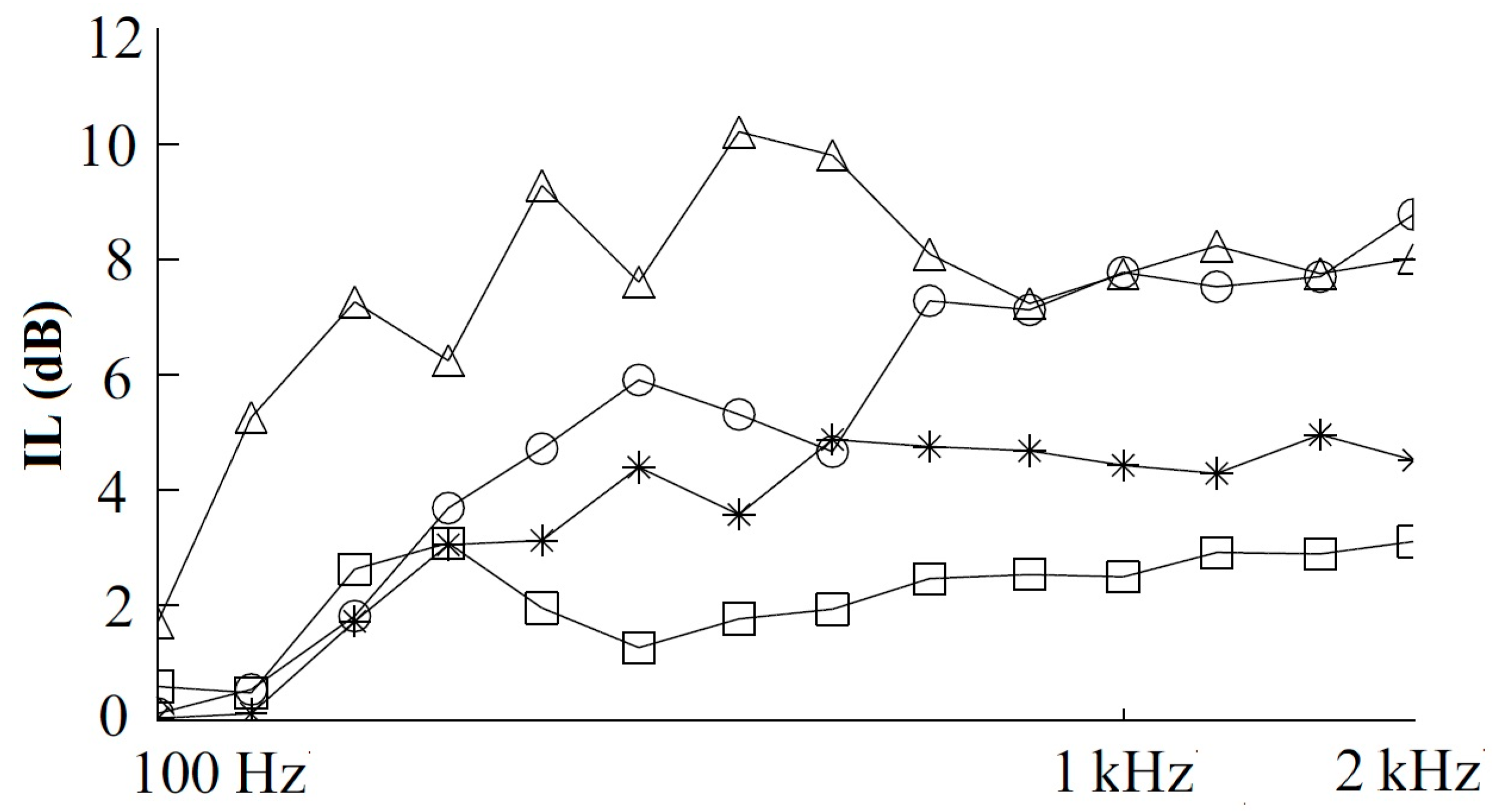
3.3. Number of Scatterers
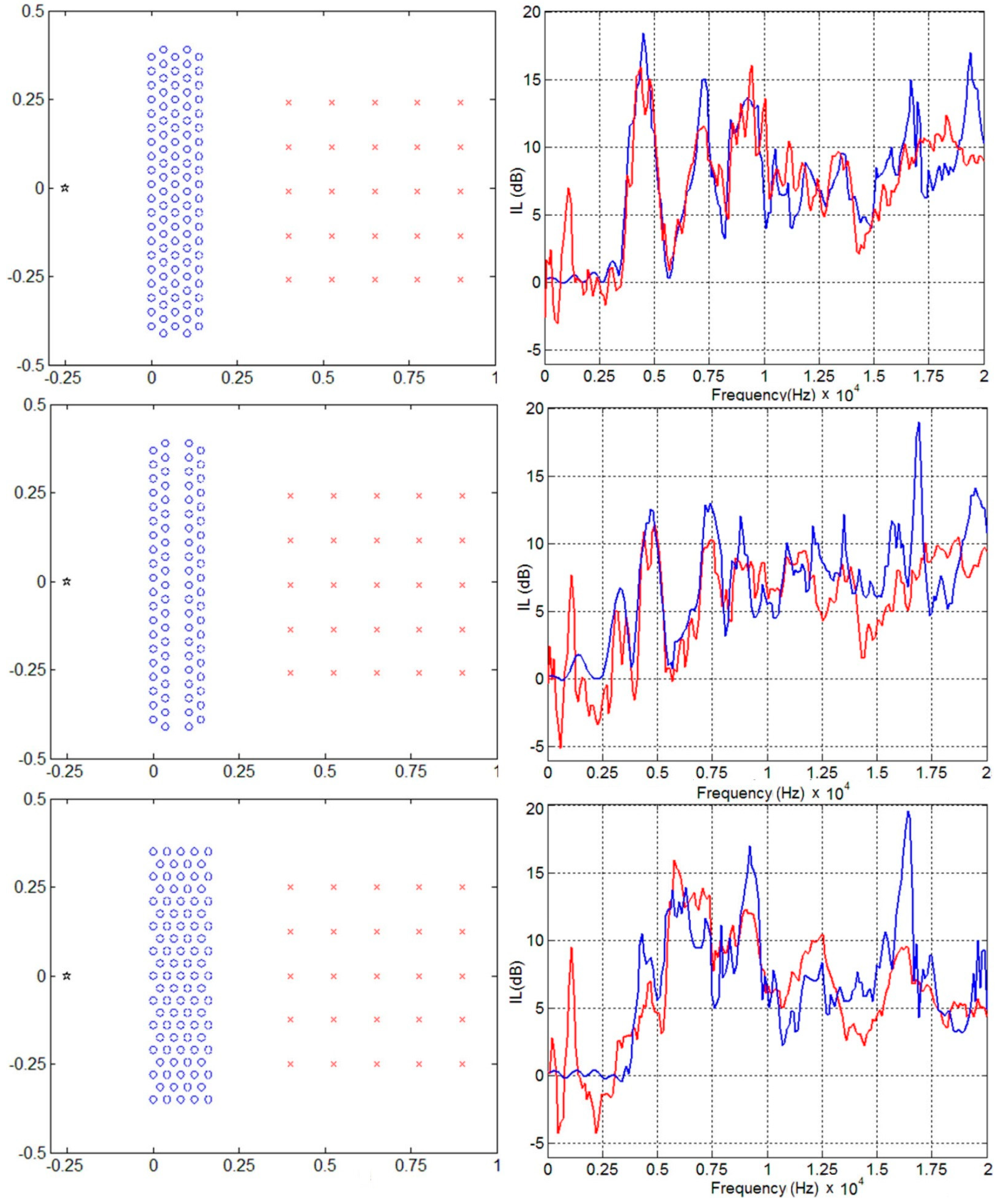
3.4. Filling Factor
4. Recent Applications
4.1. Hollow Scatterers
4.2. Scatterers Coated with Porous Material
4.3. Coupled Barriers
4.4. Low-Height Barriers
4.5. Green Barriers
5. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Licitra, G.; Ascari, E.; Fredianelli, L. Prioritizing Process in Action Plans: A Review of Approaches. Curr. Pollut. Rep. 2017, 3, 151–161. [Google Scholar] [CrossRef]
- European Commission. Report From The Commission To The European Parliament And The Council On the Implementation of the Environmental Noise Directive in accordance with Article 11 of Directive 2002/49/EC. COM/2017/0151 final; European Commission: Brussels, Belgium, 2017. [Google Scholar]
- Fredianelli, L.; Carpita, S.; Licitra, G. A procedure for deriving wind turbine noise limits by taking into account annoyance. Sci. Total Environ. 2019, 648, 728–736. [Google Scholar] [CrossRef] [PubMed]
- Muzet, A. Environmental noise, sleep and health. Sleep Med. Rev. 2007, 11, 135–142. [Google Scholar] [CrossRef] [PubMed]
- de Kluizenaar, Y.; Janssen, S.A.; van Lenthe, F.J.; Miedema, H.M.; Mackenbach, J.P. Long-term road traffic noise exposure is associated with an increase in morning tiredness. J. Acoust. Soc. Am. 2009, 126, 626–633. [Google Scholar] [CrossRef] [PubMed]
- Miedema, H.M.; Oudshoorn, C.G. Annoyance from transportation noise: Relationships with exposure metrics DNL and DENL and their confidence intervals. Environ. Health Perspect. 2001, 109, 409. [Google Scholar] [CrossRef] [PubMed]
- Babisch, W.; Beule, B.; Schust, M.; Kersten, N.; Ising, H. Traffic noise and risk of myocardial infarction. Epidemiology 2005, 33–40. [Google Scholar] [CrossRef]
- Babisch, W. Road traffic noise and cardiovascular risk. Noise Health 2008, 10, 27. [Google Scholar] [CrossRef]
- Lercher, P.; Evans, G.W.; Meis, M. Ambient noise and cognitive processes among primary schoolchildren. Environ. Behav. 2003, 35, 725–735. [Google Scholar] [CrossRef]
- Chetoni, M.; Ascari, E.; Bianco, F.; Fredianelli, L.; Licitra, G.; Cori, L. Global noise score indicator for classroom evaluation of acoustic performances in LIFE GIOCONDA project. Noise Mapp. 2016, 3. [Google Scholar] [CrossRef]
- Van Kempen, E.; Babisch, W. The quantitative relationship between road traffic noise and hypertension: A meta-analysis. J. Hypertens. 2012, 30, 1075–1086. [Google Scholar] [CrossRef]
- Licitra, G.; Moro, A.; Teti, L.; Del Pizzo, L.G.; Bianco, F. Modelling of acoustic ageing of rubberized pavements. Appl. Acoust. 2019, 146, 237–245. [Google Scholar] [CrossRef]
- Garai, M.; Guidorzi, P. European methodology for testing the airborne sound insulation characteristics of noise barriers in situ: Experimental verification and comparison with laboratory data. J. Acoust. Soc. Am. 2000, 108, 1054–1067. [Google Scholar] [CrossRef] [PubMed]
- Mir, F.; Saadatzi, M.; Ahmed, R.U.; Banerjee, S. Acoustoelastic MetaWall noise barriers for industrial application with simultaneous energy harvesting capability. Appl. Acoust. 2018, 139, 282–292. [Google Scholar] [CrossRef]
- Suslick, K.S.; Crum, L.A.; Crocker, M.J. Encyclopedia of Acoustics; John Wiley & Sons: Crocker, MJ, USA, 1997; pp. 271–282. [Google Scholar]
- Lee, J.; Kim, J.; Park, T.; Chang, S.; Kim, I. Reduction Effects of Shaped Noise Barrier for Reflected Sound. J. Civ. Environ. Eng. 2015, 5, 1. [Google Scholar]
- Gupta, A. A review on sonic crystal, its applications and numerical analysis techniques. Acoust. Phys. 2014, 60, 223–234. [Google Scholar] [CrossRef]
- Morandi, F.; Marzani, A.; De Cesaris, S.; Barbaresi, L.; Garai, M. Soni crystals as tunable noise barriers. Riv. Ital. Acust. 2017, 40, 1–19. (In italian) [Google Scholar]
- Martínez-Sala, R.; Sancho, J.; Sánchez, J.V.; Gómez, V.; Llinares, J.; Meseguer, F. Sound attenuation by sculpture. Nature 1995, 378, 241. [Google Scholar] [CrossRef]
- Sanchez-Perez, J.V.; Rubio, C.; Martinez-Sala, R.; Sanchez-Grandia, R.; Gomez, V. Acoustic barriers based on periodic arrays of scatterers. Appl. Phys. Lett. 2002, 81, 5240–5242. [Google Scholar] [CrossRef]
- Romero-García, V.; Garcia-Raffi, L.M.; Sánchez-Pérez, J.V. Evanescent waves and deaf bands in sonic crystals. AIP Adv. 2011, 1, 041601. [Google Scholar] [CrossRef]
- Gupta, A.; Chew, C.H.; Lim, K.M. Effect of periodic structure on sound propagation. In Fourth International Conference on Experimental Mechanics. Int. Soc. Opt. Photonics 2010, 7522, 75223D. [Google Scholar] [CrossRef]
- Garcia-Raffi, L.M.; Salmerón-Contreras, L.J.; Herrero-Durá, I.; Picó, R.; Redondo, J.; Sánchez-Morcillo, V.J.; Staliunas, K.; Adkins, N.J.E.; Cebrecos, A.; Romero-Garcia, V.; et al. Broadband reduction of the specular reflections by using sonic crystals: A proof of concept for noise mitigation in aerospace applications. Aerosp. Sci. Technol. 2018, 73, 300–308. [Google Scholar] [CrossRef]
- Garcia-Raffi, L.M.; Salmerón-Contreas, L.J.; Herrero-Durá, I.; Picó, R.; Redondo, J.; Sánchez-Morcillo, V.J.; Cebrecos, A.; Romero-García, V.; Staliunas, K. Reflection of sound by Sonic Crystals: An application to the aerospace engineering. In Proceedings of the EuroRegio2016, Porto, Portugal, 13–15 June 2016. [Google Scholar]
- Maldovan, M. Sound and heat revolutions in phononics. Nature 2013, 503, 209. [Google Scholar] [CrossRef] [PubMed]
- Li, X.F.; Ni, X.; Feng, L.; Lu, M.H.; He, C.; Chen, Y.F. Tunable unidirectional sound propagation through a sonic-crystal-based acoustic diode. Phys. Rev. Lett. 2011, 106, 084301. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.Y.; Ye, Z. Theoretical analysis of acoustic stop bands in two-dimensional periodic scattering arrays. Phys. Rev. E 2001, 64, 036616. [Google Scholar] [CrossRef]
- Robertson, W.M.; Rudy, J.F., III. Measurement of acoustic stop bands in two-dimensional periodic scattering arrays. J. Acoust. Soc. Am. 1998, 104, 694–699. [Google Scholar] [CrossRef]
- Sánchez-Pérez, J.V.; Caballero, D.; Mártinez-Sala, R.; Rubio, C.; Sánchez-Dehesa, J.; Meseguer, F.; Llinares, J.; Gálvez, F. Sound attenuation by a two-dimensional array of rigid cylinders. Phys. Rev. Lett. 1998, 80, 5325. [Google Scholar] [CrossRef]
- Sanchis, L.; Cervera, F.; Sanchez-Dehesa, J.; Sanchez-Perez, J.V.; Rubio, C.; Martínez-Sala, R. Reflectance properties of two-dimensional sonic band-gap crystals. J. Acoust. Soc. Am. 2001, 109, 2598–2605. [Google Scholar] [CrossRef]
- Sanchis, L.; Håkansson, A.; Cervera, F.; Sánchez-Dehesa, J. Acoustic interferometers based on two-dimensional arrays of rigid cylinders in air. Phys. Rev. B 2003, 67, 035422. [Google Scholar] [CrossRef]
- Ke, G.; Zheng, Z.C. Sound propagation around arrays of rigid and porous cylinders in free space and near a ground boundary. J. Sound Vib. 2016, 370, 43–53. [Google Scholar] [CrossRef]
- Castiñeira-Ibañez, S.; Rubio, C.; Sánchez-Pérez, J.V. Environmental noise control during its transmission phase to protect buildings. Des. Model Acoust. Barriers Based Arrays Isol. Scatt. Build. Environ. 2015, 93, 179–185. [Google Scholar] [CrossRef]
- Van der Aa, B.; Forssén, J. Shape-optimal design of graded index sonic crystal formations using natural cubic splines. Appl. Acoust. 2014, 78, 98–111. [Google Scholar] [CrossRef]
- Martins, M.; Godinho, L.; Picado-Santos, L. Numerical evaluation of sound attenuation provided by periodic structures. Arch. Acoust. 2013, 38, 503–516. [Google Scholar] [CrossRef]
- Jean, P.; Defrance, J. Sound propagation in rows of cylinders of infinite extent: Application to sonic crystals and thickets along roads. Acta Acust. United Acust. 2015, 101, 474–483. [Google Scholar] [CrossRef]
- Godinho, L.; Soares, D., Jr.; Santos, P.G. Efficient analysis of sound propagation in sonic crystals using an ACA–MFS approach. Eng. Anal. Bound. Elem. 2016, 69, 72–85. [Google Scholar] [CrossRef]
- Amado-Mendes, P.; Godinho, L.; Santos, P.G.; Dias, A.G.; Martins, M. Laboratory and full-scale experimental evaluation of the acoustic behaviour of sonic crystal noise barriers. In Proceedings of the International Congress on Acoustics, Buenos Aires, Argentina, 5–9 September 2016. [Google Scholar]
- Chong, Y. Sonic Crystal Noise Barriers. Ph.D. Thesis, The Open University, Milton Keynes, UK, 2012. [Google Scholar]
- Godinho, L.; Redondo, J.; Amado-Mendes, P. The method of fundamental solutions for the analysis of infinite 3D sonic crystals. Eng. Anal. Bound. Elem. 2019, 98, 172–183. [Google Scholar] [CrossRef]
- Romero-García, V.; Lagarrigue, C.; Groby, J.P.; Richoux, O.; Tournat, V. Tunable acoustic waveguides in periodic arrays made of rigid square-rod scatterers: Theory and experimental realization. J. Phys. D Appl. Phys. 2013, 46, 305108. [Google Scholar] [CrossRef]
- Pichard, H.; Richoux, O.; Groby, J.P. Experimental demonstrations in audible frequency range of band gap tunability and negative refraction in two-dimensional sonic crystal. J. Acoust. Soc. Am. 2012, 132, 2816–2822. [Google Scholar] [CrossRef]
- Rubio, C.; Castiñeira-Ibáñez, S.; Uris, A.; Belmar, F.; Candelas, P. Numerical simulation and laboratory measurements on an open tunable acoustic barrier. Appl. Acoust. 2018, 141, 144–150. [Google Scholar] [CrossRef]
- Alagoz, S. A sonic crystal diode implementation with a triangular scatterer matrix. Appl. Acoust. 2014, 76, 402–406. [Google Scholar] [CrossRef]
- Sigalas, M.M. Defect states of acoustic waves in a two-dimensional lattice of solid cylinders. J. Appl. Phys. 1998, 84, 3026–3030. [Google Scholar] [CrossRef]
- Peiró-Torres, M.P.; Redondo, J.; Bravo, J.M.; Pérez, J.S. Open noise barriers based on sonic crystals. Advances in noise control in transport infrastructures. Transp. Res. Procedia 2016, 18, 392–398. [Google Scholar] [CrossRef]
- Sharma, G.S.; Skvortsov, A.; MacGillivray, I.; Kessissoglou, N. Acoustic performance of periodic steel cylinders embedded in a viscoelastic medium. J. Sound Vib. 2018. [Google Scholar] [CrossRef]
- Gupta, A.; Lim, K.M.; Chew, C.H. Design of radial sonic crystal for sound attenuation from divergent sound source. Wave Motion 2015, 55, 1–9. [Google Scholar] [CrossRef]
- Morandi, F.; Miniaci, M.; Marzani, A.; Barbaresi, L.; Garai, M. Standardised acoustic characterisation of sonic crystals noise barriers: Sound insulation and reflection properties. Appl. Acoust. 2016, 114, 294–306. [Google Scholar] [CrossRef]
- Jiang, G.; Liu, Y.; Wu, Y.; Xu, W.; Kong, Q.; Zhang, C. Transmission and radiation of acoustic oblique incident through tube arrays based on phononic crystals theory. Appl. Acoust. 2017, 116, 117–126. [Google Scholar] [CrossRef]
- Santos, P.; Carbajo, J.; Rui, D.; Godinho, L.; Mendes, P.A.; Soriano, J.R. Insertion loss provided by sonic crystal type barrier—Experimental and numerical evaluation on a reduced scale model. In Proceedings of the 45° Congreso Espanol de Acustica, Murcia, Spain, 29–31 October 2014. [Google Scholar]
- Koussa, F.; Defrance, J.; Jean, P.; Blanc-Benon, P. Acoustical efficiency of a sonic crystal assisted noise barrier. Acta Acust. United Acust. 2013, 99, 399–409. [Google Scholar] [CrossRef]
- Rubio, C.; Candelas, P.; Belmar, F.; Gomez-Lozano, V.; Uris, A. Subwavelength slit acoustic metamaterial barrier. J. Phys. D Appl. Phys. 2015, 48, 395501. [Google Scholar] [CrossRef]
- Lee, H.P.; Lim, K.M. Assessment of the Performance of Sonic Crystal Noise Barriers for the Mitigation of Construction Noise. In INTER-NOISE and NOISE-CON Congress and Conference Proceedings; Institute of Noise Control Engineering: West Lafayette, IN, USA, 2016; Volume 253, pp. 2266–2272. [Google Scholar]
- Aydin, K.; Ozbay, E. Capacitor-loaded split ring resonators as tunable metamaterial components. J. Appl. Phys. 2007, 101, 024911. [Google Scholar] [CrossRef]
- Hu, X.; Chan, C.T.; Zi, J. Two-dimensional sonic crystals with Helmholtz resonators. Phys. Rev. E 2005, 71, 055601. [Google Scholar] [CrossRef]
- Lee, H.M.; Lim, K.M.; Lee, H.P. Environmental and sound divergence effects on the performance of rectangular sonic crystals with Helmholtz resonators. J. Vib. Control 2018, 24, 2483–2493. [Google Scholar] [CrossRef]
- Cavalieri, T.; Cebrecos, A.; Groby, J.P.; Chaufour, C.; Romero-García, V. Three-dimensional multiresonant lossy sonic crystal for broadband acoustic attenuation: Application to train noise reduction. Appl. Acoust. 2019, 146, 1–8. [Google Scholar] [CrossRef]
- Sánchez-Dehesa, J.; Garcia-Chocano, V.M.; Torrent, D.; Cervera, F.; Cabrera, S.; Simon, F. Noise control by sonic crystal barriers made of recycled materials. J. Acoust. Soc. Am. 2011, 129, 1173–1183. [Google Scholar] [CrossRef] [PubMed]
- Umnova, O.; Attenborough, K.; Linton, C.M. Effects of porous covering on sound attenuation by periodic arrays of cylinders. J. Acoust. Soc. Am. 2006, 119, 278–284. [Google Scholar] [CrossRef] [PubMed]
- García-Chocano, V.M.; Sánchez-Dehesa, J. Optimum control of broadband noise by barriers based on sonic crystals. arXiv, 2012; arXiv:1205.3985. [Google Scholar]
- Karimi, M.; Croaker, P.; Kessissoglou, N. Boundary element solution for periodic acoustic problems. J. Sound Vib. 2016, 360, 129–139. [Google Scholar] [CrossRef]
- Thorsson, P.J. Optimisation of low-height noise barriers using the equivalent sources method. Acta Acust. United Acust. 2000, 86, 811–820. [Google Scholar]
- Jolibois, A.; Defrance, J.; Koreneff, H.; Jean, P.; Duhamel, D.; Sparrow, V.W. In situ measurement of the acoustic performance of a full scale tramway low height noise barrier prototype. Appl. Acoust. 2015, 94, 57–68. [Google Scholar] [CrossRef]
- Koussa, F.; Defrance, J.; Jean, P.; Blanc-Benon, P. Transport noise reduction by low height sonic crystal noise barriers. In Proceedings of the Société Française d’Acoustique, Nantes, France, 23 April 2012. [Google Scholar]
- Godinho, L.; Santos, P.G.; Amado-Mendes, P.; Pereira, A.; Martins, M. Experimental and numerical analysis of sustainable sonic crystal barriers based on timber logs. In Proceedings of the EuroRegio2016, Porto, Portugal, 13–15 June 2016. [Google Scholar]
- Amado-Mendes, P.; Godinho, L.; Dias, A.G.; Santos, P.G. On the use of periodic arrays of timber logs as a sustainable noise mitigation solution. In Proceedings of the 22nd International Congress on Sound and Vibration, Florence, Italy, 12–16 July 2015. [Google Scholar]
- Martínez-Sala, R.; Rubio, C.; García-Raffi, L.M.; Sánchez-Pérez, J.V.; Sánchez-Pérez, E.A.; Llinares, J. Control of noise by trees arranged like sonic crystals. J. Sound Vib. 2006, 291, 100–106. [Google Scholar] [CrossRef]
- Gulia, P.; Gupta, A. Traffic Noise Control by Periodically Arranged Trees. In Proceedings of the International Conference on Recent Trends and Developments in Environmental Sustainability RTDES 2016, Chandigarh, India, 16 May 2016. [Google Scholar]
- Liu, Y.F.; Huang, J.K.; Li, Y.G.; Shi, Z.F. Trees as large-scale natural metamaterials for low-frequency vibration reduction. Constr. Build. Mater. 2019, 199, 737–745. [Google Scholar] [CrossRef]
- Lagarrigue, C.; Groby, J.P.; Tournat, V. Sustainable sonic crystal made of resonating bamboo rods. J. Acoust. Soc. Am. 2013, 133, 247–254. [Google Scholar] [CrossRef]
- Yahya, I. Tunable Sonic Crystals Assisted Sound Absorber with a Single and Multi Local Defect. Proc. Eng. 2017, 170, 416–421. [Google Scholar] [CrossRef]
- Kessissoglou, N.; Fard, S.M.B. Sonic crystal noise barrier using locally resonant scatterers. In Proceedings of the 22nd International Congress on Acoustics Buenos Aires, Buenos Aires, Argentina, 5–9 September 2016. [Google Scholar]
- Gulia, P.; Gupta, A. Increasing low frequency sound attenuation using compounded single layer of sonic crystal. In AIP Conference Proceedings; AIP Publishing: Bikaner, India, 2018. [Google Scholar]
- Hoare, S.; Murphy, D. Prediction of scattering effects by sonic crystal noise barriers in 2d and 3d finite difference simulations. In Proceedings of the Société Française d’Acoustique, Nantes, France, 23 April 2012. [Google Scholar]
- Batra, N.K.; Matic, P.; Everett, R.K. Sonic crystal composites for selective noise reduction. In Proceedings of the Ultrasonics Symposium, Munich, Germany, 8–11 October 2002; Volume 1, pp. 547–550. [Google Scholar]
- Zhao, H.; Wang, Y.; Wen, J.; Lam, Y.W.; Umnova, O. A slim subwavelength absorber based on coupled microslits. Appl. Acoust. 2018, 142, 11–17. [Google Scholar] [CrossRef]
- Peiró-Torres, M.; Navarro, M.P.; Bravo, J.M.; Ferri, M.; Sánchez-Pérez, J.V.; Redondo, J. Sonic Crystals acoustic screens with diffusion properties. Proc. Euronoise 2018. Available online: www.euronoise2018.eu/docs/papers/167_Euronoise2018.pdf (accessed on 28 January 2019).
- Castiñeira-Ibáñez, S.; Rubio, C.; Sánchez-Pérez, J.V. Acoustic wave diffraction at the upper edge of a two-dimensional periodic array of finite rigid cylinders. A comprehensive design model of periodicity-based devices. EPL Europhys. Lett. 2013, 101, 64002. [Google Scholar] [CrossRef]
- Morandi, F.; Miniaci, M.; Guidorzi, P.; Marzani, A.; Garai, M. Acoustic measurements on a sonic crystals barrier. Energy Procedia 2015, 78, 134–139. [Google Scholar] [CrossRef]
- Lee, H.M.; Tan, L.B.; Lim, K.M.; Lee, H.P. Sound Quality Experiments in a Student Hostel with Newly Designed Sonic Crystal Window. Acoust. Aust. 2017, 45, 505–514. [Google Scholar] [CrossRef]
- Lee, H.M.; Tan, L.B.; Lim, K.M.; Xie, J.; Lee, H.P. Field Experiment of a Sonic Crystal Window. Fluct. Noise Lett. 2018, 17, 1850032. [Google Scholar] [CrossRef]


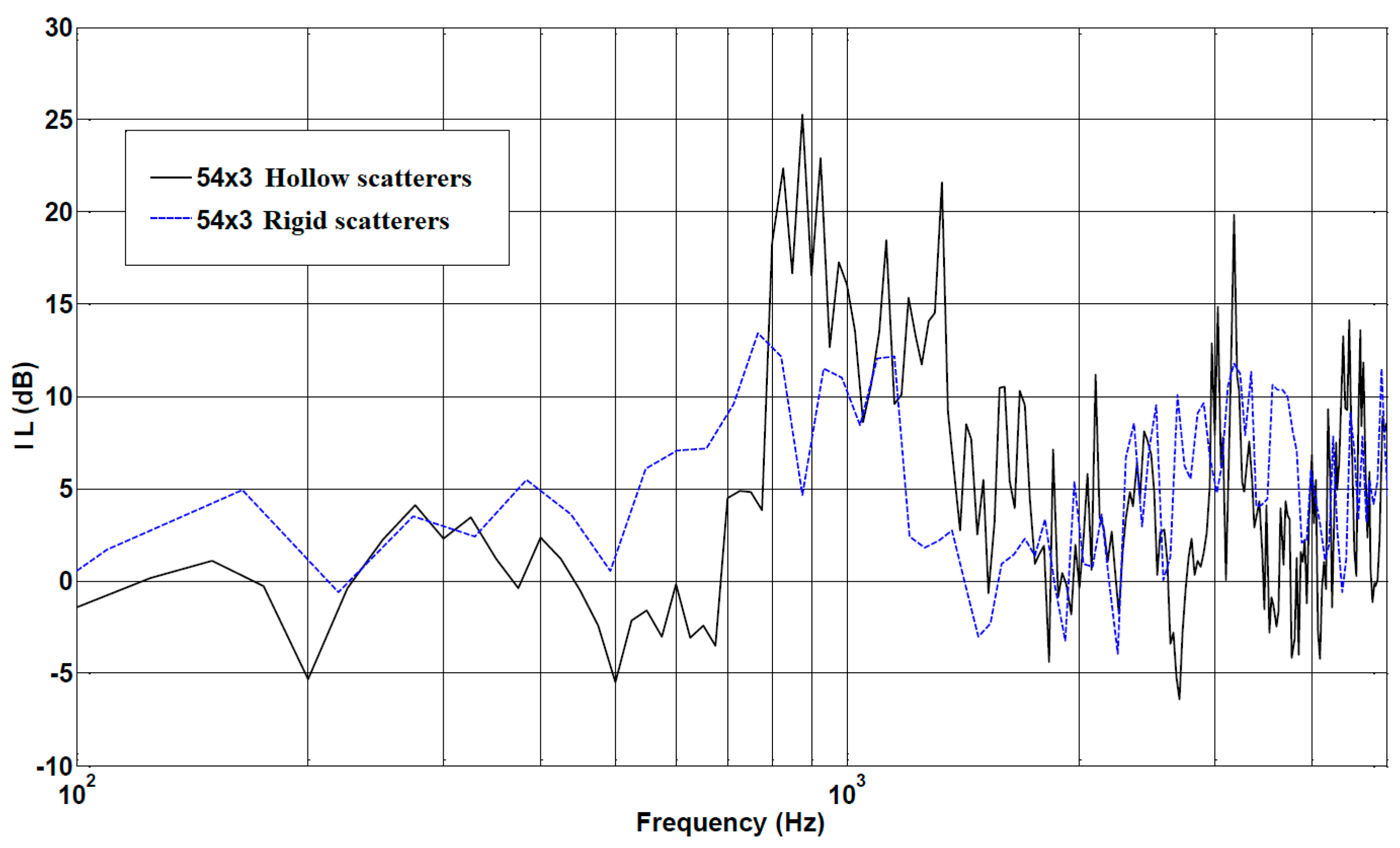
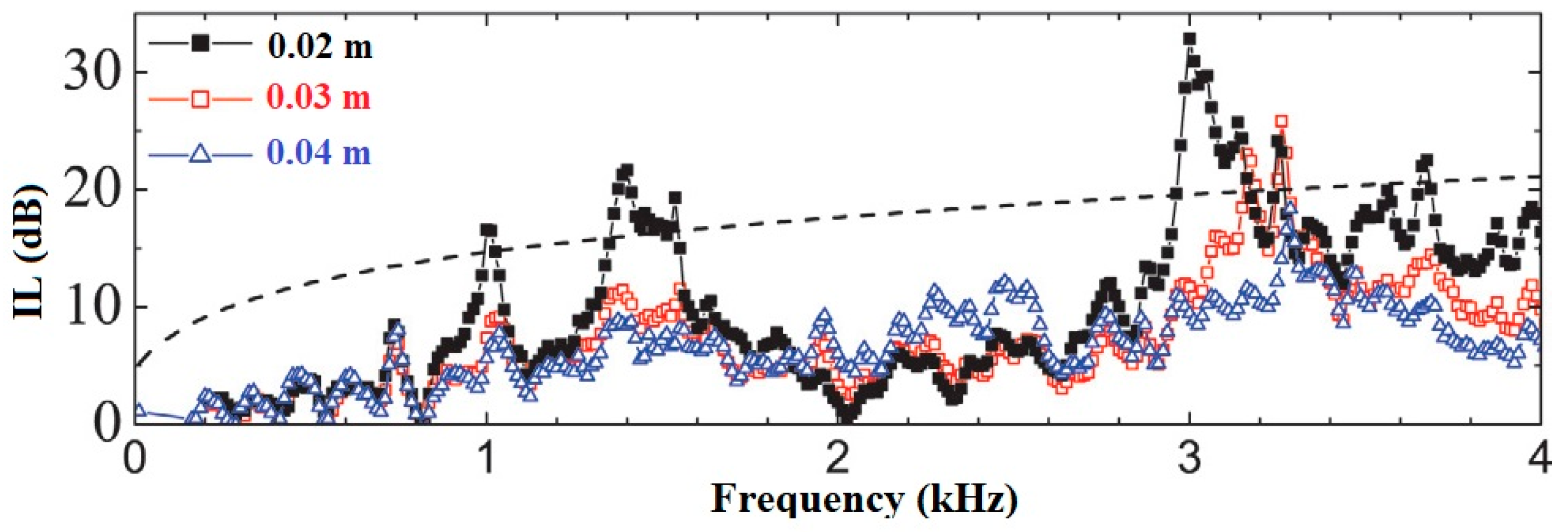
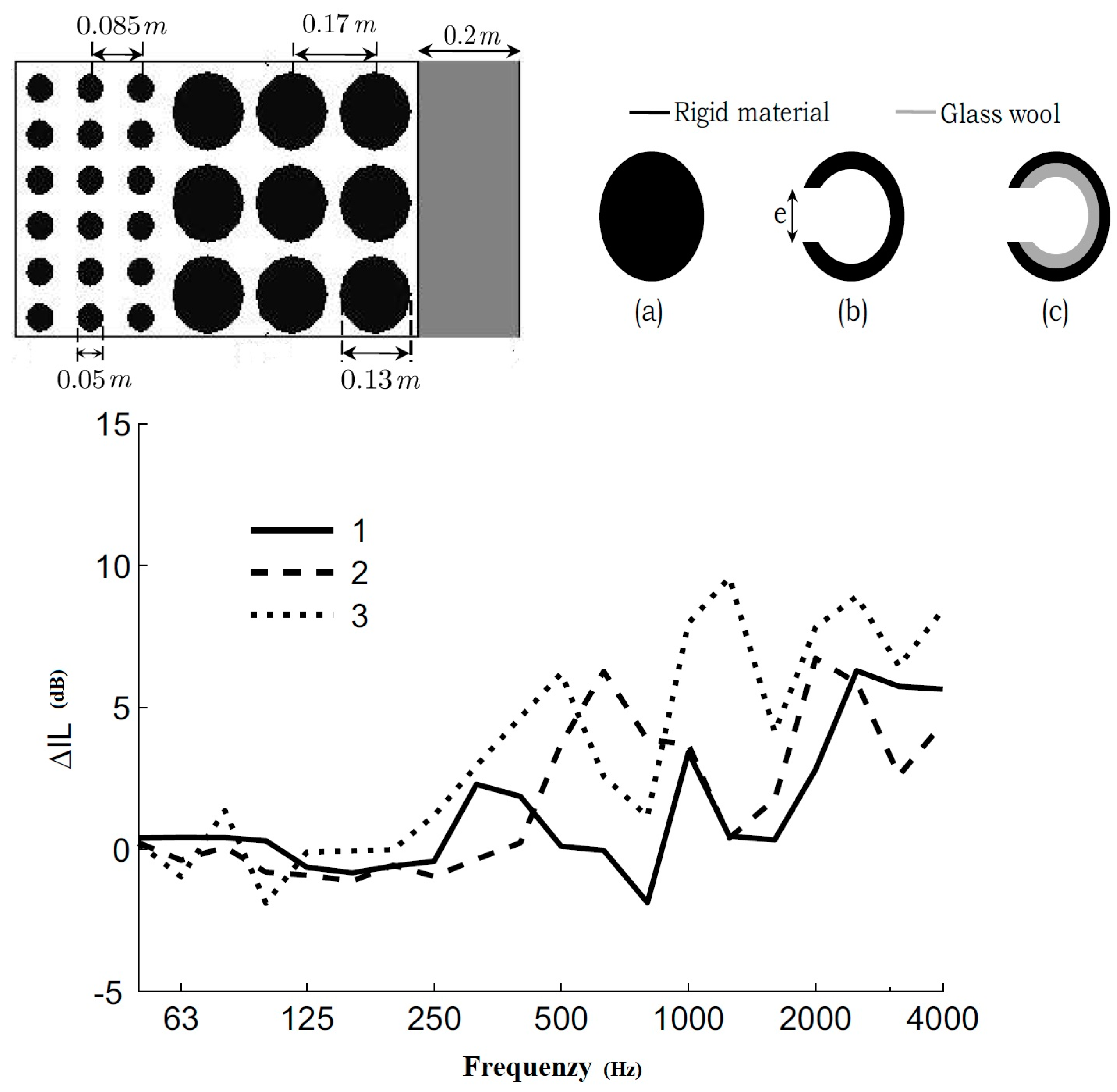

| Authors | IL (dB) | dr (m) | Scatterer’s Shape and Material | D (m) | Lattice’s Shape and Depth (m) | α (m) | Hollow | Porous | Note | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 250 Hz | 500 Hz | 1 kHz | 2 kHz | |||||||||
| Morandi et al. [49] | - | 9 | 15 | 0 | 0.4 | Polyvinyl chloride cylinders | 0.2 | Square 0.8 | 0.2 | No | No | Real dimension |
| Morandi et al. [80] | - | 9 | 15 | 18 | 0.25 | Polyvinyl chloride cylinders | 0.08 | Square 0.96 | 0.2 | Yes | No | Different configurations |
| Martins et al. [35] | - | 5 | 9 | 9 | 1 | Rigid cylinders | 0.2 | Triangular 1.4 | 0.4 | No | Yes outside | Numerical simulations |
| Santos et al. [51] | 0 | 12 | 10 | 10 | 0.65 | Polyvinyl chloride cylinders | 0.2 | 1.4 | 0.2 | No | No | In scale; various shapes |
| Amado-Mendes et al. [38] | 7 | 5 | 15 | - | 0.5 | Wooden cylinders | 0.1 0.2 | Square 1.0 | 0.1 | No | No | Real dimension |
| Jiang et al. [50] | 11 | 2 | 0 | 15 | 0.8 | Steel cylinders | 0.04 | Square 0.75 | 0.08 | No | No | Non-real scale |
| Chong [39] | 3 | 0 | 20 | - | 0.05 | Polyvinyl chloride cylinders | 0.11 | Square 0.7 | 0.16 | Yes | Yes | Non-real scale, resonant cavities |
| Jean and Defrance [36] | 7 | 10 | 9 | 9 | 10 | Wooden cylinders | 0.3 0.7 | Rectangular 2.1 | 0.40 | No | No | Cylinders of 2 different diameters |
| Sánchez-Dehesa et al. [59] | 3 | 5 | 16 | 0 | 1 | Steel cylinders covered by porous material | 0.04 0.08 | Square 0.58 | 0.11 | No | Yes | Porous material outside |
| Koussa et al. [52] | 15 | 20 | 25 | 30 | 0.4 | Aluminum cylinders | 0.05 0.13 | Rectangular, 1st section 0.3 and 2nd section 0.5 | 0.08 0.17 | No | No | 2 noise crystals combined to a conventional noise barrier |
| Koussa et al. [52] | 15 | 23 | 25 | 33 | 0.4 | Aluminum cylinders | 0.05 0.13 | Rectangular, 1st section 0.3 and 2nd section 0.5 | 0.08 0.17 | Yes | No | 2 noise crystals combined to a conventional noise barrier |
| Koussa et al. [52] | 15 | 26 | 30 | 33 | 0.4 | Aluminum cylinders | 0.05 0.13 | Rectangular, 1st section 0.3 and 2nd section 0.5 | 0.08 0.17 | Yes | Rock wool | 2 noise crystals combined to a conventional noise barrier |
| Koussa et al. [65] | 14 | 10 | 13 | 14 | 0.4 | Aluminum cylinders | 0.05 0.13 | Rectangular, 1st section 0.3 and 2nd section 0.5 | 0.08 0.17 | Yes | No | Low-height barrier of 3 different sections |
| Koussa et al. [65] | 15 | 10 | 13 | 14 | 0.4 | Aluminum cylinders | 0.05 0.13 | Rectangular, 1st section 0.3 and 2nd section 0.5 | 0.08 0.17 | Yes | Rock wool inside | Low-height barrier of 3 different sections |
| Lee et al. [57] | 1.5 | 8 | 10 | 3 | 1 | Aluminum parallelepiped | 0.04 | Square 0.37 | 0.1225 | Yes | No | Outdoor measurements, Helmholtz resonator |
| Godinho et al. [66] | 4 | 5 | 15 | 18 | 0.5 | Maritime pine timber logs | 0.1 0.2 | Square 1.0 | 0.1 0.2 | No | No | On field measurements |
| Cavalieri et al. [58] | 2 | 20 | 12 | 18 | 0.45 | Wooden rods of square cross-section | 0.05 | Square 0.3 | 0.05 | Yes | No | Helmholtz resonator |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fredianelli, L.; Del Pizzo, L.G.; Licitra, G. Recent Developments in Sonic Crystals as Barriers for Road Traffic Noise Mitigation. Environments 2019, 6, 14. https://doi.org/10.3390/environments6020014
Fredianelli L, Del Pizzo LG, Licitra G. Recent Developments in Sonic Crystals as Barriers for Road Traffic Noise Mitigation. Environments. 2019; 6(2):14. https://doi.org/10.3390/environments6020014
Chicago/Turabian StyleFredianelli, Luca, Lara Ginevra Del Pizzo, and Gaetano Licitra. 2019. "Recent Developments in Sonic Crystals as Barriers for Road Traffic Noise Mitigation" Environments 6, no. 2: 14. https://doi.org/10.3390/environments6020014
APA StyleFredianelli, L., Del Pizzo, L. G., & Licitra, G. (2019). Recent Developments in Sonic Crystals as Barriers for Road Traffic Noise Mitigation. Environments, 6(2), 14. https://doi.org/10.3390/environments6020014








