Energy-Harvesting Characteristics of a Dual-Mode Magnetic Suspension for Vehicles: Analysis and Experimental Verification
Abstract
1. Introduction
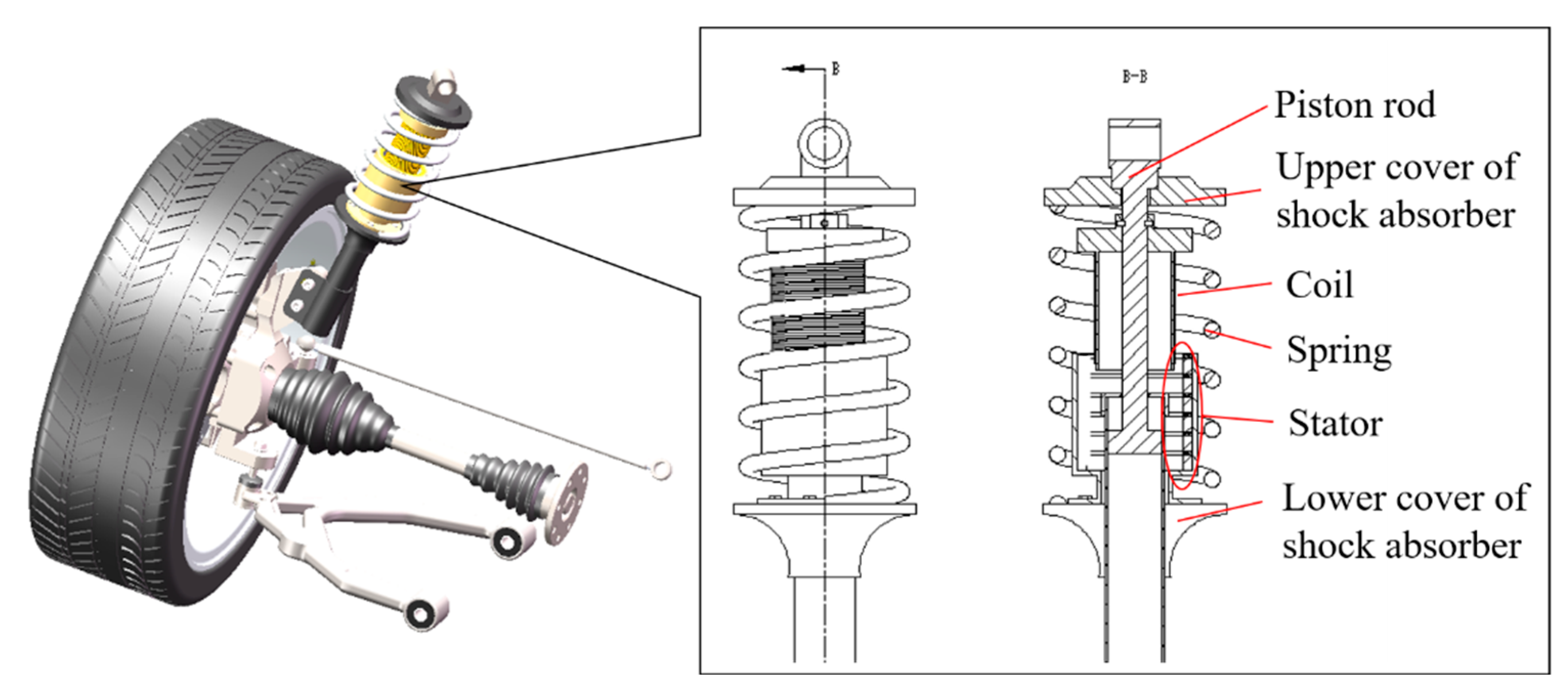
2. Structure and Working Principle of the Dual-Mode Magnetic Suspension
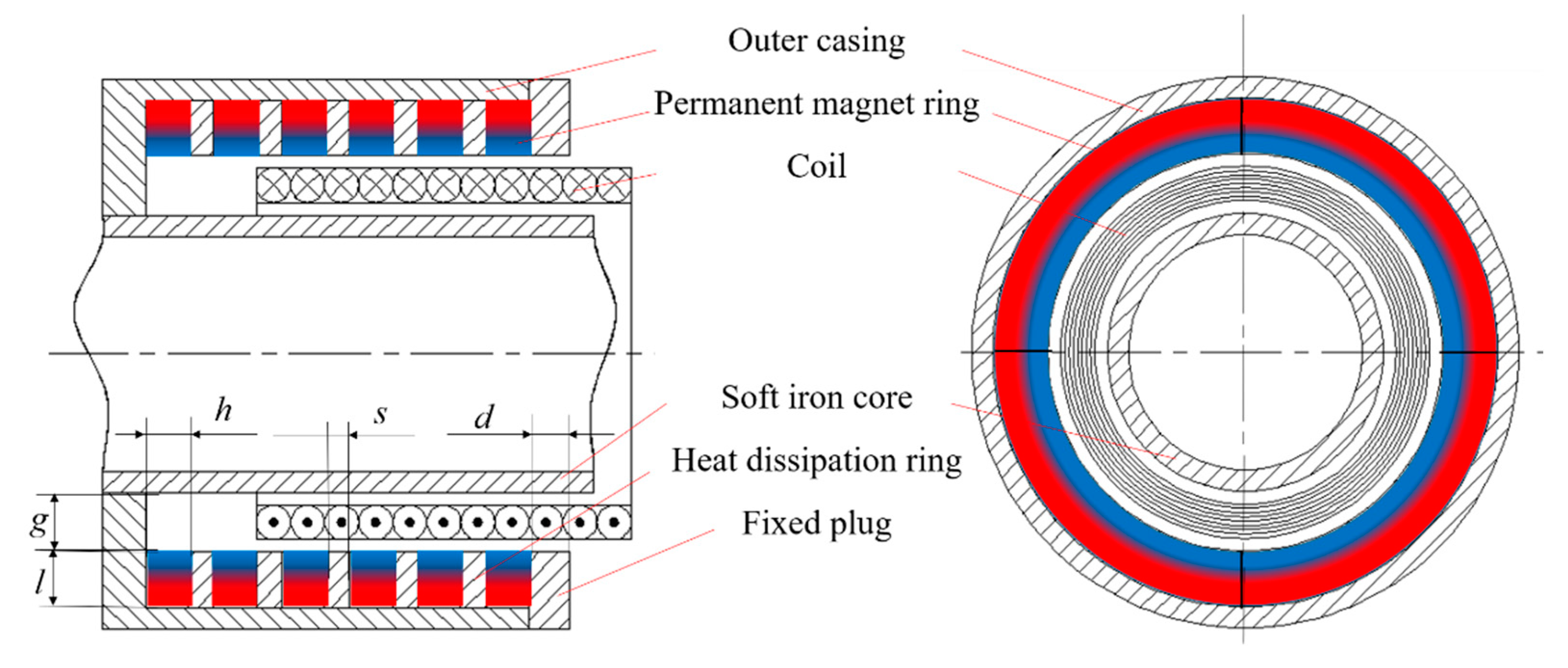
2.1. Structure
2.2. Working Principle
3. Analysis of the Effects of the Stator on the Energy-Harvesting Characteristics
3.1. Effects of the Stator Parameters on the Energy-Harvesting Characteristics
3.1.1. Effect of the Permanent Magnet Ring on the Energy Harvesting Characteristic
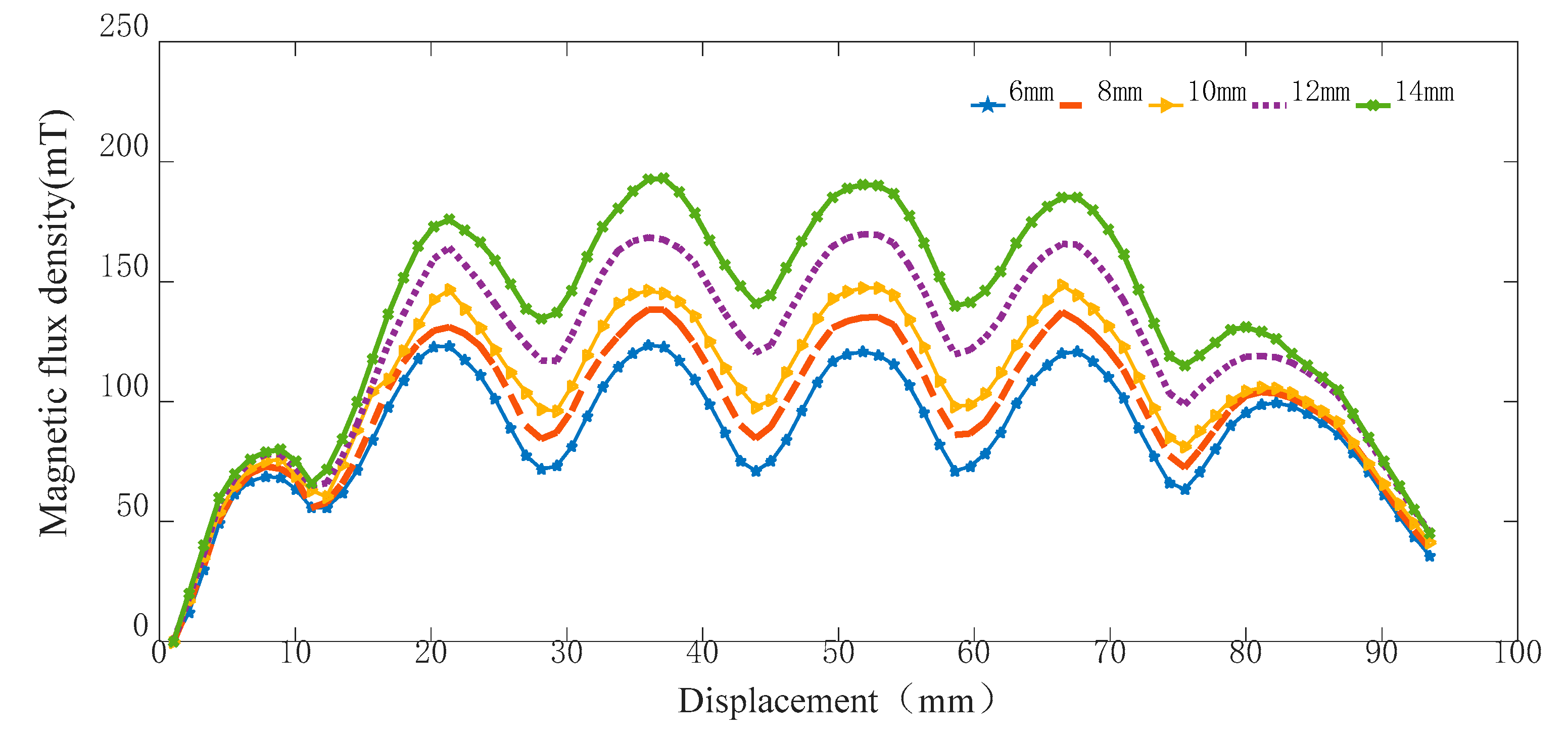
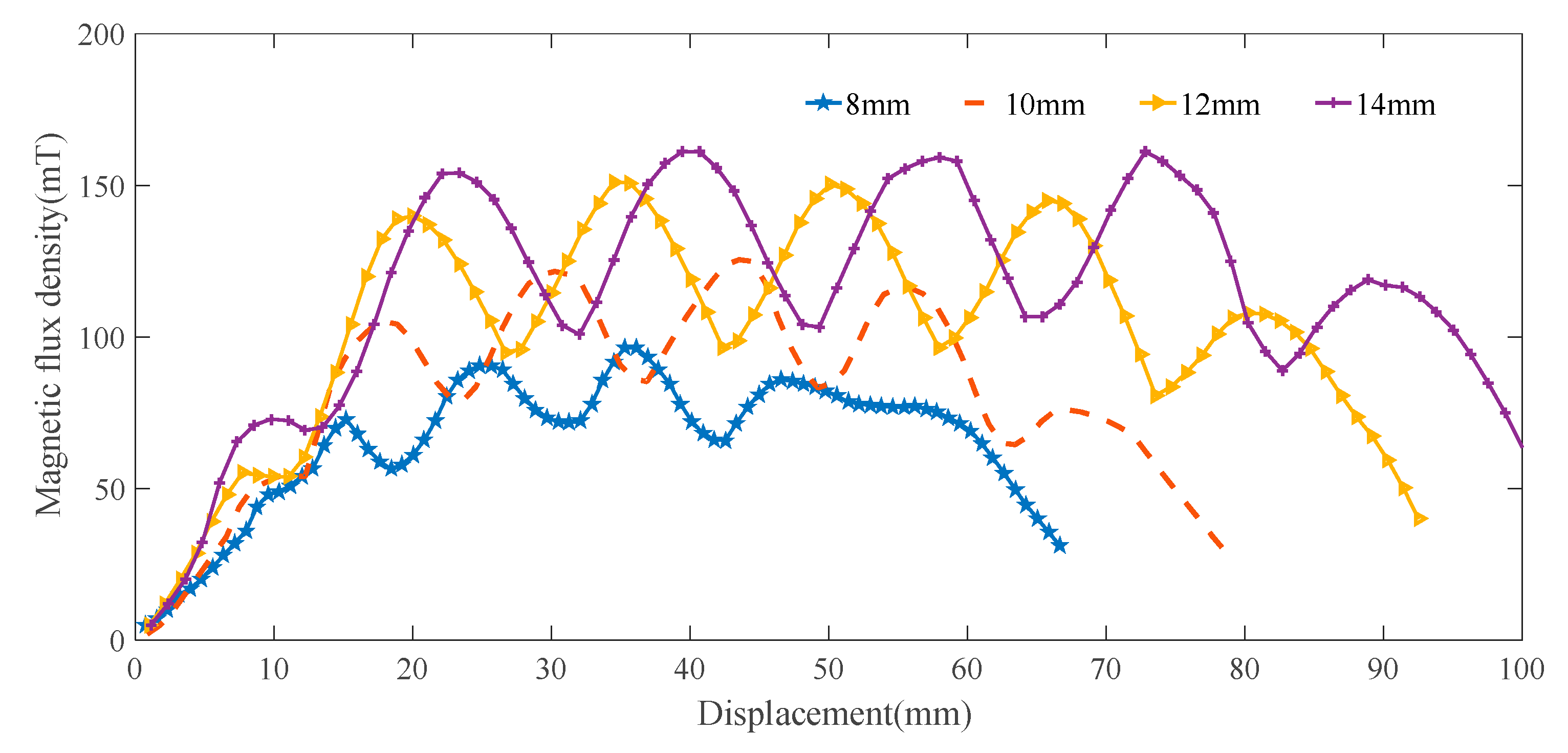
3.1.2. Effect of the Fixed Plug’s Thickness on the Energy-Harvesting Characteristic
3.1.3. Effect of the Heat Dissipation Ring’s Thickness on the Energy Harvesting Characteristic
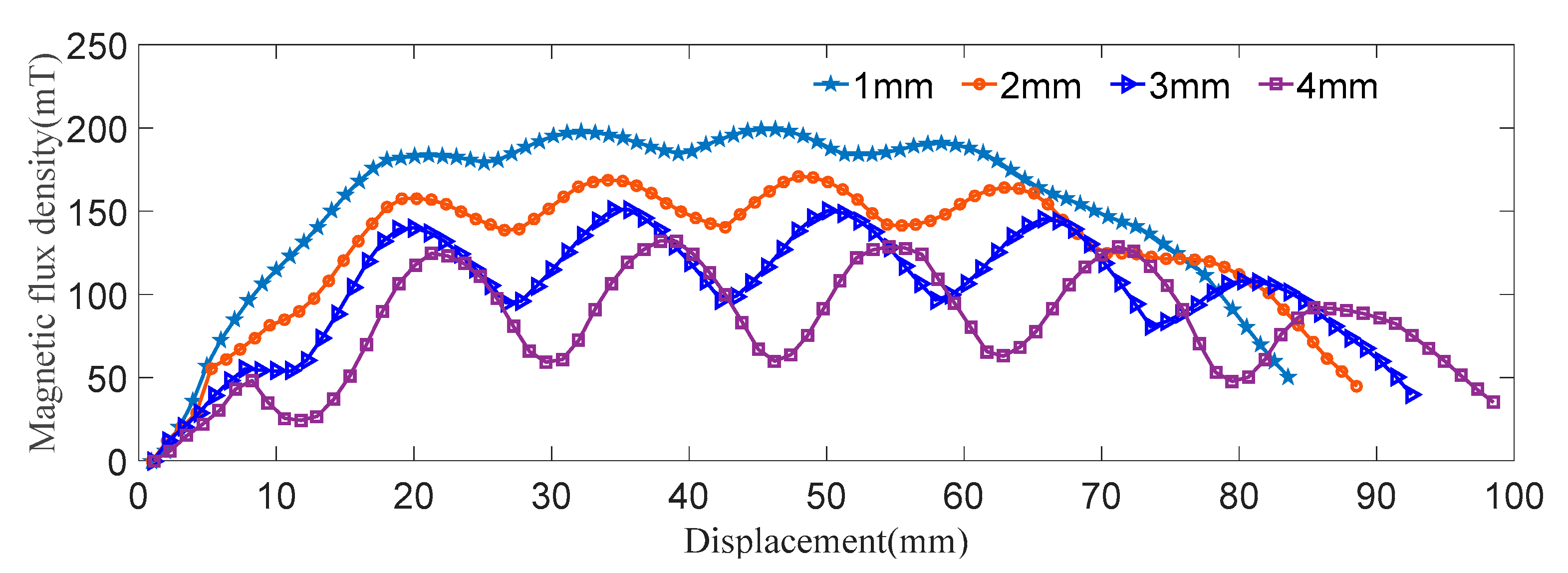
3.1.4. Effect of the Air Gap on the Energy Harvesting Characteristic
3.2. Orthogonal Analysis of the Stator Parameters
3.2.1. Orthogonal Design
3.2.2. Orthogonal Analysis
4. Simulation Results of the Dual-Mode Magnetic Suspension
4.1. Theoretical Model of the EMF
4.2. Simulation Analysis of the EMF
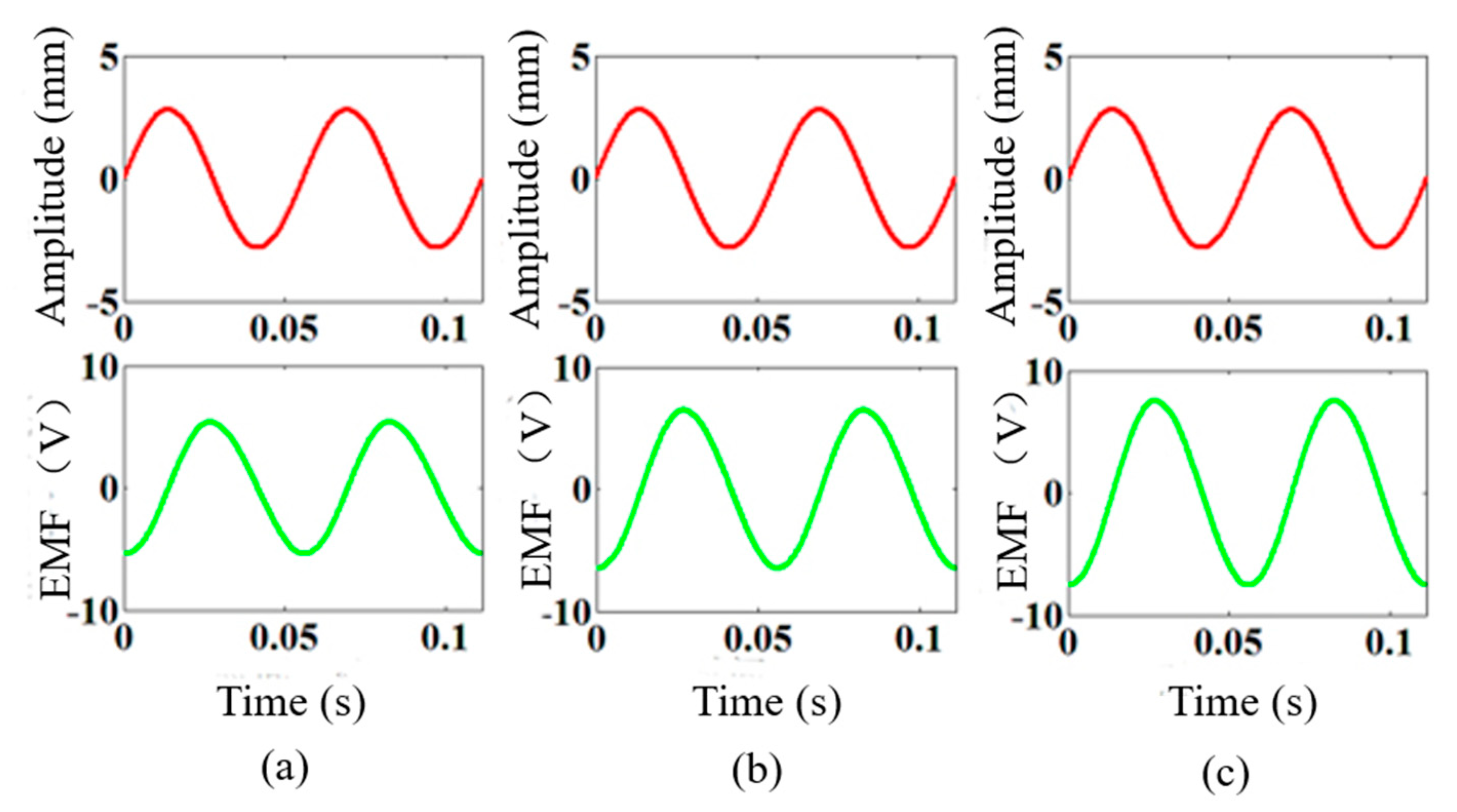
4.2.1. Effect of the Number of Coil Turns on the EMF
4.2.2. Effect of the Excitation Amplitude on the EMF
4.2.3. Effect of the Excitation Frequency on the EMF
5. Experiment Verification
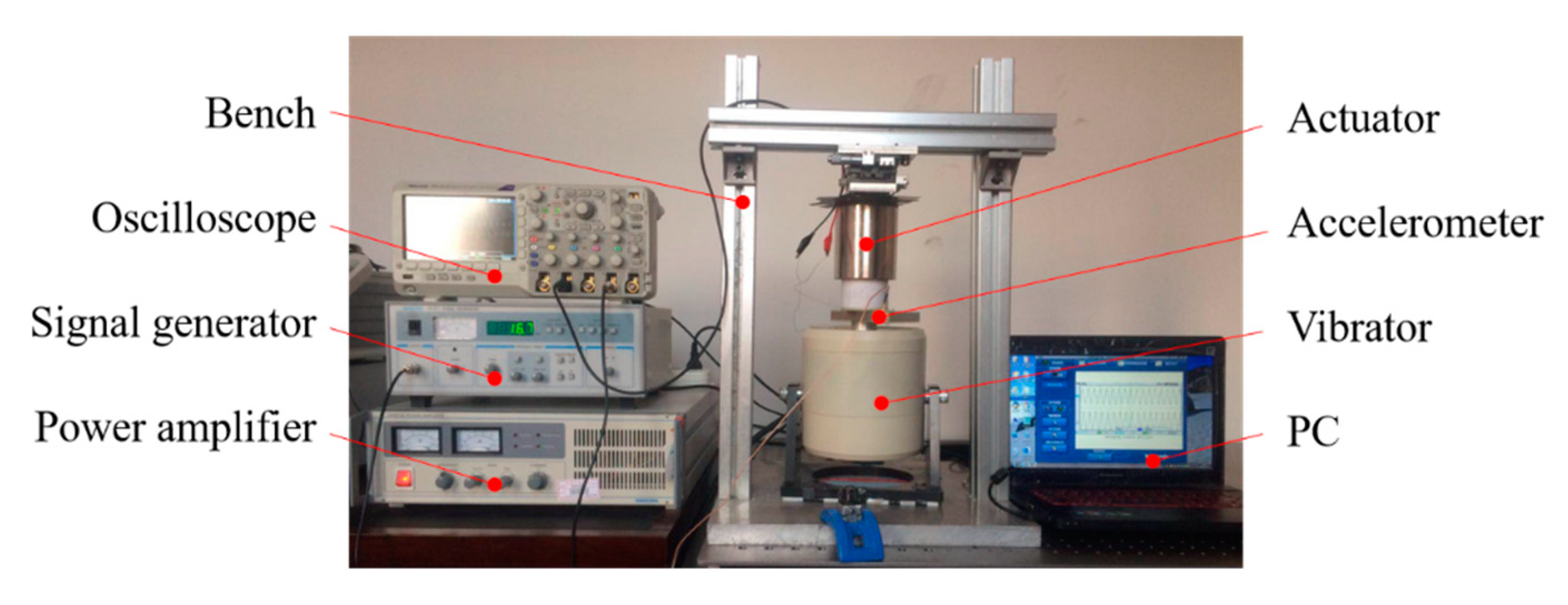
5.1. Experimental Setup
5.2. Experimental Results
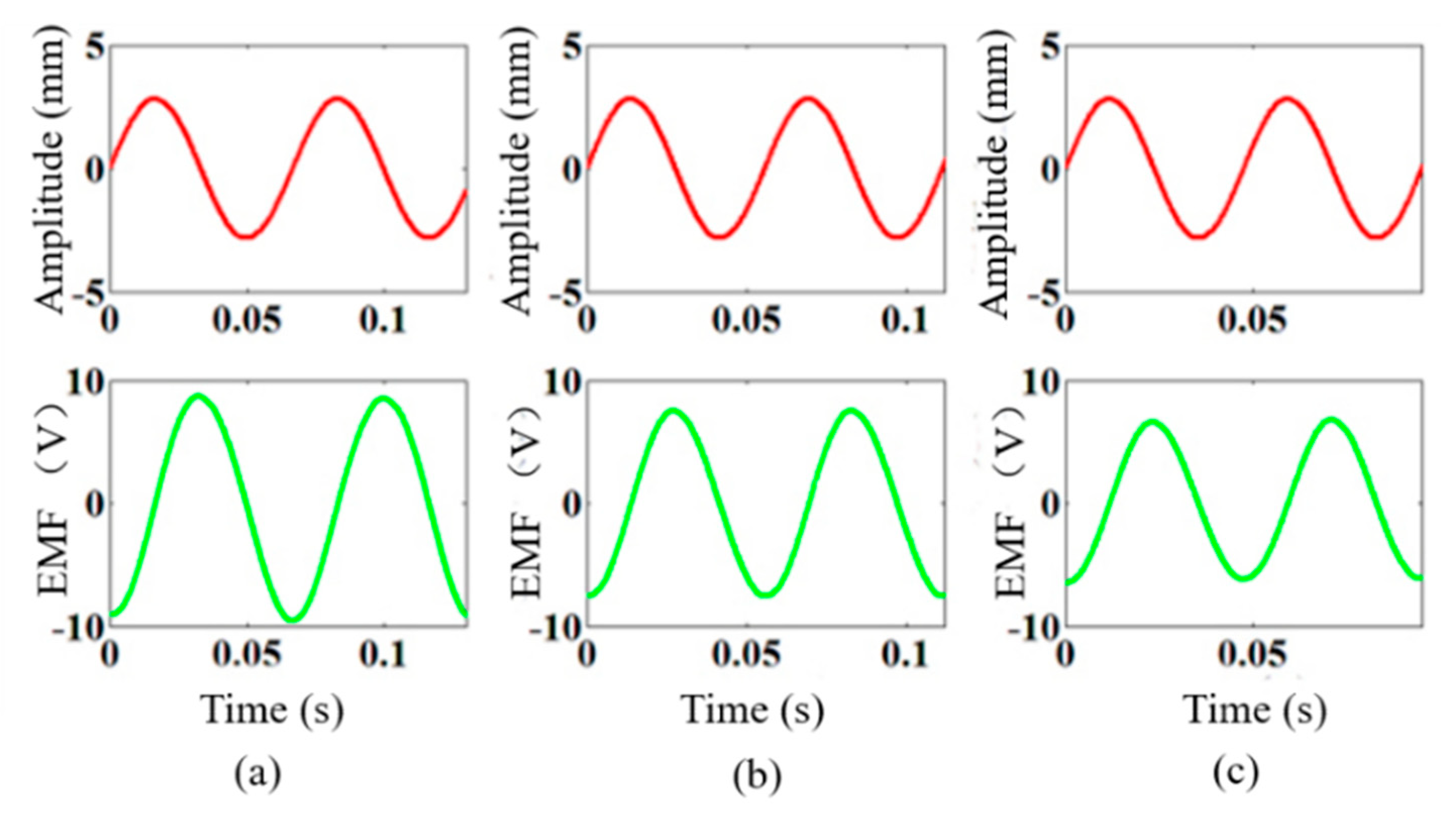
5.2.1. Experimental Results for the Effect of the Number of Coil Turns on the EMF
5.2.2. Experimental Results for the Effect of the Excitation Amplitude on the EMF
5.2.3. Experimental Results of the Effect of the Excitation Frequency on the EMF
6. Conclusions
- (1)
- The number of coil turns have the most significant effect on the energy-harvesting characteristics, with a rate of change up to 40.9%, which is followed by the amplitude of the excitation signal, up to 18.1%, and finally, the frequency of the excitation signal.
- (2)
- With the increase in the number of coil turns and the excitation amplitude, the output-induced EMF of the suspension is increased.
- (3)
- When the excitation frequency is equal to the resonance frequency, the vehicle body resonates with the excitation signal, and the induced EMF is large. Additionally, when the coil enters the air gap of 70 mm (the number of coil turns is 1319), the excitation amplitude is 3.1 mm, and the frequency is 18 Hz, the maximum induced EMF is 8.8 V.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Long, G.M.; Ding, F.; Zhang, N.; Zhang, J.; Qin, A. Regenerative active suspension system with residual energy for in-wheel motor driven electric vehicle. Appl. Energy 2020, 260, 114180. [Google Scholar] [CrossRef]
- Gu, C.; Yin, J.; Luo, J.; Chen, X.B.; Wang, J.M. Performance-oriented controls of a novel rocker-pushrod electromagnetic active vehicle suspension. Mech. Syst. Signal Process. 2018, 109, 1–14. [Google Scholar] [CrossRef]
- Zou, J.Y.; Guo, X.X.; Abdelkareem, M.A.A.; Xu, L.; Zhang, J. Modelling and ride analysis of a hydraulic interconnected suspension based on the hydraulic energy regenerative shock absorbers. Mech. Syst. Signal Process. 2019, 127, 345–369. [Google Scholar] [CrossRef]
- Zheng, P.; Gao, J.W. Damping force and energy recovery analysis of regenerative hydraulic electric suspension system under road excitation: Modelling and numerical simulation. Math. Biosci. Eng. 2019, 16, 6298–6318. [Google Scholar] [CrossRef]
- Li, S.Y.; Xu, J.; Pu, X.H.; Tao, T.; Gao, H.N.; Mei, X.S. Energy-harvesting variable/constant damping suspension system with motor based electromagnetic damper. Energy 2019, 189, 116199. [Google Scholar] [CrossRef]
- Wei, W.; Sun, F.; Jin, J.Q.; Zhao, Z.Y.; Miao, L.G.; Li, Q.; Zhang, X.Y. Proposal of energy-recycle type active suspension using magnetic force. Int. J. Appl. Electromagn. Mech. 2019, 59, 577–585. [Google Scholar] [CrossRef]
- Khoshnoud, F.; Zhang, Y.C.; Shimura, R.; Shahba, A.; Jin, G.M.; Pissanidis, G.; Chen, Y.K.; De Silva, C.W. Energy regeneration from suspension dynamic modes and self-powered actuation. IEEE/ASME Trans. Mechatron. 2015, 20, 2513–2524. [Google Scholar] [CrossRef]
- Nakano, K.; Suda, Y.; Nakadai, S. Self-powered active vibration control using a single electric actuator. J. Sound Vib. 2003, 260, 213–235. [Google Scholar] [CrossRef]
- Zhang, J.M.; Liu, J.; Liu, B.L.; Li, M. Fractional order PID control based on ball screw energy regenerative active suspension. Actuators 2022, 11, 189. [Google Scholar] [CrossRef]
- Florean-Aquino, K.H.; Arias-Montiel, M.; Linares-Flores, J.; Mendoza-Larios, J.G.; Cabrera-Amado, A. Modern semi-active control schemes for a suspension with MR actuator for vibration attenuation. Actuators 2022, 10, 22. [Google Scholar] [CrossRef]
- Yang, X.F.; Zhao, W.T.; Liu, Y.L.; Chen, L.; Meng, X.P. Design and experimental study of the energy-regenerative circuit of a hybrid vehicle suspension. Sci. Prog. 2020, 103, 0036850419874999. [Google Scholar] [CrossRef]
- Kim, J.H.; Shin, Y.J.; Chun, Y.D.; Kim, J.H. Design of 100W regenerative vehicle suspension to harvest energy from road surfaces. Int. J. Precis. Eng. Manuf. 2018, 19, 1089–1096. [Google Scholar] [CrossRef]
- Arroyo, E.; Badel, A.; Formosa, F. Energy harvesting from ambient vibrations: Electromagnetic device and synchronous extraction circuit. J. Intell. Mater. Syst. Struct. 2013, 24, 2023–2035. [Google Scholar] [CrossRef]
- Sapinski, B. Energy-harvesting linear MR damper: Prototyping and testing. Smart Mater. Struct. 2014, 23, 035021. [Google Scholar] [CrossRef]
- Sapinski, B.; Rosol, M.; Wegrzynowski, M. Investigation of an energy harvesting MR damper in a vibration control system. Smart Mater. Struct. 2016, 25, 125017. [Google Scholar] [CrossRef]
- Zhu, X.; Deng, L.; Sun, S.; Yan, T.; Yu, J.; Ma, Z.; Li, W. Development of a variable stiffness magnetorheological damper with self-powered generation capability. J. Intell. Mater. Syst. Struct. 2020, 31, 209–219. [Google Scholar] [CrossRef]
- Firoozy, P.; Ebrahimi-Nejad, S. Broadband energy harvesting from time-delayed nonlinear oscillations of magnetic levitation. J. Intell. Mater. Syst. Struct. 2020, 31, 737–755. [Google Scholar] [CrossRef]
- Zou, J.Y.; Guo, X.X.; Xu, L.; Tan, G.F.; Zhang, C.C.; Zhang, J. Design, modeling, and analysis of a novel hydraulic energy-regenerative shock absorber for vehicle suspension. Shock Vib. 2017, 2017, 3186584. [Google Scholar] [CrossRef]
- Wei, W.; Li, Q.; Xu, F.; Zhang, X.; Jin, J.; Jin, J.; Sun, F. Research on an electromagnetic actuator for vibration suppression and energy regeneration. Actuators 2020, 9, 42. [Google Scholar] [CrossRef]
- Beltran-Carbajal, F.; Valderrabano-Gonzalez, A.; Favela-Contreras, A.; Hernandez-Avila, J.L.; Lopez-Garcia, I.; Tapia-Olvera, R. An active vehicle suspension control approach with electromagnetic and hydraulic actuators. Actuators 2019, 8, 35. [Google Scholar] [CrossRef]
- Wu, H.C.; Zhou, J.; Xie, C.H.; Zhang, J.Y.; Huang, Y.M. Two-dimensional time series sample entropy algorithm: Applications to rotor axis orbit feature identification. Mech. Syst. Signal Process. 2021, 147, 107123. [Google Scholar]
- Zhou, J.; Wu, H.C.; Wang, W.Y.; Yang, K.Z.; Hu, Y.F.; Guo, X.H.; Song, C.S. Online unbalance compensation of a maglev rotor with two active magnetic bearings based on the LMS algorithm and the influence coefficient method. Mech. Syst. Signal Process. 2022, 166, 108460. [Google Scholar]
- Zhou, R.; Yan, M.; Sun, F.; Jin, J.; Li, Q.; Xu, F.; Zhang, M.; Zhang, X.; Nakano, K. Experimental validations of a magnetic energy-harvesting suspension and its potential application for self-powered sensing. Energy 2022, 239, 122205. [Google Scholar] [CrossRef]
- Zhou, R.; Sun, F.; Yan, M.; Jin, J.; Li, Q.; Xu, F.; Zhang, X.; Nakano, K. Design, analysis and prototyping of a magnetic energy-harvesting suspension for vehicles. Smart Mater. Struct. 2020, 29, 105034. [Google Scholar] [CrossRef]
- Li, Q.; Peng, Z.; Jiang, W.; Ouyang, L.; Wang, H.; Liu, J.; Zhu, M. Optimization of Ti-Zr-Cr-Fe alloys for 45 MPa metal hydride hydrogen compressors using orthogonal analysis. J. Alloys Compd. 2021, 889, 161629. [Google Scholar] [CrossRef]
- Xie, C.; Zhang, T.; Yuan, Z.; Feng, A.; Wu, L. Optimization design and internal flow analysis of prefabricated barrel in centrifugal prefabricated pumping station with double pumps. Processes 2022, 10, 1877. [Google Scholar] [CrossRef]








| Level | Magnetic Ring Radial Thickness (mm) A | Magnetic Ring Axial Height (mm) B | Heat Dissipation Ring Thickness (mm) C | Air Gap (mm) D |
|---|---|---|---|---|
| 1 | 8 | 8 | 1 | 9 |
| 2 | 10 | 10 | 2 | 10 |
| 3 | 12 | 12 | 3 | 11 |
| 4 | 14 | 14 | 4 | 12 |
| Numbering | Magnetic Ring Radial Thickness (mm) | Magnetic Ring Axial Height (mm) | Heat Dissipation Ring Thickness (mm) | Air Gap (N) | Magnetic Flux Density (mT) |
|---|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 1 | 163.625 |
| 2 | 1 | 2 | 2 | 2 | 165.926 |
| 3 | 1 | 3 | 3 | 3 | 169.317 |
| 4 | 1 | 4 | 4 | 4 | 176.305 |
| 5 | 2 | 1 | 2 | 3 | 178.128 |
| 6 | 2 | 2 | 1 | 4 | 186.419 |
| 7 | 2 | 3 | 3 | 2 | 184.526 |
| 8 | 2 | 4 | 4 | 1 | 188.513 |
| 9 | 3 | 1 | 3 | 4 | 183.614 |
| 10 | 3 | 2 | 4 | 3 | 186.325 |
| 11 | 3 | 1 | 1 | 2 | 188.146 |
| 12 | 3 | 4 | 2 | 1 | 190.182 |
| 13 | 4 | 3 | 4 | 2 | 189.379 |
| 14 | 4 | 2 | 3 | 4 | 192.353 |
| 15 | 4 | 3 | 2 | 1 | 194.476 |
| 16 | 4 | 4 | 1 | 3 | 186.479 |
| Name | Magnetic Ring Radial Thickness (mm) A | Magnetic Ring Axial Height (mm) B | Heat Dissipation Ring Thickness (mm) C | Air Gap (mm) D |
|---|---|---|---|---|
| Kj1 | 675.173 | 713.112 | 724.669 | 734.373 |
| Kj2 | 747.412 | 731.023 | 718.726 | 737.927 |
| Kj3 | 748.268 | 737.698 | 739.760 | 720.249 |
| Kj4 | 752.736 | 741.479 | 740.522 | 730.864 |
| Kjp1 | 168.775 | 178.278 | 181.167 | 183.668 |
| Kjp2 | 186.853 | 189.756 | 179.691 | 184.482 |
| Kjp3 | 187.067 | 184.424 | 184.940 | 180.062 |
| Kjp4 | 188.184 | 185.370 | 185.130 | 182.716 |
| Rj | 19.409 | 11.478 | 5.439 | 3.606 |
| Influencing factors | A > B > C > D | |||
| Best level | A4 | B3 | C2 | D1 |
| Best combination | A4 B3 C2 D1 | |||
| Description | Symbol | Numerical Value |
|---|---|---|
| Permanent magnet ring length/mm | h | 10 |
| Permanent magnet ring height/mm | l | 12.5 |
| Cooling ring height/mm | s | 3 |
| Outer fixed plug wall thickness/mm | d | 10 |
| Air gap length/mm | Xg | 90 |
| Air gap width/mm | Yg | 10 |
| NdFeB N42H remanence/T | Br | 1.18 |
| NdFeB N42H coercivity/A/m | Hc | −880,000 |
| Air permeability/H/m | μ0 | 4 π × 10−7 |
| Description | Representation Symbol | Numerical Value |
|---|---|---|
| Average diameter of the winding | Dc | 0.0635 m |
| Resistivity of copper | ρ | 1.75 × 10−8 Ω·m |
| Coil skeleton length | L | 0.09 m |
| Coil resistance | R | 62 Ω |
| Magnetic flux density | B | 0.09 T |
| Coil turns in the air gap | N1 | 1696 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, W.; Song, Y.; Xu, Y.; Zhou, R.; Sun, F.; Zhang, X. Energy-Harvesting Characteristics of a Dual-Mode Magnetic Suspension for Vehicles: Analysis and Experimental Verification. Actuators 2022, 11, 363. https://doi.org/10.3390/act11120363
Jiang W, Song Y, Xu Y, Zhou R, Sun F, Zhang X. Energy-Harvesting Characteristics of a Dual-Mode Magnetic Suspension for Vehicles: Analysis and Experimental Verification. Actuators. 2022; 11(12):363. https://doi.org/10.3390/act11120363
Chicago/Turabian StyleJiang, Weikang, Yuanyuan Song, Yongming Xu, Ran Zhou, Feng Sun, and Xiaoyou Zhang. 2022. "Energy-Harvesting Characteristics of a Dual-Mode Magnetic Suspension for Vehicles: Analysis and Experimental Verification" Actuators 11, no. 12: 363. https://doi.org/10.3390/act11120363
APA StyleJiang, W., Song, Y., Xu, Y., Zhou, R., Sun, F., & Zhang, X. (2022). Energy-Harvesting Characteristics of a Dual-Mode Magnetic Suspension for Vehicles: Analysis and Experimental Verification. Actuators, 11(12), 363. https://doi.org/10.3390/act11120363








