Preliminary Study on the Damping Effect of a Rotational Inertia Particle Damper Considering the Explosion Response of Continuous Concrete Bridges
Abstract
1. Introduction
2. Experiment Design
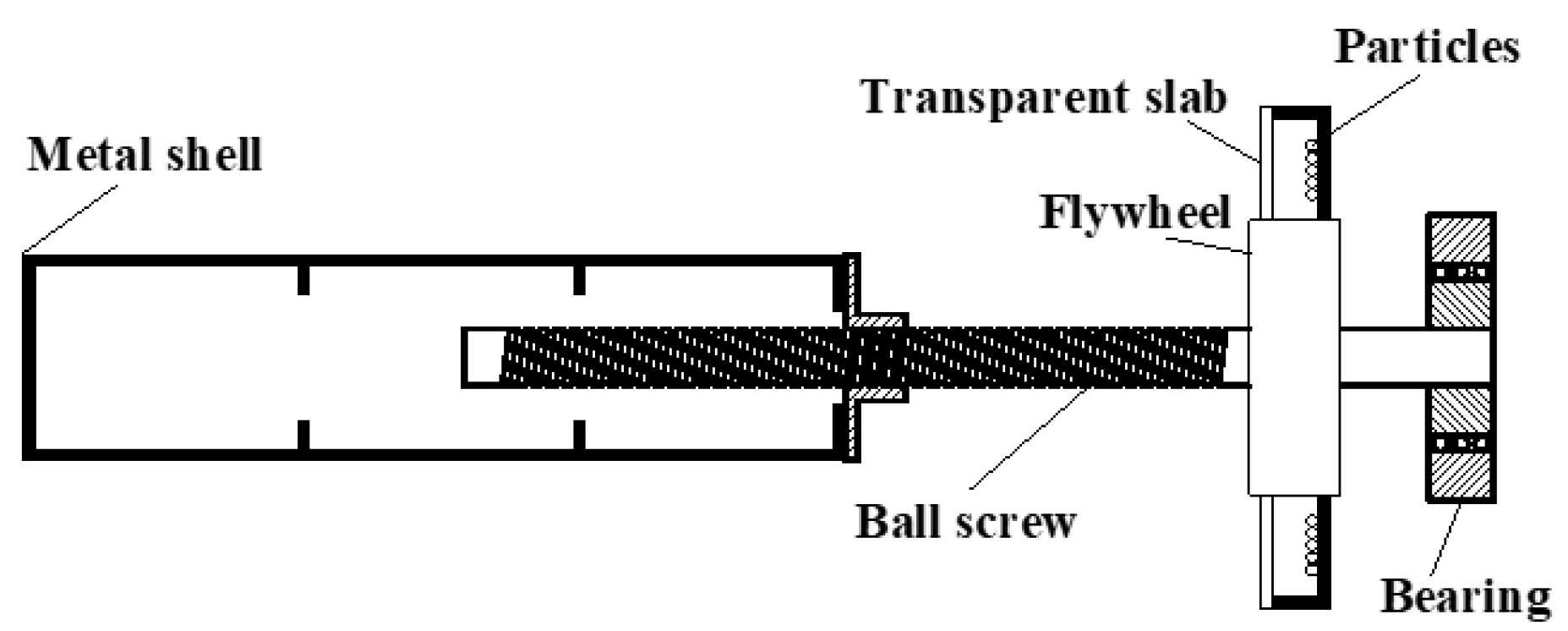
2.1. Rotational Inertia Particle Damper
2.1.1. Operating Principle of R-IPD
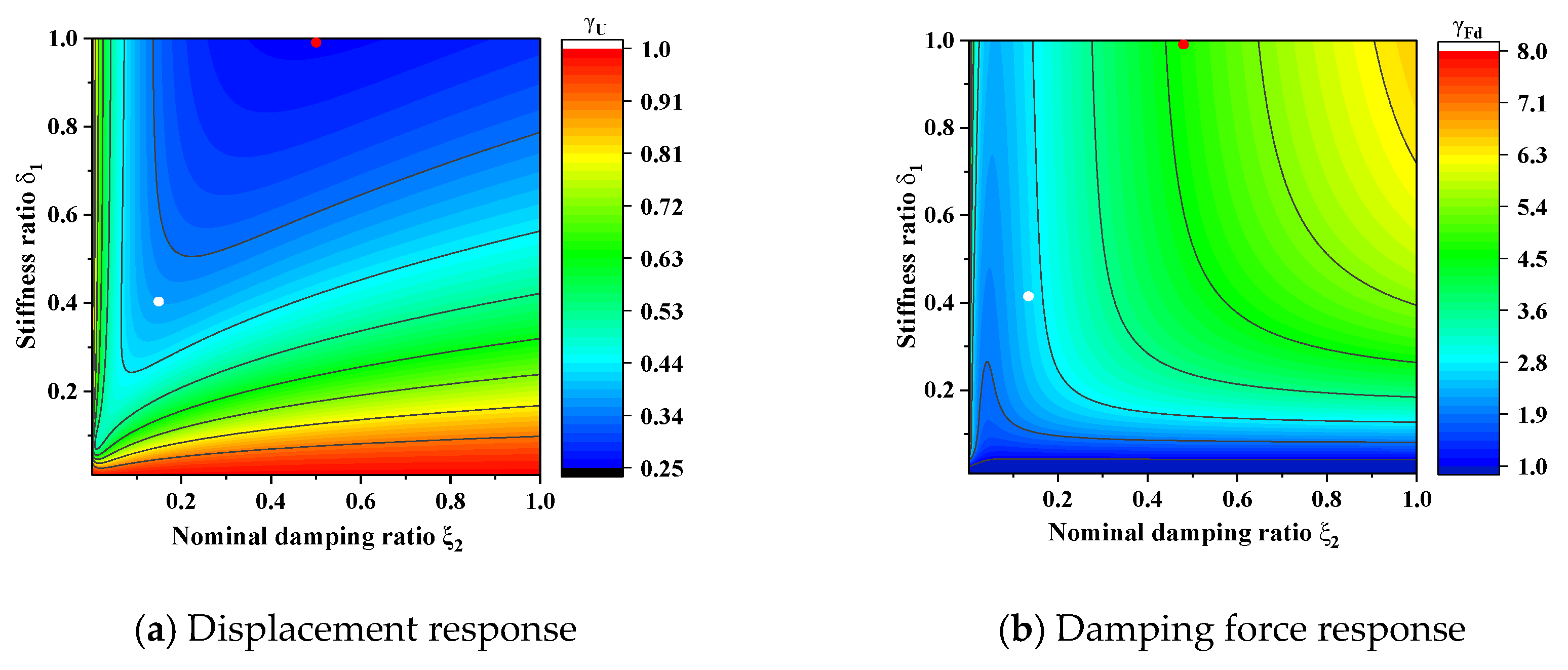
2.1.2. Parameter Optimisation Method
2.2. Model Bridge Design
2.3. R-IPD Design
2.4. Arrangement of Measurement Points
2.5. Test Cases
3. Experimental Results without R-IPDs
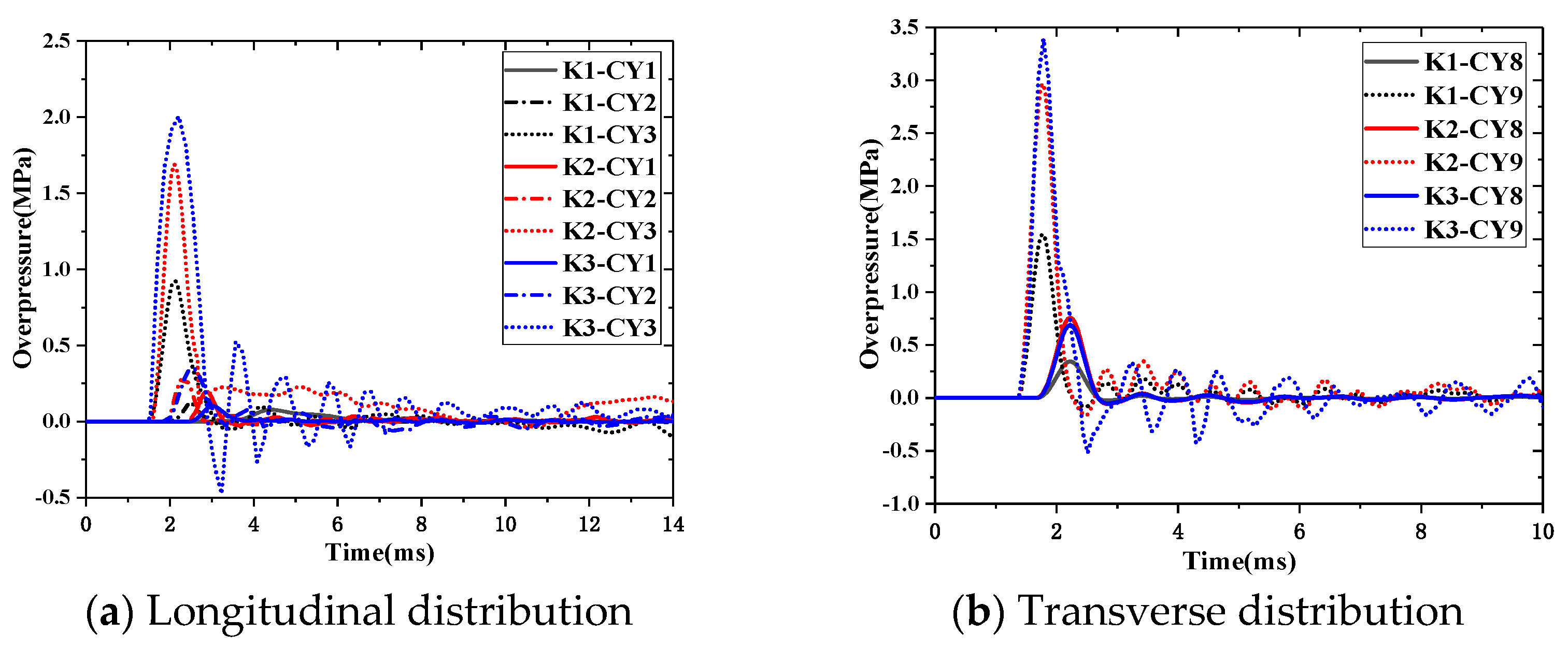
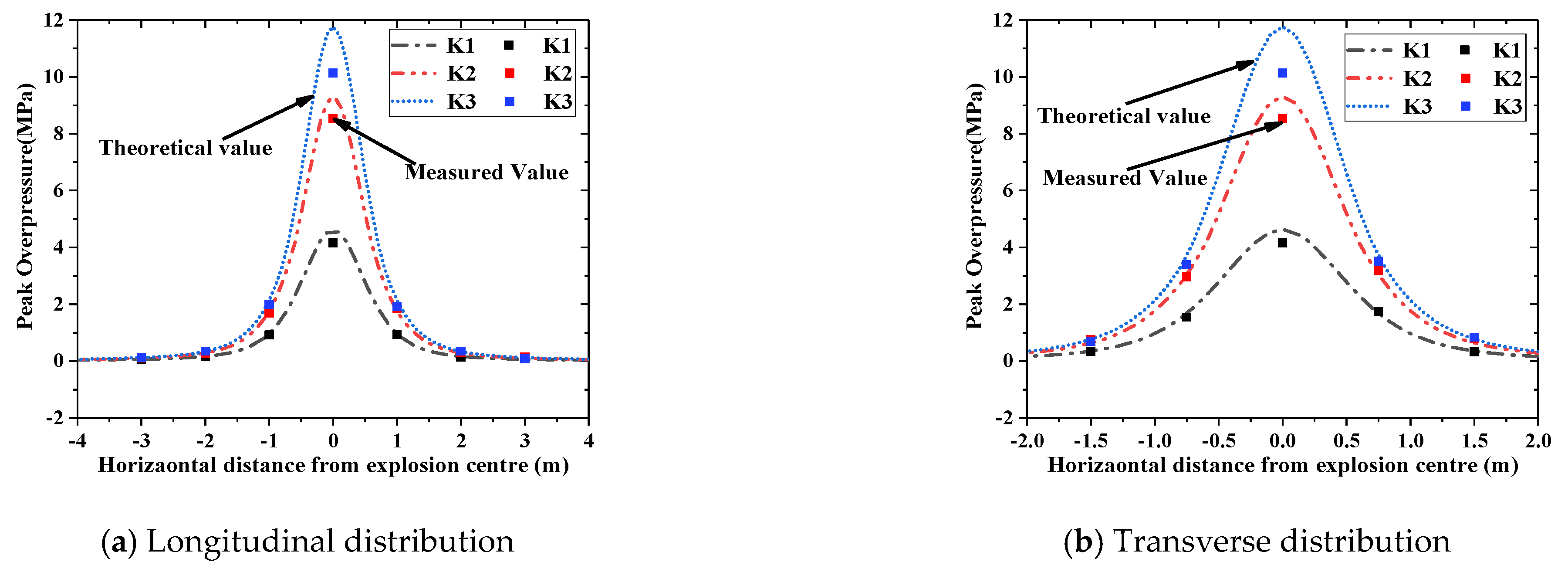
3.1. Overpressure
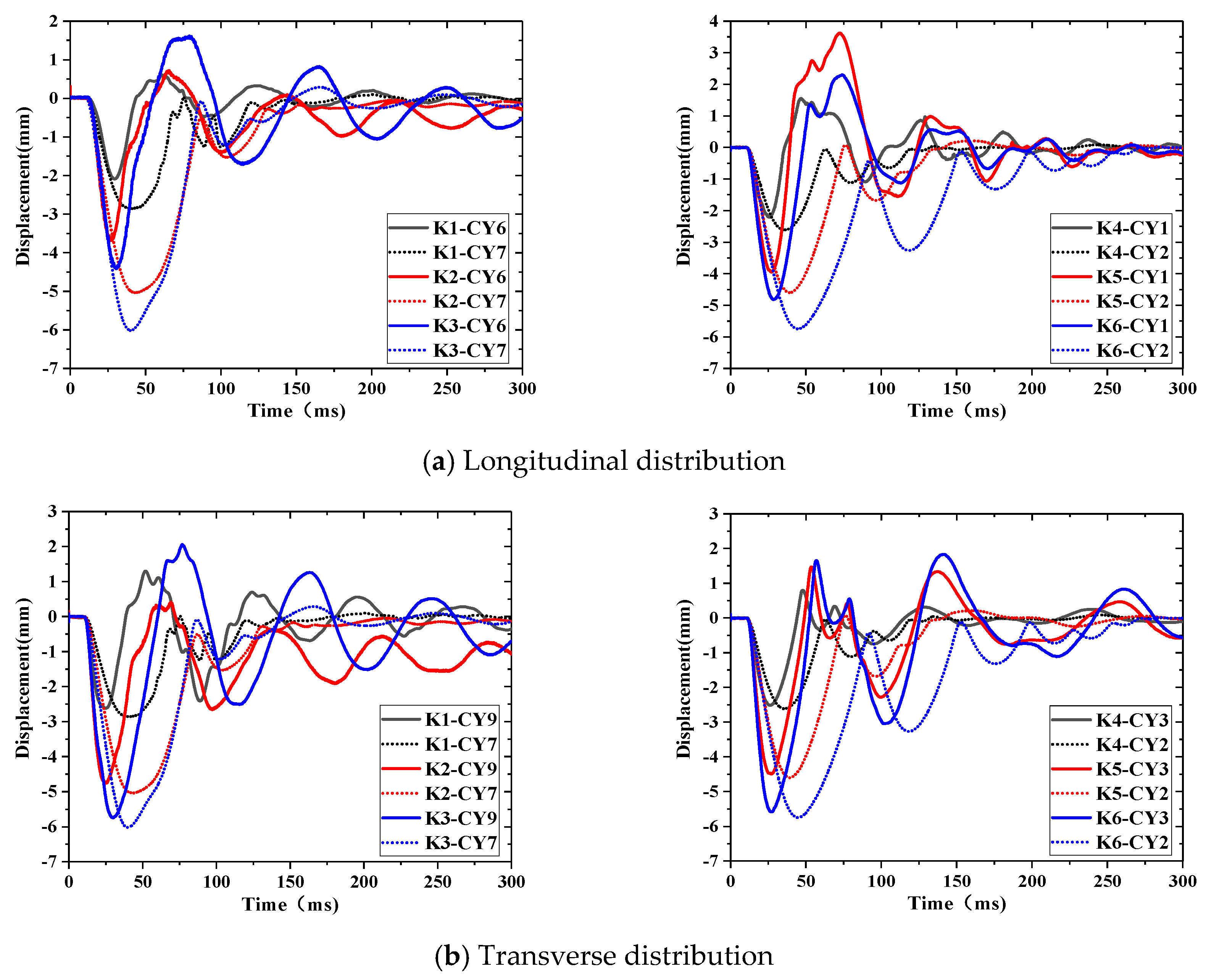
3.2. Displacement
3.3. Acceleration
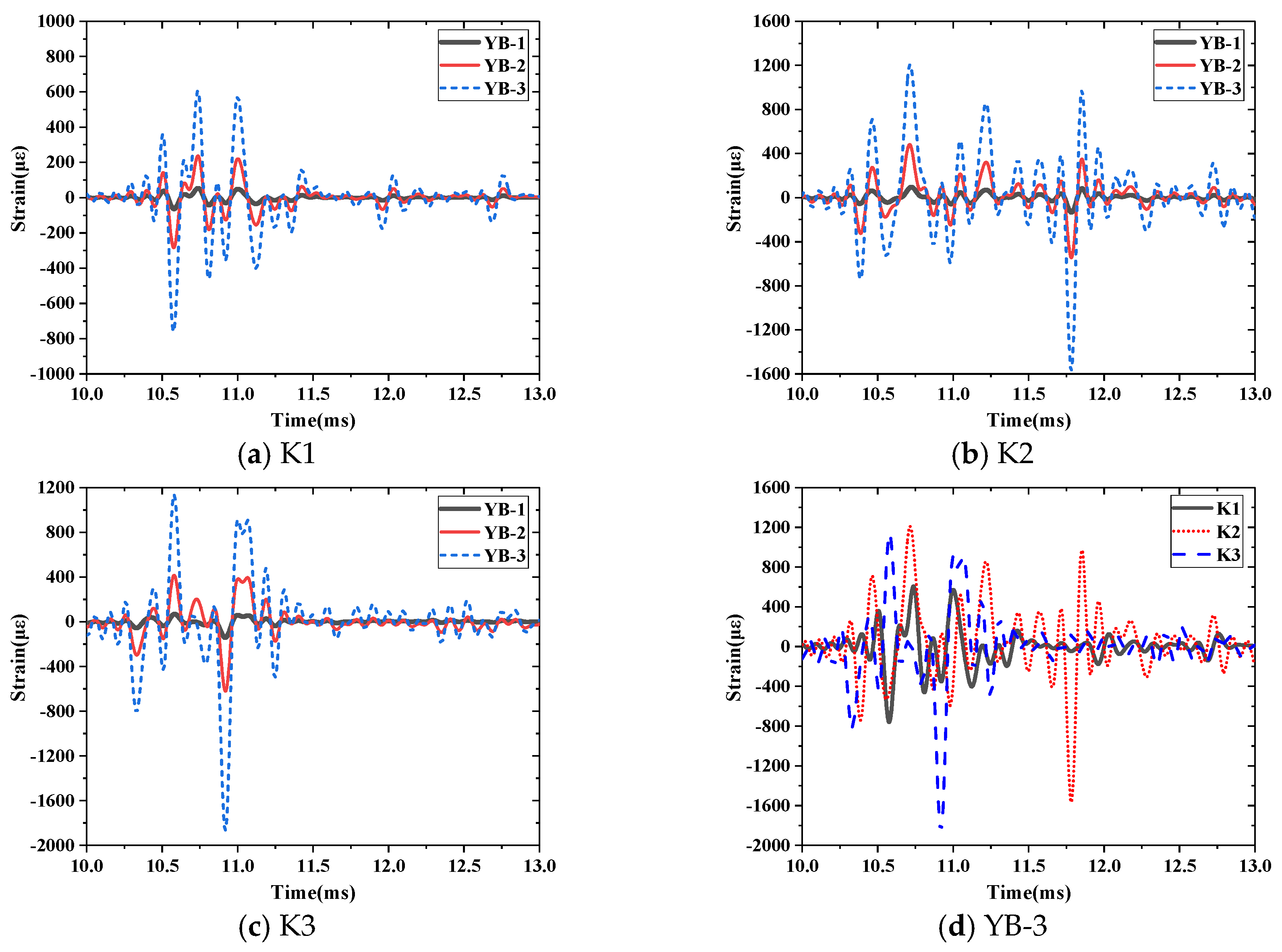
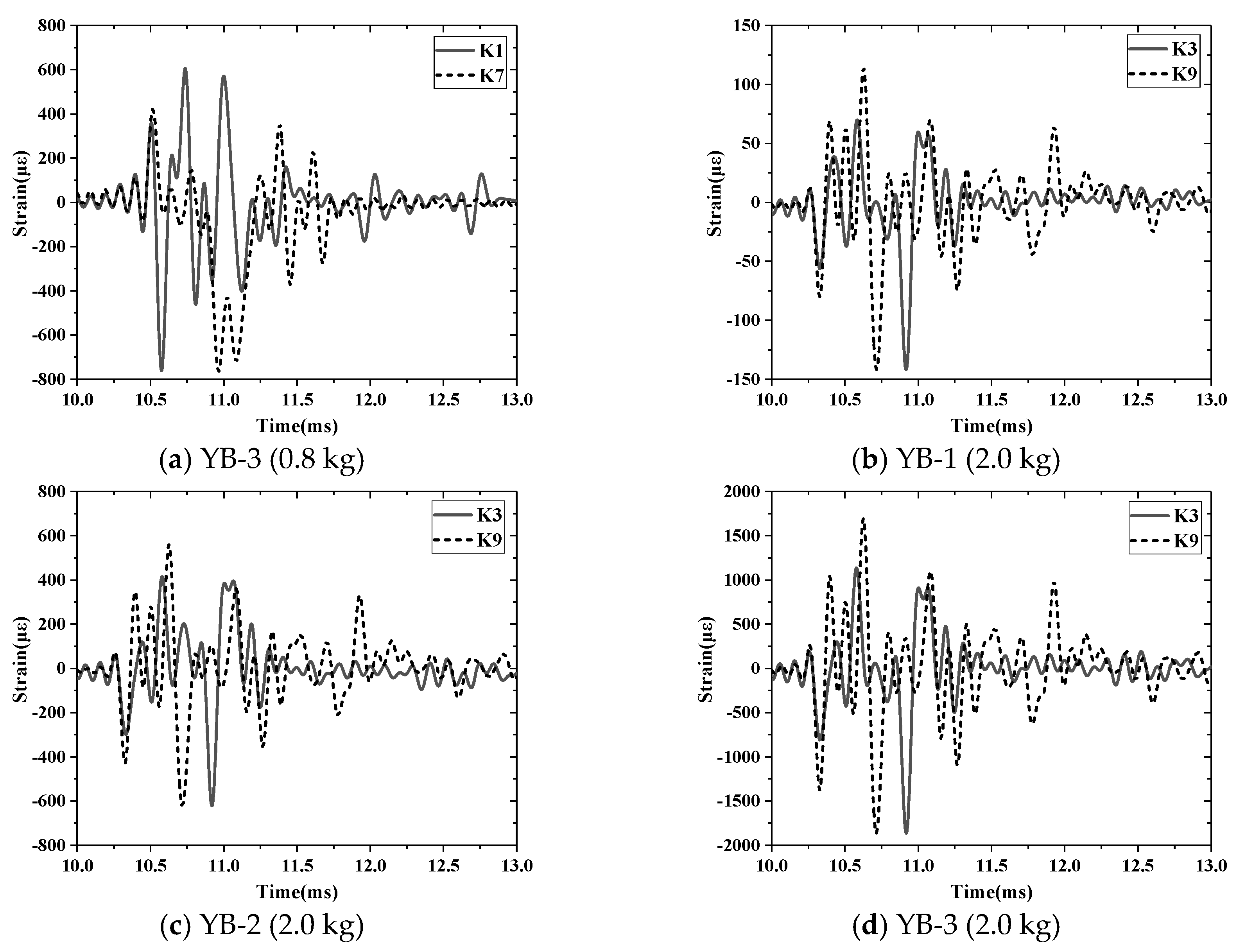
3.4. Strain
4. Experimental Results with R-IPDs
4.1. Displacement
4.2. Acceleration
4.3. Strain
5. Conclusions
- (1)
- The overpressure at the measuring point increased with an increase in the explosive equivalent (EE). The rate of increase in the peak overpressure was much faster than the rate of decrease in the distance between the measuring point and the explosion centre. For non-contact explosions, the existing theoretical methods in [46] can describe the overpressure and the distribution law of the model bridge.
- (2)
- The peak displacement of the main beam was significantly affected by its span (stiffness) when the EE was small. Additionally, the peak displacement of the middle span was larger than that of the side span. However, the local dynamic response (acceleration and strain) of the main beam was not significantly affected by its span, and the acceleration and strain responses of the main beam preceded its displacement response. With an increase in EE, the entire displacement and local dynamic responses of the main beam increased gradually.
- (3)
- The damping effect of the R-IPD on the explosion displacement response of the main beam was evident (the maximum decreasing ratio reached 12.5%). The decreasing ratio of the peak displacement of the main beam gradually increased with an increase in EE. Moreover, the decreasing ratio of the peak displacement of the midspan was greater than that of the 1/4 span. However, the damping effect on the acceleration and strain response of the main beam was not significant (less than 2%). The damping effect of the R-IPD had evident hysteresis characteristics and was directly related to the relative displacement response between the two ends of the damper. In other words, the R-IPD can obviously decrease the entire dynamic response of bridges but has little effect on the local dynamic response, such as acceleration and reinforcement strain.
- (4)
- The local response and entire response of bridge structures are quite different under explosion loads. The existing anti-explore method is mainly focused on the local response of bridge structures, which cannot be used to control their entire response. An R-IPD can be used to decrease the entire response of bridges under explosion loads. However, the parameter-optimised method of R-IPDs, as well as the dynamic response law of R-IPD under explosion loads, need to be further studied.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Stewart, M.G.; Mueller, J. Terrorism Risks for Bridges in a Multi-Hazard Environment. Int. J. Prot. Struct. 2014, 5, 275–289. [Google Scholar] [CrossRef]
- Williams, G.; Holland, C.; Williamson, E.B.; Bayrak, O.; Marchand, K.A.; Ray, J.C. Blast-Resistant Highway Bridges: Design and Detailing Guidelines; The National Academies Press: Washington, DC, USA, 2012; pp. 143–151. [Google Scholar] [CrossRef]
- Wierschem, N.E.; Luo, J.; Wilcoski, J.; Hubbard, S.A.; Fahnestock, L.A.; Spencer, B.F., Jr.; McFarland, D.M.; Quinn, D.D.; Vakakis, A.F.; Bergman, L.A. Simulating offset blast loads experimentally using shake-table-generated ground motions: Method development and validation. Struct. Control Health Monit. 2020, 27, e2480.1–e2480.14. [Google Scholar] [CrossRef]
- Temsah, Y.; Jahami, A.; Khatib, J.; Sonebi, M. Numerical analysis of a reinforced concrete beam under blast loading. In MATEC Web of Conferences; EDP Sciences: Les Ulis, France, 2018; Volume 149, p. 02063. [Google Scholar]
- Son, J.; Lee, H.-J. Performance of cable-stayed bridge pylons subjected to blast loading. Eng. Struct. 2011, 33, 1133–1148. [Google Scholar] [CrossRef]
- Li, Q.M.; Meng, H. Pressure-mpulse diagram for blast loads based on dimensionalanalysis and single-degree-of-freedom model. J. Eng. Mech. 2002, 128, 87–92. [Google Scholar] [CrossRef]
- Rong, H.C.; Li, B. Probabilistic response evaluation for RC flexural memberssubjected to blast loadings. Struct. Saf. 2007, 29, 146–163. [Google Scholar] [CrossRef]
- Zhang, D.; Yao, S.; Lu, F.; Chen, X.; Lin, G.; Wang, W.; Lin, Y. Experimental study on scaling of RC beams under close-in blast loading. Eng. Fail. Anal. 2013, 33, 497–504. [Google Scholar] [CrossRef]
- Winget, D.G.; Marchand, K.A.; Williamson, E.B. Analysis and Design of Critical Bridges Subjected to Blast Loads. J. Struct. Eng. 2005, 131, 1243–1255. [Google Scholar] [CrossRef]
- Yuan, S.; Hao, H.; Zong, Z.; Li, J. A study of RC bridge columns under contact explosion. Int. J. Impact Eng. 2017, 109, 378–390. [Google Scholar] [CrossRef]
- Hao, H.; Tang, E.K.C. Numerical simulation of a cable-stayed bridge response to blast loads, Part II: Damage prediction and FRP strengthening. Eng. Struct. 2010, 32, 3193–3205. [Google Scholar] [CrossRef]
- Tang, E.K.C.; Hao, H. Numerical simulation of a cable-stayed bridge response to blast loads, Part I: Model development and response calculations. Eng. Struct. 2010, 32, 3180–3192. [Google Scholar] [CrossRef]
- Cofer, W.F.; Matthews, D.S.; McLean, D.I. Effects of Blast Loading on Prestressed Girder Bridges. Shock Vib. 2012, 19, 186272. [Google Scholar] [CrossRef]
- Shiravand, M.; Parvanehro, P. Numerical study on damage mechanism of post-tensioned concrete box bridges under close-in deck explosion. Eng. Fail. Anal. 2017, 81, 103–116. [Google Scholar] [CrossRef]
- Wang, X.Y.; Feng, Y.J. Study of Dynamic Response and Influence Factors of Continuous Girder Bridge under Blast Loading. Blasting 2017, 34, 104–113. [Google Scholar]
- Tokal-Ahmed, Y.M. Response of Bridge Structures Subjected to Blast Loads and Protection Techniques to Mitigate the Effect of Blast Hazards on Bridge; Rutgers The State University of New Jersey: New Brunswick, NJ, USA, 2009. [Google Scholar] [CrossRef]
- Algassem, O.; Li, Y.; Aoude, H. Ability of steel fibers to enhance the shear and flexural behavior of high-strength concrete beams subjected to blast loads. Eng. Struct. 2019, 199, 109611. [Google Scholar] [CrossRef]
- Algassem, O.; Li, Y.; Aoude, H. Influence of steel fibers on the shear and flexural performance of high-strength concrete beams tested under blast loads. IOP Conf. Ser. Mater. Sci. Eng. 2017, 246, 012032. [Google Scholar] [CrossRef]
- Li, Y.; Aoude, H. Influence of steel fibers on the static and blast response of beams built with high-strength concrete and high-strength reinforcement. Eng. Struct. 2020, 221, 111031. [Google Scholar] [CrossRef]
- Liu, S.; Zhou, Y.; Zheng, Q.; Zhou, J.; Jin, F.; Fan, H. Blast responses of concrete beams reinforced with steel-GFRP composite bars. Structures 2019, 22, 200–212. [Google Scholar] [CrossRef]
- Qu, Y.; Liu, W.; Gwarzo, M.; Zhang, W.; Zhai, C.; Kong, X. Parametric study of anti-explosion performance of reinforced concrete T-shaped beam strengthened with steel plates. Constr. Build. Mater. 2017, 156, 692–707. [Google Scholar] [CrossRef]
- Liao, Z.; Tang, D.; Li, Z.; Xue, Y.; Shao, L. Study on explosion resistance performance experiment and damage assessment model of high-strength reinforcement concrete beams. Int. J. Impact Eng. 2019, 133, 103362. [Google Scholar] [CrossRef]
- Wu, C.; Oehlers, D.J.; Rebentrost, M.; Leach, J.; Whittaker, A.S. Blast testing of ultra-high performance fibre and FRP-retrofitted concrete slabs. Eng. Struct. 2009, 31, 2060–2069. [Google Scholar] [CrossRef]
- Kong, X.; Qi, X.; Gu, Y.; Lawan, I.A.; Qu, Y. Numerical evaluation of blast resistance of RC slab strengthened with AFRP. Constr. Build. Mater. 2018, 178, 244–253. [Google Scholar] [CrossRef]
- Li, J.; Wu, C.; Hao, H.; Su, Y.; Li, Z.-X. A study of concrete slabs with steel wire mesh reinforcement under close-in explosive loads. Int. J. Impact Eng. 2017, 110, 242–254. [Google Scholar] [CrossRef]
- Fouch, P.; Bruneau, M.; Asee, F. Modified steel-jacketed columns for combined blast and seismic retrofit of existing bridge columns. J. Bridge Eng. 2016, 21, 4016035. [Google Scholar] [CrossRef]
- Nurick, G.; Langdon, G.; Chi, Y.; Jacob, N. Behaviour of sandwich panels subjected to intense air blast—Part 1: Experiments. Compos. Struct. 2009, 91, 433–441. [Google Scholar] [CrossRef]
- Karagiozova, D.; Nurick, G.N.; Langdon, G.S. Behaviour of sandwich panels subject to intense air blasts Part 2: Numerical simu-lation. Compos. Struct. 2009, 91, 442–450. [Google Scholar] [CrossRef]
- Li, S.; Li, X.; Wang, Z.; Wu, G.; Lu, G.; Zhao, L. Finite element analysis of sandwich panels with stepwise graded aluminum honeycomb cores under blast loading. Compos. Part A Appl. Sci. Manuf. 2016, 80, 1–12. [Google Scholar] [CrossRef]
- Li, S.; Li, X.; Wang, Z.; Wu, G.; Lu, G.; Zhao, L. Sandwich panels with layered graded aluminum honeycomb cores under blast loading. Compos. Struct. 2017, 173, 242–254. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, X.; Song, L.; Zhou, H.; Wang, Y.; Zhang, H.; Cong, P. Mitigating confined blast response of buried steel box structure with foam concrete. Thin-Walled Struct. 2021, 169, 108473. [Google Scholar] [CrossRef]
- Ikago, K.; Saito, K.; Inoue, N. Seismic control of single-degree-of-freedom structure using tunedviscous mass damper. Earthq. Eng. Struct. Dyn. 2012, 41, 453–474. [Google Scholar] [CrossRef]
- Fricke, J.R. Lodengraf damping: An advanced vibration damping technology. SV Sound Vib. 2000, 34, 22–27. [Google Scholar]
- Panossian, H.V. Structural damping enhancement via Non-obstructive particle damping technique. J. Vib. Acoust. 1992, 114, 101–105. [Google Scholar] [CrossRef]
- Shen, B.; Xu, W.; Wang, J.; Chen, Y.; Yan, W.; Huang, J.; Tang, Z. Seismic control of super high-rise structures with double-layer tuned particle damper. Earthq. Eng. Struct. Dyn. 2021, 50, 791–810. [Google Scholar] [CrossRef]
- Huang, X.H.; Xu, W.B.; Yan, W.M. Equivalent model of a multiparticle damper considering the mass coupling coefficient. Struct. Control Health Monit. 2020, 27, e2580. [Google Scholar] [CrossRef]
- Huang, X.H.; Xu, W.B.; Wang, J.; Yan, W.M.; Chen, Y.J. Equivalent model of a multi-particle damper considering particle rolling and its analytical solution. Struct. Control Health Monit. 2021, 28, e2718. [Google Scholar] [CrossRef]
- Huang, X.H.; Xu, W.B.; Yan, W.M.; Wang, J. Equivalent model and parameter analysis of non-packed particle damper. J. Sound Vib. 2021, 491, 115775. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.; Zhou, Y. An equivalent method for optimization of particle tuned mass damper based on experimental para-metric study. J. Sound Vib. 2018, 419, 571–584. [Google Scholar] [CrossRef]
- Matuttis, H.G.; Chen, J.F. Understanding the Discrete Element Method: Simulation of Non-Spherical Particles for Granular and Multi-Body Systems; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2014. [Google Scholar]
- Lu, Z.; Wang, Z.; Masri, S.F.; Lu, X. Particle impact dampers: Past, present, and future. Struct. Control Health Monit. 2018, 25, e2058.1–e2058.25. [Google Scholar] [CrossRef]
- Zhao, G.; Ma, Y.; Yang, Z.; Wang, Q.; Li, Y. Friction mechanism and experimental investigation of the response-amplified friction damper. Struct. Control Health Monit. 2022, 29, e2953.1–e2953.19. [Google Scholar] [CrossRef]
- Crandall, S.H.; Mark, W.D. Random Vibration in Mechanical Systems; Academic Press: New York, NY, USA, 2014. [Google Scholar]
- Pan, C.; Zhang, R.; Luo, H.; Li, C.; Shen, H. Demand-based optimal design of oscillator with parallel-layout viscous inerter damper. Struct. Control Health Monit. 2017, 25, e2051. [Google Scholar] [CrossRef]
- Pan, C.; Zhang, R.F. Design of structure with inerter system based on stochastic response mitigation ratio. Struct. Control Health Monit. 2018, 25, e2169. [Google Scholar] [CrossRef]
- Randers-Pehrson, G.; Bannister, K.A. Airblast Loading Model for DYNA2D and DYNA3D. No. Arl-Tr-1310; Army Research Lab: Aberdeen Proving Ground, MD, USA, 1997.












| Type | Physical Quantity | Dimension | Similarity Coefficient |
|---|---|---|---|
| Geometric dimensions | Line size l | [L] | SL = 1/4 |
| Displacement δ | [L] | Sδ = SL = 1/4 | |
| Material characteristics | Elastic modulus E | [FL−2] | SE = 1.0000 |
| Density ρ | [FL−3] | Sρ = 4 | |
| Poisson’s ratio μ | - | Sμ = 1.0000 | |
| Strain ε | - | Sε = 1 | |
| Stress σ | [FL−2] | Sσ= SE Sε = 1.0000 | |
| Load | Uniform load P | [FL−2] | 1 |
| Concentrated load F | [F] | SF= SE SL2 = 1/16 | |
| dynamic properties | acceleration amplitude a | [LT−2] | Sa = 4 |
| Ball Screw | Flywheel | Particles | ||||||
|---|---|---|---|---|---|---|---|---|
| Diameter (cm) | Length (cm) | Lead (cm) | Diameter (cm) | Thickness (cm) | Mass (kg) | Diameter (cm) | Number | Mass (kg) |
| 3.2 | 55.0 | 0.5 | 12.0 | 4.0 | 3.5 | 0.5 | 350.0 | 0.5 |
| Mass Ratio min/m0 | Damping Ratio cd/cn | Stiffness Ratio kd/kn |
|---|---|---|
| 3041 | 20,542 | 20,542 |
| Condition | Detonation Point | Explosive Equivalent (kg) | Scale Distance (m/kg1/3) |
|---|---|---|---|
| K1 | 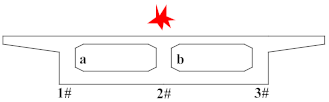  On the 1.0 m top of the geometric centre of the second span | 0.8 | 1.077 |
| K2 | 1.6 | 0.855 | |
| K3 | 2.0 | 0.794 | |
| K4 | 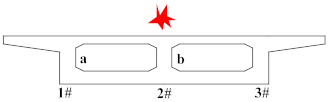  On the 1.0 m top of the geometric centre of the first span | 0.8 | 1.077 |
| K5 | 1.6 | 0.855 | |
| K6 | 2.0 | 0.794 | |
| K7–K12 | Test cases were the same as K1–K6 except with R-IPDs | ||
| Sensor | K1 | K2 | K3 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Pm/MPa | Pt/MPa | λ/% | Pm/MPa | Pt/MPa | λ/% | Pm/MPa | Pt/MPa | λ/% | |
| CY1 | 0.068 | 0.063 | −7.94 | 0.116 | 0.104 | −11.54 | 0.132 | 0.123 | −7.32 |
| CY2 | 0.162 | 0.172 | 5.81 | 0.286 | 0.305 | 6.23 | 0.350 | 0.367 | 4.63 |
| CY3 | 0.923 | 0.966 | 4.45 | 1.688 | 1.771 | 4.69 | 2.001 | 2.147 | 6.80 |
| CY4 | 4.160 | 4.622 | 10.00 | 8.535 | 9.362 | 8.83 | 10.135 | 11.832 | 14.34 |
| CY8 | 0.344 | 0.352 | 2.27 | 0.727 | 0.640 | −13.59 | 0.784 | 0.776 | −1.03 |
| CY9 | 1.544 | 1.671 | 7.60 | 2.964 | 3.050 | 2.82 | 3.392 | 3.714 | 8.67 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, M.; Xu, W.; Wang, J.; Chen, Y.; Zhou, D.; Hou, L.; Yan, Q. Preliminary Study on the Damping Effect of a Rotational Inertia Particle Damper Considering the Explosion Response of Continuous Concrete Bridges. Buildings 2023, 13, 1726. https://doi.org/10.3390/buildings13071726
Xie M, Xu W, Wang J, Chen Y, Zhou D, Hou L, Yan Q. Preliminary Study on the Damping Effect of a Rotational Inertia Particle Damper Considering the Explosion Response of Continuous Concrete Bridges. Buildings. 2023; 13(7):1726. https://doi.org/10.3390/buildings13071726
Chicago/Turabian StyleXie, Mengfei, Weibing Xu, Jin Wang, Yanjiang Chen, Daxing Zhou, Liqun Hou, and Qiushi Yan. 2023. "Preliminary Study on the Damping Effect of a Rotational Inertia Particle Damper Considering the Explosion Response of Continuous Concrete Bridges" Buildings 13, no. 7: 1726. https://doi.org/10.3390/buildings13071726
APA StyleXie, M., Xu, W., Wang, J., Chen, Y., Zhou, D., Hou, L., & Yan, Q. (2023). Preliminary Study on the Damping Effect of a Rotational Inertia Particle Damper Considering the Explosion Response of Continuous Concrete Bridges. Buildings, 13(7), 1726. https://doi.org/10.3390/buildings13071726






