Review of the Experimental Methods for Evaluation of Windows’ Thermal Transmittance: From Standardized Tests to New Possibilities
Abstract
1. Introduction
2. Experimental Methods for Windows U-Value Determination
2.1. Heat Flow Meter (HFM) Method
2.1.1. Heat Flow Meter Apparatus
2.1.2. Heat Flow Meter Sensors for In Situ Measurements
2.2. Guarded Hot Plate (GHP) Method
2.3. Hot Box (HB) Method
2.4. Infrared Thermography (IRT) Method
2.5. Developed Rapid U-Value Meter Tools
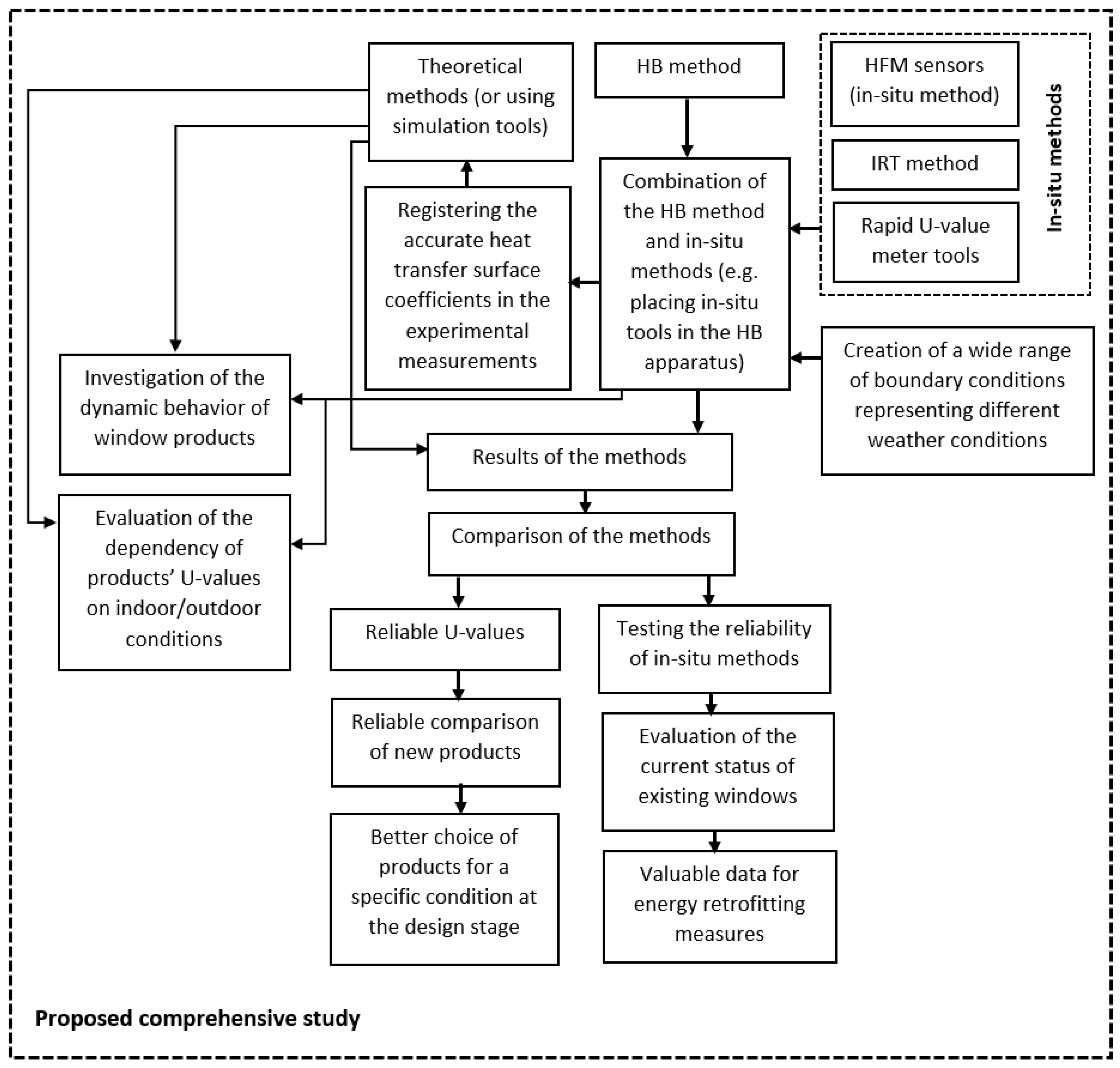
3. Critical Review
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mirrahimi, S.; Mohamed, M.F.; Haw, L.C.; Ibrahim, N.L.N.; Yusoff, W.F.M.; Aflaki, A. The effect of building envelope on the thermal comfort and energy saving for high-rise buildings in hot–humid climate. Renew. Sustain. Energy Rev. 2016, 53, 1508–1519. [Google Scholar] [CrossRef]
- Fereidani, N.A.; Rodrigues, E.; Gaspar, A.R. A review of the energy implications of passive building design and active measures under climate change in the Middle East. J. Clean. Prod. 2021, 305, 127152. [Google Scholar] [CrossRef]
- Asdrubali, F.; Baldinelli, G. Thermal transmittance measurements with the hot box method: Calibration, experimental procedures, and uncertainty analyses of three different approaches. Energy Build. 2011, 43, 1618–1626. [Google Scholar] [CrossRef]
- Kamalisarvestani, M.; Saidur, R.; Mekhilef, S.; Javadi, F. Performance, materials and coating technologies of thermochromic thin films on smart windows. Renew. Sustain. Energy Rev. 2013, 26, 353–364. [Google Scholar] [CrossRef]
- Kirimtat, A.; Koyunbaba, B.K.; Chatzikonstantinou, I.; Sariyildiz, S. Review of simulation modeling for shading devices in buildings. Renew. Sustain. Energy Rev. 2016, 53, 23–49. [Google Scholar] [CrossRef]
- Tong, S.W.; Goh, W.P.; Huang, X.; Jiang, C. A review of transparent-reflective switchable glass technologies for building facades. Renew. Sustain. Energy Rev. 2021, 152, 111615. [Google Scholar] [CrossRef]
- Bienvenido-Huertas, D.; Moyano, J.; Marín, D.; Fresco-Contreras, R. Review of in situ methods for assessing the thermal transmittance of walls. Renew. Sustain. Energy Rev. 2018, 102, 356–371. [Google Scholar] [CrossRef]
- Hee, W.J.; Alghoul, M.A.; Bakhtyar, B.; Elayeb, O.; Shameri, M.A.; Alrubaih, M.S.; Sopian, K. The role of window glazing on daylighting and energy saving in buildings. Renew. Sustain. Energy Rev. 2015, 42, 323–343. [Google Scholar] [CrossRef]
- Aguilar-Santana, J.L.; Jarimi, H.; Velasco-Carrasco, M.; Riffat, S. Review on window-glazing technologies and future prospects. Int. J. Low-Carbon Technol. 2019, 15, 112–120. [Google Scholar] [CrossRef]
- Yang, S.; Cho, H.M.; Yun, B.Y.; Hong, T.; Kim, S. Energy usage and cost analysis of passive thermal retrofits for low-rise residential buildings in Seoul. Renew. Sustain. Energy Rev. 2021, 151, 111617. [Google Scholar] [CrossRef]
- Park, S.; Kim, S.; Jeong, H.; Do, S.; Kim, J. In Situ Evaluation of the U-Value of a Window Using the Infrared Method. Energies 2021, 14, 1904. [Google Scholar] [CrossRef]
- Cuce, E.; Riffat, S.B. A state-of-the-art review on innovative glazing technologies. Renew. Sustain. Energy Rev. 2015, 41, 695–714. [Google Scholar] [CrossRef]
- Stevanović, S. Optimization of passive solar design strategies: A review. Renew. Sustain. Energy Rev. 2013, 25, 177–196. [Google Scholar] [CrossRef]
- Moghaddam, S.A.; Mattsson, M.; Ameen, A.; Akander, J.; Da Silva, M.G.; Simões, N. Low-Emissivity Window Films as an Energy Retrofit Option for a Historical Stone Building in Cold Climate. Energies 2021, 14, 7584. [Google Scholar] [CrossRef]
- Pereira, J.; Teixeira, H.; Gomes, M.D.G.; Rodrigues, A.M. Performance of Solar Control Films on Building Glazing: A Literature Review. Appl. Sci. 2022, 12, 5923. [Google Scholar] [CrossRef]
- Akram, M.W.; Hasannuzaman, M.; Cuce, E.; Cuce, P.M. Global technological advancement and challenges of glazed window, facade system and vertical greenery-based energy savings in buildings: A comprehensive review. Energy Built Environ. 2021, 4, 206–226. [Google Scholar] [CrossRef]
- Silva, T.; Vicente, R.; Rodrigues, F. Literature review on the use of phase change materials in glazing and shading solutions. Renew. Sustain. Energy Rev. 2016, 53, 515–535. [Google Scholar] [CrossRef]
- Marinoski, D.L.; Melo, A.; Weber, F.; Guths, S.; Lamberts, R. Measurement of solar factor of glazing and shading devices using a solar calorimeter. Build. Environ. 2018, 144, 72–85. [Google Scholar] [CrossRef]
- Ghosh, A. Investigation of vacuum-integrated switchable polymer dispersed liquid crystal glazing for smart window application for less energy-hungry building. Energy 2023, 265, 126396. [Google Scholar] [CrossRef]
- UNECE. Study on Mapping of Energy Efficiency Standards and Technologies in Buildings in the UNECE Region; UNECE: Geneva, Switzerland, 2018. [Google Scholar]
- Basak, C.K.; Sarkar, G.; Neogi, S. Performance evaluation of material and comparison of different temperature control strategies of a Guarded Hot Box U-value Test Facility. Energy Build. 2015, 105, 258–262. [Google Scholar] [CrossRef]
- Pavlenko, A.M.; Sadko, K. Evaluation of Numerical Methods for Predicting the Energy Performance of Windows. Energies 2023, 16, 1425. [Google Scholar] [CrossRef]
- Baldinelli, G.; Bianchi, F. Windows thermal resistance: Infrared thermography aided comparative analysis among finite volumes simulations and experimental methods. Appl. Energy 2014, 136, 250–258. [Google Scholar] [CrossRef]
- Goia, F.; Serra, V. Analysis of a non-calorimetric method for assessment of in-situ thermal transmittance and solar factor of glazed systems. Sol. Energy 2018, 166, 458–471. [Google Scholar] [CrossRef]
- Prata, J.; Simões, N.; Tadeu, A. Heat transfer measurements of a linear thermal bridge in a wooden building corner. Energy Build. 2018, 158, 194–208. [Google Scholar] [CrossRef]
- Cuce, E. Accurate and reliable U-value assessment of argon-filled double glazed windows: A numerical and experimental investigation. Energy Build. 2018, 171, 100–106. [Google Scholar] [CrossRef]
- Huang, Y.; El Mankibi, M.; Cantin, R. Identification of dynamic U-values for supply-air double windows based on experiments. In Proceedings of the CLIMA 2022 the 14th REHVA HVAC World Congress, Rotterdam, The Netherlands, 22–25 May 2022. [Google Scholar]
- ASHRAE Handbook Fundamentals, SI ed.; Refrigerating and Air-Conditioning Engineers, Inc.: Atlanta, GA, USA, 2021.
- ISO 15099; Thermal Performance of Windows, Doors and Shading Devices—Detailed Calculations. International Organization for Standardization: Geneva, Switzerland, 2003.
- Soares, N.; Martins, C.; Gonçalves, M.; Santos, P.; da Silva, L.S.; Costa, J.J. Laboratory and in-situ non-destructive methods to evaluate the thermal transmittance and behavior of walls, windows, and construction elements with innovative materials: A review. Energy Build. 2018, 182, 88–110. [Google Scholar] [CrossRef]
- EN 675; Glass in Building. Determination of Thermal Transmittance (U Value). Heat Flow Meter Method. European Committee for Standardization: Brussels, Belgium, 2011.
- ISO 10293; Glass in Building—Determination of Steady-State U Values (Thermal Transmittance) of Multiple Glazing—Heat Flow Meter Method. International Organization for Standard: Geneva, Switzerland, 1997.
- Huang, Y.; El Mankibi, M.; Cantin, R.; Coillot, M. Application of fluids and promising materials as advanced inter-pane media in multi-glazing windows for thermal and energy performance improvement: A review. Energy Build. 2021, 253, 111458. [Google Scholar] [CrossRef]
- Yüksel, N. The Review of Some Commonly Used Methods and Techniques to Measure the Thermal Conductivity of Insulation Materials. In Insulation Materials in Context of Sustainability; Almusaed, A., Almssad, A., Eds.; IntechOpen: London, UK, 2016. [Google Scholar] [CrossRef]
- ISO 9869-1; Thermal Insulation—Building Elements—Insitu Measurement of Thermal Resistance and Thermal Transmittance; Part 1: Heat Flow Meter Method. International Organization for Standardization: Geneva, Switzerland, 2014.
- ASTM C1155-95 (2021); Standard Practice for Determining Thermal Resistance of Building Envelope Components from the In-Situ Data. ASTM International: West Conshohocken, PA, USA, 2021.
- Atsonios, I.A.; Mandilaras, I.D.; Kontogeorgos, D.A.; Founti, M.A. A comparative assessment of the standardized methods for the in–situ measurement of the thermal resistance of building walls. Energy Build. 2017, 154, 198–206. [Google Scholar] [CrossRef]
- Gonçalves, M.; Serra, C.; Simões, N.; Flores-Colen, I.; Kokolsky, C.; Sprengard, C. Onsite monitoring of a wall retrofitted with an external vacuum insulation composite system. J. Build. Eng. 2021, 44, 103301. [Google Scholar] [CrossRef]
- Feng, Y.; Duan, Q.; Wang, J.; Baur, S. Approximation of building window properties using in situ measurements. Build. Environ. 2019, 169, 106590. [Google Scholar] [CrossRef]
- Aguilar-Santana, J.L.; Velasco-Carrasco, M.; Riffat, S. Thermal Transmittance (U-value) Evaluation of Innovative Window Technologies. Futur. Cities Environ. 2020, 6, 1–13. [Google Scholar] [CrossRef]
- Deconinck, A.-H.; Roels, S. Comparison of characterisation methods determining the thermal resistance of building components from onsite measurements. Energy Build. 2016, 130, 309–320. [Google Scholar] [CrossRef]
- Gaspar, K.; Casals, M.; Gangolells, M. A comparison of standardized calculation methods for in situ measurements of façades U-value. Energy Build. 2016, 130, 592–599. [Google Scholar] [CrossRef]
- Lu, X.; Memari, A.M. Comparison of the Experimental Measurement Methods for Building Envelope Thermal Transmittance. Buildings 2022, 12, 282. [Google Scholar] [CrossRef]
- greenTEG AG. gSKIN ® Application Note: U-Value Glass Measurement. 2014. Available online: http://shop.greenteg.com/wp-content/uploads/gSKIN-application-note_U-value-glass_case-study.pdf (accessed on 22 January 2023).
- O’Hegarty, R.; Kinnane, O.; Lennon, D.; Colclough, S. In-situ U-value monitoring of highly insulated building envelopes: Review and experimental investigation. Energy Build. 2021, 252, 111447. [Google Scholar] [CrossRef]
- Ficco, G.; Iannetta, F.; Ianniello, E.; Alfano, F.R.D.; Dell’Isola, M. U-value in situ measurement for energy diagnosis of existing buildings. Energy Build. 2015, 104, 108–121. [Google Scholar] [CrossRef]
- Marshall, A.; Fitton, R.; Swan, W.; Farmer, D.; Johnston, D.; Benjaber, M.; Ji, Y. Domestic building fabric performance: Closing the gap between the in situ measured and modelled performance. Energy Build. 2017, 150, 307–317. [Google Scholar] [CrossRef]
- KS F 2278; Standard Test Method for Thermal Resistance for Windows and Doors. Korean Standards Association: Seoul, Republic of Korea, 2017.
- Roulet, C.; Gass, J.; Markus, I. In-Situ U-Value Measurement: Reliable Results in Shorter Time By Dynamic Interpretation of Measured Data. ASHRAE Trans. 1985, 108, 1371–1379. [Google Scholar]
- Lu, X.; Memari, A. Application of infrared thermography for in-situ determination of building envelope thermal properties. J. Build. Eng. 2019, 26, 100885. [Google Scholar] [CrossRef]
- Yang, I.; Kim, D.; Lee, S.; Jang, H. Construction and calibration of a large-area heat flow meter apparatus. Energy Build. 2019, 203, 109445. [Google Scholar] [CrossRef]
- Tadeu, A.; Simões, I.; Simões, N.; Prata, J. Simulation of dynamic linear thermal bridges using a boundary element method model in the frequency domain. Energy Build. 2011, 43, 3685–3695. [Google Scholar] [CrossRef]
- Johra, H. Aalborg University Description of the Guarded Hot Plate Method for Thermal Conductivity Measurement with the EP500; Department of Civil Engineering, Aalborg University: Aalborg, Denmark, 2019. [Google Scholar] [CrossRef]
- ISO 10291; Glass in Building—Determination of Steady-State U Values (Thermal Transmittance) of Multiple Glazing—Guarded Hot Plate Method. International Organization for Standardization: Geneva, Switzerland, 1994.
- EN 674; Glass in Building—Determination of Thermal Transmittance (U Value)—Guarded Hot Plate Method. European Committee for Standardization (CEN): Brussels, Belgium, 2011.
- Sánchez-Palencia, P.; Martín-Chivelet, N.; Chenlo, F. Modeling temperature and thermal transmittance of building integrated photovoltaic modules. Sol. Energy 2019, 184, 153–161. [Google Scholar] [CrossRef]
- Wakili, K.G.; Raedle, W.; Krammer, A.; Uehlinger, A.; Schüler, A.; Stöckli, T. Ug-value and edge heat loss of triple glazed insulating glass units:A comparison between measured and declared values. J. Build. Eng. 2021, 44, 103031. [Google Scholar] [CrossRef]
- Lechowska, A. A CFD study and measurements of double glazing thermal transmittance under downward heat flow conditions. Energy Build. 2016, 122, 107–119. [Google Scholar] [CrossRef]
- ISO 8302; Thermal Insulation—Determination of Steady-State Thermal Resistance and Related Properties—Guarded Hot Plate Apparatus. International Organization for Standardization: Geneva, Switzerland, 1991.
- Eithun, C.F. Development of a Thermal Conductivity Apparatus: Analysis and Design; Norwegian University of Science and Technology: Trondheim, Norway, 2012. [Google Scholar]
- Thomas, W.C.; Zarr, R.R. Transient Thermal Response of a Guarded-Hot-Plate Apparatus for Operation Over an Extended Temperature Range. J. Res. Natl. Inst. Stand. Technol. 2018, 123, 123001. [Google Scholar] [CrossRef] [PubMed]
- EN 673; Glass in Building—Determination of Thermal Transmittance (U Value)—Calculation Method. European Committee for Standardization (CEN): Brussels, Belgium, 2011.
- Andreotti, M.; Calzolari, M.; Davoli, P.; Pereira, L.D.; Lucchi, E.; Malaguti, R. Design and Construction of a New Metering Hot Box for the In Situ Hygrothermal Measurement in Dynamic Conditions of Historic Masonries. Energies 2020, 13, 2950. [Google Scholar] [CrossRef]
- ASTM C1199; Standard Test Method for Measuring the Steady-State Thermal Transmittance of Fenestration Systems Using Hot Box Methods. American Society for Testing and Materials (ASTM): West Conshohocken, PA, USA, 2014.
- EN ISO 8990; Thermal Insulation—Determination of Steady-State Thermal Transmission Properties—Calibrated and Guarded Hot Box. European Standard: Brussels, Belgium, 1994.
- ASTM C1363-05; Standard Test Method for Thermal Performance of Building Materials and Envelope Assemblies by Means of a Hot Box Apparatus. American Society for Testing and Materials: West Conshohocken, PA, USA, 2005.
- GOST 26602.1-99; Windows and Doors. Methods of Determination of Resistance of Thermal Transmission. Interstate Standard of Russian Federation: Russia, 1999.
- Simões, I.; Simões, N.; Tadeu, A.; Riachos, J. Laboratory thermal transmittance assessments of homogeneous building elements using infrared thermography. In Proceedings of the 2014 International Conference on Quantitative InfraRed Thermography, Bordeaux, France, 7–11 July 2014. [Google Scholar] [CrossRef]
- Ghosh, A.; Hyde, T.J.; Neogi, S. Development and Performance Evaluation of a Virtual Pid Controller for a Guarded Hot Box Test Facility for U-Value Measurement. Int. J. Emerg. Technol. Adv. Eng. 2013, 3, 17–21. [Google Scholar]
- Transactions, T.; Techniczne, C. Measurement of thermal transmittance of multi-layer glazing with ultrathin in-ternal glass partitions. Czas. Tech. 2014, 3, 273–279. [Google Scholar] [CrossRef]
- Baldinelli, G.; Asdrubali, F.; Baldassarri, C.; Bianchi, F.; D’Alessandro, F.; Schiavoni, S.; Basilicata, C. Energy and environmental performance optimization of a wooden window: A holistic approach. Energy Build. 2014, 79, 114–131. [Google Scholar] [CrossRef]
- ISO 12567; Thermal Performance of Windows and Doors—Deter-Mination of Thermal Transmittance by the Hot-Box Method. International Organization for Standardization: Geneva, Switzerland, 2010.
- Desjarlais, A.O.; Zarr, R.R. Insulation Materials: Testing and Applications; ASTM International: West Conshohocken, PA, USA, 2002; Volume 4. [Google Scholar]
- Cho, S.; Kim, S.-H. Analysis of the Performance of Vacuum Glazing in Office Buildings in Korea: Simulation and Experimental Studies. Sustainability 2017, 9, 936. [Google Scholar] [CrossRef]
- Lechowska, A.A.; Schnotale, J.A.; Baldinelli, G. Window frame thermal transmittance improvements without frame geometry variations: An experimentally validated CFD analysis. Energy Build. 2017, 145, 188–199. [Google Scholar] [CrossRef]
- EN 12412-2; Thermal Performance of Windows, Doors and Shutters—Determination of Thermal Transmittance by Hot Box Method—Part 2: Frames. European Standard: Brussels, Belgium, 2003.
- Kim, S.-H.; Jeong, H.; Cho, S. A Study on Changes of Window Thermal Performance by Analysis of Physical Test Results in Korea. Energies 2019, 12, 3822. [Google Scholar] [CrossRef]
- Grynning, S.; Misiopecki, C.; Uvsløkk, S.; Time, B.; Gustavsen, A. Thermal performance of in-between shading systems in multilayer glazing units: Hot-box measurements and numerical simulations. J. Build. Phys. 2014, 39, 147–169. [Google Scholar] [CrossRef]
- Banionis, K.; Kumžienė, J.; Burlingis, A.; Ramanauskas, J.; Paukštys, V. The Changes in Thermal Transmittance of Window Insulating Glass Units Depending on Outdoor Temperatures in Cold Climate Countries. Energies 2021, 14, 1694. [Google Scholar] [CrossRef]
- Garai, G.E. Heat Transfer Evaluation of a Window with a “Hot Box” Set-Up in a 18th Century Stone Building by Using Comsol Software; University of Gavle: Gavle, Sweden, 2019. [Google Scholar]
- Brown, W.C.; Stephenson, D.G. Guarded Hot Box Procedure for Determining the Dynamic Response of Full-Scale Wall Specimens—Part I. ASHRAE Trans. 1993, 99, 632–642. [Google Scholar]
- Martin, K.; Campos-Celador, A.; Escudero, C.; Gómez, I.; Sala, J. Analysis of a thermal bridge in a guarded hot box testing facility. Energy Build. 2012, 50, 139–149. [Google Scholar] [CrossRef]
- Baldinelli, G.; Bianchi, F.; Lechowska, A.A.; Schnotale, J.A. Dynamic thermal properties of building components: Hot box experimental assessment under different solicitations. Energy Build. 2018, 168, 1–8. [Google Scholar] [CrossRef]
- Smith, N.; Isaacs, N.; Burgess, J.; Cox-Smith, I. Thermal performance of secondary glazing as a retrofit alternative for single-glazed windows. Energy Build. 2012, 54, 47–51. [Google Scholar] [CrossRef]
- Shen, Z.; Brooks, A.L.; He, Y.; Shrestha, S.S.; Zhou, H. Evaluating dynamic thermal performance of building envelope components using small-scale calibrated hot box tests. Energy Build. 2021, 251, 111342. [Google Scholar] [CrossRef]
- Serra, C.; Tadeu, A.; Simões, N. Heat transfer modeling using analytical solutions for infrared thermography applications in multilayered buildings systems. Int. J. Heat Mass Transf. 2017, 115, 471–478. [Google Scholar] [CrossRef]
- Serra, C.; Tadeu, A.; Simões, N. Boundary element method simulation of 3D heat diffusion in defective layered media for IRT building applications. Eng. Anal. Bound. Elements 2017, 81, 44–52. [Google Scholar] [CrossRef]
- O’Grady, M.; Lechowska, A.A.; Harte, A.M. Infrared thermography technique as an in-situ method of assessing heat loss through thermal bridging. Energy Build. 2017, 135, 20–32. [Google Scholar] [CrossRef]
- O’Grady, M.; Lechowska, A.A.; Harte, A.M. Quantification of heat losses through building envelope thermal bridges influenced by wind velocity using the outdoor infrared thermography technique. Appl. Energy 2017, 208, 1038–1052. [Google Scholar] [CrossRef]
- O’Grady, M.; Lechowska, A.A.; Harte, A.M. Application of infrared thermography technique to the thermal assessment of multiple thermal bridges and windows. Energy Build. 2018, 168, 347–362. [Google Scholar] [CrossRef]
- Asdrubali, F.; Baldinelli, G.; Bianchi, F. A quantitative methodology to evaluate thermal bridges in buildings. Appl. Energy 2012, 97, 365–373. [Google Scholar] [CrossRef]
- Kirimtat, A.; Krejcar, O. A review of infrared thermography for the investigation of building envelopes: Advances and prospects. Energy Build. 2018, 176, 390–406. [Google Scholar] [CrossRef]
- Lucchi, E. Applications of the infrared thermography in the energy audit of buildings: A review. Renew. Sustain. Energy Rev. 2018, 82, 3077–3090. [Google Scholar] [CrossRef]
- Lehmann, B.; Wakili, K.G.; Frank, T.; Collado, B.V.; Tanner, C. Effects of individual climatic parameters on the infrared thermography of buildings. Appl. Energy 2013, 110, 29–43. [Google Scholar] [CrossRef]
- Boafo, F.E.; Ahn, J.-G.; Kim, S.-M.; Kim, J.-H.; Kim, J.-T. Fenestration refurbishment of an educational building: Experimental and numerical evaluation of daylight, thermal and building energy performance. J. Build. Eng. 2019, 25, 100803. [Google Scholar] [CrossRef]
- Fokaides, P.A.; Kalogirou, S.A. Application of infrared thermography for the determination of the overall heat transfer coefficient (U-Value) in building envelopes. Appl. Energy 2011, 88, 4358–4365. [Google Scholar] [CrossRef]
- Maroy, K.; Carbonez, K.; Steeman, M.; Bossche, N.V.D. Assessing the thermal performance of insulating glass units with infrared thermography: Potential and limitations. Energy Build. 2017, 138, 175–192. [Google Scholar] [CrossRef]
- ISO 9869-2; Thermal Insulation—Building Elements—In-Situ Measurement of Thermal Resistance and Thermal Transmittance—Part 2: Infrared Method for Frame Structure Dwelling. International Organization for Standardization: Geneva, Switzerland, 2018.
- EN ISO 6946; Building Components and Building Elements—Thermal Resistance and Thermal Transmittance—Calculation Methods. International Organization for Standardization: Geneva, Switzerland, 2017.
- Sadhukhan, D.; Peri, S.; Sugunaraj, N.; Biswas, A.; Selvaraj, D.F.; Koiner, K.; Rosener, A.; Dunlevy, M.; Goveas, N.; Flynn, D.; et al. Estimating surface temperature from thermal imagery of buildings for accurate thermal transmittance (U-value): A machine learning perspective. J. Build. Eng. 2020, 32, 101637. [Google Scholar] [CrossRef]
- Gertsvolf, D.; Horvat, M.; Khademi, A.; Aslam, D.; Berardi, U. Image Processing for Future Machine Learning Algorithm Applications on Infrared Thermography of Building Envelope Systems. In Proceedings of the Cobee 2022, Montreal, ON, Canada, 25–29 July 2022. [Google Scholar]
- Sørensen, L.S. Energy Renovation of Buildings Utilizing the U-value Meter, a New Heat Loss Measuring Device. Sustainability 2010, 2, 461–474. [Google Scholar] [CrossRef]
- Pekkala, O. Precise U-Value Measurement of Installed Windows; ARCADA: Vantaa, Finland, 2020. [Google Scholar]
- Kuhn, T.E. State of the art of advanced solar control devices for buildings. Sol. Energy 2017, 154, 112–133. [Google Scholar] [CrossRef]
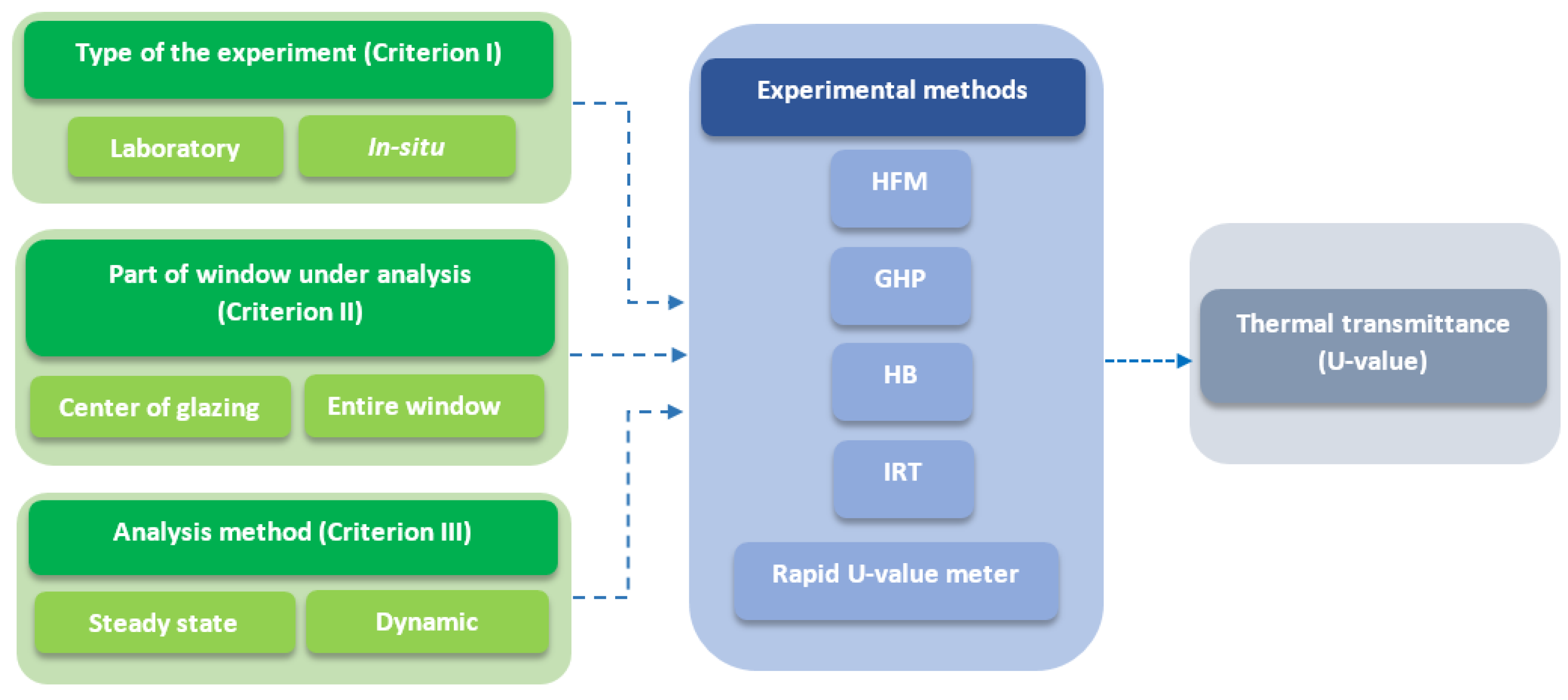



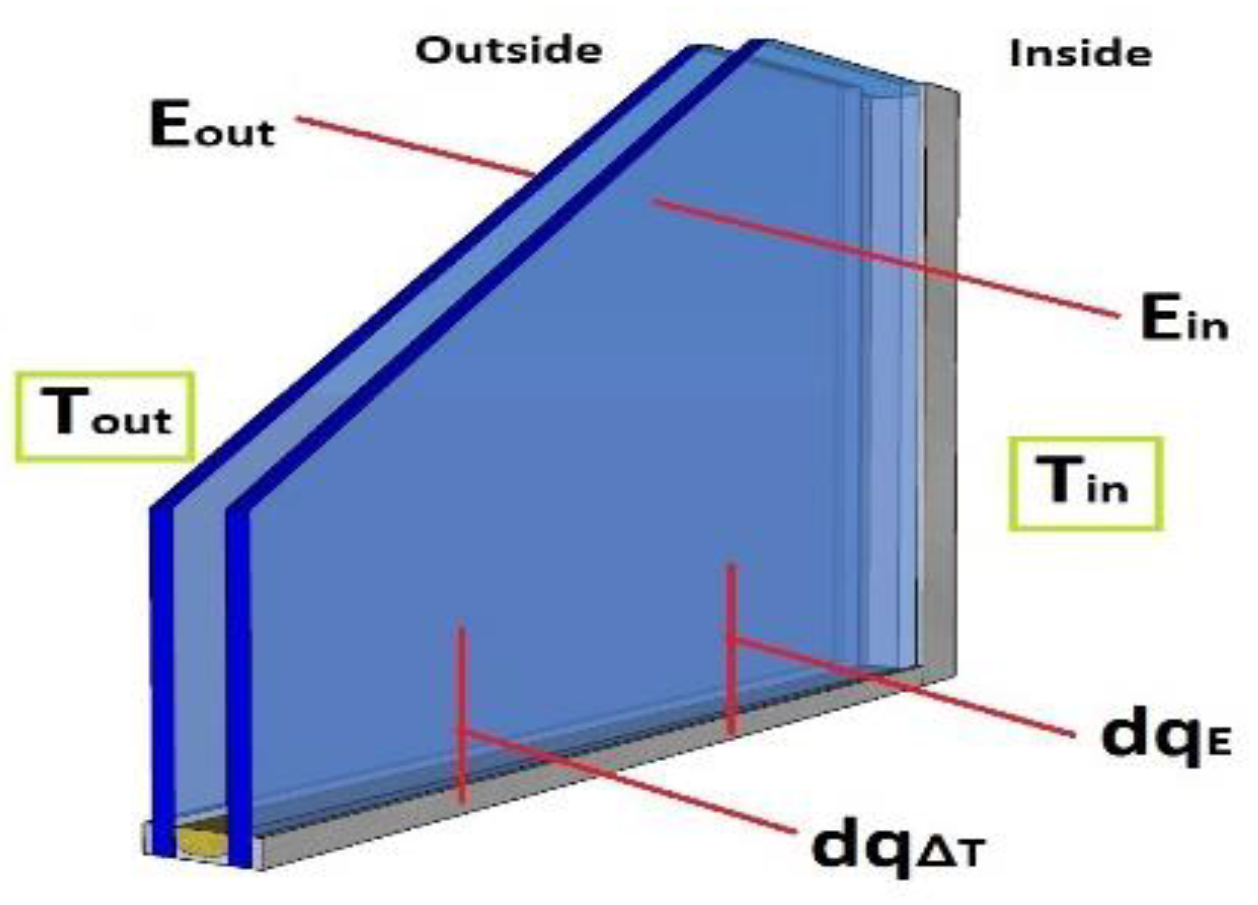
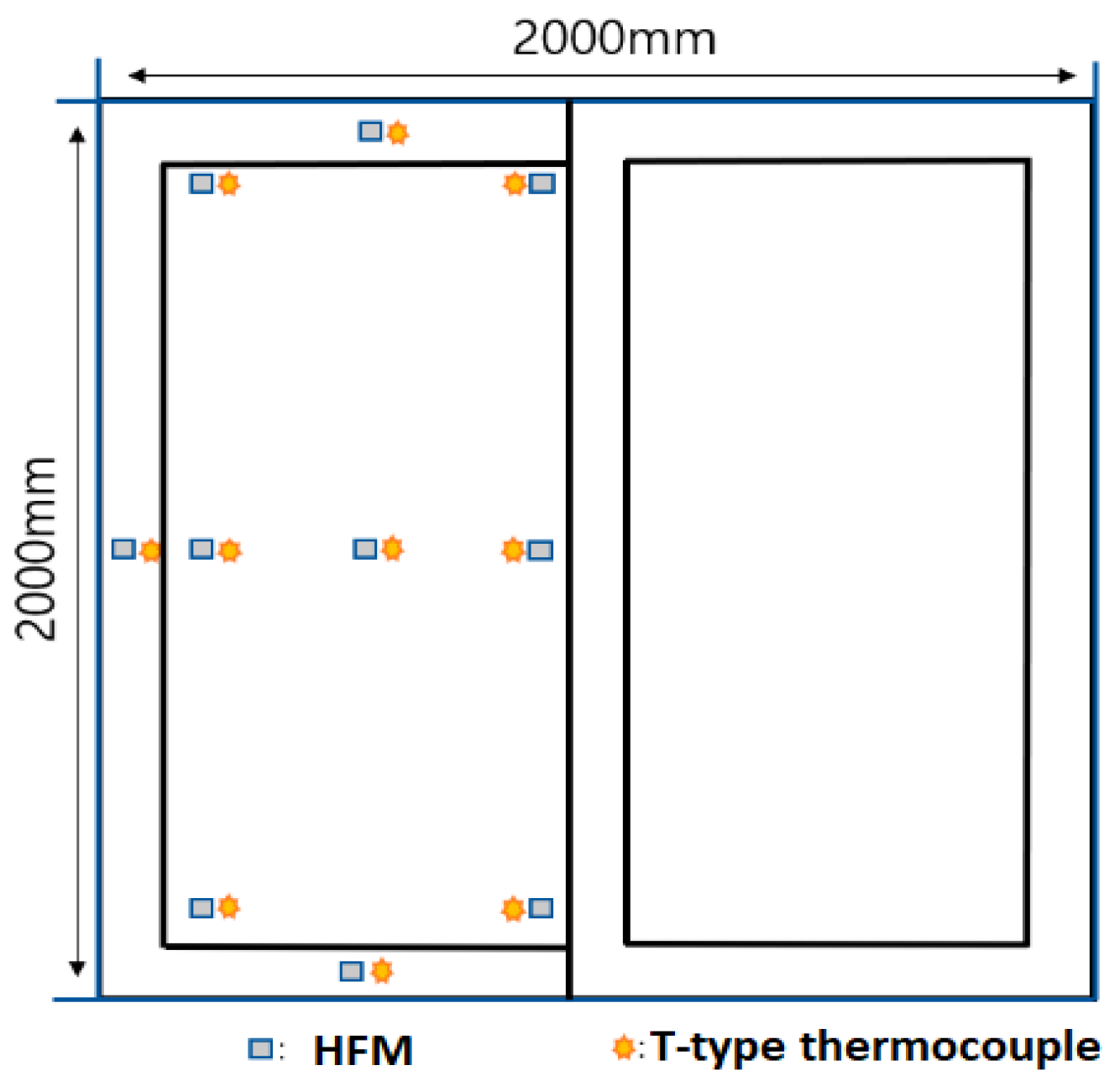
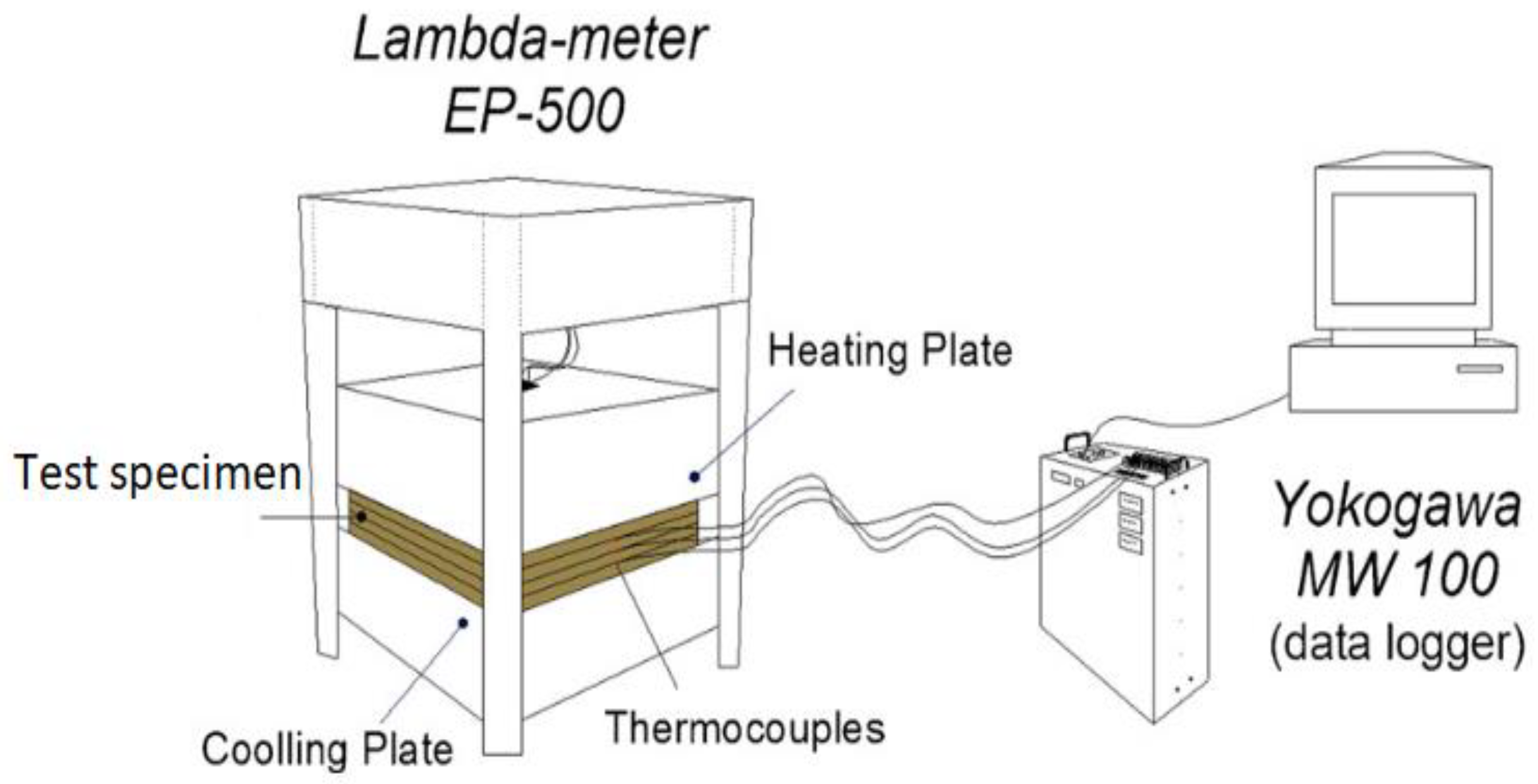

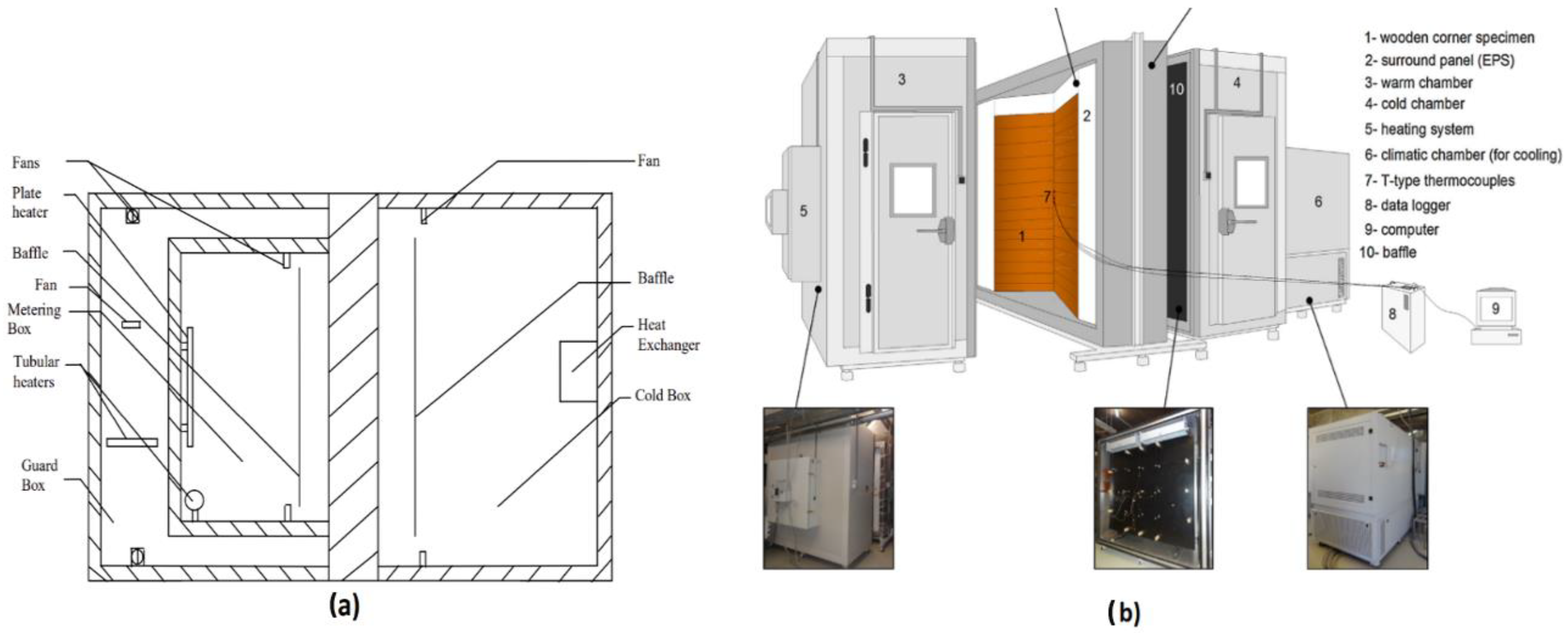
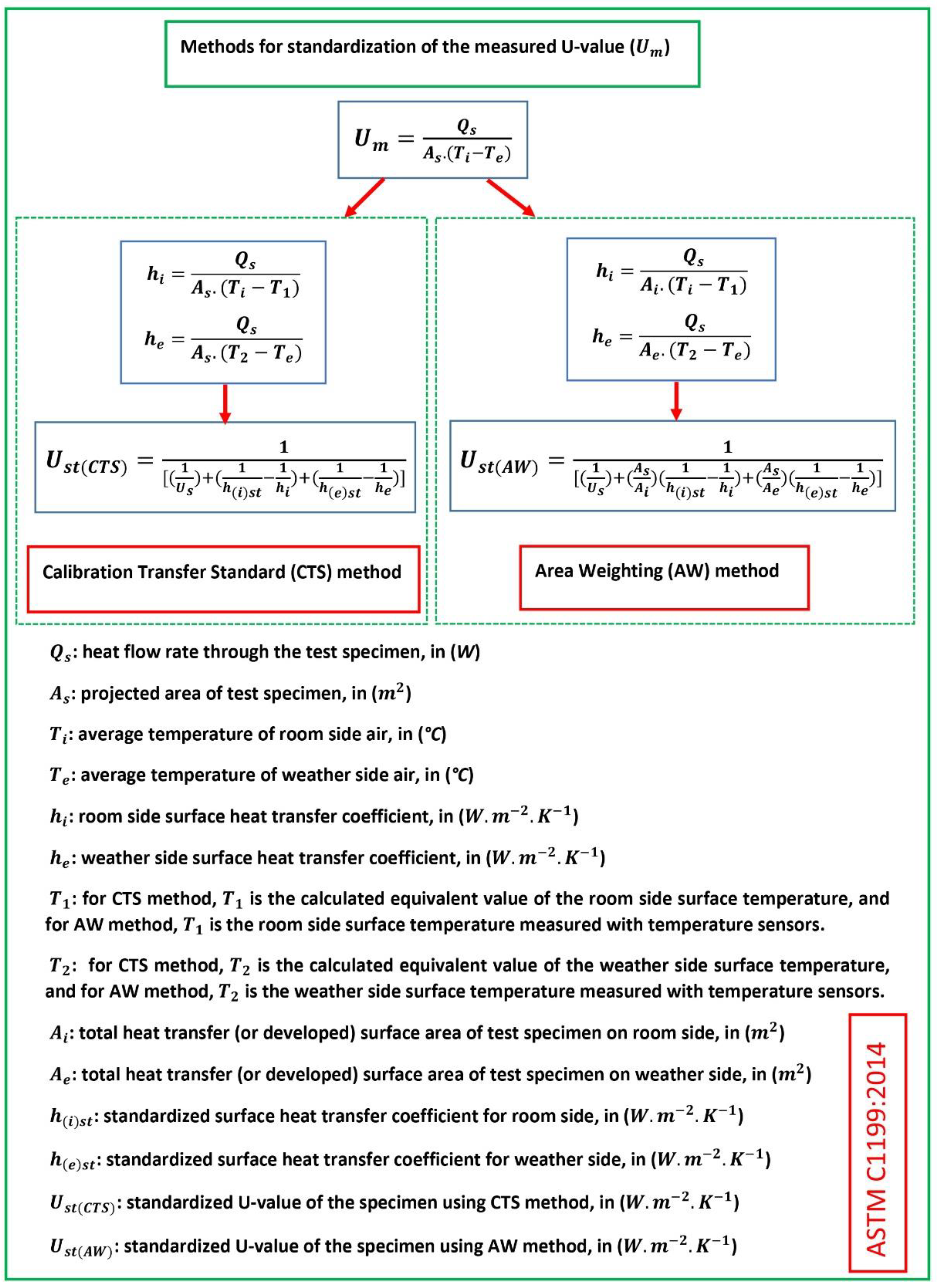
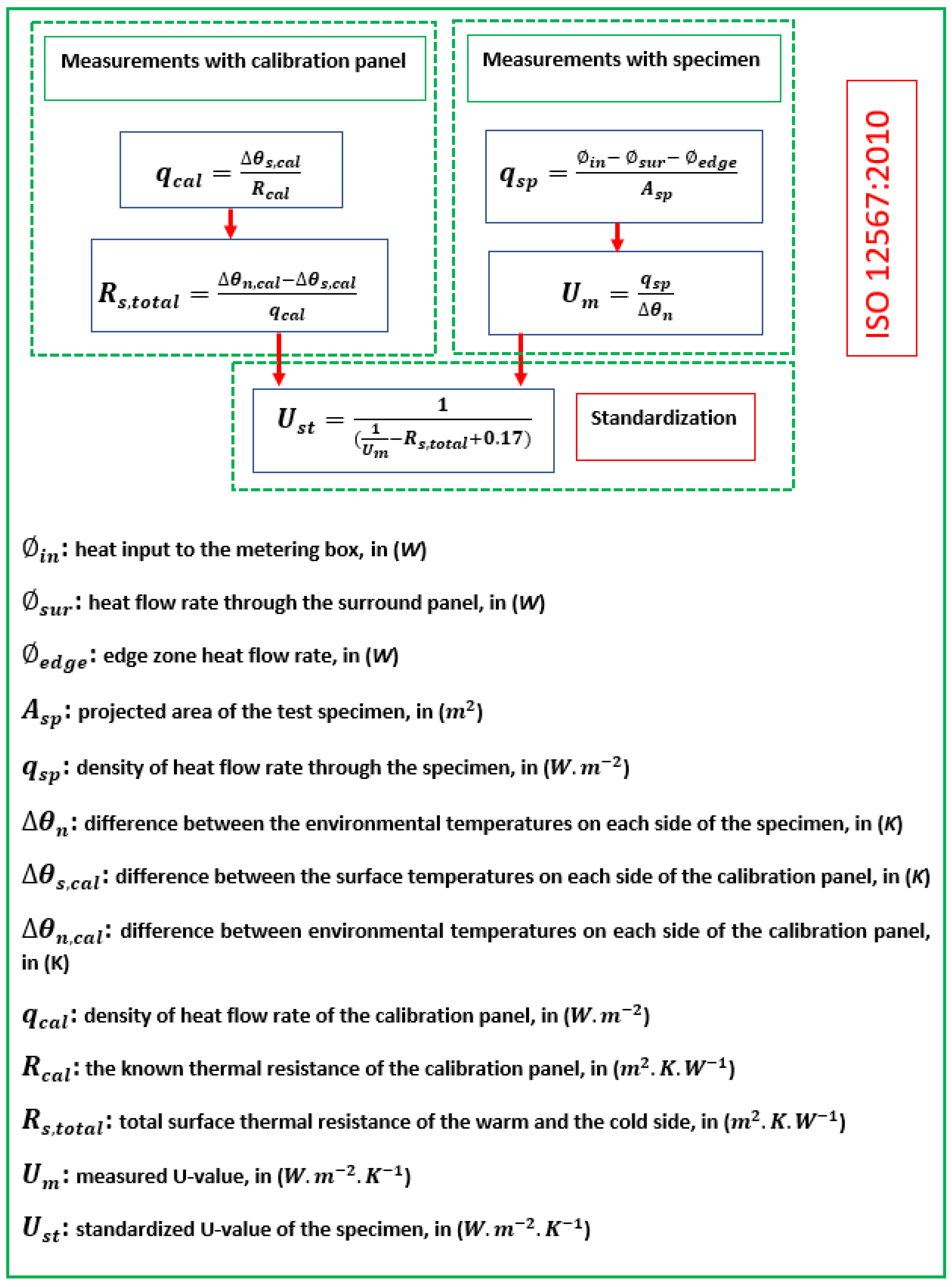

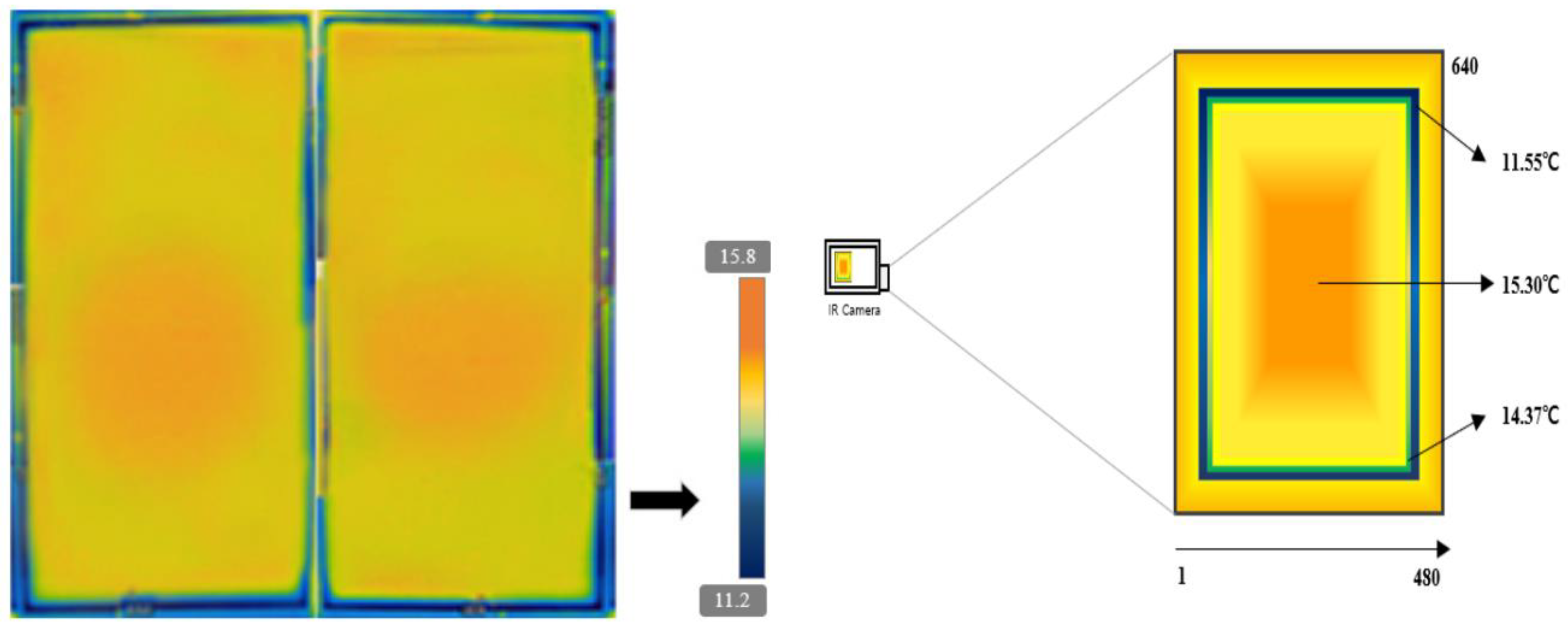


| Subject | Comment |
|---|---|
| Standards | For the HFM apparatus: EN 675:2011 [31] and ISO 10293:1997 [32]. For the in situ HFM method: ISO 9869-1:2014 [35] and ASTM C1155-95:2021 [36]. |
| Applicability of the HFM method | HFM apparatus: To determine the steady-state U-value of the glazing samples (not the entire window) in a laboratory environment [31,32]. In situ HFM method using portable sensors:
|
| Advantages | HFM apparatus:
|
| Disadvantages | HFM apparatus:
|
| Accuracy | HFM apparatus:
|
| Length of time of the test | HFM apparatus:
|
| Suggestions for the future |
|
| Subject | Comment |
|---|---|
| Standards | Standard ISO 10291:1994 [54], EN 674:2011 [55], and ISO 8302:1991 [59] |
| Applicability of the GHP method |
|
| Advantages | |
| Disadvantages |
|
| Accuracy | The accuracy of the method at room temperature and at a full temperature range would be expected to be 2% and 5%, respectively, showing a high level of accuracy [30,59,60]. |
| Length of time of the test | The time needed can vary between several minutes and several days based on the apparatus, the specimen, and their interactions [59]. Each test generally took a long time [61]. |
| Suggestions for the future |
|
| Subject | Comment |
|---|---|
| Standards | ISO 8990:1994 [65], ISO 12567:2010 [72], American ASTM C1363-05:2005 [66], ASTM C1199:2014 [64], and EN 12412-2:2003 [76]. |
| Applicability of the HB method |
|
| Advantages |
|
| Disadvantages |
|
| Accuracy | Giving reliable and accurate results with which the results of other experimental and theoretical methods can be compared for validation purposes [3,30,40,84]. |
| Length of time of the test | Relatively long measuring time, usually some days [30,85]. |
| Suggestions for the future |
|
| Subject | Comment |
|---|---|
| Standards | Standard ISO 9869-2: 2018 [98], however its primary focus is on opaque elements. |
| Applicability of the IRT method |
|
| Advantages | |
| Disadvantages |
|
| Accuracy | For the window system’s U-value determination using the IRT method, the standard deviations reported were between 3% and 20%, depending on measuring conditions [11,40,96]. |
| Length of time of the test | IRT enables a building element’s U-value to be measured in a short time, especially compared with the HFM method [30,40]. |
| Suggestions for the future |
|
| Measurement 1 | Measurement 2 | Measurement 3 | |
|---|---|---|---|
| Duration of the measurement | 7 h | 1 h | 1 h |
| Time to reach the defined quasi-steady state | 35 min | 35 min | 40 min |
| Difference between the outside and inside surface temperatures (°C) | 17.8 | 15 | 6 |
| Measured average U-value of the center part of the glazing () | 3.15 | 3.26 | 2.32 |
| Deviation of the measured U-value from the declared value (%) | +6.1% | +9.8% | −21.6% |
| Subject | HFM | GHP | HB | IRT | Rapid U-Value Meters | |
|---|---|---|---|---|---|---|
| HFM Apparatus | HFM Sensors | |||||
| In laboratory or In situ conditions | In laboratory | Both | In laboratory | In laboratory | Both | Both |
| Main advantages |
|
|
|
|
|
|
| Main disadvantage |
|
|
|
|
|
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Simões, N.; Moghaddam, S.A.; da Silva, M.G. Review of the Experimental Methods for Evaluation of Windows’ Thermal Transmittance: From Standardized Tests to New Possibilities. Buildings 2023, 13, 703. https://doi.org/10.3390/buildings13030703
Simões N, Moghaddam SA, da Silva MG. Review of the Experimental Methods for Evaluation of Windows’ Thermal Transmittance: From Standardized Tests to New Possibilities. Buildings. 2023; 13(3):703. https://doi.org/10.3390/buildings13030703
Chicago/Turabian StyleSimões, Nuno, Saman Abolghasemi Moghaddam, and Manuel Gameiro da Silva. 2023. "Review of the Experimental Methods for Evaluation of Windows’ Thermal Transmittance: From Standardized Tests to New Possibilities" Buildings 13, no. 3: 703. https://doi.org/10.3390/buildings13030703
APA StyleSimões, N., Moghaddam, S. A., & da Silva, M. G. (2023). Review of the Experimental Methods for Evaluation of Windows’ Thermal Transmittance: From Standardized Tests to New Possibilities. Buildings, 13(3), 703. https://doi.org/10.3390/buildings13030703








