Mechanical Properties of Prefabricated Cold-Formed Steel Stud Wall Panels Sheathed with Fireproof Phenolic Boards under Out-of-Plane Loading
Abstract
1. Introduction
2. Experimental Program
2.1. Experimental Parameters and Plan
2.2. Test Specimen
2.3. Material Properties
2.4. Loading Plan
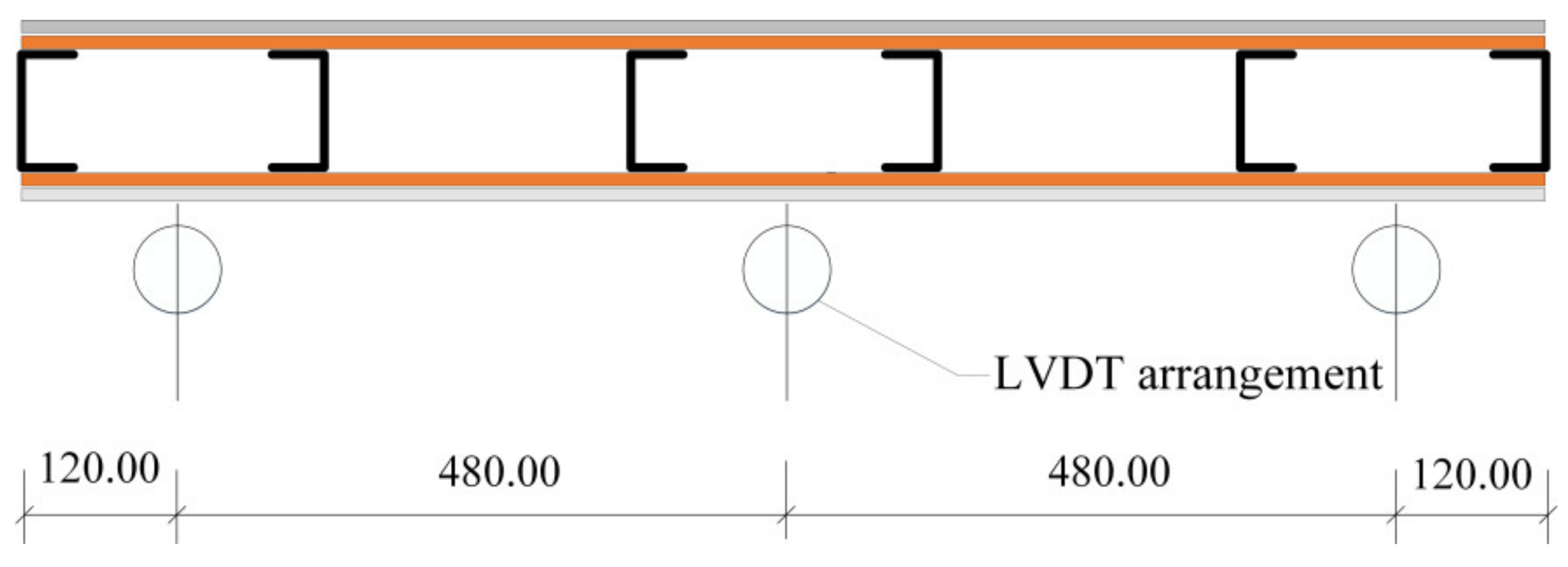
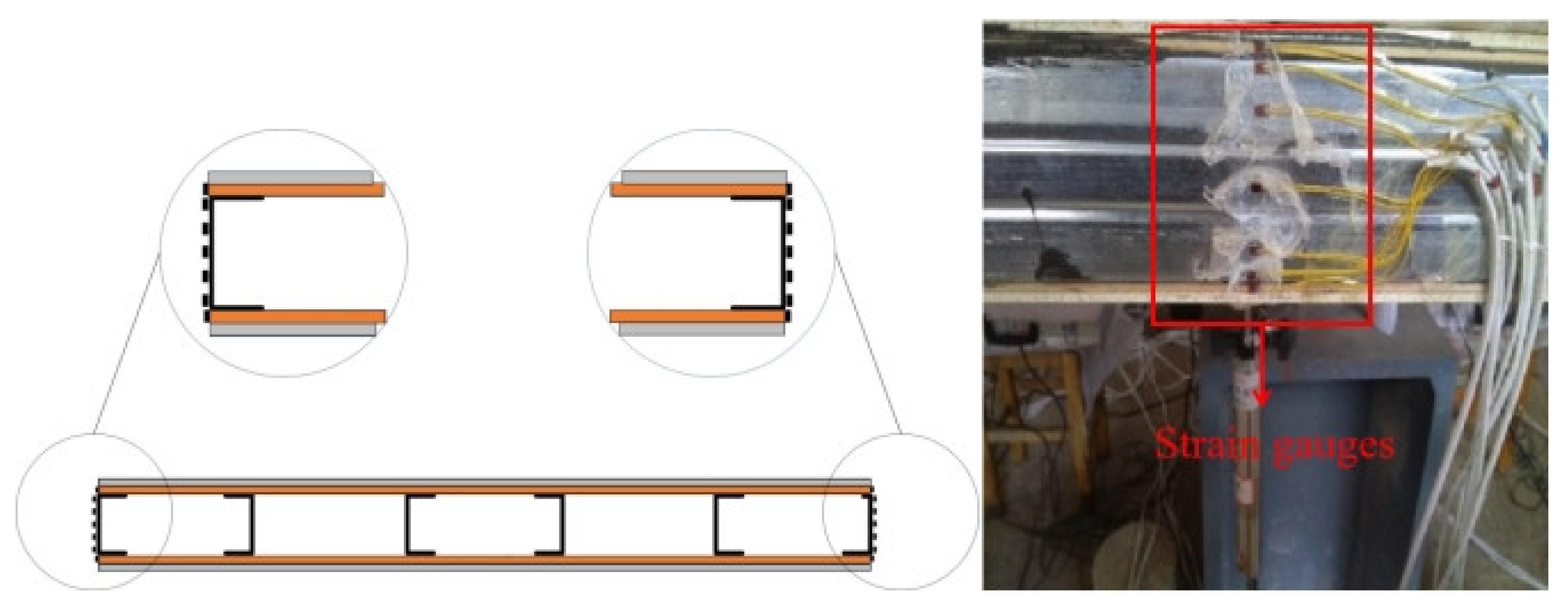
2.5. Measuring Instrument Arrangement
3. Results and Discussion
3.1. Experimental Phenomena
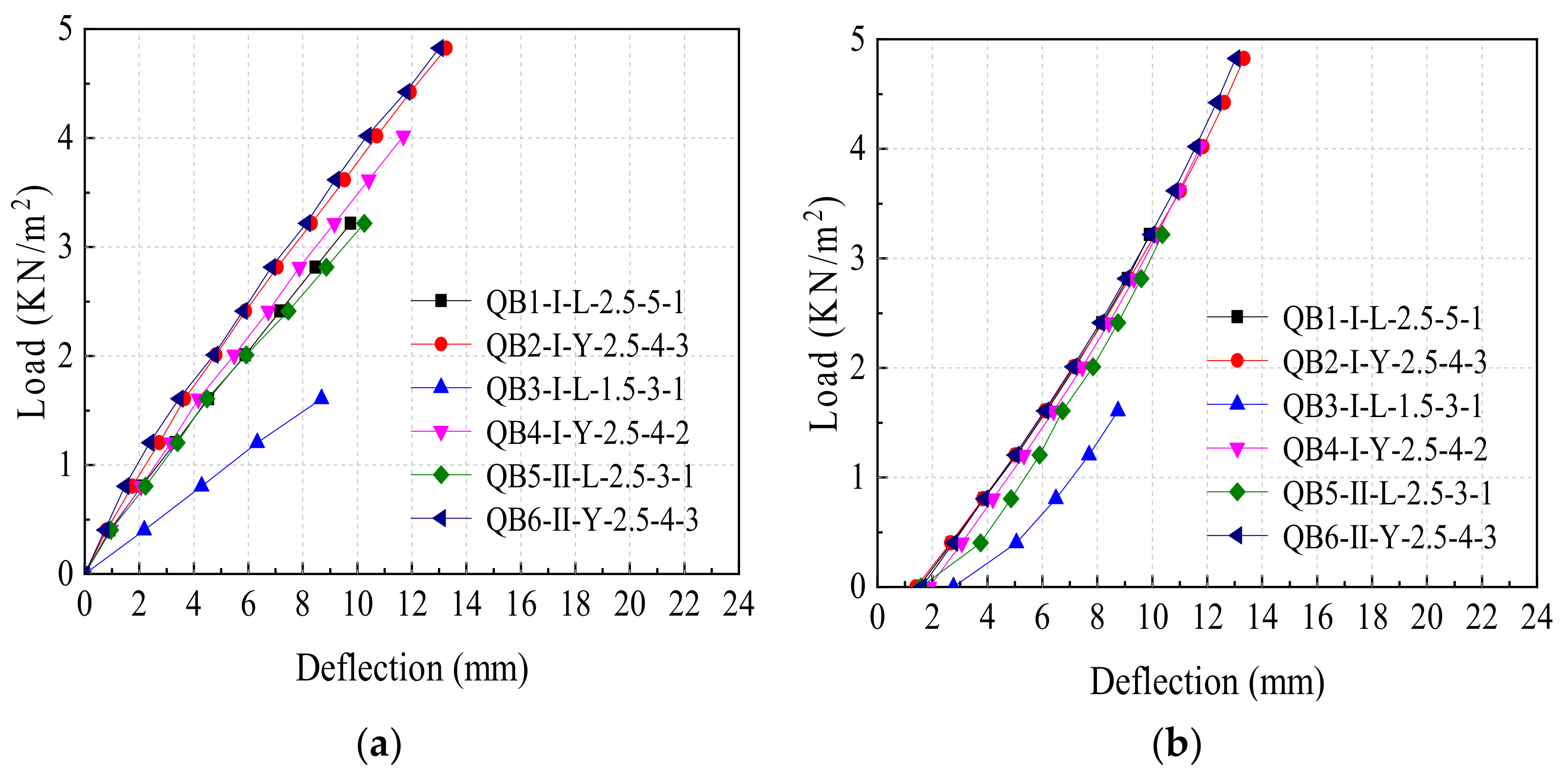
3.2. Load-Span Deflection Curve
3.3. Bending Stiffness
3.3.1. Arrangement of Light-Gauge Steel Studs
3.3.2. Connection Mode
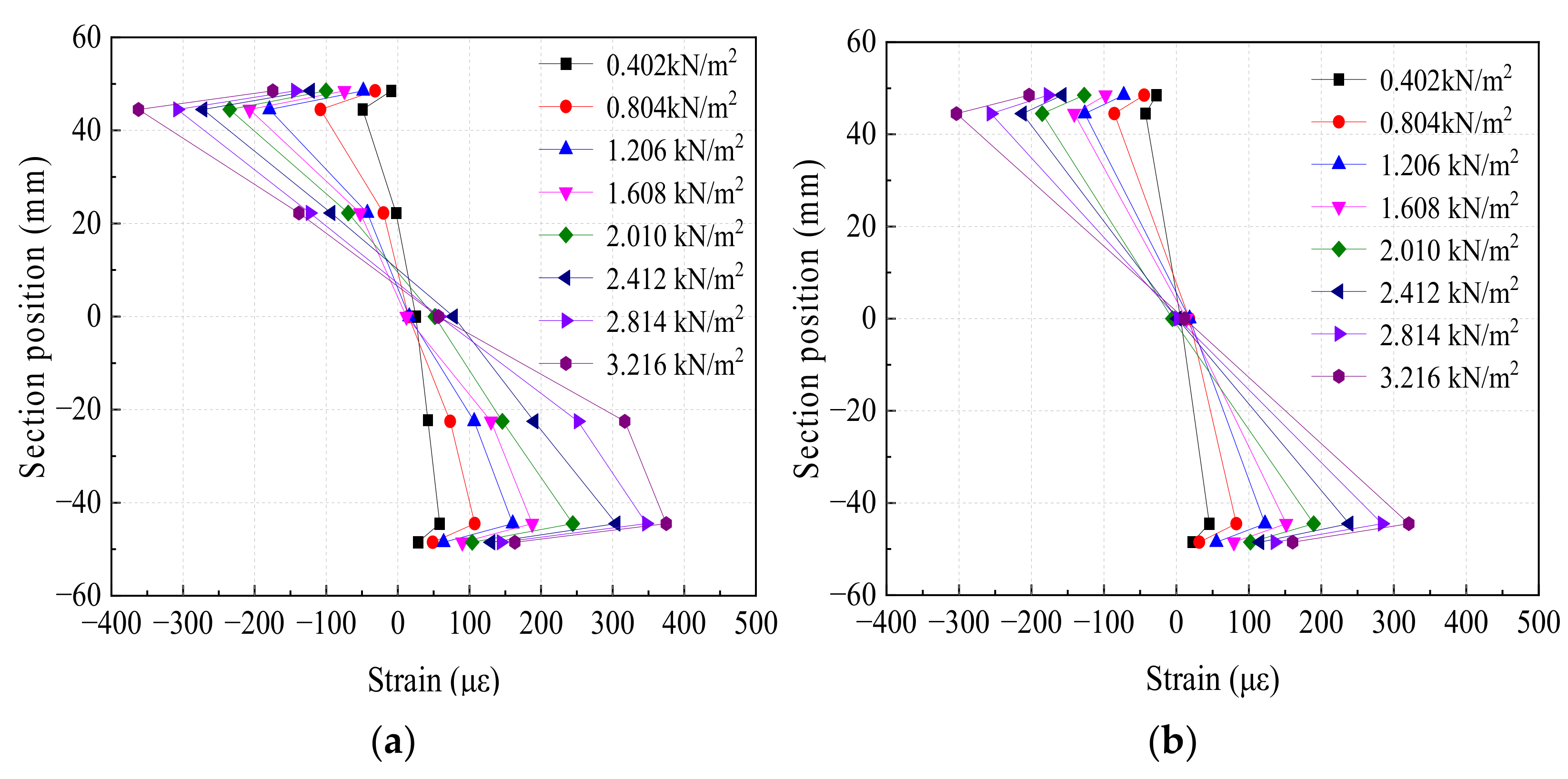
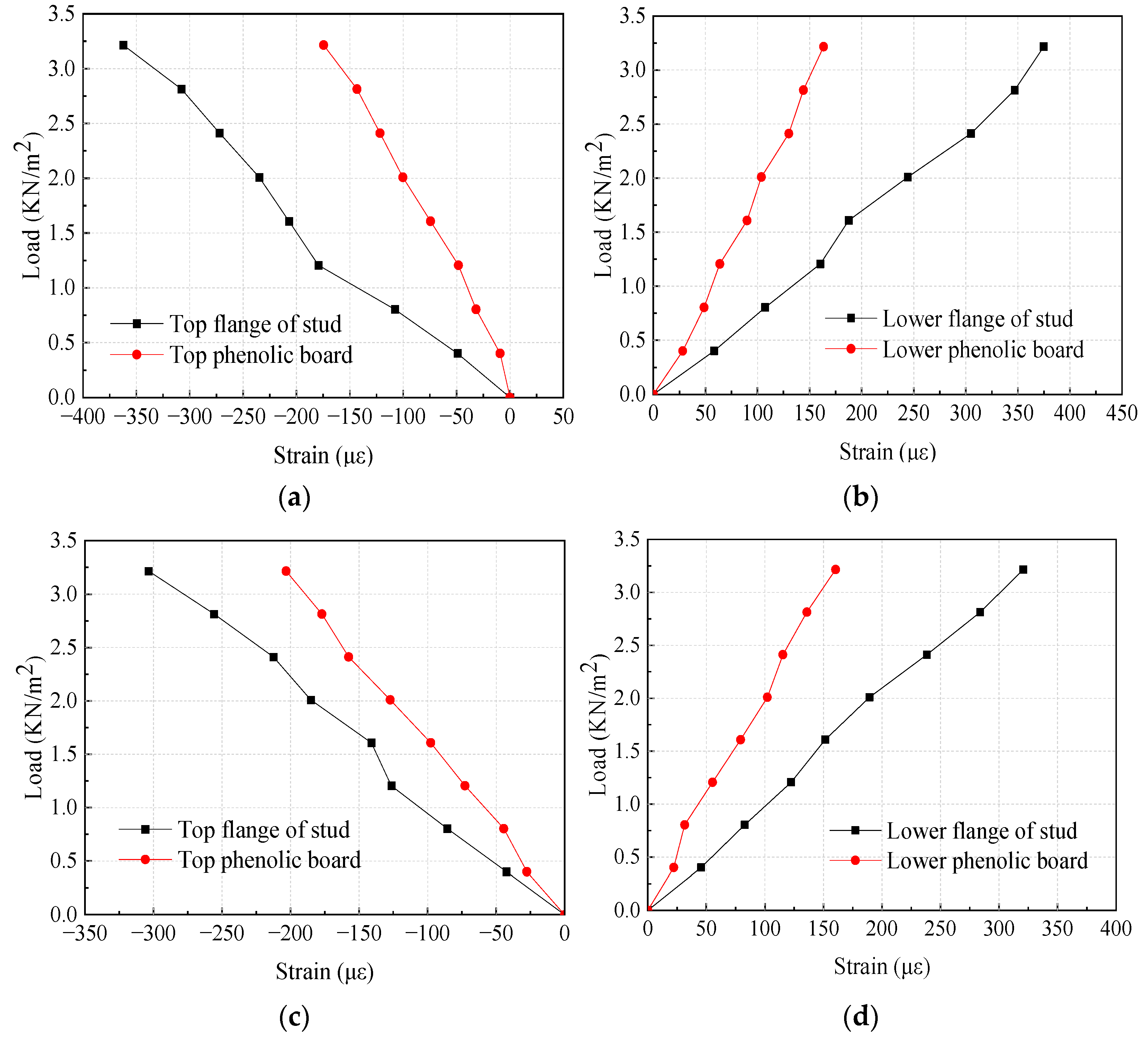
3.4. Strain Distribution
4. Theoretical Study of the Stress of LSF Wall Panels
4.1. Symmetric Slip Theory
4.1.1. The Basic Assumptions
- (1)
- Fireproof phenolic boards, silicone sealant, and cold-formed light-gauge steel studs are all elastomers. Phenolic boards and the light-gauge steel studs are in continuous and uniform contact through the silicone sealant in the longitudinal direction and maintain the same curvature. Furthemore, the longitudinal slip is a linear elastic process.
- (2)
- Under the condition of small elastic deformation, the top and lower interfaces are stressed symmetrically.
- (3)
- The out-of-plane stiffness, the bending moment, and the shear stress of the fireproof phenolic board can be ignored.
4.1.2. Differential Equation of Symmetrical Slip
4.1.3. Solution of the Mechanical Characteristic Quantity of Interface Slip under Load
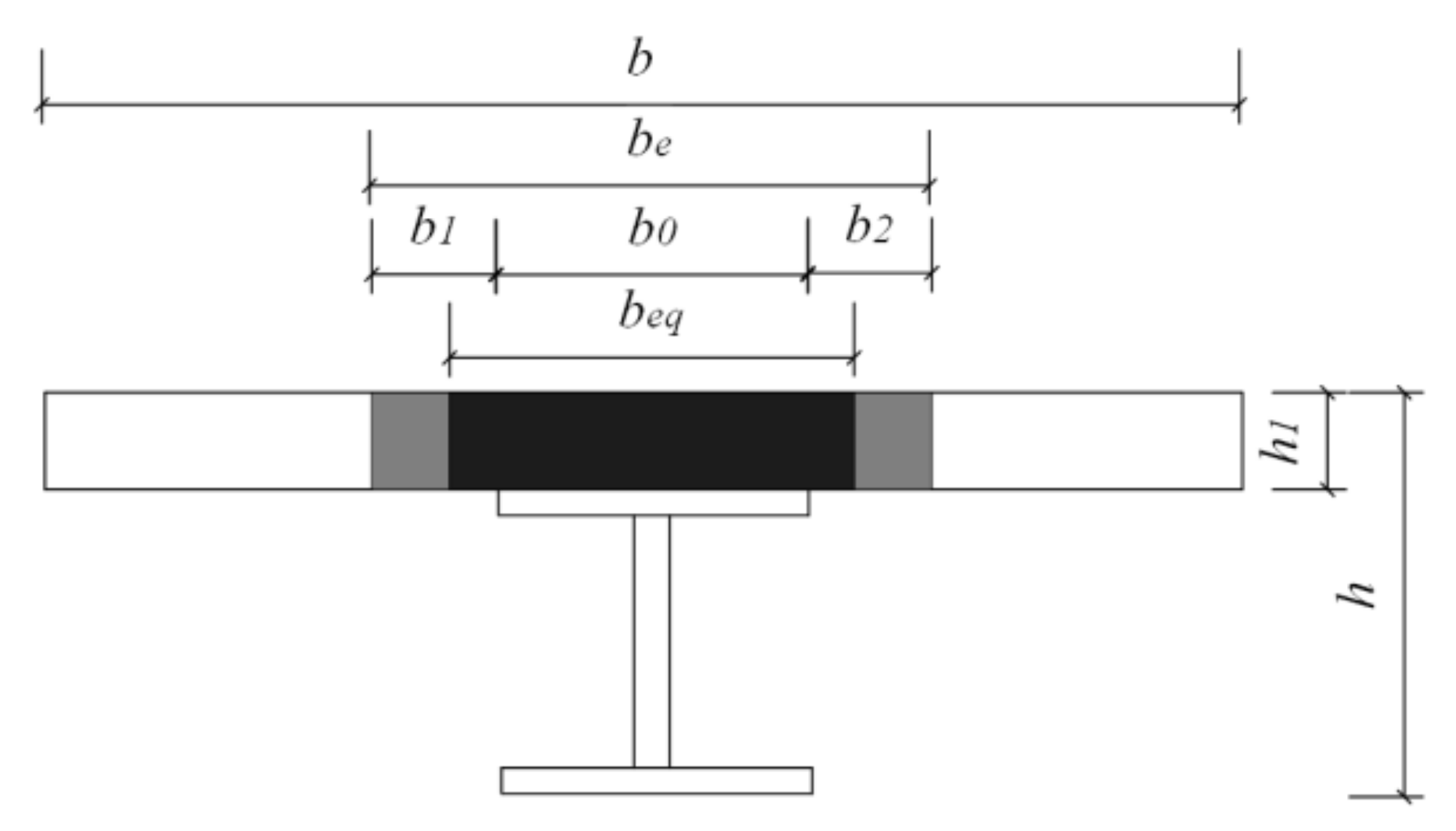
4.1.4. Composite Section Stiffness and Sheathing Effect Coefficient of LSF Wall Panels
4.2. Calculation of Elastic Deflection of LSF Wall Panels and Bending Normal Stress of Longitudinal Light-Gauge Steel Studs under Wind Load
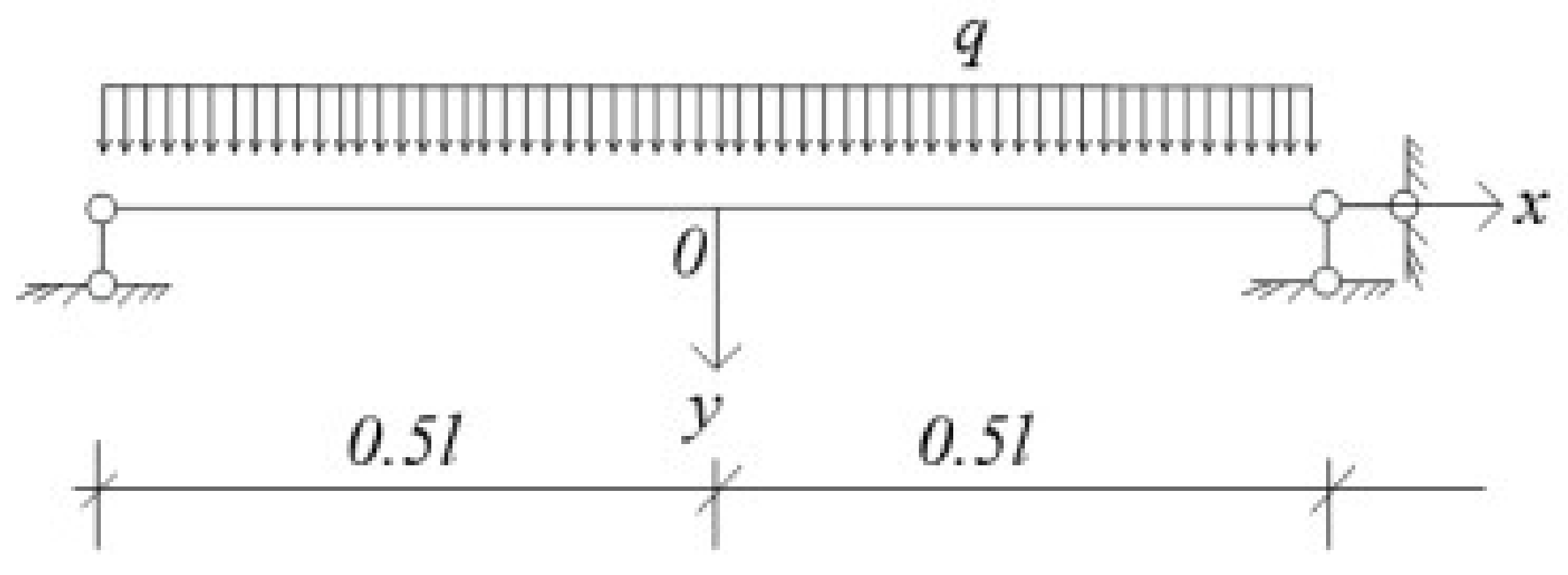
4.2.1. Calculation of Mid-Span Elastic Deflection of LSF Wall Panels
4.2.2. Calculation of Elastic Bending Normal Stress of Longitudinal Light-Gauge Steel Studs
4.2.3. Strength Checking of the Adhesive Layer between Phenolic Boards and Light-Gauge Steel Studs
4.3. Comparison between the Proposed Method and the Existing Methods
4.3.1. Transformed-Section Method without Considering Interface Slip
4.3.2. Reduced Stiffness Method Considering Interface Slip
4.3.3. Comparison of Calculated Results
5. Conclusions
- In the elastic stress stage under out-of-plane loads, the asymmetric arrangement of light-gauge steel studs of the LSF wall does not affect the overall elastic bending stiffness of wall panels. Thus, the symmetrical arrangement is recommended for practical applications.
- In the elastic stress stage under out-of-plane loads, increasing the thickness of the L-shaped connect-or and the number of self-tapping screws can improve the out-of-plane stiffness of the prefabricated LSF wall system. The overall out-of-plane stiffness of the prefabricated LSF wall system and the stiffness contribution of a single special-shaped connector can be increased by increasing the number of special-shaped connectors from two to three. The symmetrical arrangement of light-gauge steel studs of LSF wall is beneficial to improving the out-of-plane stiffness of the connectors. In addition, special-shaped connectors have greater out-of-plane stiffness than L-shaped connectors.
- Based on the sheathing effect of the wall panel and the symmetric slip theory of the interface between the fireproof phenolic board and light-gauge steel studs, the equations for calculating the elastic deflection in span and the elastic bending stress of light-gauge steel studs of the LSF wall are derived theoretically under out-of-plane loading. Compared with the transformed-section method and the reduced stiffness method in China’s steel structure design standards, the results obtained from the calculation of the method proposed in this paper are closer to the experimentally measured data.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sutarja, I.N.; Ardana, M.D.W.; Putra, I.D.G.A.D. The Post-disaster House: Simple Instant House using Lightweight Steel Structure, Bracing, and Local Wood Wall. Int. J. Eng. 2021, 34, 348–354. [Google Scholar] [CrossRef]
- Nadim, W.; Goulding, J.S. Offsite production in the UK: The way forward? a UK construction industry perspective. Constr. Innov. 2010, 10, 181–202. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, Y.-Q.; Gao, W.-J.; Kuroki, S. Life cycle environmental and cost performance of prefabricated buildings. Sustainability 2020, 12, 2609. [Google Scholar] [CrossRef]
- Chiang, Y.H.; Chan, E.H.W.; Ka-Leung Lok, L. Prefabrication and barriers to entry-a case study of public housing and institutional buildings in Hong Kong. Habitat Int. 2006, 30, 482–499. [Google Scholar] [CrossRef]
- Hong, J.-K.; Shen, Q.-P.; Li, Z.-D.; Zhang, B.-Y.; Zhang, W.-Q. Barriers to promoting prefabricated construction in china: A cost–benefit analysis. J. Clean. Prod. 2018, 172, 649–660. [Google Scholar] [CrossRef]
- Liu, W.-H.; Zhang, H.; Wang, Q.; Hua, T.-R.; Xue, H. A review and scient metric analysis of global research on prefabricated buildings. Adv. Civ. Eng. 2021, 2021, 8869315. [Google Scholar] [CrossRef]
- Zhou, M.; Chen, Y.-Y.; Su, X.-L.; An, L. Rapid construction and advanced technology for a COVID-19 field hospital in Wuhan, China. Civ. Eng. 2021, 174, 29–34. [Google Scholar] [CrossRef]
- Bhatti, A.Q.; Wahab, A. Analysis and design of emergency field isolation hospital building using innovative rapidly construction prefabricated units to treat patients infected with COVID-19. Innov. Infrastruct. Solut. 2021, 6, 90. [Google Scholar] [CrossRef]
- Chinese Standard GB/T51232-2016; Technical Standard for Prefabricated Steel Structure Building. China Architecture and Building Press: Beijing, China, 2016.
- Chinese Standard JG/T544-2018; Light-Gauge Steel Framing Wall Panel. Standards Press of China: Beijing, China, 2018.
- Vitale, P.; Spagnuolo, A.; Lubritto, C.; Arena, U. Environmental performances of residential buildings with a structure in cold formed steel or reinforced concrete. J. Clean. Prod. 2018, 189, 839–852. [Google Scholar] [CrossRef]
- Dani, A.A.; Roy, K.; Masood, R.; Fang, Z.; Lim, J.B.P. A Comparative Study on the Life Cycle Assessment of New Zealand Residential Buildings. Buildings 2022, 12, 50. [Google Scholar] [CrossRef]
- Dias, Y.; Mahendran, M. Shape optimisation of cold-formed steel framed wall studs with sheathing restraints. Thin-Walled Struct. 2020, 158, 107135. [Google Scholar] [CrossRef]
- Roy, K.; Lau, H.H.; Ting, T.; Chen, B.; Lim, J.B.P. Flexural capacity of gapped built-up cold-formed steel channel sections including web stiffeners. J. Constr. Steel Res. 2020, 172, 106154. [Google Scholar] [CrossRef]
- Chen, B.; Roy, K.; Fang, Z.; Uzzaman, A.; Chi, Y.; Lim, J.B.P. Web crippling capacity of fastened cold-formed steel channels with edge-stiffened web holes, un-stiffened web holes and plain webs under two-flange loading. Thin-Walled Struct. 2021, 163, 107666. [Google Scholar] [CrossRef]
- Ananthi, G.B.G.; Vishuvardhan, S.; Knight, G. Experimental, theoretical and numerical study on thin walled steel single and compound channel sections in axial compression. Indian J. Eng. Mater. Sci. 2015, 22, 570–580. [Google Scholar]
- Ananthi, G.B.G. A Study on Cold-Formed Steel Compound Angle Section Subjected to Axial Compression. KSCE J. Civ. Eng. 2018, 22, 1803–1815. [Google Scholar] [CrossRef]
- Ananthi, G.B.G.; Palani, G.S.; Iyer, N.R. Numerical and Theoretical Studies on Cold-Formed Steel Unlipped Channels Subjected to Axial Compression. Lat. Am. J. Solids Struct. 2015, 12, 1–17. [Google Scholar] [CrossRef]
- Roy, K.; Lim, J.B.P. Numerical investigation into the buckling behaviour of face-to-face built-up cold-formed stainless steel channel sections under axial compression. Structures 2019, 20, 42–73. [Google Scholar] [CrossRef]
- Ananthi, G.B.G.; Roy, K.; Lim, J.B.P. Tests and Finite Element Modelling of Cold-Formed Steel Zed and Hat Section Columns Under Axial Compression. Int. J. Steel Struct. 2021, 21, 1305–1331. [Google Scholar] [CrossRef]
- Ananthi, G.B.G.; Roy, K.; Lim, J.B.P. Experimental and numerical study of an innovative 4-channels cold-formed steel built-up column under axial compression. Steel Compos. Struct. 2022, 42, 513–538. [Google Scholar] [CrossRef]
- Ananthi, G.B.G.; Roy, K.; Lim, J.B.P. Behaviour and strength of back-to-back built-up cold-formed steel unequal angle sections with intermediate stiffeners under axial compression. Steel Compos. Struct. 2022, 42, 1–22. [Google Scholar]
- Shi, Y.; Ran, X.-W.; Xiao, W.; Ke, K.; Xiang, Y.; Deng, R. Experimental and numerical study of the seismic behavior of cold-formed steel walls with diagonal braces. Thin-Walled Struct. 2020, 159, 107318. [Google Scholar] [CrossRef]
- Wu, H.-H.; Sui, L.; Zhou, T.-H.; Lu, L.-F.; Li, X.-H. Estimation of lateral stiffness for gypsum-filled cold-formed steel shear walls. Structures 2021, 32, 28–37. [Google Scholar] [CrossRef]
- Ariyanayagam, A.D.; Mahendran, M. Fire tests of non-load bearing light gauge steel frame walls lined with calcium silicate boards and gypsum plasterboards. Thin-Walled Struct. 2017, 115, 86–99. [Google Scholar] [CrossRef]
- Selvaraj, S.; Madhavan, M. Criteria for selection of sheathing boards in cold-formed steel wall panels subjected to bending: Construction applications and performance-based evaluation. Pract. Period. Struct. Des. Constr. 2021, 26, 04020044. [Google Scholar] [CrossRef]
- Gunalan, S.; Mahendran, M. Experimental investigation of post-fire mechanical properties of cold-formed steels. Thin-Walled Struct. 2014, 84, 241–254. [Google Scholar] [CrossRef]
- Batista Abreu, J.C.; Vieira, L.M.C.; Abu-Hamd, M.H.; Schafer, B.W. Review: Development of performance-based fire design for cold-formed steel. Fire Sci. Rev. 2014, 3, 1. [Google Scholar] [CrossRef]
- Keerthan, P.; Mahendran, M. Numerical modelling of non-load-bearing light gauge cold-formed steel frame walls under fire conditions. J. Fire Sci. 2012, 30, 375–403. [Google Scholar] [CrossRef]
- Ranawaka, T.; Mahendran, M. Experimental study of the mechanical properties of light gauge cold-formed steels at elevated temperatures. Fire Saf. J. 2009, 44, 219–229. [Google Scholar] [CrossRef]
- Imran, M.; Mahendran, M.; Keerthan, P. Mechanical properties of cold-formed steel tubular sections at elevated temperatures. J. Constr. Steel Res. 2018, 143, 131–147. [Google Scholar] [CrossRef]
- Fang, Z.; Roy, K.; Liang, H.; Poologanathan, K.; Ghosh, K.; Mohamed, A.M.; Lim, J.B.P. Numerical Simulation and Design Recommendations for Web Crippling Strength of Cold-Formed Steel Channels with Web Holes under Interior-One-Flange Loading at Elevated Temperatures. Buildings 2021, 11, 666. [Google Scholar] [CrossRef]
- Steau, E.; Mahendran, M.; Poologanathan, K. Elevated temperature thermal properties of carbon steels used in cold-formed light gauge steel frame systems. J. Build. Eng. 2020, 28, 101074. [Google Scholar] [CrossRef]
- Li, Q.; Xia, M.-X.; Peng, H.; He, X.-J. Structural Silicone Sealant Aging Failure Analysis and the Practical Failure Model. Build. Energy Effic. 2016, 44, 30–34, 39. [Google Scholar]
- Buyl, F.D. Silicone sealants and structural adhesives. Int. J. Adhes. Adhes. 2001, 21, 411–422. [Google Scholar] [CrossRef]
- Telue, Y.; Mahendran, M. Behaviour of cold-formed steel wall frames lined with plasterboard. J. Constr. Steel Res. 2001, 57, 435–452. [Google Scholar] [CrossRef]
- Telue, Y.; Mahendran, M. Behaviour and design of cold-formed steel wall frames lined with plasterboard on both sides. Eng. Struct. 2004, 26, 567–579. [Google Scholar] [CrossRef]
- Nithyadharan, M.; Kalyanaraman, V. Behaviour of cold-formed steel shear wall panels under monotonic and reversed cyclic loading. Thin-Walled Struct. 2012, 60, 12–23. [Google Scholar] [CrossRef]
- Peterman, K.D. Experiments on the Stability of Sheathed Cold-Formed Steel Studs under Axial Load and Bending. Ph.D. Thesis, Johns Hopkins University, Baltimore, MD, USA, 2012. [Google Scholar]
- Selvaraj, S.; Madhavan, M. Studies on cold-formed steel stud panels with gypsum sheathing subjected to out-of-plane bending. J. Struct. Eng. 2018, 144, 100–117. [Google Scholar] [CrossRef]
- Selvaraj, S.; Madhavan, M. Bracing effect of sheathing in point-symmetric cold-formed steel flexural members. J. Constr. Steel Res. 2019, 157, 450–462. [Google Scholar] [CrossRef]
- Selvaraj, S.; Madhavan, M. Investigation on sheathing-fastener connection failures in cold-formed steel wall panels. Structures 2019, 20, 176–188. [Google Scholar] [CrossRef]
- Selvaraj, S.; Madhavan, M. Investigation on sheathing effect and failure modes of gypsum sheathed cold-formed steel wall panels subjected to bending. Structures 2019, 17, 87–101. [Google Scholar] [CrossRef]
- Chinese Standard GB/T228.1-2010; Metallic Materials-Tensile Testing-Part 1: Method of Test at Room Temperature. Standards Press of China: Beijing, China, 2010.
- ASTM D638-2008; Standard Test Method for Tensile Properties of Plastics. ASTM International: West Conshohocken, PA, USA, 2008.
- Chinese Standard GB50009-2012; Load Code for the Design of Building Structures. China Architecture and Building Press: Beijing, China, 2012.
- Yang, Y.-Z. Study on the Properties of the Light-Gauge Steel Truss Girder-OSB Composite Floor. Ph.D. Thesis, Chongqing University, Chongqing, China, 2014. [Google Scholar]
- Shen, H.-S.; Teng, J.-G.; Yang, J. Interfacial stresses in beams and slabs bonded with thin plate. J. Eng. Mech. 2001, 127, 399–406. [Google Scholar] [CrossRef]
- Smith, S.T.; Teng, J.-G. Interfacial stresses in plated beams. Eng. Struct. 2001, 23, 857–871. [Google Scholar] [CrossRef]
- Yang, J.; Ye, J.-Q. An improved closed-form solution to interfacial stresses in plated beams using a two-stage approach. Int. J. Mech. Sci. 2010, 52, 13–30. [Google Scholar] [CrossRef][Green Version]
- Liu, M.; Dawood, M. A closed-form solution of the interfacial stresses and strains in steel beams strengthened with externally bonded plates using ductile adhesives. Eng. Struct. 2018, 154, 66–77. [Google Scholar] [CrossRef]
- Stratford, T.; Cadei, J. Elastic analysis of adhesion stresses for the design of a strengthening plate bonded to a beam. Constr. Build. Mater. 2006, 20, 34–45. [Google Scholar] [CrossRef]
- Narayanamurthy, V.; Chen, J.F.; Cairns, R. A general analytical method for the analysis of interfacial stresses in plated beams under arbitrary loading. Adv. Struct. Eng. 2010, 13, 975–988. [Google Scholar] [CrossRef]
- Teng, J.-G.; Yu, T.; Fernando, D. Strengthening of steel structures with fiber-reinforced polymer composites. J. Constr. Steel Res. 2012, 78, 131–143. [Google Scholar] [CrossRef]
- Chinese Standard GB51367-2019; Standard for Design of Strengthening Steel Structure. China Architecture and Building Press: Beijing, China, 2019.
- Sun, X.-F. Mechanics of Materials, 6th ed.; Higher Education Press: Beijing, China, 2009; ISBN 9787040513622. [Google Scholar]
- Xue, J.-Y. Design Principle of Steel and Concrete Composite Structure; Science Press: Beijing, China, 2010; ISBN 9787030275660. [Google Scholar]
- Nie, J.-G.; Cai, C.-S.; Wang, T. Stiffness and capacity of steel-concrete composite beams with profiled sheeting. Eng. Struct. 2005, 27, 1074–1085. [Google Scholar] [CrossRef]
- Nie, J.-G.; Cai, C.-S. Steel–concrete composite beams considering shear slip effects. J. Struct. Eng. 2003, 129, 495–506. [Google Scholar] [CrossRef]
- Chinese Standard GB 50017-2017; Code for Design of Steel Structures. China Planning Press: Beijing, China, 2017.
- Nie, J.-G. Steel Concrete Composite Beam Structure-Experiment, Theory and Application; Science Press: Beijing, China, 2004; ISBN 9787030152893. [Google Scholar]
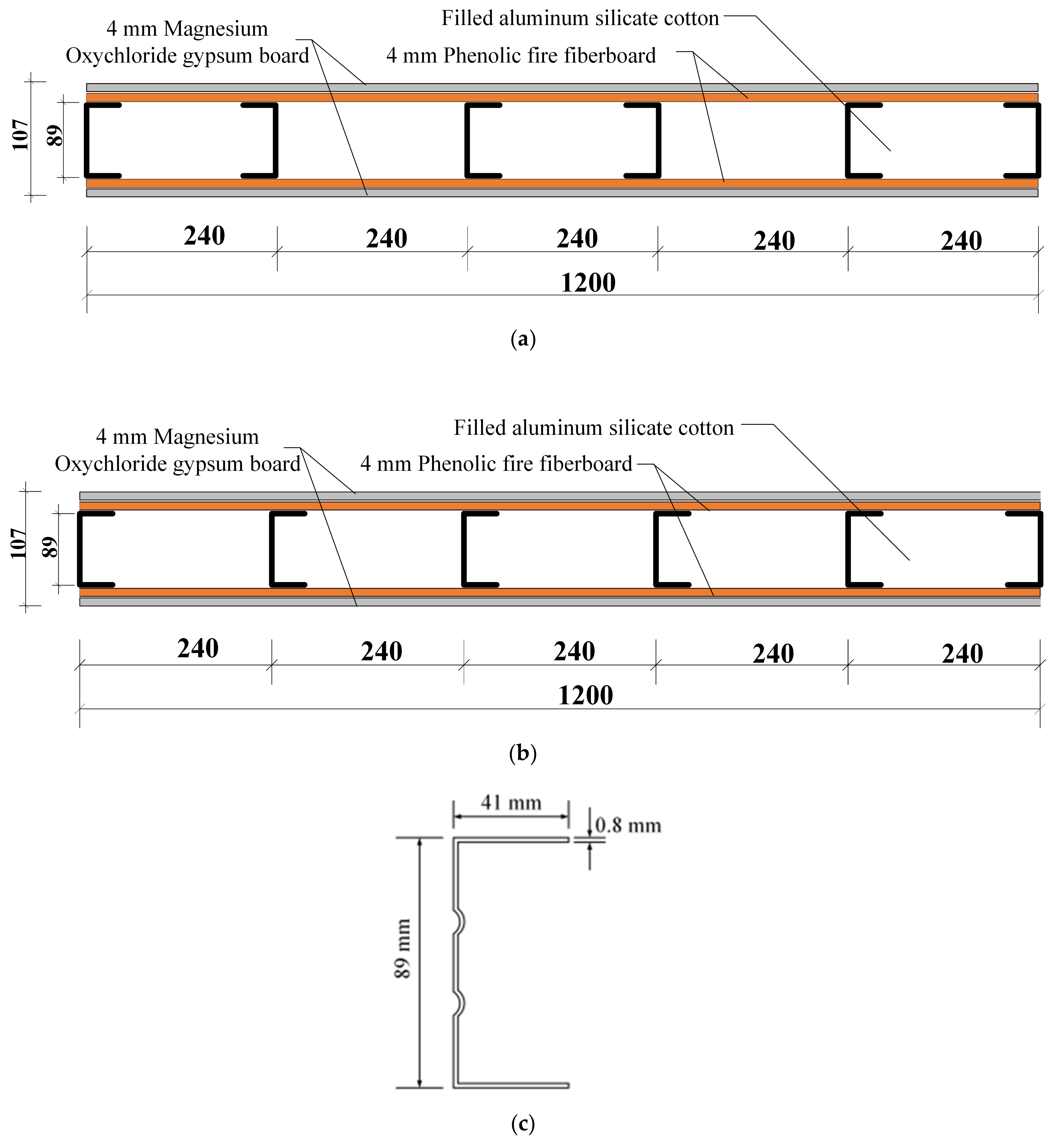

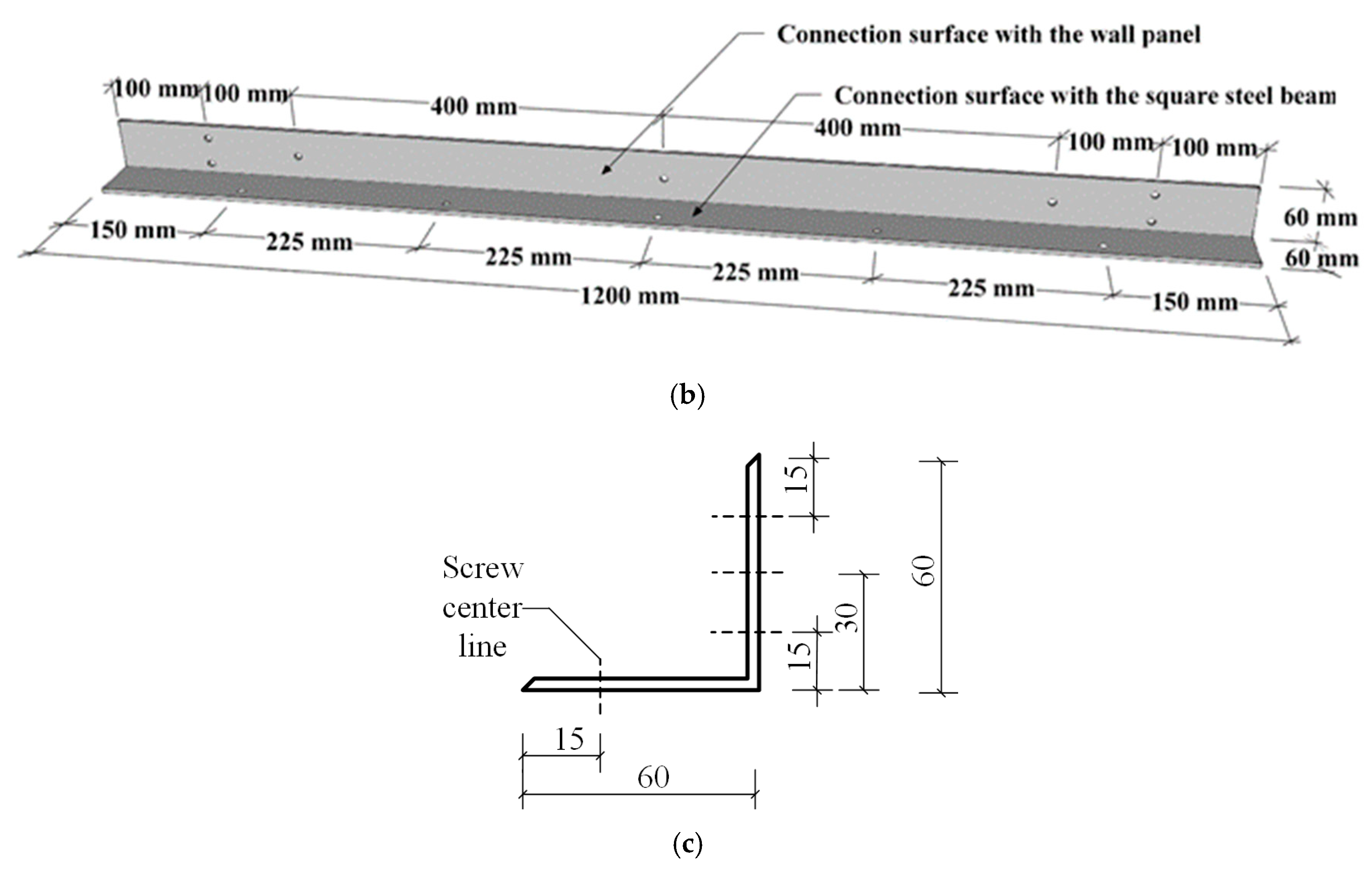

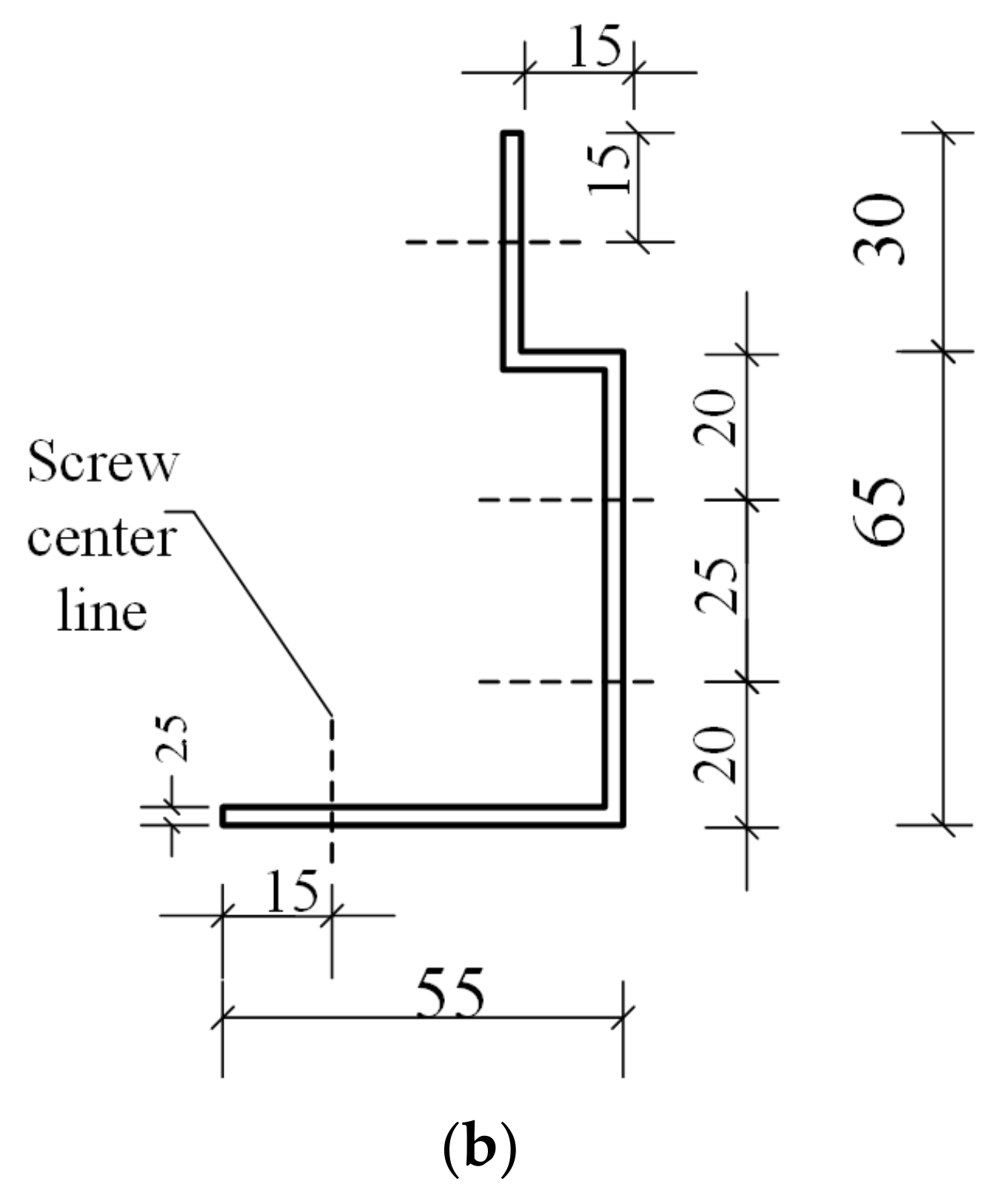

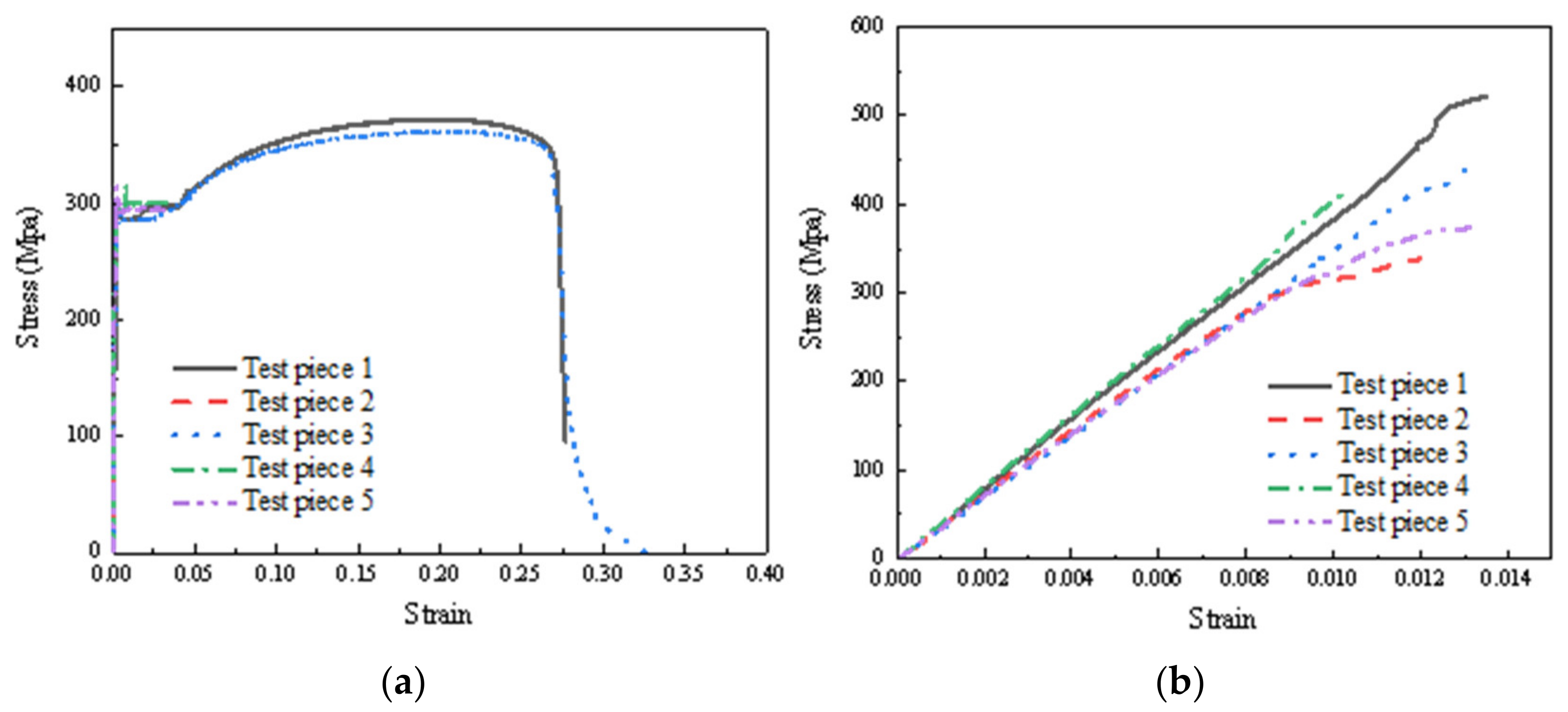








| Specimen | Wall Type | Connector Section | Thickness of Connector (mm) | Number of Single Side Connectors | Number of Single Side Screws Connected to Steel Beams |
|---|---|---|---|---|---|
| QB1-I-L-2.5-5-1 | Type I | L-shape | 2.5 | 1 | 5 |
| QB2-I-Y-2.5-4-3 | Type I | Special-shaped | 2.5 | 3 | 12 |
| QB3-I-L-1.5-3-1 | Type I | L-shape | 1.5 | 1 | 3 |
| QB4-I-Y-2.5-4-2 | Type I | Special-shaped | 2.5 | 2 | 8 |
| QB5-II-L-2.5-3-1 | Type II | L-shape | 2.5 | 1 | 3 |
| QB6-II-Y-2.5-4-3 | Type II | Special-shaped | 2.5 | 3 | 12 |
| Material | Yield Stress (Mpa) | Ultimate Stress (Mpa) | Young’s Modulus (GPa) | Poisson’s Ratio |
|---|---|---|---|---|
| Cold-formed steel | 302.4 | 377.3 | 178.3 | 0.32 |
| Fireproof phenolic board | / | 424.8 | 33.76 | 0.27 |
| Silicone sealant | / | 1.13 | 0.00067 | 0.50 |
| Loading Series | Loading Uniform Area Load (KN/m2) | Unloading Uniform Area Load (KN/m2) |
|---|---|---|
| 0-level | 0 | 4.824 |
| 1-level | 0.402 | 4.422 |
| 2-level | 0.804 | 4.020 |
| … | … | … |
| 11-level | 4.422 | 0.402 |
| 12-level | 4.824 | 0 |
| Specimen | Applying Maximum Load (KN/m2) | Loading Bending Stiffness of Specimen (KN·m2) | Unloading Bending Stiffness of Specimen (KN·m2) | Mid-Span Deflection (mm) |
|---|---|---|---|---|
| QB1-I-L-2.5-5-1 | 3.216 | 40753 | 47535 | 9.75 |
| QB2-I-Y-2.5-4-3 | 4.824 | 45166 | 52295 | 13.26 |
| QB3-I-L-1.5-3-1 | 1.608 | 23492 | 33666 | 8.68 |
| QB4-I-Y-2.5-4-2 | 4.020 | 42865 | 48616 | 11.70 |
| QB5-II-L-2.5-3-1 | 3.216 | 38882 | 47691 | 10.25 |
| QB6-II-Y-2.5-4-3 | 4.824 | 45662 | 52517 | 13.00 |
| Wall Specimen | Applying Maximum Load (KN/m2) | Mid-Span Deflection (mm) | Loading Bending Stiffness (KN·m2) | Unloading Bending Stiffness (KN·m2) |
|---|---|---|---|---|
| QB1-I-L-2.5-5-1 | 3.216 | 7.11 | 48866 | 52570 |
| QB2-I-Y-2.5-4-3 | 4.824 | 12.18 | 42980 | 46449 |
| QB3-I-L-1.5-3-1 | 1.608 | 4.96 | 31274 | 48852 |
| QB4-I-Y-2.5-4-2 | 4.020 | 9.73 | 45129 | 49175 |
| QBn-I average value | / | / | 42062 | 49262 |
| QB5-II-L-2.5-3-1 | 3.216 | 7.33 | 47463 | 47470 |
| QB6-II-Y-2.5-4-3 | 4.824 | 11.64 | 44029 | 47862 |
| QBn-II average value | / | / | 45746 | 47666 |
| Specimen | Elastic Bending Stiffness of Specimen (KN·m2) | Elastic Bending Stiffness of Wall Panel (KN·m2) | Ratio of Bending Stiffness of Specimen to the Wall Panel |
|---|---|---|---|
| QB1-I-L-2.5-5-1 | 47535 | 52570 | 0.904 |
| QB2-I-Y-2.5-4-3 | 52295 | 46449 | 1.126 |
| QB3-I-L-1.5-3-1 | 33666 | 48852 | 0.689 |
| QB4-I-Y-2.5-4-2 | 48616 | 49175 | 0.987 |
| QB5-II-L-2.5-3-1 | 47691 | 54131 | 0.881 |
| QB6-II-Y-2.5-4-3 | 52517 | 47862 | 1.097 |
| Specimen | Measured Bending Stiffness B0i (KN·m2) | Measured Conversion m0i | Calculated Value m | Ratio of Calculated Value to Measured Value |
|---|---|---|---|---|
| QB1-I-L-2.5-5-1 | 684.5 | 0.699 | 0.549 | 0.79 |
| QB2-I-Y-2.5-4-3 | 604.8 | 0.588 | 0.93 | |
| QB3-I-L-1.5-3-1 | 636.1 | 0.631 | 0.87 | |
| QB4-I-Y-2.5-4-2 | 640.3 | 0.637 | 0.86 | |
| QB5-II-L-2.5-3-1 | 618.1 | 0.606 | 0.91 | |
| QB6-II-Y-2.5-4-3 | 623.2 | 0.613 | 0.90 | |
| Average value | 634.5 | 0.629 | 0.88 |
| Load (KN/m2) | f0 (mm) | f1/f0 | f2/f0 | f3/f0 |
|---|---|---|---|---|
| 0.402 | 0.81 | 0.805 | 3.030 | 1.255 |
| 0.804 | 1.74 | 0.750 | 2.824 | 1.169 |
| 1.206 | 2.69 | 0.728 | 2.740 | 1.135 |
| 1.608 | 3.65 | 0.715 | 2.695 | 1.116 |
| 2.010 | 4.43 | 0.736 | 2.772 | 1.148 |
| 2.412 | 5.41 | 0.725 | 2.729 | 1.130 |
| 2.814 | 6.37 | 0.718 | 2.703 | 1.119 |
| 3.216 | 7.41 | 0.705 | 2.655 | 1.100 |
| 3.618 | 8.57 | 0.686 | 2.583 | 1.070 |
| 4.020 | 9.64 | 0.677 | 2.551 | 1.056 |
| 4.422 | 10.77 | 0.667 | 2.511 | 1.040 |
| 4.824 | 11.91 | 0.658 | 2.478 | 1.026 |
| Average value | / | 0.714 | 2.689 | 1.114 |
| Load (KN/m2) | σsc0 (MPa) | σsc1/σsc0 | σsc2/σsc0 | σsc3/σsc0 |
|---|---|---|---|---|
| 0.402 | −7.84 | 0.656 | 7.166 | 1.023 |
| 0.804 | −16.30 | 0.631 | 6.888 | 0.984 |
| 1.206 | −24.21 | 0.637 | 6.959 | 0.994 |
| 1.608 | −30.34 | 0.678 | 7.404 | 1.057 |
| 2.010 | −35.98 | 0.714 | 7.803 | 1.114 |
| 2.412 | −42.29 | 0.730 | 7.968 | 1.138 |
| 2.814 | −49.80 | 0.723 | 7.893 | 1.127 |
| 3.216 | −57.18 | 0.719 | 7.857 | 1.122 |
| 3.618 | −62.28 | 0.743 | 8.115 | 1.159 |
| 4.020 | −67.54 | 0.761 | 8.314 | 1.187 |
| 4.422 | −77.63 | 0.728 | 7.956 | 1.136 |
| 4.824 | −88.80 | 0.695 | 7.588 | 1.083 |
| Mean value | / | 0.701 | 7.659 | 1.094 |
| Load (KN/m2) | σst0 (MPa) | σst1/σst0 | σst2/σst0 | σst3/σst0 |
|---|---|---|---|---|
| 0.402 | 9.04 | 0.569 | 6.214 | 0.887 |
| 0.804 | 17.44 | 0.590 | 6.440 | 0.920 |
| 1.206 | 25.91 | 0.595 | 6.502 | 0.928 |
| 1.608 | 31.89 | 0.645 | 7.044 | 1.006 |
| 2.010 | 39.50 | 0.651 | 7.107 | 1.015 |
| 2.412 | 48.88 | 0.631 | 6.893 | 0.984 |
| 2.814 | 56.83 | 0.633 | 6.916 | 0.988 |
| 3.216 | 63.84 | 0.644 | 7.037 | 1.005 |
| 3.618 | 69.73 | 0.664 | 7.248 | 1.035 |
| 4.020 | 76.21 | 0.675 | 7.368 | 1.052 |
| 4.422 | 87.45 | 0.647 | 7.064 | 1.009 |
| 4.824 | 98.12 | 0.629 | 6.867 | 0.981 |
| Mean value | / | 0.631 | 6.892 | 0.984 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, G.; Chen, W.; Zhao, D.; Li, K. Mechanical Properties of Prefabricated Cold-Formed Steel Stud Wall Panels Sheathed with Fireproof Phenolic Boards under Out-of-Plane Loading. Buildings 2022, 12, 897. https://doi.org/10.3390/buildings12070897
Zhao G, Chen W, Zhao D, Li K. Mechanical Properties of Prefabricated Cold-Formed Steel Stud Wall Panels Sheathed with Fireproof Phenolic Boards under Out-of-Plane Loading. Buildings. 2022; 12(7):897. https://doi.org/10.3390/buildings12070897
Chicago/Turabian StyleZhao, Gengqi, Wanqiong Chen, Dapeng Zhao, and Ke Li. 2022. "Mechanical Properties of Prefabricated Cold-Formed Steel Stud Wall Panels Sheathed with Fireproof Phenolic Boards under Out-of-Plane Loading" Buildings 12, no. 7: 897. https://doi.org/10.3390/buildings12070897
APA StyleZhao, G., Chen, W., Zhao, D., & Li, K. (2022). Mechanical Properties of Prefabricated Cold-Formed Steel Stud Wall Panels Sheathed with Fireproof Phenolic Boards under Out-of-Plane Loading. Buildings, 12(7), 897. https://doi.org/10.3390/buildings12070897






