1. Introduction
The importance of theoretical and experimental research on the behavior of structures subjected to various types of shock impacts has attracted the attention of many scientists and practitioners to the development of methods for calculating strength and stiffness and conducting experiments to determine the degree of reliability of structures.
Dynamic effects, impacts, are the most dangerous types of loading of buildings and structures (Lyapin A. et al. [
1]). However, the complexity of the calculations of structures for dynamic actions, accompanied by the solution of systems of partial differential equations, complicates the analysis of the stress−strain state (Beskopylny A. et al. [
2], He X-T. et al. [
3]). For heterogeneous materials, the problems are considered mainly numerically (Cai K. et al. [
4]).
Goldsmith W. in his book “Impact. The theory and physical properties of colliding bodies” [
5] gives the study of impact in its classical formulation. The fundamental foundations of wave processes during impact and methods for solving partial differential equations describing processes during impact are considered. An important part of the book is devoted to the description of the technique of the experimental study of shock and wave processes.
A special type of impact on elements of reinforced concrete structures is the so-called emergency shock load, for example, Borys S. et al. [
6], Krašna S. et al. [
7], Furtado A. et al. [
8], Safi W.A. et al. [
9] and others, since they are characterized by high intensity and rare repeatability. An accidental impact can occur, for example, when erecting a multistory building or a multitiered or high structure in the event of an emergency break of a sling, careless securing of a load, the careless turning of the crane boom, etc. The numerical modelling of the impact penetration of a steel cylindrical object into a concrete structure is considered in the work of Belov N. et al. [
10]. The impact of a steel body on a concrete column was simulated with an initial velocity of 1 km/s in the longitudinal and transverse directions.
According to various estimates (Wu X. et al. [
11]), losses from accidental strikes range from tens to hundreds of millions of dollars a year. They are also often accompanied by the death of people. Most emergency strikes are delivered by bodies (strikers) of significant mass (up to several tons) at relatively low speeds (1–20 m/s). From the standpoint of the reaction of the structure, this distinguishes emergency strikes from the high-speed strikes of bombs and projectiles on protective structures, well-studied by military engineers, since it allows the use of methods based on the concepts of classical dynamics for calculations (Buzyurkin A. et al. [
12]).
When analyzing the deformation of individual reinforced concrete elements subjected to an emergency impact, it is necessary to consider the local and general effect of the impact. Local action is understood as the primary impact effect that causes local damage and destruction in the structure (penetration of the striker into the structure, as well as spalling and punching), usually before significant general deformations appear. The general impact of an impact means its secondary effect, which causes general deformations of the structure (bending, etc.).
The analysis of the general deformation of reinforced concrete beams under shock loading showed that, as in the case of static and impulsive actions, the sections of elements during loading go through three stages: before cracking in the tension zone; after cracking until reaching the yield point in tension reinforcement; after reaching the yield point to failure. Accordingly, three stages of beam operation can be considered: no cracks; conditionally elastic with cracks in the stretched zone of sections (until yield in tensioned reinforcement is achieved; plastic (or elastic-plastic) after reaching yield in reinforcement. Pan K. et al. [
13] measured crack propagation rates in three types of self-compacting steel fiber-reinforced concrete. Different loading rates were simulated experimentally for three-point bending. An increase in the ability to absorb energy is noted with increasing fiber content.
Li Y. et al. [
14], concrete specimens were tested for shock compression under various thermal effects of the freeze-thaw cycle using the Hopkinson installation. Then the dynamic crack morphology, crack block distribution, stress-strain curve, maximum stress and other dynamic mechanical properties of concrete were studied. The process of damage development and the mechanism of freezing thawed concrete materials were investigated.
High strain rates can significantly change the mechanical properties of concrete. Yu X. et al. [
15] proposed a method for assessing the basic properties of conventional concrete at high strain rates. The proposed method combined the inverse Lagrangian analysis method with optical methods using an ultra-high speed camera and digital image correlation methods. Dynamic tests showed the nonlinear behavior of stress-strain curves. In our opinion, this could be explained both by the local heterogeneity of concrete and by inertial forces upon impact.
Zhai Y. et al. [
16] experimentally investigated the mechanical characteristics in compression of various heat-treated (400–800 °C) concrete specimens at the Hopkinson installation. It was shown that in uniaxial testing, with an increase in the impact velocity, the crack initiation time increased, and the degree of fracture increased at the same rate as the loading time.
Experimental research (Radchenko A. et al. [
17]) for the action of a dynamic load have shown that the actual design diagram of the deformation of a reinforced concrete structure depended not only on the intensity of the dynamic load, as discussed in previous paragraphs. The influence of the cross-sectional shape of the element, the classes of concrete and reinforcement, the percentage of beam reinforcement were also significant. In Beskopylny A. et al. [
18], the influence of different moduli of elasticity of the material on the stress state of the column in the compressed and stretched parts was investigated. It was shown that in some cases normal tensile stresses without bimodularity were 46% less than with bimodularity, and normal compressive stresses were 29% higher than those with bimodularity. In article [
19] Beskopylny A. et al. considered a reinforced concrete beam made of bimodular material on an elastic Winkler foundation. The influence of the number of reinforcing bars located in the tensile zone on the maximum normal stress and maximum deflection was studied numerically.
The widespread use of reinforced concrete elements in various building structures subjected to static and dynamic loads makes it necessary to develop theoretical methods for calculating strength and stiffness, taking into account the mechanical characteristics of various types of concrete. An important property of concrete is bimodularity, that is, the difference in elastic moduli and Poisson’s ratios in tension and compression. For some types of fine-grained concrete, the modulus of elasticity in tension is two to three times less than in compression, for example, concrete AFB-1 (reinforced fiber concrete #1):
Ec = 1.75 · 10
4 MPa,
Et = 0.75 · 10
4 MPa [
20], fiber-reinforced concrete:
Ec = 2.25 · 10
3 MPa;
Et = 5 · 10
3 MPa [
20].
Starting with the fundamental works of Ambartsumyan S. [
20,
21,
22], Jones R. [
23], Bert C. [
24], the theory of bimodular materials began to develop actively. Zolochevsky A. et al. [
25,
26,
27] developed a theory of creep for materials with different moduli under tension and compression. A constitutive creep strain model that described the load-history-dependent behavior of initially isotropic materials with different tensile and compressive properties was presented. The model was based on kinematic hardening and introduced effective equivalent stress into the creep potential, which was based on the first and second invariants of the effective stress tensor. Practical calculations were carried out numerically.
Analytical solutions for functionally variable curved beams with different properties in tension and compression, in which the moduli of elasticity in tension and compression were taken as two different exponential functions were obtained by He X. et al. [
28,
29,
30,
31]. The authors identified an unknown neutral layer and created a simplified mechanical model related to the tension and compression subzone. Combining the boundary conditions with the conditions of continuity of the neutral layer, the authors applied the power series method to solve the stress components in pure bending. Variations of radial and circumferential stresses in various cases of bimodular functional-gradient parameters were analyzed using numerical examples.
Li X. in [
32] considered a beam made of functionally variable material with characteristics having different moduli of elasticity in tension and compression. One-dimensional and two-dimensional mechanical models for a functionally gradient beam with a bimodular effect were obtained. It was shown that, due to the introduction of the bimodular functional-gradient effect of materials, the maximum tensile and compressive bending stresses might not occur in the lower and upper parts of the beam but were determined using an extreme condition for an analytical solution.
The above review shows that the development of models for structures made of bimodular materials is an important scientific problem. The behavior of structural elements made of bimodular materials can significantly change the stress−strain state in comparison with materials with bimodularity. The stress-strain state of structures made of bimodular materials is practically not observed under shock loading. Thus, the main goal of the article is to develop a mathematical model for a reinforced concrete beam, taking into account bimodularity, and to assess the influence of the cross-sectional shape during transverse impact.
2. Materials and Methods
The paper considers a simply supported reinforced beam made of bimodular material. A load falls onto the beam from a height h in the middle of the span. The impact is considered as an absolutely plastic impact of an absolutely rigid body against an elastic system, taking into account the hypothesis about the proportionality of the dynamic and static characteristics of the stress-strain state of the body. The general impact of the impact is considered, i.e., is determined by the stress-strain state of a reinforced concrete beam before cracks appear in concrete and the development of plastic deformations in reinforcement.
The design scheme of the beam is shown in
Figure 1. The problem is solved in a general way. Section dimensions are taken as an example based on typical designs.
dt—the diameter of the reinforcement in the tension zone; ct—the distance from the reinforcement in the tension zone to the neutral axis; nt—the number of rods in the tension zone; dc—the diameter of the reinforcement in the compression zone; cc—distance (coordinate) from the reinforcement in the compression zone to the neutral axis; nc—number of rods in the compression zone.
In this paper, we consider beams made of fiber-reinforced concrete (lightweight concrete), L = 4 m, density ρ = 700 kg/cm3, and AFB-1 (heavy concrete), density ρ = 2000 kg/cm3, with cross-sections in the form of a rectangle, T-beam and I-beam. The corresponding reduced masses of beams MB are equal for beams made of fiber-reinforced concrete of rectangular cross-section MB1 = 367.2 kg, T-section MB2 = 163.2 kg and I-beam cross-section MB3 = 184.96 kg, from AFB-1 MB4 = 1049 kg, MB5 = 466.3 kg and MB6 = 528.5 kg.
The values of the load and the mass of the beams were chosen in such a way that the dynamic coefficient was not equal to 2. With the value of the falling weight equal to 100 kg for beams made of fiber-reinforced concrete for a rectangular cross-section mB L/MA = 7.71, a T-cross section mB L/MA = 3.423, an I-cross section mB L/MA = 3.9. For fiber-reinforced concrete beams of rectangular cross-section mB L/MA = 22, T-section mB L/MA = 9.77 and I-section cross-section mB L/MA = 11.1.
The dynamic coefficient is determined by the formulas [
33]:
- (a)
without considering the mass of the beam, Equation (1):
- (b)
considering the mass of the beam, Equation (2):
where
h is the height of the fall of the load;
fs is the static deflection of the beam under load without taking into account the mass of the beam;
MA is the mass of the falling weight,
MB is the reduced mass of the beam according to Cox H. [
33]. For a simply supported beam, loaded in the middle of the span,
MB = mBL where
mB is the distributed mass of the beam, L is the beam length.
The change in the coefficient
kd (Equations (1) and (2)) depending on whether the mass of the beam is taken into account is shown in
Figure 2.
Figure 2 shows the dependence of the dynamic coefficient
kd on the relative height of the beam section
2h/fs at various ratios
MB/MA. Curve 1 corresponds to
MB/MA = 0, curve 2 corresponds to
MB/MA = 0.1, curve 3 corresponds to
MB/MA = 1, curve 4 corresponds to
MB/MA = 100. The static deflection
fs was determined without considering the bimodularity of the beam material. As can be seen from
Figure 2, the dynamic coefficient
kd reaches its highest value at
MB/MA = 0 (Curve 1), which corresponds to the case if the beam mass is not taken into account in the calculations.
If the mass of the beam is significantly greater than the mass of the falling load (MB » MB, curve 4), then the dynamic coefficient is the constant kd = 2.
The Formula (2) determines normal dynamic stresses arising in the cross-section of the beam: σdt = kd·σt, σdc = kd·σc, σda = kd·σa, where σdt, σdc, σda, respectively, dynamic normal stresses arising in the tensile, compressed zone of the material beams and reinforcement; σt, σc, σa, respectively, static normal stresses arising in the tensioned, compressed zone of the material of the beam and the reinforcement.
Static stresses and deflection are determined from the action of a statically applied force in the middle of the span, taking into account the bimodularity of the material of the reinforced beam.
A model of a reinforced beam made of a bimodular material was considered as a beam consisting of rigidly connected layers with different mechanical characteristics, in particular, different moduli of elasticity for tension and compression of the material of the beam and reinforcement bars. In this case, the beam became statically indeterminate concerning internal bending moments that arise in the tensioned, compressed zones of the beam material and the reinforcement bars [
34,
35,
36,
37].
Under these assumptions, the bending moment appearing in the cross-section of the beam has the form
- (a)
equilibrium equation for a heterogeneous beam, Equation (3):
- (b)
deformation compatibility condition of a heterogeneous beam, Equation (4):
where
My, is the bending moment and beam curvature;
Myt, is the bending moment and curvature of the tensile zone beam;
Myc, is bending moment and curvature of the compressed zone beam;
Mya, is bending moment and curvature of rebars.
The equilibrium condition
= 0, expressed in terms of normal stresses, has the form, Equation (5):
where
σt, At are the normal stress and the cross-sectional area of the beam in the tensile zone,
σc, Ac are the normal stress and the cross-sectional area of the beam in the compression, compression zone,
σa, Aa are the normal stress and the cross-sectional area of the reinforcement.
The formulas for normal stresses arising in the cross-section of a beam are, Equation (6):
Substituting the normal stress Formula (6) in (5), we obtain the formula for determining the curvature of the neutral line for a reinforced beam made of bimodular material, Equation (7):
For the beam, we have the general curvature formula, Equation (8):
where
D is the reduced stiffness of a reinforced beam made of bimodular material;
Et is the tensile modulus of the material;
Ec is the elastic modulus of the material under compression;
Ea is the tensile modulus of reinforcement;
is the moment of inertia about the neutral axis of the part of the cross-section that lies in the tension zone;
is the moment of inertia about the neutral axis of the part of the cross-section that lies in the compression zone;
is the moment of inertia of the cross-section of the reinforcement, which lies in the tension zone, relative to its own central axis;
is the moment of inertia of the cross-section of the reinforcement, which lies in the compression zone, relative to its own central axis;
nt is the number of reinforcement bars in the tension zone;
nc is the number of reinforcement bars in the compression zone;
is the cross-sectional area of the reinforcement in the tension zone;
cross-sectional area of reinforcement in the compression zone;
ct distance from the reinforcement in the tension zone to the neutral axis;
cc is the distance from the reinforcement in the compression zone to the neutral axis.
Comparing Formulas (7) and (8), we obtain the expression for the reduced stiffness
D for reinforced beams made of bimodal material, Equation (9):
To determine the position of the neutral line (to determine the height of the stretching and compressed zones), consider the equilibrium equations, Equations (10) and (11):
After substituting (6) into (10), (11), we obtain, Equations (12) and (13):
where
Syt is the static moment of the cross-sectional area of the expanding zone relative to the neutral line,
Syc is the static moment of the cross-sectional area of the contracting zone relative to the neutral line,
Sya is the static moment of the area of reinforcement bars relative to the neutral line
Equations (12) and (13) are used to determine the neutral line of the cross-section of beams with an arbitrary cross-section.
Centrifugal moments of inertia Iyzt (x) = 0, Iyzc (x) = 0 since the cross-section is considered symmetric about the y-axis. Then Equation (13) is satisfied identically, and the position of the neutral line is determined from Equation (12).
For a rectangular beam (12) is written in the following form, Equation (14):
The positive root of which has the form (
k ≠ 1), Equation (15):
The height of the compressed zone
hc is found from the condition
h = hc +
ht, Equation (16):
where
hc is the height of the compressed area;
ht is the height of the stretched area,
h is the height of the cross-section,
b is the width of the cross-section
= k.
For I-beams and T-beams, expression (12) has the form, Equation (17):
where
where
d is the wall thickness of the cross-section,
bt, tt are the width and thickness of the cross-section flange in the tension zone,
bc, tc are the width and thickness of the cross-section flange in the compression zone,
nc, ccare the number and distance to the neutral line of reinforcing bars in the compressed zone, nt, ct are the the number and distance to the neutral line of reinforcing bars in the tensioned zone, Ac, At are the cross-sectional areas of the reinforcing bars in the compressed and tensile zones, respectively.
The formulas for normal stresses arising in the cross-sections of a beam under the action of static loads, taking into account (6), (8), have the form, Equation (18):
where
z is the distance from the neutral line to the point at which the normal voltage is determined.
4. Discussion
The obtained mathematical models for calculating reinforced beams of various sections and shapes show that the bimodularity of concrete can both qualitatively and quantitatively change the stress−strain state of a structure in comparison with a homogeneous material. In our examples, a significant change in the stress state is manifested in the fact that the dynamic normal tensile stresses are lower in absolute value than the dynamic normal compressive stresses. He X.-T. et al. [
3] showed, using the example of an axisymmetric plate, that the bimodular effect will significantly change the ratio of the load and the central deflection of the plate. Under the same boundary conditions, the ability to resist deformation depends on the values of the tensile modulus of elasticity, the modulus of elasticity of the neutral layer and the modulus of elasticity in compression.
The effect of bimodularity is manifested in a change in the stress−strain state. This can be seen from the considered problems of beam bending and is typical for a complex stress state. A similar trend is discussed in the work of Shah S. et al. [
38]. The authors investigated the influence of the bimodular behavior of adhesive and curing stresses on the behavior of interfacial delamination cracks in tee joints by performing sequential thermal and geometrically nonlinear finite element analysis. The authors also noted that the effect of bimodularity in comparison with the functional gradient turned out to be much more significant on the fracture behavior in the mixed mode, which indicated a slowdown in the rate of fracture propagation at the interface along certain zones.
The numerical study presented in this work shows that the stress state, taking into account the bimodularity under the action of a shock load, is qualitatively and quantitatively affected by the ratio of the elastic modulus in tension and elastic modulus in compression. For beams made of heavy concrete, for which the moduli of elasticity in tension and compression are three times higher than the corresponding moduli for lightweight concrete, the dynamic maximum stresses increase three times, which is confirmed by Hooke’s law (
Table 1 and
Table 3).
When
Et > Ec in a T-section beam, regardless of the number of rods,
σdt differs from
σdc by only 0.7% (
Table 1 and
Table 4). At
Et = Ec σdc > σdt by 57% (
Table 2). In the case of
Et < Ec σdc > σdt by 13% (
Table 3). The data presented confirm the need to take into account bimodularity when calculating the strength of beams under shock loads.
The stress state of the beam is influenced by the impact (at the same section height) and the shape of the cross-section. From the values of the maximum tensile and compressive stresses given in the tables, it can be seen that the highest stresses arise in the T-cross-section beams, the smallest in the rectangular cross-section beams, both with and without regard to the mass of the beam.
The arrangement of reinforcement bars in compression and tension zones also affects the stress state of the beam under impact. So, at
Et > Ec and the same number of reinforcing bars located in the compressed and tensile zones
σdc < σdt by 45% for a rectangular cross-section and 15% for an I-beam cross-section (
Table 1). In the absence of reinforcing bars in the compressed zone, the difference between the maximum tensile and compressive stresses increases:
σdc < σdt by 57% for a rectangular cross-section and by 30% for an I-section (
Table 5), the value of the maximum tensile normal stress in comparison with the arrangement of reinforcing bars in both the compressed and stretched zones, the increase for a rectangular cross-section is 9% and for I-beams by 13% (
Table 1 and
Table 5). As can be seen from the graphs shown in
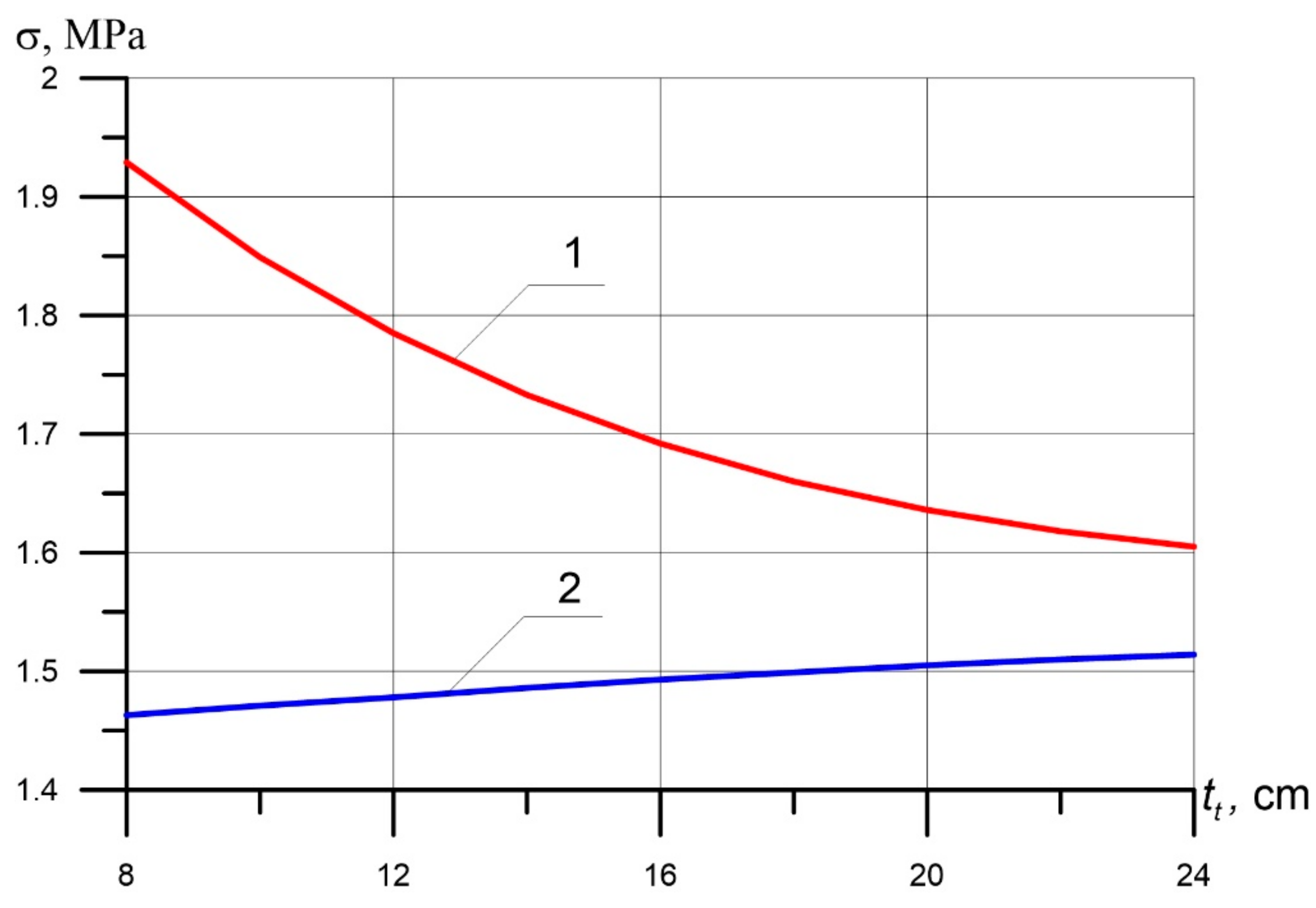
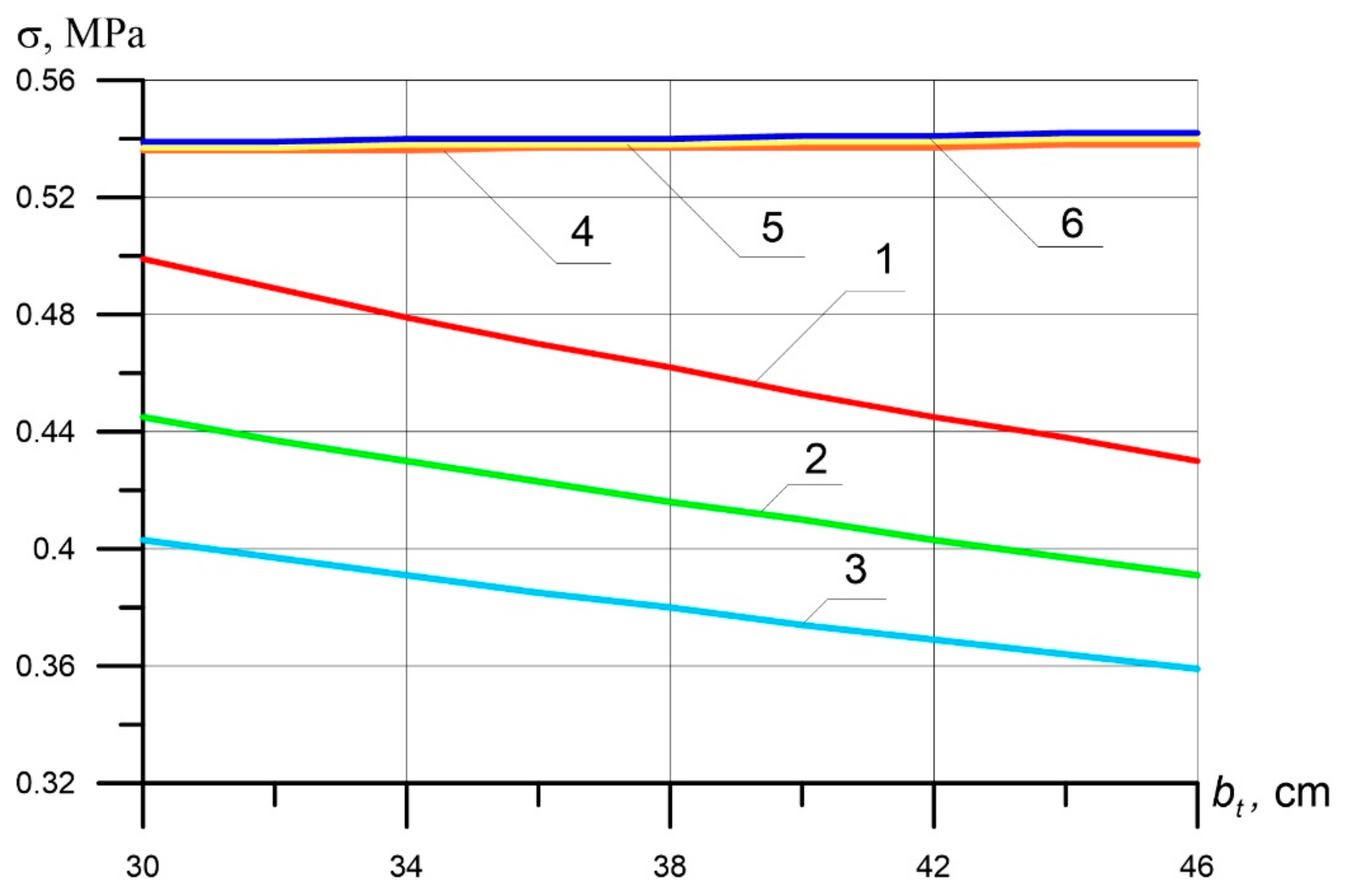
Figure 4 and
Figure 5, by varying the dimensions of the cross-section, it is possible to significantly reduce the values of the maximum tensile normal stresses of the cross-section that arise in the beam under the action of shock loads, taking into account the bimodularity of the material.
The method presented in the article allows, when calculating the strength and design of reinforced concrete beams under shock loads, the study of the effects of the mechanical properties of the material of the beam (bimodularity), the number of reinforcing bars and their location, the shape and size of the beam cross-section, the mass of the beam, the size and height of the drop load. The proposed methodology for the bimodularity accounting makes it possible to carry out calculations to optimize the structure from the point of view of their economic cost while meeting all the requirements for safety, strength and deformability. Solorzano G. et al. [
39] used a genetic algorithm with a dominance-based competitive selection technique to solve this optimal design problem. The methodology was applied in the design of rectangular isolated reinforced concrete supports.
The dynamic problems considered in this article show that taking into account the bimodularity for beams made of heavy concrete reduces the values of the maximum normal stresses (
Table 3 and
Table 4), in contrast to beams made of fiber-reinforced concrete (
Table 1 and
Table 2),
σdt decreases within 5%,
σdc decreases within 55%, both taking into account the mass of the beam and excluding the mass of the beam. Chen et al. in [
40] showed a similar effect when bimodularity not only displaced the neutral axis, which is obvious, but also changed the natural frequency of the beam vibrations.
As can be seen from
Table 2 and
Table 4, taking into account the mass of the beam reduces the dynamic normal tensile stresses and dynamic normal compressive stresses by 300−800% for lightweight concrete, by 1029–2546% for heavy concrete without taking into account bimodularity. Considering the bimodularity (
Table 1 and
Table 3), the mass of the beam reduces the dynamic normal tensile stresses and dynamic normal compressive stresses by 162–398% for lightweight concrete, by 347–2701% for heavy concrete. The installation of reinforcing bars in the tensile zone reduces the dynamic normal stresses, considering the bimodularity of the material for lightweight concrete, by an average of 771%. A numerical study can make it possible to determine the limits of applicability of using Equation (1), which is mainly used when calculating structural elements under the action of an impact, taking into account the bimodularity of the material of beams.
Experimental verification of the methodology for taking into account bimodularity when determining the maximum static deflection [
2] included in the dynamic coefficient Formula (1), (2) showed that when taking into account bimodularity, the theoretical value of the maximum deflection differs from the experimental value by 4%, without taking into account bimodularity by 6% for steel with a difference of modules in tension and compression by 2.8%. For silumin with a difference in tensile and compressive moduli by 9.7%, the experimental deflection is less than the theoretical one, taking into account bimodularity, by 0.7%, and excluding bimodularity by 5.4%. That is, the greater the difference between the moduli of elasticity in tension and compression, the more accurate the theoretical value of deflections is given by taking into account the bimodularity of the material of the beam.
5. Conclusions
The results of the study showed that the strength of reinforced concrete beams under shock loads is affected by a wide range of both mechanical and geometric characteristics. So, if we talk about the technologically justified equality of the heights of different shapes of cross-sections with the corresponding dimensions of the thickness and width of the shelves, then the maximum tensile and compressive stresses depend on taking into account the values of the elastic moduli in tension and compression.
For lightweight concrete, in our work, fiber-reinforced concrete, for which
Et < Ec, the smallest tensile and compressive dynamic normal stresses arise in a beam of rectangular cross-section with and without regard to the mass of the beam (
Table 1 and
Table 2). When designing a reinforced concrete structure, however, it is necessary to take into account the weight of the beam, since although the bearing capacity of a rectangular cross-section beam is higher than that of beams of T- and I-cross-sections, the reduced mass M is twice as large as the reduced mass of beams of I- and T-cross-sections, and this is an additional load on the columns, walls and foundations.
In beams made of heavy concrete AFB-1,
Et > Ec, the smallest tensile and compressive normal stresses also arise in a beam of rectangular cross-section with and without regard to the mass of the beam (
Table 3 and
Table 4). As for lightweight concrete, the reduced mass
MB of a rectangular beam is twice as large as the reduced mass of I-beams and T-beams. If the normal tensile stresses
σdt differ little in magnitude, then the normal compressive stresses
σdc in a rectangular cross-section beam are 65% less than in a T-cross-section beam, and by 22% less than in an I-beam cross-section.
The stress state of the beams at the same cross-sectional height can be regulated by varying the cross-sectional dimensions in the stretched zone (
Figure 4 and
Figure 5), which leads to a sharp decrease in
σdt with a slight increase in
σdc. The number and location of reinforcement bars also affect the stress state of beams. The arrangement of the rods only in the tensile zone has practically no effect on
σdc, while
σdt increases by 8% (
Table 5).
The geometric dimensions of the cross-section of the beam and the number of reinforced bars significantly affect the stress state of the reinforced concrete beam. (
Figure 7,
Table 6,
Figure 8,
Table 7,
Figure 9,
Table 8,
Figure 10,
Table 9). The simultaneous increase in the width or thickness of the I-beam flange is 1.8 times, and the number of reinforced rods in the tensioned zone leads to an increase in the normal compressive stress by 2% and a decrease in the normal tensile stress by 45%.
The proposed engineering method makes it possible to study the effect on the stress state of reinforced beams made of bimodular material under the action of shock loads, the geometric characteristics of the cross-section, the bimodularity of the material and the characteristics of reinforcement bars.