Author Contributions
Conceptualization, D.T., T.Z. and X.C.; Methodology, D.T.; Theoretical Guidance, D.T.; Review, D.T., X.C., Y.L. and J.H.; Writing—Original Draft, T.Z.; Writing—Review & Editing, T.Z.; Formal analysis, T.Z.; Software, T.Z.; Data Curation, T.Z.; Visualization, T.Z.; Resources, X.C.; Validation, Y.L. and J.H. All authors have read and agreed to the published version of the manuscript.
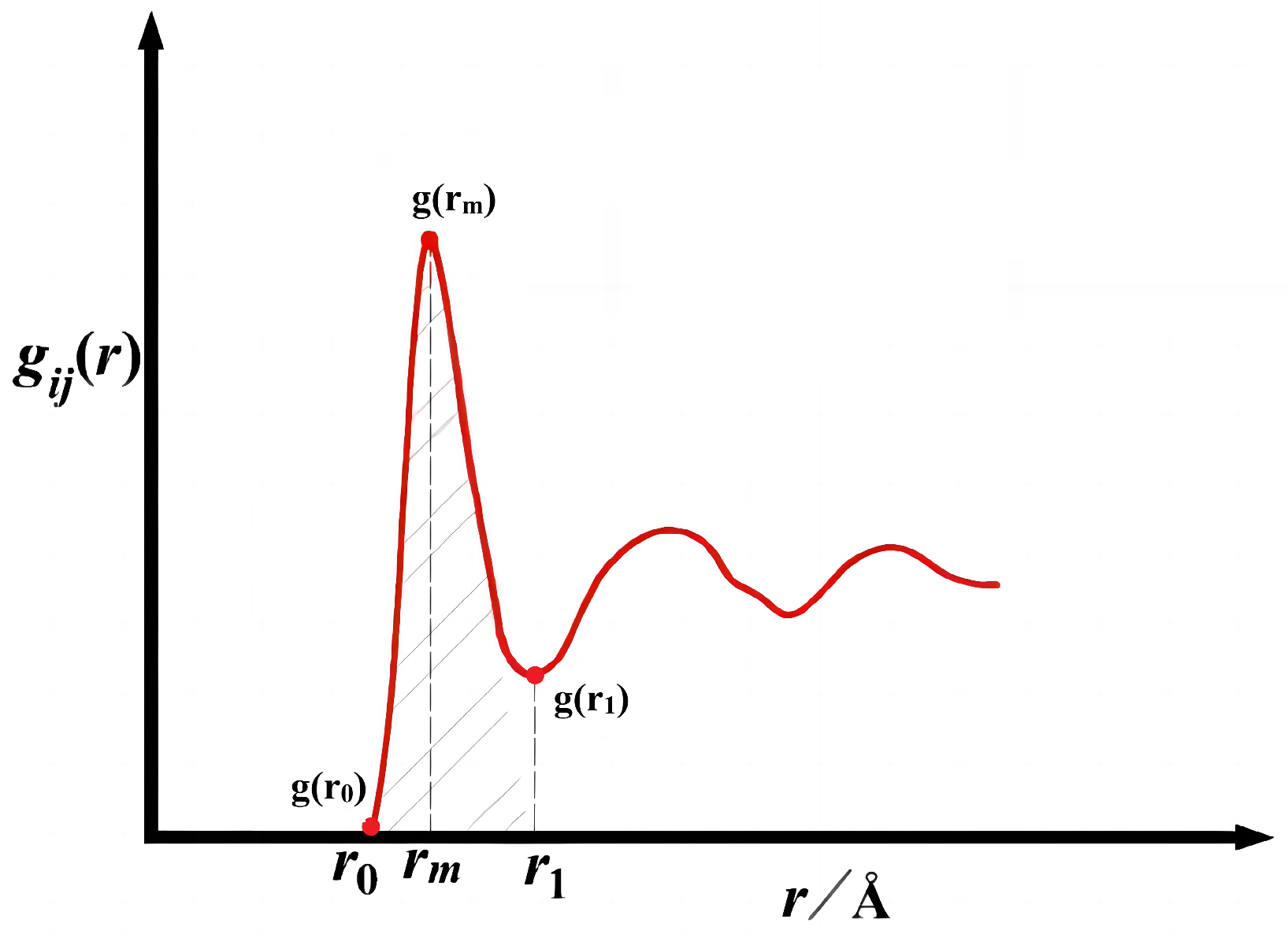
Figure 1.
The first valley and first peak of partial radial distribution function.
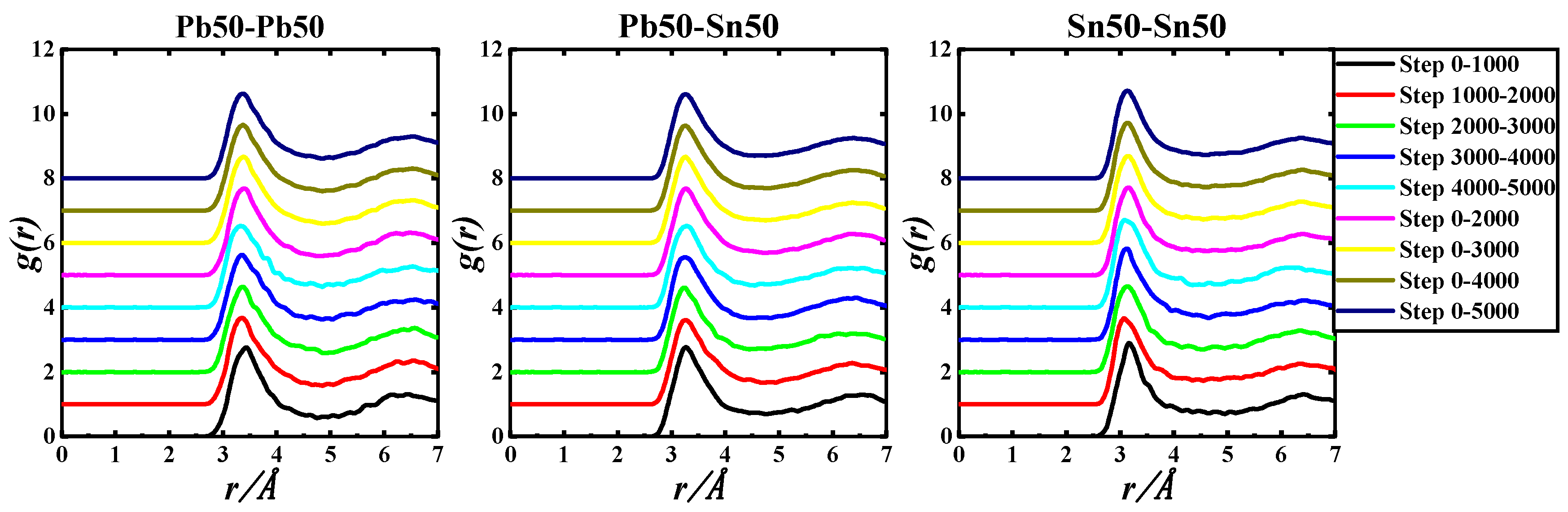
Figure 2.
gIn-In(r), gIn-Zn(r), and gZn-Zn(r) of the Pb50Sn50-1050 K system based on 5000 step PRDF data.
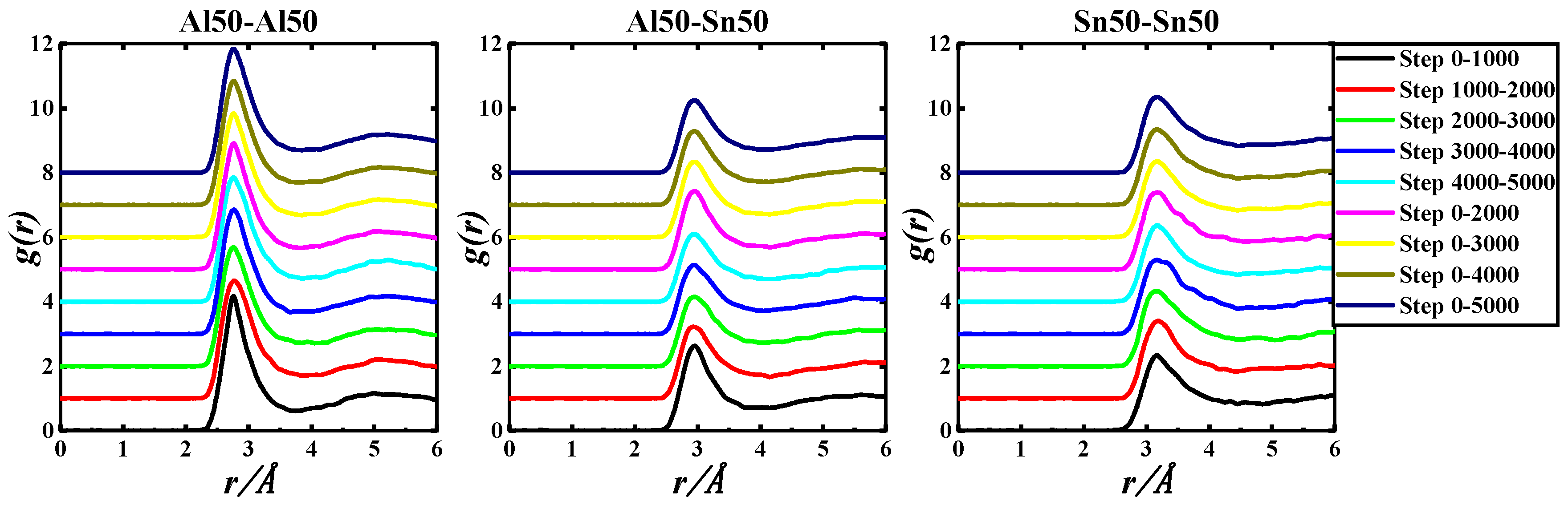
Figure 3.
gAl-Al(r), gAl-Sn(r), and gSn-Sn(r) of the Al50Sn50-973 K system based on 5000 step PRDF data.
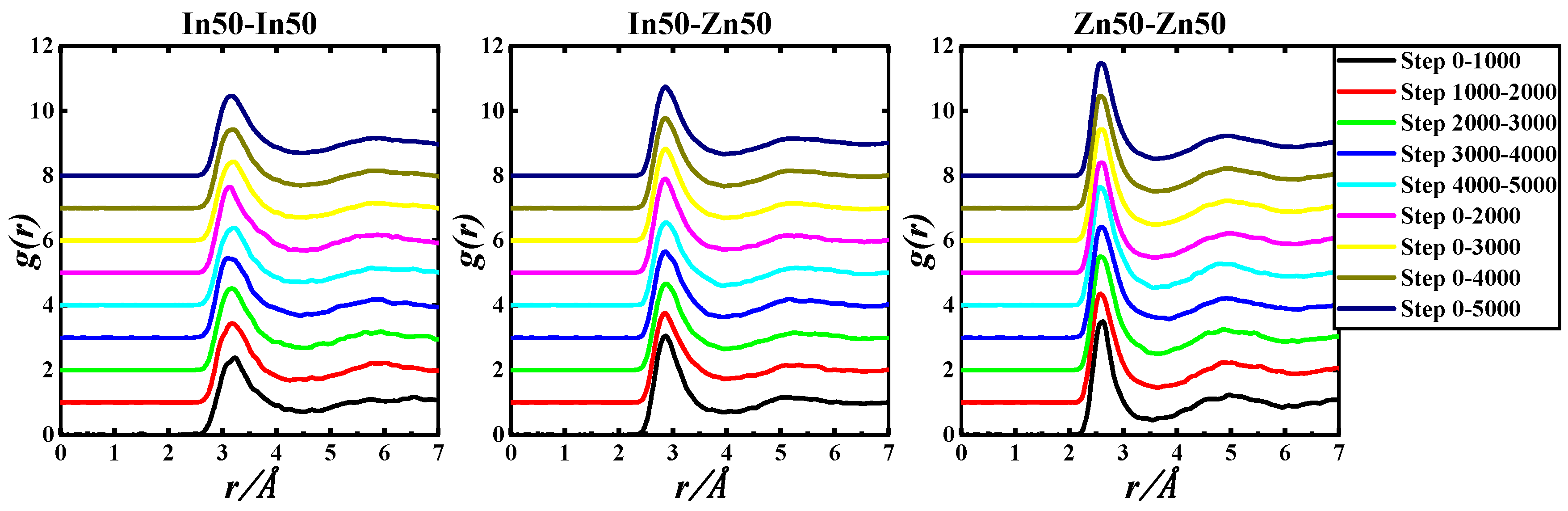
Figure 4.
gIn-In(r), gIn-Zn(r), and gZn-Zn(r) of the In50Zn50-730 K system based on 5000 step PRDF data.
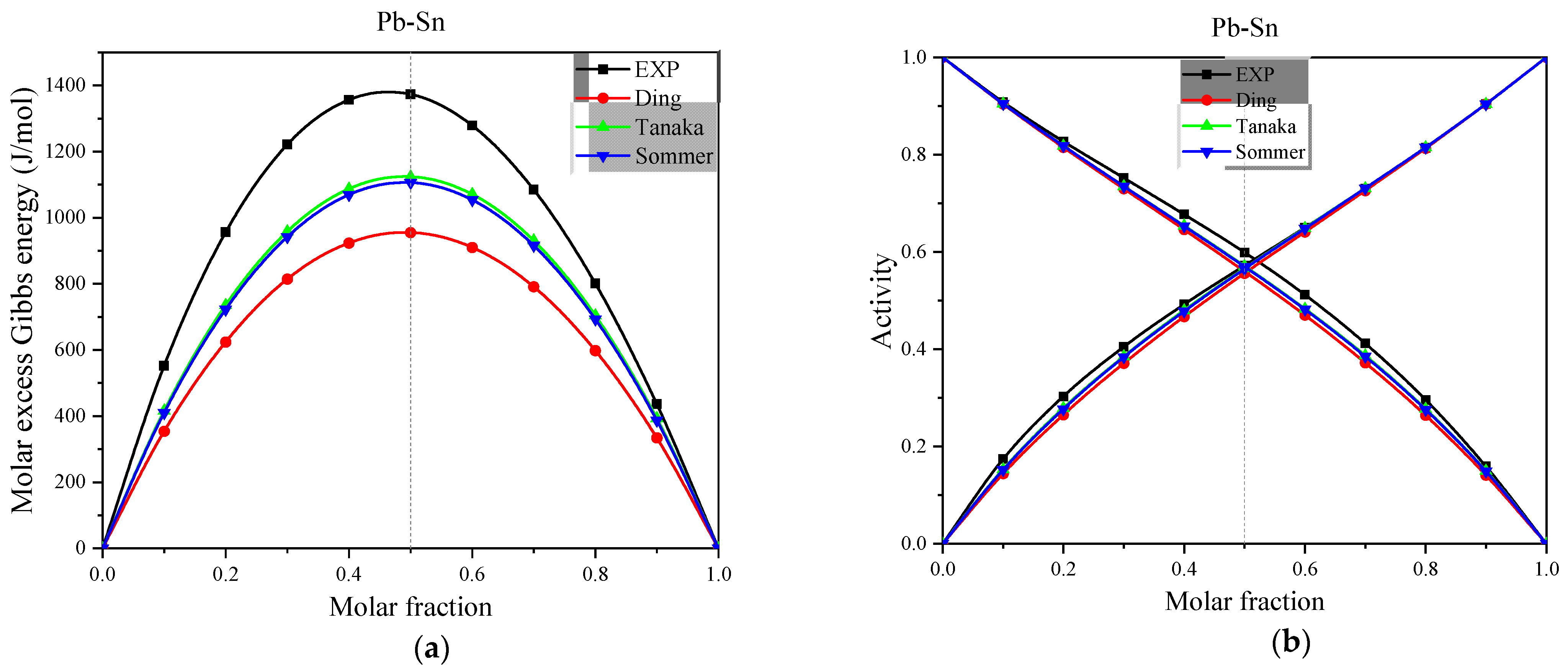
Figure 5.
(a) The experimental and calculated values of molar excess Gibbs energy for the full concentration of Pb-Sn alloys in the Miedema model at 1050 K, (b) The experimental and calculated values of activity for the full concentration of Pb-Sn alloys in the Miedema model at 1050 K.
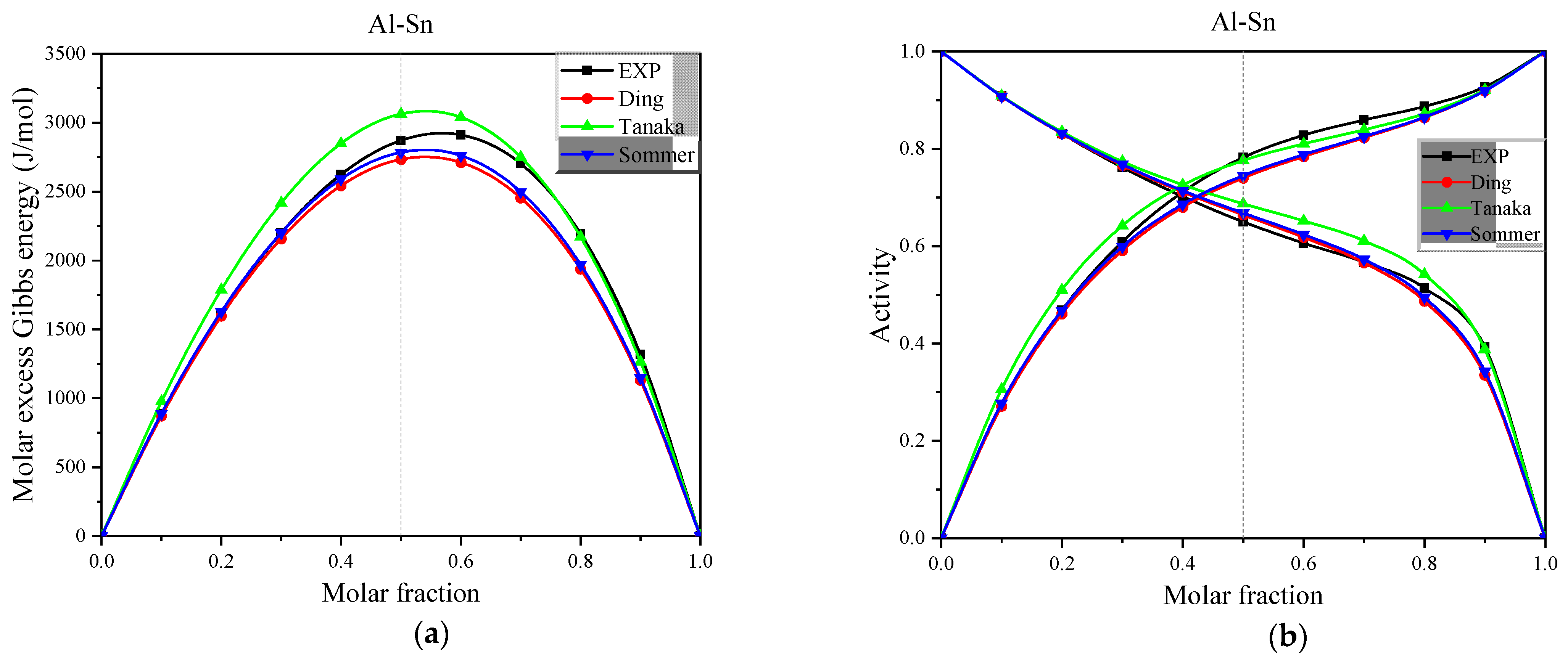
Figure 6.
(a) The experimental and calculated values of molar excess Gibbs energy for the full concentration of Al-Sn alloys in the Miedema model at 973 K, (b) The experimental and calculated values of activity for the full concentration of Al-Sn alloys in the Miedema model at 973 K.
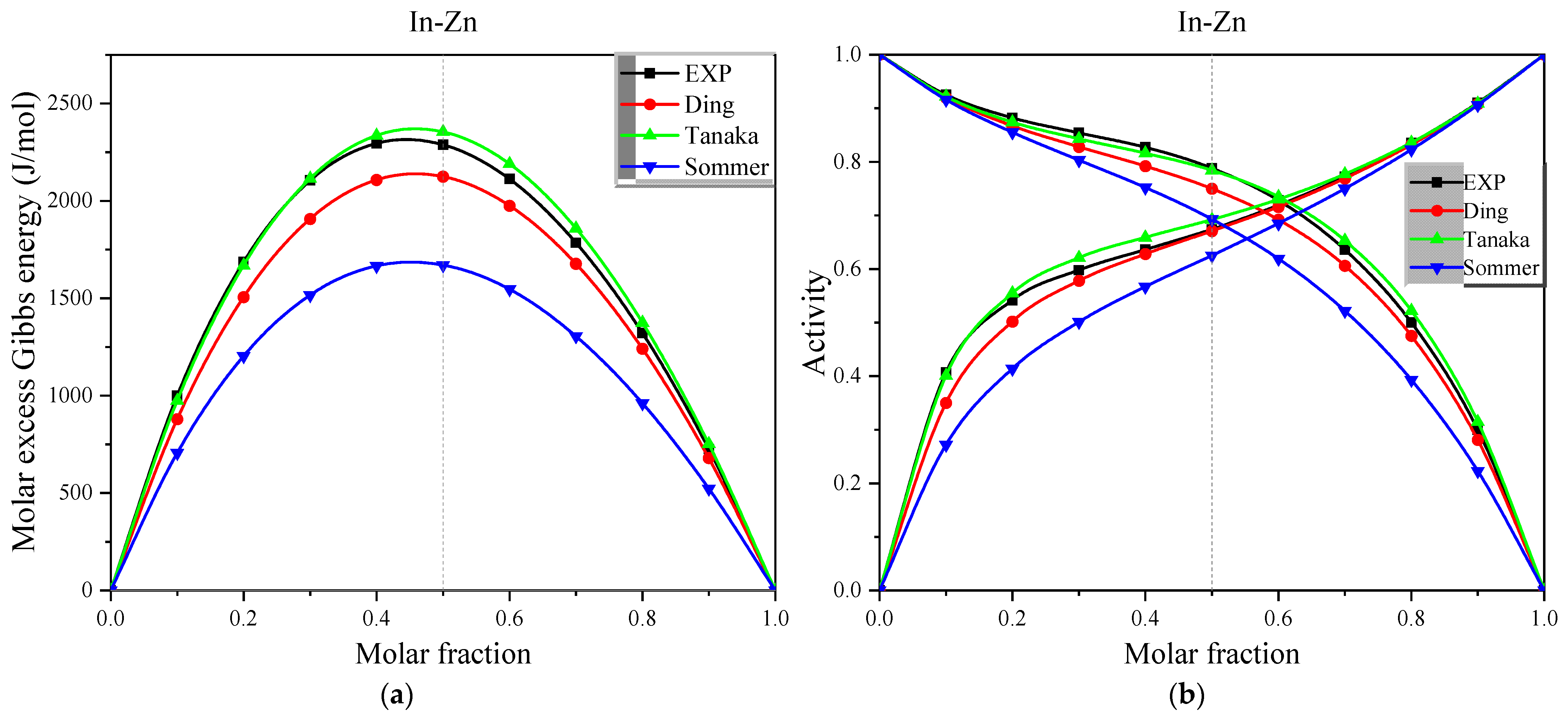
Figure 7.
(a) The experimental and calculated values of molar excess Gibbs energy for the full concentration of In-Zn alloys in the Miedema model at 730 K, (b) The experimental and calculated values of activity for the full concentration of In-Zn alloys in the Miedema model at 730 K.
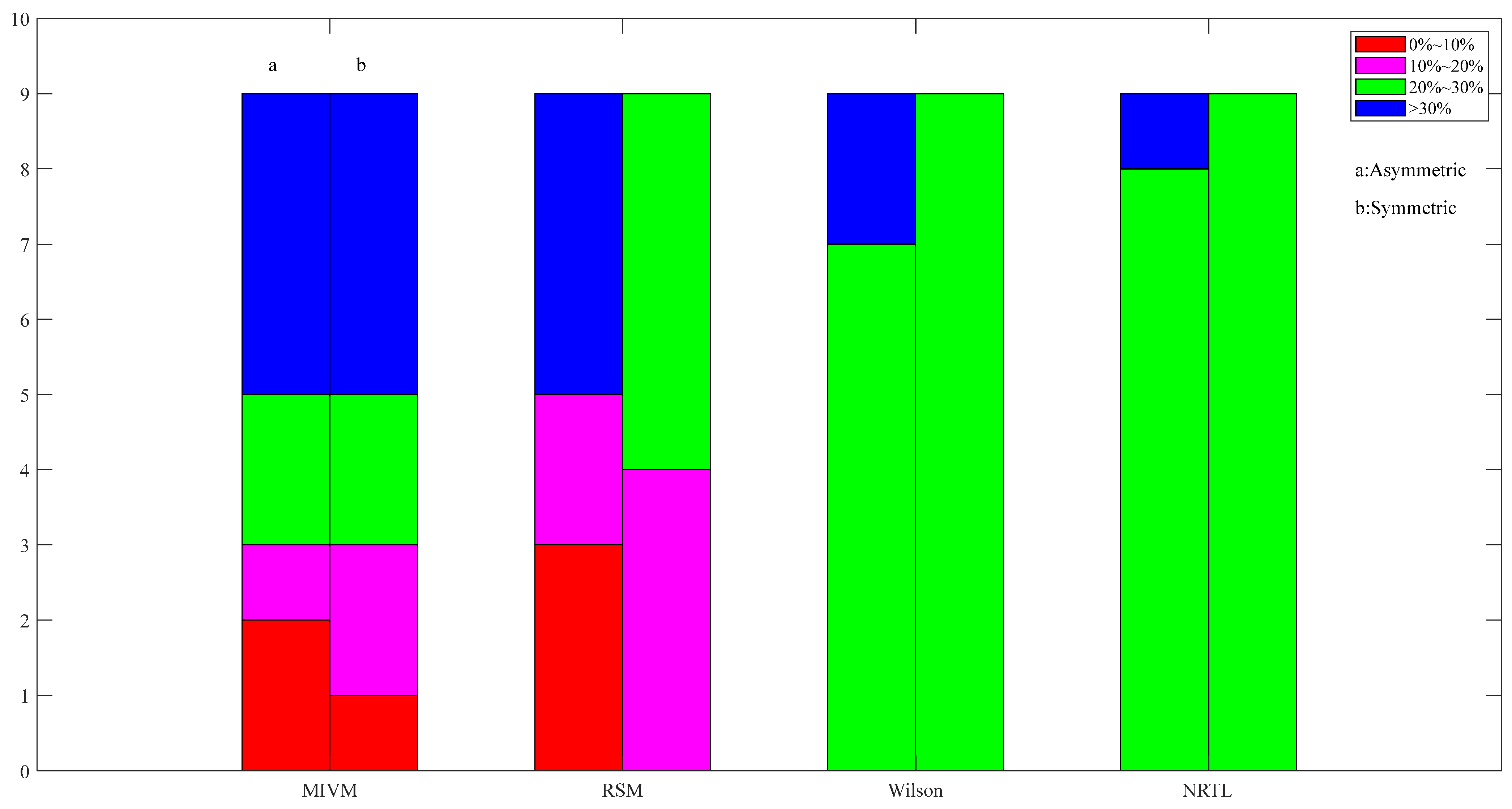
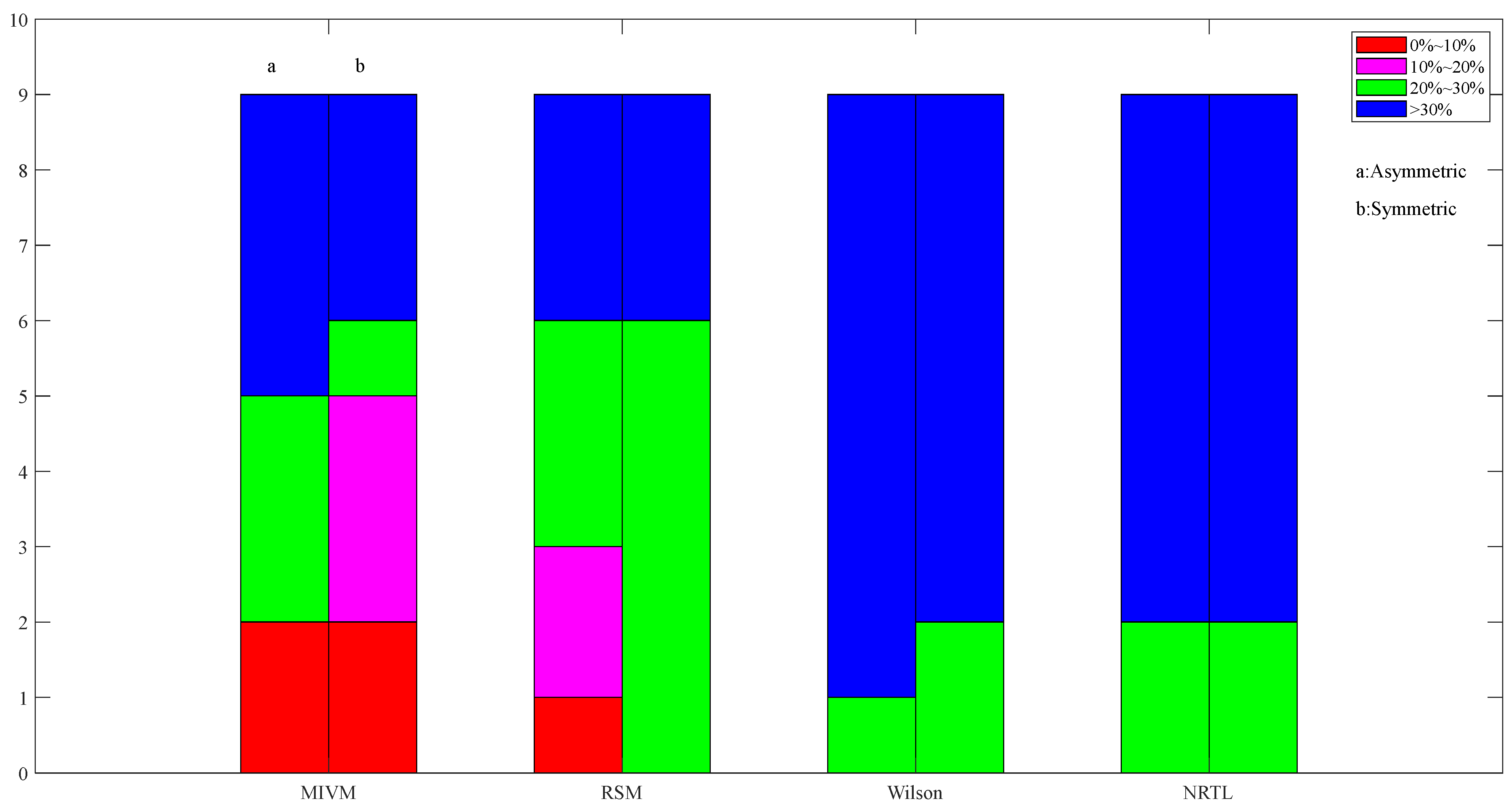
Figure 8.
Images of asymmetric and symmetric method activity ARD calculations for Pb50-Sn50 alloys in four models at 1050 K.
Figure 9.
Images of asymmetric and symmetric method activity ARD calculations for Al50-Sn50 alloys in four models at 973 K.
Figure 10.
Images of asymmetric and symmetric method activity ARD calculations for In50-Zn50 alloys in four models at 730 K.
Table 1.
The three key coordinate points of gPb-Pb(r), gPb-Sn(r), and gSn-Sn(r) in the Pb50Sn50-1050 K system.
| Parameters | Step of Pb50-Sn50 (1050 K) |
|---|
| 0–1000 | 1000–2000 | 2000–3000 | 3000–4000 | 4000–5000 | 0–2000 | 0–3000 | 0–4000 | 0–5000 |
|---|
| r0 i-i | 2.650 | 2.650 | 2.650 | 2.650 | 2.650 | 2.650 | 2.650 | 2.650 | 2.650 |
| g(r0)i-i | 0.004 | 0.004 | 0.004 | 0.004 | 0.005 | 0.004 | 0.004 | 0.004 | 0.004 |
| rm i-i | 3.450 | 3.350 | 3.350 | 3.350 | 3.350 | 3.350 | 3.350 | 3.350 | 3.350 |
| g(rm)i-i | 2.751 | 2.671 | 2.634 | 2.628 | 2.521 | 2.645 | 2.641 | 2.638 | 2.615 |
| r1 i-i | 4.750 | 4.850 | 4.850 | 4.550 | 4.850 | 4.750 | 4.850 | 4.850 | 4.850 |
| g(r1)i-i | 0.577 | 0.581 | 0.594 | 0.680 | 0.646 | 0.590 | 0.595 | 0.604 | 0.613 |
| r0 i-j | 2.550 | 2.650 | 2.550 | 2.650 | 2.550 | 2.550 | 2.550 | 2.550 | 2.550 |
| g(r0)i-j | 0.002 | 0.011 | 0.001 | 0.007 | 0.001 | 0.001 | 0.001 | 0.001 | 0.001 |
| rm i-j | 3.250 | 3.250 | 3.250 | 3.250 | 3.250 | 3.250 | 3.250 | 3.250 | 3.250 |
| g(rm)i-j | 2.748 | 2.601 | 2.614 | 2.547 | 2.508 | 2.675 | 2.654 | 2.627 | 2.603 |
| r1 i-j | 4.750 | 4.950 | 4.450 | 4.450 | 4.550 | 4.750 | 4.750 | 4.750 | 4.750 |
| g(r1)i-j | 0.699 | 0.671 | 0.704 | 0.669 | 0.677 | 0.688 | 0.700 | 0.696 | 0.700 |
| r0 j-j | 2.550 | 2.550 | 2.550 | 2.450 | 2.550 | 2.550 | 2.550 | 2.450 | 2.450 |
| g(r0)j-j | 0.003 | 0.002 | 0.013 | 0.001 | 0.001 | 0.002 | 0.006 | 0.001 | 0.001 |
| rm j-j | 3.150 | 3.050 | 3.150 | 3.150 | 3.050 | 3.150 | 3.150 | 3.150 | 3.150 |
| g(rm)j-j | 2.874 | 2.632 | 2.647 | 2.807 | 2.667 | 2.712 | 2.690 | 2.719 | 2.706 |
| r1 j-j | 4.150 | 4.150 | 4.150 | 4.650 | 4.350 | 4.350 | 4.150 | 4.550 | 4.550 |
| g(r1)j-j | 0.790 | 0.801 | 0.756 | 0.673 | 0.697 | 0.748 | 0.783 | 0.729 | 0.724 |
Table 2.
The three key coordinate points of gAl-Al(r), gAl-Sn(r), and gSn-Sn(r) in the Al50Sn50-973 K system.
| Parameters | Step of Al50-Sn50 (973 K) |
|---|
| 0–1000 | 1000–2000 | 2000–3000 | 3000–4000 | 4000–5000 | 0–2000 | 0–3000 | 0–4000 | 0–5000 |
|---|
| r0 i-i | 1.850 | 2.150 | 2.250 | 2.250 | 2.250 | 1.850 | 1.850 | 1.850 | 1.850 |
| g(r0)i-i | 0.002 | 0.001 | 0.001 | 0.004 | 0.005 | 0.001 | 0.001 | 0.001 | 0.001 |
| rm i-i | 2.750 | 2.750 | 2.750 | 2.750 | 2.750 | 2.750 | 2.750 | 2.750 | 2.750 |
| g(rm)i-i | 4.165 | 3.629 | 3.686 | 3.843 | 3.850 | 3.897 | 3.827 | 3.831 | 3.834 |
| r1 i-i | 3.750 | 3.850 | 3.850 | 3.650 | 3.850 | 3.850 | 3.850 | 3.850 | 3.850 |
| g(r1)i-i | 0.612 | 0.708 | 0.722 | 0.676 | 0.717 | 0.669 | 0.687 | 0.691 | 0.697 |
| r0 i-j | 1.750 | 2.350 | 2.350 | 2.250 | 2.350 | 1.750 | 1.750 | 1.750 | 1.750 |
| g(r0)i-j | 0.001 | 0.002 | 0.001 | 0.001 | 0.002 | 0.001 | 0.001 | 0.001 | 0.001 |
| rm i-j | 2.950 | 2.950 | 2.950 | 2.950 | 2.950 | 2.950 | 2.950 | 2.950 | 2.950 |
| g(rm)i-j | 2.634 | 2.219 | 2.149 | 2.134 | 2.094 | 2.426 | 2.334 | 2.284 | 2.246 |
| r1 i-j | 3.750 | 4.150 | 4.050 | 4.050 | 3.850 | 3.750 | 3.750 | 3.750 | 3.750 |
| g(r1)i-j | 0.713 | 0.667 | 0.728 | 0.712 | 0.770 | 0.754 | 0.767 | 0.777 | 0.776 |
| r0 j-j | 2.450 | 2.550 | 2.550 | 2.550 | 2.550 | 2.450 | 2.450 | 2.450 | 2.450 |
| g(r0)j-j | 0.003 | 0.003 | 0.005 | 0.004 | 0.001 | 0.001 | 0.001 | 0.001 | 0.001 |
| rm j-j | 3.150 | 3.150 | 3.150 | 3.150 | 3.150 | 3.150 | 3.150 | 3.150 | 3.150 |
| g(rm)j-j | 2.324 | 2.383 | 2.319 | 2.286 | 2.381 | 2.354 | 2.342 | 2.328 | 2.339 |
| r1 j-j | 4.450 | 4.450 | 4.450 | 4.450 | 4.550 | 4.450 | 4.450 | 4.450 | 4.450 |
| g(r1)j-j | 0.824 | 0.838 | 0.829 | 0.783 | 0.866 | 0.831 | 0.830 | 0.818 | 0.833 |
Table 3.
The three key coordinate points of gIn-In(r), gIn-Zn(r), and gZn-Zn(r) in the In50Zn50-730 K system.
| Parameters | Step of In50-Zn50 (730 K) |
|---|
| 0–1000 | 1000–2000 | 2000–3000 | 3000–4000 | 4000–5000 | 0–2000 | 0–3000 | 0–4000 | 0–5000 |
|---|
| r0 i-i | 2.250 | 2.550 | 2.450 | 2.550 | 2.550 | 2.250 | 2.250 | 2.250 | 2.250 |
| g(r0)i-i | 0.003 | 0.010 | 0.001 | 0.011 | 0.005 | 0.002 | 0.001 | 0.001 | 0.001 |
| rm i-i | 3.250 | 3.150 | 3.150 | 3.150 | 3.150 | 3.250 | 3.150 | 3.150 | 3.150 |
| g(rm)i-i | 2.368 | 2.424 | 2.505 | 2.429 | 2.651 | 2.370 | 2.397 | 2.405 | 2.454 |
| r1 i-i | 4.150 | 4.250 | 3.950 | 4.450 | 4.250 | 4.150 | 4.150 | 4.150 | 4.150 |
| g(r1)i-i | 0.777 | 0.680 | 0.861 | 0.680 | 0.704 | 0.744 | 0.757 | 0.771 | 0.775 |
| r0 i-j | 2.150 | 2.350 | 2.350 | 2.350 | 2.350 | 2.150 | 2.150 | 2.150 | 2.150 |
| g(r0)i-j | 0.001 | 0.010 | 0.011 | 0.004 | 0.006 | 0.001 | 0.001 | 0.001 | 0.001 |
| rm i-j | 2.850 | 2.850 | 2.850 | 2.850 | 2.850 | 2.850 | 2.850 | 2.850 | 2.850 |
| g(rm)i-j | 3.045 | 2.760 | 2.640 | 2.654 | 2.536 | 2.903 | 2.815 | 2.775 | 2.727 |
| r1 i-j | 3.950 | 3.950 | 3.950 | 3.950 | 3.950 | 3.950 | 3.950 | 3.950 | 3.950 |
| g(r1)i-j | 0.695 | 0.725 | 0.648 | 0.630 | 0.603 | 0.710 | 0.689 | 0.675 | 0.660 |
| r0 j-j | 2.050 | 2.050 | 2.150 | 2.150 | 2.150 | 2.050 | 2.050 | 2.050 | 2.050 |
| g(r0)j-j | 0.001 | 0.001 | 0.012 | 0.009 | 0.011 | 0.001 | 0.001 | 0.001 | 0.001 |
| rm j-j | 2.650 | 2.550 | 2.550 | 2.650 | 2.550 | 2.650 | 2.650 | 2.650 | 2.550 |
| g(rm)j-j | 3.455 | 3.307 | 3.452 | 3.353 | 3.605 | 3.340 | 3.371 | 3.366 | 3.404 |
| r1 j-j | 3.550 | 3.650 | 3.650 | 3.850 | 3.550 | 3.550 | 3.550 | 3.650 | 3.650 |
| g(r1)j-j | 0.455 | 0.457 | 0.505 | 0.574 | 0.542 | 0.470 | 0.486 | 0.521 | 0.527 |
Table 4.
Parameters for Miedema model calculation of Pb-Sn, Al-Sn, In-Zn systerms [
42].
| Metal | Φ | nws1/3 | V2/3 | μ | α | Tm/K | Tb/K | r/p |
|---|
| Pb | 4.1 | 1.15 | 6.9 | 0.04 | 0.73 | 1050 | 2022 | 2.1 |
| Al | 4.2 | 1.39 | 4.6 | 0.07 | 0.73 | 933 | 2793 | 1.9 |
| Sn | 4.15 | 1.24 | 6.4 | 0.04 | 0.73 | 505 | 2875 | 2.1 |
| In | 3.9 | 1.17 | 6.3 | 0.07 | 0.73 | 430 | 2345 | 1.9 |
| Zn | 4.1 | 1.32 | 4.4 | 0.1 | 0.73 | 693 | 1181 | 1.4 |
Table 5.
Parameters of four models were calculated for Pb-Sn, Al-Sn, and In-Zn systems by asymmetric and symmetric methods.
| System | Step | MIVM | RSM | Wilson | NRTL |
|---|
| Asym | Sym | Asym | Sym | Asym | Sym | Asym | Sym |
|---|
| Bij | Bji | Bij | Bji | w/kT | w/kT | Aij | Aji | Aij | Aji | τij | τji | τij | τji |
|---|
| Pb50-Sn50 (1050 K) | 0–1000 | 0.87 | 0.97 | 0.98 | 0.94 | 1.08 | 0.25 | 0.85 | 1.00 | 0.83 | 1.12 | 0.03 | 0.14 | 0.06 | 0.02 |
| 1000–2000 | 0.84 | 0.96 | 1.01 | 0.98 | 1.49 | 0.04 | 0.84 | 0.96 | 0.86 | 1.16 | 0.05 | 0.18 | 0.02 | −0.01 |
| 2000–3000 | 0.94 | 1.09 | 0.96 | 1.02 | 0.14 | 0.06 | 0.95 | 1.08 | 0.90 | 1.09 | −0.08 | 0.06 | −0.02 | 0.04 |
| 3000–4000 | 1.09 | 0.98 | 0.92 | 1.02 | 0.37 | 0.15 | 0.86 | 1.24 | 0.90 | 1.05 | 0.02 | −0.08 | −0.02 | 0.09 |
| 4000–5000 | 0.94 | 1.05 | 1.01 | 0.98 | 0.09 | 0.05 | 0.92 | 1.08 | 0.86 | 1.15 | −0.05 | 0.06 | 0.02 | −0.01 |
| 0–2000 | 0.95 | 0.98 | 0.95 | 1.02 | 0.51 | 0.11 | 0.85 | 1.08 | 0.89 | 1.09 | 0.03 | 0.05 | −0.02 | 0.05 |
| 0–3000 | 0.87 | 1.00 | 0.96 | 1.02 | 0.86 | 0.09 | 0.88 | 1.00 | 0.89 | 1.09 | 0.00 | 0.13 | −0.02 | 0.05 |
| 0–4000 | 0.99 | 1.00 | 0.95 | 1.02 | 0.11 | 0.10 | 0.87 | 1.13 | 0.89 | 1.08 | 0.01 | 0.01 | −0.02 | 0.06 |
| 0–5000 | 0.98 | 0.99 | 0.94 | 1.01 | 0.18 | 0.16 | 0.87 | 1.12 | 0.89 | 1.07 | 0.01 | 0.02 | −0.01 | 0.07 |
| Al50-Sn50 (973 K) | 0–1000 | 1.14 | 0.79 | 1.18 | 0.70 | 0.54 | 0.50 | 1.19 | 0.76 | 1.06 | 0.78 | 0.24 | −0.13 | 0.36 | −0.17 |
| 1000–2000 | 0.89 | 0.64 | 0.98 | 0.64 | 3.50 | 1.18 | 0.97 | 0.59 | 0.96 | 0.65 | 0.44 | 0.12 | 0.45 | 0.02 |
| 2000–3000 | 0.90 | 0.68 | 0.93 | 0.60 | 3.01 | 1.48 | 1.02 | 0.60 | 0.90 | 0.62 | 0.39 | 0.10 | 0.51 | 0.07 |
| 3000–4000 | 0.88 | 0.59 | 0.95 | 0.59 | 3.93 | 1.46 | 0.89 | 0.58 | 0.89 | 0.63 | 0.53 | 0.13 | 0.53 | 0.05 |
| 4000–5000 | 0.93 | 0.66 | 0.89 | 0.56 | 2.90 | 1.76 | 0.99 | 0.62 | 0.85 | 0.59 | 0.42 | 0.07 | 0.58 | 0.12 |
| 0–2000 | 1.08 | 0.79 | 1.08 | 0.67 | 0.89 | 0.83 | 1.18 | 0.72 | 1.01 | 0.72 | 0.24 | −0.08 | 0.40 | −0.08 |
| 0–3000 | 1.05 | 0.78 | 1.03 | 0.65 | 1.11 | 1.05 | 1.17 | 0.70 | 0.98 | 0.68 | 0.26 | −0.05 | 0.43 | −0.03 |
| 0–4000 | 1.03 | 0.76 | 1.01 | 0.63 | 1.32 | 1.15 | 1.15 | 0.69 | 0.95 | 0.67 | 0.27 | −0.03 | 0.46 | −0.01 |
| 0–5000 | 1.01 | 0.75 | 0.98 | 0.62 | 1.55 | 1.27 | 1.12 | 0.67 | 0.93 | 0.65 | 0.29 | −0.01 | 0.48 | 0.02 |
| In50-Zn50 (730 K) | 0–1000 | 0.95 | 1.08 | 0.81 | 1.22 | 0.17 | 0.24 | 0.66 | 1.56 | 0.74 | 1.33 | −0.08 | 0.05 | −0.20 | 0.21 |
| 1000–2000 | 0.93 | 1.05 | 0.86 | 1.14 | 0.16 | 0.00 | 0.64 | 1.53 | 0.69 | 1.41 | −0.05 | 0.07 | −0.13 | 0.15 |
| 2000–3000 | 0.85 | 0.89 | 0.79 | 1.04 | 1.61 | 0.42 | 0.54 | 1.39 | 0.64 | 1.29 | 0.12 | 0.16 | −0.04 | 0.24 |
| 3000–4000 | 0.93 | 1.06 | 0.73 | 1.04 | 0.09 | 0.94 | 0.65 | 1.52 | 0.63 | 1.20 | −0.06 | 0.08 | −0.04 | 0.32 |
| 4000–5000 | 0.76 | 0.96 | 0.74 | 0.96 | 1.88 | 0.78 | 0.58 | 1.25 | 0.58 | 1.22 | 0.05 | 0.27 | 0.04 | 0.30 |
| 0–2000 | 0.92 | 1.04 | 0.79 | 1.13 | 0.24 | 0.58 | 0.64 | 1.51 | 0.69 | 1.30 | −0.04 | 0.09 | −0.13 | 0.23 |
| 0–3000 | 0.88 | 1.01 | 0.76 | 1.17 | 0.66 | 0.47 | 0.62 | 1.44 | 0.71 | 1.25 | −0.01 | 0.13 | −0.16 | 0.28 |
| 0–4000 | 0.91 | 1.00 | 0.75 | 1.14 | 0.58 | 0.59 | 0.61 | 1.49 | 0.69 | 1.23 | 0.00 | 0.10 | −0.13 | 0.29 |
| 0–5000 | 0.88 | 0.98 | 0.83 | 1.10 | 0.82 | 0.16 | 0.60 | 1.45 | 0.67 | 1.36 | 0.02 | 0.12 | −0.09 | 0.18 |
Table 6.
Comparison of experimental and calculated values of molar excess Gibbs energy and activity for Pb-Sn alloys all at full concentration in the Miedema model at 1050 K.
| Molar Excess Gibbs Energy (J/mol) |
|---|
| xi | Exp | Ding | Tanaka | Sommer |
|---|
| 0.9 | 436 | 334 | 393 | 387 |
| 0.8 | 801 | 598 | 704 | 693 |
| 0.7 | 1085 | 791 | 931 | 916 |
| 0.6 | 1279 | 910 | 1071 | 1054 |
| 0.5 | 1373 | 955 | 1124 | 1106 |
| 0.4 | 1356 | 923 | 1087 | 1069 |
| 0.3 | 1221 | 814 | 958 | 942 |
| 0.2 | 956 | 624 | 735 | 723 |
| 0.1 | 552 | 354 | 416 | 410 |
| ARD% | 30.11% | 17.73% | 19.05% |
| SD | 325 | 197 | 210 |
| Activity |
| xi | ai-Exp | aj-Exp | ai-Ding | aj-Ding | ai-Tanaka | aj-Tanaka | ai-Sommer | aj-Sommer |
| 0.9 | 0.904 | 0.159 | 0.904 | 0.141 | 0.904 | 0.150 | 0.904 | 0.149 |
| 0.8 | 0.814 | 0.296 | 0.813 | 0.264 | 0.815 | 0.277 | 0.815 | 0.276 |
| 0.7 | 0.730 | 0.412 | 0.726 | 0.372 | 0.731 | 0.387 | 0.731 | 0.385 |
| 0.6 | 0.650 | 0.512 | 0.641 | 0.470 | 0.649 | 0.483 | 0.648 | 0.482 |
| 0.5 | 0.572 | 0.599 | 0.556 | 0.560 | 0.566 | 0.571 | 0.565 | 0.570 |
| 0.4 | 0.492 | 0.677 | 0.467 | 0.646 | 0.480 | 0.654 | 0.478 | 0.653 |
| 0.3 | 0.405 | 0.752 | 0.371 | 0.730 | 0.385 | 0.735 | 0.384 | 0.735 |
| 0.2 | 0.303 | 0.827 | 0.265 | 0.815 | 0.279 | 0.818 | 0.277 | 0.818 |
| 0.1 | 0.174 | 0.908 | 0.144 | 0.904 | 0.153 | 0.905 | 0.152 | 0.905 |
| ARD% of Single Component | 5.37% | 6.18% | 3.22% | 3.89% | 3.43% | 4.13% |
| SD of Single Component | 0.022 | 0.029 | 0.013 | 0.02 | 0.014 | 0.021 |
| ARD% | 5.77% | 3.55% | 3.78% |
| SD | 0.026 | 0.017 | 0.018 |
Table 7.
Comparison of experimental and calculated values of molar excess Gibbs energy and activity for Al-Sn alloys all at full concentration in the Miedema model at 973 K.
| Molar Excess Gibbs Energy (J/mol) |
|---|
| xi | Exp | Ding | Tanaka | Sommer |
|---|
| 0.9 | 1318 | 1130 | 1266 | 1149 |
| 0.8 | 2194 | 1937 | 2171 | 1970 |
| 0.7 | 2703 | 2454 | 2750 | 2498 |
| 0.6 | 2911 | 2711 | 3039 | 2761 |
| 0.5 | 2870 | 2733 | 3063 | 2784 |
| 0.4 | 2624 | 2542 | 2849 | 2591 |
| 0.3 | 2200 | 2158 | 2418 | 2200 |
| 0.2 | 1619 | 1596 | 1788 | 1628 |
| 0.1 | 887 | 872 | 977 | 890 |
| ARD% | 6.11% | 6.34% | 4.55% |
| SD | 160 | 147 | 130 |
| Activity |
| xi | ai-Exp | aj-Exp | ai-Ding | aj-Ding | ai-Tanaka | aj-Tanaka | ai-Sommer | aj-Sommer |
| 0.9 | 0.927 | 0.393 | 0.919 | 0.335 | 0.921 | 0.388 | 0.919 | 0.343 |
| 0.8 | 0.887 | 0.514 | 0.864 | 0.487 | 0.872 | 0.542 | 0.865 | 0.495 |
| 0.7 | 0.859 | 0.567 | 0.823 | 0.566 | 0.839 | 0.611 | 0.825 | 0.573 |
| 0.6 | 0.828 | 0.606 | 0.784 | 0.618 | 0.810 | 0.652 | 0.788 | 0.624 |
| 0.5 | 0.782 | 0.650 | 0.740 | 0.664 | 0.776 | 0.687 | 0.745 | 0.668 |
| 0.4 | 0.711 | 0.702 | 0.680 | 0.711 | 0.725 | 0.726 | 0.686 | 0.714 |
| 0.3 | 0.609 | 0.762 | 0.591 | 0.766 | 0.642 | 0.774 | 0.599 | 0.768 |
| 0.2 | 0.468 | 0.831 | 0.461 | 0.831 | 0.510 | 0.835 | 0.468 | 0.832 |
| 0.1 | 0.274 | 0.909 | 0.271 | 0.908 | 0.306 | 0.909 | 0.277 | 0.908 |
| ARD% of Single Component | 3.12% | 2.93% | 3.94% | 3.68% | 2.58% | 2.89% |
| SD of Single Component | 0.028 | 0.022 | 0.024 | 0.028 | 0.025 | 0.021 |
| ARD% | 3.03% | 3.81% | 2.74% |
| SD | 0.025 | 0.026 | 0.023 |
Table 8.
Comparison of experimental and calculated values of molar excess Gibbs energy and activity for In-Zn alloys all at full concentration in the Miedema model at 730 K.
| Molar Excess Gibbs Energy (J/mol) |
|---|
| xi | Exp | Ding | Tanaka | Sommer |
|---|
| 0.9 | 726 | 679 | 752 | 523 |
| 0.8 | 1321 | 1241 | 1375 | 961 |
| 0.7 | 1785 | 1677 | 1859 | 1305 |
| 0.6 | 2112 | 1975 | 2190 | 1546 |
| 0.5 | 2288 | 2124 | 2354 | 1670 |
| 0.4 | 2295 | 2107 | 2335 | 1666 |
| 0.3 | 2105 | 1907 | 2114 | 1516 |
| 0.2 | 1686 | 1506 | 1669 | 1203 |
| 0.1 | 1000 | 879 | 974 | 706 |
| ARD% | 8.08% | 2.68% | 27.71% |
| SD | 144 | 50 | 491 |
| Activity |
| xi | ai-Exp | aj-Exp | ai-Ding | aj-Ding | ai-Tanaka | aj-Tanaka | ai-Sommer | aj-Sommer |
| 0.9 | 0.910 | 0.300 | 0.908 | 0.281 | 0.909 | 0.315 | 0.906 | 0.223 |
| 0.8 | 0.835 | 0.500 | 0.832 | 0.475 | 0.836 | 0.522 | 0.823 | 0.393 |
| 0.7 | 0.772 | 0.636 | 0.769 | 0.606 | 0.777 | 0.653 | 0.750 | 0.522 |
| 0.6 | 0.719 | 0.728 | 0.716 | 0.692 | 0.730 | 0.735 | 0.685 | 0.619 |
| 0.5 | 0.674 | 0.788 | 0.671 | 0.750 | 0.692 | 0.784 | 0.625 | 0.693 |
| 0.4 | 0.636 | 0.827 | 0.628 | 0.792 | 0.659 | 0.816 | 0.567 | 0.752 |
| 0.3 | 0.598 | 0.854 | 0.578 | 0.828 | 0.621 | 0.843 | 0.501 | 0.803 |
| 0.2 | 0.542 | 0.882 | 0.502 | 0.867 | 0.555 | 0.874 | 0.414 | 0.855 |
| 0.1 | 0.407 | 0.925 | 0.350 | 0.920 | 0.401 | 0.922 | 0.272 | 0.916 |
| ARD% of Single Component | 3.07% | 3.93% | 1.81% | 1.91% | 11.16% | 12.34% |
| SD of Single Component | 0.024 | 0.027 | 0.014 | 0.012 | 0.077 | 0.082 |
| ARD% | 3.50% | 1.86% | 11.75% |
| SD | 0.026 | 0.013 | 0.079 |
Table 9.
The SD and ARD of molar excess Gibbs energy and activity of Pb50Sn50 alloys were estimated by asymmetric method at 1050 K.
| GEm and a | Step | MIVM | RSM | Wilson | NRTL |
|---|
| ARD% | SD | ARD% | SD | ARD% | SD | ARD% | SD |
|---|
| GEm (J/mol) | 0~1000 | 61.4% | 642 | 72.4% | 760 | 74.2% | 787 | 73.7% | 782 |
| 1000~2000 | 107.9% | 1131 | 137.0% | 1441 | 66.2% | 704 | 65.9% | 701 |
| 2000~3000 | 131.5% | 1390 | 121.8% | 1288 | 104.0% | 1100 | 103.3% | 1093 |
| 3000~4000 | 166.8% | 1758 | 159.8% | 1685 | 112.5% | 1190 | 109.8% | 1161 |
| 4000~5000 | 91.2% | 965 | 86.0% | 911 | 98.3% | 1040 | 97.7% | 1034 |
| 0~2000 | 25.4% | 265 | 37.0% | 388 | 79.4% | 939 | 87.6% | 928 |
| 0~3000 | 22.7% | 250 | 18.1% | 199 | 88.7% | 842 | 79.2% | 839 |
| 0~4000 | 85.1% | 902 | 82.6% | 876 | 98.8% | 1045 | 97.4% | 1031 |
| 0~5000 | 74.7% | 793 | 71.6% | 760 | 97.1% | 1027 | 95.8% | 1014 |
| Average | 85.2% | 899 | 87.4% | 923 | 91.0% | 964 | 90.0% | 954 |
| a | 0~1000 | 13.4% | 0.057 | 16.2% | 0.069 | 13.1% | 0.058 | 13.0% | 0.057 |
| 1000~2000 | 25.8% | 0.108 | 34.8% | 0.144 | 11.9% | 0.052 | 11.8% | 0.052 |
| 2000~3000 | 21.2% | 0.094 | 20.0% | 0.088 | 17.5% | 0.077 | 17.5% | 0.077 |
| 3000~4000 | 25.6% | 0.114 | 24.7% | 0.11 | 18.7% | 0.083 | 18.4% | 0.081 |
| 4000~5000 | 15.7% | 0.069 | 14.9% | 0.066 | 16.7% | 0.074 | 16.7% | 0.074 |
| 0~2000 | 5.2% | 0.022 | 7.8% | 0.034 | 15.3% | 0.068 | 15.1% | 0.067 |
| 0~3000 | 4.5% | 0.021 | 3.7% | 0.017 | 13.9% | 0.061 | 13.9% | 0.061 |
| 0~4000 | 14.8% | 0.065 | 14.4% | 0.064 | 16.8% | 0.074 | 16.6% | 0.073 |
| 0~5000 | 13.2% | 0.058 | 12.7% | 0.056 | 16.5% | 0.073 | 16.4% | 0.072 |
| Average | 15.5% | 0.068 | 16.6% | 0.072 | 15.6% | 0.069 | 15.5% | 0.068 |
Table 10.
The SD and ARD of molar excess Gibbs energy and activity of Pb50Sn50 alloys were estimated by symmetric method at 1050 K.
| GEm and a | Step | MIVM | RSM | Wilson | NRTL |
|---|
| ARD% | SD | ARD% | SD | ARD% | SD | ARD% | SD |
|---|
| GEm (J/mol) | 0~1000 | 14.2% | 171 | 59.7% | 632 | 88.7% | 939 | 85.9% | 911 |
| 1000~2000 | 117.3% | 1239 | 106.0% | 1122 | 104.6% | 1106 | 102.4% | 1083 |
| 2000~3000 | 83.3% | 883 | 90.8% | 962 | 97.5% | 1032 | 96.8% | 1024 |
| 3000~4000 | 53.1% | 565 | 76.7% | 813 | 92.1% | 976 | 91.7% | 971 |
| 4000~5000 | 123.7% | 1306 | 108.5% | 1148 | 105.7% | 1118 | 103.3% | 1092 |
| 0~2000 | 62.3% | 662 | 82.5% | 874 | 94.3% | 998 | 93.5% | 990 |
| 0~3000 | 71.7% | 761 | 86.2% | 914 | 95.8% | 1014 | 95.0% | 1006 |
| 0~4000 | 66.8% | 710 | 83.9% | 889 | 94.9% | 1004 | 94.2% | 997 |
| 0~5000 | 45.3% | 483 | 74.3% | 788 | 91.5% | 969 | 90.8% | 962 |
| Average | 70.8% | 753 | 85.4% | 905 | 96.1% | 1017 | 94.8% | 1004 |
| a | 0~1000 | 3.2% | 0.016 | 13.7% | 0.06 | 15.3% | 0.068 | 15.0% | 0.066 |
| 1000~2000 | 19.4% | 0.086 | 17.4% | 0.077 | 17.6% | 0.078 | 17.3% | 0.076 |
| 2000~3000 | 14.5% | 0.064 | 16.3% | 0.072 | 16.6% | 0.073 | 16.5% | 0.073 |
| 3000~4000 | 9.7% | 0.043 | 15.2% | 0.067 | 15.8% | 0.07 | 15.8% | 0.07 |
| 4000~5000 | 20.2% | 0.09 | 17.5% | 0.077 | 17.8% | 0.079 | 17.4% | 0.077 |
| 0~2000 | 11.2% | 0.049 | 15.7% | 0.069 | 16.1% | 0.071 | 16.0% | 0.071 |
| 0~3000 | 12.7% | 0.056 | 16.0% | 0.07 | 16.3% | 0.072 | 16.3% | 0.072 |
| 0~4000 | 12.0% | 0.053 | 15.8% | 0.07 | 16.2% | 0.072 | 16.1% | 0.071 |
| 0~5000 | 8.4% | 0.037 | 15.0% | 0.066 | 15.7% | 0.069 | 15.6% | 0.069 |
| Average | 12.4% | 0.055 | 15.8% | 0.07 | 16.4% | 0.072 | 16.2% | 0.072 |
Table 11.
The SD and ARD of molar excess Gibbs energy and activity of Al50Sn50 alloys were estimated by asymmetric method at 973 K.
| GEm and a | Step | MIVM | RSM | Wilson | NRTL |
|---|
| ARD% | SD | ARD% | SD | ARD% | SD | ARD% | SD |
|---|
| GEm (J/mol) | 0~1000 | 60.4% | 1359 | 43.6% | 986 | 94.9% | 2139 | 92.0% | 2075 |
| 1000~2000 | 71.1% | 1600 | 100.0% | 2242 | 65.5% | 1481 | 60.0% | 1361 |
| 2000~3000 | 56.5% | 1270 | 76.4% | 1715 | 69.8% | 1577 | 64.7% | 1467 |
| 3000~4000 | 89.5% | 2017 | 120.7% | 2716 | 59.1% | 1338 | 52.6% | 1197 |
| 4000~5000 | 51.1% | 1154 | 70.5% | 1587 | 69.7% | 1574 | 64.6% | 1464 |
| 0~2000 | 55.6% | 1249 | 38.5% | 881 | 91.3% | 2057 | 88.0% | 1985 |
| 0~3000 | 38.1% | 858 | 23.3% | 550 | 88.8% | 2001 | 85.3% | 1925 |
| 0~4000 | 22.4% | 506 | 9.1% | 253 | 86.3% | 1946 | 82.6% | 1866 |
| 0~5000 | 6.0% | 148 | 10.5% | 227 | 83.6% | 1885 | 79.7% | 1801 |
| Average | 50.1% | 1129 | 54.7% | 1240 | 78.8% | 1778 | 74.4% | 1682 |
| a | 0~1000 | 20.2% | 0.132 | 15.7% | 0.104 | 30.9% | 0.202 | 30.7% | 0.201 |
| 1000~2000 | 50.8% | 0.33 | 88.8% | 0.565 | 23.2% | 0.156 | 22.6% | 0.15 |
| 2000~3000 | 36.6% | 0.236 | 57.4% | 0.365 | 24.5% | 0.164 | 23.8% | 0.157 |
| 3000~4000 | 72.9% | 0.483 | 126.6% | 0.809 | 21.4% | 0.145 | 20.9% | 0.139 |
| 4000~5000 | 32.1% | 0.209 | 51.1% | 0.325 | 24.4% | 0.163 | 24.0% | 0.158 |
| 0~2000 | 20.4% | 0.134 | 14.8% | 0.104 | 30.0% | 0.197 | 29.7% | 0.194 |
| 0~3000 | 14.8% | 0.098 | 9.9% | 0.074 | 29.4% | 0.193 | 29.0% | 0.19 |
| 0~4000 | 9.1% | 0.061 | 6.6% | 0.051 | 28.8% | 0.19 | 28.4% | 0.186 |
| 0~5000 | 2.8% | 0.022 | 8.3% | 0.054 | 28.1% | 0.186 | 27.7% | 0.182 |
| Average | 28.8% | 0.256 | 58.3% | 0.376 | 26.7% | 0.177 | 26.3% | 0.173 |
Table 12.
The SD and ARD of molar excess Gibbs energy and activity of Al50Sn50 alloys were estimated by symmetric method at 973 K.
| GEm and a | Step | MIVM | RSM | Wilson | NRTL |
|---|
| ARD% | SD | ARD% | SD | ARD% | SD | ARD% | SD |
|---|
| GEm (J/mol) | 0~1000 | 45.1% | 1008 | 65.2% | 1484 | 87.9% | 1982 | 84.5% | 1906 |
| 1000~2000 | 37.9% | 862 | 17.8% | 466 | 71.1% | 1606 | 66.0% | 1495 |
| 2000~3000 | 67.9% | 1536 | 12.3% | 236 | 63.6% | 1439 | 57.6% | 1308 |
| 3000~4000 | 63.0% | 1428 | 12.2% | 233 | 63.7% | 1443 | 57.6% | 1307 |
| 4000~5000 | 106.1% | 2095 | 23.4% | 546 | 56.9% | 1290 | 49.9% | 1136 |
| 0~2000 | 4.4% | 87 | 41.8% | 970 | 79.5% | 1795 | 75.4% | 1703 |
| 0~3000 | 29.8% | 685 | 27.1% | 655 | 74.3% | 1678 | 69.6% | 1574 |
| 0~4000 | 44.9% | 1026 | 19.9% | 508 | 71.7% | 1620 | 66.6% | 1508 |
| 0~5000 | 63.1% | 1435 | 12.9% | 349 | 68.7% | 1553 | 63.3% | 1434 |
| Average | 51.4% | 1129 | 25.8% | 605 | 70.8% | 1601 | 65.6% | 1486 |
| a | 0~1000 | 16.2% | 0.104 | 27.8% | 0.183 | 29.2% | 0.192 | 29.4% | 0.192 |
| 1000~2000 | 22.0% | 0.145 | 20.4% | 0.137 | 24.8% | 0.165 | 24.6% | 0.162 |
| 2000~3000 | 47.7% | 0.315 | 16.1% | 0.112 | 22.7% | 0.153 | 22.4% | 0.148 |
| 3000~4000 | 42.9% | 0.285 | 16.5% | 0.114 | 22.8% | 0.153 | 22.6% | 0.149 |
| 4000~5000 | 77.8% | 0.521 | 12.1% | 0.089 | 20.7% | 0.141 | 20.3% | 0.135 |
| 0~2000 | 1.6% | 0.01 | 24.4% | 0.162 | 27.1% | 0.179 | 27.1% | 0.178 |
| 0~3000 | 15.8% | 0.105 | 22.0% | 0.147 | 25.7% | 0.171 | 25.6% | 0.169 |
| 0~4000 | 25.2% | 0.167 | 20.7% | 0.139 | 25.0% | 0.166 | 24.9% | 0.164 |
| 0~5000 | 38.1% | 0.252 | 19.2% | 0.13 | 24.2% | 0.161 | 24.0% | 0.158 |
| Average | 31.9% | 0.212 | 19.9% | 0.135 | 24.7% | 0.165 | 24.6% | 0.162 |
Table 13.
The SD and ARD of molar excess Gibbs energy and activity of In50Zn50 alloys were estimated by asymmetric method at 730 K.
| GEm and a | Step | MIVM | RSM | Wilson | NRTL |
|---|
| ARD% | SD | ARD% | SD | ARD% | SD | ARD% | SD |
|---|
| GEm (J/mol) | 0~1000 | 123.0% | 2197 | 111.2% | 1986 | 108.0% | 1929 | 101.8% | 1818 |
| 1000~2000 | 98.4% | 1757 | 89.5% | 1598 | 104.4% | 1865 | 98.2% | 1753 |
| 2000~3000 | 8.4% | 140 | 8.4% | 143 | 89.2% | 1591 | 81.8% | 1463 |
| 3000~4000 | 103.8% | 1855 | 93.8% | 1675 | 105.0% | 1874 | 98.9% | 1767 |
| 4000~5000 | 12.5% | 230 | 22.9% | 420 | 84.7% | 1511 | 80.1% | 1432 |
| 0~2000 | 92.2% | 1647 | 84.0% | 1500 | 103.3% | 1844 | 97.2% | 1736 |
| 0~3000 | 63.2% | 1131 | 57.1% | 1019 | 98.5% | 1758 | 92.7% | 1655 |
| 0~4000 | 67.9% | 1215 | 62.0% | 1108 | 100.0% | 1785 | 93.6% | 1671 |
| 0~5000 | 51.3% | 921 | 46.1% | 826 | 97.2% | 1735 | 90.9% | 1625 |
| Average | 69.0% | 1233 | 63.9% | 1142 | 98.9% | 1766 | 92.8% | 1658 |
| a | 0~1000 | 38.2% | 0.259 | 34.6% | 0.234 | 35.2% | 0.238 | 33.9% | 0.229 |
| 1000~2000 | 33.0% | 0.224 | 32.2% | 0.218 | 34.4% | 0.233 | 33.0% | 0.223 |
| 2000~3000 | 5.3% | 0.036 | 6.9% | 0.046 | 30.8% | 0.21 | 28.9% | 0.196 |
| 3000~4000 | 34.2% | 0.232 | 31.9% | 0.216 | 34.5% | 0.233 | 33.2% | 0.225 |
| 4000~5000 | 6.8% | 0.047 | 13.9% | 0.096 | 29.6% | 0.202 | 28.4% | 0.193 |
| 0~2000 | 31.6% | 0.214 | 29.5% | 0.2 | 34.1% | 0.231 | 32.8% | 0.222 |
| 0~3000 | 23.8% | 0.162 | 21.8% | 0.15 | 33.0% | 0.224 | 31.7% | 0.215 |
| 0~4000 | 25.2% | 0.171 | 23.4% | 0.16 | 33.4% | 0.226 | 31.9% | 0.216 |
| 0~5000 | 20.1% | 0.138 | 18.3% | 0.128 | 32.7% | 0.222 | 31.2% | 0.212 |
| Average | 24.2% | 0.165 | 23.6% | 0.161 | 33.1% | 0.224 | 31.7% | 0.215 |
Table 14.
The SD and ARD of molar excess Gibbs energy and activity of In50Zn50 alloys were estimated by symmetric method at 730 K.
| GEm and a | Step | MIVM | RSM | Wilson | NRTL |
|---|
| ARD% | SD | ARD% | SD | ARD% | SD | ARD% | SD |
|---|
| GEm (J/mol) | 0~1000 | 90.9% | 1621 | 84.0% | 1500 | 98.0% | 1750 | 94.6% | 1690 |
| 1000~2000 | 113.6% | 2029 | 100.0% | 1785 | 104.7% | 1869 | 99.7% | 1781 |
| 2000~3000 | 43.9% | 784 | 72.6% | 1294 | 94.0% | 1678 | 89.2% | 1594 |
| 3000~4000 | 9.6% | 178 | 38.3% | 692 | 82.5% | 1472 | 79.4% | 1419 |
| 4000~5000 | 10.2% | 192 | 48.9% | 868 | 85.6% | 1528 | 80.6% | 1442 |
| 0~2000 | 46.4% | 824 | 62.3% | 1111 | 91.8% | 1639 | 88.4% | 1579 |
| 0~3000 | 60.2% | 1070 | 69.2% | 1240 | 91.8% | 1640 | 89.1% | 1592 |
| 0~4000 | 42.0% | 744 | 61.4% | 1102 | 89.4% | 1597 | 86.7% | 1549 |
| 0~5000 | 85.9% | 1535 | 89.4% | 1595 | 100.7% | 1798 | 95.7% | 1710 |
| Average | 55.9% | 997 | 69.6% | 1243 | 93.2% | 1663 | 89.3% | 1595 |
| a | 0~1000 | 31.3% | 0.212 | 31.2% | 0.211 | 32.9% | 0.223 | 32.4% | 0.219 |
| 1000~2000 | 36.3% | 0.246 | 33.4% | 0.226 | 34.5% | 0.233 | 33.4% | 0.226 |
| 2000~3000 | 17.6% | 0.12 | 29.9% | 0.203 | 32.0% | 0.217 | 30.9% | 0.209 |
| 3000~4000 | 5.1% | 0.036 | 24.1% | 0.165 | 29.1% | 0.198 | 28.3% | 0.192 |
| 4000~5000 | 5.7% | 0.04 | 26.2% | 0.179 | 29.9% | 0.204 | 28.6% | 0.194 |
| 0~2000 | 18.5% | 0.125 | 27.9% | 0.189 | 31.4% | 0.214 | 30.8% | 0.208 |
| 0~3000 | 22.9% | 0.154 | 29.3% | 0.199 | 31.4% | 0.213 | 31.1% | 0.211 |
| 0~4000 | 17.0% | 0.114 | 28.1% | 0.191 | 30.8% | 0.21 | 30.4% | 0.206 |
| 0~5000 | 30.0% | 0.203 | 32.1% | 0.218 | 33.6% | 0.227 | 32.5% | 0.22 |
| Average | 20.5% | 0.139 | 29.1% | 0.198 | 31.7% | 0.215 | 30.9% | 0.21 |