A Creep Constitutive Model, Based on Deformation Mechanisms and Its Application to Creep Crack Growth
Abstract
:1. Introduction
2. Constitutive Model, Based on the Deformation Mechanism under a Wide Range of Stress Levels
2.1. Constitutive Model in a LS Region
2.2. Constitutive Model in a HS Region
2.3. Constitutive Model for a Wide Range of Stress Levels
3. Finite Element and Numerical Procedures
3.1. Material
3.2. Finite Element Model
3.3. Creep Damage Model and the Creep Crack Growth Simulation
3.4. Multiaxial Creep Ductility Model
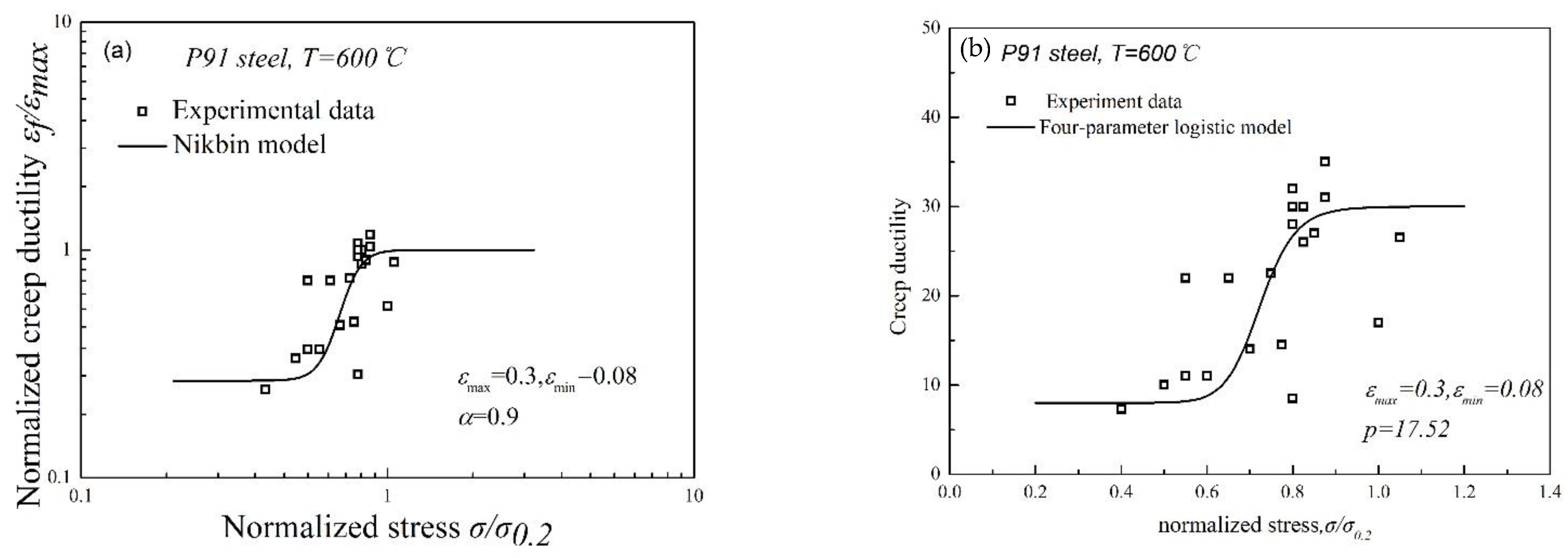
3.4.1. Stress-Independent Multiaxial Creep Ductility Model
3.4.2. Stress-Dependent Multiaxial Creep Ductility Model
4. Results and Discussion
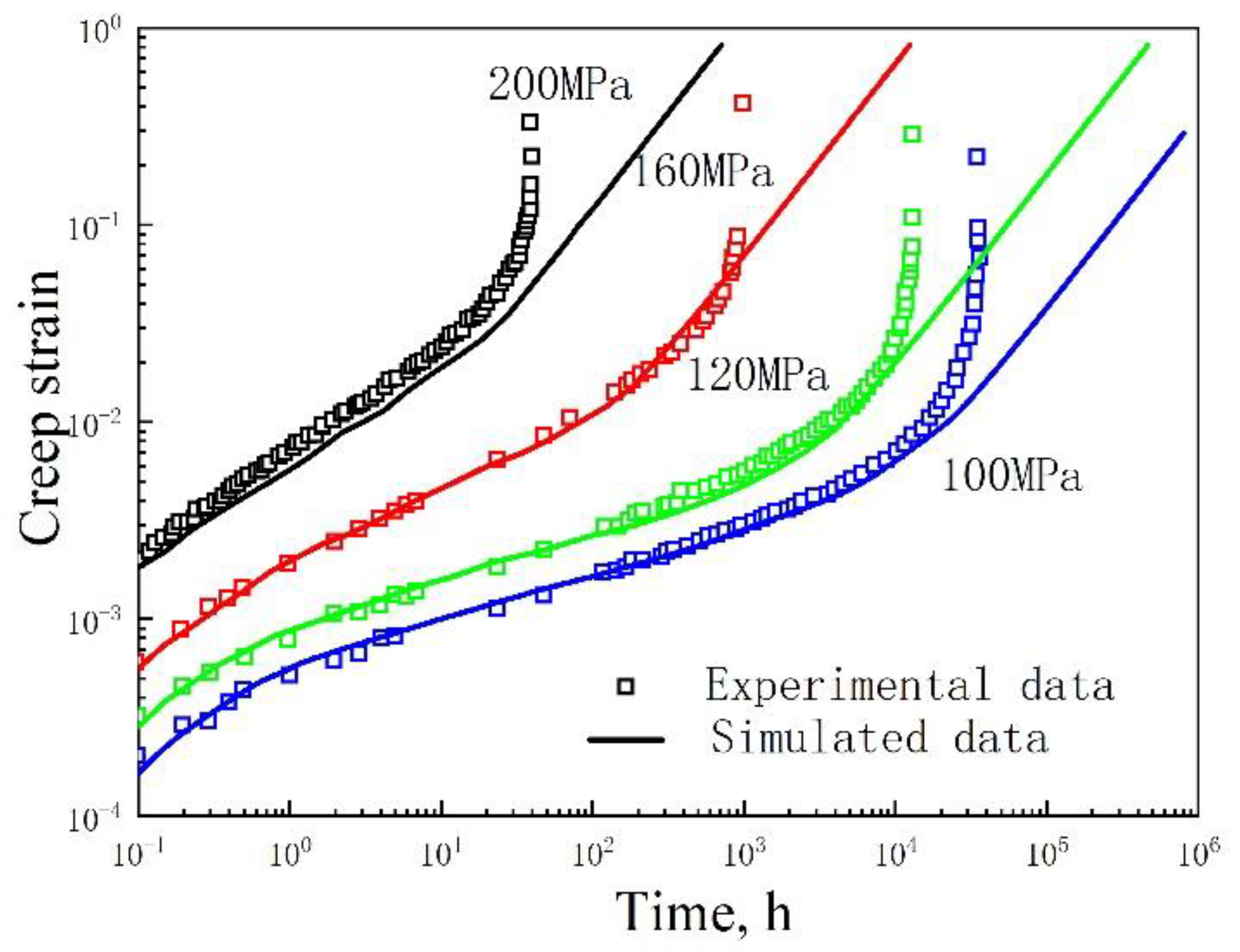
4.1. Comparison of the Uniaxial Tension Test with the Simulation under Different Levels of Stress
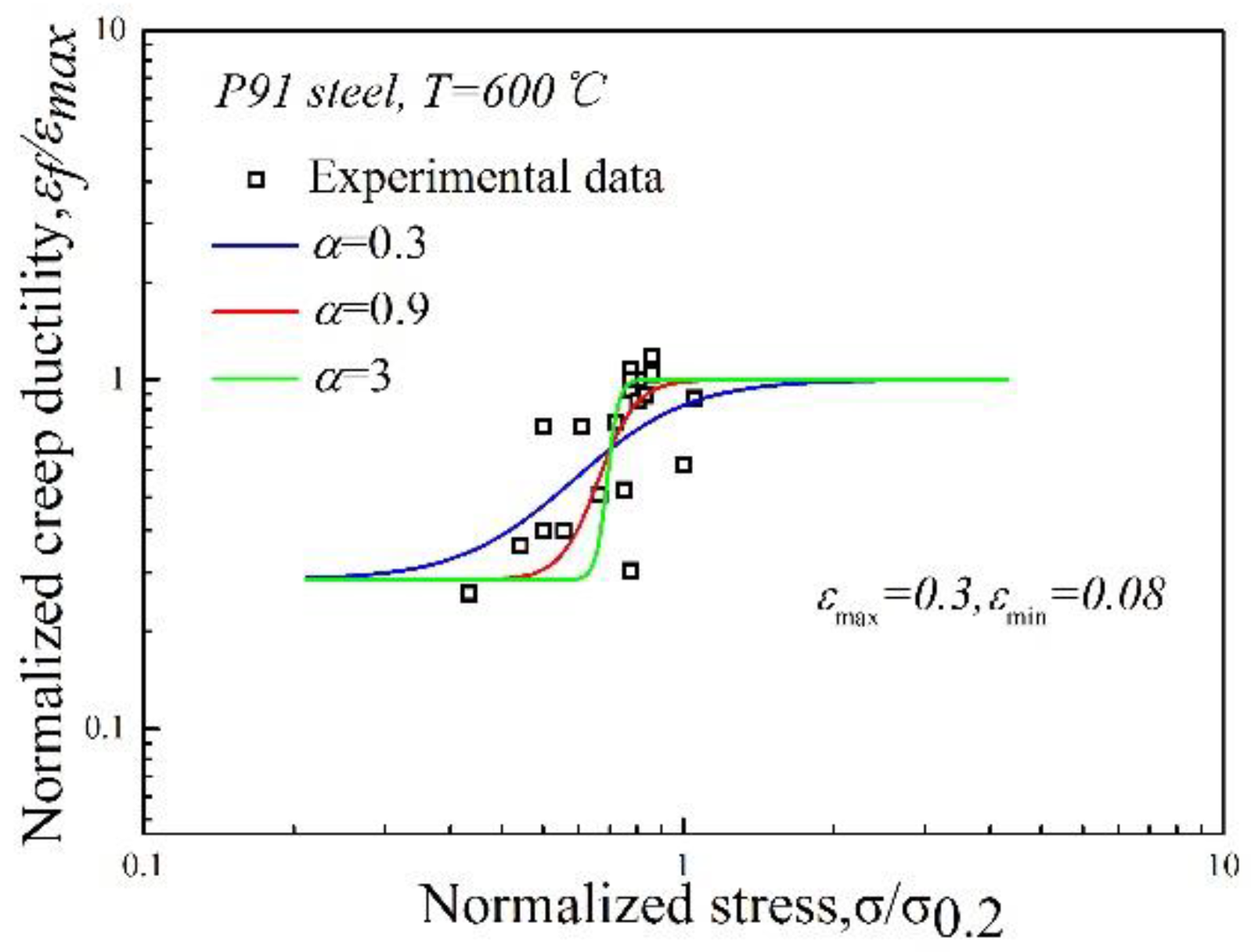
4.2. Effect of α on the CCG Rate
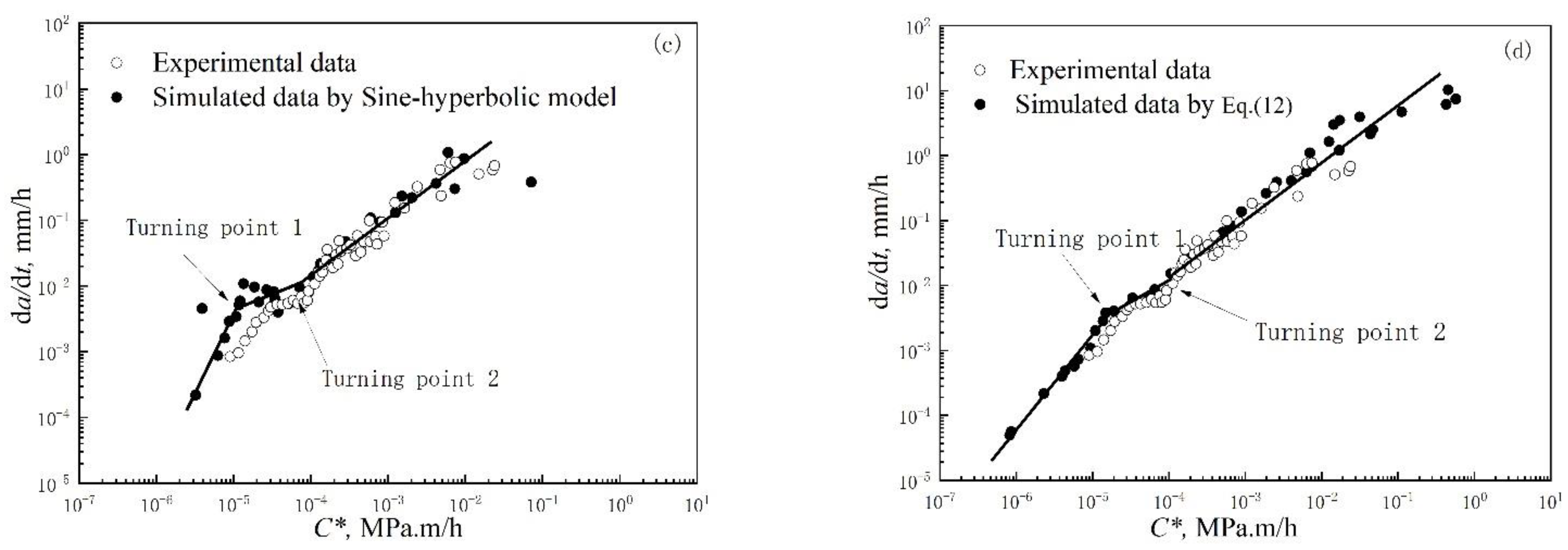
4.3. Effect of the Different Constitutive Models on the CCG Rate
4.4. Creep Crack Growth Prediction for Pressure Pipes with the Axial Surface Cracks
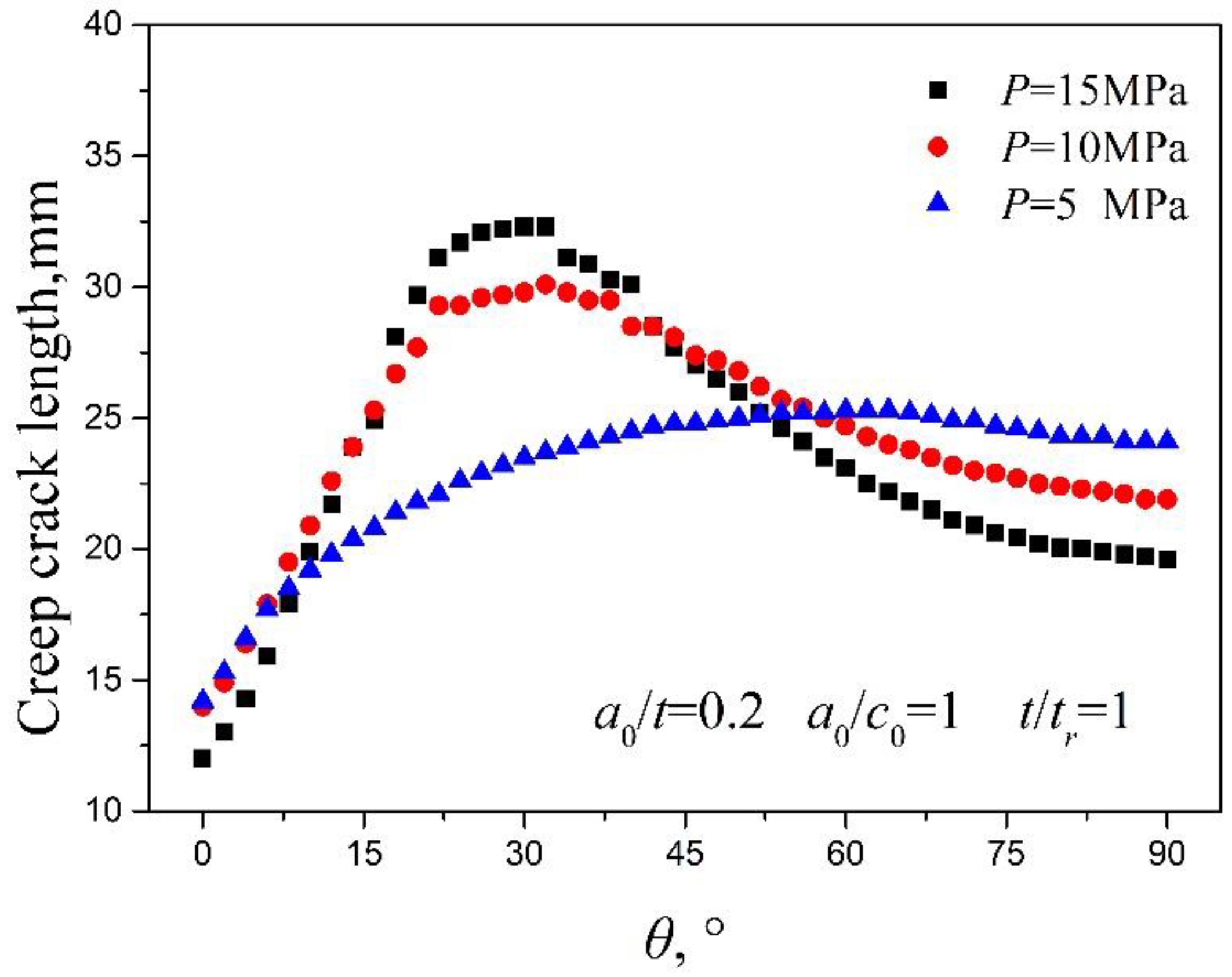
4.4.1. Effect of the Internal Pressure on the Creep Crack Growth Behavior
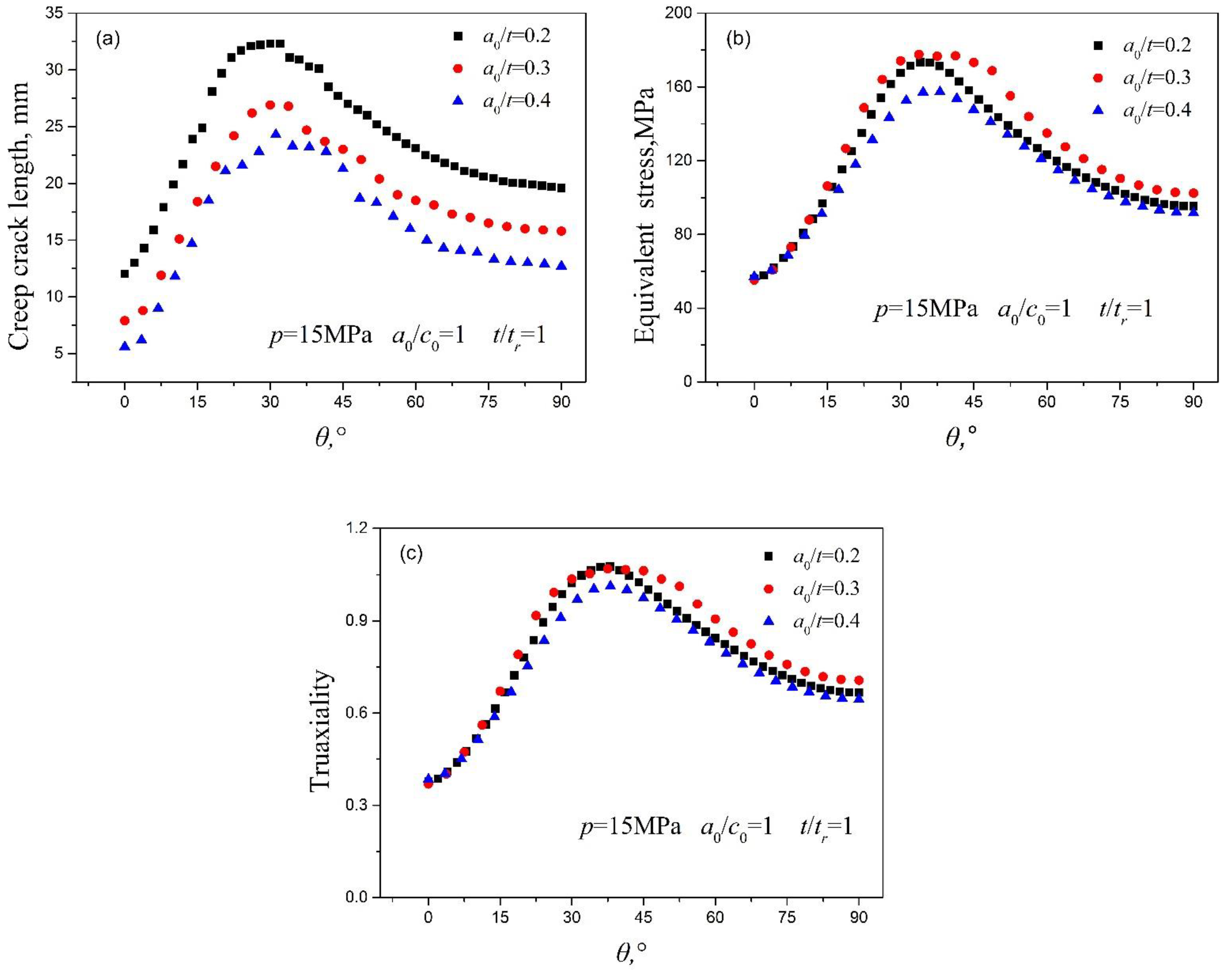
4.4.2. Effect of the Initial Crack Depth on the Creep Crack Growth Behavior
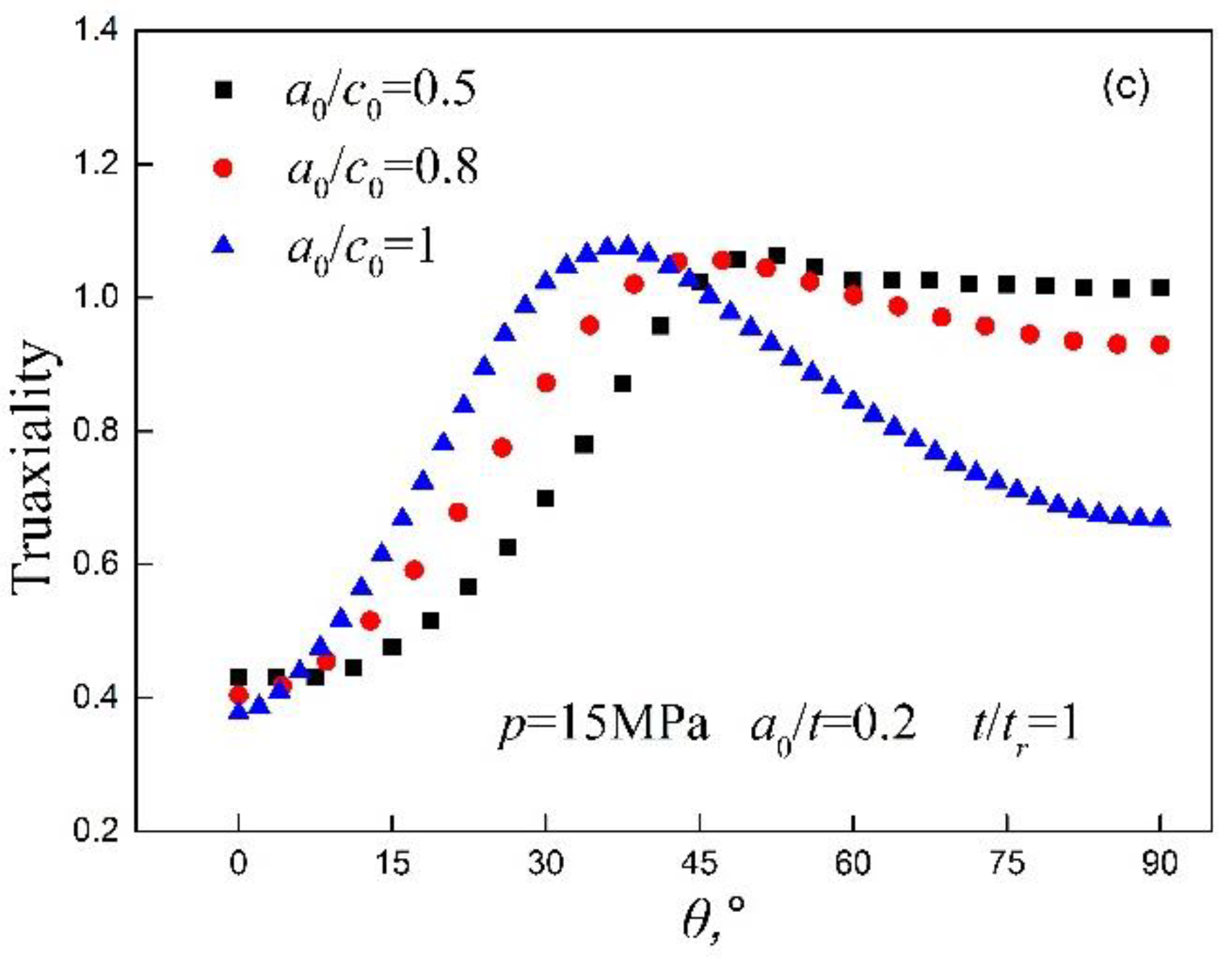
4.4.3. Effect of the Initial Crack Shape on the Creep Crack Growth Behavior
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nikbin, K.; Liu, S. Multiscale-constraint based model to predict uniaxial/multiaxial creep damage and crack growth in 316-H steels. Int. J. Mech. Sci. 2019, 156, 74–85. [Google Scholar] [CrossRef]
- Razak, N.A.; Davies, C.M.; Nikbin, K.M. Testing and assessment of cracking in P91 steels under creep-fatigue loading conditions. Eng. Fail. Anal. 2018, 84, 320–330. [Google Scholar] [CrossRef]
- Meng, Q.; Wang, Z. Extended finite element method for power-law creep crack growth. Eng. Fract. Mech. 2014, 127, 148–160. [Google Scholar] [CrossRef]
- Holdsworth, S. Advances in the assessment of creep data during the past 100 years. In Proceedings of the 9th Liege Conference: Materials for Advanced Power Engineering, Liege, Belgium, 27–29 September 2010; pp. 946–947. [Google Scholar]
- Payten, W. A reassessment of the multiaxial ductility C* creep crack growth equation based on the strain energy integral of the HRR singular field terms. Eng. Fract. Mech. 2019, 217, 106530. [Google Scholar] [CrossRef]
- Quintero, H.; Mehmanparast, A. Prediction of creep crack initiation behaviour in 316H stainless steel using stress dependent creep ductility. Int. J. Solids Struct. 2016, 97–98, 101–115. [Google Scholar] [CrossRef]
- Zhao, Y.; Gong, J.; Yong, J. Creep behaviour of P91/GTR-2CM/12Cr1MoV dissimilar joint predicted by the modified Theta method. Mater. High Temp. 2017, 34, 260–271. [Google Scholar] [CrossRef]
- Tan, J.P.; Wang, G.Z.; Xuan, F.Z.; Tu, S.T. Experimental investigation of in-plane constraint and out-of-plane constraint effect on creep crack growth. In Proceedings of the the ASME 2012 Pressure Vessels & Piping Division Conference, Toronto, ON, Canada, 15–19 July 2012. [Google Scholar]
- Zhang, J.W.; Wang, G.Z.; Xuan, F.Z.; Tu, S.T. The influence of stress-regime dependent creep model and ductility in the prediction of creep crack growth rate in Cr–Mo–V steel. Mater. Des. 2015, 65, 644–651. [Google Scholar] [CrossRef]
- Zhang, J.S. High Temperature Deformation and Fracture of Materials; Science Press: Beijing, China, 2007. [Google Scholar]
- Rieth, M.; Falkenstein, A.; Graf, P.; Heger, S.; Jäntsch, U.; Klimiankou, M.; Materna-Morris, E.; Zimmermann, H. Creep of the Austenitic Steel AISI 316L (N). Experiments and Models; Forhungcentrum Karlsruhe GmbH: Karlsruhe, Germany, 2004. [Google Scholar]
- Zhang, J.W. Numerical Prediction of Creep Crack Growth Rate and its Constraint Effect for a Wide Range of C*; East China University of Science and Technology: Shanghai, China, 2015. [Google Scholar]
- Zhang, J.W.; Wang, G.Z.; Xuan, F.Z.; Tu, S.T. Effect of stress dependent creep ductility on creep crack growth behaviour of steels for wide range of C*. Mater. High Temp. 2014, 32, 369–376. [Google Scholar] [CrossRef]
- Zhang, J.W.; Wang, G.Z.; Xuan, F.Z.; Tu, S.T. Prediction of creep crack growth behavior in Cr–Mo–V steel specimens with different constraints for a wide range of C*. Eng. Fract. Mech. 2014, 132, 70–84. [Google Scholar] [CrossRef]
- Oh, C.-S.; Kim, N.-H.; Kim, Y.-J.; Davies, C.; Nikbin, K.; Dean, D. Creep failure simulations of 316H at 550 °C: Part I—A method and validation. Eng. Fract. Mech. 2011, 78, 2966–2977. [Google Scholar] [CrossRef]
- He, J.Z.; Wang, G.Z.; Tu, S.T.; Xuan, F.Z. Characterization of 3-D creep constraint and creep crack growth rate in test specimens in ASTM-E1457 standard. Eng. Fract. Mech. 2016, 168, 131–146. [Google Scholar] [CrossRef]
- Wu, D.; Jing, H.; Xu, L.; Zhao, L.; Han, Y. Enhanced models of creep crack initiation prediction coupled the stress-regime creep properties and constraint effect. Eur. J. Mech. A/Solids 2019, 74, 145–159. [Google Scholar] [CrossRef]
- Gorash, Y.; Altenbach, H.; Lvov, G. Modelling of high-temperature inelastic behaviour of the austenitic steel AISI type 316 using a continuum damage mechanics approach. J. Strain Anal. Eng. Des. 2012, 47, 229–243. [Google Scholar] [CrossRef]
- Esposito, L.; Bonora, N.; de Vita, G. Creep modelling of 316H stainless steel over a wide range of stress. Procedia Struct. Integr. 2016, 2, 927–933. [Google Scholar] [CrossRef] [Green Version]
- Bonora, N.; Esposito, L. Mechanism Based Creep Model Incorporating Damage. J. Eng. Mater. Technol. 2010, 132, 021013. [Google Scholar] [CrossRef]
- Xue, J.-L.; Zhou, C.-Y.; Peng, J. Ultimate creep load and safety assessment of P91 steel pipe with local wall thinning at high temperature. Int. J. Mech. Sci. 2015, 93, 136–153. [Google Scholar] [CrossRef]
- Kimura, K.; Kushima, H.; Sawada, K. Long-term creep deformation property of modified 9Cr–1Mo steel. Mater. Sci. Eng. A 2009, 510–511, 58–63. [Google Scholar] [CrossRef]
- Liu, L.X. Research on Creep Property of 25Cr2NiMo1V Steel and FE Simulation on Constraint Effects; East China University of Science and Technology: Shanghai, China, 2009. [Google Scholar]
- Tan, J.P.; Tu, S.T.; Wang, G.Z.; Xuan, F.Z. Effect and mechanism of out-of-plane constraint on creep crack growth behavior of a Cr–Mo–V steel. Eng. Fract. Mech. 2013, 99, 324–334. [Google Scholar] [CrossRef]
- Robinson, E.L. Effect of temperature variation on the creep strength of steels. Trans. ASME 1938, 60, 253–259. [Google Scholar]
- Wen, J.-F.; Tu, S.-T.; Xuan, F.-Z.; Zhang, X.-W.; Gao, X.-L. Effects of Stress Level and Stress State on Creep Ductility: Evaluation of Different Models. J. Mater. Sci. Technol. 2016, 32, 695–704. [Google Scholar] [CrossRef]
- ASTM E 1457; Standard Test Method for Measurement of Creep Crack Growth Rates in Metals. Annual Book of ASTM Standards: West Conshohocken, PA, USA, 2001; Volume 3, pp. 945–966.
- Wen, J.-F.; Tu, S.-T. A multiaxial creep-damage model for creep crack growth considering cavity growth and microcrack interaction. Eng. Fract. Mech. 2014, 123, 197–210. [Google Scholar] [CrossRef]
- McClintock, F.A. A criterion for ductile fracture by the growth of holes. J. Appl. Mech. 1968, 35, 363–371. [Google Scholar] [CrossRef]
- Rice, J.R.; Tracey, D.M. On the ductile enlargement of voids in triaxial stress fields. J. Mech. Phys. Solids 1969, 17, 201–217. [Google Scholar] [CrossRef] [Green Version]
- Cocks, F.A.C.; Ashby, F.M. Intergranular fracture during power-law creep under multiaxial stresses. Met. Sci. 1980, 9, 395–402. [Google Scholar] [CrossRef]
- Spindler, M.W. The multiaxial and uniaxial creep ductility of Type 304 steel as a function of stress and strain rate. Mater. High Temp. 2004, 21, 47–54. [Google Scholar] [CrossRef]
- Zhou, Y.; Xuedong, C.; Fan, Z.; Yichun, H. An improved mechanism-based creep constitutive model using stress-dependent creep ductility. In Proceedings of the ASME 2016 Pressure Vessels and Piping Conference, American Society of Mechanical Engineers Digital Collection, Vancouver, BC, Canada, 17–21 July 2016. [Google Scholar]
- Gorash, Y. Development of a Creep-Damage Model for Non-Isothermal Long-Term Strength Analysis of High-Temperature Components Operating in a Wide Stress Range. Ph.D. Thesis, Martin-Luther-Universitat Halle-Wittenberg, Kharkiv, Ukraine, 2008. [Google Scholar]
- Kloc, L.; Sklenička, V. Transition from power-law to viscous creep behaviour of p-91 type heat-resistant steel. Mater. Sci. Eng. A 1997, 234–236, 962–965. [Google Scholar] [CrossRef]
- Kloc, L.; Sklenička, V. Confirmation of low stress creep regime in 9% chromium steel by stress change creep experiments. Mater. Sci. Eng. A 2004, 387–389, 633–638. Mater. Sci. Eng. A 2004, 387–389, 633–638. [Google Scholar] [CrossRef]
- Sklenicka, V.; Kucharova, K.; Kudrman, J.; Svoboda, M.; Kloc, L. Microstructure stability and creep behaviour of advanced high chromium ferritic steels. Kov. Mater. Met. Mater. 2005, 43, 20–33. [Google Scholar]


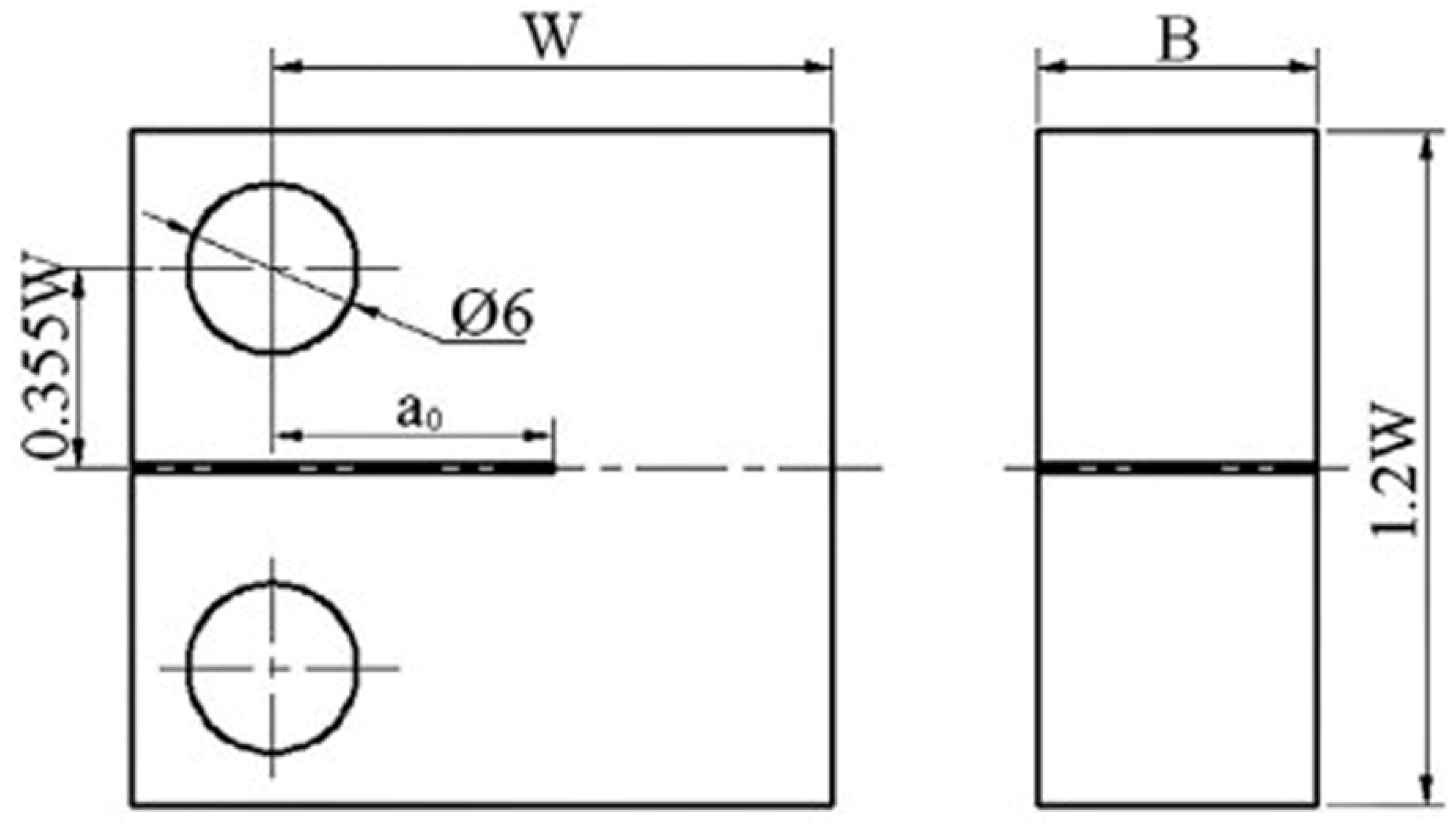









| C | Mn | Si | Cr | Ni | Mo | V | Nb | N | Al | Ti | Fe |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.108 | 0.457 | 0.221 | 9.09 | 0.217 | 0.991 | 0.206 | 0.078 | 0.047 | 0.012 | 0.005 | Bal |
| Model | Mathematical Formulation |
|---|---|
| Norton | |
| 2RN | |
| Sine-hyperbolic model | |
| Equation (12) constitutive model |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Li, J.; Zan, J.; Guo, Z.; Liu, K. A Creep Constitutive Model, Based on Deformation Mechanisms and Its Application to Creep Crack Growth. Metals 2022, 12, 2179. https://doi.org/10.3390/met12122179
Zhang J, Li J, Zan J, Guo Z, Liu K. A Creep Constitutive Model, Based on Deformation Mechanisms and Its Application to Creep Crack Growth. Metals. 2022; 12(12):2179. https://doi.org/10.3390/met12122179
Chicago/Turabian StyleZhang, Jingwei, Jie Li, Jingyi Zan, Zijian Guo, and Kanglin Liu. 2022. "A Creep Constitutive Model, Based on Deformation Mechanisms and Its Application to Creep Crack Growth" Metals 12, no. 12: 2179. https://doi.org/10.3390/met12122179
APA StyleZhang, J., Li, J., Zan, J., Guo, Z., & Liu, K. (2022). A Creep Constitutive Model, Based on Deformation Mechanisms and Its Application to Creep Crack Growth. Metals, 12(12), 2179. https://doi.org/10.3390/met12122179







