Relationship between Supplied Oil Flow Rates and Oil Film Thicknesses under Starved Elastohydrodynamic Lubrication
Abstract
:1. Introduction
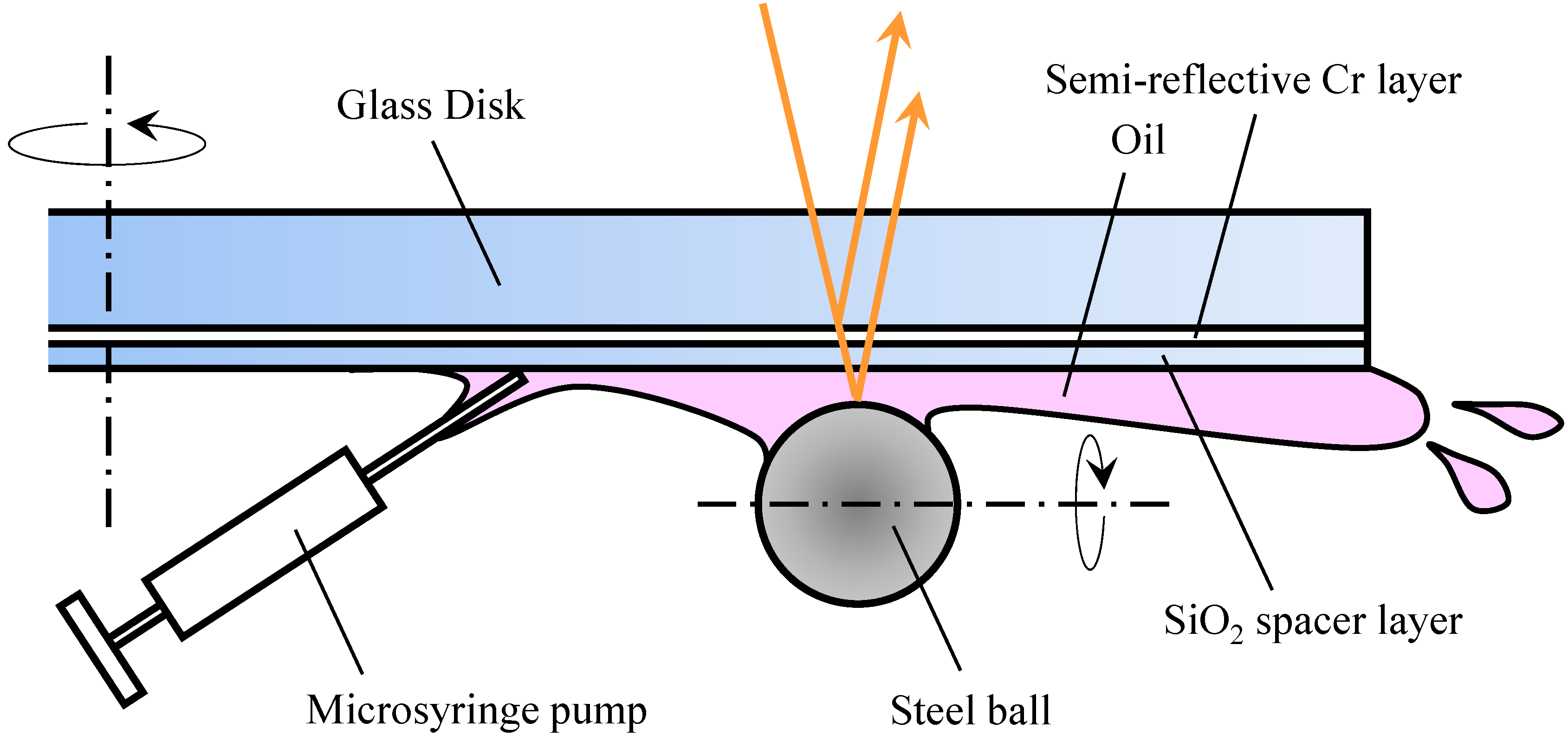
2. Experimental Methods
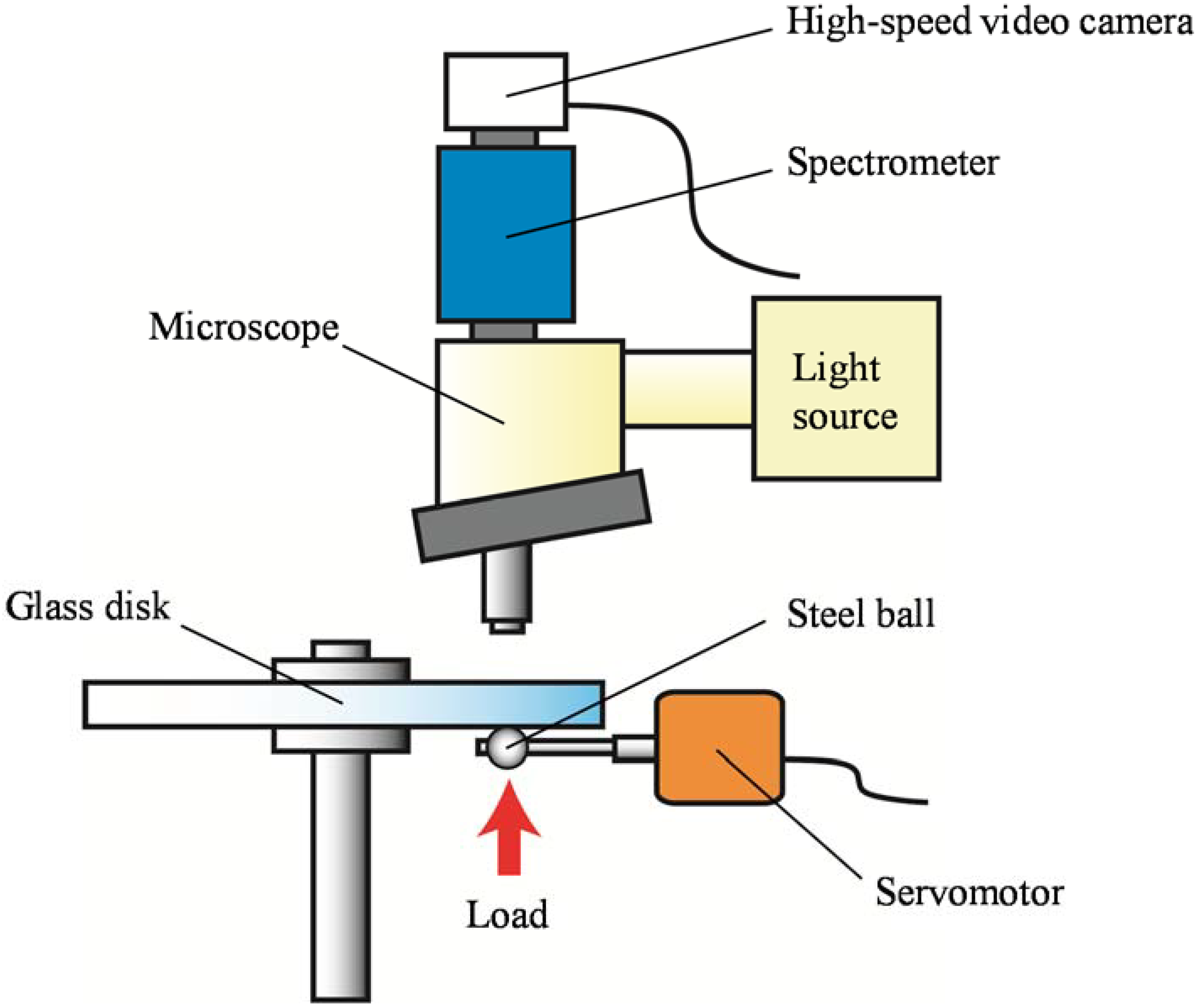
| Image Pickup Device | CMOS |
|---|---|
| Number of pixels | 512 × 512 pixels |
| Frame rate | 500 frames/s |
| Ball Specimen | Disk Specimen | |
|---|---|---|
| Diameter | 25.4 mm | 100 mm |
| Material | SUJ2 | BK7 (Glass) |
| Young’s modulus | 210 GPa | 73.1 GPa |
| Poisson’s ratio | 0.3 | 0.23 |
| Surface roughness | 8.1 nmRa | 2.3 nmRa |
| Temperature | 25 °C |
|---|---|
| Oil | Poly-alpha-olefin oil (PAO) |
| Kinematic viscosity | 19 mm2/s at 40 °C |
| Load | 29.7 N |
| Maximum contact pressure | 0.49 GPa |
| Radius of Hertzian contact | 170 μm |
| Contact radius | 40 mm |
| Rolling speed | 0.6 m/s to 1.8 m/s |
| Slide-to-roll ratio | 0% |
| Supplied oil flow rate | 0 mm3/s to 1.67 × 10−1 mm3/s |

3. Experimental Results
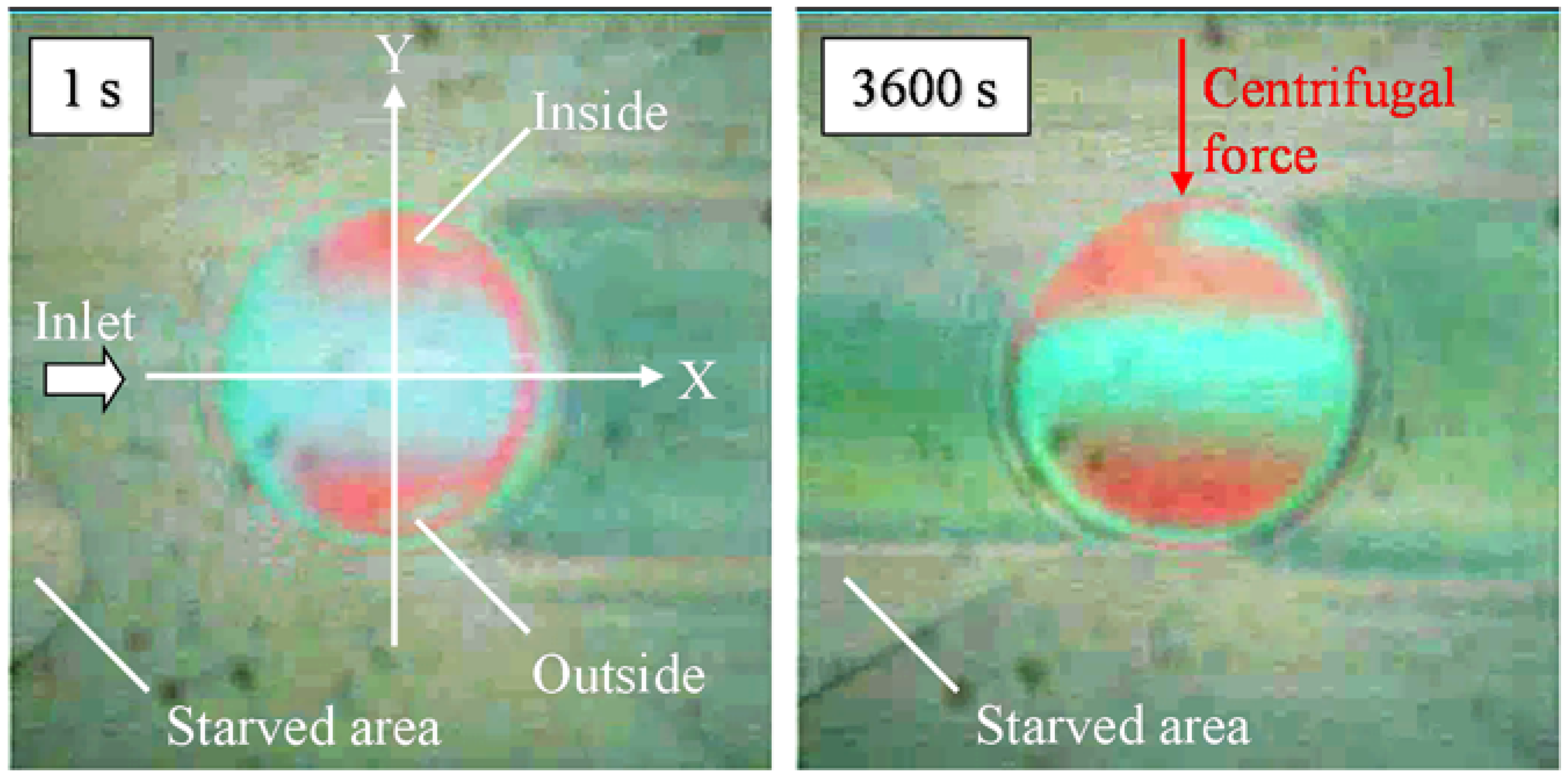
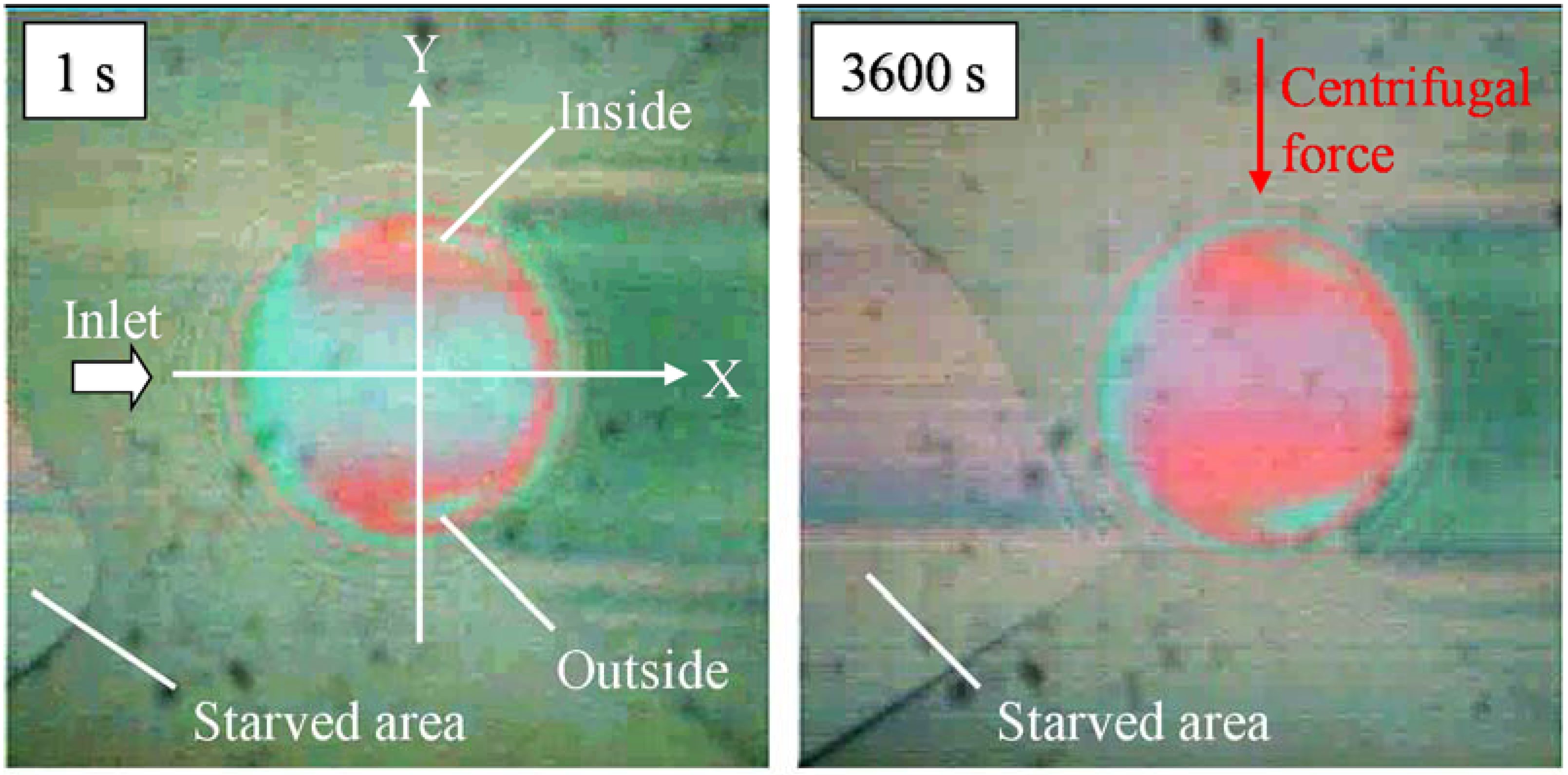
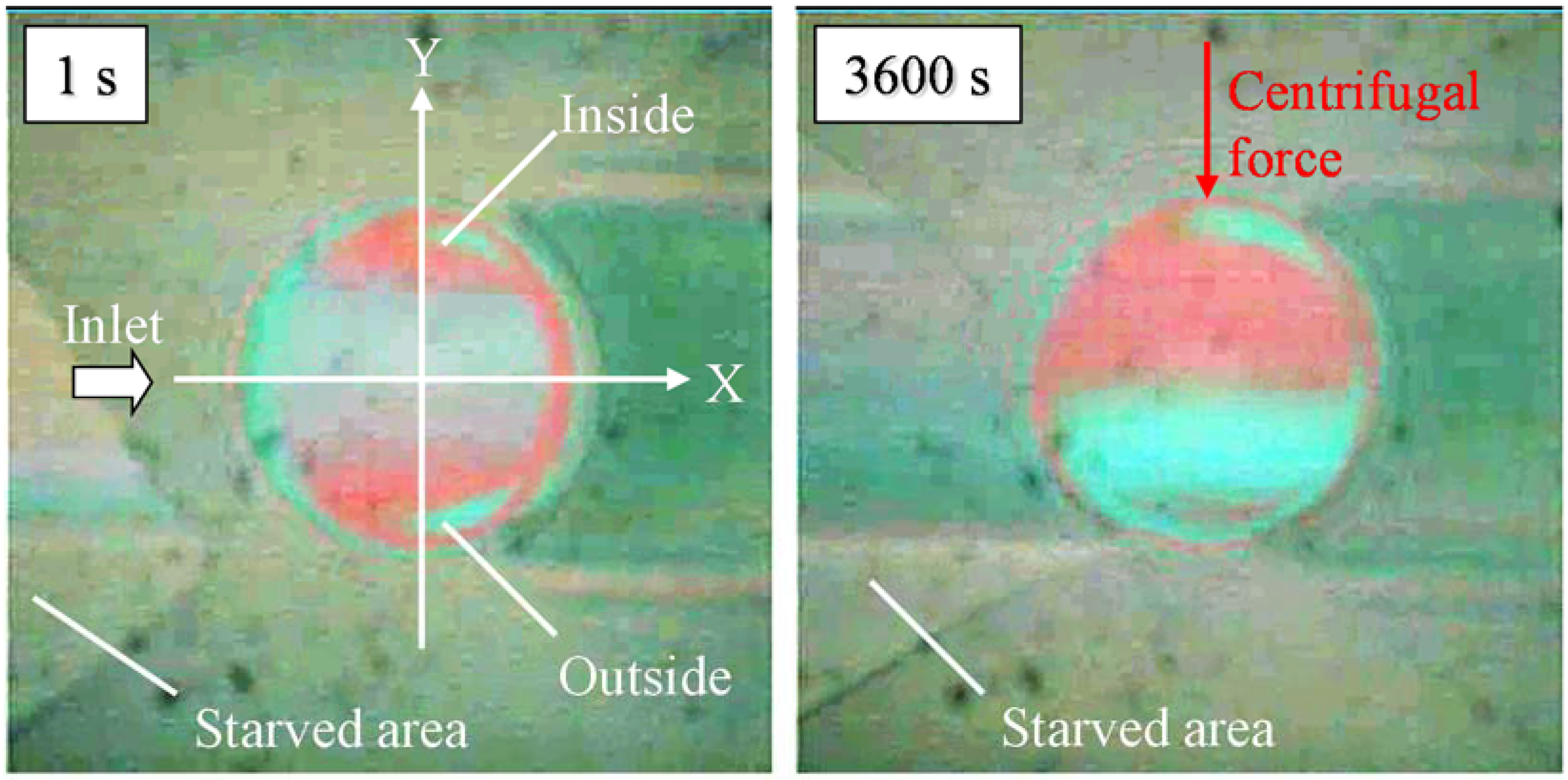
3.1. Preparatory Experiments
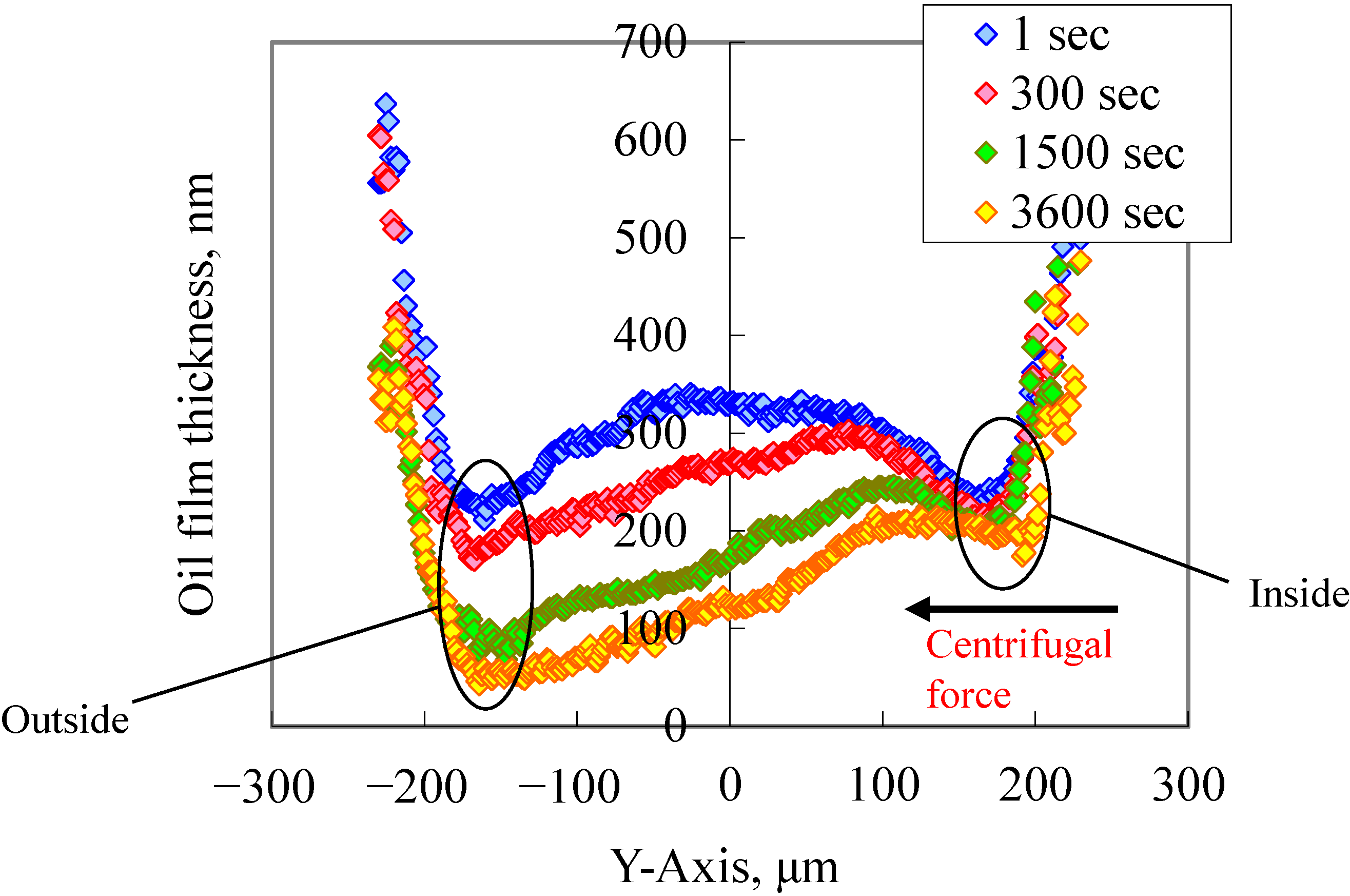
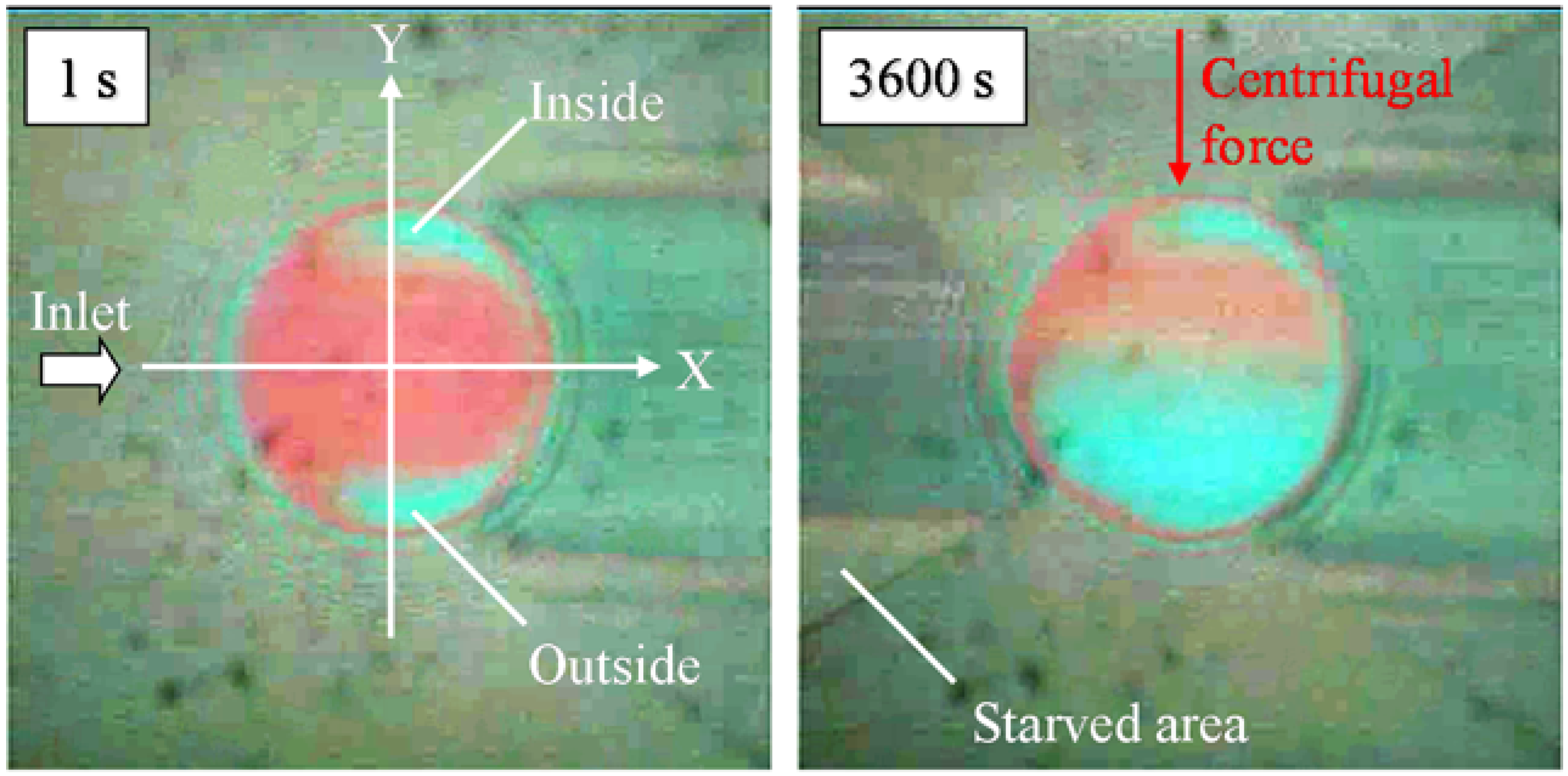

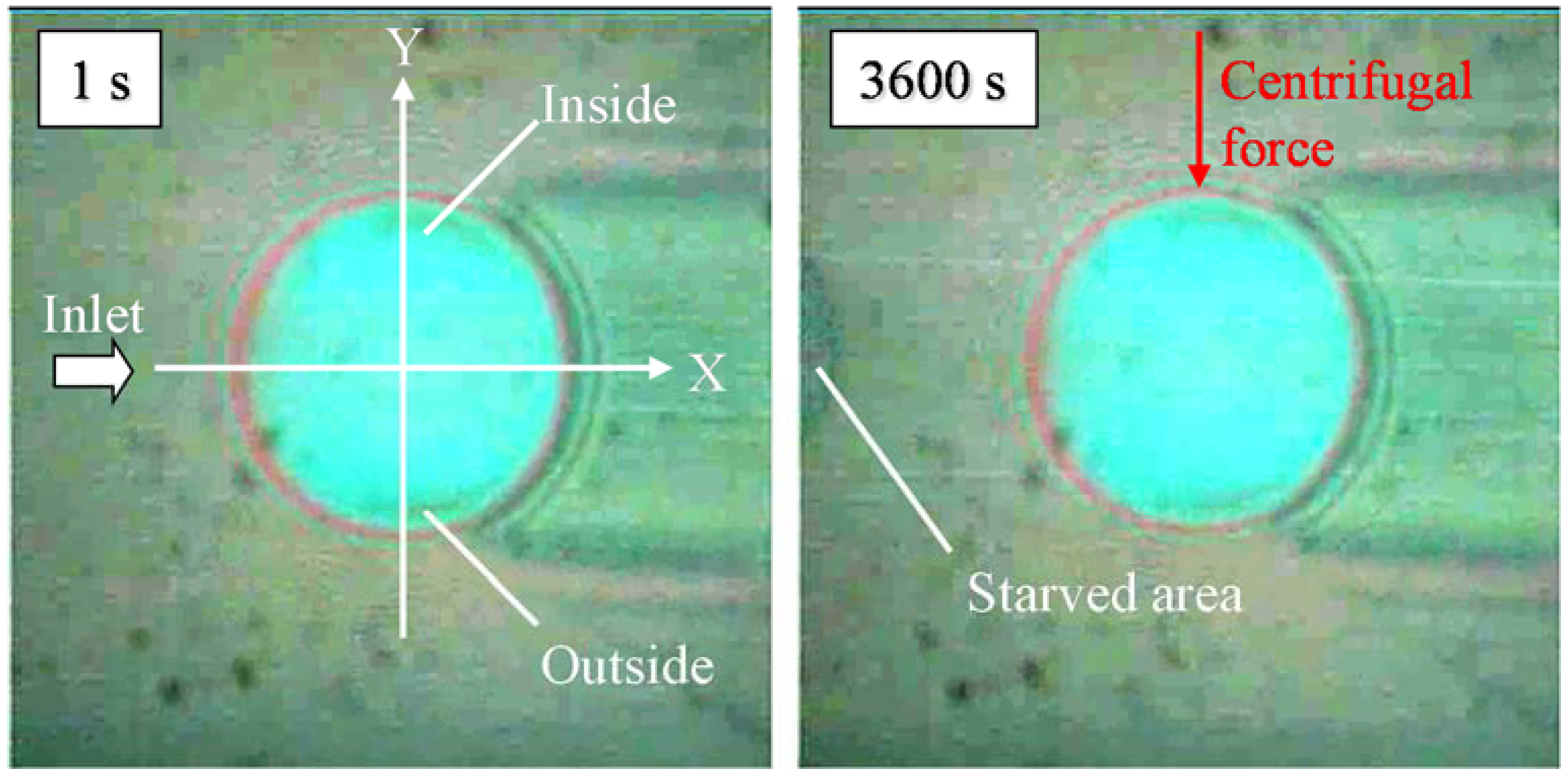
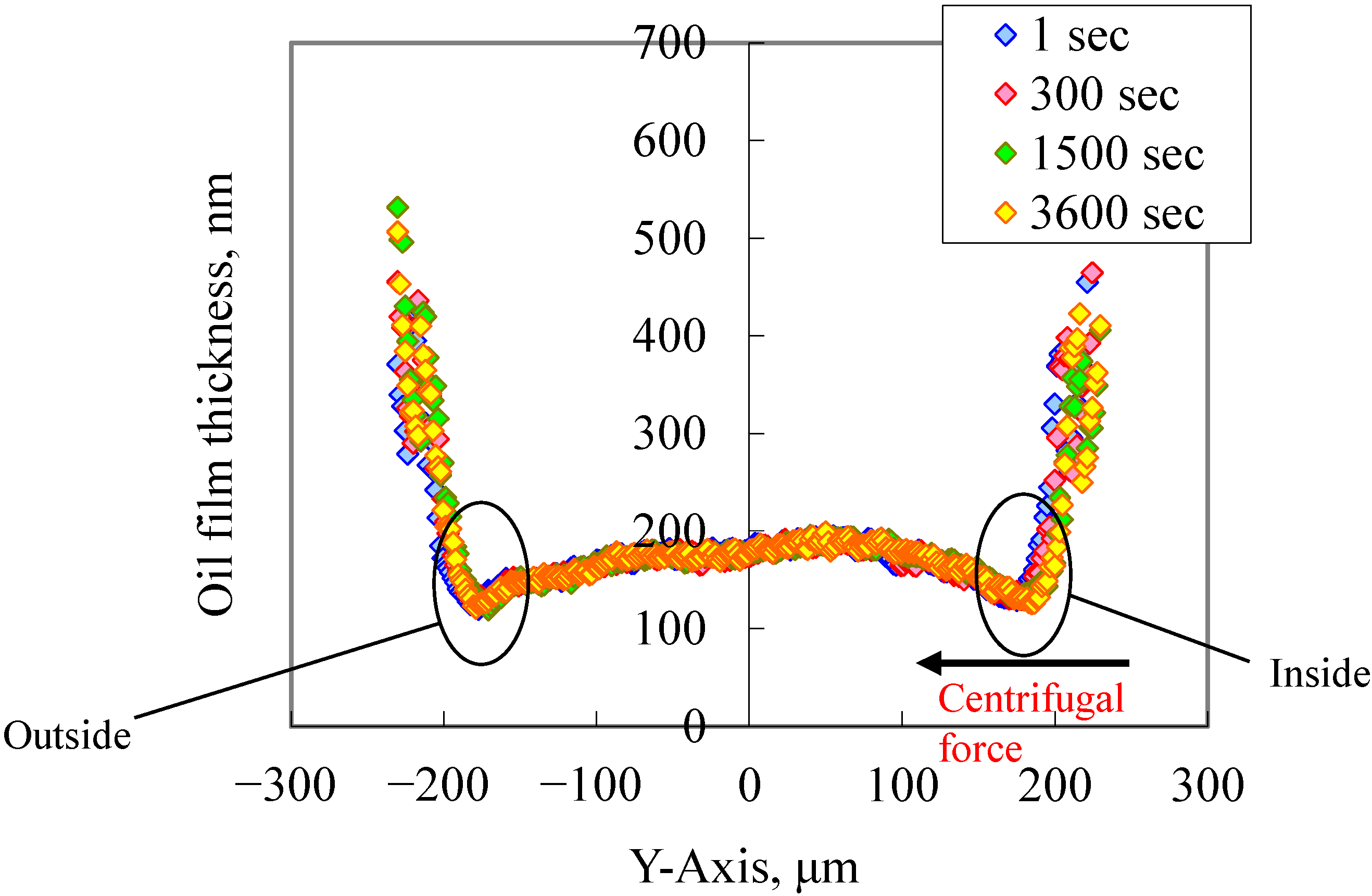
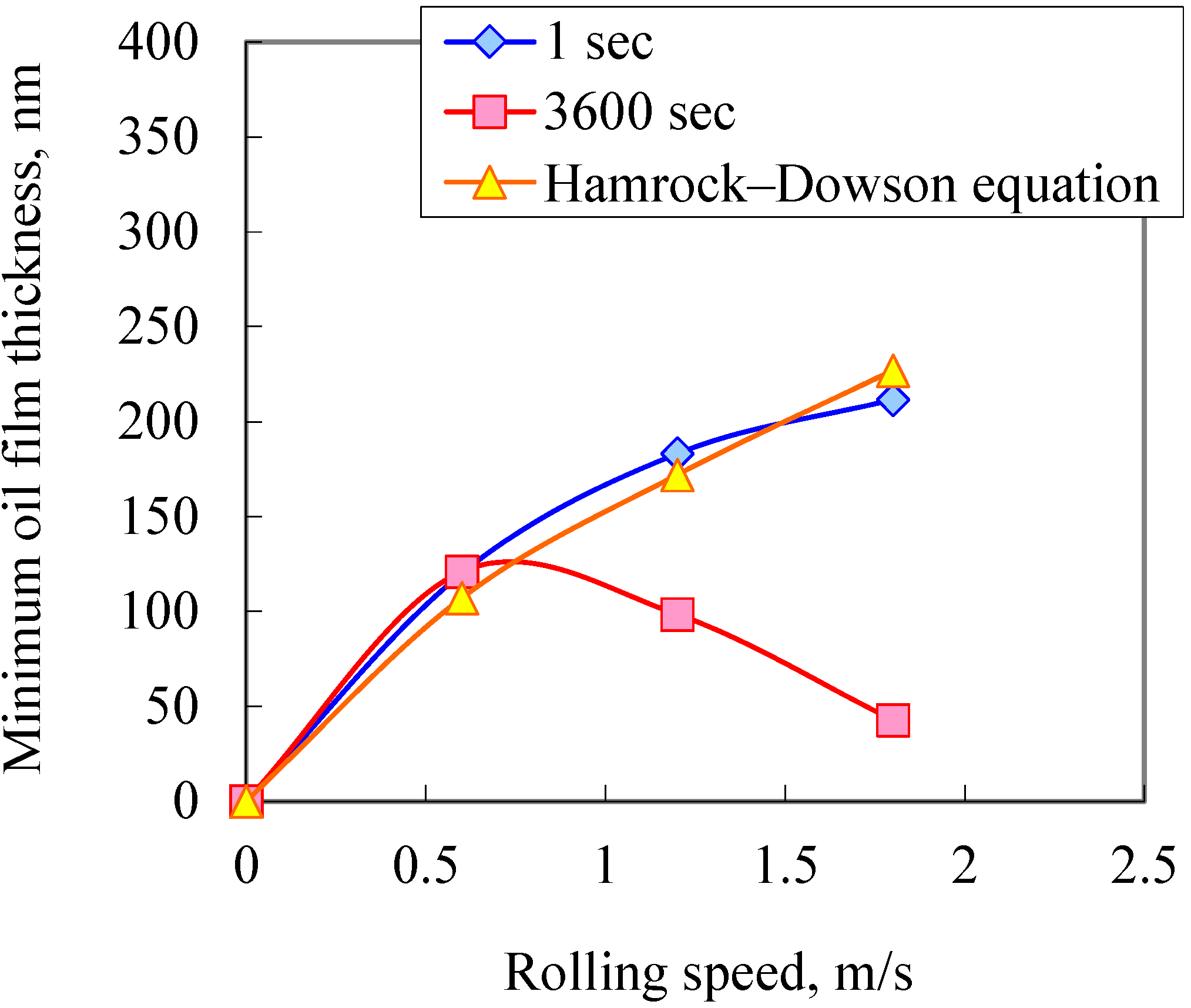

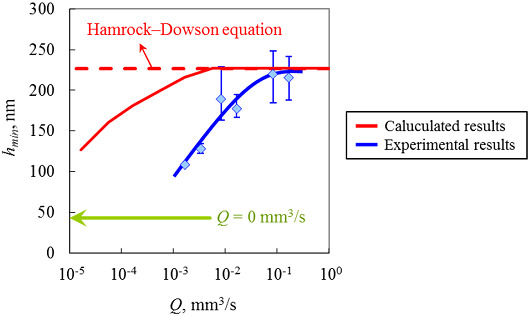
3.2. Relationship between Supplied Oil Flow Rates and Oil Film Thicknesses


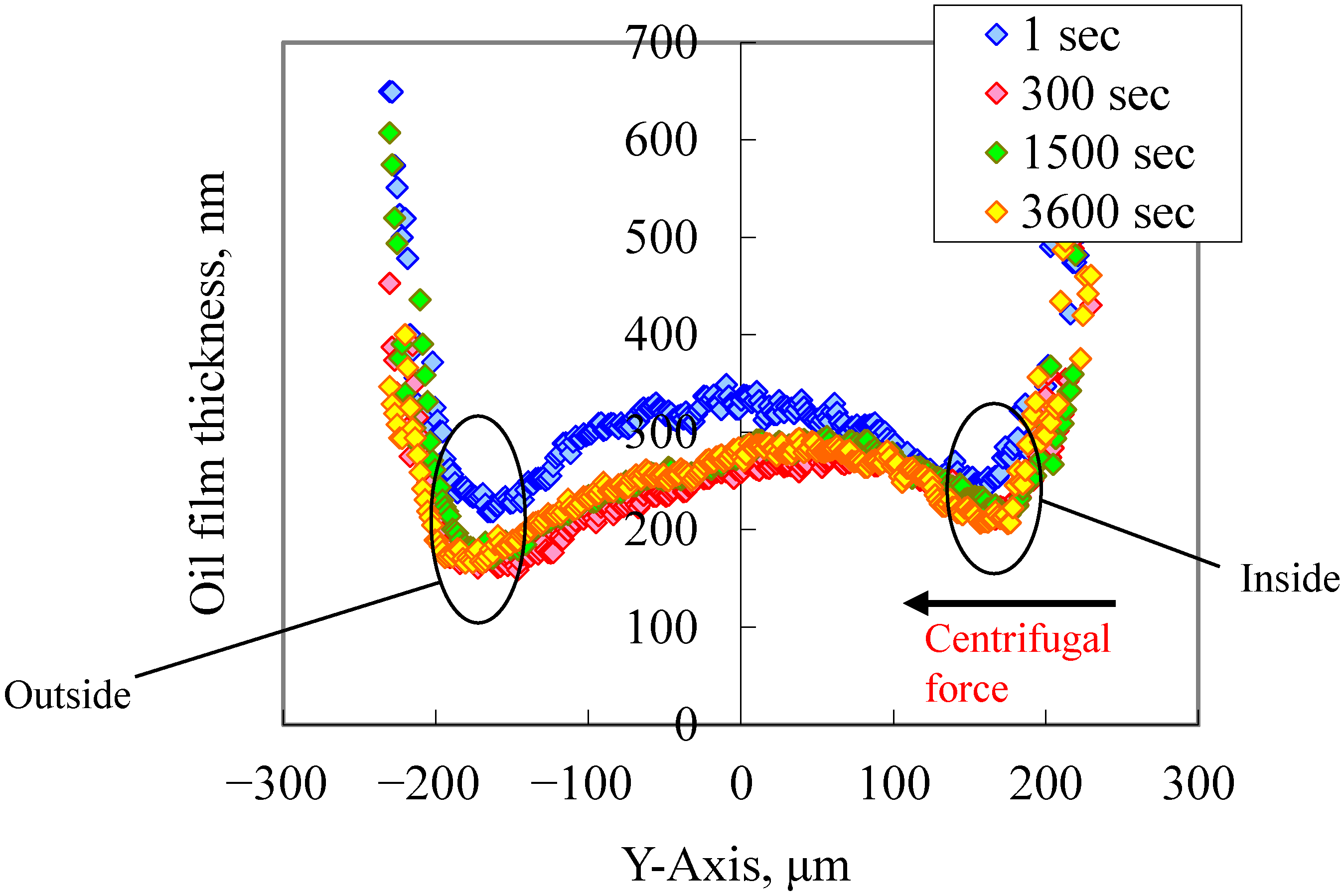
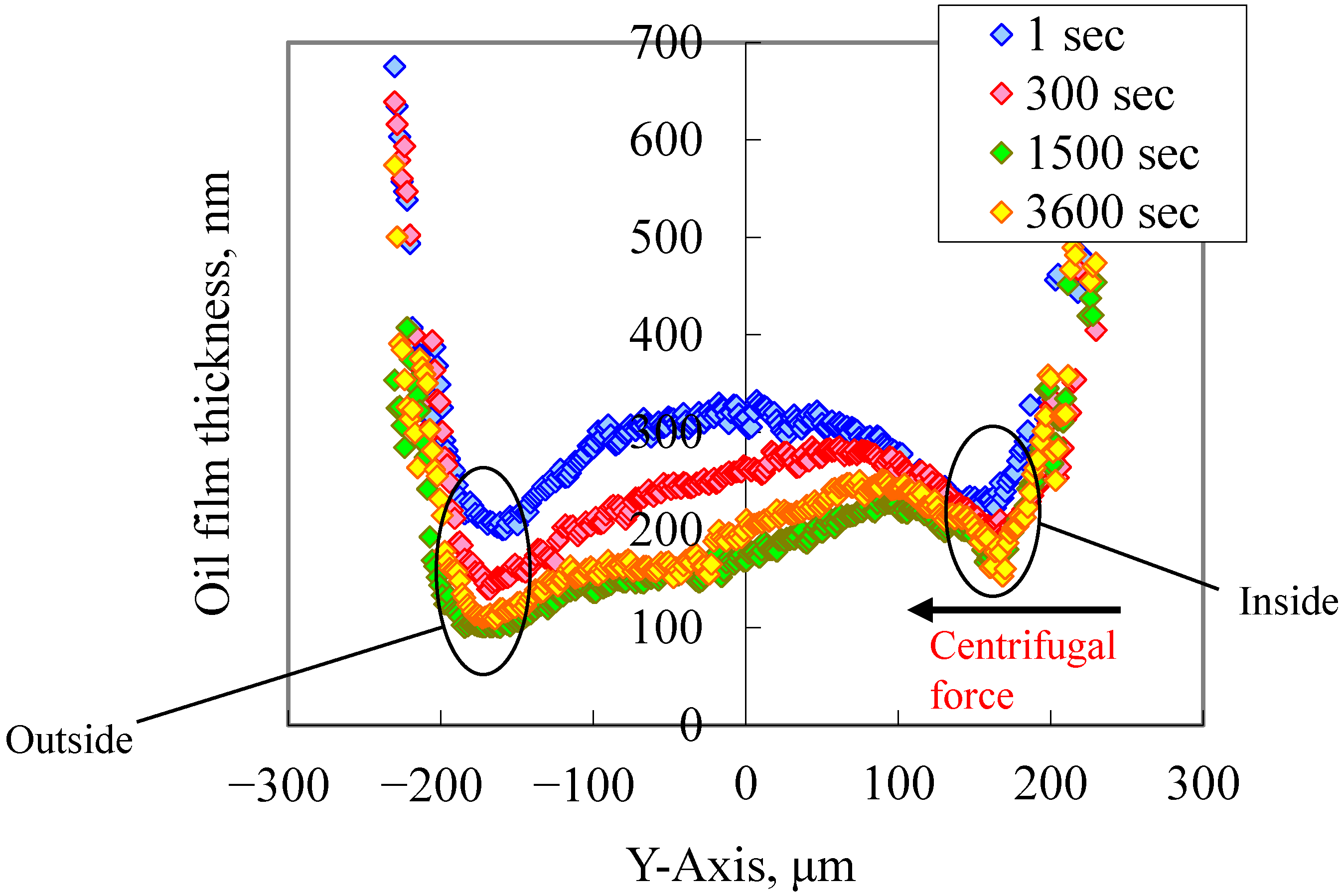
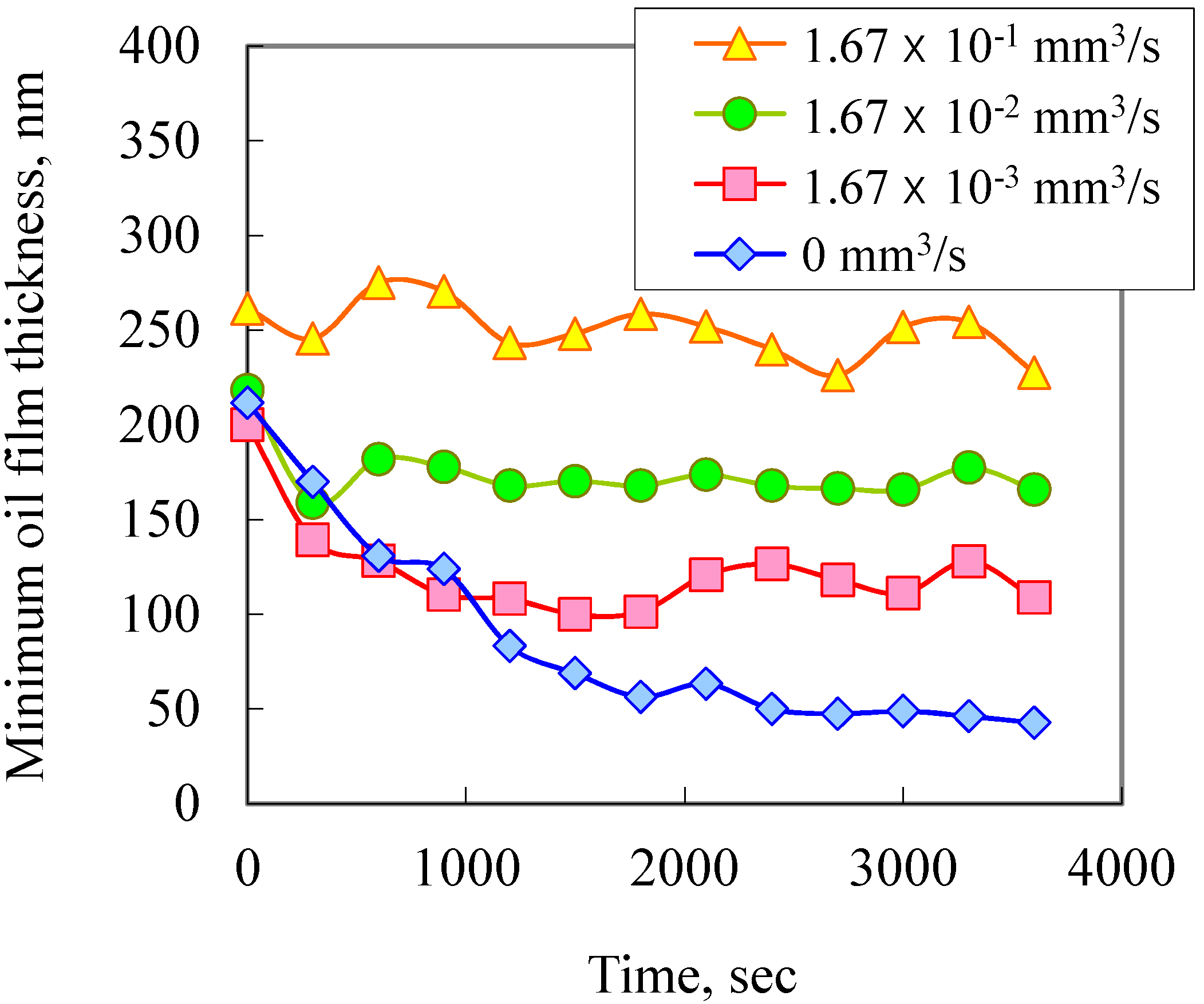
4. Discussion
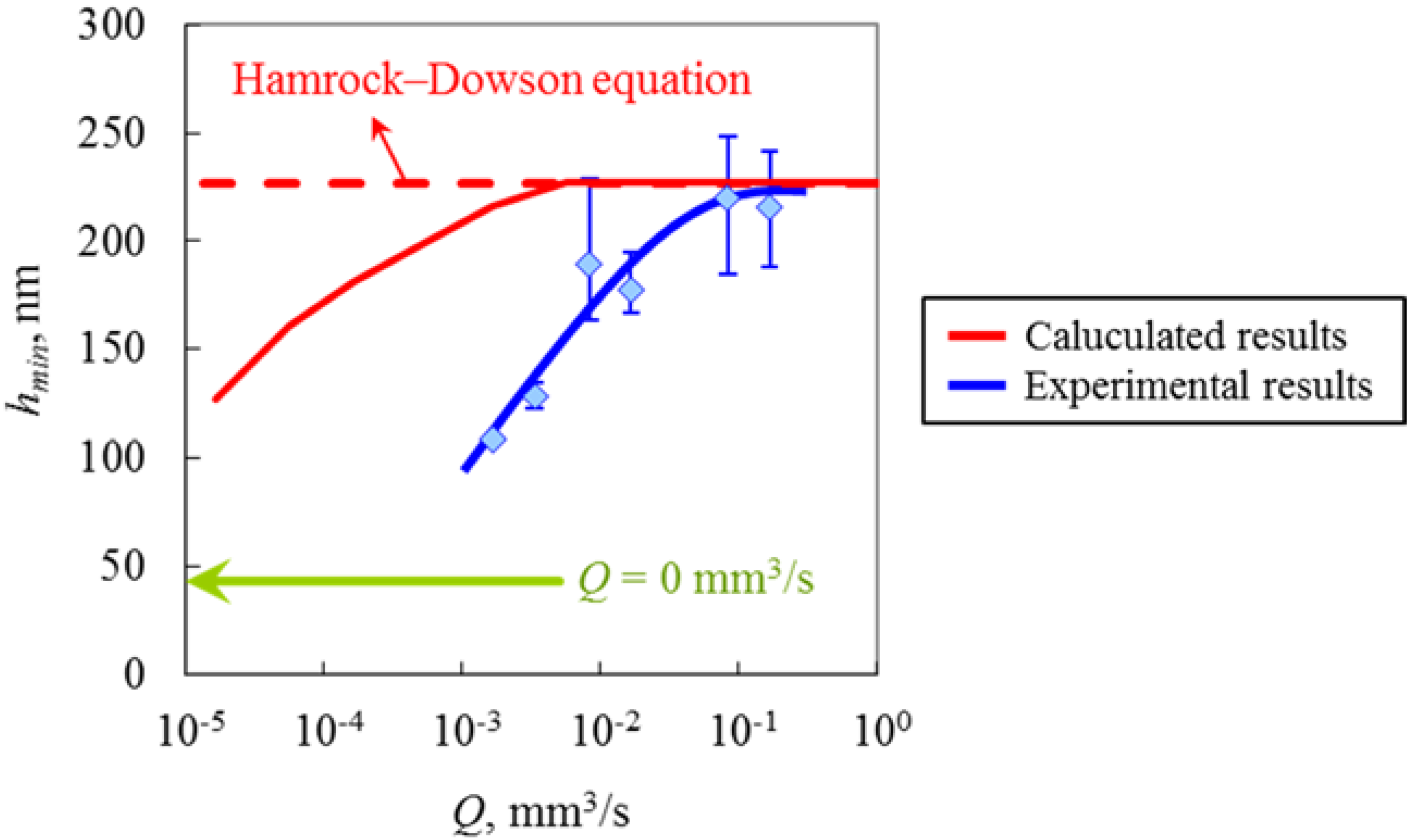
5. Conclusions
- An oil film measurement method using a microsyringe pump was developed to reproduce starved lubrication by means of an EHL testing machine.
- Based on the Hamrock-Dowson equation, with fully flooded lubrication, the oil film thickness increases with the rolling speed; with starved lubrication, the oil film thickness decreases because the lubricating oil is affected by centrifugal forces.
- The oil film thickness on the “outside” of an EHL contact area becomes lower than that on the “inside”. This is also caused by the fact that the lubricating oil is affected by centrifugal forces.
- The relationship between the supplied oil flow rate and oil film thickness was determined. In this relationship, if the supplied oil flow rate is set to approximately 10−2 mm3/s or less, the minimum oil film thickness decreases with the decrease of the supplied oil flow rate.
- The minimum oil film thickness hmin,S under starved lubrication was calculated by the supplied oil flow rate Q. However, the calculated results didn’t agree with the experimental results, which meant that hb was much thinner than h∞ because the racetracks were generated behind EHL contact areas.
Author Contributions
Conflicts of Interest
References
- Johns, P.M.; Gohar, R. Roller bearings under radial and eccentric loads. Tribol. Int. 1981, 14, 131–136. [Google Scholar] [CrossRef]
- Wedeven, L.D.; Evans, D.; Cameron, A. Optical analysis of ball bearing starvation. ASME Trans. J. Lubricat. Technol. 1971, 93, 349–363. [Google Scholar] [CrossRef]
- Lui, J.; Wen, S. Fully flooded starved and parched lubrication at a point contact system. Wear 1992, 159, 135–140. [Google Scholar] [CrossRef]
- Parinam, A.; Karan, R. Analysis of starved EHL line contacts for lubricants with linear pressure viscosity dependence. Int. J. Adv. Technol. 2014, 5, 11–27. [Google Scholar]
- Wang, W.Z.; Li, S.; Shen, D.; Zhang, S.; Hu, Y.Z. A mixed lubrication model with consideration of starvation and interasperity cavitations. Proc. Inst. Mech. Eng. J J. Eng. Tribol. 2012, 226, 1023–1038. [Google Scholar] [CrossRef]
- Mohammadpour, M.; Johns-Rahnejat, P.M.; Rahnejat, H.; Gohar, R. Boundary conditions for elastohydrodynamics of circular point contacts. Tribol. Lett. 2014, 53, 107–118. [Google Scholar] [CrossRef]
- Wolveridge, P.E.; Baglin, K.P.; Archard, J.F. The starved lubrication of cylinders in line contact. Proc. Inst. Mech. Engrs. 1970, 185, 1159–1169. [Google Scholar]
- Yang, P.; Wang, J.; Kaneta, M. Thermal and non-Newtonian numerical analyses for starved EHL line contacts. J. Tribol. 2006, 128, 282–290. [Google Scholar] [CrossRef]
- Mihailidis, A.; Agouridas, K.; Panagiotidis, K. Non-Newtonian starved thermal-elastohydrodynamic lubrication of finite line contacts. Tribol. Trans. 2013, 56, 88–100. [Google Scholar] [CrossRef]
- Chevalier, F.; Lubrecht, A.A.; Cann, P.M.E.; Colin, F.; Dalmaz, G. Film thickness in starved EHL point contacts. ASME J. Tribol. 1998, 120, 126–133. [Google Scholar] [CrossRef]
- Guangteng, G.; Cann, P.M.; Spikes, H.A. A study of parched lubrication. Wear 1992, 153, 91–105. [Google Scholar] [CrossRef]
- Cann, P.M. The transition between fully flooded and starved regimes in EHL. Tribol. Int. 2004, 37, 859–864. [Google Scholar] [CrossRef]
- Cann, P.M.E.; Chevalier, F.; Lubrecht, A.A. Track depletion and Replenishment in a grease lubricated point contact: A quantitative analysis. Tribol. Series 1997, 32, 405–413. [Google Scholar]
- Cann, P.M. Starved grease lubrication of rolling contacts. Tribol. Trans. 1999, 42, 867–873. [Google Scholar] [CrossRef]
- Svoboda, P.; Košťál, D.; Popelka, M.; Křupka, I. The experimental study of transition between fully flooded and starved regime in EHL contact. Eng. Mech. 2013, 20, 13–25. [Google Scholar]
- Damiens, B.; Venner, C.H.; Cann, P.M.E.; Lubrecht, A.A. Starved lubrication of elliptical EHD contacts. ASME J. Tribol. 2004, 126, 105–111. [Google Scholar] [CrossRef]
- Yin, C.; Yang, P.; Tan, H.; Wang, J. Thermal elastohydrodynamic lubrication of starved elliptical contacts. Tribol. Int. 2009, 42, 964–974. [Google Scholar] [CrossRef]
- Gershuni, L.; Larson, M.G.; Lugt, P.M. Lubricant replenishment in rolling bearing contacts. Tribol. Trans. 2008, 51, 643–651. [Google Scholar] [CrossRef]
- Wang, J.; Hashimoto, T.; Nishikawa, H.; Kaneta, M. Pure rolling elastohydrodynamic lubrication of short stroke reciprocating motion. Tribol. Int. 2005, 38, 1013–1021. [Google Scholar] [CrossRef]
- Chiu, Y.P. An analysis and prediction of lubricant film starvation in rolling contact systems. Tribol. Trans. 1974, 17, 22–35. [Google Scholar]
- Johnston, G.J.; Wayte, R.; Spikes, H.A. The measurement and study of very thin lubricant films in concentrated contacts. Tribol. Trans. 1991, 34, 187–194. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Isothermal elastohydrodynamic lubrication of point contacts: Part III—fully flooded results. ASME Trans. J. Lubricat. Technol. 1977, 99, 264–275. [Google Scholar] [CrossRef]
- Rauscher, J.W.; Kelly, R.E.; Cole, J.D. An asymptotic solution for the laminar flow of a thin film on a rotating disk. Trans. ASME J. Appl. Mech. 1973, 40, 43–47. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Isothermal elastohydrodynamic lubrication of point contacts: Part IV—starvation results. ASME Trans. J. Lubricat. Technol. 1977, 99, 15–23. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maruyama, T.; Saitoh, T. Relationship between Supplied Oil Flow Rates and Oil Film Thicknesses under Starved Elastohydrodynamic Lubrication. Lubricants 2015, 3, 365-380. https://doi.org/10.3390/lubricants3020365
Maruyama T, Saitoh T. Relationship between Supplied Oil Flow Rates and Oil Film Thicknesses under Starved Elastohydrodynamic Lubrication. Lubricants. 2015; 3(2):365-380. https://doi.org/10.3390/lubricants3020365
Chicago/Turabian StyleMaruyama, Taisuke, and Tsuyoshi Saitoh. 2015. "Relationship between Supplied Oil Flow Rates and Oil Film Thicknesses under Starved Elastohydrodynamic Lubrication" Lubricants 3, no. 2: 365-380. https://doi.org/10.3390/lubricants3020365
APA StyleMaruyama, T., & Saitoh, T. (2015). Relationship between Supplied Oil Flow Rates and Oil Film Thicknesses under Starved Elastohydrodynamic Lubrication. Lubricants, 3(2), 365-380. https://doi.org/10.3390/lubricants3020365