Electrical Impedance Spectroscopy for Precise Film Thickness Assessment in Line Contacts
Abstract
1. Introduction
1.1. State of the Art
1.2. Goal of the Paper
2. Materials and Methods
2.1. Equivalent Electrical Circuit of a Single EHL Contact
2.2. Electrical Impedance-Based Film Thickness Reconstruction: Maruyama’s Method
2.3. Capacitance-Based Film Thickness Reconstruction: Jablonka Method
2.4. Measurement of Electrical Impedance of Roller Bearings
2.5. Experimental Setup
3. Design of Experiments and Methodology
| Algorithm 1 Measurement protocol |
| do run-in procedure |
| do determine the resistance of oil film breakdown area at standstill ( in Equation (9)) |
| for all U, G, W: |
| if Mechanical and thermal equilibrium/steady-state do 1. Measure: |
| 2. Calculate oil film breakdown using (Equation (5)) |
| 3. Calculate central film thickness via Maruyama’s method (Equation (6)) |
| 4. Calculate central film thickness via Jablonka’s method (Equation (11)) |
4. Results
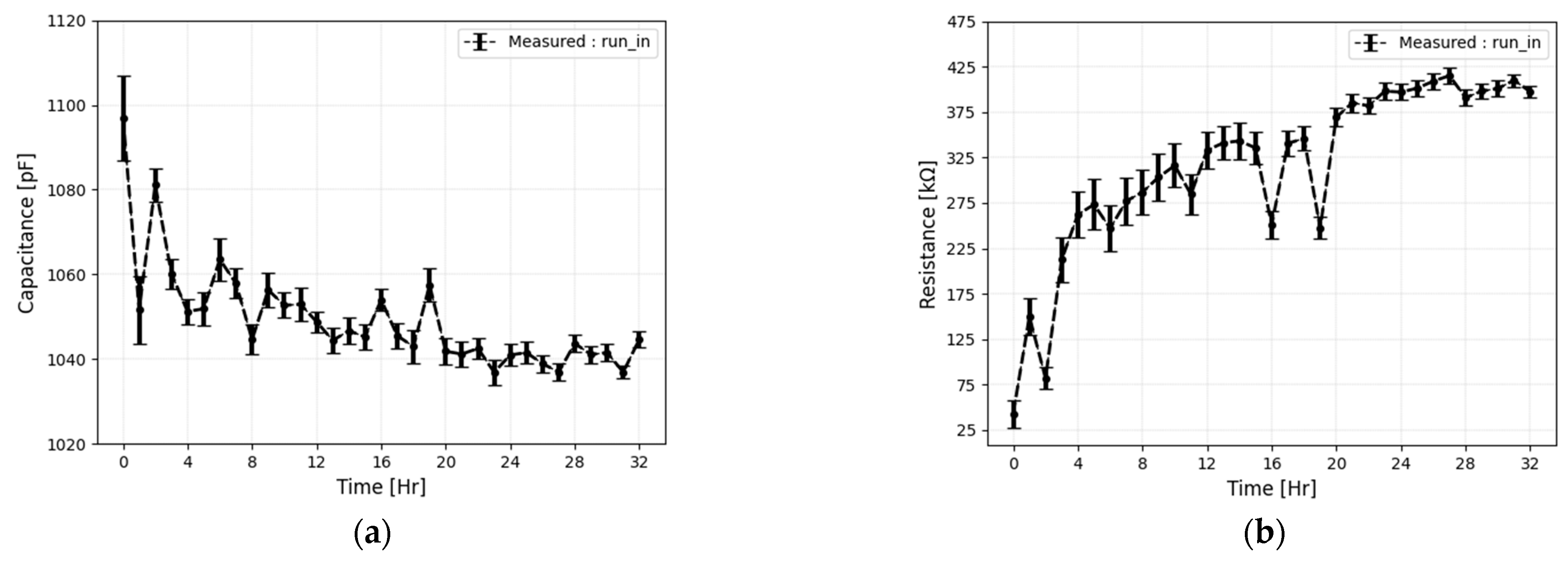
4.1. Run-in Procedure: Reaching Electrical Equilibrium
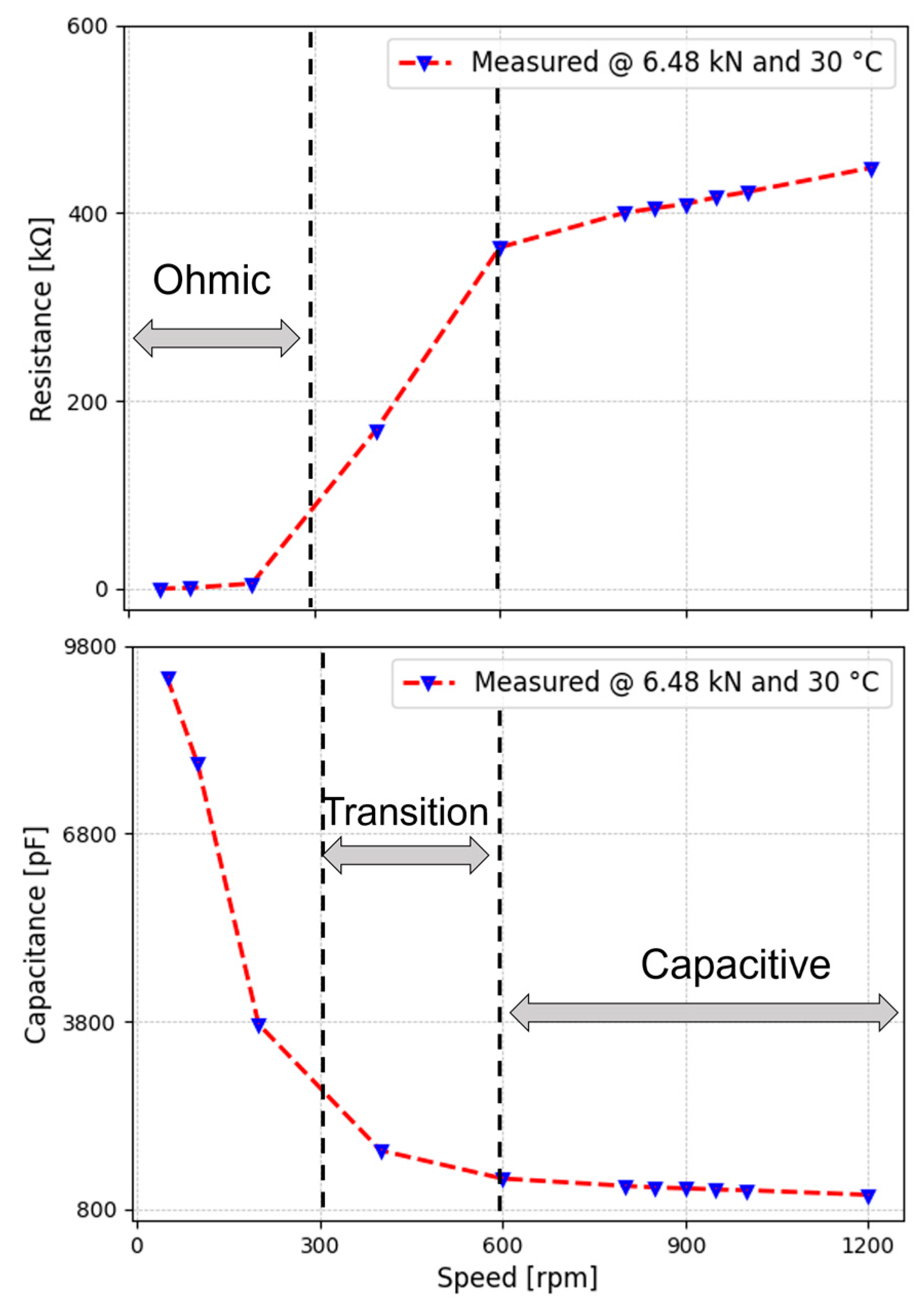
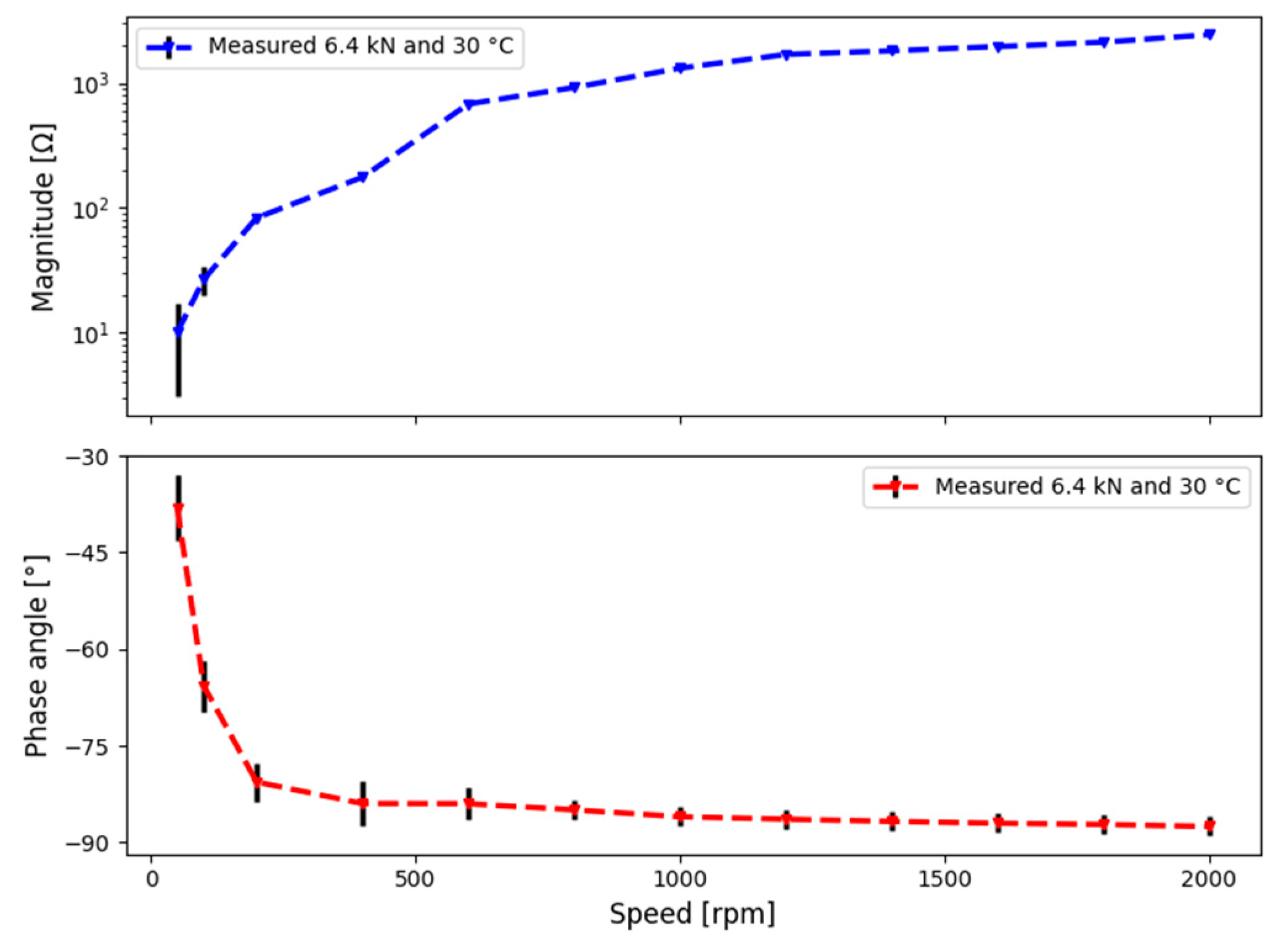
4.2. Transition from Ohmic to Capacitive Behaviour over Lubrication Regimes
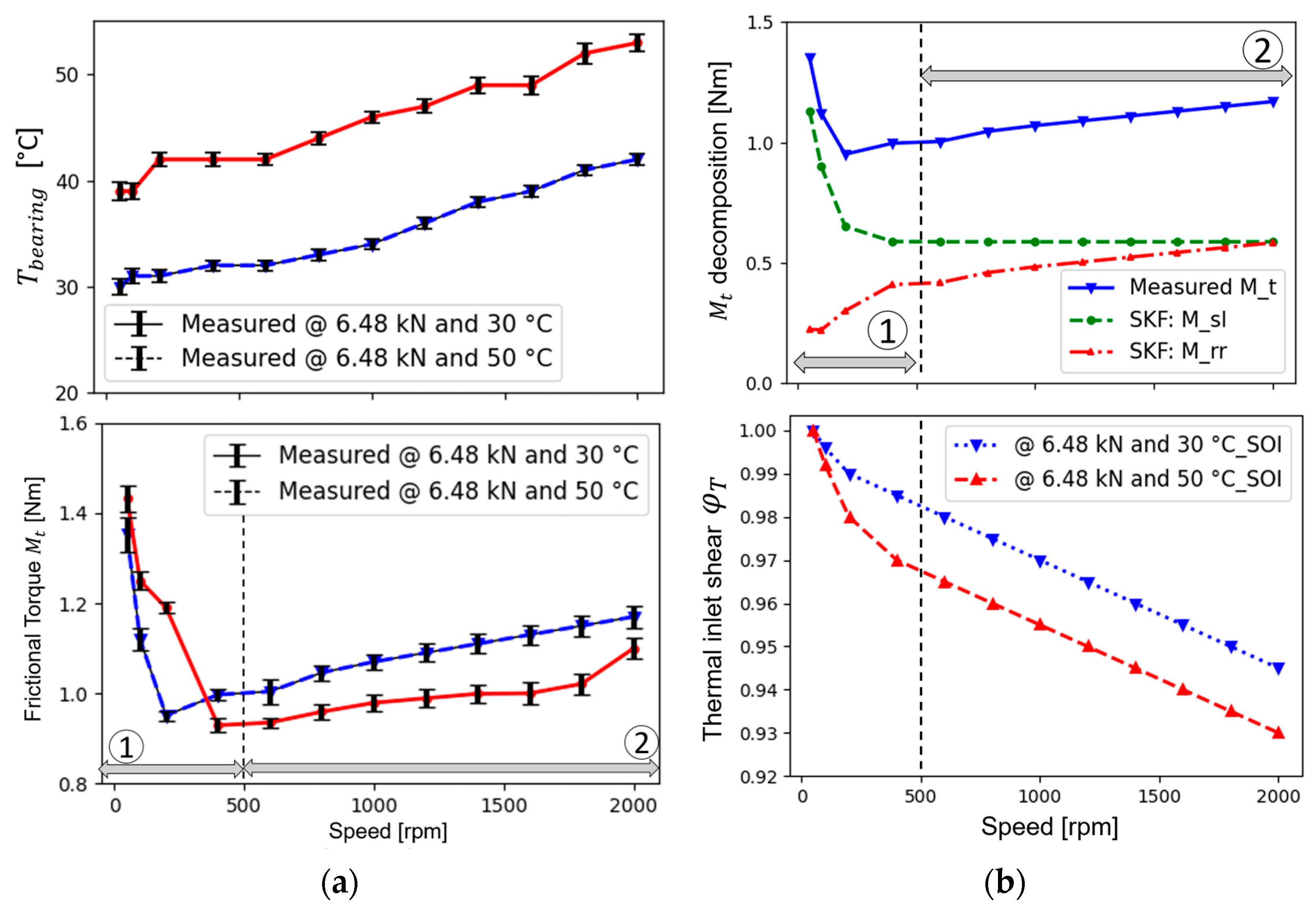
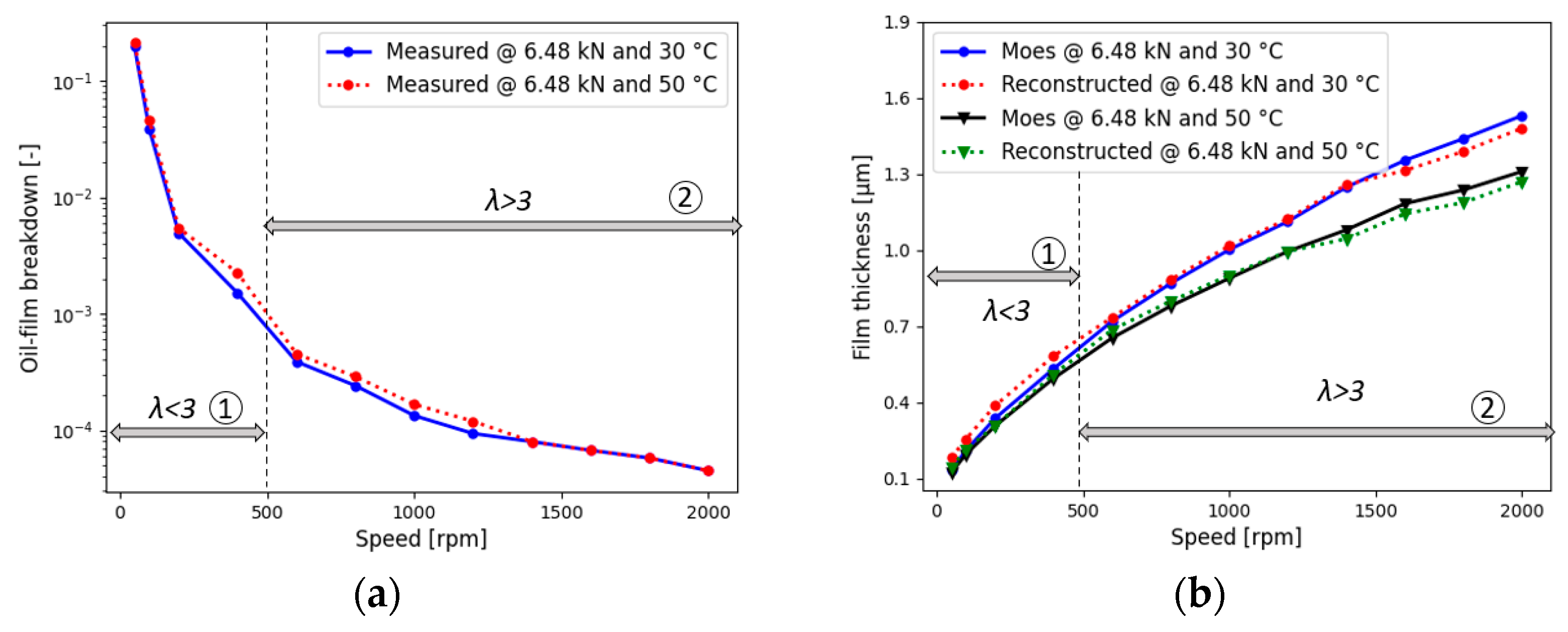
4.3. Impedance-Based Film Thickness Reconstruction: Maruyama’s Approach
4.4. Capacitance-Based Film Thickness Reconstruction: Jablonka’s Approach
5. Conclusions
- During the run-in phase of the bearing, the bearing resistance, reflecting the contact resistance, was observed to increase with time to a stationary value, whereas the capacitance was observed to decrease only slightly with time. This reflects the wear during run-in, changing the initial surface roughness of both the raceway and the roller, as proven by a priori and a posteriori measurements. Hence, monitoring the electrical impedance appears to have the potential to perform the run-in of bearings in a controlled way.
- An analogous trend was observed when migrating from boundary lubrication to mixed lubrication and finally towards EHL lubrication. Indeed, inspection of the bearing or contact impedance, and more specifically, the contact resistance and capacitance for various bearing speeds, clearly shows the transition from boundary to mixed lubrication as well as the transition from mixed to EHL lubrication. Although in boundary lubrication the resistive component is quasi-zero, it is observed to increase once mixed lubrication is established and film thickness increases. The capacitance, on the other hand, displays the inverse behaviour. Once EHL is established, the resistive contribution seems to increase at a much slower rate with increasing film thickness, whereas the capacitive component only slightly decreases.
- Monitoring the film breakdown indicator , proposed by Maruyama et al. [42,43,44], as a function of increasing rotational speed, and hence film thickness, very small values () were observed during EHL, whereas the value increases quickly with several orders of magnitude during mixed lubrication up to an order of in boundary lubrication. The breakdown indicator , however, did not show a very clear distinction once transitioning from mixed to boundary lubrication.
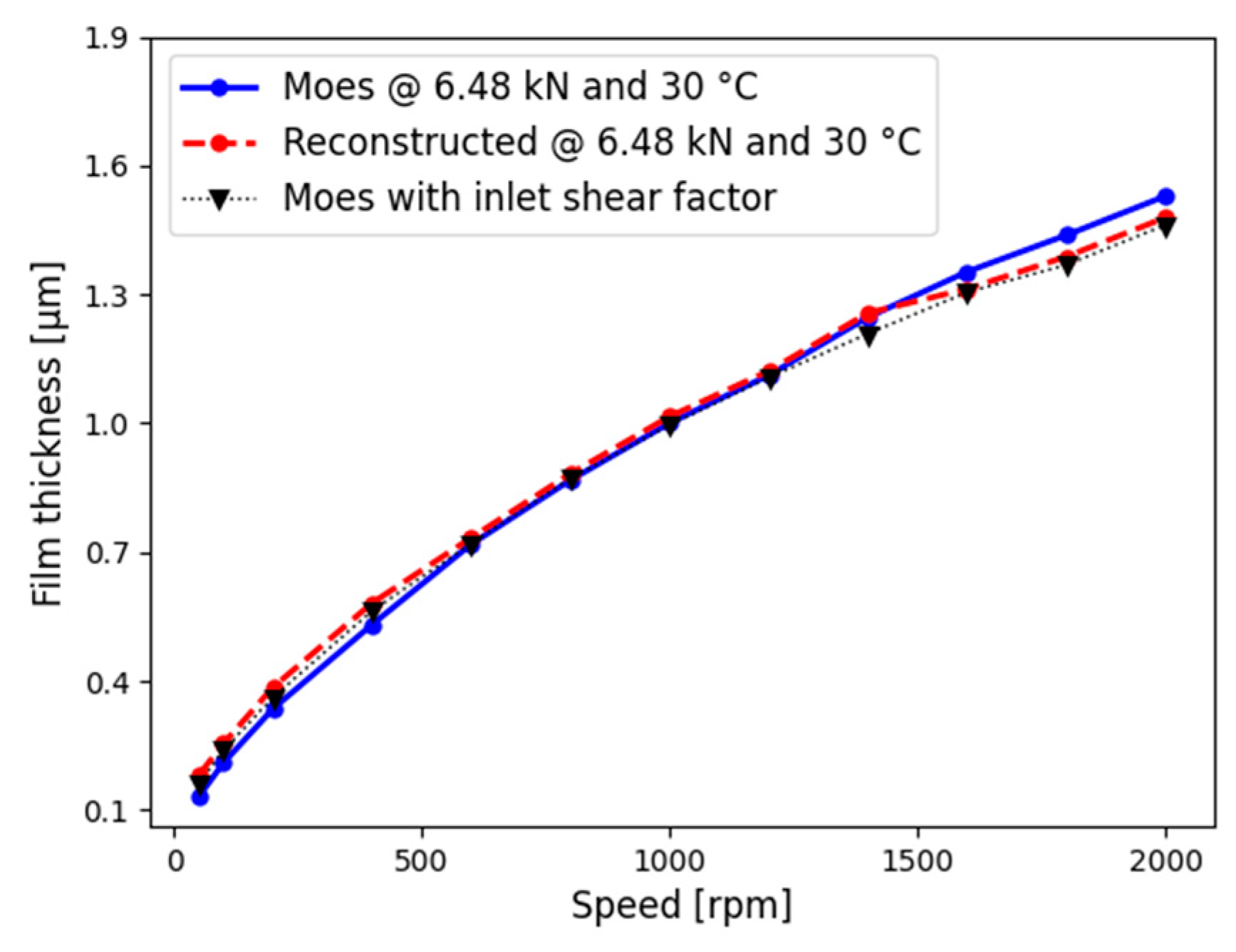
- Comparison of the impedance-based and capacitance-based film thickness estimations of, respectively, Maruyama et al. [42,43,44] and Jablonka et al. [29,30] to the analytically estimated film thickness by Moes’ correlation, corrected for inlet shear heating effects, revealed that both methods perform very well for EHL film thickness measurements. Deviations of both methods to the values of Moes were in the order of 1–2%, which is near the measurement uncertainty.
- As part of our ongoing research, our next step involves correlating measured resistance and impedance with predictions of the true-contact area using the Greenwood–Williamson/Tripp model. This correlation will bridge the gap between observed electrical behaviour and surface roughness during the operation, enhancing our understanding of the tribological dynamics of rolling bearings.
6. Patents
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| b | Hertzian half-width contact (m) |
| f | AC frequency (Hz) |
| Central film thickness of the oil (m) | |
| Indentation of the Hertzian contact (m) | |
| Average oil film thickness (m) | |
| Moes central film thickness (m) | |
| j | Imaginary unit |
| r | Roller radius (m) |
| Mean surface velocity (m/s) | |
| t | Time |
| w | Load-per-unit length (Nm) |
| Area of the Hertzian contact (m2) | |
| Flooded area (m2) | |
| Cavitation area (m2) | |
| Apparent contact area (m2) | |
| CRTB | Cylindrical roller thrust bearing |
| C | Capacitance pF |
| Single-contact capacitance (pF) | |
| Hertzian contact capacitance (pF) | |
| Inlet zone capacitance (pF) | |
| Bearing capacitance | |
| Area filled with oil and its capacitance | |
| Cavitation area and its capacitance | |
| Outside loaded area capacitance | |
| Equivalent Young’s modulus (Gpa) | |
| EHL | Elasto-hydrodynamic lubrication |
| F | Applied load (N) |
| Amplitude of alternating current (A) | |
| L | Length of the roller (m) |
| Global frictional torque (Nm) | |
| Vi | RMS input voltage (V) |
| Global frictional torque (Nm) | |
| N | Number of rolling elements |
| RBT | Roller-bearing tribometer |
| R | Resistance (Ohm) |
| Root mean square of roller surface roughness | |
| Root mean square of raceway surface roughness | |
| Resistance of the breakdown area under a stationary contact | |
| Resistance in breakdown area under a dynamic contact | |
| SRR | Slide-to-roll ratio |
| TEHL | Thermo-elasto-hydrodynamic lubrication |
| ). | |
| Bearing temperature (°C) | |
| Oil inlet temperature (°C) | |
| Oil outlet temperature (°C) | |
| Bearing temperature (°C) | |
| U | Dimensionless speed parameter |
| Amplitude of alternating voltage (V) | |
| W | Dimensionless load parameter |
| Reactance of the capacitor (Ohms) | |
| Z | Bearing impedance (Ohms) |
| Modulus of complex impedance under dynamic contact conditions (Ohms) | |
| Single-contact impedance upper and lower raceway | |
| Operating viscosity of the oil at atmospheric pressure (Pas) | |
| Oil film breakdown | |
| Phase angle under dynamic contact (degree) | |
| Phase angle under stationary contact (degree) | |
| Voltage component at 90 degrees | |
| Current component at 90 degrees | |
| Dimensionless number | |
| Pressure viscosity coefficient of lubricant (Pa−1) | |
| Dielectric constant of vacuum | |
| Relative dielectric constant of oil | |
| Dielectric constant of air | |
| Probability of the contract for an asperity with height | |
| ) | |
| Film parameter | |
| Angular frequency of AC voltage (rad/s) | |
| Cut-off frequency | |
| Thermal reduction factor of raceway |
Appendix A
References
- Doll, G.L. Causes and Effects of Bearing Damage. Roll. Bear. Tribol. 2023, 205–231. [Google Scholar] [CrossRef]
- Gohar, R. Elastohydrodynamics; World Scientific: Singapore, 2001; ISBN 1-86094-170-2. [Google Scholar]
- Dowson, D.; Higginson, G.R. Reflections on Early Studies of Elasto-Hydrodynamic Lubrication. Solid Mech. Appl. 2006, 134, 3–21. [Google Scholar] [CrossRef]
- Manjunath, M.; Fauconnier, D.; Ost, W.; De Baets, P. Experimental Analysis of Rolling Torque and Thermal Inlet Shear Heating in Tapered Roller Bearings. Machines 2023, 11, 801. [Google Scholar] [CrossRef]
- Magar, H.S.; Hassan, R.Y.A.; Mulchandani, A. Electrochemical Impedance Spectroscopy (Eis): Principles, Construction, and Biosensing Applications. Sensors 2021, 21, 6578. [Google Scholar] [CrossRef]
- Spikes, H.A. Triboelectrochemistry: Influence of Applied Electrical Potentials on Friction and Wear of Lubricated Contacts. Tribol. Lett. 2020, 68, 90. [Google Scholar] [CrossRef]
- Albahrani, S.; Philippon, D.; Vergne, P.; Bluet, J. A Review of in Situ Methodologies for Studying Elastohydrodynamic Lubrication. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2016, 230, 86–110. [Google Scholar] [CrossRef]
- Siripongse, C.; Rogers, P.R.; Cameron, A. Discharge through Oil Films. Engineering 1958, 186, 146–147. [Google Scholar]
- Gohar, R.; Cameron, A. Optical Measurement of Oil Film Thickness under Elasto-Hydrodynamic Lubrication. Nature 1963, 200, 458–459. [Google Scholar] [CrossRef]
- Dwyer-Joyce, R.S.; Donohoe, C.J.; Drinkwater, B.W. Method and Apparatus for Determining Thickness of a 40Lubricant Film. U.S. Patent 7,066,027, 27 June 2006. [Google Scholar]
- Cameron, A.; Gohar, R. Theoretical and Experimental Studies of the Oil Film in Lubricated Point Contact; The Royal Society Publishing: London, UK, 1966. [Google Scholar]
- Dwyer-Joyce, R.S.; Harper, P.; Drinkwater, B.W. A Method for the Measurement of Hydrodynamic Oil Films Using Ultrasonic Reflection. Tribol. Lett. 2004, 17, 337–348. [Google Scholar] [CrossRef]
- Dwyer-Joyce, R.S.; Reddyhoff, T.; Drinkwater, B.W. Operating Limits for Acoustic Measurement of Rolling Bearing Oil Film Thickness. Tribol. Trans. 2004, 47, 366–375. [Google Scholar] [CrossRef]
- MacConochie, I.O.; Cameron, A. The Measurement of Oil-Film Thickness in Gear Teeth. J. Basic Eng. 1960, 82, 29–34. [Google Scholar] [CrossRef]
- Dyson, A. Investigation of the Discharge-Voltage Method of Measuring the Thickness of Oil Films Formed in a Disc Machine under Conditions of Elastohydrodynamic Lubrication. Proc. Inst. Mech. Eng. 1966, 181, 633–652. [Google Scholar] [CrossRef]
- Lane, T.B.; Hughes, J.R. A Study of the Oil-Film Formation in Gears by Electrical Resistance Measurements. Br. J. Appl. Phys. 1952, 3, 315. [Google Scholar] [CrossRef]
- Furey, M.J. Metallic Contact and Friction between Sliding Surfaces. ASLE Trans. 1961, 4, 1–11. [Google Scholar] [CrossRef]
- Lord, J.; Larsson, R. Film-Forming Capability in Rough Surface EHL Investigated Using Contact Resistance. Tribol. Int. 2008, 41, 831–838. [Google Scholar] [CrossRef]
- Lugt, P.M.; Severt, R.W.M.; Fogelströ, J.; Tripp, J.H. Influence of Surface Topography on Friction, Film Breakdown and Running-in in the Mixed Lubrication Regime. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2001, 215, 519–533. [Google Scholar] [CrossRef]
- Crook, A. The Lubrication of Rollers. Philos. Trans. R. Soc. B Biol. Sci. 1958, 250, 387–409. [Google Scholar] [CrossRef]
- Crook, A.W. Elastohydrodynamic Lubrication of Rollers. Nature 1961, 190, 1182–1183. [Google Scholar] [CrossRef]
- Dyson, A.; Naylor, H.; Wilson, A.R. Paper 10: The Measurement of Oil-Film Thickness in Elastohydrodynamic Contacts. Proc. Inst. Mech. Eng. Conf. Proc. 1965, 180, 119–134. [Google Scholar] [CrossRef]
- Chua, W.H.; Stachowiak, G.W. The Study of the Dynamic Thickness of Organic Boundary Films under Metallic Sliding Contact. Tribol. Lett. 2010, 39, 151–161. [Google Scholar] [CrossRef]
- Hamilton, G.M.; Moore, S.L. First Paper: Measurement of the Oil-Film Thickness between the Piston Rings and Liner of a Small Diesel Engine. Proc. Inst. Mech. Eng. 1974, 188, 253–261. [Google Scholar] [CrossRef]
- Sherrington, I.; Smith, E.H. Experimental Methods for Measuring the Oil-Film Thickness between the Piston-Rings and Cylinder-Wall of Internal Combustion Engines. Tribol. Int. 1985, 18, 315–320. [Google Scholar] [CrossRef]
- Wilson, A.R. The relative thickness of grease and oil films in rolling bearings. Proc. Inst. Mech. Eng. 1979, 193, 185–192. [Google Scholar] [CrossRef]
- Cen, H.; Lugt, P.M. Film Thickness in a Grease Lubricated Ball Bearing. Tribol. Int. 2019, 134, 26–35. [Google Scholar] [CrossRef]
- Barz, M. Die Schmierfilmbildung in Fettgeschmierten Schnellaufenden Spindellagern. Ph.D. Dissertation, Leibniz Universität Hannover, Hanover, Germany, 1996. [Google Scholar]
- Jablonka, K.; Glovnea, R.; Bongaerts, J. Evaluation of EHD Films by Electrical Capacitance. J. Phys. D Appl. Phys. 2012, 45, 385301. [Google Scholar] [CrossRef]
- Jablonka, K.; Glovnea, R.; Bongaerts, J.; Morales-Espejel, G. The Effect of the Polarity of the Lubricant upon Capacitance Measurements of EHD Contacts. Tribol. Int. 2013, 61, 95–101. [Google Scholar] [CrossRef]
- Otsu, T.; Tanaka, H.; Izumi, N.; Sugimura, J. Effect of Surrounding Gas on Cavitation in EHL. Tribol. Online 2009, 4, 50–54. [Google Scholar] [CrossRef]
- Otsu, T.; Tanaka, H.; Sugimura, J. Initiation and Growth of Gaseous Cavity in Concentrated Contact in Various Surrounding Gases. Tribol. Int. 2012, 53, 68–75. [Google Scholar] [CrossRef]
- van Emden, E.; Venner, C.H.; Morales-Espejel, G.E. Aspects of Flow and Cavitation around an EHL Contact. Tribol. Int. 2016, 95, 435–448. [Google Scholar] [CrossRef]
- Nogi, T. An Analysis of Starved EHL Point Contacts with Reflow. Tribol. Online 2015, 10, 64–75. [Google Scholar] [CrossRef]
- Shetty, P.; Meijer, R.J.; Osara, J.A.; Lugt, P.M. Measuring Film Thickness in Starved Grease-Lubricated Ball Bearings: An Improved Electrical Capacitance Method. Tribol. Trans. 2022, 65, 869–879. [Google Scholar] [CrossRef]
- Manabe, K.; Nakano, K. Breakdown of Oil Films and Formation of Residual Films. Tribol. Int. 2008, 41, 1103–1113. [Google Scholar] [CrossRef]
- Nakano, K.; Manabe, K. Breakdown Processes of Boundary Films Formed by Oiliness Additives. Tribol. Online 2011, 6, 277–283. [Google Scholar] [CrossRef]
- Schnabel, S.; Marklund, P.; Minami, I.; Larsson, R. Monitoring of Running-in of an EHL Contact Using Contact Impedance. Tribol. Lett. 2016, 63, 35. [Google Scholar] [CrossRef]
- Nihira, T.; Manabe, K.; Tadokoro, C.; Ozaki, S.; Nakano, K. Complex Impedance Measurement Applied to Short-Time Contact Between Colliding Steel Surfaces. Tribol. Lett. 2015, 57, 29. [Google Scholar] [CrossRef]
- De Gaetano, D.; Zhu, W.; Sun, X.; Chen, X.; Griffo, A.; Jewell, G.W. Experimental Ball Bearing Impedance Analysis Under Different Speed and Electrical Conditions. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 1312–1321. [Google Scholar] [CrossRef]
- Graf, S.; Werner, M.; Koch, O.; Götz, S.; Sauer, B. Breakdown Voltages in Thrust Bearings: Behavior and Measurement. Tribol. Trans. 2023, 66, 488–496. [Google Scholar] [CrossRef]
- Maruyama, T.; Nakano, K. In Situ Quantification of Oil Film Formation and Breakdown in EHD Contacts. Tribol. Trans. 2018, 61, 1057–1066. [Google Scholar] [CrossRef]
- Maruyama, T.; Maeda, M.; Nakano, K. Lubrication Condition Monitoring of Practical Ball Bearings by Electrical Impedance Method. Tribol. Online 2019, 14, 327–338. [Google Scholar] [CrossRef]
- Maruyama, T.; Radzi, F.; Sato, T.; Iwase, S.; Maeda, M.; Nakano, K. Lubrication Condition Monitoring in EHD Line Contacts of Thrust Needle Roller Bearing Using the Electrical Impedance Method. Lubricants 2023, 11, 223. [Google Scholar] [CrossRef]
- Yu, M.; Zhang, J.; Joedicke, A.; Reddyhoff, T. Using Electrical Impedance Spectroscopy to Identify Equivalent Circuit Models of Lubricated Contacts with Complex Geometry: In-Situ Application to Mini Traction Machine. Tribol. Int. 2024, 192, 109286. [Google Scholar] [CrossRef]
- Furtmann, A. Elektrisches Verhalten von Maschinenelementen im Antriebsstrang, Leibniz Universitat Hannover. Ph.D. Dissertation, Leibniz Universität Hannover, Hanover, Germany, 2017. [Google Scholar]
- Gonda, A.; Capan, R.; Bechev, D.; Sauer, B. The Influence of Lubricant Conductivity on Bearing Currents in the Case of Rolling Bearing Greases. Lubricants 2019, 7, 108. [Google Scholar] [CrossRef]
- Kirchner, E.; Bienefeld, C.; Schirra, T.; Moltschanov, A. Predicting the Electrical Impedance of Rolling Bearings Using Machine Learning Methods. Machines 2022, 10, 156. [Google Scholar] [CrossRef]
- Hausner, S.; Bode, B.; Heine, A.; Kießling, M.; Klingebiel, J.; Thiel, C. E-Lub Tester – Lubricant Impedance & Electrical Breakdown Test Rig: flucon fluid control GmbH 2023. Available online: https://flucon.de/en/products/e-lub-tester/ (accessed on 15 January 2023).
- Muetze, A.; Binder, A. Calculation of Motor Capacitances for Prediction of the Voltage Across the Bearings in Machines of Inverter-Based Drive Systems. IEEE Trans. Ind. Appl. 2007, 43, 665–672. [Google Scholar] [CrossRef]
- Schirra, T.; Martin, G.; Puchtler, S.; Kirchner, E. Electric Impedance of Rolling Bearings—Consideration of Unloaded Rolling Elements. Tribol. Int. 2021, 158, 106927. [Google Scholar] [CrossRef]
- Moes, H. Lubrication and Beyond; Lecture Notes, Code 115531; Twente University Press: Enschede, The Netherlands, 2000; 366p, Available online: https://www.researchgate.net/publication/259757915_Moes_H_Lubrication_and_Beyond_Lecture_Notes_Code_115531_Twente_University_Press_Enschede_Netherlands_2000_366_p (accessed on 15 January 2023).
- Echávarri Otero, J.; de la Guerra Ochoa, E.; Chacón Tanarro, E.; Franco Martínez, F.; Contreras Urgiles, R.W. An Analytical Approach for Predicting EHL Friction: Usefulness and Limitations. Lubricants 2022, 10, 141. [Google Scholar] [CrossRef]
- Bearing Friction, Power Loss and Starting Torque|SKF. Available online: https://www.skf.com/group/products/rolling-bearings/principles-of-rolling-bearing-selection/bearing-selection-process/operating-temperature-and-speed/bearing-friction-power-loss-and-starting-torque (accessed on 23 August 2022).
- Spikes, H. Basics of EHL for Practical Application. Lubr. Sci. 2015, 27, 45–67. [Google Scholar] [CrossRef]
- Simon, M. Messung von Elasto-Hydrodynamischen Parametern und Ihre Auswirkung auf die Grübchentragfähigkeit Vergüteter Scheiben und Zahnräder. Ph.D. Dissertation, Technische Universität Braunschweig, Braunschweig, Germany, 1984. [Google Scholar]
- Kreil, O.; Schouten, M.J.W. Einfluss der Oberflächenstruktur auf Druckverteilung und Schmierfilmdicke im EHD-Kontakt. Ph.D. Dissertation, Technische Universität München, Munich, Germany, 2008. [Google Scholar]
- NSK Ltd. Roller Bearing Catalogue, E1254j; NSK Ltd.: Tokyo, Japan, 2019. [Google Scholar]
- Chen, S.; Han, Z.; Zeng, Q.; Wang, B.; Wang, L.; Guo, L.; Shao, Y. Influence of Manufacturing Surface Topography on Torque and Load Bearing Capacity of Hydro-Viscous Drive Clutch under Mixed Lubrication Stage. Ind. Lubr. Tribol. 2024, 76, 81–90. [Google Scholar] [CrossRef]
- Schneider, V.; Liu, H.-C.; Bader, N.; Furtmann, A.; Poll, G. Empirical Formulae for the Influence of Real Film Thickness Distribution on the Capacitance of an EHL Point Contact and Application to Rolling Bearings. Tribol. Int. 2021, 154, 106714. [Google Scholar] [CrossRef]


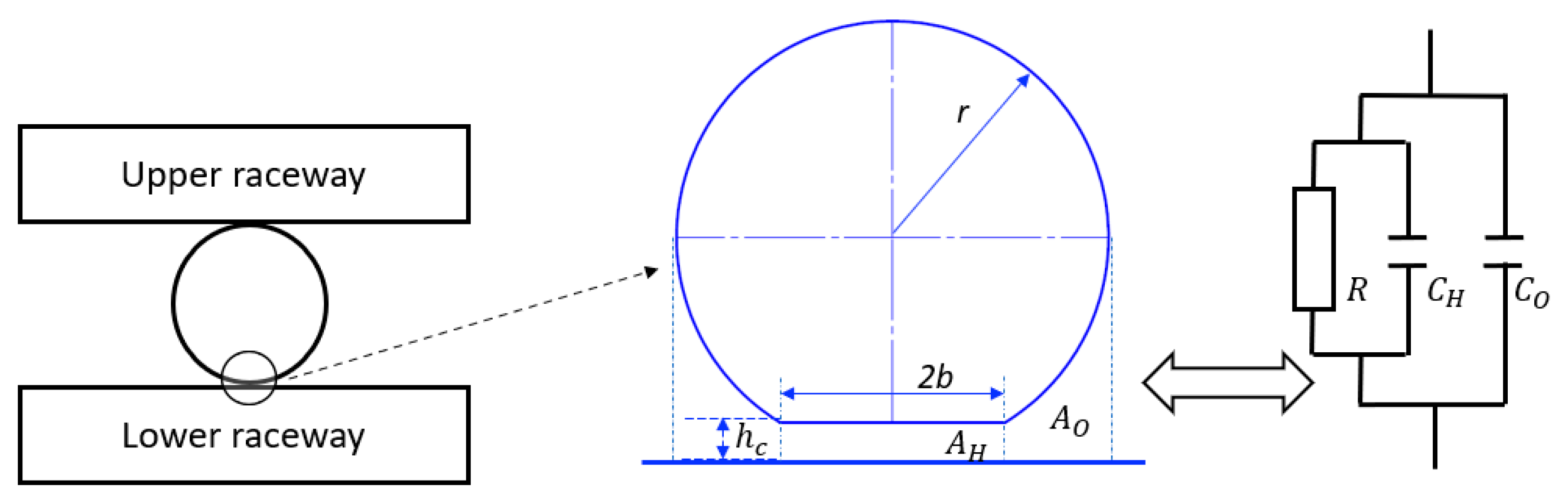
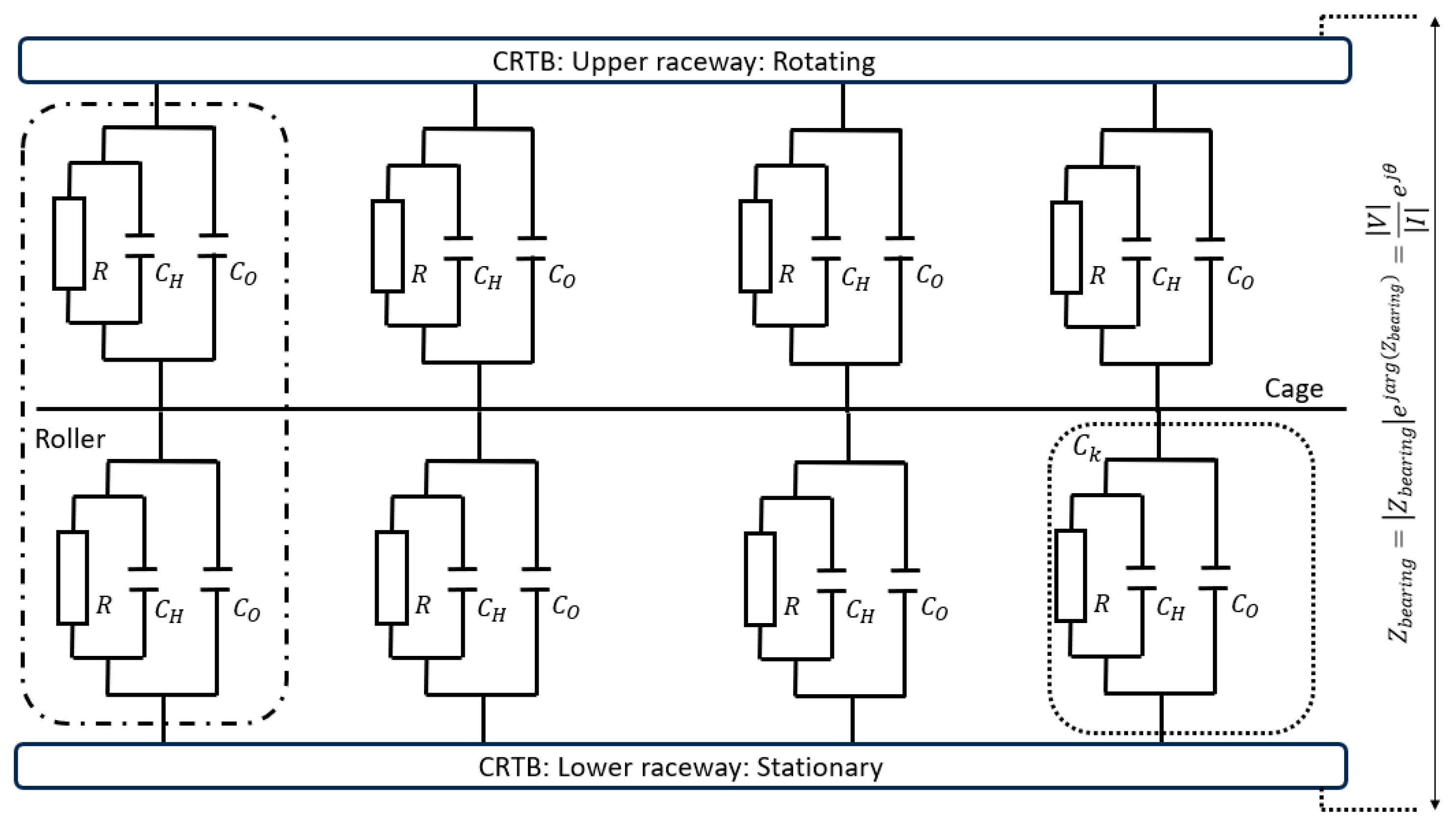
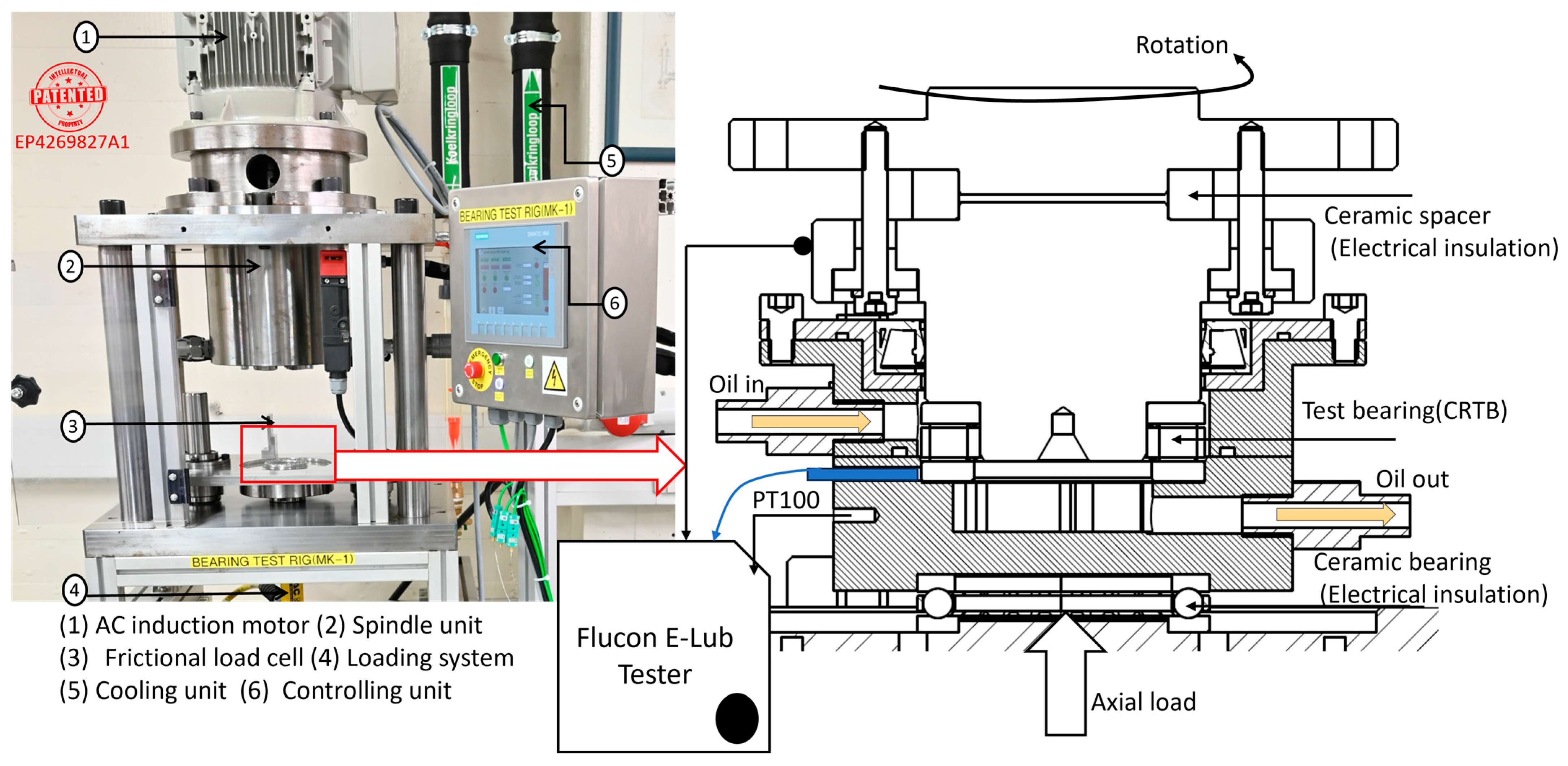




| Bearing | SKF 81208TN: Cylindrical Roller Thrust Bearing |
|---|---|
| Dimension | 40 × 68 × 19 mm |
| Length of Roller | 9 mm |
| Roller end face curvature | 0.50 mm |
| Roller Diameter | 9 mm |
| Cage material | Polyamide 6.6 |
| FVA 3A | Units | |
|---|---|---|
| Oil type | Paraffin-based solvent raffinate | |
| Density | 884.1 | kg/m3 |
| Kinematic Viscosity at 40 °C | 90.02 | mm2/s |
| Kinematic Viscosity at 100 °C | 10.41 | mm2/s |
| Viscosity-pressure Coefficient (at 200 MPa) | 2.16 × 103 bar−1 @ 25 °C 1.58 × 103 bar−1 @ 80 °C | |
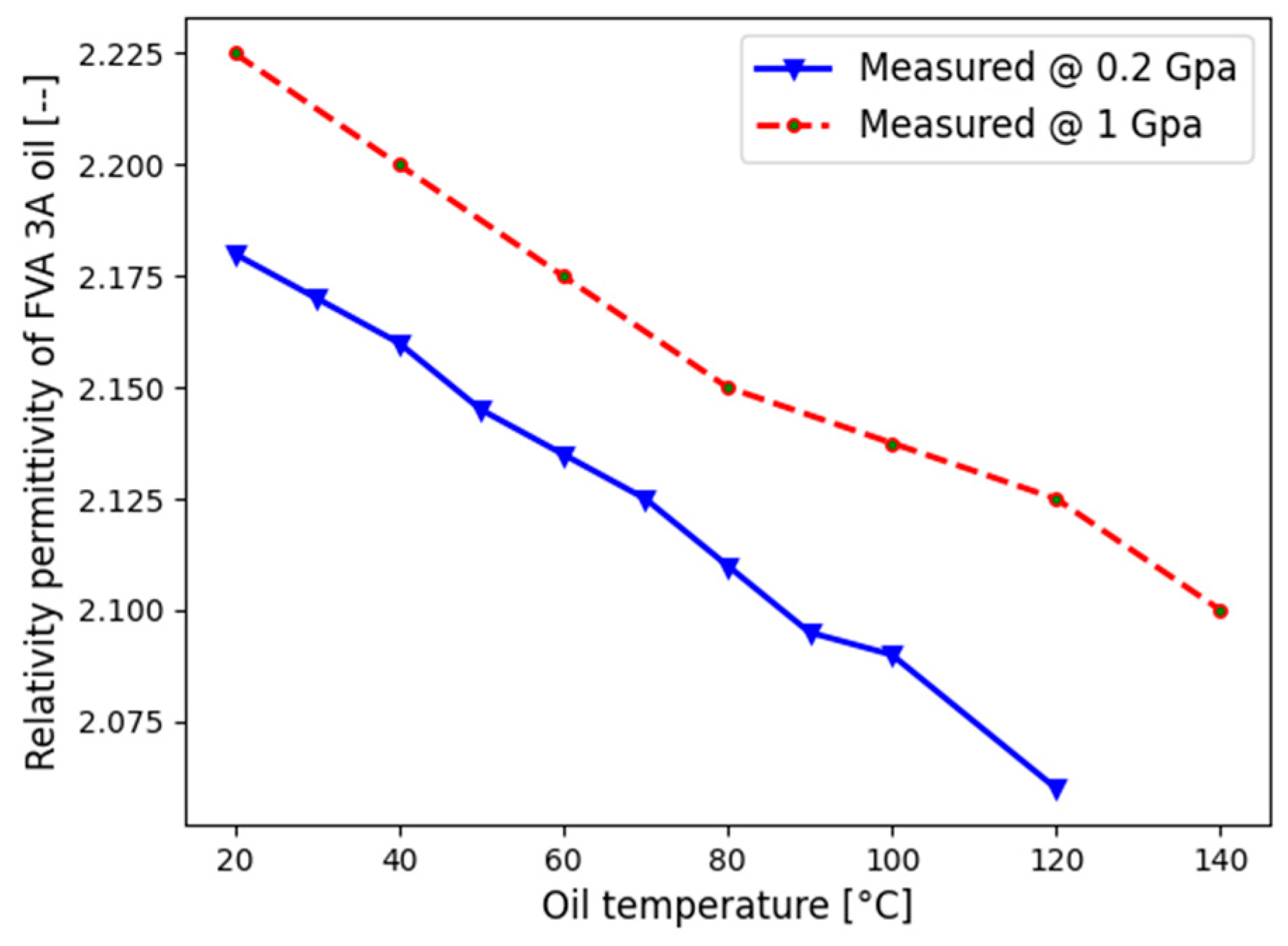
| Relativity permittivity | @ 0.2 GPa @1 GPa. The measurements were carried out at oscillating circuit frequencies of 40 kHz. | -- |
| Dielectric constant of vacuum | 8.85 × 10−12 | F/m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Manjunath, M.; Hausner, S.; Heine, A.; De Baets, P.; Fauconnier, D. Electrical Impedance Spectroscopy for Precise Film Thickness Assessment in Line Contacts. Lubricants 2024, 12, 51. https://doi.org/10.3390/lubricants12020051
Manjunath M, Hausner S, Heine A, De Baets P, Fauconnier D. Electrical Impedance Spectroscopy for Precise Film Thickness Assessment in Line Contacts. Lubricants. 2024; 12(2):51. https://doi.org/10.3390/lubricants12020051
Chicago/Turabian StyleManjunath, Manjunath, Simon Hausner, André Heine, Patrick De Baets, and Dieter Fauconnier. 2024. "Electrical Impedance Spectroscopy for Precise Film Thickness Assessment in Line Contacts" Lubricants 12, no. 2: 51. https://doi.org/10.3390/lubricants12020051
APA StyleManjunath, M., Hausner, S., Heine, A., De Baets, P., & Fauconnier, D. (2024). Electrical Impedance Spectroscopy for Precise Film Thickness Assessment in Line Contacts. Lubricants, 12(2), 51. https://doi.org/10.3390/lubricants12020051