Nonlinear Dynamic Analysis of a Spur Gear Pair System with Wear Considering the Meshing Position
Abstract
1. Introduction
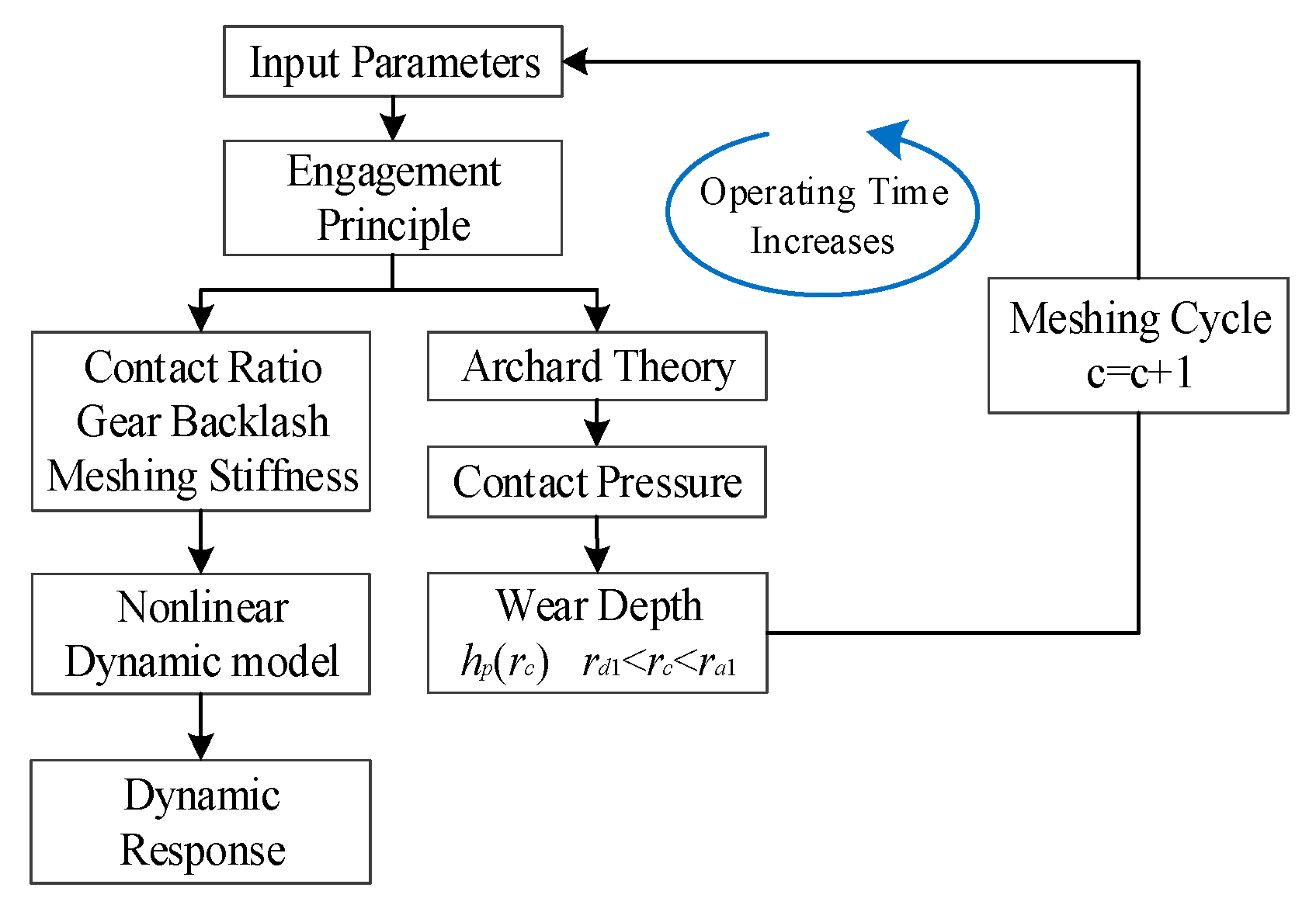
2. Nonlinear Dynamic Model of the Gear System
2.1. The Gear Backlash Function
2.2. The Contact Ratio of the Gear Pair
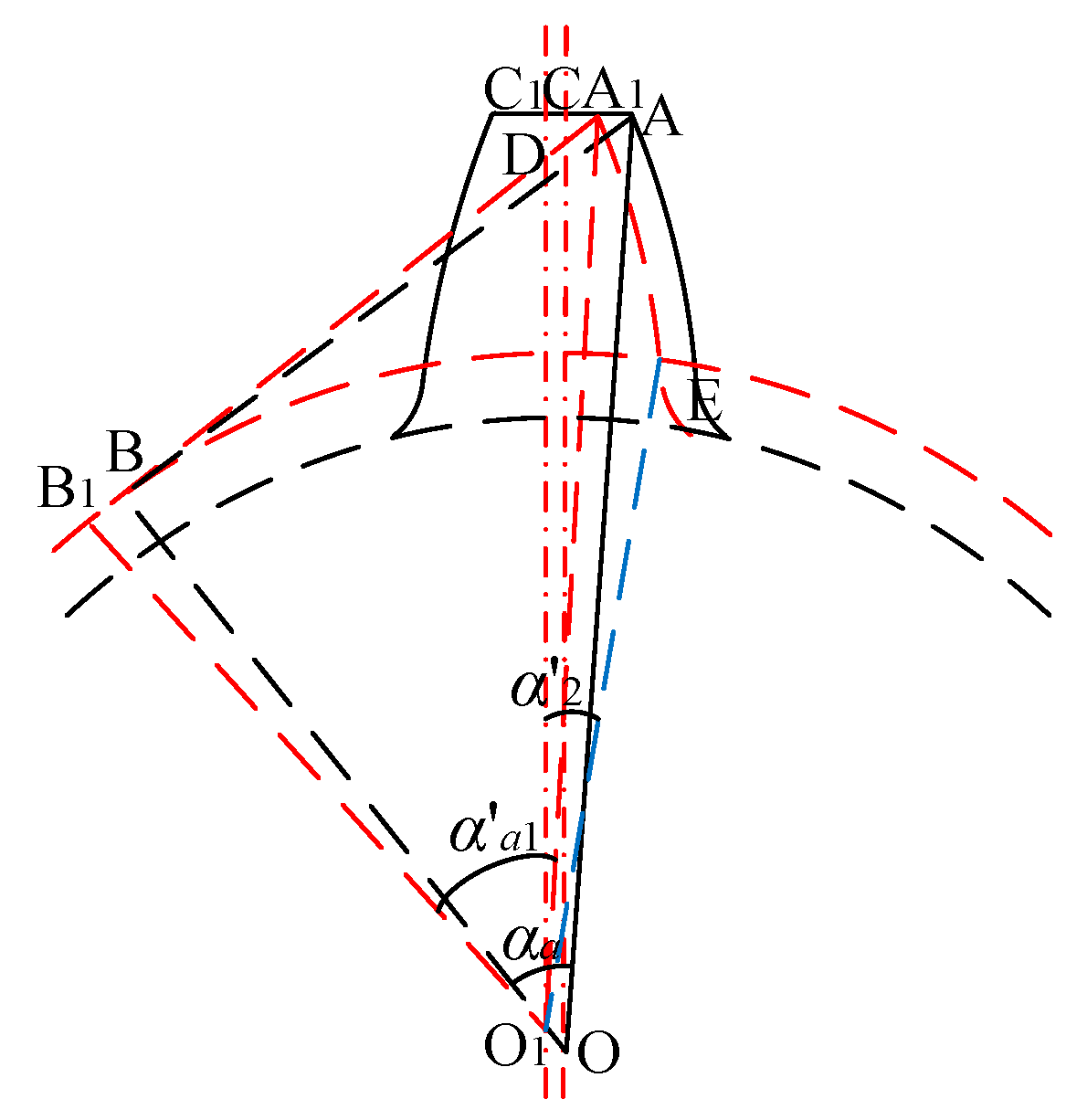
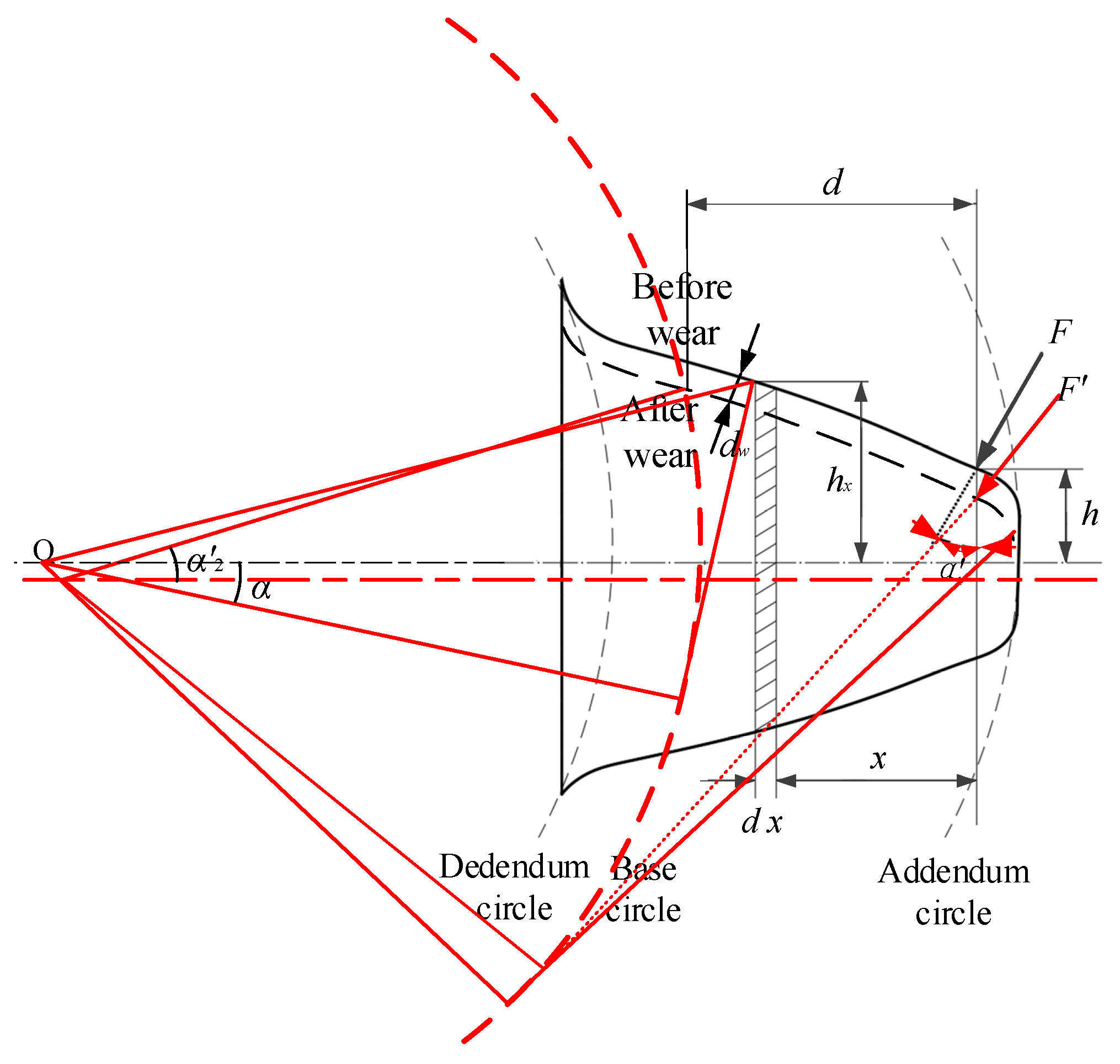
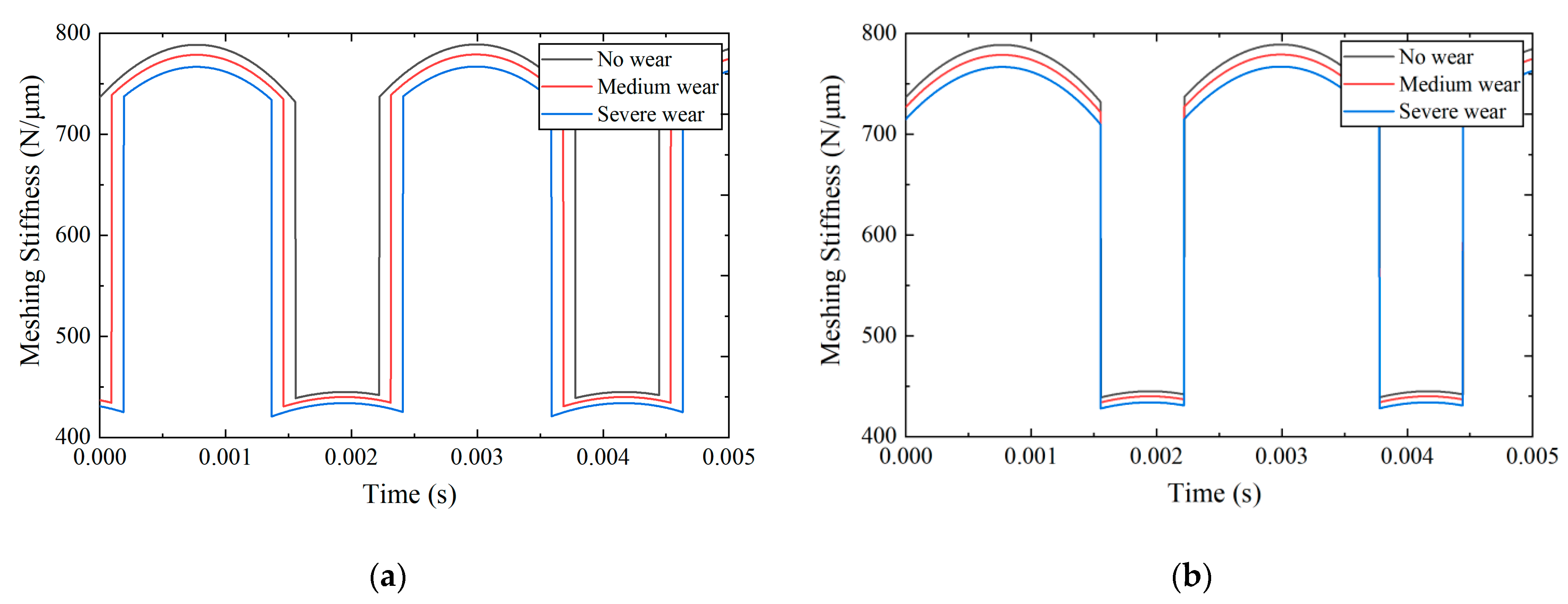
2.3. The Meshing Stiffness of the Gear Pair with Wear
2.4. Friction Force and Friction Moment
2.5. Nonlinear Dynamic Model of the Gear Pair
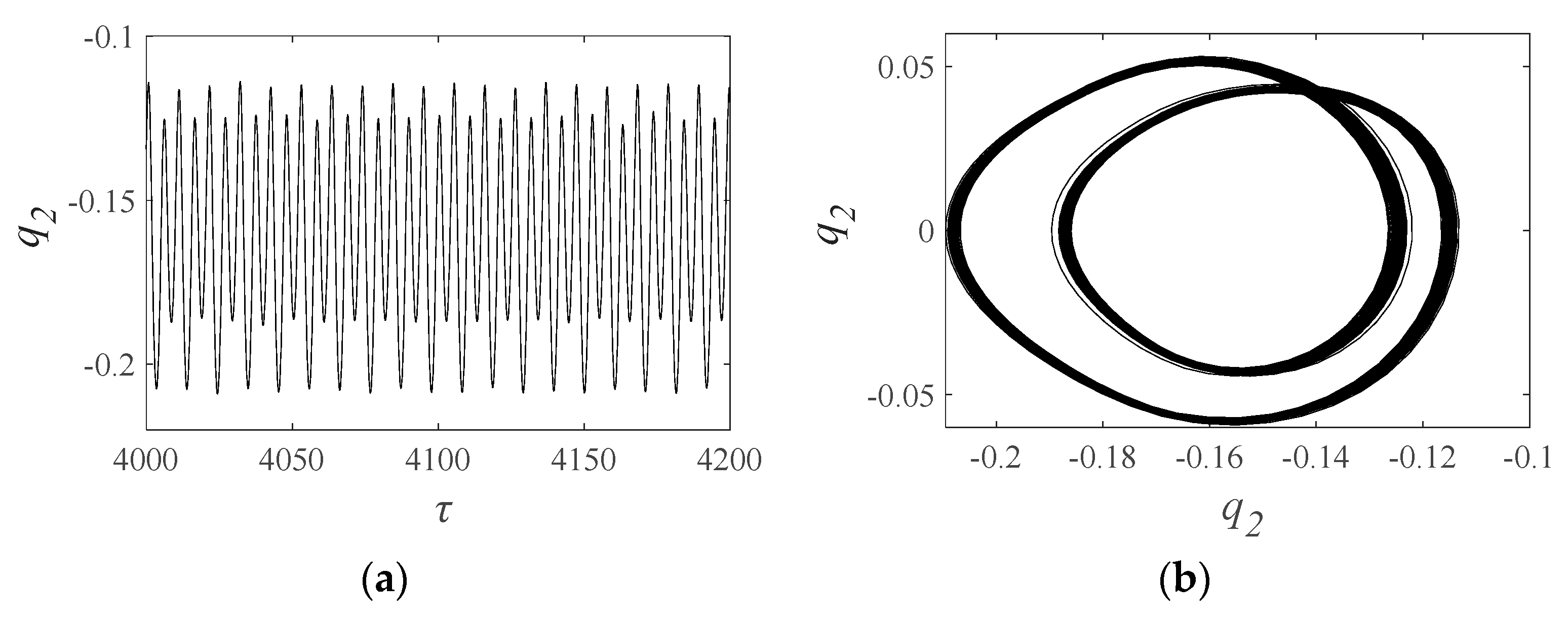
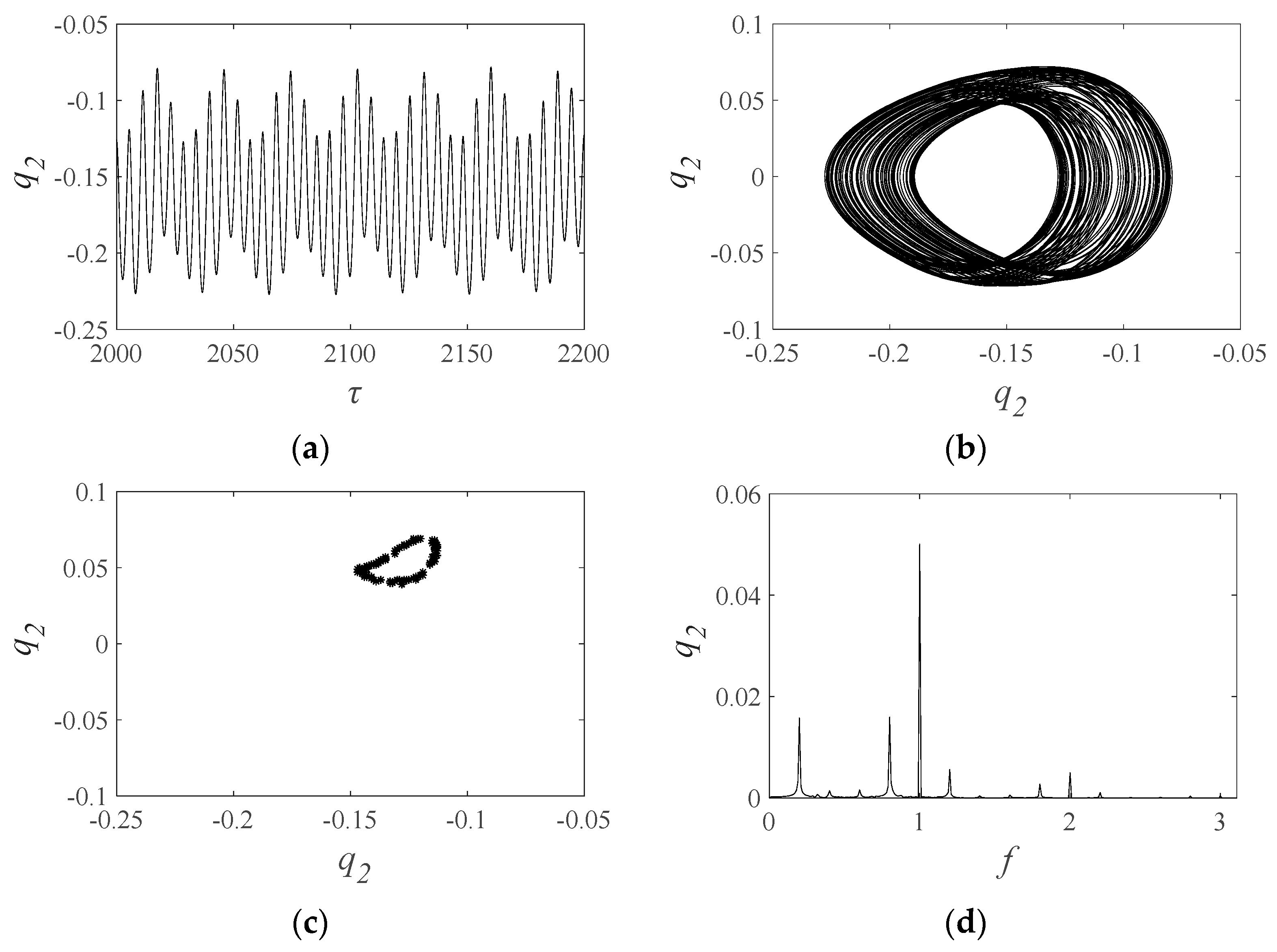
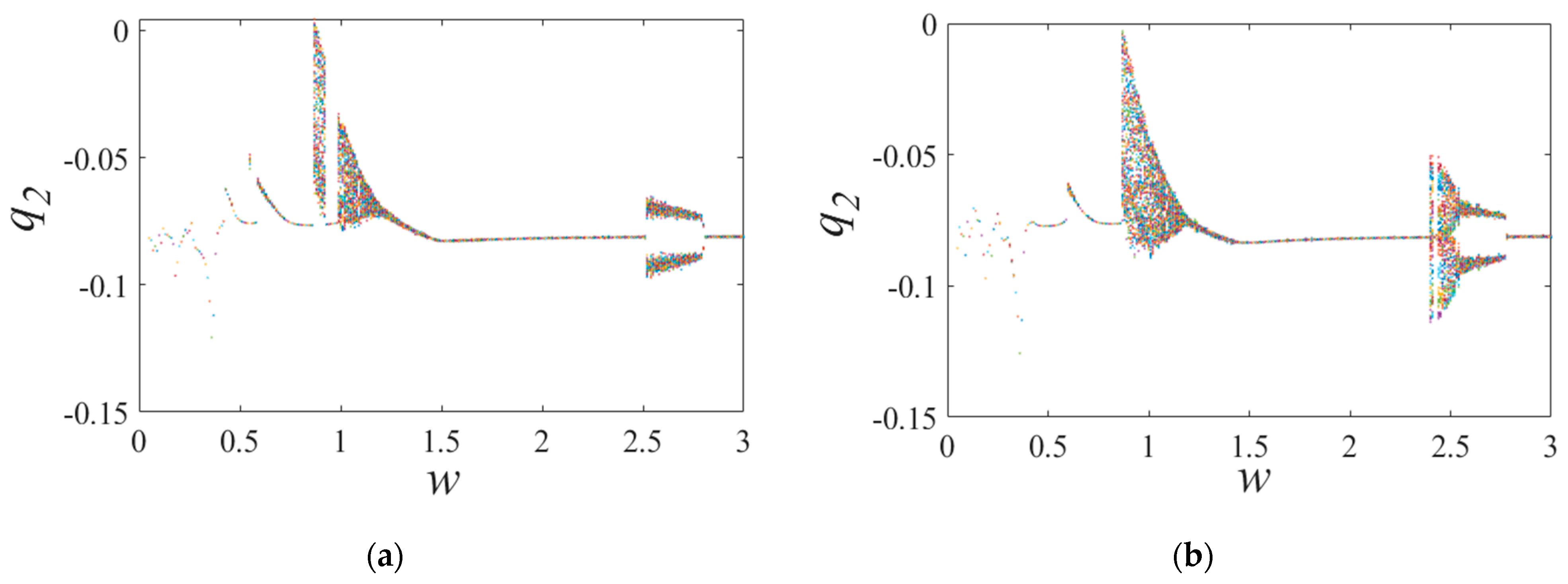
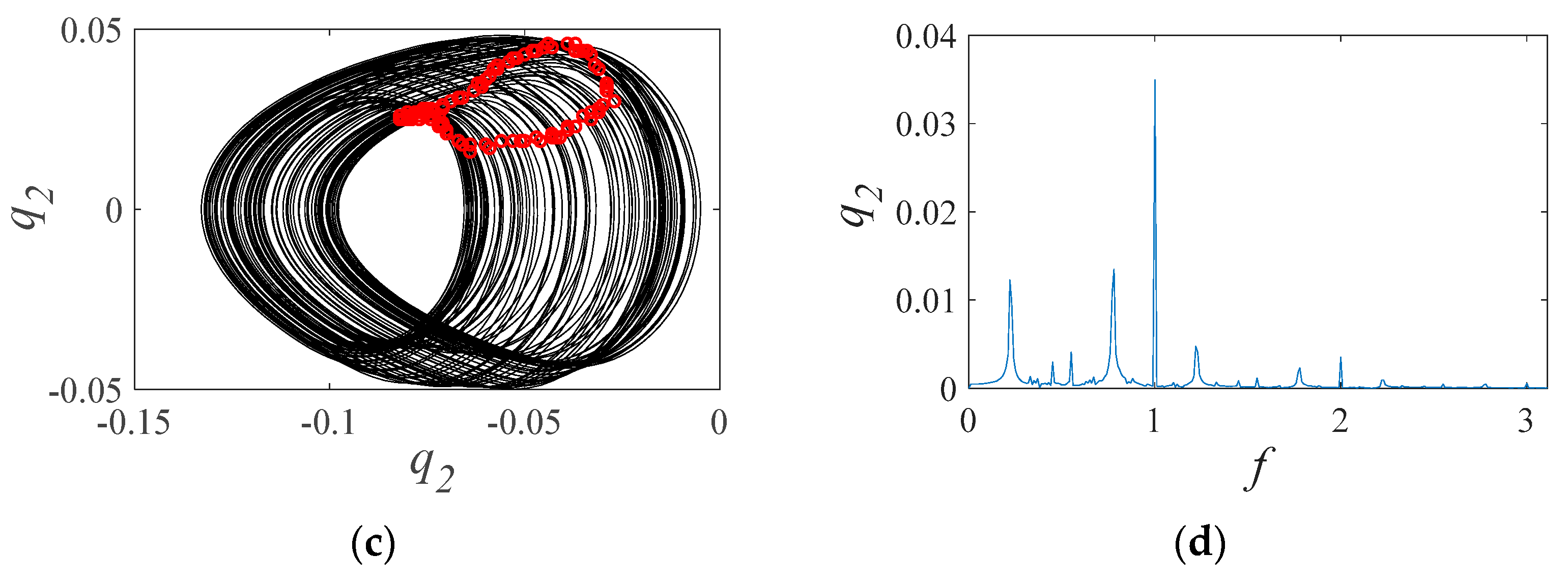
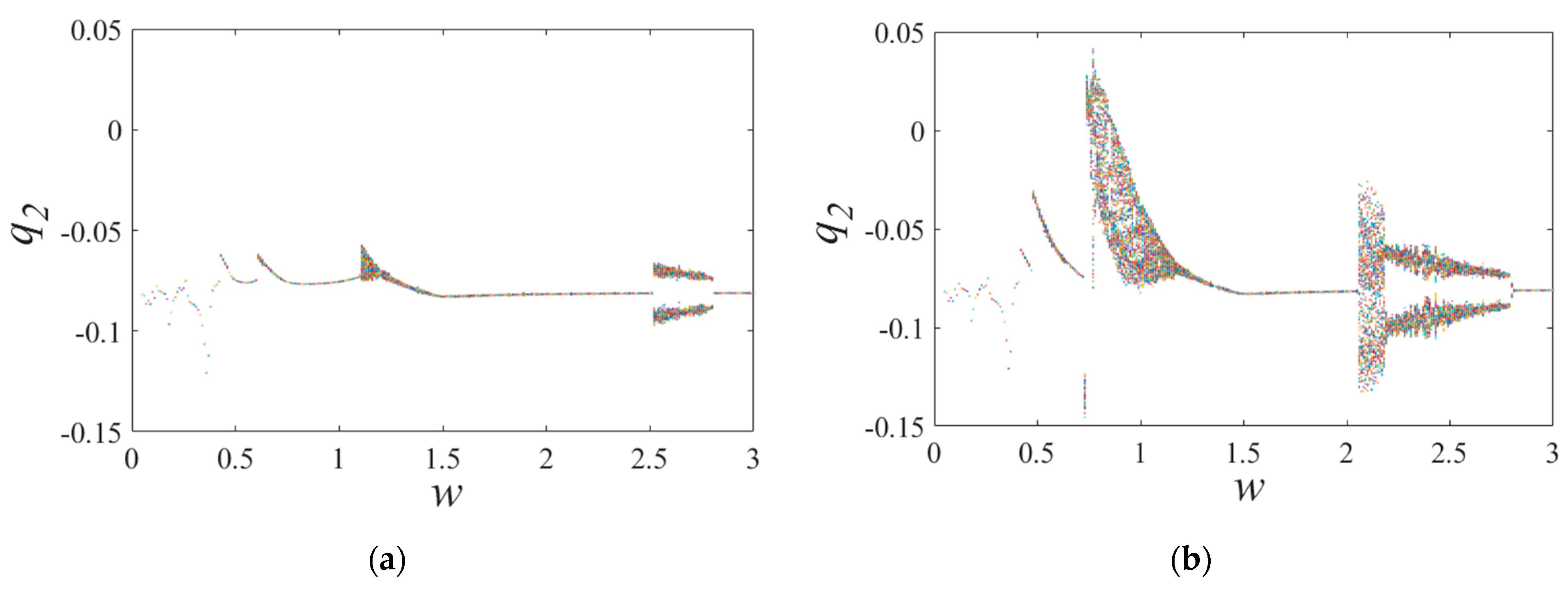
3. Numerical Results and Discussion
3.1. Influence of the Excitation Frequency
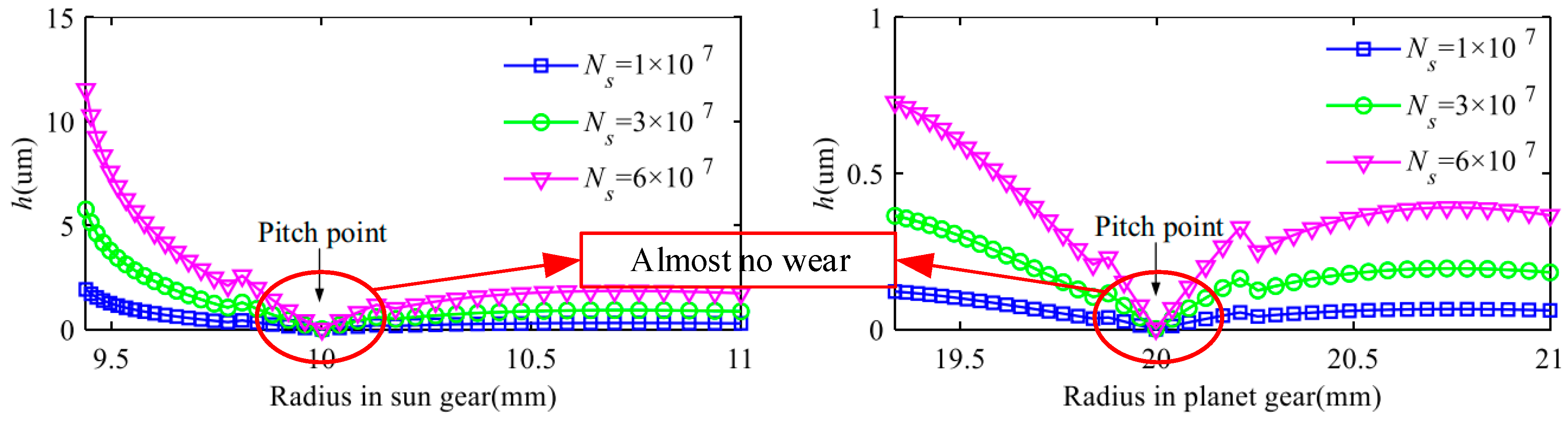
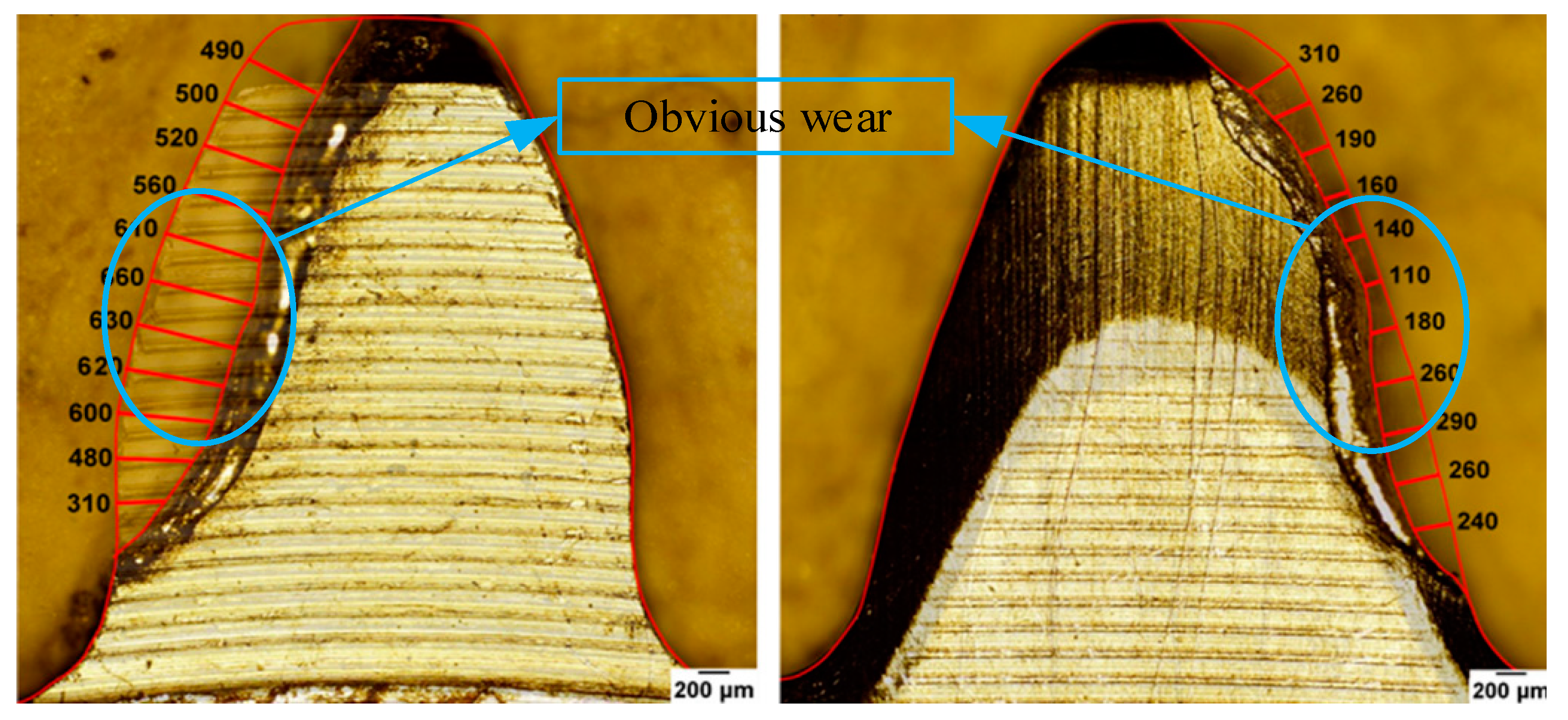
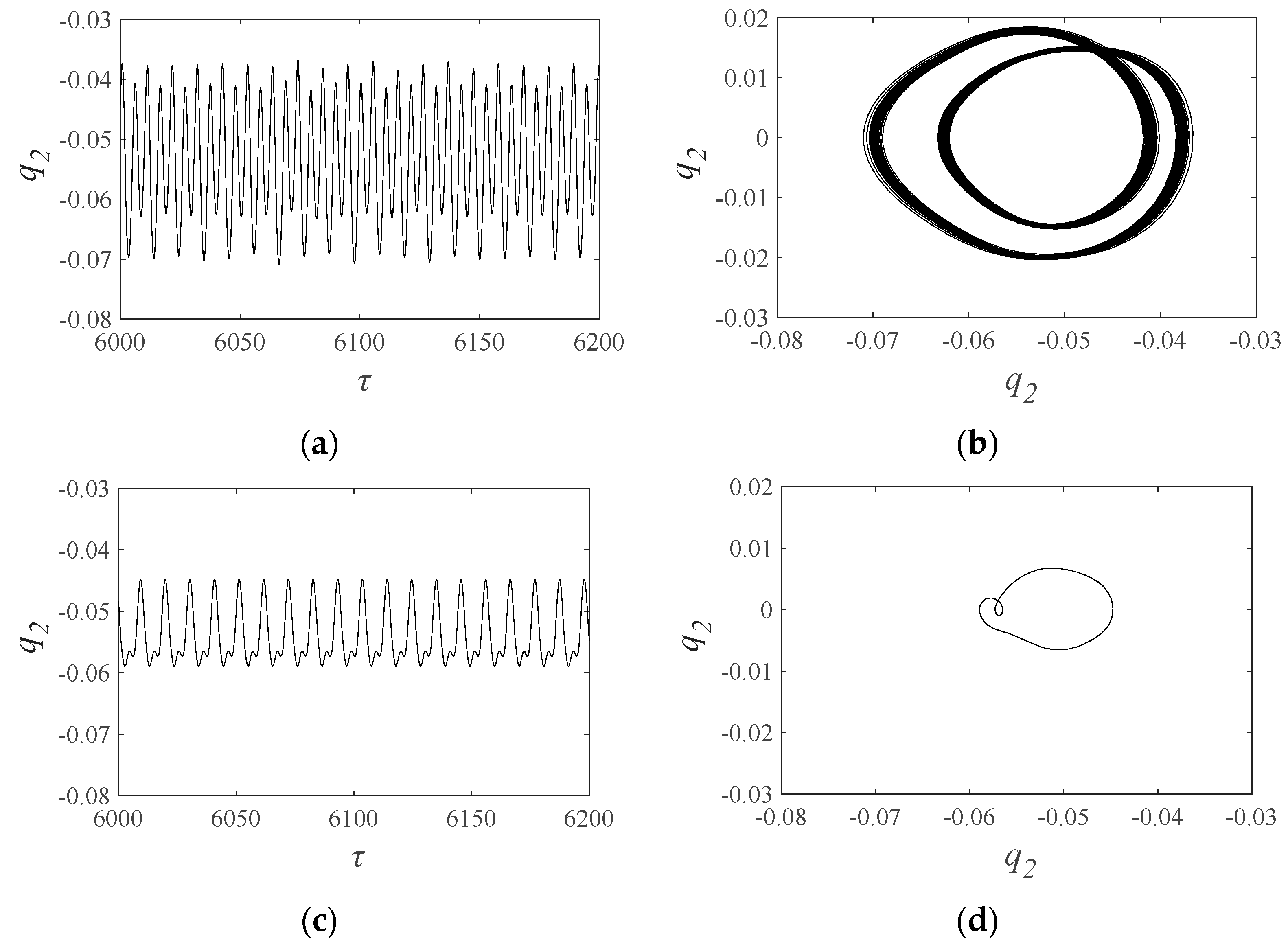
3.2. Influence of the Gear Wear
4. Conclusions
- (1)
- This paper presents a novel nonlinear dynamic model with six degrees of freedom that considers the effects of uniform wear on the contact ratio, meshing stiffness, and gear backlash, with wear volume obtained based on the Archard theory. A significant innovation of this work is its consideration of the change in meshing position with wear.
- (2)
- This paper analyzes the nonlinear dynamic characteristics of a gear pair under conditions of no wear, medium wear, and severe wear, using a range of analysis techniques such as bifurcation diagrams, spectrum waterfall diagrams, Poincaré maps, FFT spectra, phase diagrams, and time series with excitation frequency as a control parameter. The results reveal that the dynamic responses of the gear system differ significantly under different degrees of wear.
- (3)
- When the excitation frequency decreases, the bifurcation characteristics of the gear pair are more complex than those when the excitation frequency increases. When the gear teeth are under the different wear conditions, the bifurcation diagrams present different characteristics as the excitation frequency increases or decreases.
- (4)
- The study revealed that the bifurcation characteristics of the gear pair become more complex as the excitation frequency decreases. This suggests that the nonlinear dynamic behavior of gear systems is highly dependent on the excitation frequency and can exhibit significantly different characteristics at different excitation frequencies. Furthermore, the study demonstrated that the bifurcation diagrams observed under varying degrees of gear wear when the excitation frequency varies also present different characteristics.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kahraman, A. Load sharing characteristics of planetary transmissions. Mech. Mach. Theory 1994, 29, 1151–1165. [Google Scholar] [CrossRef]
- Jian, L.; Parker, R.G. Analytical Characterization of the Unique Properties of Planetary Gear Free Vibration. J. Vib. Acoust.-Trans. ASME 1999, 121, 316–321. [Google Scholar]
- Yu, X.; Sun, Y.; Li, H.; Wu, S. Nonlinear characteristics of gear pair considering fractal surface dynamic contact as internal excitation. Int. J. Non-Linear Mech. 2022, 143, 104027. [Google Scholar] [CrossRef]
- Yi, G.; Parker, R.G. Dynamic Analysis of Planetary Gears With Bearing Clearance. J. Comput. Nonlinear Dyn. 2012, 7, 041002. [Google Scholar]
- Farshidianfar, A.; Saghafi, A. Global bifurcation and chaos analysis in nonlinear vibration of spur gear systems. Nonlinear Dyn. 2014, 75, 783–806. [Google Scholar] [CrossRef]
- Sheng, L.; Li, W.; Wang, Y.; Fan, M.; Yang, X. Nonlinear dynamic analysis and chaos control of multi-freedom semi-direct gear drive system in coal cutters. Mech. Syst. Signal Proc. 2019, 116, 62–77. [Google Scholar] [CrossRef]
- Huang, K.; Cheng, Z.; Xiong, Y.; Han, G.; Li, L. Bifurcation and chaos analysis of a spur gear pair system with fractal gear backlash. Chaos Solitons Fractals 2020, 142, 110387. [Google Scholar] [CrossRef]
- Wang, S.; Zhu, R. Nonlinear dynamic analysis of GTF gearbox under friction excitation with vibration characteristics recognition and control in frequency domain. Mech. Syst. Signal Proc. 2021, 151, 107373. [Google Scholar] [CrossRef]
- Wang, J.; Liu, N.; Wang, H.; Guo, L. Research on bifurcation and chaos characteristics of planet gear transmission system with mixed elastohydrodynamic lubrication (EHL) friction. Int. J. Nonlinear Sci. Num. 2021. [Google Scholar] [CrossRef]
- Tunalioğlu, M.Ş.; Tuç, B. Theoretical and experimental investigation of wear in internal gears. Wear 2014, 309, 208–215. [Google Scholar] [CrossRef]
- Pei, J.; Han, X.; Tao, Y.; Feng, S. Study on Wear Dynamic Reliability of Gear System Based on Markov Diffusive Process. J. Tribol. 2022, 144, 021202. [Google Scholar] [CrossRef]
- Xiang, G.; Yang, T.Y.; Guo, J.; Wang, J.X. Optimization transient wear and contact performances of water-lubricated bearings under fluid-solid-thermal coupling condition using profile modification. Wear 2022, 502–503, 204379. [Google Scholar] [CrossRef]
- Chang, L.; Jeng, Y.R.; Huang, P.Y. Modeling and Analysis of the Meshing Losses of Involute Spur Gears in High-Speed and High-Load Conditions. J. Tribol. 2012, 135, 011504. [Google Scholar] [CrossRef]
- Simon, V.V. Multi-objective optimization of hypoid gears to improve operating characteristics. Mech. Mach. Theory 2020, 146, 103727. [Google Scholar] [CrossRef]
- Li, X.; Xu, J.; Yang, Z.; Chen, R.; Yang, H. The influence of tooth surface wear on dynamic characteristics of gear-bearing system based on fractal theory. J. Comput. Nonlinear Dyn. 2020, 15, 041004. [Google Scholar] [CrossRef]
- Tang, D.X.; Xiang, G.; Guo, J. On the optimal design of staved water-lubricated bearings driven by tribo-dynamic mechanism. Phys. Fluids 2023, 35, 093611. [Google Scholar] [CrossRef]
- Meng, F.S.; Xia, H.; Zhang, X.; Wang, J.X. A new tooth pitting modeling method based on matrix equation for evaluating time-varying mesh stiffness. Eng. Fail. Anal. 2022, 142, 106799. [Google Scholar] [CrossRef]
- Li, B.; Li, P.; Zhou, R.; Feng, X.; Zhou, K. Contact mechanics in tribological and contact damage-related problems: A review. Tribol. Int. 2022, 171, 107534. [Google Scholar] [CrossRef]
- Saini, V.; Maurya, U.; Thakre, G.D. Estimating the Dry-Wear Behavior of Rolling/Sliding Bearings (PB, Gunmetal, and Al6061)–Tribo Materials. J. Fail. Anal. Prev. 2023, 23, 2439–2451. [Google Scholar] [CrossRef]
- Zhang, B.; Liu, H.; Zhu, C.; Ge, Y. Simulation of the fatigue-wear coupling mechanism of an aviation gear. Friction 2021, 9, 1616–1634. [Google Scholar] [CrossRef]
- Yuksel, C.; Kahraman, A. Dynamic tooth loads of planetary gear sets having tooth profile wear. Mech. Mach. Theory 2004, 39, 695–715. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Shen, Z.; Qiao, B.; Yang, L.; Lou, W.; Yang, Z. Fault mechanism and dynamic modeling of planetary gear with gear wear. Mech. Mach. Theory 2021, 155, 104098. [Google Scholar] [CrossRef]
- Zhan, Y.; Smith, W.; Borghesani, P.; Randall, R.; Peng, Z.X. Absolute transmission error: A simple new tool for assessing gear wear. Mech. Syst. Signal Proc. 2021, 146, 107070. [Google Scholar]
- Zhang, X.; Zhong, J.; Li, W.; Mateusz, B. Nonlinear dynamic analysis of high-speed gear pair with wear fault and tooth contact temperature for a wind turbine gearbox. Mech. Mach. Theory 2022, 173, 104840. [Google Scholar] [CrossRef]
- Koffi, D.; Kassegne, K.A.; Wotodzo, K.F.; Bedja, K. Modeling and Prediction of Mechanical Behavior of Plastic Gears in Simulated Wear Situation. Solid State Phenom. 2012, 188, 232–237. [Google Scholar] [CrossRef]
- Feng, S.; Mao, J.; Xie, Y. Analysis and Calculation of Gear Mesh Stiffness with Tooth Wear. Chin. J. Mech. Eng. 2015, 51, 27–32. [Google Scholar] [CrossRef]
- Zhou, C.; Wang, H. An adhesive wear prediction method for double helical gears based on enhanced coordinate transformation and generalized sliding distance model. Mech. Mach. Theory 2018, 128, 58–83. [Google Scholar] [CrossRef]
- Geng, Z.; Xiao, K.; Wang, J.; Li, J. Investigation on Nonlinear Dynamic Characteristics of a New Rigid–Flexible Gear Transmission with Wear. J. Vib. Acoust.-Trans. ASME 2019, 14, 051008. [Google Scholar] [CrossRef]
- Sheng, L.; Li, W.; Ye, G.; Feng, K. Nonlinear dynamic analysis of gear system in shearer cutting section under wear failure. Proc. Inst. Mech. Eng. K J. Multi-Body Dyn. 2022, 236, 99–112. [Google Scholar] [CrossRef]
- Geng, Z.; Xiao, K.; Li, J.; Wang, J. Bifurcation and Chaos of a Spur Gear Transmission System With Non-Uniform Wear. J. Vib. Acoust.-Trans. 2020, 143, 031004. [Google Scholar] [CrossRef]
- Chen, Z.; Zhou, Z.; Zhai, W.; Wang, K. Improved analytical calculation model of spur gear mesh excitations with tooth profile deviations. Mech. Mach. Theory 2019, 149, 103838. [Google Scholar] [CrossRef]
- Cao, Z.; Shao, Y.; Rao, M.; Yu, W.N. Effects of the gear eccentricities on the dynamic performance of a planetary gear set. Nonlinear Dyn. 2018, 91, 1–15. [Google Scholar] [CrossRef]
- Xie, C.Y.; Hua, L.; Han, X.H.; Lan, J.; Wan, X.J.; Xiong, X.S. Analytical formulas for gear body-induced tooth deflections of spur gears considering structure coupling effect. Int. J. Mech. Sci. 2018, 148, 174–190. [Google Scholar] [CrossRef]





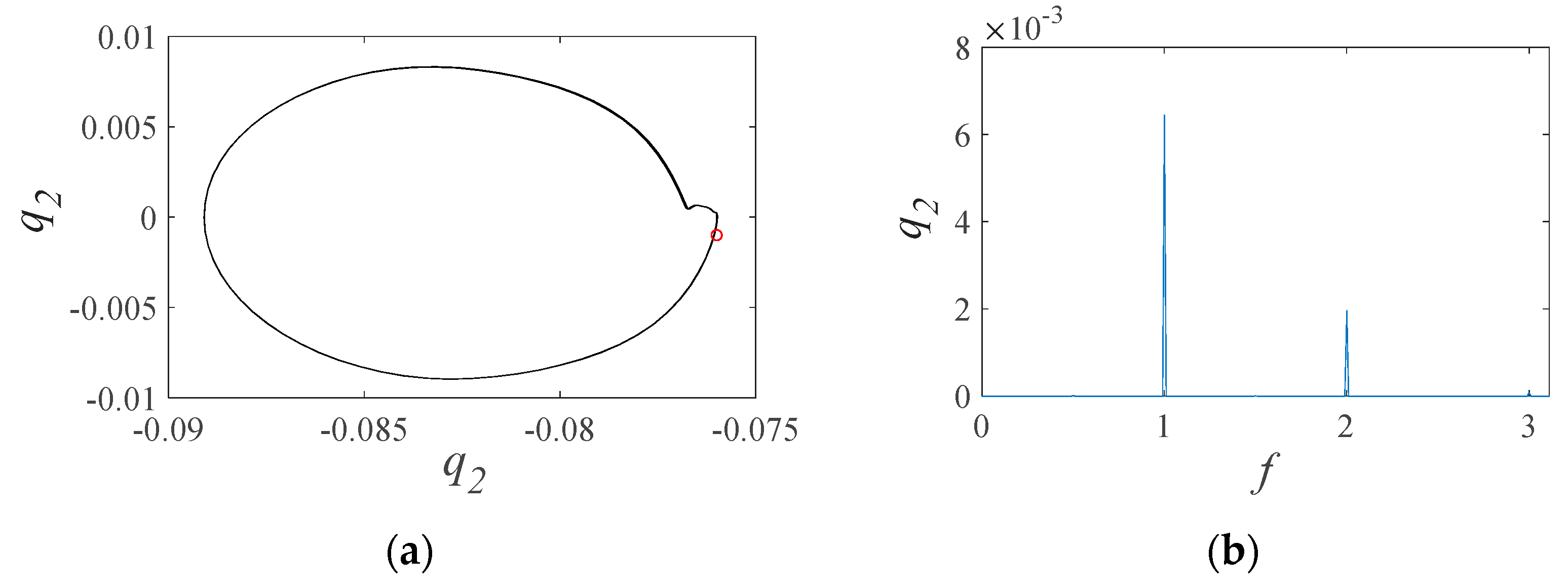






| Driving Gear | Driven Gear | |
|---|---|---|
| Number of teeth | 27 | 55 |
| Modulus (mm) | 2.5 | |
| Pressure angle | 20° | |
| Mass (kg) | 0.584 | 2.007 |
| Diameter of the shaft bore (mm) | 35 | 35 |
| Rotation inertia (kg/m2) | 0.00043 | 0.005487 |
| Contact ratio | 1.7002 | |
| Initial Gear backlash (μm) | 50 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Geng, Z.; Chen, M.; Wang, J.; Xia, Y.; Kong, Y.; Xiao, K. Nonlinear Dynamic Analysis of a Spur Gear Pair System with Wear Considering the Meshing Position. Lubricants 2024, 12, 25. https://doi.org/10.3390/lubricants12010025
Geng Z, Chen M, Wang J, Xia Y, Kong Y, Xiao K. Nonlinear Dynamic Analysis of a Spur Gear Pair System with Wear Considering the Meshing Position. Lubricants. 2024; 12(1):25. https://doi.org/10.3390/lubricants12010025
Chicago/Turabian StyleGeng, Zhibo, Min Chen, Jiao Wang, Yu Xia, Yun Kong, and Ke Xiao. 2024. "Nonlinear Dynamic Analysis of a Spur Gear Pair System with Wear Considering the Meshing Position" Lubricants 12, no. 1: 25. https://doi.org/10.3390/lubricants12010025
APA StyleGeng, Z., Chen, M., Wang, J., Xia, Y., Kong, Y., & Xiao, K. (2024). Nonlinear Dynamic Analysis of a Spur Gear Pair System with Wear Considering the Meshing Position. Lubricants, 12(1), 25. https://doi.org/10.3390/lubricants12010025






