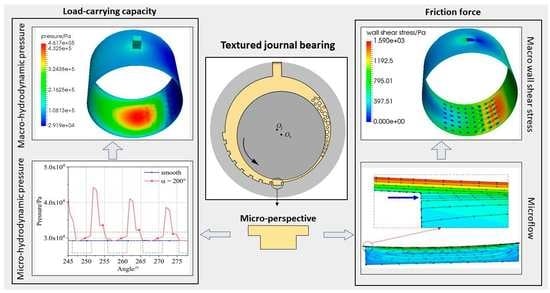
Investigation of Microflow Effects in Textures on Hydrodynamic Performance of Journal Bearings Using CFD Simulations
Abstract
1. Introduction
2. Numerical Method
2.1. Governing Equations
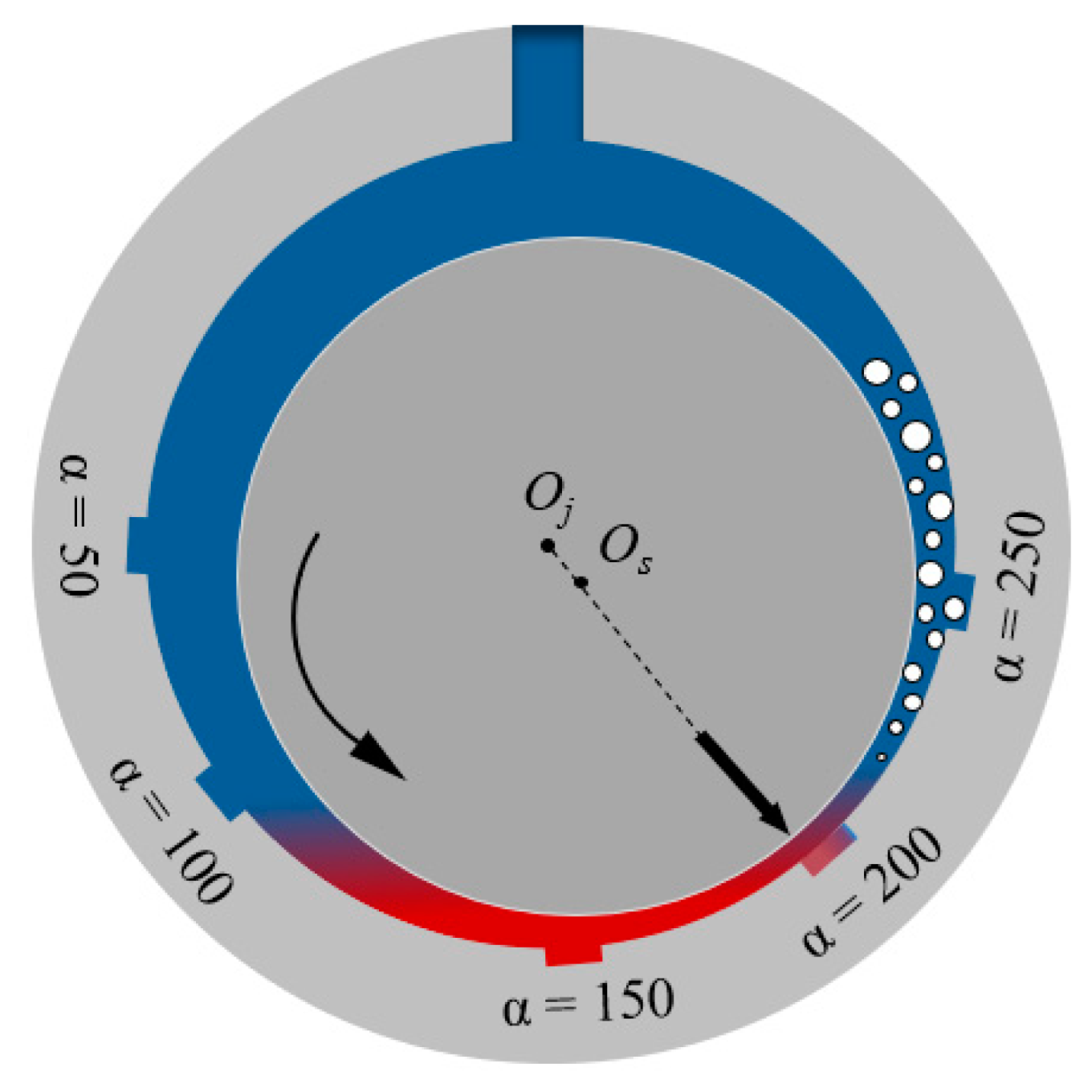
2.2. Physical Model
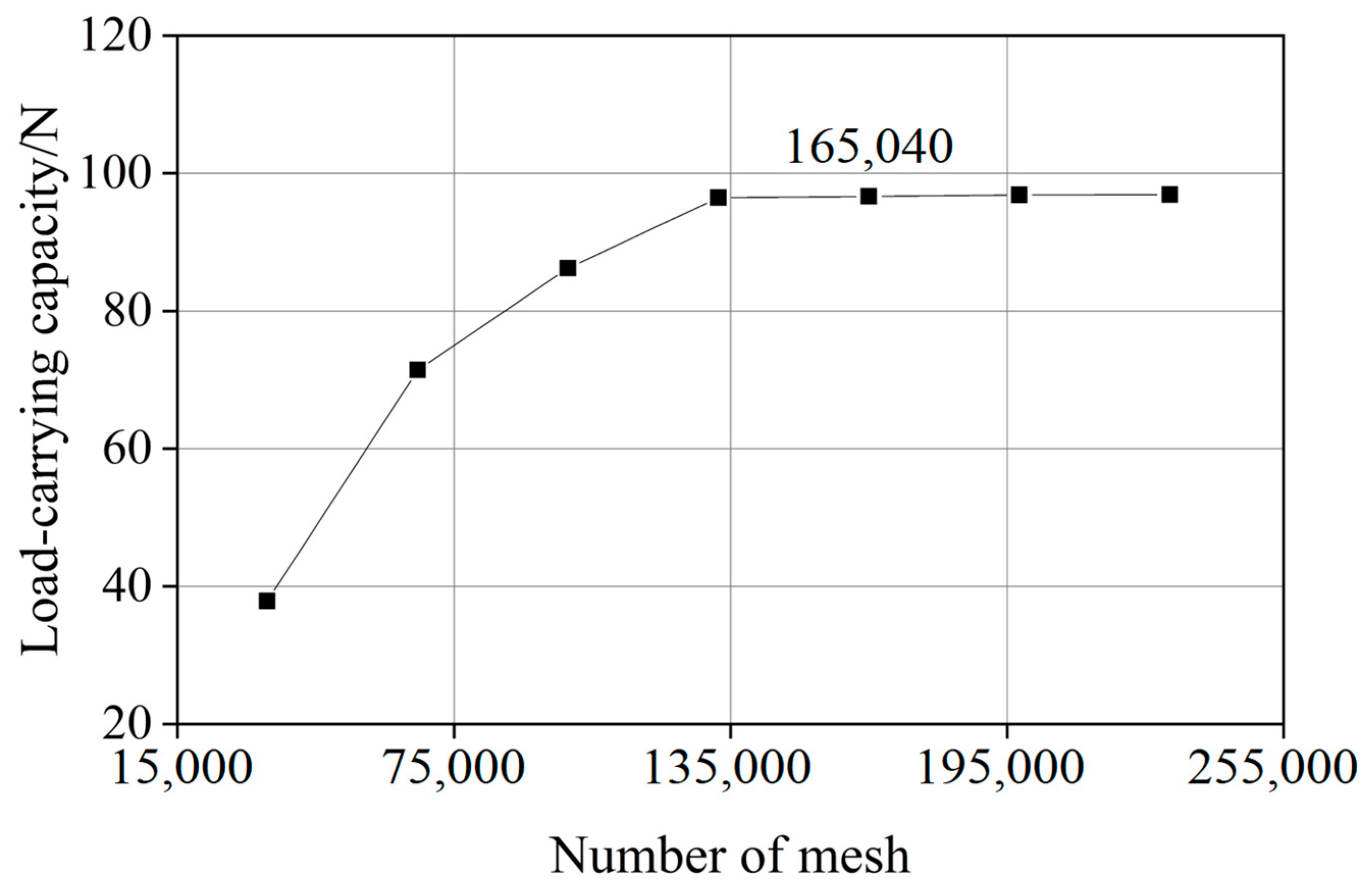
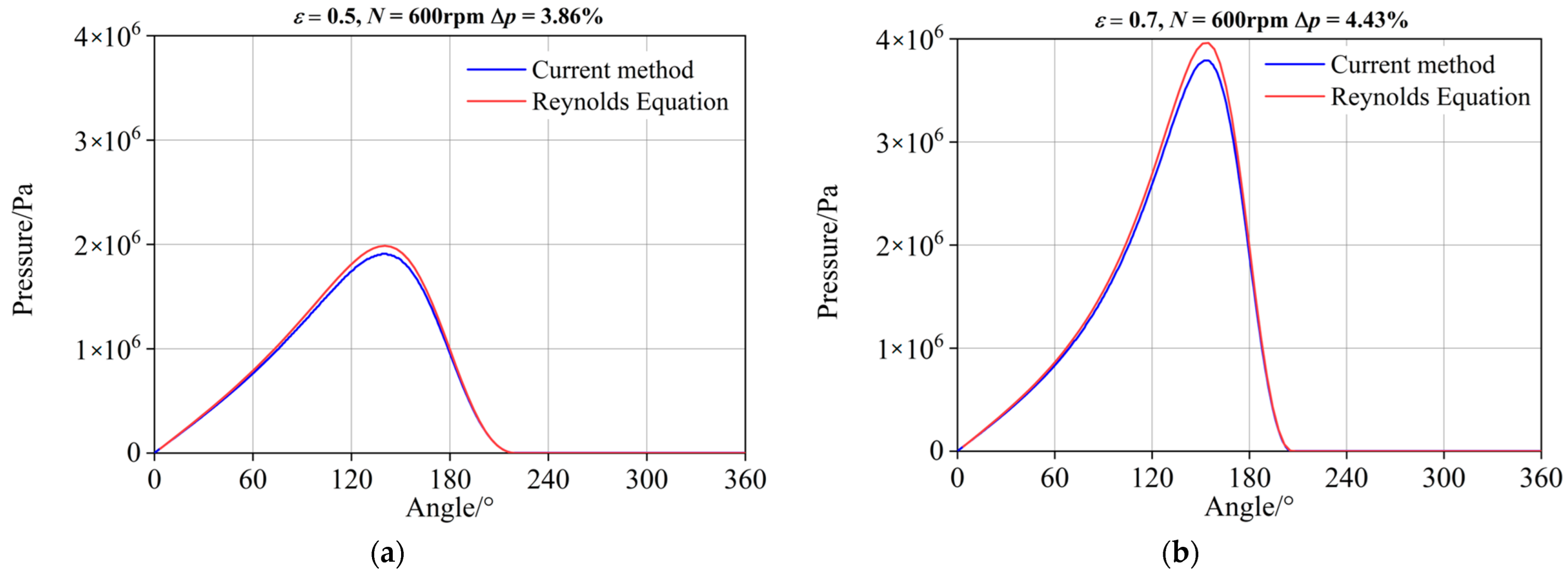
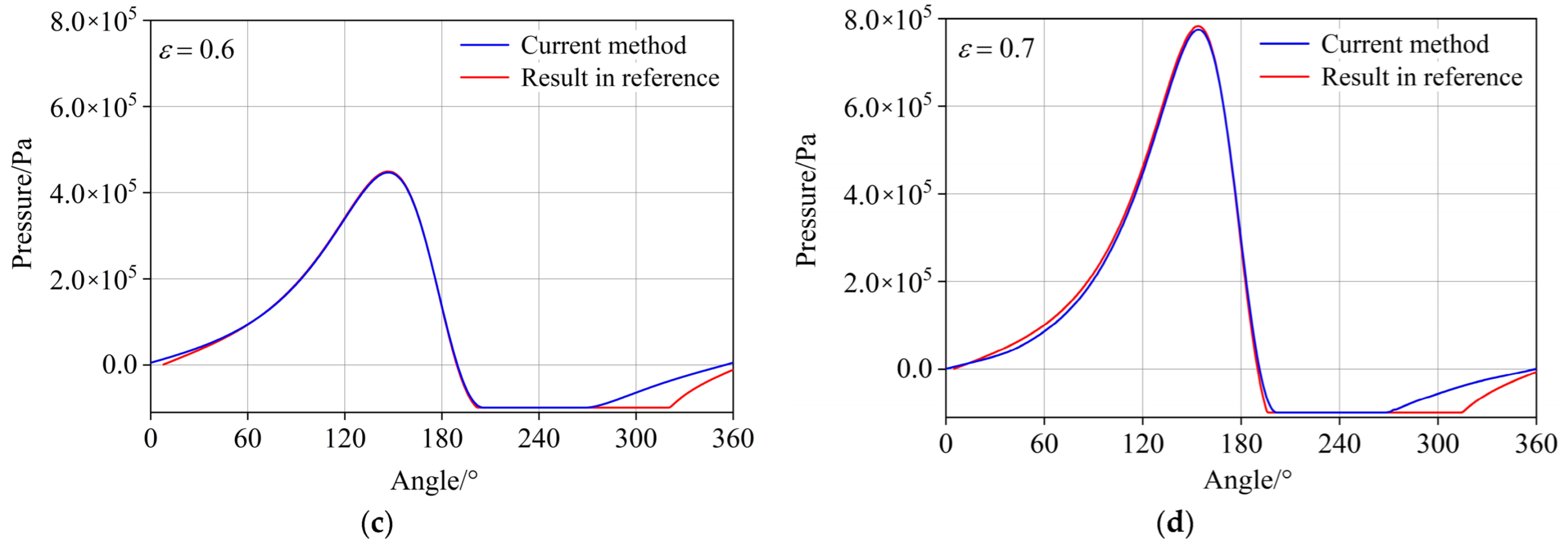
2.3. Validation
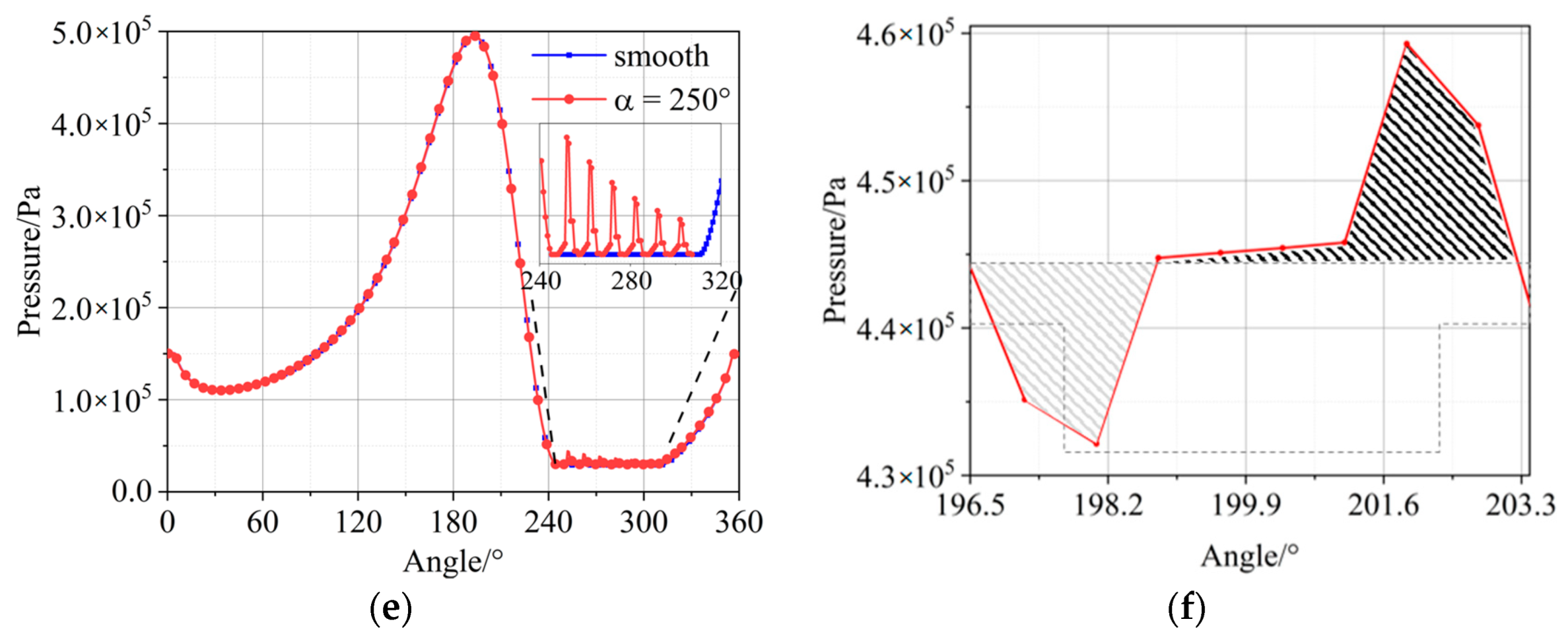
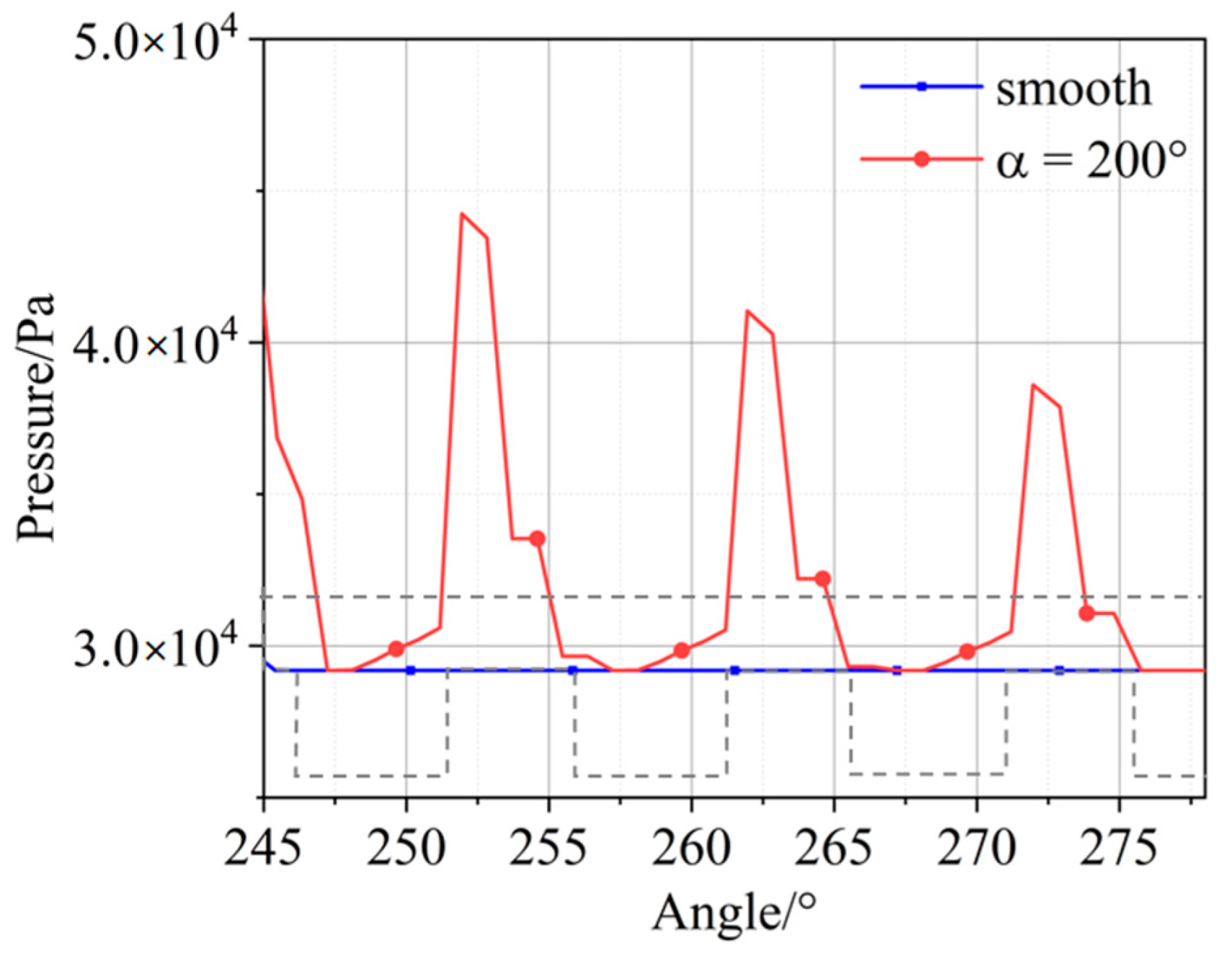
3. Results and Discussion
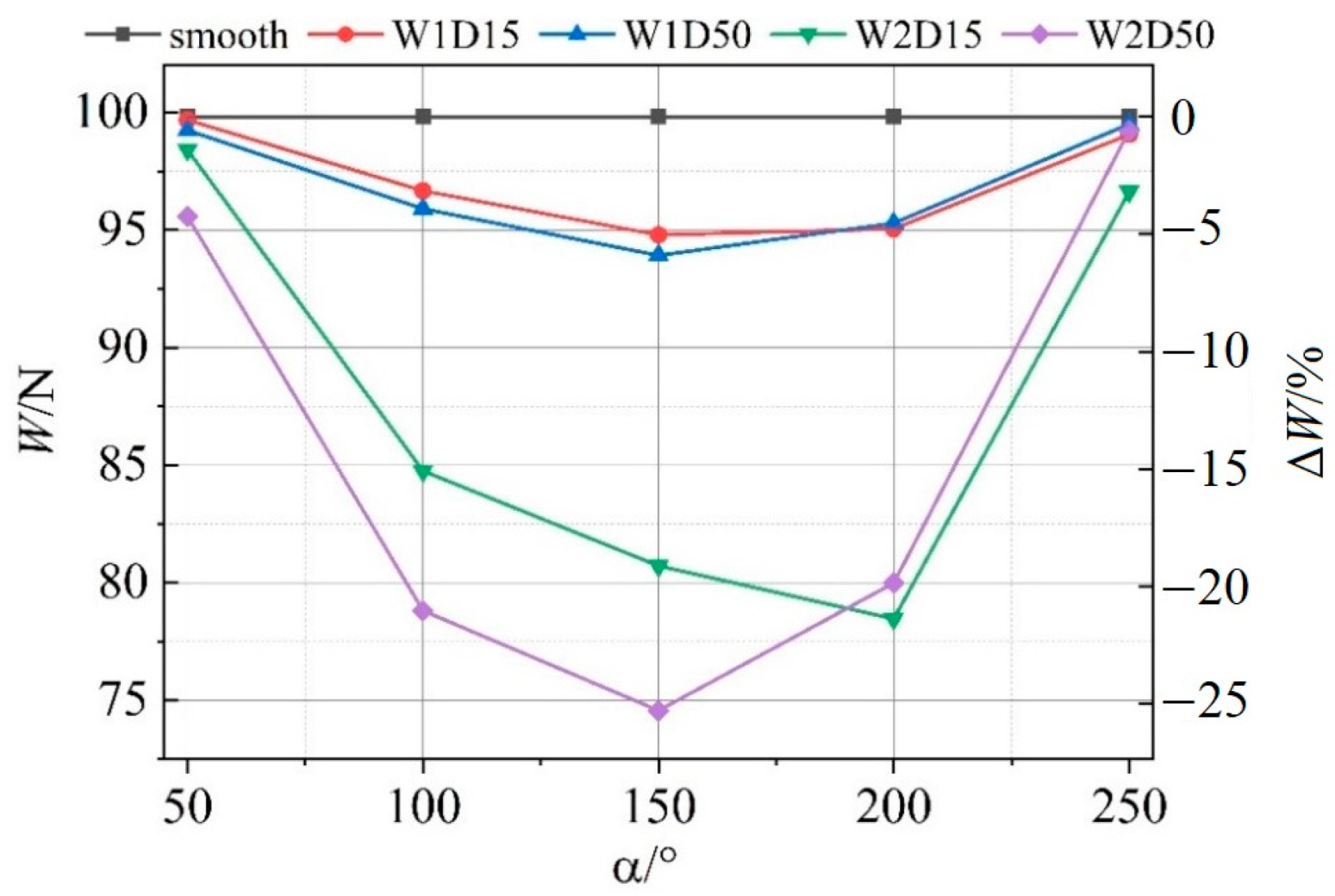
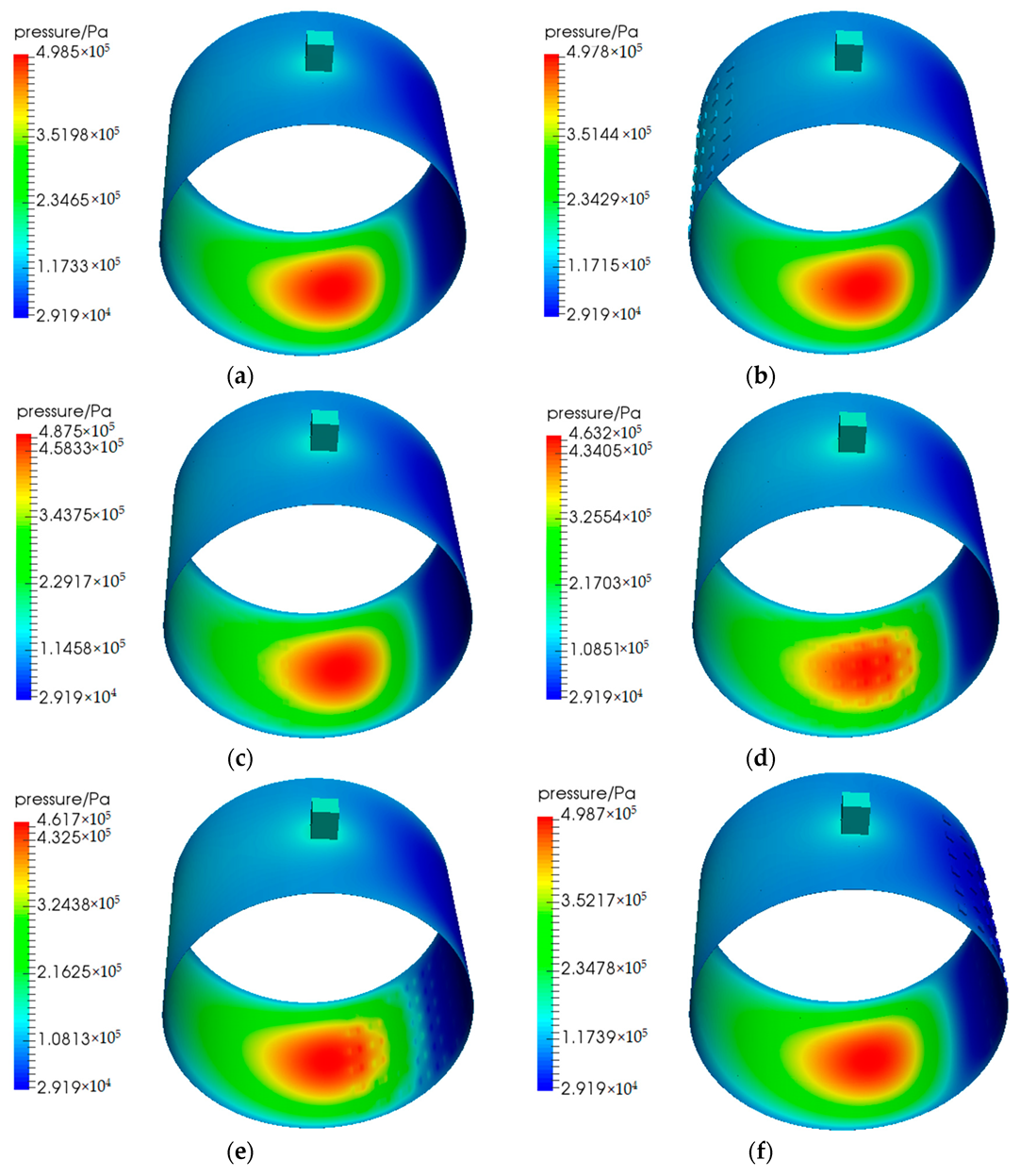
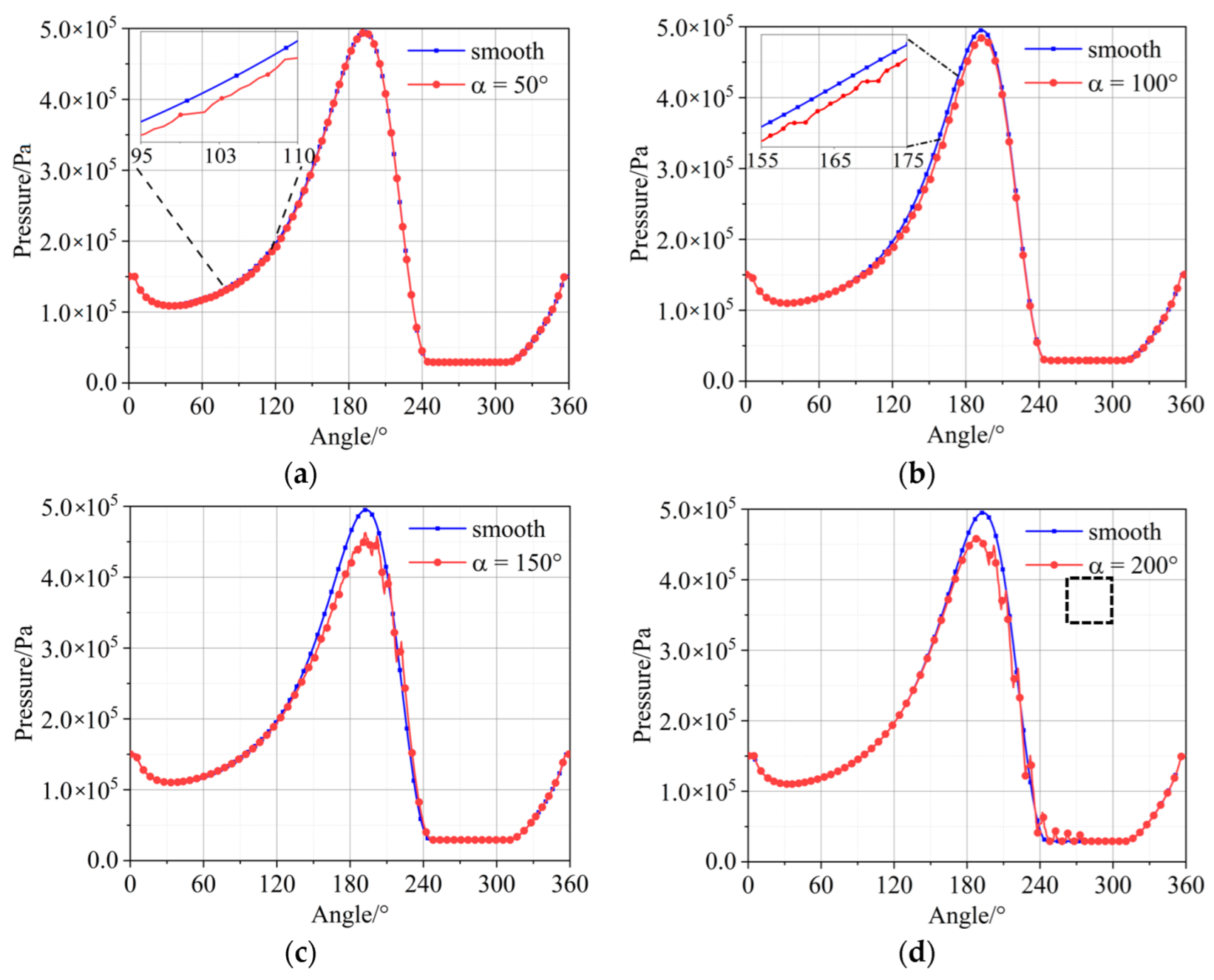
3.1. Effect of Textures on the Load-Carrying Capacity
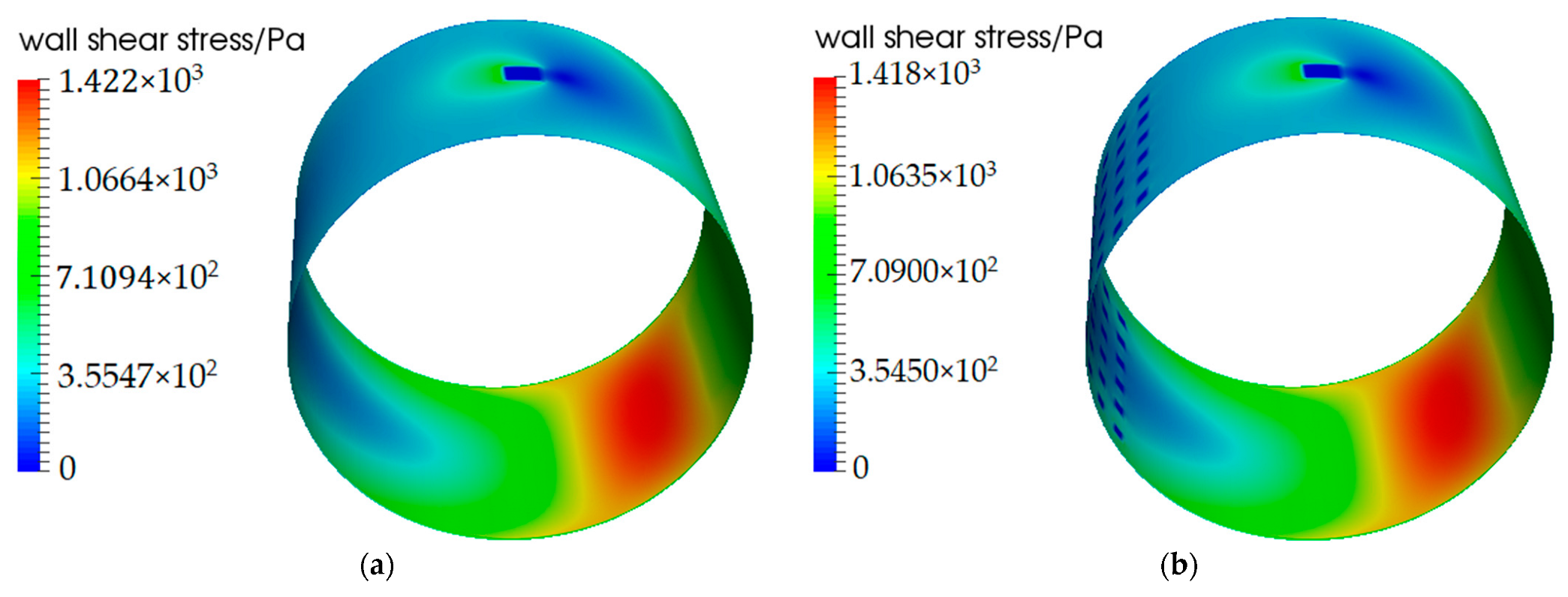
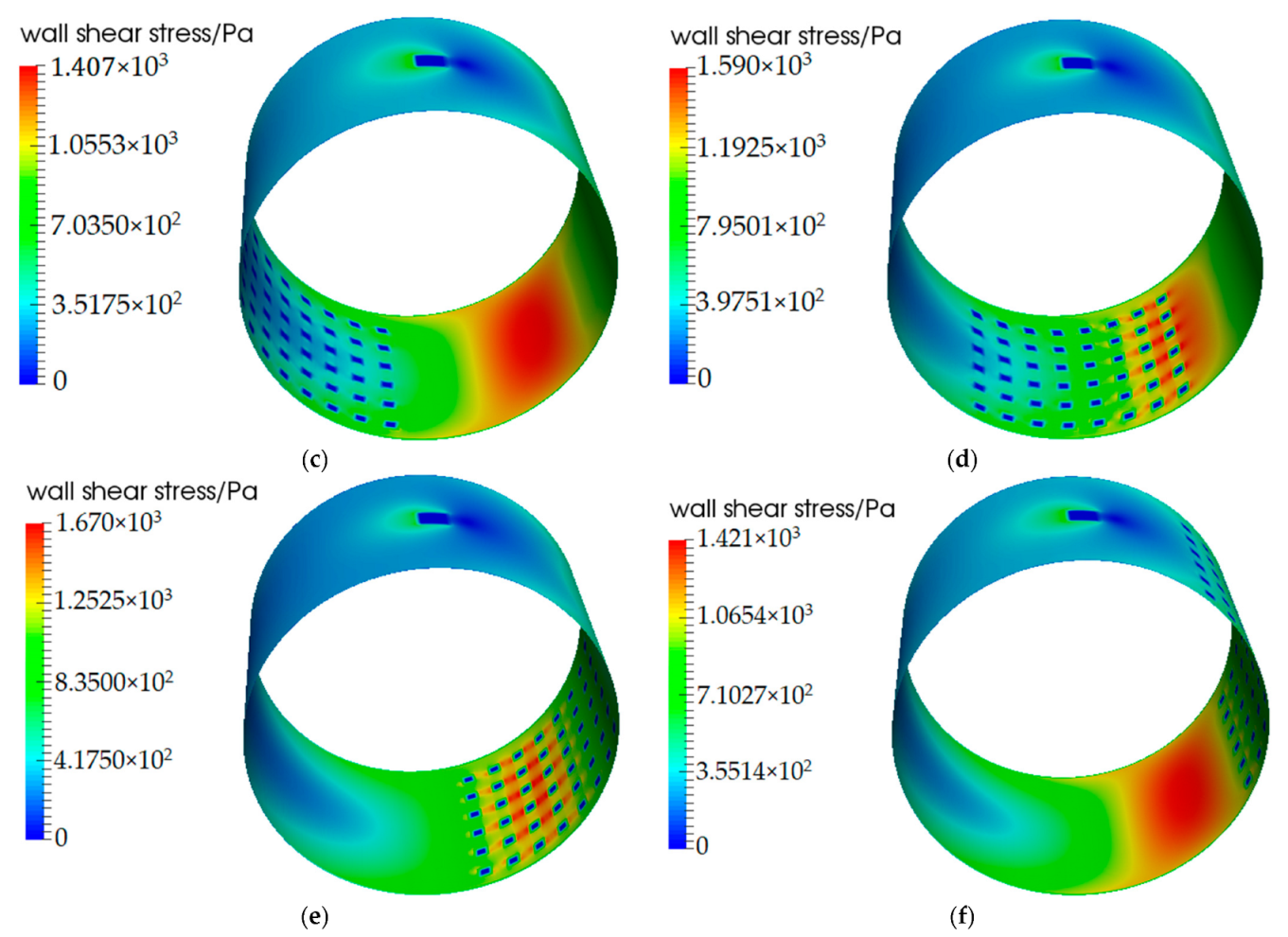
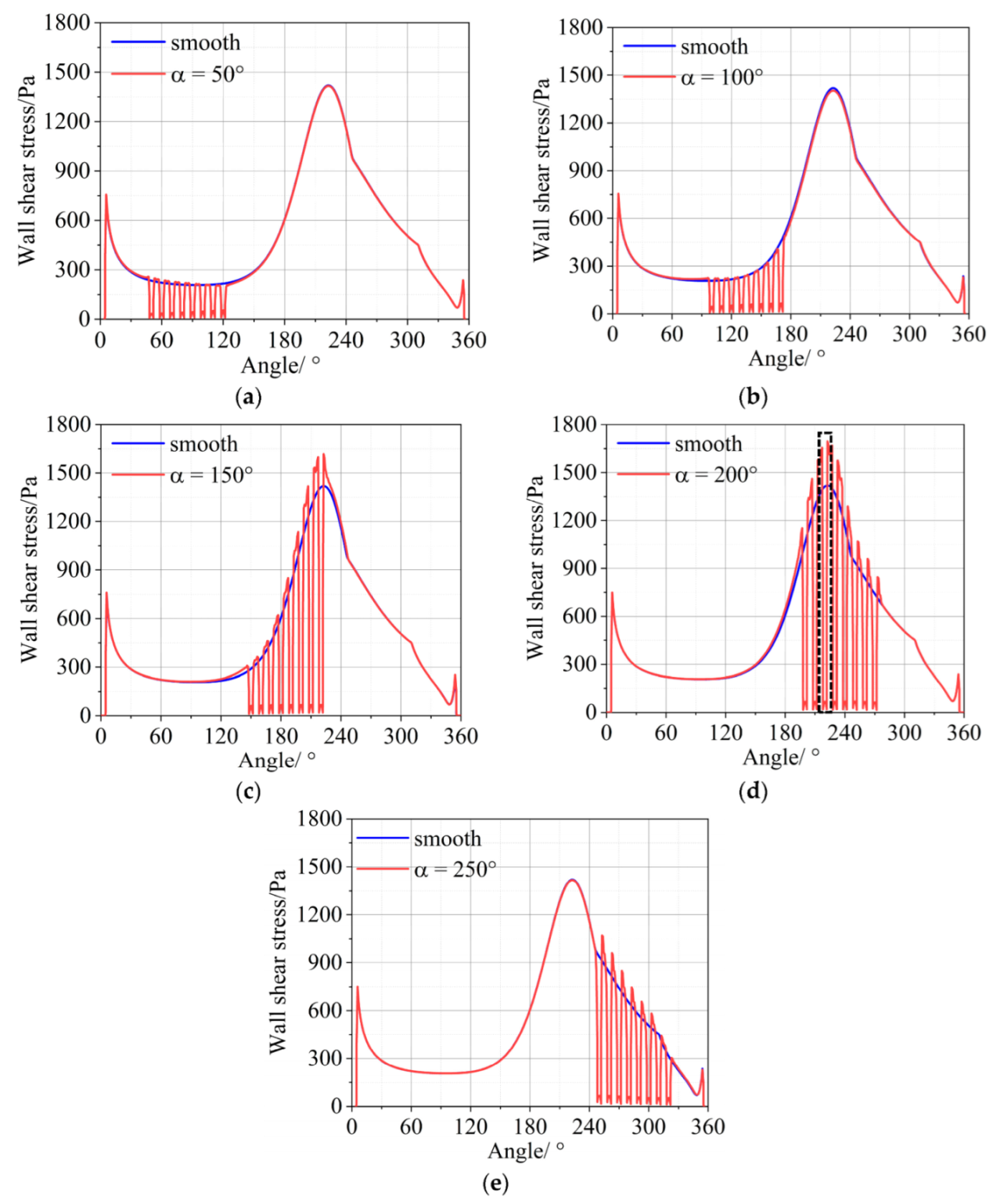
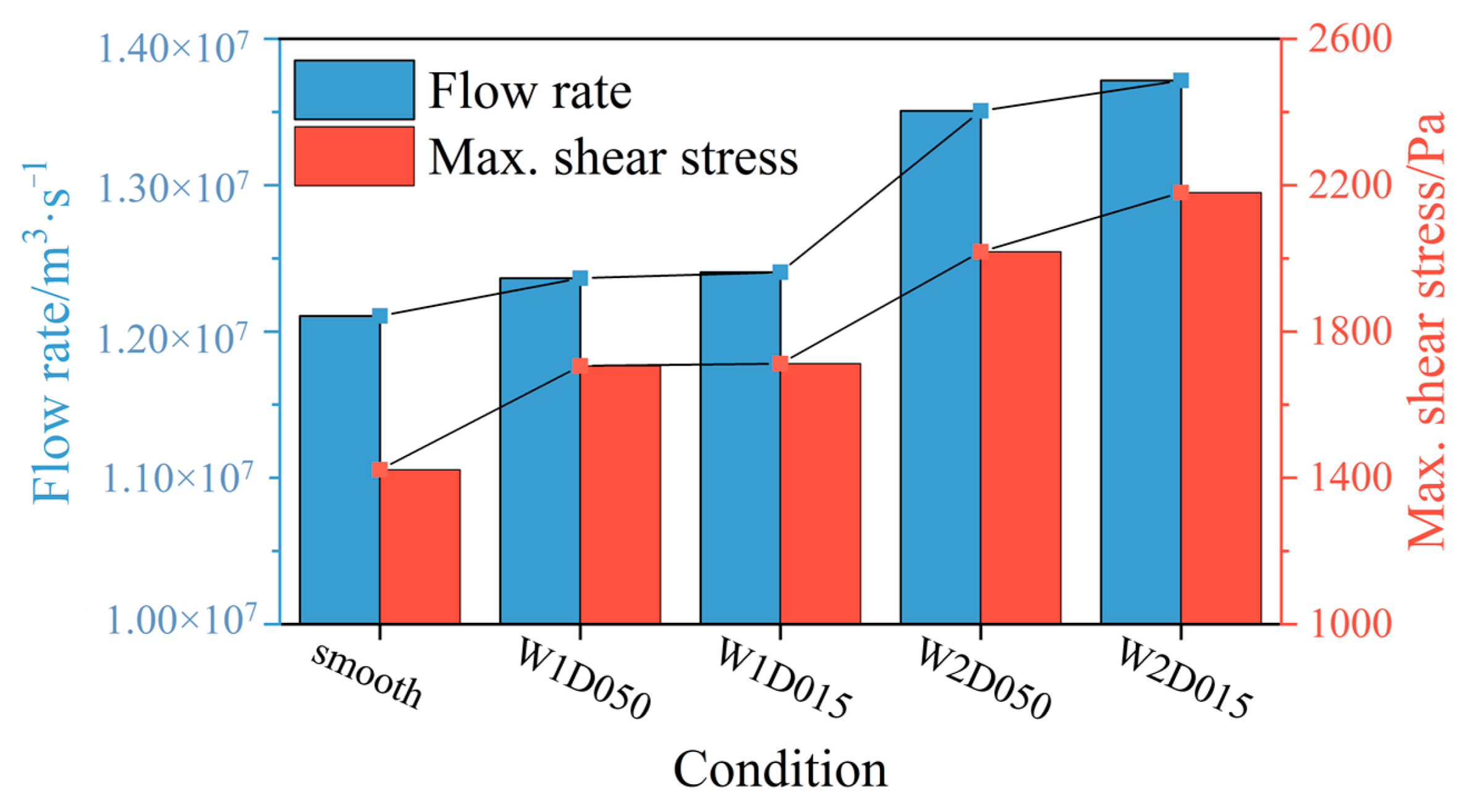
3.2. Effect of Textures on the Friction Force
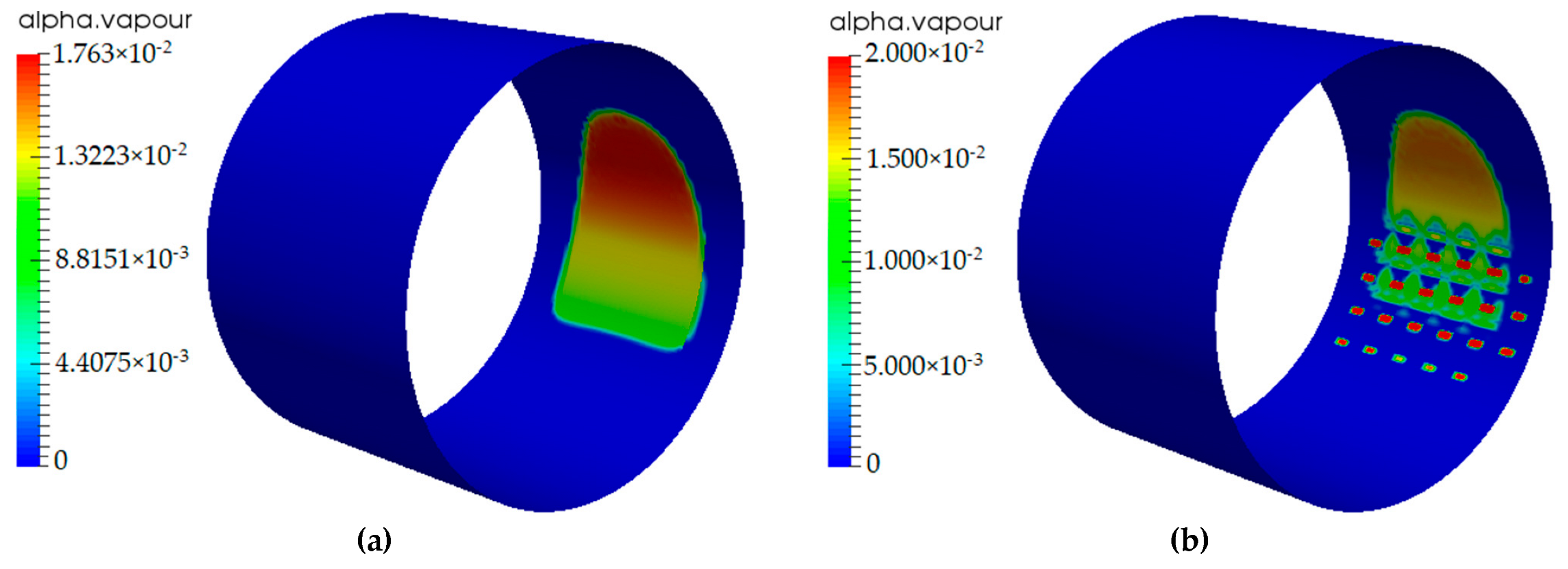
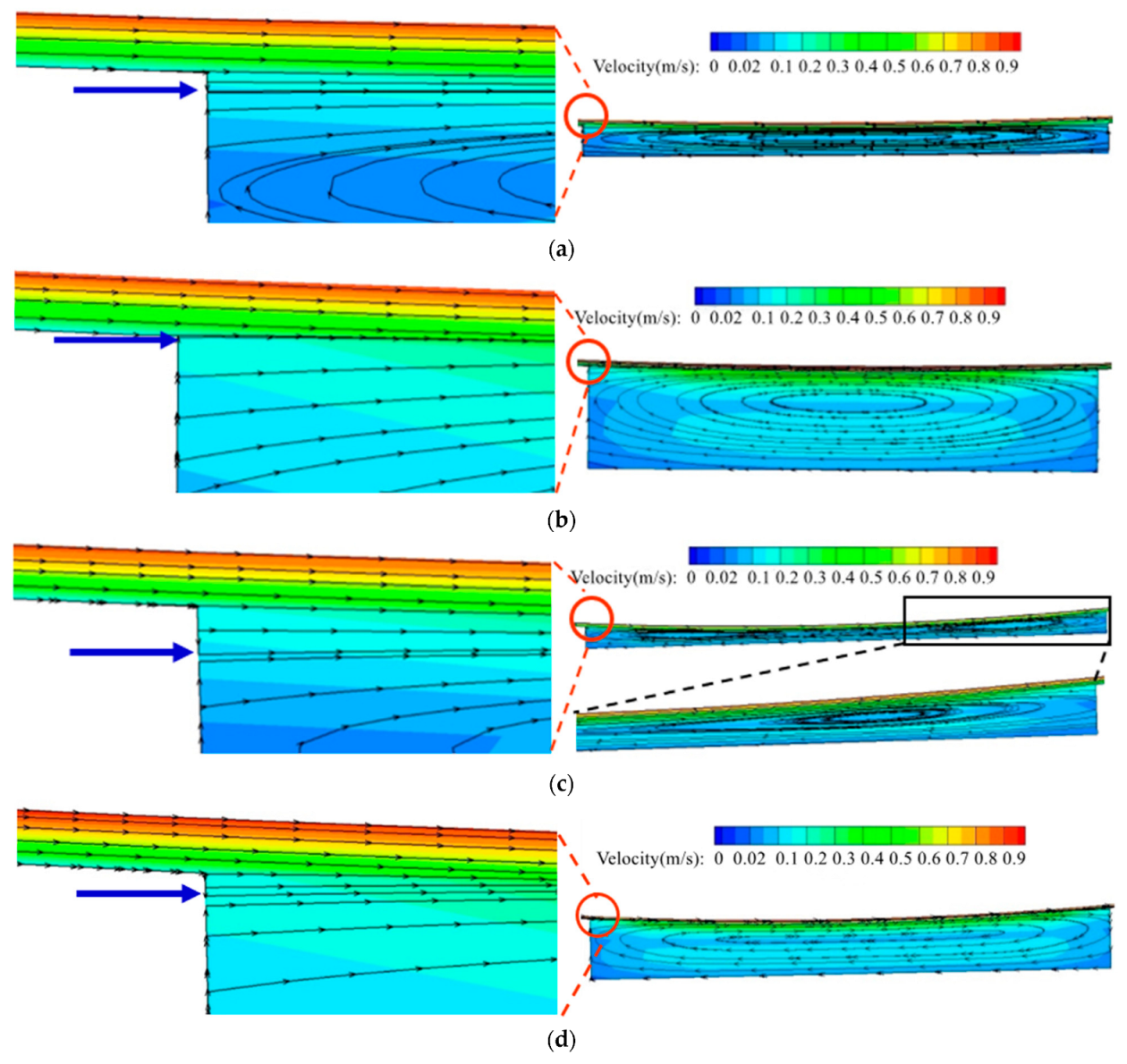
3.3. Effect of Lubrication Film on the Microflow in Textures
4. Conclusions
- (1)
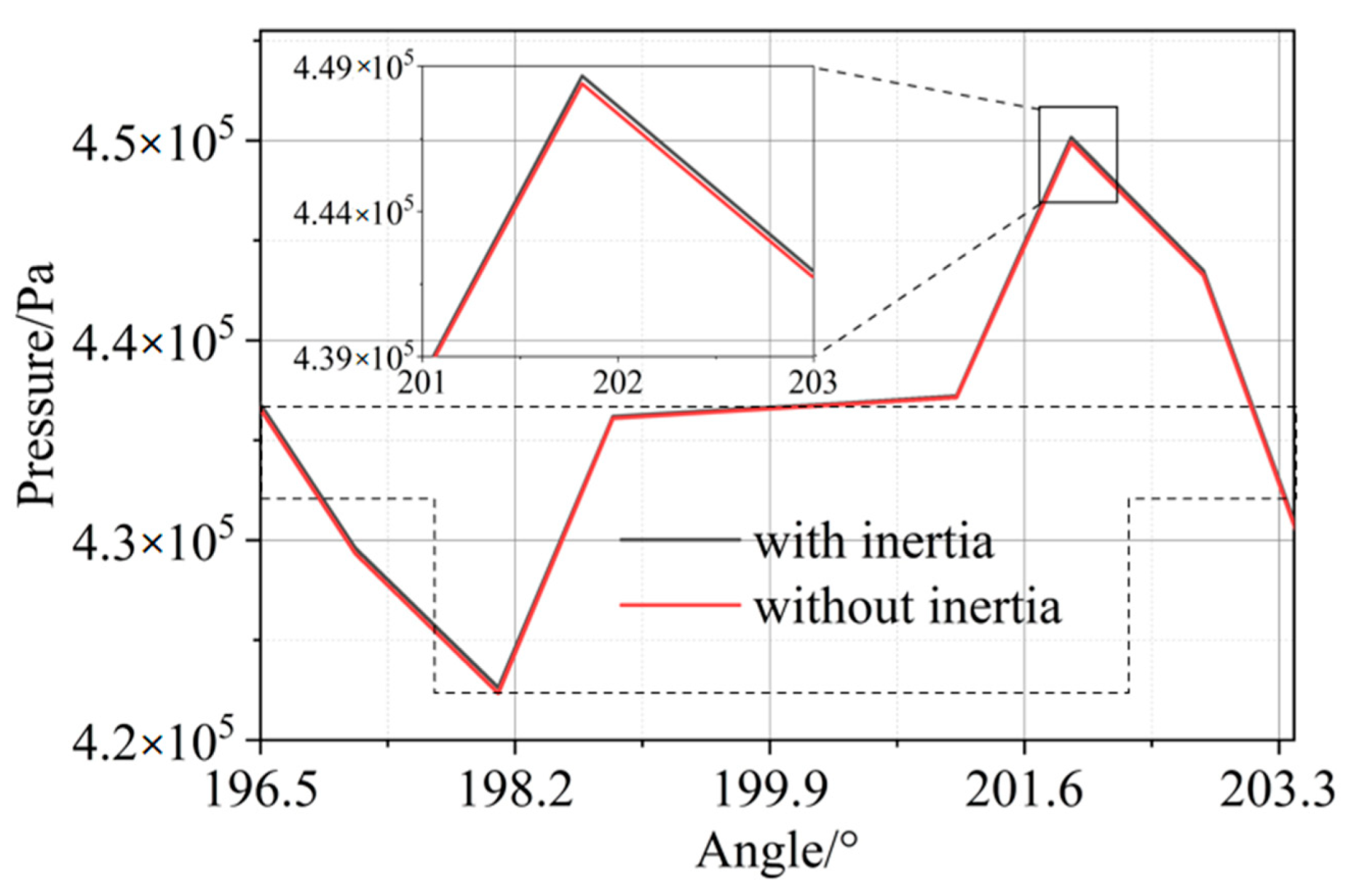
- The micro-hydrodynamic pressure of textures can be generated on one hand by the limiting pressure drop (cavitation) in the divergent gap and on the other hand by the inertia effect. Meanwhile, the increase of film thickness in textured zone disrupts the continuity of original high-pressure region of journal bearings. In the current work, the positive micro-hydrodynamic pressure effect on the load-carrying capacity is less than the negative effect caused by the discontinuity of high-pressure region, thereby the load-carrying capacity decreases by 0.3% to 25.3%.
- (2)
- Textures can significantly reduce the wall shear stress. This effect can be explained by the increased film thickness, which is most dominant when the textures are located in the region of minimum film thickness. However, it has a negative effect on the load-carrying capacity. In contrast, placing textures downstream of the high-pressure region leads to a reduced friction force, with a less severe loss of load-carrying capacity.
- (3)
- The vortex inside the textures will influence the inflow area of textures and further affect the maximum shear stress at the minimum oil film. The vortex center inside the texture moves away from the high-pressure region and the textures in the high-pressure region and the minimum oil film region have a larger lubricant inflow area.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Balakrishnan, S.; Baker, C.E.; Rahnejat, H. Fundamentals of hydrodynamic journal bearings: An analytical approach. In Tribology and Dynamics of Engine and Powertrain; Woodhead Publishing: Cambridge, UK, 2010; pp. 591–614. [Google Scholar]
- Bouyer, J.; Alexandre, Y.; Fillon, M. Experimental investigation on the influence of a multi-scratched shaft on hydrodynamic journal bearing performance. Tribol. Int. 2021, 153, 106543. [Google Scholar] [CrossRef]
- Xiang, G.; Yang, T.; Guo, J.; Wang, J.; Liu, B.; Chen, S. Optimization transient wear and contact performances of water-lubricated bearings under fluid-solid-thermal coupling condition using profile modification. Wear 2022, 502–503, 204379. [Google Scholar] [CrossRef]
- König, F.; Sous, C.; Jacobs, G. Numerical prediction of the frictional losses in sliding bearings during start-stop operation. Friction 2021, 9, 583–597. [Google Scholar] [CrossRef]
- Xie, Z.; Jiao, J.; Yang, K. Theoretical and experimental study on the fluid-structure-acoustic coupling dynamics of a new water lubricated bearing. Tribol. Int. 2023, 177, 107982. [Google Scholar] [CrossRef]
- Rasep, Z.; Muhammad Yazid, M.; Samion, S. Lubrication of textured journal bearing by using vegetable oil: A review of approaches, challenges, and opportunities. Renew. Sustain. Energy Rev. 2021, 146, 111191. [Google Scholar] [CrossRef]
- Rom, M.; König, F.; Müller, S.; Jacobs, G. Why homogenization should be the averaging method of choice in hydrodynamic lubrication. Appl. Eng. Sci. 2021, 7, 100055. [Google Scholar] [CrossRef]
- Grabon, W.; Koszela, W.; Pawlus, P.; Ochwat, S. Improving tribological behaviour of piston ring–cylinder liner frictional pair by liner surface texturing. Tribol. Int. 2013, 61, 102–108. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Y.; Zhang, S.; Xu, W.; Bai, L.; Wang, Z. Investigation and optimization of textured thrust bearings with spirally distributed dimples using multi-objective optimization method. ILT 2020, 72, 749–759. [Google Scholar] [CrossRef]
- Rosenkranz, A.; Heib, T.; Gachot, C.; Mücklich, F. Oil film lifetime and wear particle analysis of laser-patterned stainless steel surfaces. Wear 2015, 334–335, 1–12. [Google Scholar] [CrossRef]
- Yamakiri, H.; Sasaki, S.; Kurita, T.; Kasashima, N. Effects of laser surface texturing on friction behavior of silicon nitride under lubrication with water. Tribol. Int. 2011, 44, 579–584. [Google Scholar] [CrossRef]
- Brunetière, N.; Tournerie, B. Numerical analysis of a surface-textured mechanical seal operating in mixed lubrication regime. Tribol. Int. 2012, 49, 80–89. [Google Scholar] [CrossRef]
- Zhang, H.; Hua, M.; Dong, G.; Zhang, D.; Chin, K.-S. A mixed lubrication model for studying tribological behaviors of surface texturing. Tribol. Int. 2016, 93, 583–592. [Google Scholar] [CrossRef]
- Imai, N.; Kato, T. Effects of texture patterns on hydrodynamic and mixed lubrication characteristics. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2013, 227, 898–904. [Google Scholar] [CrossRef]
- König, F.; Rosenkranz, A.; Grützmacher, P.G.; Mücklich, F.; Jacobs, G. Effect of single- and multi-scale surface patterns on the frictional performance of journal bearings—A numerical study. Tribol. Int. 2020, 143, 106041. [Google Scholar] [CrossRef]
- Grützmacher, P.G.; Rosenkranz, A.; Szurdak, A.; König, F.; Jacobs, G.; Hirt, G.; Mücklich, F. From lab to application—Improved frictional performance of journal bearings induced by single- and multi-scale surface patterns. Tribol. Int. 2018, 127, 500–508. [Google Scholar] [CrossRef]
- Wang, Y.; Li, Q.; Zhang, S.; Tang, X.; Xu, W.; Wang, Z. Analysis of turbulence and blocking effects on loading capacity for elementary texture cells of infinite width under water lubrication. ILT 2021, 73, 103–112. [Google Scholar] [CrossRef]
- Gropper, D.; Wang, L.; Harvey, T.J. Hydrodynamic lubrication of textured surfaces: A review of modeling techniques and key findings. Tribol. Int. 2016, 94, 509–529. [Google Scholar] [CrossRef]
- Ma, C.; Duan, Y.; Yu, B.; Sun, J.; Tu, Q. The comprehensive effect of surface texture and roughness under hydrodynamic and mixed lubrication conditions. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2017, 231, 1307–1319. [Google Scholar] [CrossRef]
- Dobrica, M.B.; Fillon, M.; Pascovici, M.D.; Cicone, T. Optimizing surface texture for hydrodynamic lubricated contacts using a mass-conserving numerical approach. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2010, 224, 737–750. [Google Scholar] [CrossRef]
- Fowell, M.T.; Medina, S.; Olver, A.V.; Spikes, H.A.; Pegg, I.G. Parametric study of texturing in convergent bearings. Tribol. Int. 2012, 52, 7–16. [Google Scholar] [CrossRef]
- Rosenkranz, A.; Grützmacher, P.G.; Gachot, C.; Costa, H.L. Surface Texturing in Machine Elements—A Critical Discussion for Rolling and Sliding Contacts. Adv. Eng. Mater. 2019, 21, 1900194. [Google Scholar] [CrossRef]
- Etsion, I. State of the Art in Laser Surface Texturing. J. Tribol. 2005, 127, 248–253. [Google Scholar] [CrossRef]
- Marian, M.; Almqvist, A.; Rosenkranz, A.; Fillon, M. Numerical micro-texture optimization for lubricated contacts—A critical discussion. Friction 2022, 10, 1772–1809. [Google Scholar] [CrossRef]
- Hamilton, D.B.; Walowit, J.A.; Allen, C.M. A Theory of Lubrication by Microirregularities. J. Basic Eng. 1966, 88, 177–185. [Google Scholar] [CrossRef]
- Etsion, I.; Kligerman, Y.; Halperin, G. Analytical and Experimental Investigation of Laser-Textured Mechanical Seal Faces. Tribol. Trans. 1999, 42, 511–516. [Google Scholar] [CrossRef]
- Arghir, M.; Roucou, N.; Helene, M.; Frene, J. Theoretical Analysis of the Incompressible Laminar Flow in a Macro-Roughness Cell. J. Tribol. 2003, 125, 309–318. [Google Scholar] [CrossRef]
- Sahlin, F.; Glavatskih, S.B.; Almqvist, T.; Larsson, R. Two-Dimensional CFD-Analysis of Micro-Patterned Surfaces in Hydrodynamic Lubrication. J. Tribol. 2005, 127, 96–102. [Google Scholar] [CrossRef]
- Keller, D.; Jacobs, G.; Neumann, S. Development of a Low-Friction Radial Shaft Seal: Using CFD Simulations to Optimise the Microstructured Sealing Lip. Lubricants 2020, 8, 41. [Google Scholar] [CrossRef]
- Fowell, M.; Olver, A.V.; Gosman, A.D.; Spikes, H.A.; Pegg, I. Entrainment and Inlet Suction: Two Mechanisms of Hydrodynamic Lubrication in Textured Bearings. J. Tribol. 2007, 129, 336–347. [Google Scholar] [CrossRef]
- Tønder, K. Hydrodynamic effects of tailored inlet roughnesses: Extended theory. Tribol. Int. 2004, 37, 137–142. [Google Scholar] [CrossRef]
- Tala-Ighil, N.; Maspeyrot, P.; Fillon, M.; Bounif, A. Effects of surface texture on journal-bearing characteristics under steady-state operating conditions. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2007, 221, 623–633. [Google Scholar] [CrossRef]
- Tala-Ighil, N.; Fillon, M.; Maspeyrot, P. Effect of textured area on the performances of a hydrodynamic journal bearing. Tribol. Int. 2011, 44, 211–219. [Google Scholar] [CrossRef]
- Brizmer, V.; Kligerman, Y. A Laser Surface Textured Journal Bearing. J. Tribol. 2012, 134, 031702. [Google Scholar] [CrossRef]
- Kango, S.; Sharma, R.K.; Pandey, R.K. Thermal analysis of microtextured journal bearing using non-Newtonian rheology of lubricant and JFO boundary conditions. Tribol. Int. 2014, 69, 19–29. [Google Scholar] [CrossRef]
- Usman, A.; Park, C.W. Numerical optimization of surface texture for improved tribological performance of journal bearing at varying operating conditions. ILT 2018, 70, 1608–1618. [Google Scholar] [CrossRef]
- Vlădescu, S.-C.; Fowell, M.; Mattsson, L.; Reddyhoff, T. The effects of laser surface texture applied to internal combustion engine journal bearing shells—An experimental study. Tribol. Int. 2019, 134, 317–327. [Google Scholar] [CrossRef]
- Singh, N.; Awasthi, R.K. Influence of texture geometries on the performance parameters of hydrodynamic journal bearing. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2021, 235, 2056–2072. [Google Scholar] [CrossRef]
- Meng, F.M.; Zhang, L.; Liu, Y.; Li, T.T. Effect of compound dimple on tribological performances of journal bearing. Tribol. Int. 2015, 91, 99–110. [Google Scholar] [CrossRef]
- Liang, X.; Liu, Z.; Wang, H.; Zhou, X.; Zhou, X. Hydrodynamic lubrication of partial textured sliding journal bearing based on three-dimensional CFD. ILT 2016, 68, 106–115. [Google Scholar] [CrossRef]
- Shinde, A.B.; Pawar, P.M. Multi-objective optimization of surface textured journal bearing by Taguchi based Grey relational analysis. Tribol. Int. 2017, 114, 349–357. [Google Scholar] [CrossRef]
- Arif, M.; Shukla, D.K.; Kango, S.; Sharma, N. Implication of Surface Texture and Slip on Hydrodynamic Fluid Film Bearings: A Comprehensive Survey. Tribol. Online 2020, 15, 265–282. [Google Scholar] [CrossRef]
- Yamada, H.; Taura, H.; Kaneko, S. Numerical and Experimental Analyses of the Dynamic Characteristics of Journal Bearings With Square Dimples. J. Tribol. 2018, 140, 011703. [Google Scholar] [CrossRef]
- Yong, H.; Balendra, R. CFD analysis on the lubrication behaviours of journal bearing with dimples. In Proceedings of the 2009 International Conference on Mechatronics and Automation, Changchun, China, 18 September 2009. [Google Scholar]
- Wang, W.; He, Y.; Li, Y.; Wei, B.; Hu, Y.; Luo, J. Investigation on inner flow field characteristics of groove textures in fully lubricated thrust bearings. ILT 2018, 70, 754–763. [Google Scholar] [CrossRef]
- Wang, W.; He, Y.; Zhao, J.; Li, Y.; Luo, J. Numerical optimization of the groove texture bottom profile for thrust bearings. Tribol. Int. 2017, 109, 69–77. [Google Scholar] [CrossRef]
- Feldermann, A.; Neumann, S.; Jacobs, G. CFD simulation of elastohydrodynamic lubrication problems with reduced order models for fluid–structure interaction. Tribol.-Mater. Surf. Interfaces 2017, 11, 30–38. [Google Scholar] [CrossRef]
- Sagar, H.J.; el Moctar, O. Dynamics of a cavitation bubble near a solid surface and the induced damage. J. Fluids Struct. 2020, 92, 102799. [Google Scholar] [CrossRef]
- Greenshields, C.J.; Weller, H.G. Notes on Computational Fluid Dynamics: General Principles; CFD Direct Limited: Reading, UK, 2022. [Google Scholar]
- Wallis, G.B. One-Dimensional Two-Phase Flow; Dover Publications Inc.: Garden City, NY, USA, 2020. [Google Scholar]
- Wen, S.; Huang, P. Principles of Tribology (Second Edition): Mo Ca Xue Yuan Li (Di 2 Ban)/Wen Shizhu, Huang Ping Zhu, Di 1 Ban; Tsinghua University Press: Beijing, China, 2017. [Google Scholar]
- Gao, G.; Yin, Z.; Jiang, D.; Zhang, X. Numerical analysis of plain journal bearing under hydrodynamic lubrication by water. Tribol. Int. 2014, 75, 31–38. [Google Scholar] [CrossRef]
- Lin, Q.; Bao, Q.; Li, K.; Khonsari, M.M.; Zhao, H. An investigation into the transient behavior of journal bearing with surface texture based on fluid-structure interaction approach. Tribol. Int. 2018, 118, 246–255. [Google Scholar] [CrossRef]
- Xie, Z.; Jiao, J.; Yang, K.; He, T.; Chen, R.; Zhu, W. Experimental and numerical exploration on the nonlinear dynamic behaviors of a novel bearing lubricated by low viscosity lubricant. Mech. Syst. Signal Process. 2023, 182, 109349. [Google Scholar] [CrossRef]

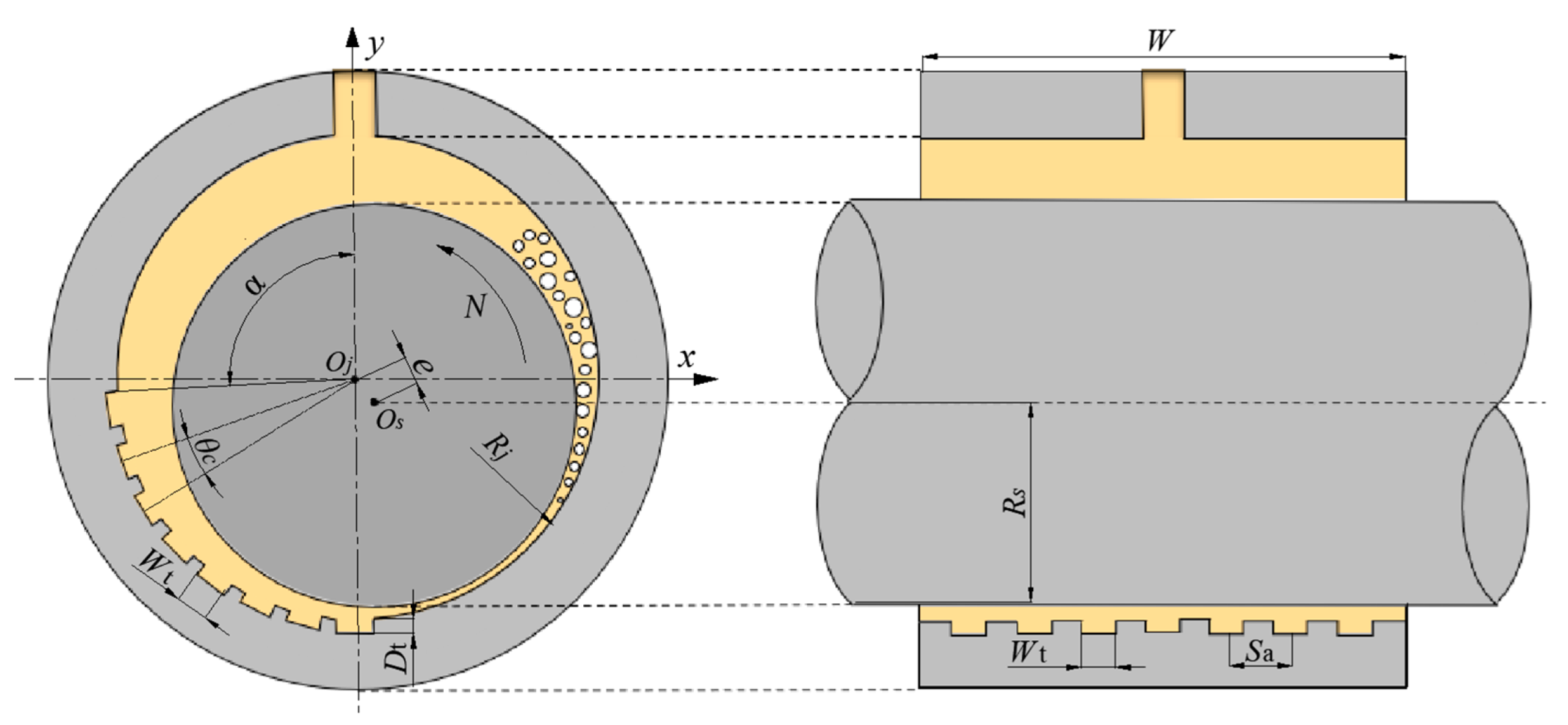



| Symbol | Parameter | Value |
|---|---|---|
| Rj/mm | Bearing radius | 15 |
| W/mm | Bearing width | 20 |
| Rs/mm | Shaft radius | 14.97 |
| e/mm | Eccentricity distance | 0.018 |
| θ/° | Attitude angle | 45 |
| α/° | Starting angle of textured zone | 50~250 |
| θc/° | Textured space in circumferential direction | 10 |
| Sa/mm | Textured space in axial direction | 3 |
| Nc/mm | Textured number in circumferential direction | 8 |
| Na/mm | Textured number in axial direction | 7 |
| N/rpm | Rotational speed | 600 |
| Pin/MPa | Inlet pressure | 0.15 |
| Pout/MPa | Outlet pressure | 0.1 |
| Psat/Pa | Saturation pressure | 29,185 |
| ρl/kg∙m−3 | Density of lubricant | 822 |
| μl/Pa∙s | Dynamic viscosity of lubricant | 0.014 |
| ρv/kg∙m−3 | Density of vapor | 1.29 |
| μv/Pa∙s | Dynamic viscosity of vapor | 5.953 × 10−6 |
| Simulation Cases | W1D15 | W1D50 | W2D15 | W2D50 |
|---|---|---|---|---|
| Wt/mm | 1 | 1 | 2 | 2 |
| Dt/mm | 0.15 | 0.50 | 0.15 | 0.50 |
| position/° | α = 50/100/150/200/250 | |||
| Item | Elements | Nodes | Average Mesh Quality | Average Skewness |
|---|---|---|---|---|
| values | 165,040 | 770,804 | 5.02 × 10−2 | 2.60 × 10−2 |
| Item | Average aspect ratio | Jacobi value (MAPDL) | Jacobi value (Gauss Point) | Orthogonal quality |
| values | 51 | 1.02 | 0.99 | 0.99 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Jacobs, G.; König, F.; Zhang, S.; von Goeldel, S. Investigation of Microflow Effects in Textures on Hydrodynamic Performance of Journal Bearings Using CFD Simulations. Lubricants 2023, 11, 20. https://doi.org/10.3390/lubricants11010020
Wang Y, Jacobs G, König F, Zhang S, von Goeldel S. Investigation of Microflow Effects in Textures on Hydrodynamic Performance of Journal Bearings Using CFD Simulations. Lubricants. 2023; 11(1):20. https://doi.org/10.3390/lubricants11010020
Chicago/Turabian StyleWang, Yujun, Georg Jacobs, Florian König, Shuo Zhang, and Stephan von Goeldel. 2023. "Investigation of Microflow Effects in Textures on Hydrodynamic Performance of Journal Bearings Using CFD Simulations" Lubricants 11, no. 1: 20. https://doi.org/10.3390/lubricants11010020
APA StyleWang, Y., Jacobs, G., König, F., Zhang, S., & von Goeldel, S. (2023). Investigation of Microflow Effects in Textures on Hydrodynamic Performance of Journal Bearings Using CFD Simulations. Lubricants, 11(1), 20. https://doi.org/10.3390/lubricants11010020