Impact of Thermal and Activation Energies on Glauert Wall Jet (WJ) Heat and Mass Transfer Flows Induced by ZnO-SAE50 Nano Lubricants with Chemical Reaction: The Case of Brinkman-Extended Darcy Model

Abstract

Heat transfer machinery or technology is rapidly expanding due to the need for effective cooling and heating systems in the requisite automotive, chemical, and aerospace industries. This study aims to provide a numerical solution to wall jet (WJ) flow with mass and heat transport phenomenon comprising of the colloidal mixture of SAE50 and zinc oxide nanoparticles immersed in a Brinkman-extended Darcy model. The idea of WJ flow suggested by Glauert is further discussed along with the impact of the activation energy, thermal radiation, and binary chemical reaction. The leading equations are transformed into ordinary differential equations through proper similarity variables and then worked out numerically by employing a very efficient bvp4c method. The importance of pertaining quantities is illustrated and well explained through several tables and graphs. The major results suggest that the velocity profiles decline while the temperature and concentration augment due to the higher impact of nanoparticles volume fraction. In addition, the shear stress and heat transfer rate are accelerated by rising the volume fraction of nanoparticles while the Sherwood number declines with bigger impacts of nanoparticle volume fraction. In addition, the radiation factor progresses the quantitative outcomes of the heat transfer rate.

1. Introduction

A prescribed wall jet (WJ) is one of the interesting flowing fields produced when a faster-moving liquid is injected into a thin layer close to a vital posited surface. The external or ambient fluid may be moving or still, but often at a slower speed than the jet being injected. Such flows are of interest to scientists and engineers for several applications, such as blades for gas turbines and automotive defrosters. In essence, a WJ can be considered as dual layers stream that has an outside area and an interior zone that continues by almost the point of larger velocity in the perpendicular direction. The flow pattern in the outer area most closely matches a free shear layer, while the inner part most closely reflects a wall boundary layer. The primary characteristics of these layers differ, and their interaction in a WJ results in a complex flow field.

Glauert [1] was the primary researcher to bring up and divulge the problem of a stationary impenetrable wall forming a wall jet. Astin and Wilks [2] presented novel outcomes that show the behavior of the jet-like of Falkner-Skan equation. Zaidi et al. [3] conducted comparison research on incompressible nanofluids over a wall jet by taking into account the suspension of carbon nanotubes. They talked about how a magnetic field affects heat and stream transport. Jafarimoghaddam and Pop [4] applied the model of Tiwari and Das [5] to inspect the jet flow and features of the heat transport phenomenon of the type of decaying exponential wall. The heat transport features in a jet flow of the Glauert type were addressed by Turkyilmazoglu [6]. The condition of velocity slip was used to describe the flow analysis. By employing the Buongiorno [7] model, Jafarimoghaddam and Shafizadeh [8] computationally evaluated the WJ stream of nanofluids and explored spatial stability. The dynamics of flow and thermal properties of a WJ through a wavy surface were explained by Kumari and Kumar [9].

An essential method for heating or cooling an object is through heat transfer. The excessive heat created by a machine or system requires either increasing or dissipating for it to operate at its best. Liquid coolants have been employed to minimize the heat of equipment such as processors and in a variety of industries such as electronics and automotive. However, regular liquid coolants display low thermal conductivity. This promotes creativity and improvement in cooling technology. The use of a fluid with metal nanoparticles in it, known as nanofluid, is one of the methods that have been found to develop the heat transfer features of liquid coolants. Metal nanoparticles are added to heat transfer fluids incorporating water, ethylene glycol, and oil to improve the heat transfer efficiency of liquid coolants since metal is a sort of substance with significant thermal conductivity (TCN). Rashid et al. [10] investigated the consequences of nanoparticles with various shapes along with the irreversibility process. The results show that the lamina shape factor of nanoparticles performs better in terms of heat exchange, irreversibility production, and temperature dispersion. The impression of stagnation-point radiative flow induced by Casson nanofluid towards a radial surface was inspected by Narender et al. [11]. The outcomes demonstrated that the rate of heat transport coefficient is augmented and the irregular radiation parameter is raised in a Casson nanofluid. Yahya et al. [12] examined the thermal characteristics of radiative flow induced by Williamson Sutterby nanofluid via a Darcy-Forchheimer spongy medium over an infinite heated plate taking into account the Cattaneo-Christov impact. Garia et al. [13] scrutinized the steady magnetohydrodynamic flow of water-based hybrid nanofluid past cone and wedge geometries with Cattaneo-Christov heat flux. Laila and Marwat [14] investigated the nanofluid flow in diverging and converging channels in the presence of inclined and heated plane walls and presented an exact solution in a limited range of physical parameters. Yaseen et al. [15] explored the features of magneto-radiative hybrid nanofluids between rotating and shrinking disks with Cattaneo-Christov heat flux. Mabood et al. [16] inspected the features of nanofluid flow through an irregular thickened stretching sheet with non-Flick’s mass and non-Fourier heat fluxes. The features of a hybrid nanofluid through a shrinking/stretching sheet in a Darcy-Forchheimer porous medium were inspected by Yaseen et al. [17]. Lately, Khan et al. [18] operated the single-phase model to inspect the nanoparticle flow induced by an erratic movable sheet with convective boundary conditions. They presented double solutions in a specific domain of the movable parameter.

Activation energy is widely considered while studying a variety of physical problems, including engineering and oil storage. In addition, the field of fluid dynamics has engrossed the devotion of researchers owing to the plentiful applications of a chemical reaction and Arrhenius activation energy (AAE). At the most fundamental level, activation energy only initiates a chemical reaction. This is because even merely starting certain chemical reactions requires energy. Other uses for activation energy include compound creation, atomic processes, and the restoration of thermal lubricants. The least amount of energy required to stimulate the particles or molecules in which physical transit occurs is known as activation energy. Arrhenius defined AE in 1889. Bestman [19] reported the primary article on AAE within binary chemical reactions. Abbas et al. [20] inspected the unsteady Casson fluid flow through a movable sheet with the features of activation energy and radiation. Hsiao [21] inspected the viscous MHD flow that experiences to increase in the extrusion system’s economic efficacy in the prevailing environment. Khan et al. [22] investigated the impression of AAE through a cross fluid under the movable wedge and presented double solutions. Ullah et al. [23] explored the impact of irregular thermal conductivity and temperature-dependent viscosity on the fluid flow of nanofluid through a rotating system between parallel plates. Yesodha et al. [24] emphasized the significance of nanofluid flow with reactants chemically from a permeable stretchable sheet with activation energy.

Inertia effects and barriers in the requisite posited porous media (PM), which could modify patterns of the given dynamics flow with features of heat transport phenomenon, are disregarded by Darcy’s model. It is indeed critical to determine the scenarios under which these effects apply. The model of Brinkman [25], which is an expansion of Darcy’s law, had better be used for no-slip circumstances, according to Hong et al. [26]. Ishak et al. [27] utilized this model to investigate the continuous flow across a vertical experiencing object (VEO) in a PM near the stagnation point through the wall temperature. Pantokratoras [28] investigated the forced convection flow and characteristics of the heat transport phenomenon through a heated plane surface soaked in a Darcy-Brinkman porous medium (DBPM). The dissipative effect on the slip flow immersed in a DBPM was lately studied by Kausar et al. [29]. Recently, Wang [30] reported the flow via a smooth channel in a Darcy-Brinkman porous medium.

The literature reviews described above demonstrated that no attempt has been undertaken to date to inspect the Glauert jet wall flow in ZnO-SAE50 nanofluids in a Darcy-Brinkman porous media including activation energy. In addition, most applications make use of turbulent jet flow. However, the turbulent state necessitates a changeable position for the transitions in cooling applications. The nanofluid has further applications related to combustion and works well for cooling in environments with a wide temperature range. Thus, in the existing problem, the consequence of AAE on the Glauert WJ flow in a DBPM within ZnO-SAE50 nanoparticles is explored numerically. The flow problem is modeled in the form of partial differential equations. The Glauert variables are utilized to change these into ordinary differential equations (ODEs) and then numerically examined via a bvp4c solver. More intriguing variables’ behavior is represented graphically.

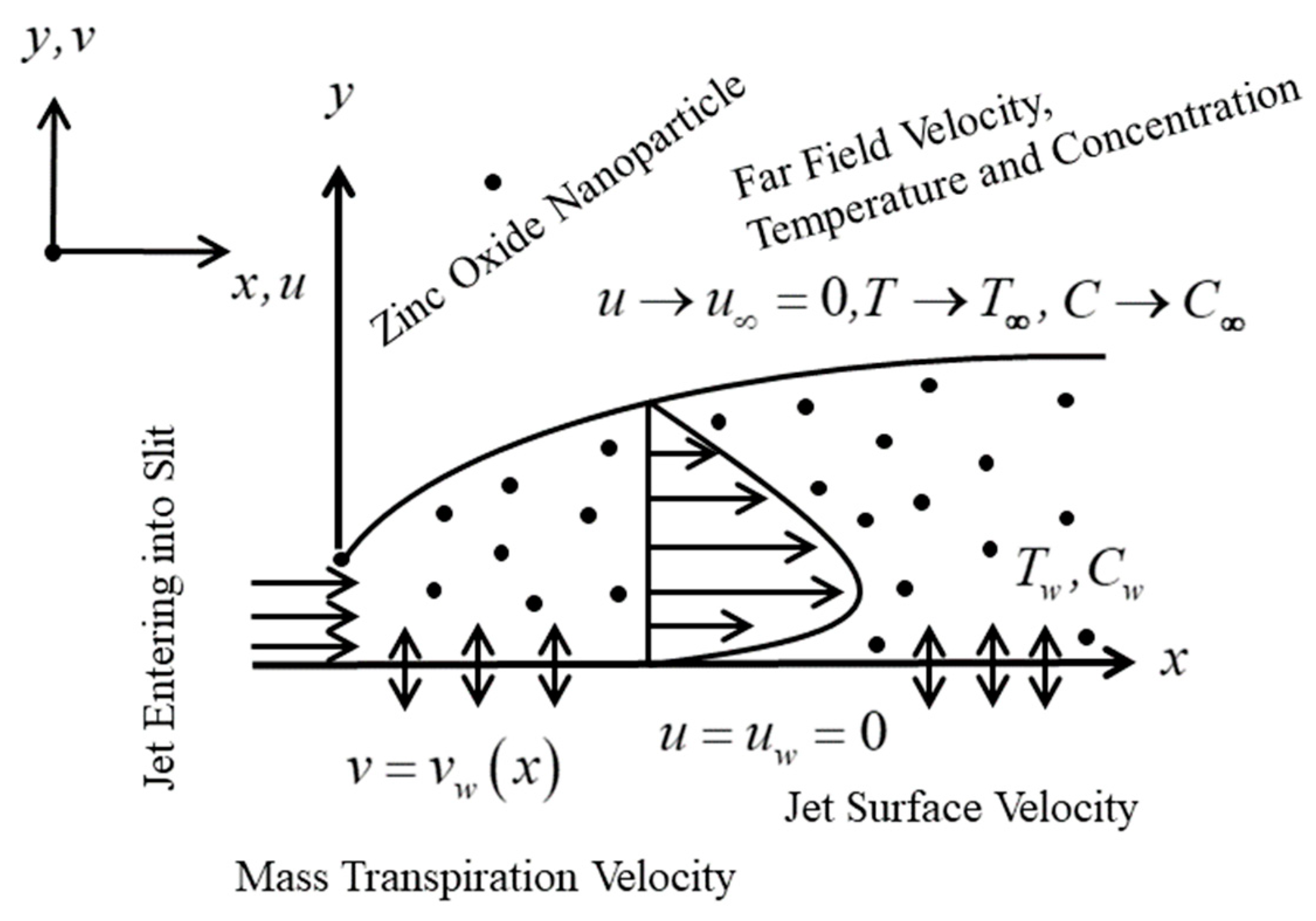
2. Materials and Methods

The schematic pattern of the Glauert model is shown in Figure 1, where a 2D wall jet flow with mass and heat transport phenomenon involving SAE50-based fluid with ZnO nanoparticles saturated in a DBPM extended model with the important impact of mass transpiration and thermal energy effect is examined. Moreover, AAE and binary chemical reaction influences are also added to the present scrutiny. The $x-$axis and $y-$axis coordinates are taken along the corresponding horizontal surface and vertical or perpendicular to it. The liquid temperature is indicated by $T$, with the fixed wall temperature by $T_w$ and the external or ambient temperature by $T_{\infty }$. Assume further, $C_w$ and $C_{\infty }$ stand for the fluid’s uniform wall and ambient concentrations, respectively. However, the thermal radiation term is involved in the equation of the energy and is symbolically indicated as $q_r$. These presumptions allow the formation of the following leading equations [3,6]:

$$\frac{\partial u}{\partial x}+\frac{\partial v}{\partial y}=0,$$

(1)

$$u\frac{\partial u}{\partial x}+v\frac{\partial u}{\partial y}=\frac{{\mu }_{eff}{\epsilon }_a{}^2}{{\rho }_{nf}}\left(\frac{{\partial }^{2}u}{\partial y^2}\right)-\frac{{\mu }_{nf}{\epsilon }_a{}^2}{K\left(x\right){\rho }_{nf}}u,$$

(2)

$$\begin{array}{l}u\frac{\partial T}{\partial x}+v\frac{\partial T}{\partial y}=\frac{k_{nf}}{{\left(\rho c_p\right)}_{nf}}\left(\frac{{\partial }^{2}T}{\partial y^2}\right)-\frac{1}{{\left(\rho c_p\right)}_{nf}}\frac{\partial q_r}{\partial y},\\ q_r=\left(-\frac{4{\sigma }^{*}}{3k^{*}}\right)\frac{\partial T^4}{\partial y},T^4\approx 4T_{\infty }^{3}T-3T_{\infty }^4,\end{array}$$

(3)

$$u\frac{\partial C}{\partial x}+v\frac{\partial C}{\partial y}=D_f\frac{{\partial }^{2}C}{\partial y^2}-k_r^2\left(x\right){\left(\frac{T}{T_{\infty }}\right)}^m{e}^{\frac{-E_0}{k_{f}T}}\left(C-C_{\infty }\right),$$

(4)

along with the boundary conditions (BCs),

$$\begin{array}{l}u=0,v=v_w\left(x\right),T=T_w,C=C_w \mathrm{at} y=0,\\ u\to 0, T\to T_{\infty }, C\to C_{\infty } \mathrm{as} y\to \infty .\end{array}\}$$

(5)

Here, $u$ and $v$ signify the elements of velocity in the respective $x-$ and $y-$axes, ${\mu }_{eff}$ the effective dynamic viscosity, ${\epsilon }_a$ the porosity parameter, $K\left(x\right)$ the variable permeability parameter. The mass transpiration velocity is further supposed in the form as $v_w\left(x\right)=-\sqrt{{\alpha }_f}{x}^{-3/4}{f}_w$, where ${\alpha }_f$ and $f_w$ describe the thermal diffusivity and the mass suction/injection, respectively. In addition, ${\sigma }^{*}$, $k^{*}$, $k_r^2\left(x\right)$, $E_a$, and $D_f$ indicate the Stefan Boltzmann constant, the mean absorption constant, the rate of variable chemical reaction, the activation energy, and the mass diffusion coefficient.

In the aforementioned stated equations, the thermal conductivity, the density, the specific heat capacity, and the dynamic viscosity of the nanofluid are symbolically denoted by $k_{nf}$, ${\rho }_{nf}$, ${\left(\rho c_p\right)}_{nf}$ and ${\mu }_{nf}$, respectively. Meanwhile, the expression or correlation of the nanofluid model can be written as [6,10]:

$$\frac{k_{nf}}{k_f}=\frac{k_{snp}+2k_f+\phi \left(2k_{snp}-2k_f\right)}{k_{snp}+2k_f-\phi \left(k_{snp}-k_f\right)},$$

(6)

$$\frac{{\mu }_{nf}}{{\mu }_f}=\frac{1}{{\left(1-\phi \right)}^{2.5}} , \frac{{\rho }_{nf}}{{\rho }_f}=\phi \left(\frac{{\rho }_{snp}}{{\rho }_f}\right)+\left(1-\phi \right),$$

(7)

$$\frac{{\left(\rho c_p\right)}_{nf}}{{\left(\rho c_p\right)}_f}=\phi \left(\frac{{\left(\rho c_p\right)}_{snp}}{{\left(\rho c_p\right)}_f}\right)+\left(1-\phi \right).$$

(8)

Therefore, Equations (6)–(8) demonstrate the physical characteristics of the ZnO-SAE50 nano-lubricants, whereas, the solid volume fraction of nanoparticles is denoted by $\phi$. In addition, the subscript $f$, and $snp$ represent the corresponding regular (viscous) fluid and the solid nanoparticles. The experimental values of physical data of the ZnO nanoparticles and ordinary base (SAE50) fluid are provided in

Table 1. The physical properties of the nanofluid [31,32].

Nanoparticles	$\mathit{k} \left(\mathbf{W}/\mathbf{m}\mathbf{K}\right)$	${\mathit{c}}_{\mathit{p}}\left(\mathbf{J}/\mathbf{k}\mathbf{g}\mathbf{K}\right)$	$\mathit{\rho } \left(\mathbf{k}\mathbf{g}/{\mathbf{m}}^3\right)$	$\mathit{\mu } \left(\mathbf{P}\mathbf{a} \mathbf{s}\right)$
SAE50	0.15	1900	0.906	0.192543
ZnO	19	544	5.606	-

.

2.1. The Similarity Transformations

Here, we enumerated the similarity transformations listed by Glauert [1] to make it easier to examine the wall jet flow model under consideration. They are as follows:

$$\xi =\frac{y}{\sqrt{{\alpha }_f}}{x}^{-3/4},\psi =\sqrt{{\alpha }_f}{x}^{1/4}F\left(\xi \right), G\left(\xi \right)=\frac{T-T_{\infty }}{T_w-T_{\infty }},S\left(\xi \right)=\frac{C-C_{\infty }}{C_w-C_{\infty }}$$

(9)

where $\psi$ is the requisite stream function and usually defined as $u=\partial \psi /\partial y$ and $v=-\partial \psi /\partial x$. Therefore, the velocity components can take place in the following acquired form:

$$u=\frac{4}{\sqrt{x}}{F}^{\prime }\left(\xi \right), v=-\sqrt{{\alpha }_f}{x}^{-3/4}\left(F\left(\xi \right)-3\xi F^{\prime }\left(\xi \right)\right).$$

(10)

2.2. The Momentum Similarity Equation

To acquire the momentum equation in a similar form, it is better to define first the variable porous medium permeability term $K\left(x\right)$ which is mathematically expressed as:

$$K\left(x\right)=K_0\sqrt{x^3},$$

(11)

where $K_0$ is the arbitrary positive constant.

Now using the Equations (9) and (11) in the early stated equations of this Section 1 and Section 2, where the equation of continuity is satisfied and the momentum equation takes place in the form:

$$\frac{{\epsilon }_b}{{\rho }_{nf}/{\rho }_f}{F}^{‴}+F{F}^{″}+2{F^{\prime }}^2-\frac{{\mu }_{nf}/{\mu }_f}{{\rho }_{nf}/{\rho }_f}{K}_a{F}^{\prime }=0,$$

(12)

where ${\epsilon }_b=\frac{{\upsilon }_{eff}{\epsilon }_a{}^2}{{\alpha }_f}$ is the modified porosity parameter.

2.3. The Energy Similarity Equation

First of all, to ease the condition of Equation (3), we substitute the approximate value of the thermal heat flux, $q_r$, to acquire the following form:

$${\left(\rho c_p\right)}_{nf}\left(u\frac{\partial T}{\partial x}+v\frac{\partial T}{\partial y}\right)=k_f\left(\frac{k_{nf}}{k_f}+\frac{4}{3}{R}_d\right)\frac{{\partial }^{2}T}{\partial y^2},$$

(13)

where $R_d=\frac{4{\sigma }^{*}{T}_{\infty }^3}{k^{*}{k}_f}$ is the radiation parameter. Further, by executing Equation (9) into above stated Equation (13), one obtains:

$$\frac{1}{{\left(\rho c_p\right)}_{nf}/{\left(\rho c_p\right)}_f}\left(\frac{k_{nf}}{k_f}+\frac{4}{3}{R}_d\right)G^{″}+F{G}^{\prime }=0.$$

(14)

2.4. The Concentration Similarity Equation

For making the concentration equation a similar one, after the exercise of transformation, here, we define the reaction rate as a function of $k_r^2\left(x\right)=k_0^2{x}^{-3/2}$. By utilizing Equation (9), the concentration Equation (4) yields.

$$\frac{1}{Le}{S}^{″}+F{S}^{\prime }-{\beta }_a{\left(1+{\delta }_{a}G\right)}^m\exp \left(\frac{-E_a}{1+{\delta }_{a}G}\right)S=0,$$

(15)

where $Le=\frac{{\alpha }_f}{D_f}$,$E_a=\frac{E_0}{k_f{T}_{\infty }}$, and ${\delta }_a=\frac{T_w-T_{\infty }}{T_{\infty }}$ are the Lewis number, the AAE parameter, and the temperature difference factor, respectively.

2.5. The Converted BCs

After substituting Equation (9) into Equation (5), the following transformed BCs are obtained:

$$\{\begin{array}{l}F\left(0\right)=f_w, F^{\prime }\left(0\right)=0, G\left(0\right)=S\left(0\right)=1 \mathrm{at} \xi =0,\\ F^{\prime }\left(\xi \right)\to 0, G\left(\xi \right)\to 0, S\left(\xi \right) \to 0 \mathrm{as} \xi \to \infty .\end{array}$$

(16)

Here, $f_w$ indicates the constant mass transpiration velocity or mass suction/injection parameter. Therefore, $f_w=0$, $f_w<0$, and $f_w>0$ signify the case of mass impermeable, injection, and suction, respectively.

2.6. The Gradients or Engineering Physical Quantities

For the considered jet problem, the following three important physical engineering quantities are used such as the friction factor $C_f$, the local Nusselt number $N{u}_x$, and the local Sherwood number (mass transfer rate) $S{h}_x$ as follows:

$$\begin{array}{l}{C}_f=\frac{1}{{\rho }_f{u}_r^2}\left({{\mu }_{nf}\frac{\partial u}{\partial y}|}_{y=0}\right),N{u}_x=\frac{x}{k_f\left(T_w-T_{\infty }\right)}\left(-k_{nf}{\frac{\partial T}{\partial y}|}_{y=0}+{\left(q_r\right)|}_{y=0}\right),\\ S{h}_x=\frac{x}{D_f\left(C_w-C_{\infty }\right)}{\left(-D_f\frac{\partial C}{\partial y}\right)|}_{y=0},\end{array}$$

(17)

where the corresponding reference velocity is denoted by $u_r=4x^{-1/2}$, see Raees et al. [33]. Further, exercising Equation (9) into the aforesaid Equation (17), we acquire,

$$2\sqrt{\frac{{\mathrm{Re}}_x}{\mathrm{Pr}}}{C}_f=\frac{{\mu }_{nf}}{{\mu }_f}{F}^{″}\left(0\right),\frac{2N{u}_x}{\sqrt{P{e}_x}}=-\left(\frac{k_{nf}}{k_f}+\frac{4}{3}{R}_d\right)G^{\prime }\left(0\right),\frac{2S{h}_x}{\sqrt{P{e}_x}}=-S^{\prime }\left(0\right),$$

(18)

where $P{e}_x=\frac{x{u}_r}{{\alpha }_f}$, ${\mathrm{Re}}_x=\frac{x{u}_r}{{\upsilon }_f}$ and $\mathrm{Pr}=\frac{{\upsilon }_f}{{\alpha }_f}$ are called the local Peclet number, the local Reynolds number, and the Prandtl number, respectively.

3. Numerical Methodology

The set of Equations (12), (14) and (15) along with BCs (16) are highly complicated and non-linear, which is difficult to work out, exactly or analytically. Therefore, the bvp4c scheme is implemented for the given jet problem to deal with the aforesaid similarity equations numerically. This scheme is based basically on a finite difference approach which is also further known as the three-stage Lobatto IIIA formula. In order to apply this scheme, the higher-order ODEs are changed into first-order ODEs by using the new variables as follows:

$$F=B_1,F^{\prime }=B_2,F^{″}=B_3,G=B_4,G^{\prime }=B_5,S=B_6,S^{\prime }=B_7,$$

(19)

$$\frac{d}{d\xi }\left(\begin{array}{c}{B}_1\\ B_2\\ B_3\\ \begin{array}{l}{B}_4\\ B_5\\ B_6\\ B_7\end{array}\end{array}\right)=\left(\begin{array}{l}{B}_2\\ B_3\\ \frac{{\rho }_{nf}/{\rho }_f}{{\epsilon }_b}\left(-B_1{B}_3-2B_2^2+\frac{{\mu }_{nf}/{\mu }_f}{{\rho }_{nf}/{\rho }_f}{K}_a{B}_2\right)\\ B_5\\ \frac{{\left(\rho c_p\right)}_{nf}/{\left(\rho c_p\right)}_f}{\left(k_{nf}/k_f+\frac{4}{3}{R}_d\right)}\left(-B_1{B}_5\right)\\ B_7\\ Le\left(-B_1{B}_7+{\beta }_a{\left(1+{\delta }_a{B}_4\right)}^m\exp \left(\frac{-E_a}{\left(1+{\delta }_a{B}_4\right)}\right)B_6\right)\end{array}\right),$$

(20)

with altered BCs are,

$$\left(\begin{array}{c}{B}_1(0)\\ B_2(0)\\ \begin{array}{l}{B}_2(\infty )\\ B_4(0)\end{array}\\ \begin{array}{l}{B}_4(\infty )\\ B_6(0)\\ B_6(\infty )\end{array}\end{array}\right)=\left(\begin{array}{l}{f}_w\\ 0\\ 0\\ 1\\ 0\\ 1\\ 0\end{array}\right).$$

(21)

In order to meet the convergence criterion, the tolerance is set as $\epsilon ={10}^{-6}$, which applied throughout the computation. Since there is only one possible solution to the aforementioned jet problem, the anticipated simulation procedure desires initial or preliminary estimates to satisfy the BCs (21). For single solution problems, the initial first guess is very simple and looks obvious but in the WJ problem, it is somewhat difficult, therefore, it is better to choose the distinct influential parameter values appropriate to acquire the essential outcomes. In addition, the range is set up to ${\xi }_{\max }=25$, for the considered simulations, which is pretty enough to hold or accomplished the accuracy of asymptotic convergence, see all the graphs. On the other hand, the essential part regarding the well-known accuracy, reliability, and authentication of the considered numerical code is mentioned in the form of table. Therefore, it is preferable to create a computational table, so that, we may match the original work with the available reported works for specific limiting circumstances to verify the developed code.

Table 2. The comparison values of shear stress $\left({\mu }_{nf}/{\mu }_f\right)F^{″}\left(0\right)$ for the higher impacts of $\phi$ when ${\epsilon }_b=1.00$, $K_a=0$ and $f_w=0$.

$$\mathit{\phi }$$	Glauert [1]	Waini et al. [34]	Present Results
0.000	$2/9\approx 0.2222$	0.2222	0.2222
0.035	-	-	0.3178
0.037	-	-	0.3656
0.039	-	-	0.4132

describes, the distinct values of the friction factor with significant impacts of $\phi$ when $f_w=0$, $K_a=0$ and ${\epsilon }_b=1.0$. From the tabular results, it is noticed that the single present outcome of friction factor is compared truly accurately with the reported works of Glauert [1] and Waini et al. [34]. The present and the previous works are excellently matched. Therefore, it gives us confidence that the developed code is convincing for finding the unavailable outcomes of the WJ model.

4. Analysis of Results

The discussion is based on the interpreting of the entire results for the considered WJ problem of the SAE50 plus zinc oxide nanoparticles owing to the impact of sundry distinguished parameters. The considered problem comprised of distinct factors such as the temperature difference parameter ${\delta }_a$, the modified porosity parameter ${\epsilon }_b$, the radiation parameter $R_d$, the solid volume fraction of nanoparticles $\phi$, the activation parameter $E_a$, the dimensionless permeability parameter $K_a$, the chemical reaction rate parameter ${\beta }_a$, and the mass suction/injection parameter $f_w$. The consequence of these constraints on the friction factor,$\left({\mu }_{nf}/{\mu }_f\right)F^{″}\left(0\right)$, the Nusselt number, $-\left(k_{nf}/k_f+\left(4/3\right)R_d\right)G^{\prime }\left(0\right)$, and the Sherwood number, $-S^{\prime }\left(0\right)$, are quantitatively provided in Table 3, Table 4 and Table 5, respectively, whereas, the velocity $F^{\prime }\left(\xi \right)$, temperature $G\left(\xi \right)$, and concentration $S\left(\xi \right)$ profiles are shown in the corresponding Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11. For the computations, we take the following default values of the constraints in the entire paper: $\phi =0.035$, $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

4.1. Research Analysis of the Tables

The quantitative results of $\left({\mu }_{nf}/{\mu }_f\right)F^{″}\left(0\right)$ due to variations in $\phi$, ${\epsilon }_b$, $K_a$, and $f_w$ are illustrated in Table 3. The outcome reveals that the $\left({\mu }_{nf}/{\mu }_f\right)F^{″}\left(0\right)$ values escalate for larger impacts of $\phi$, ${\epsilon }_b$, and $f_w$ but it shrinkages with the larger values of $K_a$, and, $f_w$. Additionally, the mass suction and blowing constraints are responsible for the biggest and smallest shear stresses that were observed in the numerical table. In contrast, the computational values of the heat transport and $-S^{\prime }\left(0\right)$ of the WJ nano-lubricants are constructed quantitatively in Table 4 and Table 5, respectively. Outcomes disclose that $-\left(k_{nf}/k_f+\left(4/3\right)R_d\right)G^{\prime }\left(0\right)$ and $-S^{\prime }\left(0\right)$ upsurge with the larger values of $\phi$, $R_d$, $Le$, ${\beta }_a$, and ${\delta }_a$ but constant with the temperature index parameter $m$. However, both gradients reduce with the enormous impression of the activation parameter, and the values of $\phi$. In addition, the largest heat transport phenomenon, and $-S^{\prime }\left(0\right)$ are detected for the distinct choices of the radiation factor and Lewis number, respectively.

Table 3. The impact of $\left({\mu }_{nf}/{\mu }_f\right)F^{″}\left(0\right)$ for the distinct values of the sundry parameters.

$$\mathit{\phi }$$	$${\mathit{K}}_{\mathit{a}}$$	$${\mathit{\epsilon }}_{\mathit{b}}$$	$${\mathit{f}}_{\mathit{w}}$$	Present Results
0.025	0.50	0.70	1.0	$1.2781\times {10}^{-9}$
0.030	-	-	-	$1.6145\times {10}^{-9}$
0.035	-	-	-	$1.9234\times {10}^{-9}$
0.035	0.30	0.70	1.0	$2.4134\times {10}^{-9}$
-	0.50	-	-	$1.9234\times {10}^{-9}$
-	0.70	-	-	$1.4560\times {10}^{-9}$
0.035	0.50	0.60	1.0	$1.4477\times {10}^{-9}$
-	-	0.65	-	$1.6433\times {10}^{-9}$
-	-	0.70	-	$1.9234\times {10}^{-9}$
0.035	0.50	0.70	1.0	$1.9234\times {10}^{-9}$
-	-	-	1.5	$2.2350\times {10}^{-7}$
-	-	-	2.0	$2.1850\times {10}^{-5}$
0.035	0.50	0.70	−0.05	$-2.2398\times {10}^{-8}$
-	-	-	−0.10	$-1.8971\times {10}^{-8}$
-	-	-	−0.15	$-1.5216\times {10}^{-8}$

Table 3. The impact of $\left({\mu }_{nf}/{\mu }_f\right)F^{″}\left(0\right)$ for the distinct values of the sundry parameters.

$$\mathit{\phi }$$	$${\mathit{K}}_{\mathit{a}}$$	$${\mathit{\epsilon }}_{\mathit{b}}$$	$${\mathit{f}}_{\mathit{w}}$$	Present Results
0.025	0.50	0.70	1.0	$1.2781\times {10}^{-9}$
0.030	-	-	-	$1.6145\times {10}^{-9}$
0.035	-	-	-	$1.9234\times {10}^{-9}$
0.035	0.30	0.70	1.0	$2.4134\times {10}^{-9}$
-	0.50	-	-	$1.9234\times {10}^{-9}$
-	0.70	-	-	$1.4560\times {10}^{-9}$
0.035	0.50	0.60	1.0	$1.4477\times {10}^{-9}$
-	-	0.65	-	$1.6433\times {10}^{-9}$
-	-	0.70	-	$1.9234\times {10}^{-9}$
0.035	0.50	0.70	1.0	$1.9234\times {10}^{-9}$
-	-	-	1.5	$2.2350\times {10}^{-7}$
-	-	-	2.0	$2.1850\times {10}^{-5}$
0.035	0.50	0.70	−0.05	$-2.2398\times {10}^{-8}$
-	-	-	−0.10	$-1.8971\times {10}^{-8}$
-	-	-	−0.15	$-1.5216\times {10}^{-8}$

Table 4. The impact of $-\left(k_{nf}/k_f+\left(4/3\right)R_d\right)G^{\prime }\left(0\right)$ for the distinct values of the sundry parameters.

$$\mathit{\phi }$$	$$\mathit{m}$$	$${\mathit{R}}_{\mathit{d}}$$	Present Results
0.025	1.00	2.00	1.0204154
0.030	-	-	1.0242804
0.035	-	-	1.0281458
0.035	0.00	2.00	1.0281458
-	1.00	-	1.0281458
-	2.00	-	1.0281458
0.035	1.00	1.50	1.0272708
-	-	2.00	1.0281458
-	-	2.50	1.0301780

Table 4. The impact of $-\left(k_{nf}/k_f+\left(4/3\right)R_d\right)G^{\prime }\left(0\right)$ for the distinct values of the sundry parameters.

$$\mathit{\phi }$$	$$\mathit{m}$$	$${\mathit{R}}_{\mathit{d}}$$	Present Results
0.025	1.00	2.00	1.0204154
0.030	-	-	1.0242804
0.035	-	-	1.0281458
0.035	0.00	2.00	1.0281458
-	1.00	-	1.0281458
-	2.00	-	1.0281458
0.035	1.00	1.50	1.0272708
-	-	2.00	1.0281458
-	-	2.50	1.0301780

Table 5. The impact of $-S^{\prime }\left(0\right)$ for the distinct values of the sundry parameters.

$$\mathit{\phi }$$	$${\mathit{\beta }}_{\mathit{a}}$$	$$\mathit{L}\mathit{e}$$	$${\mathit{\delta }}_{\mathit{a}}$$	$${\mathit{E}}_{\mathit{a}}$$	Present Results
0.025	0.50	10	0.50	0.50	10.5067960
0.030	-	-	-	-	10.5067950
0.035	-	-	-	-	10.5067940
0.035	0.30	10	0.50	0.50	10.3097350
-	0.50	-	-	-	10.5067940
-	0.70	-	-	-	10.6971130
0.035	0.50	7.5	0.50	0.50	7.99799500
-	-	0.90	-	-	9.50379410
-	-	10.5	-	-	11.0081000
0.035	0.50	10	0.50	0.50	10.5067940
-	-	-	1.00	-	10.5067940
-	-	-	1.50	-	10.9238810
0.035	0.50	10	0.50	0.50	10.5067940
-	-	-	-	1.00	10.3670720
-	-	-	-	1.50	10.2648640

Table 5. The impact of $-S^{\prime }\left(0\right)$ for the distinct values of the sundry parameters.

$$\mathit{\phi }$$	$${\mathit{\beta }}_{\mathit{a}}$$	$$\mathit{L}\mathit{e}$$	$${\mathit{\delta }}_{\mathit{a}}$$	$${\mathit{E}}_{\mathit{a}}$$	Present Results
0.025	0.50	10	0.50	0.50	10.5067960
0.030	-	-	-	-	10.5067950
0.035	-	-	-	-	10.5067940
0.035	0.30	10	0.50	0.50	10.3097350
-	0.50	-	-	-	10.5067940
-	0.70	-	-	-	10.6971130
0.035	0.50	7.5	0.50	0.50	7.99799500
-	-	0.90	-	-	9.50379410
-	-	10.5	-	-	11.0081000
0.035	0.50	10	0.50	0.50	10.5067940
-	-	-	1.00	-	10.5067940
-	-	-	1.50	-	10.9238810
0.035	0.50	10	0.50	0.50	10.5067940
-	-	-	-	1.00	10.3670720
-	-	-	-	1.50	10.2648640

4.2. Research Analysis of the Velocity Curve Profiles

Figure 2, Figure 3 and Figure 4 illustrate the velocity profiles of the SAE50 plus zinc oxide nano-lubricants for the single branch solutions due to the influence of $\phi$, $K_a$, and ${\epsilon }_b$, respectively. From the tendency of the graphical outcomes, it is perceived that the curves of velocity fulfill the criterion of asymptotic convergence, and hence mathematically satisfy the appropriate BCs owing to the successive impact of the influential parameters. To make this point clearer, the results in Figure 2 show that the velocity field curves constantly decelerate as the value of $\phi$ rises. In general, the viscosity impact is improved by the solid nanoparticle volume fraction, as a result, the WJ flow velocity decreases. Alternatively, the curves of velocity primarily decelerate and then accelerate with bigger values of $K_a$ and ${\epsilon }_b$ as elaborated in the respective Figure 3 and Figure 4. According to the basic concept of physics, if the value of $K_a$ rises, as a response, the value of ${\upsilon }_f$ also rises. Thus, the larger viscosity of the fluid arises with the higher impact of $K_a$ which physically slows down the speed of the WJ flow, in conclusion, the velocity $F^{\prime }\left(\xi \right)$ decelerates. Similarly, following the above explanation for the modified porosity parameter, one obtains ${\epsilon }_b={\upsilon }_{eff}{\epsilon }_a{}^2/{\alpha }_f$. Additionally, the space between the curves for larger $K_a$ is well enough than the space that appeared in the curves for the larger consequences of ${\epsilon }_b$.

Figure 2. The velocity profiles $F^{\prime }\left(\xi \right)$ for the change values of $\phi$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $R_d=2.0$, and $E_a=0.5$.

Figure 2. The velocity profiles $F^{\prime }\left(\xi \right)$ for the change values of $\phi$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $R_d=2.0$, and $E_a=0.5$.

Figure 3. The velocity profiles $F^{\prime }\left(\xi \right)$ for the change values of $K_a$ when $m=1.0$, $\phi =0.035$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 3. The velocity profiles $F^{\prime }\left(\xi \right)$ for the change values of $K_a$ when $m=1.0$, $\phi =0.035$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 4. The velocity profiles $F^{\prime }\left(\xi \right)$ for the change values of ${\epsilon }_b$ when $m=1.0$, $\phi =0.035$, $f_w=1.0$, $K_a=0.5$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 4. The velocity profiles $F^{\prime }\left(\xi \right)$ for the change values of ${\epsilon }_b$ when $m=1.0$, $\phi =0.035$, $f_w=1.0$, $K_a=0.5$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

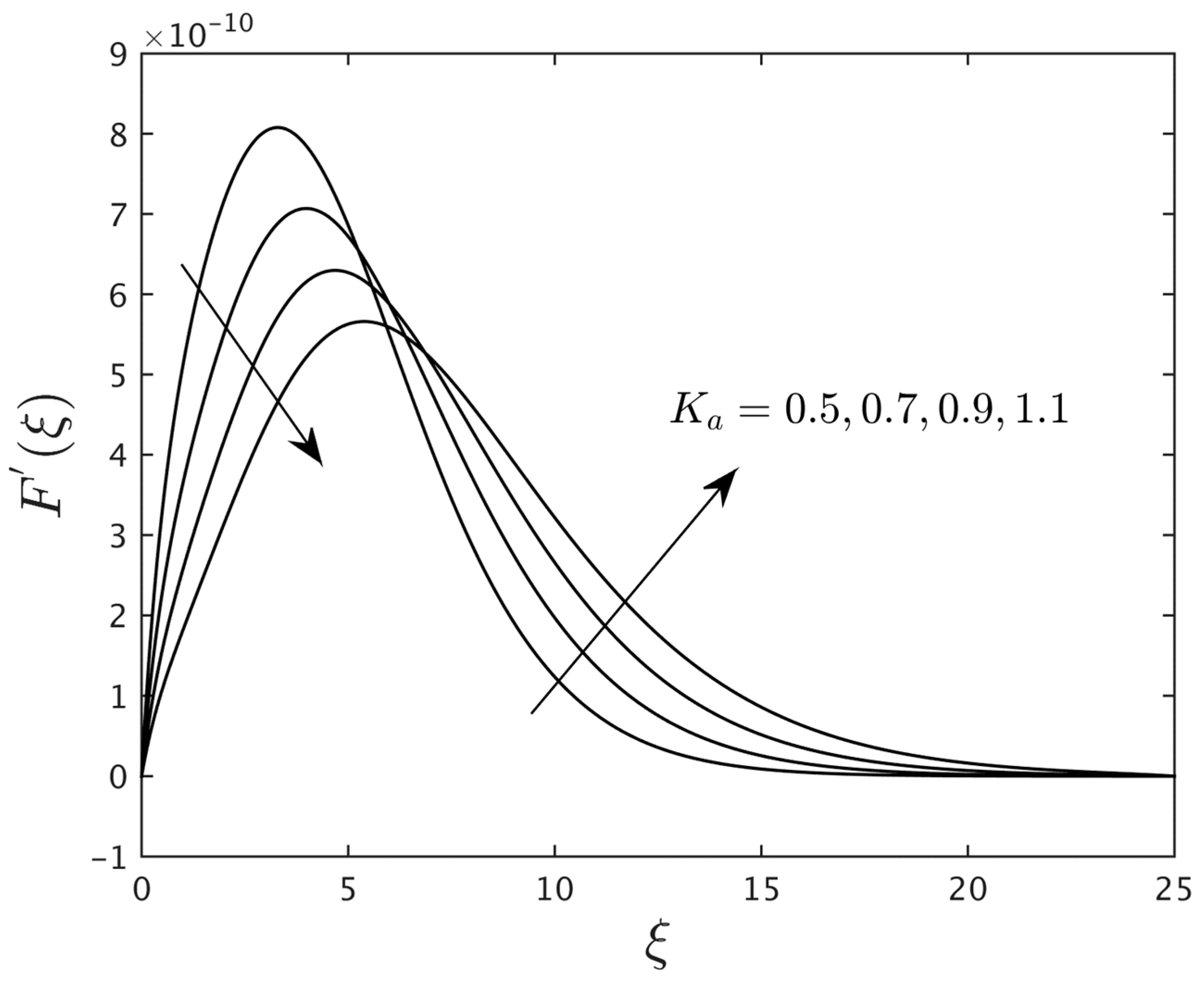
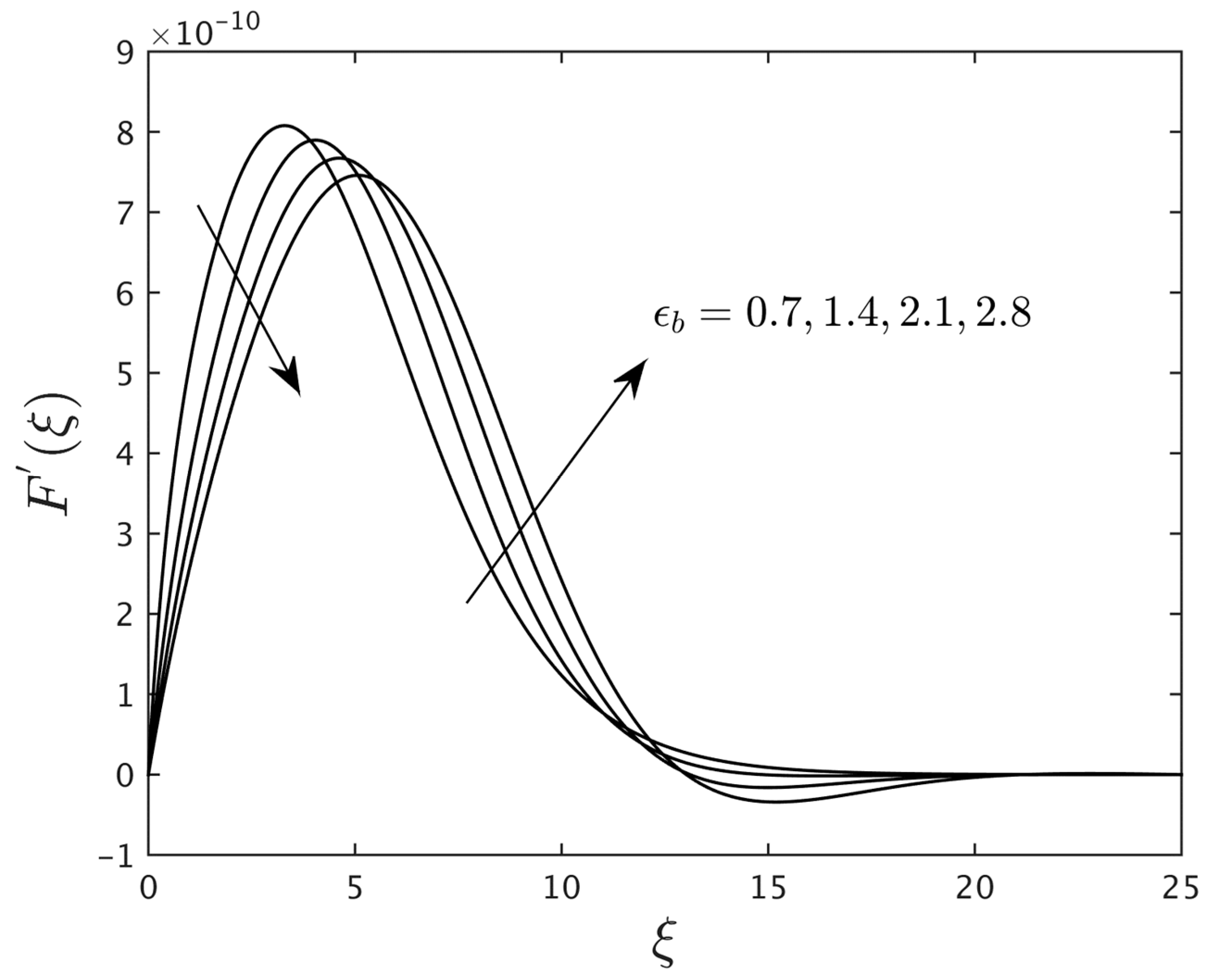
4.3. Research Analysis of the Temperature Curve Profiles

The impacts of $\phi$ and $R_d$ on $G\left(\xi \right)$ of the SAE50 plus zinc oxide (ZnO) nano-lubricants for the single branch solutions are exhibited in Figure 5 and Figure 6, respectively. From the results, it is deduced that the temperature curves and the thermal boundary layer thickness (TBLT) are constantly improved with the larger influence of $\phi$ and $R_d$. In the physical scenario, increasing radiation results in the release of extra energy into the wall jet flow, which leads to the development of the temperature field and the TBLT. Moreover, the gap between the curves is less in Figure 5 as compared to Figure 6 due to the larger values of $\phi$ and $R_d$.

Figure 5. The temperature profiles $G\left(\xi \right)$ for the change values of $\phi$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $R_d=2.0$, and $E_a=0.5$.

Figure 5. The temperature profiles $G\left(\xi \right)$ for the change values of $\phi$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $R_d=2.0$, and $E_a=0.5$.

Figure 6. The temperature profiles $G\left(\xi \right)$ curves for sundry choices of $R_d$ when ${\epsilon }_b=0.7$, $K_a=0.5$, $f_w=1.0$, $Le=10$, $m=1.0$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $\phi =0.035$.

Figure 6. The temperature profiles $G\left(\xi \right)$ curves for sundry choices of $R_d$ when ${\epsilon }_b=0.7$, $K_a=0.5$, $f_w=1.0$, $Le=10$, $m=1.0$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $\phi =0.035$.

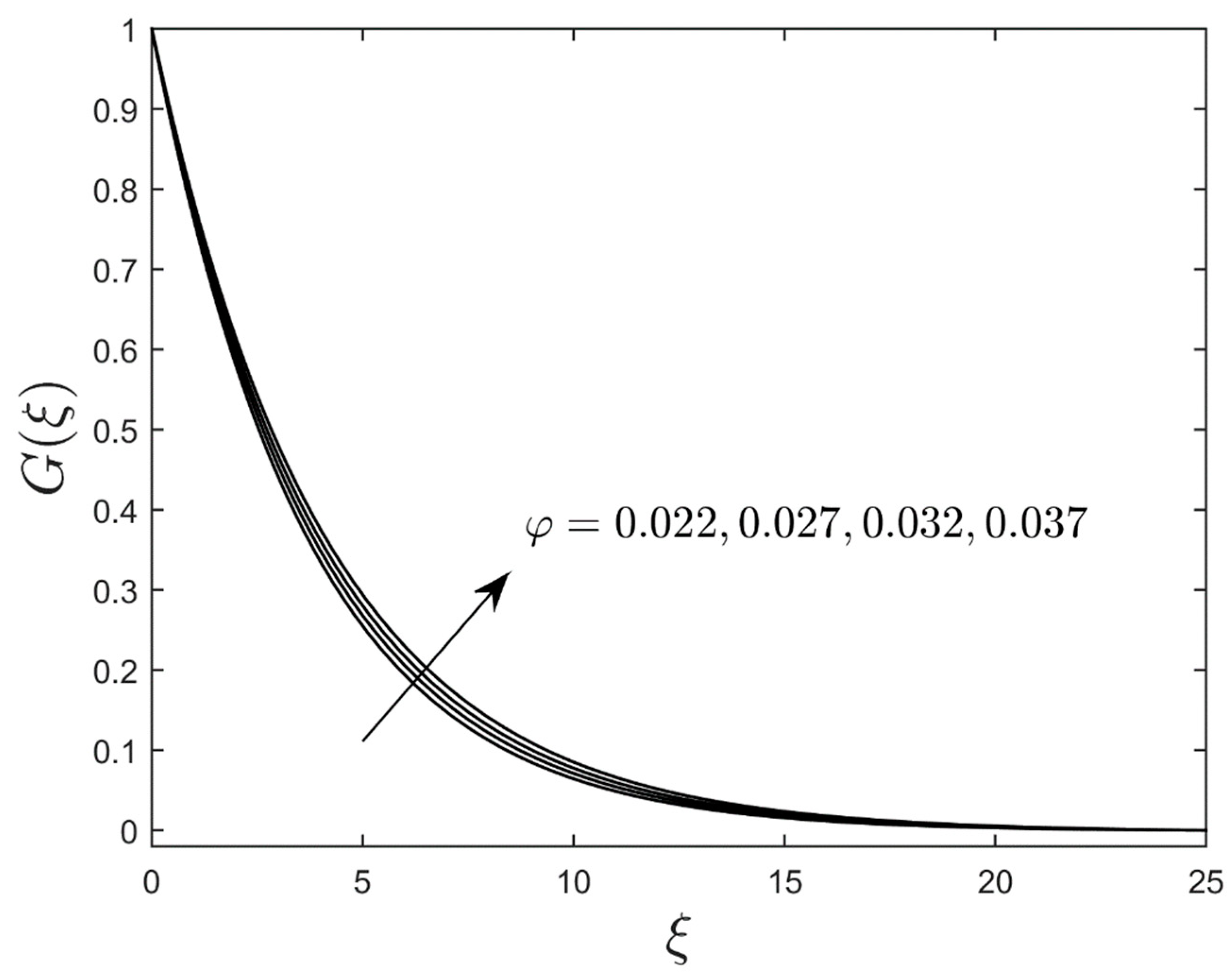
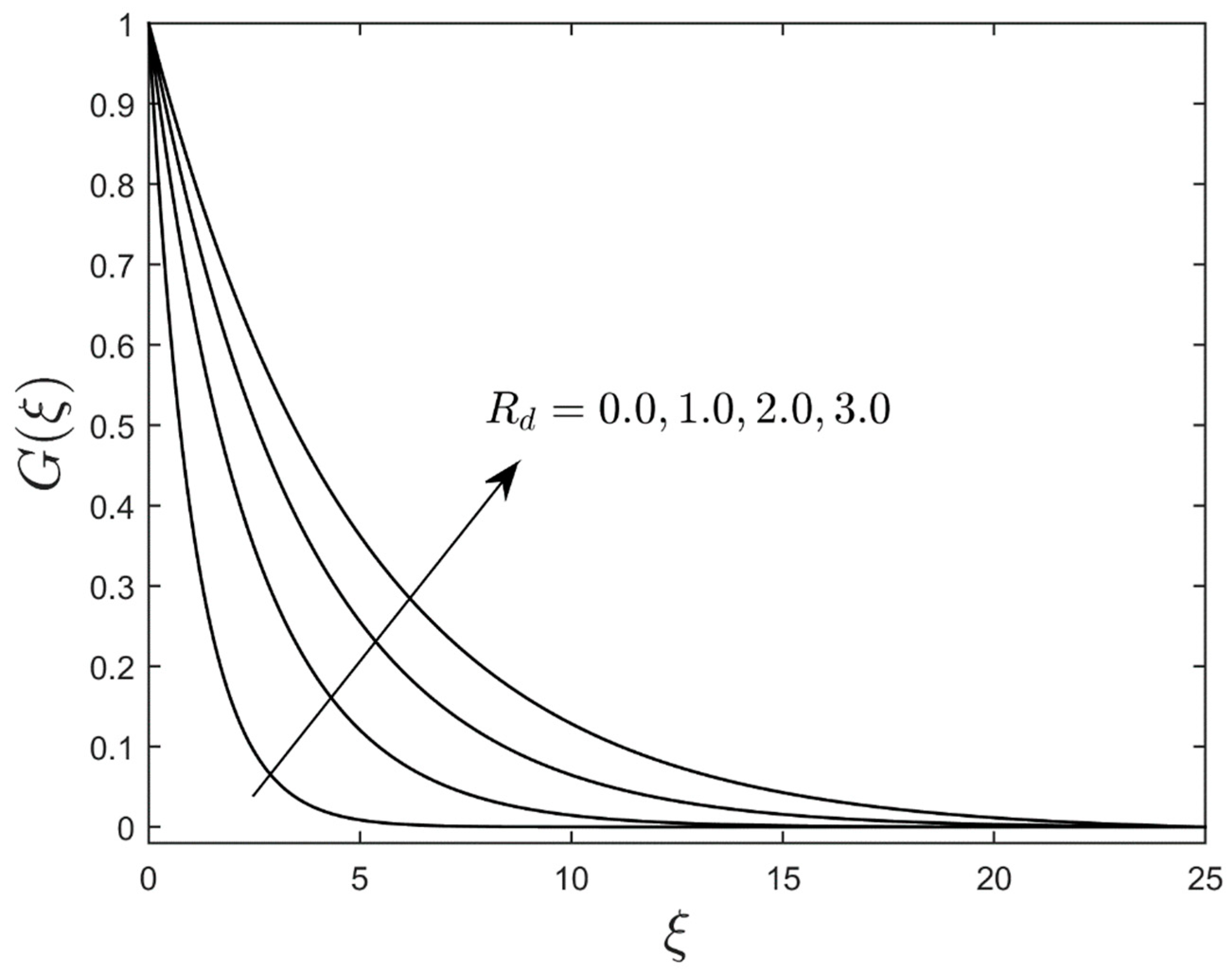
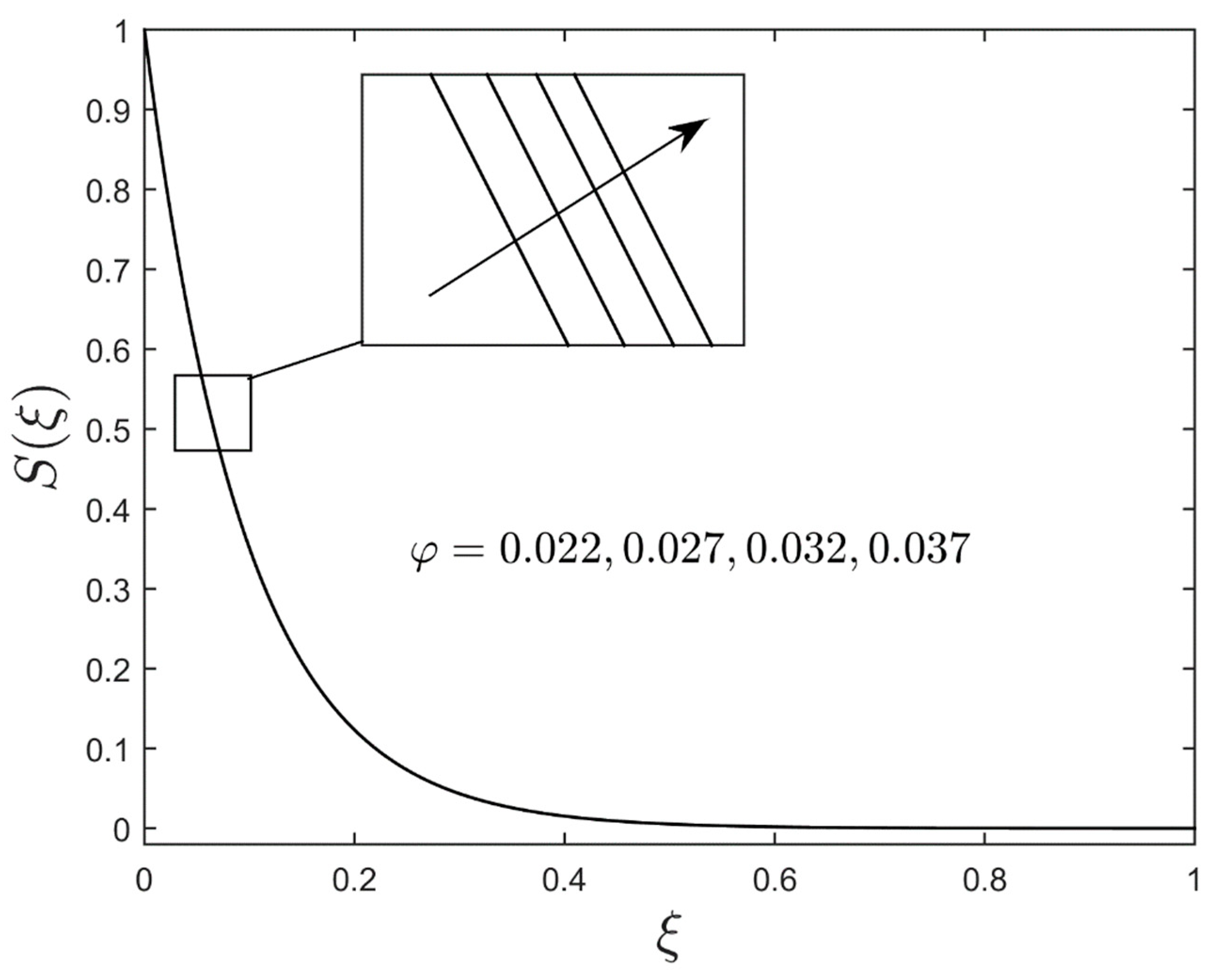
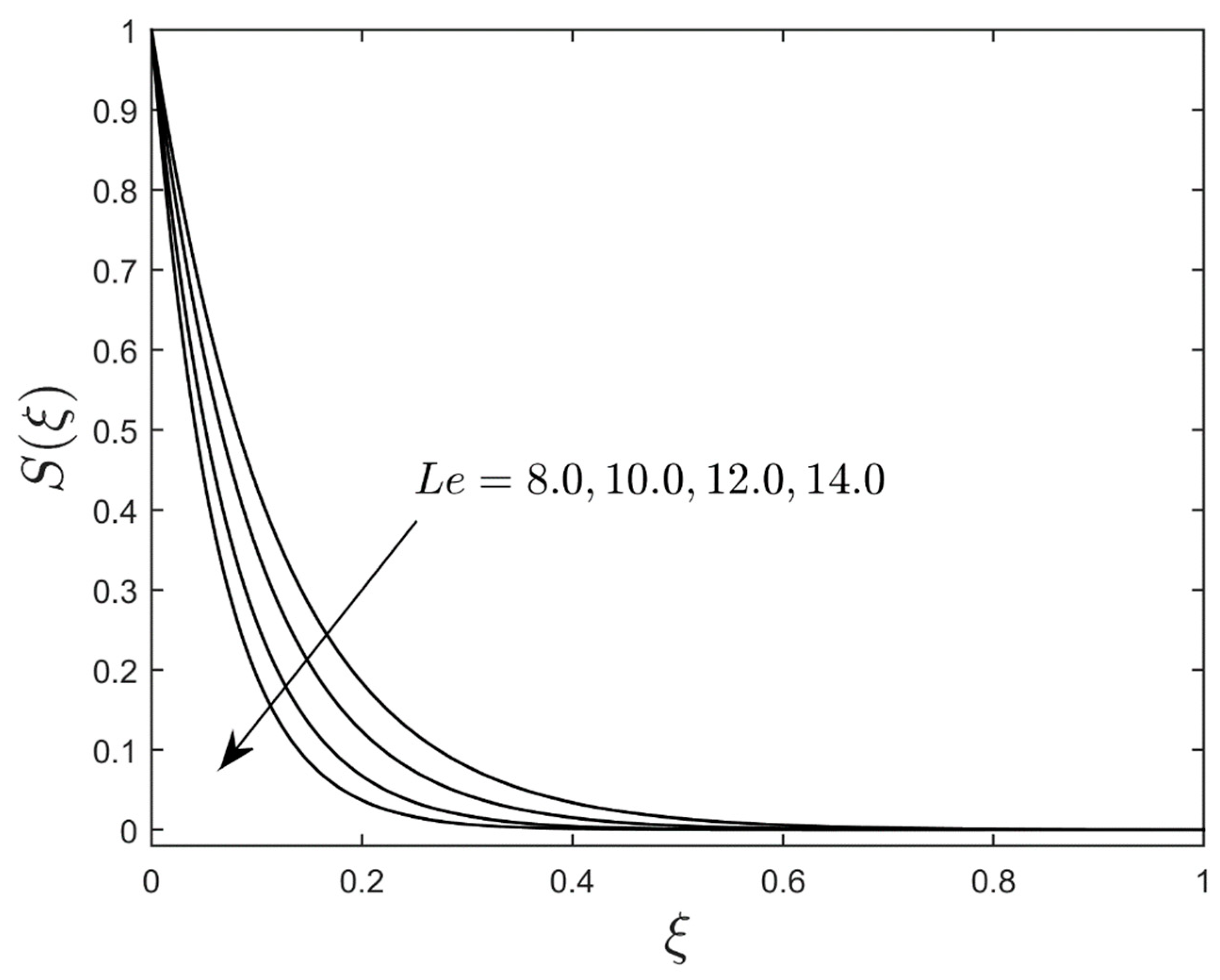
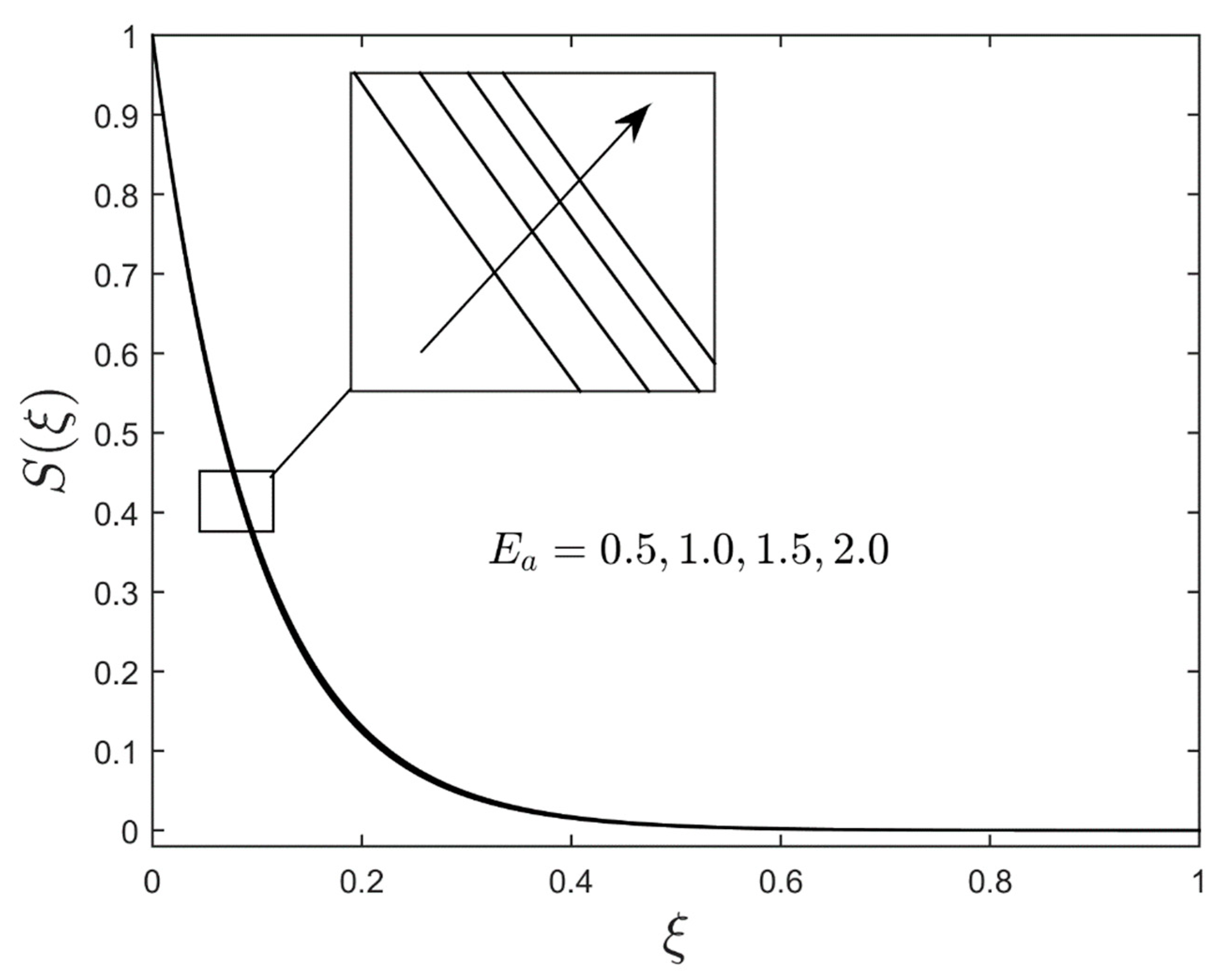
4.4. Research Analysis of the Concentration Curve Profiles

Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11 exemplify the concentration profile $S\left(\xi \right)$ of the SAE50 plus zinc oxide nano-lubricants for the single branch results owing to the influence of $\phi$, $Le$, ${\beta }_a$, ${\delta }_a$, and $E_a$, respectively. In all the graphs, it is realized that the pertinent BCs are satisfied and the pattern of the outputs converges asymptotically for varying the several distinguished constraints. Moreover, Figure 7 illustrates the effect of $\phi$ on the $S\left(\xi \right)$. For a rising value of $\phi$, the concentration profile of the wall jet flow escalates. Figure 8, Figure 9, Figure 10 and Figure 11, it is evident that the upsurging values of $Le$, ${\beta }_a$ and ${\delta }_a$ reduce the dimensionless concentration profiles of the wall jet flow, respectively, while it intensifies with the higher impacts of $E_a$. In Figure 8 such a pattern of solution curves happens due to the fact that the mass diffusivity of the fluid reduces with the huge effects of the Lewis number, as a result, the fluid is less concentrated at the surface of the wall jet. However, the activation and chemical reaction term ${\beta }_a{\left(1+{\delta }_{a}G\right)}^m\exp \left(-E_a/1+{\delta }_{a}G\right)S$ in the requisite concentration similarity equation (16) was written with the negative sign which further shows that whenever we increase the impact of ${\beta }_a$ and ${\delta }_a$, as a result, the concentration decelerates (see Figure 9 and Figure 10). In addition, for Figure 11, this exponential term such as $\exp \left(-E_a/1+{\delta }_{a}G\right)S$ is an exceptional tool that how the AAE disturbs $S\left(\xi \right)$. Henceforth, due to a superior impact of $E_a$, the thickness of the CBL and the concentration $S\left(\xi \right)$ upsurges.

Figure 7. The concentration profiles $S\left(\xi \right)$ for the change values of $\phi$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 7. The concentration profiles $S\left(\xi \right)$ for the change values of $\phi$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 8. The concentration profiles $S\left(\xi \right)$ for the change values of $Le$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $\phi =0.035$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 8. The concentration profiles $S\left(\xi \right)$ for the change values of $Le$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $\phi =0.035$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 9. The concentration profiles $S\left(\xi \right)$ for the change values of ${\beta }_a$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, $\phi =0.035$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 9. The concentration profiles $S\left(\xi \right)$ for the change values of ${\beta }_a$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, $\phi =0.035$, ${\delta }_a=0.5$, $E_a=0.5$, and $R_d=2.0$.

Figure 10. The concentration profiles $S\left(\xi \right)$ for the change values of ${\delta }_a$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, $\phi =0.035$, $E_a=0.5$, and $R_d=2.0$.

Figure 10. The concentration profiles $S\left(\xi \right)$ for the change values of ${\delta }_a$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, $\phi =0.035$, $E_a=0.5$, and $R_d=2.0$.

Figure 11. The concentration profiles $S\left(\xi \right)$ for the change values of $E_a$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $\phi =0.035$, and $R_d=2.0$.

Figure 11. The concentration profiles $S\left(\xi \right)$ for the change values of $E_a$ when $m=1.0$, $K_a=0.5$, ${\epsilon }_b=0.7$, $f_w=1.0$, $Le=10$, ${\beta }_a=0.5$, ${\delta }_a=0.5$, $\phi =0.035$, and $R_d=2.0$.

5. Conclusions

The thermal radiative heat and mass transfer analysis of 2D WJ flows conveying SAE50-ZnO nano-lubricants with mass transpiration or suction/injection velocity, and chemical reaction has been investigated. Moreover, the AAE and the Darcy-Brinkman porous medium model are also added to the investigation. The governing equations are transformed to the set of non-linear ODEs using the Glauert WJ variables, which are then workout computationally via the effective bvp4c scheme. The following are the main outcomes of the present study:

• The velocity curves of the WJ flow moderate with a superior impact of the nanoparticle volume fraction but the temperature and concentration profile curves are enhanced.
• The modified porosity parameter and the dimensionless permeability parameter impact initially decay the motion of the wall jet flow and then abruptly augmented the velocity.
• With the increasing value of the radiation parameter, the temperature profiles and the thickness of the thermal boundary layer developed.
• The concentration enriches with a higher impact of activation energy but shrinkages with $Le$, ${\beta }_a$ and ${\delta }_a$.
• The friction factor upsurges and magnitude-wise declines due to the larger impressions of the mass suction factor and mass blowing factor, respectively.
• The rate of heat transfer is boosted due to the higher influences of the nanoparticle volume fraction while the mass transfer rate decelerates.