On the Direct Extrusion of Solder Wire from 52In-48Sn Alloy
Abstract
:1. Introduction
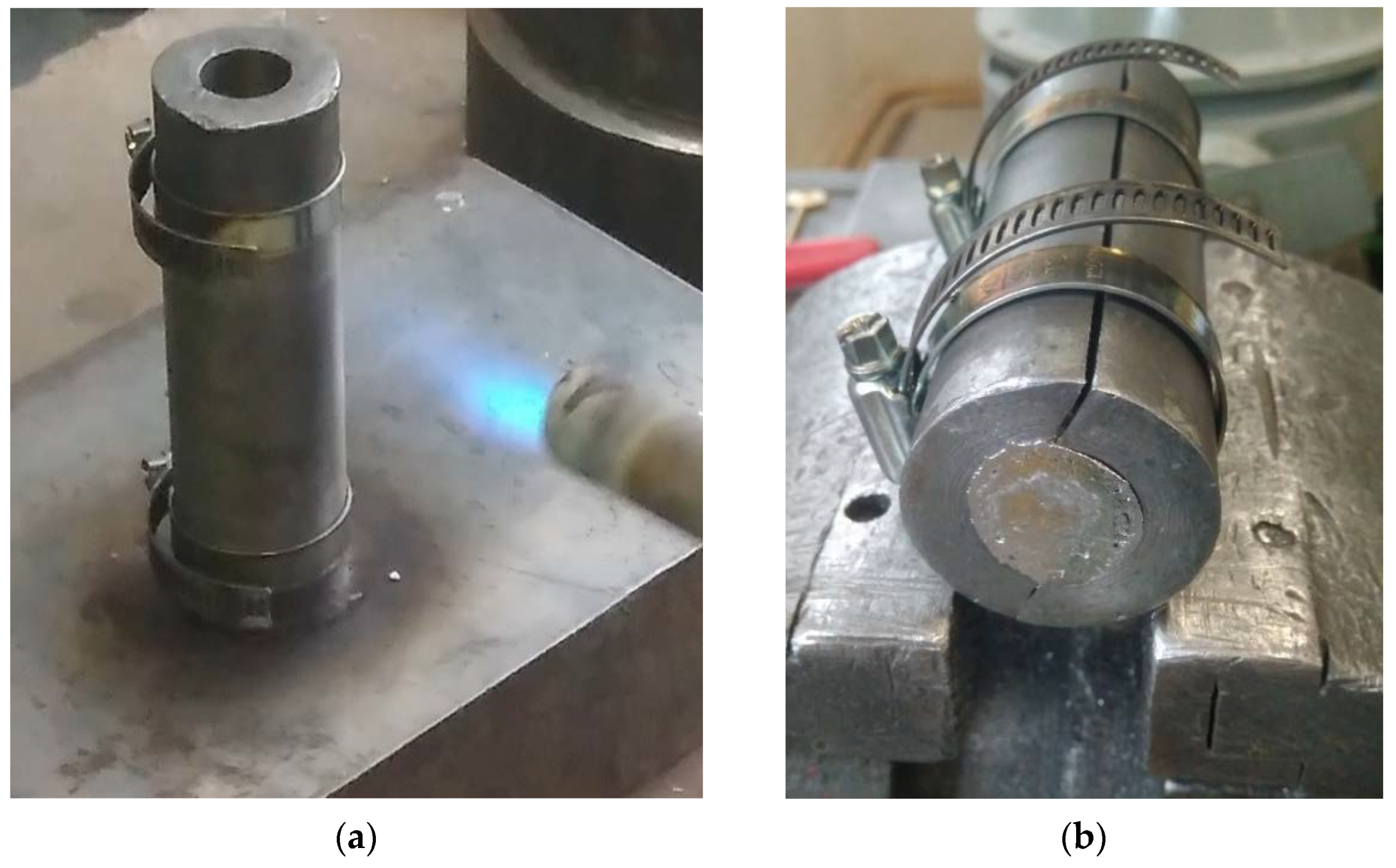
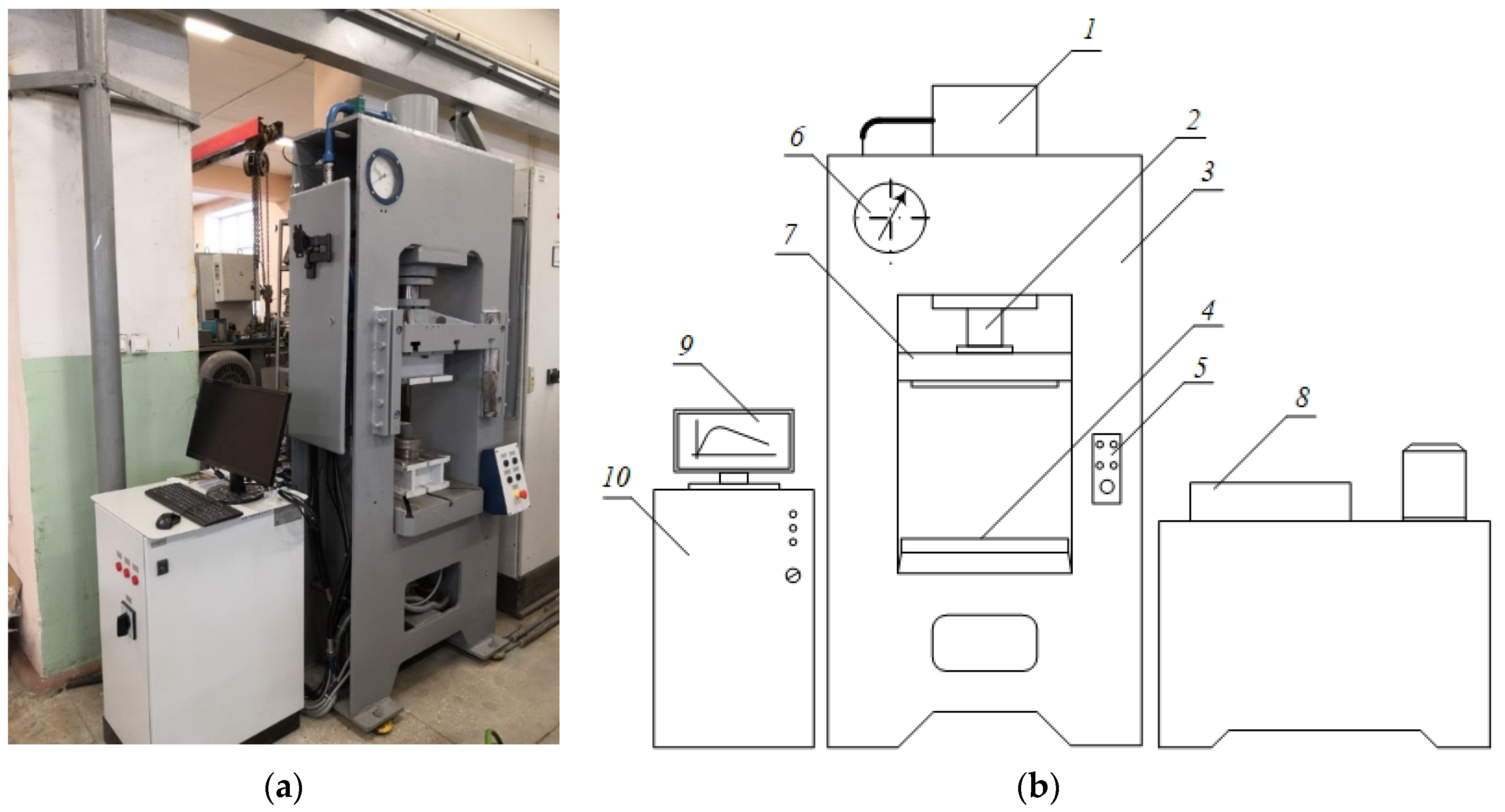
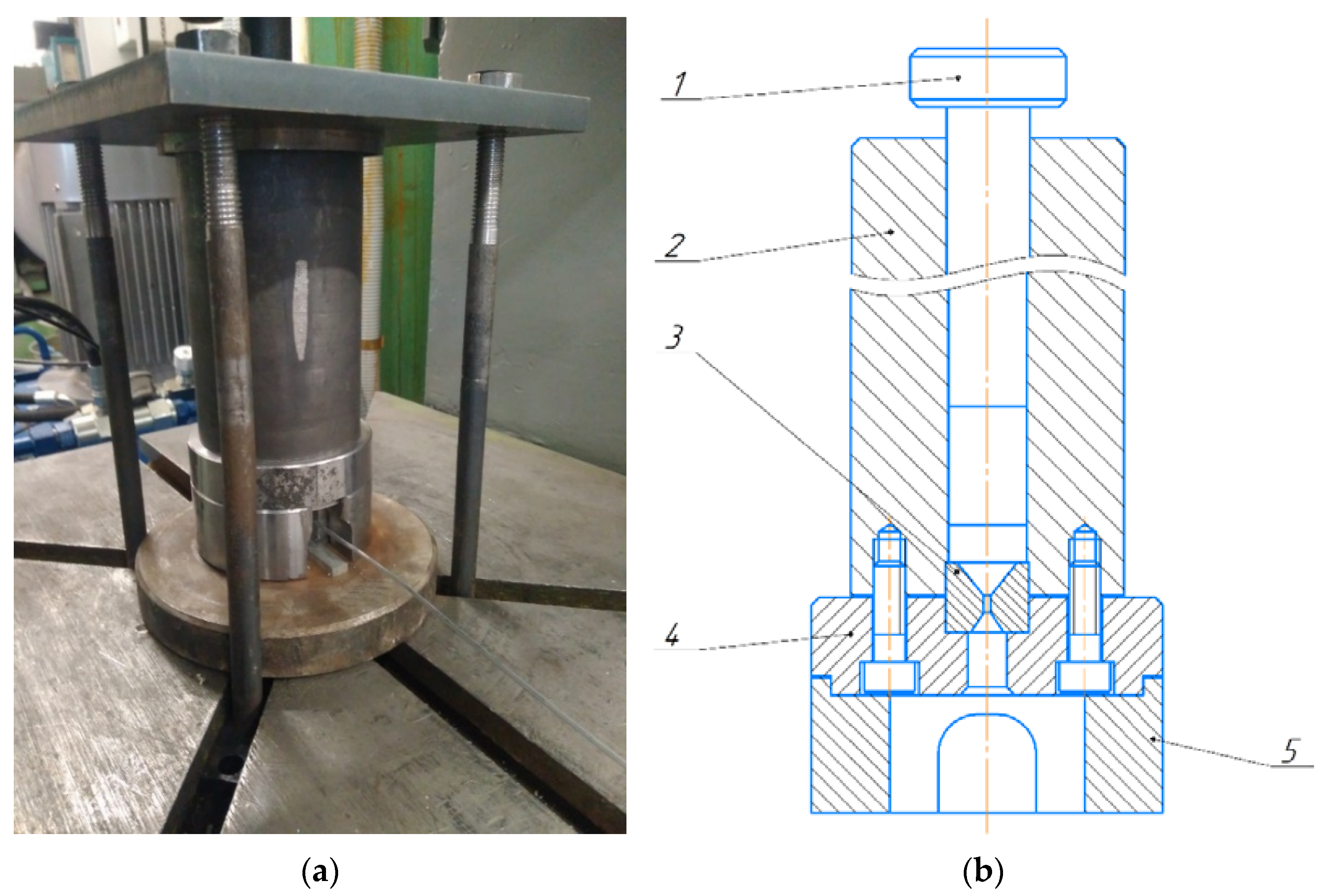
2. Materials and Methods
3. Results and Discussion
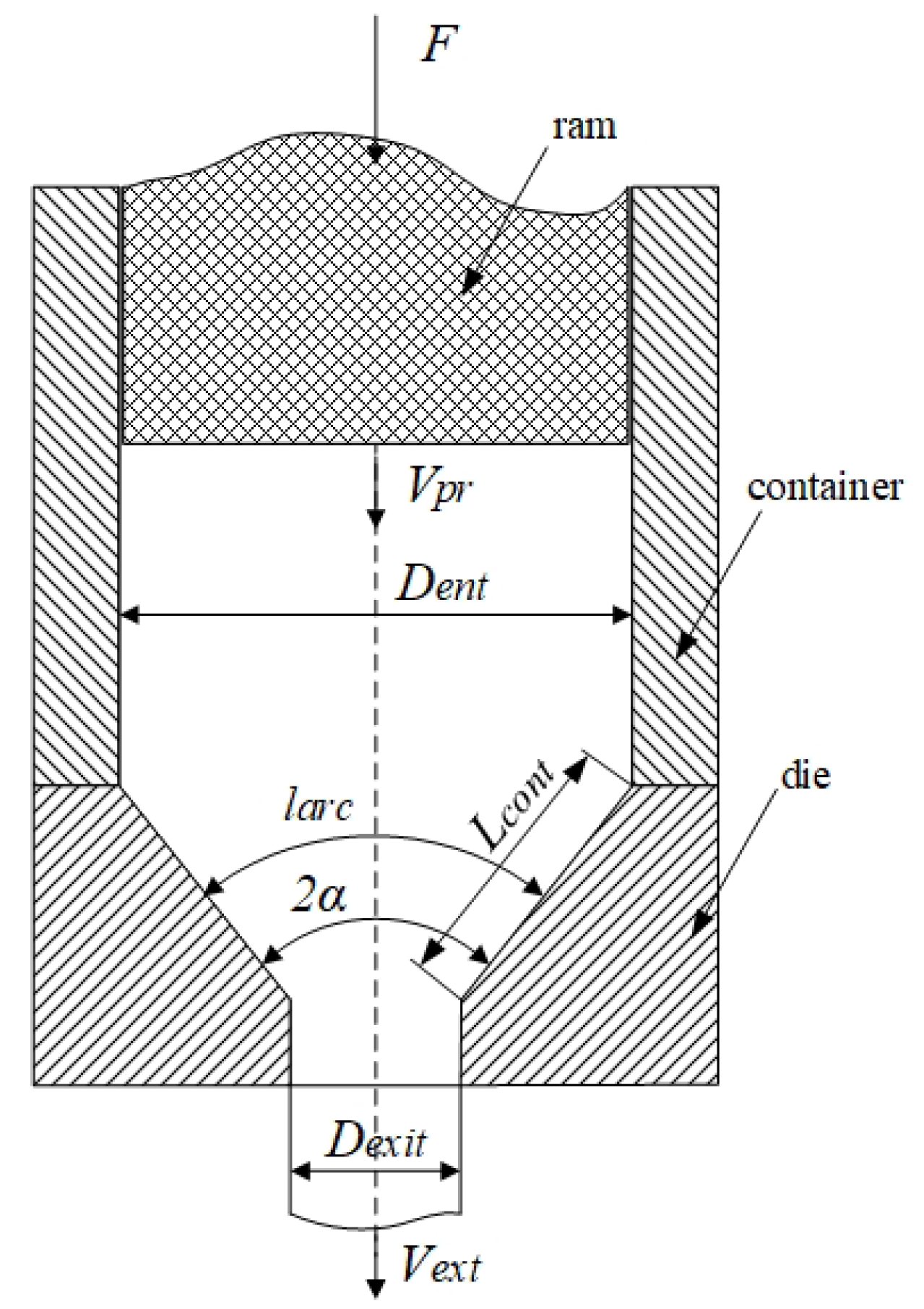
3.1. Mathematical Modeling for the Extrusion Process
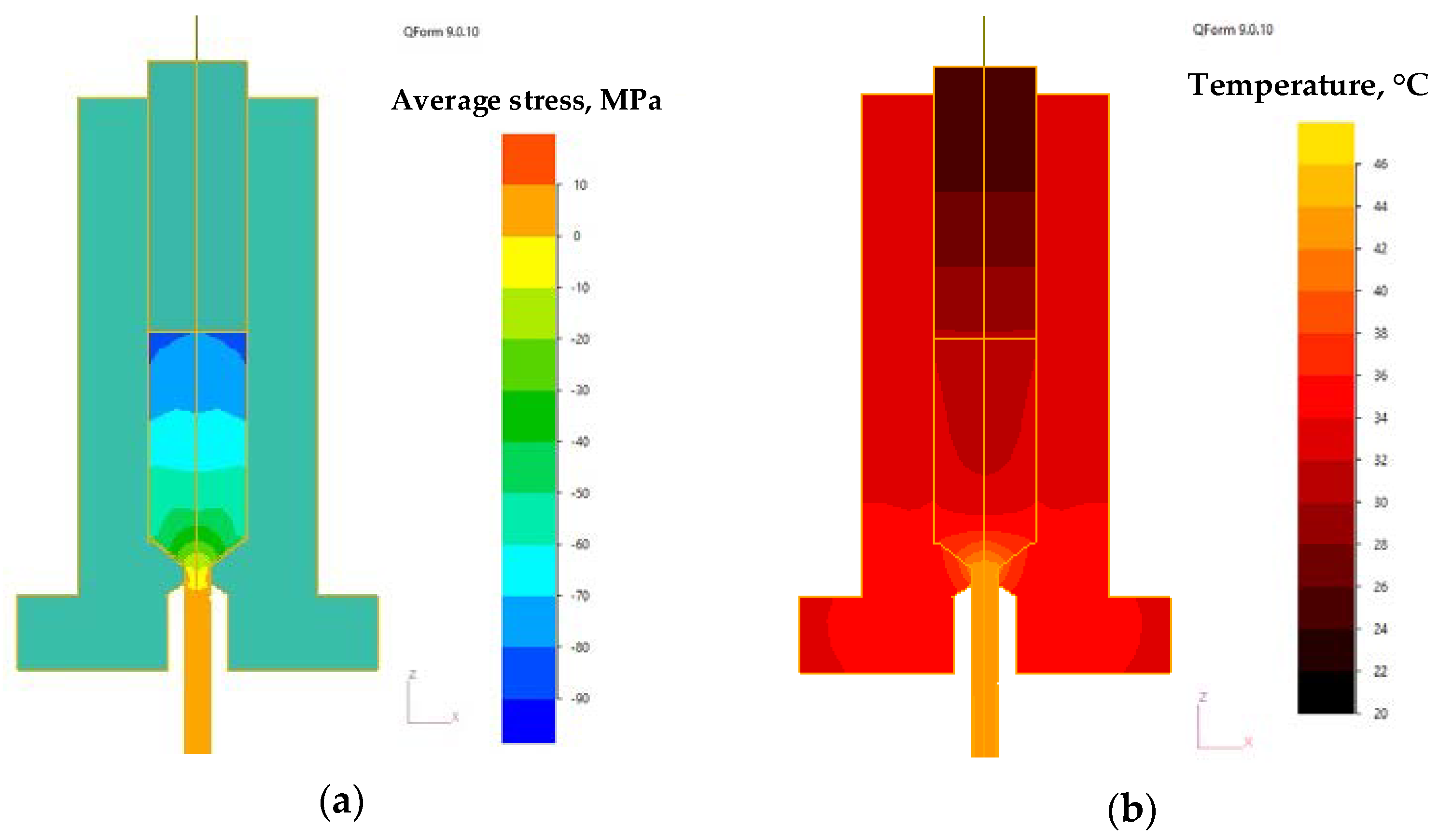
3.2. Extrusion Temperature Simulation in QForm Software
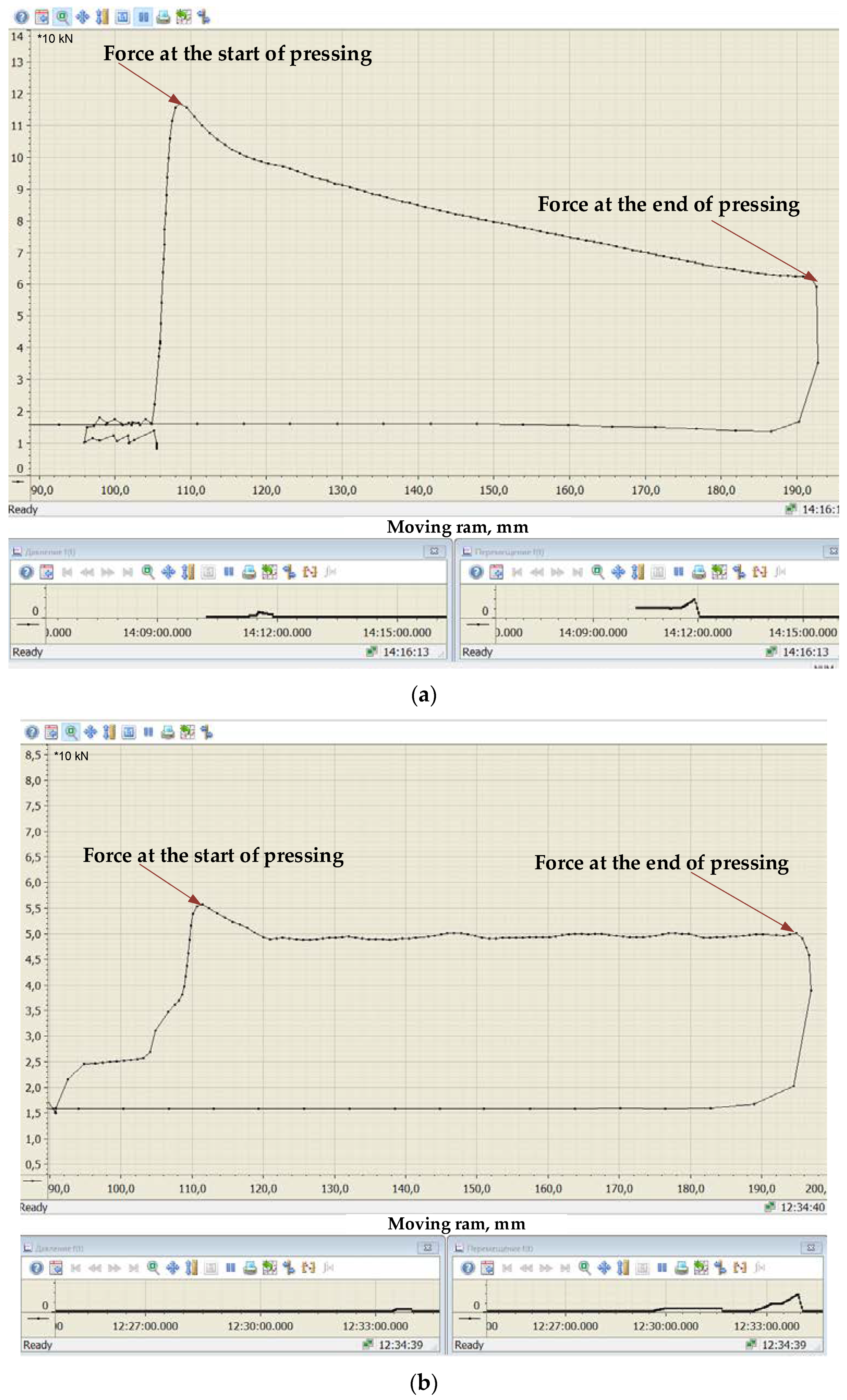
3.3. Experimental Studies of Wire and Rods Extrusion
4. Conclusions
- A mathematical model of direct extrusion has been developed, which allows us to calculate extrusion ratio, extrusion speed and pressing force. The discrepancy between the calculated and experimental results does not exceed 10%.
- In mathematical modeling, it was found that a decrease in the friction coefficient from 0.4 to 0.1 reduces the force at the start of pressing from 97 to 71 kN when extruding a 2.0 mm wire and from 94 to 54 kN when extruding a rod of Ø8.0 mm.
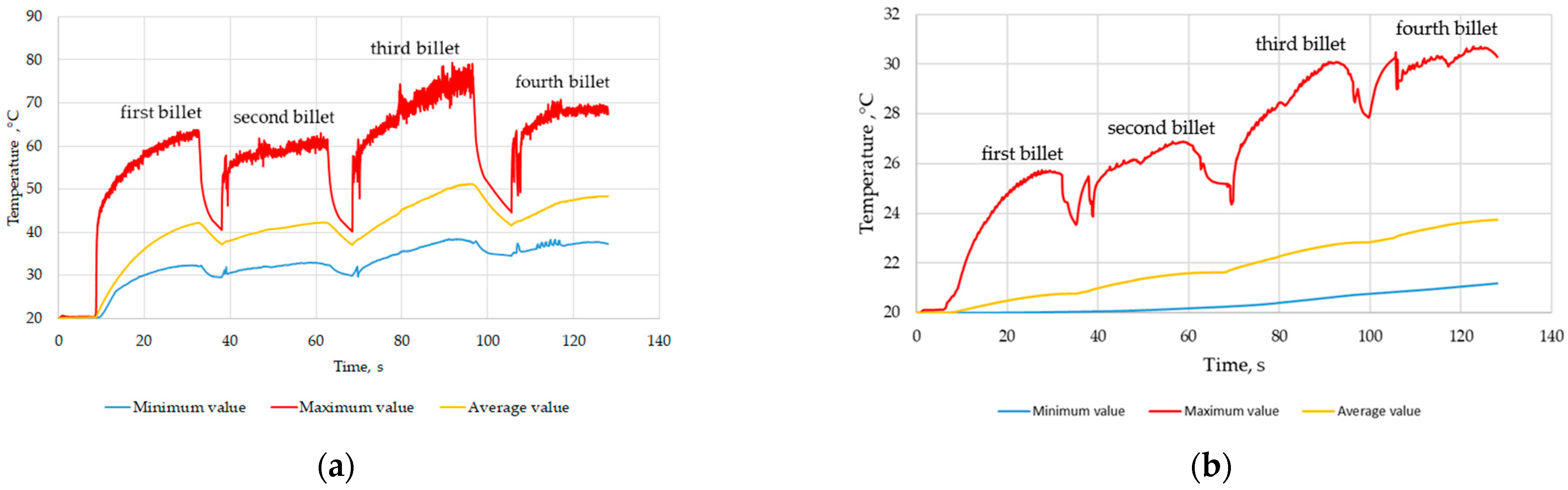
- The temperature of the solder and the tool the extrusion process is determined by simulation in software QForm, based on the finite element method. With an increase in the pressing speed from 0.5 to 3 mm/s, the maximum temperature of the Ø2 mm solder increases by 2.2 times and 1.3 times when pressing a rod Ø8.0 mm. The temperature with an increase in the pressing speed from 0.5 to 3.0 mm/s increases from 33.4 to 75.8 °C for 2.0 mm wire and from 22.5 to 30 °C for Ø8.0 mm rod. With successive pressing of four billets, the maximum temperature of Ø2.0 mm wire of solder increases to 79 °C, and the maximum temperature of the container increases by 10 °C.
- An experimental study has established that the use of graphite lubricant makes it possible to reduce the friction coefficient from 0.5 to 0.05, which reduces the temperature of the solder at the die exit from 30 to 22 °C when manufacturing a rod Ø8.0 mm at a pressing speed of 3 mm/s.
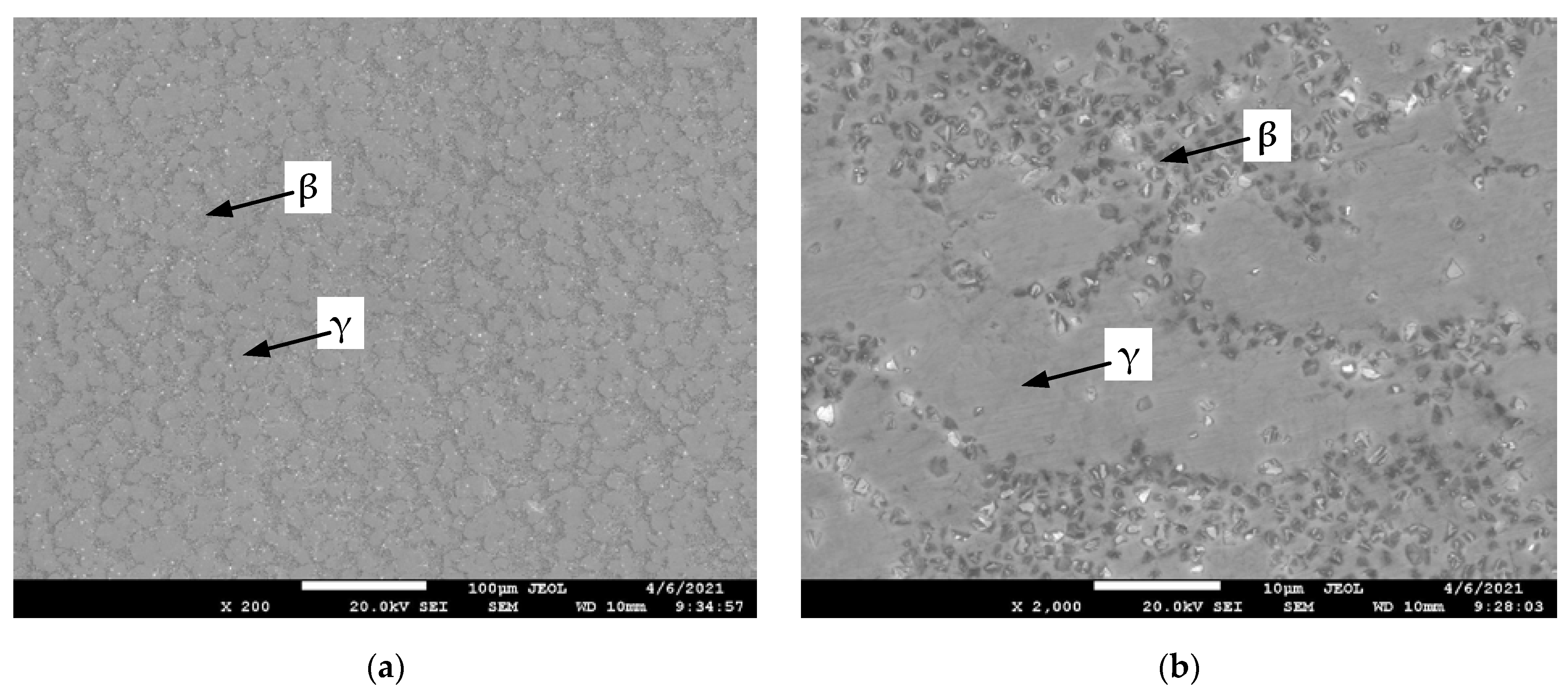
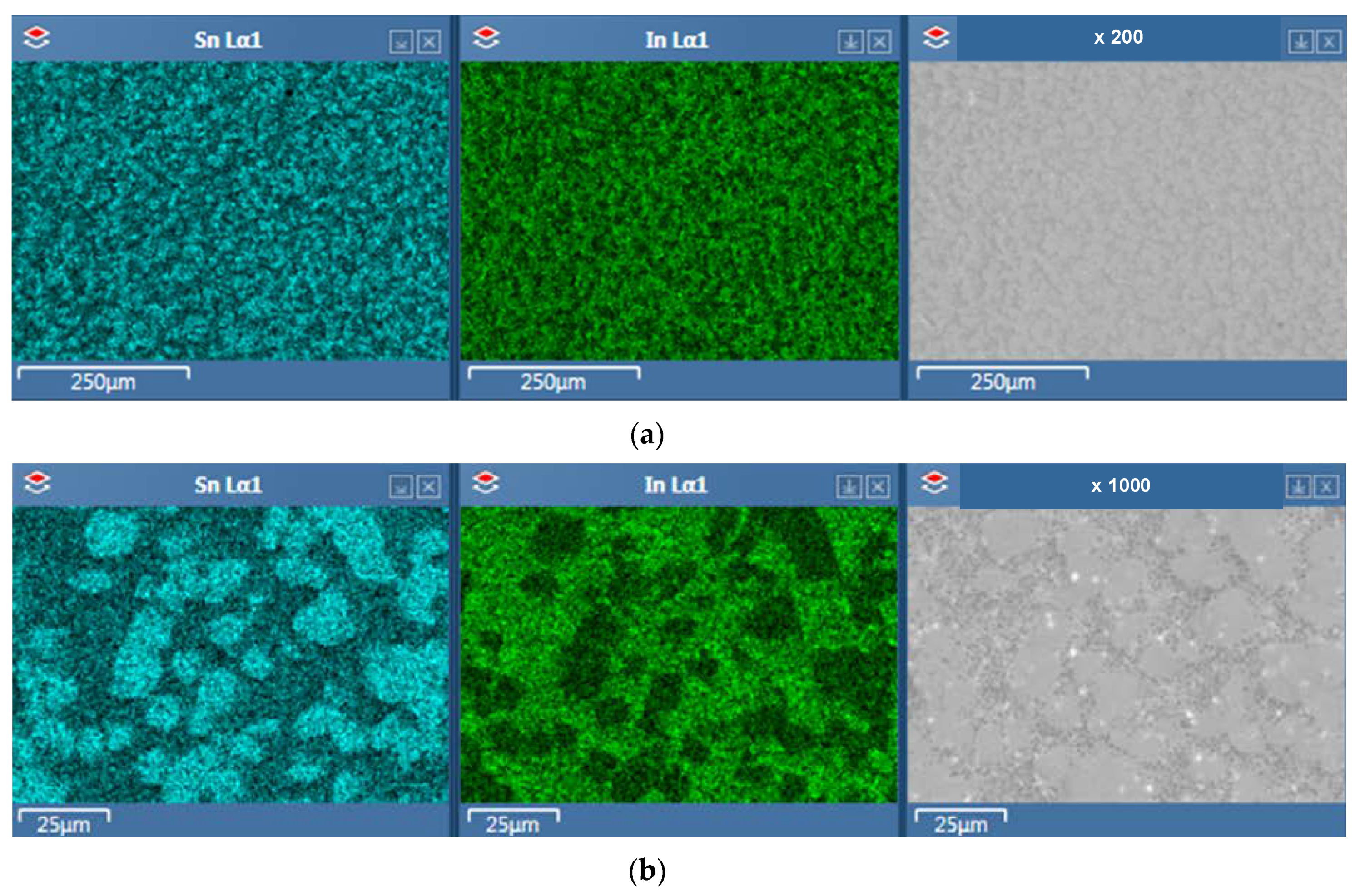
- Visual and microstructural analysis of the rod and wire showed their compliance with the customer’s requirements. The microstructure is a eutectic of phases γ and β. Energy-dispersive X-ray spectroscopy (EDS) mapping of the 52In-48Sn alloy showed that the solder obtained by direct extrusion has a uniform distribution of structural phases.
- The developed technology can be used in the manufacture of wires and rods from other low-melting alloys. In continuation of the work already done, it is planned to improve the tools and technology for obtaining rosin core solder wire.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Arabian, J. Computer Integrated Electronics Manufacturing and Testing, 1st ed.; Marcel Dekker, Inc.: New York, NY, USA, 1989. [Google Scholar] [CrossRef]
- Abtew, M.; Selvaduray, G. Lead-free solders in microelectronics. Mater. Sci. Eng. R Rep. 2000, 27, 95–141. [Google Scholar] [CrossRef]
- Raeder, C.; Felton, L.; Tanzi, V.; Knorr, D. The effect of aging on microstructure, room temperature deformation, and fracture of Sn-Bi/Cu solder joints. J. Electron. Mater. 1994, 23, 611–617. [Google Scholar] [CrossRef]
- Sommadossi, S.; Fernández Guillermet, A. Interface reaction systematics in the Cu/In–48Sn/Cu system bonded by diffusion soldering. Intermetallics 2007, 15, 912–917. [Google Scholar] [CrossRef]
- Yang, C.-H.; Zhou, S.; Lin, S.-K.; Nishikawa, H. A Computational Thermodynamics-Assisted Development of Sn-Bi-In-Ga Quaternary Alloys as Low-Temperature Pb-Free Solders. Materials 2019, 12, 631. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Tu, K.N. Low melting point solders based on Sn, Bi, and in elements. Mater. Today Adv. 2020, 8, 100115. [Google Scholar] [CrossRef]
- Chriašteľová, J.; Ožvold, M. Properties of solders with low melting point. J. Alloys Compd. 2008, 457, 323–328. [Google Scholar] [CrossRef]
- Kotadia, H.R.; Howes, P.D.; Mannan, S.H. A review: On the development of low melting temperature Pb-free solders. Microelectron. Reliab. 2014, 54, 1253–1273. [Google Scholar] [CrossRef]
- Kang, H.; Rajendran, S.H.; Jung, J.P. Low Melting Temperature Sn-Bi Solder: Effect of Alloying and Nanoparticle Addition on the Microstructural, Thermal, Interfacial Bonding, and Mechanical Characteristics. Metals 2021, 11, 364. [Google Scholar] [CrossRef]
- Efimochkin, Y.I.; Fedotov, S.V.; Rylnikov, V.S.; Afanasev-Khodykin, A.N. High-temperature brazing alloys produced by mechanical alloying. Weld. Int. 2016, 30, 229–231. [Google Scholar] [CrossRef]
- Rahman, M.M.; Ahmed, S.R.; Kaiser, M.S. On the investigation of reuse potential of SnPb-solder affected copper subjected to work-hardening and thermal ageing. Mater. Charact. 2021, 172, 110878. [Google Scholar] [CrossRef]
- EU. Directive 2002/95/EC of the European parliament and of the council of 27 January 2003 on the restriction of the use of certain hazardous substances in electrical and electronic equipment. Off. J. Eur. Union 2003, 13, 19–22. [Google Scholar]
- Gain, A.K.; Zhang, L.; Chan, Y. Microstructure, elastic modulus and shear strength of alumina (Al2O3) nanoparticles-doped tin–silver–copper (Sn–Ag–Cu) solders on copper (Cu) and gold/nickel (Au/Ni)-plated Cu substrates. J. Mater. Sci. Mater. Electron. 2015, 26, 7039–7048. [Google Scholar] [CrossRef]
- Gnecco, F.; Ricci, E.; Amore, S.; Giuranno, D.; Borzone, G.; Zanicchi, G.; Novakovic, R. Wetting behaviour and reactivity of lead free Au–In–Sn and Bi–In–Sn alloys on copper substrates. Int. J. Adhes. Adhes. 2007, 27, 409–416. [Google Scholar] [CrossRef]
- Gain, A.K.; Zhang, L.; Quadir, M.Z. Thermal aging effects on microstructures and mechanical properties of an environmentally friendly eutectic tin-copper solder alloy. Mater. Des. 2016, 110, 275–283. [Google Scholar] [CrossRef]
- Gain, A.K.; Zhang, L. Microstructure, thermal analysis and damping properties of Ag and Ni nano-particles doped Sn–8Zn–3Bi solder on OSP–Cu substrate. J. Alloys Compd. 2014, 617, 779–786. [Google Scholar] [CrossRef]
- Gain, A.K.; Zhang, L. Growth mechanism of intermetallic compound and mechanical properties of nickel (Ni) nanoparticle doped low melting temperature tin–bismuth (Sn–Bi) solder. J. Mater. Sci. Mater. Electron. 2016, 27, 781–794. [Google Scholar] [CrossRef]
- Morris, J.W.; Goldstein, J.L.F.; Mei, Z. Microstructure and mechanical properties of Sn-In and Sn-Bi solders. JOM 1993, 45, 25–27. [Google Scholar] [CrossRef]
- Okamoto, H. Binary Alloy Phase Diagrams; ASM International: Materials Park, OH, USA, 1990; Volume 3, pp. 2295–2296. [Google Scholar]
- Chuang, T.H.; Yu, C.L.; Chang, S.Y.; Wang, S.S. Phase identification and growth kinetics of the intermetallic compounds formed during in-49Sn/Cu soldering reactions. J. Electron. Mater. 2002, 31, 640–645. [Google Scholar] [CrossRef]
- Freer, J.L.; Morris, J.W. Microstructure and creep of eutectic indium/tin on copper and nickel substrates. J. Electron. Mater. 1992, 21, 647–652. [Google Scholar] [CrossRef]
- Requirements for Electronic Grade Solder Alloys and Fluxed and Non-Fluxed Solid Solders for Electronic Soldering Applications STANDARD by Association Connecting Electronics Industries, 01/01/2006. Available online: https://www.techstreet.com/J-STD-006B|Solder|Alloy.J-STD-006B|Solder|Alloy (accessed on 10 January 2021).
- Lee, N. Getting Ready for Lead-free Solders. Solder. Surf. Mt. Technol. 1997, 9, 65–69. [Google Scholar] [CrossRef]
- Available online: https://dgvictory.en.alibaba.com/ (accessed on 10 January 2021).
- Available online: https://vicmachine.en.ecplaza.net/ (accessed on 10 January 2021).
- Švejcar, J.; Juliš, M.; Klakurková, L.; Gejdoš, P.; Zikmund, T. Analysis of Causes of Defects Appearance in Wire Drawing. Defect Diffus. Forum 2020, 405, 217–222. [Google Scholar] [CrossRef]
- Sun, L.; Bai, J.; Xue, F.; Chu, C.; Meng, J. The work softening behavior of pure Mg wire during cold Drawing. Materials 2018, 11, 602. [Google Scholar] [CrossRef] [Green Version]
- Radionov, A.A.; Radionova, L.V. Energy approach to the influence of countertension on drawing. Steel Transl. 2008, 38, 358–361. [Google Scholar] [CrossRef]
- Radionova, L.V.; Shirokov, V.V.; Faizov, S.R.; Zhludov, M.A. Studies of Influence of Process Parameters on the Strain Rate at High-Speed Wire Drawing. Mater. Sci. Forum 2019, 946, 832–838. [Google Scholar] [CrossRef]
- Nienaber, M.; Yi, S.; Kainer, K.U.; Letzig, D.; Bohlen, J. On the Direct Extrusion of Magnesium Wires from Mg-Al-Zn Series Alloys. Metals 2020, 10, 1208. [Google Scholar] [CrossRef]
- Jäger, A.; Habr, S.; Tesař, K. Twinning-detwinning assisted reversible plasticity in thin magnesium wires prepared by one-step direct extrusion. Mater. Des. 2016, 110, 895–902. [Google Scholar] [CrossRef]
- Tesař, K.; Balík, K.; Sucharda, Z.; Jäger, A. Direct extrusion of thin Mg wires for biomedical applications. Trans. Nonferrous Met. Soc. China 2020, 30, 373–381. [Google Scholar] [CrossRef]
- Radionova, L.V.; Faizov, S.R.; Sarafanov, A.E. Mathematical Modelling of Low Temperature Solder Direct Extrusion. IOP Conf. Ser. Mater. Sci. Eng. 2020, 969, 012107. [Google Scholar] [CrossRef]
- Poláková, I.; Zemko, M.; Rund, M.; Džugan, J. Using DEFORM Software for Determination of Parameters for Two Fracture Criteria on DIN 34CrNiMo6. Metals 2020, 10, 445. [Google Scholar] [CrossRef] [Green Version]
- Belyakov, N.; Smirnova, O.; Alekseev, A.; Tan, H. Numerical Simulation of the Mechanical Behavior of Fiber-Reinforced Cement Composites Subjected Dynamic Loading. Appl. Sci. 2021, 11, 1112. [Google Scholar] [CrossRef]
- Biba, N.; Stebunov, S. QForm3D–cost effective simulation tool for metal forming technology. In Proceedings of the Korean Society for Technology of Plasticity Conference, 15th Forging Symposium, Changwon, Korea, 10–11 June 2010; pp. 77–80. [Google Scholar]
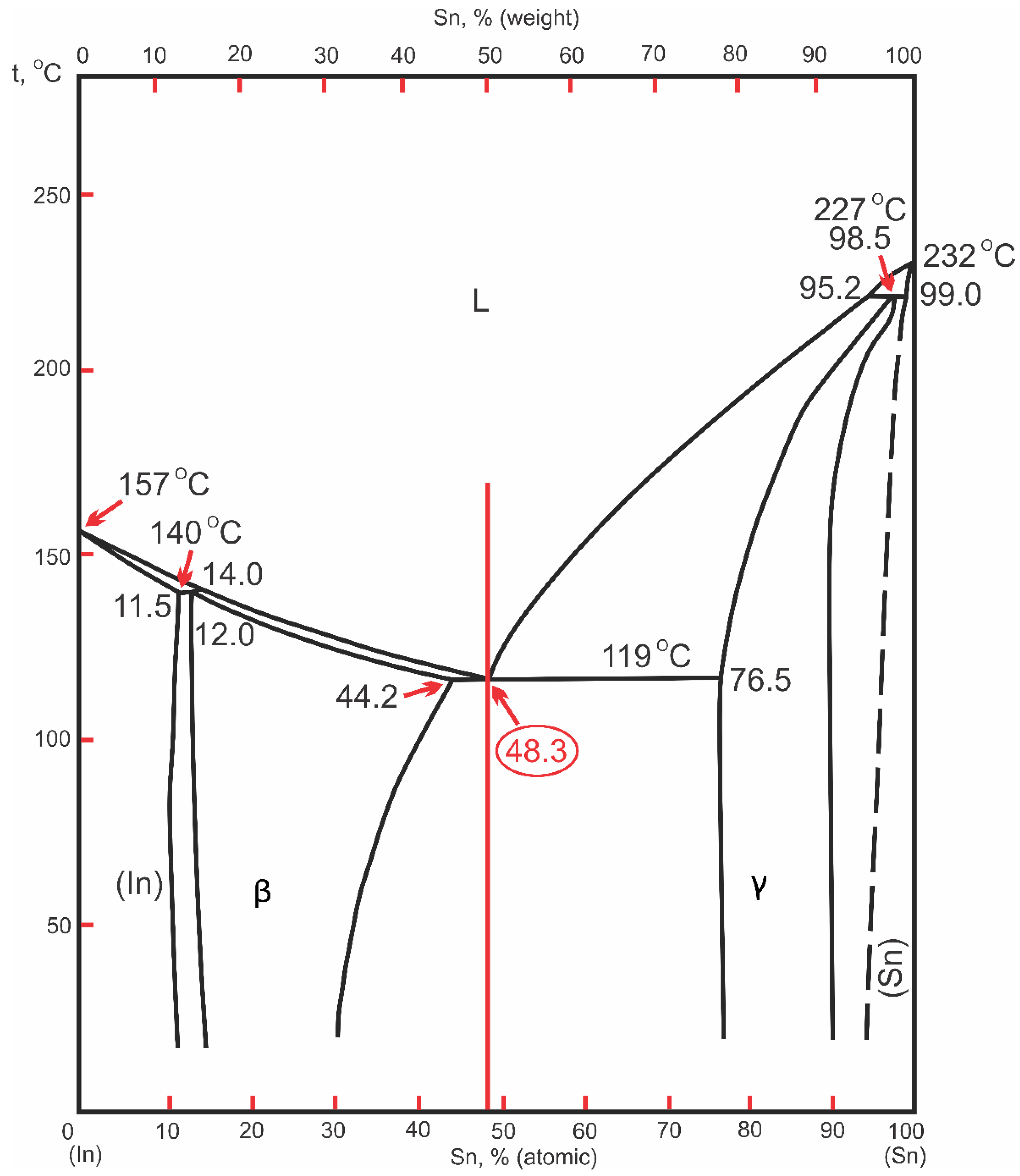
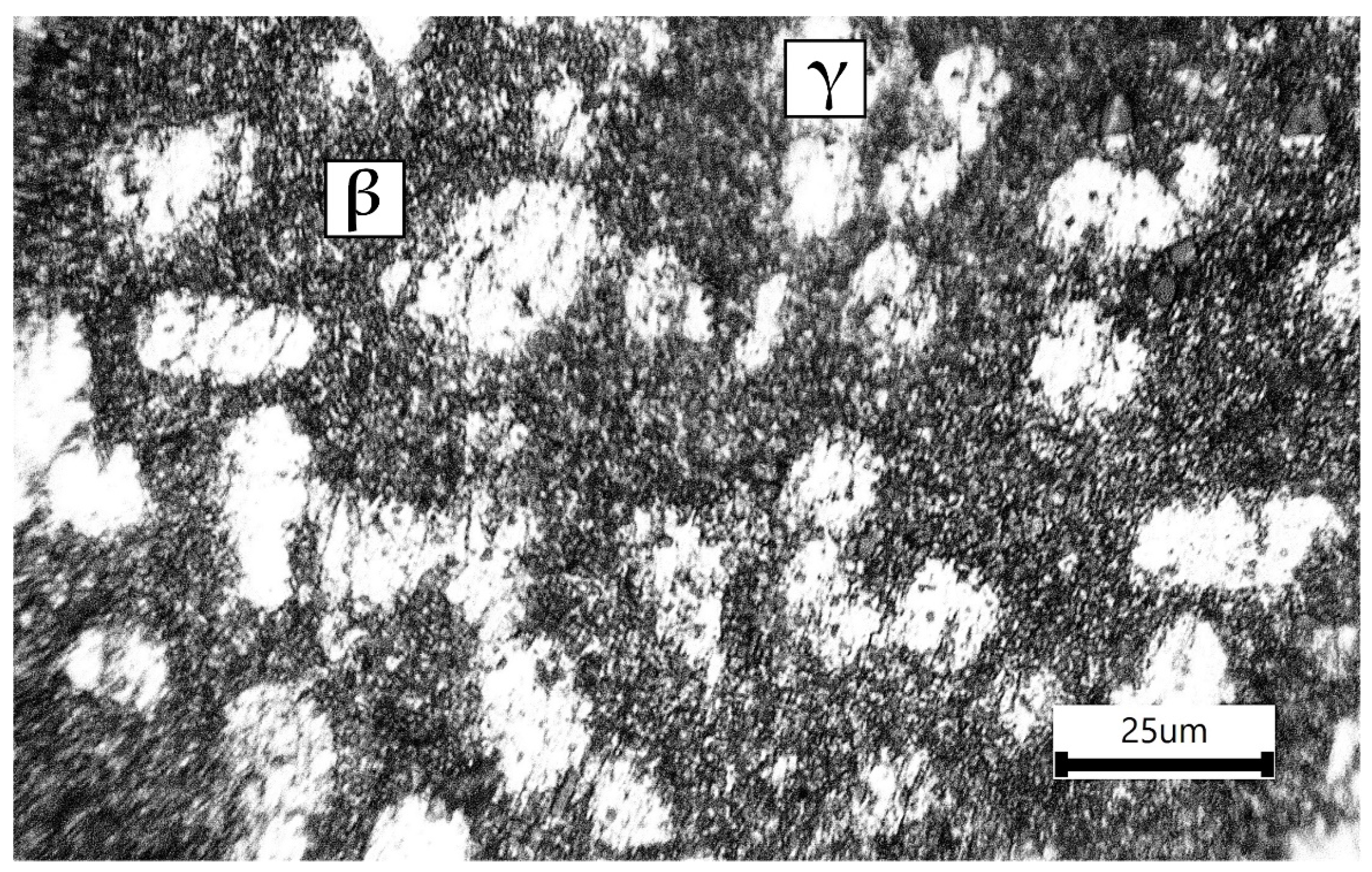
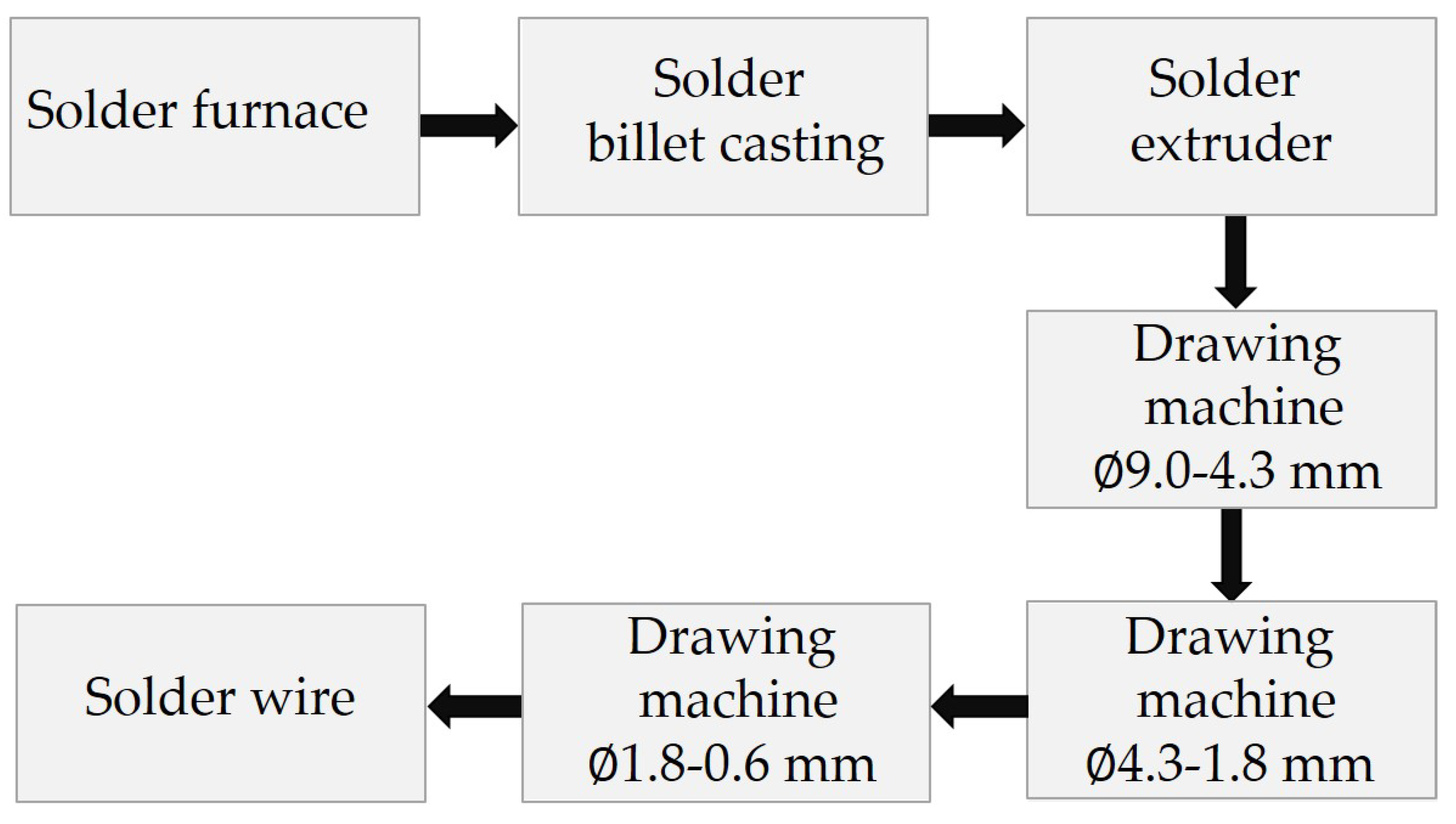
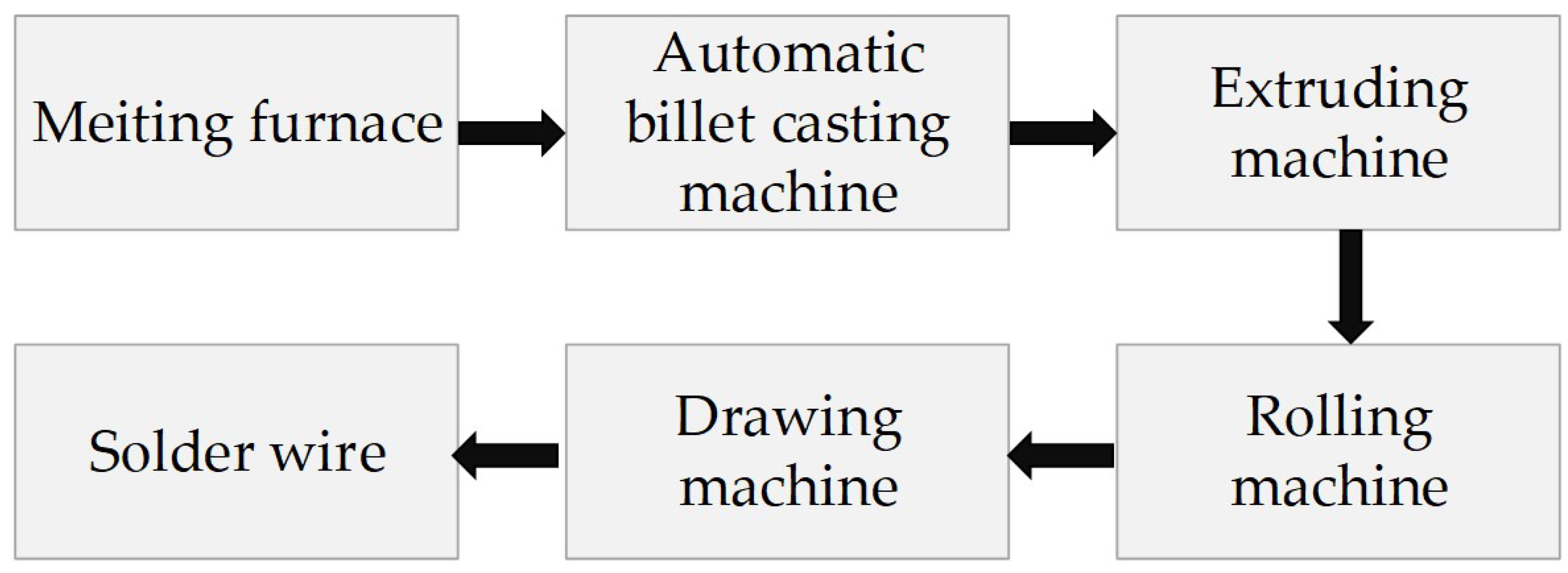




| Solidus/ Liquidus Temperature,°C | Alloy Density, g/cm3 (at 22 °C) | Thermal Conductivity, W/mK (at 85 °C) | Specific Electrical Resistance, Ohmm (at 22 °C) | Ultimate Tensile Strength, MPa | Elongation, % (at 22 °C) | Brinell Hardness, HB (at 22 °C) |
|---|---|---|---|---|---|---|
| 117/120 | 7.30 | 86 | 0.147 | 11.9 | 83 | 5 |
| Method | In | Sn | Impurities | ||||
|---|---|---|---|---|---|---|---|
| Bi | Fe | Cu | As | Sb | |||
| ICP-MS | 51.00 | balance | <0.015 | <0.01 | <0.01 | <0.006 | <0.015 |
| EDS | 51.36 | 48.64 | - | - | - | - | - |
| Parameter | Unit | Value | |
|---|---|---|---|
| Exper.1 | Exper.2 | ||
| Initial data | |||
| mm | 20 | 30 | |
| mm | 2 | 8 | |
| Billet length, L | mm | 120 | 120 |
| mm | 21.8 | 32 | |
| mm/s | 3 | 3 | |
| Billet material yield strength, σ | MPa | 10.5 | 10.5 |
| Half-angle of the die, α | ° | 18.4 | 40 |
| mm | 5 | 5 | |
| Friction coefficient, f | - | 0.5 | 0.5 |
| Calculation results | |||
| Extrusion ratio, μ | - | 118.8 | 16.0 |
| mm/s | 356.4 | 48 | |
| kN | 105 | 106 | |
| kN | 71.9 | 55.6 | |
| Parameter | Dimension | Value | |
|---|---|---|---|
| without Lubrication | with Graphite Lubricant | ||
| mm | 8 | 8 | |
| mm | 30 | 30 | |
| mm/s | 3 | 3 | |
| kN | 116 | 56 | |
| kN | 61.5 | 50 | |
| °C | 30 | 22 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Faizov, S.; Sarafanov, A.; Erdakov, I.; Gromov, D.; Svistun, A.; Glebov, L.; Bykov, V.; Bryk, A.; Radionova, L. On the Direct Extrusion of Solder Wire from 52In-48Sn Alloy. Machines 2021, 9, 93. https://doi.org/10.3390/machines9050093
Faizov S, Sarafanov A, Erdakov I, Gromov D, Svistun A, Glebov L, Bykov V, Bryk A, Radionova L. On the Direct Extrusion of Solder Wire from 52In-48Sn Alloy. Machines. 2021; 9(5):93. https://doi.org/10.3390/machines9050093
Chicago/Turabian StyleFaizov, Sergei, Aleksandr Sarafanov, Ivan Erdakov, Dmitry Gromov, Alexandra Svistun, Lev Glebov, Vitaly Bykov, Anastasia Bryk, and Liudmila Radionova. 2021. "On the Direct Extrusion of Solder Wire from 52In-48Sn Alloy" Machines 9, no. 5: 93. https://doi.org/10.3390/machines9050093
APA StyleFaizov, S., Sarafanov, A., Erdakov, I., Gromov, D., Svistun, A., Glebov, L., Bykov, V., Bryk, A., & Radionova, L. (2021). On the Direct Extrusion of Solder Wire from 52In-48Sn Alloy. Machines, 9(5), 93. https://doi.org/10.3390/machines9050093







