Development of an Automatic Elastic Torque Control System Based on a Two-Mass Electric Drive Coordinate Observer
Abstract
1. Introduction
- shock (impact) loads that result in fatigue destruction of mechanical equipment;
- vibrations that occur in the shaft lines of stands and in the electromechanical systems that are resiliently connected by the strip;
- instability of control systems due to torque limitations being applied to the electric drives.
2. Problem Formulation
- Earlier papers present research into electromechanical systems with two electric machines installed on the ends of an elastic shaft [42,43]. This enables control over the coordinates of both electric machines. These parameters include speeds and currents, which are used to calculate the torques of these machines as well as the elastic shaft torques. These coordinates are used in control algorithms.
- 2.
- Dynamic processes should be presented in the form of continuously measured physical parameters yet require minimum computing.
- Controllable coordinates are motor speed and torque as well as the applied load torque. The output coordinates are the roll speed and the elastic torque of the spindle.
- The developed solutions should not require complex mathematics (computing) and should be implementable in the controllers already used by the rolling mill APCS (automatic process control system).
3. Materials and Methods
4. Implementation
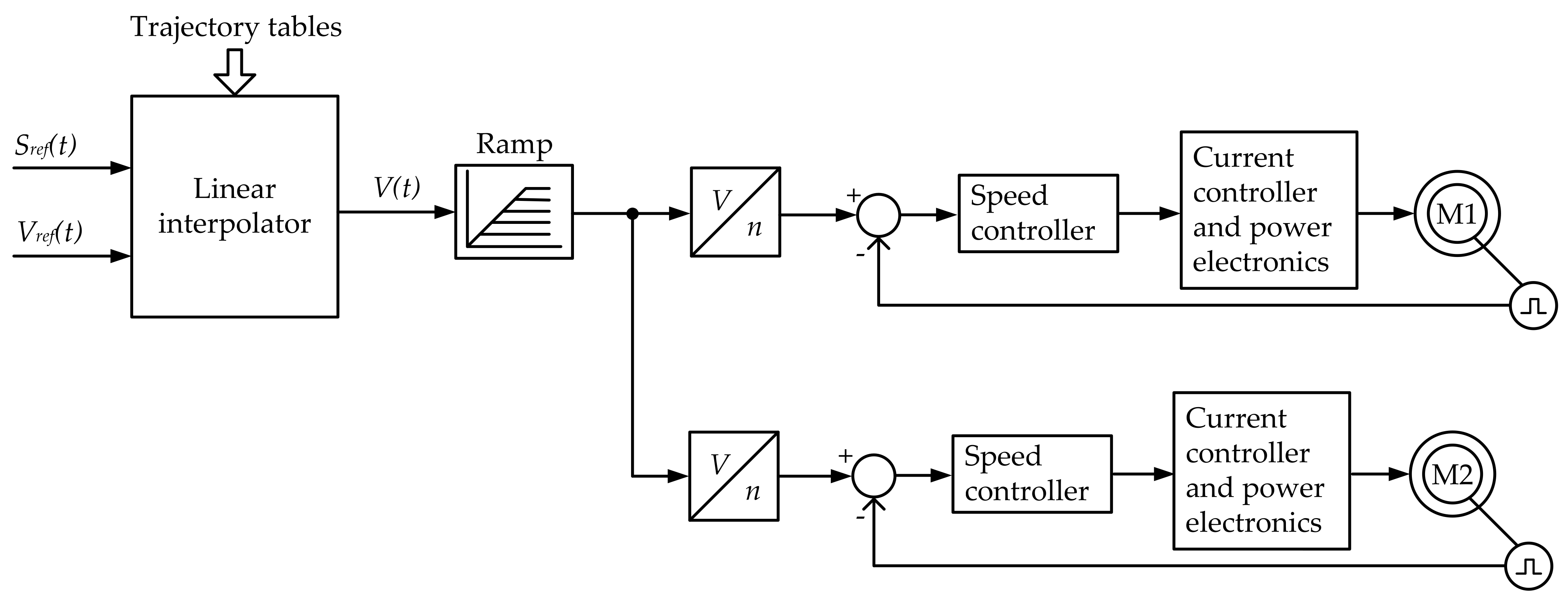
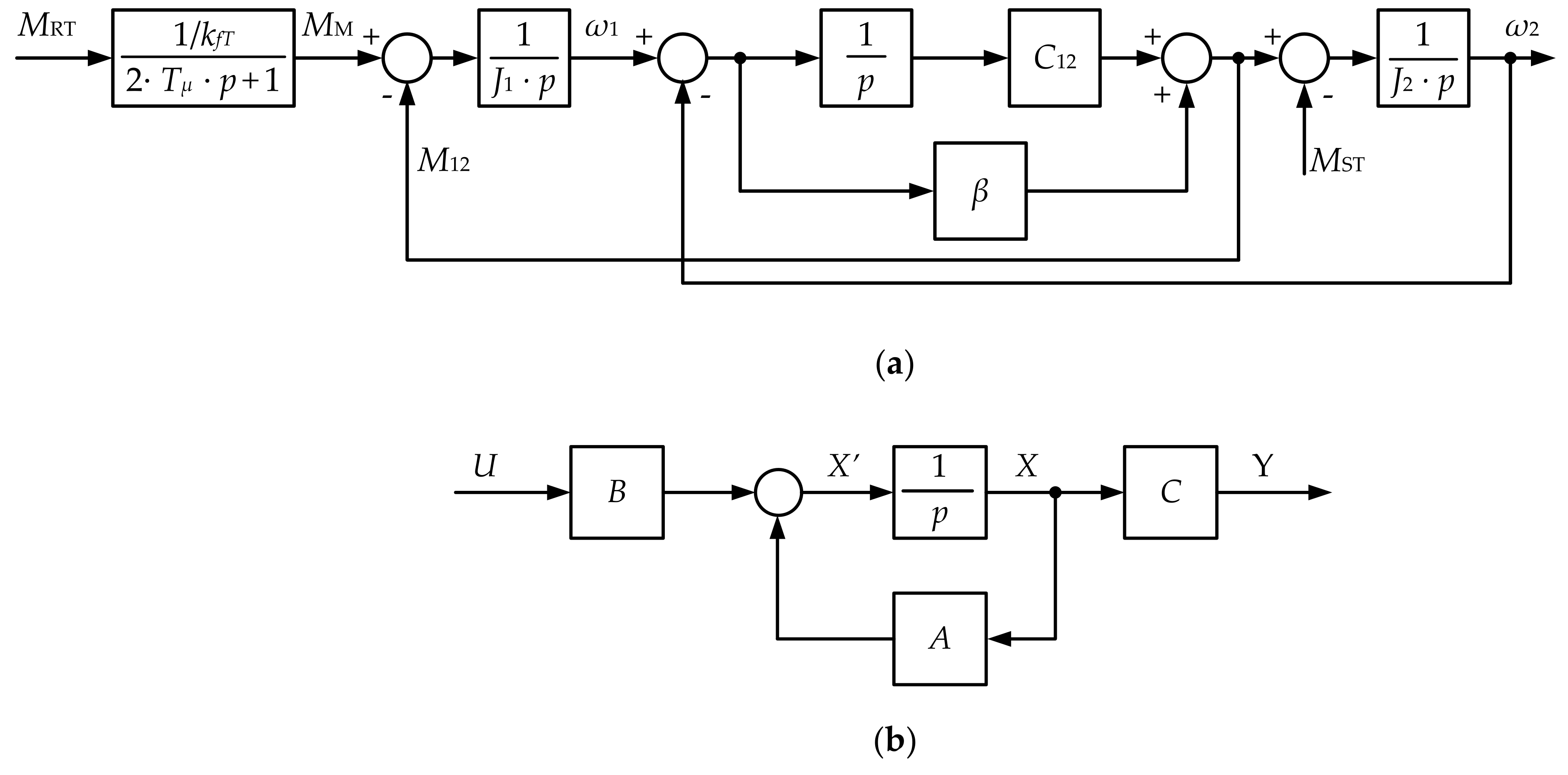
4.1. Development of the Closed Coordinate Control System for the Uncontrolled Mass
4.2. Simulation Results
- the amplitude margin is 10 dB, which is sufficient per Nyquist criterion;
- the phase plot is beak-shaped, no crossing through −180° to the left of the cutoff frequency; the phase margin is about 50°, which is also sufficient.
5. Experimental Results
- The system under consideration is a two-mass system where the first mass (the motor’s rotor) does not change its inertia.
- The stiffness of the elastic shaft (spindle) does not change either. This parameter depends on the length, diameter, and the properties of the metal that the shaft is made of. These parameters do not change even when the spindle is replaced.
- Second mass inertia depends on the mass of work and backup rolls, which is constant as well. The inertia of the rolled bar is 5% to 15% of the total second mass inertia. For configuration, use the mean bar inertia that deviates by 1.7% to 5%. Such deviations are commensurate with the error of the instrumentation sensors. Thus, they do not cause significant error in the configuration of the control algorithms.
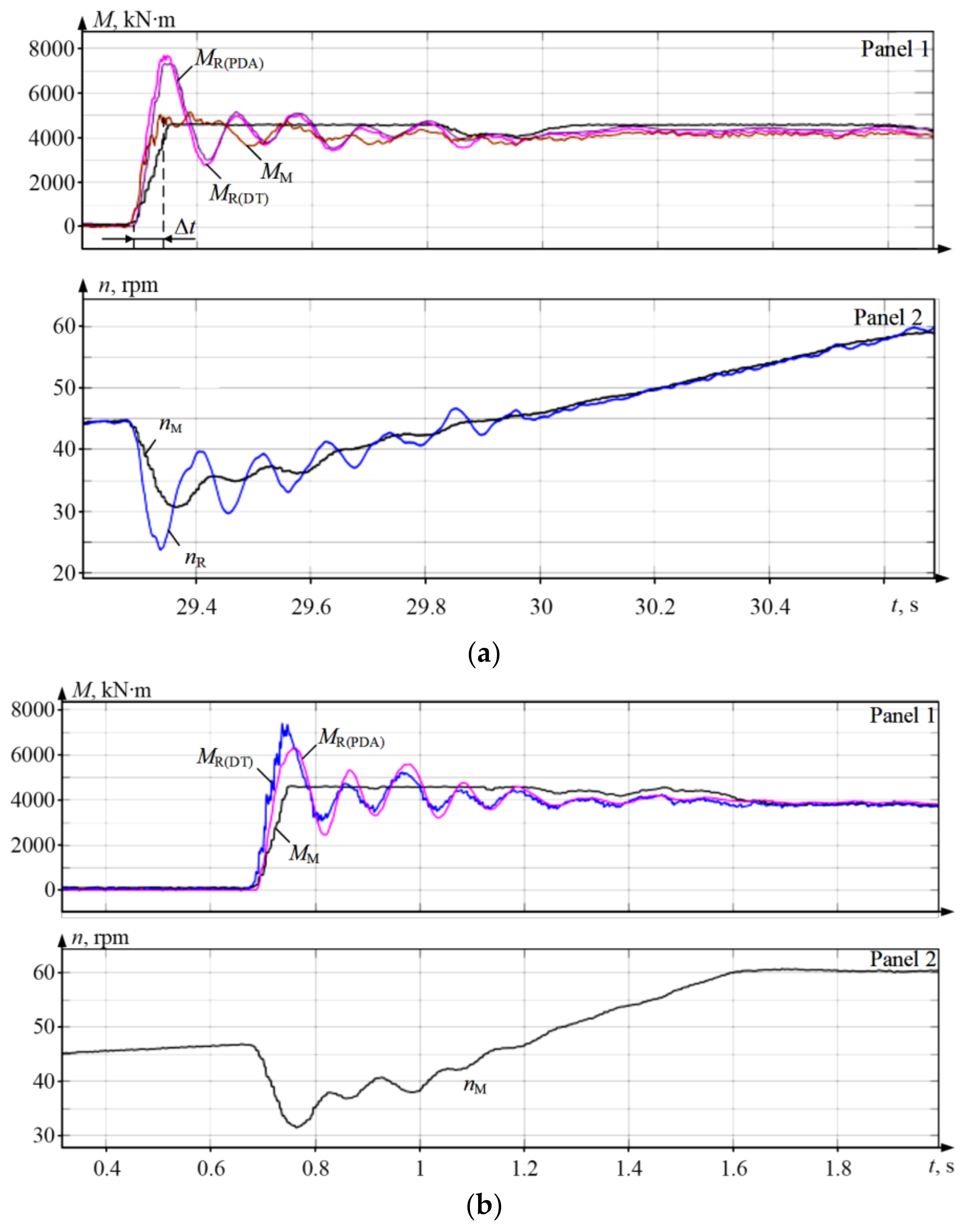
5.1. Accuracy of Reconstructed Two-Mass Coordinates
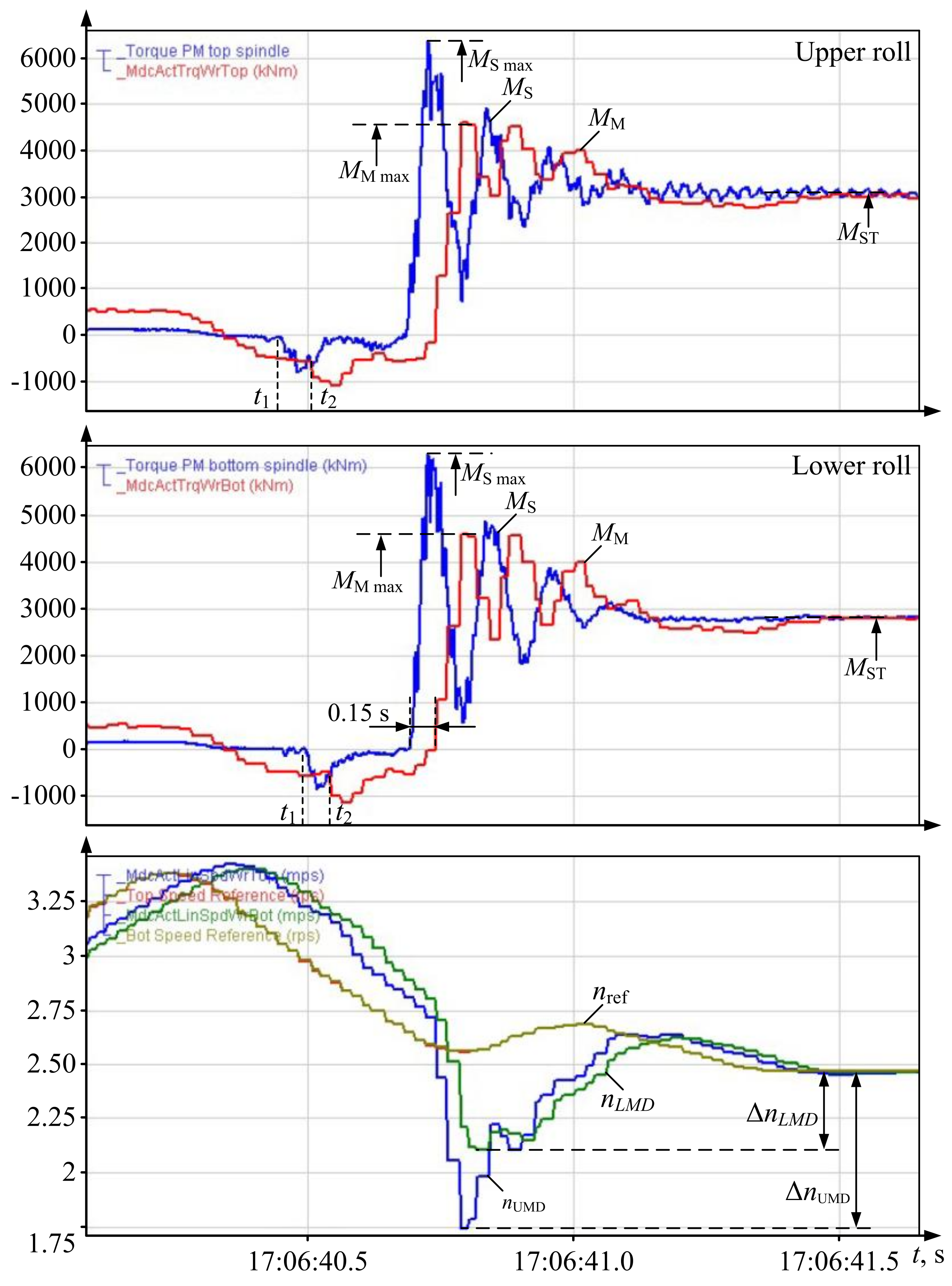
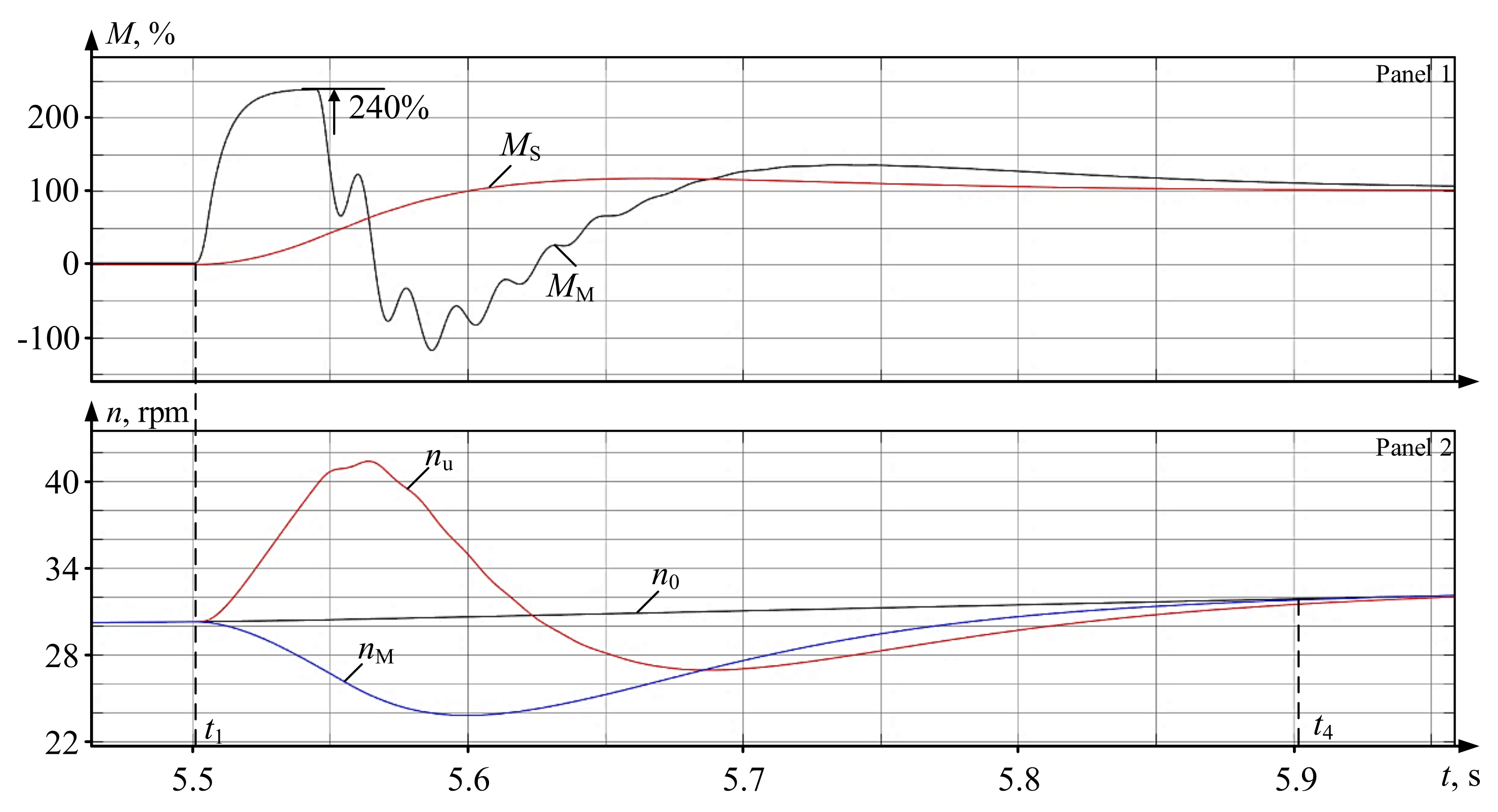
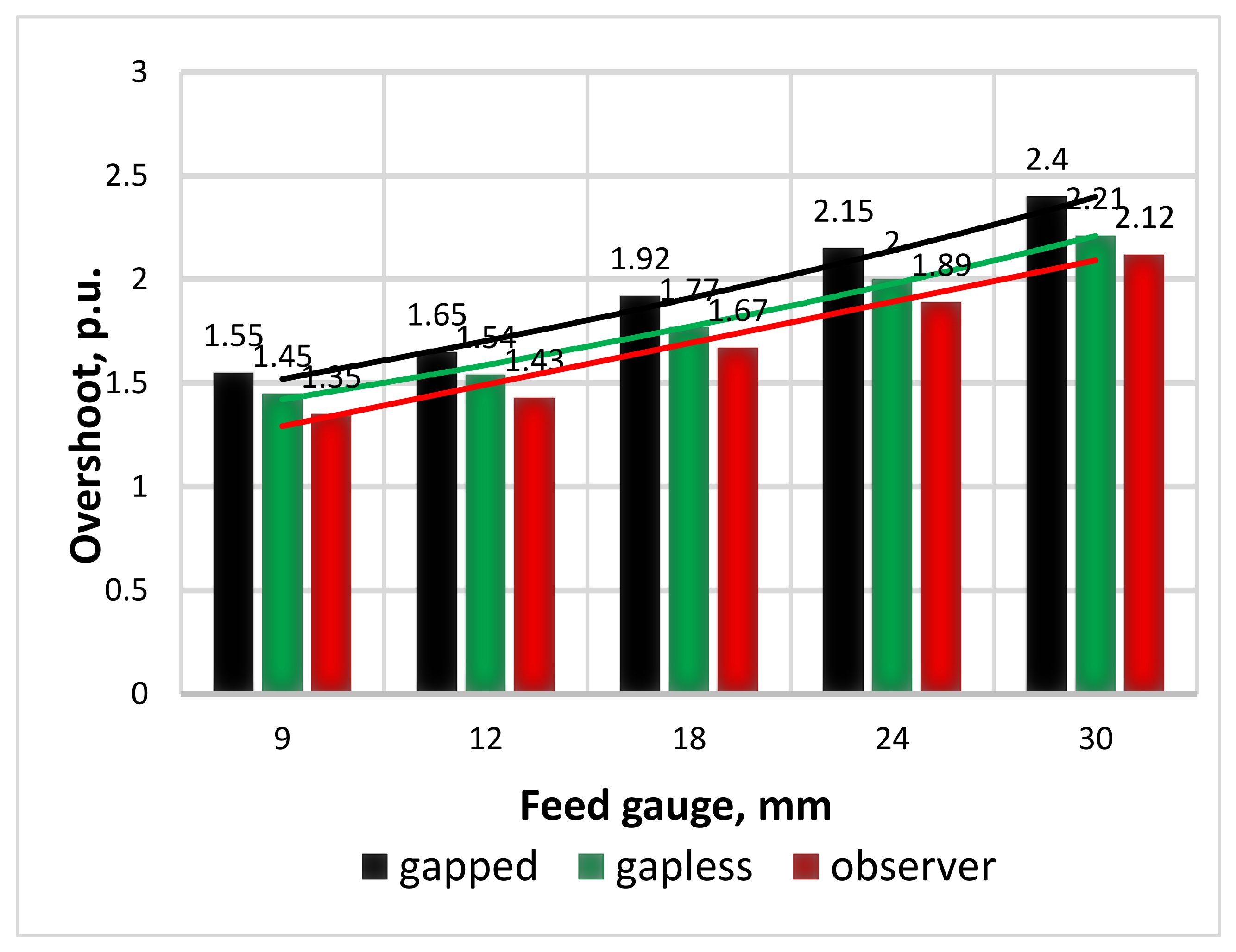
- Greater gauge is associated with greater overshoot. Thus, at 9 mm, i.e., in the later passages, the ‘gapless’ amplitude is 35%, and the ‘gapped’ amplitude is 45%. In case of 30 mm gauge (the earlier passages), it is 240% and 212%, respectively.
- The torque component that stems directly from the gap-closing shock (impact) is relatively insignificant at 6.9% at 9 mm (1.55 and 1.45 p.u. of difference in amplitudes), 8.6% at 30 mm (2.4 and 2.21 p.u., respectively). This indirectly confirms that the ‘elastic’ torque component has the maximum impact on torque overshoot [54].
- Relative difference between actual and reconstructed values decreases as the gauge increases: 6.9% at 9 mm, 5.6% at 18 mm, and 4.1% at 30 mm.
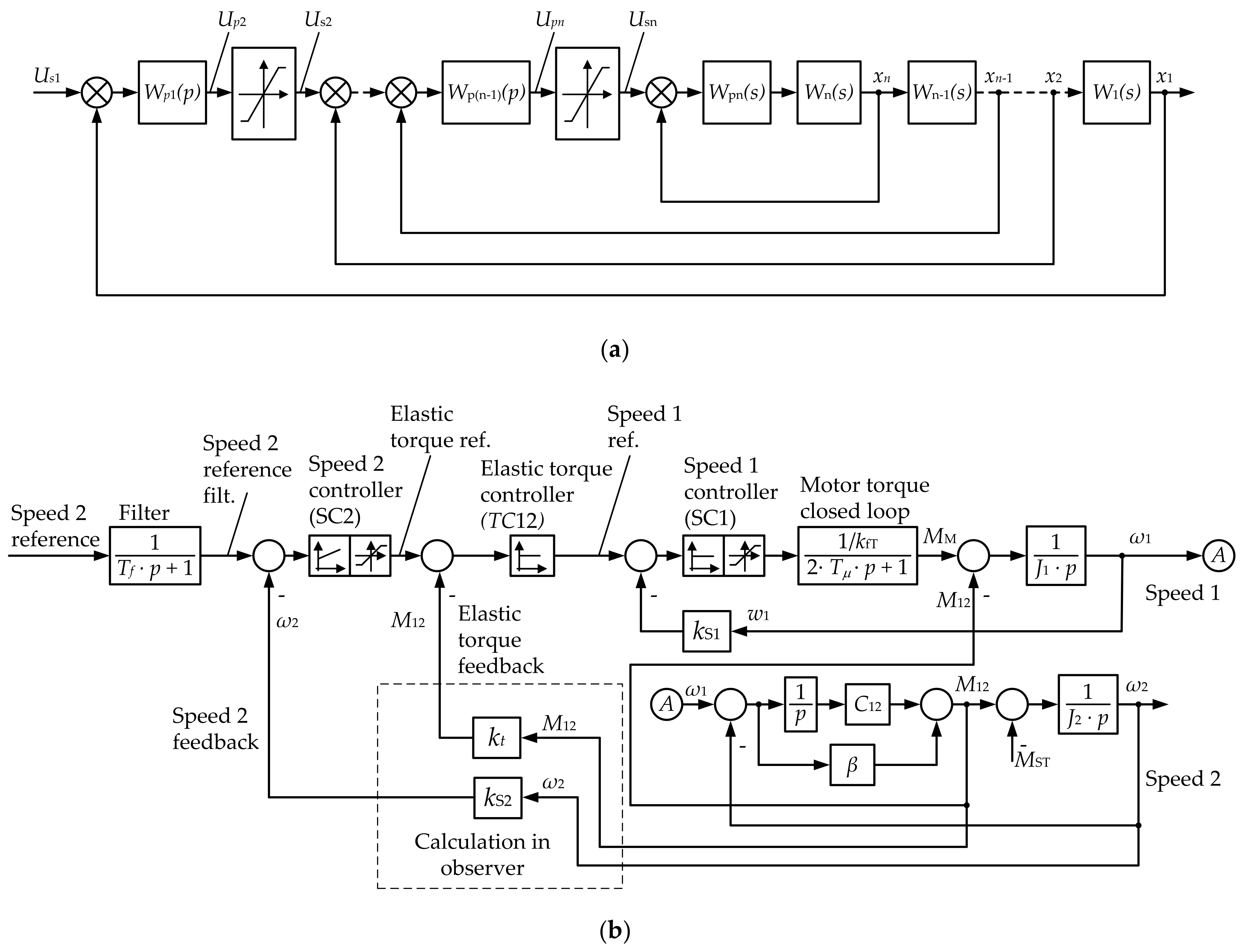
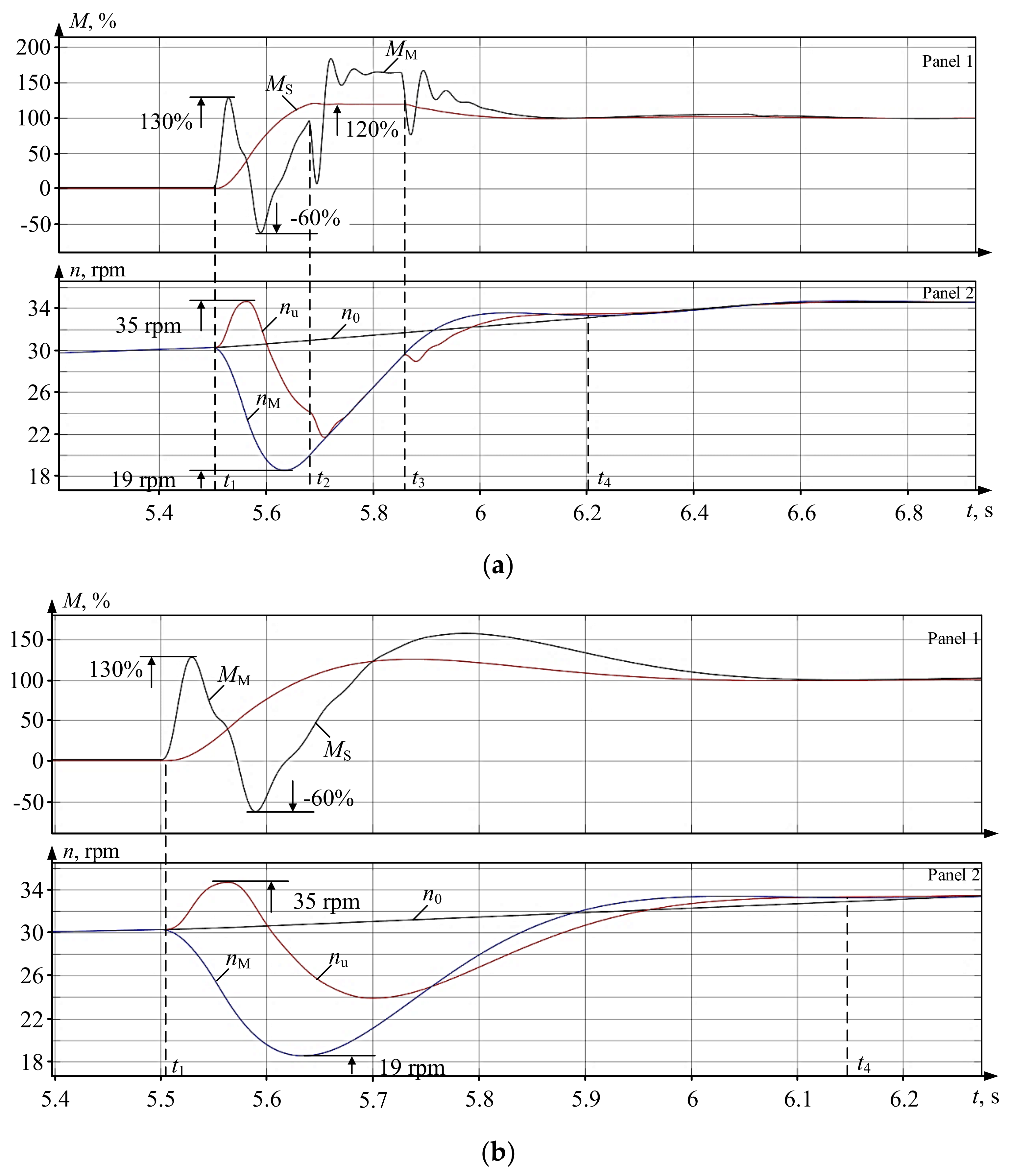
5.2. Testing the Electric Drive Coordinates in the Developed Three-Loop System
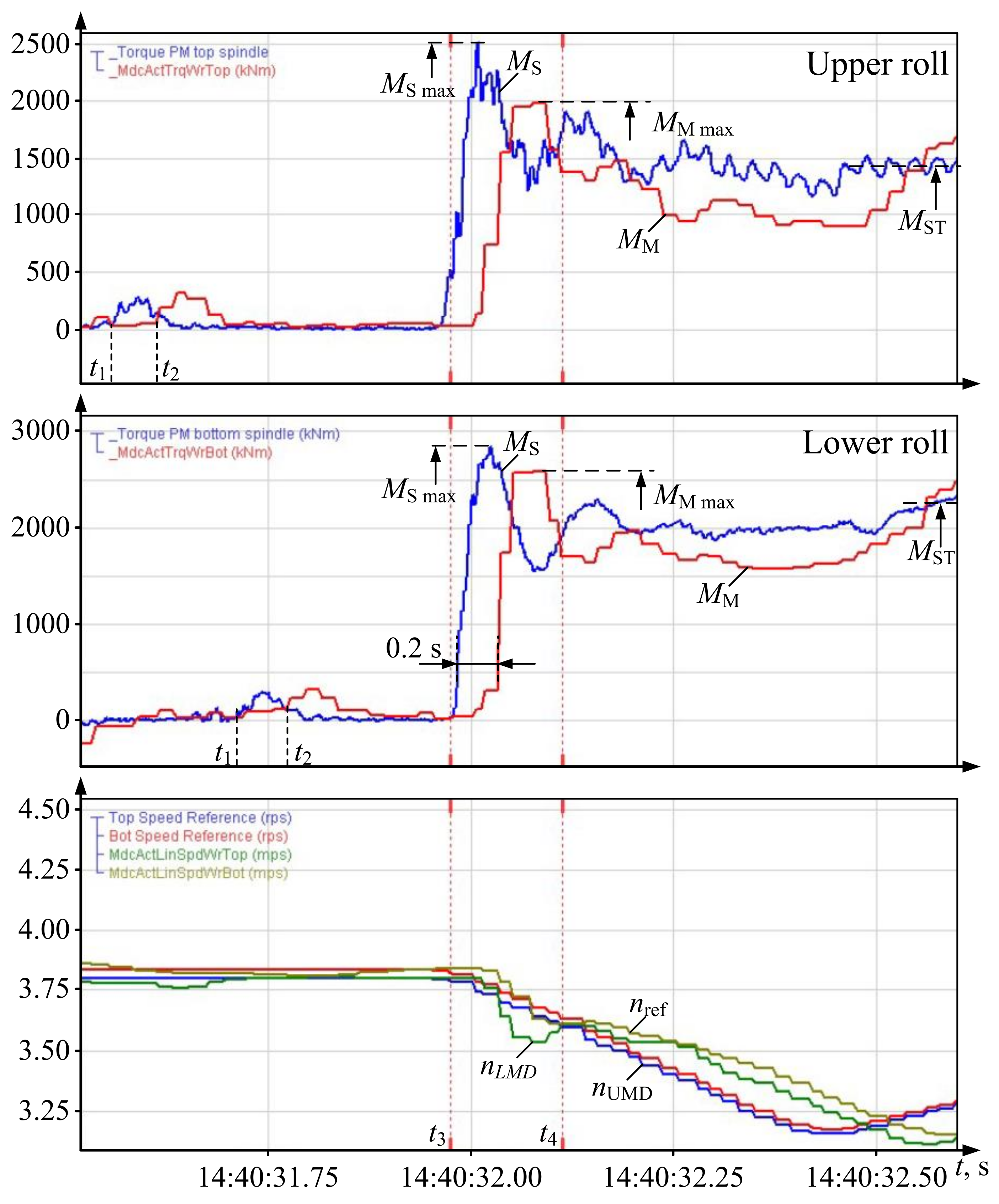
- kSm was 1.8 with the initial configuration, changed to kSm = 1.2 after implementing the new algorithm. Thus, the algorithm reduced the torque amplitude by a factor of 1.5.
- kSm was 1.4 for the UMD torque MMT, 1.12 for LMD, i.e., reduced by a factor of 1.25.
- Elastic oscillations attenuated at least twice as fast. kSm was 1.4 for the UMD torque Mmt, 1.12 for LMD, i.e., reduced by a factor of 1.25.
6. Discussion of the Results
- in case of gripping with pre-closed angular gaps in spindle joints, difference in the amplitudes of the measured vs reconstructed torque averages at 5 to 7%;
- equivalent difference in case of open gaps hits 10%.
7. Conclusions
- proportional speed controller for the first mass, whose output is the target motor torque;
- proportional controller of elastic torque. This configuration is optimal because the object structure contains an integrator, and this controller provides high performance;
- proportional speed controller for the second mass, which features a corrective proportional integral element at the input. This element increases the slope of the Bode magnitude plot in the low-frequency domain and enables astatic control over the second mass speed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kalachev, Y.N. State Observers in a Vector Electric Drive; EFO: Moscow, Russia, 2015. [Google Scholar]
- Kritzinger, W.; Karner, M.G.; Jan Henjes, J.; Sihn, W. Digital Twin in manufacturing: A categorical literature review and classification. IFAC Pap. 2018, 51, 1016–1022. [Google Scholar] [CrossRef]
- Fuller, A.; Fan, Z.; Day, C.; Barlow, C. Digital Twin: Enabling Technologies, Challenges and Open Research. IEEE Access 2020, 8, 108952–108971. [Google Scholar] [CrossRef]
- Saarakkala, S.E.; Hinkkanen, M. State-space speed control of two-mass mechanical systems: Analytical tuning and experimental evaluation. In Proceedings of the 2013 IEEE Energy Conversion Congress and Exposition, Denver, CO, USA, 15–19 September 2013. [Google Scholar] [CrossRef]
- Qiao, F.; Zhu, Q.M.; Li, S.Y.; Winfield, A. Torsional vibration suppression of a 2-mass main drive system of rolling mill with KF enhanced pole placement. In Proceedings of the 4th World Congress on Intelligent Control and Automation (Cat. No.02EX527), Shanghai, China, 10–14 June 2002. [Google Scholar] [CrossRef]
- Szabat, K.; Orłowska-Kowalska, T. Vibration Suppression in Two-Mass Drive System using PI Speed Controller and Additional Feedbacks—Comparative Study. IEEE Trans. Ind. Electron. 2007, 54, 1193–1206. [Google Scholar] [CrossRef]
- Kolganov, A.R. Electromechanotronic Systems. State-of-the-Art Controls, Implementations, and Applications: A Textbook; Infra-Inzheneriya: Moscow, Russia, 2019. [Google Scholar]
- Lebedev, S.K. Industrial Arm Motion Control: Textbook; Lenin Ivanovo State Power Engineering University: Ivanovo, Russia, 2018. [Google Scholar]
- Kolganov, A.R. Advanced Control Methods for Electromechanotronic Systems. Development, Implementation, Application; Lenin Ivanovo State Power Engineering University: Ivanovo, Russia, 2012. [Google Scholar]
- Kolganov, A.R. Mathematical Foundations of Automatic Control Theory: A University Textbook; Lenin Ivanovo State Power Engineering University: Ivanovo, Russia, 2010. [Google Scholar]
- Szabat, K.; Orlowska-Kowalska, T.; Dybkowski, M. Indirect adaptive control of induction motor drive system with an elastic coupling. IEEE Trans. Ind. Electron. 2009, 56, 4038–4042. [Google Scholar] [CrossRef]
- Szabat, K.; Orlowska-Kowalska, T. Control of the Drive System with Stiff and Elastic Couplings Using Adaptive Neuro-Fuzzy Approach. IEEE Trans. Ind. Electron. 2007, 54, 228–240. [Google Scholar] [CrossRef]
- Muszynski, R.; Deskur, J. Damping of Torsional Vibrations in High-Dynamic Industrial Drives. IEEE Trans. Ind. Electron. 2010, 57, 544–552. [Google Scholar] [CrossRef]
- Ke, C.; Wu, A.; Bing, C. Mechanical parameter identification of two-mass drive system based on variable forgetting factor recursive least squares method. Trans. Inst. Meas. Control 2018, 41, 494–503. [Google Scholar] [CrossRef]
- Bolognani, S.; Venturato, A.; Zigliotto, M. Theoretical and experimental comparison of speed controllers for elastic two-mass-systems. Proc. PESC 2000, 1087–1092. [Google Scholar] [CrossRef]
- Lukichev, D.V.; Demidova, G.L.; Brock, S. Application of fuzzy cooperative coevolution adaptive control for damping of torsional vibrations in multi-mass drive system. In Proceedings of the 2017 19th European Conference on Power Electronics and Applications (EPE’17 ECCE Europe), Warsaw, Poland, 11–14 September 2017. [Google Scholar] [CrossRef]
- Nowopolski, K.; Wicher, B.; Zawirski, K.; Tulodziecki, M. Control of electromechanical object with backlash and elasticity within interconnection between motor and load. In Proceedings of the 2015 Selected Problems of Electrical Engineering and Electronics (WZEE), Kielce, Poland, 17–19 September 2015. [Google Scholar] [CrossRef]
- Shen, Z.; Zhiqiang, G. An active disturbance rejection based approach to vibration suppression in two-inertia systems. In Proceedings of the 2010 American Control Conference, Baltimore, MA, USA, 30 June–2 July 2010. [Google Scholar] [CrossRef]
- Brock, S.; Luczak, D.; Nowopolski, K.; Pajchrowski, T.; Zawirski, K. Two Approaches to Speed Control for Multi-Mass System With Variable Mechanical Parameters. IEEE Trans. Ind. Electron. 2017, 64, 3338–3347. [Google Scholar] [CrossRef]
- Han, J. From PID to Active Disturbance Rejection Control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Wang, J.; Zhao, L.; Yu, L. Reduced-order Generalized Proportional Integral Observer Based Continuous Dynamic Sliding Mode Control for Magnetic Levitation System with Time-varying Disturbances. Int. J. Control Autom. Syst. 2020, 19, 439–448. [Google Scholar] [CrossRef]
- Wang, J.; Zhao, L.; Yu, L. Adaptive Terminal Sliding Mode Control for Magnetic Levitation Systems With Enhanced Disturbance Compensation. IEEE Trans. Ind. Electron. 2020, 68, 756–766. [Google Scholar] [CrossRef]
- Rong, J.; Yang, H.; Wang, J.; Yu, L. Design and implementation of GPI observer based sliding mode control for DC-DC buck converter *. In Proceedings of the 2019 Chinese Automation Congress (CAC), Hangzhou, China, 22–24 November 2019. [Google Scholar] [CrossRef]
- Wang, J.; Rong, J.; Yu, L. Reduced-order extended state observer based event-triggered sliding mode control for DC-DC buck converter system With parameter perturbation. Asian J. Control 2020, 23, 1591–1601. [Google Scholar] [CrossRef]
- Wang, J.; Wang, F. Robust sensorless FCS-PCC control for inverter-based induction machine systems with high-order disturbance compensation. J. Power Electron. 2020, 20, 1222–1231. [Google Scholar] [CrossRef]
- Drozdz, K.; Janiszewski, D.; Szabat, K. Application of fuzzy Kalman filter in adaptive control structure of two-mass system. In Proceedings of the 2014 16th International Power Electronics and Motion Control Conference and Exposition, Antalya, Turkey, 21–24 September 2014. [Google Scholar] [CrossRef]
- Pajchrowski, T.; Janiszewski, D. Control of multi-mass system by on-line trained neural network based on Kalman filter. In Proceedings of the 2015 17th European Conference on Power Electronics and Applications (EPE’15 ECCE-Europe), Geneva, Switzerland, 8–10 September 2015. [Google Scholar] [CrossRef]
- Szabat, K.; Orlowska-Kowalska, T. Adaptive Control of the Electrical Drives with the Elastic Coupling using Kalman Filter. In Adaptive Control; Kwanho, Y., Ed.; IntechOpen: London, UK, 2009. [Google Scholar] [CrossRef]
- Szabat, K.; Serkies, P.; Cychowski, M. Application of the MPC to the robust control of the two-mass drive system. In Proceedings of the 2011 IEEE International Symposium on Industrial Electronics, Gdansk, Poland, 27–30 June 2011. [Google Scholar] [CrossRef]
- Hidehiro, I. Vibration Suppression Controller of Multi-Mass Resonance System Using Fuzzy Controller. Mod. Fuzzy Control Syst. Its Appl. 2017, 19, 399–417. [Google Scholar] [CrossRef][Green Version]
- Lozynskyy, A.; Demkiv, L. Analysis of Fuzzy Controller Application Efficiency in Two-Mass Systems with Variable Moment of Inertia of Second Mass. Energy Eng. Control Syst. 2016, 2, 59–68. [Google Scholar] [CrossRef]
- Khramshin, V.R. Dynamic loads reduction in universal stands of rolling mill. Metallurg 2015, 4, 41–47. [Google Scholar] [CrossRef]
- Radionov, A.A. Mathematical modelling of the interrelated electric and mechanical systems of continuous sub-group of the rolling mill stands. Part 1. Development of the mathematical model. Bull. South Ural. State Univ. Ser. Power Eng. 2015, 15, 59–73. [Google Scholar] [CrossRef]
- Galkin, V.V.; Karandaev, A.; Golovin, V.; Rodionov, A.; Khramshin, V.; Gasiyarov, V.; Zalogin, O. Algorithm of calculation of high-speed and loading modes of electric drives of cages of the rolling mill at the rolling of thick strips. Izv. TulGU. Tech. Sci. 2010, 3, 12–17. [Google Scholar]
- Khramshin, V.R.; Karandaev, A.S.; Gasiyarov, V.R.; Zinchenko, M.A.; Loginov, B.M. Limiting Dynamic Loads in the Main Line of a Rolling Mill through an Automated Drive. In Proceedings of the International Russian Automation Conference, Sochi, Russia, 6–12 September 2020. [Google Scholar] [CrossRef]
- Khramshin, V.R.; Gasiyarov, V.R.; Karandaev, A.S.; Baskov, S.N.; Loginov, B.M. Constraining the Dynamic Torque of a Rolling Mill Stand Drive. Bull. South Ural. State Univ. Ser. Power Eng. 2018, 18, 101–111. [Google Scholar] [CrossRef][Green Version]
- Radionov, A.A.; Gasiyarov, V.R.; Tverskoi, M.M.; Khramshin, V.R.; Loginov, B.M. Implementation of telemetric on-line monitoring system of elastic torque of rolling mill line of shafting. In Proceedings of the 2017 2nd International Ural Conference on Measurements (UralCon), Chelyabinsk, Russia, 16–19 October 2017. [Google Scholar] [CrossRef]
- Krot, P.V. Nonlinear Vibrations and Backlashes Diagnostics in the Rolling Mills Drive Trains. In Proceedings of the 6th EUROMECH Nonlinear Dynamics Conference (ENOC 2008), St. Petersburg, Russia, 30 June–4 July 2008. [Google Scholar] [CrossRef]
- Lukyanov, S.I.; Karandaev, A.S.; Evdokimov, S.A.; Sarvarov, A.S.; Petushkov, M.Y.; Khramshin, V.R. The development and implementation of intelligent systems for electrical equipment state diagnostics. Bull. Nosov Magnitogorsk State Tech. Univ. 2014, 1, 129–136. [Google Scholar]
- Karandaev, A.S. Experimental Parametrization of the Dual-Mass Electromechanical System of a Rolling Mill. Russ. Electromech. 2021, 64, 24–35. [Google Scholar] [CrossRef]
- Shubin, A.G. Feasibility of the Ways to Limit Dynamic Loads of Electromechanical Systems of a Rolling Stand. Electrotech. Syst. Complexes 2018, 1, 14–25. [Google Scholar] [CrossRef]
- Bouheraoua, M.; Wang, J.; Atallah, K. Influence of Control Structures and Load Parameters on Performance of a Pseudo Direct Drive. Machines 2014, 2, 158–175. [Google Scholar] [CrossRef]
- Orlowska-Kowalska, T.; Kaminski, M.; Szabat, K. Implementation of a Sliding-Mode Controller with an Integral Function and Fuzzy Gain Value for the Electrical Drive with an Elastic Joint. Ind. Electron. IEEE Trans. 2010, 57, 1309–1317. [Google Scholar] [CrossRef]
- Szabat, K.; Orlowska-Kowalska, T. Performance Improvement of Industrial Drives with Mechanical Elasticity Using Nonlinear Adaptive Kalman Filter. Ind. Electron. IEEE Trans. 2008, 55, 1075–1084. [Google Scholar] [CrossRef]
- Cychowski, M.; Szabat, K.; Orlowska-Kowalska, T. Constrained Model Predictive Control of the Drive System with Mechanical Elasticity. Ind. Electron. IEEE Trans. 2009, 56, 1963–1973. [Google Scholar] [CrossRef]
- Orlowska-Kowalska, T.; Dybkowski, M.; Szabat, K. Adaptive Sliding-Mode Neuro-Fuzzy Control of the Two-Mass Induction Motor Drive Without Mechanical Sensors. Ind. Electron. IEEE Trans. 2010, 57, 553–564. [Google Scholar] [CrossRef]
- Thomsen, S.; Hoffmann, N.; Fuchs, F.W. PI Control, PI-Based State Space Control and Model-Based Predictive Control for Drive Systems with Elastically Coupled Loads—A Comparative Study. Ind. Electron. IEEE Trans. 2011, 58, 3647–3657. [Google Scholar] [CrossRef]
- Sieklucki, G. Optimization of Observers in a Two-Mass System—Dual LQ Problem. In Proceedings of the 2018 International Symposium on Electrical Machines (SME), Andrychow, Poland, 10–13 June 2018. [Google Scholar] [CrossRef]
- Karandaev, A.S.; Baskov, S.N.; Gasiyarova, O.A.; Loginov, B.M.; Khramshin, V.R. Calculating Simulation Model Parameters for Electromechanical System of Rolling Mill Stand. In Proceedings of the International Ural Conference on Electrical Power Engineering (Ural-Con), Magnitogorsk, Russian, 24–26 September 2021. [Google Scholar] [CrossRef]
- Radionov, A.A. Conceptual Directions of Creating Digital Twins for Electrotechnical Systems of Rolling Mill Facilities. Russ. Electromechanics 2021, 64, 54–68. [Google Scholar] [CrossRef]
- Klyuchev, V.I. Electric Drive Theory: A University Textbook; Energoatomizdat: Moscow, Russia, 2001. [Google Scholar]
- Radionov, A.A.; Gasiyarov, V.R.; Karandaev, A.S.; Khramshin, V.R. Use of automated electric drives for limiting dynamic loads in shaft lines of roll mill stands. J. Eng. 2019, 2019, 3578–3581. [Google Scholar] [CrossRef]
- Gasiyarov, V.R. Method for dynamic load limitation at mechatronic systems of the plate mill stand. Bull. South Ural. State Univ. Ser. Mech. Eng. 2019, 19, 5–18. [Google Scholar] [CrossRef][Green Version]
- Gasiyarov, V.R.; Khramshin, V.R.; Voronin, S.S.; Lisovskaya, T.A.; Gasiyarova, O.A. Dynamic Torque Limitation Principle in the Main Line of a Mill Stand: Explanation and Rationale for Use. Machines 2019, 7, 76. [Google Scholar] [CrossRef]
- Lebedev, S.K. Electromechatronic Positioning Systems with Load Observers: A Monograph; Lenin Ivanovo State Power Engineering University: Ivanovo, Russia, 2016. [Google Scholar]
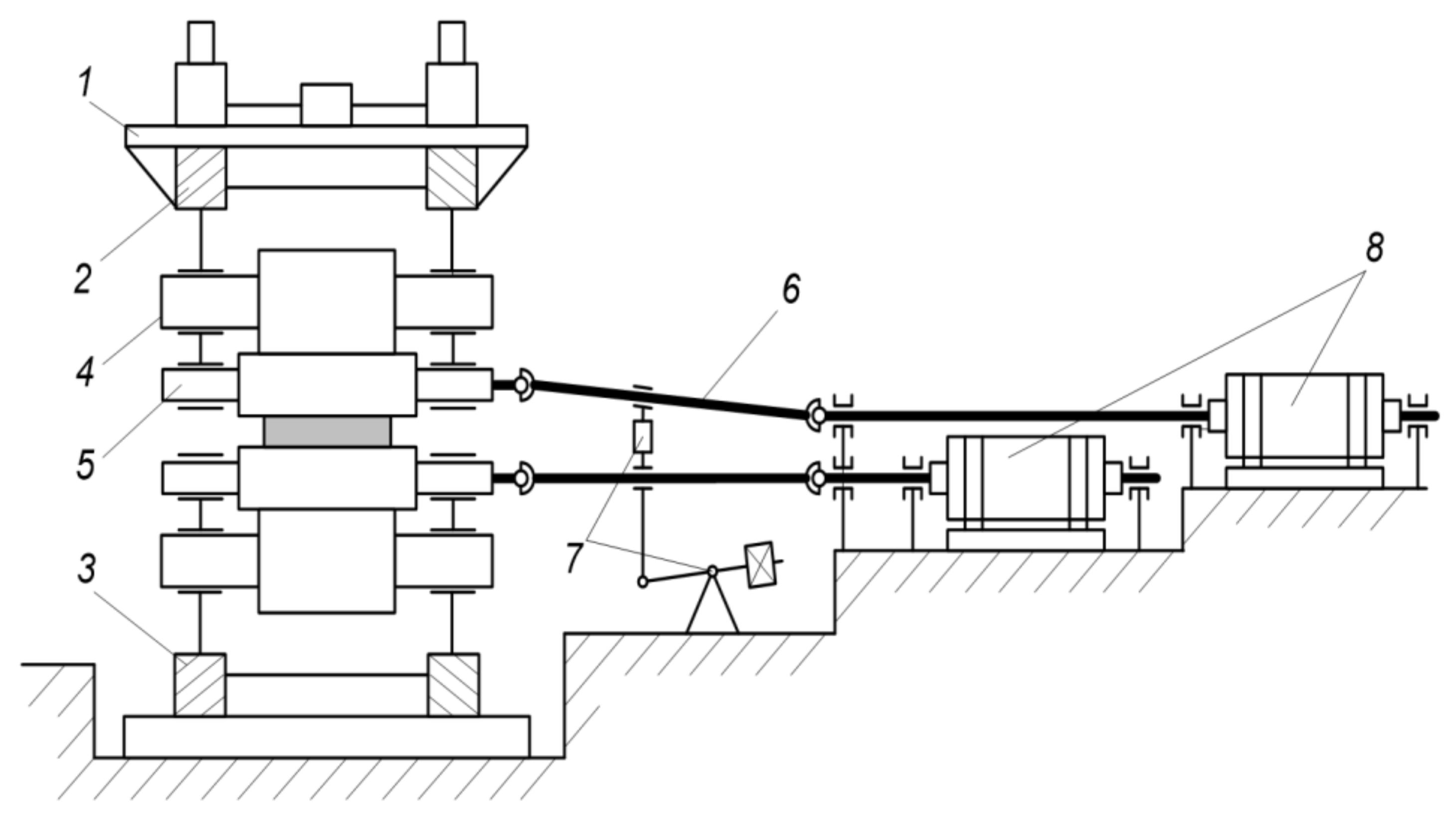
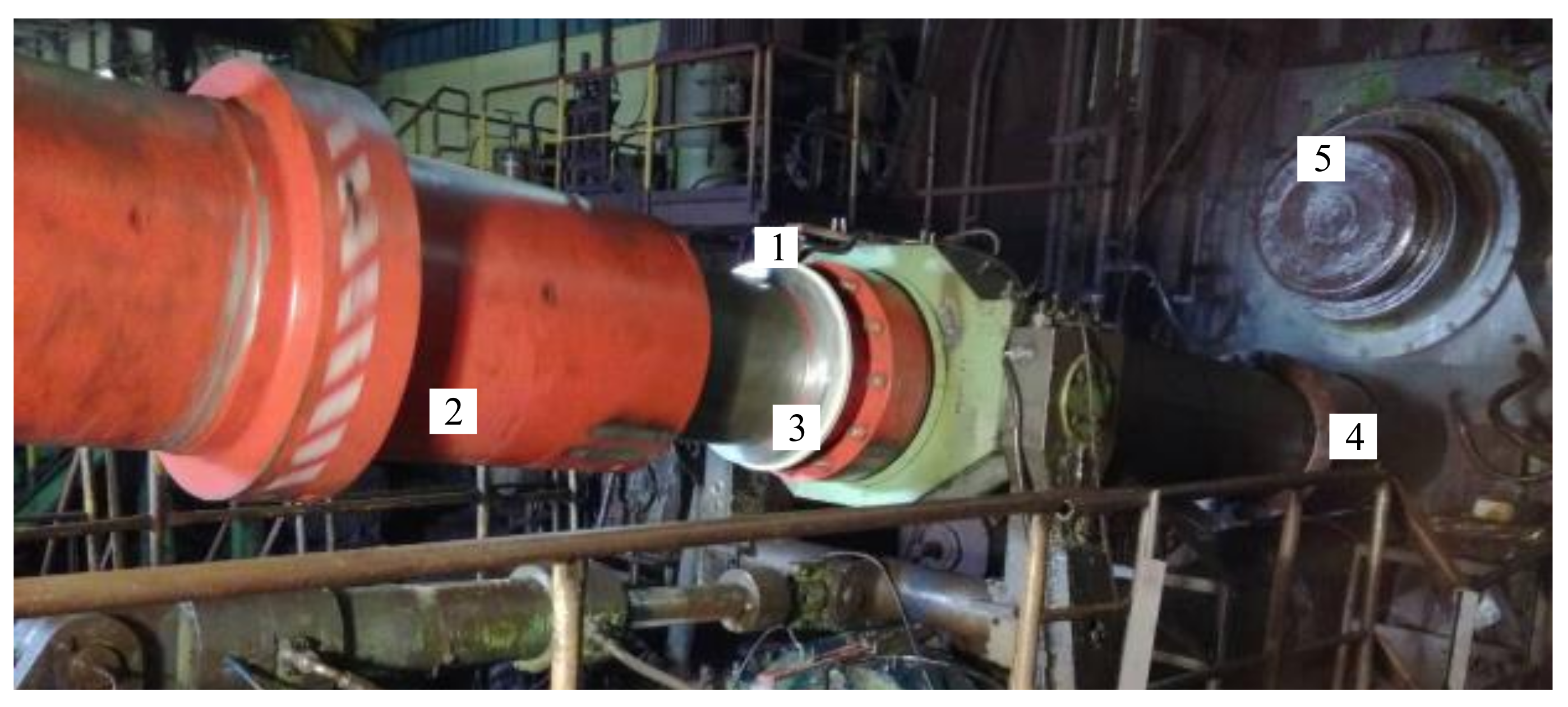




| Parameter | Symbol | Dimensions | Value |
|---|---|---|---|
| Moment of inertia of the first moving mass (the motor) | J1 | kg∙m2 | 125,000 |
| Moment of inertia of the second moving mass (the roll) | J2 | kg∙m2 | 114,571 |
| Elastic coupling rigidity | c12 | N∙m/rad | 5,934,842 |
| Eigenfrequency of elastic oscillations | ω12 | rad/s | 9.96 |
| Electric drive acceleration | ε0 | rad/s2 | 1–3 |
| Transmission gap | δ | rad | 0.017–0.051 (1–3°) |
| Mean elastic torque | M12 | MN∙m | 1.9 |
| Damping ratio | β | - | 2.817 |
| Attenuation decrement | ξ | - | 0.172 |
| Speed controller gain | ksc | - | 19.5 |
| Speed controller time constant | Tsc | s | 0.0041 |
| Gauge | Measured Values | Observer Values | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Mmax (Gapped) | Mmax (Gapless) | ΔMmax (Gap/Gapl.) | Mmax(Obs.) | |ΔMmax| (Obs./Gap) | |ΔMmax| (Obs./Gapl.) | ||||
| mm | p.u. | p.u. | p.u. | % | p.u. | p.u. | % | p.u. | % |
| 9 | 1.55 | 1.45 | 0.1 | 6.9 | 1.35 | 0.2 | 13.8 | 0.1 | 6.9 |
| 12 | 1.65 | 1.54 | 0.11 | 7.1 | 1.43 | 0.22 | 14.3 | 0.11 | 7.1 |
| 18 | 1.92 | 1.77 | 0.15 | 8.5 | 1.67 | 0.25 | 14.1 | 0.1 | 5.6 |
| 24 | 2.15 | 2 | 0.15 | 7.5 | 1.89 | 0.26 | 12.1 | 0.11 | 5.5 |
| 30 | 2.4 | 2.21 | 0.19 | 8.6 | 2.12 | 0.28 | 11.7 | 0.09 | 4.1 |
| Parameter | Oscillogram Value | |||||
|---|---|---|---|---|---|---|
| UMD | LMD | |||||
| Shaft torque | Mst | MSmax | kSm | Mst | MSmax | kSm |
| kN∙m | - | kN∙m | - | |||
| 1400 | 2500 | 1.8 | 2250 | 2700 | 1.2 | |
| Motor torque | Mst | MSmax | kSm | Mst | MSmax | kSm |
| kN∙m | - | kN∙m | - | |||
| 1400 | 2000 | 1.4 | 2250 | 2550 | 1.12 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Radionov, A.A.; Karandaev, A.S.; Gasiyarov, V.R.; Loginov, B.M.; Gartlib, E.A. Development of an Automatic Elastic Torque Control System Based on a Two-Mass Electric Drive Coordinate Observer. Machines 2021, 9, 305. https://doi.org/10.3390/machines9120305
Radionov AA, Karandaev AS, Gasiyarov VR, Loginov BM, Gartlib EA. Development of an Automatic Elastic Torque Control System Based on a Two-Mass Electric Drive Coordinate Observer. Machines. 2021; 9(12):305. https://doi.org/10.3390/machines9120305
Chicago/Turabian StyleRadionov, Andrey A., Alexandr S. Karandaev, Vadim R. Gasiyarov, Boris M. Loginov, and Ekaterina A. Gartlib. 2021. "Development of an Automatic Elastic Torque Control System Based on a Two-Mass Electric Drive Coordinate Observer" Machines 9, no. 12: 305. https://doi.org/10.3390/machines9120305
APA StyleRadionov, A. A., Karandaev, A. S., Gasiyarov, V. R., Loginov, B. M., & Gartlib, E. A. (2021). Development of an Automatic Elastic Torque Control System Based on a Two-Mass Electric Drive Coordinate Observer. Machines, 9(12), 305. https://doi.org/10.3390/machines9120305








