Detachment Detection in Cam Follower System Due to Nonlinear Dynamics Phenomenon
Abstract
:1. Introduction
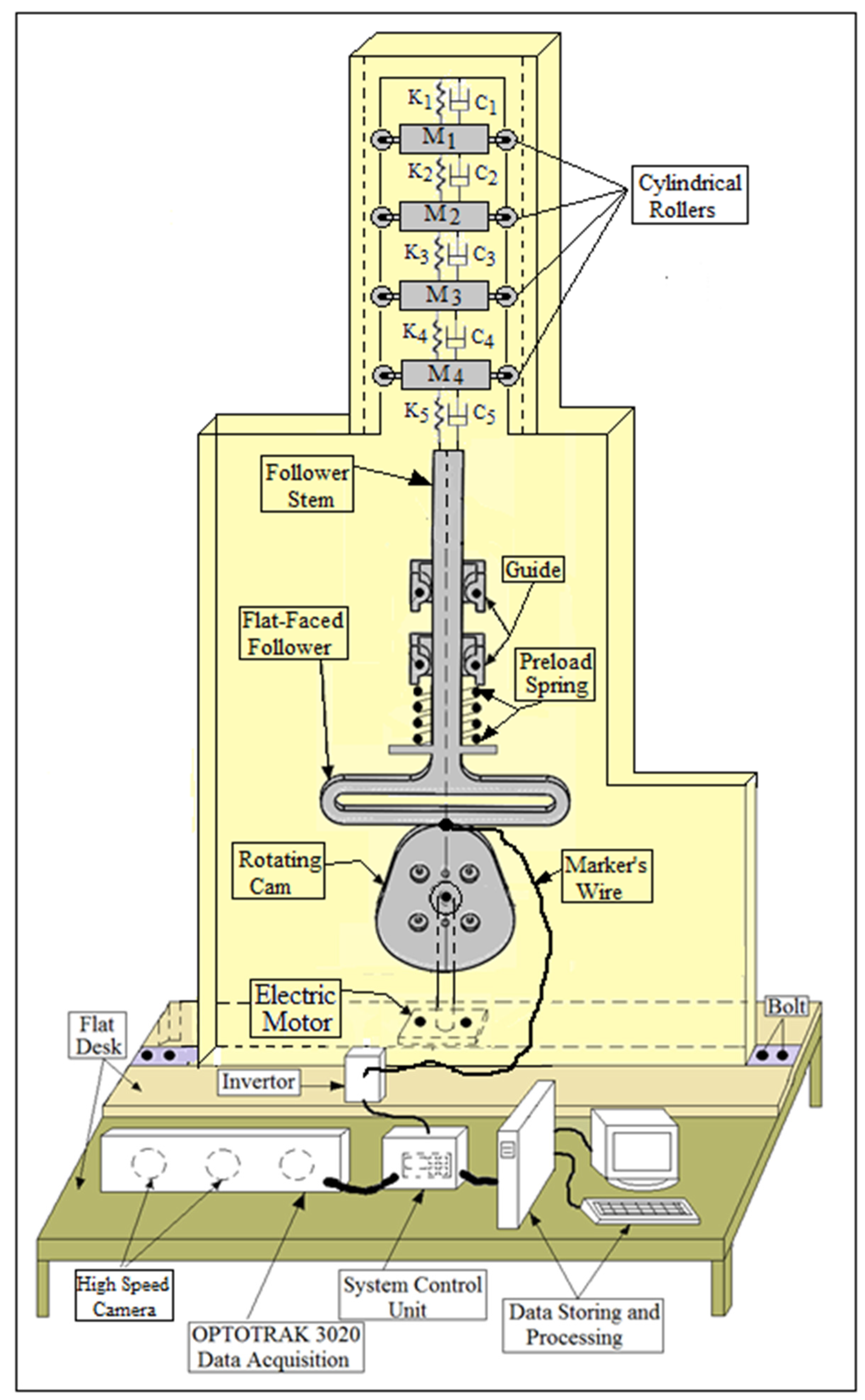
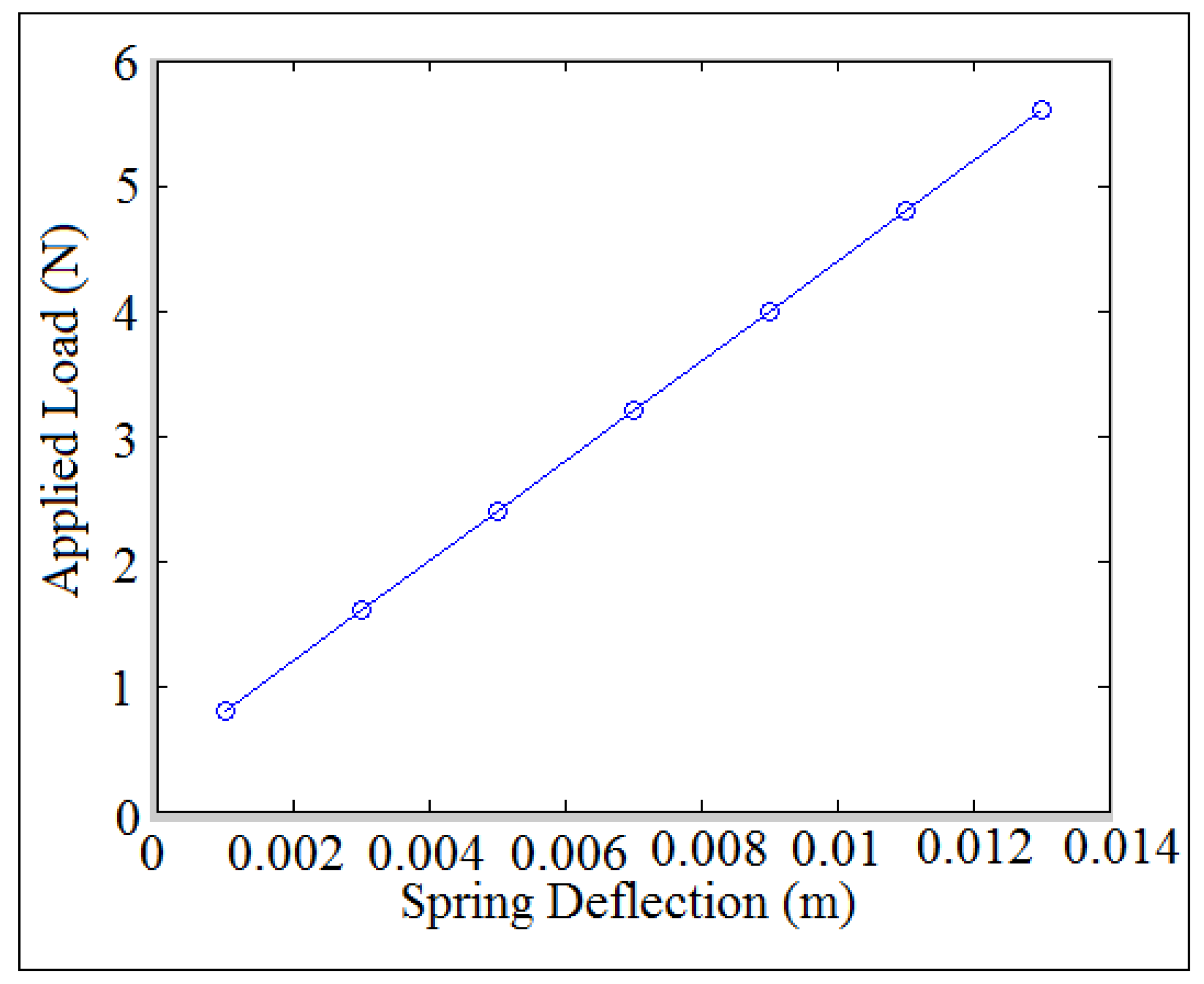
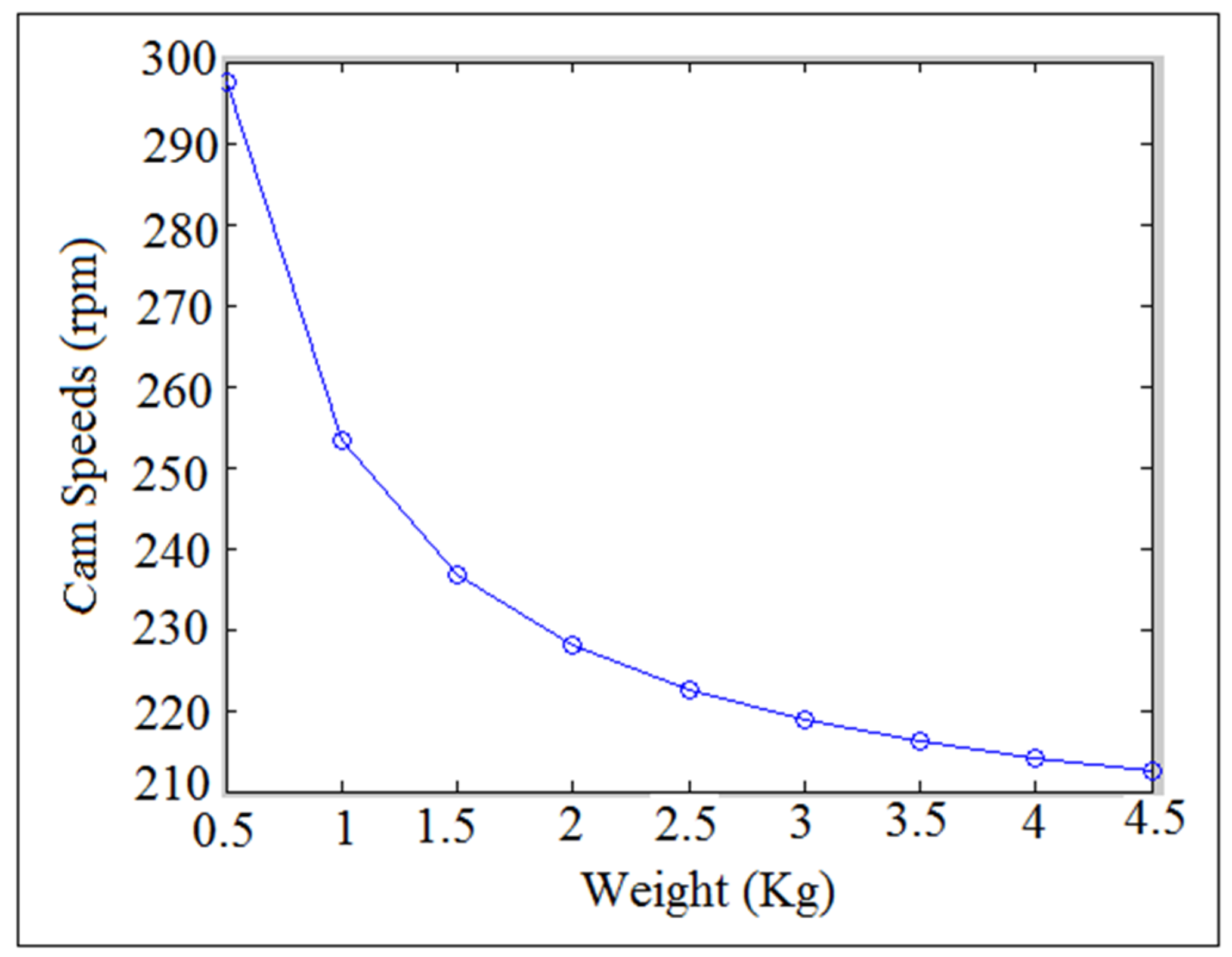
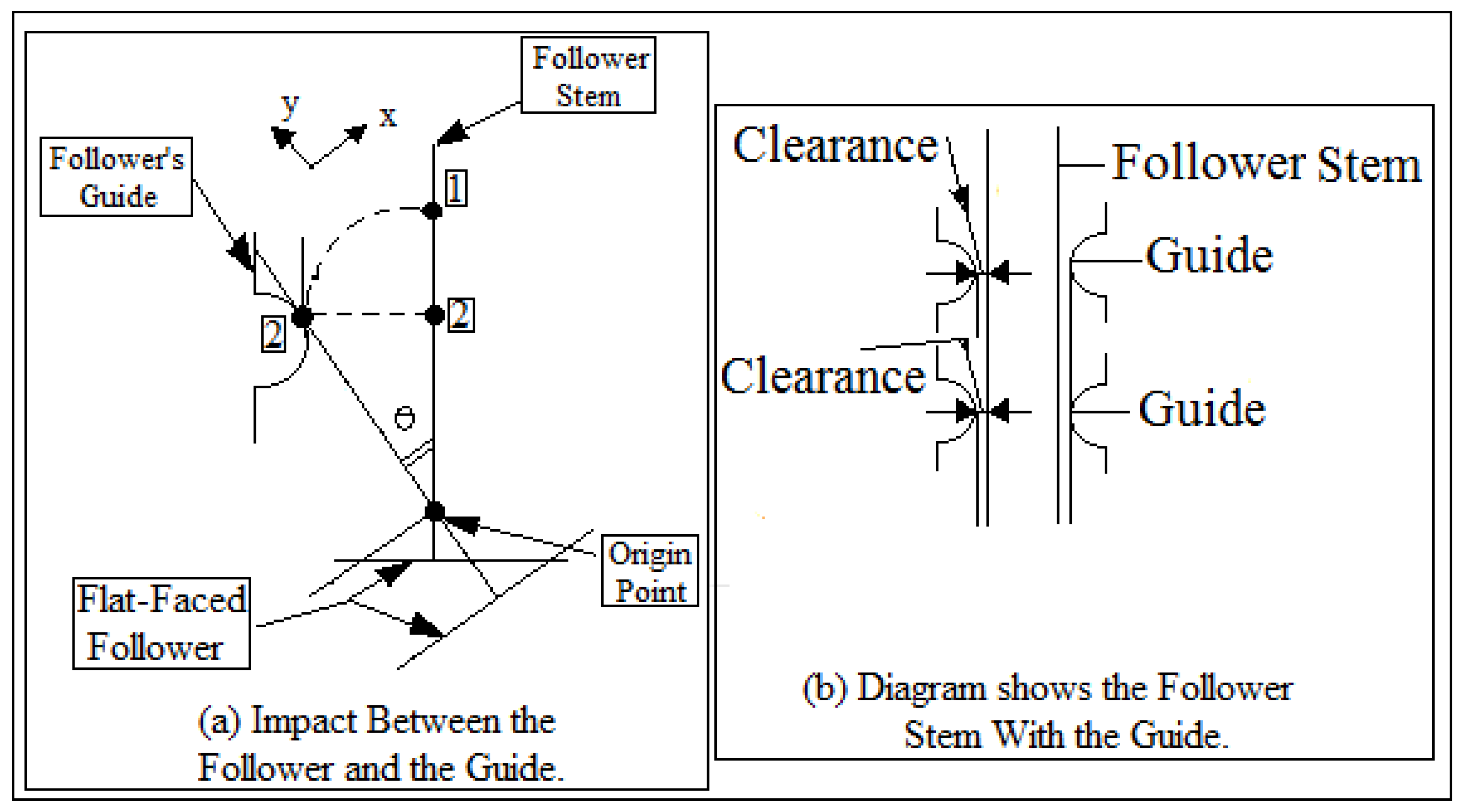
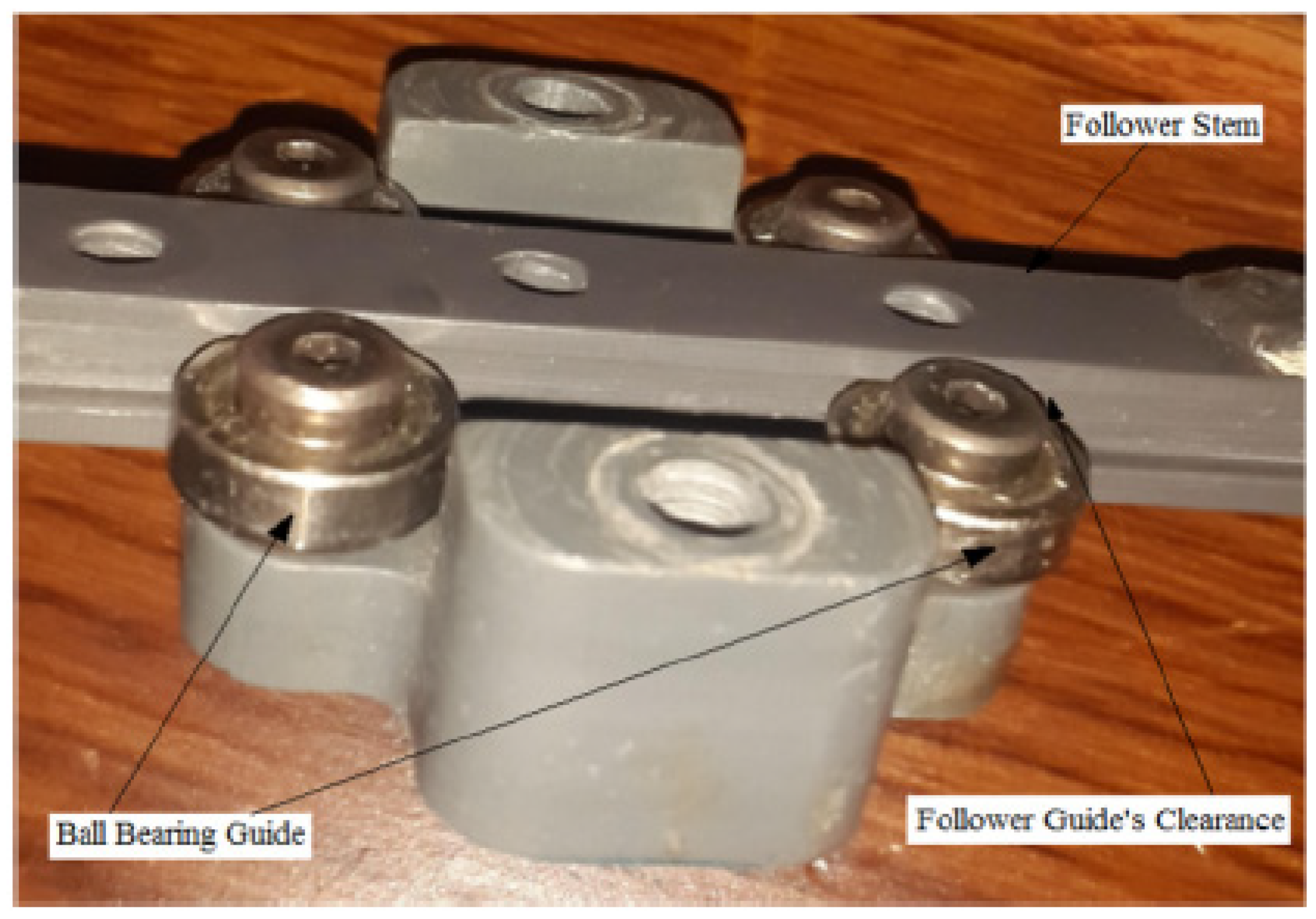
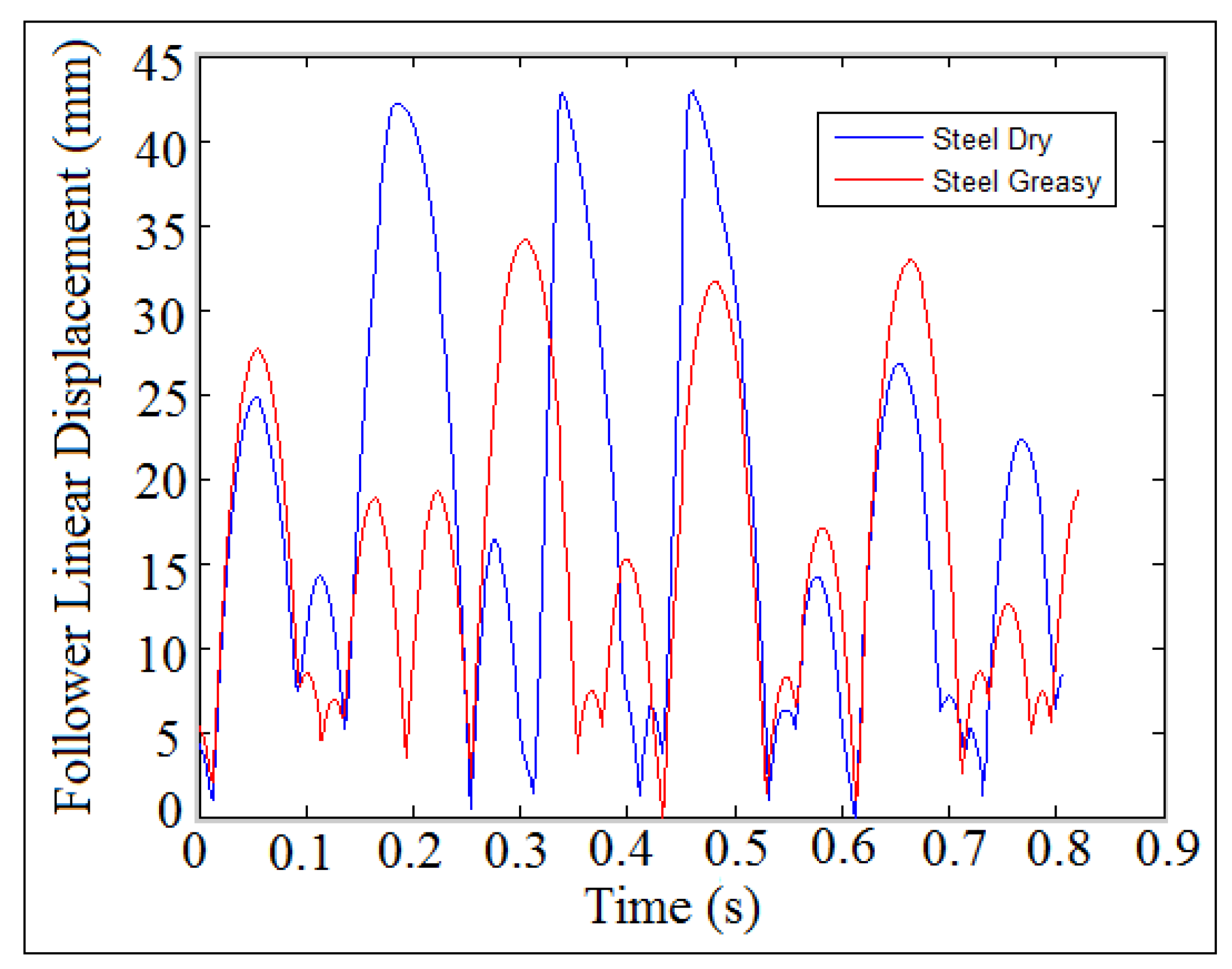
2. Experiment Test
- Δh is the change in follower displacement when the follower is turned with an angle (θ) about z-axis, mm.
- g is the gravitational acceleration, mm/s2
- is the change in time when the follower displacement has been changed, s.
- r is the minimum radius in the cam profile which is equal to (22.5 mm), [16].
- S is the total force acting on the follower in the direction of the axis when the mechanism is stopped in the minimum lift position, N.
- is the weight which locates at the end of the follower stem, kg.
- ω is the critical speed of the cam, rpm.
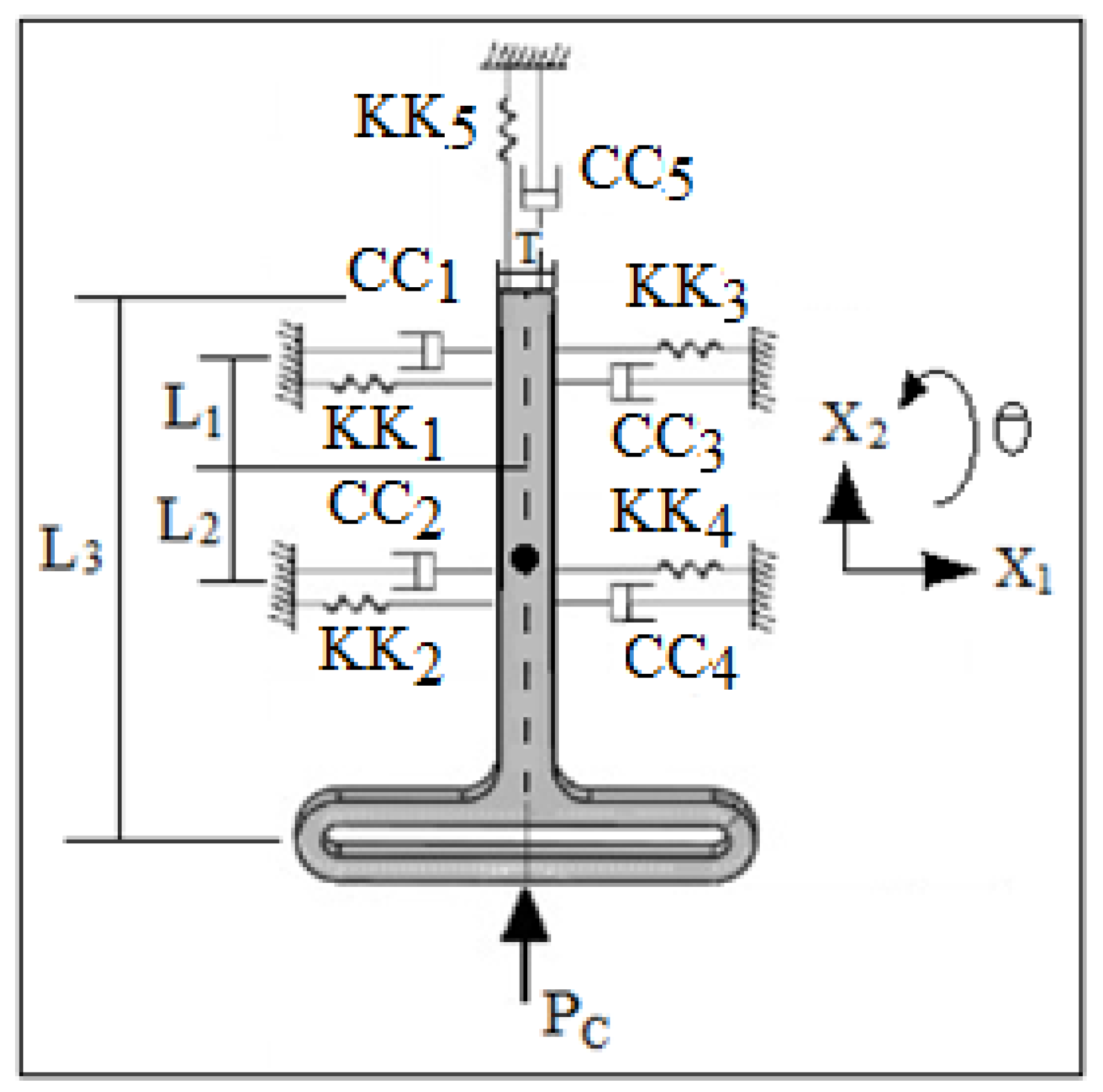
3. Equations of Motion of Three Degrees of Freedom System
4. Numerical Simulation
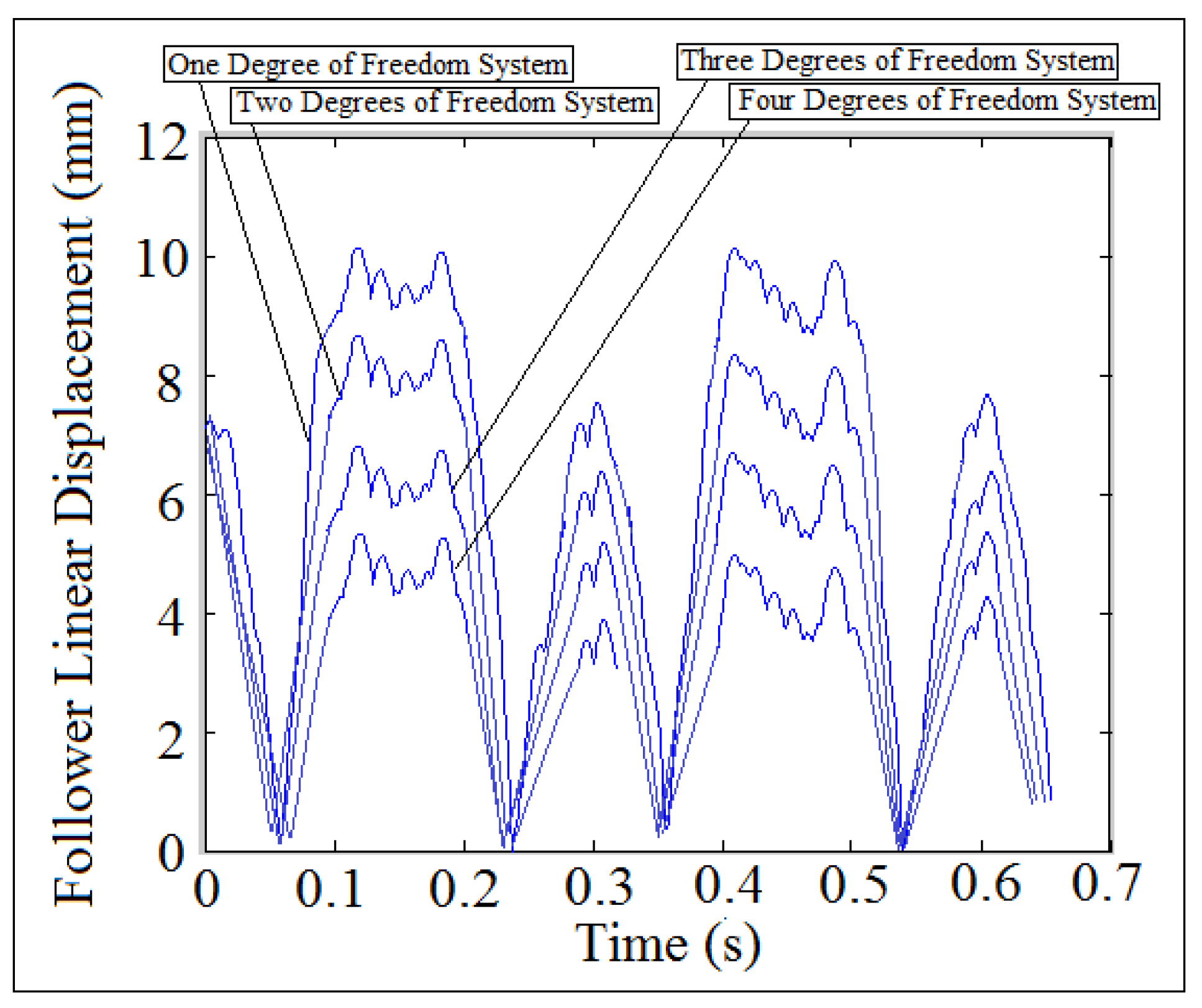
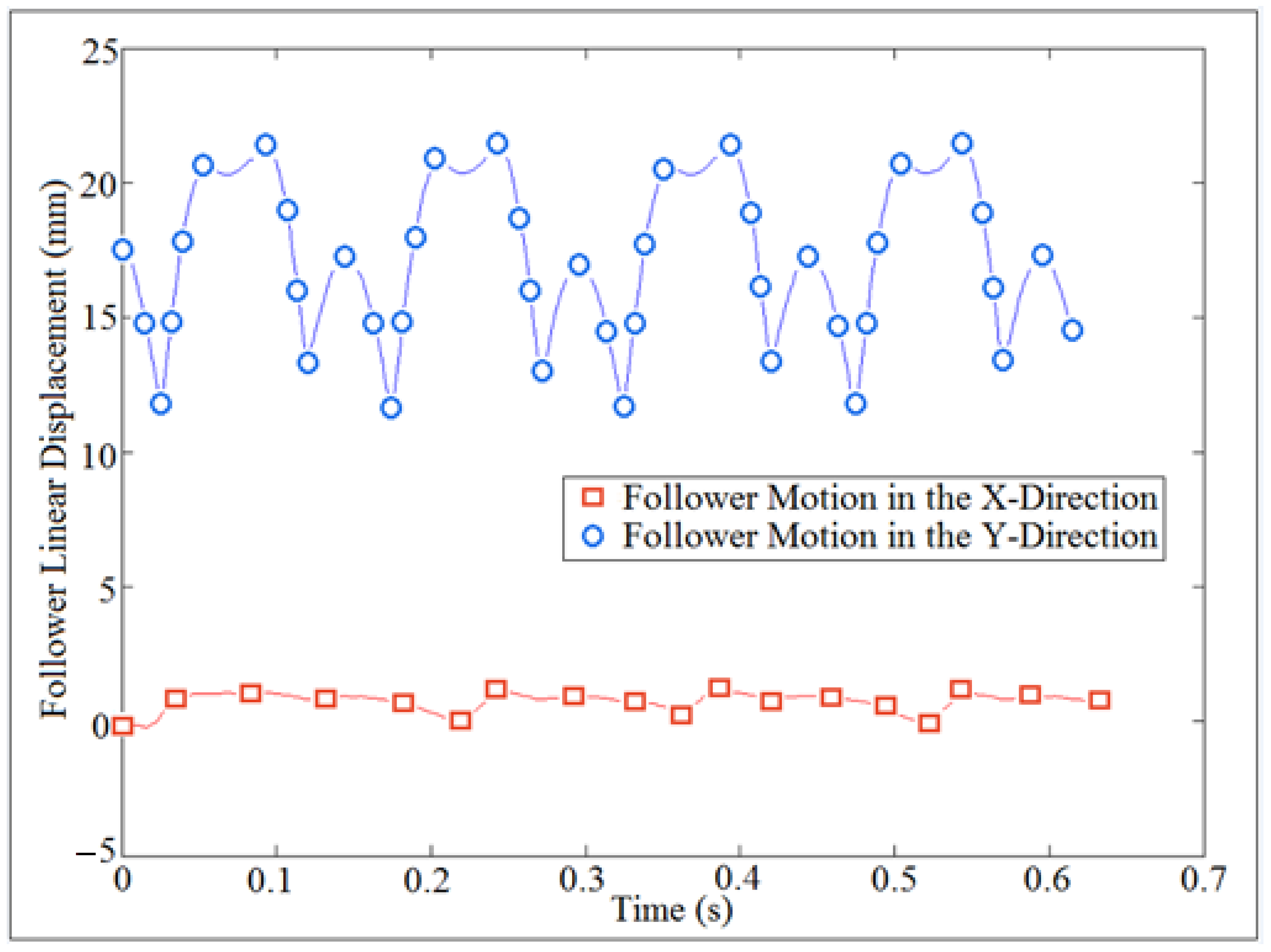
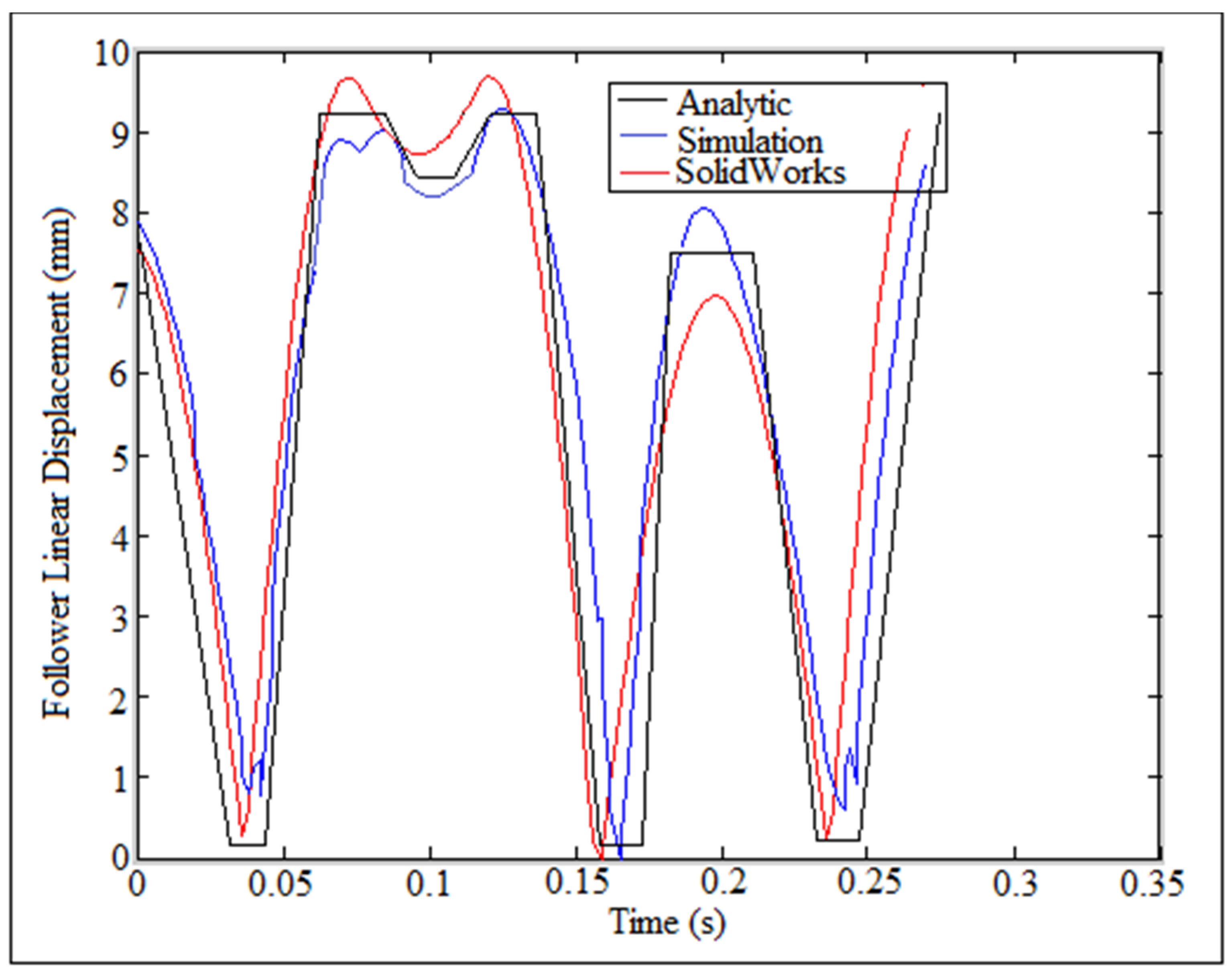
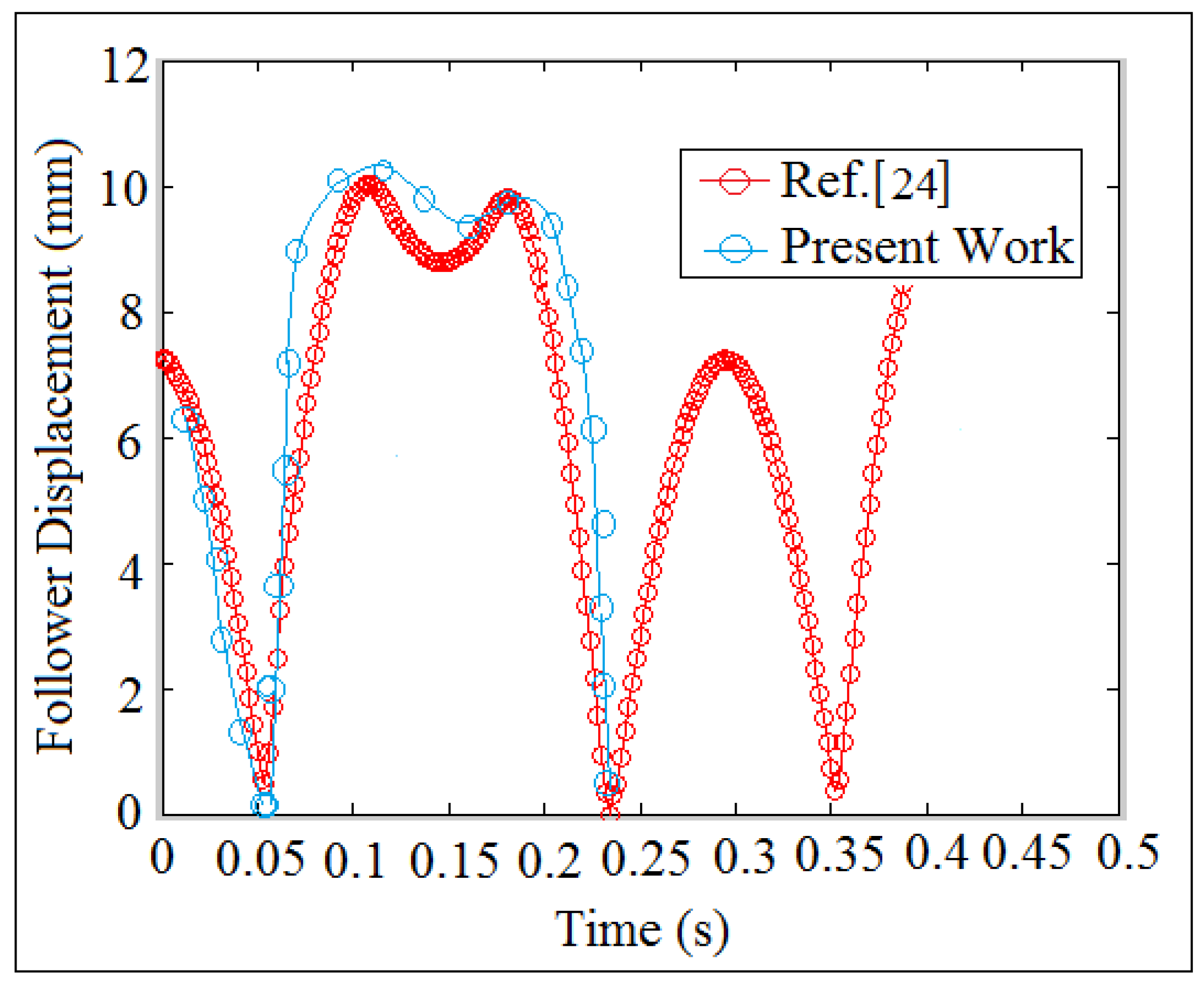
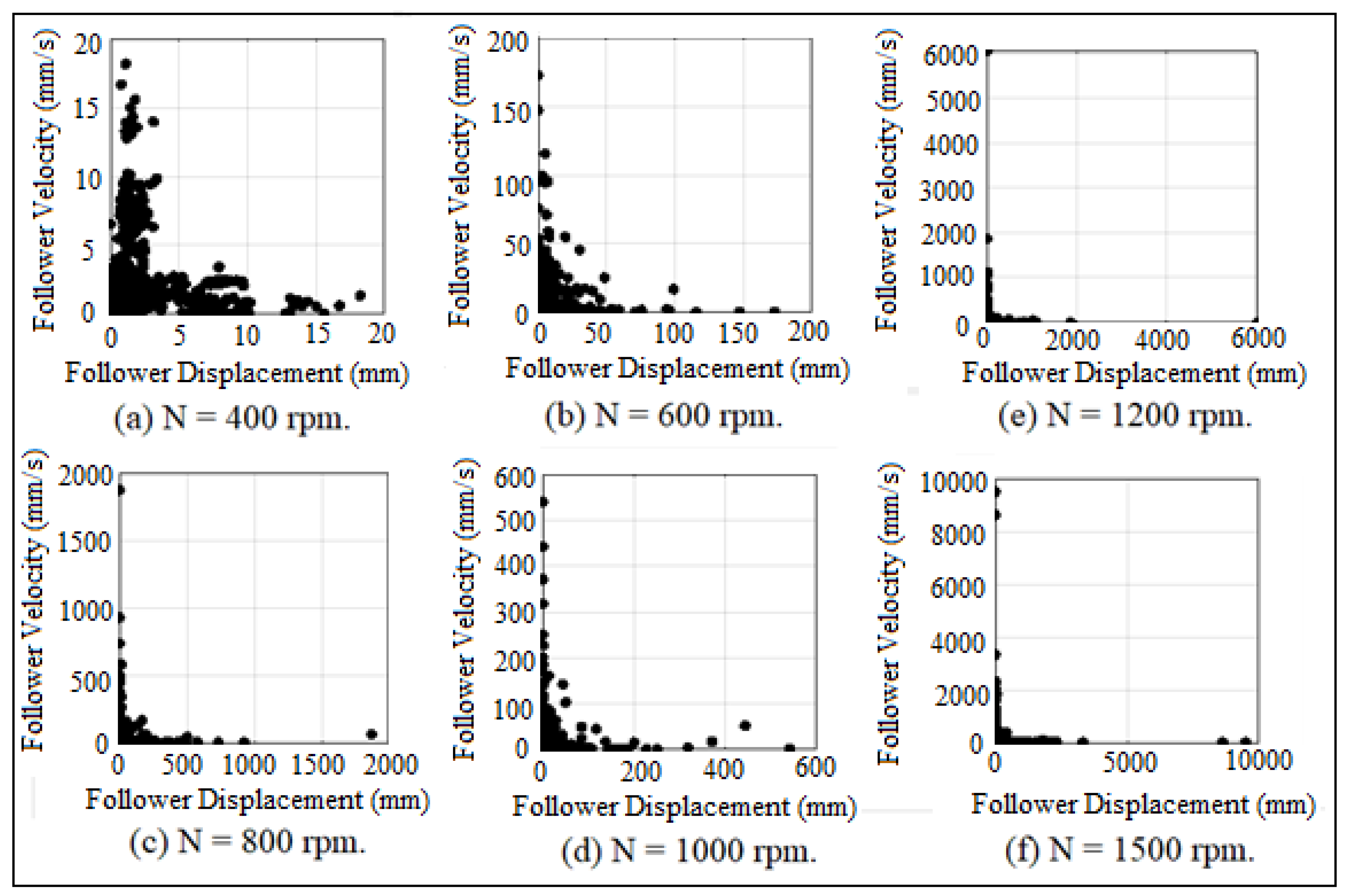
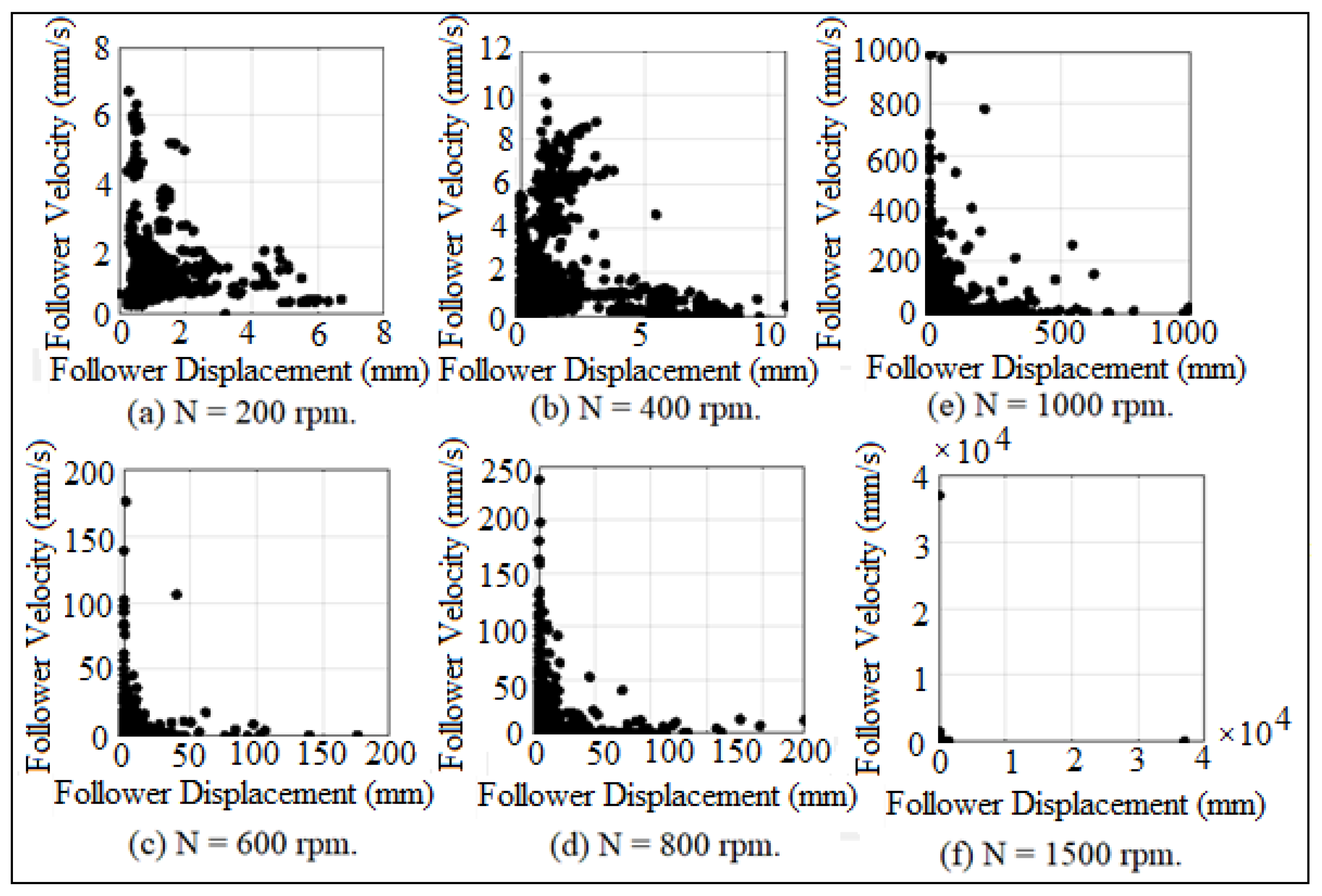
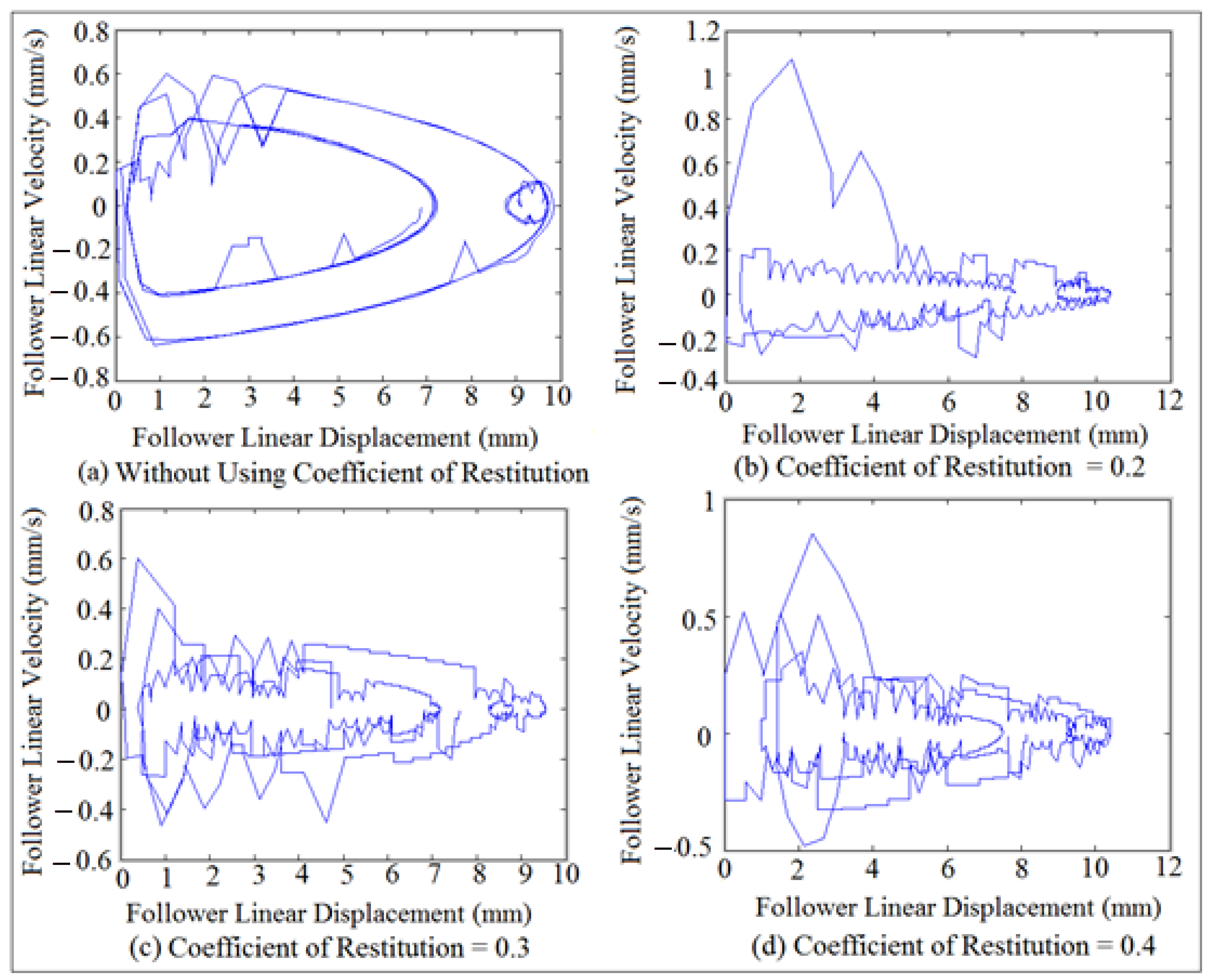
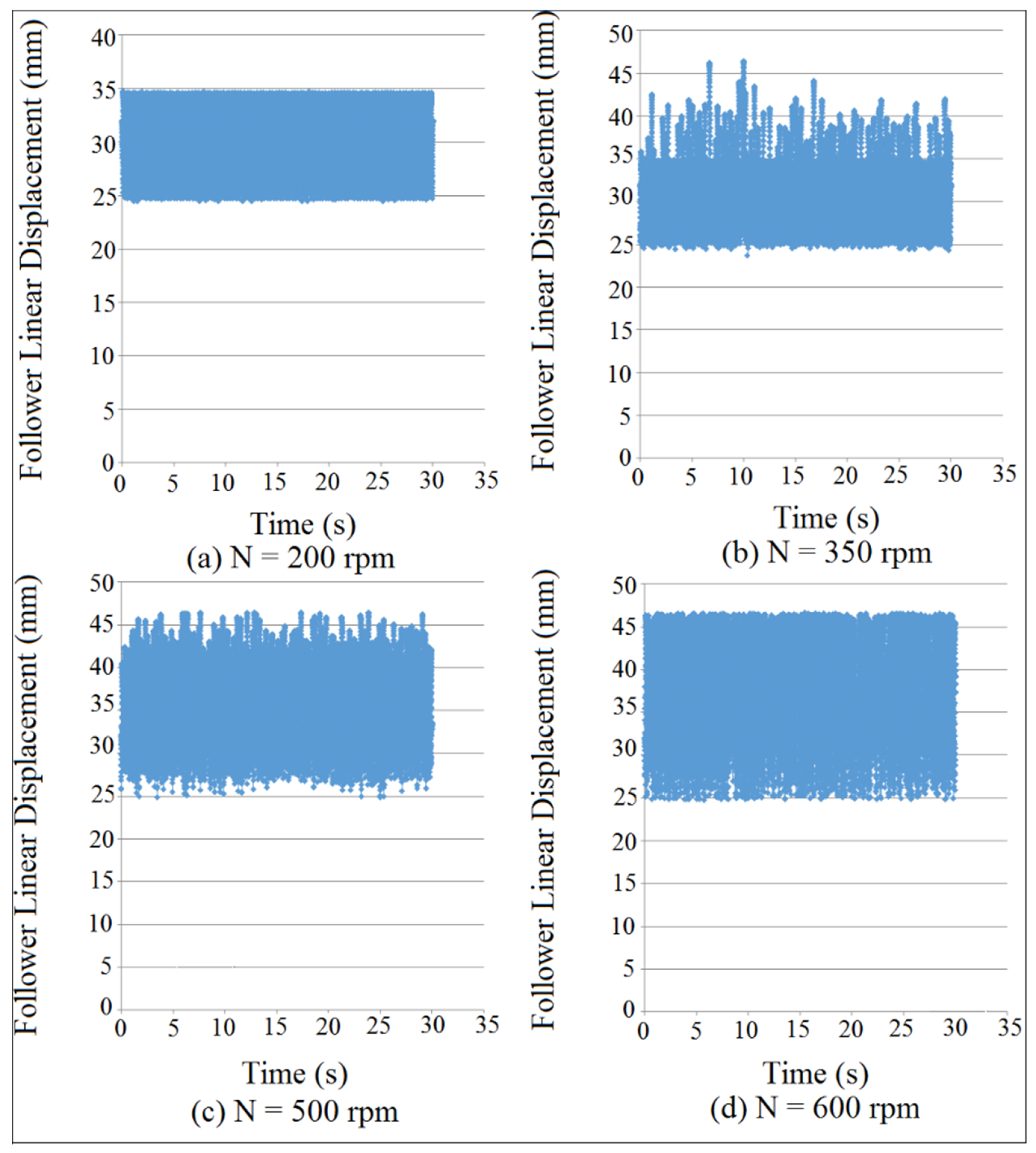
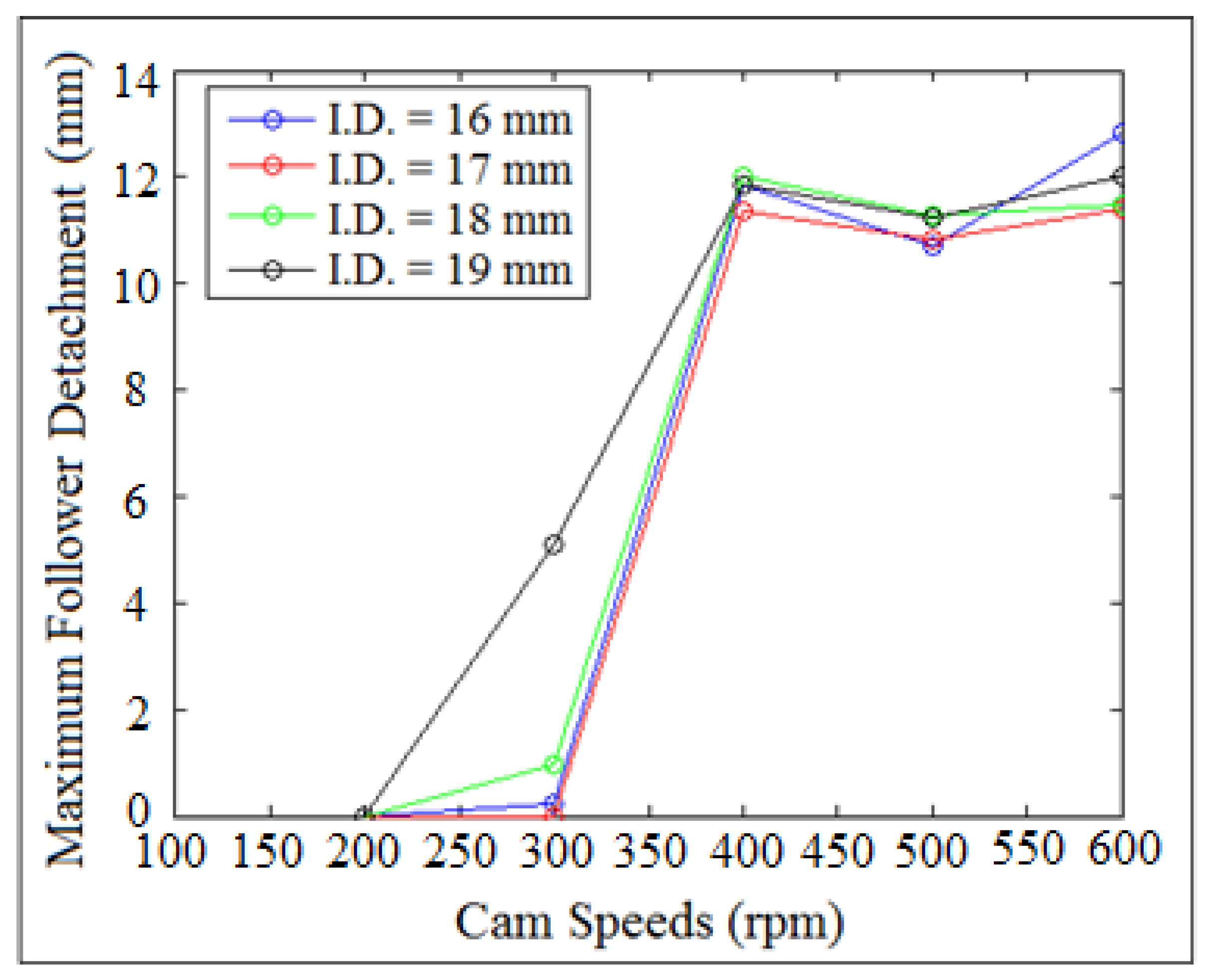
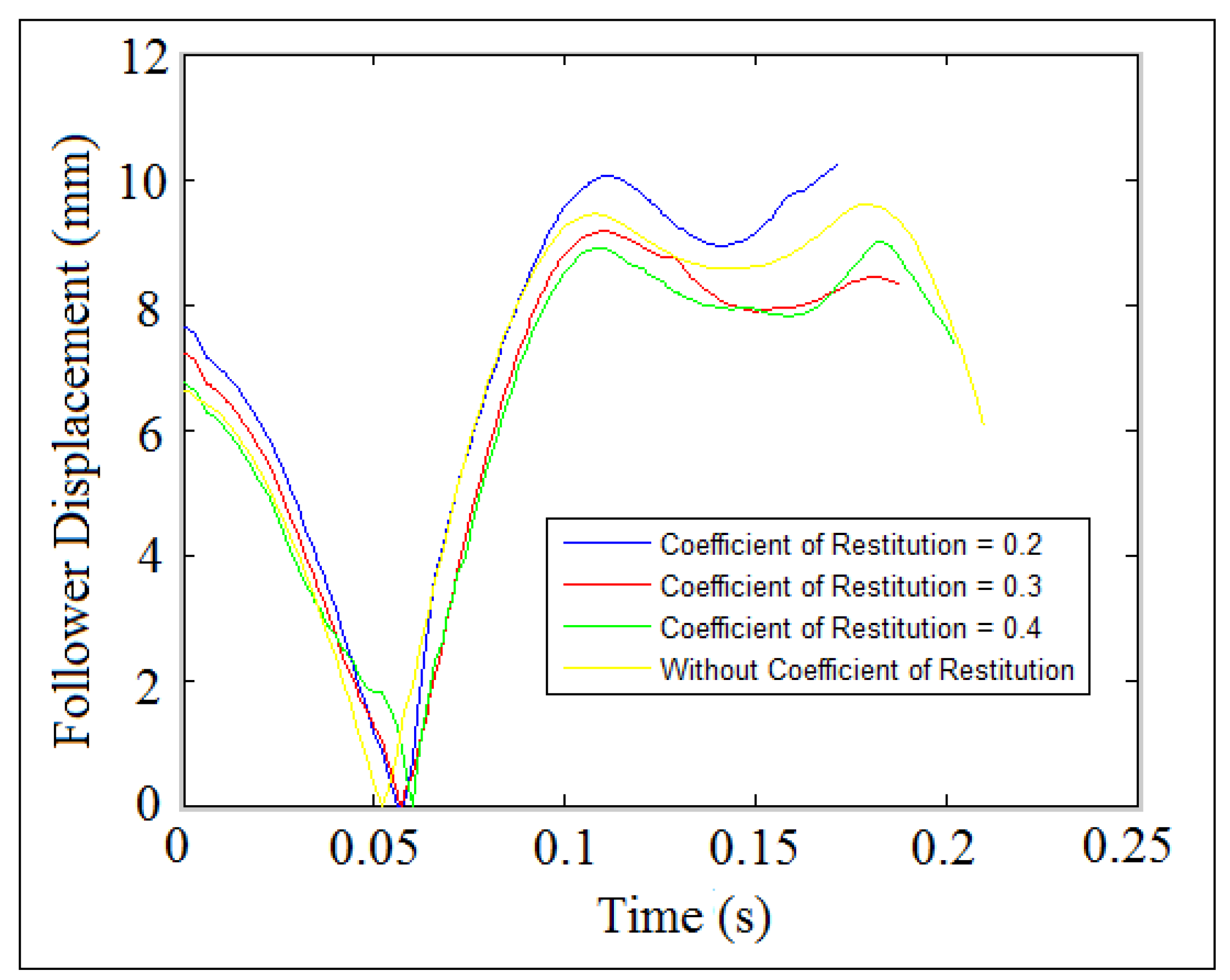
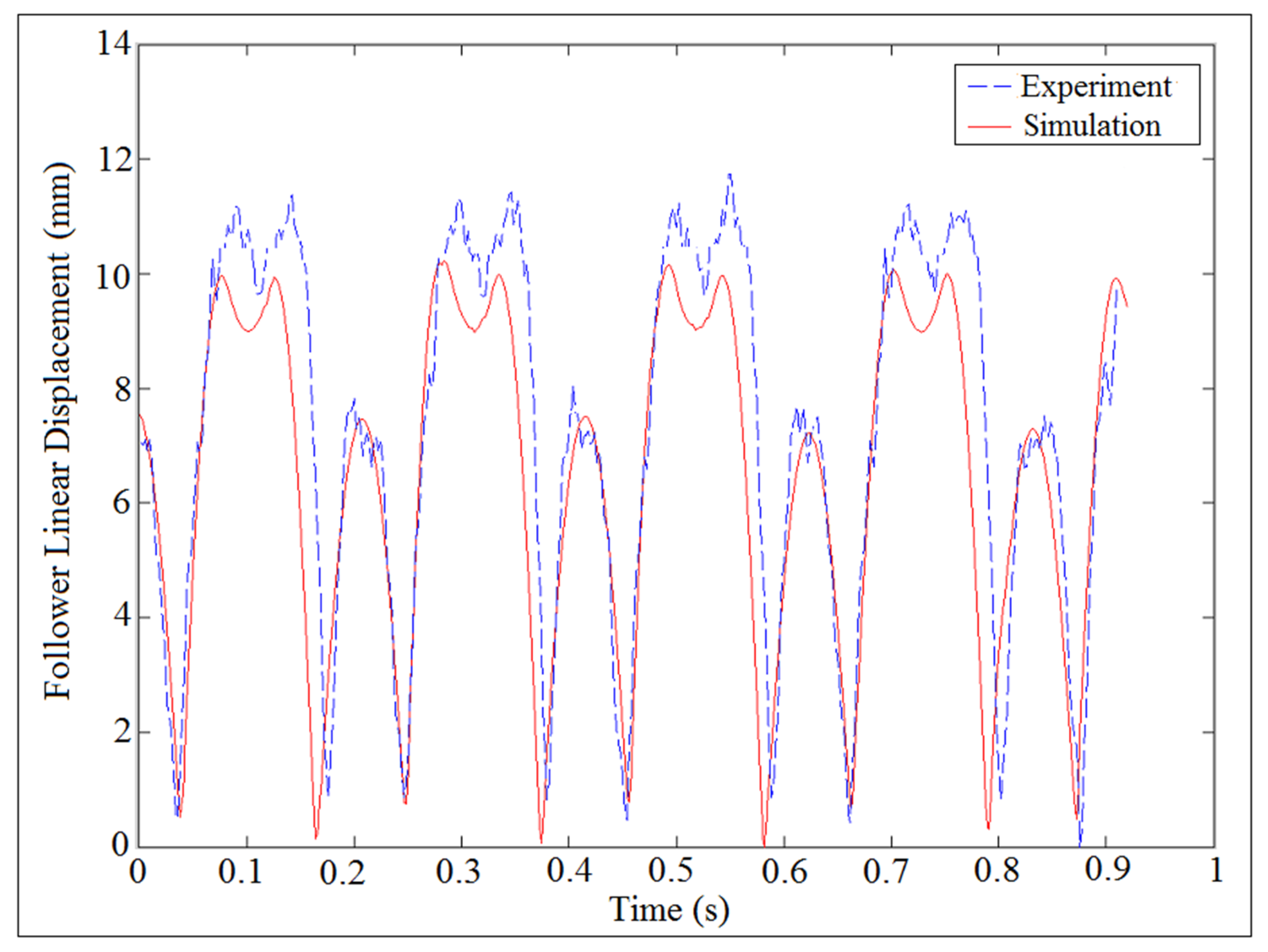
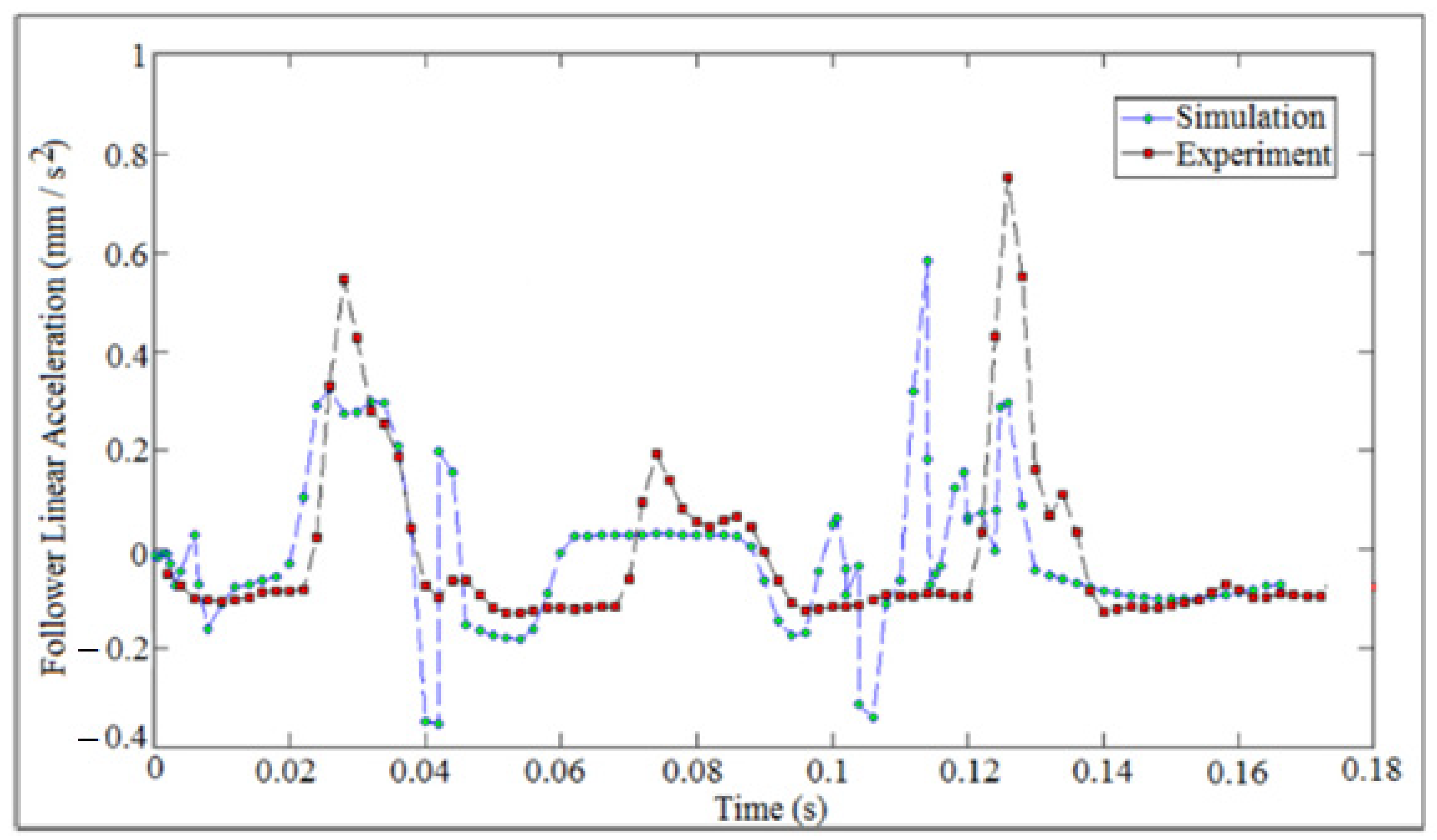
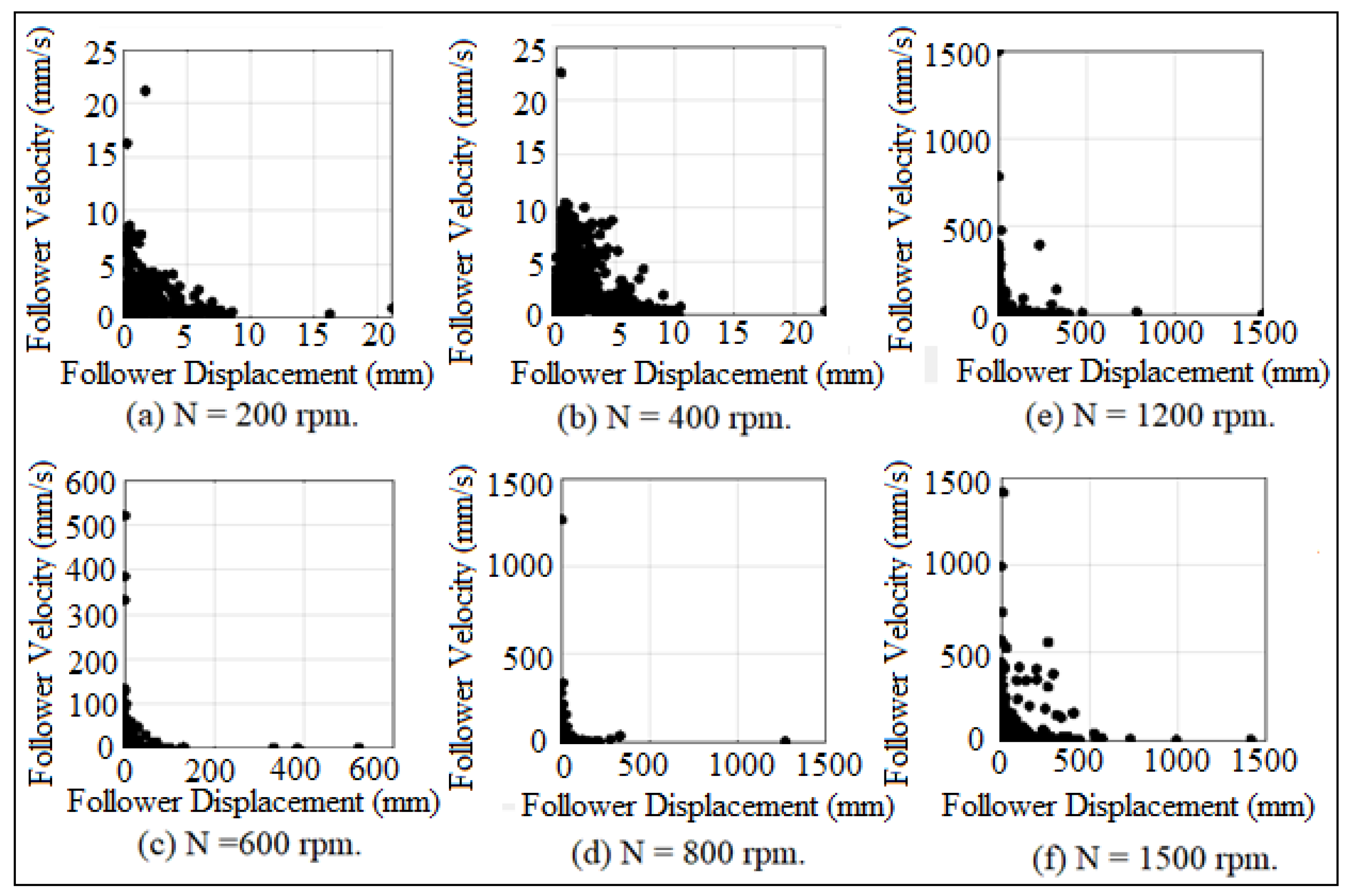
4.1. Detachment Detection through Follower Displacement
- (a)
- SolidWorks program was used in the modeling of computer aided design (CAD), [21]. In the numerical simulation, the follower moved with three degrees of freedom (up-down, right-left, and rotation about z-axes). The two rollers in both sides between the wall and the mass of the spring–damper system helped the multi-degrees of freedom moved up and down as indicated in Figure 5. In SolidWorks program, there were three types of integrator, (GSTIFF), (SI2-GSTIFF) and (WSTIFF), in which the integrator of the type (GSTIFF) was selected. The (GSTIFF) solves the complex nonlinear dynamics system, and it can be operated over a range of speeds of the cam. The integrator (GSTIFF) works with maximum iteration (50), initial integrator step size (0.0001), minimum integrator step size (0.0000001), and maximum integrator step size (0.001). The dimensions of cam, flat-faced follower, and the two guides are taken from Ref. [22] while the dimensions of the outside frame and the four mass boxes are assumed to be arbitrary. The SolidWorks simulation setup can be seen in Appendix B. In regards to the multi-degrees of freedom at the very end of the follower stem, all the springs have the stiffness (K1 = K2 = K3 = K4 = K5 = 73.56 N/mm) while the coefficients of the damping have the values (C1 = C2 = C3 = C4 = C5 = 9.19 Ns/mm). The values of the masses of the four boxes are (M1 = M2 = M3 = M4 = 0.2625 Kg, Mtotal = 1.05 Kg) to reduce the peak of follower displacement of the lift position. The spring constants are the spring index (C = 3), number of turn (n = 6), modulus of rigidity (G = 80 GPa.), coil diameter (d = 2.5 mm), and outside diameter (OD = 10 mm). In regards to the spring between the follower stem and the vertical table, the spring index (C = 3), number of turn (n = 6), modulus of rigidity (G = 80 GPa.), coil diameter (d = 2.5 mm), outside diameter (OD = 10 mm), and the spring stiffness (K = 400 N/m).
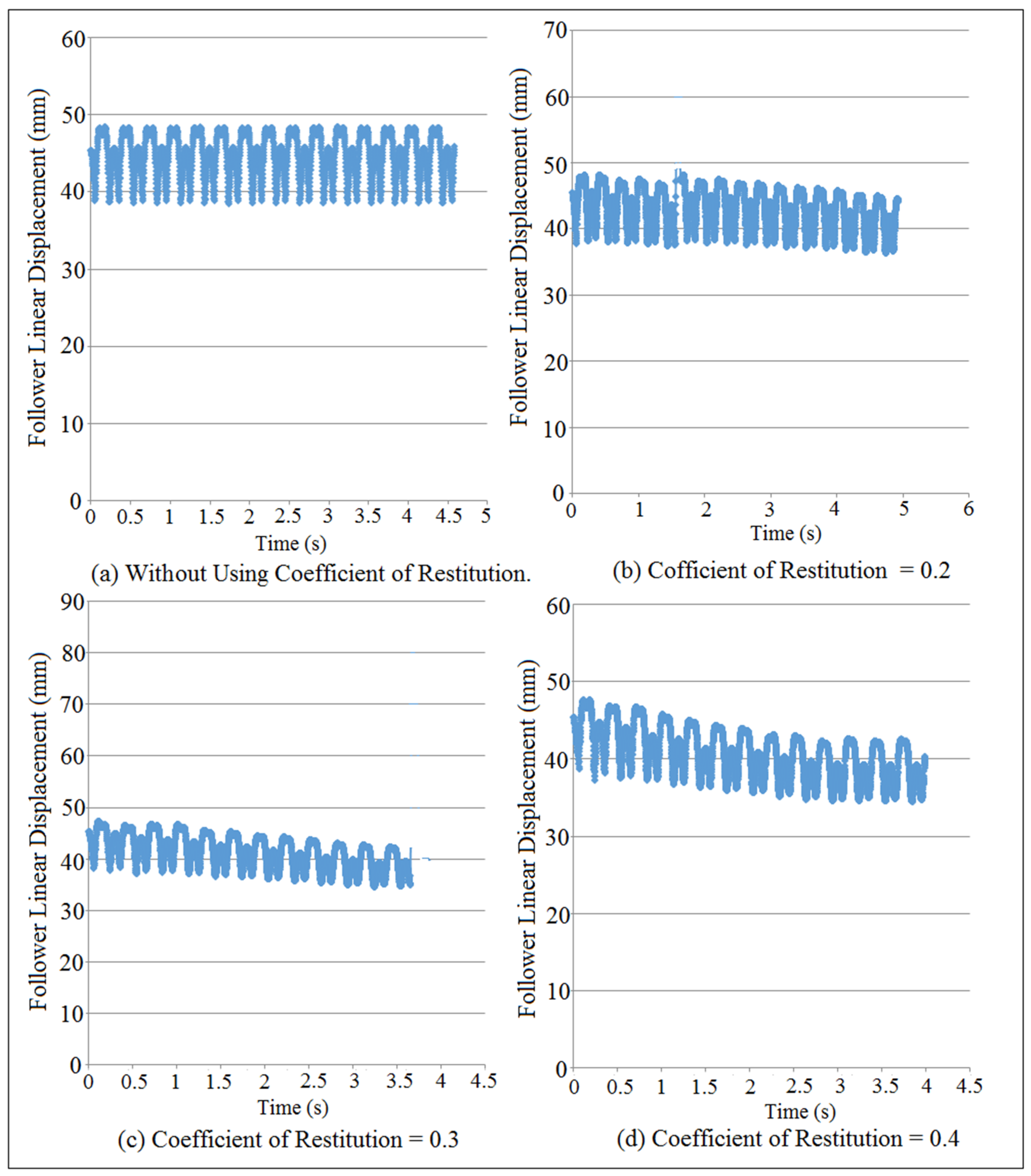
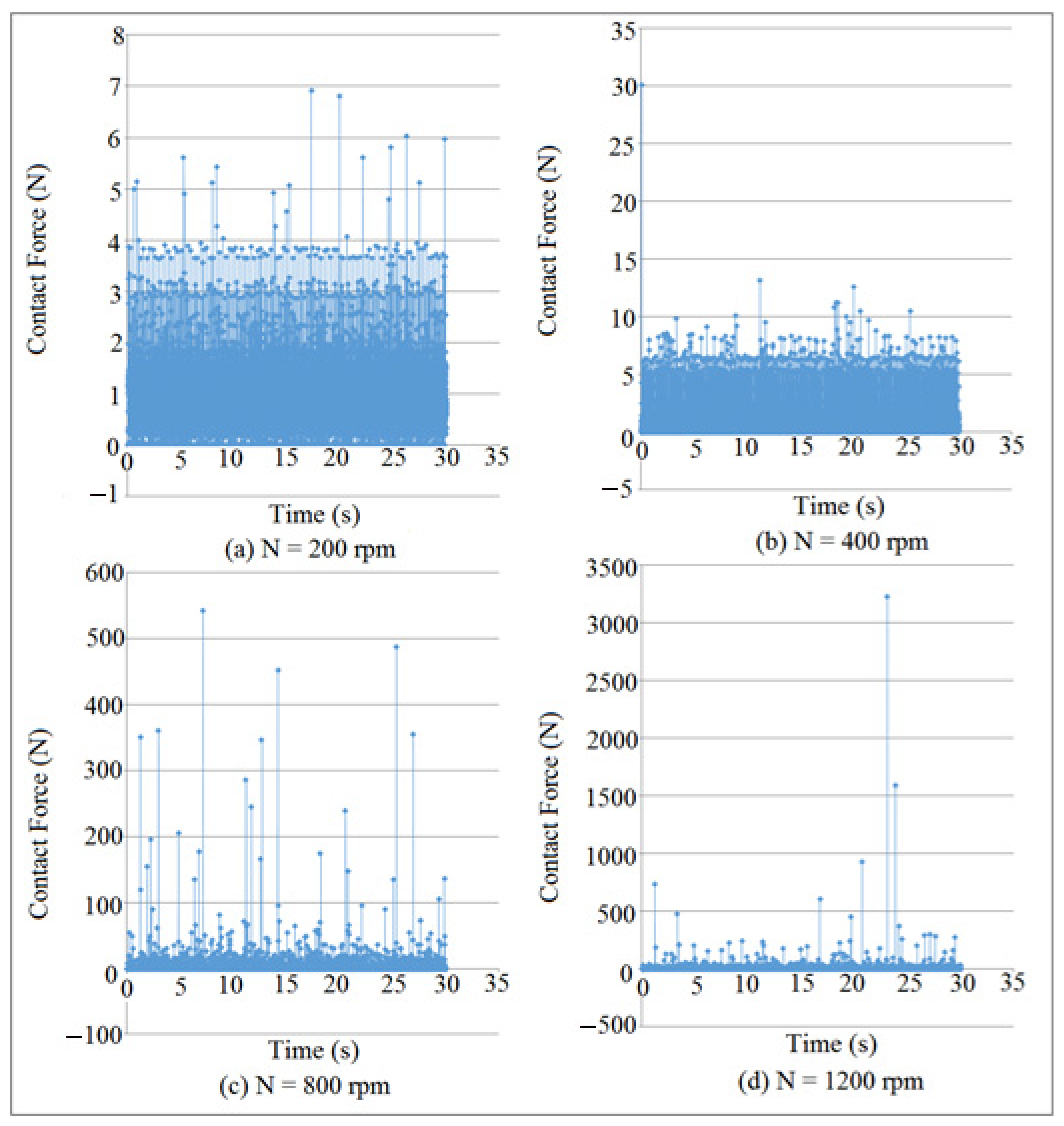
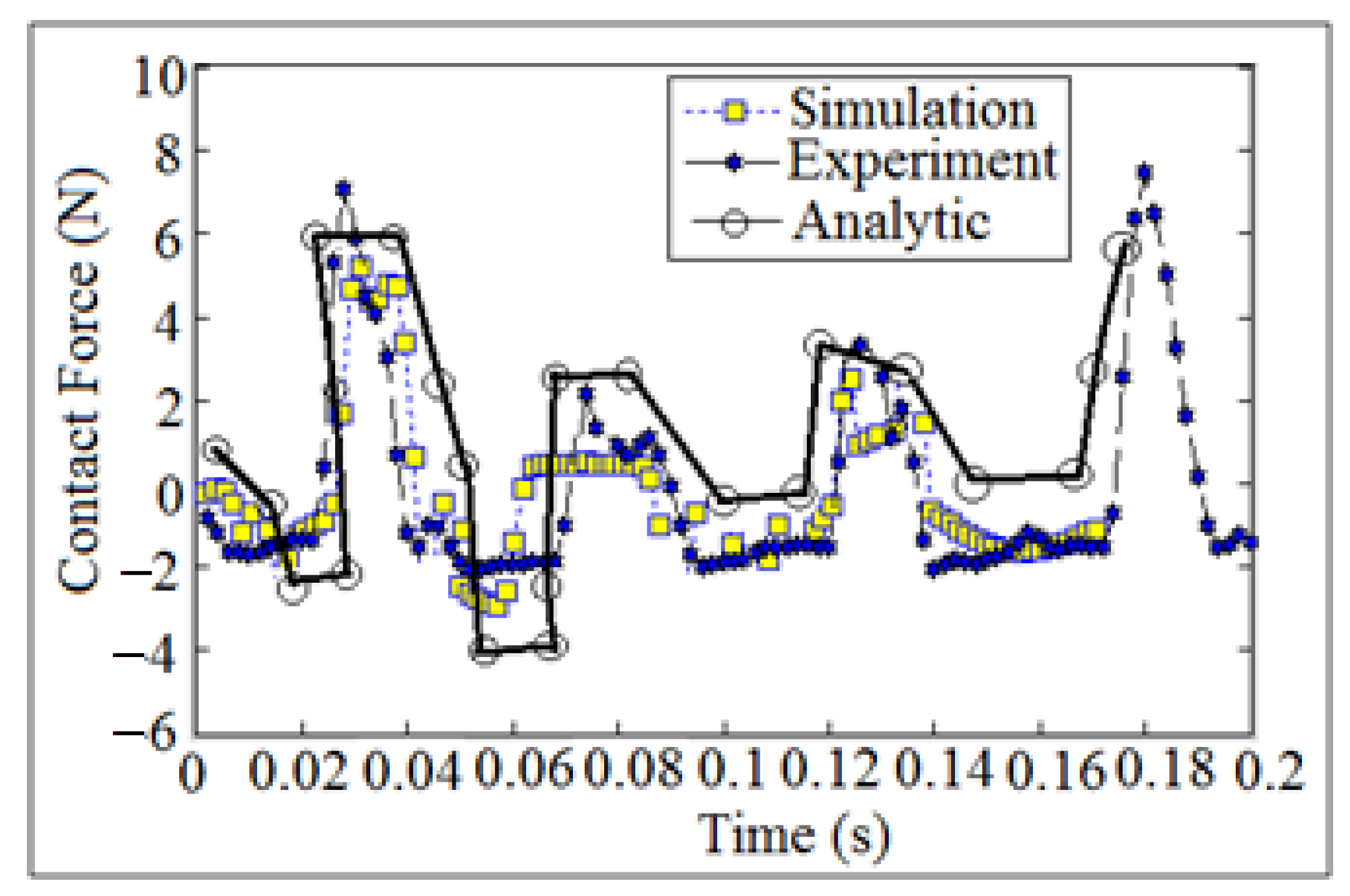
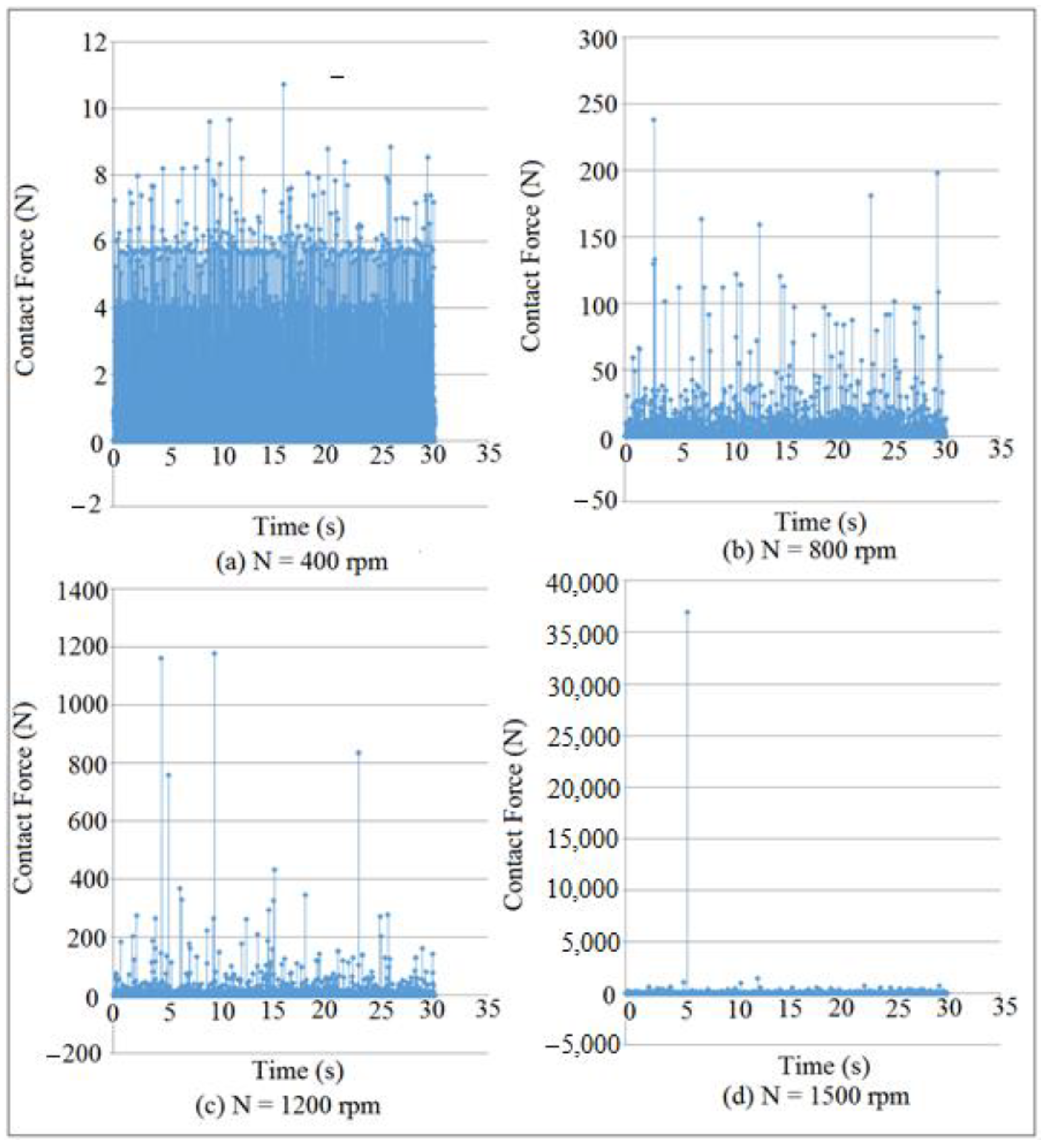
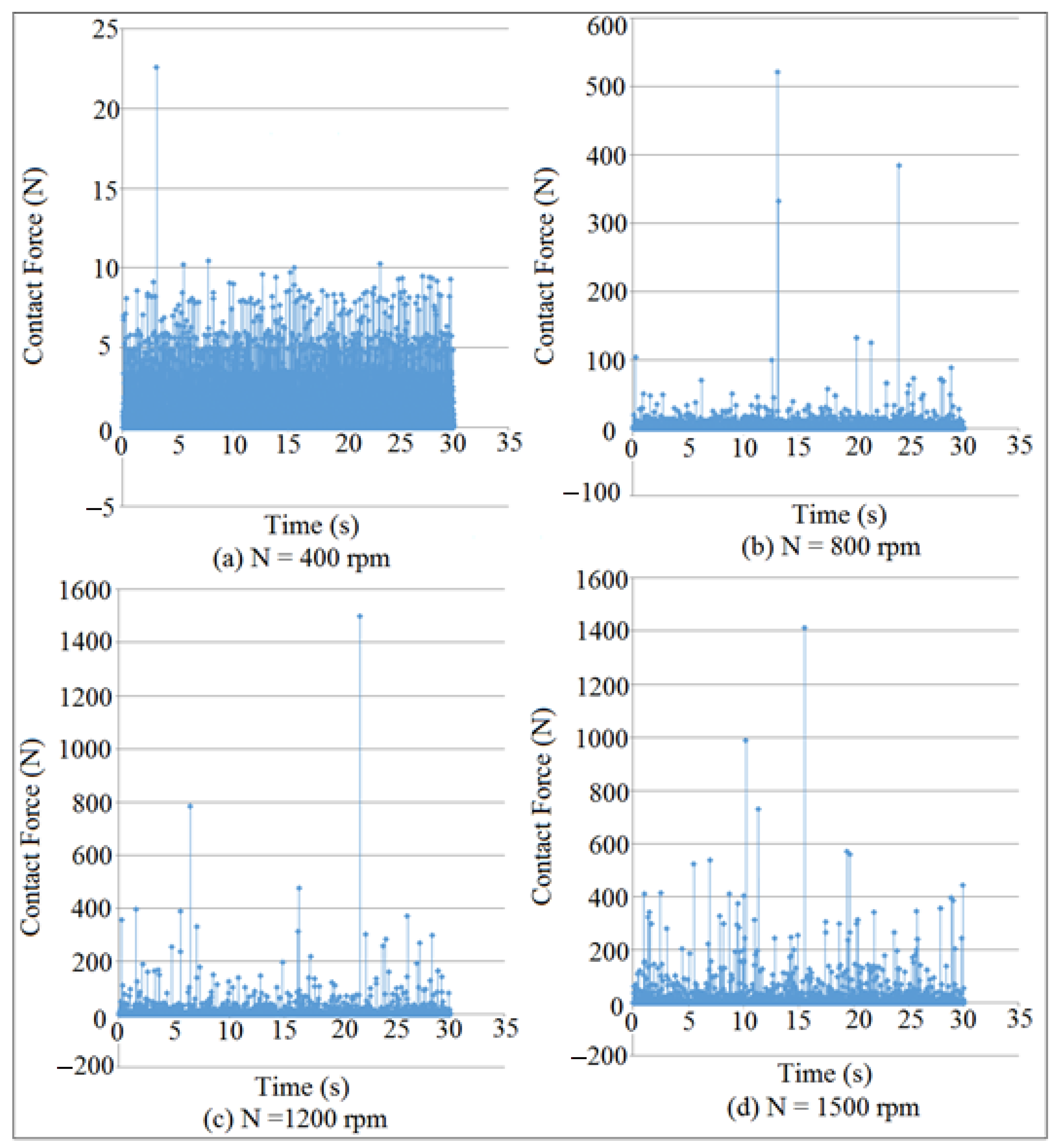
4.2. Detachment Detection through Contact Force
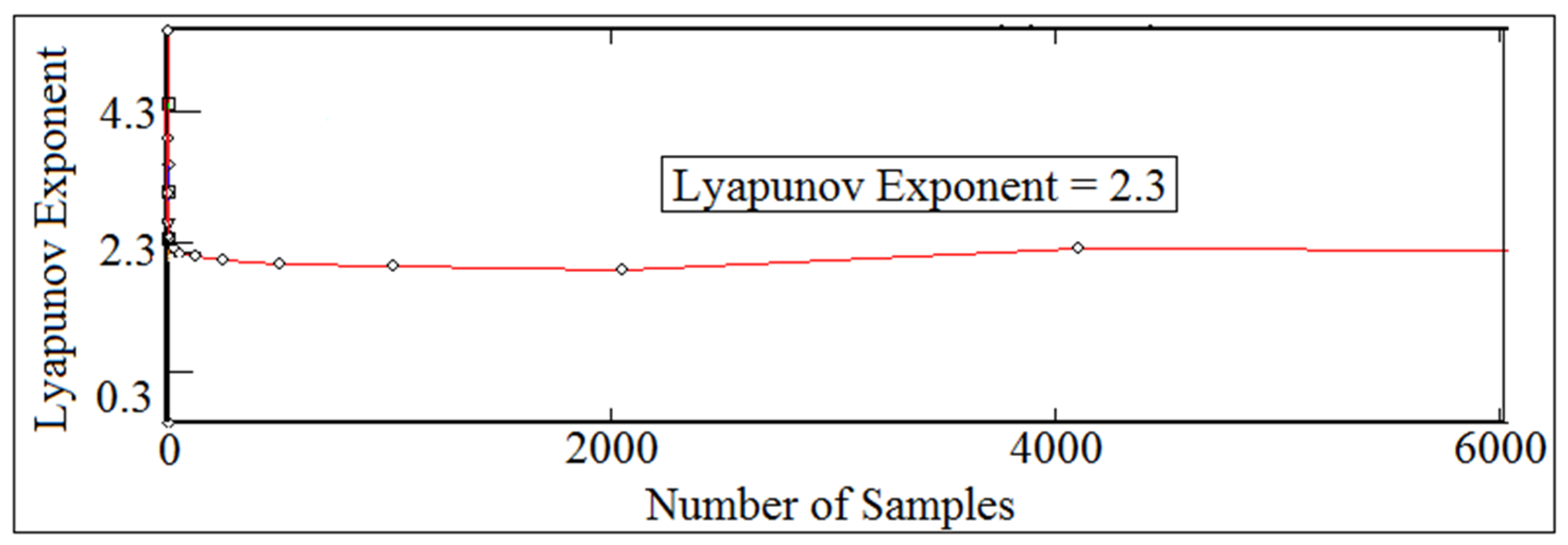
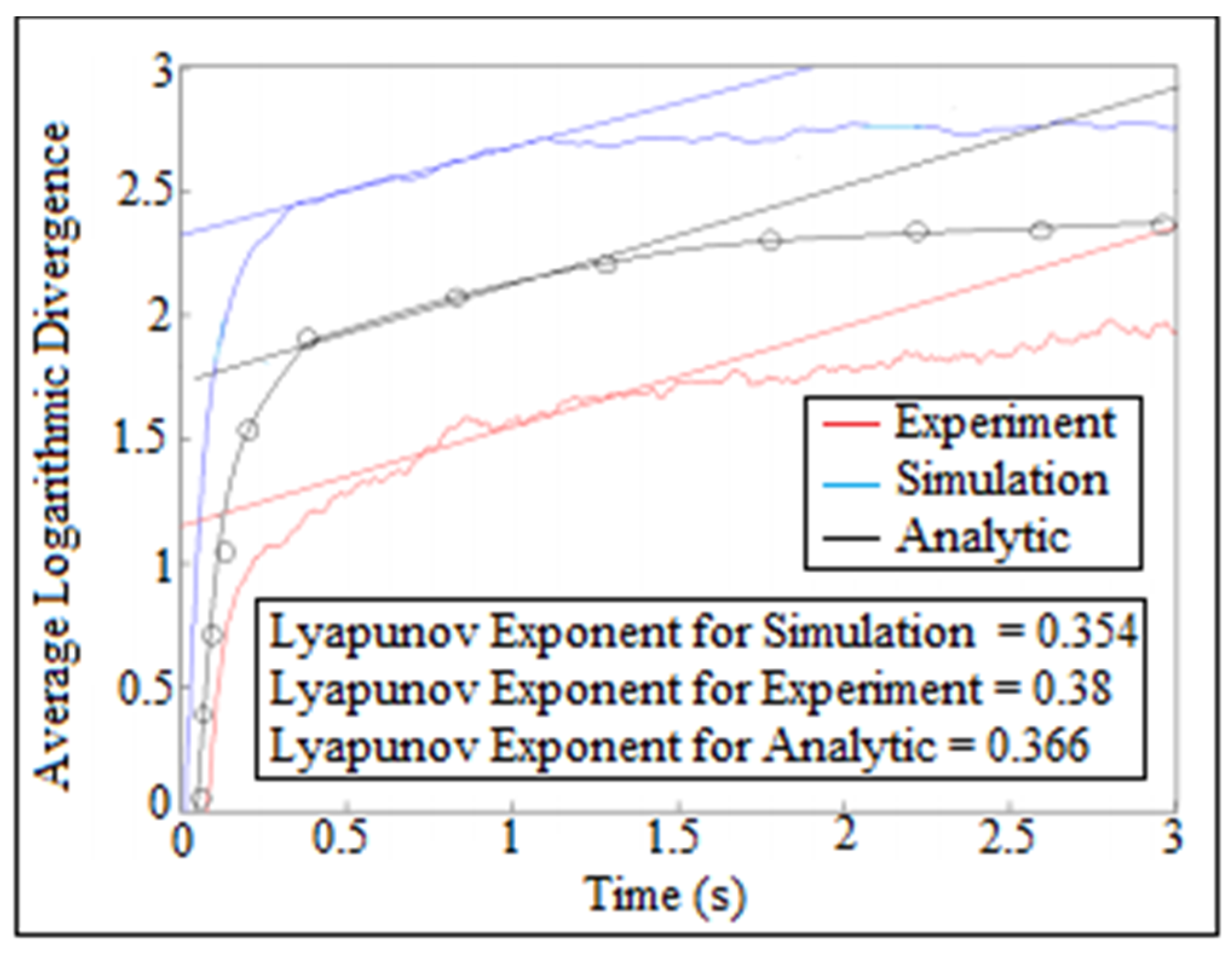
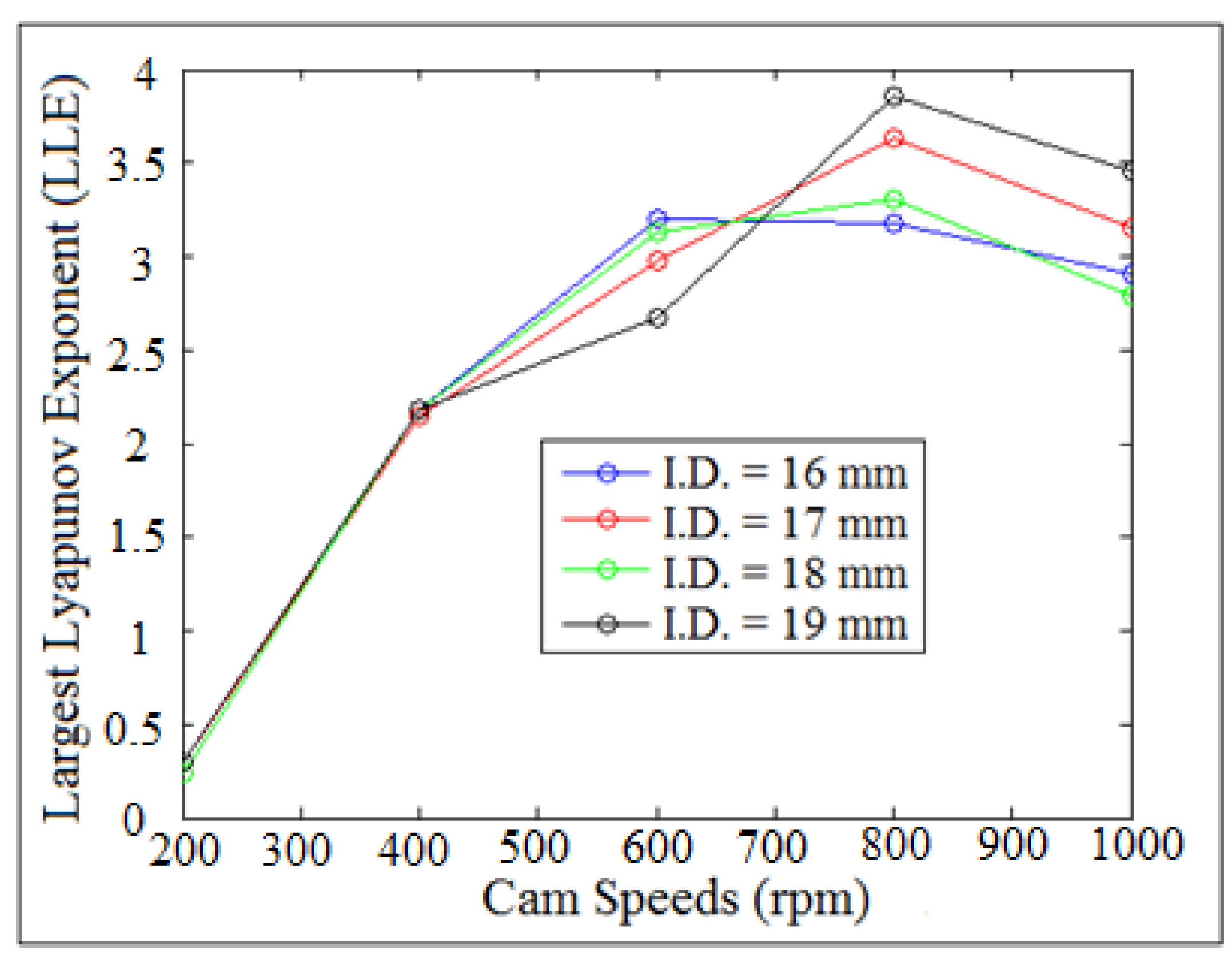
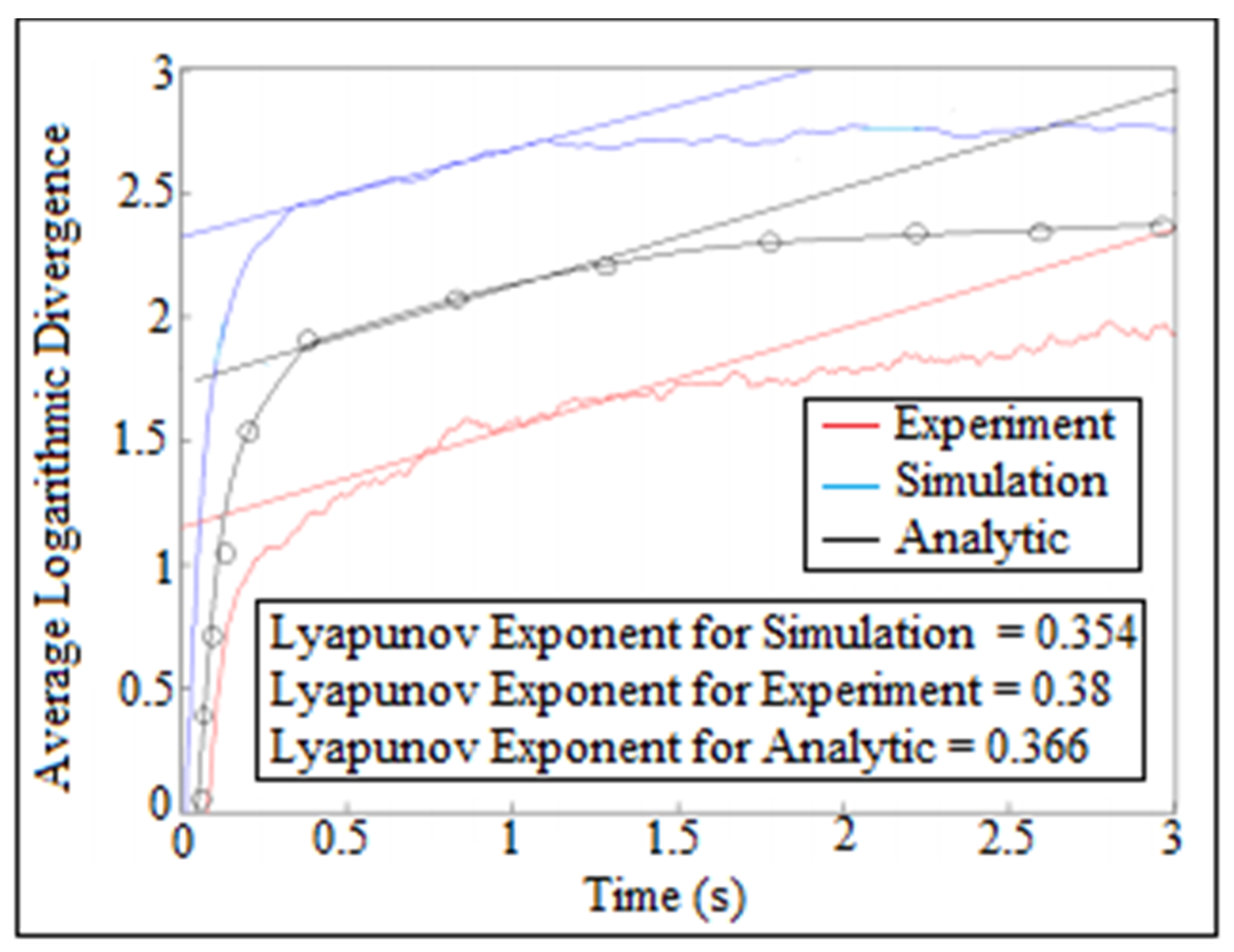
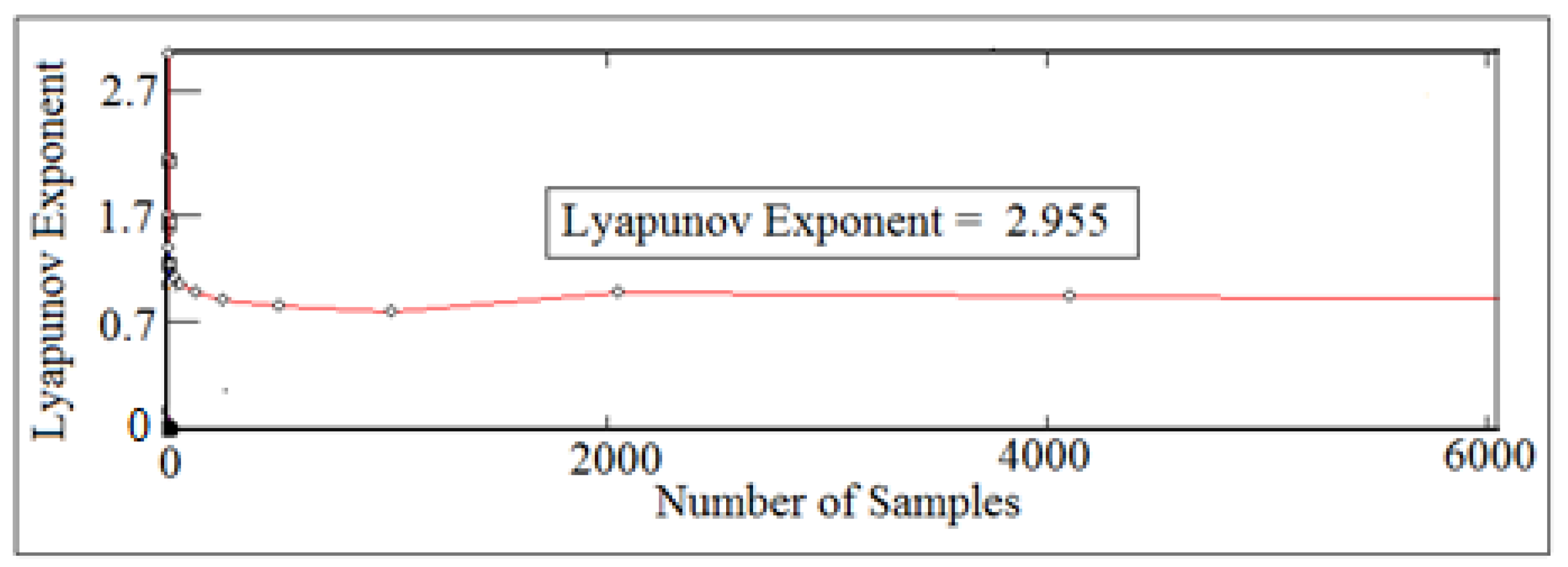
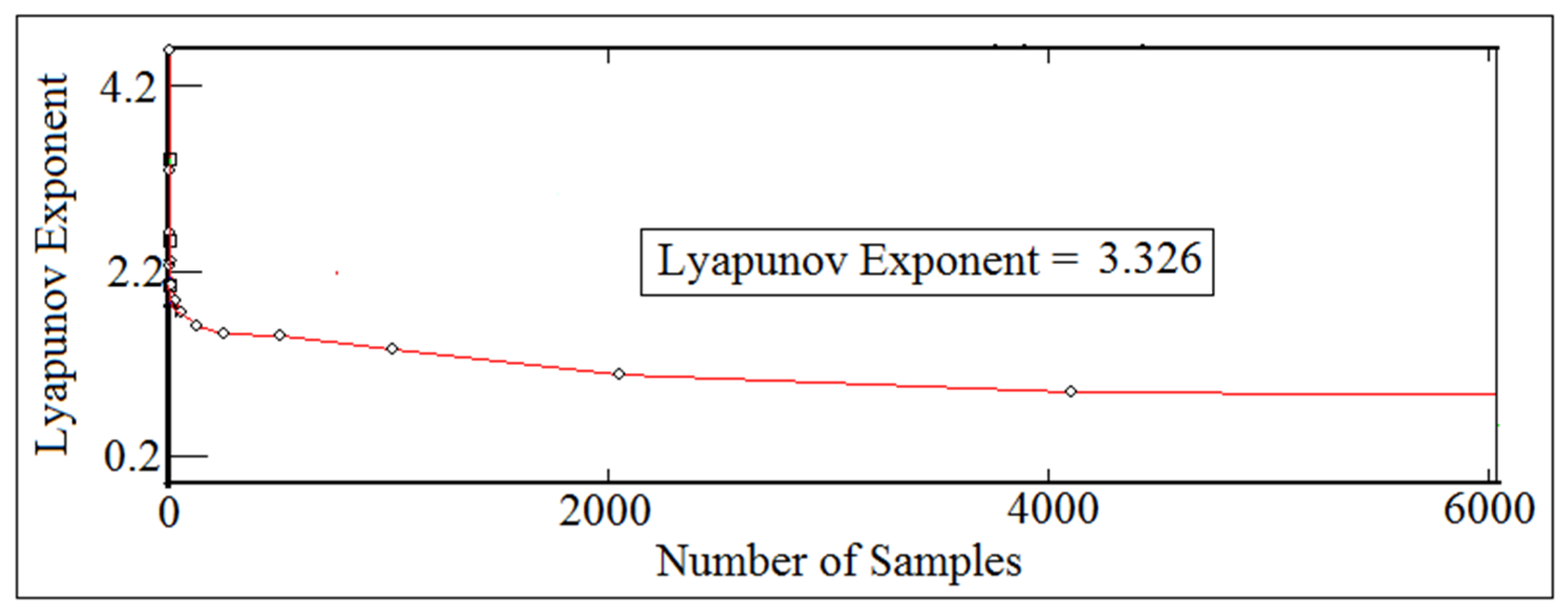
5. Detachment Detection through Largest Lyapunov Exponent Parameter
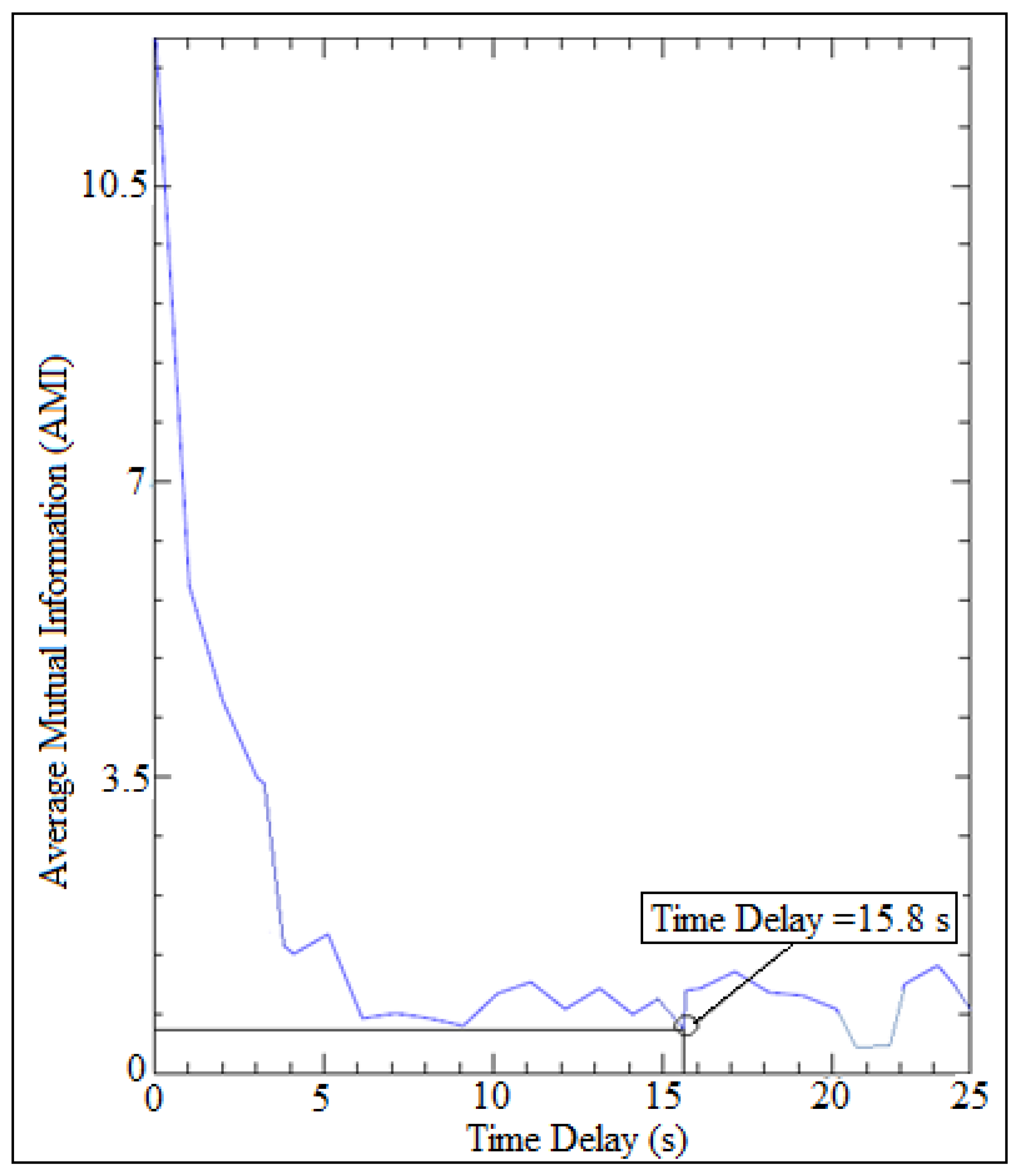
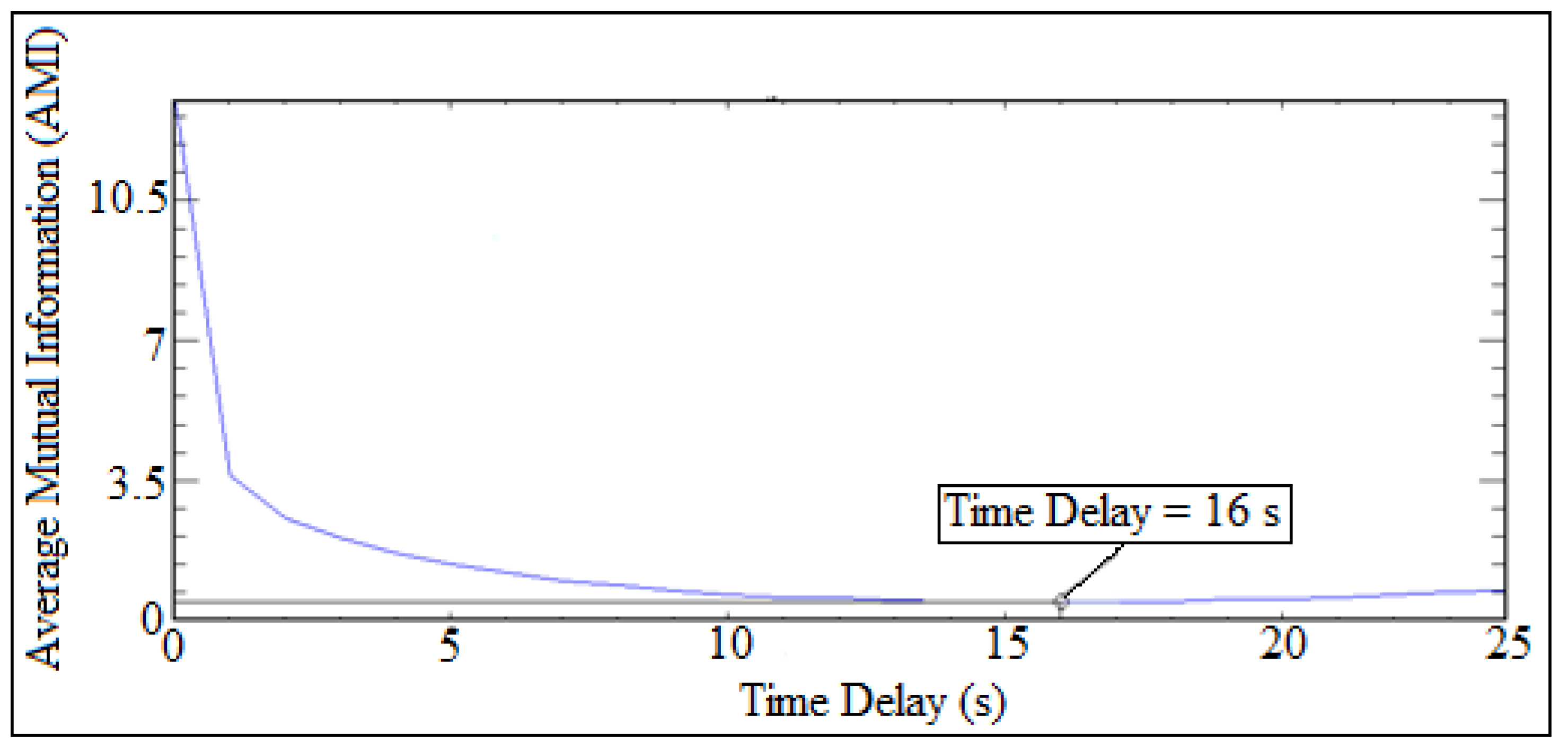
- is the local time delay.
- is the optimal for independence of time series.
- P is the embedding dimensions.
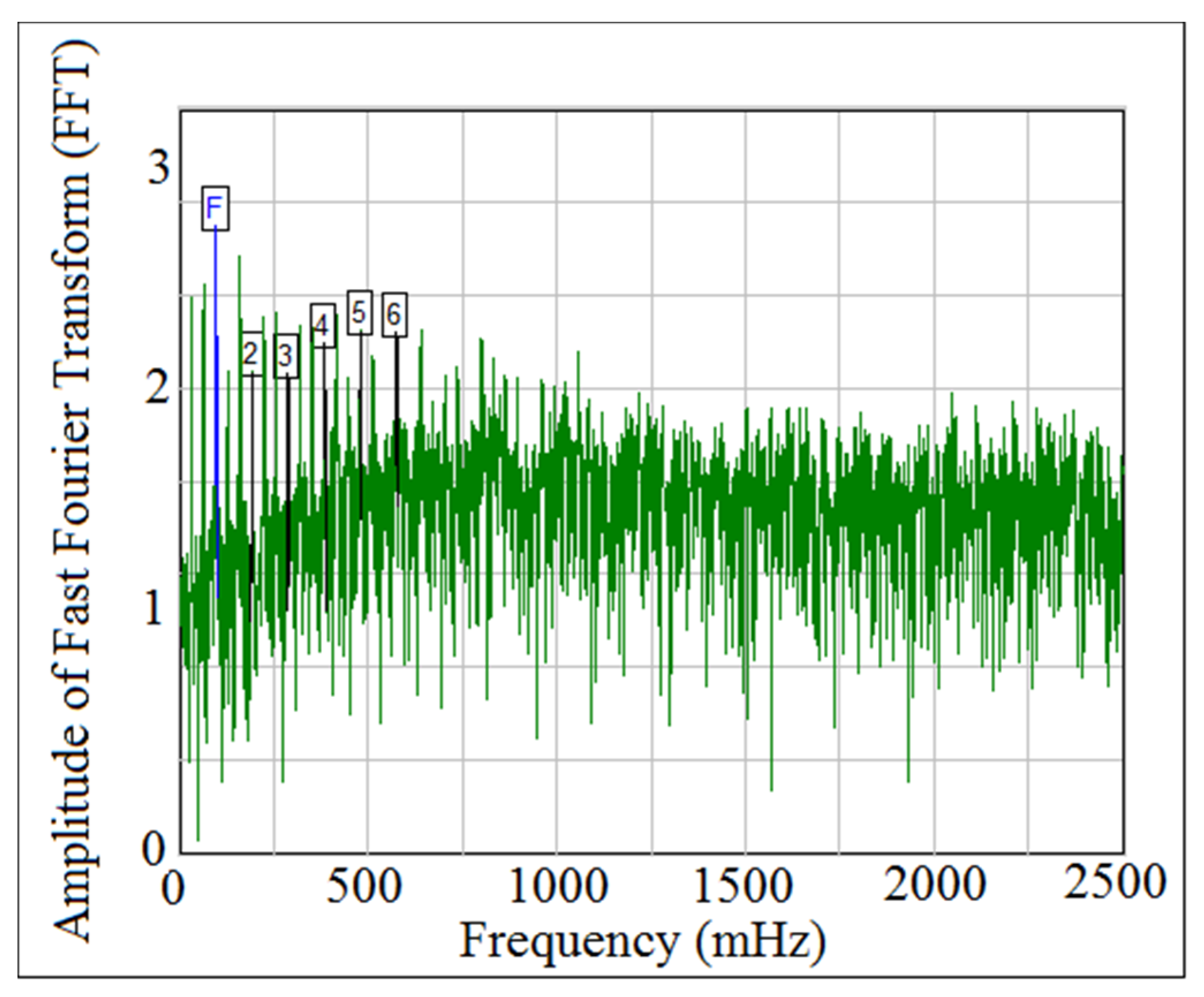
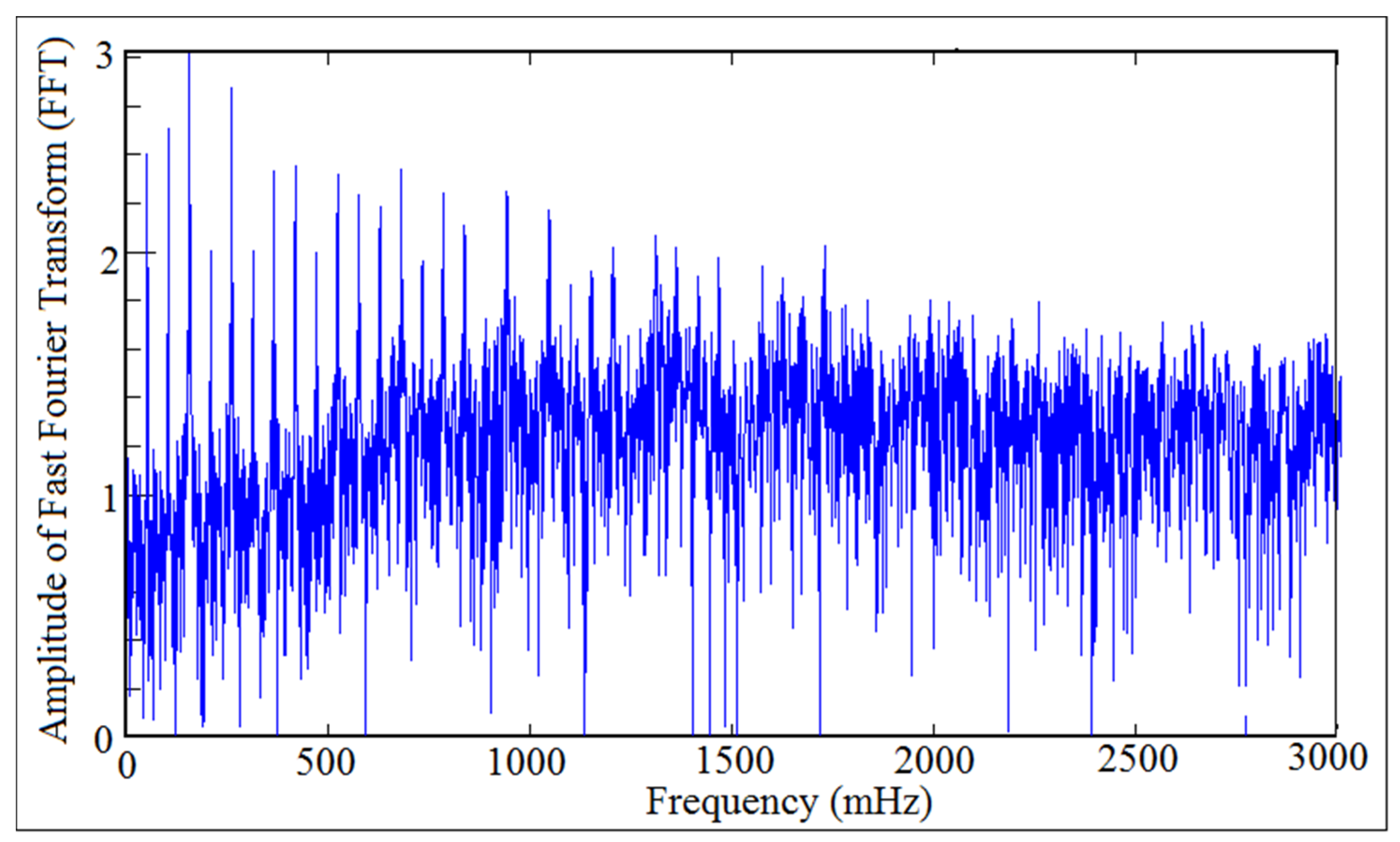
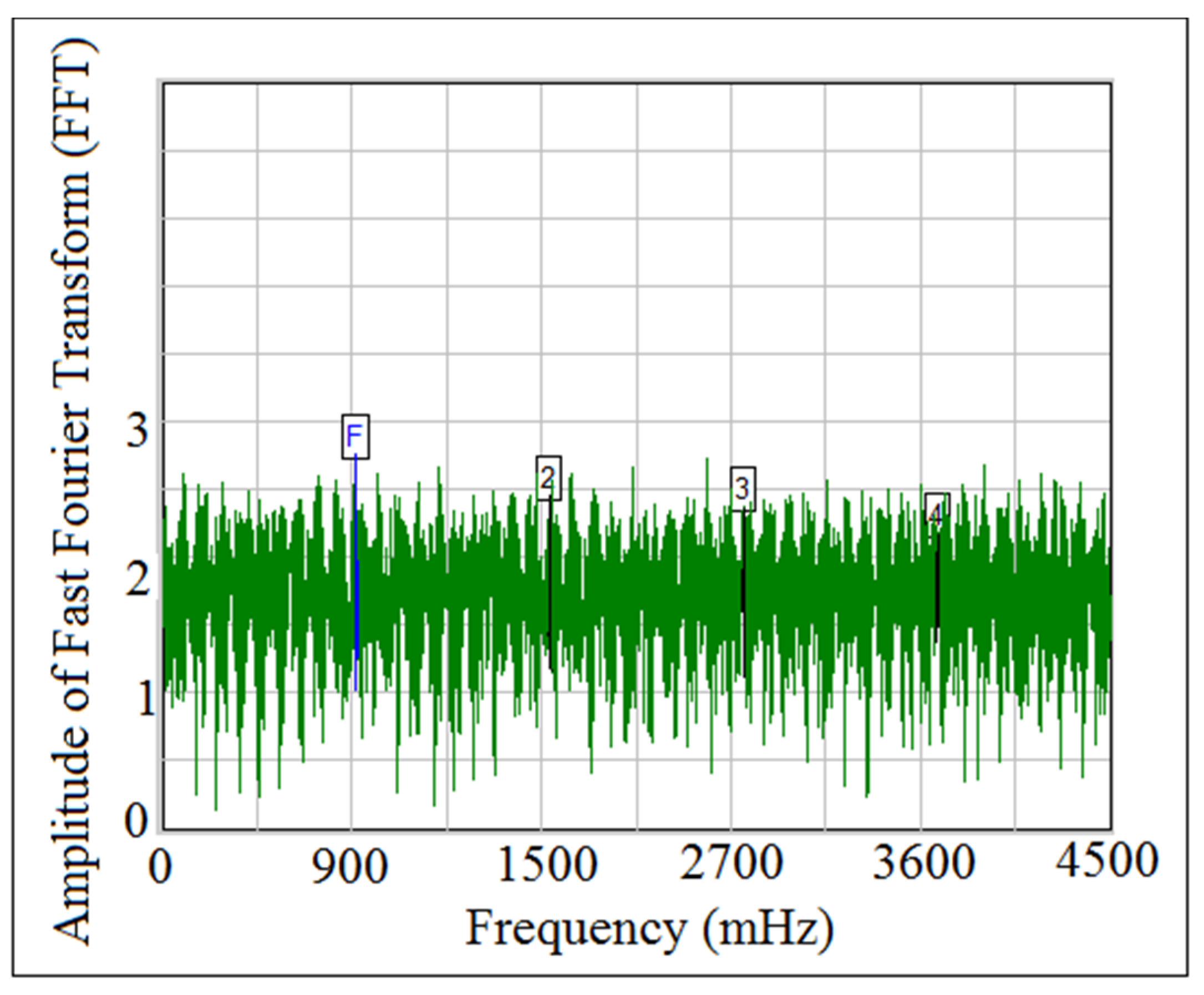
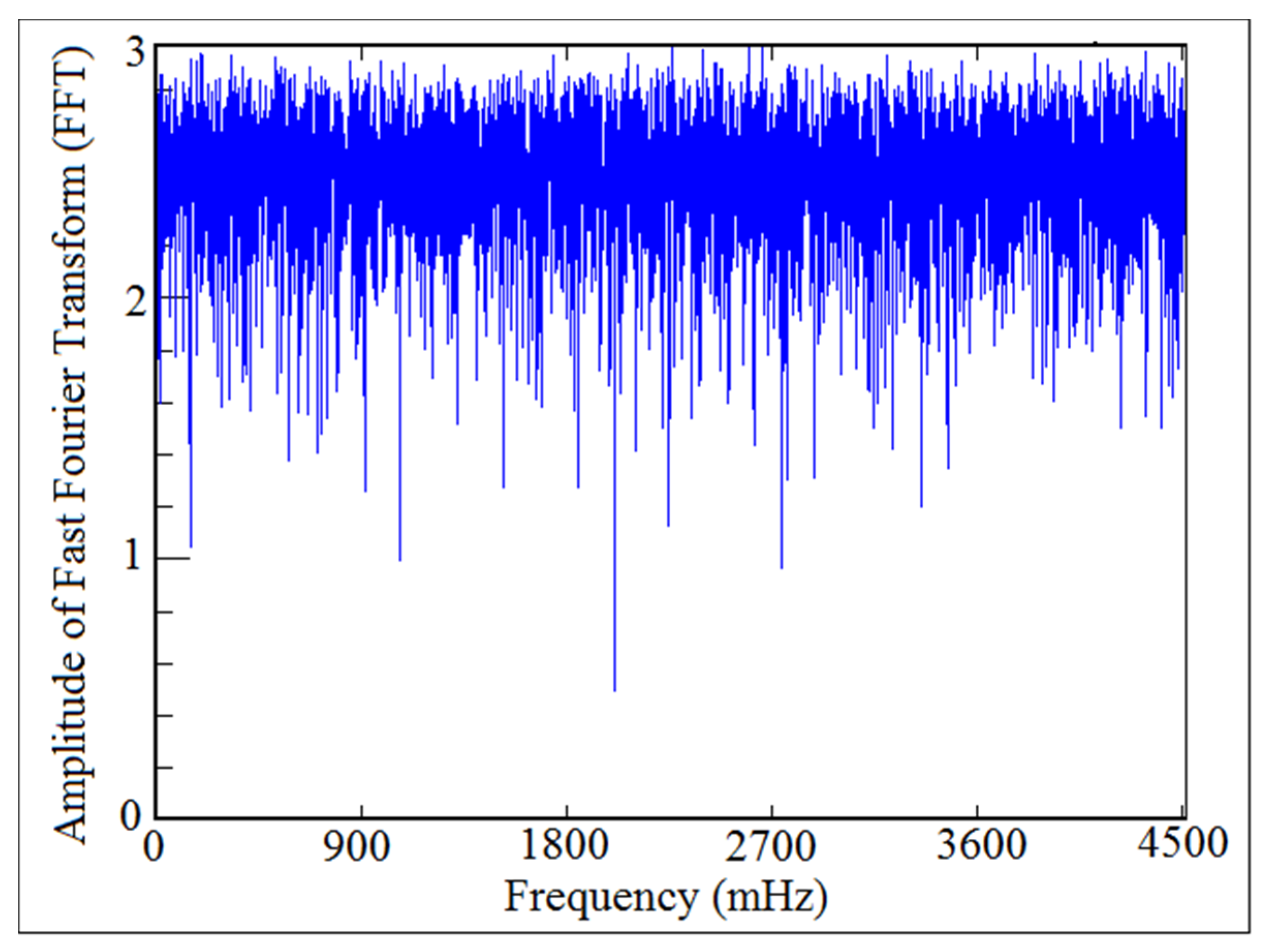
6. Detachment Detection through Fast Fourier Transform (FFT)
7. Detachment Detection through Poincare’ Map
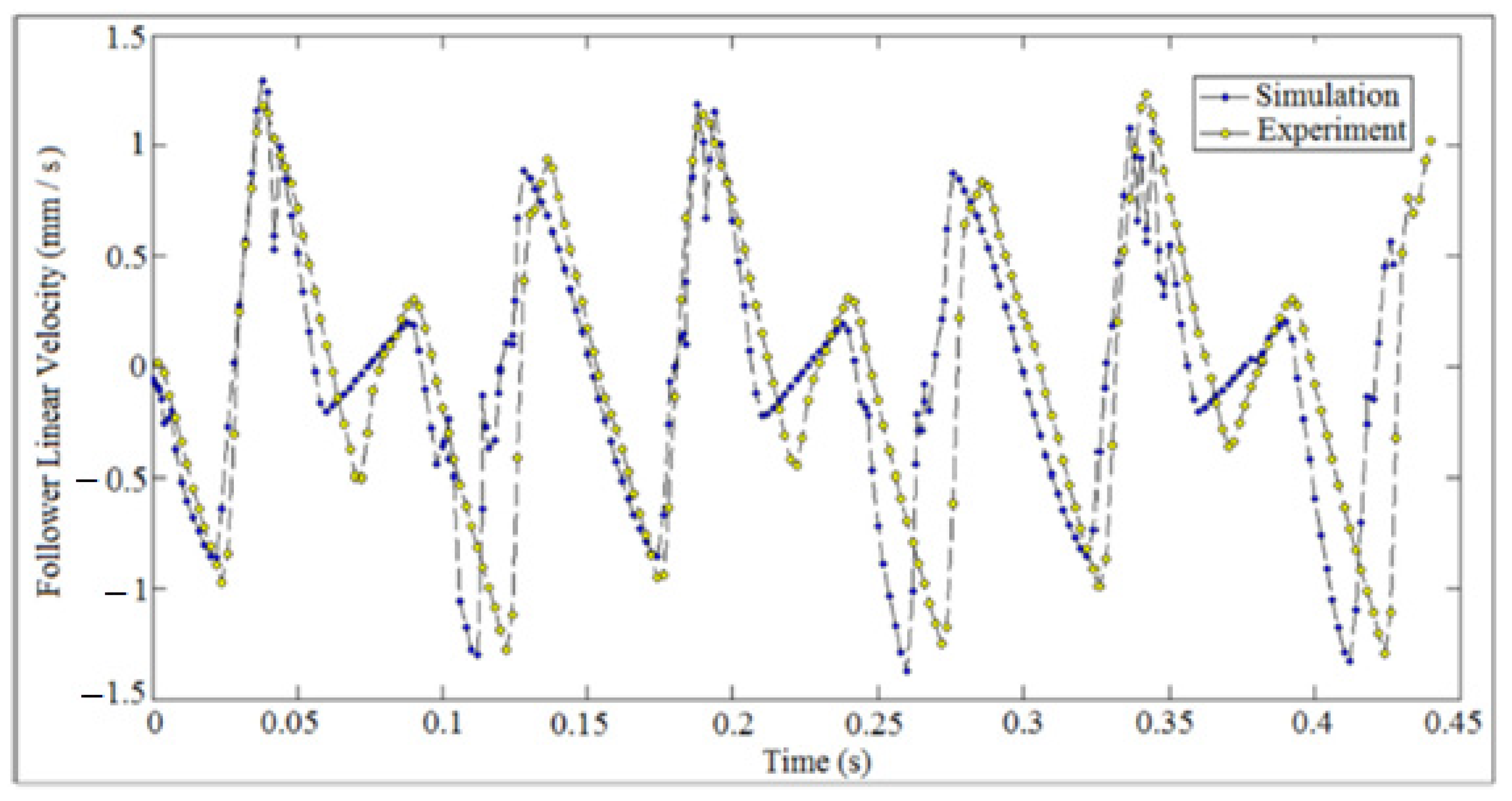
8. Results and Discussions
9. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Article Highlights
- The detachment between the cam and the follower was detected using largest Lyapunov exponent parameter, power density function using (FFT), and Poincare’ maps due to the nonlinear dynamics phenomenon of the follower.
- Multi-degrees of freedom (spring-damper-mass) systems at the very end of the follower were used to improve the dynamic performance and to reduce the detachment height between the cam and the follower.
- Friction and impact were considered between the cam and the follower and between the follower and its guides.
- The experiment test was carried out using high speed camera at the foreground of the OPTOTRAK 30/20 equipment, while the numerical simulation was done using SolidWorks program.
Nomenclatures
| Normal Letters | |
| I.D. | Internal distance of the follower guide from inside, mm. |
| N | Cam speed, rpm. |
| I | Polar moment of inertia, . |
| Dimensions of the follower stem, mm. | |
| Spring stiffness, N/mm. | |
| Damping coefficient, Ns/mm. | |
| Spring stiffness of the three degrees of freedom, N/mm. | |
| Damping coefficient of the three degrees of freedom, Ns/mm. | |
| Radius of the base circle of the cam, mm. | |
| m | Mass of the follower, kg. |
| PC | Contact force between the cam and the follower, N. |
| d(t) | Rate of change in the distance between nearest neighbors. |
| dj(i) | Distance between the jth pair at (i) nearest neighbors, mm. |
| t | Single time series, s. |
| y(i) | Curve fitting of least square method for the follower displacement data. |
| FFT | Fast Fourier Transform of the power density function. |
| LLE | Largest Lyapunov exponent parameter. |
| Greek Letters | |
| θ | Rotational angle of the follower, Degree. |
| Ø | Phase shift angle of the vibration mode shape, Degree. |
| Pressure angle, degree. | |
| λ | Lyapunov exponent. |
| Critical speed of the cam, rpm. | |
| Latin Letters | |
| Eigenvalue problem, rpm. | |
| Discrete time steps, s. | |
| Preload extension, mm. | |
| D | Average displacement between trajectories at (t = 0). |
| Horizontal movement of the follower in the x-direction, mm. | |
| Vertical movement of the follower in the y-direction, mm. | |
| X | Equivalent solution of the follower movement, mm. |
| , | Velocity, and acceleration of the roller follower, mm/s, mm/s2. |
Appendix A
Appendix B
- (a)
- Ten parts such as (polydynecam.SLDPRT, flat-facedfollower.SLDPRT,guideno1.SLDPRT, guideno2.SLDPRT, outsideframe.SLDPRT, massboxno1.SLDPRT, massboxno2.SLDPRT, massboxno3.SLDPRT, massboxno4.SLDPRT, and cylindricalrollers.SLDPRT) has been created using the modeling computer aided design (CAD) of SolidWorks program. It can be assembled the above nine parts using camfollowerassembly.SLDASM to obtain Figure 5.
- (b)
- Select Motion Analysis from Motion Study Tab and select number frames per second equal to 1000 to make the solution of the dynamic motion more accurate.
- (c)
- Select the Option of Units from Document Properties Tab and select MMGS.
- (d)
- Select Gravity from Motion Study Tab and select the y-direction.
- (e)
- Select Contact from Motion Study Tab and check mark the box of Use Contact Groups in which there will be two boxes. Select the cam geometry in the first box and select the follower geometry in the second box to make the contact between the cam and the follower. Also there will be another two boxes to make the contact between the follower and the two guides.
- (f)
- Select Motor button from Motion Study Tab and select Rotary Motor and the cam speed should be constant.
- (g)
- Select Mechanical Mate from Mate button to make the mate between the cam and the follower.
- (h)
- Select the integrator type GSTIFF from Advanced Motion Analysis Options Tab.
- (i)
- Select Force button from Motion Study Tab and enter the spring force between the follower and the installation table as an external constant force.
- (j)
- Select Spring button from Motion Study Tab to apply multi-degrees of freedom (spring-damper-mass system) and enter the spring constant and the damping coefficient and select the top and bottom surfaces of mass box no. 2, 3, and 4. To apply the spring and damper on mass box no. 1 select the bottom surface of the outside frame and top surface of mass box no. 1. Moreover, to apply the spring and damper on mass box no. 4 select the bottom surface of mass box no. 4 and the very end top of follower stem.
- (k)
- Select Calculate from the Motion Study Tab to solve for the motion study.
- (l)
- Select Results button from Motion Study Table Select Displacement and Linear Displacement to obtain the follower displacement against time and select lower surface of guide no.2 and the contact point between the cam and the follower. On the other hand, select from Results button Forces and select Contact Force and select lower surface of guide no.2 and select the contact point between the cam and the follower to obtain the contact force against time.
References
- Sundar, S.; Dreyer, J.T.; Singh, R. Rotational sliding contact dynamics in a non-linear cam-follower system as excited by a periodic motion. J. Vib. Acoust. 2013, 332, 4280–4295. [Google Scholar] [CrossRef]
- Yan, H.S.; Tsay, W.J. A variable-speed approach for preventing cam-follower separation. J. Adv. Mech. Des. Syst. Manuf. 2008, 2, 12–23. [Google Scholar] [CrossRef] [Green Version]
- Jamali, U.H.; Al-Hamood, A.; Abdulla, O.I.; Senatore, I.; Schlattmann, J. Lubrication analyses of cam and flat-faced follower. Lubricants 2019, 7, 31. [Google Scholar] [CrossRef] [Green Version]
- Pugliese, G.; Ciulli, E.; Fazzolari, F. Experimental aspects of a cam-follower contact. In IFToMM World Congress on Mechanism and Machine Science; Springer: Berlin/Heidelberg, Germany, 2019; pp. 3815–3824. [Google Scholar]
- Ciulli, E.; Fazzolari, F.; Pugliese, G. Contact Force Measurements in Cam and Follower Lubricated Contacts. Front. J. Front. Mech. Eng. 2020, 6, 601410. [Google Scholar] [CrossRef]
- Alzate, R.; Di Bernardo, M.; Montanaro, U.; Santini, S. Experimental and numerical verification of bifurcations and chaos in cam-follower impacting systems. J. Nonlinear Dyn. 2007, 50, 409–429. [Google Scholar] [CrossRef] [Green Version]
- Desai, H.D.; Patel, V.K. Computer aided kinematic and dynamicanalysis of cam and follower. In Proceedings of the World Congress on Engineering WCE 2010, London, UK, 30 June–2 July 2010; Volume II. [Google Scholar]
- Yang, Y.-F.; Lu, Y.; Jiang, T.-D.; Lu, N. Modeling and nonlinear response of the cam-follower oblique-impact system. Disc. Dyn. Nat. Soc. 2016, 2016, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Lassaad, W.; Mohamed, T.; Yassine, D.; Fakher, C.; Taher, F.; Mohamed, H. Nonlinear dynamic behavior of a cam mechanism with oscillating roller follower in presence of profile error. Front. J. Front. Mech. Eng. 2013, 8, 127–136. [Google Scholar] [CrossRef]
- DasGupta, A.; Ghosh, A. On the determination of basic dimensions of a cam with a translating roller-follower. J. Mech. Des. 2004, 126, 143–147. [Google Scholar] [CrossRef]
- Yousuf, L.S.; Marghitu, B.D. Nonlinear dynamics behavior of cam-follower system using concave curvatures profile. Adv. Mech. Eng. 2020, 12, 1687814020945920. [Google Scholar] [CrossRef]
- Belliveau, K.D. An Investigation of Incipient Jump in Industrial Cam Follower Systems. Master’s Thesis, Worcester Polytechnic Institute, Worcester, MA, USA, 2002. [Google Scholar]
- Hejma, P.; Svoboda, M.; Kampo, J.; Soukup, J. Analytic analysis of a cam mechanism. Procedia Eng. 2017, 177, 3–10. [Google Scholar] [CrossRef]
- Yousuf, L.S. Non-periodic motion reduction in globoidal cam with roller follower mechanism. Proc. Inst. Mech. Eng. C J. Mech. Eng. Sci. 2021, 09544062211033642. [Google Scholar] [CrossRef]
- Sinha, A.; Bharti, K.S.; Samantaray, A.K.; Bhattacharyya, R. Sommerfeld effect in a single-DOF system with base excitation from motor driven mechanism. Mech. Mach. Theory 2020, 148, 103808. [Google Scholar] [CrossRef]
- Yousuf, L.S. Investigation of chaos in a polydyne cam with flat-faced follower mechanism. J. King Saud Univ. Eng. Sci. 2021, 33, 507–516. [Google Scholar] [CrossRef]
- Norton, R.L. Cam Design and Manufacturing Handbook; Industrial Press: New York, NY, USA, 2002. [Google Scholar]
- Sinha, A. Vibration of Mechanical Systems, 1st ed.; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Shakoor, M.M. Fatigue Life Investigation for Cams with Translating Roller-Follower and Translating Flat-Face Follower Systems. Ph.D. Thesis, Iowa State University, Ames, IA, USA, 2006. [Google Scholar]
- Hamza, F.; Abderazek, H.; Lakhdar, S.; Ferhat, D.; Yıldız, A.R. Optimum design of cam-roller follower mechanism using a new evolutionary algorithm. Int. J. Adv. Manuf. Syst. 2018, 99, 1267–1282. [Google Scholar] [CrossRef]
- Planchard, D. SolidWorks 2016 Reference Guide: A Comprehensive Reference Guide with over 250 Standalone Tutorials; Sdc: Kansas, KS, USA, 2015. [Google Scholar]
- Yousuf, L.S.; Marghitu, D.B. Experimental and Simulation of a Cam and Translated Roller Follower Over a Range of Speeds. In ASME International Mechanical Engineering Congress and Exposition; ASME: New York, NY, USA, 2016; p. V04AT05A057. [Google Scholar]
- Rothbart, H.A. Cam Design Handbook; McGraw-Hill Education: New York, NY, USA, 2004. [Google Scholar]
- Nguyen, T.T.N.; Hüsing, M.; Corves, B. Motion Design of Cam Mechanisms by Using Non-Uniform Rational B-Spline. Ph.D. Thesis, Rheinisch-Westfälischen Aachen University of Technology, Aachen, Germany, 2018. [Google Scholar]
- Wu, L.I.; Liu, C.H.; Chen, T.W. Disc cam mechanisms with concave-face follower. Proc. Inst. Mech. Eng. C J. Mech. Eng. Sci. 2009, 223, 1443–1448. [Google Scholar] [CrossRef]
- Parlitz, U. Estimating Lyapunov Exponents from Time Series. Chaos Detection and Predictability; Springer: Berlin/Heidelberg, Germany, 2016; pp. 1–34. [Google Scholar]
- Terrier, P.; Fabienne, R. Maximum Lyapunov exponent revisited: Long-term attractor divergence of gait dynamics is highly sensitive to the noise structure of stride intervals. Gait Posture 2018, 66, 236–241. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hussain, V.S.; Spano, M.L.; Lockhart, T.E. Effect of data length on time delay and embedding dimension for calculating the Lyapunov exponent in walking. J. R. Soc. Interface 2020, 17, 20200311. [Google Scholar] [CrossRef] [PubMed]
- Garcia, M.M.; Morales, I.; Rodriguez, J.M.; Marin, M.R. Selection of embedding dimension and delay time in phase space reconstruction via symbolic dynamics. J. Entropy 2021, 23, 221. [Google Scholar] [CrossRef] [PubMed]
- Stoica, P.; Moses, R.L. Spectral Analysis of Signals; Pearson Prentice Hall Upper Saddle River: Upper Saddle River, NJ, USA, 2005. [Google Scholar]
- Abu-Mahfouz, I. Experimental investigation of non-linear behavior of a cam-follower mechanism. In SEM Annual Conference and Exposition on Experimental and Applied Mechanics; Elsevier: Springfield, MA, USA, 2005. [Google Scholar]









Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yousuf, L.S. Detachment Detection in Cam Follower System Due to Nonlinear Dynamics Phenomenon. Machines 2021, 9, 349. https://doi.org/10.3390/machines9120349
Yousuf LS. Detachment Detection in Cam Follower System Due to Nonlinear Dynamics Phenomenon. Machines. 2021; 9(12):349. https://doi.org/10.3390/machines9120349
Chicago/Turabian StyleYousuf, Louay S. 2021. "Detachment Detection in Cam Follower System Due to Nonlinear Dynamics Phenomenon" Machines 9, no. 12: 349. https://doi.org/10.3390/machines9120349
APA StyleYousuf, L. S. (2021). Detachment Detection in Cam Follower System Due to Nonlinear Dynamics Phenomenon. Machines, 9(12), 349. https://doi.org/10.3390/machines9120349




