A Variable-Length Rational Finite Element Based on the Absolute Nodal Coordinate Formulation
Abstract
:1. Introduction
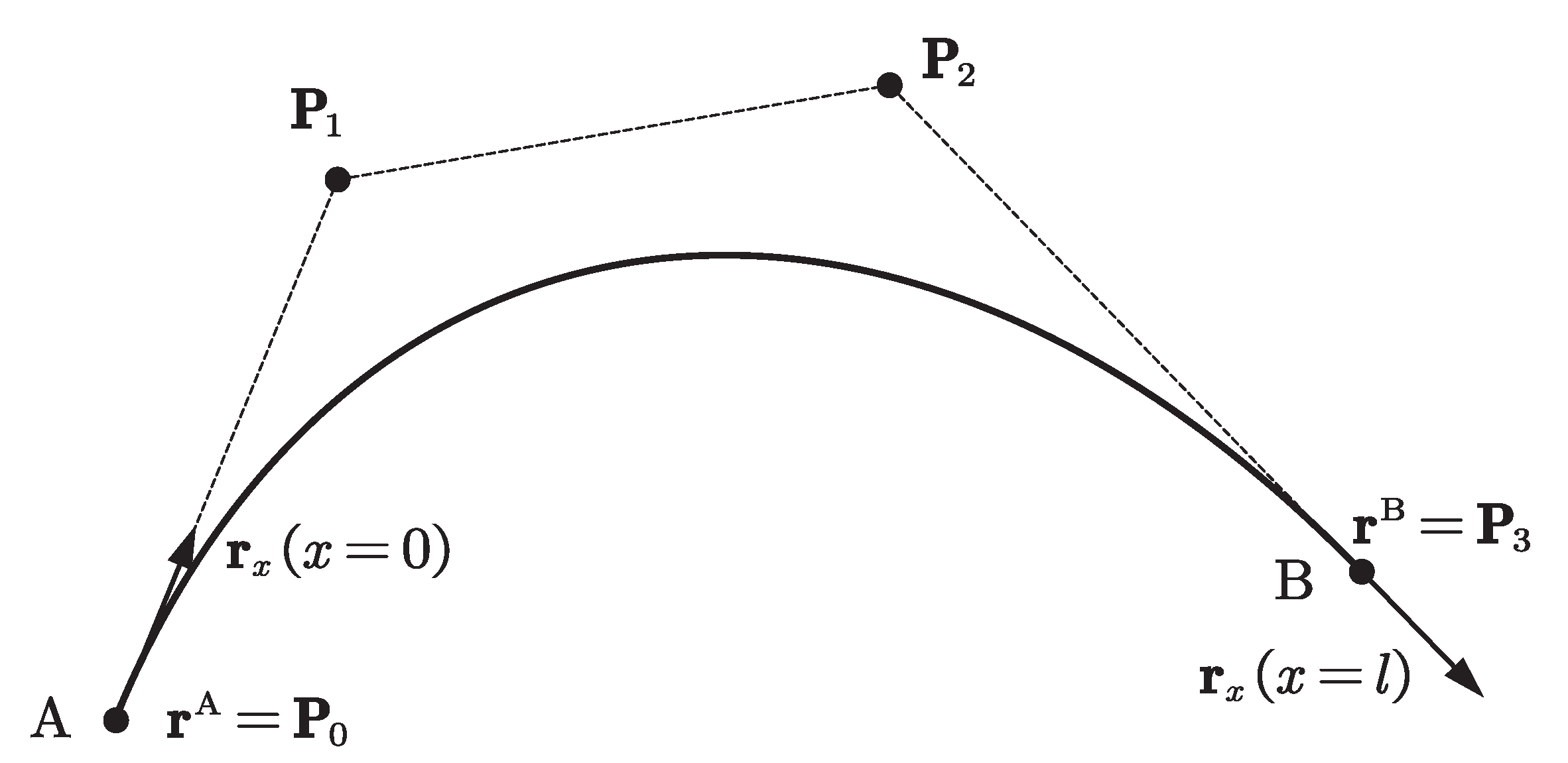
2. RANCF Finite Element
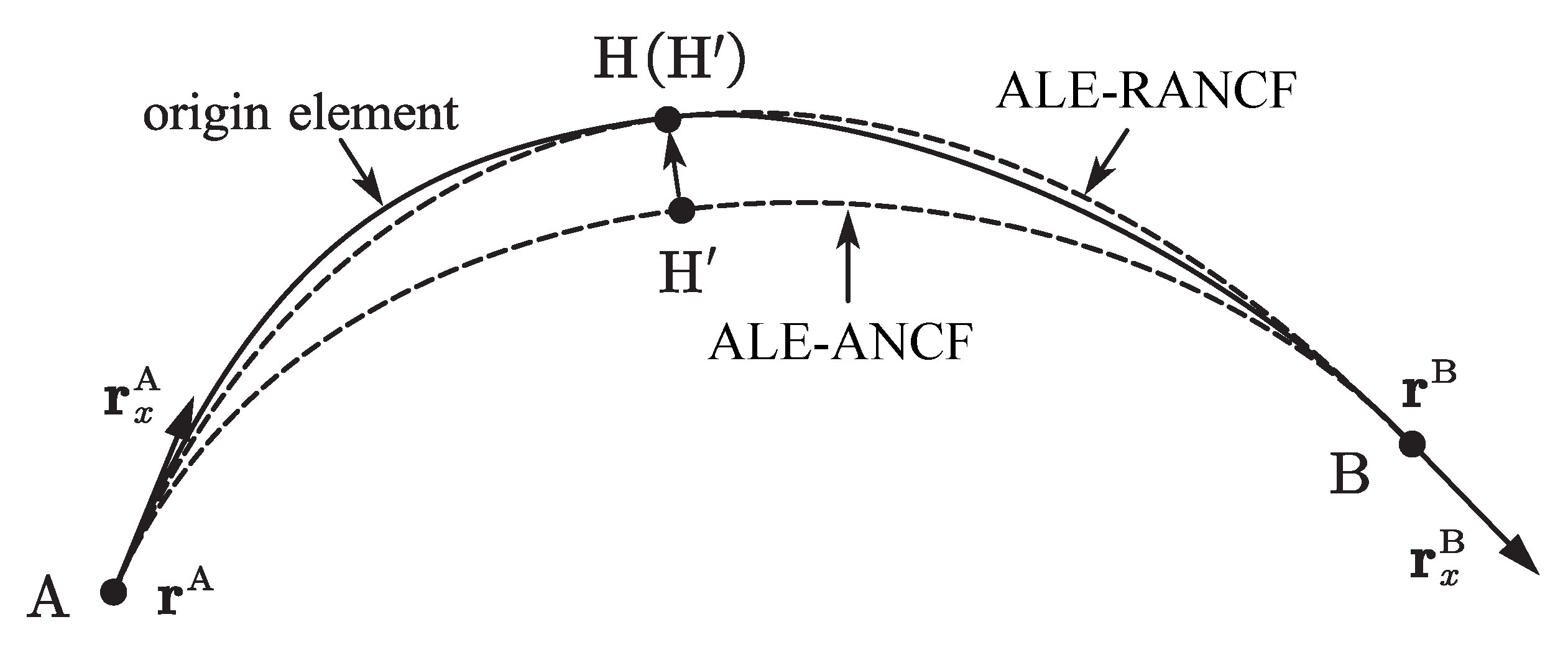
3. ALE-RANCF Finite Element
4. Element Length Control
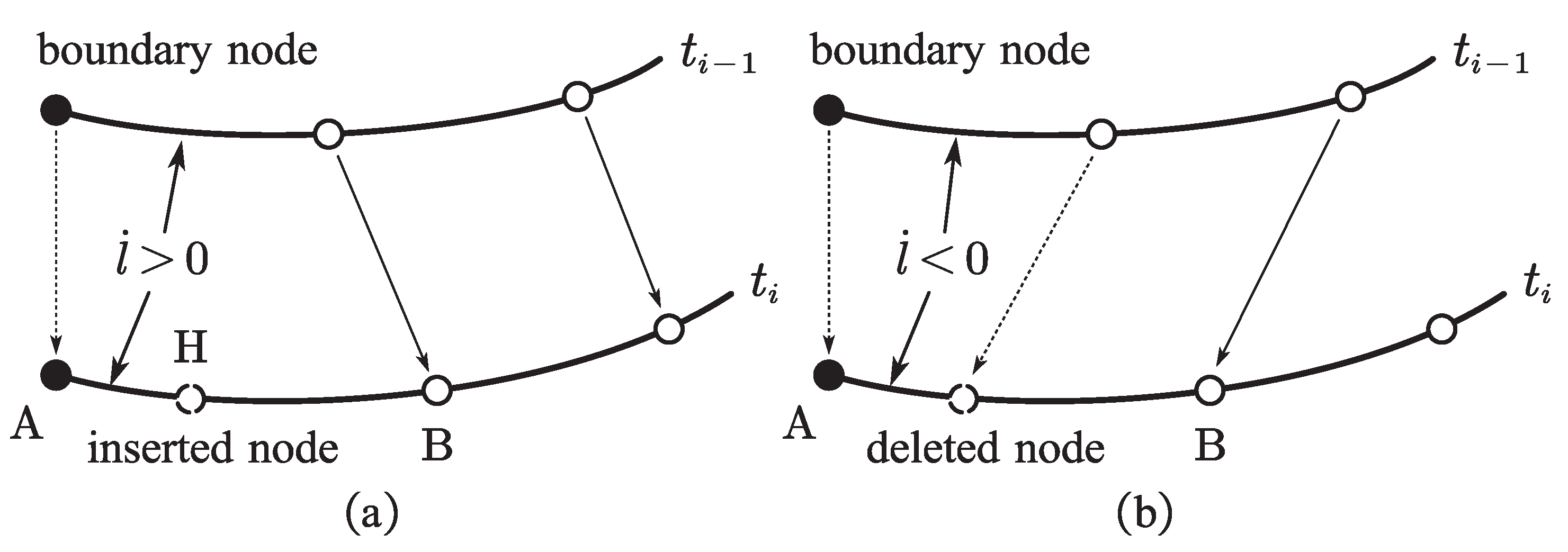
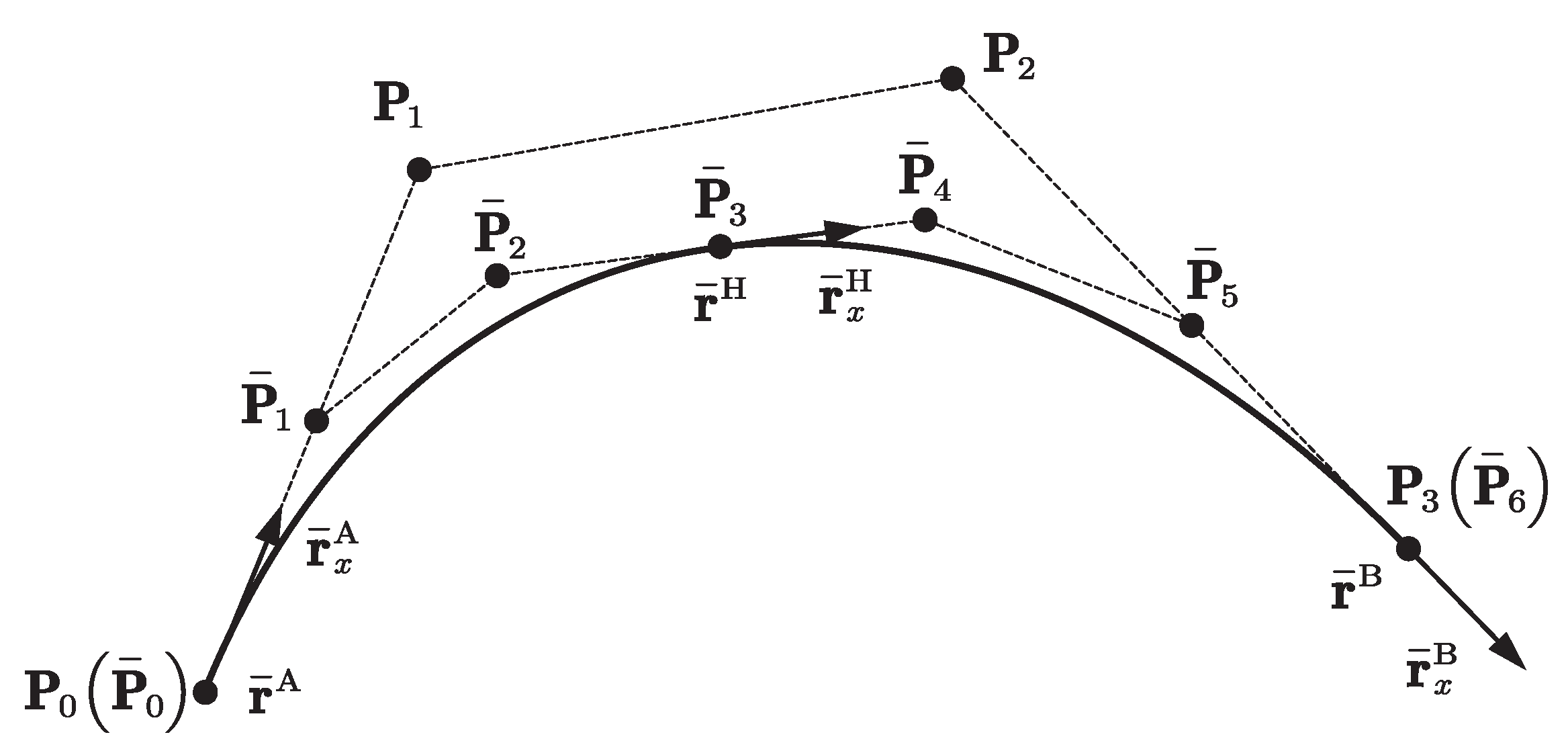
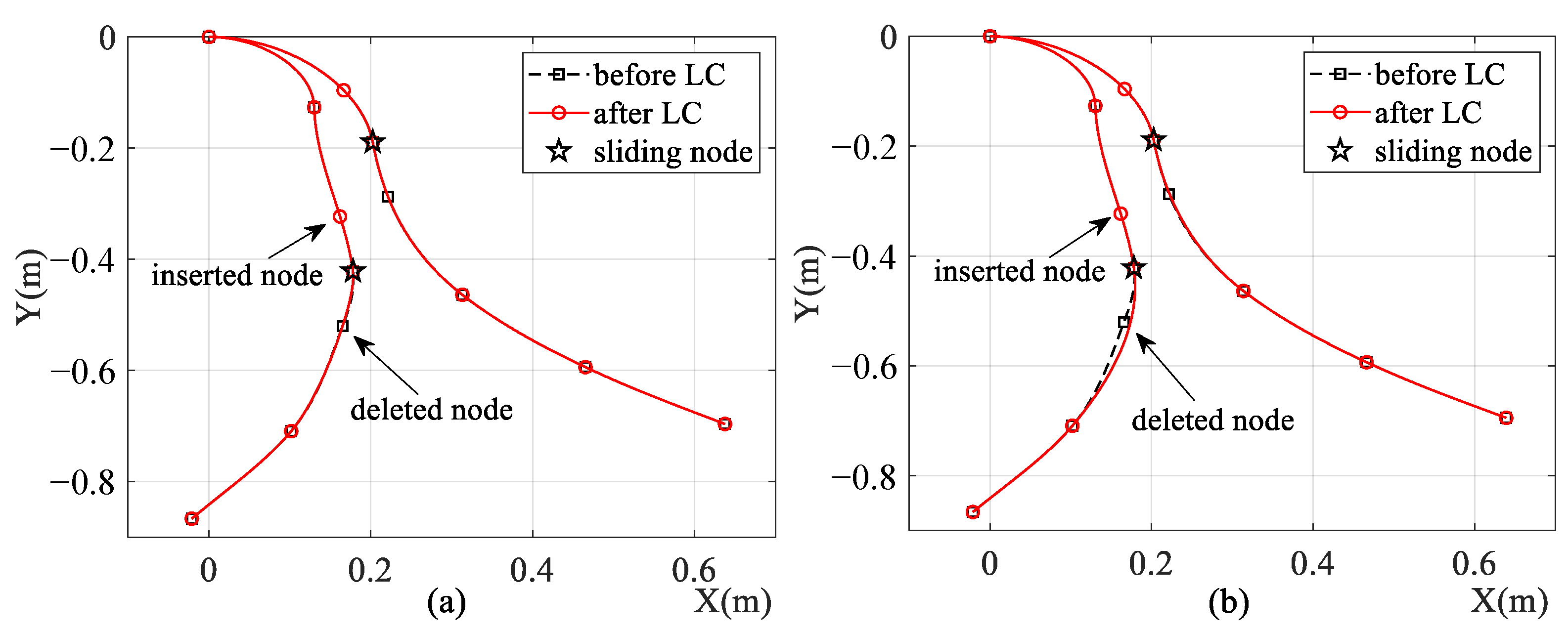
4.1. Element Segmentation
4.2. Element Merging
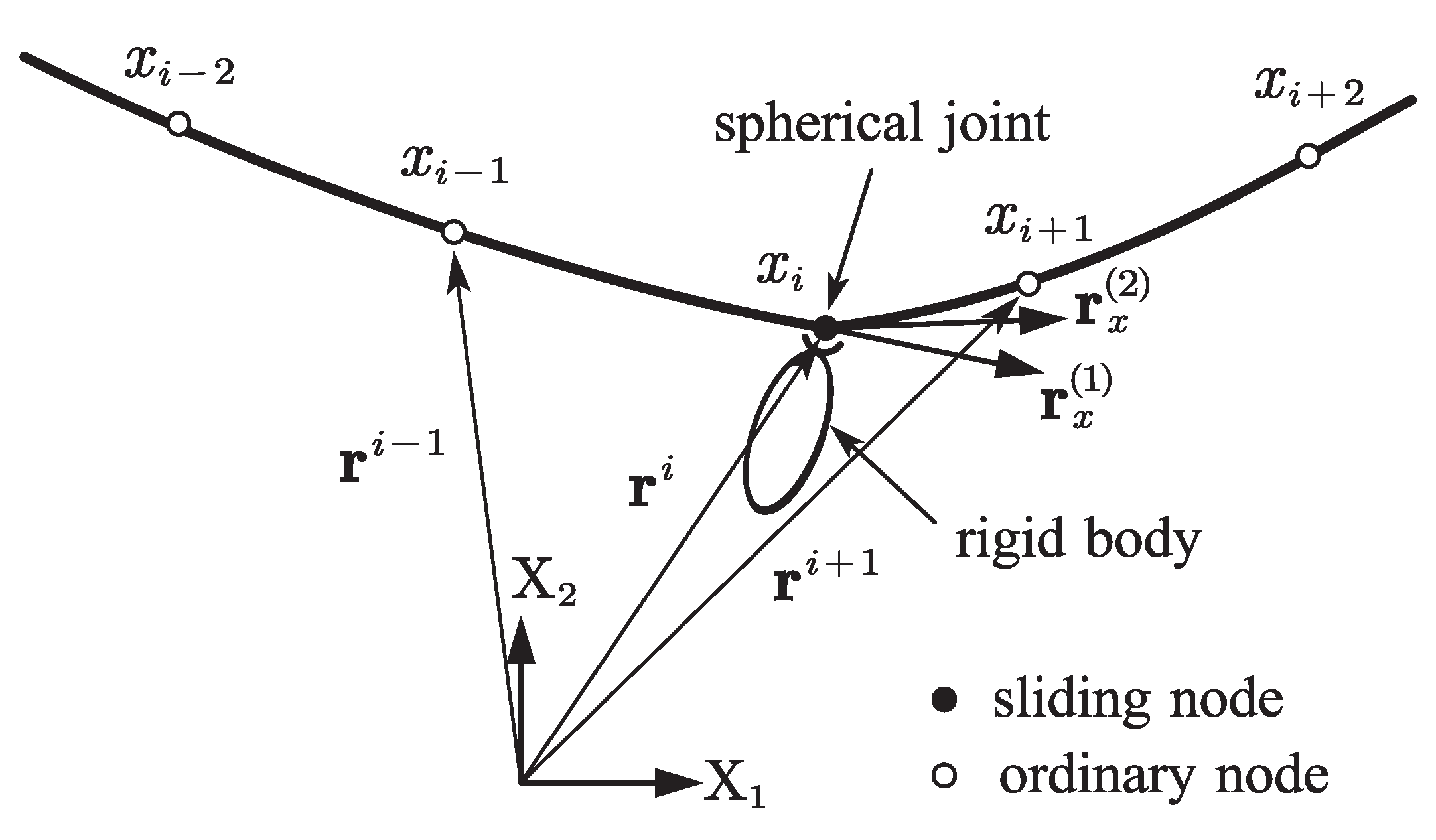
5. Sliding Joint Model
6. Numerical Examples
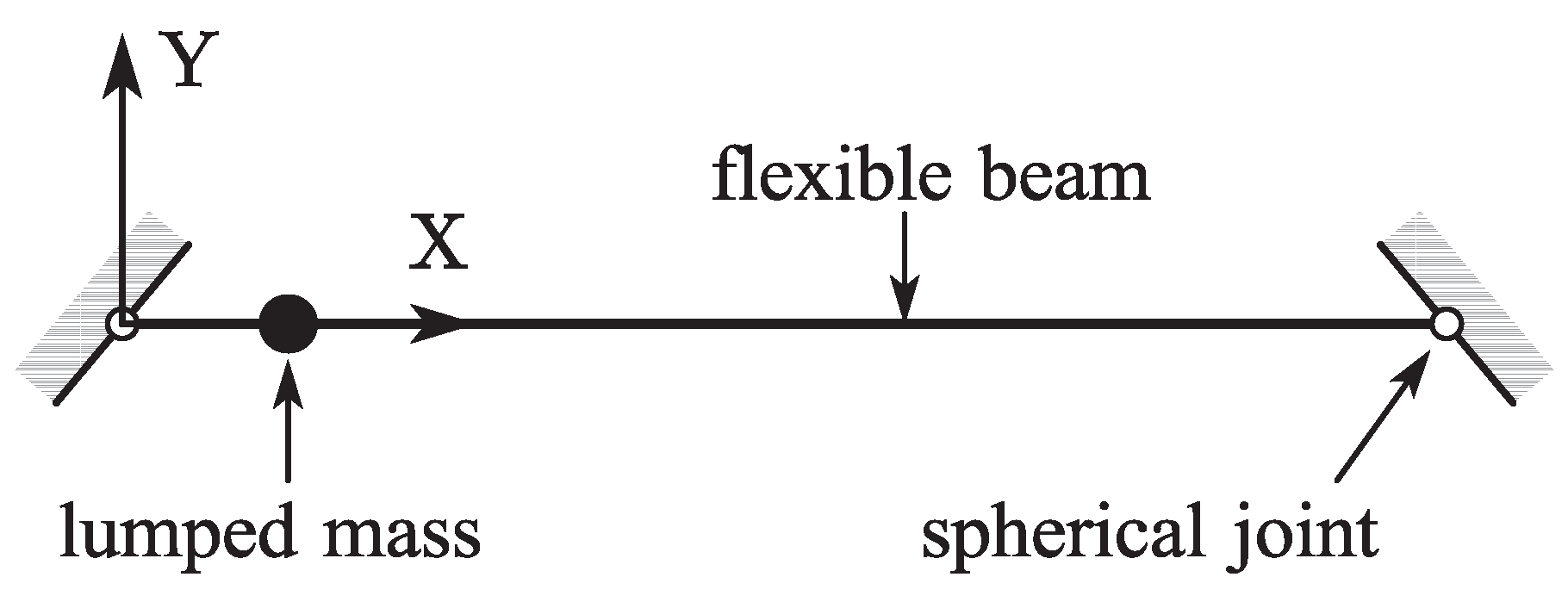
6.1. A Falling Beam with a Sliding Lumped Mass
6.2. A Suspended Beam with a Sliding Lumped Mass
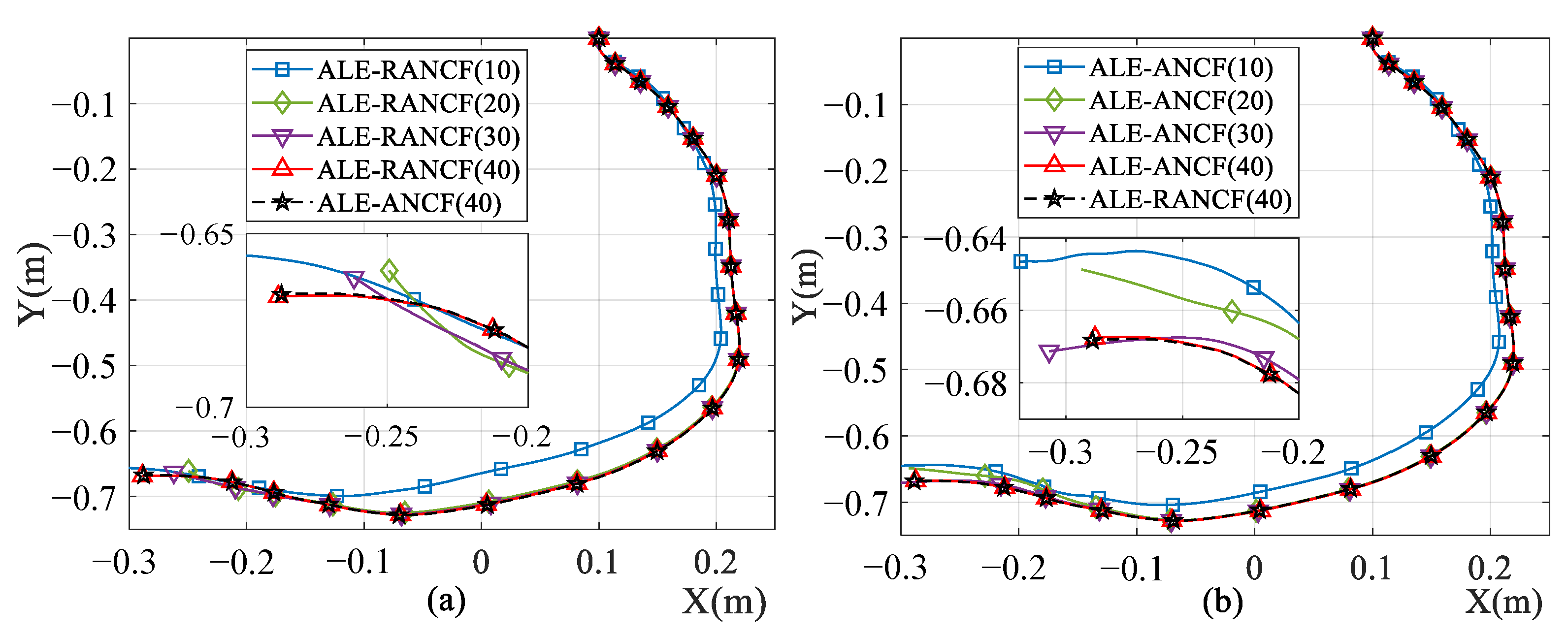
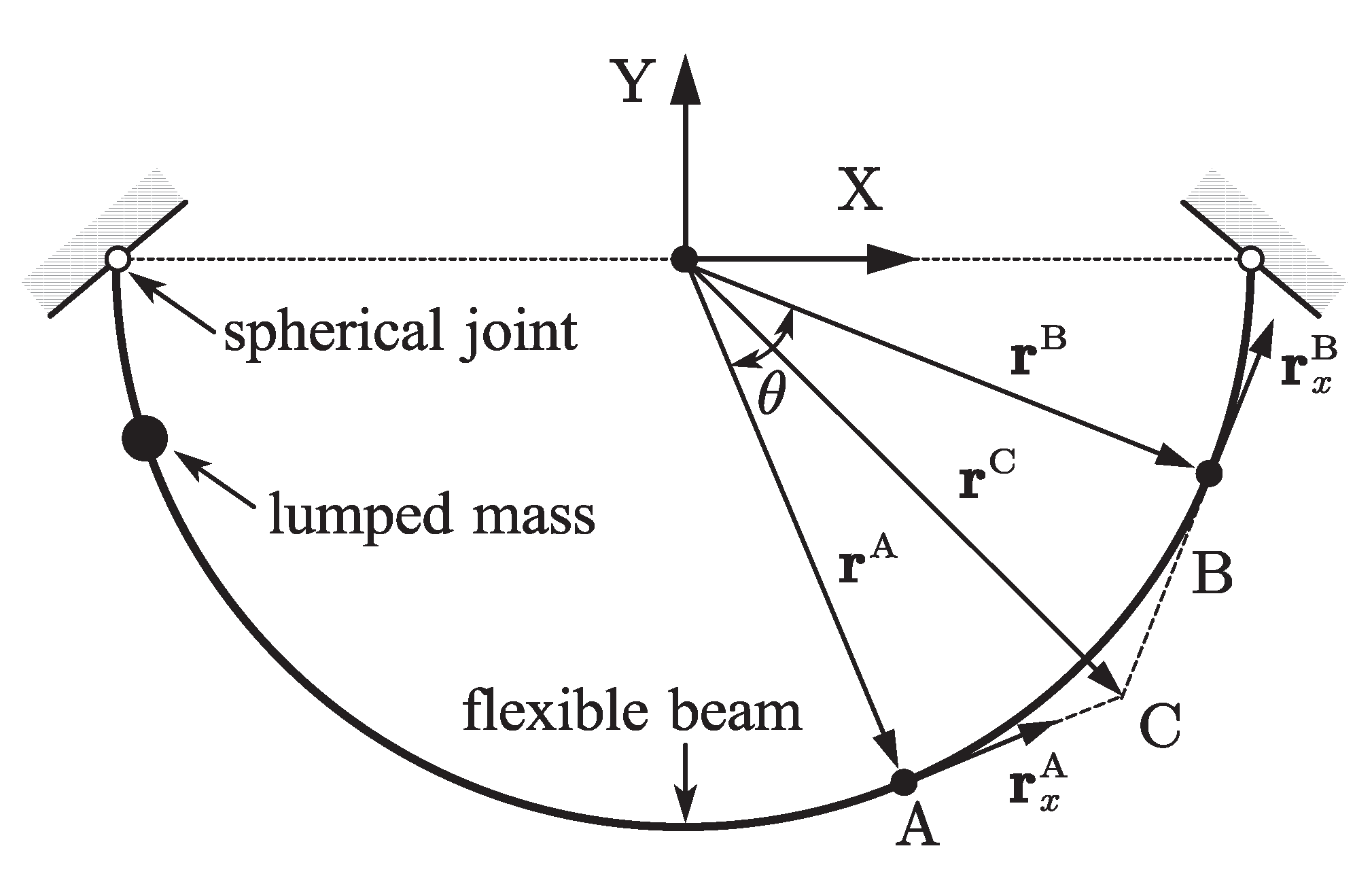
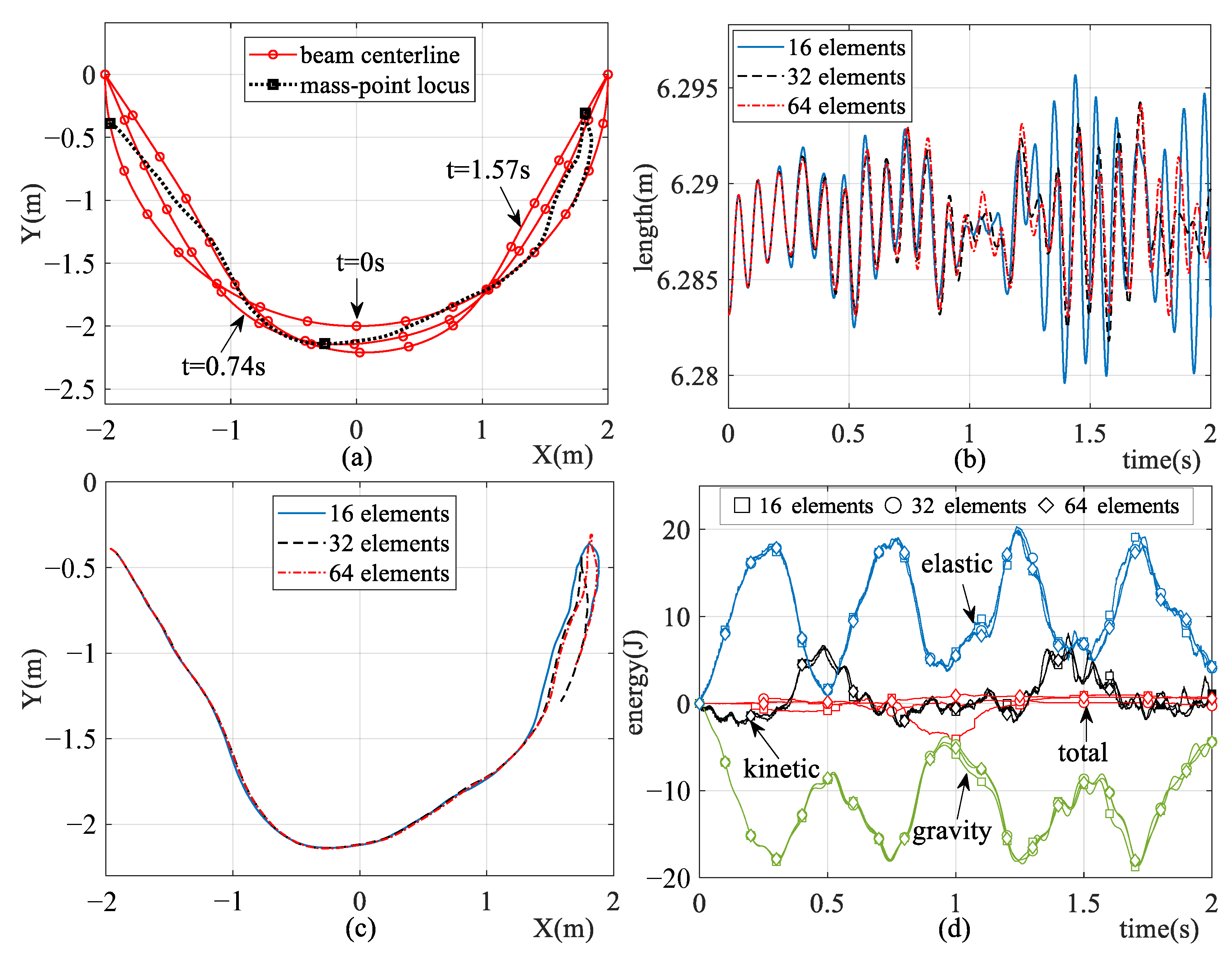
6.3. A Suspended Semicircular Beam with a Sliding Lumped Mass
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| ALE | Arbitrary Lagrange–Euler |
| ANCF | Absolute Nodal Coordinate Formulation |
| RANCF | Rational Absolute Nodal Coordinate Formulation |
| CAD | Computer-Aided Design |
| CAA | Computer-Aided Analysis |
| NURBS | Nonuniform Rational B-Splines |
| ICADA | Integration of Computer-Aided Design and Analysis |
References
- Sugiyama, H.; Escalona, J.L.; Shabana, A.A. Formulation of three-dimensional joint constraints using the absolute nodal coordinates. Nonlinear Dyn. 2003, 31, 167–195. [Google Scholar] [CrossRef]
- Muñoz, J.J.; Jelenić, G. Sliding joints in 3D beams: Conserving algorithms using the master-slave approach. Multibody Syst. Dyn. 2006, 16, 237–261. [Google Scholar] [CrossRef] [Green Version]
- Mizuno, Y.; Sugiyama, H. Sliding and nonsliding joint constraints of B-spline plate elements for integration with flexible multibody dynamics simulation. J. Comput. Nonlinear Dyn. 2014, 9, 011001. [Google Scholar] [CrossRef]
- Lee, S.H.; Park, T.W.; Seo, J.H.; Yoon, J.W.; Jun, K.J. The development of a sliding joint for very flexible multibody dynamics using absolute nodal coordinate formulation. Multibody Syst. Dyn. 2008, 20, 223–237. [Google Scholar] [CrossRef]
- Gu, Y.; Lan, P.; Cui, Y.; Li, K.; Yu, Z. Dynamic interaction between the transmission wire and cross-frame. Mech. Mach. Theory 2021, 155, 104068. [Google Scholar] [CrossRef]
- Kulkarni, S.; Pappalardo, C.M.; Shabana, A.A. Pantograph/catenary contact formulations. ASME J. Vib. Acoust. 2016, 139, 011010. [Google Scholar] [CrossRef]
- Tang, J.; Ren, G.; Zhu, W.; Ren, H. Dynamics of variable-length tethers with application to tethered satellite deployment. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 3411–3424. [Google Scholar] [CrossRef]
- Luo, C.; Sun, J.; Wen, H.; Jin, D. Dynamics of a tethered satellite formation for space exploration modeled via ANCF. Acta Astronaut. 2020, 177, 882–890. [Google Scholar] [CrossRef]
- Zhang, H.; Guo, J.Q.; Liu, J.P.; Ren, G.X. An efficient multibody dynamic model of arresting cable systems based on ALE formulation. Mech. Mach. Theory 2020, 151, 103892. [Google Scholar] [CrossRef]
- Hong, D.; Ren, G. A modeling of sliding joint on one-dimensional flexible medium. Multibody Syst. Dyn. 2011, 26, 91–106. [Google Scholar] [CrossRef]
- Hong, D.; Tang, J.; Ren, G. Dynamic modeling of mass-flowing linear medium with large amplitude displacement and rotation. J. Fluids Struct. 2011, 27, 1137–1148. [Google Scholar] [CrossRef]
- Gerstmayr, J.; Sugiyama, H.; Mikkola, A. Review on the absolute nodal coordinate formulation for large deformation analysis of multibody systems. J. Comput. Nonlinear Dyn. 2013, 8, 031016. [Google Scholar] [CrossRef]
- Shabana, A.A. Definition of ANCF finite elements. J. Comput. Nonlinear Dyn. 2015, 10, 054506. [Google Scholar] [CrossRef]
- Ren, H.; Fan, W.; Zhu, W.D. An accurate and robust geometrically exact curved beam formulation for multibody dynamic analysis. ASME J. Vib. Acoust. 2017, 140, 011012. [Google Scholar] [CrossRef]
- Otsuka, K.; Wang, Y.; Fujita, K.; Nagai, H.; Makihara, K. Multifidelity modeling of deployable wings: Multibody dynamic simulation and wind tunnel experiment. AIAA J. 2019, 57, 4300–4311. [Google Scholar] [CrossRef]
- Kim, E.; Kim, H.; Cho, M. Model order reduction of multibody system dynamics based on stiffness evaluation in the absolute nodal coordinate formulation. Nonlinear Dyn. 2017, 87, 1901–1915. [Google Scholar] [CrossRef]
- Qi, Z.; Wang, J.; Wang, G. An efficient model for dynamic analysis and simulation of cable-pulley systems with time-varying cable lengths. Mech. Mach. Theory 2017, 116, 383–403. [Google Scholar] [CrossRef]
- Peng, Y.; Wei, Y.; Zhou, M. Efficient modeling of cable-pulley system with friction based on arbitrary-Lagrangian-Eulerian approach. Appl. Math. Mech. 2017, 38, 1785–1802. [Google Scholar] [CrossRef]
- Wang, J.; Qi, Z.; Wang, G. Hybrid modeling for dynamic analysis of cable-pulley systems with time-varying length cable and its application. J. Sound Vib. 2017, 406, 277–294. [Google Scholar] [CrossRef]
- Sanborn, G.G.; Shabana, A.A. A rational finite element method based on the absolute nodal coordinate formulation. Nonlinear Dyn. 2009, 58, 565. [Google Scholar] [CrossRef]
- Lan, P.; Shabana, A.A. Rational finite elements and flexible body dynamics. J. Vib. Acoust. 2010, 132, 041007. [Google Scholar] [CrossRef]
- Yamashita, H.; Sugiyama, H. Numerical convergence of finite element solutions of nonrational B-spline element and absolute nodal coordinate formulation. Nonlinear Dyn. 2012, 67, 177–189. [Google Scholar] [CrossRef]
- Mikkola, A.; Shabana, A.A.; Sanchez-Rebollo, C.; Jimenez-Octavio, J.R. Comparison between ANCF and B-spline surfaces. Multibody Syst. Dyn. 2013, 30, 119–138. [Google Scholar] [CrossRef]
- Nada, A.A. Use of B-spline surface to model large-deformation continuum plates: Procedure and applications. Nonlinear Dyn. 2013, 72, 243–263. [Google Scholar] [CrossRef]
- Hughes, T.J.R.; Cottrell, J.A.; Bazilevs, Y. Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput. Methods Appl. Mech. Eng. 2005, 194, 4135–4195. [Google Scholar] [CrossRef] [Green Version]
- Piegl, L.A.; Tiller, W. The NURBS Book, 2nd ed.; Springer: New York, NY, USA, 1997. [Google Scholar] [CrossRef]
- Sanborn, G.G.; Shabana, A.A. On the integration of computer aided design and analysis using the finite element absolute nodal coordinate formulation. Multibody Syst. Dyn. 2009, 22, 181–197. [Google Scholar] [CrossRef]
- Fan, W.; Zhu, W.D. An accurate singularity-free formulation of a three-dimensional curved Eule-Bernoulli beam for flexible multibody dynamic analysis. ASME J. Vib. Acoust. 2016, 138, 051001. [Google Scholar] [CrossRef]
- Gerstmayr, J.; Shabana, A.A. Analysis of thin beams and cables using the absolute nodal co-ordinate formulation. Nonlinear Dyn. 2006, 45, 109–130. [Google Scholar] [CrossRef]
- Berzeri, M.; Shabana, A. Development of simple models for the elastic forces in the absolute nodal coordinate formulation. J. Sound Vib. 2000, 235, 539–565. [Google Scholar] [CrossRef]
- Shabana, A.A. Dynamics of Multibody Systems; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar] [CrossRef]
- Arnold, M.; Brüls, O. Convergence of the generalized-α scheme for constrained mechanical systems. Multibody Syst. Dyn. 2007, 18, 185–202. [Google Scholar] [CrossRef]


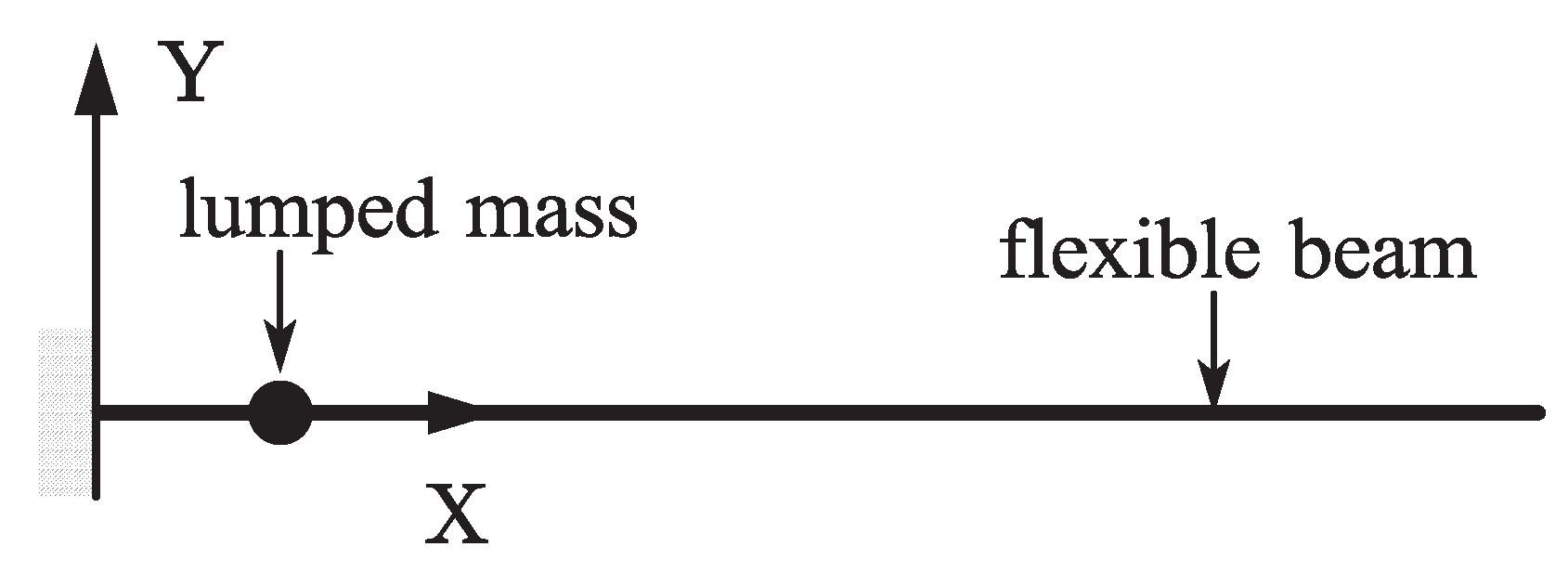



| Number of Elements | LC Times | ALE-RANCF | ALE-ANCF | ||
|---|---|---|---|---|---|
| Motion Time(s) | LC Time(s) | Motion Time(s) | LC Time(s) | ||
| 5 | 3 | 59.9 | 16.1 | 34.6 | / |
| 10 | 9 | 97.1 | 69.1 | 58.3 | / |
| 20 | 18 | 169.2 | 157.0 | 103.1 | / |
| 30 | 26 | 292.4 | 238.3 | 192.4 | / |
| 40 | 35 | 478.0 | 378.2 | 325.0 | / |
| Number of Elements | Size of Set | Maximum | Minimum | Deviation |
|---|---|---|---|---|
| 5 | 6 | 1.32 | 0.44 | 0.27 |
| 10 | 24 | 1.18 | 0.12 | 0.29 |
| 20 | 57 | 1.15 | 0.73 | 0.06 |
| 30 | 81 | 1.16 | 0.95 | 0.03 |
| 40 | 108 | 1.07 | 0.72 | 0.04 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, Z.; Ouyang, B. A Variable-Length Rational Finite Element Based on the Absolute Nodal Coordinate Formulation. Machines 2022, 10, 174. https://doi.org/10.3390/machines10030174
Ding Z, Ouyang B. A Variable-Length Rational Finite Element Based on the Absolute Nodal Coordinate Formulation. Machines. 2022; 10(3):174. https://doi.org/10.3390/machines10030174
Chicago/Turabian StyleDing, Zhishen, and Bin Ouyang. 2022. "A Variable-Length Rational Finite Element Based on the Absolute Nodal Coordinate Formulation" Machines 10, no. 3: 174. https://doi.org/10.3390/machines10030174
APA StyleDing, Z., & Ouyang, B. (2022). A Variable-Length Rational Finite Element Based on the Absolute Nodal Coordinate Formulation. Machines, 10(3), 174. https://doi.org/10.3390/machines10030174






