Study on Nonlinear Correlation in Modal Coefficients of the Bionic Airfoil
Abstract
:1. Introduction
2. Test Case
2.1. Geometric Construction
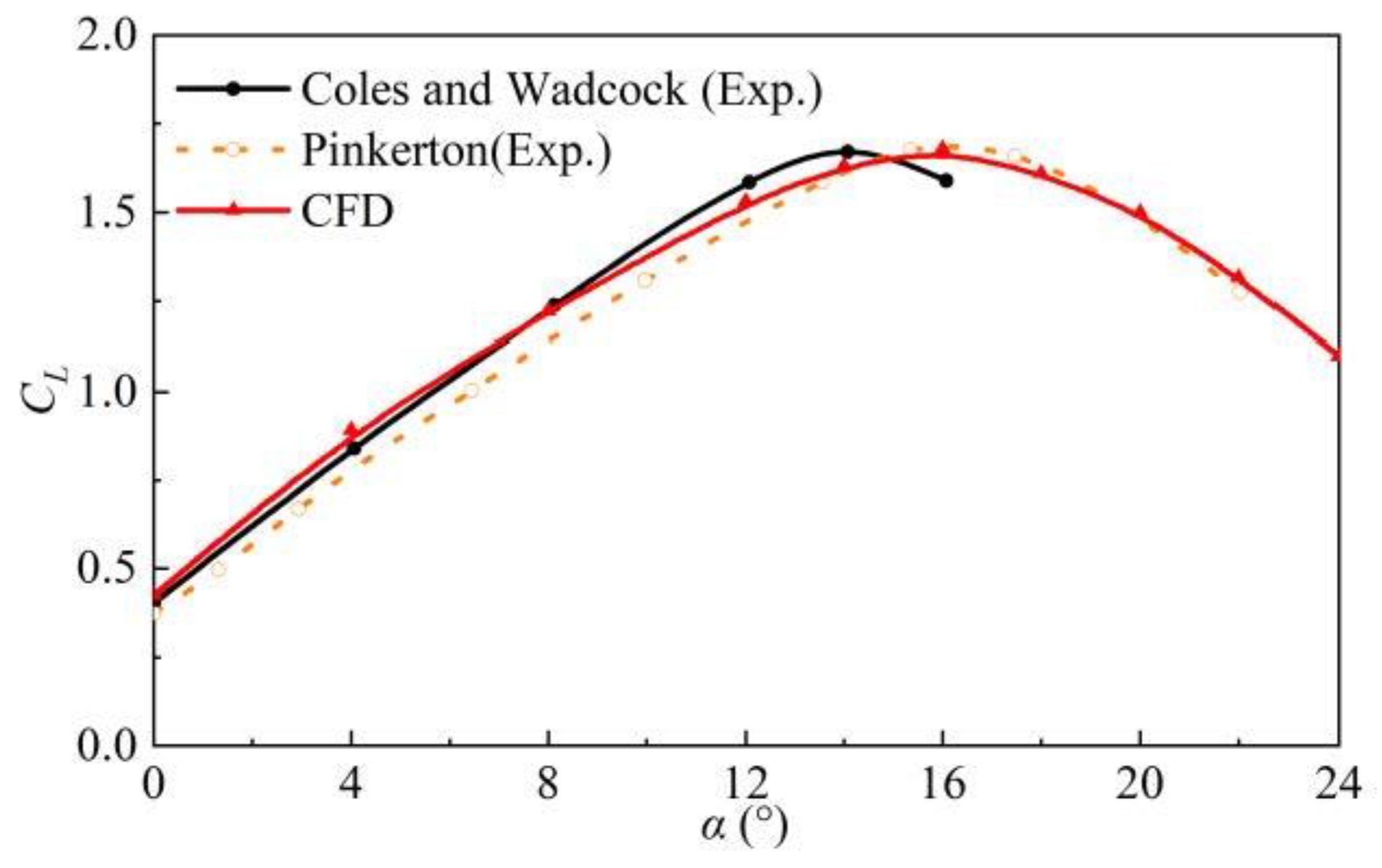
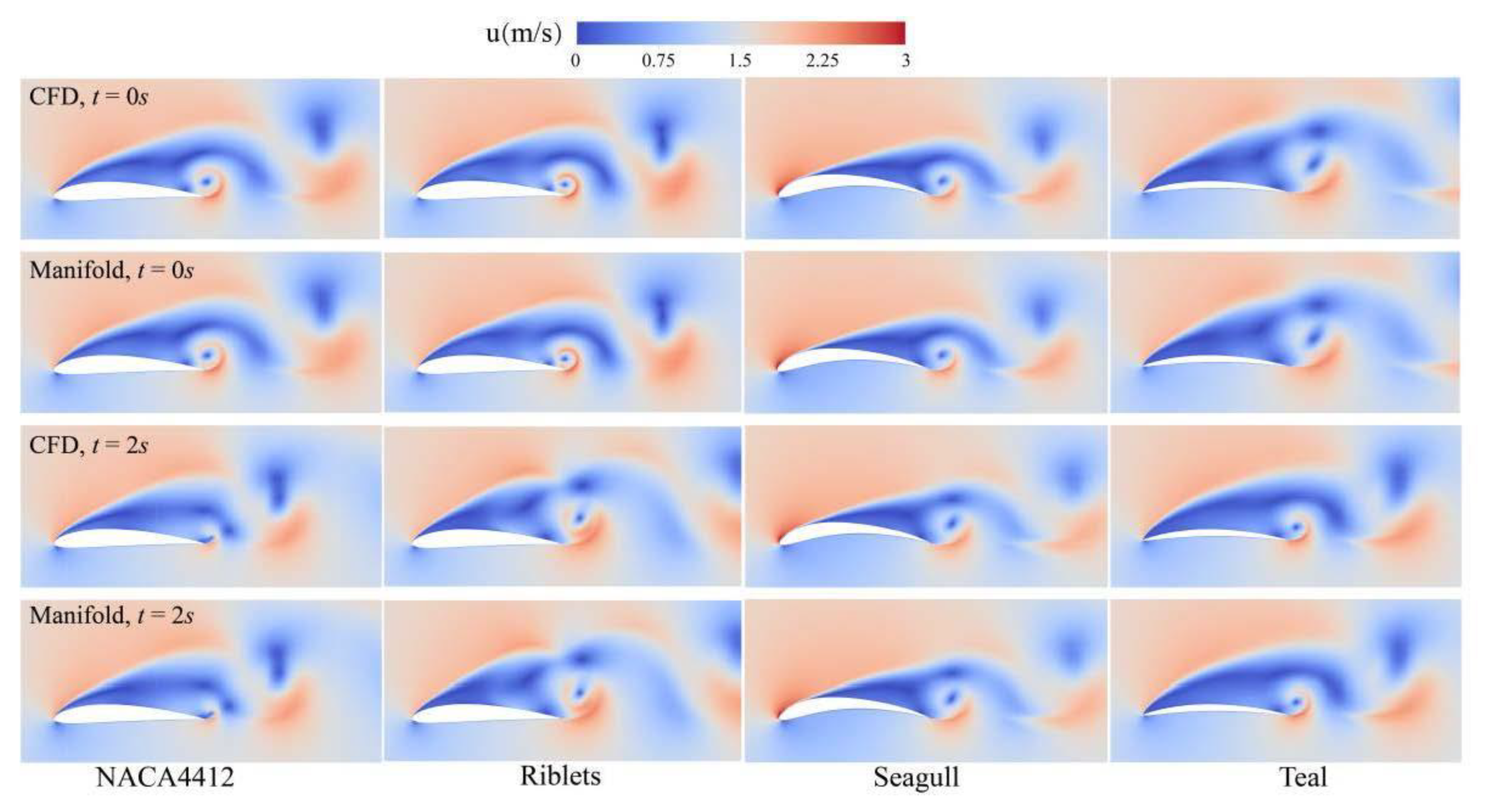
2.2. Numerical Method and Experimental Verification
3. Nonlinear Dependence of Modal Coefficients
3.1. Dynamic Mode Decomposition
3.2. Nonlinear Correlation
4. Construction of the Minimum Reduced-Order Model
4.1. The Sparse Identification of Nonlinear Dynamics
4.2. Manifold Dynamics and Flow Field Reconstruction
5. Conclusions
- (1)
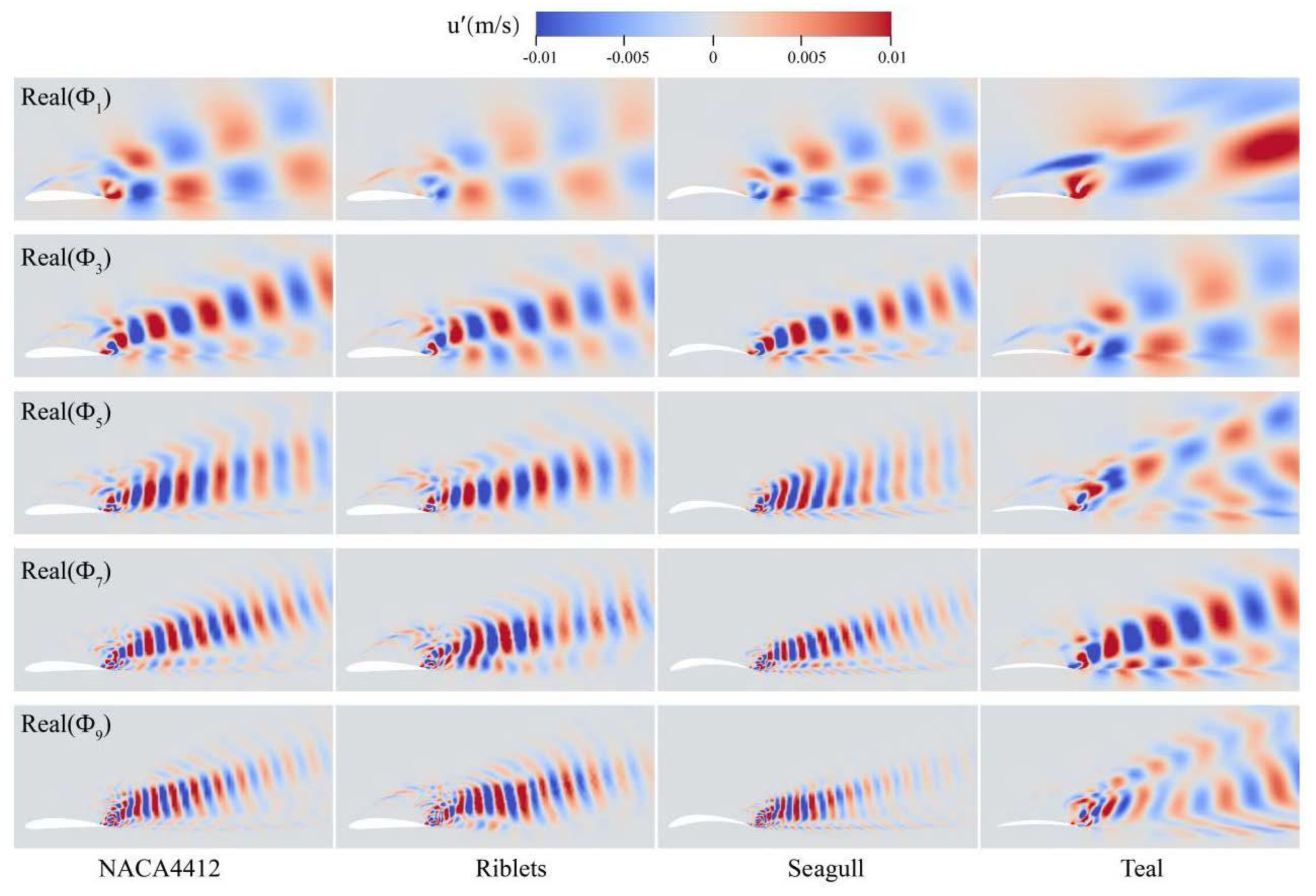
- The Seagull airfoil in the bionic airfoil has a more significant lift coefficient, while the Riblets has the smallest lift coefficient. The modes of airfoils are antisymmetric vortex-shedding structures. Under a large number of tiny serrations, the vortex-shedding structure in the middle of the upper surface of the Riblets airfoil disappears, and the high-frequency trailing edge vortex-shedding structure is different from the NACA4412 airfoil. The lift coefficient of the Teal airfoil is similar to the NACA4412 airfoil, but the vortex shedding frequency is significantly higher than the Seagull airfoil and the NACA4412 airfoil. The NACA4412, Riblets, and Seagull airfoil are mainly dominated by the trailing edge shedding vortex, while the Teal airfoil also includes the leading-edge LSB.
- (2)
- The first twenty DMD modes of the three airfoils are sufficient to ensure the accuracy of the velocity field reconstruction, and the spatial distribution of the modes is very similar. The mode frequency is multiple when the modal system is sorted by frequency. The relationship between the modal coefficients of the four airfoils is pure harmonic, and the shape is pure oscillation. The difference between modal coefficients is the difference between amplitude and phase. The phase difference between the paired modal coefficients is 90°, and the order of Lissajous orbits is conducive to building the specific function expression of nonlinear correlation.
- (3)
- The NACA4412, Riblets, Seagull, and Teal airfoil modal coefficients have the same type of nonlinear correlation, which is related to the frequency corresponding to the mode. The active mode has only a pair of active modes corresponding to the fundamental frequency. The rest of the modal coefficients can be represented by the active modes’ polynomial functions. The two-dimensional manifold equations constructed by the four airfoils are consistent, and the absolute value of the coefficient corresponds to the fundamental frequency of the airfoil vortex shedding. The flow field reconstructed based on manifold dynamics is in high agreement with the numerical simulation results, validating the accuracy of the simple, compact, and interpretable flow field equations.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Aabid, A.; Parveez, B.; Parveen, N.; Khan, S.A.; Zayan, J.M.; Shabbir, O. Reviews on Design and Development of Unmanned Aerial Vehicle (Drone) for Different Applications. J. Mech. Eng. Res. Dev. 2022, 45, 53–69. [Google Scholar]
- Wright, A.K.; Wood, D.H. The starting and low wind speed behaviour of a small horizontal axis wind turbine. J. Wind Eng. Ind. Aerod. 2004, 92, 1265–1279. [Google Scholar] [CrossRef]
- Zhao, M.; Zhang, M.; Xu, J. Numerical simulation of flow characteristics behind the aerodynamic performances on an airfoil with leading edge protuberances. Eng. Appl. Comp. Fluid 2017, 11, 193–209. [Google Scholar] [CrossRef] [Green Version]
- Lewthwaite, M.T.; Amaechi, C.V. Numerical investigation of winglet aerodynamics and dimple effect of NACA 0017 airfoil for a freight aircraft. Inventions 2022, 7, 31. [Google Scholar] [CrossRef]
- Tian, H.; Shan, X.; Cao, H.; Xie, T. Enhanced performance of airfoil-based piezoaeroelastic energy harvester: Numerical simulation and experimental verification. Mech. Syst. Signal Process. 2022, 162, 108065. [Google Scholar] [CrossRef]
- Li, Z.; Shi, D.; Li, S.; Yang, X.; Miao, G. A systematical weight function modified critical distance method to estimate the creep-fatigue life of geometrically different structures. Int. J. Fatigue 2019, 126, 6–19. [Google Scholar] [CrossRef]
- Tripathi, D.; Vishal, S.; Bose, C.; Venkatramani, J. Stall-induced fatigue damage in nonlinear aeroelastic systems under stochastic inflow: Numerical and experimental analyses. Int. J. Nonlin. Mech. 2022, 142, 104003. [Google Scholar] [CrossRef]
- Plante, F.; Dandois, J.; Beneddine, S.; Laurendeau, É.; Sipp, D. Link between subsonic stall and transonic buffet on swept and unswept wings: From global stability analysis to nonlinear dynamics. J. Fluid Mech. 2021, 908, A16. [Google Scholar] [CrossRef]
- Akram, M.T.; Kim, M.H. Aerodynamic shape optimization of NREL S809 airfoil for wind turbine blades using reynolds-averaged navier stokes model—Part II. Appl. Sci. 2021, 11, 2211. [Google Scholar] [CrossRef]
- Jung, Y.S.; Vijayakumar, G.; Ananthan, S.; Baeder, J. Local correlation-based transition models for high-Reynolds-number wind-turbine airfoils. Wind Energy Sci. 2022, 7, 603–622. [Google Scholar] [CrossRef]
- Błoński, D.; Strzelecka, K.; Kudela, H. Vortex Trapping Cavity on Airfoil: High-Order Penalized Vortex Method Numerical Simulation and Water Tunnel Experimental Investigation. Energies 2021, 14, 8402. [Google Scholar] [CrossRef]
- Ma, A.; Gibeau, B.; Ghaemi, S. Time-resolved topology of turbulent boundary layer separation over the trailing edge of an airfoil. J. Fluid Mech. 2020, 891, 1–32. [Google Scholar] [CrossRef]
- Raheem, M.A.; Edi, P.; Pasha, A.A.; Rahman, M.M.; Juhany, K.A. Numerical study of variable camber continuous trailing edge flap at off-design conditions. Energies 2019, 12, 3185. [Google Scholar] [CrossRef] [Green Version]
- Zhao, M.; Zhao, Y.; Liu, Z. Dynamic mode decomposition analysis of flow characteristics of an airfoil with leading edge protuberances. Aerosp. Sci. Technol. 2020, 98, 105684. [Google Scholar] [CrossRef]
- Yuan, Y.; Yu, X.; Yang, X.; Xiao, Y.; Xiang, B.; Wang, Y. Bionic building energy efficiency and bionic green architecture: A review. Renew. Sust. Energ. Rev. 2017, 74, 771–787. [Google Scholar] [CrossRef]
- Lotfabadi, P.; Alibaba, H.Z.; Arfaei, A. Sustainability; as a combination of parametric patterns and bionic strategies. Renew. Sust. Energ. Rev. 2016, 57, 1337–1346. [Google Scholar] [CrossRef]
- Wang, T.; Wang, Z.; Zhang, B. Mechanism Design and Experiment of a Bionic Turtle Dredging Robot. Machines 2021, 9, 86. [Google Scholar] [CrossRef]
- Howe, M.S. Aerodynamic noise of a serrated trailing edge. J. Fluid Struct. 1991, 5, 33–45. [Google Scholar] [CrossRef]
- Avallone, F.; Van der Velden, W.C.P.; Ragni, D. Benefits of curved serrations on broadband trailing-edge noise reduction. J. Sound Vib. 2017, 400, 167–177. [Google Scholar] [CrossRef] [Green Version]
- Huang, X. Theoretical model of acoustic scattering from a flat plate with serrations. J. Fluid Mech. 2017, 819, 228–257. [Google Scholar] [CrossRef]
- Miklosovic, D.S.; Murray, M.M.; Howle, L.E.; Fish, F.E. Leading-edge tubercles delay stall on humpback whale (Megaptera novaeangliae) flippers. Phys. Fluid. 2004, 16, 39–42. [Google Scholar] [CrossRef]
- Hansen, K.L.; Kelso, R.M.; Dally, B.B. Performance variations of leading-edge tubercles for distinct airfoil profiles. AIAA J. 2011, 49, 185–194. [Google Scholar] [CrossRef]
- Rostamzadeh, N.; Hansen, K.L.; Kelso, R.M.; Dally, B.B. The formation mechanism and impact of streamwise vortices on NACA 0021 airfoil’s performance with undulating leading edge modification. Phys. Fluid. 2014, 26, 107101. [Google Scholar] [CrossRef]
- Srinivas, K.S.; Datta, A.; Bhattacharyya, A.; Kumar, S. Free-stream characteristics of bio-inspired marine rudders with different leading-edge configurations. Ocean Eng. 2018, 170, 148–159. [Google Scholar] [CrossRef]
- Kant, R.; Bhattacharyya, A. A bio-inspired twin-protuberance hydrofoil design. Ocean Eng. 2020, 218, 108209. [Google Scholar] [CrossRef]
- Chang, H.; Cai, C.; Li, D.; Zuo, Z.; Wang, H.; Liu, S. Effects of Reynolds Number and Protuberance Amplitude on Twin-Protuberance Airfoil Performance. AIAA J. 2022, 60, 3775–3788. [Google Scholar] [CrossRef]
- Pereira, L.T.L.; Ragni, D.; Avallone, F.; Scarano, F. Aeroacoustics of sawtooth trailing-edge serrations under aerodynamic loading. J. Sound Vib. 2022, 537, 117202. [Google Scholar] [CrossRef]
- Wang, L.; Liu, X.; Li, D. Noise reduction mechanism of airfoils with leading-edge serrations and surface ridges inspired by owl wings. Phys. Fluid. 2021, 33, 015123. [Google Scholar] [CrossRef]
- Li, D.; Chang, H.; Zuo, Z.; Wang, H.; Liu, S. Aerodynamic characteristics and mechanisms for bionic airfoils with different spacings. Phys. Fluid. 2021, 33, 064101. [Google Scholar] [CrossRef]
- Huang, S.; Hu, Y.; Wang, Y. Research on aerodynamic performance of a novel dolphin head-shaped bionic airfoil. Energy 2021, 214, 118179. [Google Scholar] [CrossRef]
- Liu, T.; Kuykendoll, K.; Rhew, R.; Jones, S. Avian wing geometry and kinematics. AIAA J. 2006, 44, 954–963. [Google Scholar] [CrossRef]
- Song, L.; Tian, K.; Jiao, X.; Feng, R.; Wang, L.; Tian, R. Design and optimization of seagull airfoil wind energy conversion device. Int. J. Green Energy 2021, 18, 1046–1063. [Google Scholar] [CrossRef]
- Li, D.; Liu, X. A comparative study on aerodynamic performance and noise characteristics of two kinds of long-eared owl wing models. J. Mech. Sci. Technol. 2017, 31, 3821–3830. [Google Scholar] [CrossRef]
- Huang, S.; Qiu, H.; Wang, Y. Aerodynamic performance of horizontal axis wind turbine with application of dolphin head-shape and lever movement of skeleton bionic airfoils. Energ. Convers. Manag. 2022, 267, 115803. [Google Scholar] [CrossRef]
- Zargar, O.A.; Lin, T.; Zebua, A.G.; Lai, T.J.; Shih, Y.C.; Hu, S.C.; Leggett, G. The effects of surface modification on aerodynamic characteristics of airfoil DU 06 W 200 at low Reynolds numbers. Int. J. Thermo. 2022, 16, 100208. [Google Scholar] [CrossRef]
- Mallik, W.; Raveh, D.E. Aerodynamic damping investigations of light dynamic stall on a pitching airfoil via modal analysis. J. Fluid. Struct. 2020, 98, 103111. [Google Scholar] [CrossRef]
- Schmid, P.J. Dynamic mode decomposition and its variants. Annu. Rev. Fluid Mech. 2022, 54, 225–254. [Google Scholar] [CrossRef]
- Naderi, M.H.; Eivazi, H.; Esfahanian, V. New method for dynamic mode decomposition of flows over moving structures based on machine learning (hybrid dynamic mode decomposition). Phys. Fluids 2019, 31, 127102. [Google Scholar] [CrossRef]
- Mohan, A.T.; Gaitonde, D.V. Analysis of airfoil stall control using dynamic mode decomposition. J. Aircr. 2017, 54, 1508–1520. [Google Scholar] [CrossRef]
- Tirandaz, M.R.; Rezaeiha, A. Effect of airfoil shape on power performance of vertical axis wind turbines in dynamic stall: Symmetric Airfoils. Renew. Energy 2021, 173, 422–441. [Google Scholar] [CrossRef]
- Callaham, J.L.; Brunton, S.L.; Loiseau, J.C. On the role of nonlinear correlations in reduced-order modelling. J. Fluid Mech. 2022, 938, A1. [Google Scholar] [CrossRef]
- Brunton, S.L.; Proctor, J.L.; Kutz, J.N. Discovering governing equations from data by sparse identification of nonlinear dynamical systems. Proc. Natl. Acad. Sci. USA 2016, 113, 3932–3937. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sanei, M.; Razaghi, R. Numerical investigation of three turbulence simulation models for S809 wind turbine airfoil. Proc. Inst. Mech. Eng. Part A J. Power Energy 2018, 232, 1037–1048. [Google Scholar] [CrossRef]
- Winter, O.; Sváček, P. On numerical simulation of flexibly supported airfoil in interaction with incompressible fluid flow using laminar–turbulence transition model. Comput. Math. Appl. 2021, 83, 57–73. [Google Scholar] [CrossRef]
- Yang, X.; Wang, J.; Jiang, B.; Li, Z.A.; Xiao, Q. Numerical Study of Effect of Sawtooth Riblets on Low-Reynolds-Number Airfoil Flow Characteristic and Aerodynamic Performance. Processes 2021, 9, 2102. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef] [Green Version]
- Coles, D.; Wadcock, A.J. Flying-Hot-Wire Study of 2-Dimensional Mean Flow Past an NACA 4412 Airfoil at Maximum Lift. Bull. Am. Phys. Soc. 1978, 23, 991. [Google Scholar]
- Pinkerton, R.M. The Variation with Reynolds Number of Pressure Distribution over an Airfoil Section (No. NACA-TR-613); US Government Printing Office: Washington, WA, USA, 1938.
- Noack, B.R.; Afanasiev, K.; Morzyński, M.; Tadmor, G.; Thiele, F. A hierarchy of low-dimensional models for the transient and post-transient cylinder wake. J. Fluid Mech. 2003, 497, 335–363. [Google Scholar] [CrossRef] [Green Version]
- Loiseau, J.C.; Noack, B.R.; Brunton, S.L. Sparse reduced-order modelling: Sensor-based dynamics to full-state estimation. J. Fluid Mech. 2018, 844, 459–490. [Google Scholar] [CrossRef] [Green Version]
- Rowley, C.W.; Mezić, I.; Bagheri, S.; Schlatter, P.; Henningson, D.S. Spectral analysis of nonlinear flows. J. Fluid Mech. 2009, 641, 115–127. [Google Scholar] [CrossRef] [Green Version]
- Cai, Z.; Dang, Z.; Wen, M.; Lv, Y.; Duan, H. Application of Compressed Sensing Based on Adaptive Dynamic Mode Decomposition in Signal Transmission and Fault Extraction of Bearing Signal. Machines 2022, 10, 353. [Google Scholar] [CrossRef]
- Gibeau, B.; Koch, C.R.; Ghaemi, S. Active control of vortex shedding from a blunt trailing edge using oscillating piezoelectric flaps. Phys. Rev. Fluid. 2019, 4, 054704. [Google Scholar] [CrossRef]
- Lopez-Paz, D.; Hennig, P.; Schölkopf, B. The randomized dependence coefficient. Adv. Neural Inf. Process. Syst. 2013, 26, 1–9. [Google Scholar]
- Rudy, S.H.; Brunton, S.L.; Proctor, J.L.; Kutz, J.N. Data-driven discovery of partial differential equations. Sci. Adv. 2017, 3, 3932–3937. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Loiseau, J.C. Data-driven modeling of the chaotic thermal convection in an annular thermosyphon. Theor. Comp. Fluid Dyn. 2020, 34, 339–365. [Google Scholar] [CrossRef]
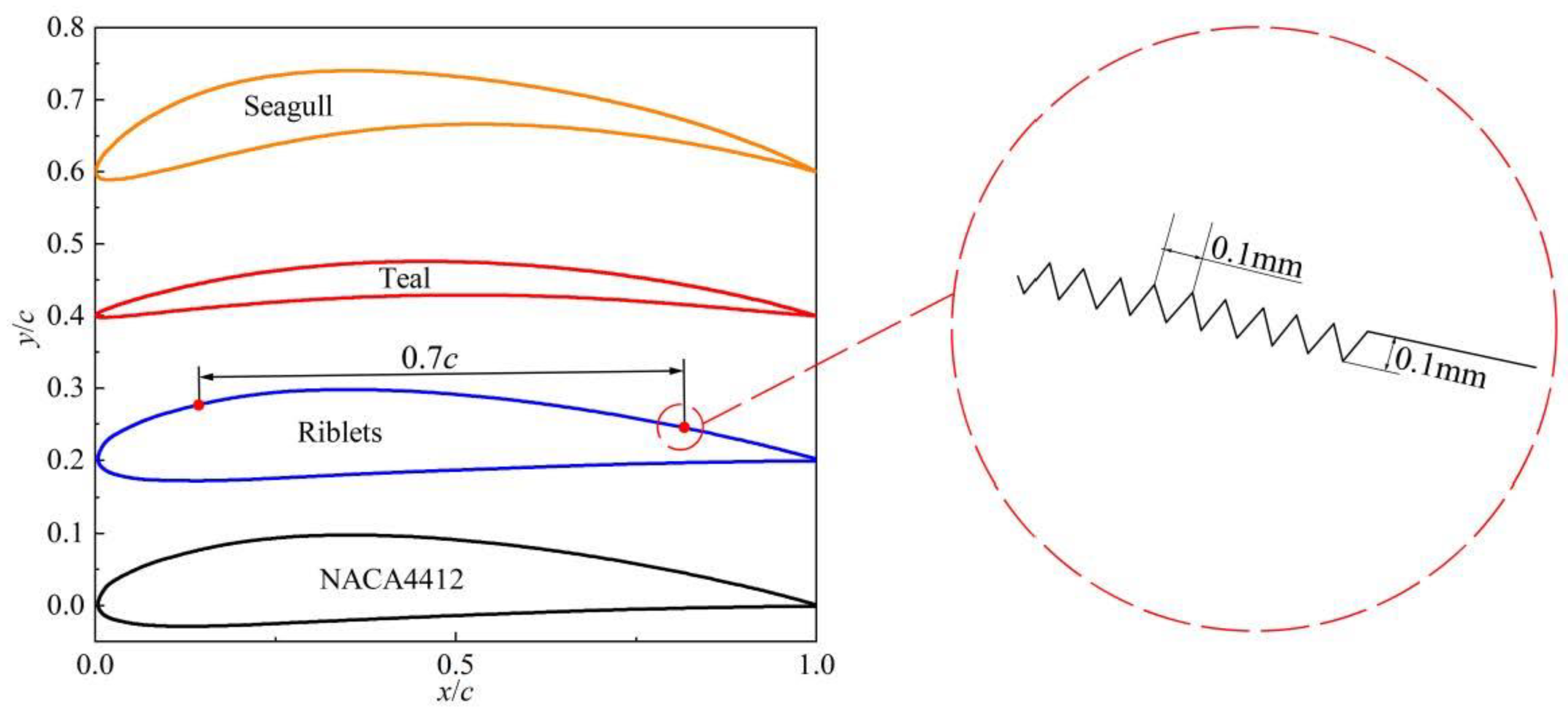






| Coefficient | Seagull | Teal |
|---|---|---|
| S1 | 3.8735 | 3.9917 |
| S2 | −0.807 | −0.3677 |
| S3 | 0.771 | 0.0239 |
| A1 | −15.246 | 1.7804 |
| A2 | 26.482 | −13.6875 |
| A3 | −18.975 | 18.276 |
| A4 | 4.6232 | −8.279 |
| Coefficient | Seagull | Teal |
|---|---|---|
| ac | 0.14 | 0.11 |
| at | 0.1 | 0.05 |
| bc | 1.33 | 4 |
| bt | 3.54 | 4 |
| dc | 1.4 | 1.4 |
| dt | 1.4 | 1.4 |
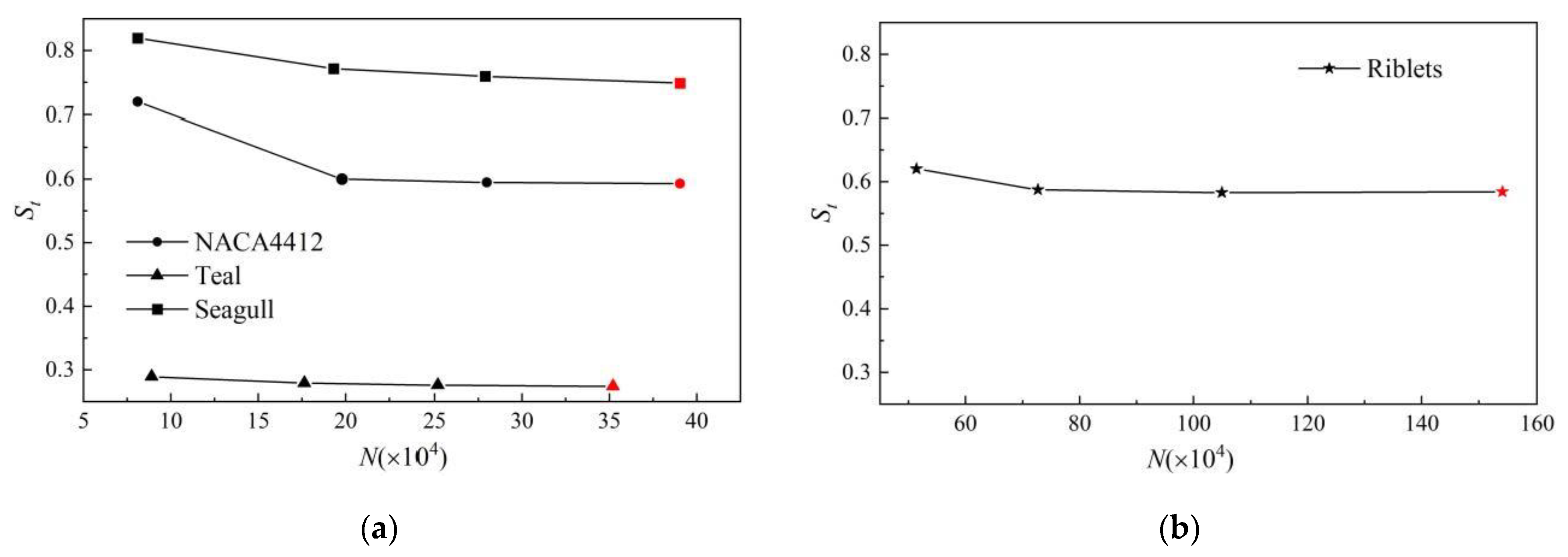
| Coefficient | NACA4412 | Riblets | Seagull | Teal |
|---|---|---|---|---|
| ω (rad/s) | 5.446 | 5.364 | 6.878 | 2.518 |
| St | 0.593 | 0.584 | 0.749 | 0.274 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, Q.; Wang, J.; Jiang, B.; Ding, Y.; Yang, X. Study on Nonlinear Correlation in Modal Coefficients of the Bionic Airfoil. Machines 2023, 11, 88. https://doi.org/10.3390/machines11010088
Xiao Q, Wang J, Jiang B, Ding Y, Yang X. Study on Nonlinear Correlation in Modal Coefficients of the Bionic Airfoil. Machines. 2023; 11(1):88. https://doi.org/10.3390/machines11010088
Chicago/Turabian StyleXiao, Qianhao, Jun Wang, Boyan Jiang, Yanyan Ding, and Xiaopei Yang. 2023. "Study on Nonlinear Correlation in Modal Coefficients of the Bionic Airfoil" Machines 11, no. 1: 88. https://doi.org/10.3390/machines11010088
APA StyleXiao, Q., Wang, J., Jiang, B., Ding, Y., & Yang, X. (2023). Study on Nonlinear Correlation in Modal Coefficients of the Bionic Airfoil. Machines, 11(1), 88. https://doi.org/10.3390/machines11010088









