Numerical Examination of the Dynamic Evolution of Fluctuations in Cavitation and Pressure in a Centrifugal Pump during Startup
Abstract
1. Introduction
2. Materials and Methods
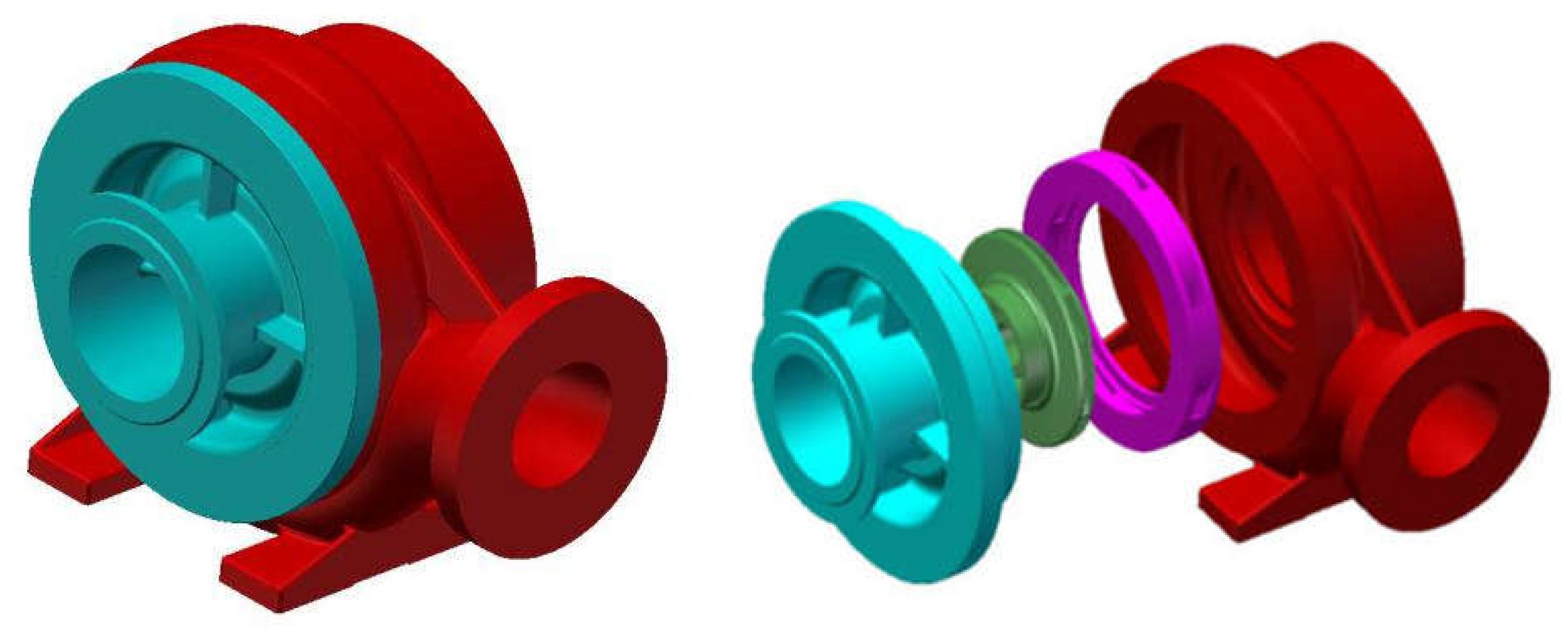
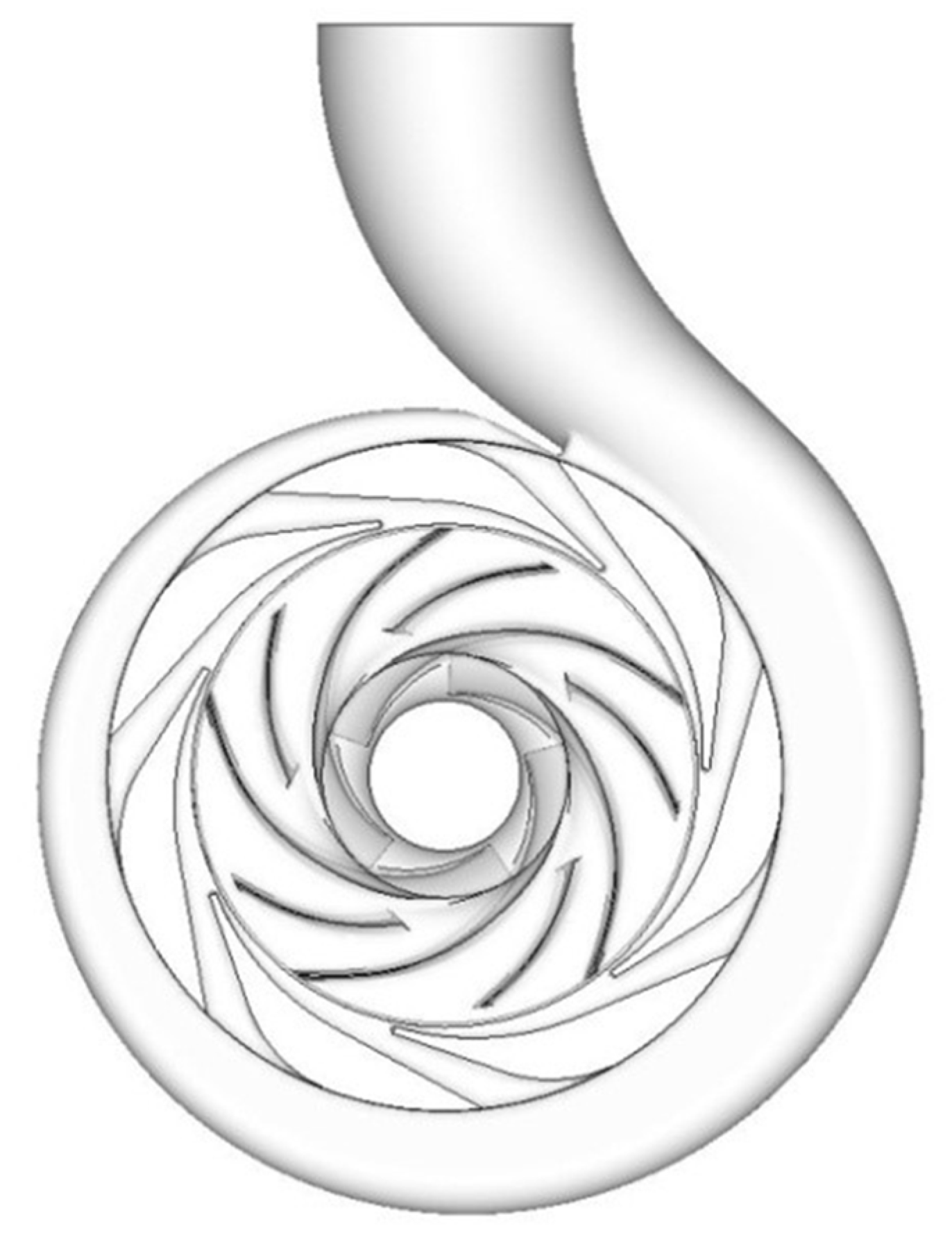
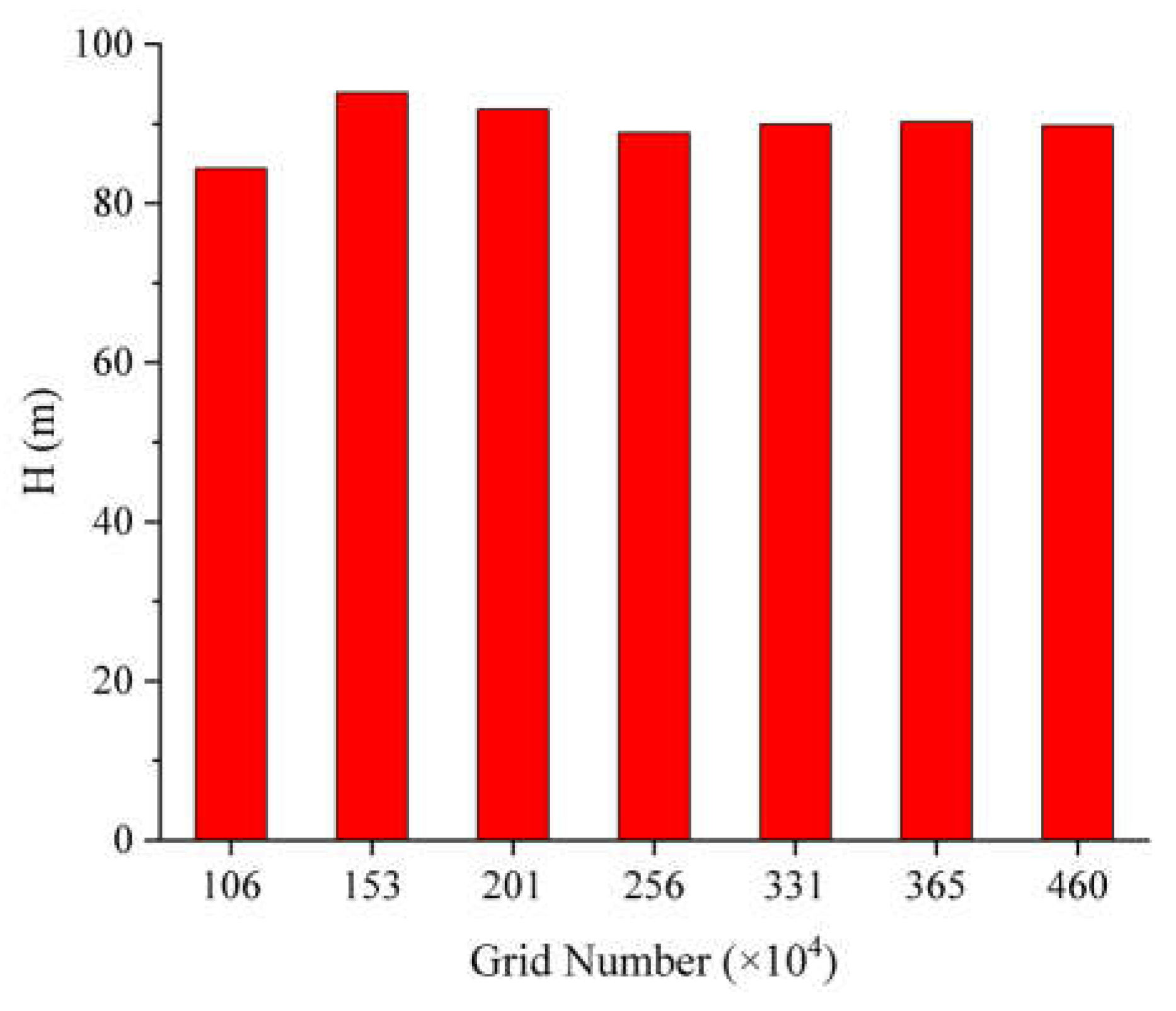
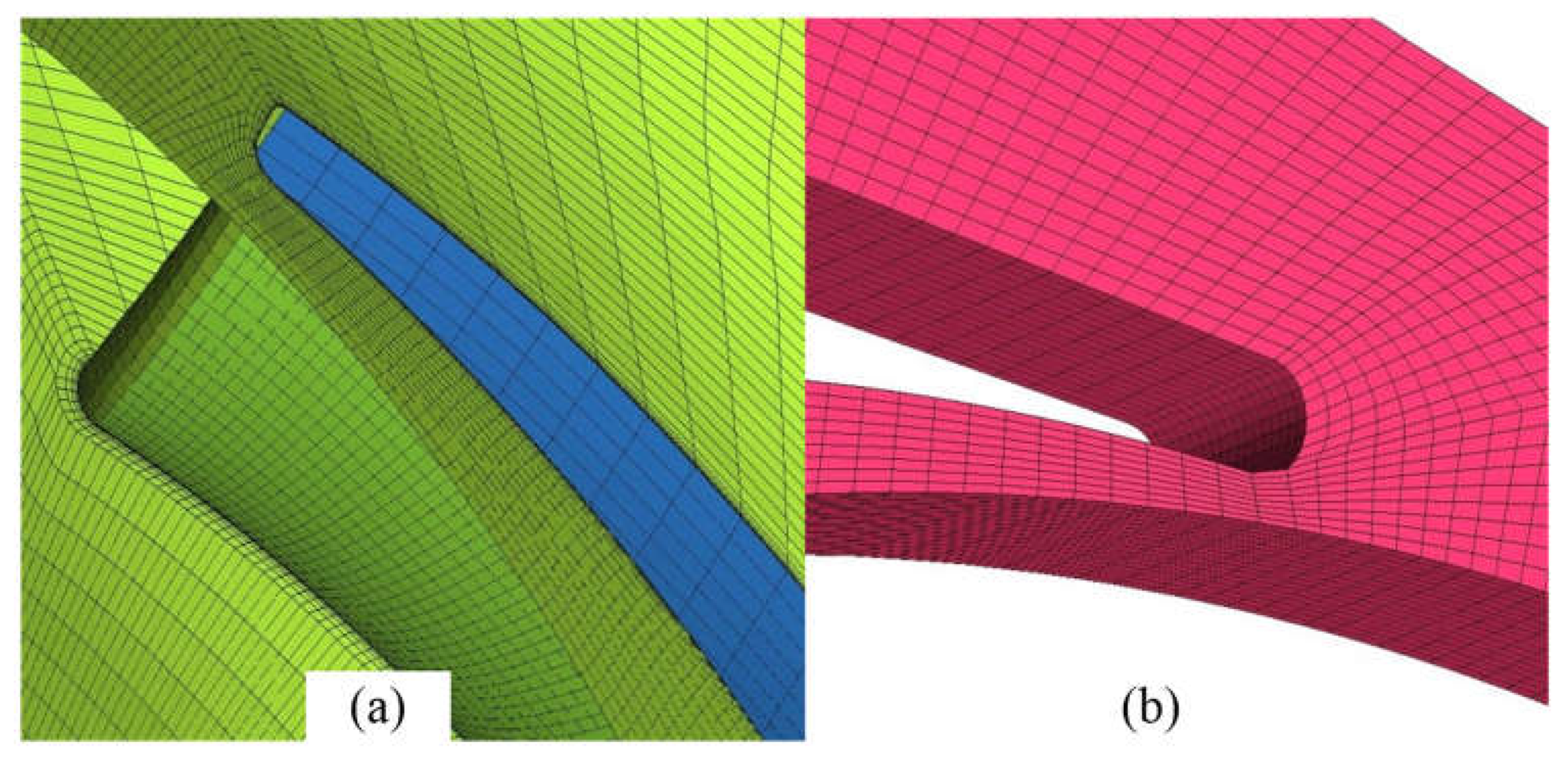
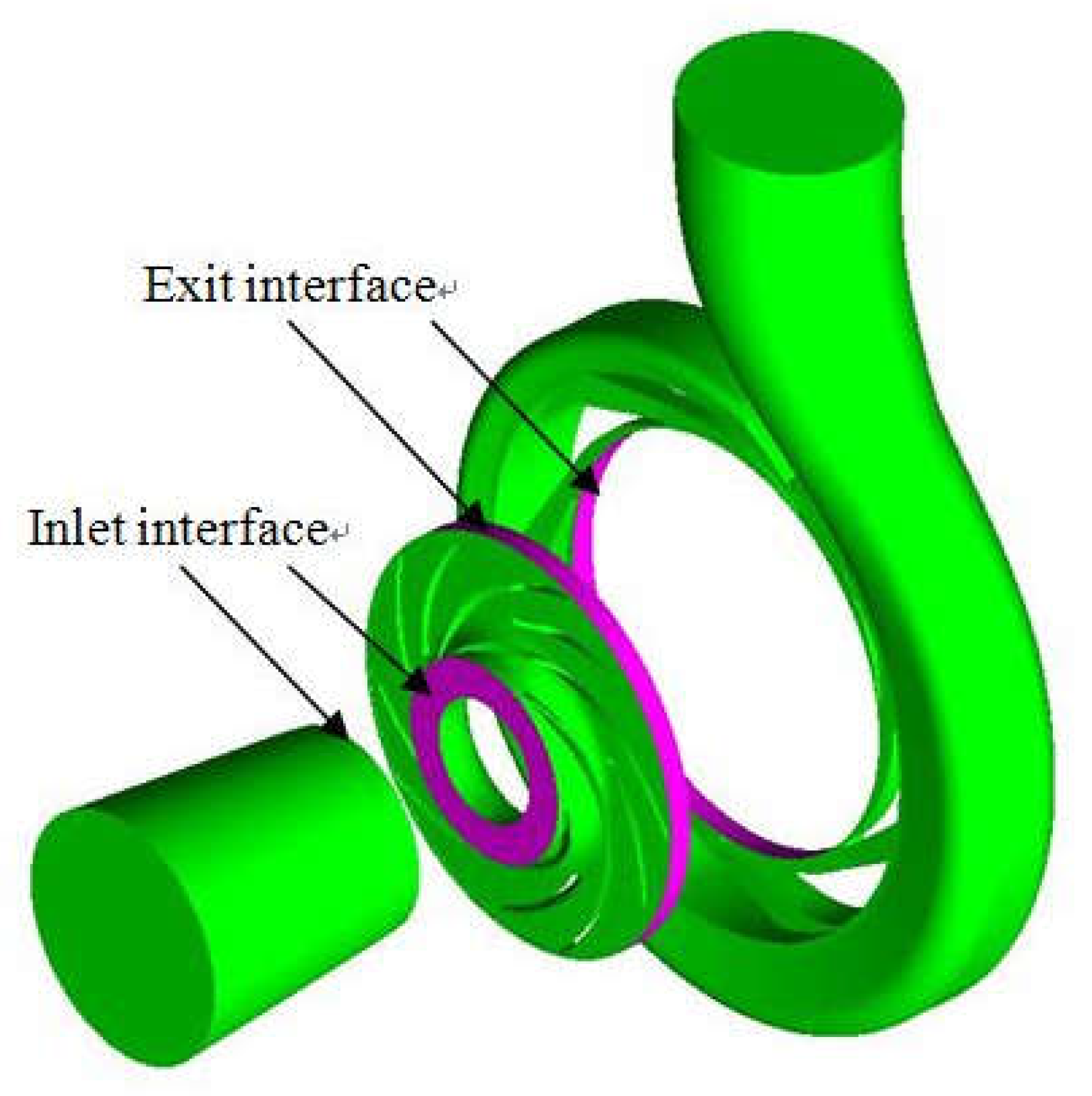
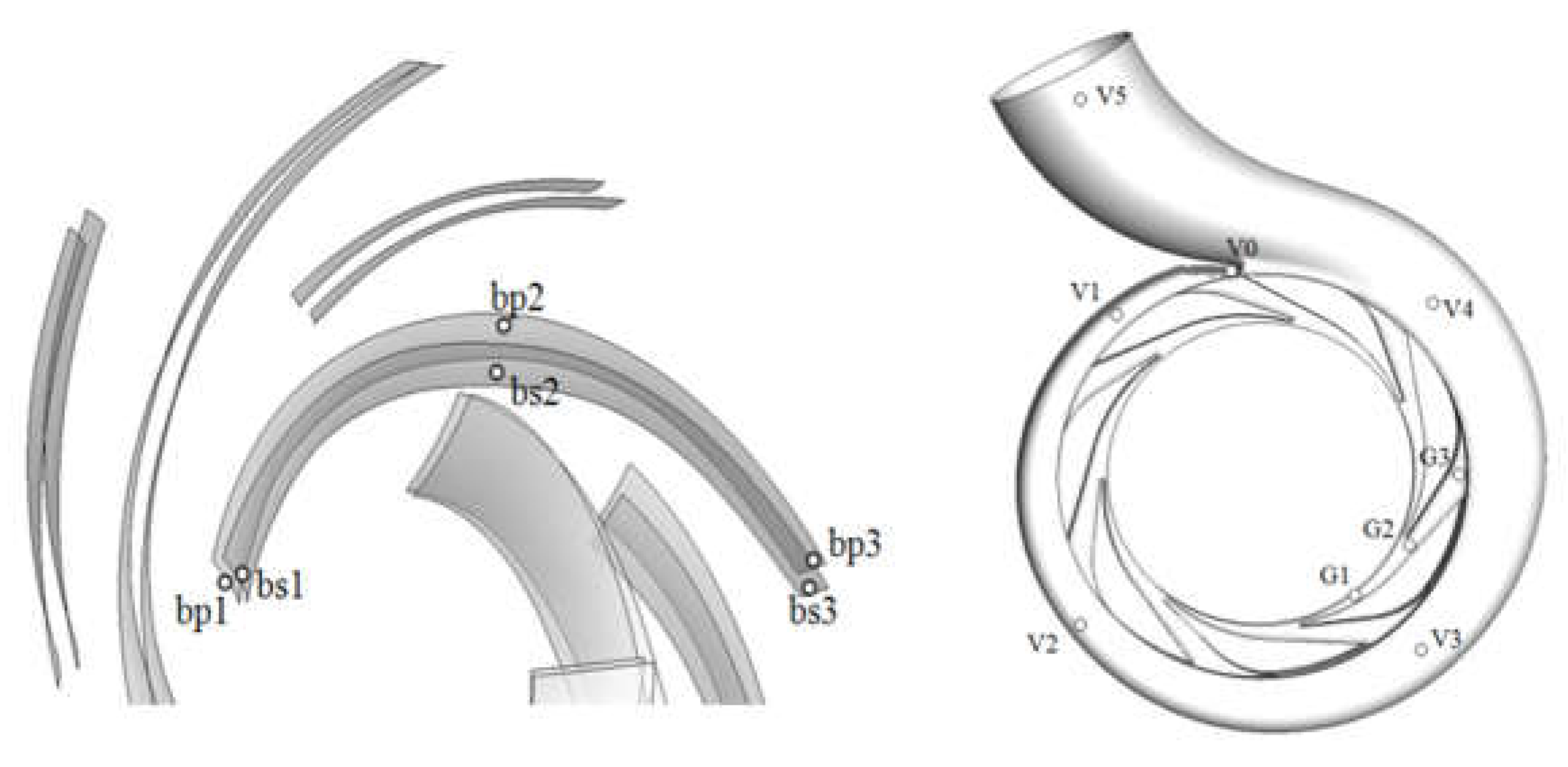
2.1. Computational Domain and Mesh System
2.2. Governing Equations
2.3. Cavitation Model
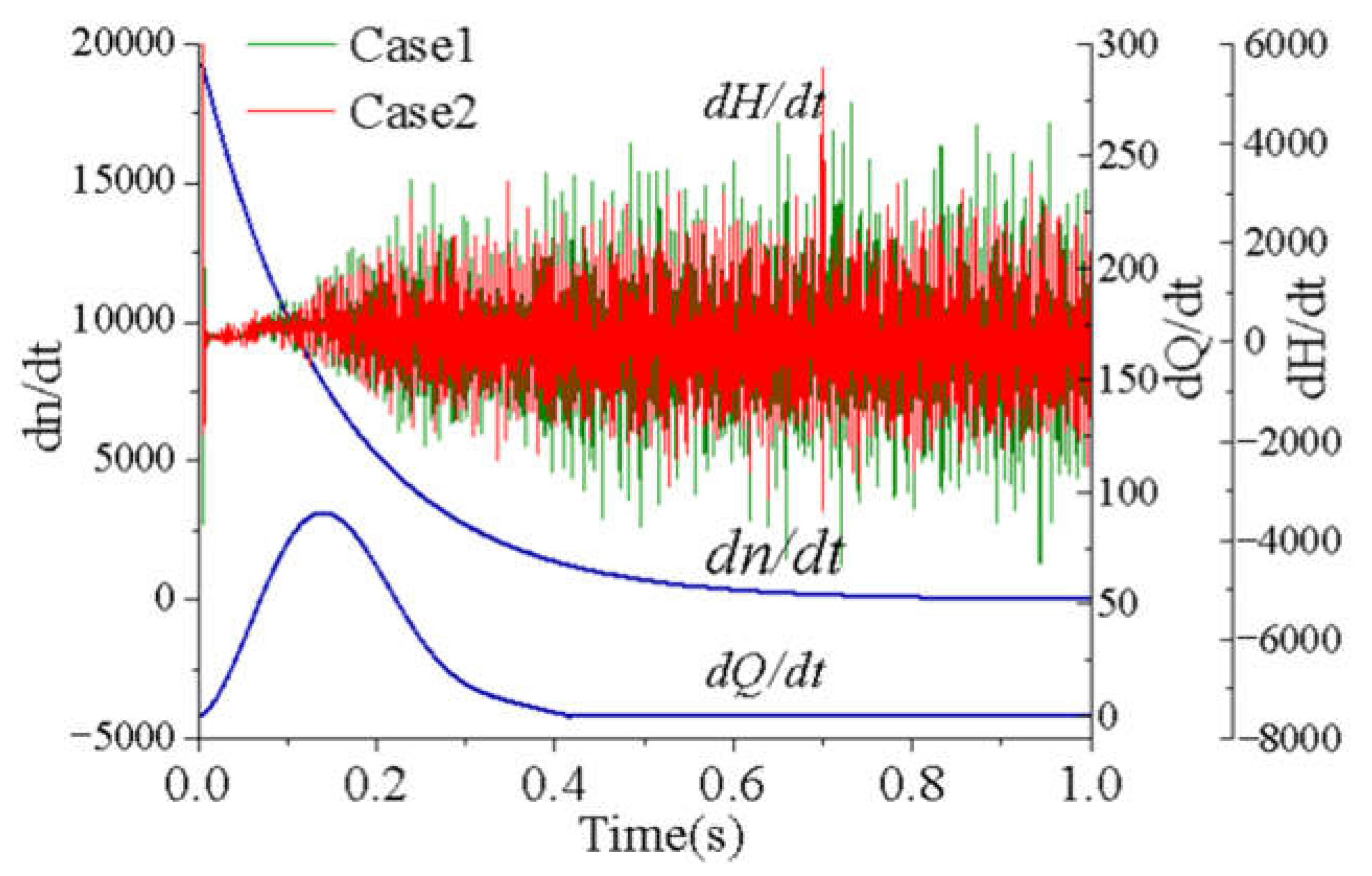
2.4. Numerical Method and Conditions
3. Results
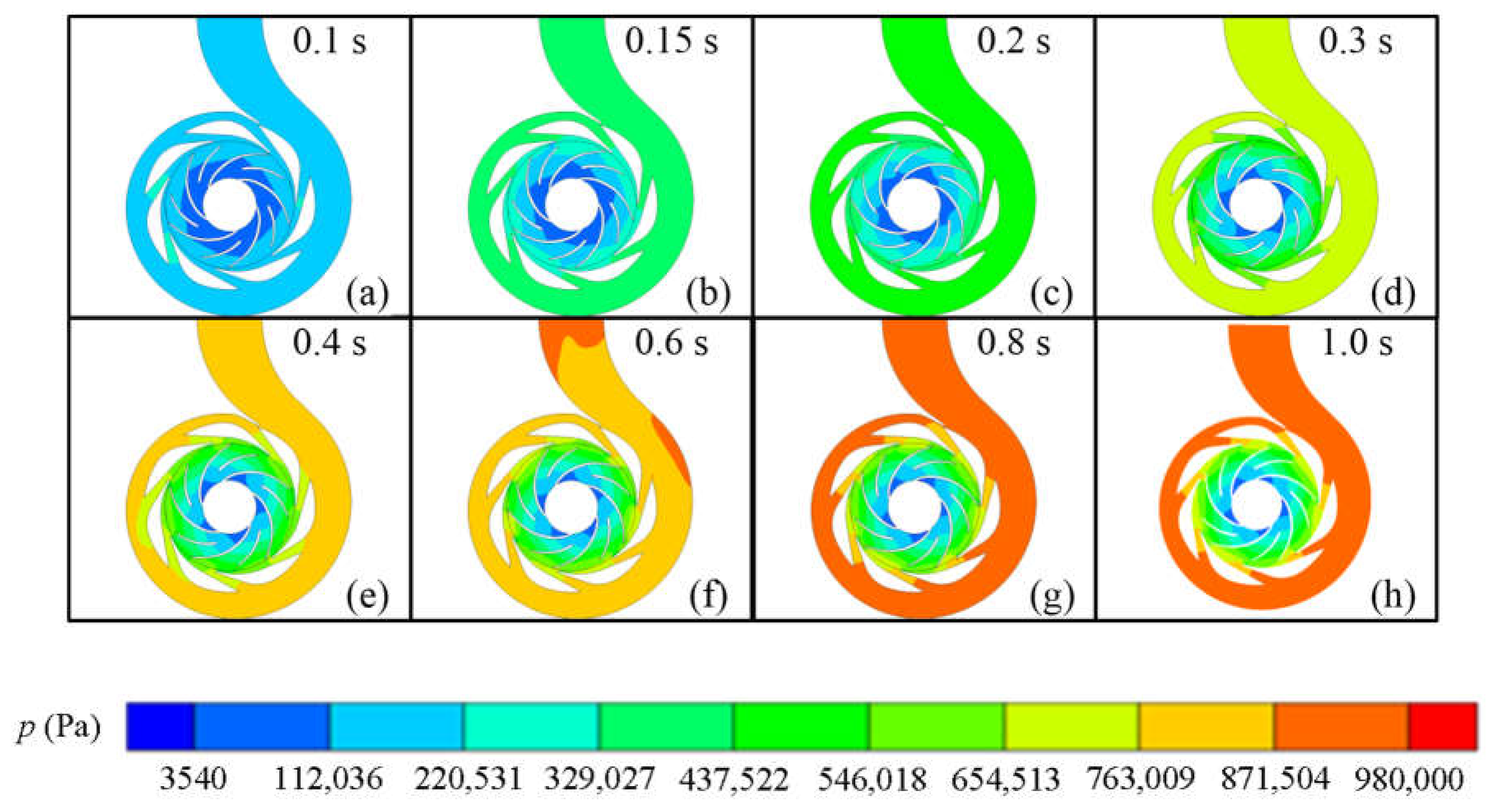
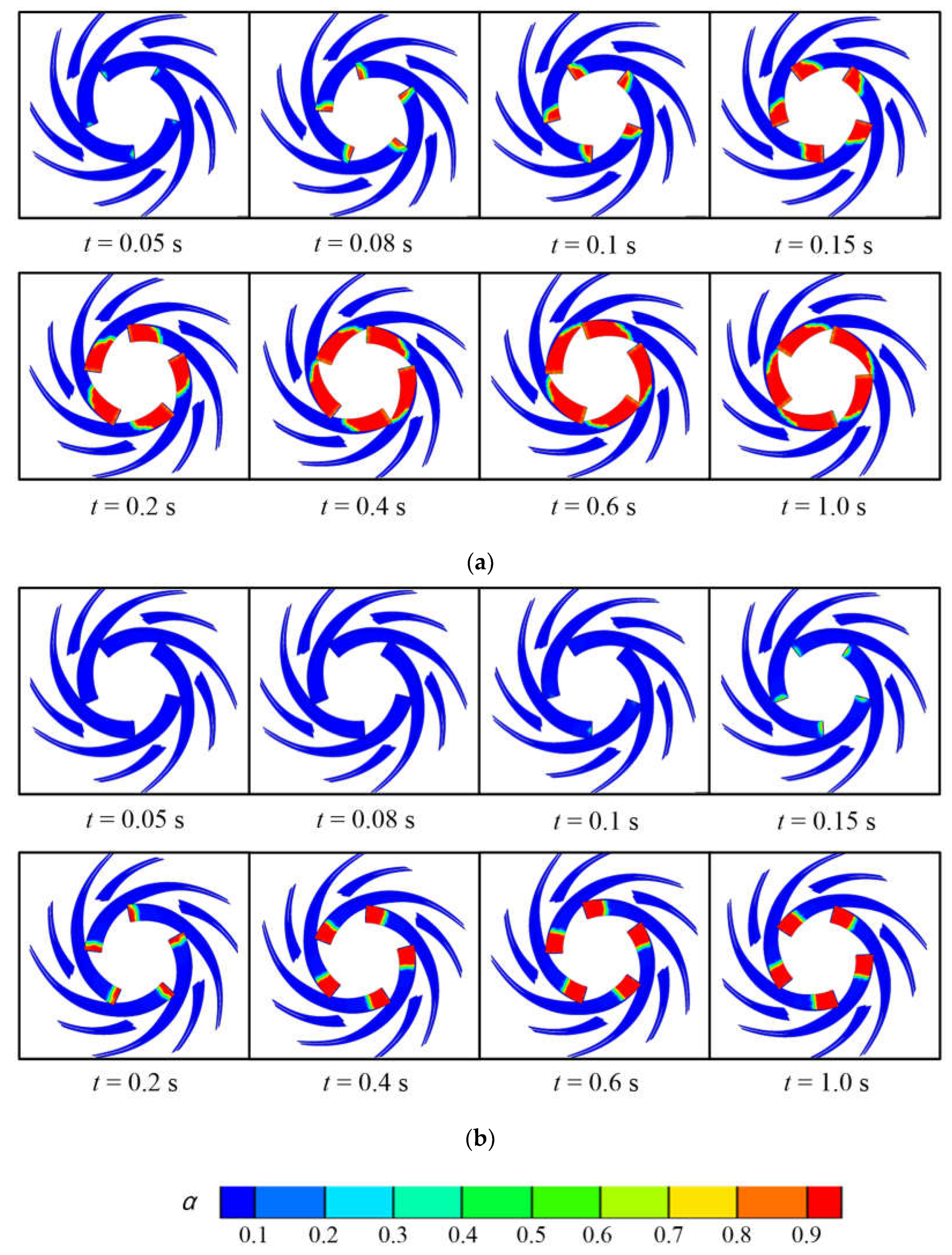
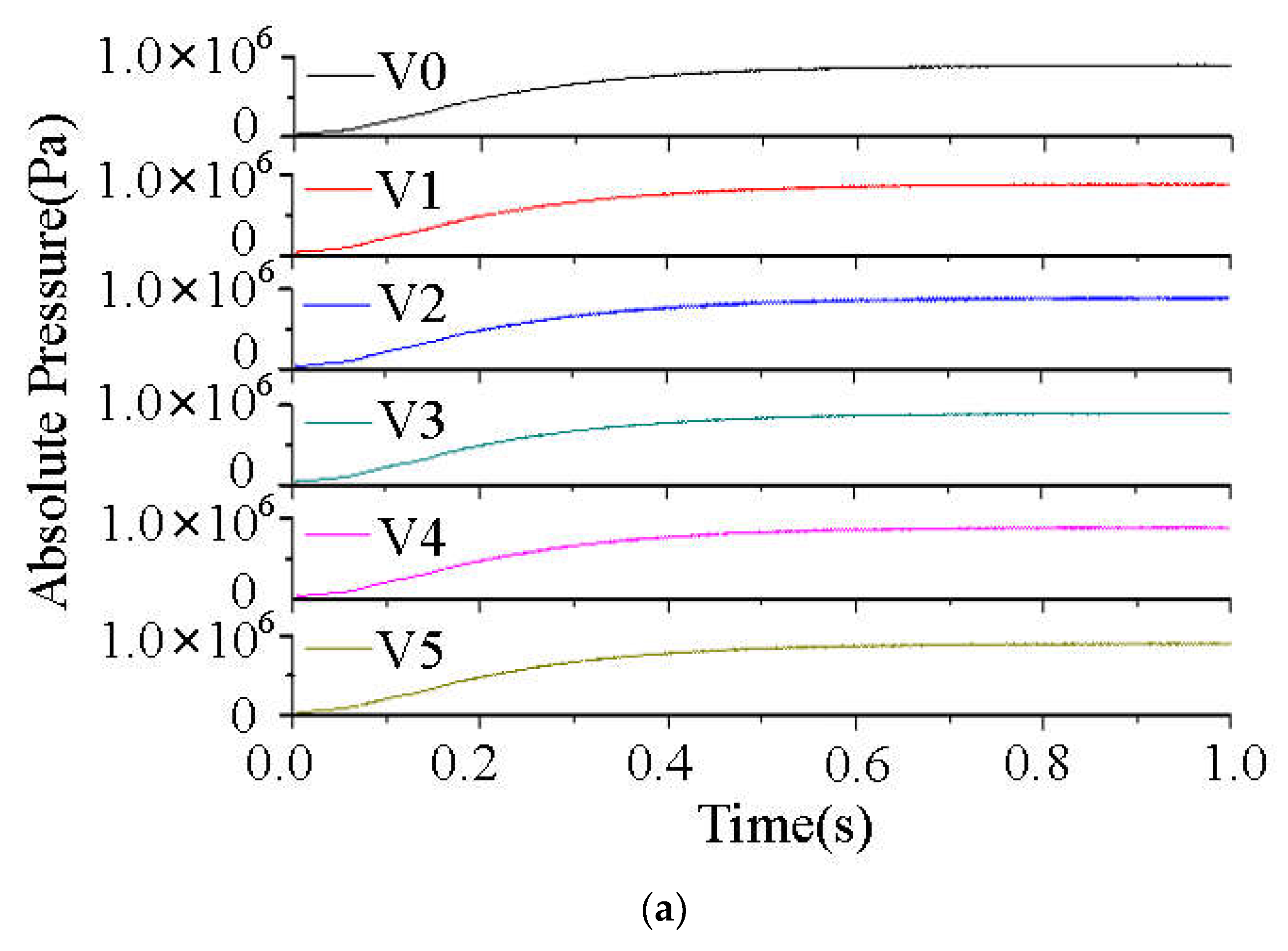
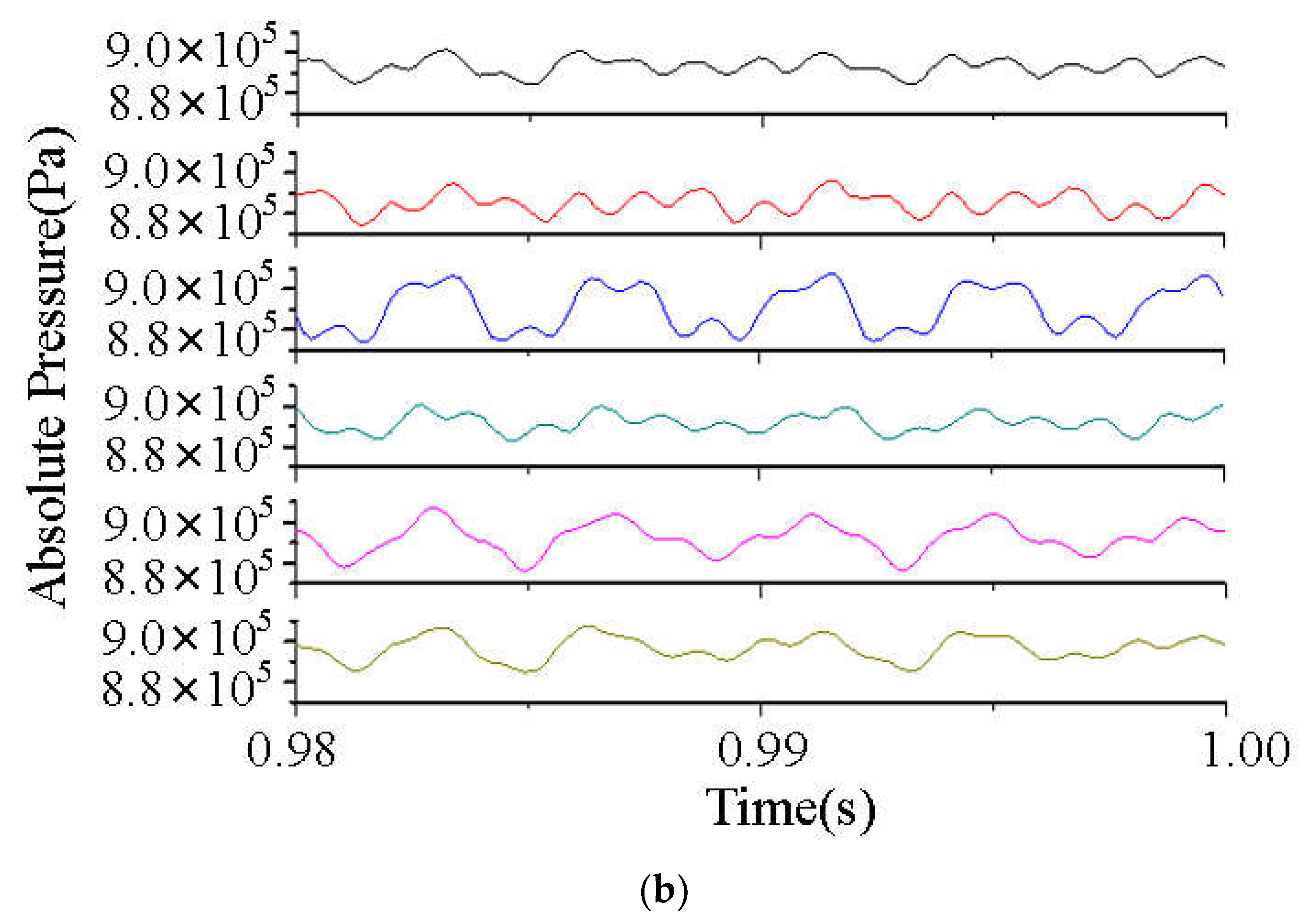
3.1. Evolution of Cavitation during the Startup Process
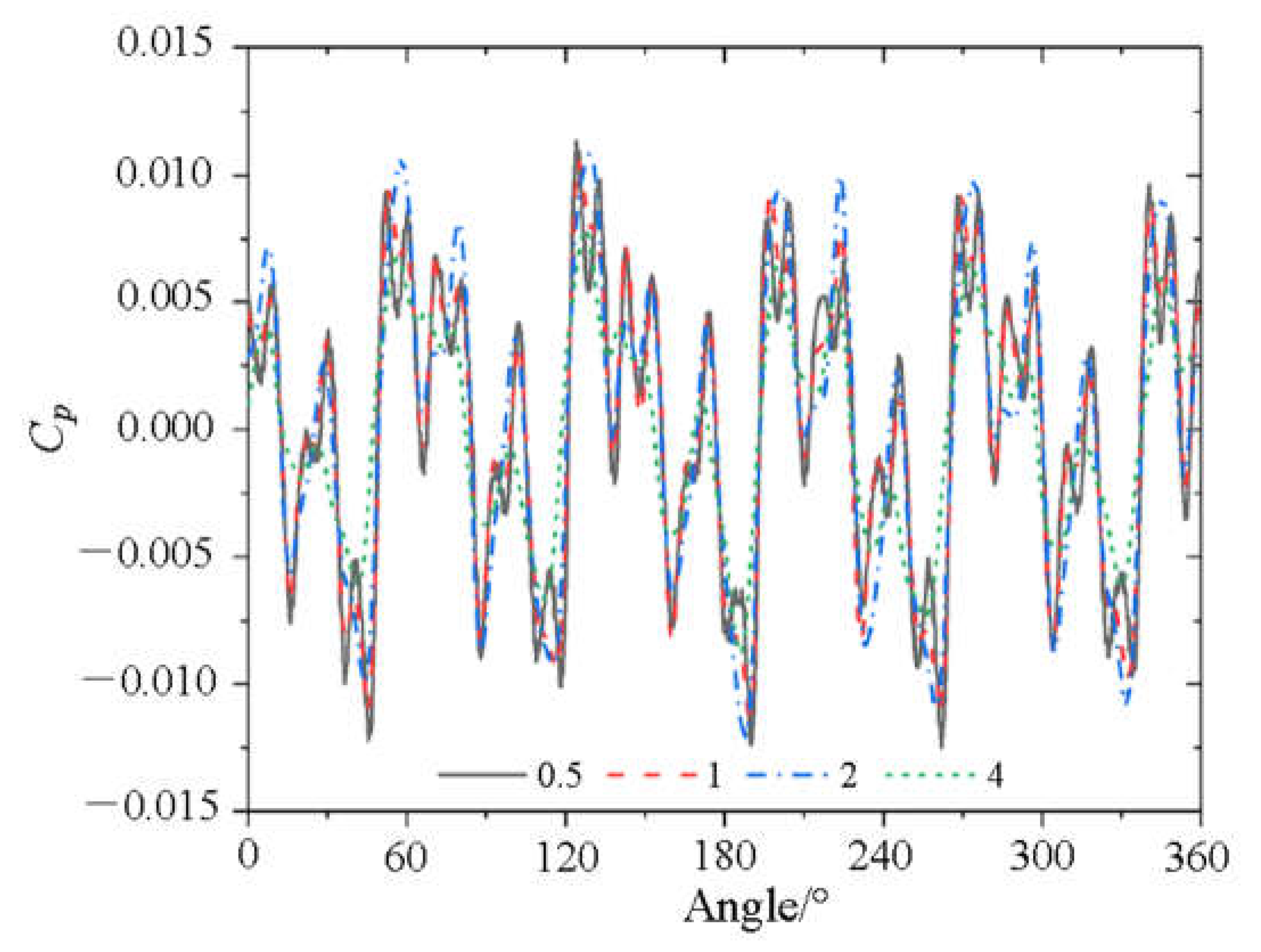
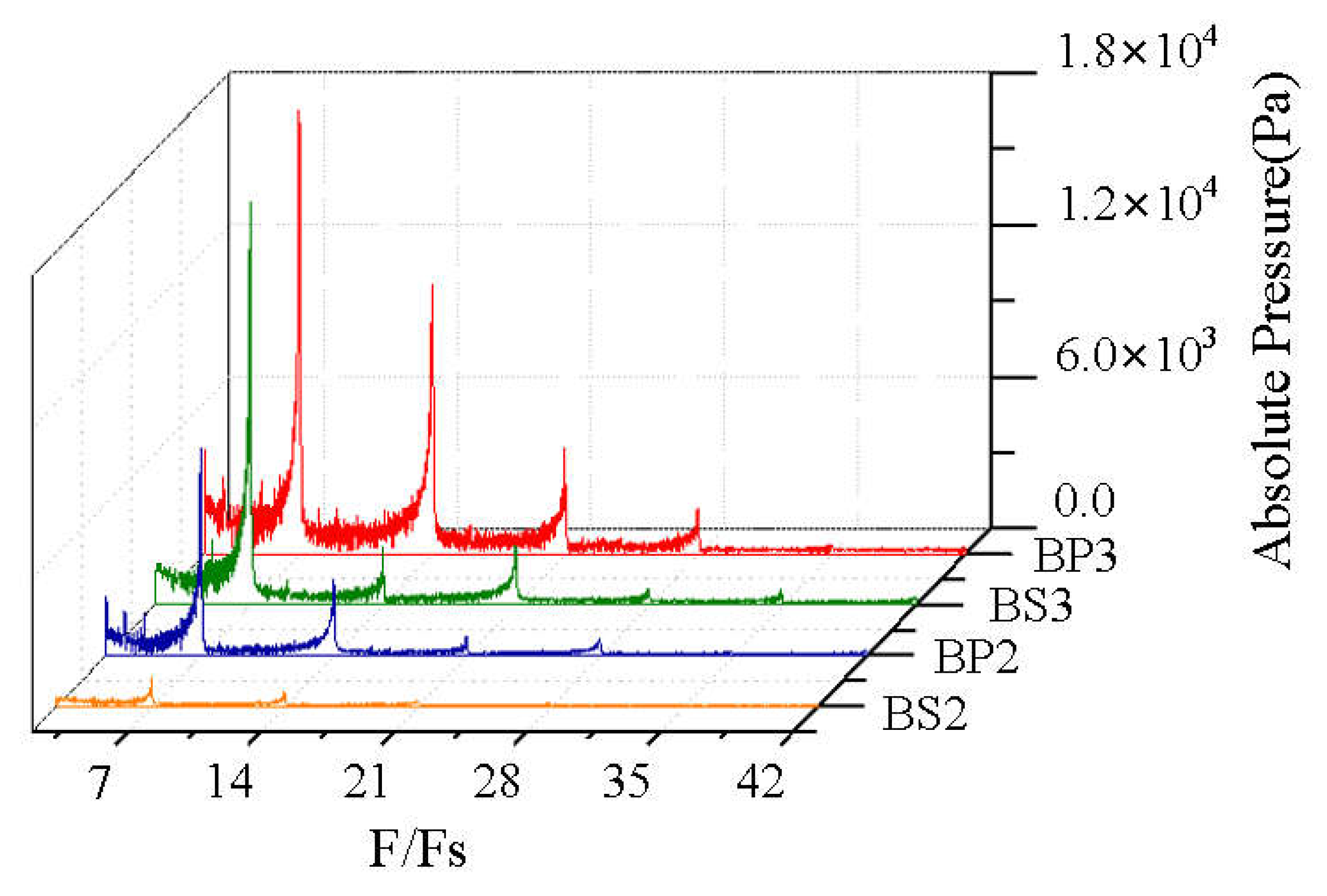
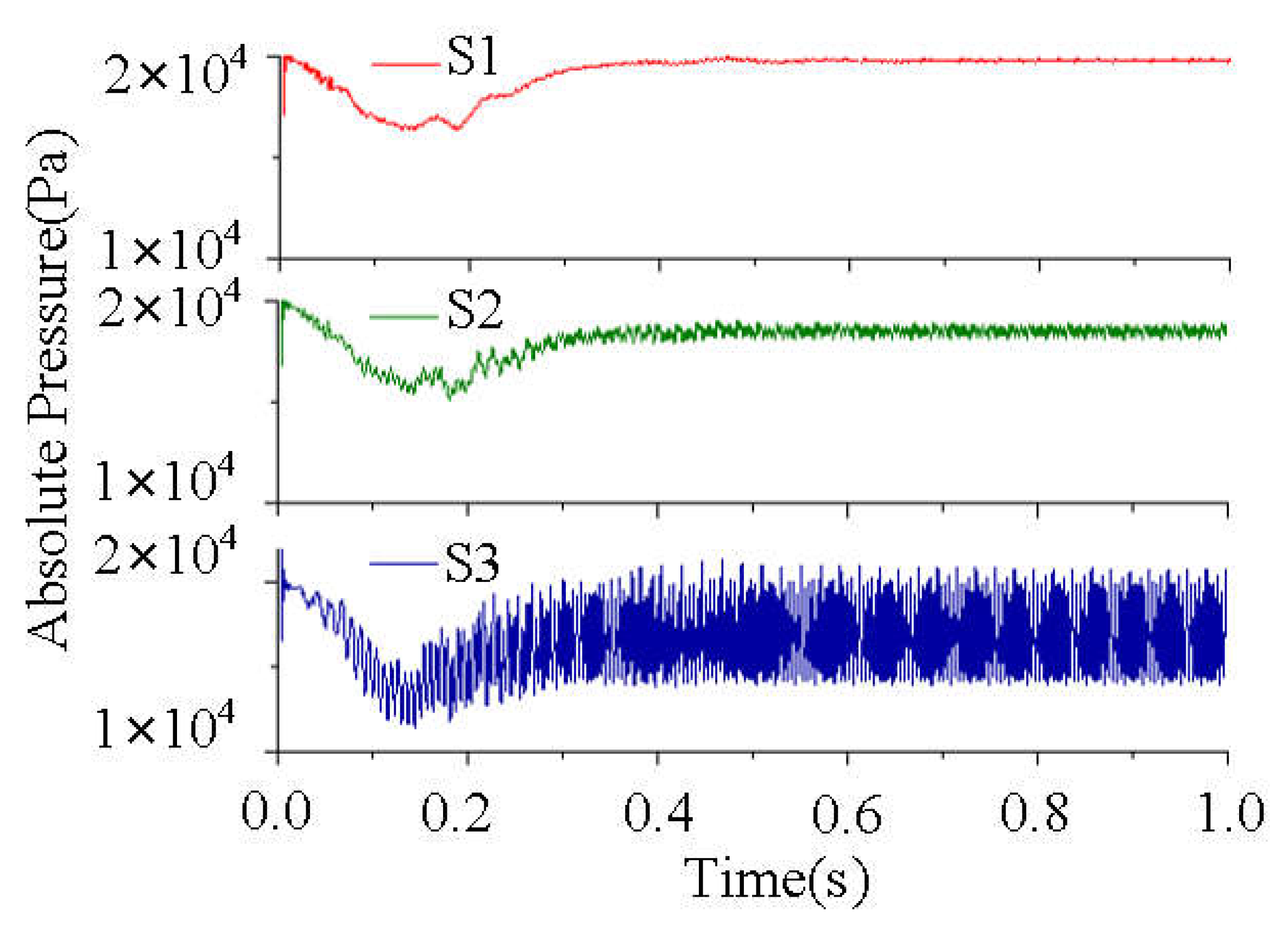
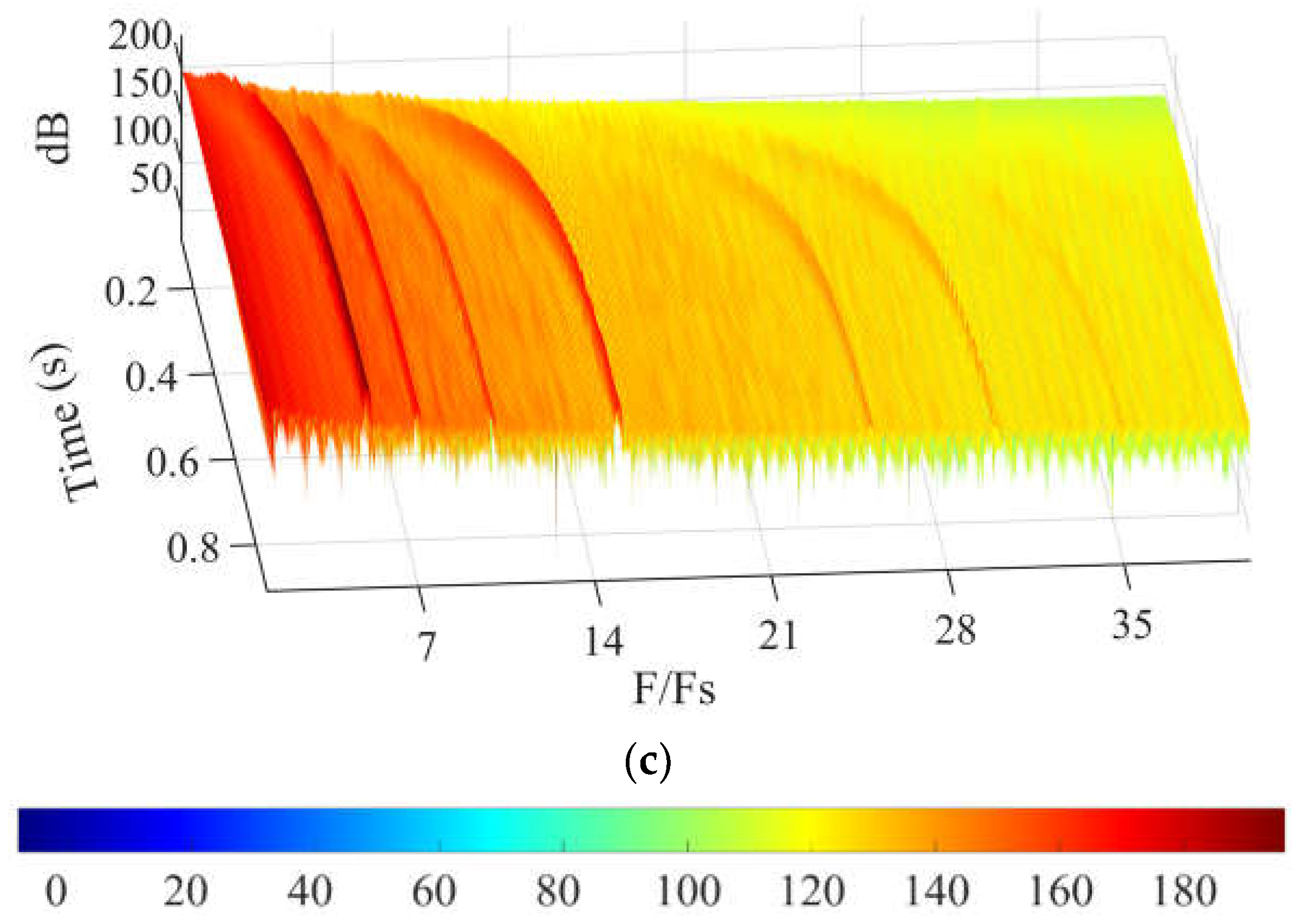
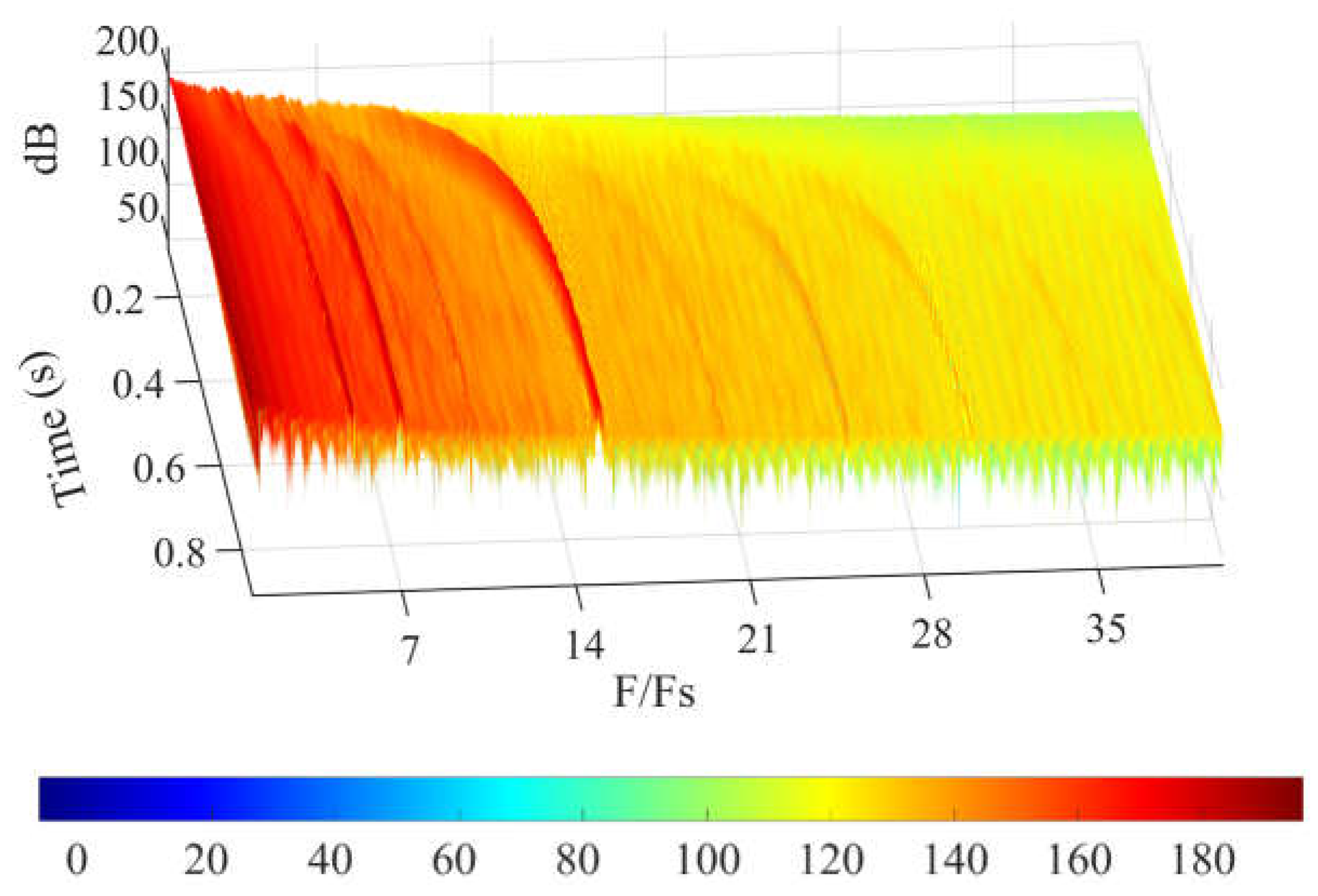
3.2. Analysis of Fluctuations in Pressure
4. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Grover, R.B.; Koranne, S.M. Analysis of pump start-up transients. Nucl. Eng. Des. 1981, 67, 137–141. [Google Scholar] [CrossRef]
- Tsukamoto, H.; Ohashi, H. Transient characteristics of a centrifugal pump during starting period. J. Fluids Eng. 1982, 104, 6–13. [Google Scholar] [CrossRef]
- Tsukamoto, H.; Matsunaga, S.; Yoneda, H.; Hata, S. Transient characteristics of a centrifugal pump during stopping period. J. Fluids Eng. 1986, 108, 392–399. [Google Scholar] [CrossRef]
- Lefebvre, P.J.; Barker, W.P. Centrifugal pump performance during transient operation. J. Fluids Eng. 1995, 117, 123–128. [Google Scholar] [CrossRef]
- Thanapandi, P.; Prasad, R. Centrifugal pump transient characteristics and analysis using the method of characteristics. Int. J. Mech. Sci. 1995, 37, 77–89. [Google Scholar] [CrossRef]
- Zhang, Y. Transient Internal Flow and Performance of Centrifugal Pumps during Startup Period. Ph.D. Thesis, Zhejiang University, Hangzhou, China, 2013. (In Chinese). [Google Scholar]
- Li, Z.; Wu, D.; Wang, L.; Huang, B. Numerical simulation of the transient flow in a centrifugal pump during starting period. J. Fluids Eng. 2010, 132, 081102. [Google Scholar] [CrossRef]
- Liu, J.; Li, Z.; Wang, L.; Jiao, L. Numerical simulation of the transient flow in a radial flow pump during stopping period. J. Fluids Eng. 2011, 133, 111101. [Google Scholar] [CrossRef]
- Wu, D.; Wu, P.; Li, Z.; Wang, L. The transient flow in a centrifugal pump during the discharge valve rapid opening process. Nucl. Eng. Des. 2010, 240, 4061–4068. [Google Scholar] [CrossRef]
- Singh, K.K.; Mahajani, S.M.; Shenoy, K.T.; Patwardhan, A.W.; Ghosh, S.K. CFD modeling of pilot-scale pump-mixer: Single-phase head and power characteristics. Chem. Eng. Sci. 2007, 62, 1308–1322. [Google Scholar] [CrossRef]
- Brennen, C.E. A review of the dynamics of cavitating pumps. J. Fluids Eng. 2013, 135, 061301. [Google Scholar] [CrossRef]
- Luo, X.; Bin, J.I.; Tsujimoto, Y. A review of cavitation in hydraulic machinery. J. Hydrodyn. Ser. B 2016, 28, 335–358. [Google Scholar] [CrossRef]
- Ji, B.; Luo, X.W.; Arndt, R.E.; Peng, X.; Wu, Y. Large eddy simulation and theoretical investigations of the transient cavitating vortical flow structure around a NACA66 hydrofoil. Int. J. Multiph. Flow 2015, 68, 121–134. [Google Scholar] [CrossRef]
- Ji, B.; Luo, X.; Wu, Y. Unsteady cavitation characteristics and alleviation of pressure fluctuations around marine propellers with different skew angles. J. Mech. Sci. Technol. 2014, 28, 1339–1348. [Google Scholar] [CrossRef]
- Peng, G.; Yang, C.; Oguma, Y.; Shimizu, S. Numerical analysis of cavitation cloud shedding in a submerged water jet. J. Hydrodyn. Ser. B 2016, 28, 986–993. [Google Scholar] [CrossRef]
- Patrik, Z.I.M.A.; Fürst, T.; SedláŘ, M.; Komárek, M.; Huzlík, R. Determination of frequencies of oscillations of cloud cavitation on a 2-D hydro-foil from high-speed camera observations. J. Hydrodyn. Ser. B 2016, 28, 369–378. [Google Scholar]
- Ji, B.; Long, Y.; Long, X.P.; Qian, Z.D.; Zhou, J.J. Large eddy simulation of turbulent attached cavitating flow with special emphasis on large scale structures of the hydrofoil wake and turbulence–cavitation interactions. J. Hydrodyn. Ser. B 2017, 29, 27–39. [Google Scholar] [CrossRef]
- Ji, B.; Luo, X.; Arndt, R.E.; Wu, Y. Numerical simulation of three dimensional cavitation shedding dynamics with special emphasis on cavitation–vortex interaction. Ocean. Eng. 2014, 87, 64–77. [Google Scholar] [CrossRef]
- Tanaka, T.; Tsukamoto, H. Transient behavior of a cavitating centrifugal pump at rapid change in operating conditions—Part 1: Transient phenomena at opening/closure of discharge valve. J. Fluids Eng. 1999, 121, 841–849. [Google Scholar] [CrossRef]
- Tanaka, T.; Tsukamoto, H. Transient behavior of a cavitating centrifugal pump at rapid change in operating conditions—Part 2: Transient phenomena at pump startup/shutdown. J. Fluids Eng. 1999, 121, 850–856. [Google Scholar] [CrossRef]
- Tanaka, T.; Tsukamoto, H. Transient behavior of a cavitating centrifugal pump at rapid change in operating conditions—Part 3: Classifications of transient phenomena. J. Fluids Eng. 1999, 121, 857–865. [Google Scholar] [CrossRef]
- Wu, D.; Wang, L.; Hao, Z.; Li, Z.; Bao, Z. Experimental study on hydrodynamic performance of a cavitating centrifugal pump during transient operation. J. Mech. Sci. Technol. 2010, 24, 575–582. [Google Scholar] [CrossRef]
- Duplaa, S.; Coutier-Delgosha, O.; Dazin, A.; Roussette, O.; Bois, G.; Caignaert, G. Experimental study of a cavitating centrifugal pump during fast startups. J. Fluids Eng. 2010, 132, 021301. [Google Scholar] [CrossRef]
- Duplaa, S.; Coutier-Delgosha, O.; Dazin, A.; Bois, G.; Caignaert, G.; Roussette, O. Cavitation inception in fast startup. In Proceedings of the12th International Symposium on Transport Phenomena and Dynamics of Rotating Machinery, Honolulu, HI, USA, 17–22 February 2008. [Google Scholar]
- Duplaa, S.; Coutier-Delgosha, O.; Dazin, A.; Bois, G.; Caignaert, G. Experimental characterization and modelling of a cavitating centrifugal pump operating in fast start-up conditions. In Proceedings of the 13th International Symposium on Transport Phenomena and Dynamics of Rotating Machinery, Honolulu, HI, USA, 22–29 April 2010. [Google Scholar]
- Meng, L. The Study of Cavitation Influence on Centrifugal Pump Startup Process. Ph.D. Thesis, China Agricultural University, Beijing, China, 2016. (In Chinese). [Google Scholar]
- Yakhot, V.S.; Orszag, S.A.; Thangam, S.; Gatski, T.B.; Speziale, C. Development of turbulence models for shear flows by a double expansion technique. Phys. Fluids A Fluid Dyn. 1992, 4, 1510–1520. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Fluent Inc. FLUENT User’s Guide; Fluent Inc.: New York, NY, USA, 2011. [Google Scholar]
- Hinze, J.O. Turbulence: An Introduction to Its Mechanism and Theory; Mcgraw-Hill Book Company: New York, NY, USA, 1959. [Google Scholar]
- Schnerr, G.H.; Sauer, J. Physical and numerical modeling of unsteady cavitation dynamics. In Proceedings of the 4th International Conference on Multiphase Flow, New Orleans, LA, USA, 27 May–1 June 2001. [Google Scholar]
- Li, Z.; Pourquie, M.; Van Terwisga, T.J.C. A numerical study of steady and unsteady cavitation on a 2D hydrofoil. J. Hydrodyn. Ser. B 2010, 22, 770–777. [Google Scholar] [CrossRef]
- Li, D.; Grekula, M.; Lindell, P. Towards numerical prediction of unsteady sheet cavitation on hydrofoils. J. Hydrodyn. Ser. B 2010, 22, 741–746. [Google Scholar] [CrossRef]
- Jiang, C.X.; Shuai, Z.J.; Zhang, X.Y.; Li, W.Y.; Li, F.C. Numerical study on evolution of axisymmetric natural supercavitation influenced by turbulent drag-reducing additives. Appl. Therm. Eng. 2016, 107, 797–803. [Google Scholar] [CrossRef]
- Jiang, C.X.; Shuai, Z.J.; Zhang, X.Y.; Li, W.Y.; Li, F.C. Numerical study on the transient behavior of water-entry supercavitating flow around a cylindrical projectile influenced by turbulent drag-reducing additives. Appl. Therm. Eng. 2016, 104, 450–460. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Jiang, C.X.; Lv, S.; Wang, X.; Yu, T.; Jian, J.; Shuai, Z.J.; Li, W.Y. Clocking effect of outlet RGVs on hydrodynamic characteristics in a centrifugal pump with an inlet inducer by CFD method. Eng. Appl. Comput. Fluid Mech. 2021, 15, 222–235. [Google Scholar] [CrossRef]
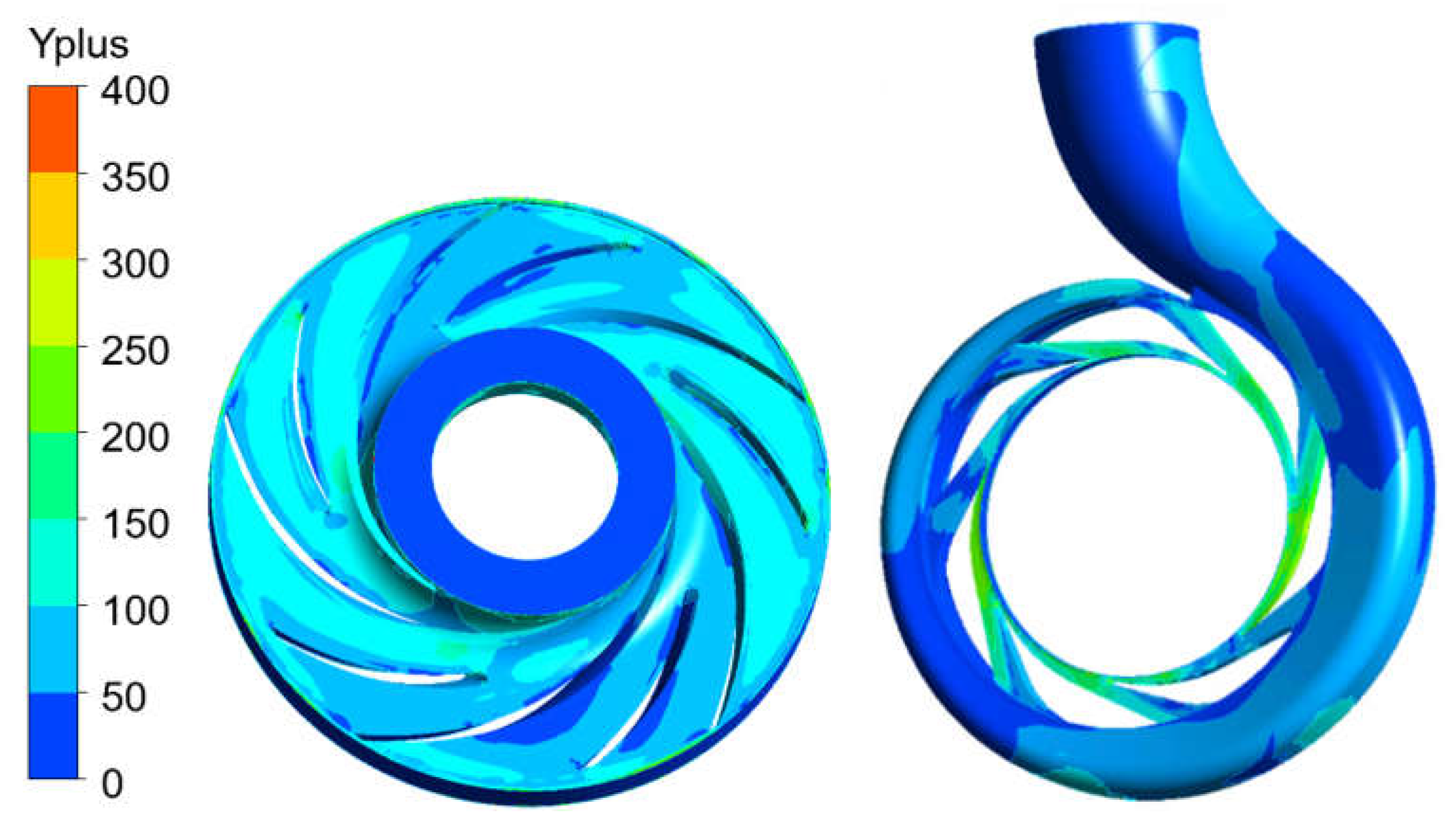









| Parameter | Pump |
|---|---|
| Impeller exit diameter (mm) | 250 |
| Impeller exit width (mm) | 13.5 |
| Number of inlet vane blades | 3 |
| Number of impeller blades | 5 |
| Number of outlet vane blades | 7 |
| Nominal flow coefficient (m3/h) | 58.96 |
| Nominal head (m) | 89 |
| Speed of rotation (r/min) | 2980 |
| Time Constant (s) | Final Mass Flow Rate (kg/s) | Inlet Pressure (kPa) | |
|---|---|---|---|
| Case1 | 0.15 | 16.4 | 20 |
| Case2 | 0.15 | 16.4 | 50 |
| Case 1 | Case 2 | |||
|---|---|---|---|---|
| H (m) | η (%) | H (m) | η (%) | |
| Experiment | 87.72 | 37.56 | 88.43 | 38.25 |
| CFD | 89.90 | 38.82 | 90.68 | 39.57 |
| Error % | 2.49 | 3.35 | 2.54 | 3.45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Cao, H.; Chen, Y.; Ni, S.; Zhao, G.; Jiang, C. Numerical Examination of the Dynamic Evolution of Fluctuations in Cavitation and Pressure in a Centrifugal Pump during Startup. Machines 2023, 11, 67. https://doi.org/10.3390/machines11010067
Liu S, Cao H, Chen Y, Ni S, Zhao G, Jiang C. Numerical Examination of the Dynamic Evolution of Fluctuations in Cavitation and Pressure in a Centrifugal Pump during Startup. Machines. 2023; 11(1):67. https://doi.org/10.3390/machines11010067
Chicago/Turabian StyleLiu, Shaofeng, Haifeng Cao, Yuxuan Chen, Shiwei Ni, Guofeng Zhao, and Chenxing Jiang. 2023. "Numerical Examination of the Dynamic Evolution of Fluctuations in Cavitation and Pressure in a Centrifugal Pump during Startup" Machines 11, no. 1: 67. https://doi.org/10.3390/machines11010067
APA StyleLiu, S., Cao, H., Chen, Y., Ni, S., Zhao, G., & Jiang, C. (2023). Numerical Examination of the Dynamic Evolution of Fluctuations in Cavitation and Pressure in a Centrifugal Pump during Startup. Machines, 11(1), 67. https://doi.org/10.3390/machines11010067








