Analysis of 3-DOF Cutting Stability of Titanium Alloy Helical Milling Based on PKM and Machining Quality Optimization
Abstract
:1. Introduction
2. Cutting Stability Analysis Based on Hybrid PKM
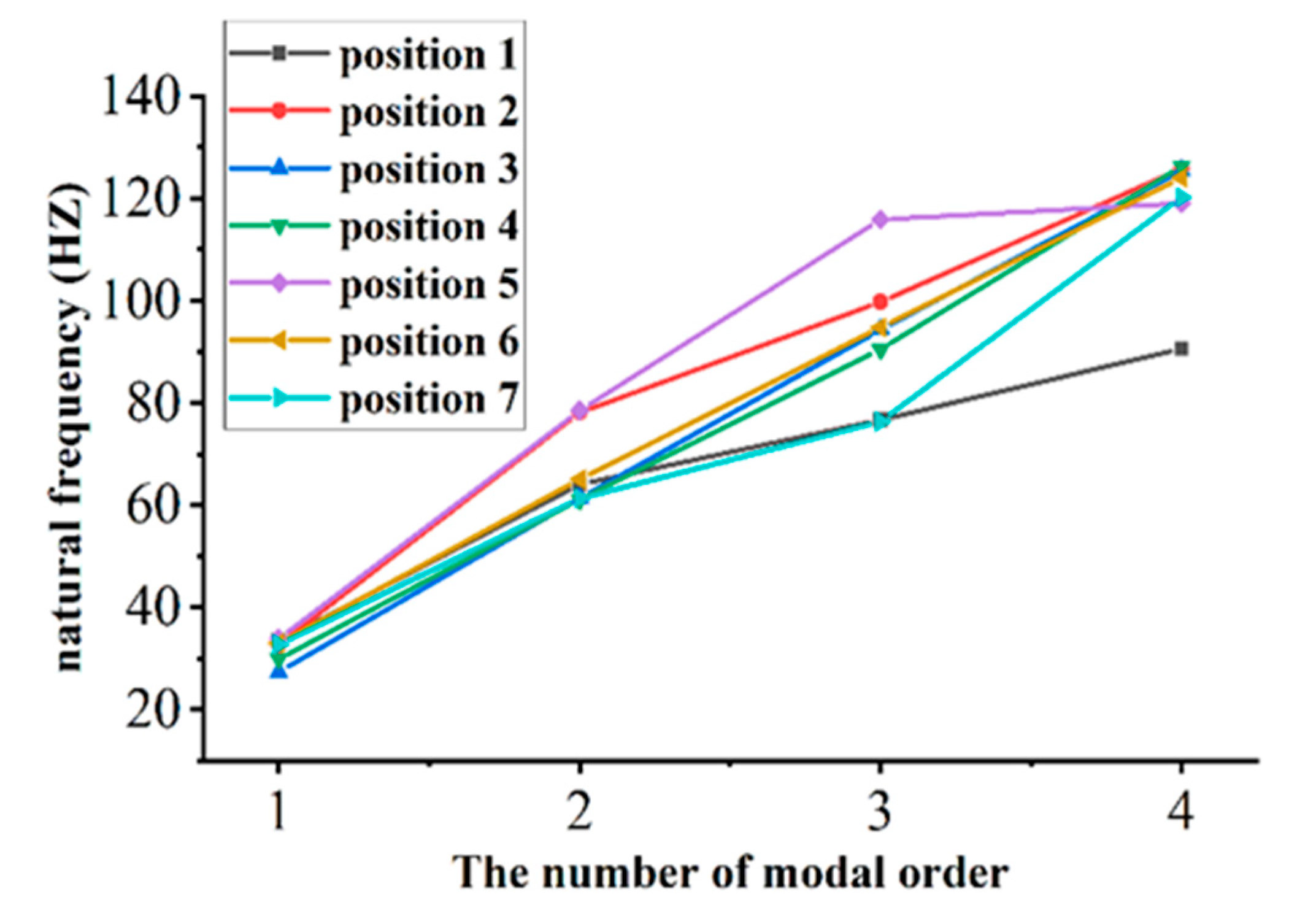
2.1. Modal Analysis of PKM
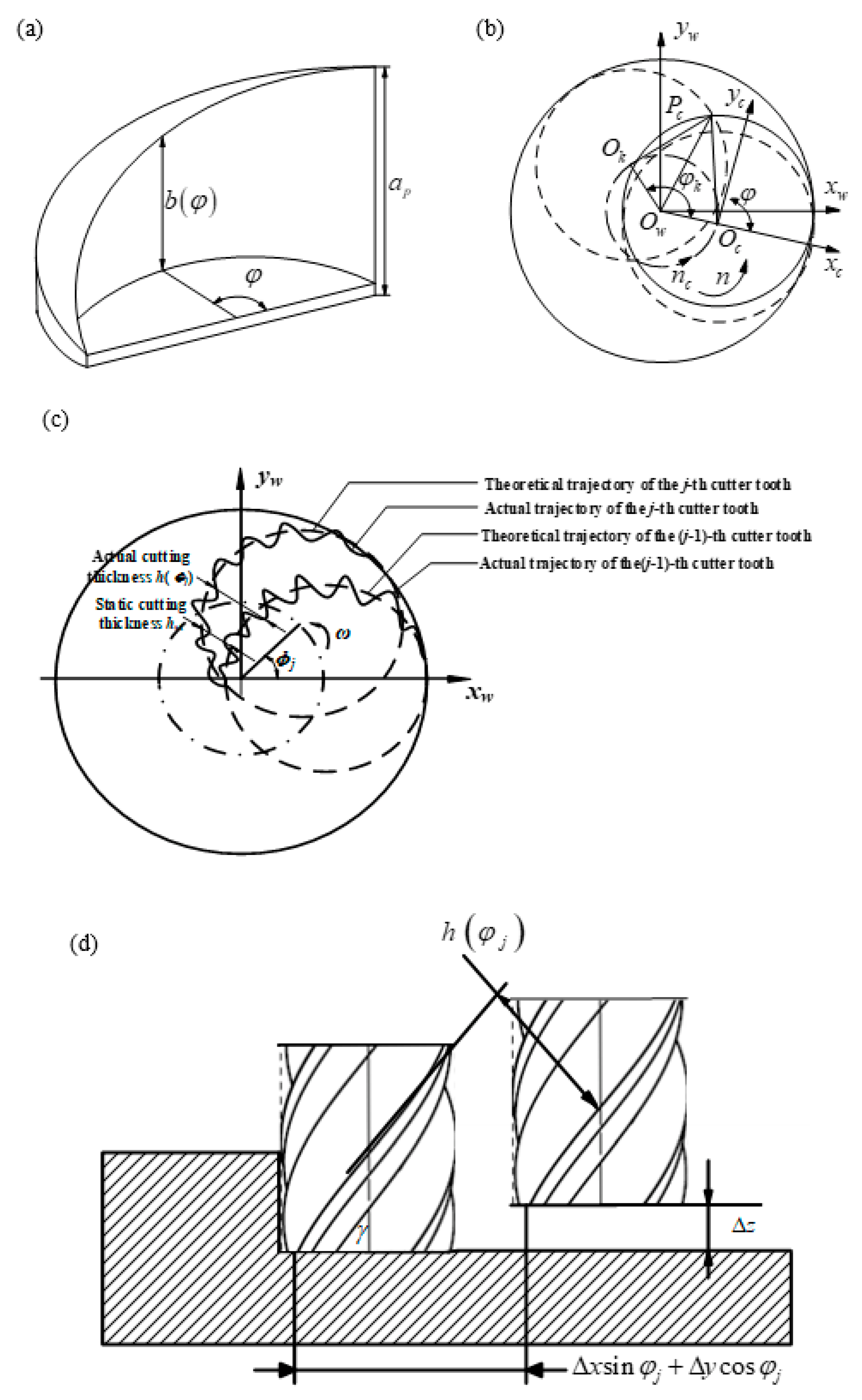
2.2. Cutting Forces Modeling and Identification of Cutting Forces Coefficients of Helical Milling Based on PKM
2.3. 3-DOF Cutting Stability Analysis of Helical Milling Based on the PKM
3. Experimental Platform Set-Up
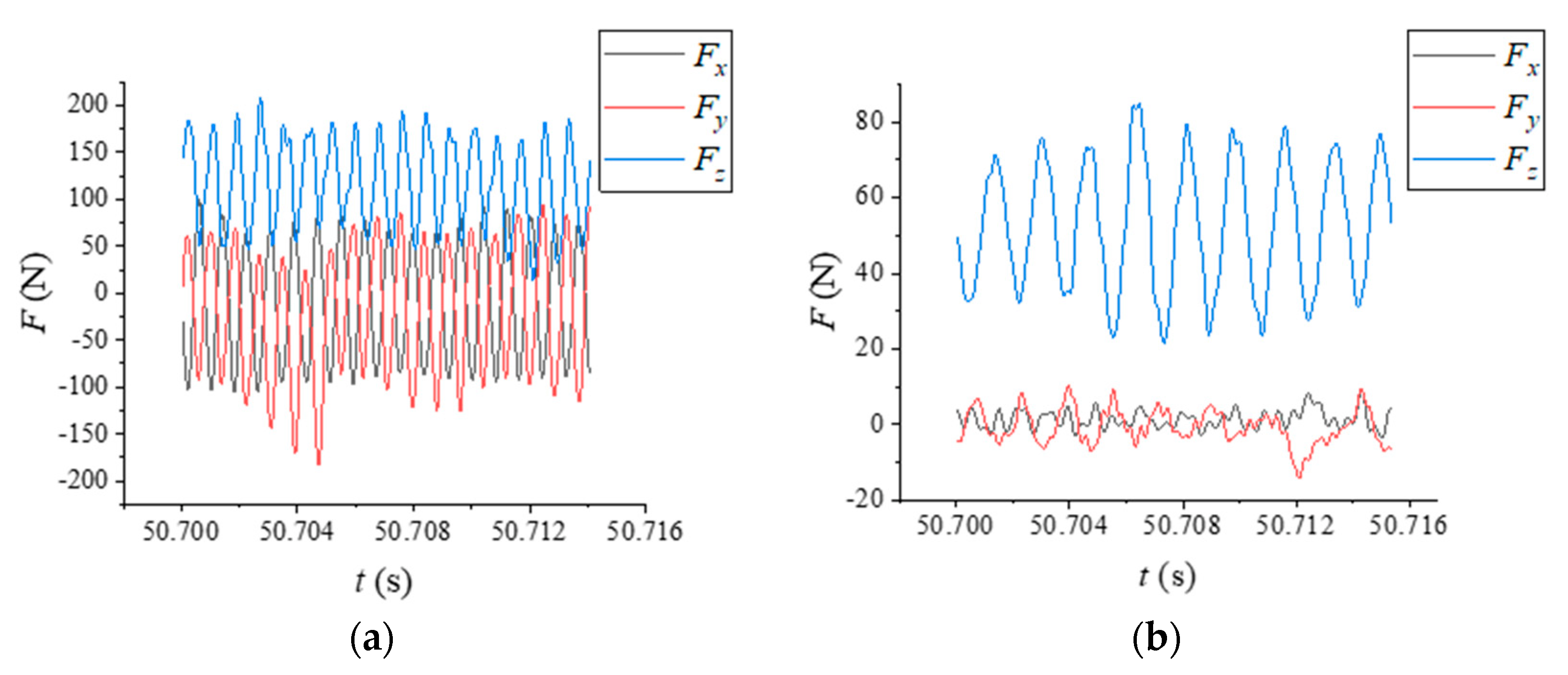
3.1. Cutting Force Coefficients Identification and Stability Verification
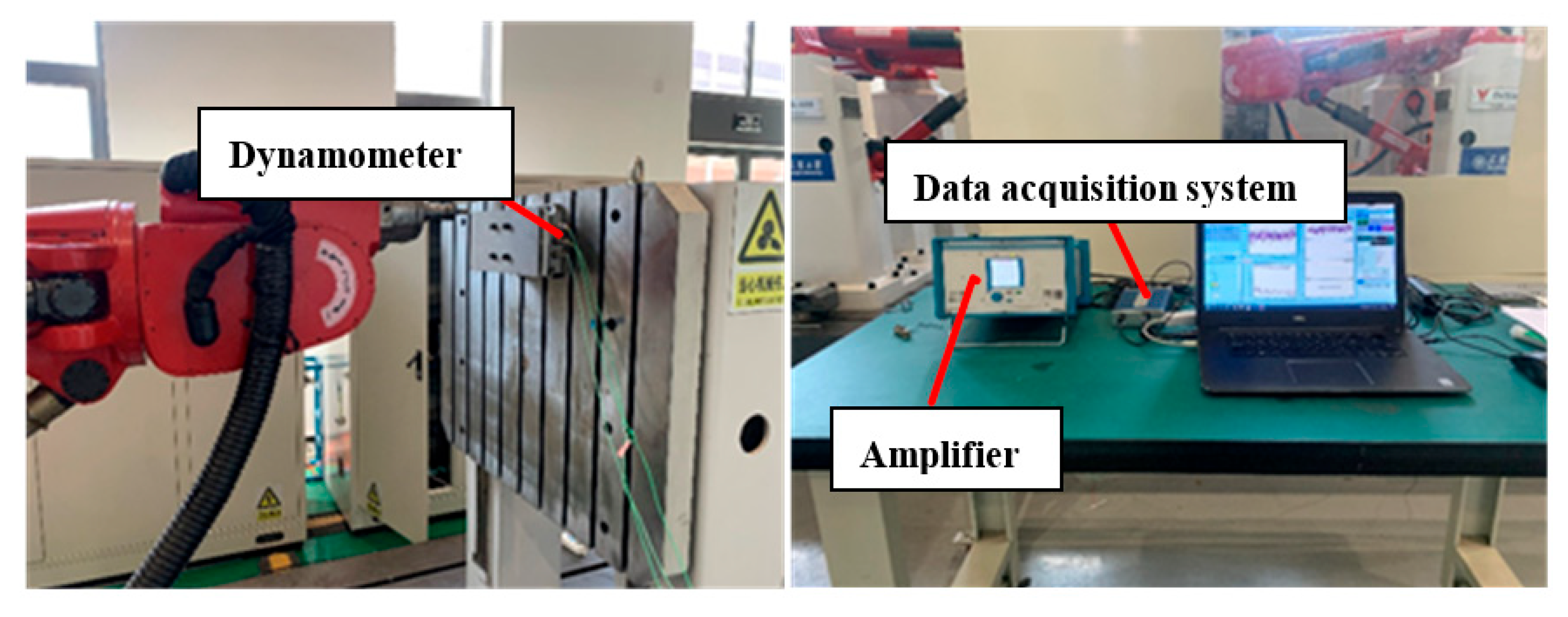
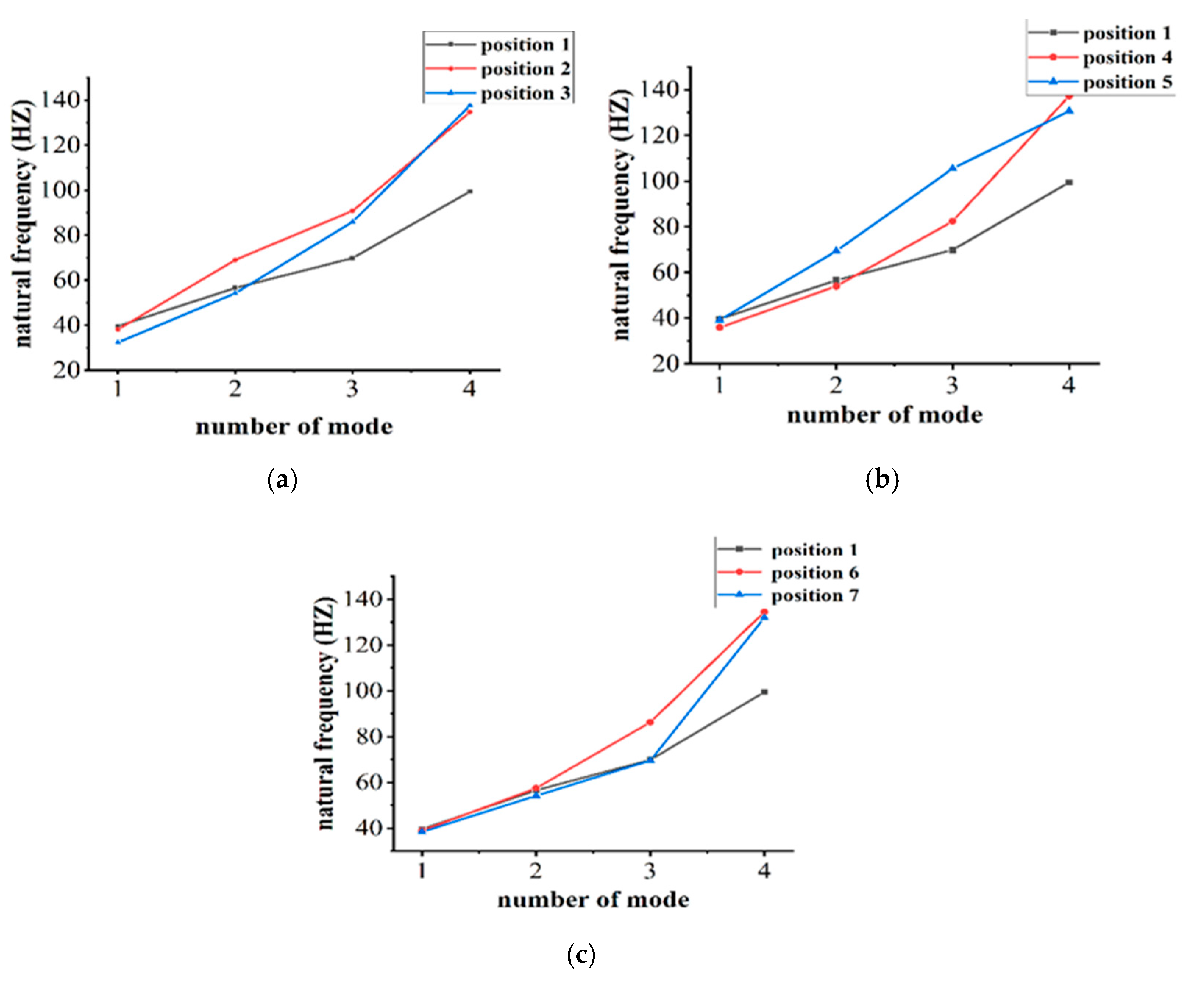
3.2. Experimental Platform Set-Up to Indentify of Modal Parameters of TriMule
4. Results and Discussion
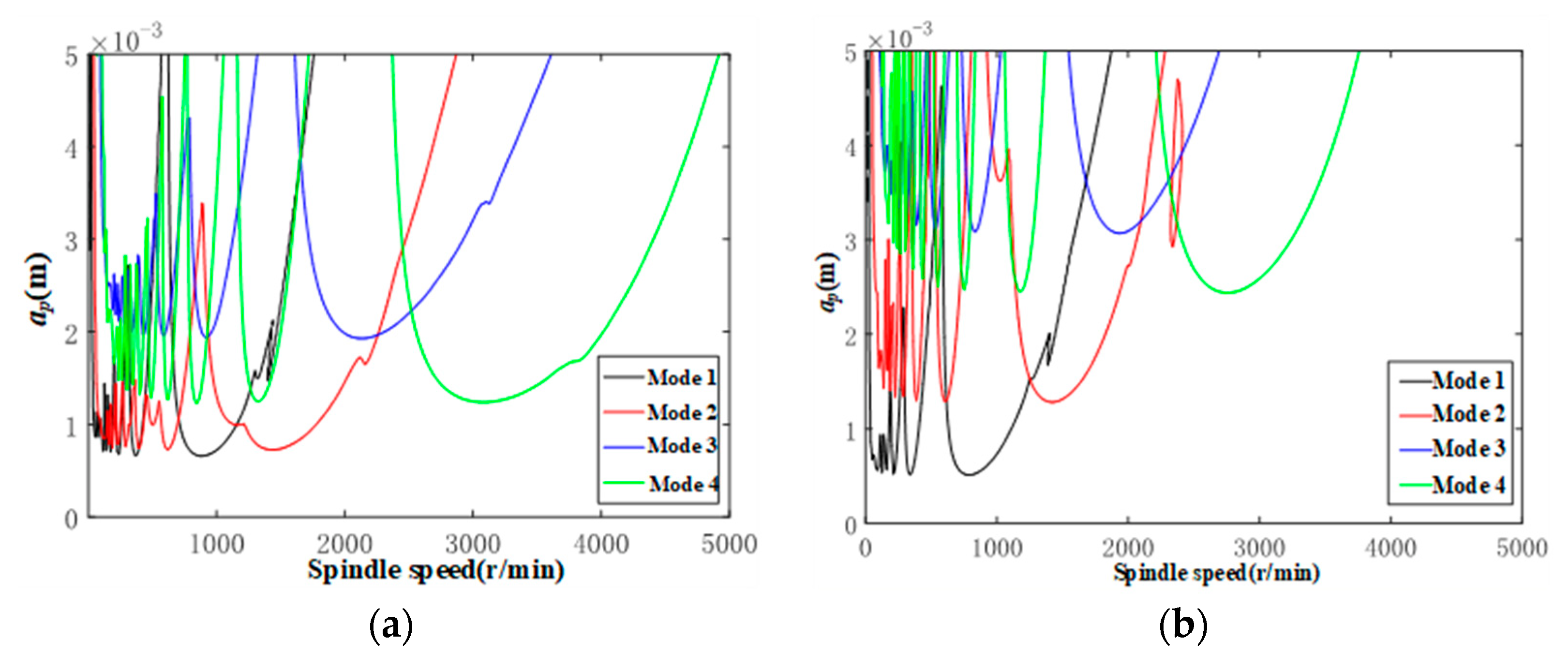
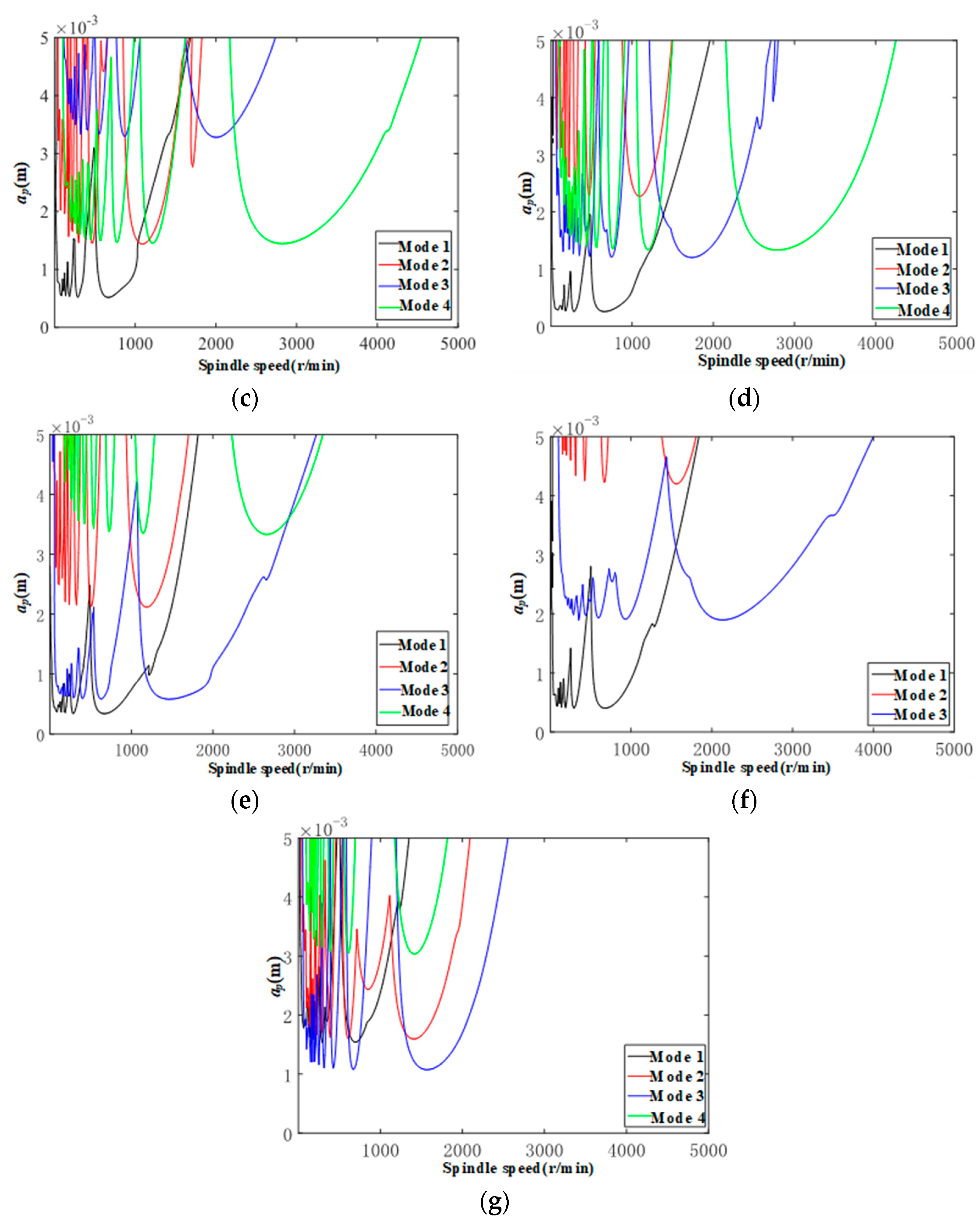
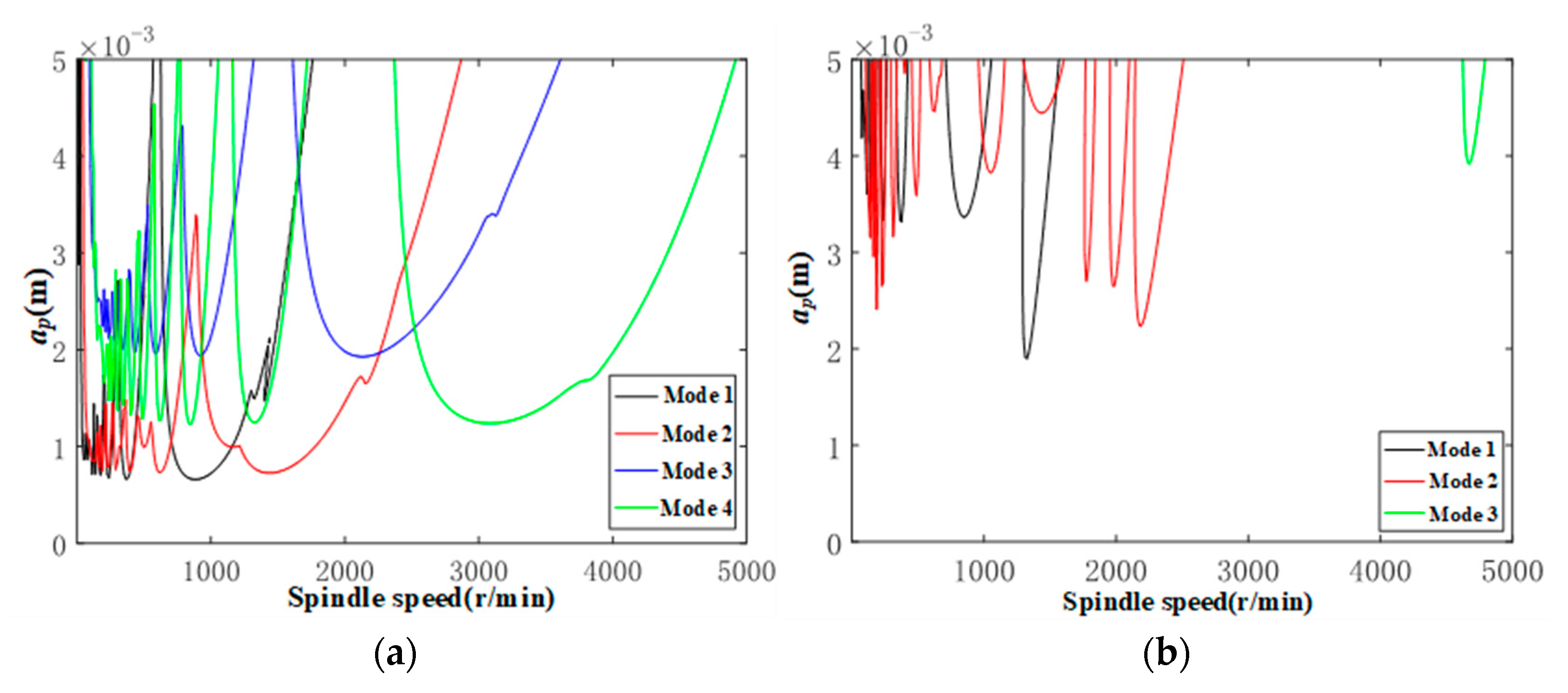
4.1. Cutting Stability Lobes of Titanium Alloy Helical Milling Based TriMule
4.2. Validation of Cutting Stability Lobes of TriMule Based Titanium Alloy Helical Milling
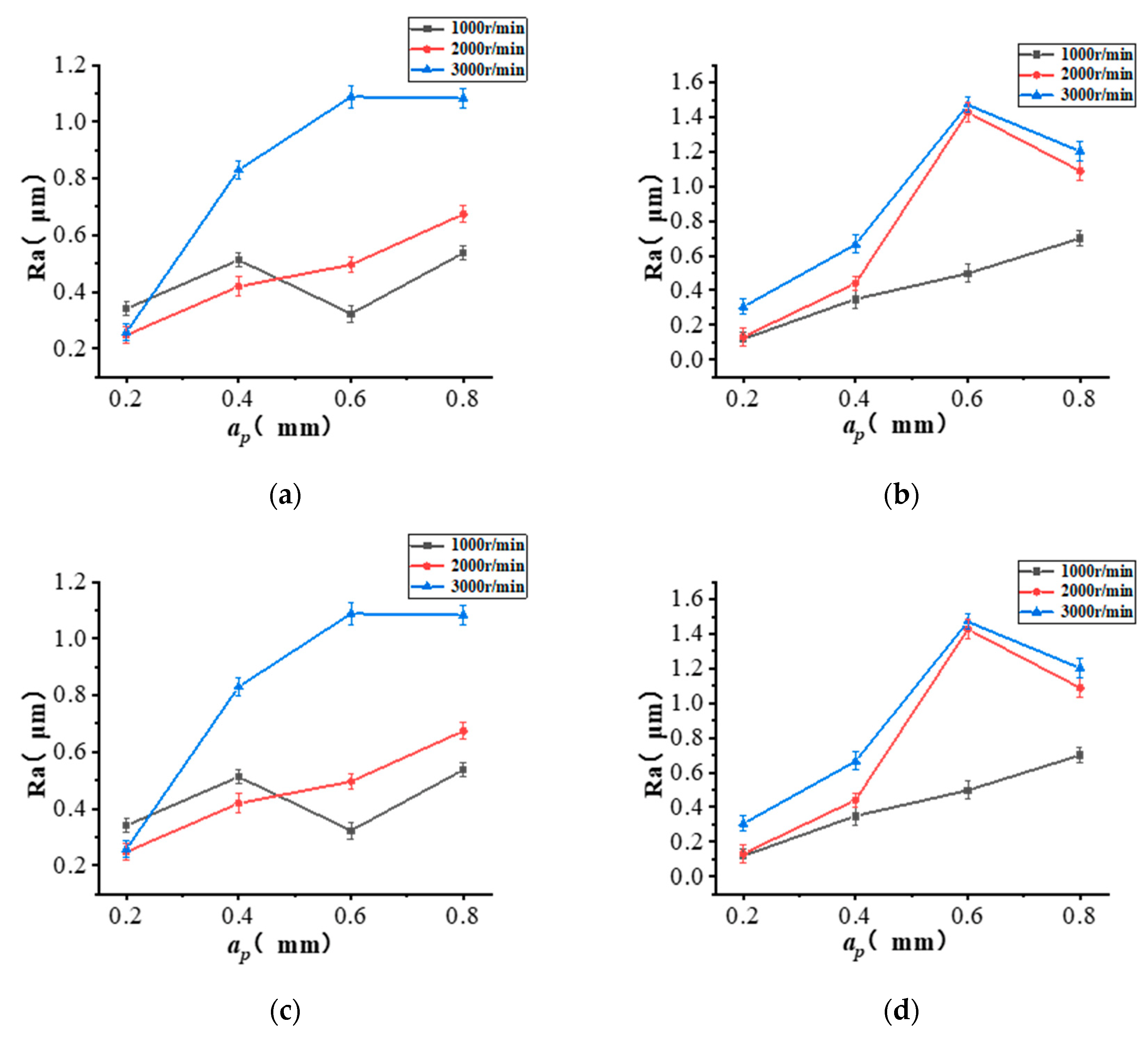
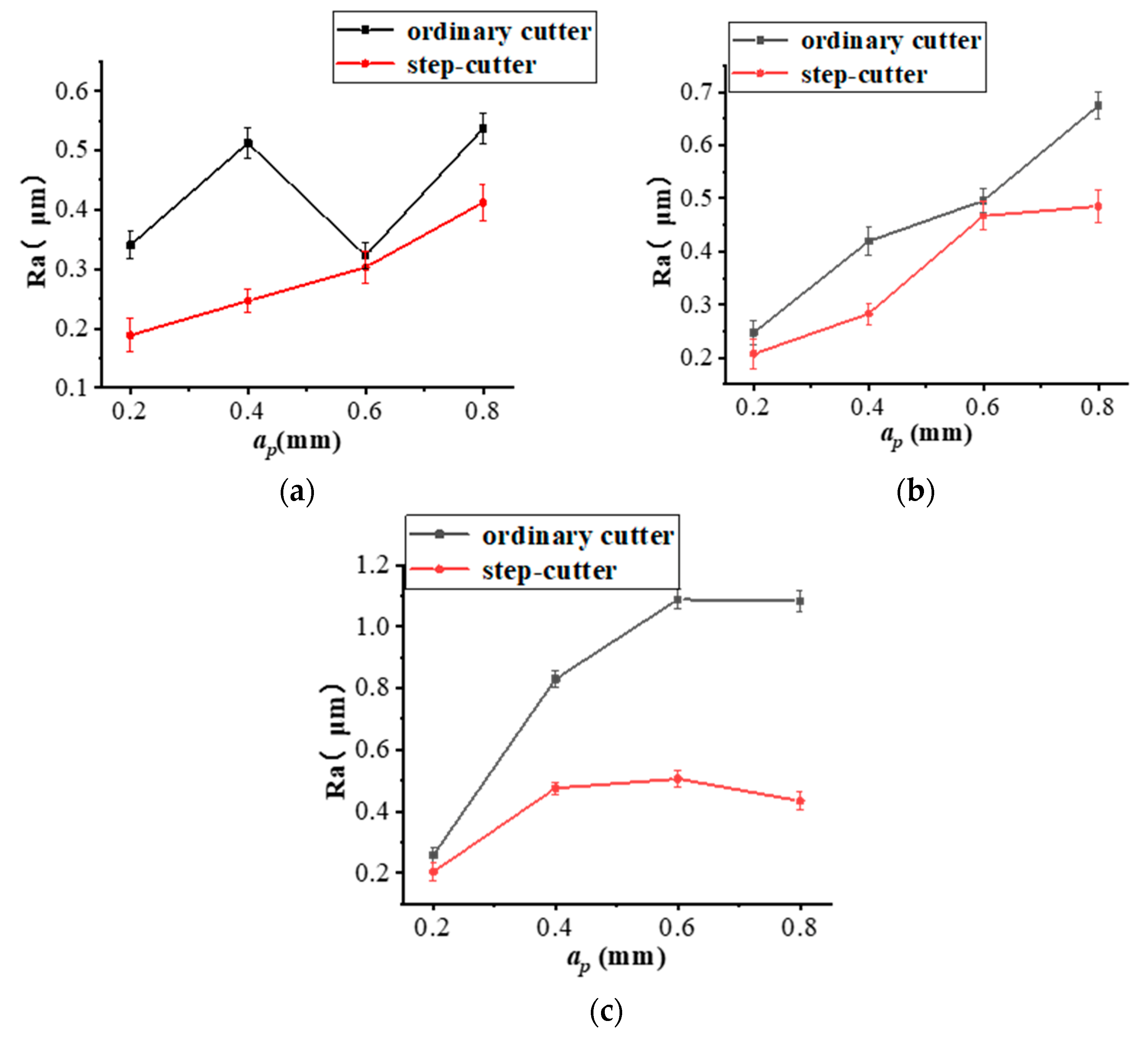
4.3. Optimization of Cutting Stability Lobes of Titanium Alloy Helical Milling Based TriMule
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mohd Yusuf, S.; Cutler, S.; Gao, N. Review: The Impact of Metal Additive Manufacturing on the Aerospace Industry. Metals 2019, 9, 1286. [Google Scholar] [CrossRef] [Green Version]
- Pereira, R.B.D.; Brandão, L.C.; de Paiva, A.P.; Ferreira, J.R.; Davim, J.P. A review of helical milling process. Int. J. Mach. Tools Manuf. 2017, 120, 27–48. [Google Scholar] [CrossRef]
- Altintas, Y.; Brecher, C.; Weck, M.; Witt, S. Virtual Machine Tool. CIRP Ann. 2005, 54, 115–138. [Google Scholar] [CrossRef]
- Piras, G.; Cleghorn, W.L.; Mills, J.K. Dynamic finite-element analysis of a planar high-speed, high-precision parallel manipulator with flexible links. Mech. Mach. Theory 2005, 40, 849–862. [Google Scholar] [CrossRef]
- Luo, H.-w.; Wang, H.; Zhang, J.; Li, Q. Rapid Evaluation for Position-Dependent Dynamics of a 3-DOF PKM Module. Adv. Mech. Eng. 2015, 6, 326–334. [Google Scholar] [CrossRef]
- Law, M.; Ihlenfeldt, S.; Wabner, M.; Altintas, Y.; Neugebauer, R. Position-dependent dynamics and stability of serial-parallel kinematic machines. CIRP Ann. 2013, 62, 375–378. [Google Scholar] [CrossRef]
- Mousavi, S.; Gagnol, V.; Bouzgarrou, B.C.; Ray, P. Dynamic modeling and stability prediction in robotic machining. Int. J. Adv. Manuf. Technol. 2016, 88, 3053–3065. [Google Scholar] [CrossRef]
- Li, J.; Li, B.; Shen, N.; Qian, H.; Guo, Z. Effect of the cutter path and the workpiece clamping position on the stability of the robotic milling system. Int. J. Adv. Manuf. Technol. 2016, 89, 2919–2933. [Google Scholar] [CrossRef]
- Shi, M.; Qin, X.; Li, H.; Shang, S.; Jin, Y.; Huang, T. Cutting force and chatter stability analysis for PKM-based helical milling operation. Int. J. Adv. Manuf. Technol. 2020, 111, 3207–3224. [Google Scholar] [CrossRef]
- Cao, H.; Zhou, K.; Chen, X. Stability-based selection of cutting parameters to increase material removal rate in high-speed machining process. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2015, 230, 227–240. [Google Scholar] [CrossRef]
- Mejri, S.; Gagnol, V.; Le, T.-P.; Sabourin, L.; Ray, P.; Paultre, P. Dynamic characterization of machining robot and stability analysis. Int. J. Adv. Manuf. Technol. 2015, 82, 351–359. [Google Scholar] [CrossRef]
- Polini, W.; Turchetta, S. Cutting force, tool life and surface integrity in milling of titanium alloy Ti-6Al-4V with coated carbide tools. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2014, 230, 694–700. [Google Scholar] [CrossRef]
- Zain, A.M.; Haron, H.; Sharif, S. Application of GA to optimize cutting conditions for minimizing surface roughness in end milling machining process. Expert Syst. Appl. 2010, 37, 4650–4659. [Google Scholar] [CrossRef]
- Muñoz-Escalona, P.; Maropoulos, P.G. A geometrical model for surface roughness prediction when face milling Al 7075-T7351 with square insert tools. J. Manuf. Syst. 2015, 36, 216–223. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Sun, Y.; Geng, Y.; Shan, D. Experimental research of milling force and surface quality for TC4 titanium alloy of micro-milling. Int. J. Adv. Manuf. Technol. 2015, 79, 705–716. [Google Scholar] [CrossRef]
- Zhang, R.; Li, A.; Song, X. Surface quality adjustment and controlling mechanism of machined surface layer in two-step milling of titanium alloy. Int. J. Adv. Manuf. Technol. 2022, 119, 2691–2707. [Google Scholar] [CrossRef]
- Chen, C.; Peng, F.; Yan, R.; Tang, X.; Li, Y.; Fan, Z. Rapid prediction of posture-dependent FRF of the tool tip in robotic milling. Robot. Comput.-Integr. Manuf. 2020, 64. [Google Scholar] [CrossRef]
- Huang, T.; Dong, C.; Liu, H. Five-Degree-of-Freedom Parallel Robot with Multi-Shaft Rotary Brackets. Patent No. WO/2017/005015, 1 December 2017. [Google Scholar]
- Wang, H.; Qin, X.; Ren, C.; Wang, Q. Prediction of cutting forces in helical milling process. Int. J. Adv. Manuf. Technol. 2011, 58, 849–859. [Google Scholar] [CrossRef]
- Guimu, Z.; Chao, Y.; Chen, S.R.; Libao, A. Experimental study on the milling of thin parts of titanium alloy (TC4). J. Mater. Process. Technol. 2003, 138, 489–493. [Google Scholar] [CrossRef]
- Kao, Y.-C.; Nguyen, N.-T.; Chen, M.-S.; Su, S.-T. A prediction method of cutting force coefficients with helix angle of flat-end cutter and its application in a virtual three-axis milling simulation system. Int. J. Adv. Manuf. Technol. 2014, 77, 1793–1809. [Google Scholar] [CrossRef]
- Peng, C.; Wang, L.; Liao, T.W. A new method for the prediction of chatter stability lobes based on dynamic cutting force simulation model and support vector machine. J. Sound Vib. 2015, 354, 118–131. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, Q.; Zhang, B. Three-dimensional chatter stability prediction of milling based on the linear and exponential cutting force model. Int. J. Adv. Manuf. Technol. 2014, 72, 1175–1185. [Google Scholar] [CrossRef]
- Yi, J.; Chen, K.; Xu, Y. Microstructure, Properties, and Titanium Cutting Performance of AlTiN–Cu and AlTiN–Ni Coatings. Coatings 2019, 9, 818. [Google Scholar] [CrossRef] [Green Version]

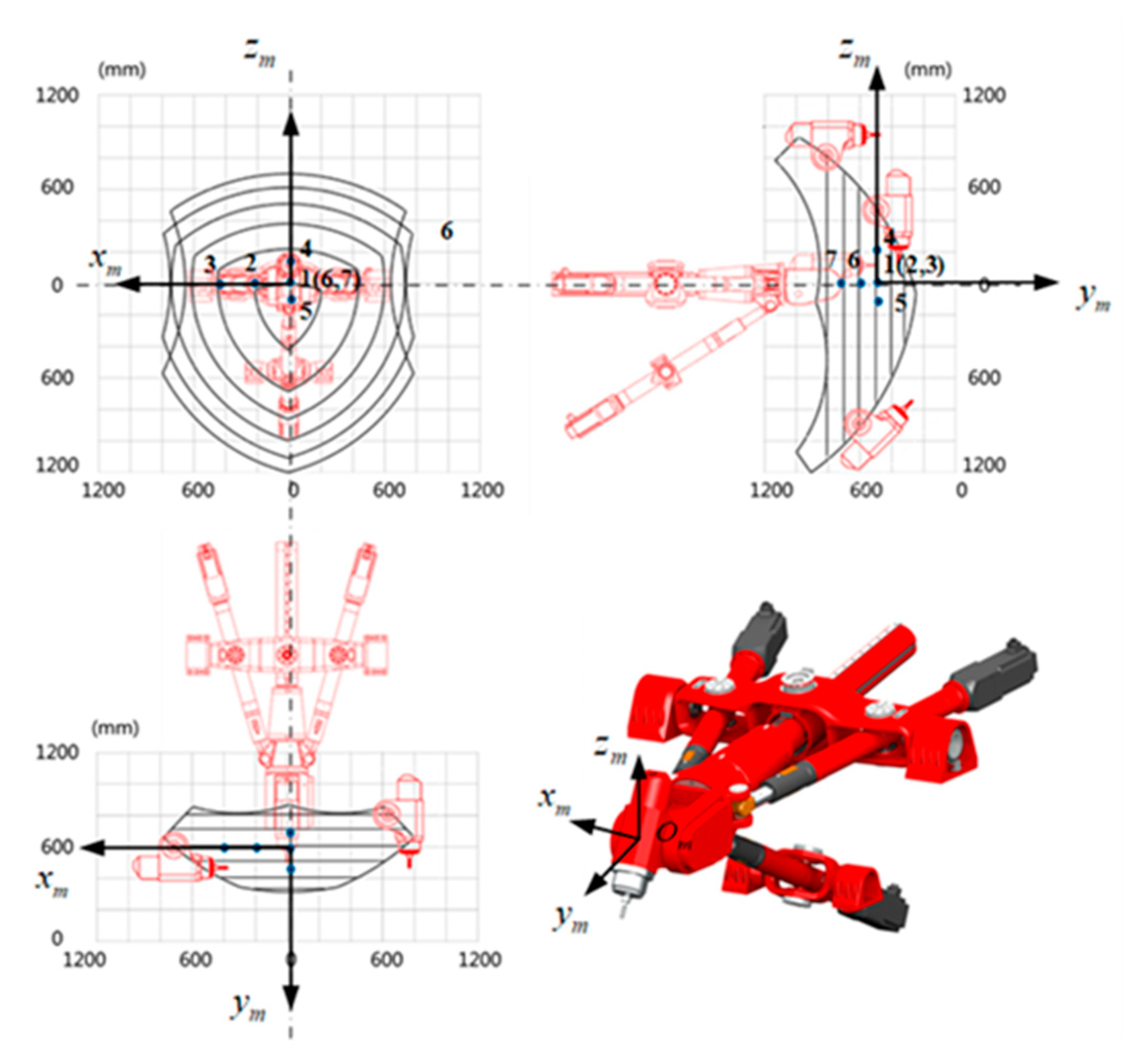
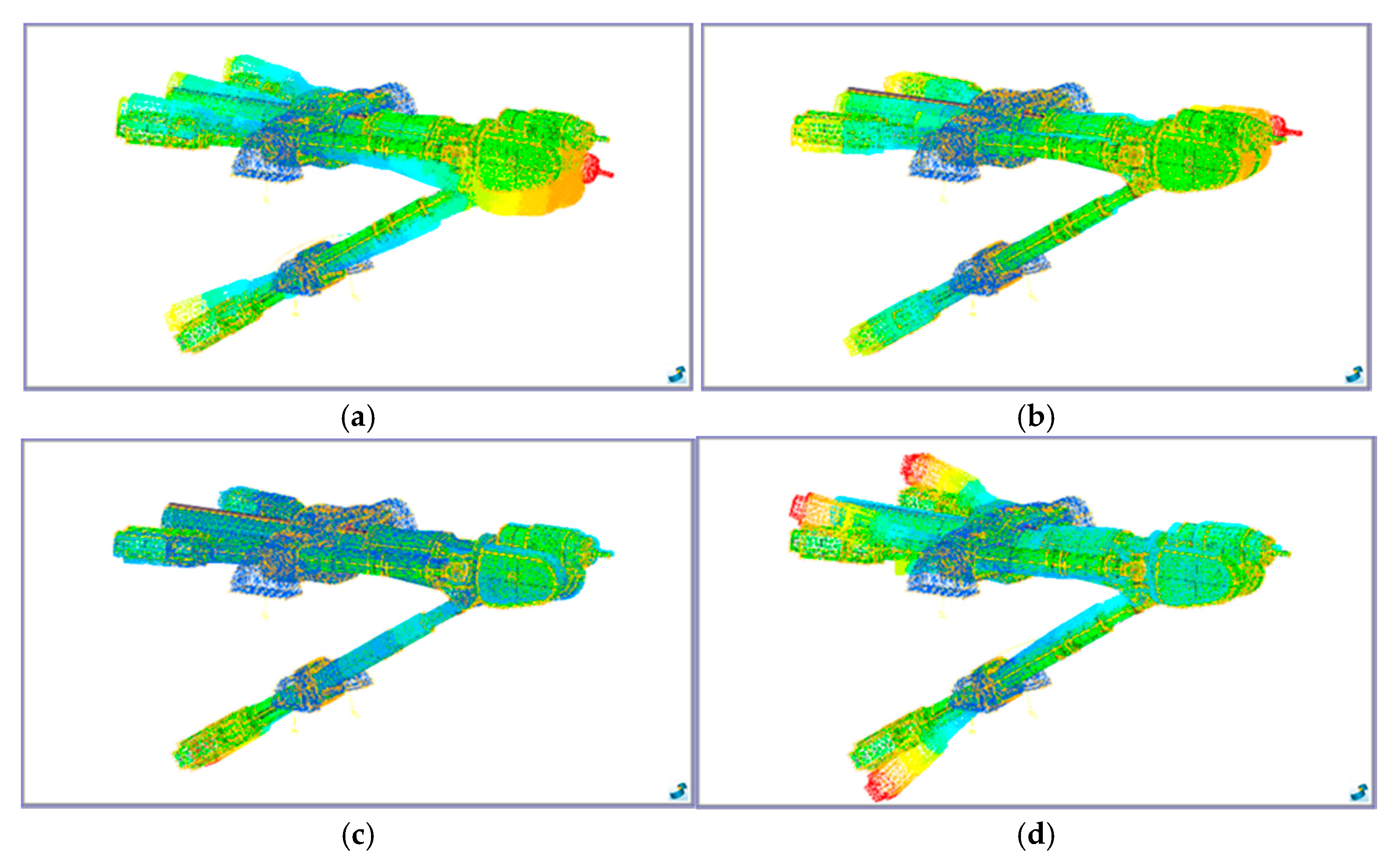



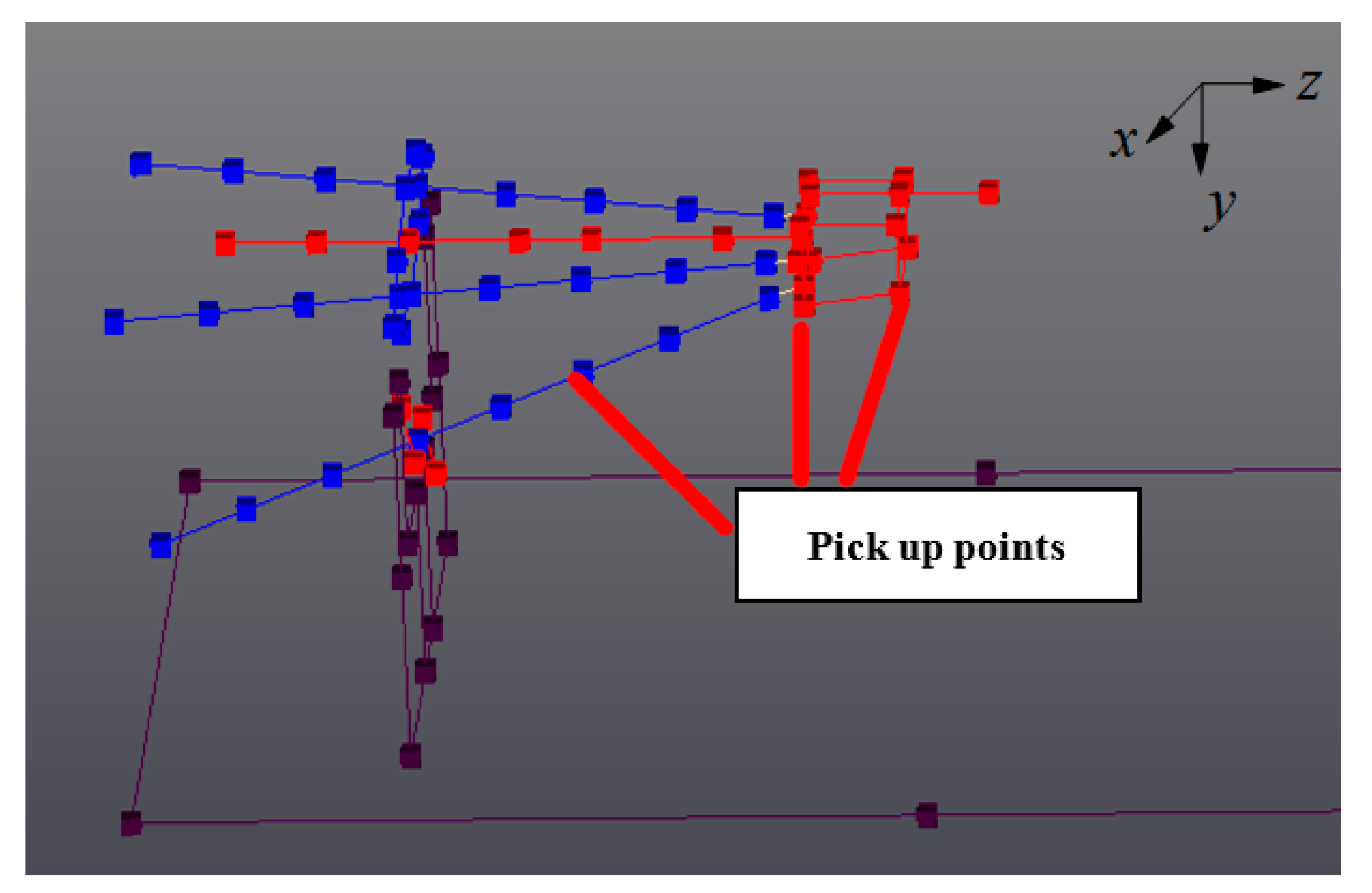




| Number of Positon | x (mm) | y (mm) | z (mm) | Angle of A/C Wrist |
|---|---|---|---|---|
| 1 | 0 | 0 | 0 | 0 |
| 2 | 200 | 0 | 0 | 0 |
| 3 | 400 | 0 | 0 | 0 |
| 4 | 0 | 0 | 100 | 0 |
| 5 | 0 | 0 | −50 | 0 |
| 6 | 0 | −50 | 0 | 0 |
| 7 | 0 | 100 | 0 | 0 |
| Properties | Density (g/cm3) | Tensile Strength (MPa) | Yield Strength (MPa) | Elastic Modulus (GPa) | Shear Modulus (MPa) | Poisson Ratio |
|---|---|---|---|---|---|---|
| Value | 4.5 | 895 | 825 | 110 | 0.342 | 0.342 |
| Properties | Diameter | Rake Angle | Tool Clearance | Helix Angel | Number of Teeth | Materials |
|---|---|---|---|---|---|---|
| Values | 12 mm | 8° | 15° | 40° | 4 | K44 UF |
| Spindle Speed (r/min) | ap (mm) | Feed Engagement (mm) | Feed Speed (mm/min) |
|---|---|---|---|
| 2000 | 0.2 | 0.02 | 160 |
| 2000 | 0.2 | 0.03 | 240 |
| 2000 | 0.2 | 0.04 | 320 |
| 2000 | 0.2 | 0.05 | 400 |
| 2000 | 0.4 | 0.02 | 160 |
| 2000 | 0.4 | 0.03 | 240 |
| 2000 | 0.4 | 0.04 | 320 |
| 2000 | 0.4 | 0.05 | 400 |
| 2000 | 0.6 | 0.02 | 160 |
| 2000 | 0.6 | 0.03 | 240 |
| 2000 | 0.6 | 0.04 | 320 |
| 2000 | 0.6 | 0.05 | 400 |
| Number | Spindle Speed (r/min) | ap (mm) | Feed Engagement (mm) | Feed Speed (mm/min) |
|---|---|---|---|---|
| 1 | 1000 | 0.2 | 0.02 | 80 |
| 2 | 2000 | 0.2 | 0.02 | 160 |
| 3 | 3000 | 0.2 | 0.02 | 240 |
| 4 | 1000 | 0.4 | 0.02 | 80 |
| 5 | 2000 | 0.4 | 0.02 | 160 |
| 6 | 3000 | 0.4 | 0.02 | 240 |
| 7 | 1000 | 0.6 | 0.02 | 80 |
| 8 | 2000 | 0.6 | 0.02 | 160 |
| 9 | 3000 | 0.6 | 0.02 | 240 |
| 10 | 1000 | 0.8 | 0.02 | 80 |
| 11 | 2000 | 0.8 | 0.02 | 160 |
| 12 | 3000 | 0.8 | 0.02 | 240 |
| Number of Teeth | Longth of Cutting Edge I (mm) | Longth of Cutting Edge II (mm) | Rake Angel | Tool Clearance | Helix Angle | Transient Mode |
|---|---|---|---|---|---|---|
| 4 | 11 | 5 | 8° | 15° | 40° | Right angle |
| Direction | Number of Mode | ωn (Hz) | ξ | m (kg) |
|---|---|---|---|---|
| 1 | 23.62 | 9.15 | 2.31 × 10−8 | |
| x | 2 | 29.23 | 9.80 | 2.10 × 10−8 |
| 3 | 34.82 | 6.03 | 2.07 × 10−8 | |
| 4 | 40.93 | 4.22 | 7.77 × 10−9 | |
| 1 | 23.54 | 7.79 | 2.08 × 10−8 | |
| y | 2 | 33.56 | 6.59 | 1.058 × 10−8 |
| 3 | 56.52 | 4.19 | 3.86 × 10−8 | |
| 4 | 68.07 | 2.76 | 3.63 × 10−10 | |
| 1 | 23.76 | 7.52 | 2.79 × 10−8 | |
| z | 2 | 29.23 | 10.07 | 7.68 × 10−8 |
| 3 | 34.33 | 5.53 | 2.32 × 10−8 | |
| 4 | 39.83 | 3.48 | 2.13 × 10−8 |
| Depth of Axial Cutting (mm) | kr (N/mm2) | kt (N/mm2) | ka (N/mm2) | kre (N/mm) | kte (N/mm) | kae (N/mm) |
|---|---|---|---|---|---|---|
| 0.2 | 1044.5 | 477.4 | 287.9 | 299.1 | 54.3 | 32.5 |
| 0.4 | 1221.0 | 584.5 | 316.5 | 306.3 | 56.8 | 38.8 |
| 0.6 | 1383.1 | 648.8 | 357.2 | 311.5 | 58.5 | 48.2 |
| The average | 1216.2 | 570.2 | 320.5 | 305.6 | 56.5 | 39.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, X.; Shi, M.; Hou, Z.; Li, S.; Li, H.; Liu, H. Analysis of 3-DOF Cutting Stability of Titanium Alloy Helical Milling Based on PKM and Machining Quality Optimization. Machines 2022, 10, 404. https://doi.org/10.3390/machines10050404
Qin X, Shi M, Hou Z, Li S, Li H, Liu H. Analysis of 3-DOF Cutting Stability of Titanium Alloy Helical Milling Based on PKM and Machining Quality Optimization. Machines. 2022; 10(5):404. https://doi.org/10.3390/machines10050404
Chicago/Turabian StyleQin, Xuda, Mengrui Shi, Zhuojie Hou, Shipeng Li, Hao Li, and Haitao Liu. 2022. "Analysis of 3-DOF Cutting Stability of Titanium Alloy Helical Milling Based on PKM and Machining Quality Optimization" Machines 10, no. 5: 404. https://doi.org/10.3390/machines10050404
APA StyleQin, X., Shi, M., Hou, Z., Li, S., Li, H., & Liu, H. (2022). Analysis of 3-DOF Cutting Stability of Titanium Alloy Helical Milling Based on PKM and Machining Quality Optimization. Machines, 10(5), 404. https://doi.org/10.3390/machines10050404







