Temperature Dynamic Characteristics Analysis and Thermal Load Dissipation Assessment for Airliner Hydraulic System in a Full Flight Mission Profile
Abstract
:1. Introduction
2. Thermal Dynamic Modeling of Hydraulic Components
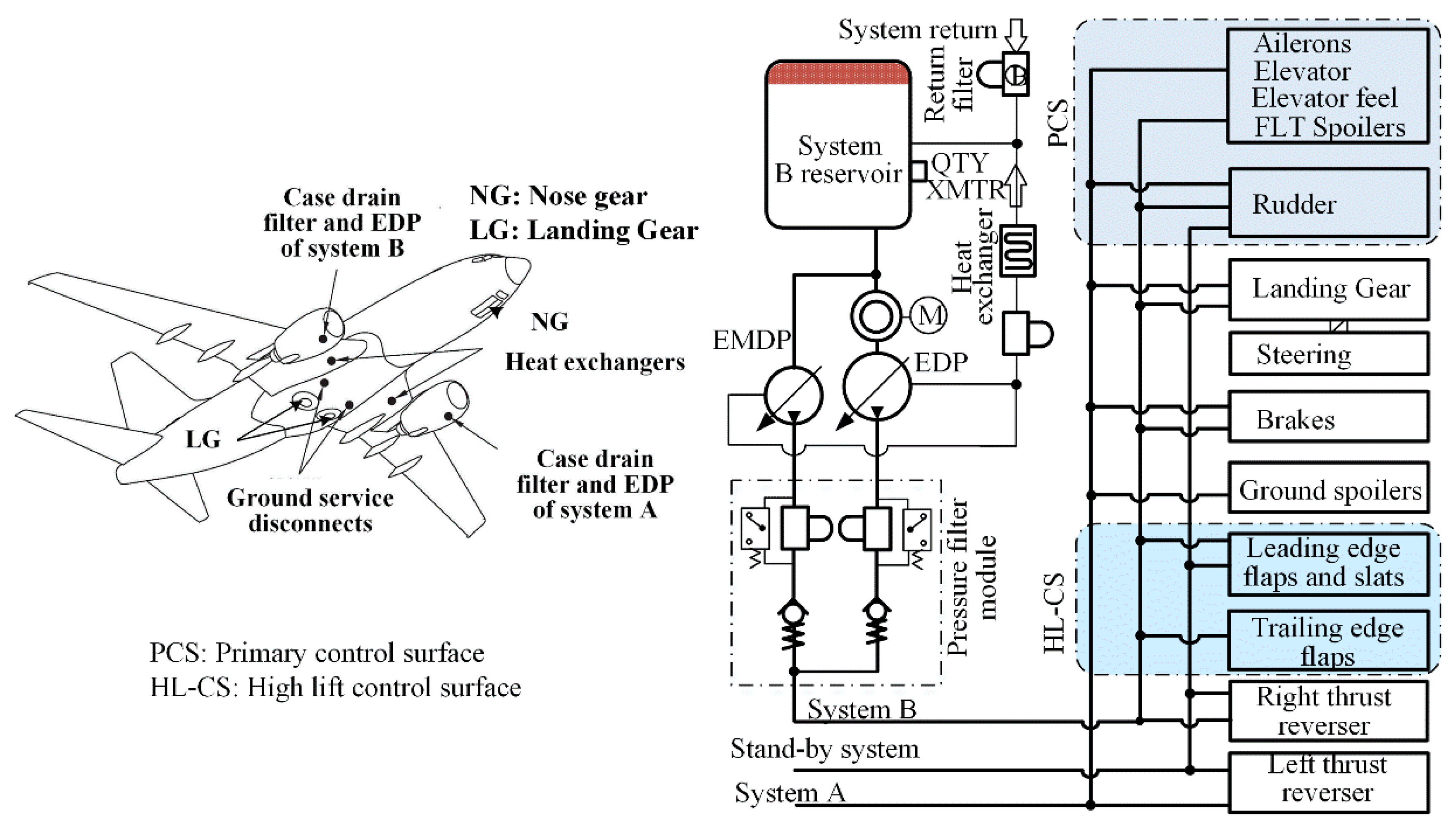
2.1. The Scheme Description of Thermal Dynamic Modeling
2.2. Thermal Dynamic Modeling of Key Hydraulic Components
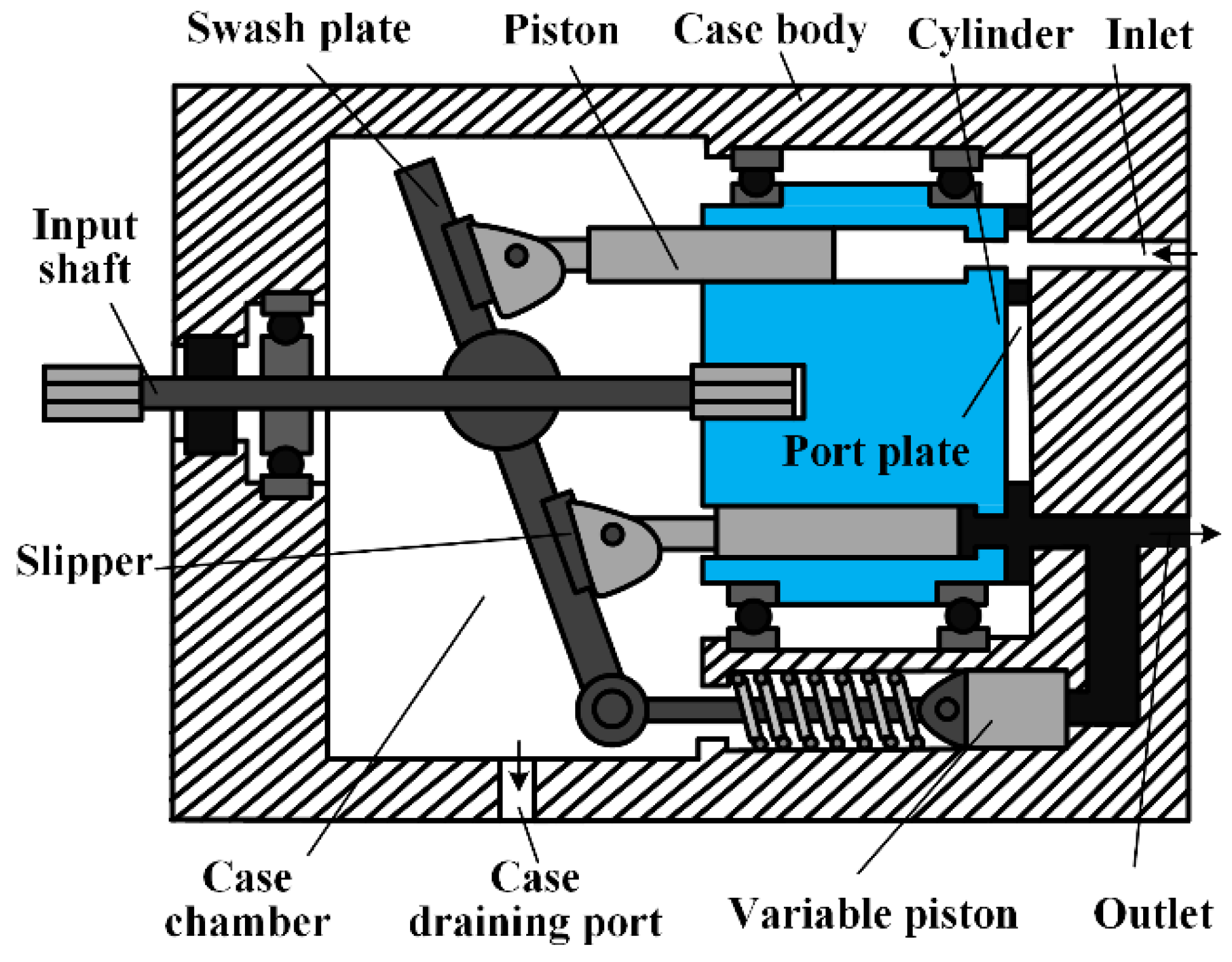
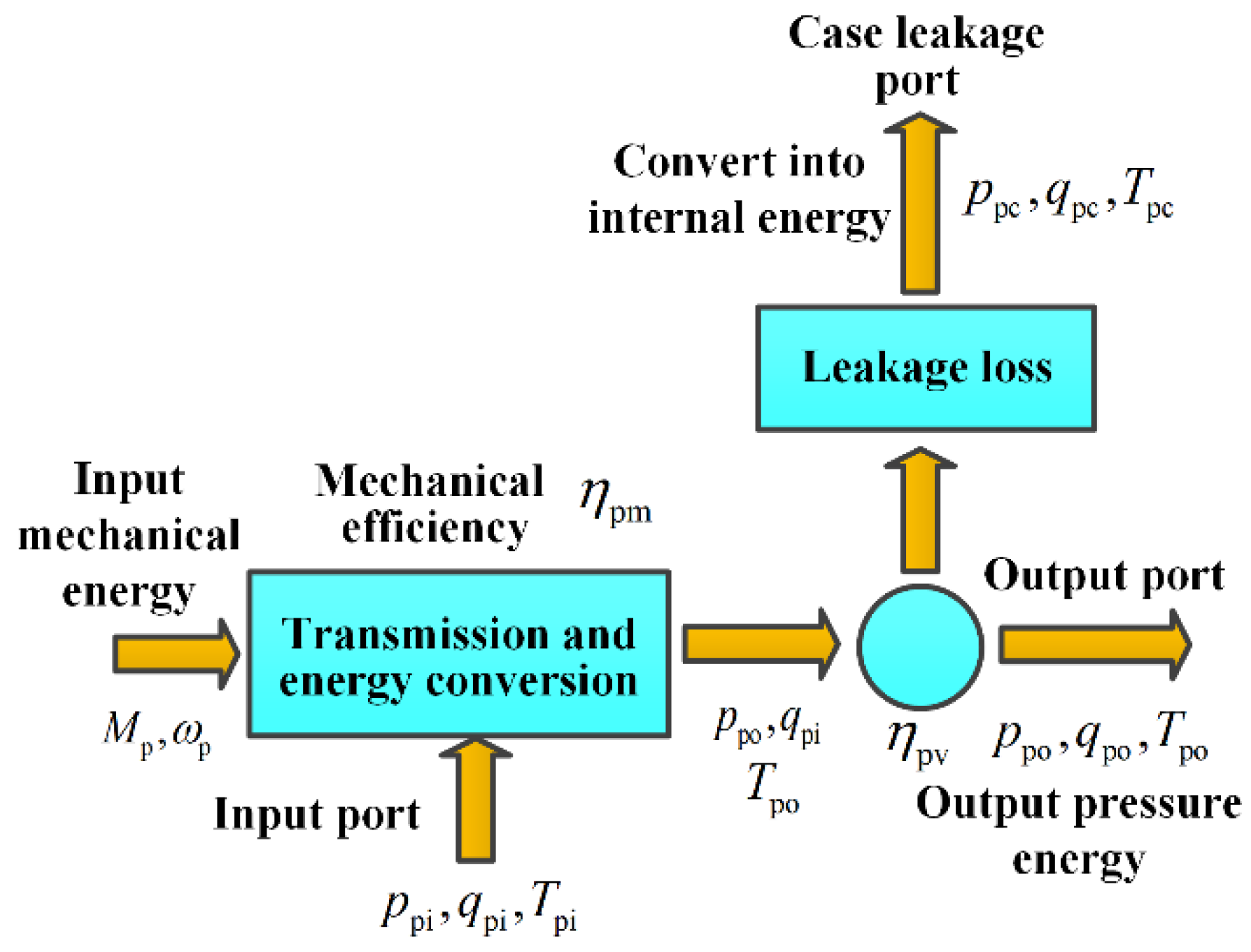
2.2.1. Axial Piston Pump
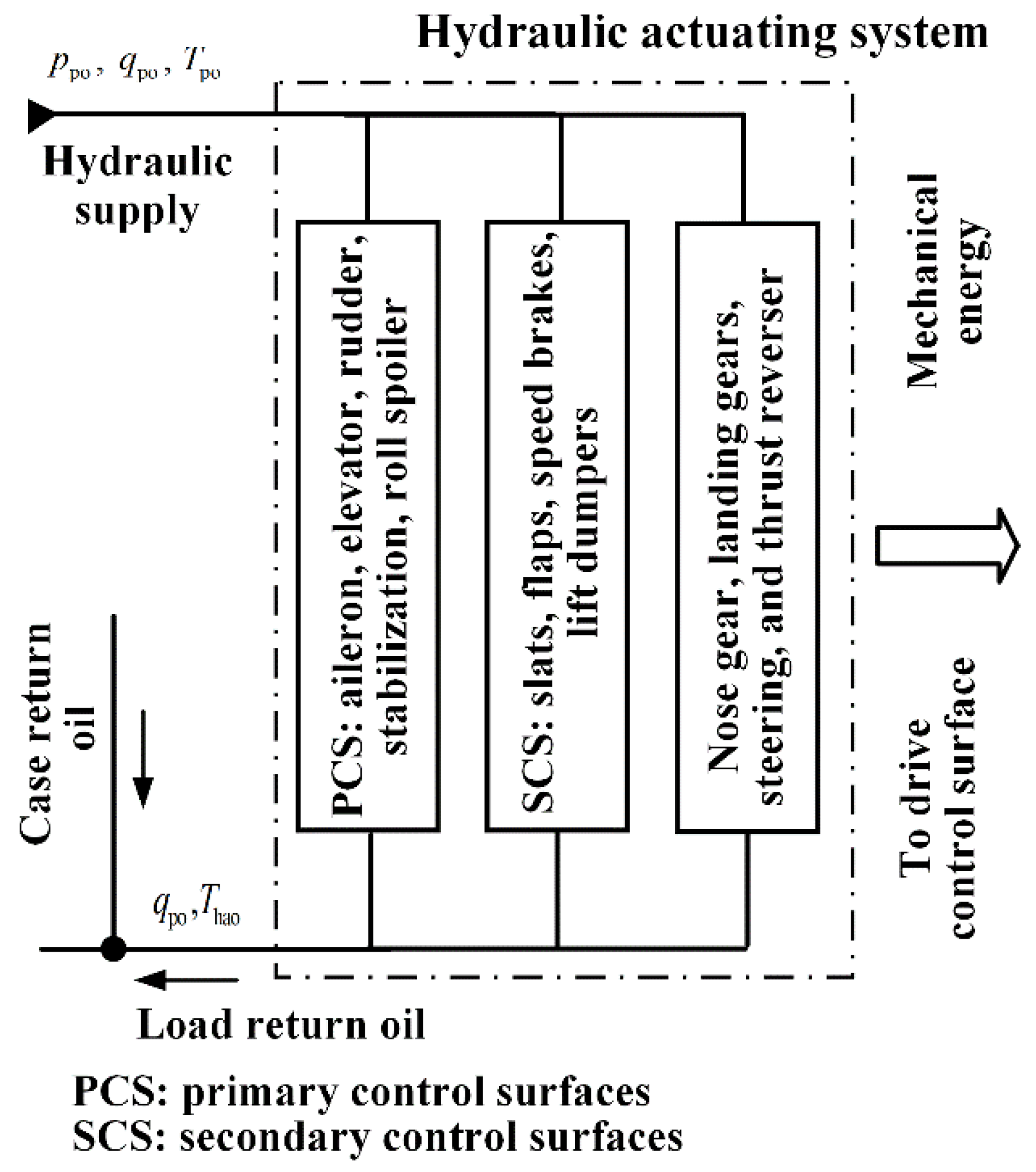
2.2.2. Hydraulic Actuating Subsystem
2.2.3. Hydraulic Oil Tank
2.2.4. Thermal Management Module
3. Working Condition and Simulation Model Establishment
3.1. Working Condition Used for Simulation Calculation
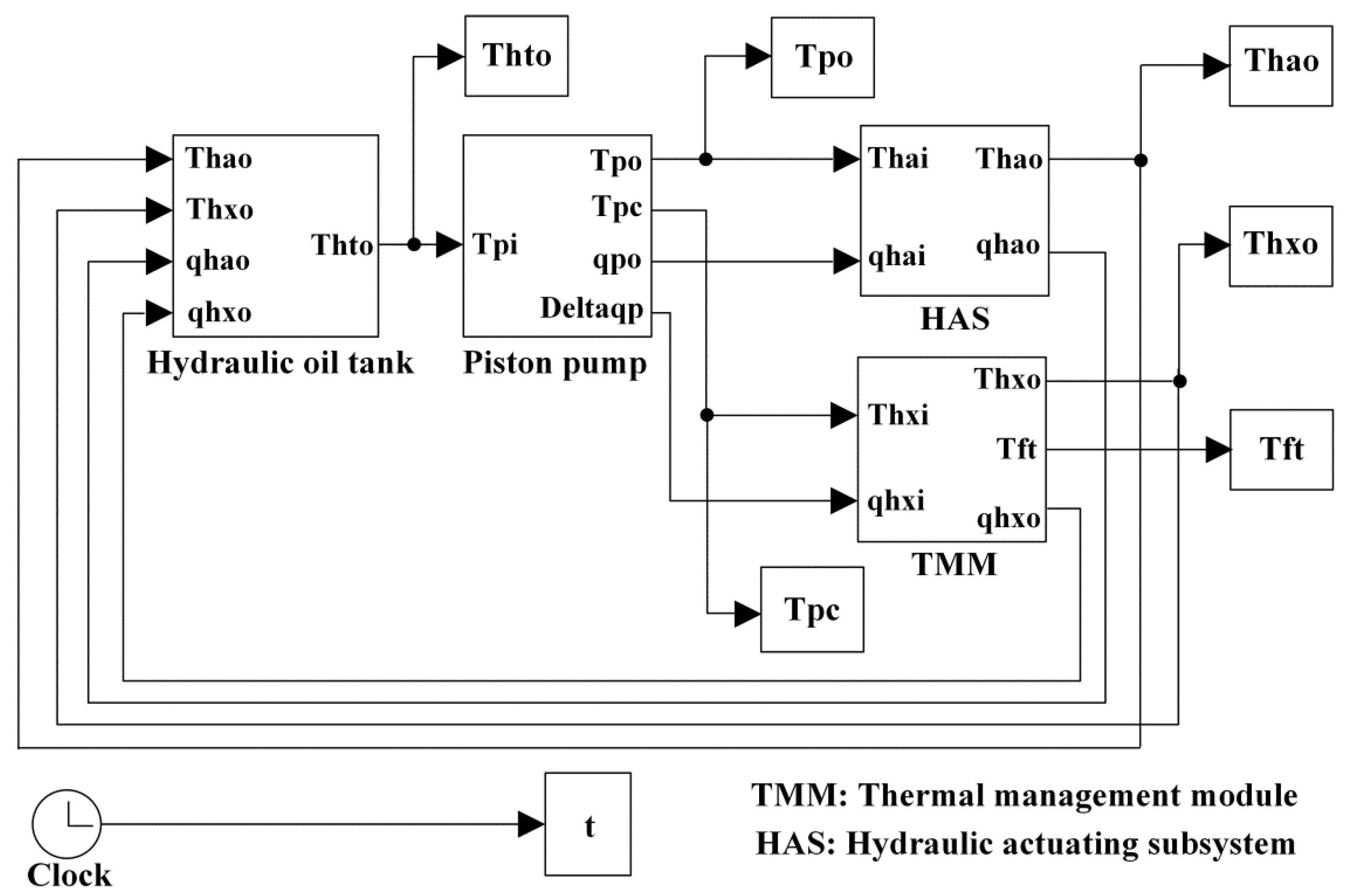
3.2. Thermal Dynamic Simulation Model
4. The Analysis of Simulation Results
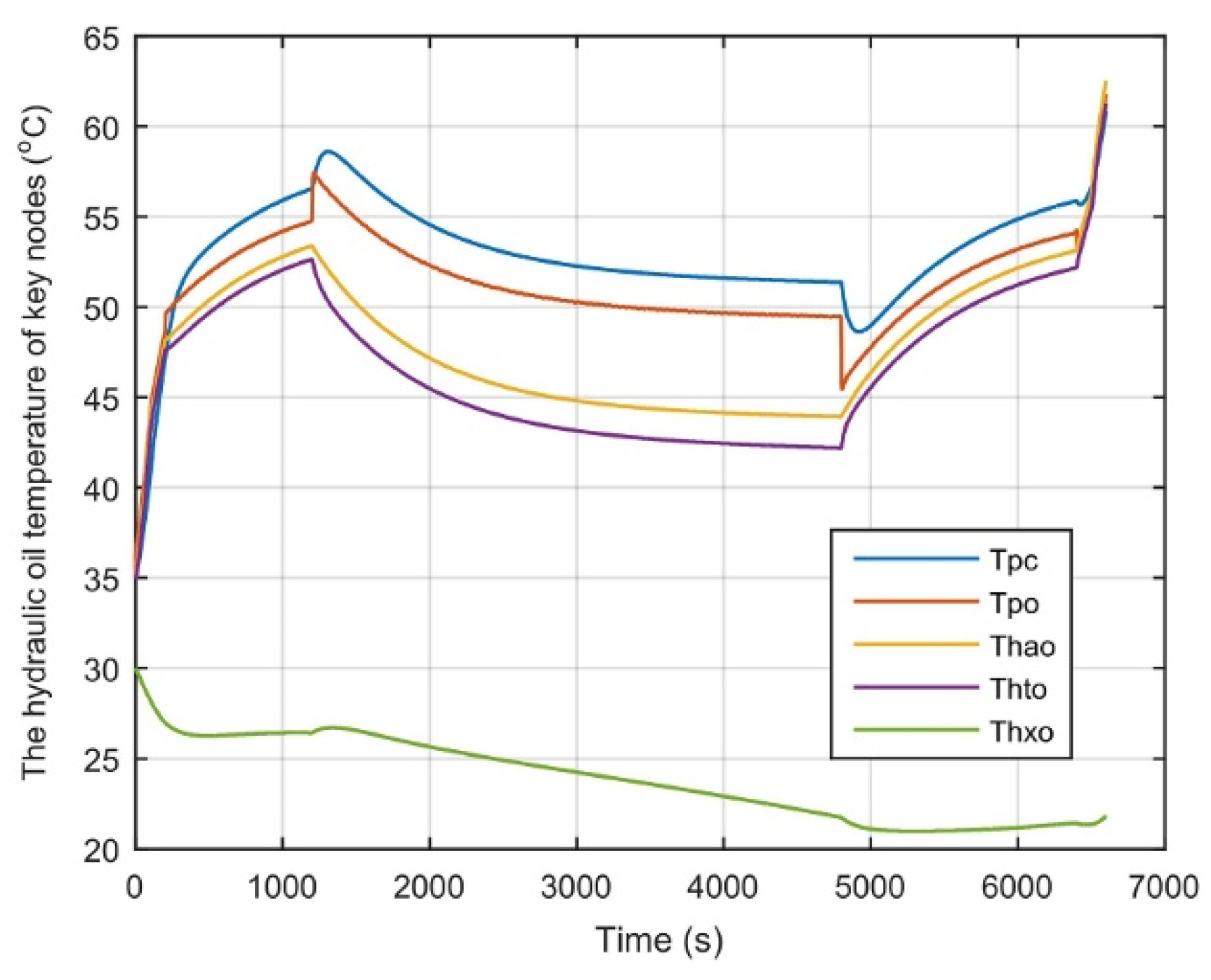
4.1. Temperature Characteristic Analysis at Key Nodes of Hydraulic System
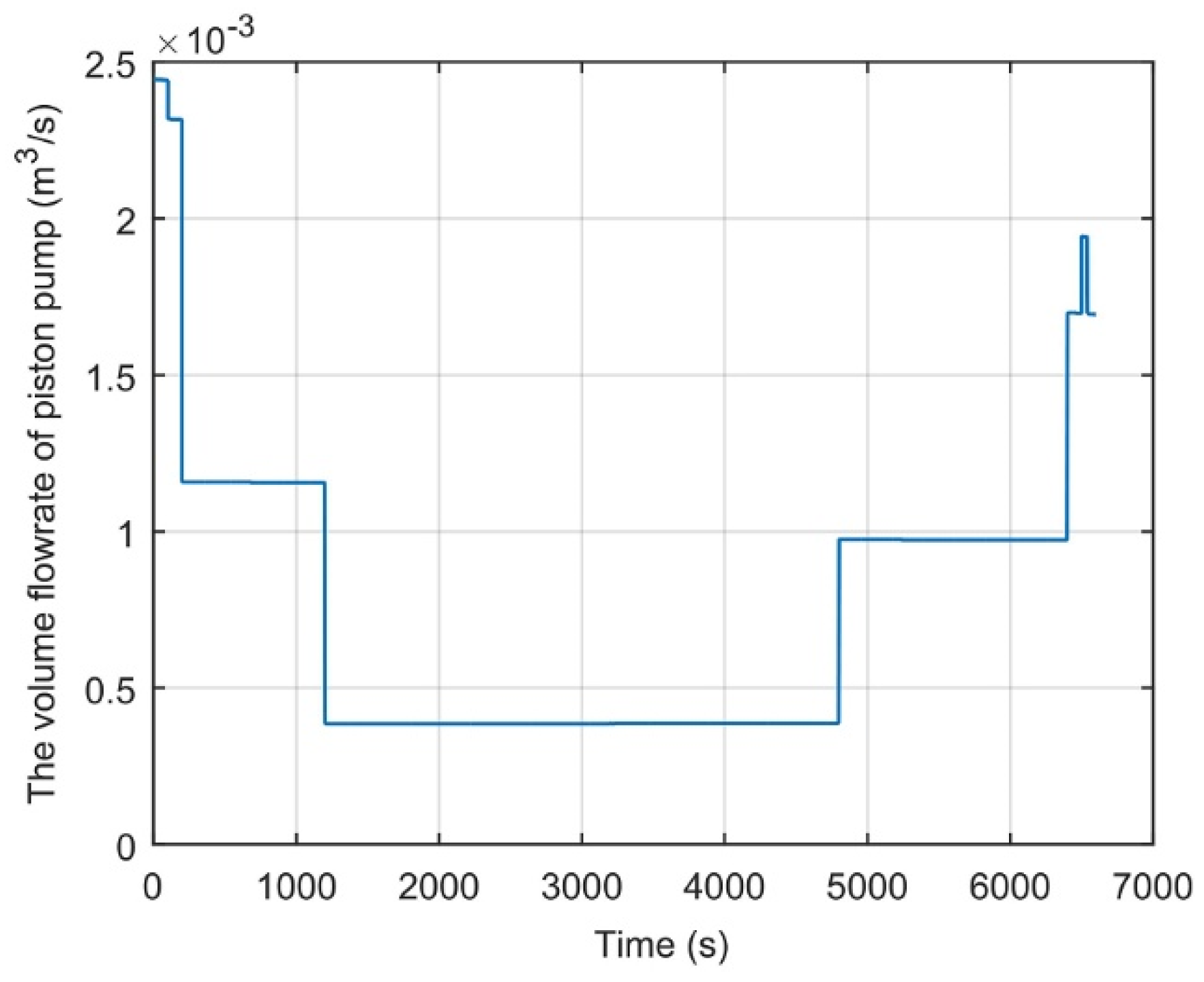
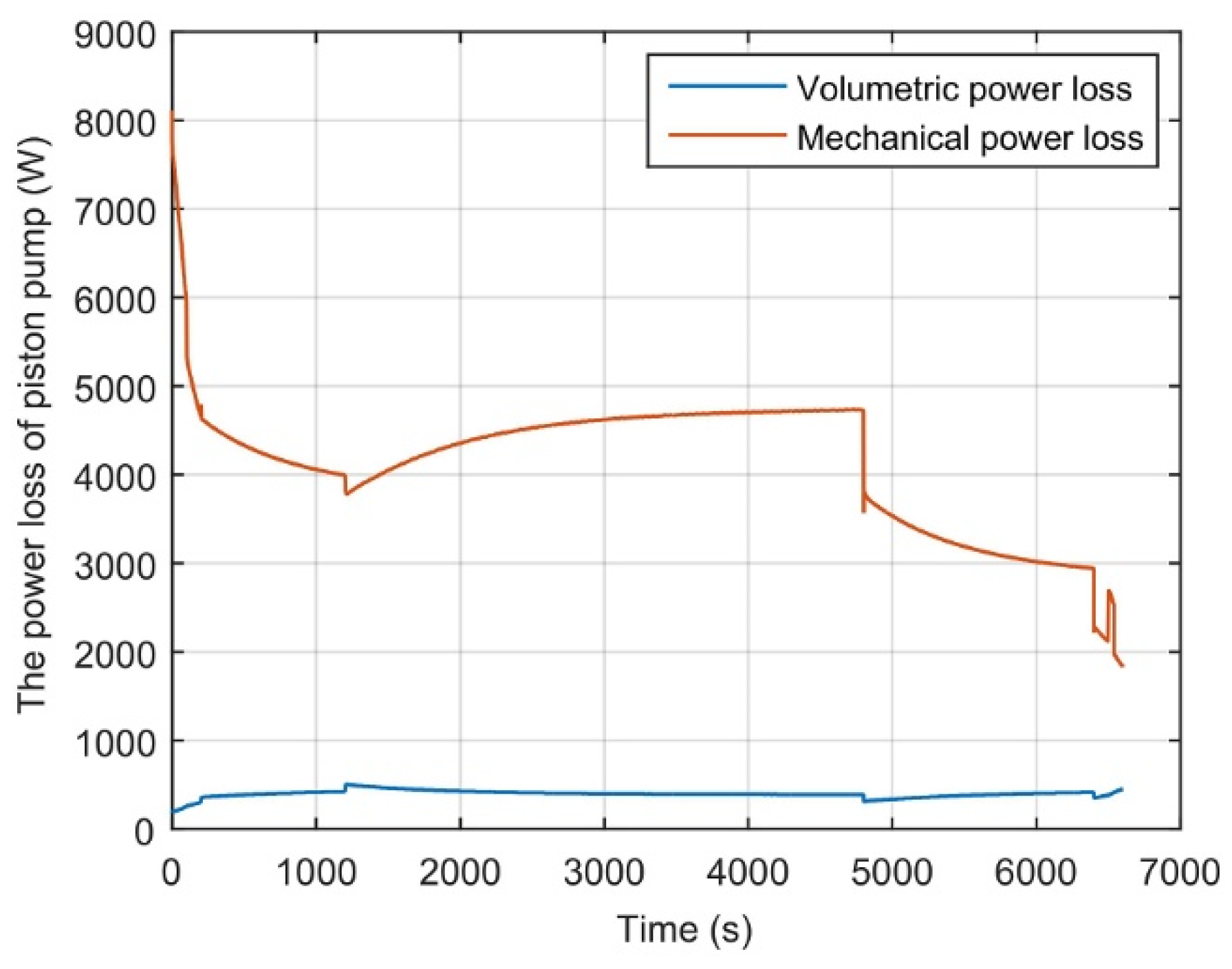
4.2. Power Loss Characteristic Analysis of the Axial Piston Pump
4.3. The Thermal Characteristic Analysis of Thermal Management Module
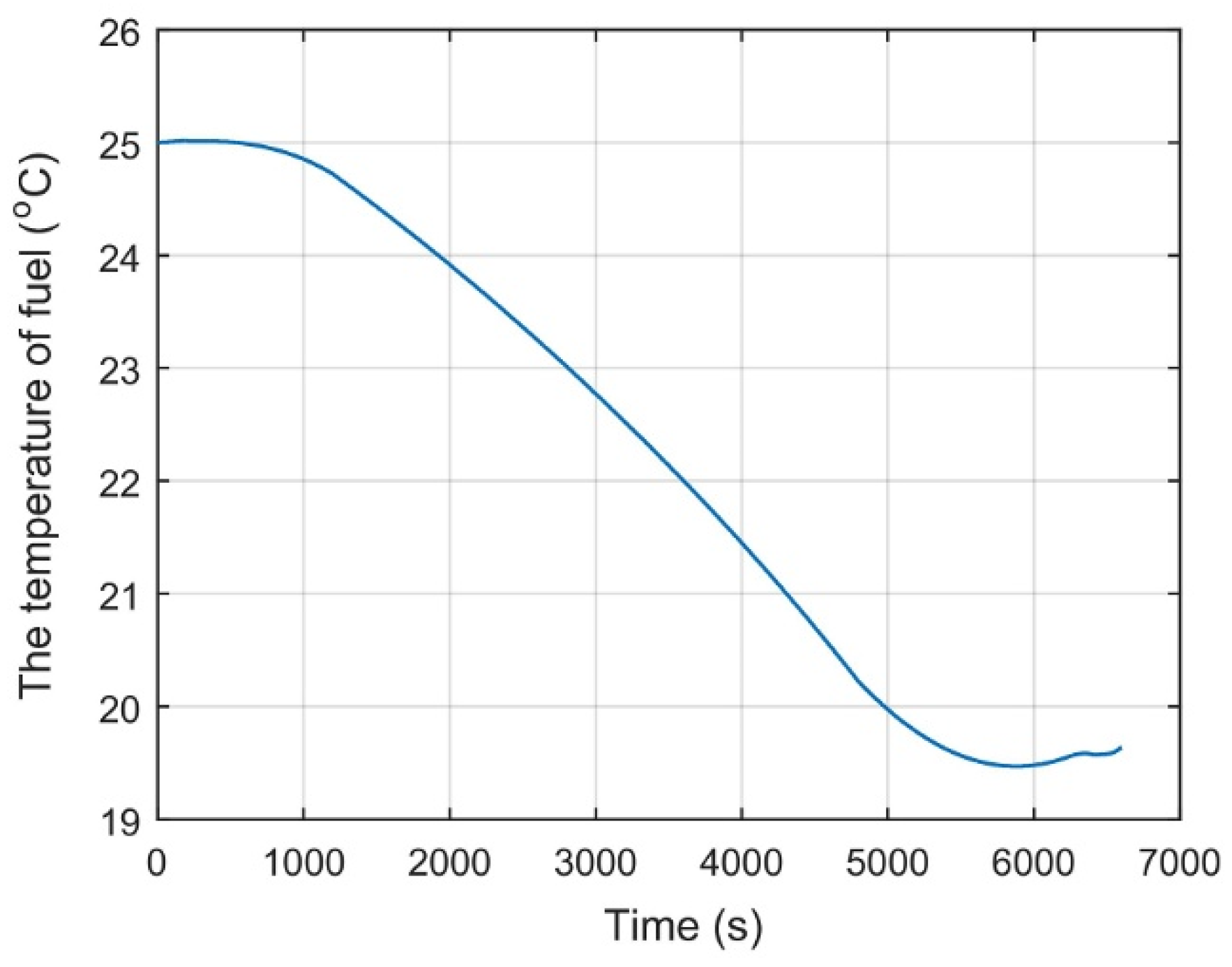
4.3.1. Fuel Temperature Inside the Fuel Tank
4.3.2. Inlet and Outlet Temperature of Heat Exchanger
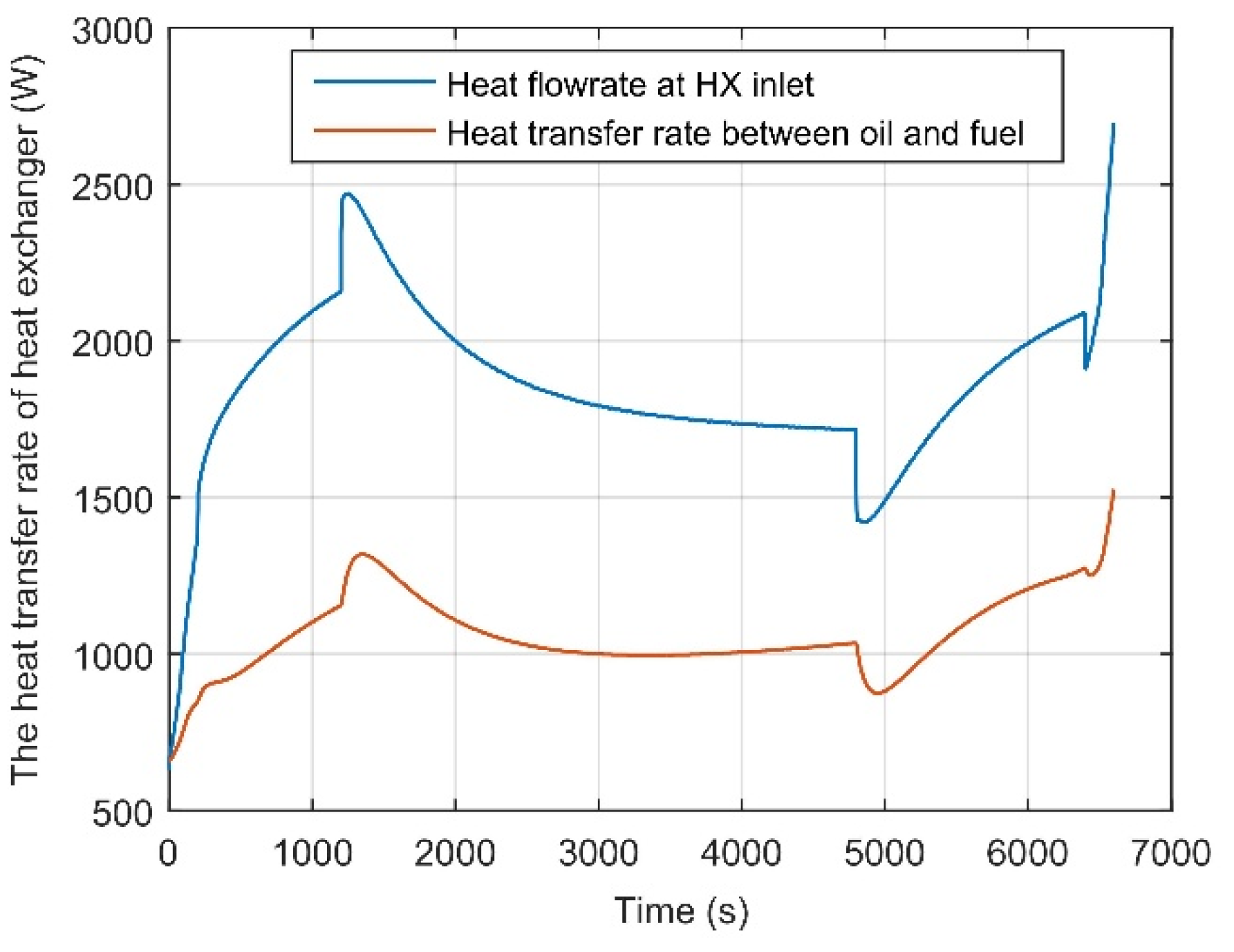
4.3.3. Heat Transfer Analysis of Heat Exchanger
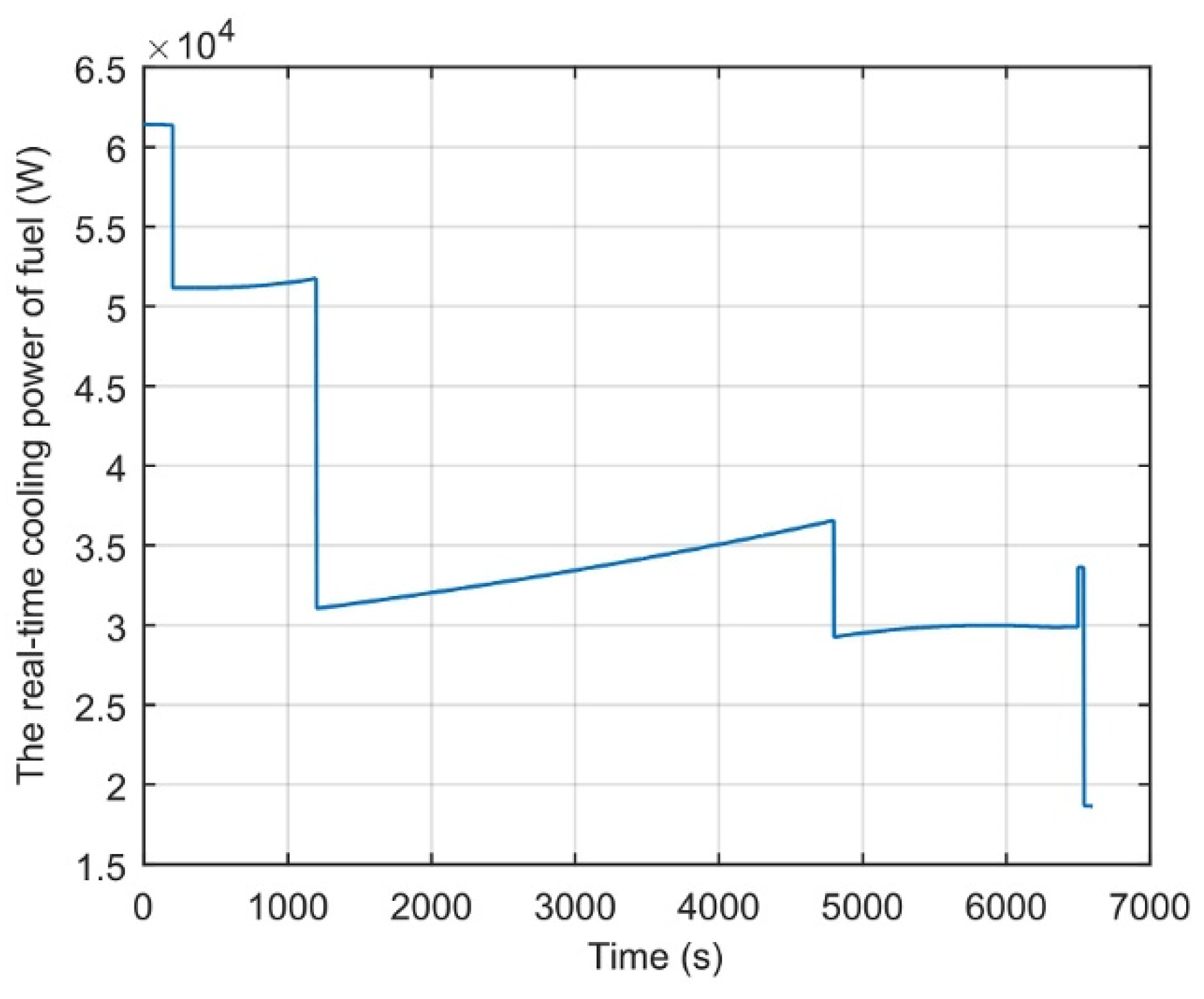
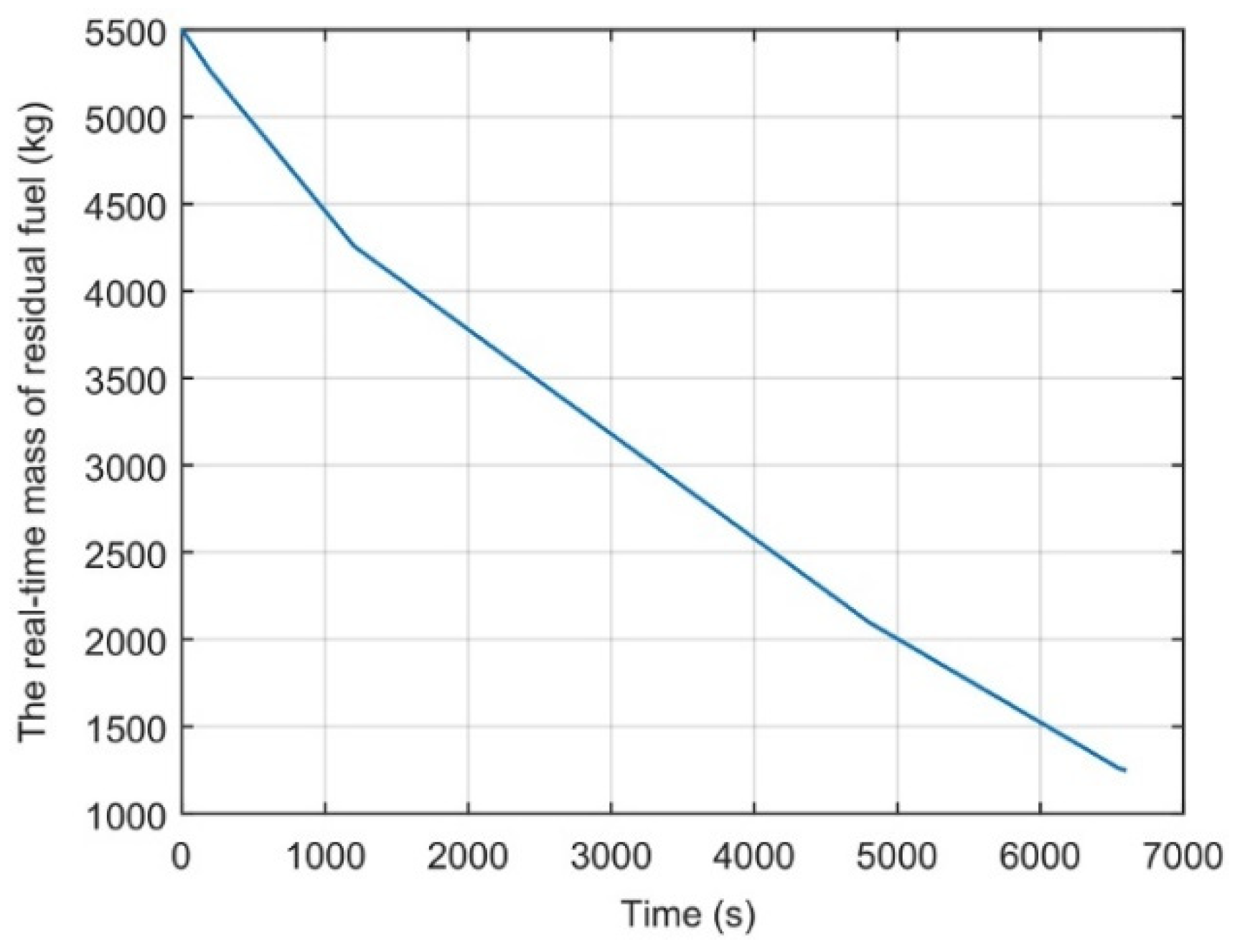
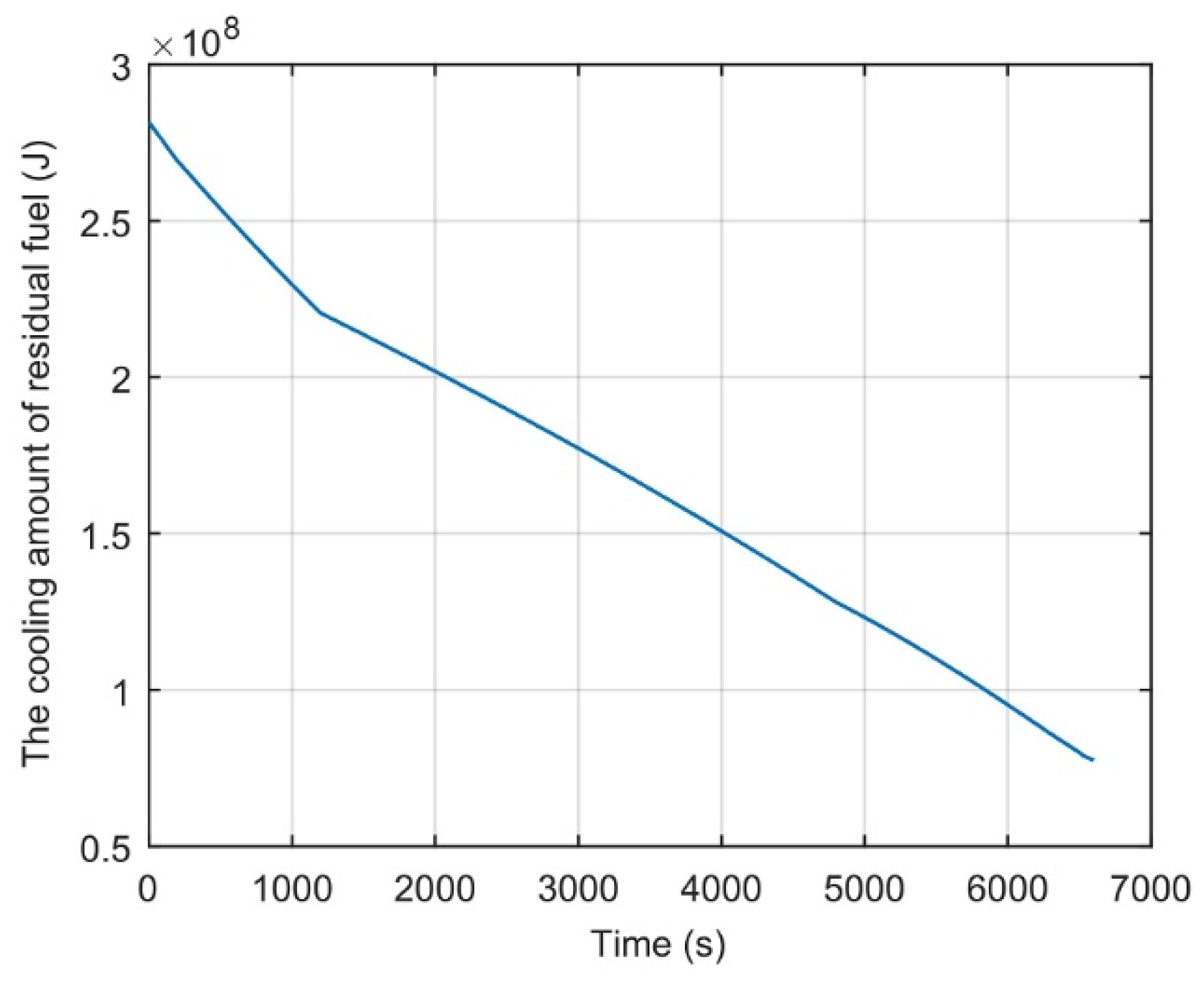
4.3.4. The Cooling Ability Analysis of Fuel Heat Sink
4.4. Verification of the Model of Electro-Hydraulic Servo Subsystem
5. Conclusions
- (1)
- The established power losses model of the pump and the actuating system and the thermal dynamic model of the entire hydraulic system are effective. The temperature rise of the hydraulic system appears in stages of climbing and landing, and the maximum temperature node is at the housing port of the pump.
- (2)
- The thermal management scheme, i.e., to adopt the heat exchanger assembled at the case returning oil pipeline of the piston pump and immersed in the wing root of the fuel tank, is feasible. It can ensure the temperature rises of the hydraulic system and the fuel tank are less than the allowable values.
- (3)
- The temperature, the real-time cooling power, and the residual cooling amount of the fuel heat sink in the fuel tank meet the requirements of the full flight mission profile.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Notations | |
| Heat transfer area of pump body () | |
| Heat transfer areas of unilateral wing fuel tank and half center fuel tank () | |
| Heat transfer area of hydraulic actuating subsystem () | |
| Heat transfer areas of hydraulic oil tank and heat exchanger () | |
| Heat transfer area between pump outlet and case port () | |
| Specific heat capacity of hydraulic actuator () | |
| Specific heat capacities of fuel and specific heat capacity of hydraulic oil () | |
| Specific heat capacities of high-pressure pipeline and low-pressure pipeline () | |
| Specific heat capacities of heat exchanger and piston pump, respectively () | |
| Viscous friction coefficient, mechanical friction coefficient, and leakage flow coefficient of piston pump (-) | |
| Instantaneous displacement and maximum displacement of piston pump () | |
| Equivalent factor of product of specific heat capacity and mass of hydraulic actuating subsystem (-) | |
| Equivalent factors of products of specific heat capacity and mass of hydraulic oil tank (-) and heat exchanger (-) | |
| Equivalent factors of products of specific heat capacity and mass of the pump housing body (-) and the pump cylinder (-) | |
| Heat transfer coefficient between pump case body and nacelle air () | |
| Heat transfer coefficients, between unilateral wing fuel tank and wing surface, between half center fuel tank and cold air inside airborne equipment bay () | |
| Heat transfer coefficients, between hydraulic actuating subsystem and cold air inside airborne equipment bay, between hydraulic oil tank and equipment bay () | |
| Heat transfer coefficient between hydraulic oil and fuel of heat exchanger () | |
| Heat transfer coefficient between leakage port and outlet () | |
| Mass of hydraulic actuators () | |
| Initial fuel mass and residual fuel mass of fuel tank () | |
| Mass flow-rate which engine fuel consumes () | |
| Oil mass of hydraulic actuating subsystem () | |
| Oil mass of hydraulic oil tank and oil mass of heat exchanger, respectively () | |
| Net mass of high-pressure pipeline and net mass of hydraulic oil tank () | |
| Net mass of heat exchanger, net mass of low-pressure pipeline (kg) | |
| Net mass of piston pump (kg) | |
| Oil mass in pump discharge chamber, oil mass of pump case chamber (kg) | |
| Theoretical torque, viscous friction torque and mechanical friction torque of piston pump () | |
| Input shaft torque of piston pump (), rotational speed of piston pump (), | |
| Case port pressure, inlet pressure and of pump, outlet pressure of piston pump () | |
| Pressure difference between the outlet pressure and inlet pressure () | |
| Input shaft power and output hydraulic power of the piston pump (W) | |
| The power loss of hydraulic actuating subsystem (W) | |
| Mechanical power loss and volumetric power loss of piston pump (W) | |
| Volume flow rate at the outlet of hydraulic actuating subsystem () | |
| Volume flow rate at the outlet of heat exchanger () | |
| Input volume flowrate and output volume flowrate of piston pump () | |
| Leakage flowrate of piston pump () | |
| Theoretical volume flowrate of piston pump () | |
| Residual cooling amount which fuel heat sink can provide in current moment (J) | |
| Cooling flowrate of fuel heat sink (W) | |
| Heat flowrate of heat exchange between fuel and external air outside fuel tank (W) | |
| Heat flowrate between pump case body and nacelle air (W) | |
| Heat flowrate of heat exchange between hydraulic actuating subsystem and cold air from airborne equipment bay (W) | |
| Heat flowrate of heat exchange between fuel and hydraulic oil inside thermal management module (W) | |
| Heat flowrate between the discharge chamber and case returning chamber (W) | |
| Heat tranfer rate around the control volume surface (W) | |
| Threshold fuel temperature inside fuel tank () | |
| Fuel temperature inside fuel tank () | |
| Temperature of the cold air inside airborne equipment bay () | |
| Oil temperature at the outlet of hydraulic actuating subsystem () | |
| Environment temperature of hydraulic oil tank () | |
| Oil temperature at the outlet of hydraulic oil tank () | |
| Oil temperatures at the inlet and outlet of heat exchanger () | |
| Oil temperature at case port of piston pump () | |
| Temperature of the nacelle air () | |
| Inlet oil temperature and outlet oil temperature of piston pump () | |
| The logarithmic mean temperature difference of heat exchanger () | |
| Density of the hydraulic oil () and dynamic viscosity of hydraulic oil () | |
| Inclination angle coefficient of swash plate of piston pump (-) | |
| Rotational angular speed of piston pump (), | |
| Overall efficiency, mechanical efficiency and volumetric efficiency of the piston pump (-) | |
Abbreviations
| EBHA | Electric-Backup-Hydraulic actuator |
| EHA | Electric-hydrostatic actuator |
| EDP | Aerengine driven pump |
| EMDP | Electric motor driven pump |
| HX | Heat exchanger |
References
- Wheeler, P. Technology for the More and All Electric Aircraft of the Future. In Proceedings of the 2016 IEEE International Conference on Automatica (ICA-ACCA), Curico, Chile, 19–21 October 2016. [Google Scholar]
- Sarlioglu, B.; Morris, C.T. More Electric Aircraft: Review, Challenges, and Opportunities for Commercial Transport Aircraft. IEEE Trans. Transp. Electrif. 2015, 1, 54–64. [Google Scholar] [CrossRef]
- Fitch, E.C.; Hong, I.T. HyPneu Thermal Manual; BarDyne Inc.: Stillwater, OK, USA, 2004. [Google Scholar]
- Wang, S.P.; Tomovic, M.; Liu, H. Commercial Aircraft Hydraulic System; Shanghai Jiao Tong University Press: Shanghai, China, 2016. [Google Scholar]
- Li, D.; Hang, J.; Li, Y.; Dong, S. Fuel Flowrate Control for Aeroengine and Fuel Thermal Management for Airborne System of Aircraft-An Overview. Appl. Sci. 2022, 12, 279. [Google Scholar] [CrossRef]
- Guo, S.R.; Chen, J.H.; Lu, Y.L.; Wang, Y. Hydraulic piston pump in civil aircraft: Current status, future directions and critical technologies. Chin. J. Aeronaut. 2020, 33, 16–30. [Google Scholar] [CrossRef]
- Yin, Y.B.; Wang, Z.Y.; Li, J.; Tang, H.S. Thermal Characteristics of Aircraft Hydraulic Pump. J. Fluid Power Transm. Control. 2016, 1, 11–15. (In Chinese) [Google Scholar]
- Zhang, J.; Li, Y.; Xu, B.; Chen, X.; Pan, M. Churning losses analysis on the thermal-hydraulic model of a high-speed electro-hydrostatic actuator pump. Int. J. Heat Mass Transf. 2018, 127, 1023–1030. [Google Scholar] [CrossRef]
- Wang, K.; Huang, X.P.; Wang, H.X. Thermal-hydraulic System Modeling and Simulation of Large Aircraft Hydraulic Systems Based on AMESim. J. Fluid Power Transm. Control 2016, 3, 23–27. (In Chinese) [Google Scholar]
- Li, Y.L.; Hou, Y.Y.; Cao, K.Q.; Hu, L.M. Thermal Performance Simulation and Comparing Analysis for Aircraft Hydraulic System with Different Pumping Source Structure. J. Mech. Sci. Technol. Aerosp. Eng. 2016, 35, 1470–1476. (In Chinese) [Google Scholar]
- Li, J.; Tang, G.; Cao, J.; Shi, X. Thermodynamic Analysis and Calculations on Hydraulic Steering Units of Aircraft Hydraulic System. J. Chin. Mech. Eng. 2015, 26, 2070–2074. (In Chinese) [Google Scholar]
- Du, Z.; Li, K. Application of real-time simulation on the integrated management of aircraft hydraulic system. Aeronaut. Sci. Technol. 2016, 27, 71–74. [Google Scholar]
- Li, D.; Dong, S.J.; Wang, J.; Li, Y.H. State-of-art and some considerations on thermal load analysis and thermal management for hydraulic system in MEA. J. Eng. 2018, 13, 399–405. [Google Scholar] [CrossRef]
- Harris, R.M. The Modeling and Simulation of Temperature Effects in Hydraulic Systems. Ph.D. Thesis, School of Mechanical Engineering, University of Bath, Bath, UK, 1990. [Google Scholar]
- Li, D.; Dong, S.J.; Wang, J.; Li, Y.H. Thermal dynamics and thermal management strategy for a civil aircraft hydraulic system. Therm. Sci. 2020, 24, 2311–2318. [Google Scholar] [CrossRef]
- Jonsson, G.; Palsson, O.P. An Application of Extended Kalman Filtering to Heat Exchanger Models. J. Dyn. Sys. Meas. Control 1994, 116, 257–264. [Google Scholar] [CrossRef]
- Dooley, M.; Lui, N.; Newman, R.; Lui, C. Aircraft Thermal Management-Heat Sink Challenge. In Proceedings of the SAE 2014 Aerospace Systems and Technology Conference, Cincinnati, OH, USA, 23 September 2017; SAE Technical Paper 2014-01-2193. Available online: https://www.sae.org/publications/technical-papers/content/2014-01-2193/ (accessed on 29 March 2022).
- Glickstein, M.R.; Griethuysen, V.J.V.; Hodge, E.S. Integration of High Heat Sink Fuels in Aircraft Thermal Management Systems. In Proceedings of the ASME Turbo Expo 2003: Power for Land, Sea and Air, Atlanta, GA, USA, 16–19 June 2003; pp. 75–82. [Google Scholar]
- German, B.J. Tank heating model for aircraft fuel thermal systems with recirculation. J. Propul. Power 2012, 28, 204–210. [Google Scholar] [CrossRef]
- Alyanak, E.J.; Allison, D.L. Fuel thermal management system consideration in conceptual design sizing. In Proceedings of the 57th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, San Diego, CA, USA, 4–8 January 2016. AIAA Paper 2016-0670. [Google Scholar]
- Doman, D.B. Fuel flow topology and control for extending aircraft thermal endurance. J. Thermophys. Heat Transf. 2018, 32, 35–50. [Google Scholar] [CrossRef]
- Doman, D.B. Fuel Flow Control for Extending Aircraft Thermal Endurance Part I: Underlying Principles. In Proceedings of the 2016 AIAA Guidance, Navigation, and Control Conference, San Diego, CA, USA, 4–8 January 2016. AIAA Paper 2016-1621. [Google Scholar]
- Huang, P.G.; Doman, D.B. Thermal Management of Single and Dual Tank Fuel-Flow Topologies Using an Optimal Control Strategy. J. Thermal Sci. Eng. Appl 2018, 10, 041019. [Google Scholar] [CrossRef]
- Pangborn, H.C.; Hey, J.E.; Deppen, T.O.; Alleyne, A.G.; Fisher, T.S. Hardware-in-the-Loop Validation of Advanced Fuel Thermal Management Control. J. Thermophys. Heat Transf. 2017, 32, 901–909. [Google Scholar] [CrossRef]
- Hong, G.; Wei, T.; Ding, X.F.; Duan, C.W. Multi-Objective Optimal Design of Electro-Hydrostatic Actuator Driving Motors for Low Temperature Rise and High Power Weight Ratio. Energies 2018, 11, 1173. [Google Scholar] [CrossRef] [Green Version]
- Boeing 737-600/700/800/900 Aircraft Maintenance Manual (SDS), Company, Chapter 29: Hydraulic Power, Seattle, Boeing Airplane Commercial, June 15 2016, 29-10-24.




| Action | t (s) | H (m) | Ma |
|---|---|---|---|
| Taxi | 0~60 | 0 | 0~0.25 |
| Take-off | 60~100 | 0~25 | 0.25~0.3 |
| Climb1 | 100~200 | 25~450 | 0.3~0.5 |
| Climb2 | 200~1200 | 450~11000 | 0.5~0.8 |
| Cruise | 1200~4800 | 11000 | 0.8 |
| Decline | 4800~6400 | 11000~450 | 0.8~0.4 |
| Approach | 6400~6500 | 450~25 | 0.4~0.3 |
| Land | 6500~6540 | 25~0 | 0.3~0.25 |
| Taxi | 6540~6600 | 0 | 0.25~0 |
| Action | t (s) | ||||||
|---|---|---|---|---|---|---|---|
| Taxi | 0~60 | 3750.0 | 18.0 | 30 | 10000 | 30 | 30 |
| Take-off | 60~100 | 3750.0 | 18.0 | 30 | 16000 | 30 | 30 |
| Climb1 | 100~200 | 3562.5 | 18.0 | 30 | 6000 | 30 | 30 |
| Climb2 | 200~1200 | 3562.5 | 19.5 | 20 | 1000 | 20 | 20 |
| Cruise | 1200~4800 | 3562.5 | 20.5 | 10 | 500 | 10 | 10 |
| Decline | 4800~6400 | 3000.0 | 19.5 | 20 | 2000 | 20 | 20 |
| Approach | 6400~6500 | 2625.0 | 18.0 | 30 | 7000 | 30 | 30 |
| Land | 6500~6540 | 3000.0 | 18.0 | 30 | 17000 | 30 | 30 |
| Taxi | 6540~6600 | 2625.0 | 18.0 | 30 | 13000 | 30 | 30 |
| Action | t (s) | |||
|---|---|---|---|---|
| Taxi | 0~60 | 15 | 15 | 15 |
| Take-off | 60~100 | 15 | 15 | 15 |
| Climb1 | 100~200 | 15 | 15 | 15 |
| Climb2 | 200~1200 | 20 | 20 | 20 |
| Cruise | 1200~4800 | 25 | 25 | 25 |
| Decline | 4800~6400 | 20 | 20 | 20 |
| Approach | 6400~6500 | 15 | 15 | 15 |
| Land | 6500~6540 | 15 | 15 | 15 |
| Taxi | 6540~6600 | 15 | 15 | 15 |
| Time t (s) | Test of Ground Bench | Simulation Result | ||
|---|---|---|---|---|
| Temperature at Supply Port, K | Temperature at Return Port, K | Temperature at Supply Port, K | Temperature at Return Port, K | |
| 0 | 308.2 | 311.2 | 308.2 | 309.6 |
| 100 | 311.7 | 320.2 | 311.7 | 319.8 |
| 200 | 315.4 | 323.2 | 315.4 | 322.1 |
| 300 | 316.2 | 323.5 | 316.2 | 322.6 |
| 400 | 318.0 | 324.2 | 318.0 | 324.3 |
| 500 | 318.5 | 325.6 | 318.5 | 325.7 |
| 600 | 319.2 | 326.2 | 319.2 | 326.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Dong, S.; Wang, J.; Li, Y. Temperature Dynamic Characteristics Analysis and Thermal Load Dissipation Assessment for Airliner Hydraulic System in a Full Flight Mission Profile. Machines 2022, 10, 258. https://doi.org/10.3390/machines10040258
Li D, Dong S, Wang J, Li Y. Temperature Dynamic Characteristics Analysis and Thermal Load Dissipation Assessment for Airliner Hydraulic System in a Full Flight Mission Profile. Machines. 2022; 10(4):258. https://doi.org/10.3390/machines10040258
Chicago/Turabian StyleLi, Dong, Sujun Dong, Jun Wang, and Yunhua Li. 2022. "Temperature Dynamic Characteristics Analysis and Thermal Load Dissipation Assessment for Airliner Hydraulic System in a Full Flight Mission Profile" Machines 10, no. 4: 258. https://doi.org/10.3390/machines10040258
APA StyleLi, D., Dong, S., Wang, J., & Li, Y. (2022). Temperature Dynamic Characteristics Analysis and Thermal Load Dissipation Assessment for Airliner Hydraulic System in a Full Flight Mission Profile. Machines, 10(4), 258. https://doi.org/10.3390/machines10040258







