Study on Mechanism of Roundness Improvement by the Internal Magnetic Abrasive Finishing Process Using Magnetic Machining Tool
Abstract
1. Introduction
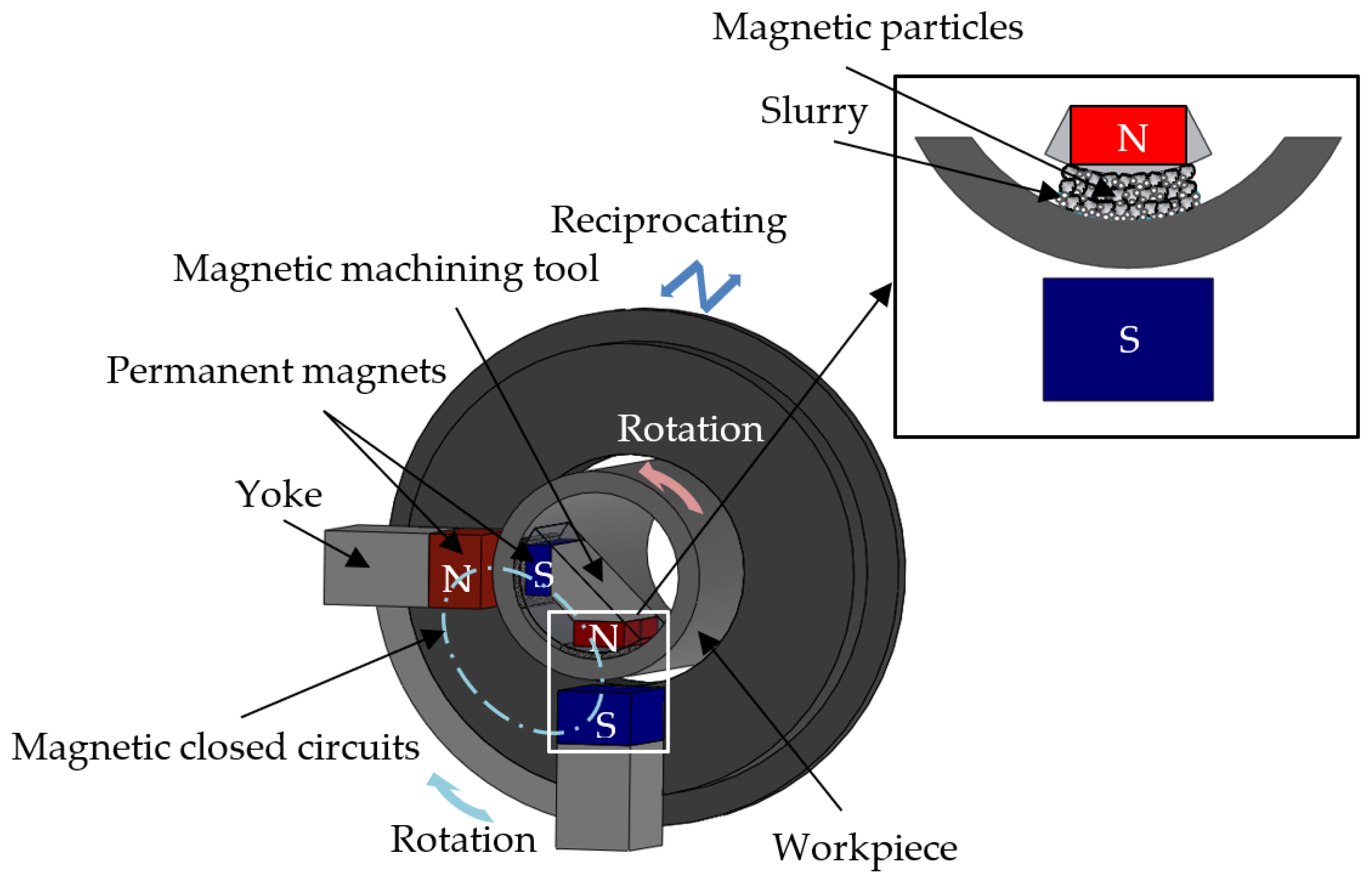
2. Processing Principle
3. Mechanism of Roundness Improvement
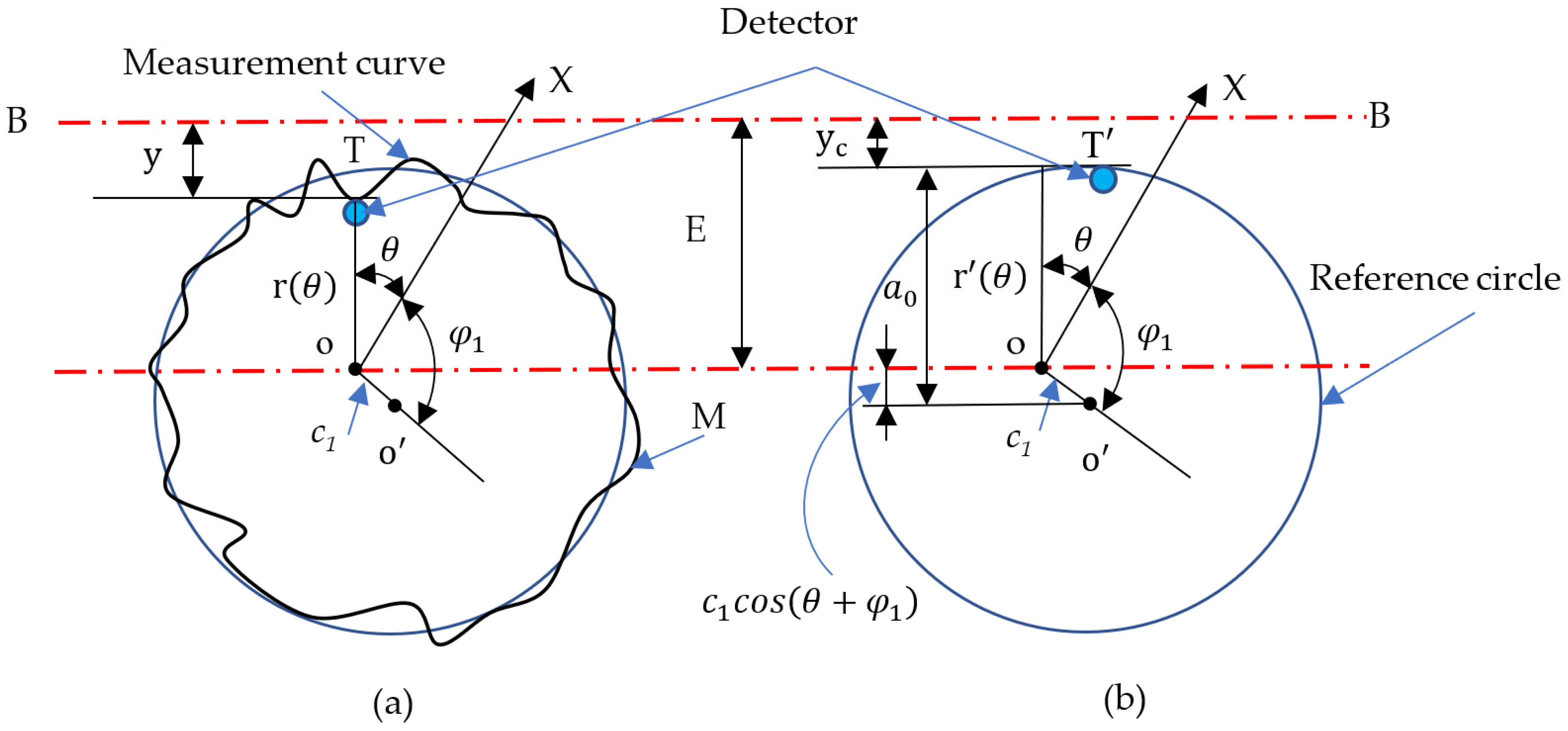
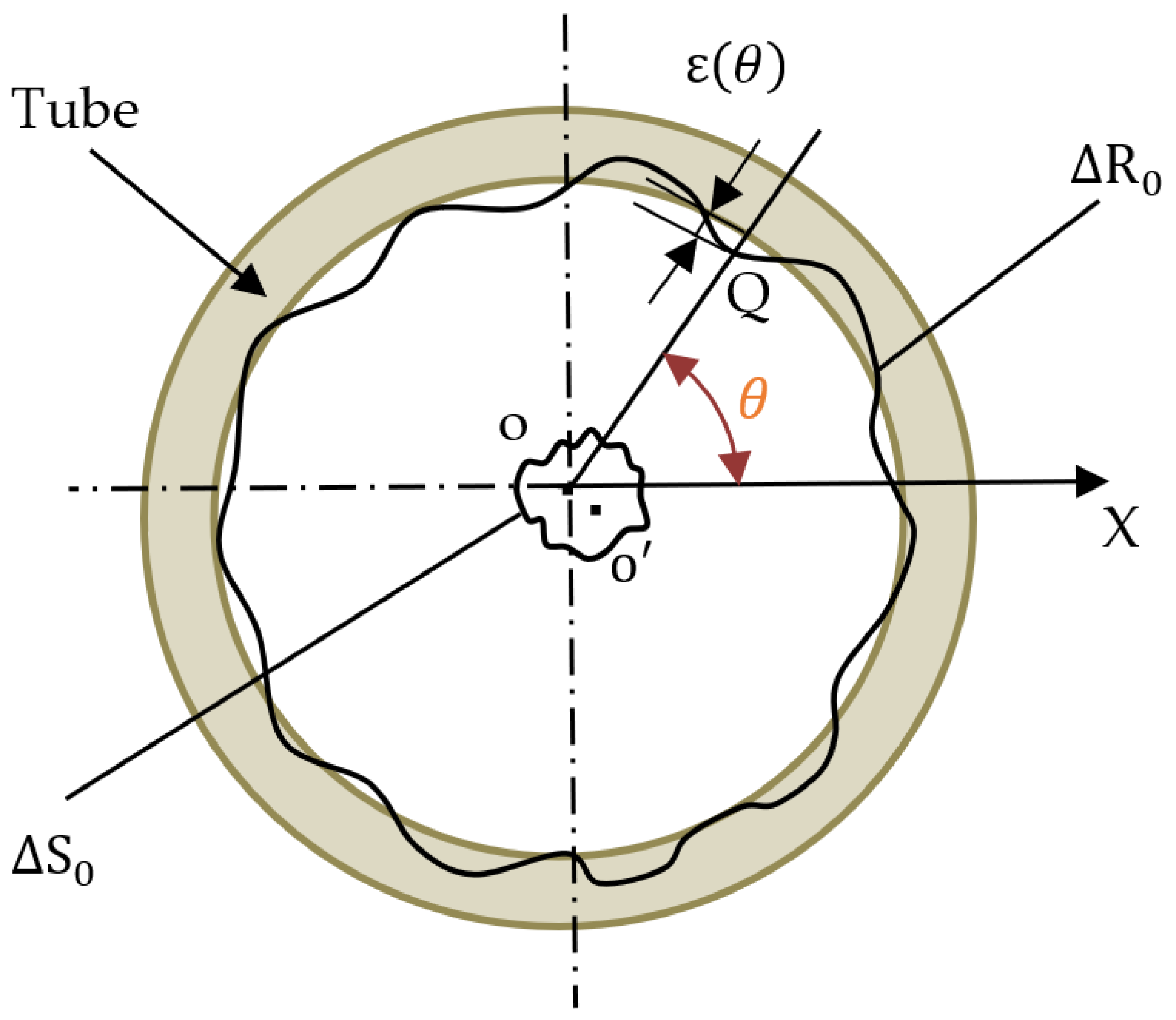
3.1. Fourier Series Expression of Roundness Curve
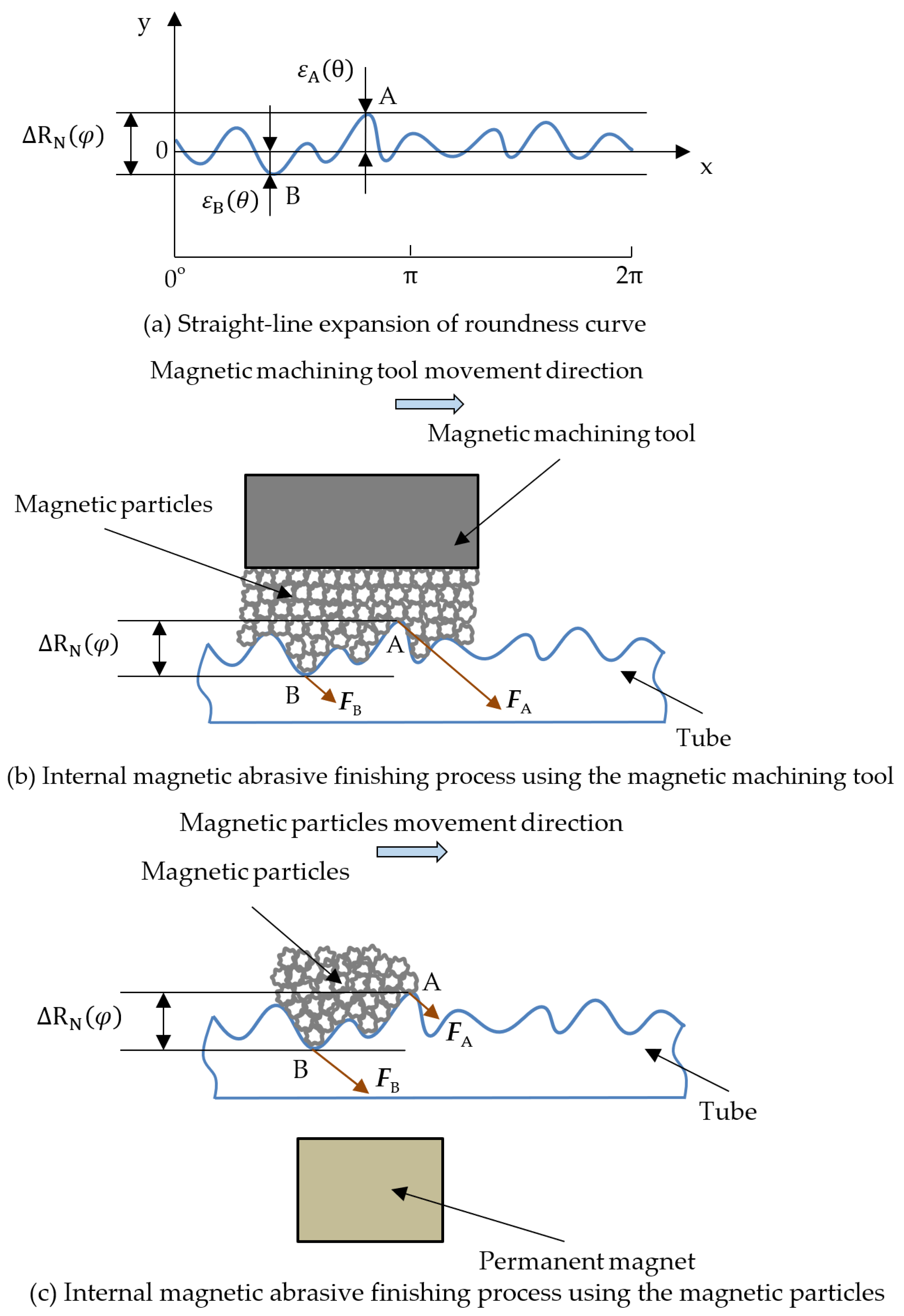
3.2. The Changing Process of Roundness
3.3. Analysis of the Influencing Factors of Roundness Improvement
3.3.1. Discussion on the Influence of Eccentricity
3.3.2. Discussion on the Influence of Finishing Force
4. The Experimental Verification of SUS304 Stainless Steel Tube
4.1. Experimental Setup and Conditions
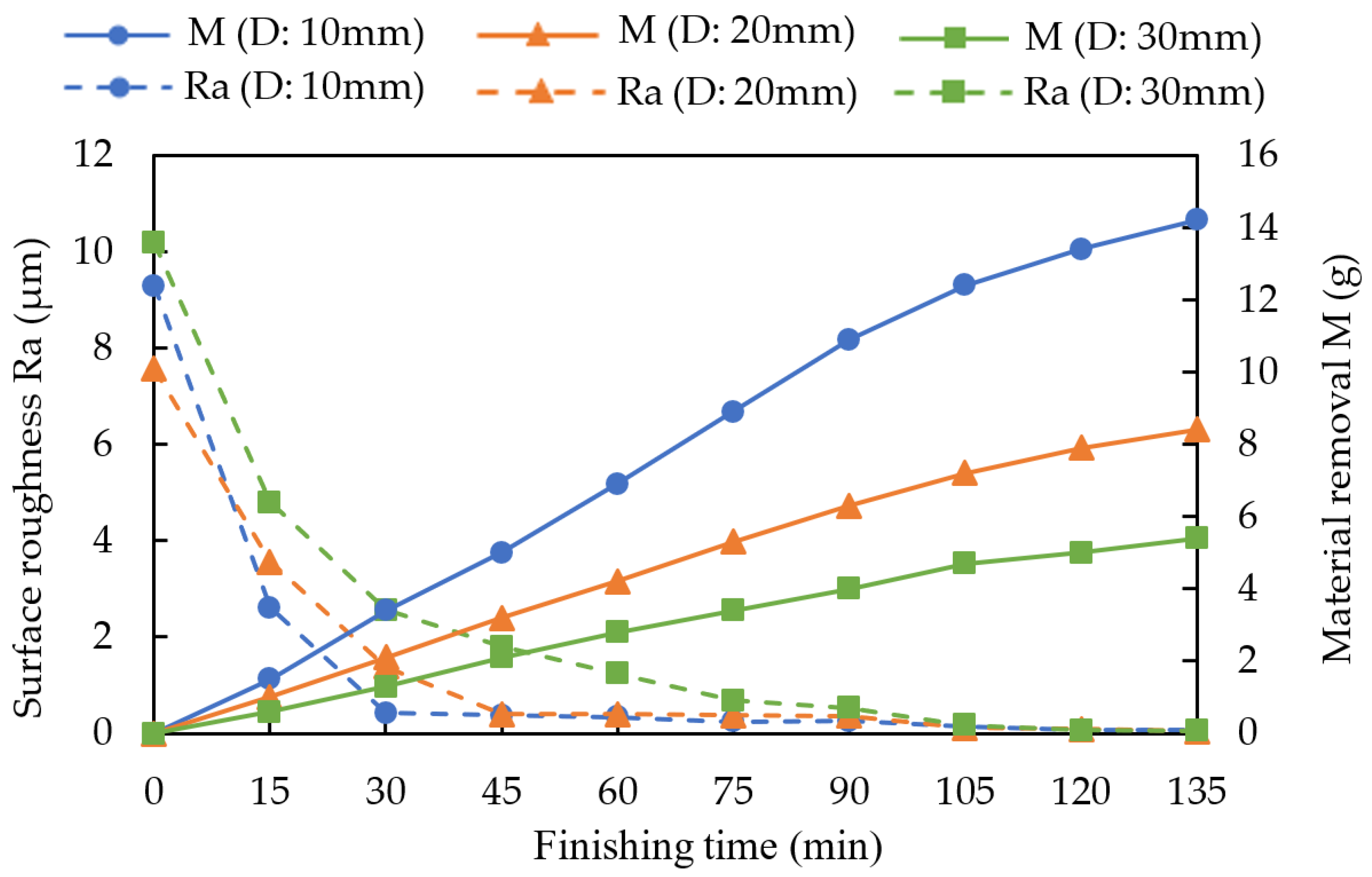
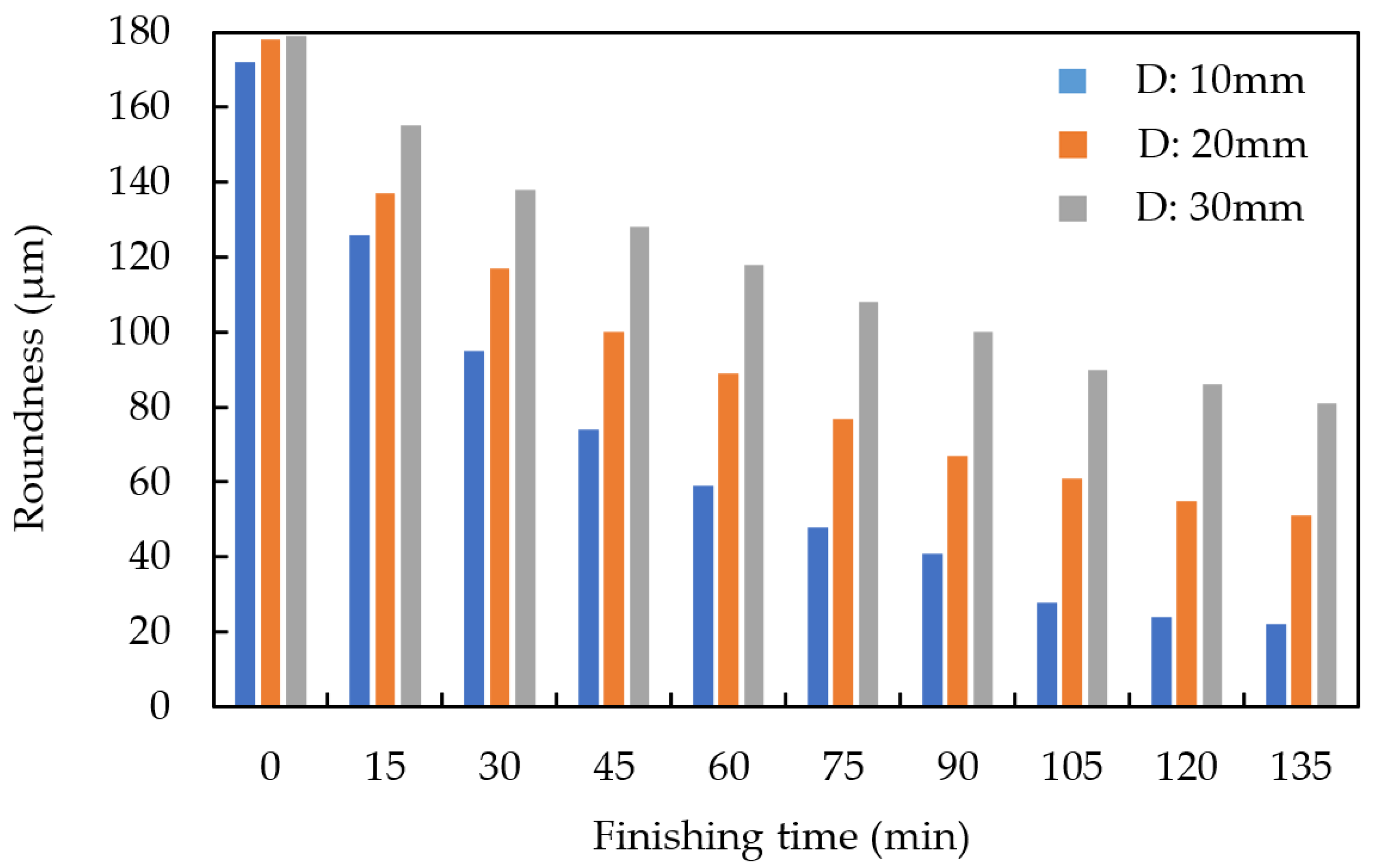
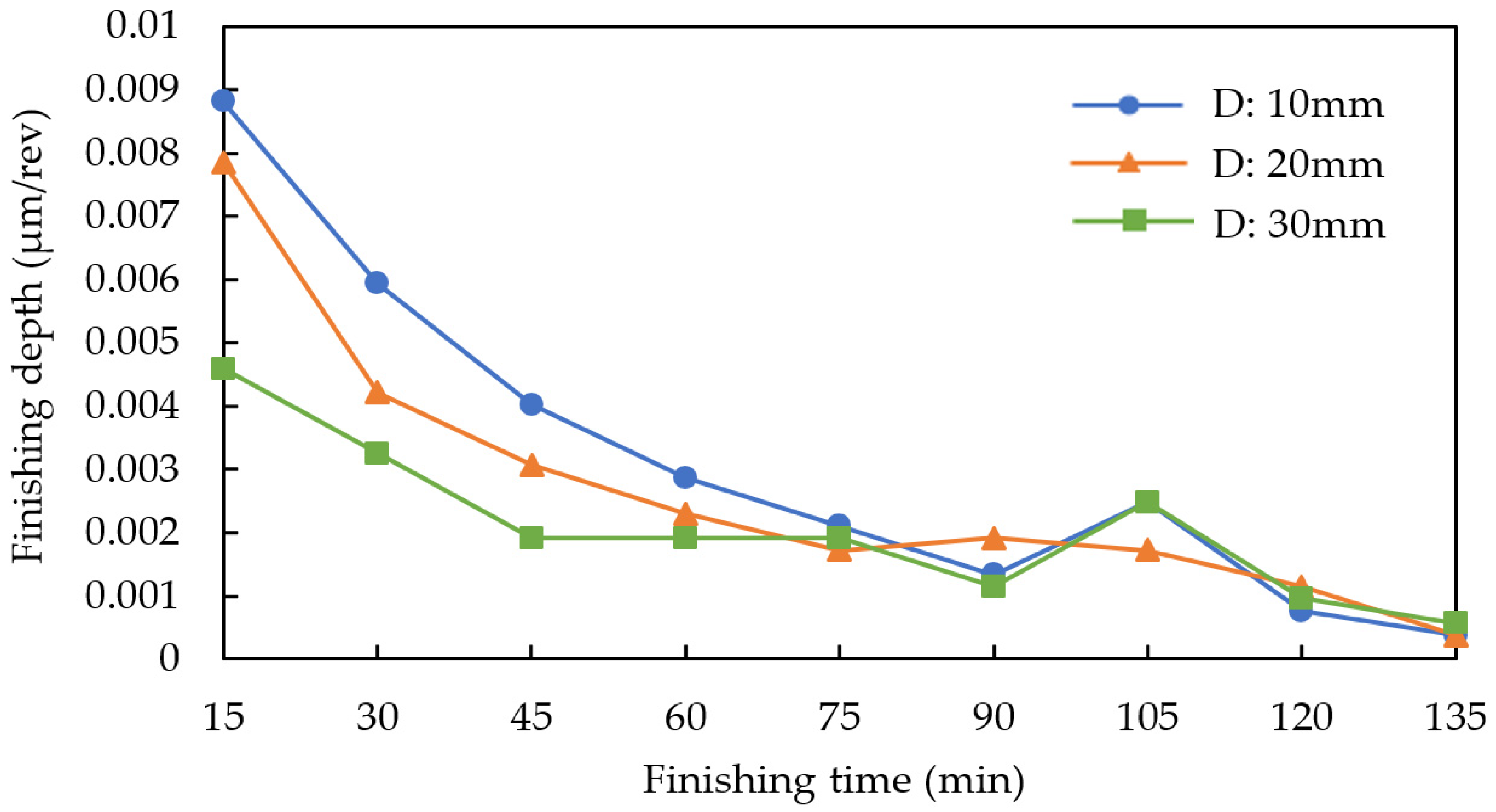
4.2. Experimental Results and Discussion
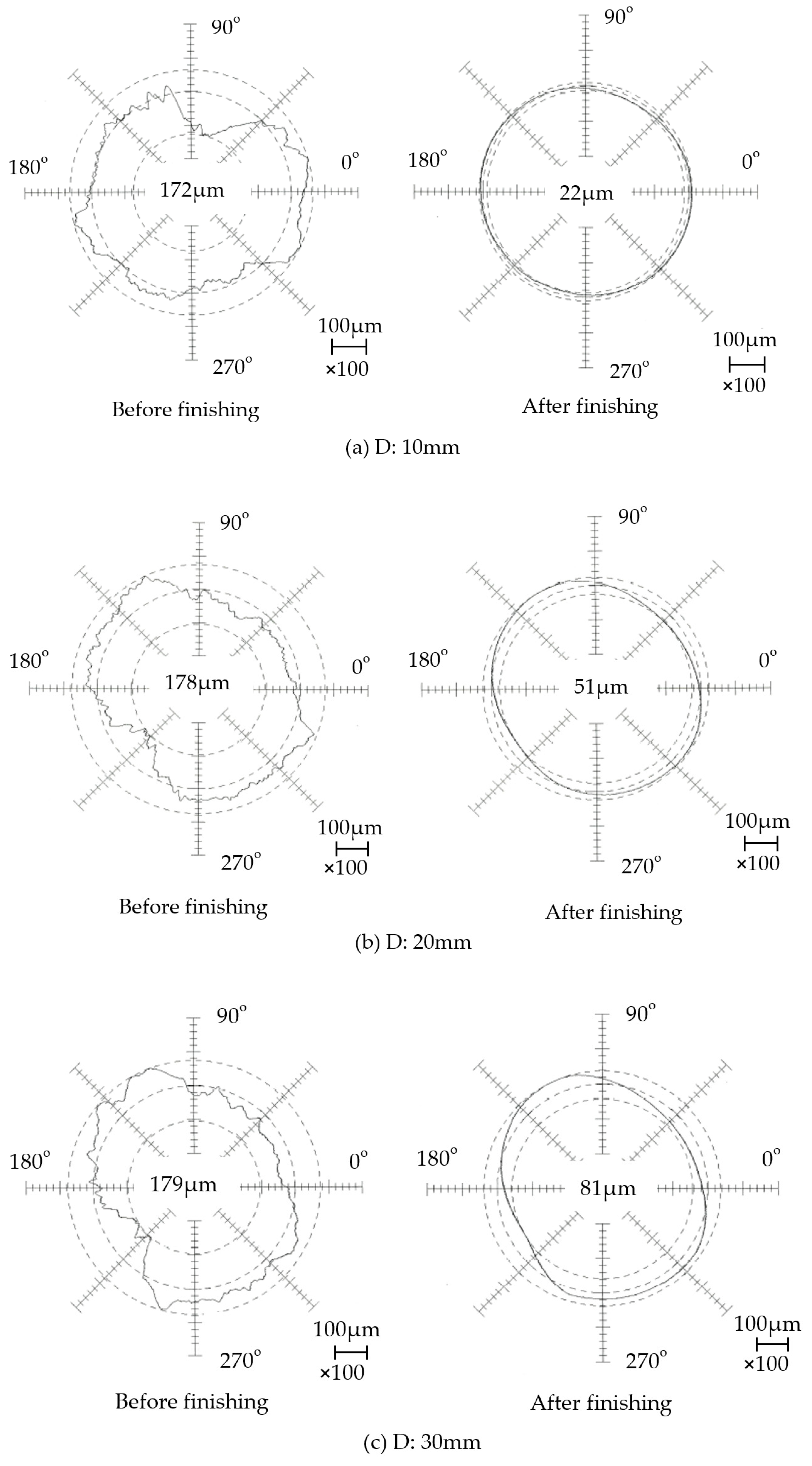
4.3. Confirmation of Mechanism Analysis Results and Experimental Results
5. Conclusions
- The mechanism of roundness improvement was analyzed theoretically. The roundness curve expression was derived using the principle of roundness measurement by assumed center method and the expression of the roundness curve expanded by Fourier series was obtained.
- The factors influencing roundness improvement—eccentricity and finishing force—were discussed. When the workpiece is clamped at both ends, the influence of eccentricity can be ignored. Moreover, it is clear that the magnetic abrasive finishing process using the magnetic machining tool was effective in improving the roundness of the internal surface of the thick tubes.
- Confirming the mechanism analysis results and the experimental results, it can be concluded that the internal magnetic abrasive finishing process using the magnetic machining tool was effective for improving the roundness of thick tubes with a thickness from 10 mm to 30 mm. As the thickness of the tube increased, the finishing force decreased, causing the roundness improvement to decrease. For different tube thicknesses, the roundness was improved from 172 µm to 22 µm in the case of 10 mm, from 178 µm to 51 µm in the case of 20 mm, and from 179 µm to 81 µm in the case of 30 mm.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zou, Y.H.; Shinmura, T.; Wang, F. Study on a magnetic deburring method by the application of the plane magnetic abrasive machining process. Adv. Mater. Res. 2009, 76–78, 276–281. [Google Scholar] [CrossRef]
- Jain, V.K. Magnetic field assisted abrasive based micro-/nano-finishing. J. Mater. Process. Technol. 2009, 209, 6022–6038. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Shinmura, T. Internal finishing process for alumina ceramic components by a magnetic field assisted finishing process. Precis. Eng. 2004, 28, 135–142. [Google Scholar] [CrossRef]
- Shinmura, T.; Takazawa, K.; Hatano, E.; Matsunaga, M.; Matsuo, T. Study on magnetic abrasive finishing. Ann. CIRP 1990, 39, 325–328. [Google Scholar] [CrossRef]
- Shinmura, T.; Yamaguchi, H. Study on a new internal finishing process by the application of magnetic abrasive machining: Internal finishing of stainless steel tube and clean gas bomb. JSME Int. J. Ser. C 1995, 38, 798–804. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Shinmura, T.; Kobayashi, A. Development of an internal magnetic abrasive finishing process for nonferromagnetic complex shaped tubes. JSME Int. J. Ser. C 2001, 44, 275–281. [Google Scholar] [CrossRef]
- Kang, J.; Yamaguchi, H. Internal finishing of capillary tubes by magnetic abrasive finishing using a multiple pole-tip system. Precis. Eng. 2012, 36, 510–516. [Google Scholar] [CrossRef]
- Kang, J.; George, A.; Yamaguchi, H. High-speed internal finishing of capillary tubes by magnetic abrasive finishing. Procedia CIRP 2012, 1, 414–418. [Google Scholar] [CrossRef]
- Jha, S.; Jain, V.K. Design and development of the magnetorheological abrasive flow finishing (MRAFF) process. Int. J. Mach. Tools Manuf. 2004, 44, 1019–1029. [Google Scholar] [CrossRef]
- Jha, S.; Jain, V.K.; Komanduri, R. Effect of extrusion pressure and number of finishing cycles on surface roughness in magnetorheological abrasive flow finishing (MRAFF) process. Int. J. Adv. Manuf. Technol. 2007, 33, 725–729. [Google Scholar] [CrossRef]
- Das, M.; Jain, V.K.; Ghoshdastidar, P.S. Analysis of magnetorheological abrasive flow finishing (MRAFF) process. Int. J. Adv. Manuf. Technol. 2007, 38, 613–621. [Google Scholar] [CrossRef]
- Kim, J. Polishing of ultra-clean inner surfaces using magnetic force. Int. J. Adv. Manuf. Technol. 2003, 21, 91–97. [Google Scholar] [CrossRef]
- Kim, J.; Choi, M. Stochastic approach to experimental analysis of cylindrical lapping process. Int. J. Mach. Tools Manuf. 1995, 35, 51–59. [Google Scholar] [CrossRef]
- Chen, K.Y.; Tu, T.Y.; Fan, Y.H.; Wang, A.C.; Fu, P.K. Study on the polishing characteristics of the rotating cylinder-based magnetic gel abrasive finishing. Processes 2021, 9, 1794. [Google Scholar] [CrossRef]
- Cheng, K.C.; Chen, K.Y.; Tsui, H.P.; Wang, A.C. Characteristics of the polishing effects for the stainless tubes in magnetic finishing with gel abrasive. Processes 2021, 9, 1561. [Google Scholar] [CrossRef]
- Zou, Y.H.; Shinmura, T. A study on the magnetic field assisted machining process for internal finishing using a magnetic machining jig. Key Eng. Mater. 2004, 257–258, 505–510. [Google Scholar] [CrossRef]
- Muhamad, M.R.; Zou, Y.H.; Sugiyama, H. Development of a new internal finishing of tube by magnetic abrasive finishing process combined with electrochemical machining. Int. J. Mech. Eng. Appl. 2015, 3, 22–29. [Google Scholar] [CrossRef][Green Version]
- González, H.; Calleja, A.; Pereira, O.; Ortega, N.; López de Lacalle, L.N.; Barton, M. Super abrasive machining of integral rotary components using grinding flank tools. Metals 2018, 8, 24. [Google Scholar] [CrossRef]
- Rodriguez, A.; López de Lacalle, L.N.; Pereira1, O.; Fernandez, A.; Ayesta, I. Isotropic finishing of austempered iron casting cylindrical parts by roller burnishing. Int. J. Adv. Manuf. Technol. 2020, 110, 753–761. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Shinmura, T. Study of an internal magnetic abrasive finishing using a pole rotation system: Discussion of the characteristic abrasive behavior. Precis. Eng. 2000, 24, 237–244. [Google Scholar] [CrossRef]
- Zou, Y.H.; Shinmura, T. Mechanism of a magnetic field assisted finishing process using a magnetic machining jig. Key Eng. Mater. 2007, 339, 106–113. [Google Scholar] [CrossRef]
- Zou, Y.H.; Shinmura, T. Development of magnetic field assisted machining process using magnetic machining jig. JSME Int. J. Ser. C 2002, 68, 233–239. (In Japanese) [Google Scholar] [CrossRef]
- Nakata, K. Engineering Analysis: A Mathematical Approach for Engineers, 1st ed.; Ohm Press: Saitama, Japan, 1972; pp. 79–84. [Google Scholar]
- Shinmura, T.; Takazawa, K.; Hatano, E. Study on magnetic abrasive finishing: Rounding condition and its confirmation by experiment. Bull. Jpn. Soc. Precis. Eng. 1986, 52, 1598–1603. (In Japanese) [Google Scholar] [CrossRef]
- Shinmura, T.; Aizawa, T. Study on Internal Finishing of a Non-ferromagnetic Tubing by Magnetic Abrasive Machining Process. Bull. Jpn. Soc. Precis. Eng. 1989, 23, 37–41. (In Japanese) [Google Scholar]
- Nakano, K. Precision Shape Measurement Practice: Interpretation of Geometric Tolerances and Measurement of Geometric Deviations, 1st ed.; Kaibundo Publishing Co., Ltd.: Tokyo, Japan, 1992; pp. 61–63. [Google Scholar]
- Yamaguchi, H.; Shinmura, T. Study on a new internal finishing process by the application of magnetic abrasive machining: Discussion of the roundness. Bull. Jpn. Soc. Precis. Eng. 1996, 62, 1617–1621. (In Japanese) [Google Scholar] [CrossRef]


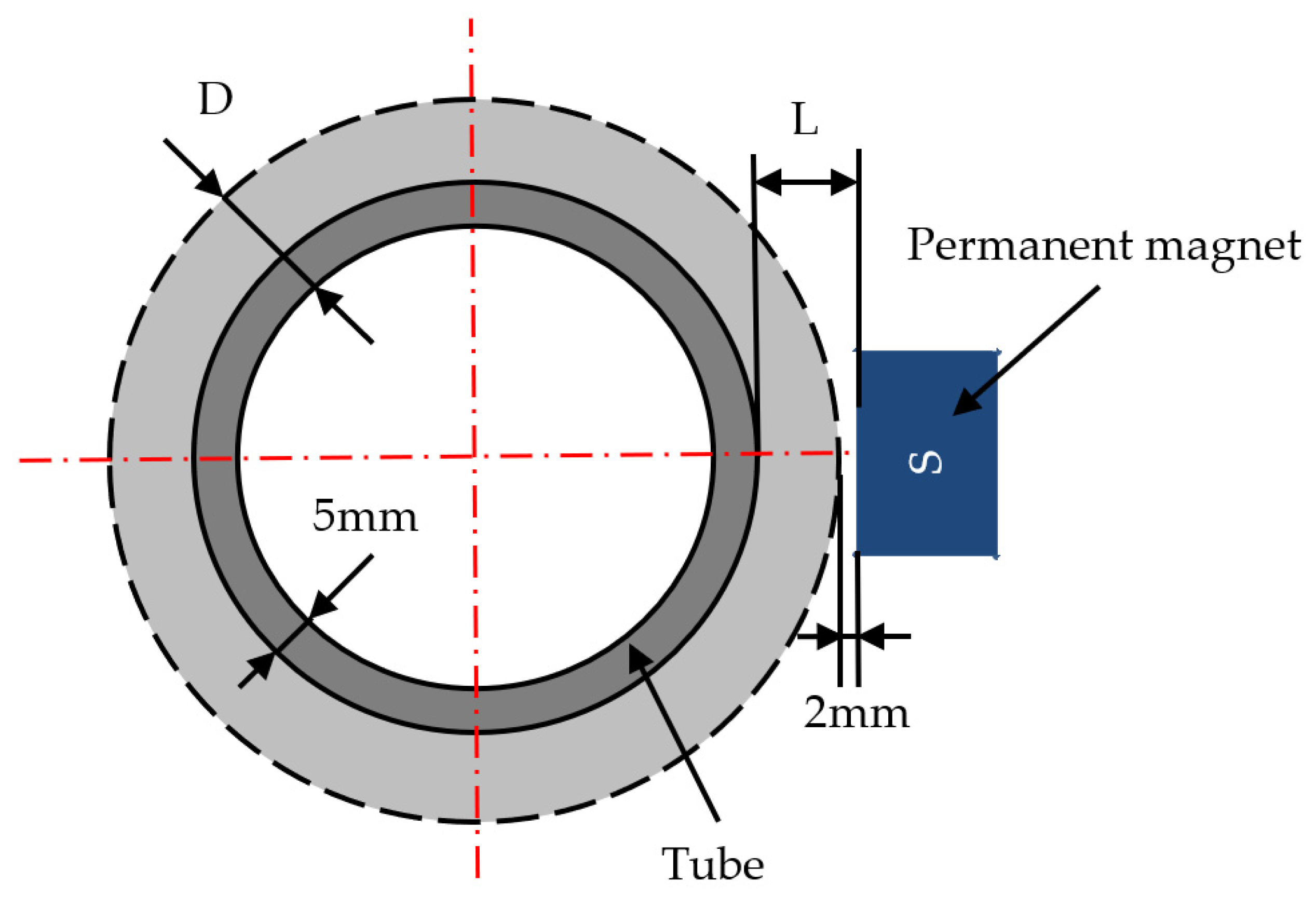
| Parameters | Conditions | |
|---|---|---|
| Workpiece | SUS304 stainless steel tube Ø89.1 × 79.1 × 200 mm Rotational speed: 162 min−1 | |
| Magnetic machining tool | Magnet: Nd-Fe-B rare earth permanent magnet Yoke: SS400 steel Molding material: Polymer | |
| Finishing unit | Magnet: Ferrite magnet 50 × 35 × 26 mm Yoke: SS400 steel Rotational speed: 186 min−1 Reciprocating speed: 1500 mm/min | |
| Magnetic particles | Electrolytic iron particles: 1680 µm in mean dia., 24 g | |
| Abrasive particles | 1st-stage (15 × 6 min) | WA #400, 2.5 g |
| 2nd-stage (15 min) | WA #3000, 2.5 g | |
| 3rd-stage (15 min) | WA #6000, 2.5 g | |
| 4th-stage (15 min) | WA #10000, 2.5 g | |
| Grinding fluid | Water-soluble grinding fluid (SCP-23): 30 g | |
| Thickness of tube (D) | 10 mm, 20 mm, 30 mm | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Zou, Y. Study on Mechanism of Roundness Improvement by the Internal Magnetic Abrasive Finishing Process Using Magnetic Machining Tool. Machines 2022, 10, 112. https://doi.org/10.3390/machines10020112
Liu J, Zou Y. Study on Mechanism of Roundness Improvement by the Internal Magnetic Abrasive Finishing Process Using Magnetic Machining Tool. Machines. 2022; 10(2):112. https://doi.org/10.3390/machines10020112
Chicago/Turabian StyleLiu, Jiangnan, and Yanhua Zou. 2022. "Study on Mechanism of Roundness Improvement by the Internal Magnetic Abrasive Finishing Process Using Magnetic Machining Tool" Machines 10, no. 2: 112. https://doi.org/10.3390/machines10020112
APA StyleLiu, J., & Zou, Y. (2022). Study on Mechanism of Roundness Improvement by the Internal Magnetic Abrasive Finishing Process Using Magnetic Machining Tool. Machines, 10(2), 112. https://doi.org/10.3390/machines10020112







