On the Phenomenology of an Accelerated Large-Scale Universe

Abstract

In this review paper, several new results towards the explanation of the accelerated expansion of the large-scale universe is discussed. On the other hand, inflation is the early-time accelerated era and the universe is symmetric in the sense of accelerated expansion. The accelerated expansion of is one of the long standing problems in modern cosmology, and physics in general. There are several well defined approaches to solve this problem. One of them is an assumption concerning the existence of dark energy in recent universe. It is believed that dark energy is responsible for antigravity, while dark matter has gravitational nature and is responsible, in general, for structure formation. A different approach is an appropriate modification of general relativity including, for instance, $f(R)$ and $f(T)$ theories of gravity. On the other hand, attempts to build theories of quantum gravity and assumptions about existence of extra dimensions, possible variability of the gravitational constant and the speed of the light (among others), provide interesting modifications of general relativity applicable to problems of modern cosmology, too. In particular, here two groups of cosmological models are discussed. In the first group the problem of the accelerated expansion of large-scale universe is discussed involving a new idea, named the varying ghost dark energy. On the other hand, the second group contains cosmological models addressed to the same problem involving either new parameterizations of the equation of state parameter of dark energy (like varying polytropic gas), or nonlinear interactions between dark energy and dark matter. Moreover, for cosmological models involving varying ghost dark energy, massless particle creation in appropriate radiation dominated universe (when the background dynamics is due to general relativity) is demonstrated as well. Exploring the nature of the accelerated expansion of the large-scale universe involving generalized holographic dark energy model with a specific Nojiri-Odintsov cut-off is presented to finalize the paper.

1. Introduction

The accelerated expansion of the large-scale universe (LSU) is one of the long standing problems of physics [1,2,3,4]. In this case according to recent understanding of symmetries and physics of LSU, dark energy (DE) (≈70%) should be used to provide acceleration to expanding universe [5,6]. On the other hand, DE is not enough and according to astrophysical data, dark matter (DM) should be involved in the energy budget of universe [7,8]. Various energy conditions have been developed in order to distinguish DE and DM (

Table 1. Four energy conditions used in modern cosmology.

The Energy Condition	Mathematical Expression
Null energy condition (NEC)	$\rho +P\ge 0$
Dominant energy condition (DEC)	$\rho \ge 0\mathrm{and}\rho \pm P\ge 0$
Strong energy condition (SEC)	$\rho +3P\ge 0\mathrm{and}\rho +P\ge 0$
Weak energy condition (WEC)	$\rho \ge 0\mathrm{and}\rho +P\ge 0$

) according to known symmetries of LSU. Introduction of these ideas lunched a search of the correct candidates for both of them: DE breaks Strong Energy Condition, dominates DM in LSU giving accelerated expansion and preserves symmetries.

Since the subject of this review is directly related to accelerated expansion of LSU and DE, any future discussion on topics related to DM problem will be suppressed in a proper way. Moreover, any additional disscusion on inflation (accelerated expansion of early universe) will be suppressed alike [9,10,11,12,13,14] (and references therein). Cosmological constant (CC) is the first and the simplest model of DE ever suggested and used in modern cosmology. The minimal model of modern cosmology is ΛCDM concordance model, with Λ CC and non-relativistic pressureless cold dark matter (CDM). In this model the dynamics of the background is described by the field equations of GR. However, mainly two problems arise discussed in recent scientific literature very intensively with the CC model of DE [15,16,17]. In particular, the fine-tuning problem indicates the absence of a fundamental mechanism, which sets the CC to zero or to a very small value (in some sense violating symmetry). The second problem is the cosmological coincidence problem (CCP) with the question about comparability of the the densities of DE and DM in LSU.

In order to solve the mentioned problems various models of dynamical DE models have been introduced without violating/recovering symmetries of LSU [5,6]. Among DE models are quintessence, k-essence, tachyonic, phantom and quinton DE models, as well as dark fluids like Chaplygin gas (and its modifications), van der Waals gas and polytropic gas involving also viscosity [18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47,48] and references therein. In

Table 2. The values of EoS parameter ω allowing one to have various forms of the matter in the universe due to the barotropic fluid.

EoS Parameter	Matter
$\omega =0$	dust
$\omega =1/3$	radiation
$\omega \in (1/3,1)$	hard universe
$\omega =1$	stiff matter
$\omega \in (-1/3,-1)$	quintessence
$\omega =-1$	cosmological constant
$\omega <-1$	phantom matter
$\omega >1$	ekpyrotic matter

the type of the fluids which can be modeled using equation of state parameter (EoS) of the barotropic fluid: $P=\omega \rho$ are summarized. In scientific literature an active discussion on possible modification of this simple EoS are held. In general, one can consider dark fluids with more general EoS, like [42,43]

$$P_{DE}=P({\rho }_{DE},H,\dot{H}),$$

(1)

and some explicit examples of dark fluids of this type are [42,43]

$$P=-\rho -A{\rho }^{\alpha }-B{H}^{2\beta },$$

(2)

and

$$P={\omega }_f\rho +{\omega }_H{H}^2+{\omega }_{dH}\dot{H}.$$

(3)

Another interesting approach in this direction is, for instance, consideration of an implicit relation between P, ρ and H [42,43]

$$F(P,\rho ,H)=0.$$

(4)

One of the alternative models of DE is holographic DE based on the holographic principle which establishes a link between the ultraviolet (UV) cut-off, defined through ${\rho }_{\mathrm{\Lambda }}$, and the infrared (IR) cut-off. In scientific literature different options for IR cut-off like Hubble horizon, particle horizon, event horizon, Ricci scale or generalized IR cut-off, among many others, have been considered. The starting point of holographic models is an expression for DE energy density from which EoS is then derived. There is an active discussion on various modifications of this idea (to mention a few) including modified holographic Ricci DE

$$\rho =\frac{2}{\xi -\eta }\left(\dot{H}+\frac{3\xi }{2}{H}^2\right),$$

(5)

extended holographic Ricci DE

$$\rho =3M_{Pl}^2(\xi H^2+\eta \dot{H}),$$

(6)

ξ and η are free constants. Another option which can play the role of dynamical DE is the so called Veneziano ghost DE with the following energy density

$$\rho =\alpha H+\beta H^2,$$

(7)

where α and β are the constants of the model. Moreover, discussed models of holographic dark energy models belongs to generalization considered in Reference [24] which allows us to unify symmetry in early universe and LSU in sense of accelerated expansion.

In recent scientific literature there is an active interest towards in interacting DE models, which is a non-gravitational coupling between DE and DM revealing interesting symmetry [49] (and references therein). There are various forms of linear and non linear forms of interactions considered in modern cosmology. There are various modifications of considered interactions. Some of the examples (phenomenological) of non-gravitational interaction are

$$Q=3Hbq\rho ,$$

(8)

$$Q=3Hb\rho ,$$

(9)

and

$$Q=3Hb\frac{{\rho }_{de}{\rho }_{dm}}{{\rho }_{de}+{\rho }_{dm}},$$

(10)

On the other hand, it has been demonstrated successfully, that to solve the problems of modern cosmology and LSU, one can modify GR [50,51] (and references therein). The key ingredient of the modification of GR is related with an appearing of a term in the field equations, which is in a naive way eventually associated with DE. Various modifications of GR exist involving, for instance, extra dimensions, variable gravitational constant and the speed of light. Moreover, scalar-tensor, tensor-vector-scalar, scalar-tensor-vector theories (to mention a few) very actively studied to answer open problems in modern cosmology can be involved. Toy models as Horava-Lifschitz gravity proposed for quantum gravity, Brans-Dicke theory and particle physics theories such as Kaluza-Klein theory (an attempt to unify gravity and electrodynamics), also can be involved. To finalize the introductory part of this review one would like to mention about geometrical tools like statefinder analysis with $(r,s)$ parameters, $Om$, $({\omega }_{de},{\omega }^{\prime })$ and statefinder hierarchy analysis designed to study DE and cosmological models [52,53,54,55,56]. For instance, $Om$ analysis suggests to study the following parameter

$$Om=\frac{x^2-1}{{(1+z)}^3-1},$$

(11)

where $x=H/H_0$, $H_0$ it is the value of the Hubble parameter at $z=0$ (for the definition of the other parameters and tools one refers to Appendix A). On the other hand, there is an active discussion on the models via phase space analysis and providing the thermodynamics of the models is another interesting approach relevant to the study of the models.

The goal of this paper is to present various novel results concerning to the solution of accelerated expansion of LSU. There are two groups of the models discussed here. In case of the first group of the models, GR has been considered as the theory to describe the background dynamics of the universe. However, considered cosmological models involve new phenomenological modifications of the ghost DE to explain the accelerated expansion of LSU. The study of massless particle creation in appropriate radiation dominated universe is also had been carried out. On the other hand, in the second group of the models, one has gathered various cosmological models either involving new forms of the nonlinear interactions between dark components, or new parameterization of EoS of the DE. It should be mentioned that in the considered models either mentioned cosmological problems are solved due to the existence of interaction, or the problems do not rise at all.

The paper is organized as follows: In Section 2, new cosmological models involving various models of the varying ghost dark energy allowing to explain the accelerated expansion of LSU universe are discussed. Considered models are able to explain the accelerated expansion of LSU, transition between decelerated expanding universe and accelerated expanding universe. Moreover, in these models CCP either is solved, or does not observe at all. Moreover, massless particle creation has been demonstrated for appropriate radiation dominated expanding universe, which evolves to LSU containing suggested interacting varying ghost DE models. In Section 3, discussion is about cosmological models which involve either new forms of interaction, or new parameterizations of EoS of the DE (dark fluid) including holographic DE model with specific model of Nojiri-Odintsov cut-off. In Section 3.3, we summarize the discussed material.

2. Varying Ghost Sark Energy Models

The ghost DE can be used to explain accelerated expansion of LSU. New phenomenological modifications of the ghost DE named as varying ghost DE have been suggested recently. The first model tries to operate on the existence of some dynamics between the ghost DE and a fluid making sense of the proposed effect [57]. On the other hand, the second model of the varying ghost DE has been considered and obtained results are presented in Section 2.1 corresponding to Reference [58]. For the other two models of the varying ghost DE presented in this section (Section 2.2 and Section 2.3), possible relationship between the energy density of the DM and the energy density of the ghost DE has been considered [59,60] having an aim to find possible origin of DE from an interplay with DM and geometry. Detailed presentation of the forms of the varying ghost DE models has been given in appropriate subsections completing assumptions which have been used to construct cosmological models for LSU. Within appropriate cosmographic analysis and constraints from observational data demonstrated applicability of considered cosmological models to the problems of LSU. Moreover, in case of two cosmological models (for the cosmological model presented in Section 2.3 this question has been left as a subject for future discussion) having the background dynamics given according to GR, massless particle creation possibility has been demonstrated.

Many authors had discussed the physics of the matter creation believing that particles are created because the positive and negative frequency parts of the fields become mixed during expansion of universe [61,62,63,64,65,66,67,68,69,70,71,72,73,74,75,76,77,78,79]. If one considers GR, then there is no creation of massless particles (no photon, graviton or any other kind of massless particles) in a radiation dominated universe due to conformal invariance of the metric. However, recently in case of modified theories of gravity, in particular, in case of $f(R)$ theory of gravity massless particle creation has been reported [80,81,82]. The study of particle creation problem is complicated, since exists the problem of the definition of the particles and the vacuum states. However, the problem may be solved by a Bogoliubov transformation and with a suitable boundary condition the total number of created particles $N_k$ and antiparticles $\overline{N_k}$ reads as [80] (and references therein)

$$N_k=\overline{N_k}=|{\beta }_k{(\eta )|}^2=\frac{1}{4{\omega }_k(\eta )}|{\chi }_k^{\prime }{|}^2+\frac{{\omega }_k(\eta )}{4}{|{\chi }_k|}^2-\frac{1}{2},$$

(12)

where

$${\omega }^2(\eta )=k^2+m^2{a}^2(\eta )-\frac{a^{″}(\eta )}{a(\eta )},$$

(13)

${\omega }_k$ represents the frequency of the particle and k is the Fourier mode or wavenumber of the particle, while η it is the conformal time which is related to the physical time with the relation

$$\eta =\int \frac{dt}{a},$$

(14)

with ${}^{\prime }$ denotes the derivative with respect to the conformal time η, while the equation of motion for the decoupled modes ${\chi }_k(\eta )$ is a second order differential equation. More details for this issue can be found in Reference [80]. As the last step before starting the discussion of the results one would like to remind that to describe the background dynamics of LSU in scope of GR, which contains radiation ($P_r=(1/3){\rho }_r$), the varying ghost DE coupled to the pressureless DM via an interaction, the following set of equations should be considered (throughout this section geometrical units are considered, i.e., $8\pi G=c=1$.)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}(\rho +3P),$$

(15)

$${\dot{\rho }}_r+4H{\rho }_r=0,$$

(16)

$${\dot{\rho }}_{de}+3H({\rho }_{de}+P_{de})=-Q,$$

(17)

$${\dot{\rho }}_{dm}+3H{\rho }_{dm}=Q.$$

(18)

Cosmographic and appropriate study of additional questions concerning considered varying ghost DE models are presented in appropriate three subsections of this section.

2.1. Cosmological Model with ${\rho }_{de}=\alpha H+\beta a^m{H}^2$

The varying ghost DE has been suggested recently assuming that one of the parameters to determine the energy density of the ghost DE could be a varying quantity. In particular, one of the models of the varying ghost DE has the following energy density [57]

$${\rho }_{de}={\alpha }_0{a}^{m}H+\widehat{\beta }{H}^2,$$

(19)

instead of

$${\rho }_{de}=\alpha H+\widehat{\beta }{H}^2,$$

(20)

where ${\alpha }_0$, $\widehat{\beta }$ and m are constants and should be determined from observational data. The next model considered in Reference [58] has the following form

$${\rho }_{de}=\alpha H+\beta a^m{H}^2.$$

(21)

It is easy to see, that the parameterization is according to the scale factor of universe in the form of the power law. On the other hand, to gain a comprehensive understanding of the background dynamics the author of Reference [58] assumed an existence of interaction between DE and DM and considered the following form of the interaction

$$Q=3Hb\rho .$$

(22)

In Equation (22) ρ is the total energy density of the effective dark fluid consisting of considered varying ghost DE and DM, while b is a constant. The first goal is to discuss theoretical results corresponding to the cosmographic analysis of this model for appropriate constraints on the parameters of the model. Obtained results will be used to study particle creation in this toy cosmological model.

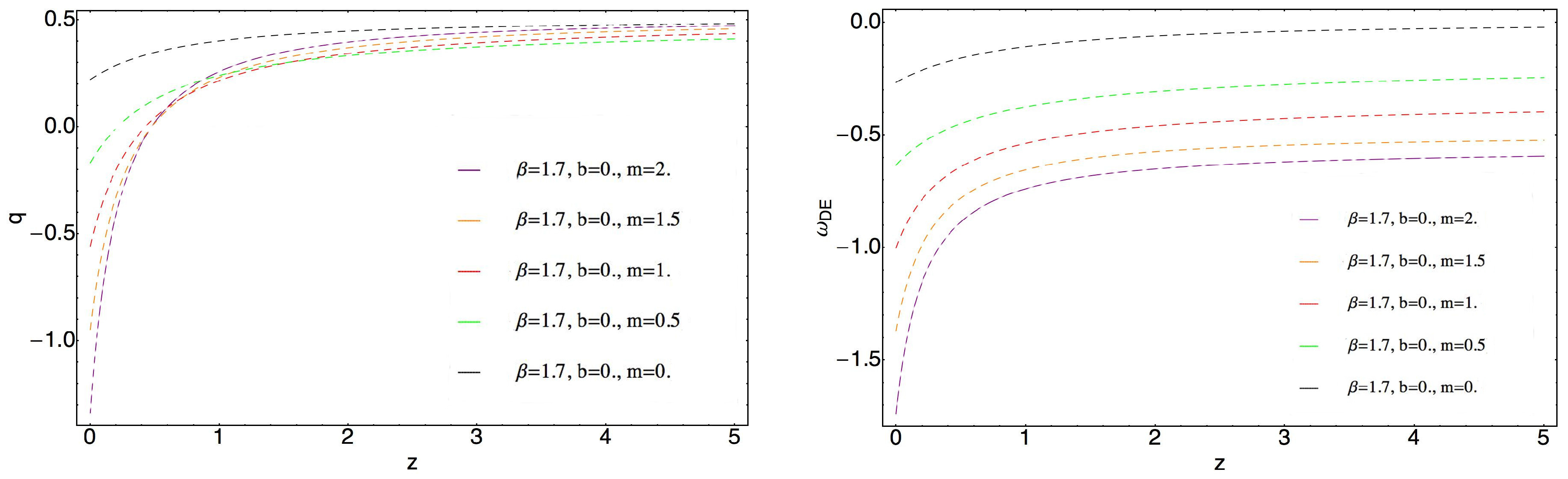
2.1.1. Non-Interacting Model

Consideration of the non-interacting model implies Q equal to 0 in Equations (17) and (18). In this case the first cosmological parameter which was studied is the deceleration parameter q (Figure 1) and one sees that for appropriate values of parameter m one can obtain a transit universe. Moreover, it can be seen that (when $b=0$) decrease of m causes vanishing of possibility to have a transit universe, in particular, when $m=0$, which corresponds to the usual ghost DE case, the transit universe is not possible. One can see that suggested modification allows to make the model very flexible with respect to future observational data. On the other hand, the graphical behavior of the deceleration parameter q and known fact that $0<q\le -1$, imposes some constraints on parameter m. In particular, for this model the following constraint on m $0<m\le 1.5$ will be obtained. Moreover, the right plot of Figure 1 demonstrates that for appropriate values of parameters, the phantom divide crossing is also possible. 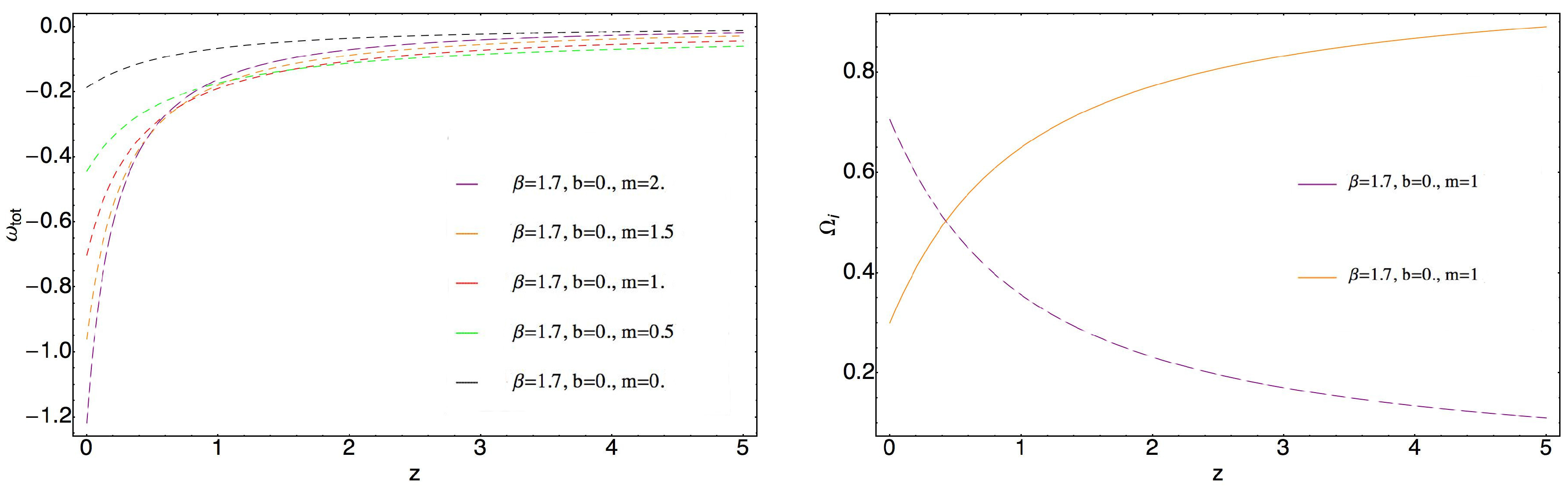Figure 2 represents the graphical behavior of EoS parameter of the total effective fluid defined as

$${\omega }_{tot}=\frac{P_{de}+(1/3){\rho }_r}{{\rho }_{de}+{\rho }_{dm}+{\rho }_r},$$

(23)

and the density parameters ${\mathrm{\Omega }}_{de}$ and ${\mathrm{\Omega }}_{dm}$ and one sees that the model is free from CCP. Present day values of the statefinder pair $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$ and q are presented in

Table 3. Present day values of $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$ and q for various values of the parameter m, when ${\mathrm{\Omega }}_{dm}\approx 0.3$, ${\mathrm{\Omega }}_r\approx 7\times {10}^{-5}$, while $b=0$ and $H_0=0.72$. $m=0$ corresponds to the usual ghost DE.

m	$(\mathit{r},\mathit{s})$	$({\mathit{\omega }}_{\mathit{de}}^{\prime },{\mathit{\omega }}_{\mathit{de}})$	q
1	$(9.9,-2.937)$	$(-5.96,-0.973)$	$-0.51$
$0.5$	$(3.854,-1.531)$	$(-2.38,-0.605)$	$-0.121$
$0.0$	$(3.069,-2.974)$	$(-1.68,-0.237)$	$0.268$

. In this case $\beta =1.7$. $\alpha =0.3$, $m=1.0$, $H_0=0.72$, ${\mathrm{\Omega }}_{dm}\approx 0.3$ and ${\mathrm{\Omega }}_r\approx 7\times {10}^{-5}$, with the transition redshift $z_{tr}\approx 0.42$ has been obtained from the distance modulus comparison with observational data. This is the model of universe, where the varying ghost DE in LSU has quintessence nature, while at $z=0$ it is a cosmological constant with ${\omega }_{de}=-1$. On the other hand, the total effective fluid will have only quintessence behavior in LSU.

2.1.2. Interacting Model

The energy density of DM in the case of interacting model according to Equations (18) and (22) reads as

$${\dot{\rho }}_{dm}+3H(1-b){\rho }_{dm}=3Hb{\rho }_{de}.$$

(24)

In this case also the parameter m has significant effect on behavior of the deceleration parameter q and EoS parameter of the varying ghost DE given by Equation (Figure 3 of Reference [58]). In this model the best fit of the distance modulus to observational data is possible when $\beta =0.7$, $\alpha =0.3$, $b=0.03$, $m=0.5$, ${\mathrm{\Omega }}_{dm}\approx 0.3$, ${\mathrm{\Omega }}_r\approx 7\times {10}^{-5}$, $H_0=0.72$, and the transition redshift is $z_{tr}\approx 0.42$. This model is also free from CCP (see Figure 4 of Reference [58]) with $z_{tr}\approx 0.42$. Bottom panels of Figures 3 and 4 of Reference [58] present graphical behavior of q, ${\omega }_{de}$, ${\omega }_{tot}$ and ${\mathrm{\Omega }}_i$ depending on the interaction parameter b for a fixed value of the parameter m. In

Table 4. Present day values of the statefinder pair $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$ and q for various values of the parameter m, when ${\mathrm{\Omega }}_{dm}\approx 0.3$, ${\mathrm{\Omega }}_r\approx 7\times {10}^{-5}$, while $b=0.03$ and $H_0=0.72$. $m=0$ corresponds to the usual ghost DE. $\alpha =0.3$ and $\beta =1.7$.

m	$(\mathit{r},\mathit{s})$	$({\mathit{\omega }}_{\mathit{de}}^{\prime },{\mathit{\omega }}_{\mathit{de}})$	q
1	$(10.73,-2.827)$	$(-6.315,-1.099)$	$-0.648$
$0.5$	$(3.838,-1.246)$	$(-2.361,-0.732)$	$-0.299$
$0.0$	$(2.213,-1.084)$	$(-1.29,-0.364)$	$0.130$

and

Table 5. Present day values of the statefinder pair $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$ and q for various values of the interaction parameter b, when ${\mathrm{\Omega }}_{dm}\approx 0.3$, ${\mathrm{\Omega }}_r\approx 7\times {10}^{-5}$, while $m=0.5$ and $H_0=0.72$. $\alpha =0.3$ and $\beta =1.7$.

b	$(\mathit{r},\mathit{s})$	$({\mathit{\omega }}_{\mathit{de}}^{\prime },{\mathit{\omega }}_{\mathit{de}})$	q
$0.0$	$(3.797,-1.475)$	$(-2.348,-0.612)$	$-0.132$
$0.01$	$(3.796,-1.382)$	$(-2.348,-0.652)$	$-0.174$
$0.03$	$(3.838,-1.246)$	$(-2.361,-0.732)$	$-0.26$
$0.05$	$(3.938,-1.161)$	$(-2.393,-0.812)$	$-0.343$
$0.07$	$(4.095,-1.111)$	$(-2.445,-0.891)$	$-0.428$

the present day values of the statefinder pair $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$ and q parameters are presented. Comparison of theoretical results with observational data left only quintessence behavior for DE and the total effective fluid. Numerical analysis presented in Reference [58] directly indicates creation of massless particles in an appropriate radiation dominated expanding universe which evolving gives LSU (with suggested varying ghost DE) compatible with recent observational data. On the other hand, massless particles in these models (non-interacting and interacting) are not created immediately. Creation requires some time to start. Moreover, the total number of created massless particles $N_k$ for both models is an increasing function. Moreover, massless particle creation will adopt a periodic character in recent LSU.

2.2. Cosmological Model with ${\rho }_{de}=\alpha H+\beta {\rho }_{dm}^m{H}^2$

In this subsection one will present the results corresponding to a model of the low redshift universe with another model of the varying ghost DE having the following energy density [59]

$${\rho }_{de}=\alpha H+\beta {\rho }_{dm}^m{H}^2,$$

(25)

where α, β and m are constants and ${\rho }_{dm}$ is the energy density of the pressureless DM. Such consideration is an attempt to have better understanding of the seeds for DE formation in early universe due to existence of DM. It is easy to see that Equations (25) and (17) with Equation (22) allows us to obtain EoS parameter of the varying ghost DE in terms of ${\mathrm{\Omega }}_{de}$ and ${\mathrm{\Omega }}_{dm}$ as

$${\omega }_{de}=\frac{A{\mathrm{\Omega }}_{dm}^2+B{\mathrm{\Omega }}_{dm}+C}{3\left(6H({\mathrm{\Omega }}_{de}-1)-\alpha \right){\mathrm{\Omega }}_{de}{\mathrm{\Omega }}_{dm}},$$

(26)

where $A=6H{\mathrm{\Omega }}_{de}-18bH-\alpha$, $B=-{\mathrm{\Omega }}_{de}(\alpha +6H(-3b(m+1)+3m+1))+2\alpha (2-3(b-1)m)+6H{\mathrm{\Omega }}_{de}^2$ and $C=-6bm{\mathrm{\Omega }}_{de}\left(\alpha -3H{\mathrm{\Omega }}_{de}\right)$. To obtain Equation (26) $P_{de}={\omega }_{de}{\rho }_{de}$ and that

$$1={\mathrm{\Omega }}_{de}+{\mathrm{\Omega }}_{dm}+{\mathrm{\Omega }}_r,$$

(27)

where

$${\mathrm{\Omega }}_i=\frac{{\rho }_i}{3H^2},$$

(28)

is taken into account. On the other hand, for this model the deceleration parameter reads as

$$q=-1-\frac{\dot{H}}{H^2}=\frac{1}{2}\left(2-(1-3{\omega }_{de}){\mathrm{\Omega }}_{de}-{\mathrm{\Omega }}_{dm}\right).$$

(29)

The study presented in Reference [59] indicates that the decrease of m leads to increase of transition redshift and to decrease of the present day values of the deceleration parameter q and ${\omega }_{de}$. Moreover, the increase of b leads to increase the transition redshift and to decrease of the present day values of ${\omega }_{de}$ and q. Moreover, in low redshift universe parameter b has a significant impact on these parameters. $b=0.01$, $\alpha =0.75$ and $\beta =0.85$, $m=-0.2$, $H_0=0.69$, ${\mathrm{\Omega }}_{de}\approx 0.7$, ${\mathrm{\Omega }}_{dm}\approx 0.3$ and ${\mathrm{\Omega }}_r\approx 0.3\times {10}^{-5}$ constraints on the model parameters have been obtained during the comparison of the modulus distance with observational data to have the best fit. Present day values of the statefinder pair $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$ and q are presented in

Table 6. Present day values of $(r,s)$, $({\omega }_{de}^{\prime },{\omega }_{de})$, q and appropriate redshift transition for the various values of the parameter m, when $b=0.01$, $\alpha =0.75$ and $\beta =0.85$, $H_0=0.69$, ${\mathrm{\Omega }}_{de}\approx 0.7$, ${\mathrm{\Omega }}_{dm}\approx 0.3$ and ${\mathrm{\Omega }}_r\approx 0.3\times {10}^{-5}$. $m=0$ corresponds to the usual ghost DE.

m	$(\mathit{r},\mathit{s})$	$({\mathit{\omega }}_{\mathit{de}}^{\prime },{\mathit{\omega }}_{\mathit{de}})$	q	${\mathit{z}}_{\mathit{tr}}$
$0.0$	$(2.247,-0.711)$	$(-1.173,-0.576)$	$-0.085$	$0.18$
$-0.1$	$(2.515,-0.745)$	$(-1.033,-0.647)$	$-0.177$	$0.3$
$-0.15$	$(2.852,-0.842)$	$(-1.057,-0.707)$	$-0.233$	$0.38$
$-0.2$	$(3.381,-0.996)$	$(-1.171,-0.761)$	$-0.297$	$0.42$
$-0.4$	$(8.883,-2.268)$	$(-3.237,-1.042)$	$-0.658$	$0.58$

. Graphical behavior of ${\mathrm{\Omega }}_{de}$ and ${\mathrm{\Omega }}_{dm}$ against redshift z is presented in Figure 2 of Reference [59].

Statefinder Hierarchy and Thermodynamics

It is easy to see, that if one starts from the first law of thermodynamics

$$Td{S}_{de}=d{E}_{de}+P_{de}dV,$$

(30)

and with an assumption that DE, DM, radiation and event horizon are in thermal equilibrium, after some mathematics for $dS/dH$ for the varying ghost DE, Equation (25), one obtains [59]

$$\frac{d{S}_{de}}{dH}=\frac{8{\pi }^2}{3H^4}\left(-\alpha -9H{\omega }_{de}{\mathrm{\Omega }}_{de}-3H{\mathrm{\Omega }}_{de}+\frac{6m\left(b{\mathrm{\Omega }}_{de}+(b-1){\mathrm{\Omega }}_{dm}\right)\left(\alpha -3H{\mathrm{\Omega }}_{de}\right)}{{\mathrm{\Omega }}_{dm}\left((3{\omega }_{de}-1){\mathrm{\Omega }}_{de}-{\mathrm{\Omega }}_{dm}+4\right)}\right),$$

(31)

where ${\omega }_{de}$ is given by Equation (26) and the case $b=0$, corresponds to thermodynamics of the non-interacting varying ghost DE model. Moreover, the graphical behavior of $S_3^{(1)}$ according to Equation (A11) against redshift z presented in Reference [59] indicates that $S_3^{(1)}$ in statefinder hierarchy is a good indicator for this model. Moreover, it gives clear indication about possible deviation from ΛCDM model. The cosmographic analysis demonstrated that suggested cosmological model is a good model for low redshift universe. Moreover, this is a model with radiation like effective fluid for high redshift, which evolves to a quintessence DE in present universe. Another interesting result of Reference [59] is the demonstration of massless particle creation in a radiation dominated universe (see Figure 4 of Reference [59]).

2.3. Cosmological Model with ${\rho }_{de}=\alpha {\rho }_{dm}^{m}H+\beta H^2$

In Section 2.1 and Section 2.2 the subject of study was accelerated expansion of LSU, where a varying ghost DE of a certain type can take the role of DE. The model of the varying ghost DE with

$${\rho }_{de}=\alpha {\rho }_{dm}^{m}H+\beta H^2,$$

(32)

presented in Reference [60] is another phenomenological modification of the ghost DE and completes the logical chain of considered modifications. It is obvious α, m and β should accepts appropriate values to have ${\rho }_{de}>0$. One sees that the power law function of the energy density of the DM is considered. The ghost DE model, Equation (32), may also be understood as an example of a fluid introduced in References [43,44]. The first model considered in Reference [60] is a cosmological scenario where DE and DM do not interact. One starts with the presentation of the results corresponding to this scenario taking into account the constraints which were obtained Reference [60] according to the best fit of theoretical results to luminosity distance.

2.3.1. Non-Interacting Model

In the case of suggested modification in non-interacting model the EoS of the varying ghost DE has the following form

$${\omega }_{de}=\frac{(2m-1)\left(\beta -3{\mathrm{\Omega }}_{\mathrm{de}}\right)}{{\mathrm{\Omega }}_{\mathrm{de}}\left(\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6\right)},$$

(33)

with ${\mathrm{\Omega }}_{de}\to 0$ ${\omega }_{de}\to \infty$, while in the case ${\mathrm{\Omega }}_{de}=1$, ${\omega }_{de}=2m-1$. On the other hand, the deceleration parameter q of this model accepts the following form

$$q=\frac{(6-9m){\mathrm{\Omega }}_{\mathrm{de}}+\beta (3m-1)-3}{\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6}.$$

(34)

Despite ${\omega }_{de}\to \infty$, for the deceleration parameter q one have

$$q=\frac{\beta (3m-1)-3}{\beta -6},$$

(35)

while for ${\mathrm{\Omega }}_{de}=1$

$$q=\frac{(\beta -3)(3m-1)-3}{\beta -3}.$$

(36)

Demand discussed in Reference [60] on $q\in [-1,0)$, ${\omega }_{de}\in [-1,0)$ and for simplicity $0\le \beta <1$, gives the following constraints on ${\mathrm{\Omega }}_{de}$ and m

$$\frac{1}{3}<{\mathrm{\Omega }}_{\mathrm{de}}\le 1,$$

(37)

$$\frac{\beta -\beta {\mathrm{\Omega }}_{\mathrm{de}}-3{\mathrm{\Omega }}_{\mathrm{de}}^2+3{\mathrm{\Omega }}_{\mathrm{de}}}{2\beta -6{\mathrm{\Omega }}_{\mathrm{de}}}\le m<\frac{\beta -6{\mathrm{\Omega }}_{\mathrm{de}}+3}{3\beta -9{\mathrm{\Omega }}_{\mathrm{de}}}.$$

(38)

The study of Reference [60] provides a self-consistent picture about the behavior of the deceleration parameter, EoS parameter of the varying ghost DE and EoS of the effective fluid for low and high redshift. In particular, the attention of the authors has been concentrated on the study of the impact of m parameter on the behavior and present day values of mentioned cosmological parameters. It has been found that correct dynamics of ${\omega }_{de}$, ${\omega }_{tot}$ and ${\mathrm{\Omega }}_{de}$ provides a phase transition from a decelerated expanding universe to recent accelerated expanding universe and with an increase of m the transition redshift will decrease. For the reconstruction of thermodynamics of DE model one should start from the first law of thermodynamics and after some trivial mathematics for the dynamics of the entropy $S_{\mathrm{de}}$ will be obtained

$$T\frac{d{S}_{\mathrm{de}}}{dH}=-\frac{4\pi \left((6-9m){\mathrm{\Omega }}_{\mathrm{de}}+\beta (3m-1)-3\right)\left({\mathrm{\Omega }}_{\mathrm{de}}\left(\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6m-3\right)+\beta (2m-1)\right)}{H^2\left(\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6\right)\left(-3(m-1){\mathrm{\Omega }}_{\mathrm{de}}+\beta m-3\right)},$$

(39)

On the other hand, in Reference [60] the forms of two parameters of statefinder analysis are presented corresponding to this case.

2.3.2. Interacting Model with $Q=3bH({\rho }_{de}+{\rho }_{dm})$

The consideration of interaction term given by Equation (22), gives EoS parameter and the deceleration parameter q of the model as follows

$${\omega }_{de}=\frac{-{\mathrm{\Omega }}_{\mathrm{de}}\left(6b(m-1)+\beta +(6m-3){\mathrm{\Omega }}_{\mathrm{de}}-2\beta m-6m+3\right)+2b(\beta m-3)+\beta -2\beta m}{\left({\mathrm{\Omega }}_{\mathrm{de}}-1\right){\mathrm{\Omega }}_{\mathrm{de}}\left(\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6\right)},$$

(40)

$$q=-\frac{3(2m-1)(3b-\beta +3)}{\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6}+\frac{3bm}{{\mathrm{\Omega }}_{\mathrm{de}}-1}-3m+2.$$

(41)

As it is pointed in Reference [60], for ${\mathrm{\Omega }}_{de}\to 0$, ${\omega }_{de}\to \infty$, but q is finite and is equal to

$$q=-\frac{3(2m-1)(3b-\beta +3)}{\beta -3}-3bm-3m+2,$$

(42)

and ${\mathrm{\Omega }}_{de}=1$ case should be excluded, since with ${\mathrm{\Omega }}_{de}\to 1$, ${\omega }_{de}$ and q tend to infinity. It is easy to see that the dynamics of the entropy of the varying ghost DE reads as

$$T\frac{d{S}_{\mathrm{de}}}{dH}=\frac{4\pi }{3H^2}\left(\frac{18(2m-1)(3b-\beta +3)}{\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6}-\frac{18bm}{{\mathrm{\Omega }}_{\mathrm{de}}-1}+A+A_1\right),$$

(43)

where

$$A=\frac{{(\beta -3)}^{2}m(2m-1)}{{(m-1)}^2\left(-3(m-1){\mathrm{\Omega }}_{\mathrm{de}}+\beta m-3\right)},$$

(44)

and

$$A_1=\frac{3(2-3m){\mathrm{\Omega }}_{\mathrm{de}}}{m-1}+\frac{(2m-1)(-\beta +3m(3m-5)+9)}{{(m-1)}^2}.$$

(45)

The graphical behavior of cosmological parameters and the present day values of these parameters summarized in Reference [60] directly indicate viability of considered model according to observational data.

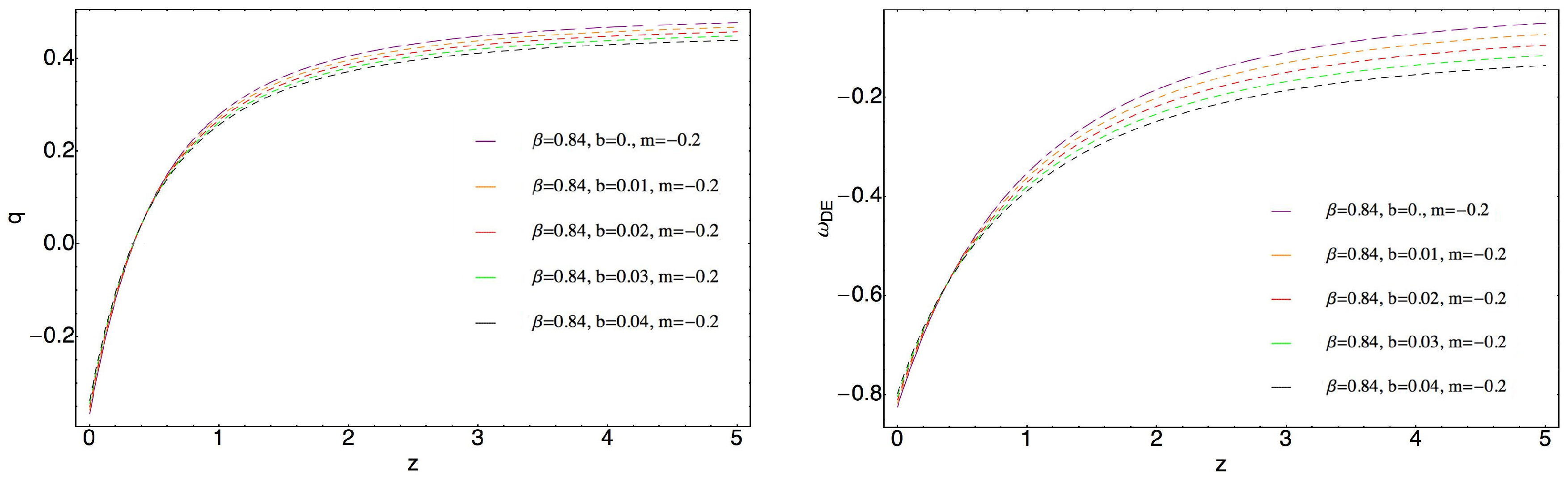
2.3.3. Interacting Model with $Q=3bHq({\rho }_{de}+{\rho }_{dm})$

Another model considered in Reference [60] assumes that the non-gravitational interaction is sign changeable and reads as

$$Q=3bHq({\rho }_{de}+{\rho }_{dm}).$$

(46)

In Figure 3 the redshift dependent behavior of the deceleration parameter q and EoS parameter of the varying ghost DE, Equation (32), corresponding to different values of the parameter b for fixed values of β and m are plotted. It can be seen, that the considered sign changeable interaction, Equation (46), leaves unaffected the transition redshift and a distinguishing interacting models from non interacting is not possible. An estimation of the present day values of $(r,s)$ and $({\omega }_{de}^{\prime },{\omega }_{de})$ for this model gives the same picture (for small values of b). The considered model is a model of a universe where

$${\omega }_{de}=\frac{-{\mathrm{\Omega }}_{\mathrm{de}}\left(3b(m-1)+\beta +(6m-3){\mathrm{\Omega }}_{\mathrm{de}}-2\beta m-6m+3\right)+b(\beta m-3)+\beta -2\beta m}{{\mathrm{\Omega }}_{\mathrm{de}}\left({\mathrm{\Omega }}_{\mathrm{de}}\left(9b(m-1)+\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-9\right)+b(9-3\beta m)-\beta +6\right)},$$

(47)

$$q=-\frac{\left({\mathrm{\Omega }}_{\mathrm{de}}-1\right)\left(\beta +(9m-6){\mathrm{\Omega }}_{\mathrm{de}}-3\beta m+3\right)}{{\mathrm{\Omega }}_{\mathrm{de}}\left(9b(m-1)+\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-9\right)+b(9-3\beta m)-\beta +6}.$$

(48)

and the dynamics of the entropy of the varying ghost DE, Equation (32), has the following form

$$T\frac{d{S}_{\mathrm{de}}}{dH}=\frac{4\pi \left({\mathrm{\Omega }}_{\mathrm{de}}\left(\beta +(9m-6){\mathrm{\Omega }}_{\mathrm{de}}-3(\beta +2)m+6\right)+\beta (2m-1)\right)}{H^2\left(-3(m-1){\mathrm{\Omega }}_{\mathrm{de}}+\beta m-3\right)}-\frac{12\pi {\omega }_{\mathrm{de}}{\mathrm{\Omega }}_{\mathrm{de}}}{H^2}.$$

(49)

There ${\omega }_{de}$ is given by Equation (47). It is evident from Equation (48), that when ${\mathrm{\Omega }}_{de}=1$, then $q=0$, therefore in order to have LSU with accelerated expansion with $q\in [-1,0)$, ${\omega }_{de}\in [-1,0)$ and for simplicity $0\le b<1$ with $0\le \beta <1$, it is necessary, that

$$\frac{1}{3}<{\mathrm{\Omega }}_{\mathrm{de}}<1,$$

(50)

$$\frac{\left({\mathrm{\Omega }}_{\mathrm{de}}-1\right)\left({\mathrm{\Omega }}_{\mathrm{de}}\left(-9b+\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-3\right)+3b-\beta \right)}{\left((3b-2){\mathrm{\Omega }}_{\mathrm{de}}-b+2\right)\left(\beta -3{\mathrm{\Omega }}_{\mathrm{de}}\right)}\le m<\frac{\beta -6{\mathrm{\Omega }}_{\mathrm{de}}+3}{3\beta -9{\mathrm{\Omega }}_{\mathrm{de}}}.$$

(51)

For universe with ${\mathrm{\Omega }}_{de}=0$, i.e., matter dominated universe

$$q=\frac{\left(\beta -3\beta m+3\right)}{b(9-3\beta m)-\beta +6}.$$

(52)

2.3.4. Interacting Model with $Q=3bH({\rho }_{dm}-{\rho }_{de})$

Discussed sign changeable interaction, Equation (46), is only an option. The following formulation

$$Q=3bH({\rho }_{dm}-{\rho }_{de}),$$

(53)

provides another option and indicates that when ${\rho }_{de}>{\rho }_{dm}$ DM will be transferred to DE, otherwise DE will be transferred to DM. If one considers the interaction, Equation (53), then in such universe, EoS parameter of the varying ghost DE has the following form [60]

$${\omega }_{de}=\frac{A_3+2b(\beta m-3)+\beta -2\beta m}{\left({\mathrm{\Omega }}_{\mathrm{de}}-1\right){\mathrm{\Omega }}_{\mathrm{de}}\left(\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6\right)},$$

(54)

and the deceleration parameter reads as [60]

$$q=-\frac{3(2m-1)(b(2\beta -9)-\beta +3)}{\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-6}-\frac{3bm}{{\mathrm{\Omega }}_{\mathrm{de}}-1}+6bm-6b-3m+2,$$

(55)

where $A_3={\mathrm{\Omega }}_{\mathrm{de}}\left(3(4b(m-1)-2m+1){\mathrm{\Omega }}_{\mathrm{de}}-2b(2\beta m+3m-9)+(\beta +3)(2m-1)\right)$. In order to obtain LSU with accelerated expansion with $q\in [-1,0)$, ${\omega }_{de}\in [-1,0)$ and for simplicity $0\le b<1$ with $0\le \beta <1$, it is necessary [60]

$$\frac{1}{3}<{\mathrm{\Omega }}_{\mathrm{de}}<1$$

(56)

$$\frac{\left({\mathrm{\Omega }}_{\mathrm{de}}-1\right)\left({\mathrm{\Omega }}_{\mathrm{de}}\left(-12b+\beta +3{\mathrm{\Omega }}_{\mathrm{de}}-3\right)+6b-\beta \right)}{2\left((2b-1){\mathrm{\Omega }}_{\mathrm{de}}-b+1\right)\left(\beta -3{\mathrm{\Omega }}_{\mathrm{de}}\right)}\le m<\frac{\left({\mathrm{\Omega }}_{\mathrm{de}}-1\right)\left(6(3b-1){\mathrm{\Omega }}_{\mathrm{de}}-9b+\beta +3\right)}{3\left((1-2b){\mathrm{\Omega }}_{\mathrm{de}}+b-1\right)\left(\beta -3{\mathrm{\Omega }}_{\mathrm{de}}\right)}.$$

(57)

The study of Reference [60] shows that one has the model with the correct behavior of cosmological parameters providing phase transition between decelerated and accelerated expansion of LSU. Moreover, in Reference [60] another model of interaction with ${\rho }_{de}-{\rho }_{dm}$ also has been considered. The difference between these two models is the sign of the parameter b, therefore consideration of $|b|$ instead of b will describe physics of two models. Results related to these two models of the interaction are compared in Figures 7 and 8 of Reference [60]. For the dynamics of the entropy of the varying ghost DE considered in this subsection has the following form [60]

$$T\frac{d{S}_{\mathrm{de}}}{dH}=\frac{4\pi \left({\mathrm{\Omega }}_{\mathrm{de}}\left(\beta +(9m-6){\mathrm{\Omega }}_{\mathrm{de}}-3(\beta +2)m+6\right)+\beta (2m-1)\right)}{H^2\left(-3(m-1){\mathrm{\Omega }}_{\mathrm{de}}+\beta m-3\right)}-\frac{12\pi {\omega }_{\mathrm{de}}{\mathrm{\Omega }}_{\mathrm{de}}}{H^2},$$

(58)

where ${\omega }_{\mathrm{de}}$ is given by Equation (54), and interaction parameter b has been changed to $|b|$. The study of Reference [60] shows also that $S_3$ parameter from the statefinder hierarchy is a good indicator to distinguish the models, therefore attention can be concentrated on it only. Moreover, the study of Reference [60] shows that $Om$ is a good analysis for considered models. However, $Om$ analysis is better for low redshif analysis.

3. Alternative Look at the Problem

The purpose of this section is to discuss various cosmological models applicable to accelerated expansion of LSU providing an alternative look to the problem. In particular, two models considered in this section belong to the class of the models where the dynamics of the background is still according to GR, however exotic forms of EoS and the interaction term between DE and DM is used. Instead of directly solving the field equations for these models, phase space analysis has been performed. It is known that in the phase space of a dynamical system all its possible states are represented making it very useful tool for modern cosmology. To analyse the dynamical system of the interacting polytropic gases considered in this section, one follows to existing scientific literature (see for instance [83]). There is a huge number of articles presenting the phase space analysis of different cosmological models [83,84,85,86,87,88,89,90] (to mention a few).

3.1. LSU with Interacting Polytropic Gas

Polytropic gas with non-linear EoS of the following

$$P_p=K{\rho }_p^{1+1/n},$$

(59)

where K and n are two constants, has important applications in astrophysics and it is one of the models of dark fluids used in modern cosmology. In Reference [91] various cosmological scenarios with non-linear interacting polytropic gas models has been considered. Namely, two phenomenological possibilities for interaction term are considered: non-linear and sign changeable non-linear interactions. In particular, the following forms of interaction will be considered

$$Q=3Hb\left(\rho +\frac{\widehat{\rho }}{\rho }\right),$$

(60)

and

$$Q=3Hbq\left(\rho +\frac{\widehat{\rho }}{\rho }\right),$$

(61)

ρ is the energy density either of the effective fluid or one of the components of the effective fluid. $\widehat{\rho }={\rho }_i{\rho }_j$ is the product of the energy densities of DE and DM.

3.1.1. Interacting Model with $Q=3Hb\left({\rho }_p+{\rho }_m+\frac{{\rho }_m^2}{{\rho }_p+{\rho }_m}\right)$

The study of Reference [91] starts with the model, when the following interaction is considered

$$Q=3Hb\left({\rho }_p+{\rho }_m+\frac{{\rho }_m^2}{{\rho }_p+{\rho }_m}\right).$$

(62)

The autonomous system of this model reads as

$$x^{\prime }=-3b\left(2+(x-2)x\right)-3(1-x)y,$$

(63)

$$y^{\prime }=3y(1+y)-\frac{3(1+n)y\left(x+y+b(2+(x-2)x)\right)}{nx}.$$

(64)

It has been found, that only one physically reasonable stable critical point $(E.1.1)$ exists

$$(x,y)=\left(\frac{2b-1+\sqrt{1-4b^2}}{2b},-1\right),$$

(65)

when

$$n\le -1,0<b<\frac{1}{4}\sqrt{\frac{2n^2-1}{n^2}}-\frac{1}{4n},$$

(66)

or

$$-1<n<0,0<b<\frac{1}{2}.$$

(67)

Moreover, this solution is simply a late time scaling attractor, because

$$r=\frac{{\mathrm{\Omega }}_m}{{\mathrm{\Omega }}_p}=\frac{\sqrt{1-4b^2}}{2(1-2b^2)}-\frac{1}{2}.$$

(68)

The study shows, that this late time scaling attractor describes the state of universe with ${\omega }_{eff}=-1$, $q=-1$ and EoS of the polytropic gas exhibits a phantom behavior (quintessence behavior is not possible) with

$${\omega }_p=-\frac{2b}{2b+\sqrt{1-4b^2}-1},$$

(69)

when

$$n\le -1,0<b\le \frac{1}{2\sqrt{2}},$$

(70)

or

$$-1<n<0,0<b<\frac{1}{2},$$

(71)

or

$$-\sqrt{\frac{1-4b^2}{{(1-8b^2)}^2}}+\frac{2b}{1-8b^2}<n\le -1,\frac{1}{2\sqrt{2}}<b<\frac{1}{2},$$

(72)

3.1.2. Interacting Model with $Q=3Hb\left({\rho }_p+{\rho }_m+\frac{{\rho }_p^2}{{\rho }_p+{\rho }_m}\right)$

In the second model conidered in Reference [91] the following form for the interacting has been considered

$$Q=3Hb\left({\rho }_p+{\rho }_m+\frac{{\rho }_p^2}{{\rho }_p+{\rho }_m}\right).$$

(73)

The study shows that only one late time scaling attractor exists ($E.1.2$)

$$(x,y)=\left(\frac{\sqrt{1-4(b-1)b}-1}{2b},-1\right),$$

(74)

obtained from the following autonomous system

$$x^{\prime }=-3b(1+x^2)-3(1-x)y,$$

(75)

$$y^{\prime }=3y(1+y)-\frac{3(1+n)y\left(b+x(1+bx)+y\right)}{nx}.$$

(76)

For this model, it is not hard to see that ${\omega }_{eff}=-1$, $q=-1$, while

$${\omega }_p=-\frac{2b}{\sqrt{1-4(b-1)b}-1},$$

(77)

and

$$r=\frac{{\mathrm{\Omega }}_m}{{\mathrm{\Omega }}_p}=\frac{2b+\sqrt{1-4(b-1)b}}{2(1-b)}.$$

(78)

Discussed late time scaling attractor represents a universe where the polytropic gas is phantom DE, when one of the following conditions take a place

$$n\le -1,0<b<\frac{n-1}{2n},$$

(79)

or

$$-1<n<0,0<b<1.$$

(80)

3.1.3. Interacting Model with $Q=3Hb\left({\rho }_p+{\rho }_m+\frac{{\rho }_p{\rho }_m}{{\rho }_p+{\rho }_m}\right)$

The third model studied in Reference [91] is a cosmological model with the following form of interaction

$$Q=3Hb\left({\rho }_p+{\rho }_m+\frac{{\rho }_p{\rho }_m}{{\rho }_p+{\rho }_m}\right),$$

(81)

having four critical points and only one is a physically reasonable and has the following form (E.1.3)

$$(x,y)=\left(\frac{1+b-\sqrt{1+(5b-2)b}}{2b},-1\right),$$

(82)

According to Reference [91] this solution is a late time scaling attractor describing universe with ${\omega }_{eff}=-1$, $q=-1$,

$${\omega }_p=-\frac{2b}{1+b-\sqrt{1+(5b-2)b}},$$

(83)

and

$$r=\frac{{\mathrm{\Omega }}_m}{{\mathrm{\Omega }}_p}=\frac{3b+\sqrt{1+(5b-2)b}-1}{2(1-b)},$$

(84)

where the polytropic gas is phantom DE, when

$$n\le -1,0<b<\frac{2n-5}{10n}+\frac{1}{10}\sqrt{\frac{5+4n^2}{n^2}},$$

(85)

or

$$-1<n<0,0<b<1.$$

(86)

3.1.4. Sign Changeable Interactions

On the other hand in Reference [91] an attempt has been forwarded to construct new forms of sign changeable interactions for the interaction terms considered in Section 3.1.1, Section 3.1.2 and Section 3.1.3. In particular, the deceleration parameter q has been involved. The result (critical points) of the study presented in Reference [91] is summarized in

Table 7. Critical points for the models where sign changeable non linear interactions are given via Equations (87)–(89).

Critical Points	Q	x	y
$E.2.1$	Equation (87)	$\frac{1+2b\pm \sqrt{1-4b^2}}{2b}$	$-1$
$E.2.2$	Equation (88)	$\frac{1\pm \sqrt{1-4b(1+b)}}{2b}$	$-1$
$E.2.3$	Equation (89)	$\frac{1}{2}(1\pm \sqrt{5})$	0
$E.2.4$	Equation (89)	$\frac{b-1\pm \sqrt{1+(2+5b)b}}{2b}$	$-1$

.

In particular, the forms for Q have been considered

$$Q=3Hbq\left({\rho }_p+{\rho }_m+\frac{{\rho }_m^2}{{\rho }_p+{\rho }_m}\right),$$

(87)

$$Q=3Hbq\left({\rho }_p+{\rho }_m+\frac{{\rho }_p^2}{{\rho }_p+{\rho }_m}\right),$$

(88)

and

$$Q=3Hbq\left({\rho }_p+{\rho }_m+\frac{{\rho }_p{\rho }_m}{{\rho }_p+{\rho }_m}\right).$$

(89)

Analysis show that obtained critical points are not stable. Compared with the interactions terms in Section 3.1.1, Section 3.1.2 and Section 3.1.3, appropriate sign changeable interactions do not allow to obtain late time attractors.

3.2. LSU with a Varying Polytropic Gas

In this section one will discuss results of the study presented in Reference [92]. The study of Reference [92] involves new idea/ phenomenological model of dark fluid constructed in analogue with a varying Chaplygin gas of the following form

$$P_c=A{\rho }_c-\frac{B{H}^{-k}}{{\rho }^{\alpha }}.$$

(90)

In particular, in Reference [92] the following model of varying polytropic gas

$$P_{de}=A{H}^{-k}{\rho }_{de}^{1+1/n},$$

(91)

where A, B, k and n are constants, is considered. In addition, pressureless DM to be the second component of recent low redshift universe is assumed to exist. Moreover, the forms of interactions considered in Reference [92] are particular examples obtained from

$$Q=q^m\left(3Hb{\rho }_i+\gamma {\dot{\rho }}_i\right),$$

(92)

m, b and γ are constants. There the subscript i stands for the energy density either of effective fluid, or one of dark components. The study in Reference [92] starts form the models containing fixed sign interactions, which correspond to $m=0$ in Equation (92) with $q\in [-1,0)$, ${\omega }_{de}\in [-1.05,0)$, $k\in (-2,2)$ and $n\in (-2,2)$. The lower limit on ${\omega }_{de}$ is due to PLANCK 2015 satellite experiment constraints [93].

3.2.1. Interaction $Q=3Hb{\rho }_{de}+\gamma {\dot{\rho }}_{de}$

The first cosmological model studied in Reference [92] involves the following interaction

$$Q=3Hb{\rho }_{de}+\gamma {\dot{\rho }}_{de},$$

(93)

between varying polytropic DE, Equation (91), and CDM. The autonomous system of this model reads as [92]

$$x^{\prime }=3x(1+y)-\frac{3(x+bx+y)}{1+\gamma },$$

(94)

$$y^{\prime }=\frac{3}{2}(2+k)(1+y)y-\frac{3(1+n)(x+bx+y)y}{n(1+\gamma )x},$$

(95)

and admits only one physically reasonable stable critical point $(C.1.1)$ (is either a stable node or stable focus) of the following form

$$(x,y)=\left(\frac{1}{1+b},-1\right).$$

(96)

It is easy to see, that straightforward calculations give

$$r=\frac{{\mathrm{\Omega }}_{dm}}{{\mathrm{\Omega }}_{de}}=b,$$

(97)

indicating that a solution of CCP directly depends on parameter b of interaction Equation (93). $C.1.1$ describes the universe where ${\omega }_{eff}=-1$, $q=-1$ and EoS parameter of the varying polytropic DE reads as

$${\omega }_{de}=-1-b.$$

(98)

In case of considered constraints in Reference [92] one will have only phantom polytropic dark fluid. The study of Reference [92] reveals possible phase transition to accelerated expending low redshift universe. On the other hand, the study of this model has been completed by estimation of the present day values of $({\omega }_{de}^{\prime },{\omega }_{de})$ and statefinder parameters $(r,s)$, which are summarized in

Table 8. Present day values of $({\omega }_{de}^{\prime },{\omega }_{de})$ and statefinder parameters $(r,s)$ for the cosmological model where $H_0=0.72$, ${\mathrm{\Omega }}_{de}^0=0.7$ and ${\mathrm{\Omega }}_{de}^0=0.3$, while the interaction is given by Equation (93).

b	γ	$({\mathit{\omega }}_{\mathit{de}}^{\prime },{\mathit{\omega }}_{\mathit{de}})$	$(\mathit{r},\mathit{s})$
$0.0$	$0.0$	$(-0.453,-0.998)$	$(4.982,-1.266)$
$0.02$	$0.0$	$(-0.483,-0.998)$	$(4.857,-1.226)$
$0.02$	$0.01$	$(-0.483,-0.998)$	$(4.858,-1.226)$
$0.02$	$0.02$	$(-0.482,-0.998)$	$(4.859,-1.227)$
$0.02$	$0.03$	$(-0.482,-0.998)$	$(4.861,-1.228)$

of this work.

3.2.2. Interaction $Q=3Hb{\rho }_{dm}+\gamma {\dot{\rho }}_{dm}$

The second cosmological model considered in Reference [92] admits the following form of the interaction

$$Q=3Hb{\rho }_{dm}+\gamma {\dot{\rho }}_{dm}.$$

(99)

giving two late time attractors (

Table 9. Critical points for the cosmological model, where interaction between varying polytropic dark fluid Equation (91) is given via Equation (99).

$\mathit{Critical}\mathit{Points}$	x	y	Type of Stability	Acceleration
$C.2.1$	1	$-1$	Stable node	Yes
$C.2.2$	1	0	Stable node	No

). However, an attractor $C.2.2$ with $x=1$ and $y=0$, can not describe accelerated expansion of recent LSU, therefore it is out of the future discussion. Late time attractor $C.2.1$ with $x=1$ and $y=-1$ describes the state of low redshift universe with ${\omega }_{eff}=-1$, ${\omega }_{de}=-1$ and $q=-1$ i.e it describes de Sitter universe (according to the constraints considered in Reference [92]). On the other hand in

Table 10. Present day values of $({\omega }_{de}^{\prime },{\omega }_{de})$ and statefinder parameters $(r,s)$ for the cosmological model where $H_0=0.72$, ${\mathrm{\Omega }}_{de}^0=0.7$ and ${\mathrm{\Omega }}_{de}^0=0.3$, while the interaction is given via Equation (99). The values of the parameters are due to the behavior of the cosmological parameters presented in Figure 2 of Reference [92]. $m=-2$.

b	γ	$({\mathit{\omega }}_{\mathit{de}}^{\prime },{\mathit{\omega }}_{\mathit{de}})$	$(\mathit{r},\mathit{s})$
$0.0$	$0.0$	$(-0.453,-0.998)$	$(4.982,-1.266)$
$0.02$	$0.0$	$(-0.466,-0.998)$	$(4.928,-1.249)$
$0.02$	$0.01$	$(-0.459,-0.998)$	$(4.955,-1.258)$
$0.02$	$0.03$	$(-0.447,-0.998)$	$(5.011,-1.227)$

present day values of $({\omega }_{de}^{\prime },{\omega }_{de})$ and statefinder parameters $(r,s)$ are summarized and the study revealed possible phase transition to accelerated expending low redshift universe.

3.2.3. Interaction $Q=3Hb({\rho }_{dm}+{\rho }_{de})+\gamma ({\dot{\rho }}_{dm}+{\dot{\rho }}_{de})$

Another possibility studied in Reference [92] is cosmological model where the interaction between dark components reads as

$$Q=3Hb({\rho }_{dm}+{\rho }_{de})+\gamma ({\dot{\rho }}_{dm}+{\dot{\rho }}_{de}),$$

(100)

with only one late time attractor (C.3.1 with $r=b/(1-b)$) of the following form

$$(x,y)=\left(1-b,-1\right),$$

(101)

${\omega }_{eff}=-1$, $q=-1$ and

$${\omega }_{de}=-\frac{1}{1-b},$$

(102)

giving a phantom LSU when $b\in (0,1)$. On the other hand, when $b=0$ polytropic dark fluid is a CC with ${\omega }_{de}=-1$. In Reference [92] the graphical behavior of the cosmological parameters reveals the phase transition between decelerated and accelerated expansion.

3.2.4. Interaction $Q=q\left(3Hb{\rho }_{de}+\gamma {\dot{\rho }}_{de}\right)$

In addition to considered three forms of interactions, in Reference [92] some forms of sign changeable interactions also has been discussed to complete the study. In particular the following form of sign changeable interaction

$$Q=q\left(3Hb{\rho }_{de}+\gamma {\dot{\rho }}_{de}\right),$$

(103)

has been considered and demonstrated, that only one critical point exists (E.4.1 which is either stable node or stable focus) with

$$(x,y)=\left(\frac{1}{1-b},-1\right),$$

(104)

${\omega }_{de}=-1+b$, ${\omega }_{eff}=-1$ and $q=-1$. On the other hand, for this model ${\mathrm{\Omega }}_{dm}/{\mathrm{\Omega }}_{de}$ is equal to $-b$ and in Reference [92] $0\le b<1$ was assumed, which directly indicates that $C.4.1$ is physically reasonable only with $b=0$. Therefore, according to Reference [92] $C.4.1$ describes a state of LSU, where only varying polytropic dark energy given by Equation (91) will dominate (an example of de Sitter universe). Moreover, the considered model has phase transition to an accelerated expanding low redshift universe.

If interaction of the following form is considered

$$Q=q\left(3Hb{\rho }_{dm}+\gamma {\dot{\rho }}_{dm}\right),$$

(105)

between varying polytropic DE and DM, then only one late time attractor will exist for this model, which is an example de Sitter universe admitting phase transition (see Reference [92]).

3.2.5. Interaction $Q=q\left(3Hb({\rho }_{dm}+{\rho }_{de})+\gamma ({\dot{\rho }}_{dm}+{\dot{\rho }}_{de})\right)$

Autonomous system of cosmological model where interaction between suggested DE and DM has the following form (the last model considered in Reference [92])

$$Q=q\left(3Hb({\rho }_{dm}+{\rho }_{de})+\gamma ({\dot{\rho }}_{dm}+{\dot{\rho }}_{de})\right),$$

(106)

has one critical point (C.6.1)

$$(x,y)=(1+b,-1),$$

(107)

and describe a model of universe with $q=-1$, ${\omega }_{eff}=-1$ and

$${\omega }_{de}=-\frac{1}{1+b}.$$

(108)

However, in this case $C.6.1$ is a physically reasonable solution if $b=0$ (according to considered constrains), describing de Sitter universe. Moreover, it is easy to see that the explicit forms of the trace

$$Tr=\frac{3(2+3b-(2+b+k(1+b))n+2(1+n)\gamma )}{2(1+b)n},$$

(109)

and the determinant

$$Det=\frac{9k}{2}-\frac{9}{n},$$

(110)

of the appropriate Jacobian matrix indicate for $C.6.1$ attractor to be either stable node or stable focus. Present day values of $({\omega }_{de}^{\prime },{\omega }_{de})$ and statefinder parameters $(r,s)$, for different values of the parameters of all models studied in Reference [92] are summarized in appropriate tables (for more details on this issue one referees the readers to Reference [92]).

3.3. Cosmological Models with Generalized Holographic DE

In this subsection, following Reference [94], one will consider particular model of the generalized holographic DE with the Nojiri- Odintsov cut-off defined as [24].

$${\rho }_{de}=\frac{3c^2}{L^2},$$

(111)

with

$$\frac{c}{L}=\frac{1}{L_f}\left[{\alpha }_0+{\alpha }_1{L}_f+{\alpha }_2{L}_f^2\right]$$

(112)

where $L_f$ it is the future horizon and defined as

$$L_f=a{\int }_t^{\infty }\frac{dt}{a},$$

(113)

while c, ${\alpha }_0$, ${\alpha }_1$ and ${\alpha }_2$ are numerical constants.

3.3.1. Models with $Q=3Hb({\rho }_{de}+{\rho }_{dm})$

Generalized holographic DE model of Reference [24] includes arbitrary cut-off depending on $L_f$, scale factor, H etc and their derivatives. In Reference [94] particular class of such generalized holographic DE model with Nojiri-Odintsov cut-off as Equation (112) has been considered. The cosmological model of LSU, where the interaction between the dark energy and dark matter is given by $Q=3Hb({\rho }_{de}+{\rho }_{dm})$ contains the DE with the following EoS parameter

$${\omega }_{de}=-\frac{3b{H}^2{L}_f+2\sqrt{{\mathrm{\Omega }}_{de}}{\dot{L}}_f({\alpha }_1+2{\alpha }_2{L}_f)+H{\mathrm{\Omega }}_{de}(H{L}_f+2)}{3H^2{L}_f{\mathrm{\Omega }}_{de}},$$

(114)

while the deceleration parameter q reads as

$$q=\frac{(1-3b)H^2{L}_f-2\sqrt{{\mathrm{\Omega }}_{de}}{\dot{L}}_f({\alpha }_1+2{\alpha }_2{L}_f)-H{\mathrm{\Omega }}_{de}(H{L}_f+2)}{2H^2{L}_f}.$$

(115)

Analysis of Reference [94] shows that in such model $z_{tr}\approx 0.682$ (transition redshift) when $b=0$ and the present day value of q defined by Equation (115) will decrease, the transition redshift $z_{tr}$ will increase, when the value of the interaction parameter b will increase. Moreover, an increase of the interaction parameter b will speed up an increase of the amount of ${\mathrm{\Omega }}_{de}$ and this model is free from CCP. Moreover, the study of Reference [94] indicates clearly, that the impact of the interaction is imprinted into the dynamics of ${\mathrm{\Omega }}_{de}$ and ${\mathrm{\Omega }}_{dm}$. Moreover, for $b\in [0,0.03]$ the value of the EoS parameter of DE (recent epoch) satisfies to the constraints according to the Planck satellite 2015 experiments. On the other hand, in this case with an increase of b the EoS will change its nature from quintessence to phantom (for higher redshifts), while for the lower redshifts gives an increase of ${\omega }_{de}^{\prime }$ and a decrease of ${\omega }_{de}$. Another constraint on the parameter b is $b\in [0,0.005]$ due to the demand for DE to have only the quintessence nature. From

Table 11. Present day values of the deceleration parameter q, $({\omega }_{de},{\omega }_{de}^{\prime })$ of interacting dark energy, $(r,s)$ statefinder parameters and the value of the transition redshift $z_{tr}$ for several values of the interaction parameter b, when the interaction is given by $Q=3Hb({\rho }_{de}+{\rho }_{dm})$. The best fit of the theoretical results to the recent observational data has been obtained for $H_0=0.7$, ${\alpha }_0=0.15$, ${\alpha }_1=0.25$, $c=0.75$.

b	q	$({\mathit{\omega }}_{\mathit{de}},{\mathit{\omega }}_{\mathit{de}}^{\prime })$	$(\mathit{r},\mathit{s})$	${\mathit{z}}_{\mathit{tr}}$
$0.0$	$-0.522$	$(-0.978,0.249)$	$(2.86,-0.61)$	$0.682$
$0.01$	$-0.537$	$(-0.993,0.257)$	$(2.76,-0.57)$	$0.728$
$0.03$	$-0.567$	$(-1.022,0.269)$	$(2.57,-0.49)$	$0.832$
$0.05$	$-0.596$	$(-1.051,0.279)$	$(2.39,-0.42)$	$0.954$
$0.07$	$-0.626$	$(-1.079,0.284)$	$(2.23,-0.36)$	$1.101$

one sees that an increase of b will decrease and increase the present day values of r and s parameters, respectively.

3.3.2. Models with Non-Linear Interactions

The cosmographic study of Reference [94] has been completed with an assumption about possible existence of the interaction between DE and DM of the following form

$$Q=3bH\frac{{\rho }_{de}{\rho }_{dm}}{{\rho }_{de}+{\rho }_{dm}}.$$

(116)

Straightforward calculations showed that such assumption gives a universe, where the EoS parameter of DE and the deceleration parameter read as

$${\omega }_{de}=\frac{1}{3}\left(3b{\mathrm{\Omega }}_{de}-3b-\frac{2{\dot{L}}_f({\alpha }_1+2{\alpha }_2{L}_f)}{H^2{L}_f\sqrt{{\mathrm{\Omega }}_{de}}}-\frac{2}{H{L}_f}-1\right)$$

(117)

and

$$q=\frac{3b{H}^2{L}_f{\mathrm{\Omega }}_{de}^2-H{\mathrm{\Omega }}_{de}(H(3b{L}_f+L_f)+2)-2\sqrt{{\mathrm{\Omega }}_{de}}{\dot{L}}_f({\alpha }_1+2{\alpha }_2{L}_f)+H^2{L}_f}{2H^2{L}_f}.$$

(118)

The appropriate comparison of the results for the cosmological models where the interaction is given by $Q=3Hb({\rho }_{de}+{\rho }_{dm})$, Equation (116),

$$Q=3bH\frac{{\rho }_{de}^2}{{\rho }_{de}+{\rho }_{dm}},$$

(119)

and

$$Q=3bH\frac{{\rho }_{dm}^2}{{\rho }_{de}+{\rho }_{dm}},$$

(120)

is presented in Reference [94]. The analysis shows, that with the interaction $Q=3Hb({\rho }_{de}+{\rho }_{dm})$ one will obtain the highest value for the transition redshift $z_{tr}$, while Equations (116), (119) and (120) interactions will increase $z_{tr}$. Moreover the study shoes that the maximum and minimal present day values for the deceleration parameter will be obtained in case of $b=0$ and $Q=3Hb({\rho }_{de}+{\rho }_{dm})$, respectively. It also has been found that the $z_{tr}$ significantly will be affected in case of $Q=3Hb({\rho }_{de}+{\rho }_{dm})$ and the dynamics of ${\mathrm{\Omega }}_{de}$ and ${\mathrm{\Omega }}_{dm}$ carry information about the nature of the interactions. Moreover, the author of Reference [94] demonstrated, that with $Q=3Hb({\rho }_{de}+{\rho }_{dm})$ and Equation (120) DE is phantom, while in case of $b=0$ and when the interaction terms are given by Equations (116) and (119), DE is quintessence (for higher redshifts). However, during the evolution DE will change the nature giving either a quintessence universe, or a phantom universe. Such behavior has been found directly imprinted on the behavior of the other parameters.

Besides cosmographic analysis, the models have been studied by $Om$ and $Om3$ analysis and the graphical behavior (redshift dependent) of the $Om$ and $Om3$ parametes are presented in Figure 4 and Figure 5, respectively. Figures demonstrate departures from ΛCDM model (${\mathrm{\Omega }}_{dm}^0=0.3$ line). Moreover, one can see clearly, that $Om$ analysis is good enough for these models, while $Om3$ analysis shows that at lower redshifts non-gravitational interaction can be switched off. On the other hand $S_3$ parameter (corresponding to the statefinder hierarchy analysis) is good to study the models for lower redshifts only (Figure 6). In Reference [94] the validity of the generalized second law of thermodynamics

$${\dot{S}}_{tot}={\dot{S}}_{de}+{\dot{S}}_{dm}+{\dot{S}}_h,$$

(121)

with $S_h=8{\pi }^2{L}^2$ it is the entropy associated with the horizon, has been confirmed. On the other hand, $b\in [0,0.01)$ has been obtained from the validity of the generalized second law of thermodynamics. One can see that in this case the DE will have phantom nature at higher redshifts.

4. Conclusions

Accelerated expansion of LSU is one of the long standing problems. There are various attempts to solve this problem and in this review paper one has presented some of the possible new solutions of this problem. In particular, it has been demonstrated, that the problem of accelerated expansion can be solved by the new models of the varying ghost DE. Moreover, for two models massless particle creation in appropriate readiation dominated universe has been observed numerically. On the other hand within phase space analysis two alternative possibilities have been demonstrated. In the first case new models of nonlinear and nonlinear sing changeable interactions have been considered between polytropic DE and CDM. On the other hand for the second case, the solution to the problem has been demonstrated within new phenomenological modification of polytropic gas. Finally, the third alternative possibility presented in this work is due to the generalized holographic DE model with a specific form of cut-off. In this case, various forms of non-gravitational interaction between DE and DM have been considered to complete the study. Presented all models either are free from CPP, or the CCP can be avoided due to appropriate interaction. All models give phase transition between decelerated and accelerated expansion.

Comparison of the results with observational data proved the viability of suggested scenarios. On the other hand, one should try to deepen the position of these models involving into the game other forms of non-gravitational interaction, find scalar field description of the models (find the form of the potential) and construct, for instance, the $f(R)$ theory. There is another important direction of research related to the classification and understanding of the formation of finite time singularities in these models [95,96,97,98,99,100]. One hopes that results of this study will be reported soon elsewhere.