FIMP Dark Matter in Bulk Viscous Non-Standard Cosmologies
Abstract
1. Introduction
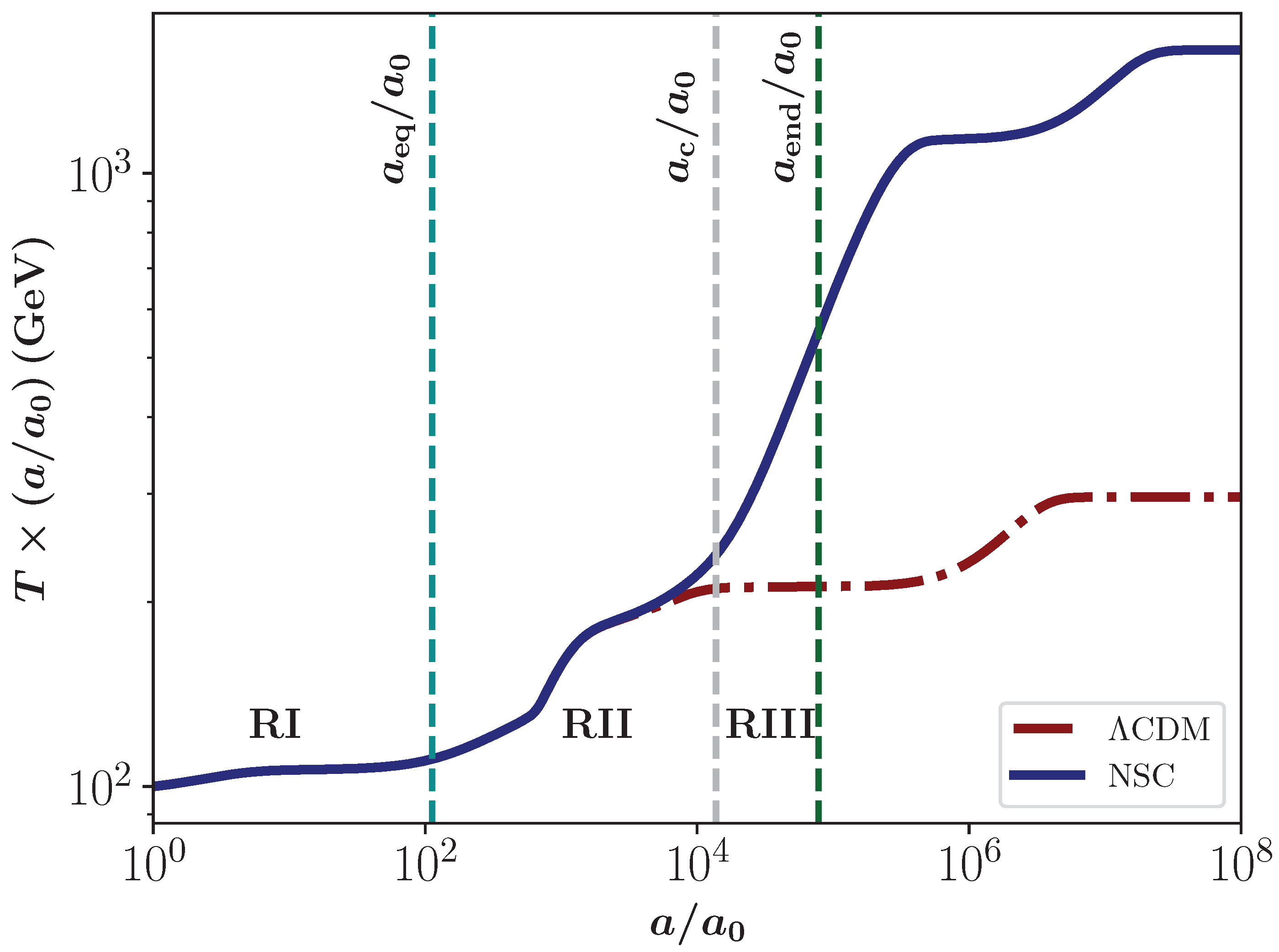
2. Non-Standard Cosmologies
2.1. FIMPs in Non-Standard Cosmologies
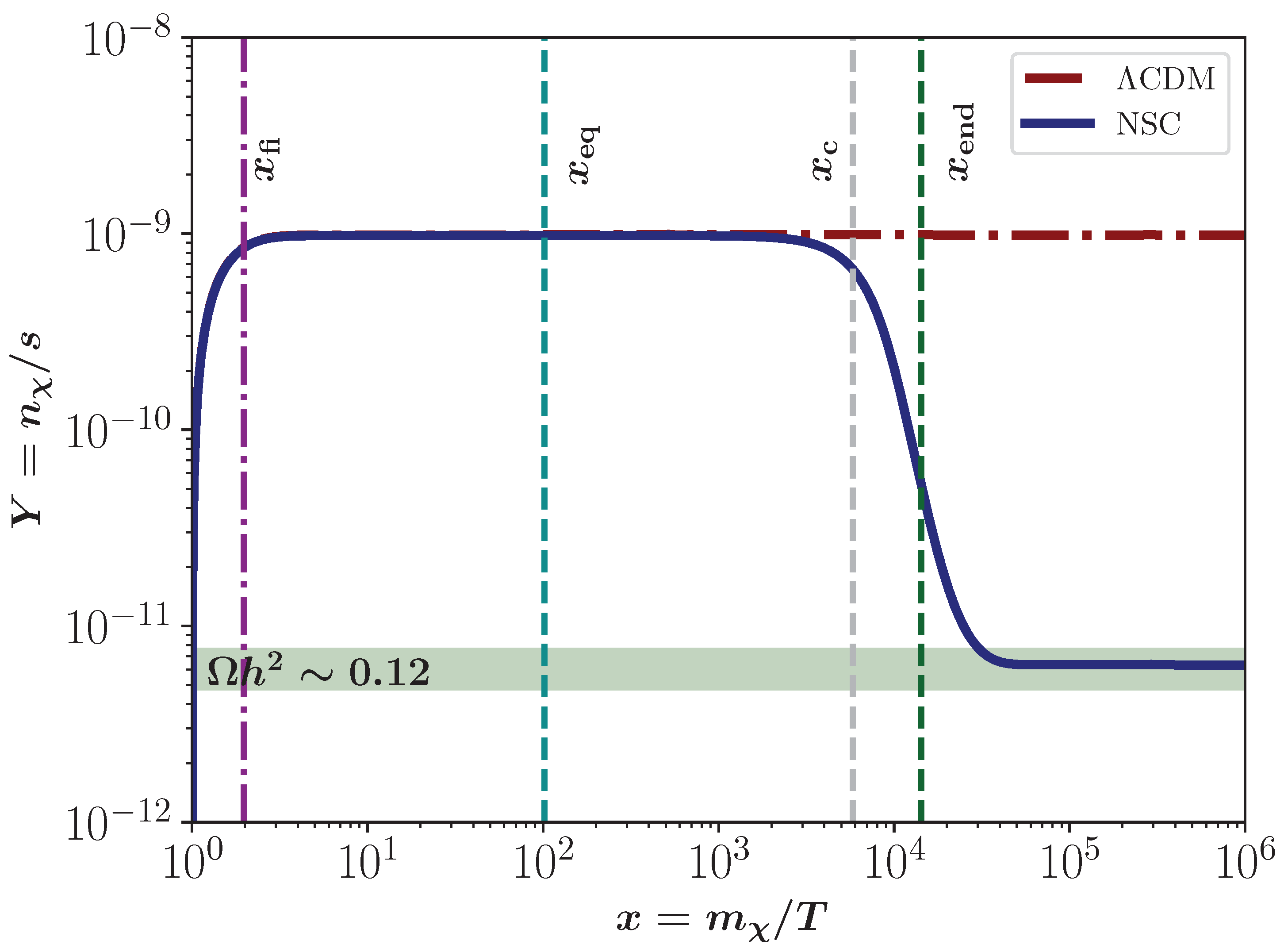
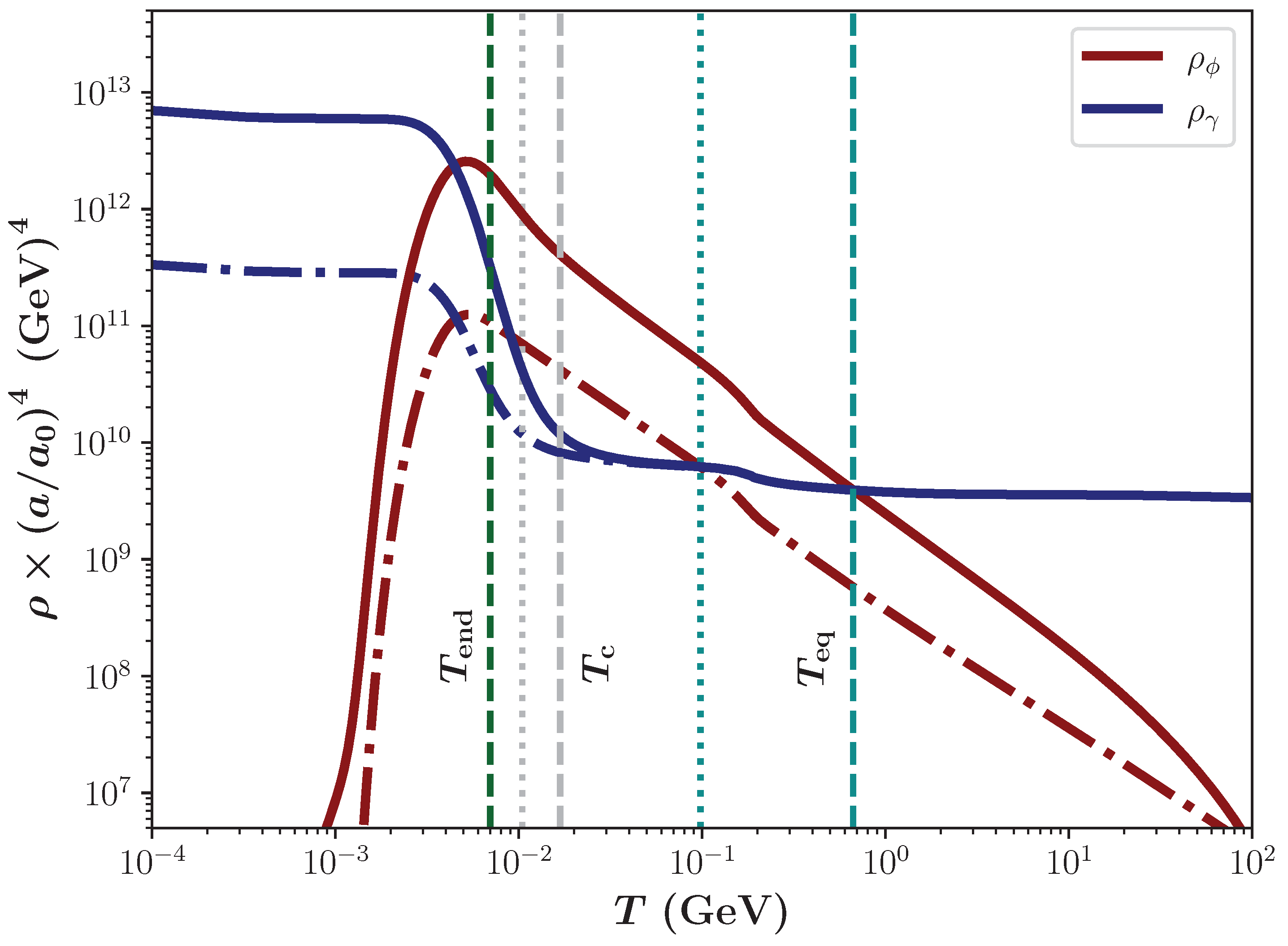
- RI: In this region, the radiation component of the universe still dominates its expansion, i.e., is almost the same as in the CDM model. The condition () is necessary for this region to exist. This behavior is illustrated in Figure 3, for a NSC with , GeV, GeV, GeV−2, and . It can be observed that the DM freezes its abundance before the decay of , and as the decays become significant, the DM relic density is diluted to reach its current value, allowing the parameters , which were previously ruled out in the CDM scenario.
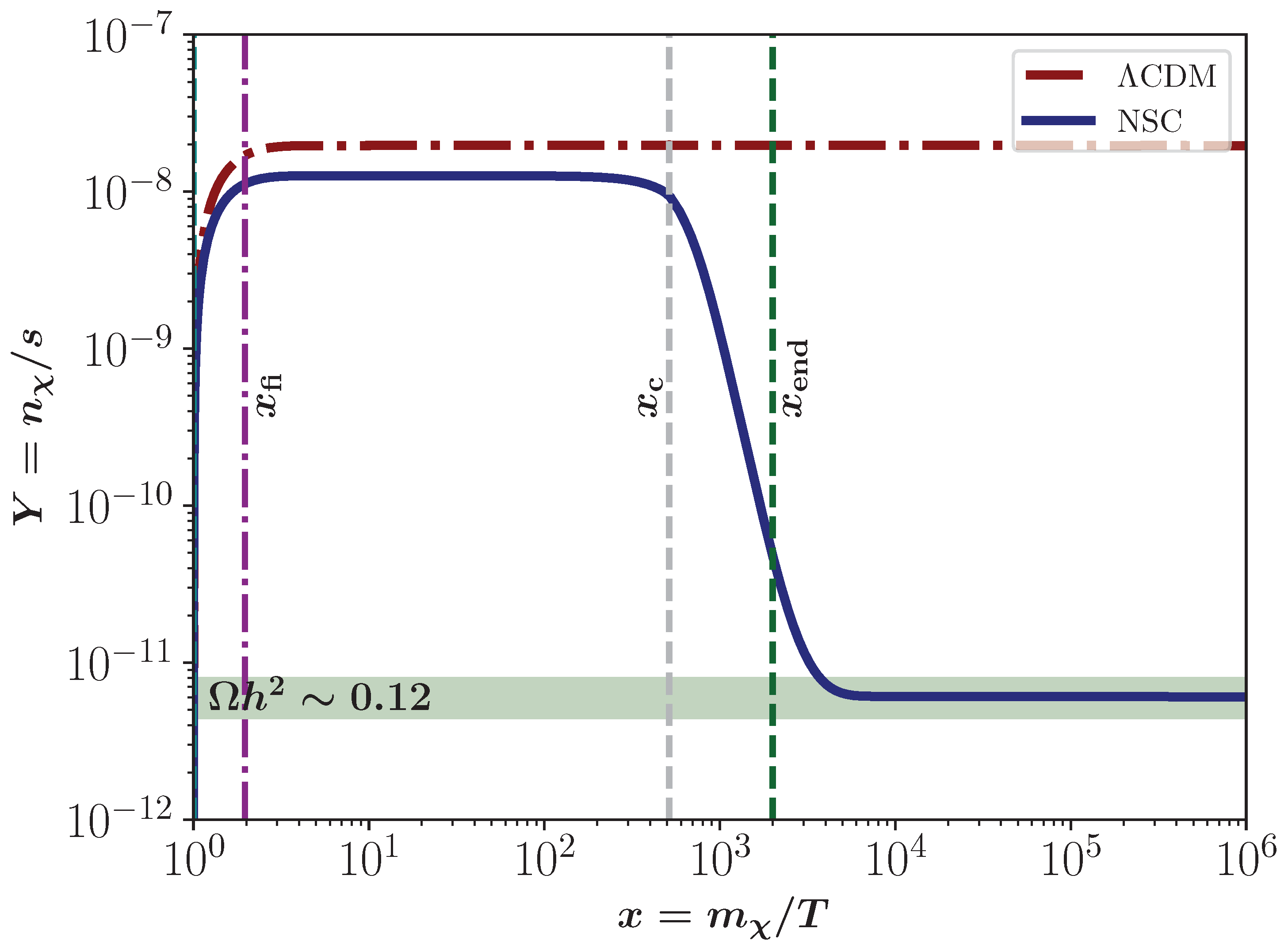
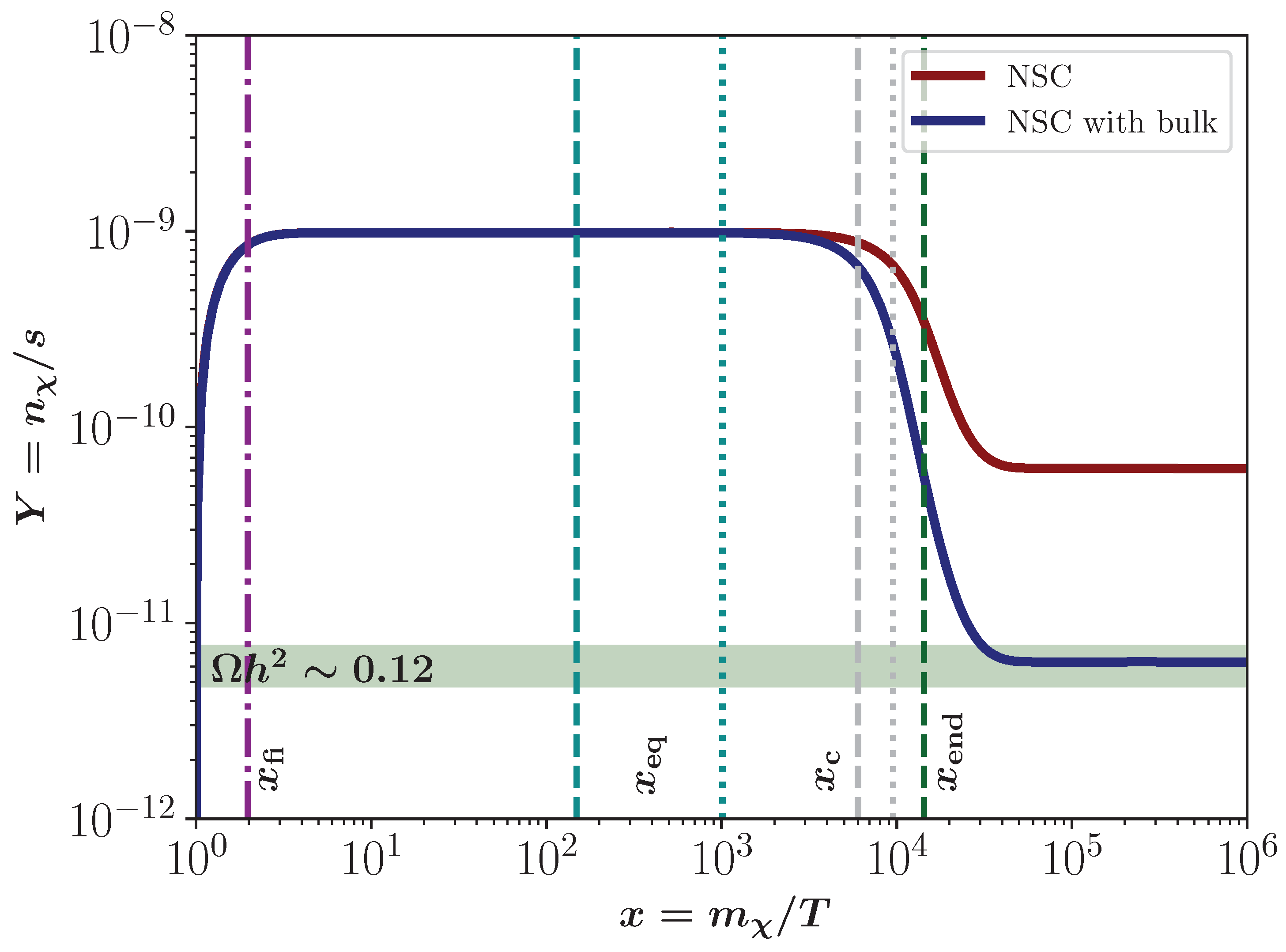
- RII: In this region, the expansion of the universe is dominated by the energy density of the field, i.e., . Figure 4 shown the evolution of the DM yield for , GeV, GeV, GeV−2, and . In this case, the freeze-in happens at different but closer times, and it can be seen that the DM yield in the CDM model is slightly higher than the NSC scenario. This is produced by the expansion rate of the universe different to . Nevertheless, after the decay of , the entropy injection dilutes the DM relic density, bringing it to its current value.
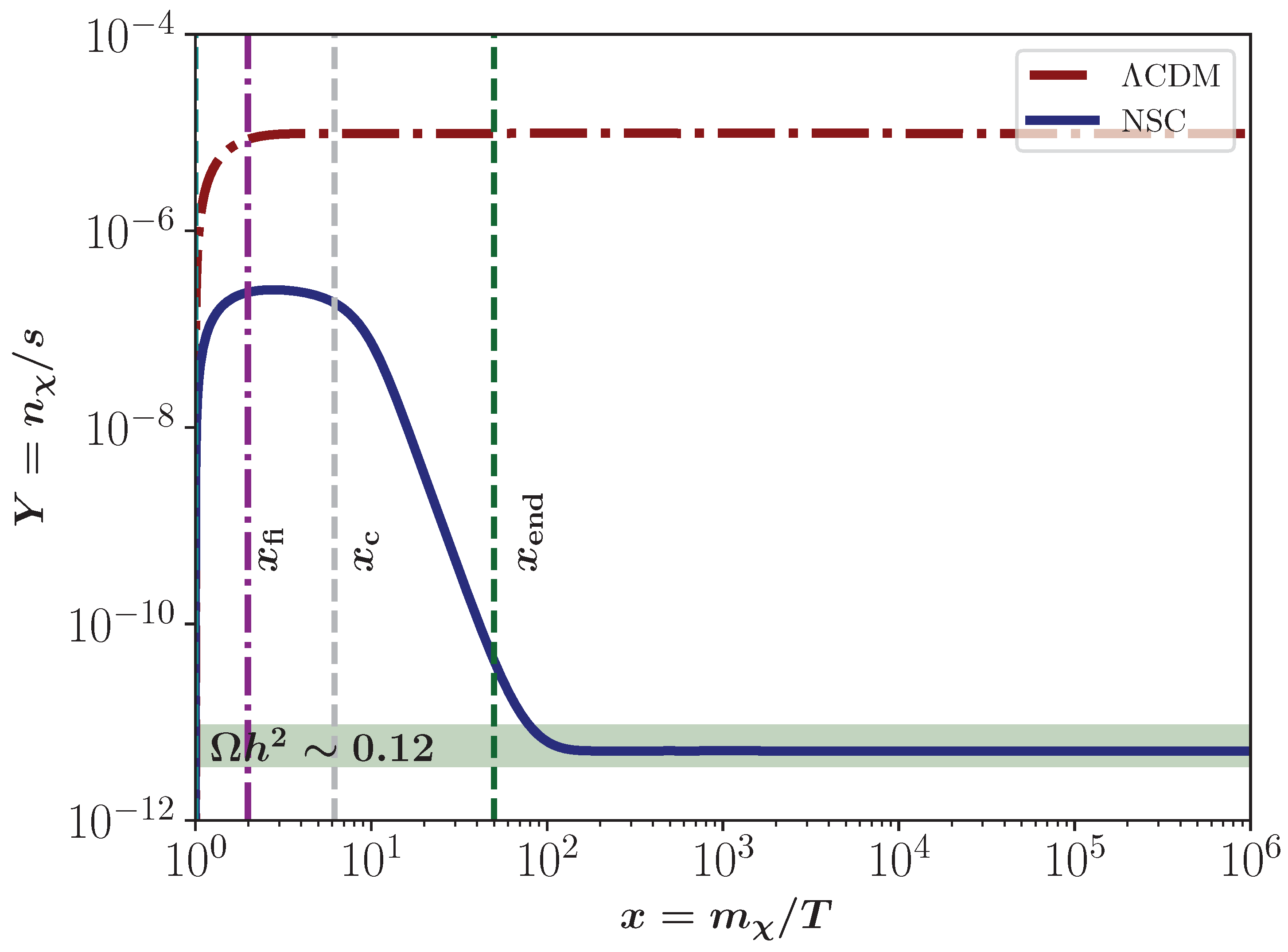
- RIII: For this case, the universe expansion is still dominated by the field, but the decays begin to inject entropy to the SM bath. The expansion rate can be approximated as , which means a decaying epoch. Figure 5 shows the evolution of the DM yield for , GeV, GeV, GeV−2, and . Once again, the particles freeze their abundance earlier in the NSC scenario compared to CDM model, resulting in a lower DM yield. As the decay of becomes significant, the resulting entropy injection dilutes the DM relic density, bringing it to its present value.
- RIV: Finally, in this region the filed has already fully decay and the CDM model is recovered. This region is not of our interest.
3. Bulk Viscous Non-Standard Cosmologies
3.1. Comparison Between Scenarios
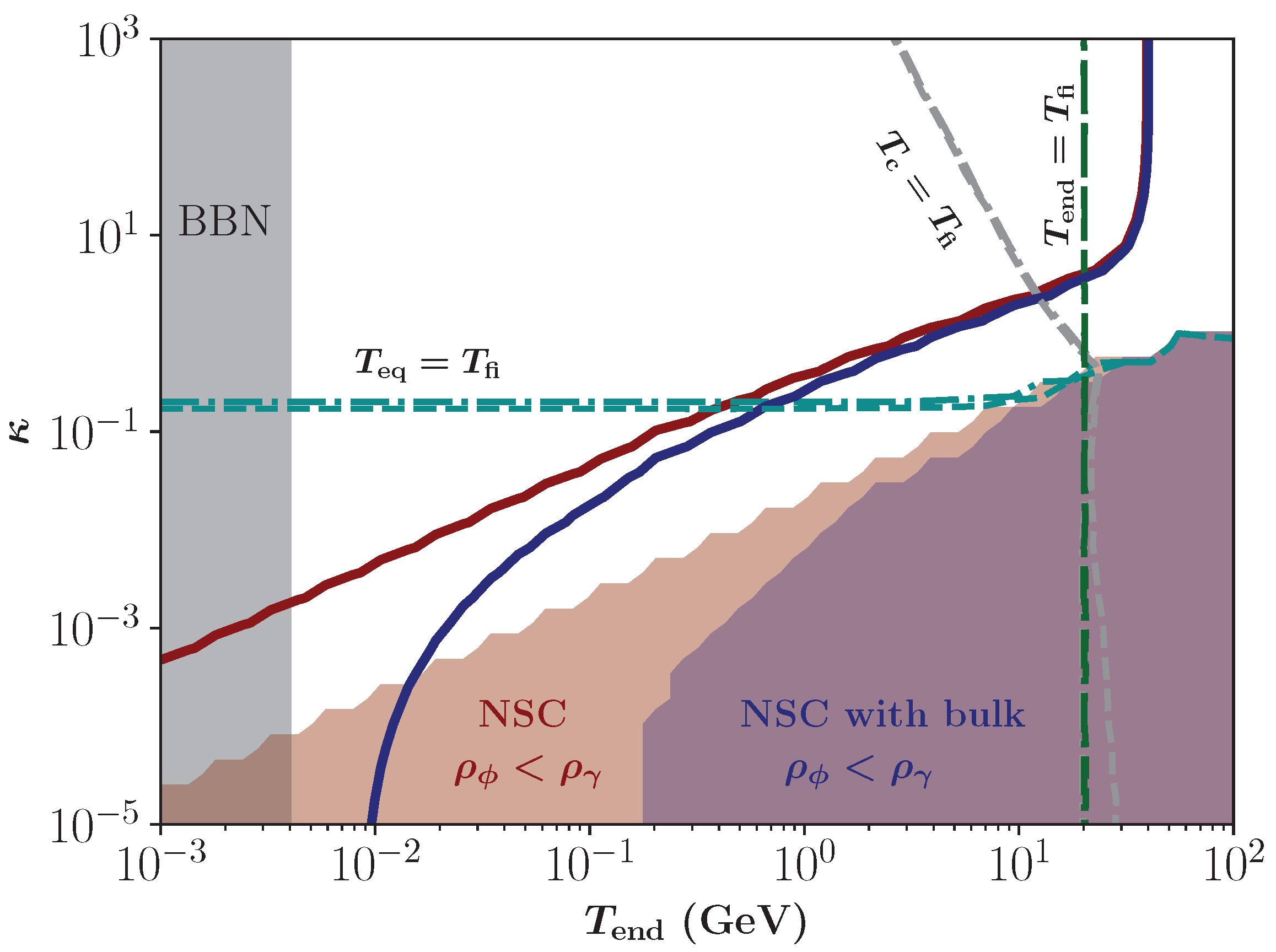
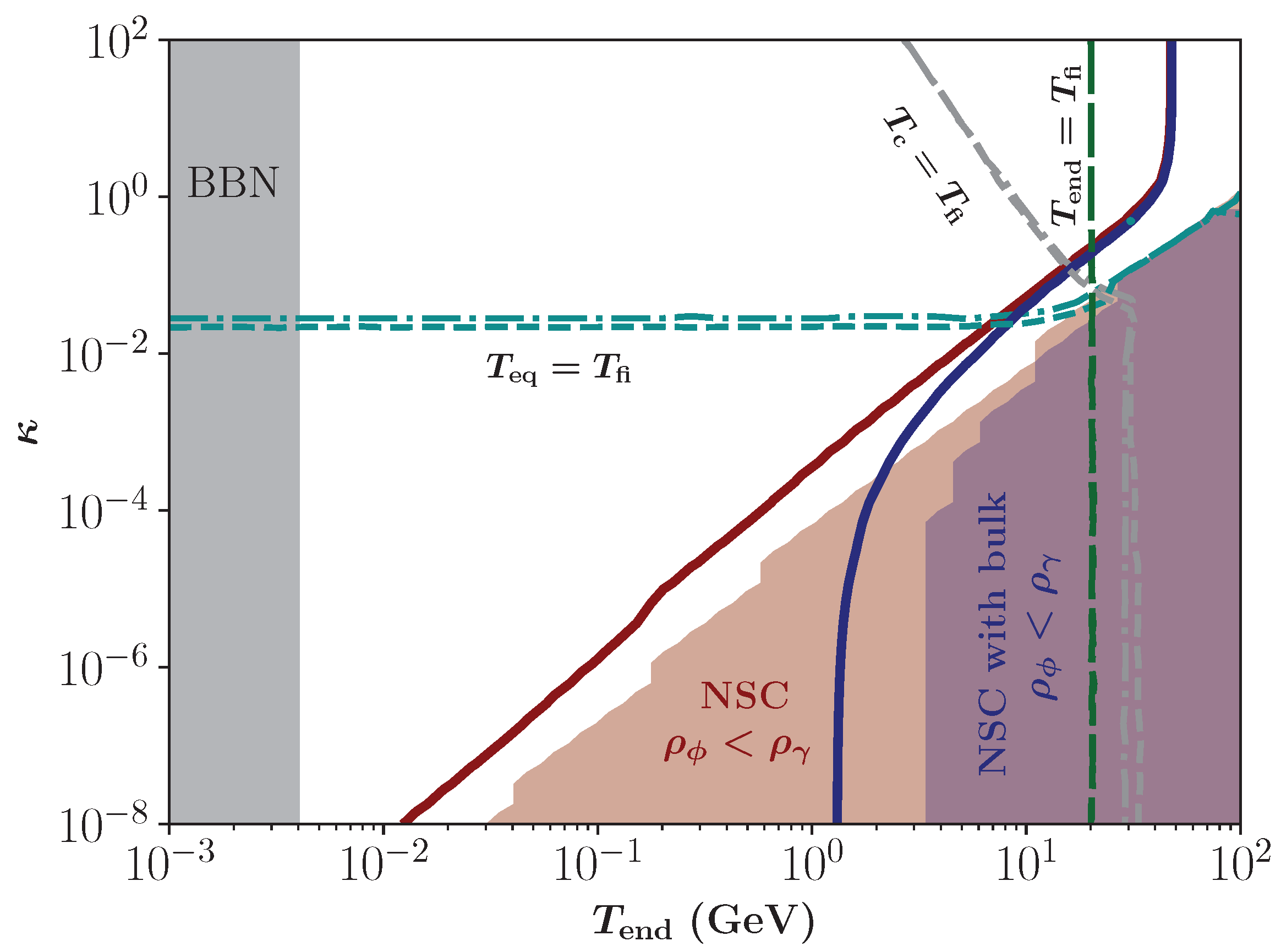
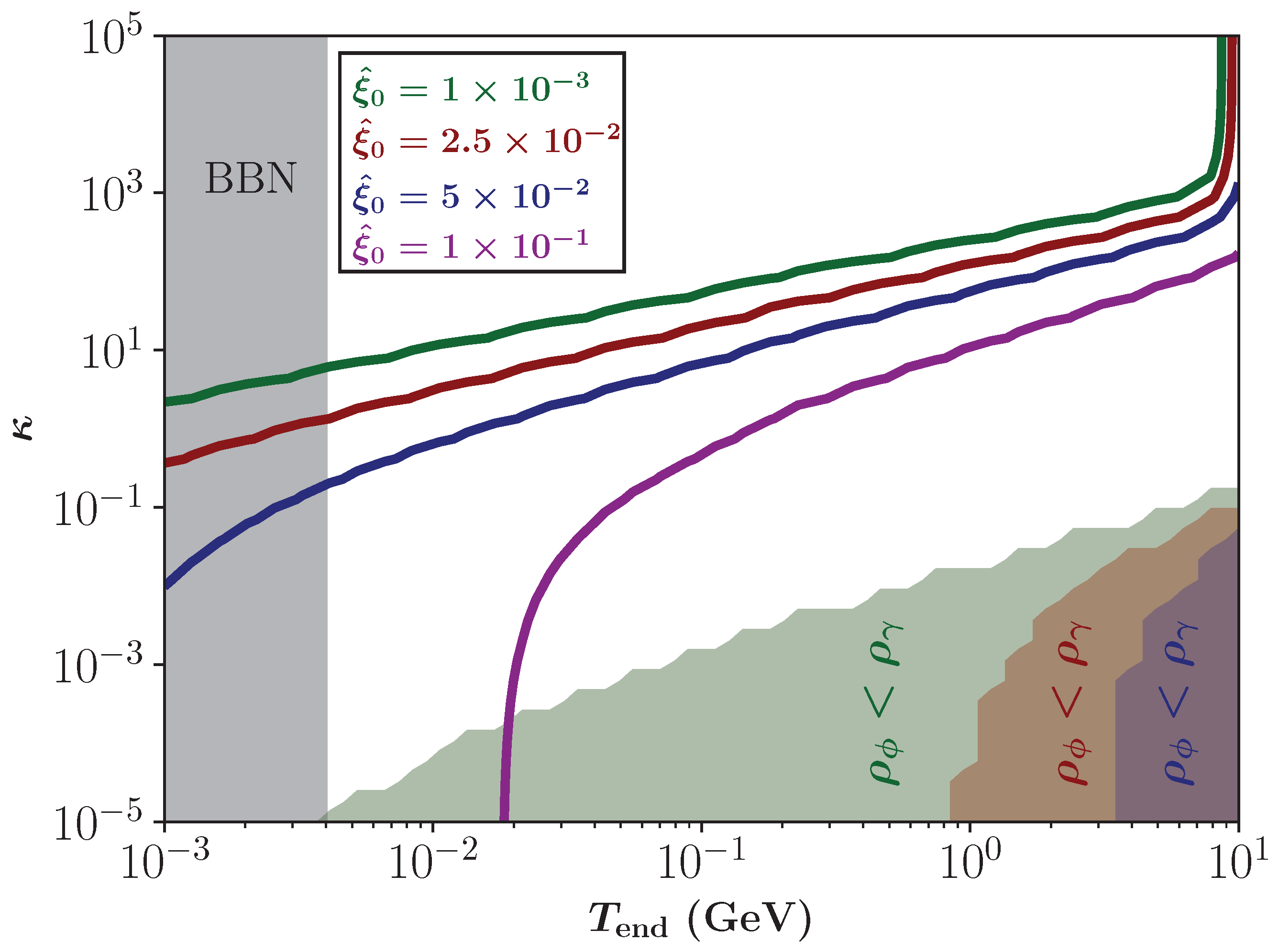
3.2. Parameter Spaces in the Bulk Viscous Non-Standard Cosmologies
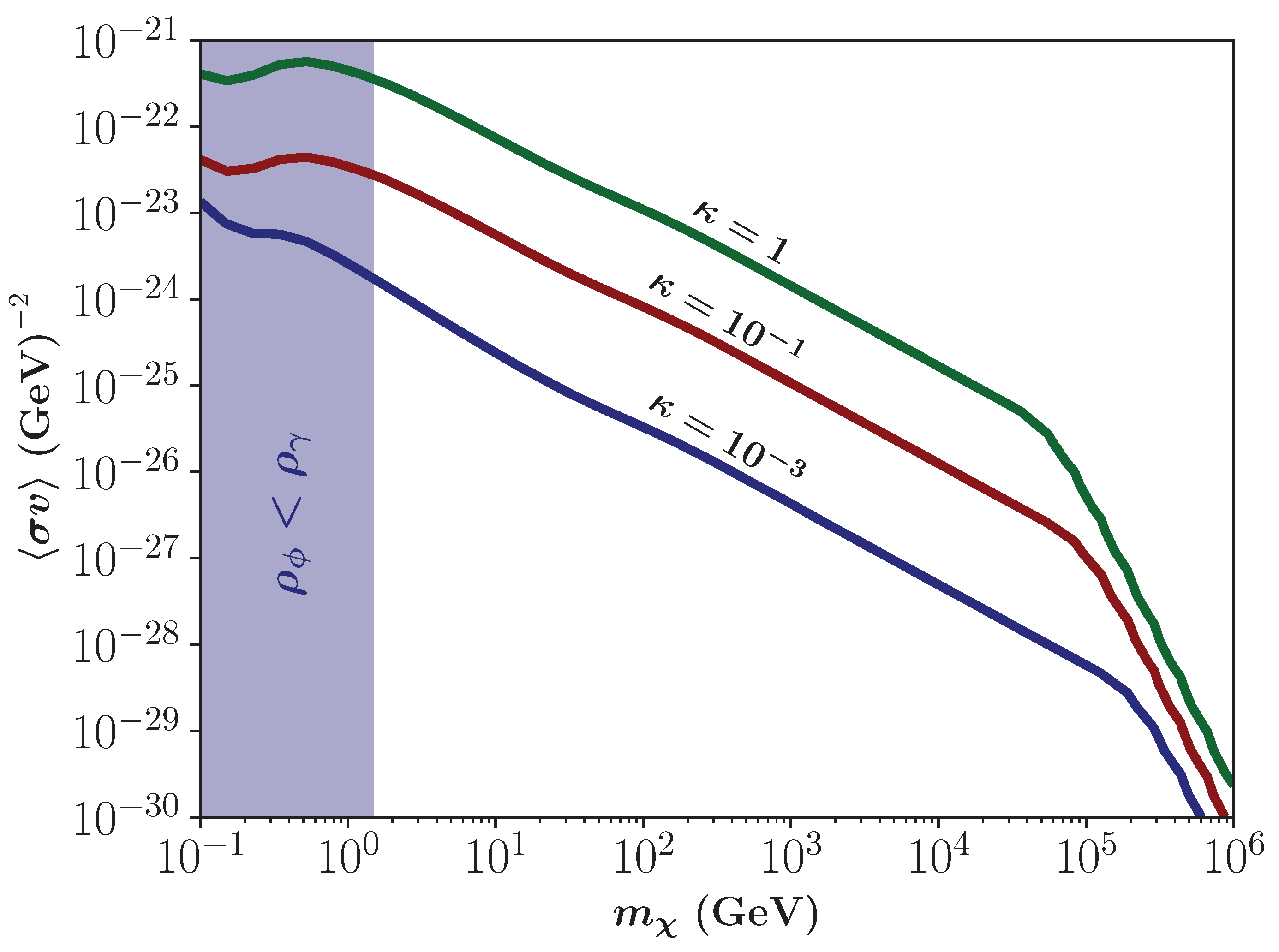
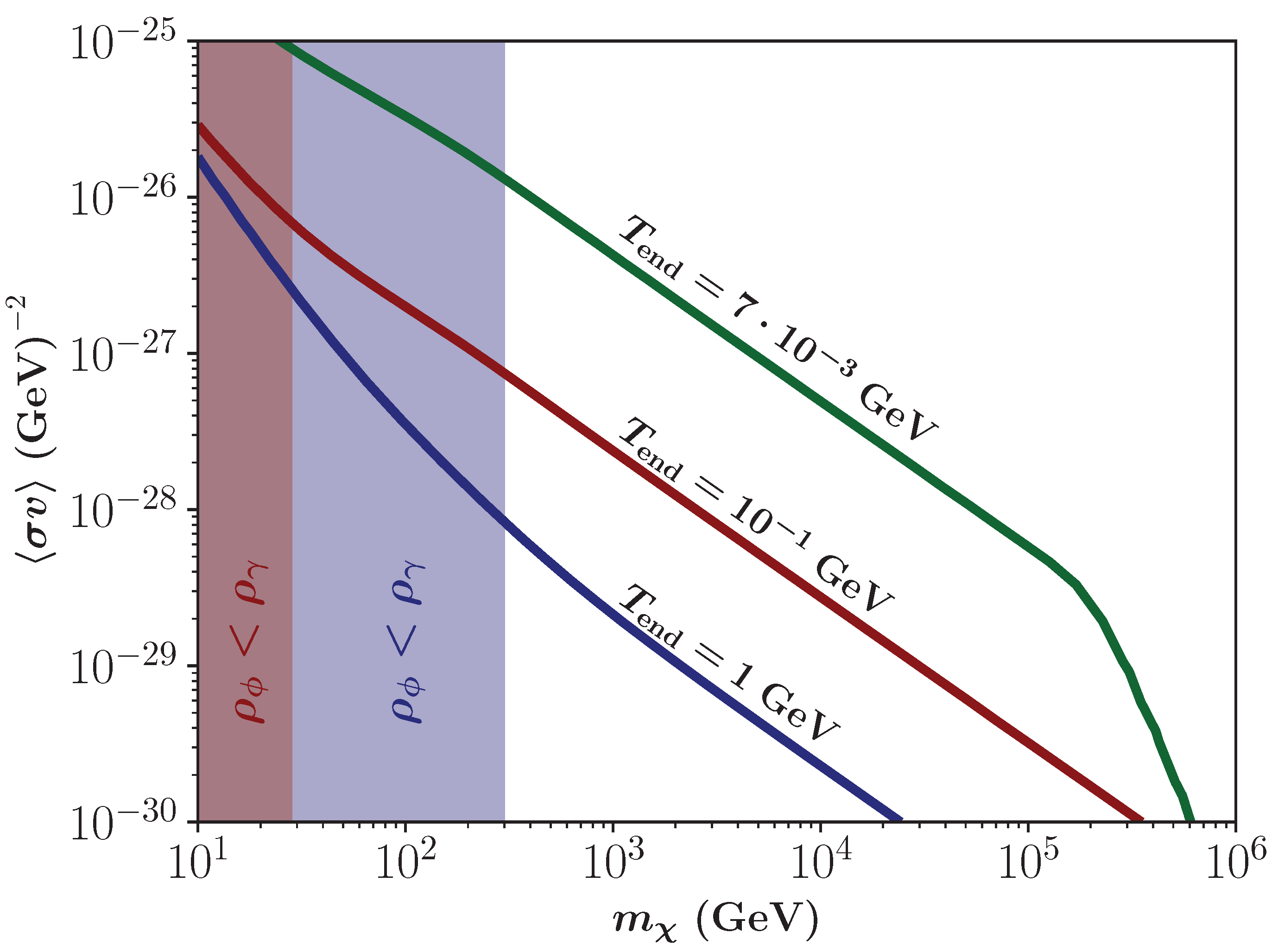
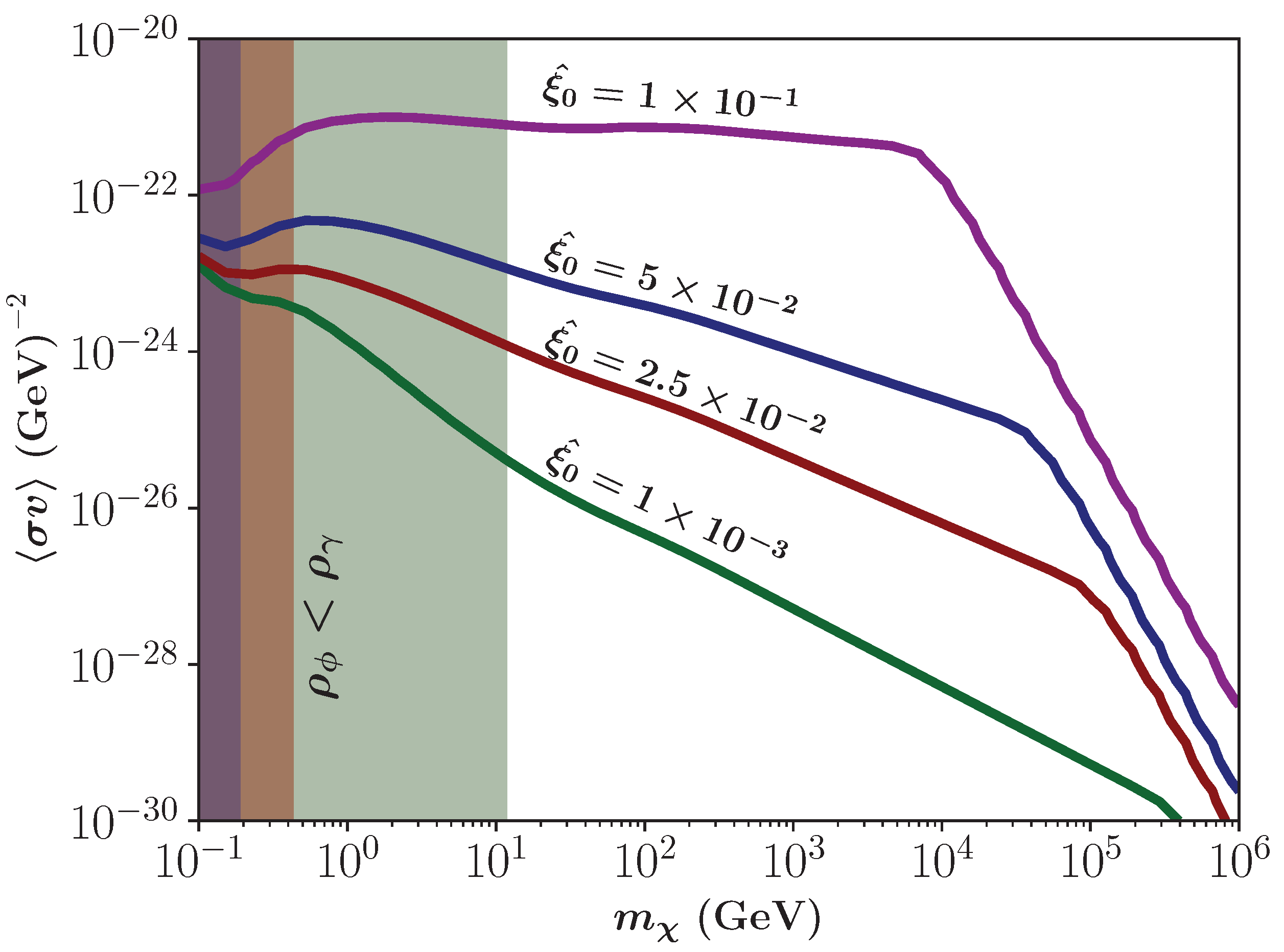
3.3. FIMPs for a Non-Constant Totally Thermal Averaged DM Production Cross-Section
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DM | Dark Matter |
| DE | Dark Energy |
| CDM | Cold Dark Matter |
| WIMPs | Weakly Interacting Massive Particles |
| FIMPs | Feebly Interacting Massive Particles |
| SM | tandard Model |
| WDM | Warm Dark Matter |
| NSCs | Non-Standard Cosmologies |
| BBN | Big Bang Nucleosynthesis |
| RI | Region I |
| RII | Region II |
| RIII | Region III |
| RVI | Region VI |
| UV | UltraViolet |
| IR | InfraRed |
References
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E.; et al. Measurements of Ω and Λ from 42 High Redshift Supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Scolnic, D.; Brout, D.; Carr, A.; Riess, A.G.; Davis, T.M.; Dwomoh, A.; Jones, D.O.; Ali, N.; Charvu, P.; Chen, R.; et al. The Pantheon+ Analysis: The Full Data Set and Light-curve Release. Astrophys. J. 2022, 938, 113. [Google Scholar] [CrossRef]
- Moresco, M.; Cimatti, A.; Jimenez, R.; Pozzetti, L.; Zamorani, G.; Bolzonella, M.; Dunlop, J.; Lamareille, F.; Mignoli, M.; Pearce, H.; et al. Improved constraints on the expansion rate of the Universe up to z~1.1 from the spectroscopic evolution of cosmic chronometers. J. Cosmol. Astropart. Phys. 2012, 2012, 006. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, H.; Yuan, S.; Zhang, T.J.; Sun, Y.C. Four new observational H(z) data from luminous red galaxies in the Sloan Digital Sky Survey data release seven. Res. Astron. Astrophys. 2014, 14, 1221–1233. [Google Scholar] [CrossRef]
- Moresco, M. Raising the bar: New constraints on the Hubble parameter with cosmic chronometers at z ∼ 2. Mon. Not. Roy. Astron. Soc. 2015, 450, L16–L20. [Google Scholar] [CrossRef]
- Eisenstein, D.J.; Zehavi, I.; Hogg, D.W.; Scoccimarro, R.; Blanton, M.R.; Nichol, R.C.; Scranton, R.; Seo, H.J.; Tegmark, M.; Zheng, Z.; et al. Detection of the Baryon Acoustic Peak in the Large-Scale Correlation Function of SDSS Luminous Red Galaxies. Astrophys. J. 2005, 633, 560–574. [Google Scholar] [CrossRef]
- Bennett, C.L.; Larson, D.; Weiland, J.L.; Jarosik, N.; Hinshaw, G.; Odegard, N.; Smith, K.M.; Hill, R.S.; Gold, B.; Halpern, M.; et al. Nine-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Final Maps and Results. Astrophys. J. Suppl. 2013, 208, 20. [Google Scholar] [CrossRef]
- Aghanim, N. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6, Erratum in Astron. Astrophys. 2021, 652, C4. [Google Scholar] [CrossRef]
- Riess, A.G.; Yuan, W.; Macri, L.M.; Scolnic, D.; Brout, D.; Casertano, S.; Jones, D.O.; Murakami, Y.; Anand, G.S.; Breuval, L.; et al. A Comprehensive Measurement of the Local Value of the Hubble Constant with 1 km s−1 Mpc−1 Uncertainty from the Hubble Space Telescope and the SH0ES Team. Astrophys. J. Lett. 2022, 934, L7. [Google Scholar] [CrossRef]
- Wong, K.C.; Suyu, S.H.; Chen, G.C.-F.; Rusu, C.E.; Millon, M.; Sluse, D.; Bonvin, V.; Fassnacht, C.D.; Taubenberger, S.; Auger, M.W.; et al. H0LiCOW – XIII. A 2.4 per cent measurement of H0 from lensed quasars: 5.3σ tension between early- and late-Universe probes. Mon. Not. Roy. Astron. Soc. 2020, 498, 1420–1439. [Google Scholar] [CrossRef]
- Jungman, G.; Kamionkowski, M.; Griest, K. Supersymmetric dark matter. Phys. Rept. 1996, 267, 195–373. [Google Scholar] [CrossRef]
- King, S.F.; Roberts, J.P. Natural Dark Matter from Type I String Theory. J. High Energy Phys. 2007, 2007, 024. [Google Scholar] [CrossRef]
- Steigman, G.; Turner, M.S. Cosmological Constraints on the Properties of Weakly Interacting Massive Particles. Nucl. Phys. B 1985, 253, 375–386. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D.; Silk, J. Particle dark matter: Evidence, candidates and constraints. Phys. Rept. 2005, 405, 279–390. [Google Scholar] [CrossRef]
- Arcadi, G.; Dutra, M.; Ghosh, P.; Lindner, M.; Mambrini, Y.; Pierre, M.; Profumo, S.; Queiroz, F.S. The waning of the WIMP? A review of models, searches, and constraints. Eur. Phys. J. C 2018, 78, 203. [Google Scholar] [CrossRef] [PubMed]
- Roszkowski, L.; Sessolo, E.M.; Trojanowski, S. WIMP dark matter candidates and searches—current status and future prospects. Rept. Prog. Phys. 2018, 81, 066201. [Google Scholar] [CrossRef]
- Arcadi, G.; Cabo-Almeida, D.; Dutra, M.; Ghosh, P.; Lindner, M.; Mambrini, Y.; Neto, J.P.; Pierre, M.; Profumo, S.; Queiroz, F.S. The Waning of the WIMP: Endgame? Eur. Phys. J. C 2025, 85, 1522024. [Google Scholar] [CrossRef]
- Singh, E.; Bharadwaj, H.; Devisharan. Exploring new physics via effective interactions. Int. J. Mod. Phys. A 2024, 39, 2450038. [Google Scholar] [CrossRef]
- Bernal, N.; Heikinheimo, M.; Tenkanen, T.; Tuominen, K.; Vaskonen, V. The Dawn of FIMP Dark Matter: A Review of Models and Constraints. Int. J. Mod. Phys. A 2017, 32, 1730023. [Google Scholar] [CrossRef]
- Chu, X.; Hambye, T.; Tytgat, M.H.G. The Four Basic Ways of Creating Dark Matter Through a Portal. J. Cosmol. Astropart. Phys. 2012, 2012, 034. [Google Scholar] [CrossRef]
- Hall, L.J.; Jedamzik, K.; March-Russell, J.; West, S.M. Freeze-In Production of FIMP Dark Matter. J. High Energy Phys. 2010, 2010, 080. [Google Scholar] [CrossRef]
- Abi, B.; Acciarri, R.; Acero, M.A.; Adamov, G.; Adams, D.; Adinolfi, M.; Ahmad, Z.; Ahmed, J.; Alion, T.; Monsalve, S.A.; et al. Deep Underground Neutrino Experiment (DUNE), Far Detector Technical Design Report, Volume I Introduction to DUNE. J. Instrum. 2020, 15, T08008. [Google Scholar] [CrossRef]
- Feng, J.L. Dark Matter Candidates from Particle Physics and Methods of Detection. Ann. Rev. Astron. Astrophys. 2010, 48, 495–545. [Google Scholar] [CrossRef]
- Alves, A., Jr.; Filho, L.M.A.; Barbosa, A.F.; Bediaga, I.; Cernicchiaro, G.; Guerrer, G.; Lima, H.P., Jr.; Machado, A.A.; Magnin, J.; Marujo, F.; et al. The LHCb Detector at the LHC. J. Instrum. 2008, 3, S08005. [Google Scholar] [CrossRef]
- Steigman, G.; Dasgupta, B.; Beacom, J.F. Precise Relic WIMP Abundance and its Impact on Searches for Dark Matter Annihilation. Phys. Rev. D 2012, 86, 023506. [Google Scholar] [CrossRef]
- Frigerio, M.; Hambye, T.; Masso, E. Sub-GeV dark matter as pseudo-Goldstone from the seesaw scale. Phys. Rev. X 2011, 1, 021026. [Google Scholar] [CrossRef]
- Wang, Z.; Reyimuaji, Y.; Yalikun, N. A Z4 symmetric inverse seesaw model for neutrino masses and FIMP dark matter. arXiv 2024, arXiv:2412.15672. [Google Scholar]
- Babu, K.S.; Chakdar, S.; Das, N.; Ghosh, D.K.; Ghosh, P. FIMP dark matter from flavon portals. J. High Energy Phys. 2023, 2023, 143. [Google Scholar] [CrossRef]
- Junius, S. Searching for Feebly Interacting Dark Matter in Colliders and Beam-Dump Experiments. Ph.D. Thesis, Vrije Universiteit Brussel, Brussels, Belgium, 2022. [Google Scholar]
- Barman, B.; Bhattacharya, S.; Jahedi, S.; Pradhan, D.; Sarkar, A. Lepton Collider as a window to Reheating. arXiv 2024, arXiv:2406.11963. [Google Scholar]
- Bélanger, G.; Chakraborti, S.; Pukhov, A. Feebly-interacting dark matter. Eur. Phys. J. ST 2024, 233, 11–12. [Google Scholar] [CrossRef]
- Blinnikov, S.I.; Khlopov, M.Y. On Possible Effects of ’Mirror’ Particles. Sov. J. Nucl. Phys. 1982, 36, 472. [Google Scholar]
- Blinnikov, S.I.; Khlopov, M. Possible astronomical effects of mirror particles. Sov. Astron. 1983, 27, 371–375. [Google Scholar]
- Khlopov, M.Y.; Beskin, G.M.; Bochkarev, N.E.; Pustylnik, L.A.; Pustylnik, S.A. Observational Physics of Mirror World. Sov. Astron. 1991, 35, 21. [Google Scholar]
- D’Eramo, F.; Lenoci, A. Lower mass bounds on FIMP dark matter produced via freeze-in. J. Cosmol. Astropart. Phys. 2021, 10, 045. [Google Scholar] [CrossRef]
- McDonald, J. Warm Dark Matter via Ultra-Violet Freeze-In: Reheating Temperature and Non-Thermal Distribution for Fermionic Higgs Portal Dark Matter. J. Cosmol. Astropart. Phys. 2016, 2016, 035. [Google Scholar] [CrossRef]
- Klasen, M.; Yaguna, C.E. Warm and cold fermionic dark matter via freeze-in. J. Cosmol. Astropart. Phys. 2013, 11, 039. [Google Scholar] [CrossRef]
- de Giorgi, A.; Vogl, S. Warm dark matter from a gravitational freeze-in in extra dimensions. J. High Energy Phys. 2023, 2023, 032. [Google Scholar] [CrossRef]
- Kuo, J.L.; Lattanzi, M.; Cheung, K.; Valle, J.W.F. Decaying warm dark matter and structure formation. J. Cosmol. Astropart. Phys. 2018, 12, 026. [Google Scholar] [CrossRef]
- Bode, P.; Ostriker, J.P.; Turok, N. Halo formation in warm dark matter models. Astrophys. J. 2001, 556, 93–107. [Google Scholar] [CrossRef]
- de Vega, H.J.; Sanchez, N.G. Warm dark matter in the galaxies:theoretical and observational progresses. Highlights and conclusions of the chalonge meudon workshop 2011. arXiv 2011, arXiv:1109.3187. [Google Scholar]
- Klypin, A.A.; Kravtsov, A.V.; Valenzuela, O.; Prada, F. Where are the missing Galactic satellites? Astrophys. J. 1999, 522, 82–92. [Google Scholar] [CrossRef]
- Moore, B.; Ghigna, S.; Governato, F.; Lake, G.; Quinn, T.R.; Stadel, J.; Tozzi, P. Dark matter substructure within galactic halos. Astrophys. J. Lett. 1999, 524, L19–L22. [Google Scholar] [CrossRef]
- Weinberg, D.H.; Bullock, J.S.; Governato, F.; Kuzio de Naray, R.; Peter, A.H.G. Cold dark matter: Controversies on small scales. Proc. Nat. Acad. Sci. USA 2015, 112, 12249–12255. [Google Scholar] [CrossRef]
- Viel, M.; Lesgourgues, J.; Haehnelt, M.G.; Matarrese, S.; Riotto, A. Constraining warm dark matter candidates including sterile neutrinos and light gravitinos with WMAP and the Lyman-alpha forest. Phys. Rev. D 2005, 71, 063534. [Google Scholar] [CrossRef]
- Newton, O.; Leo, M.; Cautun, M.; Jenkins, A.; Frenk, C.S.; Lovell, M.R.; Helly, J.C.; Benson, A.J.; Cole, S. Constraints on the properties of warm dark matter using the satellite galaxies of the Milky Way. J. Cosmol. Astropart. Phys. 2021, 2021, 062. [Google Scholar] [CrossRef]
- Maartens, R. Causal thermodynamics in relativity. arXiv 1996, arXiv:astro-ph/9609119. [Google Scholar]
- Pandey, K.L.; Karwal, T.; Das, S. Alleviating the H0 and σ8 anomalies with a decaying dark matter model. J. Cosmol. Astropart. Phys. 2020, 2020, 026. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Di Valentino, E.; Paliathanasis, A.; Lu, J. Challenging bulk viscous unified scenarios with cosmological observations. Phys. Rev. D 2019, 100, 103518. [Google Scholar] [CrossRef]
- Di Valentino, E.; Mena, O.; Pan, S.; Visinelli, L.; Yang, W.; Melchiorri, A.; Mota, D.F.; Riess, A.G.; Silk, J. In the realm of the Hubble tension—a review of solutions. Class. Quant. Grav. 2021, 38, 153001. [Google Scholar] [CrossRef]
- Normann, B.D.; Brevik, I.H. Can the Hubble tension be resolved by bulk viscosity? Mod. Phys. Lett. A 2021, 36, 2150198. [Google Scholar] [CrossRef]
- Brevik, I.; Grøn, O.; de Haro, J.; Odintsov, S.D.; Saridakis, E.N. Viscous Cosmology for Early- and Late-Time Universe. Int. J. Mod. Phys. D 2017, 26, 1730024. [Google Scholar] [CrossRef]
- Eckart, C. The Thermodynamics of Irreversible Processes. I. The Simple Fluid. Phys. Rev. 1940, 58, 267–269. [Google Scholar] [CrossRef]
- Eckart, C. The Thermodynamics of Irreversible Processes. III. Relativistic Theory of the Simple Fluid. Phys. Rev. 1940, 58, 919–924. [Google Scholar] [CrossRef]
- Stewart, J.M. On transient relativistic thermodynamics and kinetic theory. Proc. R. Soc. A 1977, 357, 59–75. [Google Scholar] [CrossRef]
- Israel, W.; Stewart, J.M. On transient relativistic thermodynamics and kinetic theory. II. Proc. R. Soc. A 1979, 365, 43–52. [Google Scholar] [CrossRef]
- Israel, W. Nonstationary irreversible thermodynamics: A Causal relativistic theory. Ann. Phys. 1976, 100, 310–331. [Google Scholar] [CrossRef]
- Maartens, R. Dissipative cosmology. Class. Quant. Grav. 1995, 12, 1455–1465. [Google Scholar] [CrossRef]
- Floerchinger, S.; Tetradis, N.; Wiedemann, U.A. Accelerating Cosmological Expansion from Shear and Bulk Viscosity. Phys. Rev. Lett. 2015, 114, 091301. [Google Scholar] [CrossRef] [PubMed]
- Zimdahl, W. ‘Understanding’ cosmological bulk viscosity. Mon. Not. Roy. Astron. Soc. 1996, 280, 1239. [Google Scholar] [CrossRef]
- Wilson, J.R.; Mathews, G.J.; Fuller, G.M. Bulk Viscosity, Decaying Dark Matter, and the Cosmic Acceleration. Phys. Rev. D 2007, 75, 043521. [Google Scholar] [CrossRef]
- Mathews, G.J.; Lan, N.Q.; Kolda, C. Late Decaying Dark Matter, Bulk Viscosity and the Cosmic Acceleration. Phys. Rev. D 2008, 78, 043525. [Google Scholar] [CrossRef]
- Hofmann, S.; Schwarz, D.J.; Stoecker, H. Damping scales of neutralino cold dark matter. Phys. Rev. D 2001, 64, 083507. [Google Scholar] [CrossRef]
- Foot, R.; Vagnozzi, S. Dissipative hidden sector dark matter. Phys. Rev. D 2015, 91, 023512. [Google Scholar] [CrossRef]
- Foot, R.; Vagnozzi, S. Solving the small-scale structure puzzles with dissipative dark matter. J. Cosmol. Astropart. Phys. 2016, 2016, 013. [Google Scholar] [CrossRef]
- Goswami, G.; Chakravarty, G.K.; Mohanty, S.; Prasanna, A.R. Constraints on cosmological viscosity and self interacting dark matter from gravitational wave observations. Phys. Rev. D 2017, 95, 103509. [Google Scholar] [CrossRef]
- Alford, M.G.; Bovard, L.; Hanauske, M.; Rezzolla, L.; Schwenzer, K. Viscous Dissipation and Heat Conduction in Binary Neutron-Star Mergers. Phys. Rev. Lett. 2018, 120, 041101. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. The Final state and thermodynamics of dark energy universe. Phys. Rev. D 2004, 70, 103522. [Google Scholar] [CrossRef]
- Capozziello, S.; Cardone, V.F.; Elizalde, E.; Nojiri, S.; Odintsov, S.D. Observational constraints on dark energy with generalized equations of state. Phys. Rev. D 2006, 73, 043512. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Inhomogeneous equation of state of the universe: Phantom era, future singularity and crossing the phantom barrier. Phys. Rev. D 2005, 72, 023003. [Google Scholar] [CrossRef]
- Cataldo, M.; Cruz, N.; Lepe, S. Viscous dark energy and phantom evolution. Phys. Lett. B 2005, 619, 5–10. [Google Scholar] [CrossRef]
- Brevik, I.H. Crossing of the W = -1 barrier in two-fluid viscous modified gravity. Gen. Rel. Grav. 2006, 38, 1317–1328. [Google Scholar] [CrossRef]
- Cruz, N.; Lepe, S.; Peña, F. Crossing the phantom divide with dissipative normal matter in the Israel–Stewart formalism. Phys. Lett. B 2017, 767, 103–109. [Google Scholar] [CrossRef]
- Velten, H.; Schwarz, D. Dissipation of dark matter. Phys. Rev. D 2012, 86, 083501. [Google Scholar] [CrossRef]
- Acquaviva, G.; Beesham, A. Nonlinear bulk viscosity and the stability of accelerated expansion in FRW spacetime. Phys. Rev. D 2014, 90, 023503. [Google Scholar] [CrossRef]
- Cruz, M.; Cruz, N.; Lepe, S. Accelerated and decelerated expansion in a causal dissipative cosmology. Phys. Rev. D 2017, 96, 124020. [Google Scholar] [CrossRef]
- Cruz, N.; González, E.; Lepe, S.; Sáez-Chillón Gómez, D. Analysing dissipative effects in the ΛCDM model. J. Cosmol. Astropart. Phys. 2018, 12, 017. [Google Scholar] [CrossRef]
- Cruz, N.; González, E.; Jovel, J. Study of a Viscous ΛWDM Model: Near-Equilibrium Condition, Entropy Production, and Cosmological Constraints. Symmetry 2022, 14, 1866. [Google Scholar] [CrossRef]
- Cruz, N.; González, E.; Jovel, J. A non-singular early-time viscous cosmological model. Mod. Phys. Lett. A 2023, 38, 2350088. [Google Scholar] [CrossRef]
- Gómez, G.; Palma, G.; González, E.; Rincón, A.; Cruz, N. A new parametrization for bulk viscosity cosmology as extension of the ΛCDM model. Eur. Phys. J. Plus 2023, 138, 738. [Google Scholar] [CrossRef]
- Cruz, N.; Gomez, G.; González, E.; Palma, G.; Rincon, A. Exploring models of running vacuum energy with viscous dark matter from a dynamical system perspective. Phys. Dark Univ. 2023, 42, 101351. [Google Scholar] [CrossRef]
- Fabris, J.C.; Goncalves, S.V.B.; de Sa Ribeiro, R. Bulk viscosity driving the acceleration of the Universe. Gen. Rel. Grav. 2006, 38, 495–506. [Google Scholar] [CrossRef]
- Avelino, A.; Nucamendi, U. Can a matter-dominated model with constant bulk viscosity drive the accelerated expansion of the universe? J. Cosmol. Astropart. Phys. 2009, 2009, 006. [Google Scholar] [CrossRef]
- Li, B.; Barrow, J.D. Does Bulk Viscosity Create a Viable Unified Dark Matter Model? Phys. Rev. D 2009, 79, 103521. [Google Scholar] [CrossRef]
- Avelino, A.; Nucamendi, U. Exploring a matter-dominated model with bulk viscosity to drive the accelerated expansion of the Universe. J. Cosmol. Astropart. Phys. 2010, 2010, 009. [Google Scholar] [CrossRef]
- Hipolito-Ricaldi, W.S.; Velten, H.E.S.; Zimdahl, W. The Viscous Dark Fluid Universe. Phys. Rev. D 2010, 82, 063507. [Google Scholar] [CrossRef]
- Velten, H.; Schwarz, D.J. Constraints on dissipative unified dark matter. J. Cosmol. Astropart. Phys. 2011, 2011, 016. [Google Scholar] [CrossRef]
- Gagnon, J.S.; Lesgourgues, J. Dark goo: Bulk viscosity as an alternative to dark energy. J. Cosmol. Astropart. Phys. 2011, 2011, 026. [Google Scholar] [CrossRef]
- Bruni, M.; Lazkoz, R.; Rozas-Fernandez, A. Phenomenological models for Unified Dark Matter with fast transition. Mon. Not. Roy. Astron. Soc. 2013, 431, 2907–2916. [Google Scholar] [CrossRef]
- Cruz, M.; Cruz, N.; Lepe, S. Phantom solution in a non-linear Israel–Stewart theory. Phys. Lett. B 2017, 769, 159–165. [Google Scholar] [CrossRef]
- Cruz, N.; González, E.; Palma, G. Exact analytical solution for an Israel–Stewart cosmology. Gen. Rel. Grav. 2020, 52, 62. [Google Scholar] [CrossRef]
- Cruz, N.; González, E.; Palma, G. Testing dissipative dark matter in causal thermodynamics. Mod. Phys. Lett. A 2021, 36, 2150032. [Google Scholar] [CrossRef]
- Mak, M.K.; Harko, T. Full causal dissipative cosmologies with stiff matter. Int. J. Mod. Phys. D 2004, 13, 273–280. [Google Scholar] [CrossRef]
- Padmanabhan, T.; Chitre, S.M. Viscous universes. Phys. Lett. A 1987, 120, 433–436. [Google Scholar] [CrossRef]
- Barrow, J.D. String-Driven Inflationary and Deflationary Cosmological Models. Nucl. Phys. B 1988, 310, 743–763. [Google Scholar] [CrossRef]
- Maartens, R.; Mendez, V. Nonlinear bulk viscosity and inflation. Phys. Rev. D 1997, 55, 1937–1942. [Google Scholar] [CrossRef]
- Avelino, A.; Leyva, Y.; Urena-Lopez, L.A. Interacting viscous dark fluids. Phys. Rev. D 2013, 88, 123004. [Google Scholar] [CrossRef]
- Hernández-Almada, A.; García-Aspeitia, M.A.; Magaña, J.; Motta, V. Stability analysis and constraints on interacting viscous cosmology. Phys. Rev. D 2020, 101, 063516. [Google Scholar] [CrossRef]
- Brevik, I.H.; Gorbunova, O.; Shaido, Y.A. Viscous FRW cosmology in modified gravity. Int. J. Mod. Phys. D 2005, 14, 1899–1906. [Google Scholar] [CrossRef]
- Brevik, I.H.; Gorbunova, O. Dark energy and viscous cosmology. Gen. Rel. Grav. 2005, 37, 2039–2045. [Google Scholar] [CrossRef]
- Brevik, I.H.; Gorbunova, O. Viscous Dark Cosmology with Account of Quantum Effects. Eur. Phys. J. C 2008, 56, 425–428. [Google Scholar] [CrossRef]
- Brevik, I.; Gorbunova, O.; Saez-Gomez, D. Casimir Effects Near the Big Rip Singularity in Viscous Cosmology. Gen. Rel. Grav. 2010, 42, 1513–1522. [Google Scholar] [CrossRef]
- Brevik, I.; Nojiri, S.; Odintsov, S.D.; Saez-Gomez, D. Cardy-Verlinde formula in FRW Universe with inhomogeneous generalized fluid and dynamical entropy bounds near the future singularity. Eur. Phys. J. C 2010, 69, 563–574. [Google Scholar] [CrossRef]
- Brevik, I.; Elizalde, E.; Nojiri, S.; Odintsov, S.D. Viscous Little Rip Cosmology. Phys. Rev. D 2011, 84, 103508. [Google Scholar] [CrossRef]
- Contreras, F.; Cruz, N.; González, E. Generalized equations of state and regular universes. J. Phys. Conf. Ser. 2016, 720, 012014. [Google Scholar] [CrossRef]
- Contreras, F.; Cruz, N.; Elizalde, E.; González, E.; Odintsov, S. Linking little rip cosmologies with regular early universes. Phys. Rev. D 2018, 98, 123520. [Google Scholar] [CrossRef]
- Cruz, N.; González, E.; Jovel, J. Singularities and soft-Big Bang in a viscous ΛCDM model. Phys. Rev. D 2022, 105, 024047. [Google Scholar] [CrossRef]
- Barta, D. Effect of viscosity and thermal conductivity on the radial oscillation and relaxation of relativistic stars. Class. Quant. Grav. 2019, 36, 215012. [Google Scholar] [CrossRef]
- Bravo Medina, S.; Nowakowski, M.; Batic, D. Viscous Cosmologies. Class. Quant. Grav. 2019, 36, 215002. [Google Scholar] [CrossRef]
- González, E.; Maldonado, C.; Mite, N.S.; Salinas, R. WIMP Dark Matter in bulk viscous non-standard cosmologies. J. Cosmol. Astropart. Phys. 2024, 10, 088. [Google Scholar] [CrossRef]
- Giudice, G.F.; Kolb, E.W.; Riotto, A. Largest temperature of the radiation era and its cosmological implications. Phys. Rev. D 2001, 64, 023508. [Google Scholar] [CrossRef]
- Hamdan, S.; Unwin, J. Dark Matter Freeze-out During Matter Domination. Mod. Phys. Lett. A 2018, 33, 1850181. [Google Scholar] [CrossRef]
- D’Eramo, F.; Fernandez, N.; Profumo, S. Dark Matter Freeze-in Production in Fast-Expanding Universes. J. Cosmol. Astropart. Phys. 2018, 2018, 046. [Google Scholar] [CrossRef]
- D’Eramo, F.; Fernandez, N.; Profumo, S. When the Universe Expands Too Fast: Relentless Dark Matter. J. Cosmol. Astropart. Phys. 2017, 2017, 012. [Google Scholar] [CrossRef]
- Visinelli, L. (Non-)thermal production of WIMPs during kination. Symmetry 2018, 10, 546. [Google Scholar] [CrossRef]
- Drees, M.; Hajkarim, F. Neutralino Dark Matter in Scenarios with Early Matter Domination. J. High Energy Phys. 2018, 12, 042. [Google Scholar] [CrossRef]
- Bernal, N.; Cosme, C.; Tenkanen, T. Phenomenology of Self-Interacting Dark Matter in a Matter-Dominated Universe. Eur. Phys. J. C 2019, 79, 99. [Google Scholar] [CrossRef]
- Bernal, N.; Cosme, C.; Tenkanen, T.; Vaskonen, V. Scalar singlet dark matter in non-standard cosmologies. Eur. Phys. J. C 2019, 79, 30. [Google Scholar] [CrossRef]
- Maldonado, C.; Unwin, J. Establishing the Dark Matter Relic Density in an Era of Particle Decays. J. Cosmol. Astropart. Phys. 2019, 2019, 037. [Google Scholar] [CrossRef]
- Arias, P.; Bernal, N.; Herrera, A.; Maldonado, C. Reconstructing Non-standard Cosmologies with Dark Matter. J. Cosmol. Astropart. Phys. 2019, 10, 047. [Google Scholar] [CrossRef]
- Bernal, N.; Elahi, F.; Maldonado, C.; Unwin, J. Ultraviolet Freeze-in and Non-Standard Cosmologies. J. Cosmol. Astropart. Phys. 2019, 11, 026. [Google Scholar] [CrossRef]
- Arias, P.; Bernal, N.; Karamitros, D.; Maldonado, C.; Roszkowski, L.; Venegas, M. New opportunities for axion dark matter searches in nonstandard cosmological models. J. Cosmol. Astropart. Phys. 2021, 11, 003. [Google Scholar] [CrossRef]
- Bernal, N.; Xu, Y. WIMPs during reheating. J. Cosmol. Astropart. Phys. 2022, 12, 017. [Google Scholar] [CrossRef]
- Silva-Malpartida, J.; Bernal, N.; Jones-Pérez, J.; Lineros, R.A. From WIMPs to FIMPs: Impact of Early Matter Domination. J. Cosmol. Astropart. Phys. 2025, 2025, 003. [Google Scholar] [CrossRef]
- Drees, M.; Hajkarim, F. Dark Matter Production in an Early Matter Dominated Era. J. Cosmol. Astropart. Phys. 2018, 2018, 057. [Google Scholar] [CrossRef]
- Sarkar, S. Big bang nucleosynthesis and physics beyond the standard model. Rept. Prog. Phys. 1996, 59, 1493–1610. [Google Scholar] [CrossRef]
- Hannestad, S. What is the lowest possible reheating temperature? Phys. Rev. D 2004, 70, 043506. [Google Scholar] [CrossRef]
- De Bernardis, F.; Pagano, L.; Melchiorri, A. New constraints on the reheating temperature of the universe after WMAP-5. Astropart. Phys. 2008, 30, 192–195. [Google Scholar] [CrossRef]
- Kofman, L.; Linde, A.D.; Starobinsky, A.A. Towards the theory of reheating after inflation. Phys. Rev. D 1997, 56, 3258–3295. [Google Scholar] [CrossRef]
- Ackerman, L.; Fischler, W.; Kundu, S.; Sivanandam, N. Constraining the Inflationary Equation of State. J. Cosmol. Astropart. Phys. 2011, 2011, 024. [Google Scholar] [CrossRef]
- Garcia, M.A.G.; Kaneta, K.; Mambrini, Y.; Olive, K.A. Reheating and Post-inflationary Production of Dark Matter. Phys. Rev. D 2020, 101, 123507. [Google Scholar] [CrossRef]
- Garcia, M.A.G.; Kaneta, K.; Mambrini, Y.; Olive, K.A. Inflaton Oscillations and Post-Inflationary Reheating. J. Cosmol. Astropart. Phys. 2021, 2021, 012. [Google Scholar] [CrossRef]
- Silva-Malpartida, J.; Bernal, N.; Jones-Pérez, J.; Lineros, R.A. From WIMPs to FIMPs with low reheating temperatures. J. Cosmol. Astropart. Phys. 2023, 2023, 015. [Google Scholar] [CrossRef]
- Haque, M.R.; Maity, D.; Mondal, R. Thermal and non-thermal dark matters with gravitational neutrino reheating. Phys. Rev. D 2025, 111, 063546. [Google Scholar] [CrossRef]
- Gelmini, G.B.; Gondolo, P. Neutralino with the right cold dark matter abundance in (almost) any supersymmetric model. Phys. Rev. D 2006, 74, 023510. [Google Scholar] [CrossRef]
- Gelmini, G.; Gondolo, P.; Soldatenko, A.; Yaguna, C.E. The Effect of a late decaying scalar on the neutralino relic density. Phys. Rev. D 2006, 74, 083514. [Google Scholar] [CrossRef]
- Freese, K.; Montefalcone, G.; Shams Es Haghi, B. Dark Matter production during Warm Inflation via Freeze-In. Phys. Rev. Lett. 2024, 133, 211001. [Google Scholar] [CrossRef]
- Chowdhury, D.; Dudas, E.; Dutra, M.; Mambrini, Y. Moduli Portal Dark Matter. Phys. Rev. D 2019, 99, 095028. [Google Scholar] [CrossRef]
- Elahi, F.; Kolda, C.; Unwin, J. UltraViolet Freeze-in. J. High Energy Phys. 2015, 2015, 048. [Google Scholar] [CrossRef]
- Biswas, A.; Ganguly, S.; Roy, S. Fermionic dark matter via UV and IR freeze-in and its possible X-ray signature. J. Cosmol. Astropart. Phys. 2020, 2020, 043. [Google Scholar] [CrossRef]
- Shakya, B. Sterile Neutrino Dark Matter from Freeze-In. Mod. Phys. Lett. A 2016, 31, 1630005. [Google Scholar] [CrossRef]
- McDonald, J.; Sahu, N. keV Warm Dark Matter via the Supersymmetric Higgs Portal. Phys. Rev. D 2009, 79, 103523. [Google Scholar] [CrossRef]
- Lebedev, O.; Lee, H.M.; Mambrini, Y. Vector Higgs-portal dark matter and the invisible Higgs. Phys. Lett. B 2012, 707, 570–576. [Google Scholar] [CrossRef]
- Bernal, N.; Dutra, M.; Mambrini, Y.; Olive, K.; Peloso, M.; Pierre, M. Spin-2 Portal Dark Matter. Phys. Rev. D 2018, 97, 115020. [Google Scholar] [CrossRef]
- Krauss, M.B.; Morisi, S.; Porod, W.; Winter, W. Higher Dimensional Effective Operators for Direct Dark Matter Detection. J. High Energy Phys. 2014, 2014, 056. [Google Scholar] [CrossRef]
- Barman, B.; Bernal, N.; Xu, Y.; Zapata, O. Ultraviolet freeze-in with a time-dependent inflaton decay. J. Cosmol. Astropart. Phys. 2022, 2022, 019. [Google Scholar] [CrossRef]
- Bernal, N.; Deka, K.; Losada, M. Dark Matter Ultraviolet Freeze-in in General Reheating Scenarios. Phys. Rev. D 2025, 111, 055034. [Google Scholar] [CrossRef]
- Weinberg, S.; Dicke, R.H. Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Barranco, J.; Bernal, A.; Delepine, D. Diffuse neutrino supernova background as a cosmological test. J. Phys. G 2018, 45, 055201. [Google Scholar] [CrossRef]
- Lambiase, G.; Mohanty, S.; Stabile, A. PeV IceCube signals and Dark Matter relic abundance in modified cosmologies. Eur. Phys. J. C 2018, 78, 350. [Google Scholar] [CrossRef]

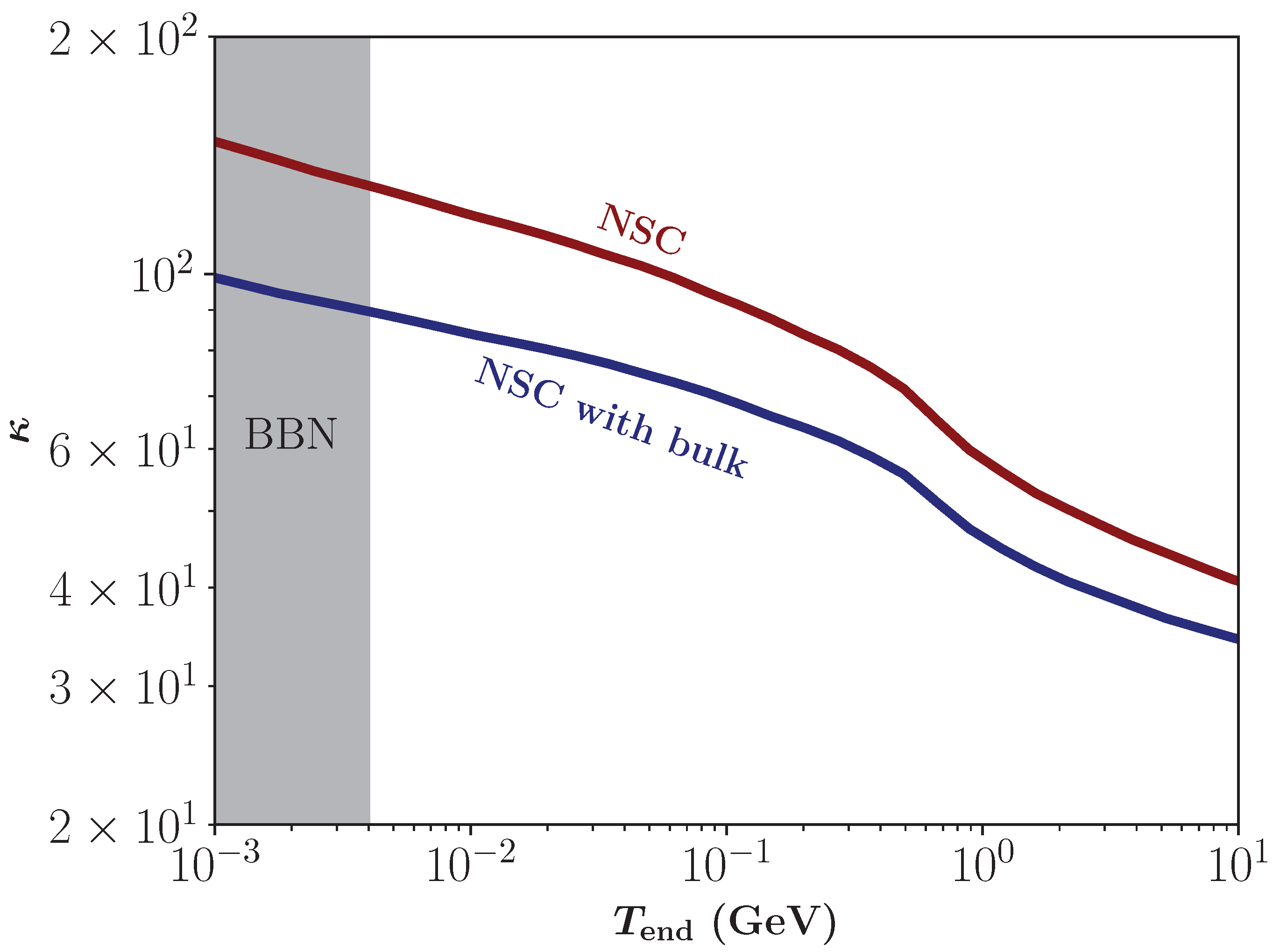
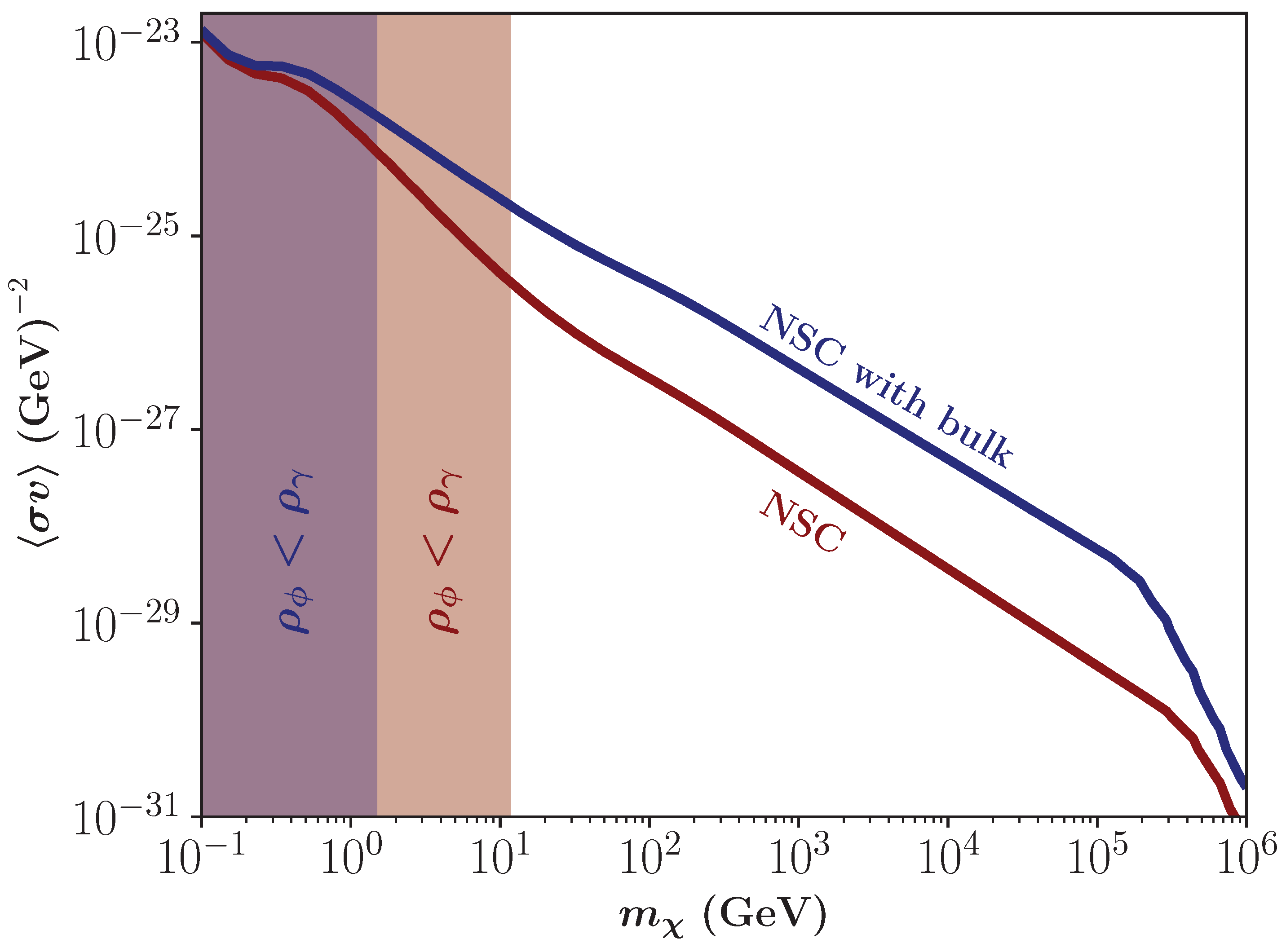
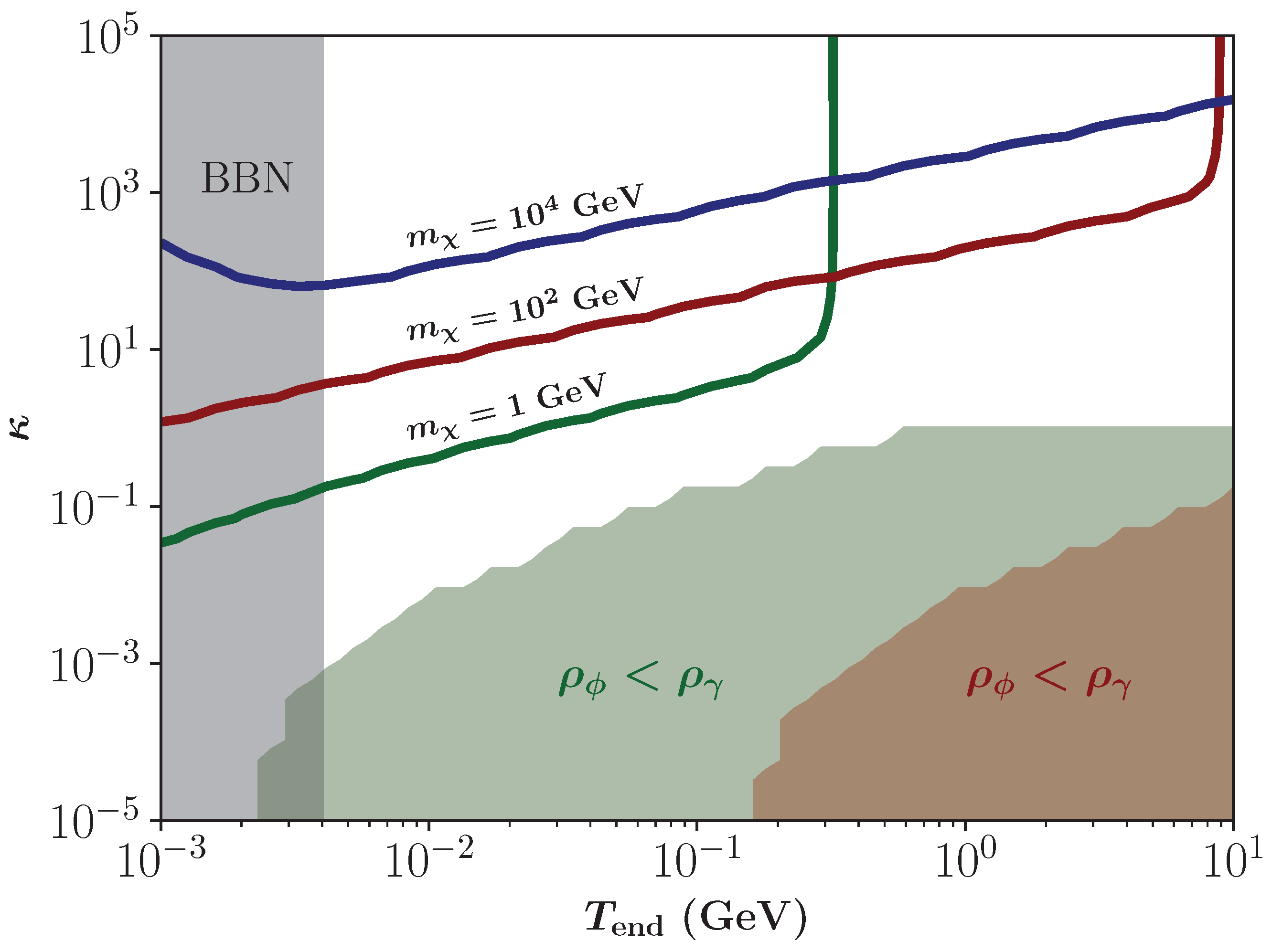
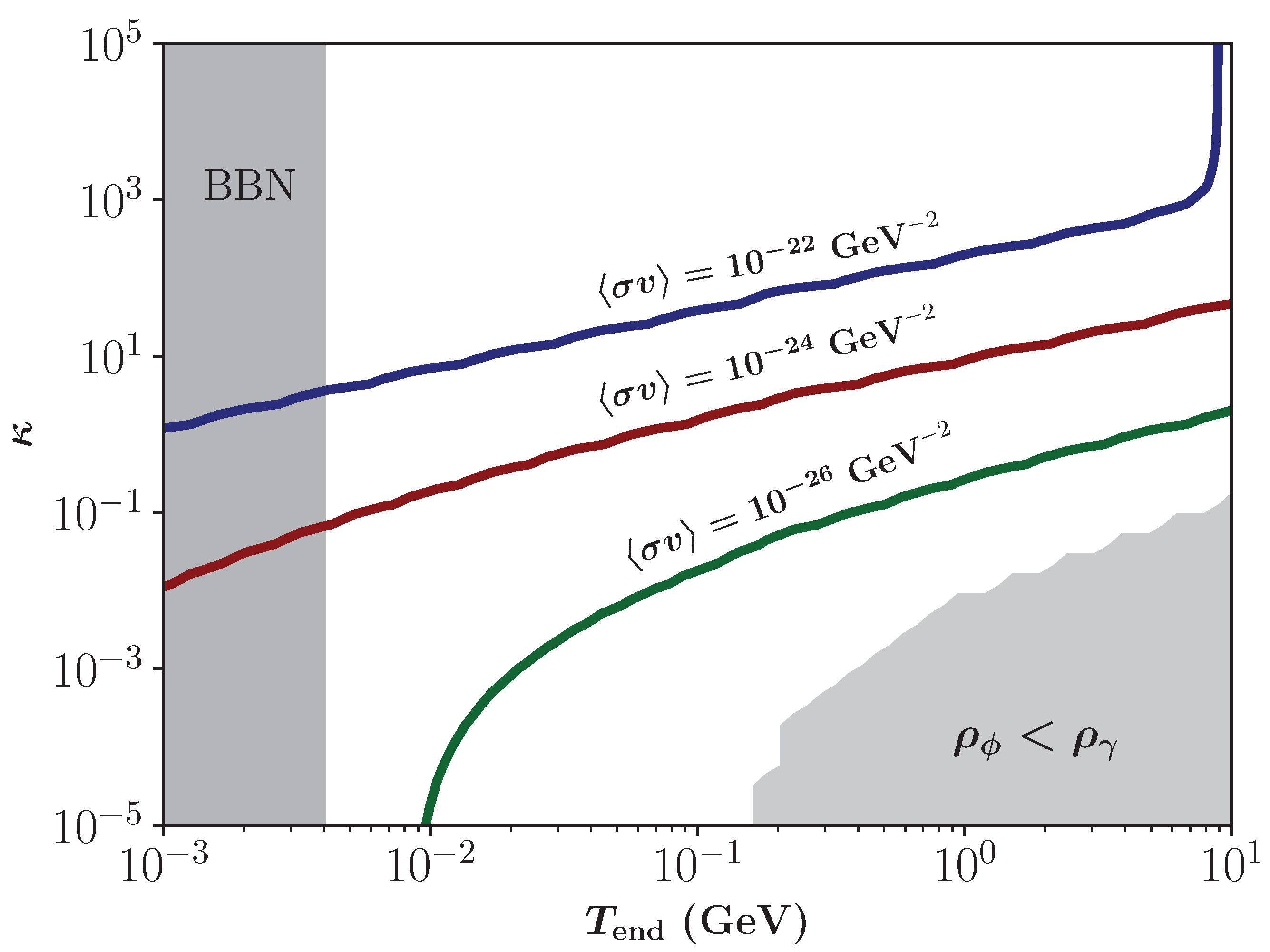
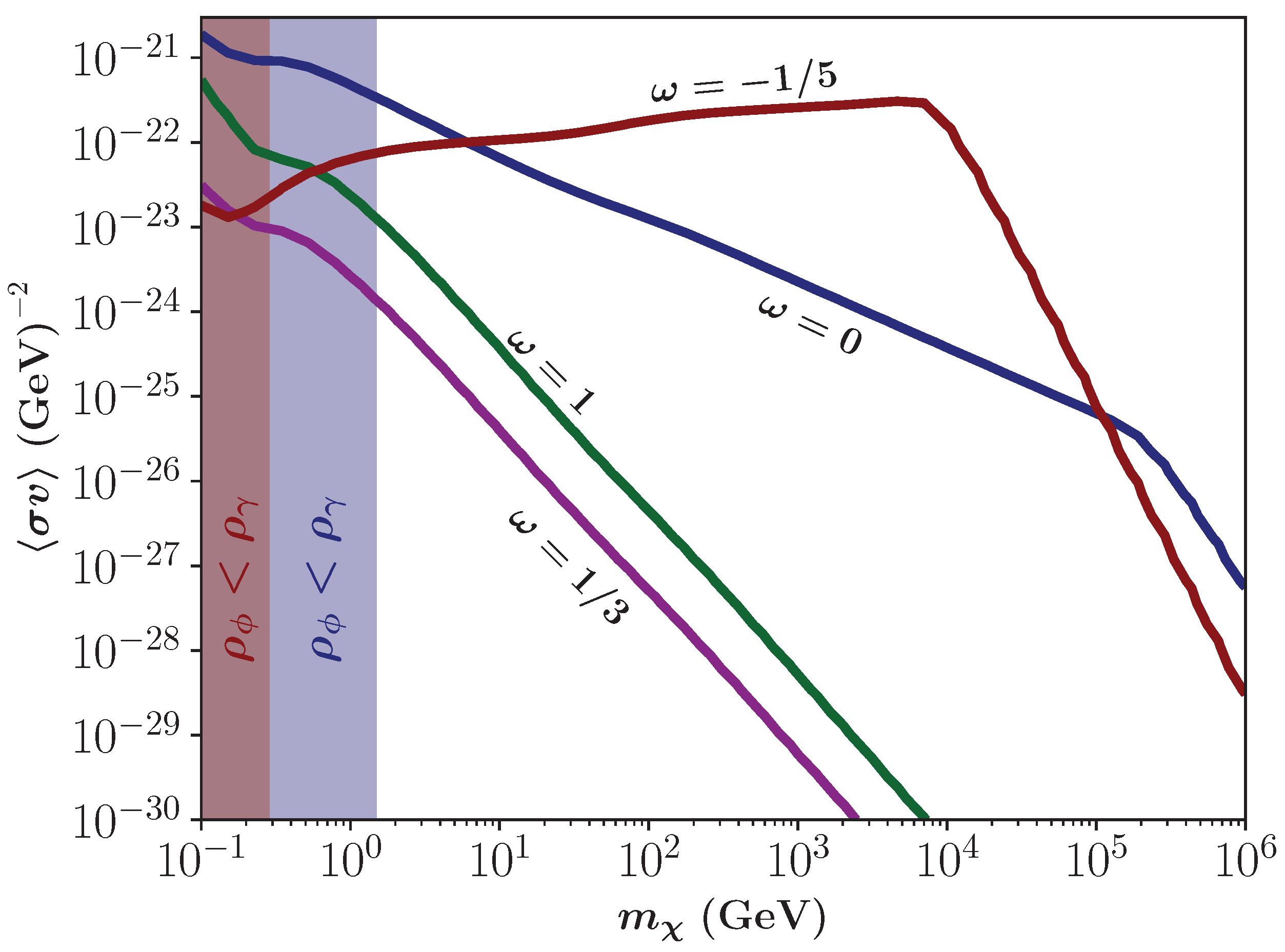

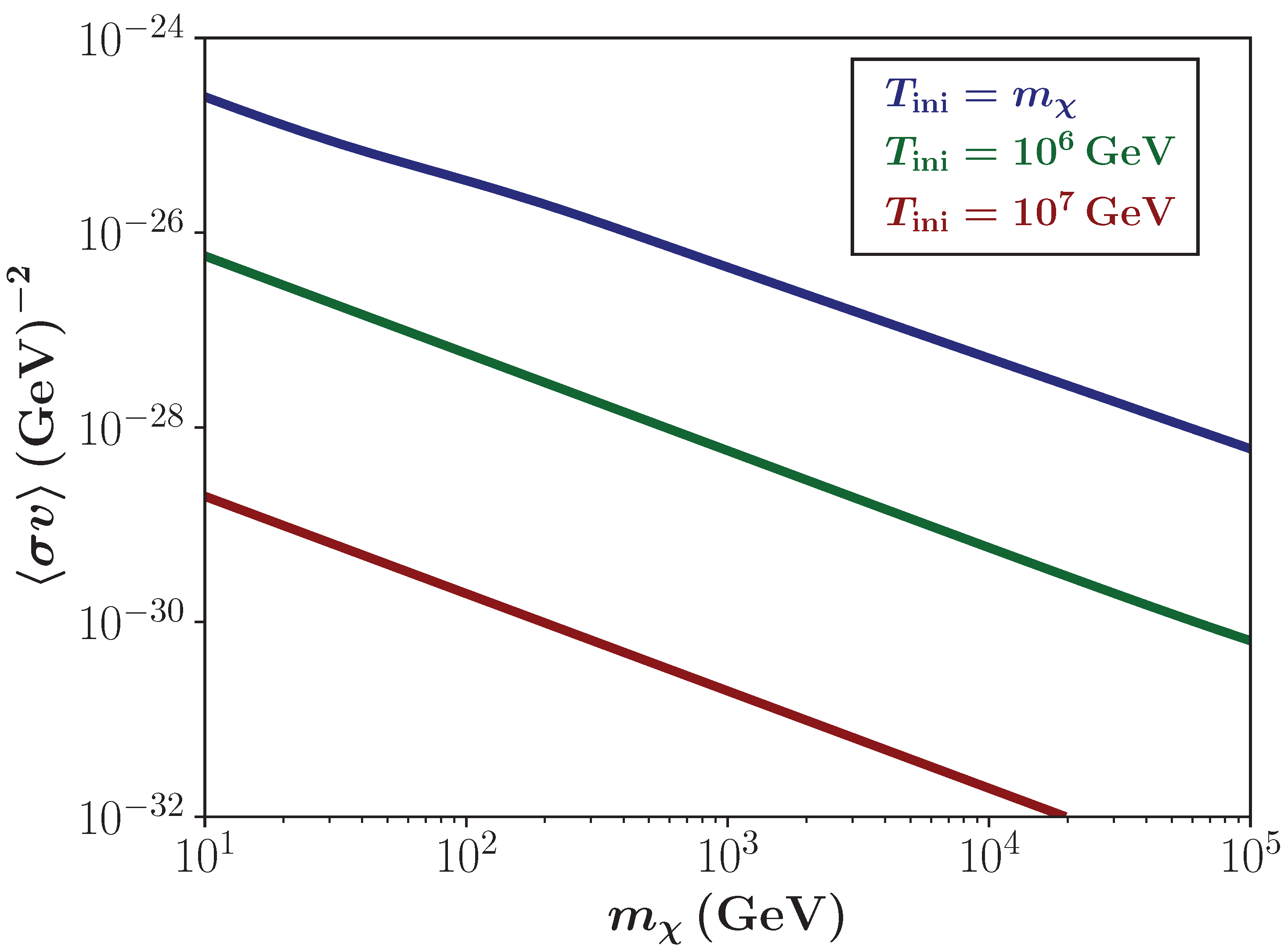


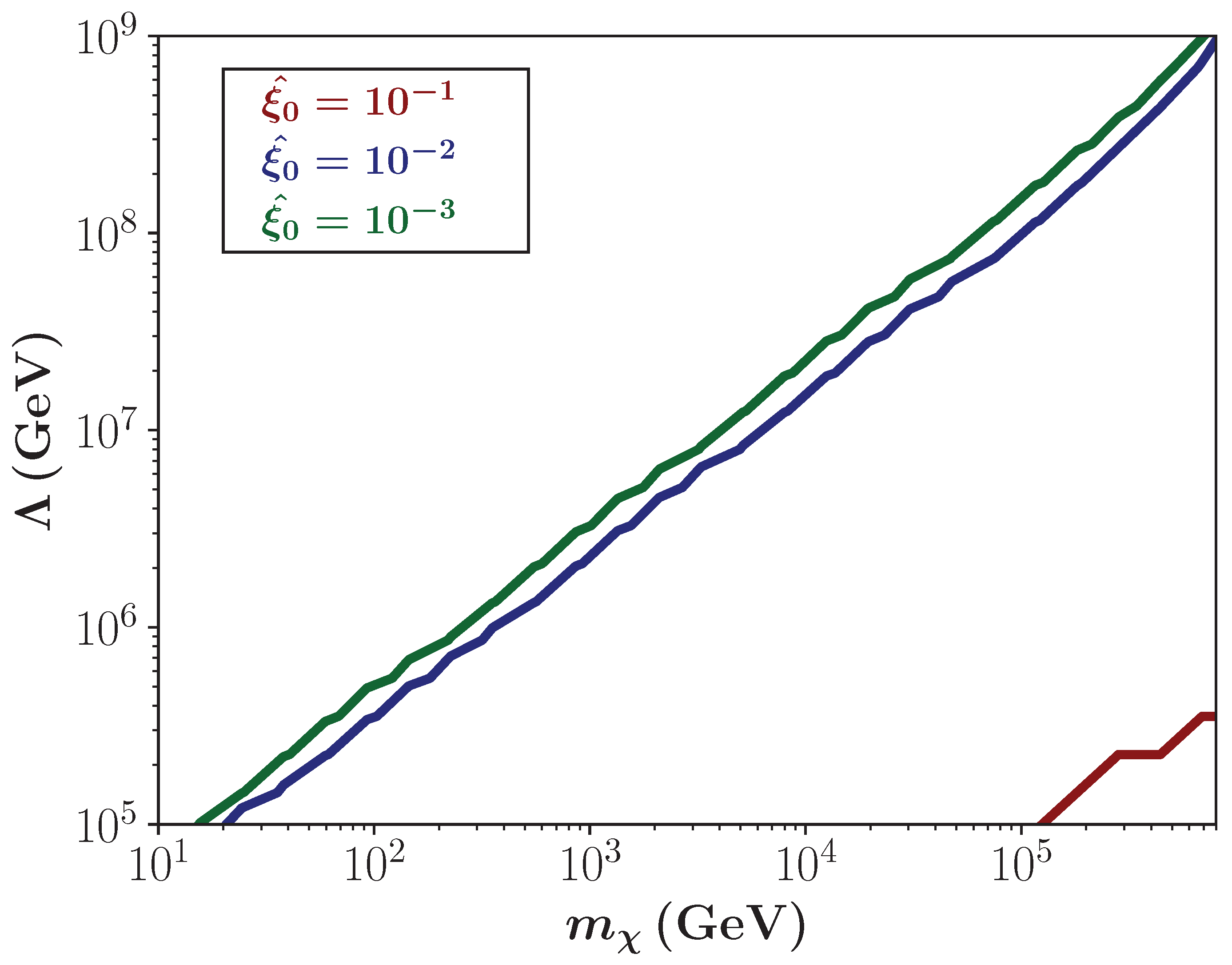
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
González, E.; Maldonado, C.; Mite, N.S.; Salinas, R. FIMP Dark Matter in Bulk Viscous Non-Standard Cosmologies. Symmetry 2025, 17, 731. https://doi.org/10.3390/sym17050731
González E, Maldonado C, Mite NS, Salinas R. FIMP Dark Matter in Bulk Viscous Non-Standard Cosmologies. Symmetry. 2025; 17(5):731. https://doi.org/10.3390/sym17050731
Chicago/Turabian StyleGonzález, Esteban, Carlos Maldonado, N. Stefanía Mite, and Rodrigo Salinas. 2025. "FIMP Dark Matter in Bulk Viscous Non-Standard Cosmologies" Symmetry 17, no. 5: 731. https://doi.org/10.3390/sym17050731
APA StyleGonzález, E., Maldonado, C., Mite, N. S., & Salinas, R. (2025). FIMP Dark Matter in Bulk Viscous Non-Standard Cosmologies. Symmetry, 17(5), 731. https://doi.org/10.3390/sym17050731





