A Study on the Influence of Disturbance Factors’ Coupling Effects on the Dynamic Response of the Symmetrical Structure Press Mechanism
Abstract
1. Introduction
2. Establishment of a Clearance Model
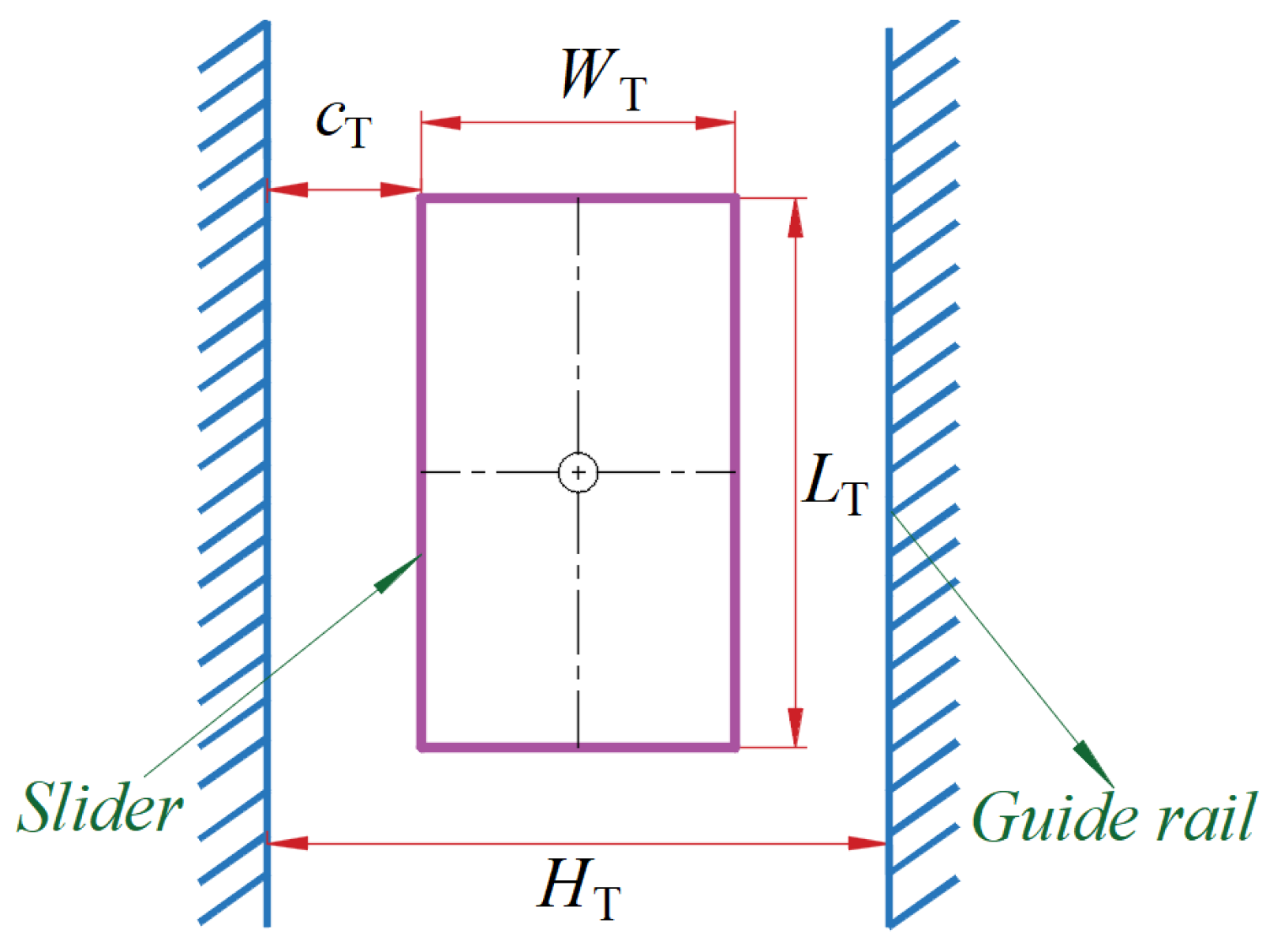
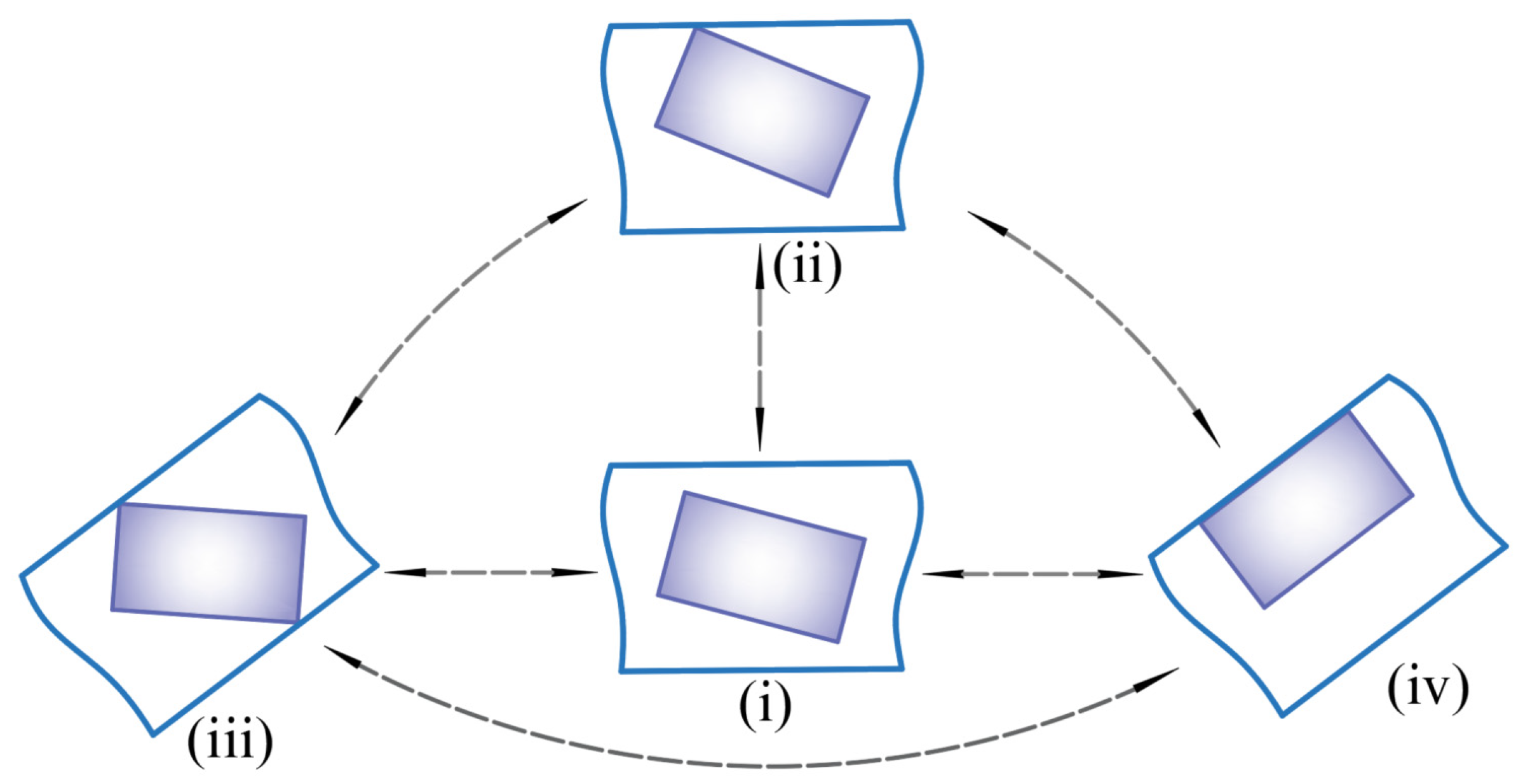
2.1. Clearance of the Translational Pair
2.1.1. Mathematical Model of Clearance of the Translational Pair
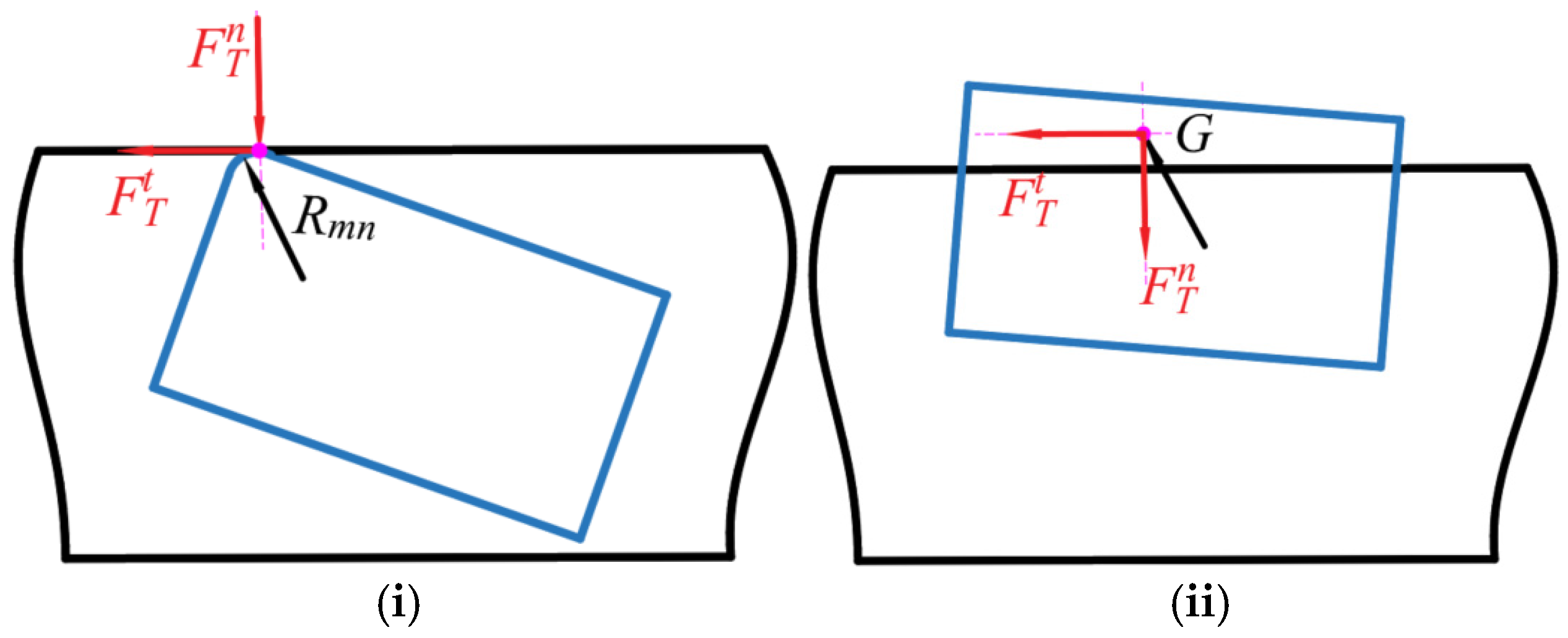
2.1.2. Collision Force Model for the Translational Clearance Pair
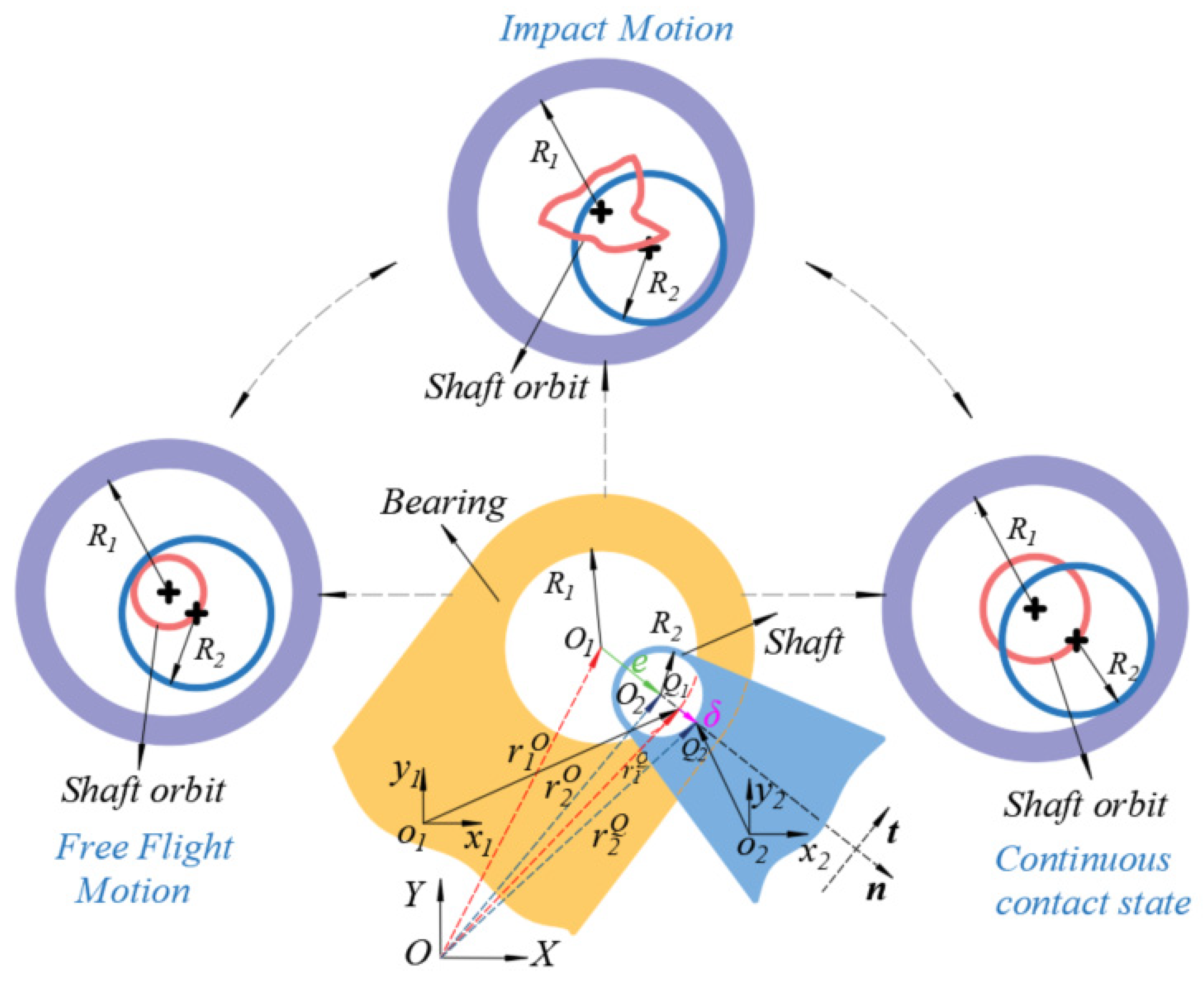
2.2. Clearance of the Revolute Pair
2.2.1. Mathematical Model of Clearance of the Revolute Pair
2.2.2. Collision Force Model of the Revolute Clearance Pair
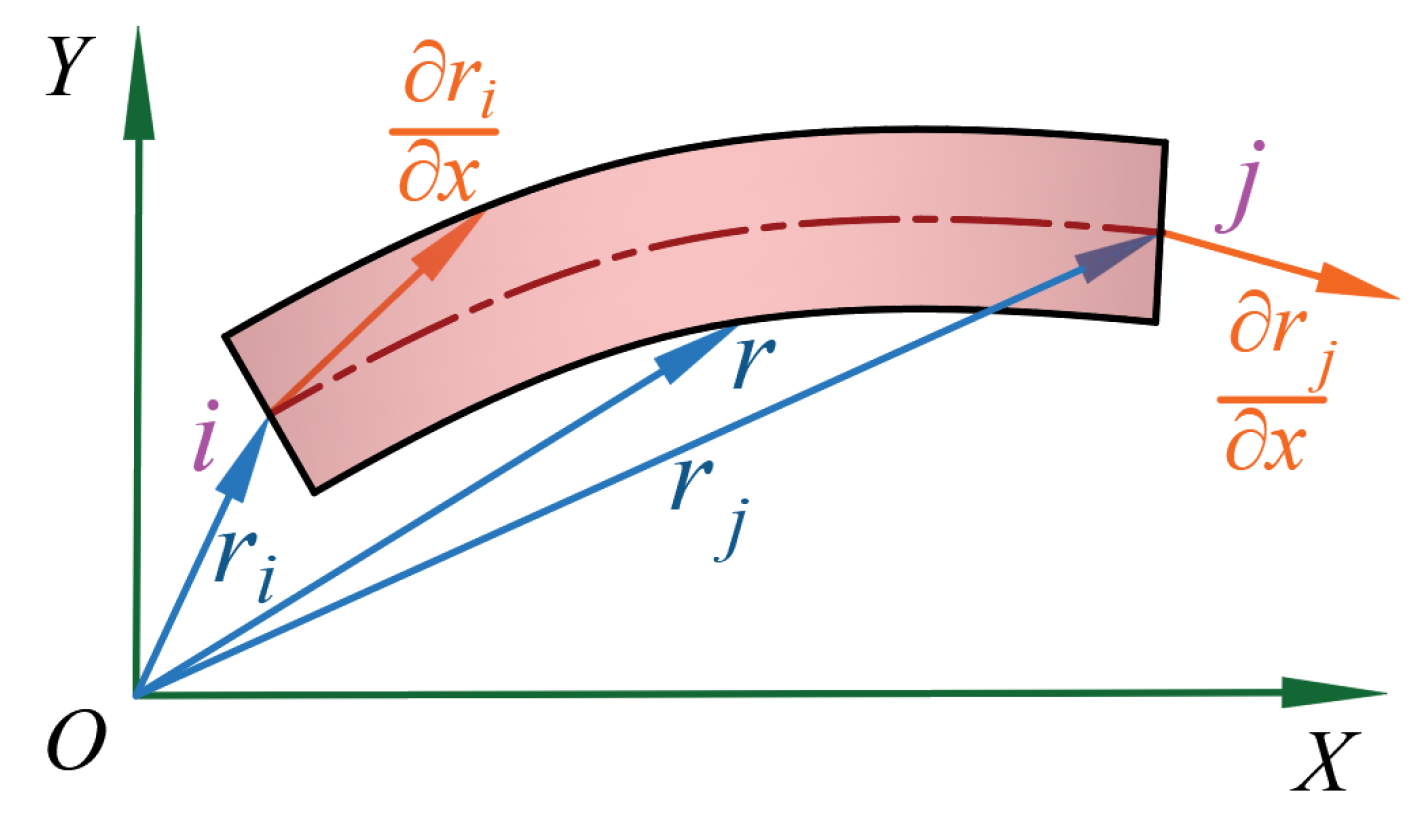
3. Establishment of Beam Unit Model
3.1. Rigid Beam Unit
3.2. Flexible Beam Unit
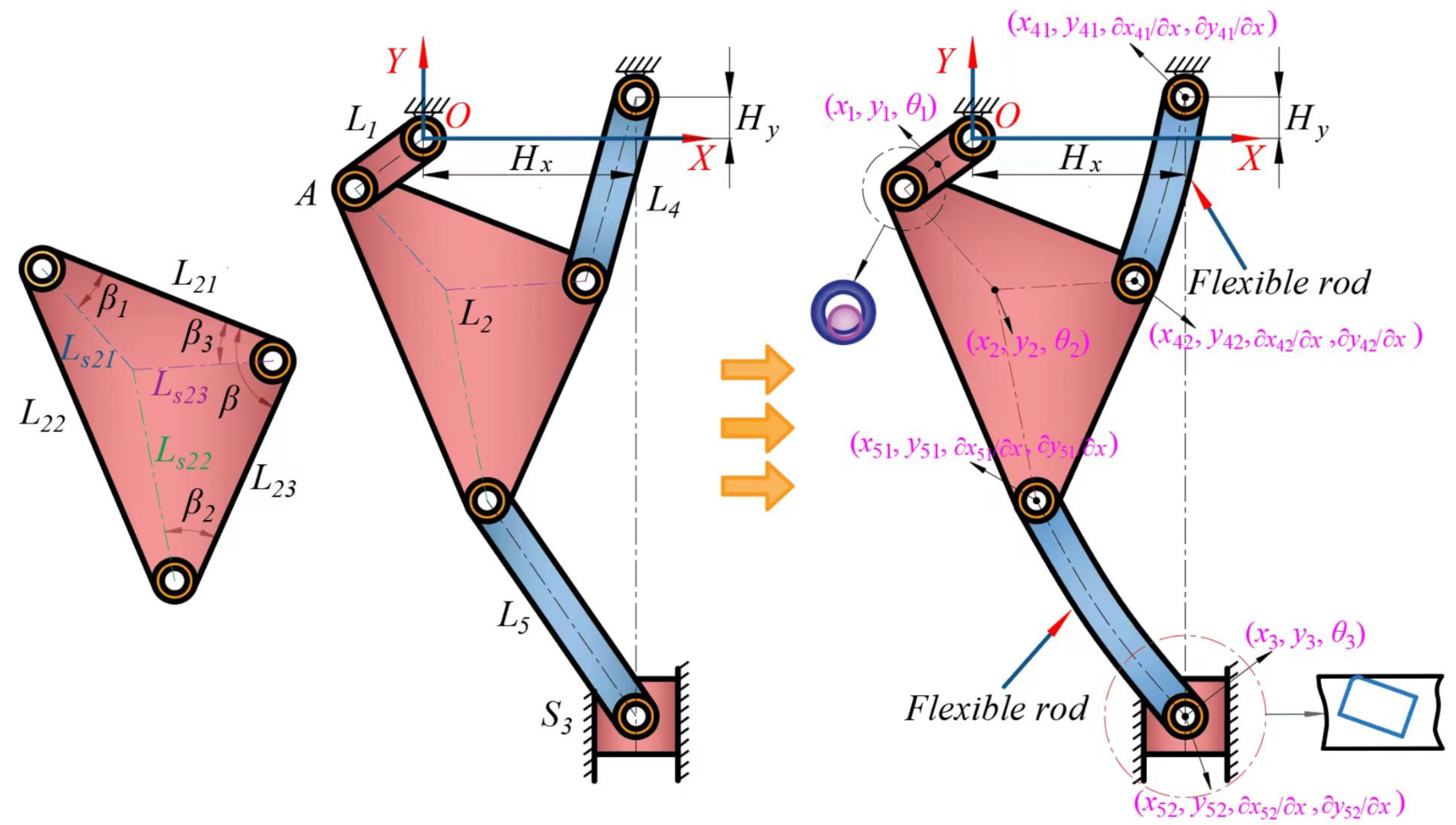
4. Rigid–Flexible Coupling Dynamic Modeling of a Mechanism with Revolute Clearance and Translational Clearance
5. Dynamic Response Analysis and Chaos Identification
5.1. Solution of Dynamic Equations for Mechanisms with Compound Clearances
5.2. Simulation Parameters
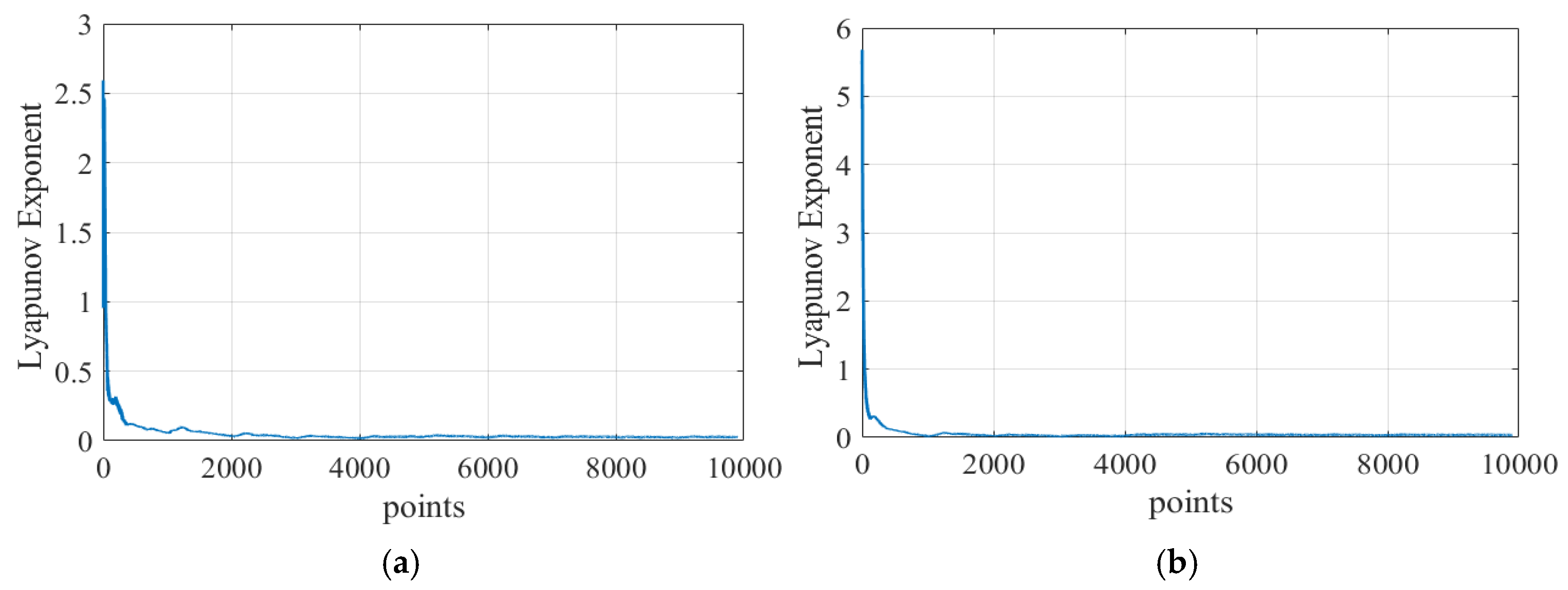
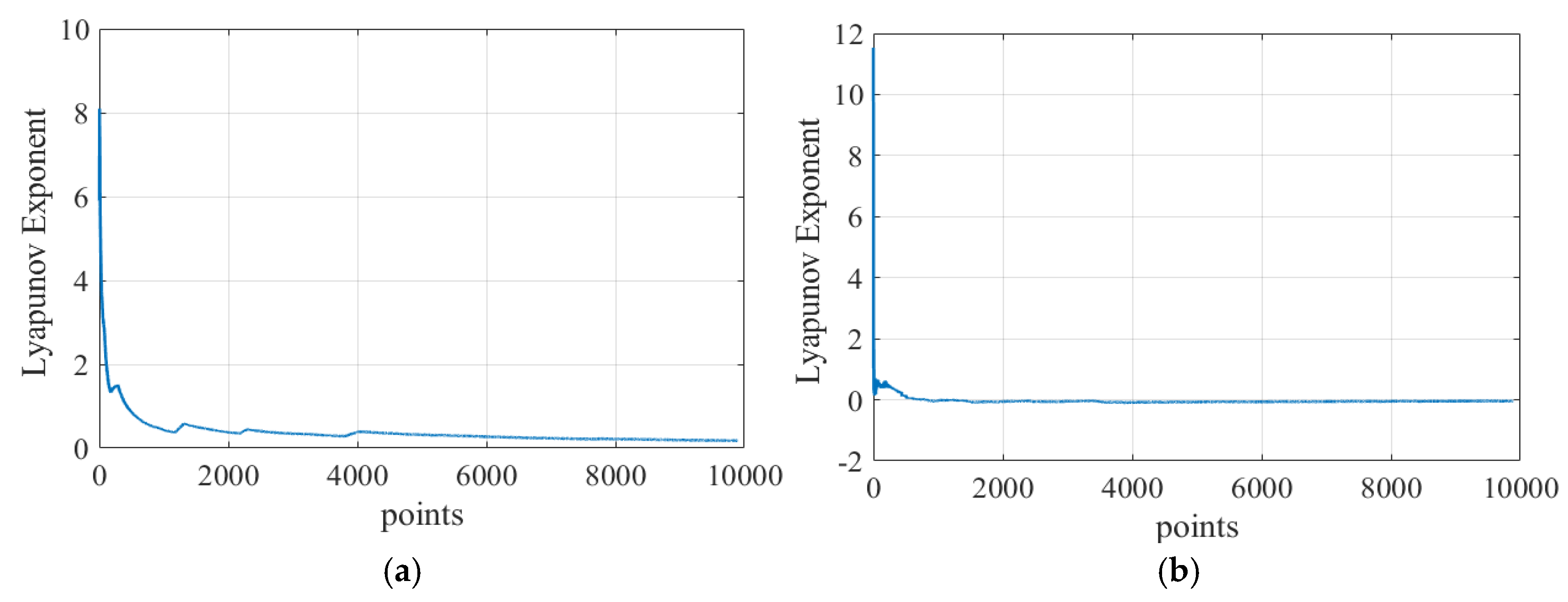
5.3. Chaos Identification
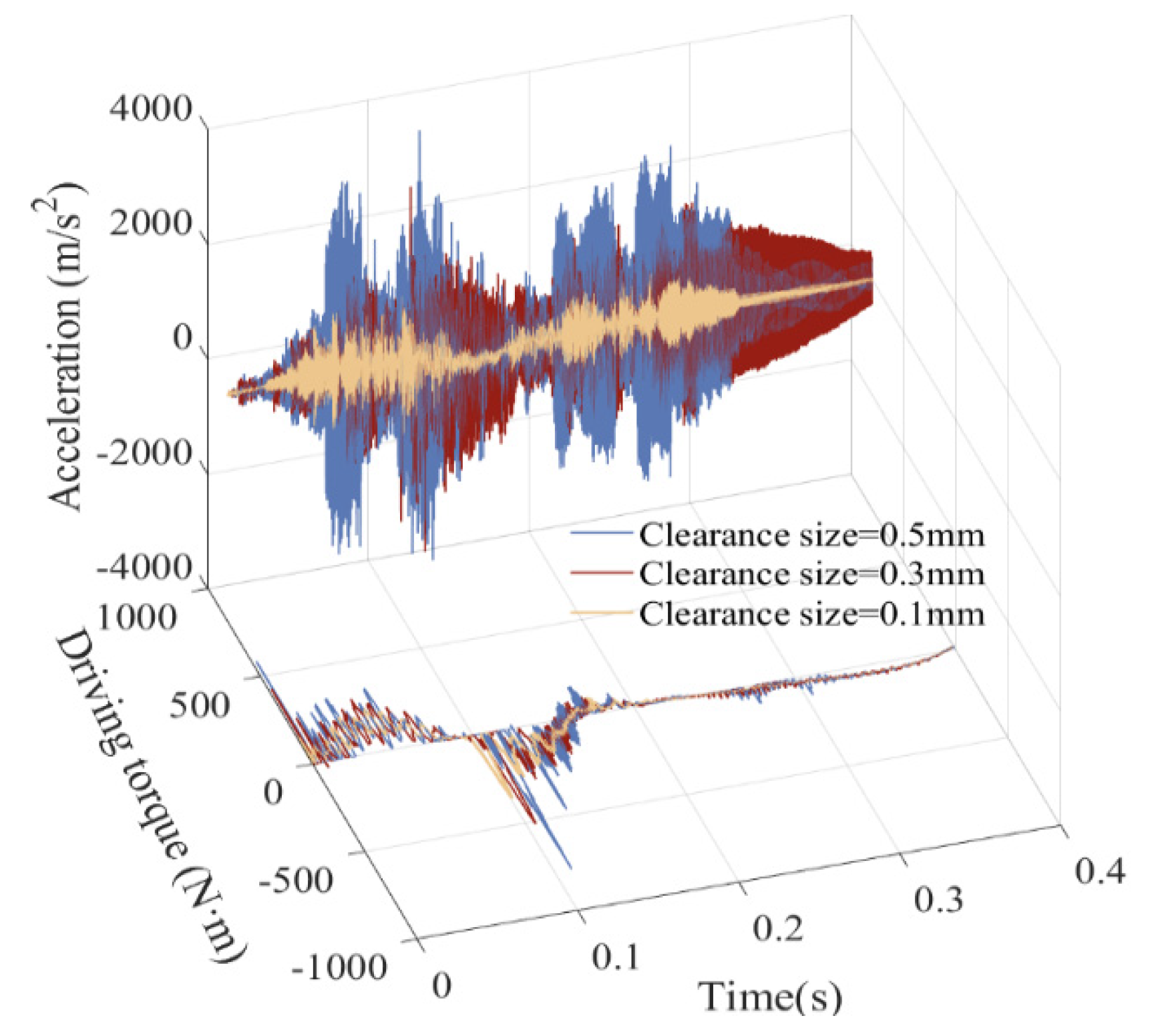
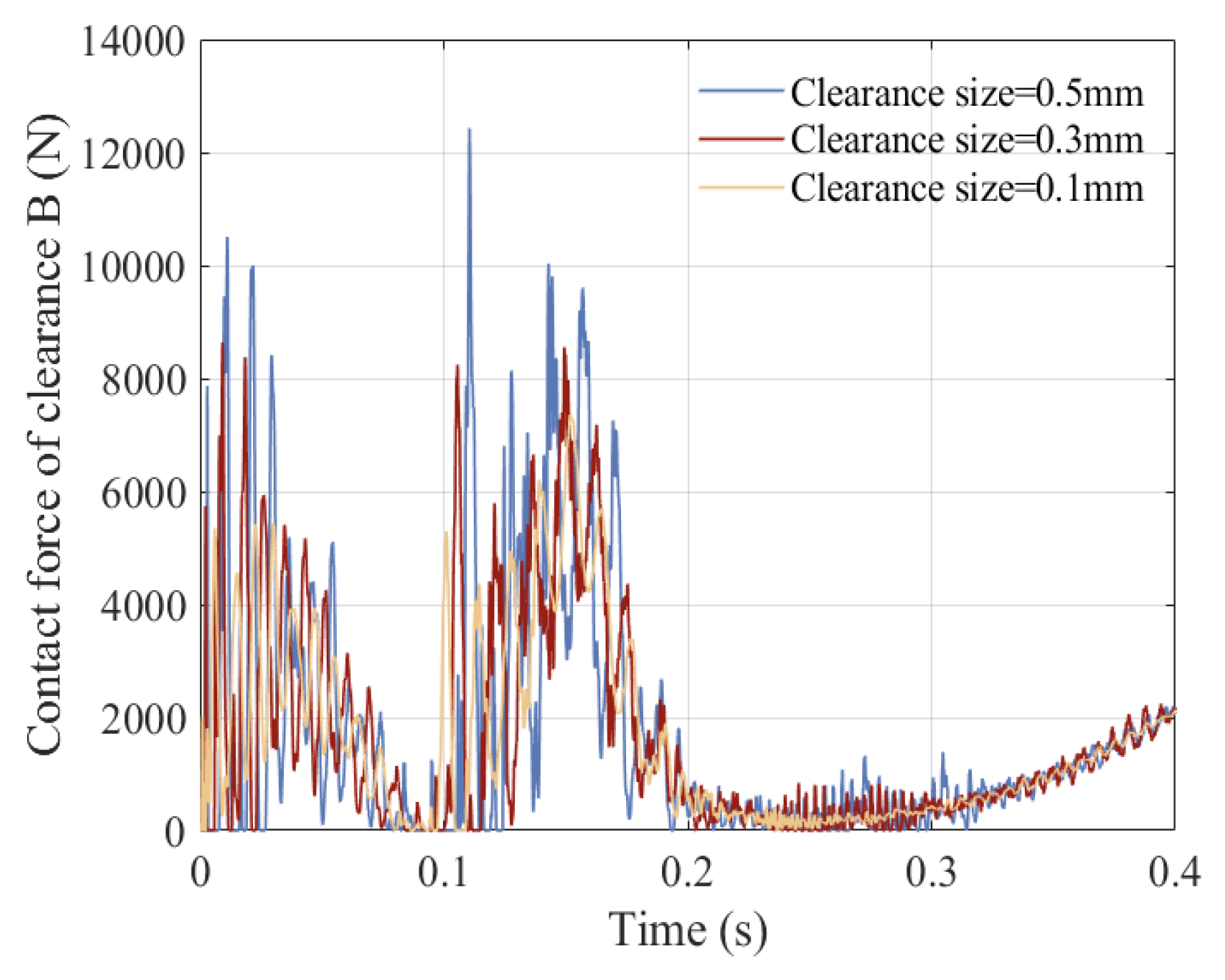
5.4. Influence of Clearance Values on the Dynamic Response
5.5. The Influence of Driving Velocities on Dynamic Response
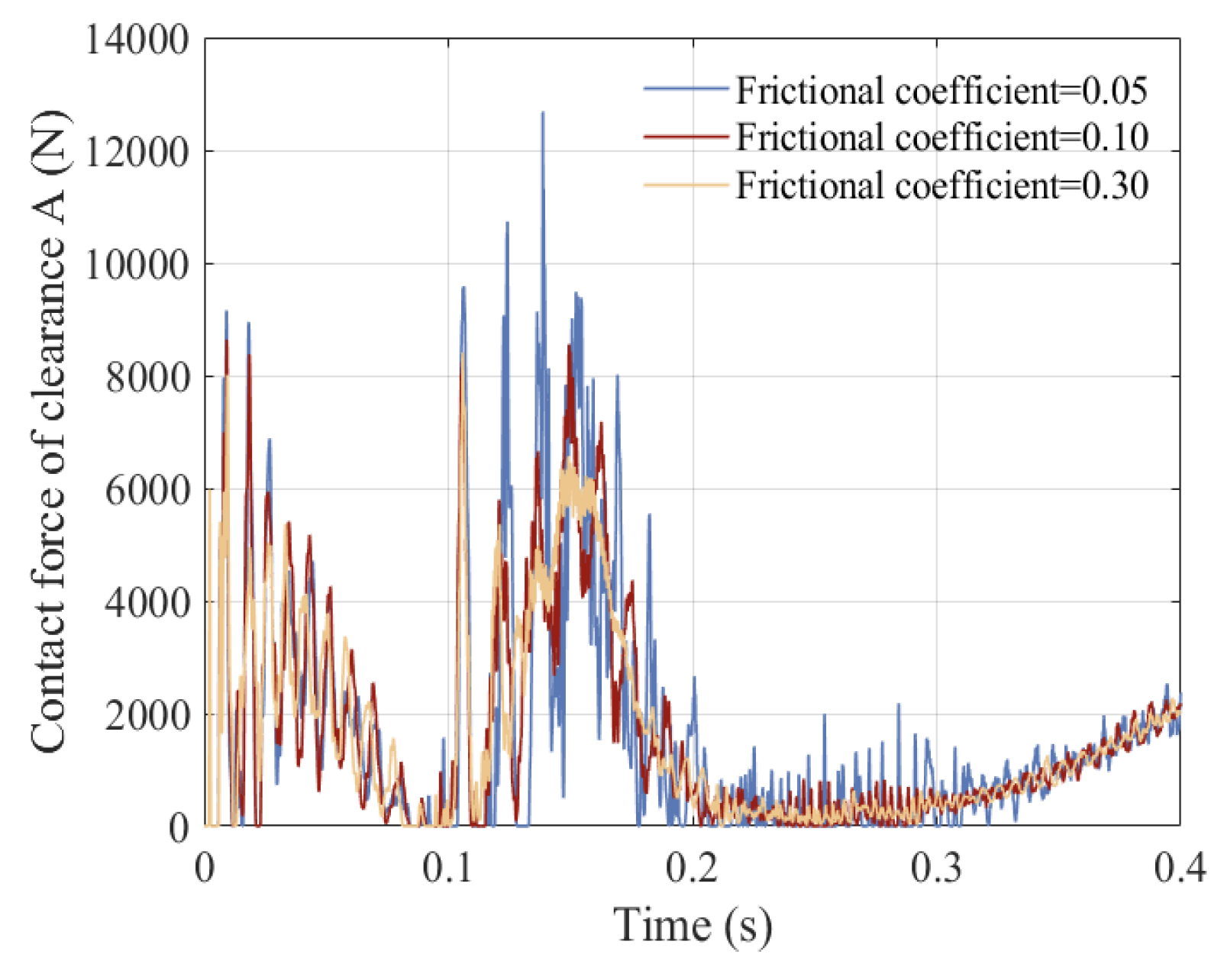
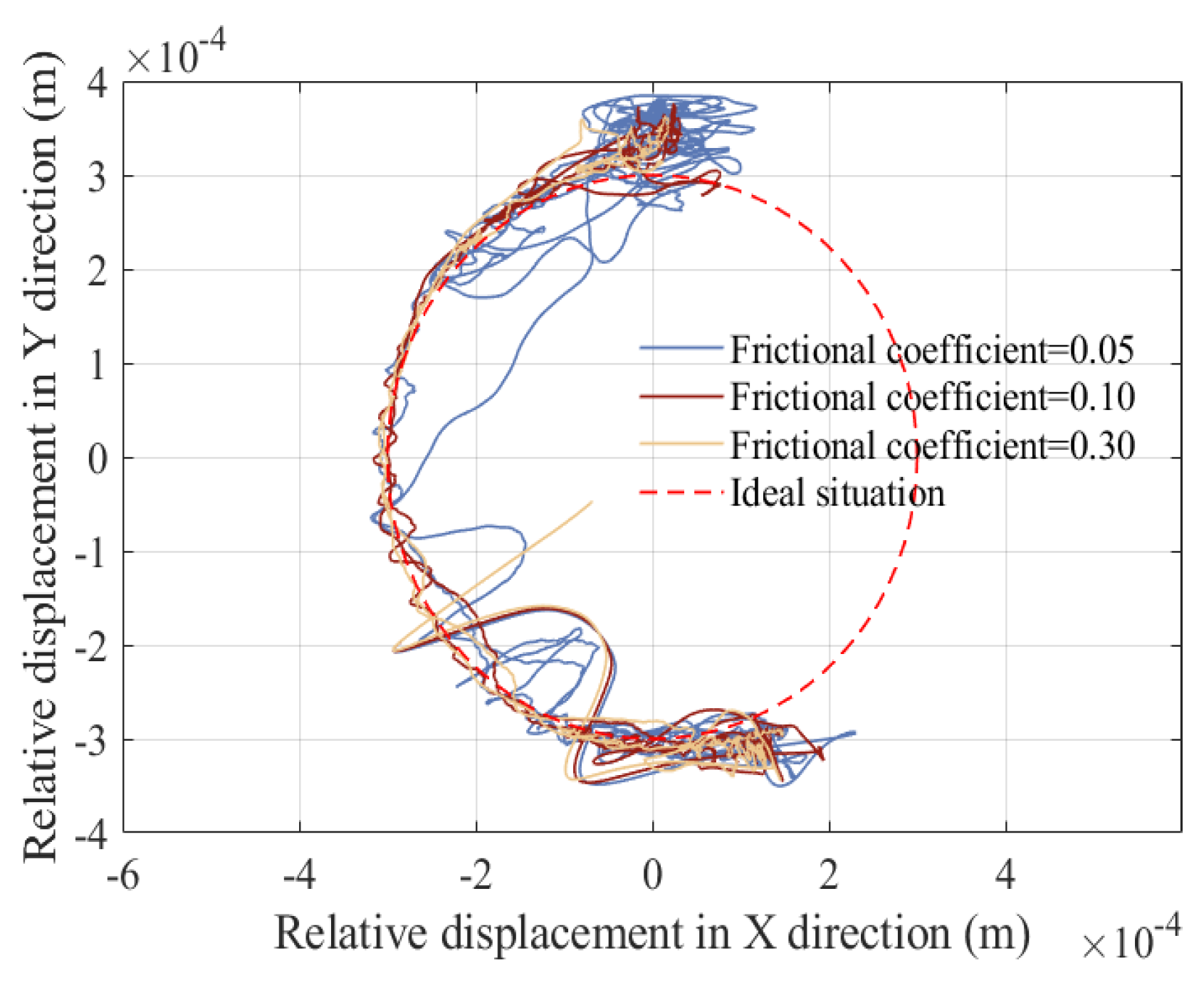
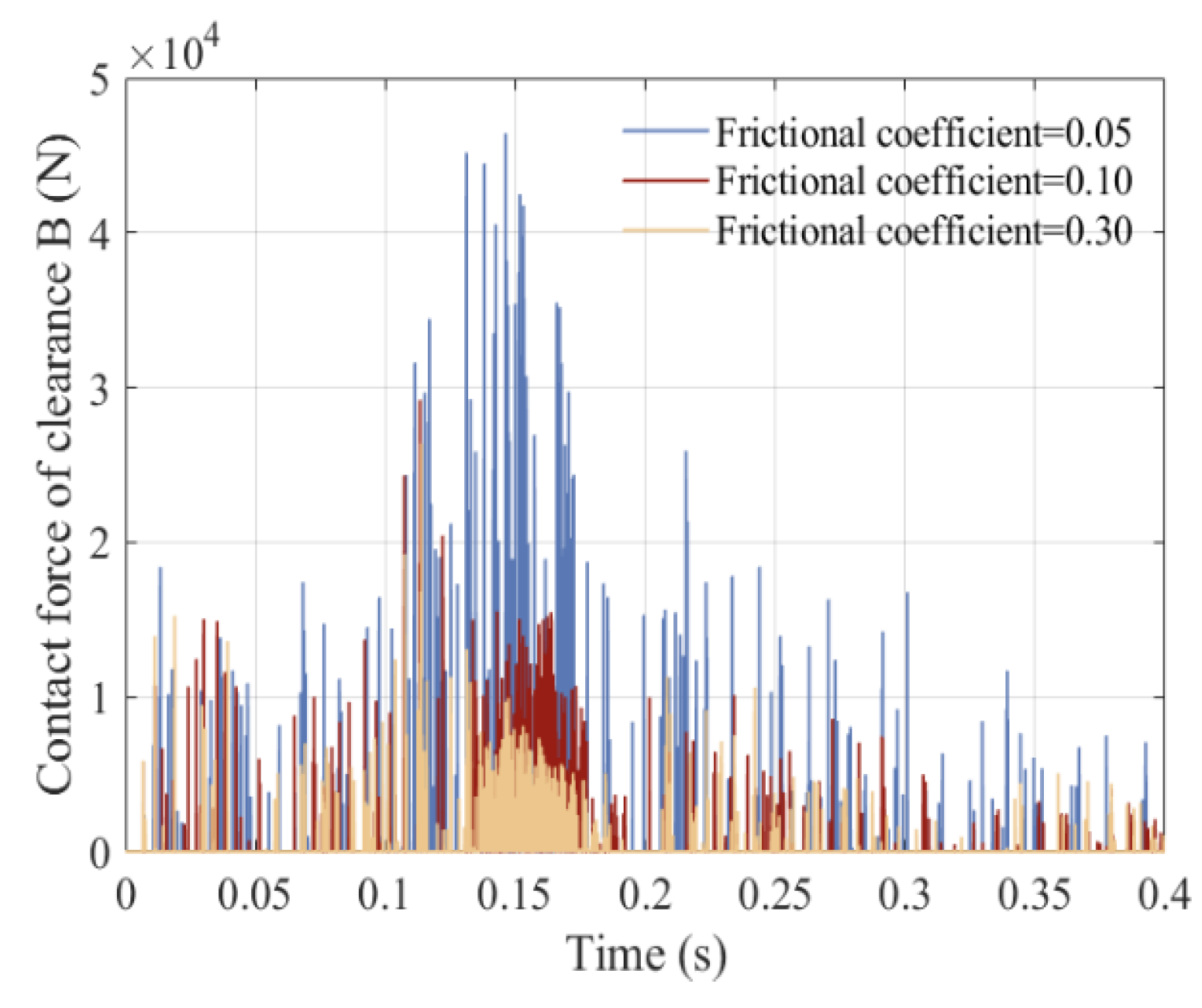
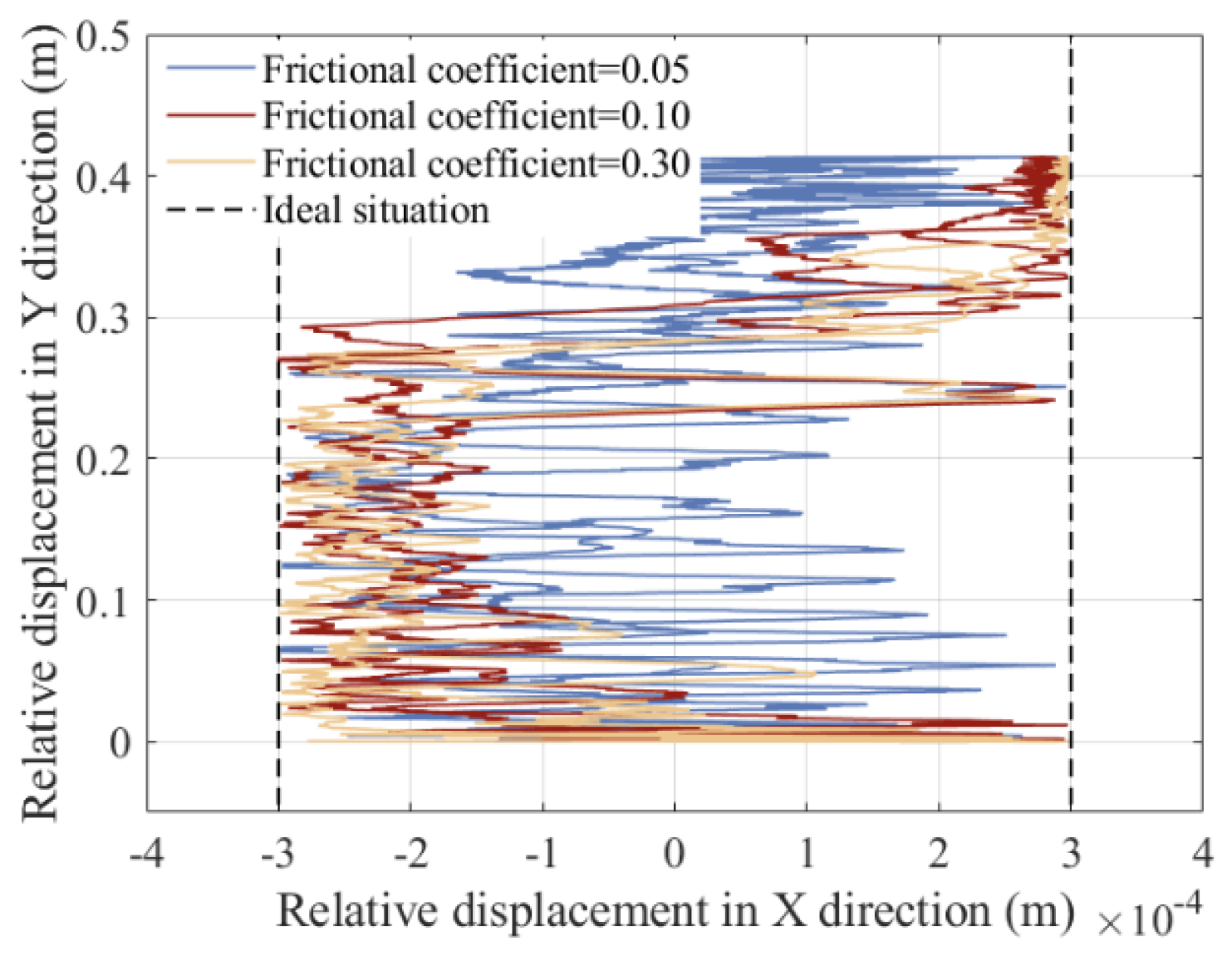
5.6. Influence of Frictional Coefficients on the Dynamic Response
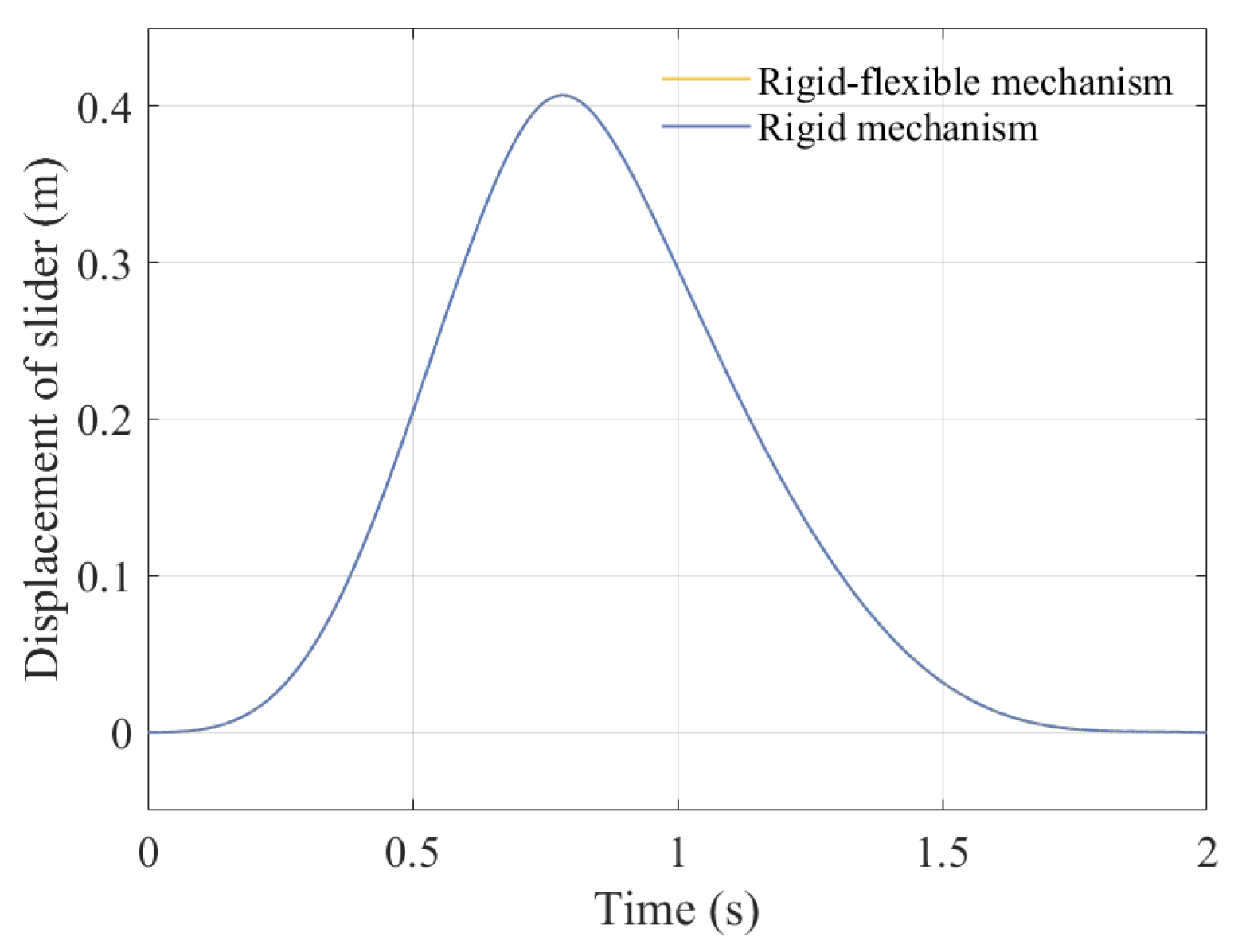
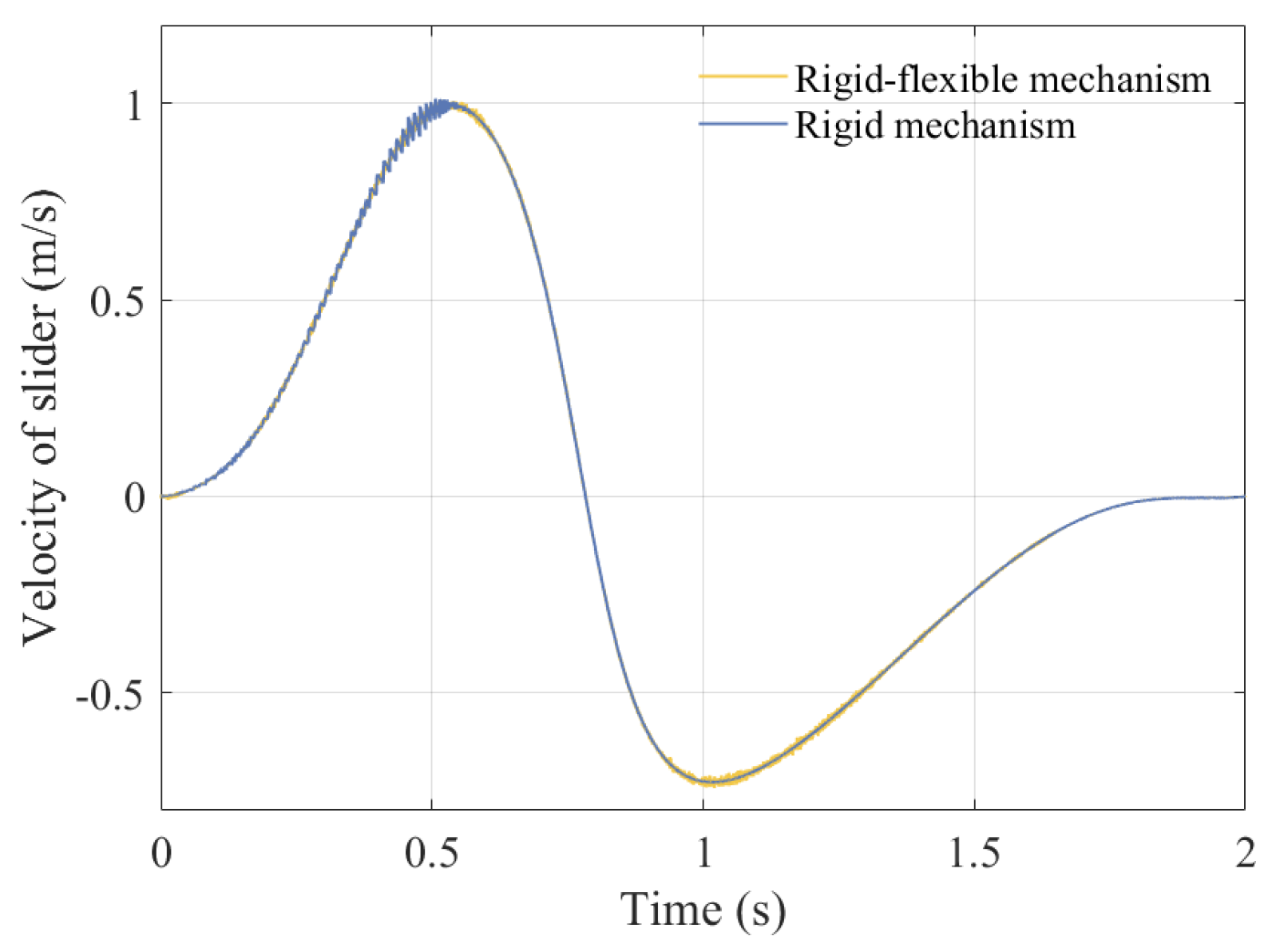
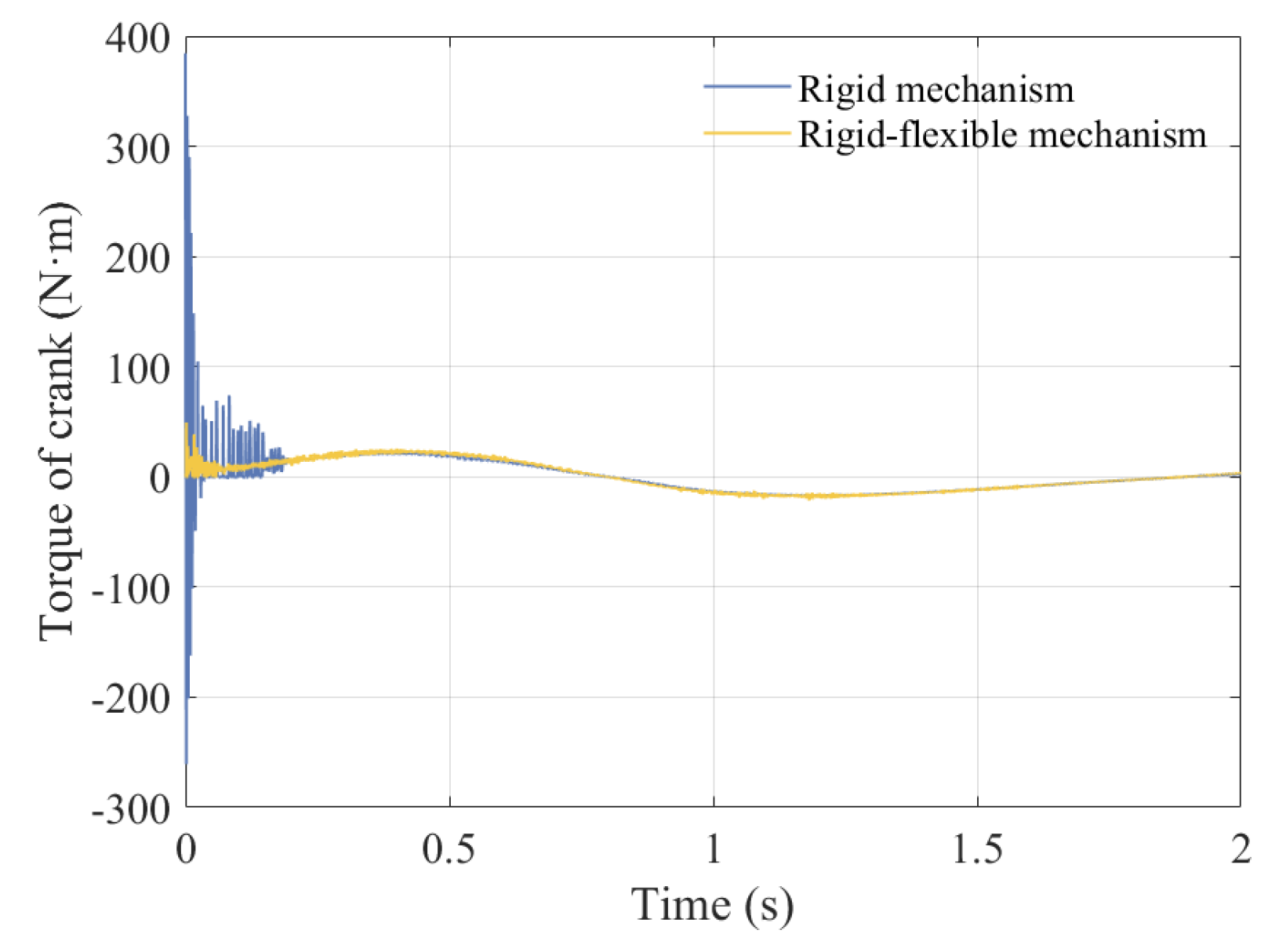
5.7. Comparison of Dynamic Response Between Rigid Mechanisms with Clearance and Rigid–Flexible Coupling Mechanisms with Clearance
6. Conclusions
- (1)
- A dynamic model of a mechanism considering multiple disturbance factors (including translational clearance, revolute clearance, and component flexibility) was established. The coupling effect between the revolute clearance, the translational clearance, and the elasticity of the components can make the motion of the mechanism unstable and produce a certain degree of vibration, and the dynamic response curve will also have certain fluctuations.
- (2)
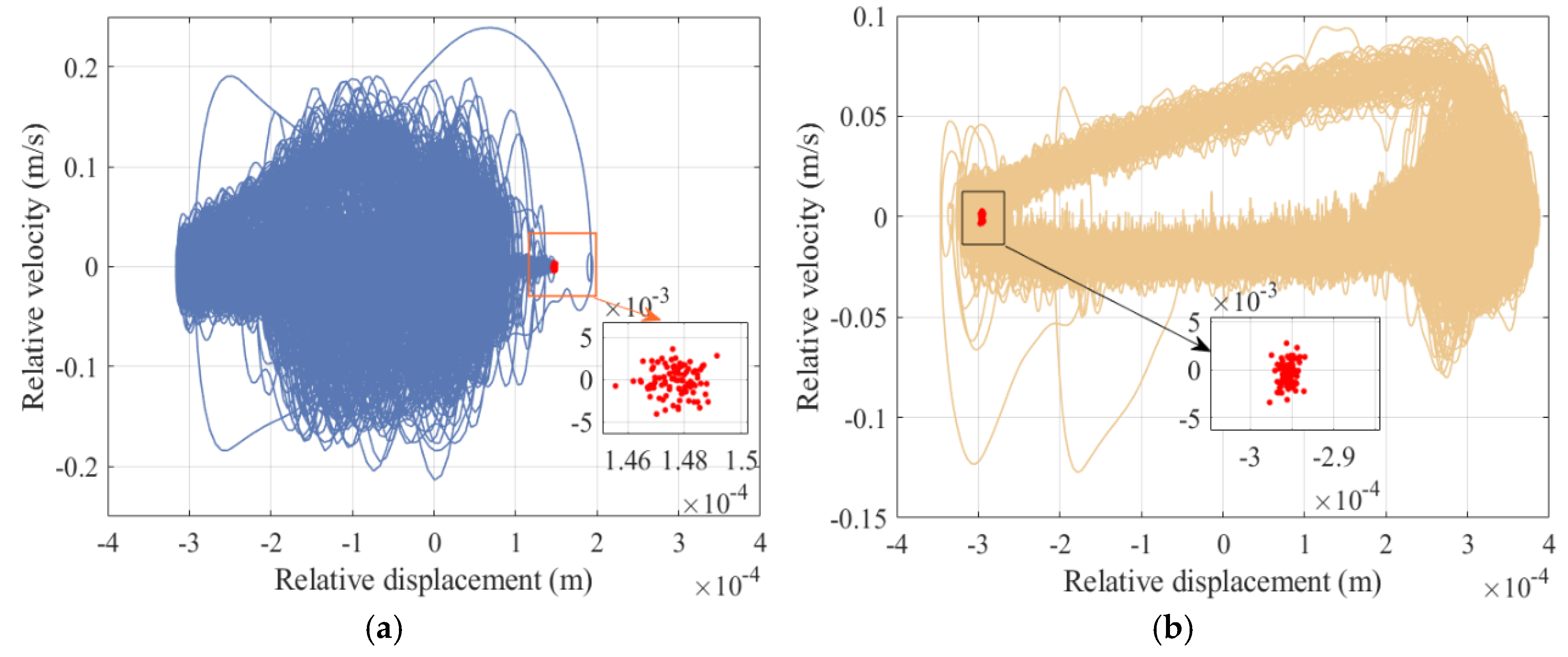
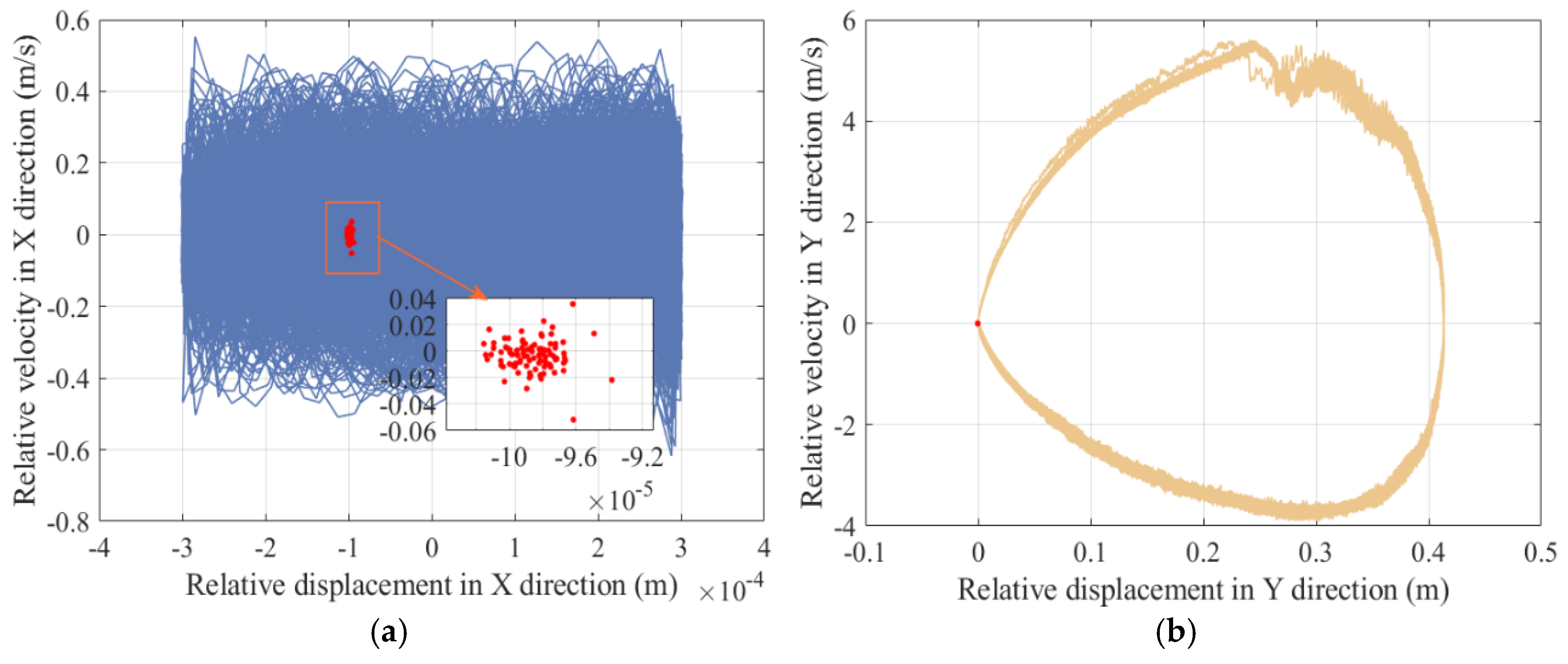
- The motion state of the mechanism was quantitatively and qualitatively determined using phase diagrams, Poincaré mappings, and maximum Lyapunov exponents. It was found that revolute pair A (X direction and Y direction) and translational pair B (X direction) are more prone to chaotic states, whereas translational pair B (Y direction) is more prone to periodic motion.
- (3)
- The effect of various parameters on dynamics were analyzed, including friction coefficient, driving speed, and clearance value. As a result, it was found that with the increase in driving speed and clearance value, as well as the decrease in friction coefficient, the stability of the mechanism weakened, and the vibration of the mechanism’s dynamic response intensified.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Marques, F.; Isaac, F.; Dourado, N.; Flores, P. An enhanced formulation to model spatial revolute joints with radial and axial clearances. Mech. Mach. Theory 2017, 116, 123–144. [Google Scholar] [CrossRef]
- Muvengei, O.; Kihiu, J.; Ikua, B. Dynamic analysis of planar rigid-body mechanical systems with two-clearance revolute joints. Nonlinear Dyn. 2013, 73, 259–273. [Google Scholar] [CrossRef]
- Ambrósio, J.; Pombo, J. A unified formulation for mechanical joints with and without clearances/bushings and/or stops in the framework of multibody systems. Multibody Syst. Dyn. 2018, 42, 317–345. [Google Scholar] [CrossRef]
- Gao, S.; Fan, S.; Zhang, H. Research on the Motion Accuracy Reliability of Rigid-Flexible Coupling Mechanism with Lubrication Clearances. In Proceedings of the International Conference on Mechanical Design, Singapore, 26–28 July 2024. [Google Scholar]
- Jin, G.; Wang, Z.; Liang, D.; Wei, Z.; Chang, B.; Zhou, Y. Modeling and dynamics characteristics analysis of six-bar rocking feeding mechanism with lubricated clearance joint. Arch. Appl. Mech. 2023, 93, 2831–2854. [Google Scholar] [CrossRef]
- Guo, J.; Wang, Y.; Zhang, X.; Cao, S.; Liu, Z. Dynamic characteristic of rudder loop with rough revolute joint clearance. Nonlinear Dyn. 2024, 112, 3179–3194. [Google Scholar] [CrossRef]
- Chen, X.; Tang, Y. Dynamic Modeling, Response, and Chaos Analysis of 2-DOF Hybrid Mechanism with Revolute Clearances. Shock. Vib. 2020, 2020, 9172853. [Google Scholar] [CrossRef]
- Li, S.; Zheng, Y.; Wu, H.; Zhang, J.; Ohsaki, M.; Yang, C.; Luo, Y. Dynamics analysis of deployment process of the Bennett linkage with revolute clearance joints. Nonlinear Dyn. 2024, 112, 10911–10935. [Google Scholar] [CrossRef]
- Wang, X.; Rui, X. Dynamics modeling and simulation of tracked armored vehicle with planar clearance trunnion-bearing revolute joint. J. Mech. Sci. Technol. 2021, 35, 2285–2302. [Google Scholar] [CrossRef]
- Chen, X.; Wang, T.; Gao, S. Dynamic response errors and accuracy reliability for mechanism with multiple lubrication clearance joints. Arch. Appl. Mech. 2023, 93, 525–550. [Google Scholar] [CrossRef]
- Fu, Y.; Sun, X.; Song, Z.; Xu, J. Compound control method for reliability of the robotic arms with clearance joint. Meccanica 2024, 59, 1961–1983. [Google Scholar] [CrossRef]
- Jia, Y.; Chen, X.; Zhang, L.; Ning, C. Dynamic characteristics and reliability analysis of parallel mechanism with clearance joints and parameter uncertainties. Meccanica 2023, 58, 813–842. [Google Scholar] [CrossRef]
- Jiang, S.; Meng, K.; Xin, Y.C.; Zhu, J.N.; Lin, Y.P.; Li, Y. Chaos Identification and Dynamics Analysis of Mechanism Considering the Coupling Effect of Clearance and Elastic Deformation. Mech. Solids 2024, 59, 2179–2211. [Google Scholar] [CrossRef]
- Liang, D.; Lv, T.; Wang, Z. Research on reliability and dynamic characteristics of planar complex multi-bar mechanism considering clearance and irregular wear effect. Commun. Nonlinear Sci. Numer. Simul. 2025, 145, 108708. [Google Scholar] [CrossRef]
- Chen, X.; Jiang, S. Nonlinear dynamic behavior analysis of multi-linkage mechanism with multiple lubrication clearances. Eur. J. Mech. B/Fluids 2022, 91, 177–193. [Google Scholar] [CrossRef]
- Chen, X.; Mu, X. Theoretical and experimental studies on effect of impact load on dynamic characteristics of multi-link press mechanisms with clearances. Nonlinear Dyn. 2024, 112, 81–100. [Google Scholar] [CrossRef]
- Ma, X.-F.; Li, T.-J. Wave Analysis of Planar Deployable Structures with Revolute Clearance Joints Based on Spectral Element Method. Int. J. Appl. Mech. 2018, 10, 1850090. [Google Scholar] [CrossRef]
- Wu, X.; Sun, Y.; Wang, Y.; Chen, Y. Passive chaos suppression for the planar slider-crank mechanism with a clearance joint by attached vibro-impact oscillator. Mech. Mach. Theory 2022, 174, 104882. [Google Scholar] [CrossRef]
- Lin, Y.P.; Wang, J.C.; Zhang, J.L.; An, M.X.; Jiang, S. Research on the Dynamic and Reliability of Planar Multi-Link Mechanisms with Multiple Clearances. Mech. Solids 2024, 59, 1537–1558. [Google Scholar] [CrossRef]
- Gao, S.; Fan, S.; Wang, W. The research on the failure mechanism and dynamic reliability of lubricated clearance. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2024, 238, 9248–9263. [Google Scholar] [CrossRef]
- Wang, J.; Meng, B.; Zhang, L.; Yu, C. Degradation modeling and reliability estimation for mechanical transmission mechanism considering the clearance between kinematic pairs. Reliab. Eng. Syst. Saf. 2024, 247, 110093. [Google Scholar] [CrossRef]
- Chen, X.; Yang, W. Dynamic modeling and analysis of spatial parallel mechanism with revolute joints considering radial and axial clearances. Nonlinear Dyn. 2021, 106, 1929–1953. [Google Scholar] [CrossRef]
- Bai, Z.; Ning, Z.; Zhou, J. Study on Wear Characteristics of Revolute Clearance Joints in Mechanical Systems. Micromachines 2022, 13, 1018. [Google Scholar] [CrossRef]
- Huang, J.; Hu, B.; Sun, S.; Xiao, M.; Peng, C.; Wang, H. Mesh Stiffness and Nonlinear Dynamic Model for a Gear Drive with Revolute Pair Clearance. Processes 2023, 11, 230. [Google Scholar] [CrossRef]
- Huang, S.; Wang, F.; Pan, T.; Wang, Z.; Zhou, H. Research on a monitoring model of revolute pair clearance based on dynamic features and thermal imaging fusion. Infrared Phys. Technol. 2023, 135, 104967. [Google Scholar] [CrossRef]
- Zhuang, F.; Wang, Q. Modeling and simulation of the nonsmooth planar rigid multibody systems with frictional translational joints. Multibody Syst. Dyn. 2013, 29, 403–423. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, J.; Ning, F.; Chai, C.; Zhang, L.; Li, H. Dynamic analysis of crank slider mechanism considering 3D translational joint clearance based on variable contact area. Mech. Ind. 2025, 26, 5. [Google Scholar] [CrossRef]
- Xiao, S.; Liu, S.; Wang, H.; Lin, Y.; Song, M.; Zhang, H. Nonlinear dynamics of coupling rub-impact of double translational joints with subsidence considering the flexibility of piston rod. Nonlinear Dyn. 2020, 100, 1203–1229. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Q. Modeling and simulation of a frictional translational joint with a flexible slider and clearance. Multibody Syst. Dyn. 2016, 38, 367–389. [Google Scholar] [CrossRef]
- Qian, M.; Qin, Z.; Yan, S.; Zhang, L. A comprehensive method for the contact detection of a translational clearance joint and dynamic response after its application in a crank-slider mechanism. Mech. Mach. Theory 2020, 145, 103717. [Google Scholar] [CrossRef]
- Wu, X.; Sun, Y.; Wang, Y.; Chen, Y. Dynamic analysis of the double crank mechanism with a 3D translational clearance joint employing a variable stiffness contact force model. Nonlinear Dyn. 2019, 99, 1937–1958. [Google Scholar] [CrossRef]
- Zheng, X.; Li, J.; Wang, Q.; Liao, Q. A methodology for modeling and simulating frictional translational clearance joint in multibody systems including a flexible slider part. Mech. Mach. Theory 2019, 142, 103603. [Google Scholar] [CrossRef]
- Liu, S.; Cui, Y.; Fu, Y.; Li, B.; Lv, B.; Qian, Y. Modeling of lubricated translational joints in rigid-partially flexible multibody systems and its application in two-stroke marine diesel engines. Tribol. Int. 2022, 165, 107244. [Google Scholar] [CrossRef]
- Flores, P.; Leine, R.; Glocker, C. Modeling and analysis of planar rigid multibody systems with translational clearance joints based on the non-smooth dynamics approach. Multibody Syst. Dyn. 2009, 23, 165–190. [Google Scholar] [CrossRef]
- Zhuang, F.-F.; Wang, Q. Modeling and analysis of rigid multibody systems with driving constraints and frictional translation joints. Acta Mech. Sin. 2014, 30, 437–446. [Google Scholar] [CrossRef]
- Liu, S.; Cui, Y.; Fu, Y.; Li, B.; Lv, B.; Qian, Y. Tribo-dynamic performances and vibration transmission of lubricated translational joints in marine engines. Int. J. Mech. Sci. 2022, 231, 107599. [Google Scholar] [CrossRef]
- Ting, K.-L.; Hsu, K.-L.; Yu, Z.; Wang, J. Clearance-induced output position uncertainty of planar linkages with revolute and prismatic joints. Mech. Mach. Theory 2017, 111, 66–75. [Google Scholar] [CrossRef]
- Bai, Z.; Liu, T.; Li, J.; Zhao, J. Numerical and experimental study on dynamic characteristics of planar mechanism with mixed clearances. Mech. Based Des. Struct. Mach. 2022, 51, 6142–6165. [Google Scholar] [CrossRef]
- Tan, H.; Li, L.; Huang, Q.; Jiang, Z.; Li, Q.; Zhang, Y.; Yu, D. Influence of two kinds of clearance joints on the dynamics of planar mechanical system based on a modified contact force model. Sci. Rep. 2023, 13, 20569. [Google Scholar] [CrossRef]
- Dong, X.; Sun, Y.; Wu, X.; Wang, R. Dynamic modeling and performance analysis of toggle-linkage presses considering mixed clearances and flexibility. Int. J. Non-linear Mech. 2022, 147, 104243. [Google Scholar] [CrossRef]
- Jiang, S.; Chen, X. Test study and nonlinear dynamic analysis of planar multi-link mechanism with compound clearances. Eur. J. Mech. A/Solids 2021, 88, 104260. [Google Scholar] [CrossRef]
- Jiang, S.; Zhao, M.; Liu, J.; Lin, Y.; Xiao, L.; Jia, Y. Dynamic response and nonlinear characteristics of multi-link mechanism with clearance joints. Arch. Appl. Mech. 2023, 93, 3461–3493. [Google Scholar] [CrossRef]
- Chen, X.; Jiang, S.; Deng, Y. Dynamic Responses of Planar Multilink Mechanism considering Mixed Clearances. Shock. Vib. 2020, 2020, 8725845. [Google Scholar] [CrossRef]
- Xiao, L.; Yan, F.; Chen, T.; Zhang, S.; Jiang, S. Study on nonlinear dynamics of rigid-flexible coupling multi-link mechanism considering various kinds of clearances. Nonlinear Dyn. 2023, 111, 3279–3306. [Google Scholar] [CrossRef]
- Wu, X.; Sun, Y.; Wang, Y.; Chen, Y. Correlation dimension and bifurcation analysis for the planar slider-crank mechanism with multiple clearance joints. Multibody Syst. Dyn. 2021, 52, 95–116. [Google Scholar] [CrossRef]
- Tan, H.; Hu, Y.; Li, L. Effect of friction on the dynamic analysis of slider-crank mechanism with clearance joint. Int. J. Non-linear Mech. 2019, 115, 20–40. [Google Scholar] [CrossRef]
- Jia, Y.; Chen, X. Dynamic response analysis for multi-degrees-of-freedom parallel mechanisms with various types of three-dimensional clearance joints. Int. J. Adv. Robot. Syst. 2021, 18, 1–9. [Google Scholar]
- Ege, M.; Kucuk, S. Energy Minimization of New Robotic-Type Above-Knee Prosthesis for Higher Battery Lifetime. Appl. Sci. 2023, 13, 3868. [Google Scholar] [CrossRef]
- Kucuk, S. Simulation and design tool for performance analysis of planar parallel manipulators. Simulation 2012, 88, 542–556. [Google Scholar] [CrossRef]
- Ambrosio, J.A.C. Impact of Rigid and Flexible Multibody Systems: Deformation Description and Contact Models. Virtual Nonlinear Multibody Syst. 2003, 103, 57–81. [Google Scholar]




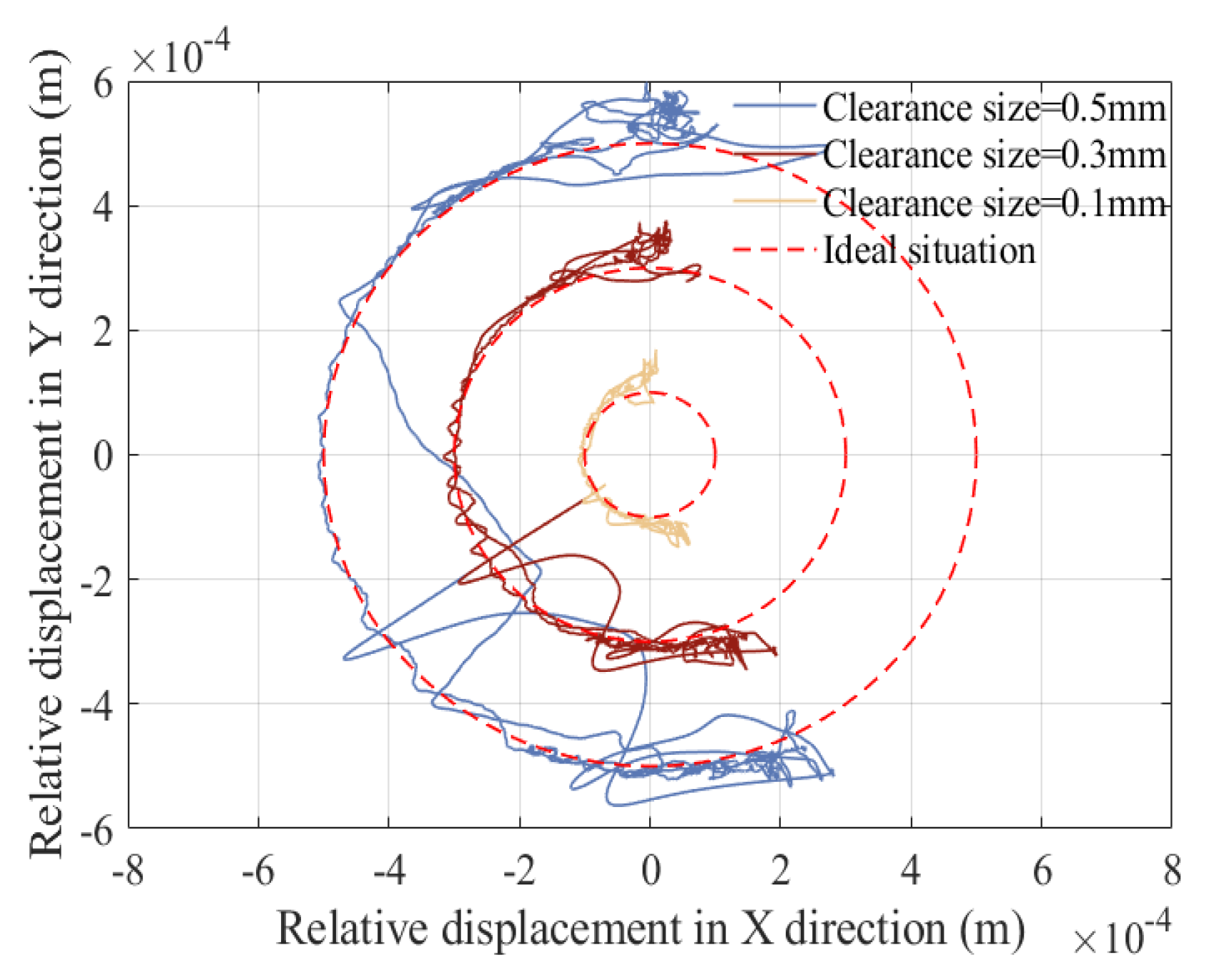
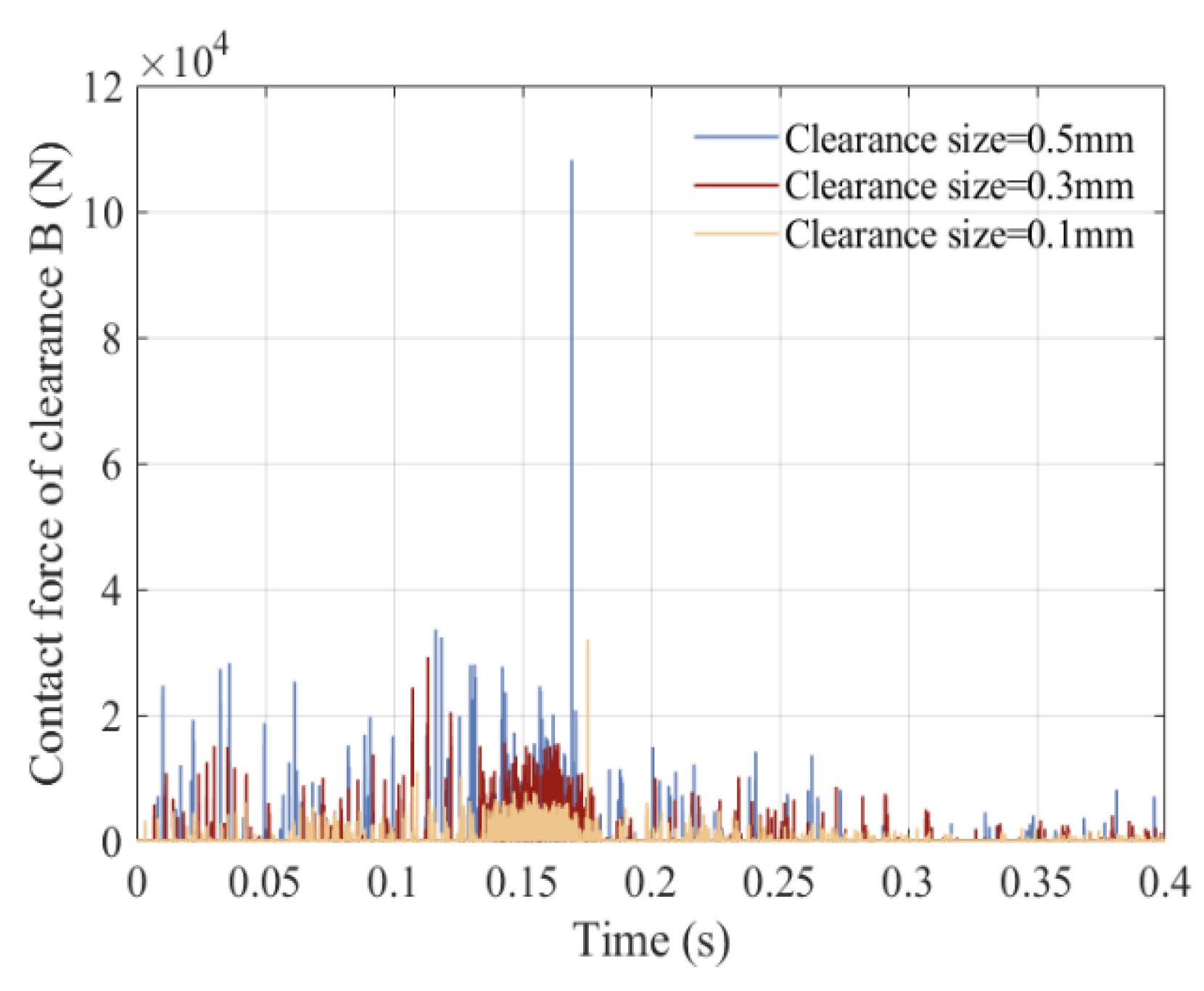

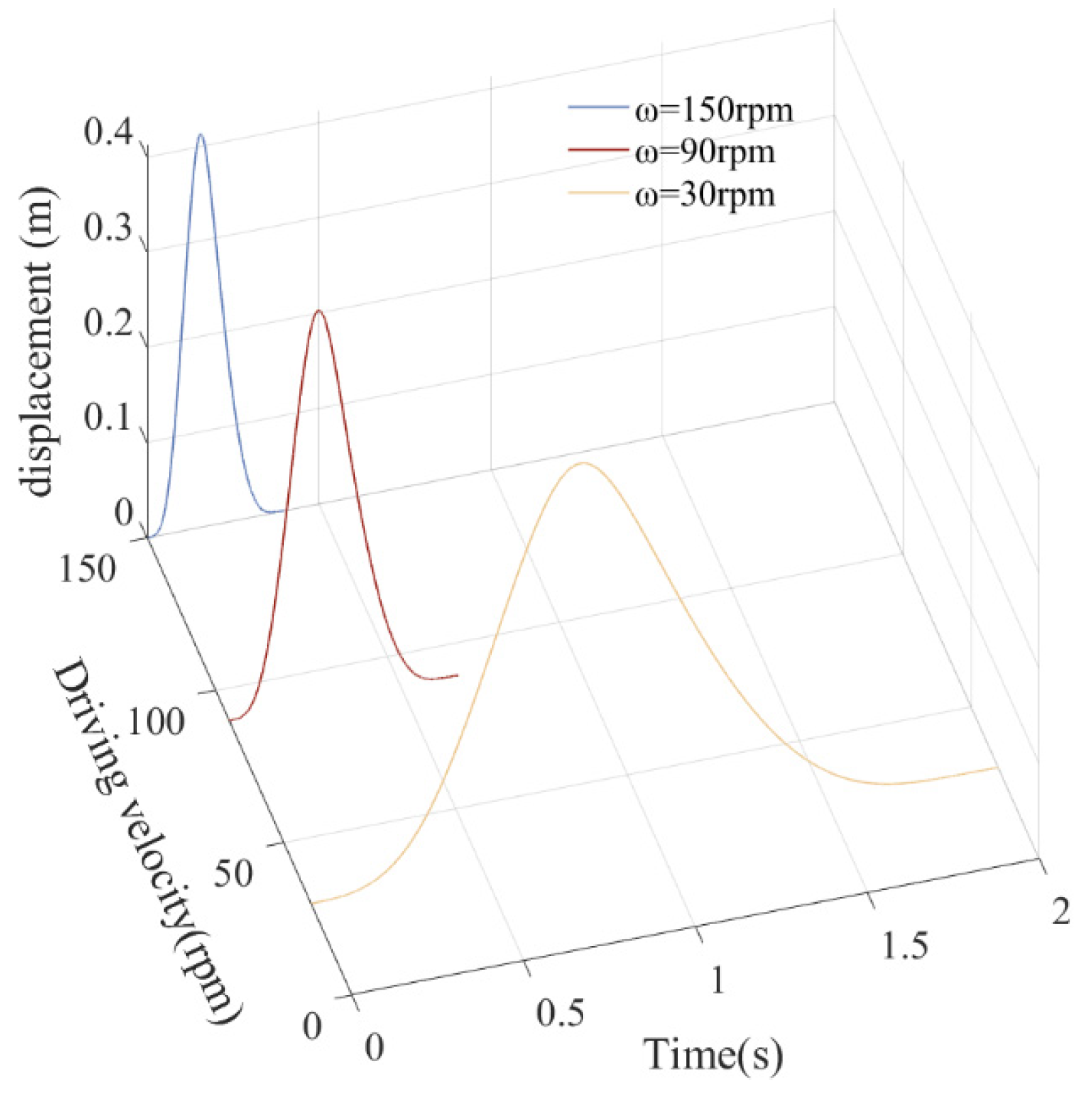
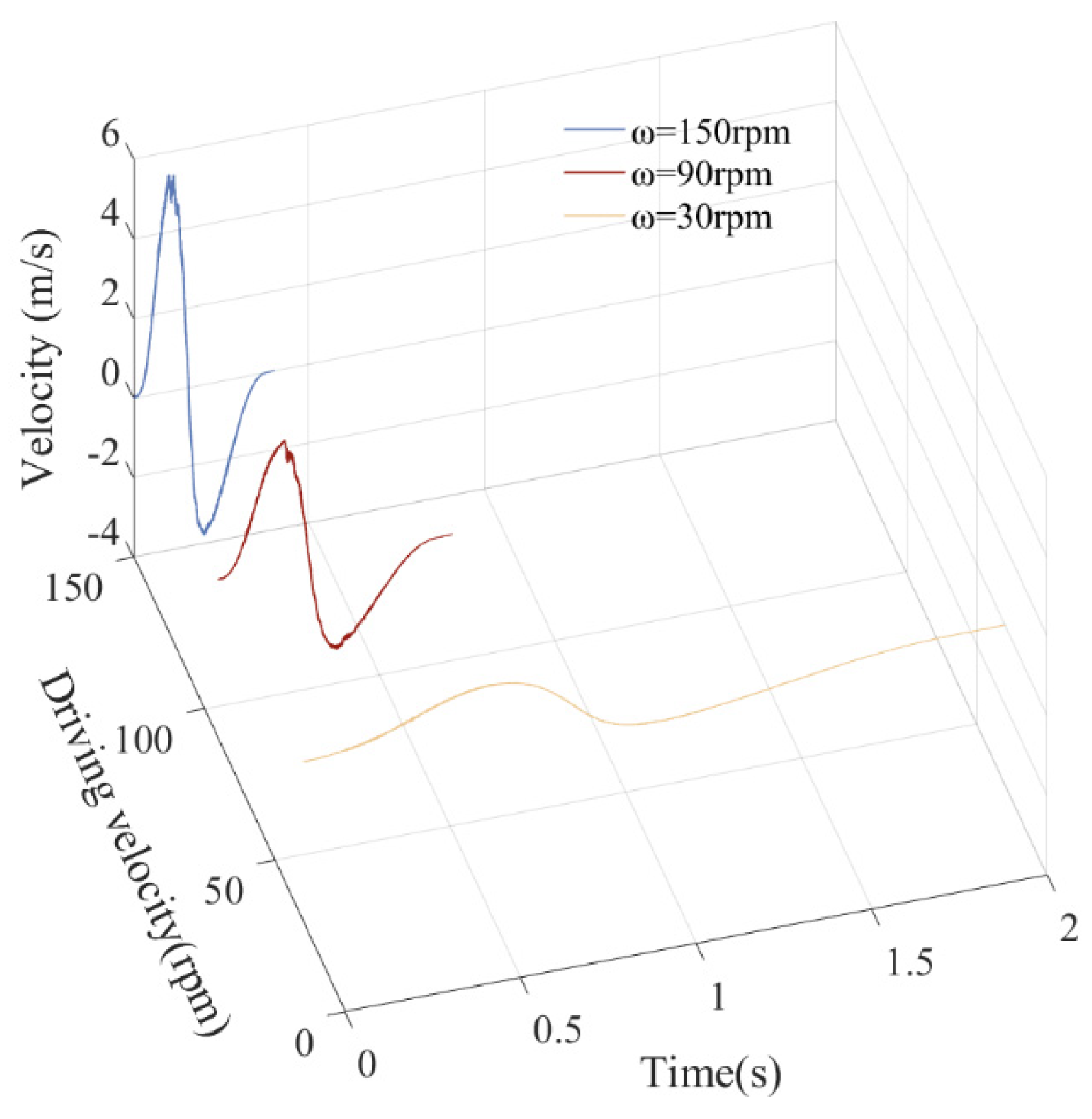

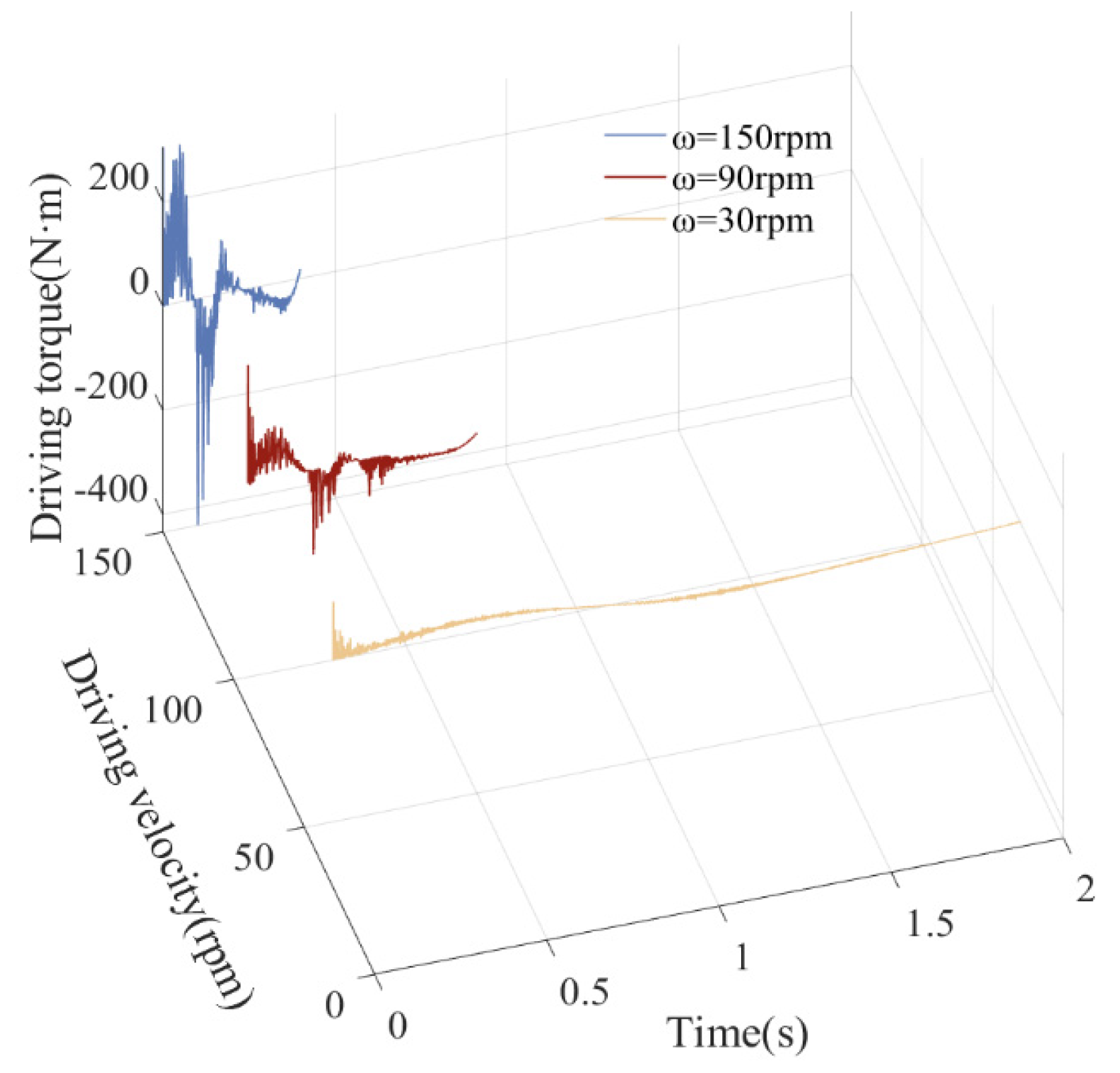



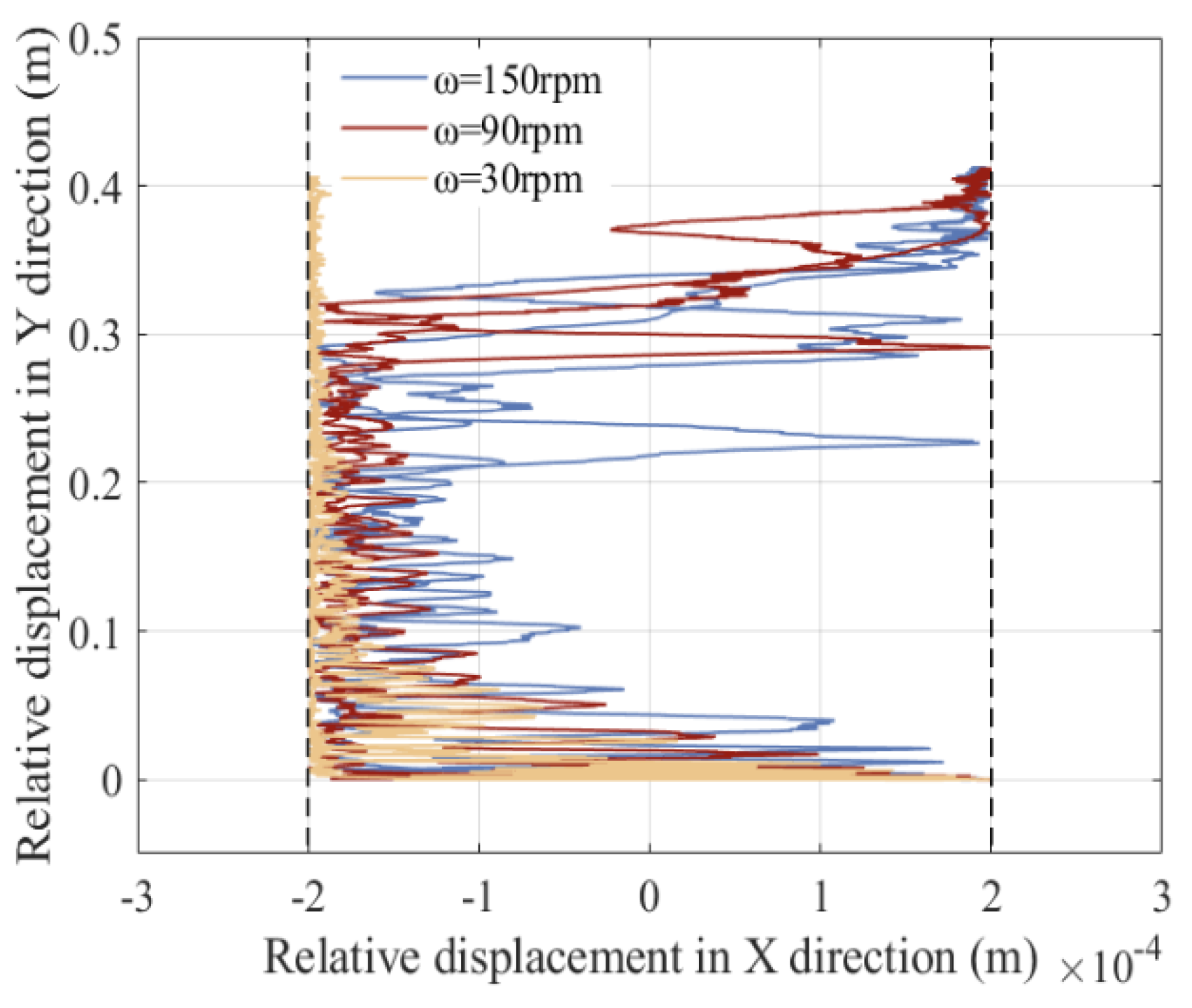
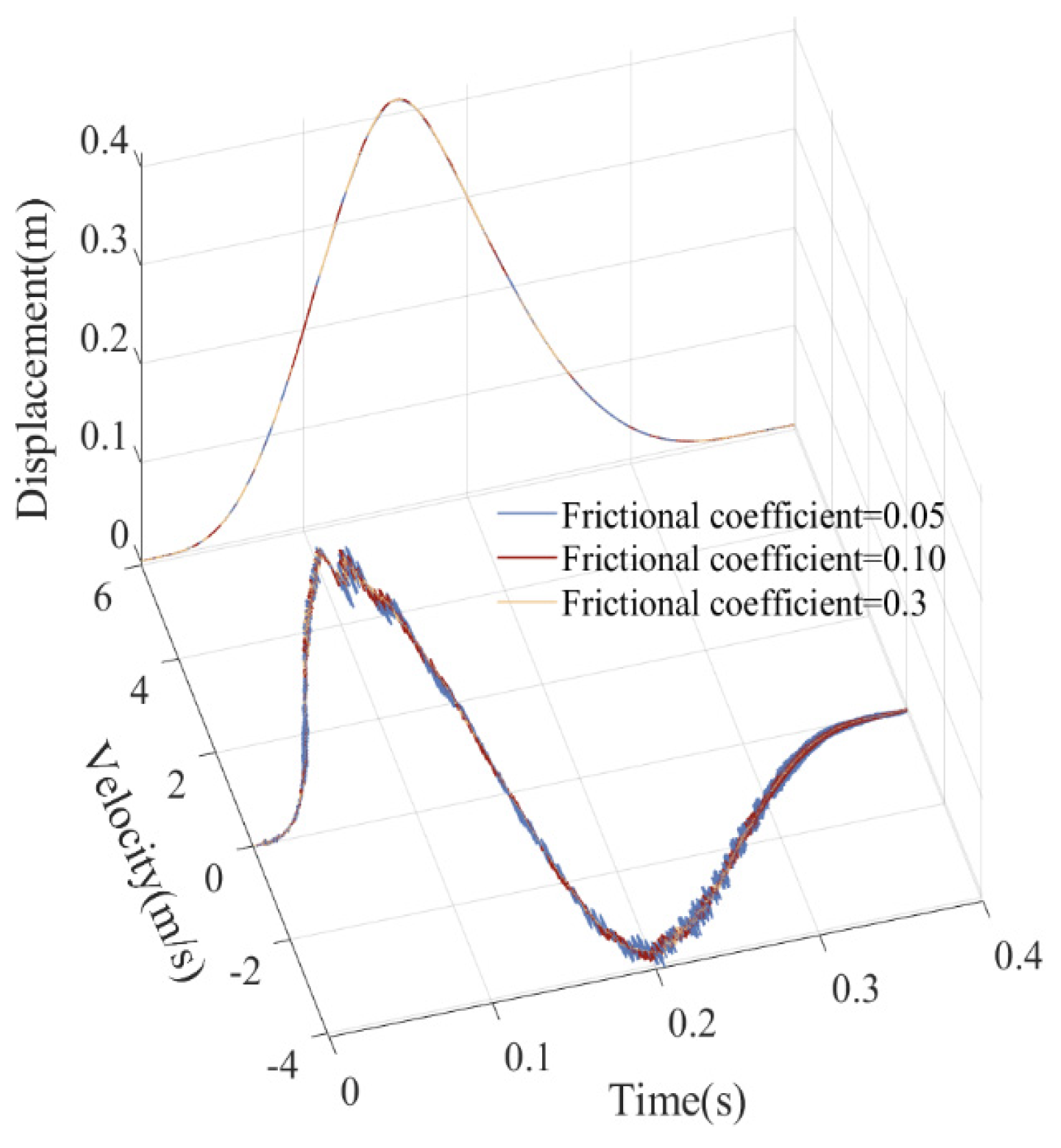
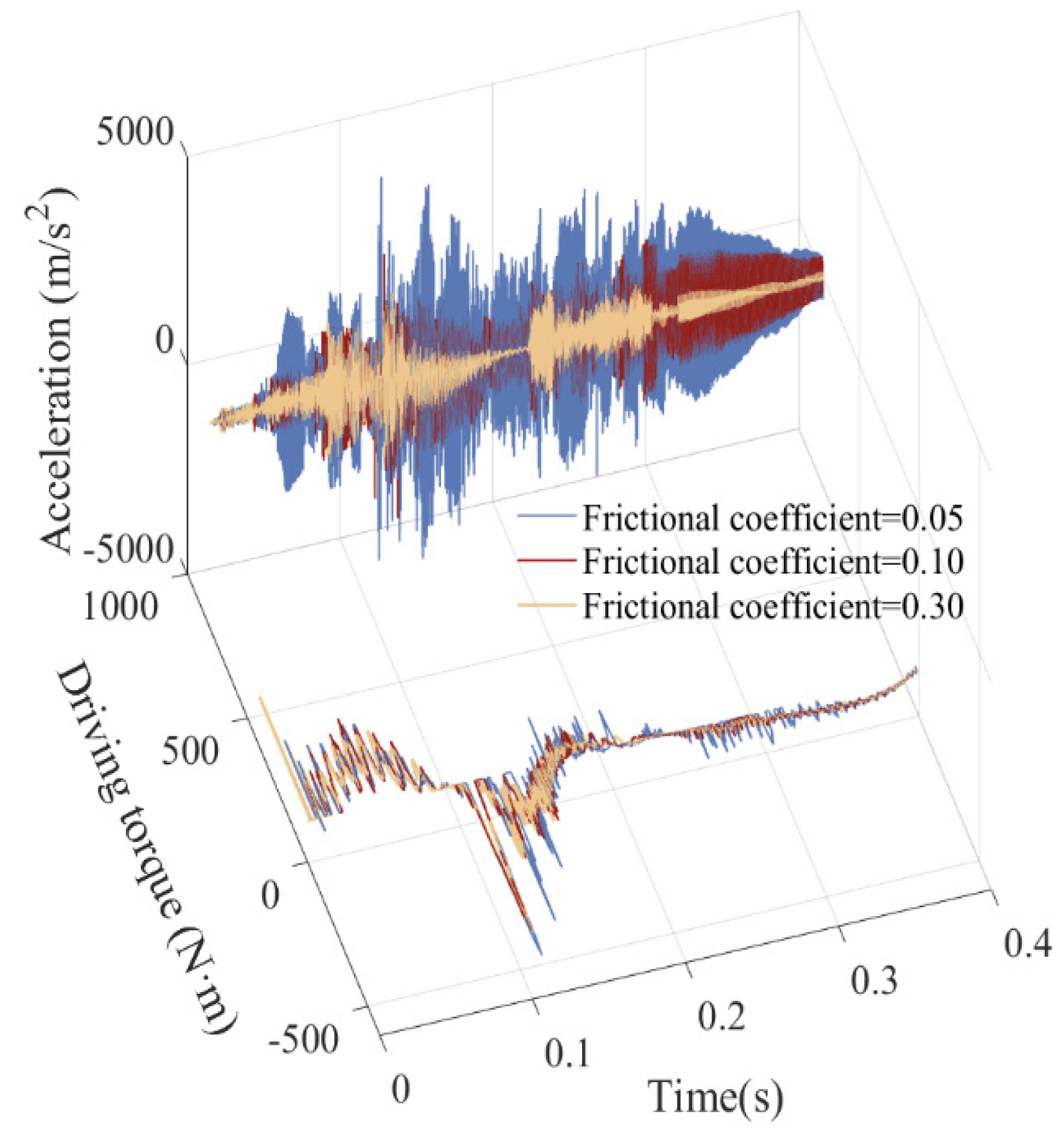



| Component | Length (mm) | Mass (kg) | Moment of Inertia (kg·m2) |
|---|---|---|---|
| Crank 1 | 75 | 0.738 (m1) | 1.977 × 10−9 (J1) |
| Rod 2 | 584 (L2) | 4.708 (m2) | 546.91 × 10−9 (J2) |
| Rod 3 | 555.14 (L31) | 21.483 (m3) | 1348.94 × 10−9 (J3) |
| 985 (L32) | |||
| 473.66 (L33) | |||
| Rod 4 | 450 (L4) | 3.663 (m4) | 69.26 × 10−9 (J4) |
| Slider 5 | 150 (LT) | 0.922 (m5) | 1.059 × 10−9 (J5) |
| 100 (HT) |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Restitution coefficient ce | 0.9 [7] | Limited speed v1/(m/s) | 0.001 [7] |
| Elastic modulus Ei (i = 1, 2, m, n)/GPa | 207 [23] | Limited speed v0/(m/s) | 0.0001 [7] |
| Poisson’s ratio νi (i = 1, 2, m, n) | 0.3 [7] | Cross-sectional area Aa/m2 | 0.001 |
| Density ρ/(g/cm3) | 7.85 | Second moment of area If/m4 | 3.333 × 10−8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niu, J.; Zhang, H.; Jiang, S. A Study on the Influence of Disturbance Factors’ Coupling Effects on the Dynamic Response of the Symmetrical Structure Press Mechanism. Symmetry 2025, 17, 730. https://doi.org/10.3390/sym17050730
Niu J, Zhang H, Jiang S. A Study on the Influence of Disturbance Factors’ Coupling Effects on the Dynamic Response of the Symmetrical Structure Press Mechanism. Symmetry. 2025; 17(5):730. https://doi.org/10.3390/sym17050730
Chicago/Turabian StyleNiu, Jun, Hao Zhang, and Shuai Jiang. 2025. "A Study on the Influence of Disturbance Factors’ Coupling Effects on the Dynamic Response of the Symmetrical Structure Press Mechanism" Symmetry 17, no. 5: 730. https://doi.org/10.3390/sym17050730
APA StyleNiu, J., Zhang, H., & Jiang, S. (2025). A Study on the Influence of Disturbance Factors’ Coupling Effects on the Dynamic Response of the Symmetrical Structure Press Mechanism. Symmetry, 17(5), 730. https://doi.org/10.3390/sym17050730





