On the Approximations and Symmetric Properties of Frobenius–Euler–Şimşek Polynomials Connecting Szász Operators
Abstract
1. Introduction and Preliminaries
2. Voronovskaja-Type Theorem and Order of Approximation
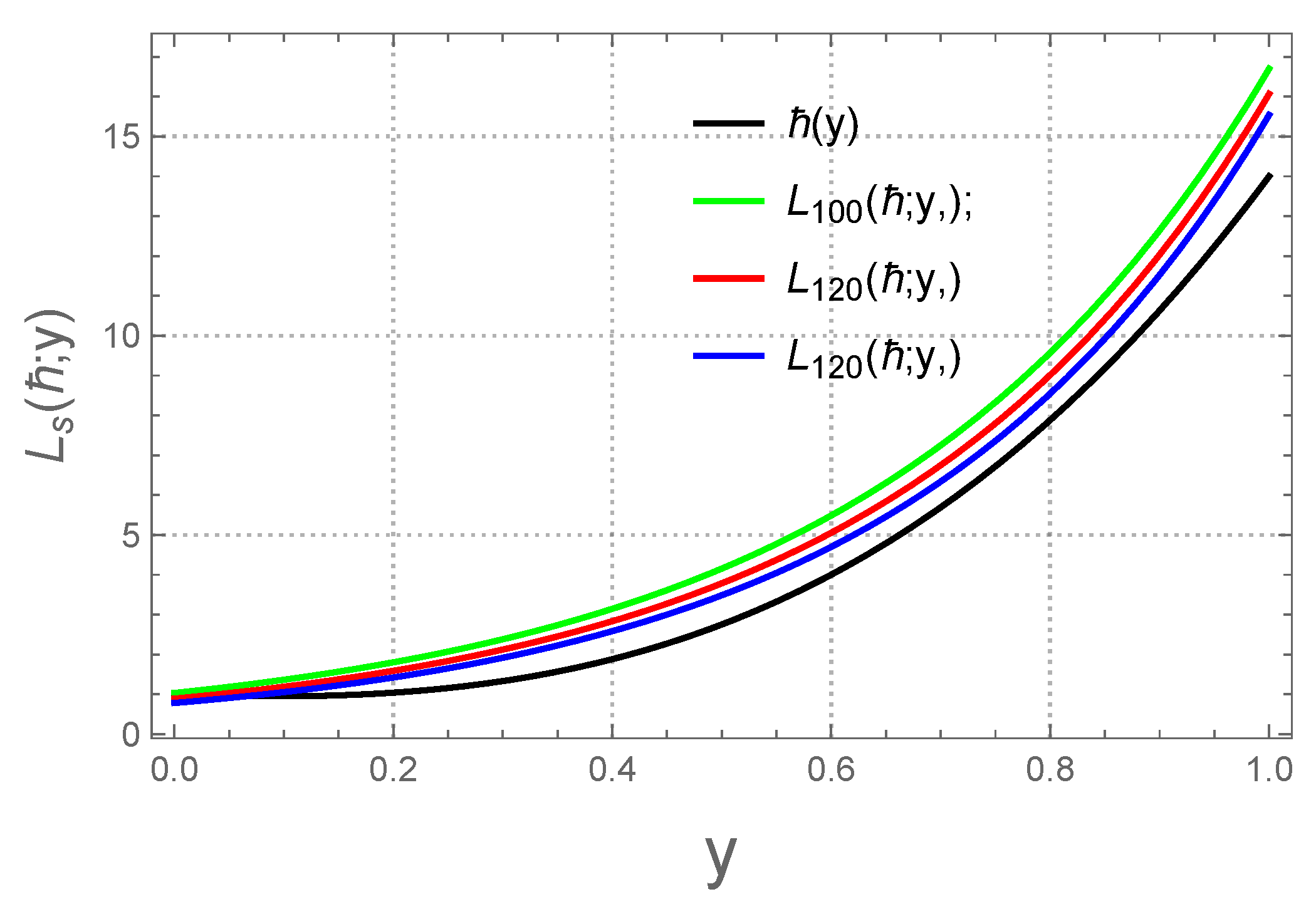
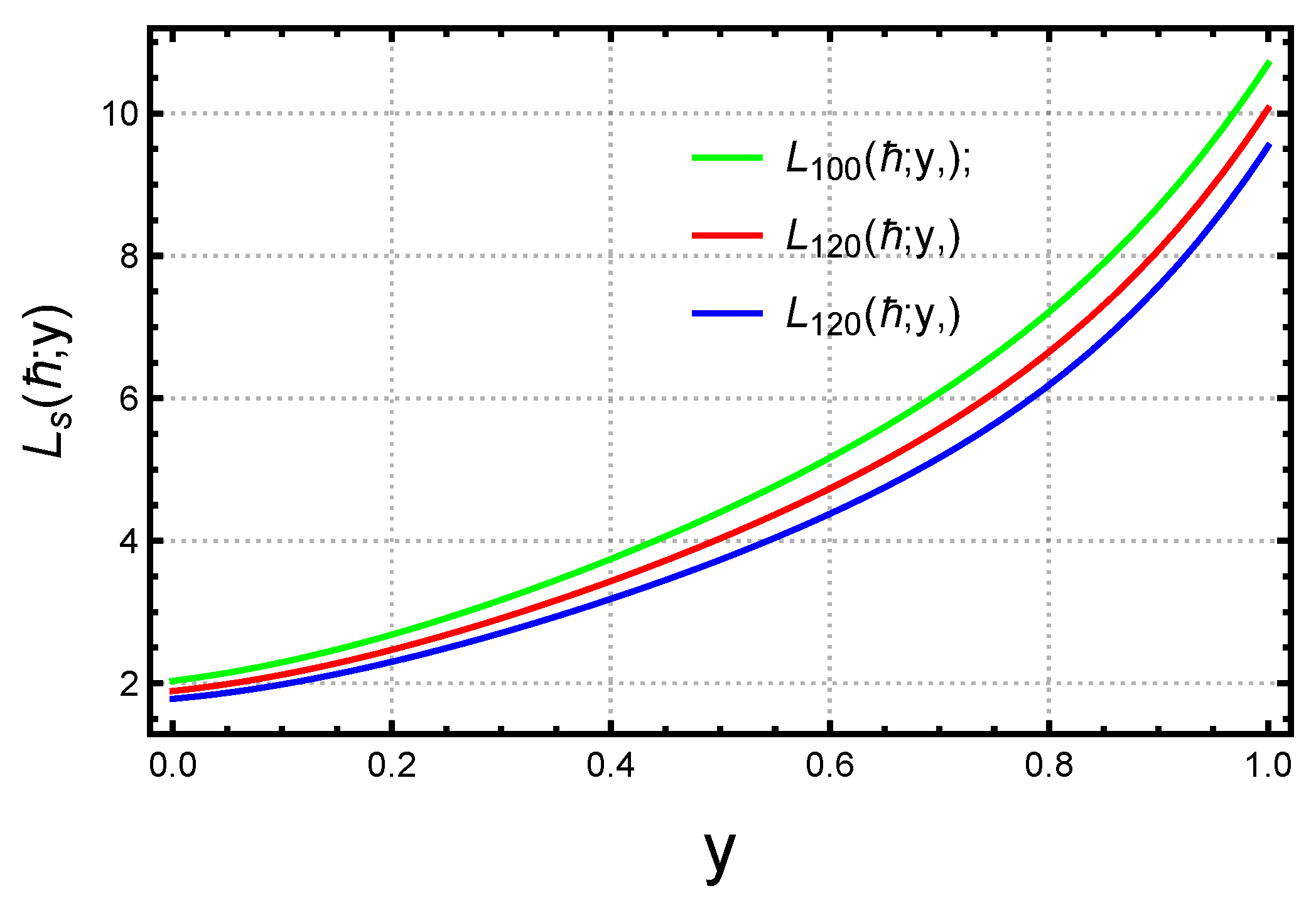
3. Convergence Analysis via Numerical and Graphical Methods
Significance of Numerical Analysis
4. Results on Local Approximation
5. A Bivariate Frobenius–Şimşek–Euler Polynomial Representation of Szász Operators
- ,
- for .
6. Error Bounds and Approximation Order
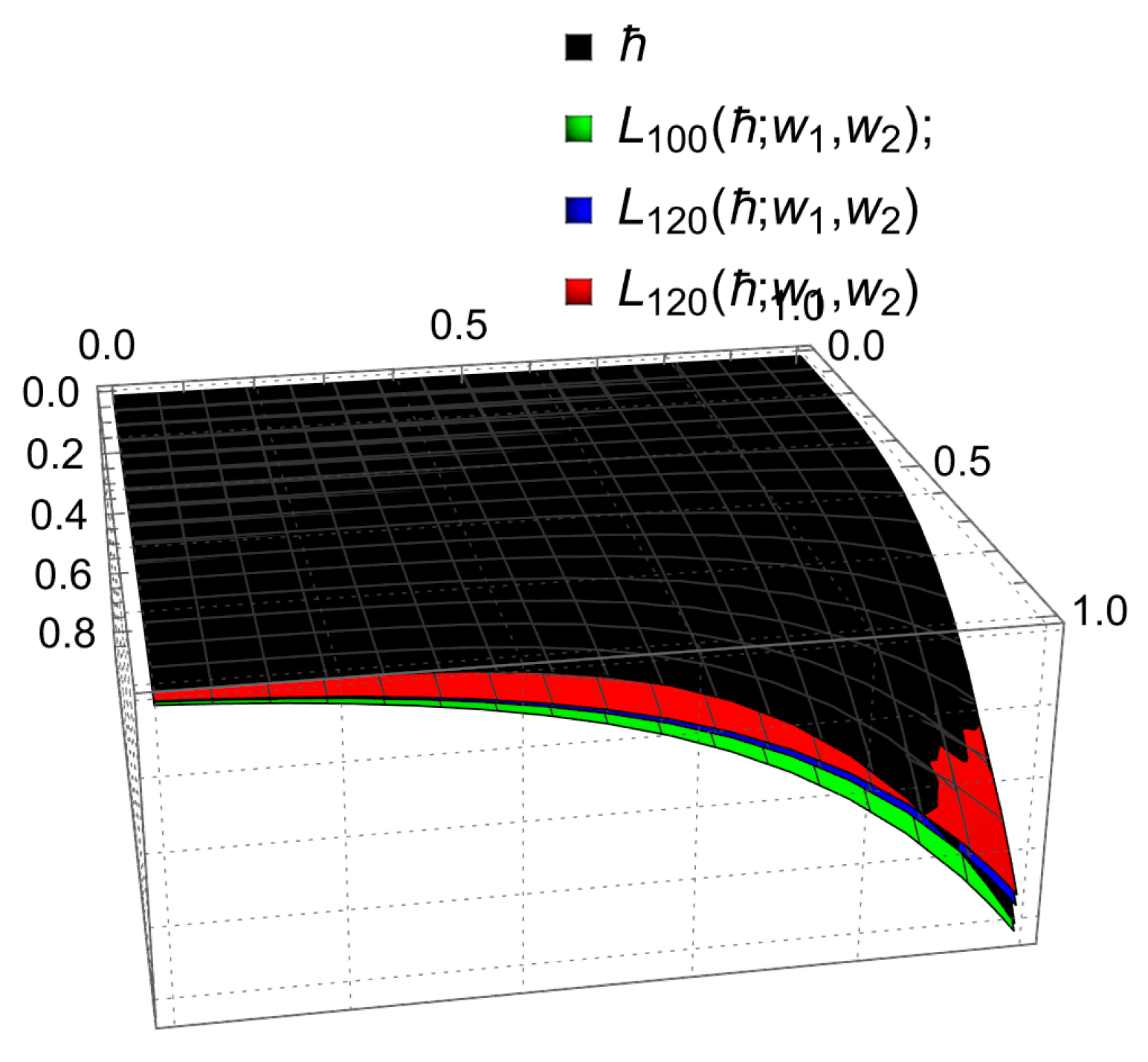
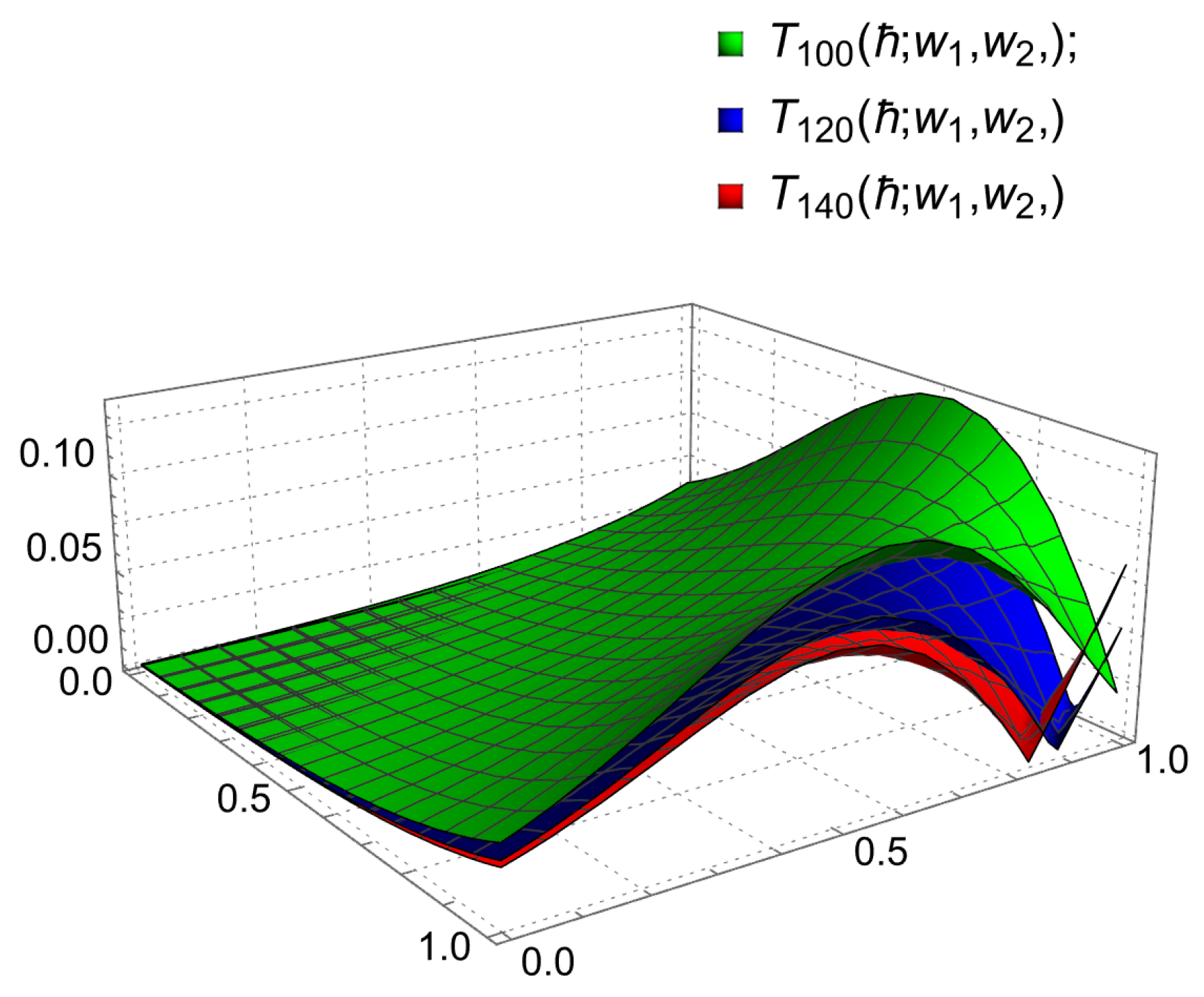
7. Bivariate Operators: Graphical and Tabular Representations
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bernstein, S.N. Démonstration du théorème de Weierstrass fondée sur le calcul de probabilités. Commun. Soc. Math. Kharkow. 1913, 13, 1–2. [Google Scholar]
- Weierstrass, K. Über die analytische Darstellbarkeit sogenannter willkürlicher Functionen einer reellen Veränderlichen. Sitzungsberichte KöNiglichen PreußIschen Akad. Wiss. Berl. 1885, 2, 633–639. [Google Scholar]
- Agyuz, E. Onthe convergence properties of generalized Szász–Kantorovich type operators involving Frobenious–Euler–Simsek-type polynomials. Aims Math. 2024, 9, 28195–28210. [Google Scholar] [CrossRef]
- Khan, K.; Lobiyal, D.K. Bézier curves based on Lupăs (p, q)-analogue of Bernstein functions in CAGD. Comput. Appl. Math. 2017, 317, 458–477. [Google Scholar] [CrossRef]
- Khan, K.; Lobiyal, D.K.; Kilicman, A. Bézier Curves and Surfaces Based on Modified Bernstein Polynomials. Azerb. J. Math. 2019, 9, 3–21. [Google Scholar]
- Izadbakhsh, A.; Kalat, A.A.; Khorashadizadeh, S. Observer-based adaptive control for HIV infection therapy using the Baskakov operator. Biomed. Signal Process. Control 2021, 65, 102343. [Google Scholar] [CrossRef]
- Uyan, H.; Aslan, A.O.; Karateke, S.; Büyükyazıcı, İ. Interpolation for neural network operators activated with a generalized logistic-type function. J. Inequalities Appl. 2024, 2024, 125. [Google Scholar] [CrossRef]
- Zhang, Q.; Mu, M.; Wang, X. A Modified Robotic Manipulator Controller Based on Bernstein-Kantorovich-Stancu Operator. Micromachines 2022, 14, 44. [Google Scholar] [CrossRef]
- Özger, F. Weighted statistical approximation properties of univariate and bivariate λ-Kantorovich operators. Filomat 2019, 33, 3473–3486. [Google Scholar] [CrossRef]
- Özger, F.; Ansari, K.J. Statistical convergence of bivariate generalized Bernstein operators via four-dimensional infinite matrices. Filomat 2022, 36, 507–525. [Google Scholar] [CrossRef]
- Aslan, R. Rate of approximation of blending type modified univariate and bivariate λ-Schurer-Kantorovich operators. Kuwait J. Sci. 2024, 51, 100168. [Google Scholar] [CrossRef]
- Aslan, R. Approximation properties of univariate and bivariate new class-Bernstein–Kantorovich operators and its associated GBS operators. Comp. Appl. Math. 2023, 42, 34. [Google Scholar] [CrossRef]
- Ayman-Mursaleen, M.; Heshamuddin, M.; Rao, N.; Sinha, B.K.; Yadav, A.K. Hermite polynomials linking Szász–Durrmeyer operators. Comp. Appl. Math. 2024, 43, 223. [Google Scholar] [CrossRef]
- Ayman-Mursaleen, M.; Rao, N.; Rani, M.; Kilicman, A.; Al-Abied, A.A.H.A.; Malik, P. A Note on Approximation of Blending Type Bernstein–Schurer–Kantorovich Operators with Shape Parameter α. J. Math. 2023, 2023, 5245806. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Acar, T.; Alotaibi, A. Construction of a new family of Bernstein-Kantorovich operators. Math. Methods Appl. Sci. 2017, 40, 7749–7759. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Ahmad, N.; Özger, F.; Alotaibi, A.; Hazarika, B. Approximation by the parametric generalization of Baskakov–Kantorovich operators linking with Stancu operators. Iran. J. Sci. Technol. Trans. 2021, 45, 593–605. [Google Scholar] [CrossRef]
- Mursaleen, M.; Ansari, K.J.; Khan, A. Approximation properties and error estimation of q-Bernstein shifted operators. Numer. Algorithms 2020, 84, 207–227. [Google Scholar] [CrossRef]
- Mursaleen, M.; Naaz, A.; Khan, A. Improved approximation and error estimations by King type (p, q)-Szász-Mirakjan Kantorovich operators. Appl. Math. Comput. 2019, 348, 2175–2185. [Google Scholar] [CrossRef]
- Khan, A.; Mansoori, M.; Khan, K.; Mursaleen, M. Phillips-type q-Bernstein operators on triangles. J. Funct. Spaces 2021, 2021, 6637893. [Google Scholar] [CrossRef]
- Nasiruzzaman, M. Approximation properties by Szász–Mirakjan operators to bivariate functions via Dunkl analogue. Iran. J. Sci. Technol. Trans. 2011, 45, 259–269. [Google Scholar] [CrossRef]
- Braha, N.L.; Loku, V.; Mansour, T.; Mursaleen, M. A new weighted statistical convergence and some associated approximation theorems. Math. Methods Appl. Sci. 2022, 45, 5682–5698. [Google Scholar] [CrossRef]
- Rao, N.; Farid, M.; Ali, R.A. Study of Szász–Durremeyer-Type Operators Involving Adjoint Bernoulli Polynomials. Mathematics 2024, 12, 3645. [Google Scholar] [CrossRef]
- Rao, N.; Farid, M.; Raiz, M. Symmetric Properties of λ-Szász Operators Coupled with Generalized Beta Functions and Approximation Theory. Symmetry 2024, 16, 1703. [Google Scholar] [CrossRef]
- Rao, N.; Farid, M.; Raiz, M. Approximation Results: Szász– Kantorovich Operators Enhanced by Frobenius–Euler–Type Polynomials. Axioms 2025, 14, 252. [Google Scholar] [CrossRef]
- Çetin, N. Approximation and geometric properties of complex α-Bernstein operator. Results Math. 2019, 74, 40. [Google Scholar] [CrossRef]
- Çetin, N.; Radu, V.A. Approximation by generalized Bernstein-Stancu operators. Turk. J. Math. 2019, 43, 2032–2048. [Google Scholar] [CrossRef]
- Arı, D.A.; Yılmaz, G.U. A Note On Kantorovich Type Operators Which Preserve Affine Functions. Fundam. J. Math. Appl. 2024, 1, 53–58. [Google Scholar]
- Çiçek, H.; İzgi, A. Approximation by Modified Bivariate Bernstein-Durrmeyer and GBS Bivariate Bernstein-Durrmeyer Operators on a Triangular Region. Fundam. J. Math. Appl. 2022, 5, 135–144. [Google Scholar] [CrossRef]
- Aslan, R. Some Approximation Results on λ–Szász-Mirakjan-Kantorovich Operators. Fundam. J. Math. Appl. 2021, 4, 150–158. [Google Scholar] [CrossRef]
- Baytunç, E.; Aktuğlu, H.; Mahmudov, N. A New Generalization of Szász-Mirakjan Kantorovich Operators for Better Error Estimation. Fundam. J. Math. Appl. 2023, 6, 194–210. [Google Scholar] [CrossRef]
- Qazza, A.; Bendib, I.; Hatamleh, R.; Saadeh, R.; Ouannas, A. Finite-time synchronization control for fractional-order reaction–diffusion systems with Neumann boundary conditions. Partial. Diff. Equations Appl. Math. 2024, 13, 101125. [Google Scholar]
- Qazza, A.; Bendib, I.; Hatamleh, R.; Saadeh, R.; Ouannas, A. Dynamics of the Gierer–Meinhardt reaction–diffusion system: Insights into finite-time stability and control strategies. Partial. Differ. Equ. Appl. Math. 2025, 13, 101142. [Google Scholar] [CrossRef]
- Batiha, I.M.; Ogilat, O.; Bendib, I.; Ouannas, A.; Jebril, I.H.; Anakira, N. Finite-time dynamics of the fractional-order epidemic model: Stability, synchronization, and simulations. Chaos, Solitons Fractals X 2024, 130, 100118. [Google Scholar] [CrossRef]
- Sadek, L.; Baleanu, D.; Abdo, M.S.; Shatanawi, W. Introducing novel θ-fractional operators: Advances in fractional calculus. J. King Saud-Univ.-Sci. 2024, 36, 103352. [Google Scholar] [CrossRef]
- Sadek, L. Controllability, observability, and stability of ϕ-conformable fractional linear dynamical systems. Asian J. Control. 2024, 26, 2476–2494. [Google Scholar] [CrossRef]
- Sadek, L.; Lazar, T.A. On Hilfer cotangent fractional derivative and a particular class of fractional problems. Aims Math. 2023, 8, 28334–28352. [Google Scholar] [CrossRef]
- Sadek, L.; Jarad, F. The general Caputo–Katugampola fractional derivative and numerical approach for solving the fractional differential equations. Alex. Eng. J. 2025, 121, 539–557. [Google Scholar] [CrossRef]
- Hou, Q.; Li, Y.; Singh, V.P.; Sun, Z. Physics-informed neural network for diffusive wave model. J. Hydrol. 2024, 637, 131261. [Google Scholar] [CrossRef]
- Feng, G.; Yu, S.; Wang, T.; Zhang, Z. Discussion on the Weak Equivalence Principle for a Schwarzschild Gravitational Field Based on the Light-Clock Model. Ann. Phys. 2025, 473, 169903. [Google Scholar] [CrossRef]
- Sun, W.; Jin, Y.; Lü, G. Genuine Multipartite Entanglement from a Thermodynamic Perspective. Phys. Rev. A 2024, 109, 042422. [Google Scholar] [CrossRef]
- Lenze, B. On Lipschitz type maximal functions and their smoothness spaces. Nederl. Akad. Indag. Math. 1988, 50, 53–63. [Google Scholar] [CrossRef]
- Simsek, Y. Applications of constructed new families of generating-type functions interpolating new and known classes of polynomials and numbers. Math. Methods Appl. Sci. 2021, 44, 11245–11268. [Google Scholar] [CrossRef]
- Araci, S.; Duran, U.; Acikgoz, M. Symmetric Identities Involving q-Frobenius-Euler Polynomials under Sym (5). Turk. J. Anal. Number Theory 2015, 3, 90–93. [Google Scholar] [CrossRef]
- Kucukoglu, I. Generating functions for multiparametric Hermite-based Peters-type Simsek numbers and polynomials in several variables. Montes Taurus J. Pure Appl. Math. 2024, 6, 217–238. [Google Scholar]
- Kucukoglu, I.; Simsek, Y. Identities and relations on the q-Apostol type Frobenius-Euler numbers and polynomials. J. Korean Math. Soc. 2019, 56, 265–284. [Google Scholar]
- DeVore, R.A.; Lorentz, G.G. Constructive Approximation; Springer: Berlin, Germany, 1993. [Google Scholar]
- Altomare, F.; Campiti, M. Korovkin-type approximation theory and its applications. De Gruyter Ser. Stud. Math. 1994, 17, 266–274. [Google Scholar]
- Özarslan, M.A.; Aktuğlu, H. Local approximation for certain King type operators. Filomat 2013, 27, 173–181. [Google Scholar] [CrossRef]
- Volkov, V.I. On the convergence of sequences of linear positive operators in the space of continuous functions of two variables. Dokl. Akad. Nauk SSSR (NS) 1957, 115, 17–19. (In Russian) [Google Scholar]
- Stancu, F. Aproximarca Funcţiilor de Două şi Mai Multe Variabile Prin şiruri de Operatori Liniari şi Pozitivi. Ph.D. Thesis, Babeṣ-Bolyai University, Cluj-Napoca, Romania, 1984. (In Romanian). [Google Scholar]
| 0.1 | 2.29412 | 2.11053 | 1.98317 |
| 0.2 | 2.68187 | 2.46853 | 2.30031 |
| 0.3 | 2.68187 | 2.91136 | 2.70543 |
| 0.4 | 3.17009 | 3.43292 | 3.18315 |
| 0.5 | 4.40275 | 4.03316 | 3.73363 |
| 0.6 | 5.16538 | 4.73213 | 4.37807 |
| 0.7 | 6.07566 | 5.57675 | 5.16583 |
| 0.8 | 7.21082 | 6.64978 | 6.18452 |
| 0.9 | 8.69213 | 8.08191 | 7.57317 |
| 0.1, 0.1 | 0.00291072 | 0.00203763 | 0.00173559 |
| 0.2, 0.2 | 0.00547611 | 0.00394756 | 0.00340736 |
| 0.3, 0.3 | 0.102474 | 0.00759292 | 0.006634474 |
| 0.4, 0.4 | 0.0187716 | 0.0142067 | 0.0125241 |
| 0.5, 0.5 | 0.0327879 | 0.0250319 | 0.0221139 |
| 0.6, 0.6 | 0.0529577 | 0.0399807 | 0.034999 |
| 0.7, 0.7 | 0.0763755 | 0.055093 | 0.0467623 |
| 0.8, 0.8 | 0.0931754 | 0.0592102 | 0.0456669 |
| 0.9, 0.9 | 0.0838775 | 0.317595 | 0.106255 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rao, N.; Farid, M.; Raiz, M. On the Approximations and Symmetric Properties of Frobenius–Euler–Şimşek Polynomials Connecting Szász Operators. Symmetry 2025, 17, 648. https://doi.org/10.3390/sym17050648
Rao N, Farid M, Raiz M. On the Approximations and Symmetric Properties of Frobenius–Euler–Şimşek Polynomials Connecting Szász Operators. Symmetry. 2025; 17(5):648. https://doi.org/10.3390/sym17050648
Chicago/Turabian StyleRao, Nadeem, Mohammad Farid, and Mohd Raiz. 2025. "On the Approximations and Symmetric Properties of Frobenius–Euler–Şimşek Polynomials Connecting Szász Operators" Symmetry 17, no. 5: 648. https://doi.org/10.3390/sym17050648
APA StyleRao, N., Farid, M., & Raiz, M. (2025). On the Approximations and Symmetric Properties of Frobenius–Euler–Şimşek Polynomials Connecting Szász Operators. Symmetry, 17(5), 648. https://doi.org/10.3390/sym17050648







