The Generalized Euler Method for Analyzing Zoonotic Disease Dynamics in Baboon–Human Populations

Abstract

This study presents a novel fractional-order mathematical model to investigate zoonotic disease transmission between humans and baboons, incorporating the Generalized Euler Method and highlighting key control strategies such as sterilization, restricted food access, and reduced human–baboon interaction. The model’s structure exhibits an inherent symmetry in the transmission dynamics between baboon and human populations, reflecting balanced interaction patterns. This symmetry is further analyzed through the stability of infection-free and endemic equilibrium points, guided by the basic reproduction number ${\mathcal{R}}_0$. Theoretical analyses confirmed the existence, uniqueness, and boundedness of solutions, while sensitivity analysis identified critical parameters influencing disease spread. Numerical simulations validated the effectiveness of intervention strategies, demonstrating the impact of symmetrical measures on minimizing zoonotic disease risks and promoting balanced population health outcomes. This work contributes to epidemiological modeling by illustrating how symmetry in control interventions can optimize zoonotic disease management.

1. Introduction

Zoonotic diseases, or infections that are transmitted between animals and humans, represent a significant public health challenge worldwide [1]. As human activities increasingly encroach on wildlife habitats, the risk of zoonotic disease transmission has escalated [2]. Among the various species that can act as reservoirs for zoonotic diseases, baboons (genus Papio) are notable for their frequent interactions with human populations, particularly in regions like Al-Baha, Saudi Arabia [3]. These interactions, often resulting from competition for food and habitat, create opportunities for the transmission of diseases that can spread between humans and baboons [4].

In the Al-Baha region, the presence of baboons has been linked to potential zoonotic outbreaks, largely due to their proximity to human settlements and the overlap in resource use [5]. Diseases such as tuberculosis and other viral infections, which can be transmitted through direct contact or contaminated resources, pose a serious risk to both baboon and human populations [6]. Moreover, the behavior of feeding baboons, particularly by local residents and tourists, exacerbates this interaction, increasing the chances of zoonotic disease transmission [7].

Mathematical modeling plays a crucial role in analyzing disease transmission dynamics in both human and animal populations [8]. These models enable the examination of intricate relationships by simulating various parameters, such as population density, disease spread rates, and preventive interventions, to better understand disease behavior [9]. This research adopts a modeling framework to capture the transmission dynamics of zoonotic diseases between baboons and humans in the Al-Baha region. By combining mathematical approaches with real-world data, we aim to enhance knowledge of zoonotic infections and aid public health initiatives in mitigating outbreak risks. The outcomes of this study also hold potential significance for managing wildlife populations and controlling infectious diseases in regions characterized by frequent human-animal interactions [10].

In recent years, fractional calculus has emerged as a powerful tool for describing systems that exhibit memory and hereditary properties [11]. Its growing popularity is evident in fields such as engineering [12], plant pathology [13], biology [14], medicine [15], behavioral sciences [16], viscoelastic materials [17], electromagnetic waves [18], quantum mechanics [19], Langevin equations [20], physics [21,22,23,24], diabetes modeling [25,26,27,28,29,30,31], cybersecurity [32], smoking dynamics [33], epidemiology [34,35], influenza [36], infectious disease outbreaks [37], epidemic modeling [38], cancer research [39], COVID-19 [40], monkeypox [41], zoonotic viruses [42], and alcohol-related studies [43]. Epidemiological models, in particular, have found extensive applications in various disciplines, such as healthcare, chemistry, physics, and economic modeling [44,45,46,47,48,49,50,51,52,53]. Sadek et al. (2024) expanded fractional calculus by proposing innovative $\mathsf{\Theta }$-fractional operators and demonstrating their effectiveness in optimization problems using the Galerkin-Bell technique [49]. In a related contribution, Sadek (2023) introduced a cotangent-based fractional derivative, offering an alternative framework for solving complex differential equations [50]. Building on this concept, Sadek and Lazar (2023) applied the Hilfer cotangent fractional operator to a specialized set of problems, bridging classical and fractional calculus perspectives [51]. In epidemiology, Sadek et al. (2023) proposed a fractional-order COVID-19 model for Morocco that incorporated vaccination and isolation measures, enhancing accuracy by accounting for memory effects [52]. Additionally, Sadek et al. (2022) utilized fractional calculus to describe the low-temperature synthesis of TiO₂ nanopowder via the sol-gel technique, improving nanomaterial production models [53]. These advancements underscore fractional calculus’ versatility across multiple scientific domains.

Compared to traditional integer-order models, fractional calculus provides distinct advantages when describing dynamic systems. Despite this, solving fractional differential equations (FDEs) analytically can be highly challenging, necessitating numerical methods. Numerous techniques have been developed to approximate FDE solutions. For instance, the generalized Euler scheme [54,55] efficiently handles Caputo-type FDEs and is relatively simple to implement. However, it may not be suitable for solving higher-order equations. Other numerical approaches, including the Adomian decomposition method [56], the homotopy perturbation method [57,58], the homotopy analysis method [59,60], the Taylor matrix technique [61,62], and the Haar wavelet approach [63], have been successfully employed to study fractional systems.

This research focuses on understanding the transmission dynamics of zoonotic diseases in a baboon-human population system, while integrating control measures such as sterilization, food access restriction, and minimizing human-baboon interactions [64]. The mathematical analysis ensures the model’s reliability by demonstrating the existence, uniqueness, positivity, and boundedness of its solutions [65]. Furthermore, the basic reproductive number (${\mathcal{R}}_0$) is calculated to evaluate the likelihood of disease outbreak or eradication [66]. Sensitivity analysis identifies critical parameters affecting disease spread, offering guidance for designing effective control strategies [67].

This study introduces a fractional-order epidemiological model for zoonotic disease transmission between baboons and humans, with a focus on key interventions, including sterilization, limiting food access, and reducing contact. A rigorous mathematical examination confirms the model’s well-posedness by establishing solution properties such as existence, uniqueness, and positivity. The basic reproductive number (${\mathcal{R}}_0$) is derived to assess the conditions under which the disease persists or is eliminated. A sensitivity analysis pinpoints influential factors affecting disease dynamics. Additionally, the numerical solutions of the fractional-order system are approximated using the Generalized Euler Method, which is known for its computational efficiency and accuracy in solving FDEs. The simulations provide valuable insights into the potential impact of various control strategies on reducing zoonotic disease risk.

The remainder of this paper is structured as follows: Section 2 describes the model formulation, including fractional-order differential equations. Section 3 provides a theoretical analysis of the model and its well-posedness. Section 4 discusses the equilibrium stability and computation of ${\mathcal{R}}_0$. Section 5 outlines the numerical approach, specifically the Generalized Euler Method. Finally, Section 6 interprets the results, and Section 7 concludes with recommendations for future work.

2. Baboon and Human Population Model Description

The following model aims to simulate the dynamics of baboon and human populations in the context of zoonotic disease transmission. The model incorporates control strategies such as sterilization, restricted food access, and reduced human–baboon interactions. The baboon population dynamics are modeled using the following equations, where we account for natural growth, disease transmission, and control actions. The combined system of equations modeling the dynamics of baboon and human populations with zoonotic disease transmission is as follows:

$$\begin{array}{cc}\hfill {\displaystyle \frac{d{\mathrm{S}}_b}{dt}}& =r{\mathrm{S}}_b\left(1-{\displaystyle \frac{{\mathrm{S}}_b+{\mathrm{I}}_b+{\mathrm{R}}_b}{K}}\right)-{\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-H_s\left(t\right){\mathrm{S}}_b-H_f\left(t\right){\mathrm{S}}_b,\hfill \\ \hfill {\displaystyle \frac{d{\mathrm{I}}_b}{dt}}& ={\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-{\gamma }_b{\mathrm{I}}_b-H_s\left(t\right){\mathrm{I}}_b-H_f\left(t\right){\mathrm{I}}_b,\hfill \\ \hfill {\displaystyle \frac{d{\mathrm{R}}_b}{dt}}& ={\gamma }_b{\mathrm{I}}_b-H_f\left(t\right){\mathrm{R}}_b,\hfill \\ \hfill {\displaystyle \frac{d{\mathrm{S}}_h}{dt}}& =-{\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-H_i\left(t\right){\mathrm{S}}_h,\hfill \\ \hfill {\displaystyle \frac{d{\mathrm{I}}_h}{dt}}& ={\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-{\gamma }_h{\mathrm{I}}_h-H_i\left(t\right){\mathrm{I}}_h,\hfill \\ \hfill {\displaystyle \frac{d{\mathrm{R}}_h}{dt}}& ={\gamma }_h{\mathrm{I}}_h.\hfill \end{array}$$

The variables and parameters used in the baboon-human disease framework are defined as follows: $S_b$, $I_b$, and $R_b$ represent the susceptible, infected, and recovered populations of baboons, respectively. Likewise, $S_h$, $I_h$, and $R_h$ denote the corresponding human populations. The key parameters include r, the natural growth rate of the baboon population; K, the carrying capacity based on the availability of resources; ${\beta }_b$, the disease transmission rate within the baboon population; ${\beta }_h$, the transmission rate from baboons to humans; ${\gamma }_b$, the recovery rate of infected baboons; and ${\gamma }_h$, the recovery rate of infected humans.

Additionally, $H_s\left(t\right)$, $H_f\left(t\right)$, and $H_i\left(t\right)$ represent control interventions targeting the baboon population, specifically sterilization measures, food restriction, and reduction in human contact, respectively. These definitions form the foundation for understanding the dynamics of disease transmission between baboons and humans within the model.

The fractional-order formulation of the model, for $0<\tau <1$, is given by:

$$\begin{array}{cc}\hfill {\mathcal{D}}^{\tau }{\mathrm{S}}_b\left(\mathtt{t}\right)& =r{\mathrm{S}}_b\left(1-{\displaystyle \frac{{\mathrm{S}}_b+{\mathrm{I}}_b+{\mathrm{R}}_b}{K}}\right)-{\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-{\mathrm{H}}_s\left(\mathtt{t}\right){\mathrm{S}}_b-{\mathrm{H}}_f\left(\mathtt{t}\right){\mathrm{S}}_b,\hfill \\ \hfill {\mathcal{D}}^{\tau }{\mathrm{I}}_b\left(\mathtt{t}\right)& ={\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-{\gamma }_b{\mathrm{I}}_b-{\mathrm{H}}_s\left(\mathtt{t}\right){\mathrm{I}}_b-{\mathrm{H}}_f\left(\mathtt{t}\right){\mathrm{I}}_b,\hfill \\ \hfill {\mathcal{D}}^{\tau }{\mathrm{R}}_b\left(\mathtt{t}\right)& ={\gamma }_b{\mathrm{I}}_b-{\mathrm{H}}_f\left(\mathtt{t}\right){\mathrm{R}}_b,\hfill \\ \hfill {\mathcal{D}}^{\tau }{\mathrm{S}}_h\left(\mathtt{t}\right)& =-{\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-{\mathrm{H}}_i\left(\mathtt{t}\right){\mathrm{S}}_h,\hfill \\ \hfill {\mathcal{D}}^{\tau }{\mathrm{I}}_h\left(\mathtt{t}\right)& ={\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-{\gamma }_h{\mathrm{I}}_h-{\mathrm{H}}_i\left(\mathtt{t}\right){\mathrm{I}}_h,\hfill \\ \hfill {\mathcal{D}}^{\tau }{\mathrm{R}}_h\left(\mathtt{t}\right)& ={\gamma }_h{\mathrm{I}}_h.\hfill \end{array}$$

(1)

The parameters in the fractional-order model were determined through empirical data, assumptions, and numerical calibration. Epidemiological data from the Al-Baha region informed estimates for key parameters such as the transmission rate from baboons to humans (${\beta }_h$) and the human recovery rate (${\gamma }_h$), while control parameters ($H_s,H_f,H_i$) were based on plausible intervention strategies and validated through sensitivity analysis. Numerical simulations ensured the model outputs aligned with observed disease dynamics.

3. Foundational Concepts

This section presents key definitions and some fundamental properties of fractional calculus. Let ${\mathbb{R}}_{+}$ denote the set of non-negative real numbers. For any fractional order $\tau \in {\mathbb{R}}_{+}$, the fractional integral $J_m^{\tau }f\left(t\right)$ of a function $f:{\mathbb{R}}_{+}\to \mathbb{R}$ is defined by

$$J_m^{\tau }f\left(t\right)={\displaystyle \frac{1}{\Gamma \left(\tau \right)}}{\int }_m^t{(t-\xi )}^{\tau -1}f\left(\xi \right)d\xi ,t\ge m,$$

where $\Gamma \left(z\right)={\int }_0^{\infty }{e}^{-u}{u}^{z-1}du$ is the Euler Gamma function.

Definition 1 

([68]). The Caputo fractional derivative of order $\tau >0$ for $f\left(t\right)$, where $n-1<\tau <n$ and $n\in \mathbb{N}$, is given by:

$${}_a{}^C\mathcal{D}^{\tau }f\left(t\right)={\displaystyle \frac{1}{\Gamma (n-\tau )}}{\int }_a^t{\displaystyle \frac{f^{\left(n\right)}\left(\zeta \right)}{{(t-\zeta )}^{\tau +1-n}}}d\zeta ,t\ge a.$$

Consider the following fractional-order initial value problem:

$$\left\{\begin{array}{c}{\mathcal{D}}^{\tau }Z\left(t\right)=h\left(Z\left(t\right)\right),\hfill \\ Z\left(0\right)=Z_0,\hfill \end{array}\right.$$

(2)

where ${\mathcal{D}}^{\tau }$ denotes the Caputo fractional derivative, and $h\left(Z\left(t\right)\right):\mathbb{R}\times {\mathbb{R}}^2\to {\mathbb{R}}^2$ is a vector function. Let ${\mathbb{R}}^2$ be a complete metric space under an appropriate norm $\parallel ·\parallel$, and define:

$$\begin{array}{c}\mathcal{J}=[t_0-r,t_0+r],\mathcal{D}=\{Z\in {\mathbb{R}}^2:\parallel Z-Z_0\parallel \le c\},\\ \mathcal{S}=\{(t,Z)\in \mathbb{R}\times {\mathbb{R}}^2:t\in \mathcal{J},Z\in \mathcal{D}\}.\end{array}$$

Lemma 1

([69]). Let $h:\mathcal{S}\to {\mathbb{R}}^2$ be a function satisfying the following conditions:

(1) 

$h\left(Z\right(t\left)\right)$ is Lebesgue measurable in t on $\mathcal{J}$,

(2) 

$h\left(Z\right(t\left)\right)$ is continuous in Z on $\mathcal{D}$,

(3) 

There exists a function $k\left(t\right)\in L^2\left(\mathcal{J}\right)$ such that:

$$\parallel h\left(Z\right(t\left)\right)\parallel \le k\left(t\right),$$

for almost every $t\in \mathcal{J}$ and all $Z\in \mathcal{D}$,

(4) 

There is a function $\mu \left(t\right)\in L^4\left(\mathcal{J}\right)$ such that:

$$\parallel h\left(Z_1\left(t\right)\right)-h\left(Z_2\left(t\right)\right)\parallel \le \mu \left(t\right)\parallel Z_1\left(t\right)-Z_2\left(t\right)\parallel ,$$

for almost every $t\in \mathcal{J}$ and any $Z_1,Z_2\in \mathcal{D}$.

Then, the initial value problem (2) has a unique solution on $[t_0-ϵ,t_0+ϵ]$ for some $ϵ>0$.

Lemma 2 

([70]). Assume that $h:{\mathbb{R}}_{+}\times {\mathbb{R}}^2\to {\mathbb{R}}^2$ satisfies the following criteria:

(1) 

$h\left(Z\right(t\left)\right)$ is Lebesgue measurable with respect to t on $\mathbb{R}$,

(2) 

$h\left(Z\right(t\left)\right)$ is continuous in Z on ${\mathbb{R}}^2$,

(3) 

The partial derivative ${\displaystyle \frac{\partial h\left(Z\right(t\left)\right)}{\partial Z}}$ is continuous on ${\mathbb{R}}^2$,

(4) 

There exist constants $\alpha >0$ and $\beta >0$ such that:

$$\parallel h\left(Z\right(t\left)\right)\parallel \le \alpha +\beta \parallel Z\parallel ,$$

for almost every $t\in \mathbb{R}$ and all $Z\in {\mathbb{R}}^2$.

Then, the initial value problem (2) has a unique solution $Z\left(t\right)$ defined on $(-\infty ,+\infty )$.

Lemma 3 

([71,72]). Let $u\left(t\right)$ be a function for which ${\mathcal{D}}^{\tau }u\left(t\right)$ exists and satisfies the following inequality:

$$\left\{\begin{array}{c}{\mathcal{D}}^{\tau }u\left(t\right)\le -\eta u\left(t\right)+\kappa ,\hfill \\ u\left(t_0\right)=u_0,\hfill \end{array}\right.$$

where $0<\tau <1$, $(\eta ,\kappa )\in {\mathbb{R}}^2$, and $\eta \ne 0$. Then:

$$u\left(t\right)\le \left(u\left(t_0\right)-{\displaystyle \frac{\kappa }{\eta }}\right)E_{\tau ,1}\left[-\eta {(t-t_0)}^{\tau }\right]+{\displaystyle \frac{\kappa }{\eta }},$$

where $E_{\tau ,1}\left(t\right)={\sum }_{k=0}^{\infty }{\displaystyle \frac{t^k}{\Gamma (k\tau +1)}}$ denotes the Mittag-Leffler function.

4. Existence, Uniqueness, and Positivity of Solution

Let us consider the domain of interest as follows:

$$\begin{array}{cc}\hfill \mathrm{\Psi }& =\{(S_b,I_b,R_b,S_h,I_h,R_h)\in {\mathbb{R}}_{+}^6:S_b\ge 0,I_b\ge 0,R_b\ge 0,S_h\ge 0,I_h\ge 0,R_h\ge 0,\hfill \\ & \mathrm{and}max\left(|S_b|,|I_b|,|R_b|,|S_h|,|I_h|,|R_h|\right)\le M\}.\hfill \end{array}$$

In this region, the system possesses a unique, non-negative, and bounded solution.

Theorem 1.

Given any initial state $Y_0=(S_b\left(0\right),I_b\left(0\right),R_b\left(0\right),S_h\left(0\right),I_h\left(0\right),R_h\left(0\right))\in \mathrm{\Psi }$, the system’s solution $Y=(S_b\left(t\right),I_b\left(t\right),R_b\left(t\right),S_h\left(t\right),I_h\left(t\right),R_h\left(t\right))$ remains in Ψ for all $t\ge 0$ and is unique.

Proof. 

Define the vector-valued function $G\left(Y\right)=(G_1\left(Y\right),G_2\left(Y\right),G_3\left(Y\right),G_4\left(Y\right),G_5\left(Y\right),G_6\left(Y\right))$, where each component is expressed as:

$$\begin{array}{cc}& G_1\left(Y\right)=r{S}_b\left(1-{\displaystyle \frac{S_b+I_b+R_b}{K}}\right)-{\beta }_b{S}_b{I}_b-P_s\left(t\right)S_b-P_f\left(t\right)S_b,\hfill \\ & G_2\left(Y\right)={\beta }_b{S}_b{I}_b-{\gamma }_b{I}_b-P_s\left(t\right)I_b-P_f\left(t\right)I_b,\hfill \\ & G_3\left(Y\right)={\gamma }_b{I}_b-P_f\left(t\right)R_b,\hfill \\ & G_4\left(Y\right)=-{\beta }_h{S}_h{I}_b-P_i\left(t\right)S_h,\hfill \\ & G_5\left(Y\right)={\beta }_h{S}_h{I}_b-{\gamma }_h{I}_h-P_i\left(t\right)I_h,\hfill \\ & G_6\left(Y\right)={\gamma }_h{I}_h.\hfill \end{array}$$

Now, for any $Y,\overline{Y}\in \mathrm{\Psi }$, we have:

$$\begin{array}{cc}\hfill \parallel G\left(\overline{Y}\right)-G\left(Y\right)\parallel & =|G_1\left(\overline{Y}\right)-G_1\left(Y\right)|+|G_2\left(\overline{Y}\right)-G_2\left(Y\right)|+|G_3\left(\overline{Y}\right)-G_3\left(Y\right)|\hfill \\ & +|G_4\left(\overline{Y}\right)-G_4\left(Y\right)|+|G_5\left(\overline{Y}\right)-G_5\left(Y\right)|+|G_6\left(\overline{Y}\right)-G_6\left(Y\right)|\hfill \\ & \le (r+{\beta }_{b}K+P_s\left(t\right)+P_f\left(t\right))|\overline{S_b}-S_b|+({\beta }_{b}K+{\gamma }_b+P_s\left(t\right)+P_f\left(t\right))|\overline{I_b}-I_b|\hfill \\ & +({\gamma }_b+P_f\left(t\right))|\overline{R_b}-R_b|+({\beta }_{h}K+P_i\left(t\right))|\overline{S_h}-S_h|\hfill \\ & +({\beta }_{h}K+{\gamma }_h+P_i\left(t\right))|\overline{I_h}-I_h|+{\gamma }_h|\overline{R_h}-R_h|\hfill \\ & \le \Lambda |\overline{Y}-Y|,\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill \Lambda & =max\{r+{\beta }_{b}K+P_s\left(t\right)+P_f\left(t\right),{\beta }_{b}K+{\gamma }_b+P_s\left(t\right)+P_f\left(t\right),{\gamma }_b+P_f\left(t\right),{\beta }_{h}K+P_i\left(t\right),\hfill \\ & {\beta }_{h}K+{\gamma }_h+P_i\left(t\right),{\gamma }_h\}.\hfill \end{array}$$

Since $G\left(Y\right)$ adheres to the Lipschitz condition, it follows that the solution to the given system exists and is unique. □

Theorem 2.

If the initial values satisfy $S_b\left(0\right)\ge 0$, $I_b\left(0\right)\ge 0$, $R_b\left(0\right)\ge 0$, $S_h\left(0\right)\ge 0$, $I_h\left(0\right)\ge 0$, and $R_h\left(0\right)\ge 0$, then the solution of system (1) remains non-negative for all $t\ge 0$.

Proof. 

To demonstrate this, we evaluate the right-hand side of the system at the respective boundaries:

$$\begin{array}{cc}& {\mathcal{D}}^{\tau }{S}_b\left(t\right){|}_{S_b=0}=0,\hfill \\ & {\mathcal{D}}^{\tau }{I}_b\left(t\right){|}_{I_b=0}=0,\hfill \\ & {\mathcal{D}}^{\tau }{R}_b\left(t\right){|}_{R_b=0}={\gamma }_b{I}_b\ge 0,\hfill \\ & {\mathcal{D}}^{\tau }{S}_h\left(t\right){|}_{S_h=0}=0,\hfill \\ & {\mathcal{D}}^{\tau }{I}_h\left(t\right){|}_{I_h=0}={\beta }_h{S}_h{I}_b\ge 0,\hfill \\ & {\mathcal{D}}^{\tau }{R}_h\left(t\right){|}_{R_h=0}={\gamma }_h{I}_h\ge 0.\hfill \end{array}$$

Since all the evaluated terms are either zero or non-negative at $S_b=0$, $I_b=0$, $R_b=0$, $S_h=0$, $I_h=0$, and $R_h=0$, none of the variables can drop below zero. Hence, the solution remains non-negative for all $t\ge 0$. By applying standard results on the positivity of differential equations, it follows that the non-negativity of the solution is preserved throughout the evolution of the system. □

Theorem 3.

The solutions of system (1) are uniformly bounded within the region

$$\Omega =\left\{(S_b,I_b,R_b,S_h,I_h,R_h)\in {\mathbb{R}}_{+}^6:0\le S_b+I_b+R_b+S_h+I_h+R_h\le {\displaystyle \frac{\alpha M}{\lambda }}\right\},$$

where the parameter λ is defined as $\lambda ={\displaystyle \frac{\alpha M}{L}}$.

Proof. 

Define a function representing the total population size at any time t:

$$P\left(t\right)=S_b\left(t\right)+I_b\left(t\right)+R_b\left(t\right)+S_h\left(t\right)+I_h\left(t\right)+R_h\left(t\right).$$

By adding all the equations in the system, we obtain:

$$\begin{array}{cc}\hfill {\mathcal{D}}^{\tau }P\left(t\right)& =\alpha S_b\left(1-{\displaystyle \frac{S_b+I_b+R_b}{L}}\right)-G_s\left(t\right)S_b-G_f\left(t\right)S_b\hfill \\ \hfill & -G_s\left(t\right)I_b-G_f\left(t\right)I_b-G_f\left(t\right)R_b-G_i\left(t\right)S_h-G_i\left(t\right)I_h.\hfill \end{array}$$

Since $G_s\left(t\right),G_f\left(t\right),G_i\left(t\right)\ge 0$, we can establish the following upper bound for ${\mathcal{D}}^{\tau }P\left(t\right)$:

$${\mathcal{D}}^{\tau }P\left(t\right)\le \alpha M-\lambda P\left(t\right).$$

Thus, we have:

$${\mathcal{D}}^{\tau }P\left(t\right)+\lambda P\left(t\right)\le \alpha M.$$

Following the approach outlined in [73], we obtain:

$$0\le P\left(t\right)\le P\left(0\right)E_{\nu }(-\lambda t^{\nu })+t^{\nu }{E}_{\nu ,\nu +1}(-\lambda t^{\nu }),$$

where $E_{\nu }$ represents the Mittag-Leffler function. Considering the asymptotic behavior of this function as derived in [74], we further conclude:

$$0\le P\left(t\right)\le {\displaystyle \frac{\alpha M}{\lambda }},\mathrm{as}t\to \infty .$$

Hence, if the initial conditions place the solutions within ${\Omega }^{+}$, they remain uniformly bounded inside the region $\Omega$ over time. □

Remark 1.

The set Ω is positively invariant under the dynamics of the system, meaning that any solution that starts in Ω will remain within this bounded region.

5. Equilibrium Points and Stability

At equilibrium, we solve for ${\mathrm{S}}_b$ and ${\mathrm{S}}_h$, with ${\mathrm{I}}_b={\mathrm{I}}_h=0$, leading to the infection-free equilibrium:

$$E_0=({\mathrm{S}}_b^0,0,0,{\mathrm{S}}_h^0,0,0),$$

where

$${\mathrm{S}}_b^0={\displaystyle \frac{Kr}{H_s+H_f}},{\mathrm{S}}_h^0={\displaystyle \frac{K}{H_i}}.$$

According to the framework presented in [75], the basic reproduction number ${\mathcal{R}}_0$ is expressed as

$${\mathcal{R}}_0={\displaystyle \frac{{\beta }_b{S}_b^0}{{\gamma }_b+H_s}}+{\displaystyle \frac{{\beta }_h{S}_h^0}{{\gamma }_h+H_i}}.$$

Lemma 4.

The equilibrium point $E_0=(S_b^0,0,0,S_h^0,0,0)$ is LAS within the domain Ω when ${\mathcal{R}}_0<1$, but it becomes unstable if ${\mathcal{R}}_0>1$.

Proof. 

At the disease-free equilibrium $E_0=(S_b^0,0,0,S_h^0,0,0)$, the Jacobian matrix associated with system (1) takes the form:

$$J\left(E_0\right)=\left[\begin{array}{cccccc}-r& 0& -{\beta }_b{S}_b^0& 0& 0& 0\\ 0& -H_i& 0& -{\beta }_h{S}_h^0& 0& 0\\ 0& 0& {\beta }_b{S}_b^0-({\gamma }_b+H_s)& 0& 0& 0\\ 0& 0& 0& {\beta }_h{S}_h^0-({\gamma }_h+H_i)& 0& 0\\ 0& 0& 0& 0& -H_f& 0\\ 0& 0& 0& 0& 0& -H_f\end{array}\right].$$

The characteristic equation for this matrix can be derived as follows:

$$(\sigma +r)(\sigma +H_i)(\sigma -{\beta }_b{\mathrm{S}}_b^0+{\gamma }_b+H_s)(\sigma -{\beta }_h{\mathrm{S}}_h^0+{\gamma }_h+H_i)=0.$$

Thus, the eigenvalues are

$${\sigma }_1=-r,{\sigma }_2=-H_i,{\sigma }_3={\beta }_b{\mathrm{S}}_b^0-({\gamma }_b+H_s),{\sigma }_4={\beta }_h{\mathrm{S}}_h^0-({\gamma }_h+H_i).$$

For stability, all eigenvalues must have negative real parts. ${\sigma }_1$ and ${\sigma }_2$ are always negative. The eigenvalues ${\sigma }_3$ and ${\sigma }_4$ depend on the basic reproduction number ${\mathcal{R}}_0$:

$${\mathcal{R}}_0={\displaystyle \frac{{\beta }_b{\mathrm{S}}_b^0}{{\gamma }_b+H_s}}+{\displaystyle \frac{{\beta }_h{\mathrm{S}}_h^0}{{\gamma }_h+H_i}}.$$

If ${\mathcal{R}}_0<1$, then ${\sigma }_3<0$ and ${\sigma }_4<0$, and $E_0$ is LAS. If ${\mathcal{R}}_0>1$, then ${\sigma }_3>0$ or ${\sigma }_4>0$, and $E_0$ is unstable. □

Theorem 4.

The equilibrium point $E_0=(S_b^0,0,0,S_h^0,0,0)$ is GAS.

Proof. 

Let us define a LF that is positive definite as follows:

$$\begin{array}{c}{L}_1(S_b,I_b,R_b,S_h,I_h,R_h)=(S_b-S_b^0-S_b^0\ln {\displaystyle \frac{S_b}{S_b^0}})+(S_h-S_h^0-S_h^0\ln {\displaystyle \frac{S_h}{S_h^0}}).\end{array}$$

From Lemma 4, one obtains

$$\begin{array}{c}\begin{array}{cc}\hfill {\mathcal{D}}^{\tau }{L}_1& =\left({\displaystyle \frac{S_b-S_b^0}{S_b}}\right){\mathcal{D}}^{\tau }{S}_b+\left({\displaystyle \frac{S_h-S_h^0}{S_h}}\right){\mathcal{D}}^{\tau }{S}_h\hfill \\ & =\left({\displaystyle \frac{S_b-S_b^0}{S_b}}\right)\left(r{\mathrm{S}}_b\left(1-{\displaystyle \frac{{\mathrm{S}}_b+{\mathrm{I}}_b+{\mathrm{R}}_b}{K}}\right)-{\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-H_s\left(t\right){\mathrm{S}}_b-H_f\left(t\right){\mathrm{S}}_b\right)\hfill \\ & +\left({\displaystyle \frac{S_h-S_h^0}{S_h}}\right)\left(-{\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-H_i\left(t\right){\mathrm{S}}_h\right)\hfill \\ & =\left(S_b-S_b^0\right)\left(r\left(1-{\displaystyle \frac{{\mathrm{S}}_b+{\mathrm{I}}_b+{\mathrm{R}}_b}{K}}\right)-{\beta }_b{\mathrm{I}}_b-H_s\left(t\right)-H_f\left(t\right)\right)\hfill \\ & +\left(S_h-S_h^0\right)\left(-{\beta }_h{\mathrm{I}}_b-H_i\left(t\right)\right)\hfill \\ & =-{\left(S_b-S_b^0\right)}^2.\hfill \end{array}\end{array}$$

Hence, it follows that ${\mathcal{D}}^{\tau }{L}_1(S_b,I_b,R_b,S_h,I_h,R_h)<0$ holds for all $(S_b,I_b,R_b,S_h,I_h,R_h)\in \Omega$. Additionally, the condition ${\mathcal{D}}^{\tau }{L}_1(S_b,I_b,R_b,S_h,I_h,R_h)=0$ leads to $S_b=S_b^0,I_b=I_b^0,R_b=R_b^0,S_h=S_h^0,I_h=I_h^0,R_h=R_h^0$. Thus, the equilibrium point $E^0$ forms the unique invariant set such that

$${\mathcal{D}}^{\tau }{L}_2(S_b,I_b,R_b,S_h,I_h,R_h)=0.$$

Applying the fractional LaSalle Invariance Principle [76,77] and utilizing the result from Lemma 3, the equilibrium $E^0$ is GAS within the domain $\Omega$. □

For $I^{\ast }>0$, $E^{\ast }=(S^{\ast },I_b^{\ast },R_b^{\ast },S_h^{\ast },I_h^{\ast },R_h^{\ast })$ represents the endemic equilibrium, corresponding to the steady-state solution of the given model. Using the steady-state conditions:

$${\mathcal{D}}^{\tau }{S}_b=0,{\mathcal{D}}^{\tau }{I}_b=0,{\mathcal{D}}^{\tau }{R}_b=0,{\mathcal{D}}^{\tau }{S}_h=0,{\mathcal{D}}^{\tau }{I}_h=0,{\mathcal{D}}^{\tau }{R}_h=0,$$

and substituting from the second and third equations, we express $I_b^{\ast }$ and $R_b^{\ast }$:

$$R_b^{\ast }={\displaystyle \frac{{\gamma }_b}{H_f}}{I}_b^{\ast }.$$

By inserting this result into the fourth equation, we find:

$$I_h^{\ast }={\displaystyle \frac{{\beta }_h{S}_h^{\ast }}{{\gamma }_h+H_i}}{I}_b^{\ast }.$$

Now, substituting this into the first equation, we solve for $S_b^{\ast }$:

$$S_b^{\ast }={\displaystyle \frac{Kr}{H_s}}+{\displaystyle \frac{{\beta }_b{a}_2}{H_s}}{I}_b^{\ast }.$$

Thus, the endemic equilibrium can be expressed as:

$$E^{\ast }=(S_b^{\ast },I_b^{\ast },R_b^{\ast },S_h^{\ast },I_h^{\ast },R_h^{\ast }).$$

Lemma 5.

If ${\mathcal{R}}_0>1$, $E^{\ast }$ is LAS in Ω.

Proof. 

For the first-order integer model, the Jacobian matrix $J\left(E^{\ast }\right)$ at $E^{\ast }$ is given by

$$J\left(E^{\ast }\right)=\left[\begin{array}{cccccc}-r& 0& -{\beta }_b{S}_b^{\ast }& 0& 0& 0\\ 0& -H_i& 0& -{\beta }_h{S}_h^{\ast }& 0& 0\\ 0& 0& {\gamma }_b-H_f& 0& 0& 0\\ 0& 0& 0& {\gamma }_h-H_i& 0& 0\\ 0& 0& 0& 0& -H_f& 0\\ 0& 0& 0& 0& 0& -H_f\end{array}\right].$$

Evaluating the Jacobian matrix at $E^{\ast }$ gives the characteristic equation

$$P\left(\xi \right)=det\left(\xi I-J\left(E^{\ast }\right)\right)=0,$$

where I is the identity matrix. The characteristic polynomial is

$$P\left(\xi \right)={\xi }^6+a_1{\xi }^5+a_2{\xi }^4+a_3{\xi }^3+a_4{\xi }^2+a_5\xi +a_6,$$

where the coefficients $a_1,a_2,\dots ,a_6$ are defined in terms of the parameters of the model.

If the Routh–Hurwitz criterion is satisfied, the endemic equilibrium is locally stable:

$$a_1>0,a_2>0,a_3>0,a_4>0,a_5>0,a_6>0.$$

Thus, $E^{\ast }$ is LAS when ${\mathcal{R}}_0>1$, as all conditions of the Routh–Hurwitz criterion are satisfied. □

Theorem 5.

The positive endemic equilibrium point $E^{\ast }=(S_b^{\ast },I_b^{\ast },R_b^{\ast },S_h^{\ast },I_h^{\ast },R_h^{\ast })$ of the model is GAS.

Proof. 

Consider this positive definite LF:

$$\begin{array}{c}{L}_2(S_b,I_b,R_b,S_h,I_h,R_h)=(S_b-S_b^{\ast }-S_b^{\ast }\ln {\displaystyle \frac{S_b}{S_b^{\ast }}})+(I_b-I_b^{\ast }-I_b^{\ast }\ln {\displaystyle \frac{I_b}{I_b^{\ast }}})+(R_b-R_b^{\ast }-R_b^{\ast }\ln {\displaystyle \frac{R_b}{R_b^{\ast }}})\\ +(S_h-S_h^{\ast }-S_h^{\ast }\ln {\displaystyle \frac{S_h}{S_h^{\ast }}})+(I_h-I_h^{\ast }-I_h^{\ast }\ln {\displaystyle \frac{I_h}{I_h^{\ast }}})+(R_h-R_h^{\ast }-R_h^{\ast }\ln {\displaystyle \frac{R_h}{R_h^{\ast }}}).\end{array}$$

From Lemma 4, one obtains

$$\begin{array}{c}\begin{array}{cc}\hfill {\mathcal{D}}^{\tau }{L}_2& =\left({\displaystyle \frac{S_b-S_b^{\ast }}{S_b}}\right){\mathcal{D}}^{\tau }{S}_b+\left({\displaystyle \frac{I_b-I_b^{\ast }}{I_b}}\right){\mathcal{D}}^{\tau }{I}_b+\left({\displaystyle \frac{R_b-R_b^{\ast }}{R_b}}\right){\mathcal{D}}^{\tau }{R}_b\hfill \\ & +\left({\displaystyle \frac{S_h-S_h^{\ast }}{S_h}}\right){\mathcal{D}}^{\tau }{S}_h+\left({\displaystyle \frac{I_h-I_h^{\ast }}{I_h}}\right){\mathcal{D}}^{\tau }{I}_h+\left({\displaystyle \frac{R_h-R_h^{\ast }}{R_h}}\right){\mathcal{D}}^{\tau }{R}_h\hfill \\ & =\left({\displaystyle \frac{S_b-S_b^{\ast }}{S_b}}\right)\left(r{\mathrm{S}}_b\left(1-{\displaystyle \frac{{\mathrm{S}}_b+{\mathrm{I}}_b+{\mathrm{R}}_b}{K}}\right)-{\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-H_s\left(t\right){\mathrm{S}}_b-H_f\left(t\right){\mathrm{S}}_b\right)\hfill \\ & +\left({\displaystyle \frac{I_b-I_b^{\ast }}{I_b}}\right)\left({\beta }_b{\mathrm{S}}_b{\mathrm{I}}_b-{\gamma }_b{\mathrm{I}}_b-H_s\left(t\right){\mathrm{I}}_b-H_f\left(t\right){\mathrm{I}}_b\right)+\left({\displaystyle \frac{R_b-R_b^{\ast }}{R_b}}\right)\left({\gamma }_b{\mathrm{I}}_b-H_f\left(t\right){\mathrm{R}}_b\right)\hfill \\ & +\left({\displaystyle \frac{S_h-S_h^{\ast }}{S_h}}\right)\left(-{\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-H_i\left(t\right){\mathrm{S}}_h\right)+\left({\displaystyle \frac{I_h-I_h^{\ast }}{I_h}}\right)\left({\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b-{\gamma }_h{\mathrm{I}}_h-H_i\left(t\right){\mathrm{I}}_h\right)\hfill \\ & +\left({\displaystyle \frac{R_h-R_h^{\ast }}{R_h}}\right)\left({\gamma }_h{\mathrm{I}}_h\right)\hfill \\ & =\left(S_b-S_b^{\ast }\right)\left(r\left(1-{\displaystyle \frac{{\mathrm{S}}_b+{\mathrm{I}}_b+{\mathrm{R}}_b}{K}}\right)-{\beta }_b{\mathrm{I}}_b-H_s\left(t\right)-H_f\left(t\right)\right)\hfill \\ & +\left(I_b-I_b^{\ast }\right)\left({\beta }_b{\mathrm{S}}_b-{\gamma }_b-H_s\left(t\right)-H_f\left(t\right)\right)+\left(R_b-R_b^{\ast }\right)\left({\displaystyle \frac{{\gamma }_b{\mathrm{I}}_b}{R_b}}-H_f\left(t\right)\right)\hfill \\ & +\left(S_h-S_h^{\ast }\right)\left(-{\beta }_h{\mathrm{I}}_b-H_i\left(t\right)\right)+\left(I_h-I_h^{\ast }\right)\left({\displaystyle \frac{{\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b}{I_h}}-{\gamma }_h-H_i\left(t\right)\right)+\left(R_h-R_h^{\ast }\right)\left({\displaystyle \frac{{\gamma }_h{\mathrm{I}}_h}{R_h}}\right)\hfill \\ & =-{\left(S_b-S_b^{\ast }\right)}^2-{\left(R_b-R_b^{\ast }\right)}^2\left({\displaystyle \frac{{\gamma }_b{\mathrm{I}}_b}{R_b{R}_b^{\ast }}}\right)-\left(I_h-I_h^{\ast }\right)\left({\displaystyle \frac{{\beta }_h{\mathrm{S}}_h{\mathrm{I}}_b}{I_h{I}_h^{\ast }}}\right)-{\left(R_h-R_h^{\ast }\right)}^2\left({\displaystyle \frac{{\gamma }_h{\mathrm{I}}_h}{R_h{R}_h^{\ast }}}\right)\hfill \end{array}\end{array}$$

Thus, ${\mathcal{D}}^{\tau }{L}_2(S_b,I_b,R_b,S_h,I_h,R_h)<0$ for all $(S_b,I_b,R_b,S_h,I_h,R_h)\in \Omega$. Furthermore, ${\mathcal{D}}^{\tau }{L}_2(S_b,I_b,R_b,S_h,I_h,R_h)=0$ implies that $S_b=S_b^{\ast }$, $I_b=I_b^{\ast }$, $R_b=R_b^{\ast }$, $S_h=S_h^{\ast }$, $I_h=I_h^{\ast }$, and $R_h=R_h^{\ast }$. Therefore, the singleton $\left\{E^{\ast }\right\}$ is the only invariant set such that ${\mathcal{D}}^{\tau }{L}_2(S_b,I_b,R_b,S_h,I_h,R_h)=0$.

Applying the fractional LaSalle Invariance Principle and using Lemma 3, $E^{\ast }$ is GAS on $\Omega$. □

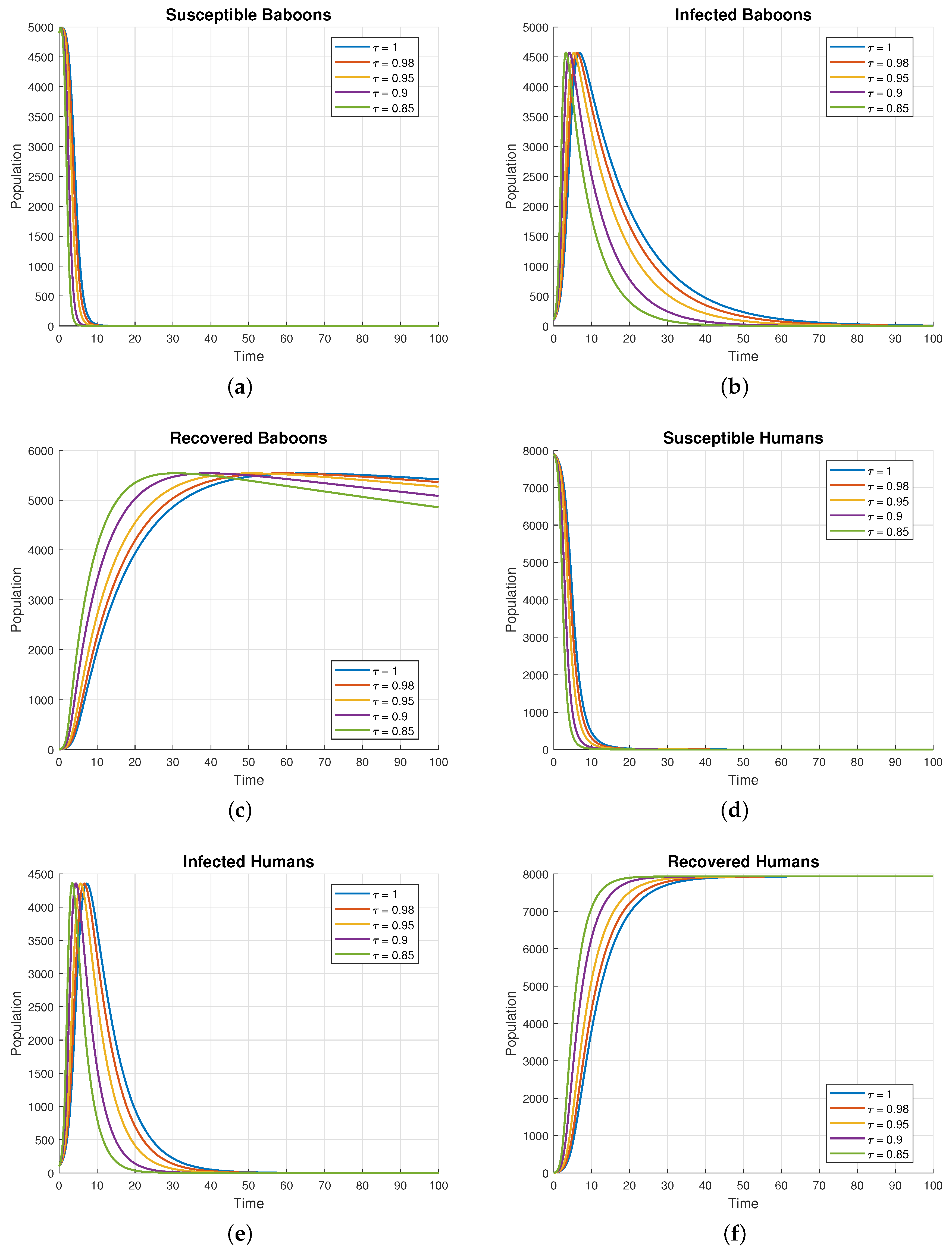
6. Utilization of the Extended Euler Approach

Based on a framework inspired by fractional numerical schemes, we implement a generalized Euler technique to approximate the solutions of the proposed system. Let us examine the fractional-order differential equation:

$${\mathcal{D}}^{\tau }x=g(t,x),t\in [0,T],0<\tau \le 1.$$

The interval $[0,b]$ is partitioned into k segments of equal width $h=b/k$, with discretization points $t_j=jh$ for $j=0,1,\dots ,k$. Utilizing the fractional Taylor series expansion and assuming that x, ${\mathcal{D}}^{\tau }x$, and ${\mathcal{D}}^{2\tau }x$ are continuous on $[0,b]$, we can write:

$$x\left(t\right)=x\left(t_0\right)+\left({\mathcal{D}}^{\tau }x\left(t\right)\right)\left(t_0\right){\displaystyle \frac{t^{\tau }}{\Gamma (\tau +1)}}+\left({\mathcal{D}}^{2\tau }x\left(t\right)\right)\left(a_1\right){\displaystyle \frac{t^{2\tau }}{\Gamma (2\tau +1)}}.$$

By substituting $\left({\mathcal{D}}^{\tau }x\right)\left(t_0\right)=g(t_0,x\left(t_0\right))$ and ignoring the second-order term for sufficiently small h, we derive:

$$x\left(t_1\right)=x\left(t_0\right)+g(t_0,x\left(t_0\right)){\displaystyle \frac{h^{\tau }}{\Gamma (\tau +1)}}+\left({\mathcal{D}}^{2\tau }x\left(t\right)\right)\left(a_1\right){\displaystyle \frac{h^{2\tau }}{\Gamma (2\tau +1)}}.$$

For small step size h, the term involving $h^{2\tau }$ becomes negligible, yielding:

$$x\left(t_1\right)=x\left(t_0\right)+{\displaystyle \frac{h^{\tau }}{\Gamma (\tau +1)}}g(t_0,x\left(t_0\right)).$$

By repeating this process, we generate a sequence of approximate solutions $x\left(t_j\right)$. For $t_{j+1}=t_j+h$, the generalized Euler formula becomes:

$$x\left(t_{j+1}\right)=x\left(t_j\right)+{\displaystyle \frac{h^{\tau }}{\Gamma (\tau +1)}}g(t_j,x\left(t_j\right)),$$

(3)

for $j=0,1,\dots ,k-1$. Notably, when $\tau =1$, this method simplifies to the classical Euler scheme.

Applying this extended numerical approach (3) to the given system (1) leads to the following iterative expressions:

$$\begin{array}{cc}\hfill S_b^{(n+1)}& =S_b^{\left(n\right)}+m\left[r{S}_b^{\left(n\right)}\left(1-{\displaystyle \frac{S_b^{\left(n\right)}+I_b^{\left(n\right)}+R_b^{\left(n\right)}}{K}}\right)-{\beta }_b{S}_b^{\left(n\right)}{I}_b^{\left(n\right)}-H_s^{\left(n\right)}{S}_b^{\left(n\right)}-H_f^{\left(n\right)}{S}_b^{\left(n\right)}\right],\hfill \\ \hfill I_b^{(n+1)}& =I_b^{\left(n\right)}+m\left[{\beta }_b{S}_b^{\left(n\right)}{I}_b^{\left(n\right)}-{\gamma }_b{I}_b^{\left(n\right)}-H_s^{\left(n\right)}{I}_b^{\left(n\right)}-H_f^{\left(n\right)}{I}_b^{\left(n\right)}\right],\hfill \\ \hfill R_b^{(n+1)}& =R_b^{\left(n\right)}+m\left[{\gamma }_b{I}_b^{\left(n\right)}-H_f^{\left(n\right)}{R}_b^{\left(n\right)}\right],\hfill \\ \hfill S_h^{(n+1)}& =S_h^{\left(n\right)}+m\left[-{\beta }_h{S}_h^{\left(n\right)}{I}_b^{\left(n\right)}-H_i^{\left(n\right)}{S}_h^{\left(n\right)}\right],\hfill \\ \hfill I_h^{(n+1)}& =I_h^{\left(n\right)}+m\left[{\beta }_h{S}_h^{\left(n\right)}{I}_b^{\left(n\right)}-{\gamma }_h{I}_h^{\left(n\right)}-H_i^{\left(n\right)}{I}_h^{\left(n\right)}\right],\hfill \\ \hfill R_h^{(n+1)}& =R_h^{\left(n\right)}+m\left[{\gamma }_h{I}_h^{\left(n\right)}\right],\hfill \end{array}$$

where the parameter $0<m={\displaystyle \frac{h^{\tau }}{\Gamma (\tau +1)}}<1$ serves as the step size. The initial values are $S_b\left(0\right)=S_{b0}$, $I_b\left(0\right)=I_{b0}$, $R_b\left(0\right)=R_{b0}$, $S_h\left(0\right)=S_{h0}$, $I_h\left(0\right)=I_{h0}$, and $R_h\left(0\right)=R_{h0}$.

This extended numerical scheme offers an effective tool for approximating fractional dynamics while maintaining computational efficiency.

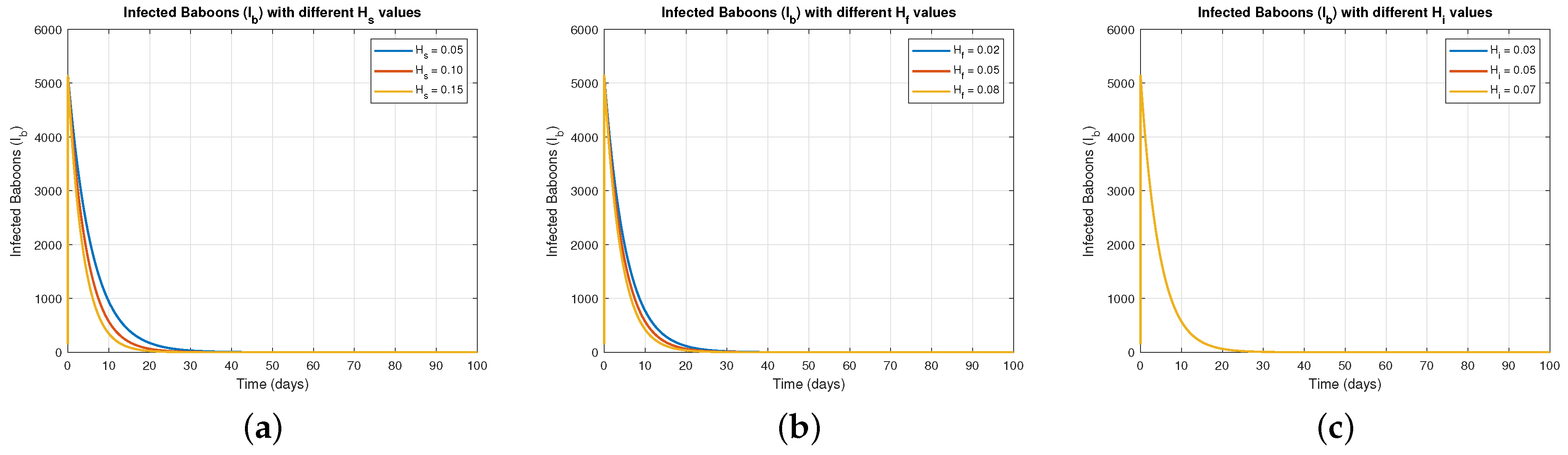
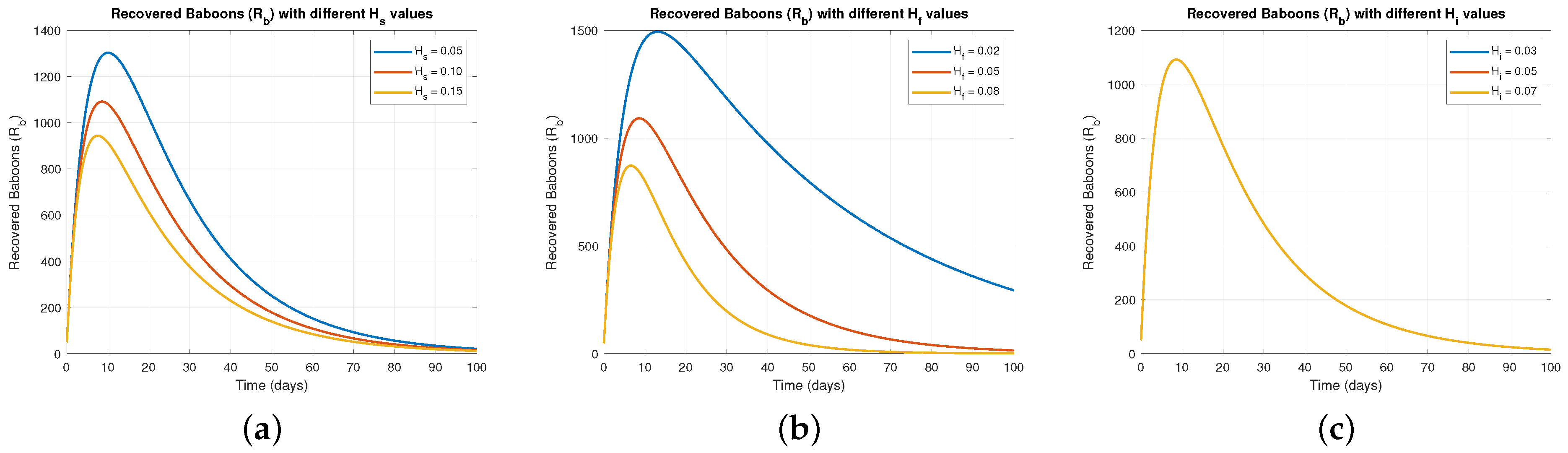
6.1. Numerical Experiments

This part presents the implementation of the Modified Euler Approach to numerically estimate the solutions of the fractional-order zoonotic disease framework. This numerical scheme is specifically advantageous for fractional differential systems due to its ability to maintain computational accuracy while capturing the memory-dependent effects intrinsic to fractional calculus. The simulations investigate how various control interventions—such as sterilizing baboons, restricting food availability, and minimizing human–baboon encounters—affect disease transmission patterns.

For the simulations, real-world data on zoonotic disease transmission between humans and baboons in Al-Baha were incorporated, as reported by the Ministry of Environment, Agriculture, and Water in Saudi Arabia’s Al-Baha region. The parameter values and their respective sources are summarized in

Table 1. Parameter values and initial conditions used in the zoonotic disease model [78].

Parameter	Value	Source
${\mathtt{k}}_b$	0.3	Estimated
${\mathtt{k}}_h$	0.1	Estimated
${\gamma }_b$	0.07	Estimated
${\gamma }_h$	0.15	Estimated
$H_s$	0.1	Assumed
$H_f$	0.05	Assumed
$H_i$	0.05	Assumed
r	0.05	Assumed
K	3000	Estimated
$\mu$	0.01	Estimated

, with initial population values set as follows: ${\mathtt{S}}_b\left(0\right)=5000$, ${\mathtt{I}}_b\left(0\right)=150$, ${\mathtt{R}}_b\left(0\right)=50$, ${\mathtt{S}}_h\left(0\right)=8000$, ${\mathtt{I}}_h\left(0\right)=100$, and ${\mathtt{R}}_h\left(0\right)=100$.

To analyze the dynamics of disease transmission, we conducted simulations under various control scenarios. The model parameters were selected based on empirical estimates from epidemiological studies in the Al-Baha region, where frequent human–baboon interactions contribute to zoonotic disease spread. The key findings from our simulations are summarized below:

• Baseline Dynamics: Without any control interventions, the infected baboon ($I_b$) and infected human ($I_h$) populations exhibited sustained growth, leading to persistent zoonotic disease transmission. The basic reproduction number ${\mathcal{R}}_0$ remained above 1, indicating an endemic situation.
• Effect of Sterilization ($H_s$): Increasing the sterilization rate of baboons significantly reduced the susceptible baboon population ($S_b$), thereby lowering the overall infection rate. However, sterilization alone was not sufficient to eliminate the disease entirely, as transmission still occurred through infected individuals.
• Effect of Food Access Restriction ($H_f$): Limiting food availability for baboons decreased human–baboon interactions, leading to a decline in transmission. The simulations showed that moderate values of $H_f$ contributed to a substantial reduction in both $I_b$ and $I_h$, making this an effective intervention strategy.
• Effect of Reducing Human–Baboon Interaction ($H_i$): Implementing measures such as public awareness campaigns and habitat modifications reduces direct contact between baboons and humans. The simulations revealed that increasing $H_i$ led to a significant decline in $I_h$, confirming that minimizing interaction is one of the most effective strategies for controlling zoonotic disease spread.
• Combined Control Strategies: The most effective disease mitigation was achieved when sterilization, food restriction, and interaction reduction were applied simultaneously. In such cases, the model predicted that ${\mathcal{R}}_0$ dropped below 1, leading to disease eradication over time.

The numerical simulations in this study explored the dynamics of zoonotic disease transmission between baboons and humans under various control scenarios. The simulations focused on how changes in control measures, represented by the parameters $H_s$, $H_f$, and $H_i$, impacted susceptible, infected, and recovered populations for both baboons and humans. These parameters correspond to sterilization, food access restriction, and interaction reduction controls, respectively. Below is an interpretation of each figure in the manuscript, followed by explanations of the trends and outcomes observed in each simulation.

Figure 1 examine how different levels of the control parameters $H_s$, $H_f$, and $H_i$ affect the infected baboon population. In Figure 1a shows that increasing $H_s$ gradually reduced the infected baboon population $I_b$, indicating that sterilization effectively limits disease spread. Figure 1b displays a similar trend for varying $H_f$, suggesting that restricting food access significantly decreases infection rates by reducing contact around food sources. Figure 1c illustrates that increased human interaction control ($H_i$) lowers infection levels in baboons, demonstrating the importance of minimizing contact between humans and baboons to prevent disease spillover.

Figure 2 explores the impact of different control intensities $H_s$, $H_f$, and $H_i$ on the infected human population ($I_h$). Figure 2a demonstrates that increasing $H_s$ exerts minimal direct influence on $I_h$, since sterilization primarily impacts baboons. Figure 2b indicates that food restriction ($H_f$) effectively reduces $I_h$, as limiting food availability curtails baboon-human encounters. Figure 2c highlights that increased $H_i$ values substantially lower $I_h$, underlining the critical role of minimizing human-baboon interactions in controlling zoonotic transmission.

Figure 3 focuses on how control measures $H_s$, $H_f$, and $H_i$ affect the recovered baboon population ($R_b$). Figure 3a shows that increasing $H_s$ results in a steady rise in $R_b$, as more baboons recover due to reduced transmission. Figure 3b,c depict similar recovery trends with higher values of $H_f$ and $H_i$, confirming that effective control measures reduce infection and enhance recovery rates.

Figure 4 focuses on how control measures $H_s$, $H_f$, and $H_i$ affect the recovered baboon population ($R_h$). Figure 4a shows that increasing $H_s$ results in a steady rise in $R_h$, as more baboons recover due to reduced transmission. Figure 4b,c depict similar recovery trends with higher values of $H_f$ and $H_i$, confirming that effective control measures reduce infection and enhance recovery rates.

Figure 5 illustrates the population dynamics of baboons in different compartments—$S_b\left(t\right)$, $I_b\left(t\right)$, $R_b\left(t\right)$, $S_h\left(t\right)$, $I_h\left(t\right)$, $R_h\left(t\right)$—under varying fractional-order $\tau$. The subfigures (a–f) within Figure 5 focus on how different initial control parameter values influence the overall population trajectories:

6.2. Error Estimation

In this section, we estimate the convergence rate of our six-component numerical scheme. Convergence rate is determined by

$$p={\displaystyle \frac{\log \left|\frac{u^{\frac{h}{2}}-u^h}{u^{\frac{h}{4}}-u^{\frac{h}{2}}}\right|}{\log 2}},$$

where $u^h=\{{\mathrm{S}}_b^h\left(t\right),{\mathrm{I}}_b^h\left(t\right),{\mathrm{R}}_b^h\left(t\right),{\mathrm{S}}_h^h\left(t\right),{\mathrm{I}}_h^h\left(t\right),{\mathrm{R}}_h^h\left(t\right)\}$, $u^{{\displaystyle \frac{h}{2}}}=\{{\mathrm{S}}_b^{{\displaystyle \frac{h}{2}}}\left(t\right),{\mathrm{I}}_b^{{\displaystyle \frac{h}{2}}}\left(t\right),{\mathrm{R}}_b^{{\displaystyle \frac{h}{2}}}\left(t\right),{\mathrm{S}}_h^{{\displaystyle \frac{h}{2}}}\left(t\right),{\mathrm{I}}_h^{{\displaystyle \frac{h}{2}}}\left(t\right),{\mathrm{R}}_h^{{\displaystyle \frac{h}{2}}}\left(t\right)\}$, and $u^{{\displaystyle \frac{h}{4}}}=\{{\mathrm{S}}_b^{{\displaystyle \frac{h}{4}}}\left(t\right),{\mathrm{I}}_b^{{\displaystyle \frac{h}{4}}}\left(t\right),{\mathrm{R}}_b^{{\displaystyle \frac{h}{4}}}\left(t\right),{\mathrm{S}}_h^{{\displaystyle \frac{h}{4}}}\left(t\right),{\mathrm{I}}_h^{{\displaystyle \frac{h}{4}}}\left(t\right),{\mathrm{R}}_h^{{\displaystyle \frac{h}{4}}}\left(t\right)\}$ are the approximate solutions for step sizes h, ${\displaystyle \frac{h}{2}}$, and ${\displaystyle \frac{h}{4}}$, respectively.

Table 2. The rate of convergence for different values of h for ${\mathrm{S}}_b\left(t\right)$ and ${\mathrm{S}}_h\left(t\right)$.

h	${\mathrm{S}}_{\mathit{b}} \left(\mathit{t}\right)$	$\mathit{p} \left({\mathrm{S}}_{\mathit{b}}\right)$	${\mathrm{S}}_{\mathit{h}} \left(\mathit{t}\right)$	$\mathit{p} \left({\mathrm{S}}_{\mathit{h}}\right)$
${\displaystyle \frac{1}{100}}$	−1.230587514160928	1.904959580936555	0.751972948680281	0.785531902054452
${\displaystyle \frac{1}{200}}$	−1.061110242113278	1.940190794821020	0.415109454355213	0.879128933122776
${\displaystyle \frac{1}{400}}$	−1.015855771334397	1.961017182073904	0.219682130799205	0.933763944099137
${\displaystyle \frac{1}{800}}$	−1.004063271904520	1.972167801697337	0.113429154201297	0.962477934907692
${\displaystyle \frac{1}{1600}}$	−1.001034400255673	1.977926785981624	0.057806699720829	0.977150215826183
${\displaystyle \frac{1}{3200}}$	−1.000262432420641	1.980853052009642	0.029262661073358	0.984573990764699
${\displaystyle \frac{1}{6400}}$	−1.000066464983257		0.014762798186914
${\displaystyle \frac{1}{12800}}$	−1.000016818587168		0.007434930967961

,

Table 3. The rate of convergence for different values of h for ${\mathrm{I}}_b\left(t\right)$ and ${\mathrm{I}}_h\left(t\right)$.

h	${\mathrm{I}}_{\mathit{b}} \left(\mathit{t}\right)$	$\mathit{p} \left({\mathrm{I}}_{\mathit{b}}\right)$	${\mathrm{I}}_{\mathit{h}} \left(\mathit{t}\right)$	$\mathit{p} \left({\mathrm{I}}_{\mathit{h}}\right)$
${\displaystyle \frac{1}{100}}$	−2.326508213491328	1.067858535219673	2.063882999678037	3.578639814091108
${\displaystyle \frac{1}{200}}$	−1.647800735475855	1.006794709692697	1.992390832959806	0.463370460207230
${\displaystyle \frac{1}{400}}$	−1.324039249963567	0.992944397031469	1.986406985255091	0.118777817473332
${\displaystyle \frac{1}{800}}$	−1.162919129852769	0.990829292631269	1.990747009184933	0.699165428640857
${\displaystyle \frac{1}{1600}}$	−1.081964120101717	0.991036225398347	1.994744030260617	0.865555010973675
${\displaystyle \frac{1}{3200}}$	−1.041228494373794	0.991461358994093	1.997205909082042	0.932675206008964
${\displaystyle \frac{1}{6400}}$	−1.020733737952112	0.991752429070755	1.998557074903865
${\displaystyle \frac{1}{12800}}$	−1.010425530442698		1.999264931751799

and

Table 4. The rate of convergence for different values of h for ${\mathrm{R}}_b\left(t\right)$ and ${\mathrm{R}}_h\left(t\right)$.

h	${\mathrm{R}}_{\mathit{b}} \left(\mathit{t}\right)$	$\mathit{p} \left({\mathrm{R}}_{\mathit{b}}\right)$	${\mathrm{R}}_{\mathit{h}} \left(\mathit{t}\right)$	$\mathit{p} \left({\mathrm{R}}_{\mathit{h}}\right)$
${\displaystyle \frac{1}{100}}$	−0.810858602235998	1.134148250045712	17.710724336596417	0.919794628750672
${\displaystyle \frac{1}{200}}$	−0.910831556058634	1.051723457397754	18.810610778750505	0.955734196405311
${\displaystyle \frac{1}{400}}$	−0.956379615764291	1.020129659296684	19.391993440099736	0.973775158693165
${\displaystyle \frac{1}{800}}$	−0.978351614908976	1.005880122139417	19.691742213503858	0.982881681370900
${\displaystyle \frac{1}{1600}}$	−0.989185393271114	0.998981951425179	19.844365880125473	0.987471151984859
${\displaystyle \frac{1}{3200}}$	−0.994580249280774	0.995555981083686	19.921588585883410	0.989778019827995
${\displaystyle \frac{1}{6400}}$	−0.997279581417658		19.960536712925116
${\displaystyle \frac{1}{12800}}$	−0.998633411352176		19.980149246718597

indicate the rate of convergence, typically approaching first-order and second-order accuracy. However, chaotic systems, like the one studied, are highly sensitive to initial conditions, so these results may exhibit variations due to the nature of the system.

7. Discussion

This study presents a fractional-order mathematical model to analyze zoonotic disease transmission between baboons and humans, incorporating control measures such as sterilization, food access restriction, and reduced human–baboon interactions. The mathematical model developed in this study highlights the complex interactions between baboon and human populations, concerning zoonotic disease transmission. The inclusion of fractional-order derivatives allows the model to account for memory and hereditary properties inherent in biological systems, providing a more accurate representation of disease dynamics compared to traditional models. The stability analysis of the infection-free and endemic equilibrium points confirmed that the basic reproduction number ${\mathcal{R}}_0$ is a critical determinant of disease persistence or eradication. A basic reproduction number less than one indicates that the disease will eventually be eradicated, while a value greater than one suggests the disease may persist. The sensitivity analysis identified critical parameters, such as the transmission rates between baboons and humans and the recovery rates of both populations. Interventions that reduced human–baboon contact, such as sterilization and limiting access to food resources, proved to be effective in lowering transmission rates. The findings underscore the importance of combining these control measures with public health strategies aimed at improving human recovery rates through medical interventions. The numerical simulations conducted in this study supported the theoretical results, demonstrating that optimal control strategies can significantly reduce zoonotic disease risks. Sterilization of baboons, along with restricted food access and reduced interactions with humans, emerged as an effective approach for controlling zoonotic disease transmission.

The numerical simulations confirmed the effectiveness of various intervention strategies in controlling zoonotic disease transmission. Notably, reducing human–baboon interactions ($H_i$) played a crucial role in mitigating infections, while food restriction ($H_f$) emerged as a practical and impactful control measure. The Generalized Euler Method provided a robust numerical framework for approximating the fractional-order system, ensuring accurate predictions of disease dynamics. These findings highlight the importance of integrating mathematical modeling with public health policies to design optimal zoonotic disease control strategies.

The feasibility of implementing control measures for human–baboon interactions involves assessing sterilization, food restriction, and interaction reduction, each with unique challenges and potential solutions. Sterilization, while moderately feasible, is resource-intensive, ethically complex, and slow in impact, though alternatives such as immunocontraceptives and small-scale trials may enhance effectiveness. Food restriction is more practical, requiring secured waste disposal and public education, though enforcement must be sustained to prevent baboons from seeking alternative food sources. Reducing human–baboon interactions is similarly feasible but demands long-term public behavioral change, supported by wildlife corridors, deterrents, and fines for feeding. Empirical studies, including macaque sterilization in urban areas, bear-proofing programs in North America, and eco-tourism regulations in Kruger National Park, provide valuable validation of these interventions. A multi-faceted approach combining these strategies, alongside education, community engagement, and continuous monitoring, offers the most effective solution for mitigating human–baboon conflicts.

The stability analysis confirmed that the basic reproduction number (${\mathcal{R}}_0$) is a critical threshold for disease persistence, with ${\mathcal{R}}_0<1$ leading to eradication and ${\mathcal{R}}_0>1$ indicating endemic conditions. The sensitivity analysis highlighted that transmission and recovery rates significantly influence disease spread. Numerical simulations using the Generalized Euler Method demonstrated that food access restriction ($H_f$) and interaction reduction ($H_i$) were particularly effective in controlling the disease, while sterilization ($H_s$) alone was insufficient. The Generalized Euler Method provided computational efficiency and accuracy in approximating the fractional-order system, confirming its suitability for complex epidemiological modeling. The results underscore the importance of integrating mathematical modeling with public health strategies, such as limiting human–wildlife interactions, promoting awareness campaigns, and implementing wildlife management policies. Future work should incorporate spatial dynamics, refine parameter estimates using empirical data, and explore alternative numerical methods to enhance predictive accuracy. Overall, this research contributes to a deeper understanding of zoonotic disease control and informs evidence-based intervention strategies.

8. Conclusions

This study developed a fractional-order mathematical model to investigate zoonotic disease transmission between baboons and humans, incorporating key control strategies such as sterilization, food access restriction, and reduced human–baboon interactions. Theoretical analysis established the model’s well-posedness, including the existence, uniqueness, non-negativity, and boundedness of solutions. The basic reproduction number (${\mathcal{R}}_0$) was derived to assess disease persistence, confirming that ${\mathcal{R}}_0<1$ leads to eradication, while ${\mathcal{R}}_0>1$ results in endemic conditions. A sensitivity analysis identified critical parameters influencing transmission, emphasizing the effectiveness of intervention measures. Numerical simulations using the Generalized Euler Method demonstrated that reducing human–baboon interactions ($H_i$) and restricting food access ($H_f$) significantly lowered infection rates, while sterilization ($H_s$) contributed to long-term control but was less effective as a standalone measure. The study highlights the importance of integrating mathematical modeling with public health policies, to develop optimal zoonotic disease management strategies. Future research should extend the model by incorporating spatial dynamics, refining parameter estimates using real-world data, and exploring alternative numerical schemes for improved computational efficiency. The findings provide valuable insights for policymakers and wildlife management authorities to implement targeted interventions and mitigate zoonotic disease risks.