Thermal Performance Analysis of Integrated Energy Management System for Mold Cooling/Heat Pump/Material Preheating of Injection-Molding Machine
Abstract
1. Introduction
2. Physical Mechanism and Simulation Software
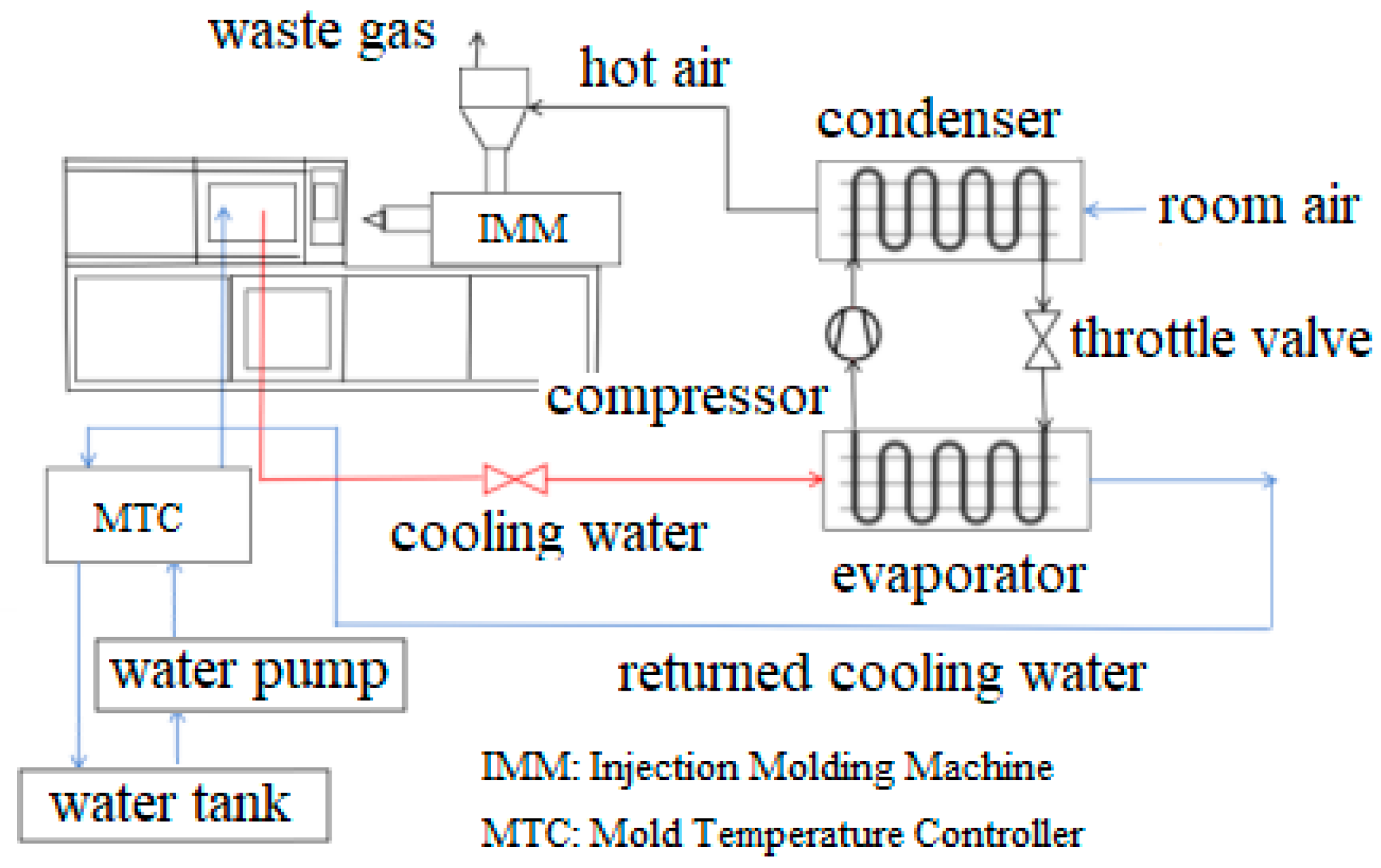
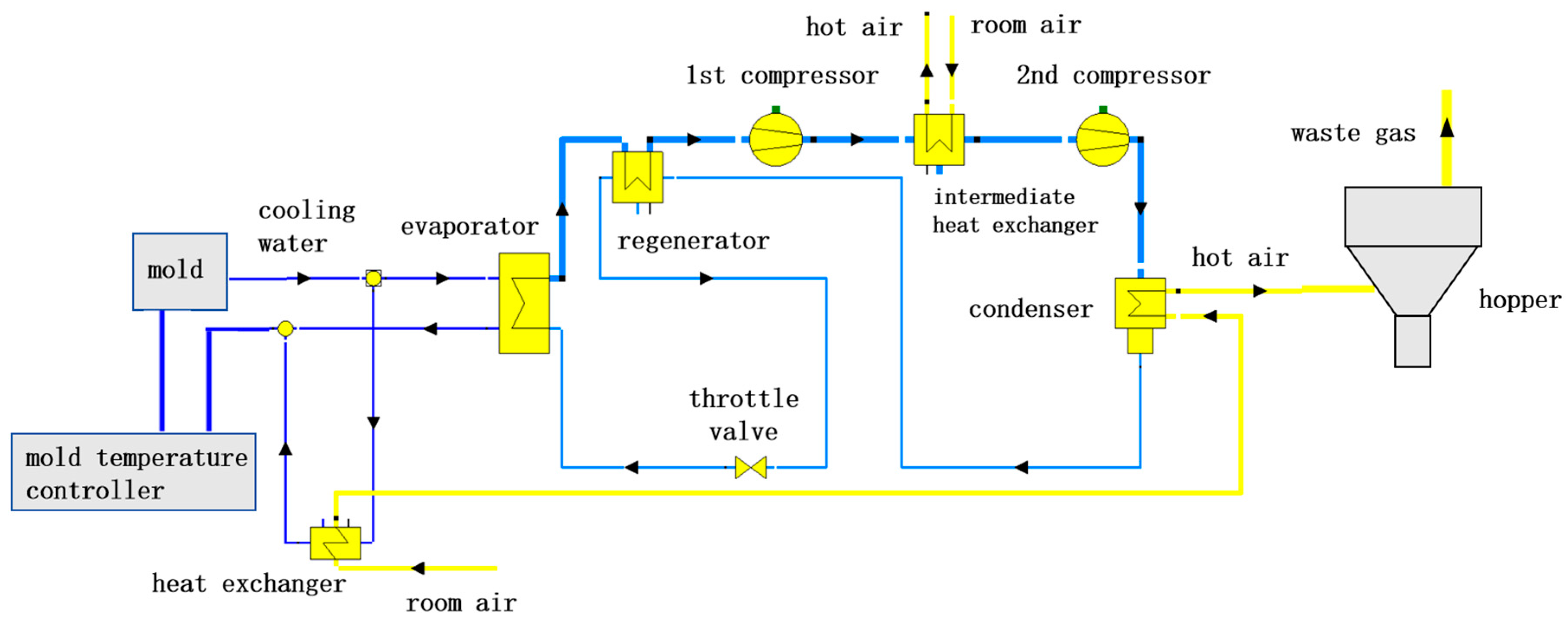
2.1. Mold Cooling/Heat Pump/Material Preheating Combined Cycle System
2.2. Mathematical and Physical Modeling
- (1)
- Compressor
- (2)
- Throttle valve
- (3)
- Evaporator
- (4)
- Condenser
- (5)
- Regenerator
2.3. Thermal Performance Evaluation Index
2.4. Simulation Software
3. Results and Discussion
3.1. Parametric Analysis of Different Mold Cooling/Heat Pump/Material Preheating Combined Cycle Configurations
3.1.1. Mold Cooling/Basic Heat Pump/Material Preheating Combined Cycle (MC/BHP/MPCC)
3.1.2. Mold Cooling/Regenerative Heat Pump/Material Preheating Combined Cycle Model (MC/RHP/MPCC)
3.1.3. Mold Cooling/Heat Pump/Material Preheating Integrated Energy Management System (MC/HP/MP-IEMS)
3.1.4. Mold Cooling/Dual-Stage Compression Heat Pump/Material Preheating Integrated Energy Management System (MC/DCHP/MP-IEMS)
3.2. Performance Comparison of Different Integrated Energy Management Systems for Mold Cooling/Heat Pump/Material Preheating
3.2.1. T-q Diagrams for Four Integrated Energy Management Systems
3.2.2. T-s Diagram of Four Integrated Energy Management Systems
3.2.3. P-h Diagram of Four Integrated Energy Management Systems
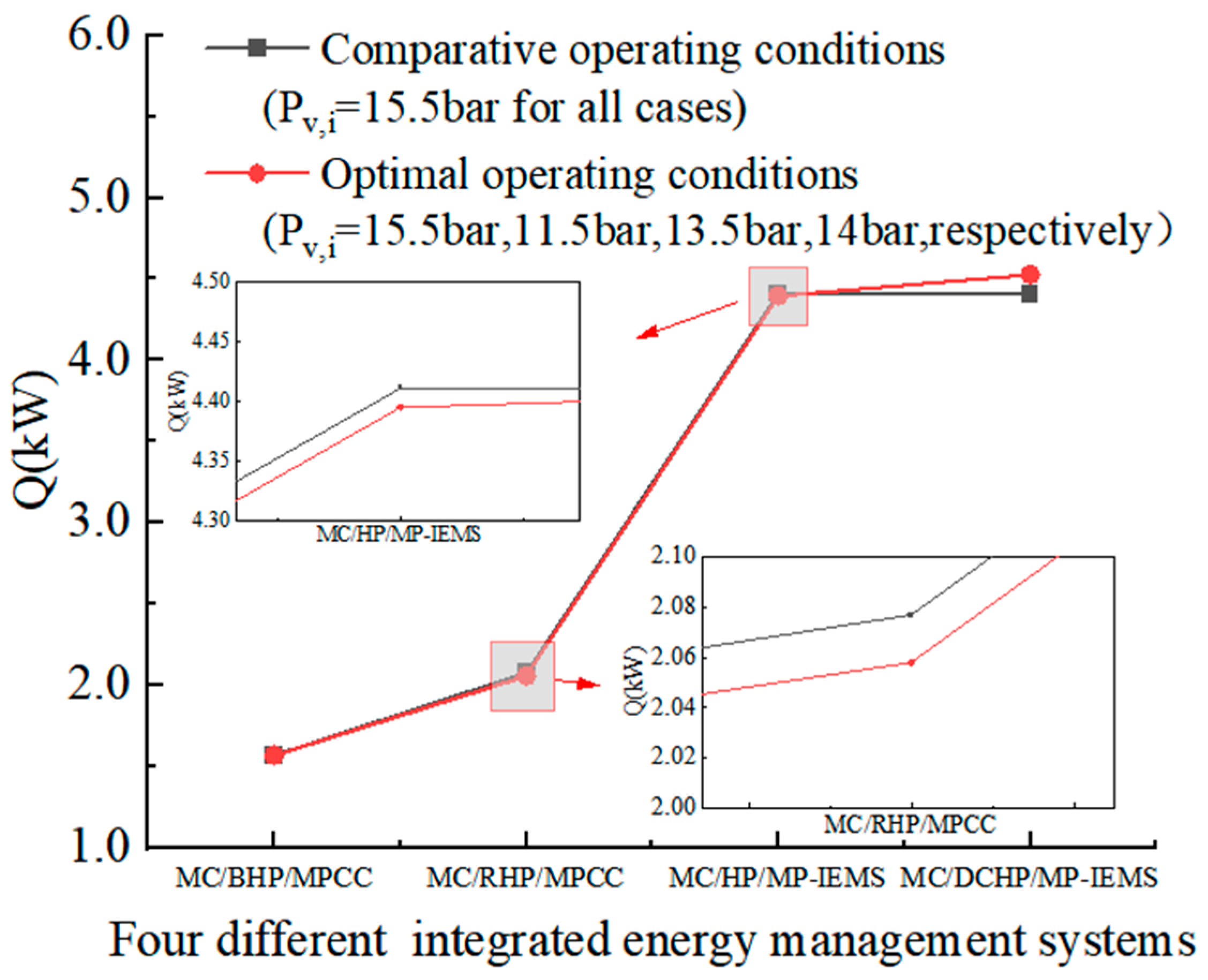
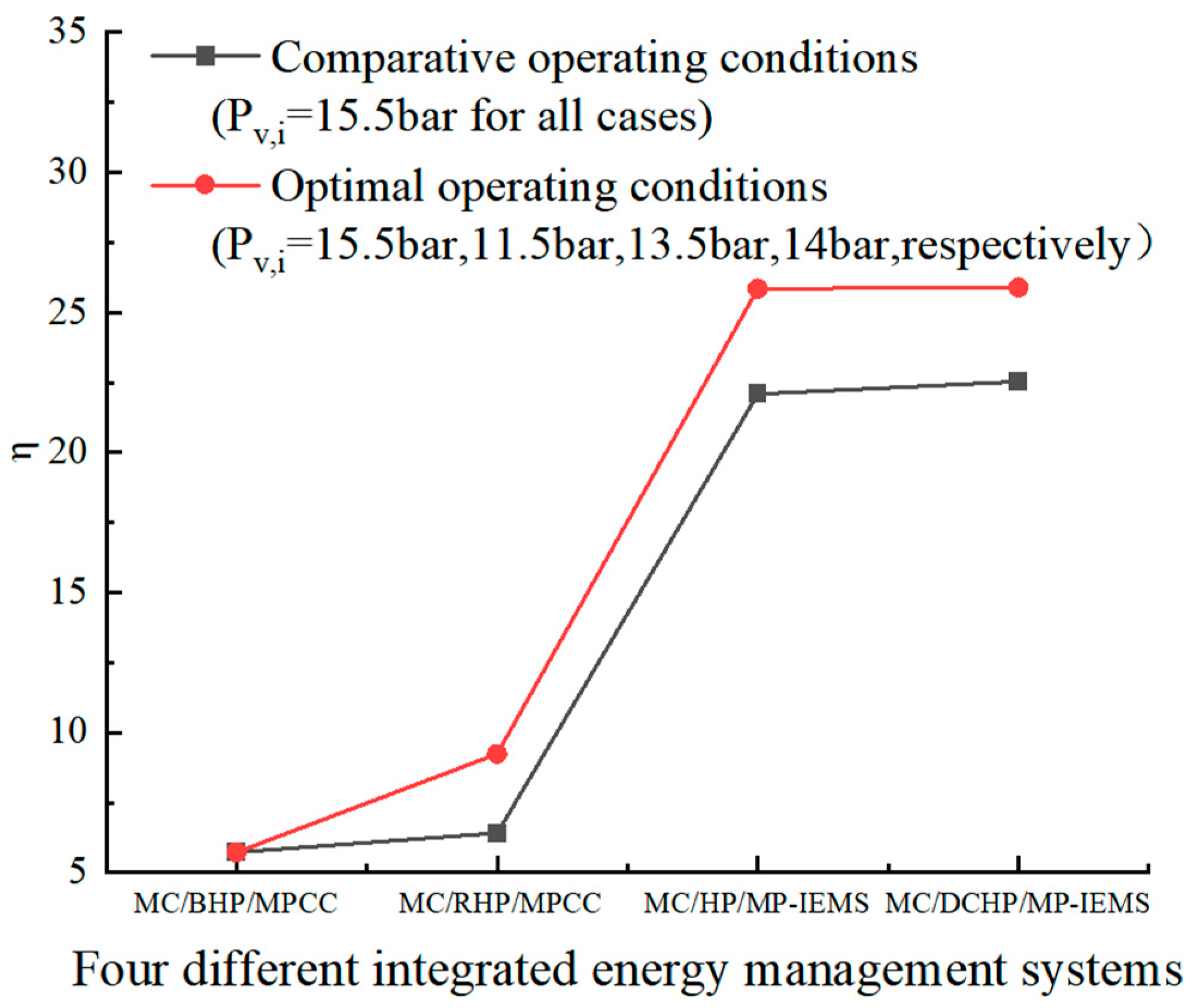
3.3. Comparison of Thermal Performance of Different Mold Cooling/Heat Pump/Material Preheating Integrated Energy Management Systems Under Different Working Conditions
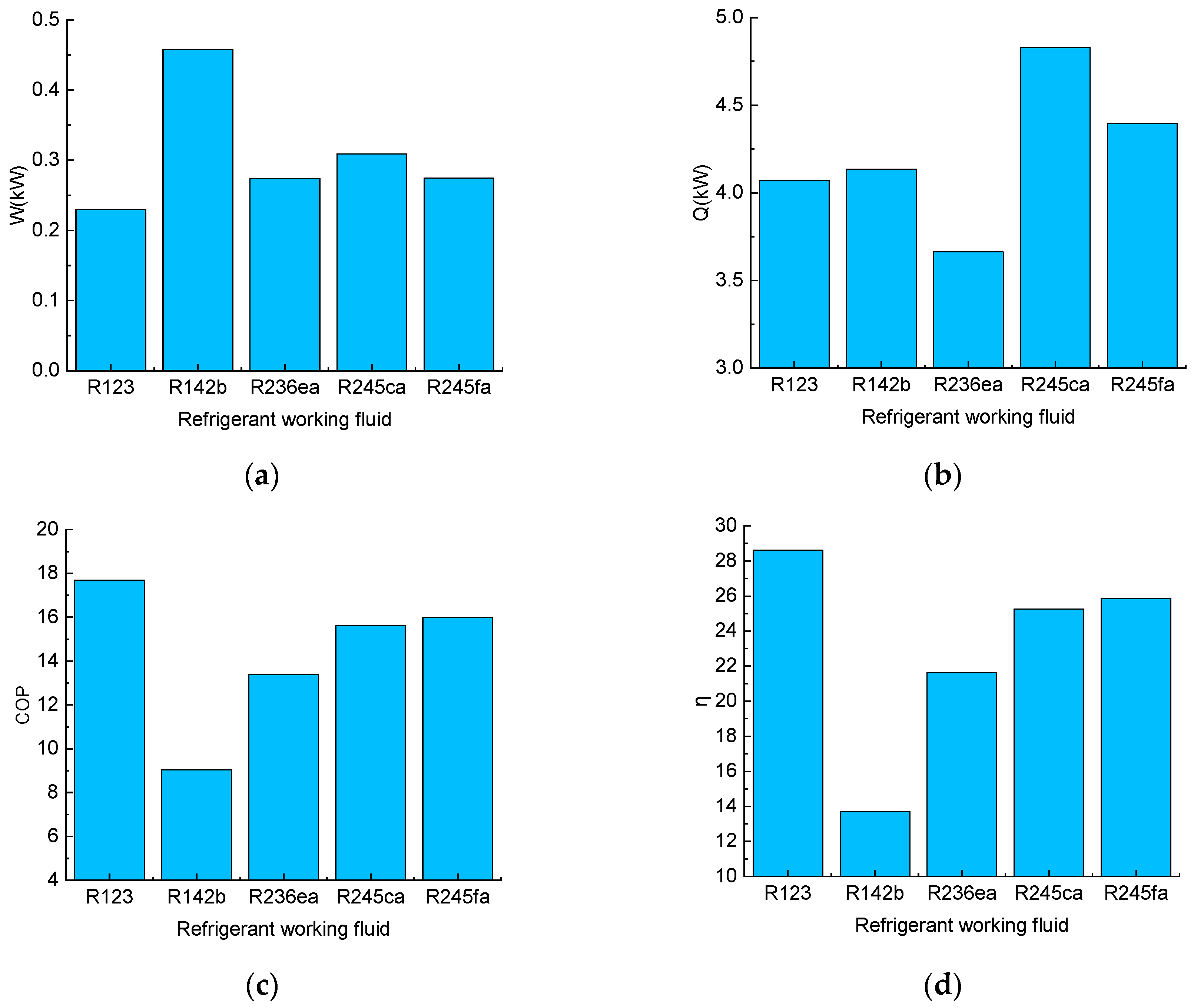
3.4. Effect of Refrigerant Types on Thermal Performance of Different Mold Cooling/Heat Pump/Material Preheating Integrated Energy Management Systems
4. Conclusions
- (1)
- Simulation analysis was performed on four integrated energy management systems, examining the pressure, temperature, specific enthalpy, void fraction, and heat at the inlet and outlet of each component during their cycles. This analysis yielded energy consumption, heat production, coefficient of performance (COP), and whole cycle energy efficiency (η) for each system. Additionally, T-q, T-s, and P-h diagrams were analyzed. The results revealed that MC/HP/MP-IEMS and MC/DCHP/MP-IEMS exhibited the best thermodynamic performance. Both systems consumed 0.32 kW of energy. Compared to MC/BHP/MPCC, MC/HP/MP-IEMS improves COP by 138% (from 13.66) and η by 286% (from 22.09), while MC/DCHP/MP-IEMS enhances COP by 144% (from 14.00) and η by 293% (from 22.53). However, given the complexity of the structure of MC/DCHP/MP-IEMS, MC/HP/MP-IEMS is recommended as the optimal integrated energy management system solution for injection-molding machines.
- (2)
- The paper compares energy consumption, heat production, COP, and whole cycle energy efficiency of different integrated energy management systems using MC/HP/MP-IEMS as an example, under both comparative and optimal conditions. In MC/RHP/MPCC, MC/HP/MP-IEMS, and MC/DCHP/MP-IEMS, the optimal condition is obtained when the outlet pressure of the throttle valve is 5.5 bar, and the outlet pressure of the compressor is 11.5 bar, 13.5 bar, and 14 bar, respectively. Under these conditions, COP and η values increase to approximately 9, 16, 16, and 9, 26, 25, respectively.
- (3)
- The paper investigates the thermodynamic properties of five different refrigerants using MC/HP/MP-IEMS as an example. Among them, R123 exhibits the best performance with a COP value of 17.69 and whole cycle energy efficiency of 28.61. However, due to its ozone-depleting potential, R123 will be phased out by 2030. R245fa, which is slightly inferior to R123 in terms of total power consumption, performs remarkably well with a COP of 16 and a whole-cycle energy efficiency of 25.5, making it a viable alternative. R245ca can also serve as a substitute. The remaining two refrigerants, R236ea, and R142b, demonstrate inferior overall performance compared to the aforementioned three.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Abbreviations | |
| COP | coefficient of performance |
| MC/BHP/MPCC | mold cooling/basic heat pump/material preheating combined cycle |
| MC/RHP/MPCC | mold cooling/regenerative heat pump/material preheating combined cycle |
| MC/HP/MP-IEMS | mold cooling/heat pump/material preheating integrated energy management system |
| MC/DCHP/MP-IEMS | mold cooling/dual-stage compression heat pump/material preheating integrated energy management system |
| PC | polycarbonate |
| Symbols | |
| T | temperature, °C |
| P | pressure, bar |
| q | transferred heat, kW |
| h | specific enthalpy, kJ/kg |
| m | mass flow rate, kg/s |
| X | gas void fraction, % |
| W | total power consumption, kW |
| W1 | 1st compression power consumption, kW |
| W2 | 2nd compression power consumption, kW |
| s | entropy, kJ/(kg·K) |
| Q | heat generation of the system, kW |
| Greek symbols | |
| actual value | |
| η | system efficiency |
| Subscripts | |
| air | hot air generated in the condenser |
| air1 | hot air generated in the cooling water/air heat exchanger |
| air2 | hot air generated in the intermediate heat exchanger |
| cf | cold fluid |
| C | condensate |
| vaporization | |
| hot fluid | |
| regenerator | |
| inlet | |
| Theoretical maximum value | |
| outlet | |
| compressor | |
| saturation | |
| time | |
| throttle valve | |
| liquid refrigerant | |
| absorb |
References
- Liu, H.; Zhang, X.; Quan, L.; Zhang, H. Research on energy consumption of injection molding machine driven by five different types of electro-hydraulic power units. J. Clean. Prod. 2020, 242, 118355. [Google Scholar] [CrossRef]
- Iwko, J.; Wroblewski, R.; Steller, R. Experimental study on energy consumption in the plasticizing unit of the injection molding machine. Polimery 2018, 63, 362–371. [Google Scholar] [CrossRef]
- Lovrec, D.; Ti, V.; Taner, T. Dynamic behaviour of different hydraulic drive concepts-comparison and limits. Int. J. Simul. Model 2017, 16, 448–457. [Google Scholar] [CrossRef]
- Xinhua News Agency. Opinions of the Central Committee of the Communist Party of China and the State Council on Fully and Faithfully Implementing the New Development Philosophy to Achieve Carbon Peaking and Carbon Neutrality. Available online: https://www.gov.cn/xinwen/2021-10/25/content_5644687.htm (accessed on 25 October 2021).
- Park, H.S.; Nguyen, T.T. Optimization of injection molding process for car fender in consideration of energy efficiency and product quality. J. Comput. Des. Eng. 2014, 1, 256–265. [Google Scholar] [CrossRef]
- Lucchetta, G.; Masato, D.; Sorgato, M. Optimization of mold thermal control for minimum energy consumption in injection molding of polypropylene parts. J. Clean. Prod. 2018, 182, 217–226. [Google Scholar] [CrossRef]
- Mattila, J.; Virvalo, T. Energy-efficient motion control of a hydraulic manipulator. IEEE Int. Conf. Robot. Autom. 2000, 3, 3000–3006. [Google Scholar]
- Matsuo, S.; Himeno, T.; Bessho, M.; Tsutsui, K.; Kato, N.; Mizuno, T. World’s first 3,000 ton mold clamping force electric injection molding machine. Mitsubishi Heavy Ind. Ltd. Tech. Rev. 2006, 43, 1–6. [Google Scholar]
- Imamura, T.; Sawada, Y.; Ichikawa, M.; Nakamura, H. Energy-saving hybrid hydraulic system comprising highly efficient IPM motor and inverter, for injection molding and manufacturing machine. In Proceedings of the JFPS International Symposium on Fluid Power, Toyama, Japan, 15–18 September 2008; Volume 9, pp. 117–120. [Google Scholar]
- Zhang, H.; Ren, L.; Gao, Y.; Jin, B. A comprehensive study of energy conservation in electric-hydraulic injection-molding equipment. Energy 2017, 10, 1768. [Google Scholar] [CrossRef]
- Takahashi, K.E.; Okamoto, M.; Hiraki, E.; Tanaka, T. Simulation analysis of energy-saving effect of an energy recovery system for electric motor drive system in the injection molding machine. In Proceedings of the Power Electronics & Motion Control Conference, Ohrid, Macedonia, 21 October 2010. [Google Scholar]
- Lu, N.Y.; Gong, G.X.; Yang, Y.; Lu, J.H. Multi-objective process parameter optimization for energy saving in injection molding process. J. Zhejiang Univ. Sci. A (App. Phys. Eng.) 2012, 13, 382–394. [Google Scholar] [CrossRef]
- Yin, K.H. Dynamic Optimisation for Energy Efficiency of Injection Moulding Process. Ph.D. Thesis, University of Nottingham, Nottingham, UK, 2015. [Google Scholar]
- Mianehrow, H.; Abbasian, A. Energy monitoring of plastic injection molding process running with hydraulic injection molding machines. J. Clean Prod. 2017, 148, 804–810. [Google Scholar] [CrossRef]
- Meekers, I.; Refalo, P.; Rochman, A. Analysis of process parameters affecting energy consumption in plastic injection moulding. Procedia CIRP. 2018, 69, 342–347. [Google Scholar] [CrossRef]
- Moussaoui, H. Achieving energy savings and process optimization in plastic injection molding: A design of experiments study. J. Clean. Prod. 2024, 477, 143835. [Google Scholar] [CrossRef]
- Othman, N.A. Sustainable Manufacturing: Optimization of Electrical Energy Consumption in Plastic Injection Molding Process. Master’s Thesis, Universiti Utara Malaysia, Kedah, Malaysia, 2015. [Google Scholar]
- Rashid, O.; Low, K.W.Q.; Pittman, J.F.T. Mold cooling in thermoplastics injection molding: Effectiveness and energy efficiency. J. Clean Prod. 2020, 264, 121375. [Google Scholar] [CrossRef]
- Brückner, S.; Liu, S.; Miró, L.; Radspieler, M.; Cabeza, L.F.; Lävemann, E. Industrial waste heat recovery technologies: An economic analysis of heat transformation technologies. Appl. Energy 2015, 151, 157–167. [Google Scholar] [CrossRef]
- Jouhara, H.; Khordehgah, N.; Almahmoud, S.; Delpech, B.; Chauhan, A.; Tassou, S.A. Waste heat recovery technologies and applications. Therm. Sci. Eng. Prog. 2018, 6, 268–289. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Fazli, M.; Morad, M.R. A recent review of waste heat recovery by organic rankine cycle. Appl. Therm. Eng. 2018, 143, 660–675. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, G.; Li, L.; Yang, Q.; Tang, B.; Liu, Y. Expansion devices for organic rankine cycle (ORC) using in low temperature heat recovery: A review. Energy Convers. Manag. 2019, 199, 111944. [Google Scholar] [CrossRef]
- Wang, E.; Yu, Z.; Zhang, H.; Yang, F. A regenerative supercritical-subcritical dual-loop organic rankine cycle system for energy recovery from the waste heat of internal combustion engines. Appl. Energy 2017, 190, 574–590. [Google Scholar] [CrossRef]
- Michael, L.; O’Toole, K. A new technology for cost effective low grade waste heat recovery. Energy Procedia 2017, 123, 188–195. [Google Scholar]
- Xiao, S.Q.; Nefodov, D.; Richter, M.; Wördemann, M.; Urbaneck, T. Large heat pumps with hot water store in local heating systems–Investigation of operation strategies. J. Energy Storage 2023, 63, 106924. [Google Scholar] [CrossRef]
- Szymiczek, J.; Szczotka, K.; Banaś, M.; Jura, P. Efficiency of a compressor heat pump system in different cycle designs: A simulation study for low-enthalpy geothermal resources. Energies 2022, 15, 5546. [Google Scholar] [CrossRef]
- Heath, E.A. Amendment to the Montreal protocol on substances that deplete the ozone layer. Int. J. Mater. Res. 2017, 56, 193–205. [Google Scholar]
- Xie, Y.; Hu, P.; Zhu, N.; Lei, F.; Xing, L.; Xu, L.; Sun, Q. A hybrid short-term load forecasting model and its application in ground source heat pump with cooling storage system. Renew. Energy 2020, 161, 1244–1259. [Google Scholar] [CrossRef]
- Yong, H.E.; Chung, Y.; Min, S.P.; Hong, S.B.; Min, S.K. Deep learning-based prediction method on performance change of air source heat pump system under frosting conditions. Energy 2021, 228, 120542. [Google Scholar]
- Wang, C.R.; Yu, X.; Han, C. Performance prediction of a ground source heat pump system using denoised long short-term memory neural network optimised by fast non-dominated sorting genetic algorithm-II. Geothermics 2014, 120, 103002. [Google Scholar]
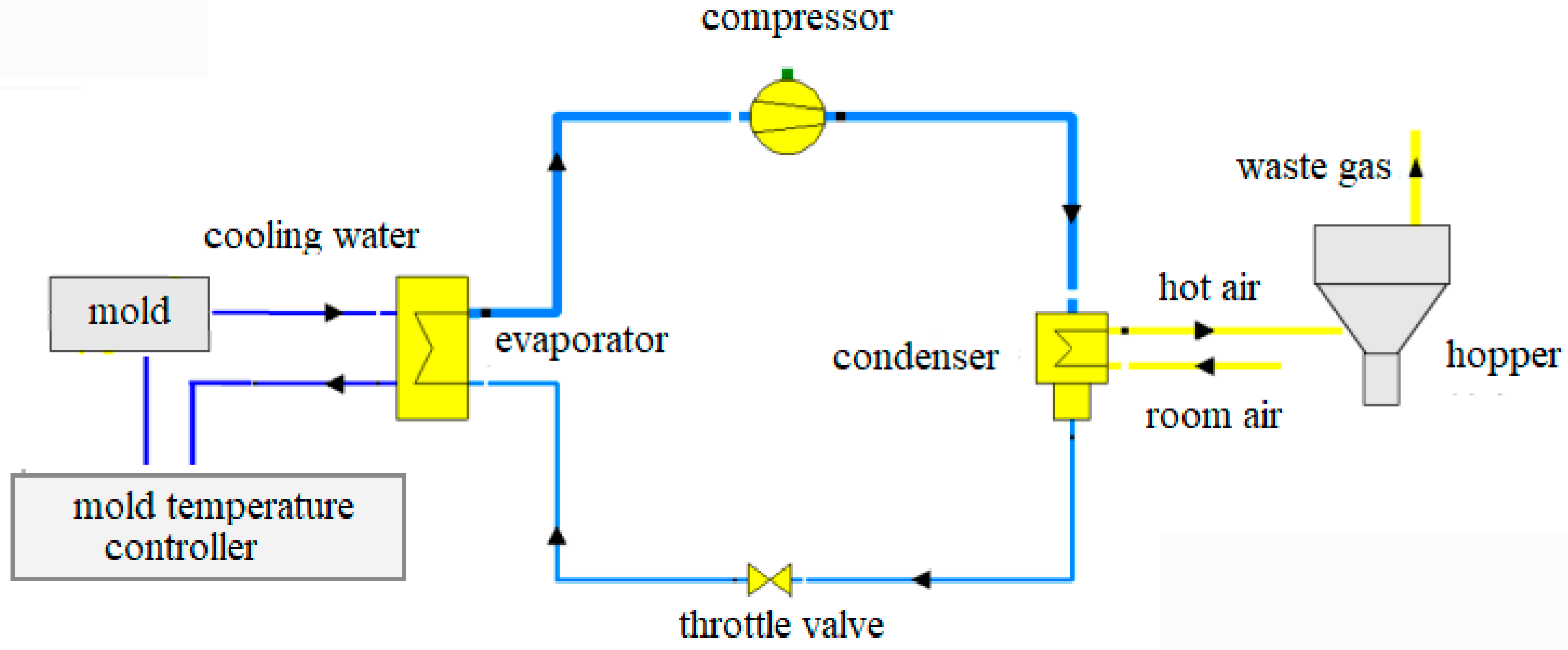
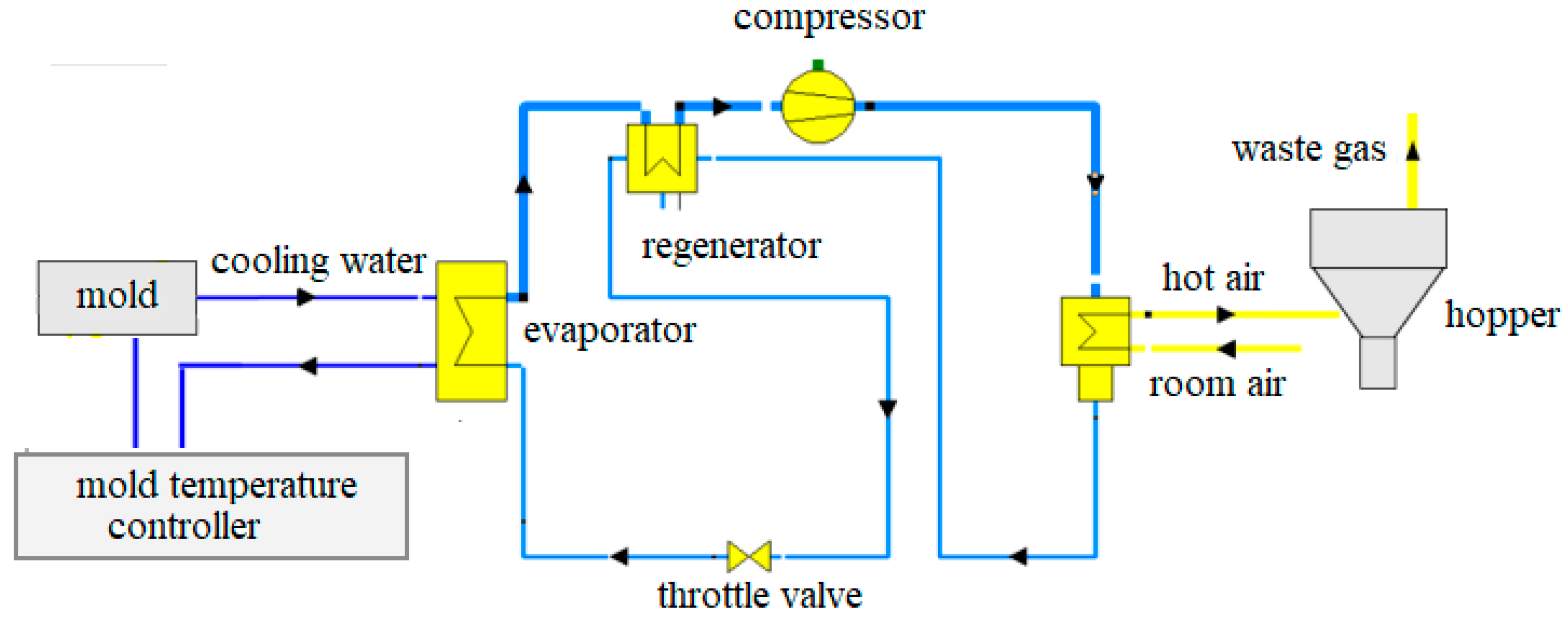
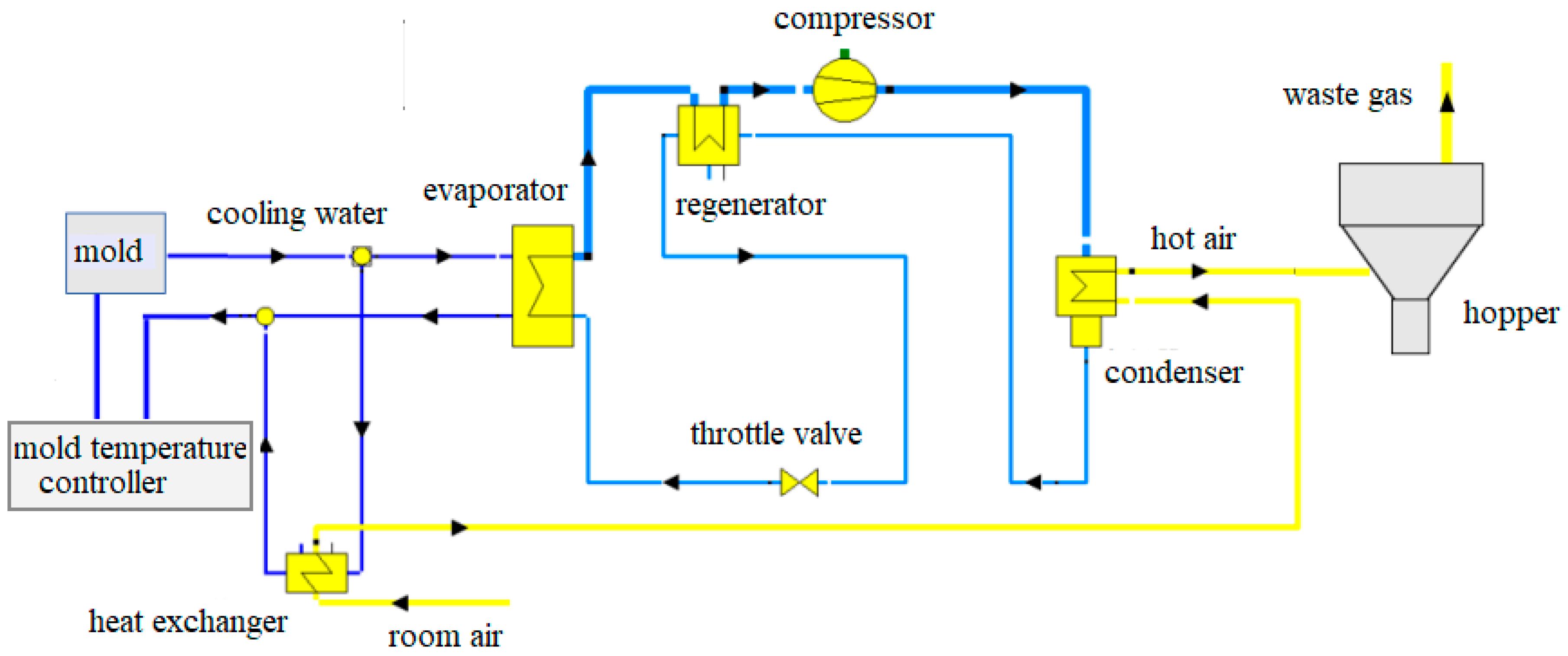
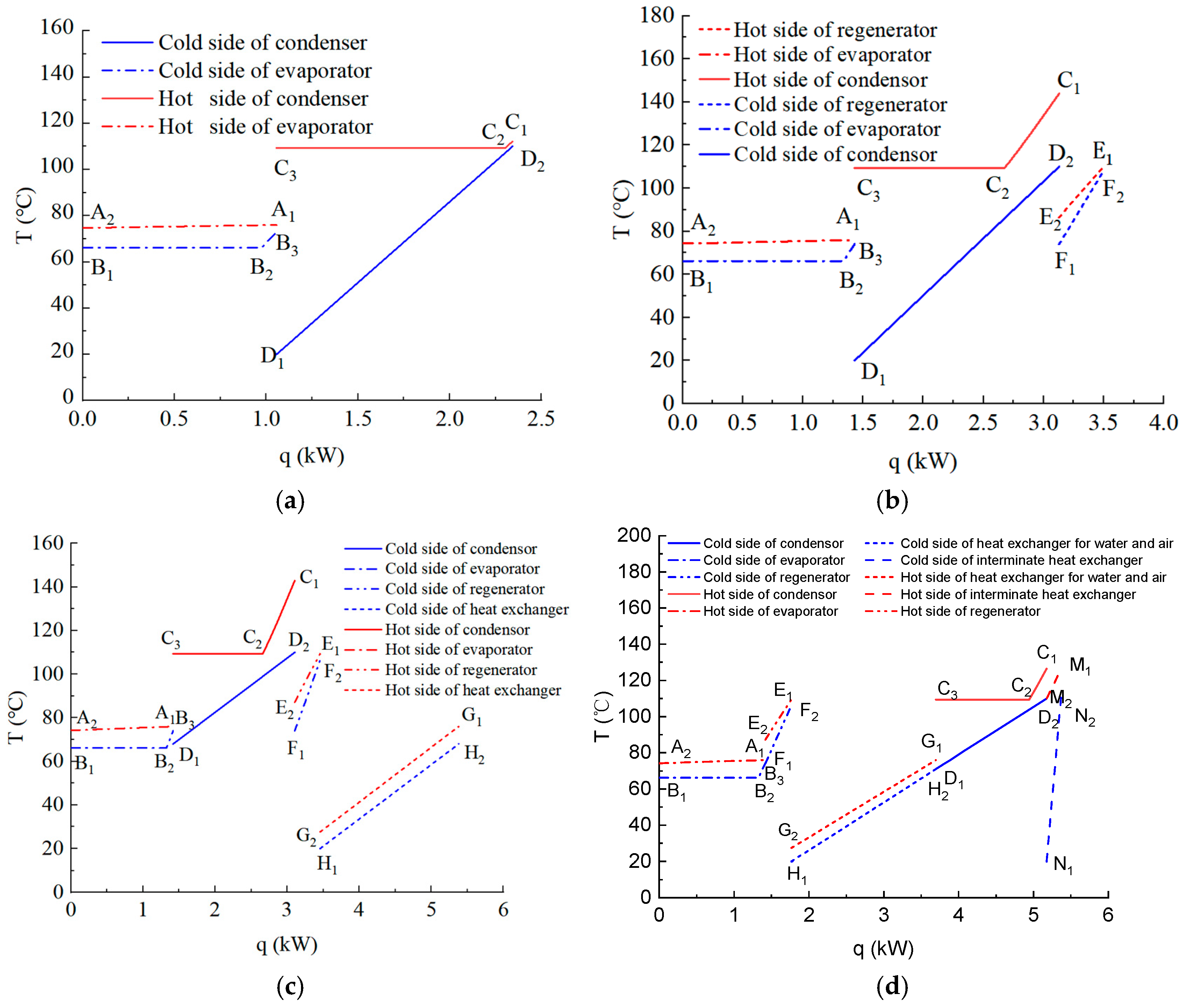
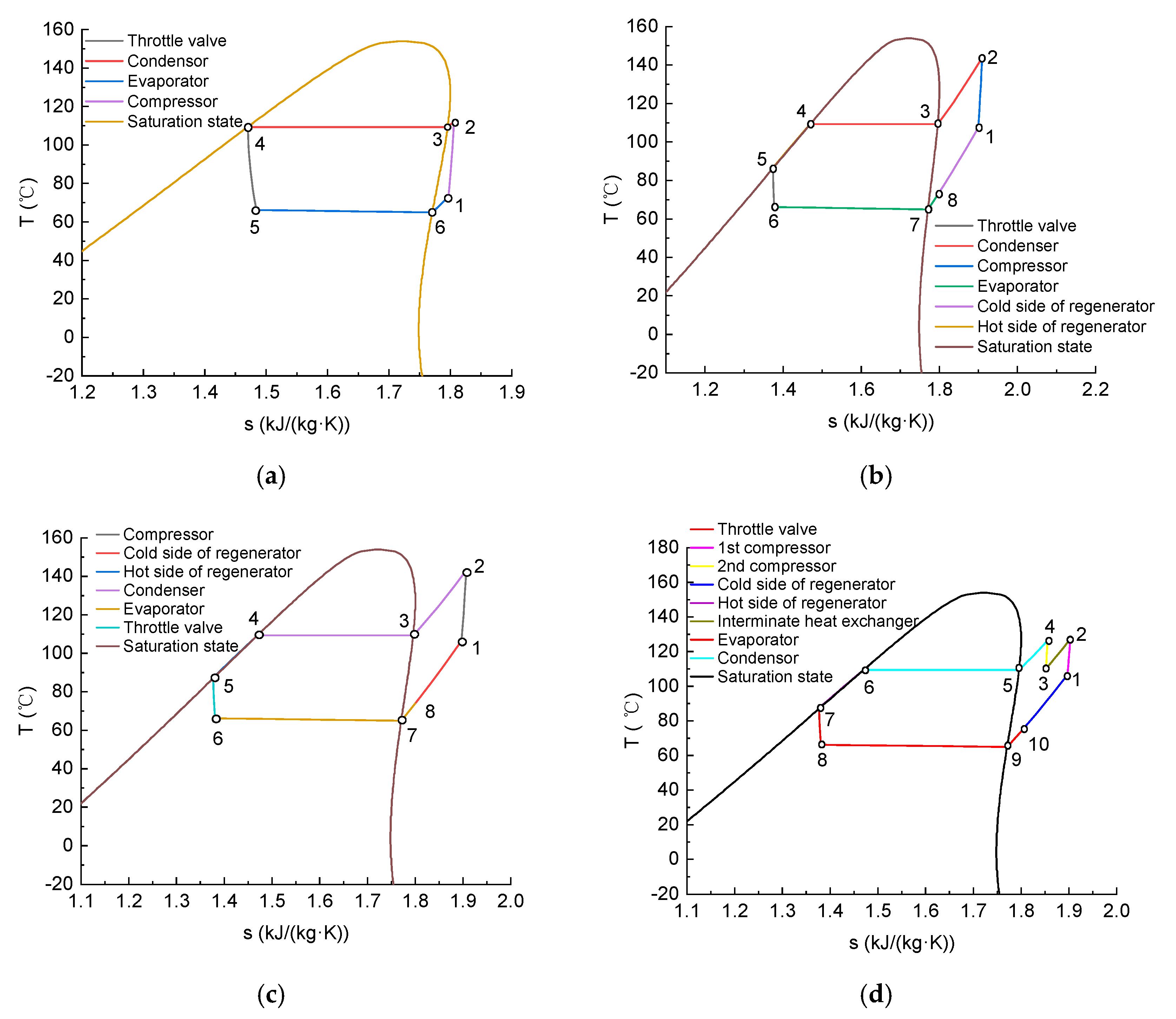
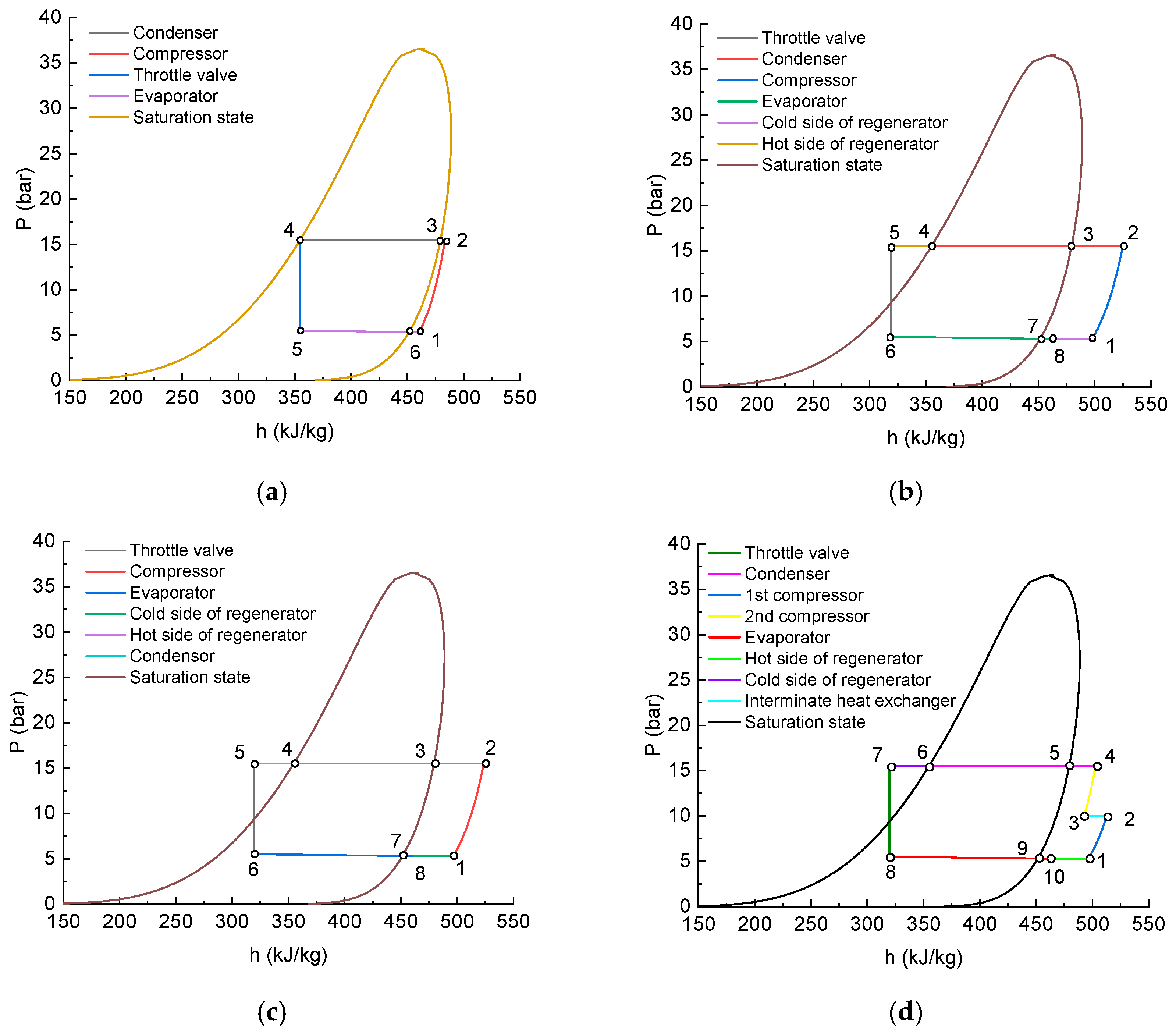


| Items | T/°C | P/bar | q/kW | h/kJ·kg−1 | m/kg·s−1 | X | |
|---|---|---|---|---|---|---|---|
| Cooling water | inlet | 76.00 | 1.0 | 63.64 | 318.22 | 0.2 | 0 |
| outlet | 74.74 | 0.9 | 62.59 | 312.93 | 0.2 | 0 | |
| Cold side of Evaporator | inlet | 66.19 | 5.5 | 3.55 | 354.86 | 0.01 | 0.40 |
| outlet | 73.00 | 5.3 | 4.60 | 460.48 | 0.01 | 1 | |
| Compressor | inlet | 73.00 | 5.3 | 4.60 | 460.48 | 0.01 | 1 |
| outlet | 112.16 | 15.5 | 4.83 | 483.41 | 0.01 | 1 | |
| Hot side of condenser | inlet | 112.16 | 15.5 | 4.83 | 483.41 | 0.01 | 1 |
| outlet | 109.40 | 15.5 | 3.55 | 354.86 | 0.01 | 0 | |
| Throttle valve | inlet | 109.40 | 15.5 | 3.55 | 354.86 | 0.01 | 0 |
| outlet | 66.19 | 5.5 | 3.55 | 354.86 | 0.01 | 0.40 | |
| Cold side of condenser-air | inlet | 20.00 | 1.0 | 0.29 | 20.10 | 0.014 | 1 |
| outlet | 110.0 | 1.0 | 1.57 | 110.82 | 0.014 | 1 | |
| Motor | W | 0.27 kW | |||||
| Total Power Consumption | Heat Produced by the Heat Pump | Coefficient of Performance in Heat Pump | Whole Cycle Energy Efficiency |
|---|---|---|---|
| /kW | /kW | COP | η |
| 0.27 | 1.57 | 5.73 | 5.73 |
| Total Power Consumption | Heat Production by the Heat Pump | Coefficient of Performance in Heat Pump | Whole Cycle Energy Efficiency |
|---|---|---|---|
| /kW | /kW | COP | η |
| 0.32 | 2.08 | 6.41 | 6.41 |
| Total Power Consumption | Heat Production by the Heat Pump | Coefficient of Performance in Heat Pump | Whole Cycle Energy Efficiency |
|---|---|---|---|
| /kW | /kW | COP | η |
| 0.323 | 4.41 | 13.66 | 22.09 |
| Total Power Consumption | Heat Production by the Heat Pump | Coefficient of Performance in Heat Pump | Whole Cycle Energy Efficiency |
|---|---|---|---|
| /kW | /kW | COP | η |
| 0.32 | 4.41 | 14.00 | 22.53 |
| Code | Molecular Formula | Molecular Weight | Safety | Standard Boiling Point/°C | Critical Temperature/°C | Critical Pressure/MPa | ODP | GWP |
|---|---|---|---|---|---|---|---|---|
| R123 | CHClF2CF3 | 152.93 | B1 | 27.8 | 183.7 | 3.66 | 0.02 | 120 |
| R142b | CClF2CH3 | 100.5 | A2 | −9.1 | 137.1 | 4.06 | 0.065 | 2400 |
| R236ea | CHF2-CHF-CF3 | 152.05 | - | 6.5 | 139.23 | 3.41 | 0 | 710 |
| R245ca | CH2FCF2CHF2 | 134.05 | - | 25 | 174.42 | 3.93 | 0 | 350 |
| R245fa | CF3-CH2-CHF2 | 134.05 | B1 | 15.3 | 157.5 | 3.64 | 0 | 858 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, Y.; Hu, H.; Ding, Y.; Wang, T.; Xie, P.; Yang, W. Thermal Performance Analysis of Integrated Energy Management System for Mold Cooling/Heat Pump/Material Preheating of Injection-Molding Machine. Symmetry 2025, 17, 637. https://doi.org/10.3390/sym17050637
Tang Y, Hu H, Ding Y, Wang T, Xie P, Yang W. Thermal Performance Analysis of Integrated Energy Management System for Mold Cooling/Heat Pump/Material Preheating of Injection-Molding Machine. Symmetry. 2025; 17(5):637. https://doi.org/10.3390/sym17050637
Chicago/Turabian StyleTang, Yuxuan, Hemin Hu, Yumei Ding, Tao Wang, Pengcheng Xie, and Weimin Yang. 2025. "Thermal Performance Analysis of Integrated Energy Management System for Mold Cooling/Heat Pump/Material Preheating of Injection-Molding Machine" Symmetry 17, no. 5: 637. https://doi.org/10.3390/sym17050637
APA StyleTang, Y., Hu, H., Ding, Y., Wang, T., Xie, P., & Yang, W. (2025). Thermal Performance Analysis of Integrated Energy Management System for Mold Cooling/Heat Pump/Material Preheating of Injection-Molding Machine. Symmetry, 17(5), 637. https://doi.org/10.3390/sym17050637





