Design of Fast Response Compound Control System for Hypersonic Skid-to-Turn Missile
Abstract
1. Introduction
- (1)
- Control Requirements of Aircraft
- (2)
- Advantages of Optimal Control
- (3)
- Limitations of Adaptive Control
- (4)
- Compound Control Strategies in Aircraft
2. STT Missile Model
2.1. Pitch Channel Model
2.2. Yaw Channel Model
3. Controller Design
3.1. Controller Design of Aerodynamic System
3.1.1. Aerodynamic System Controller in Pitch Channel
3.1.2. Aerodynamic System Controller in Yaw Channel
3.2. Controller Design of Lateral Thrust System
3.2.1. Lateral Thrust System Controller in Pitch Channel
3.2.2. Lateral Thrust System Controller in Yaw Channel
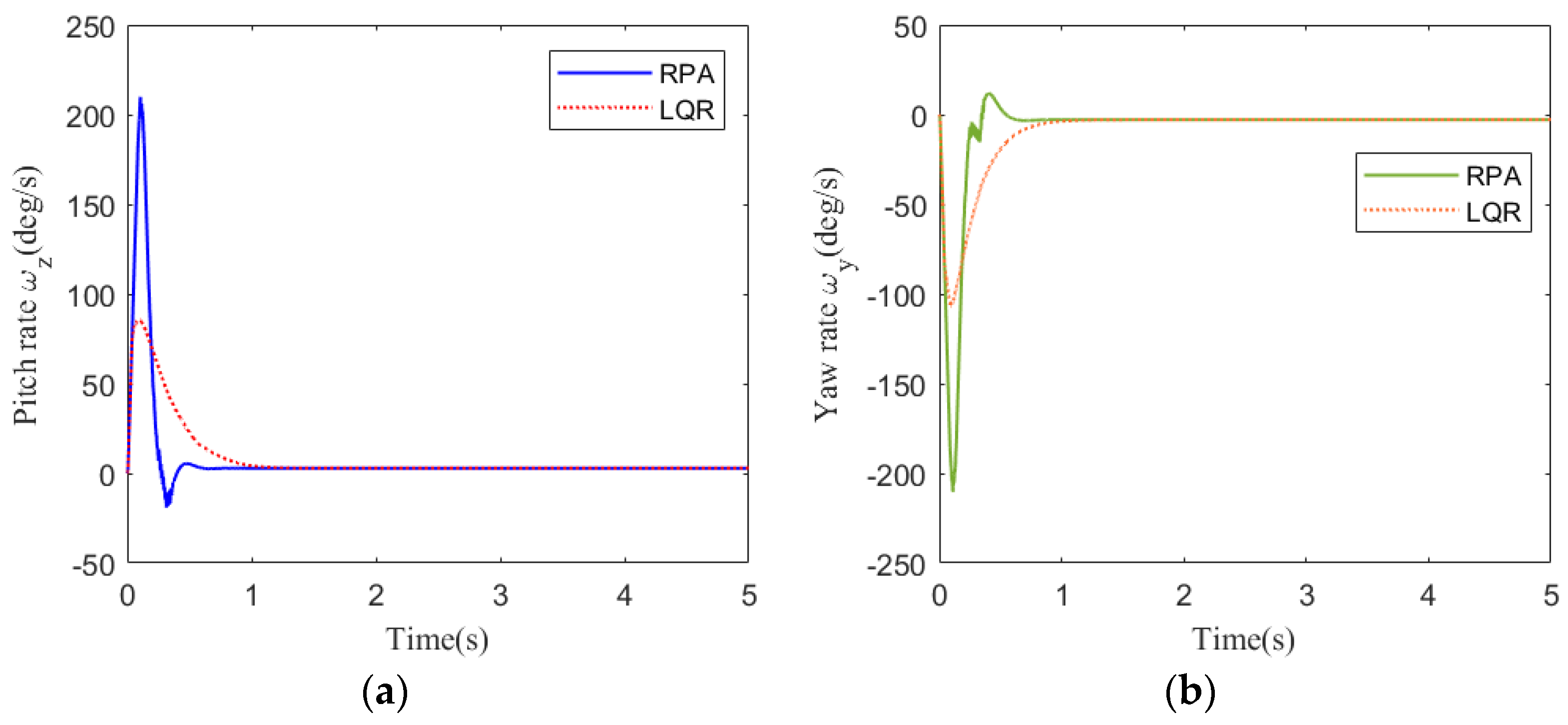
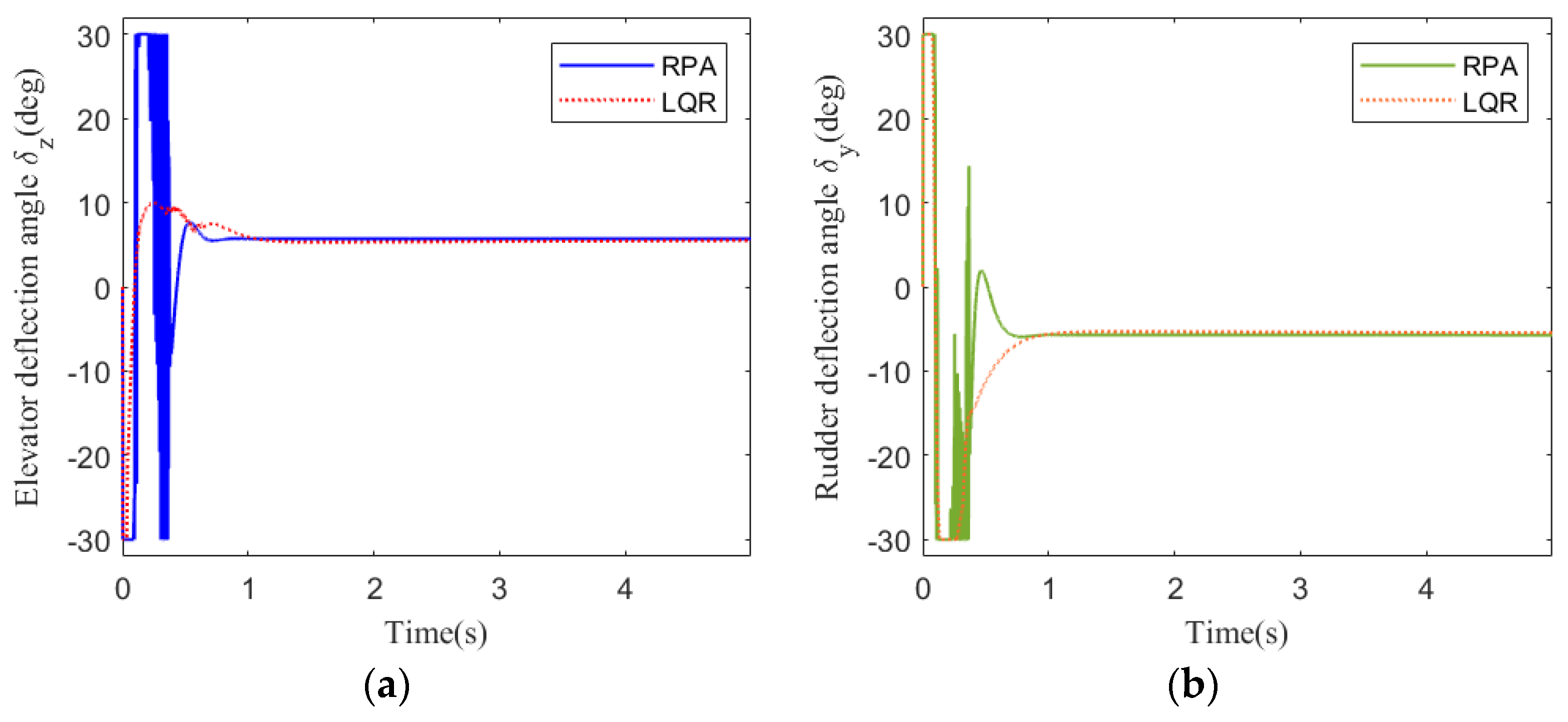
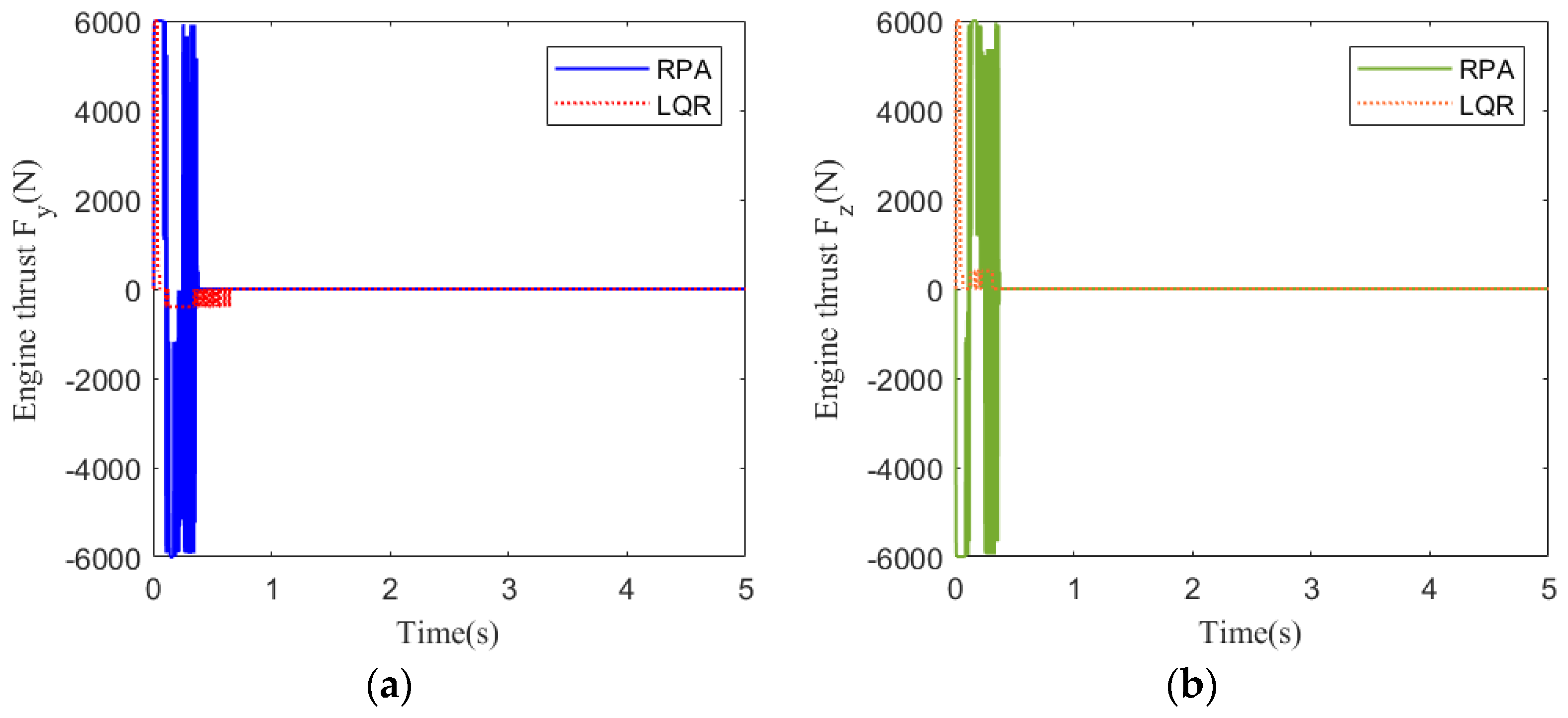
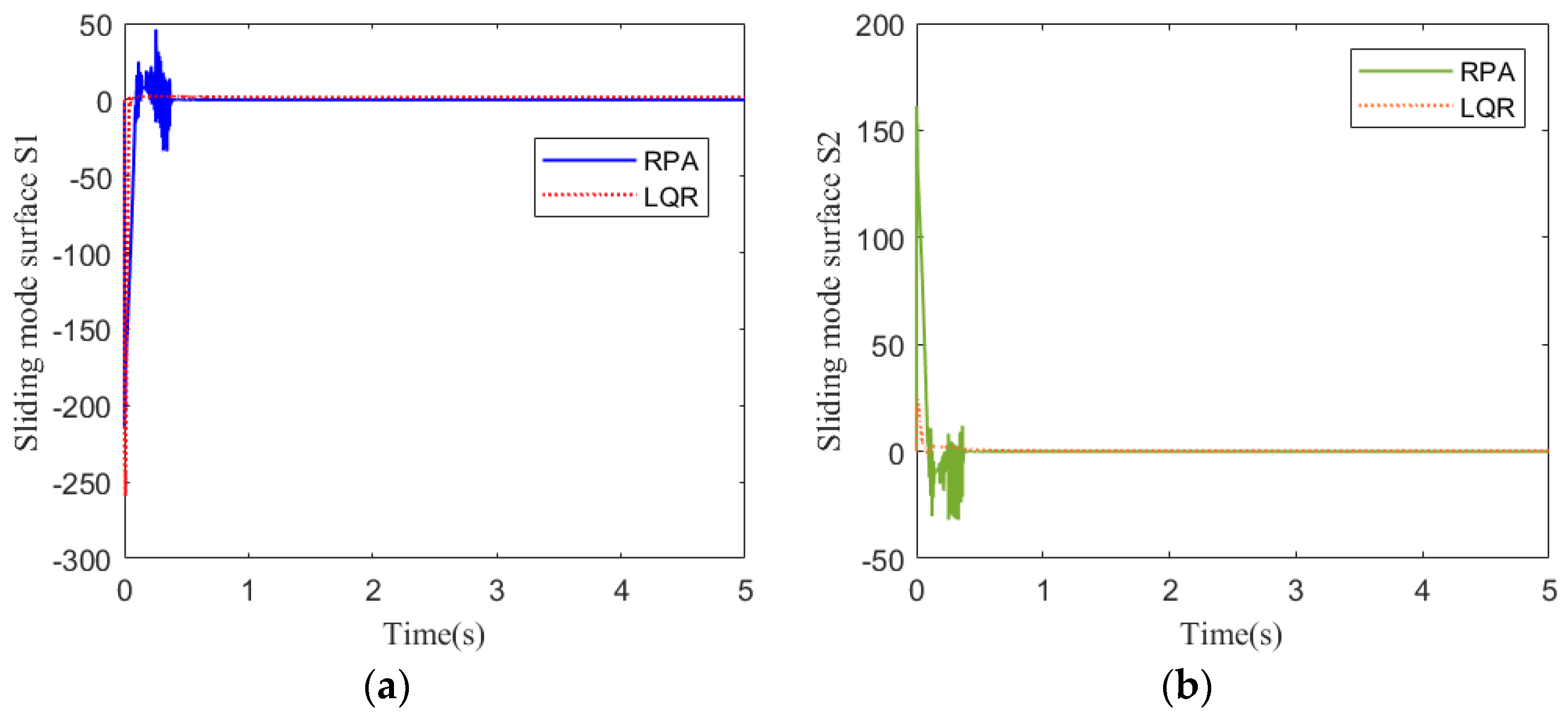
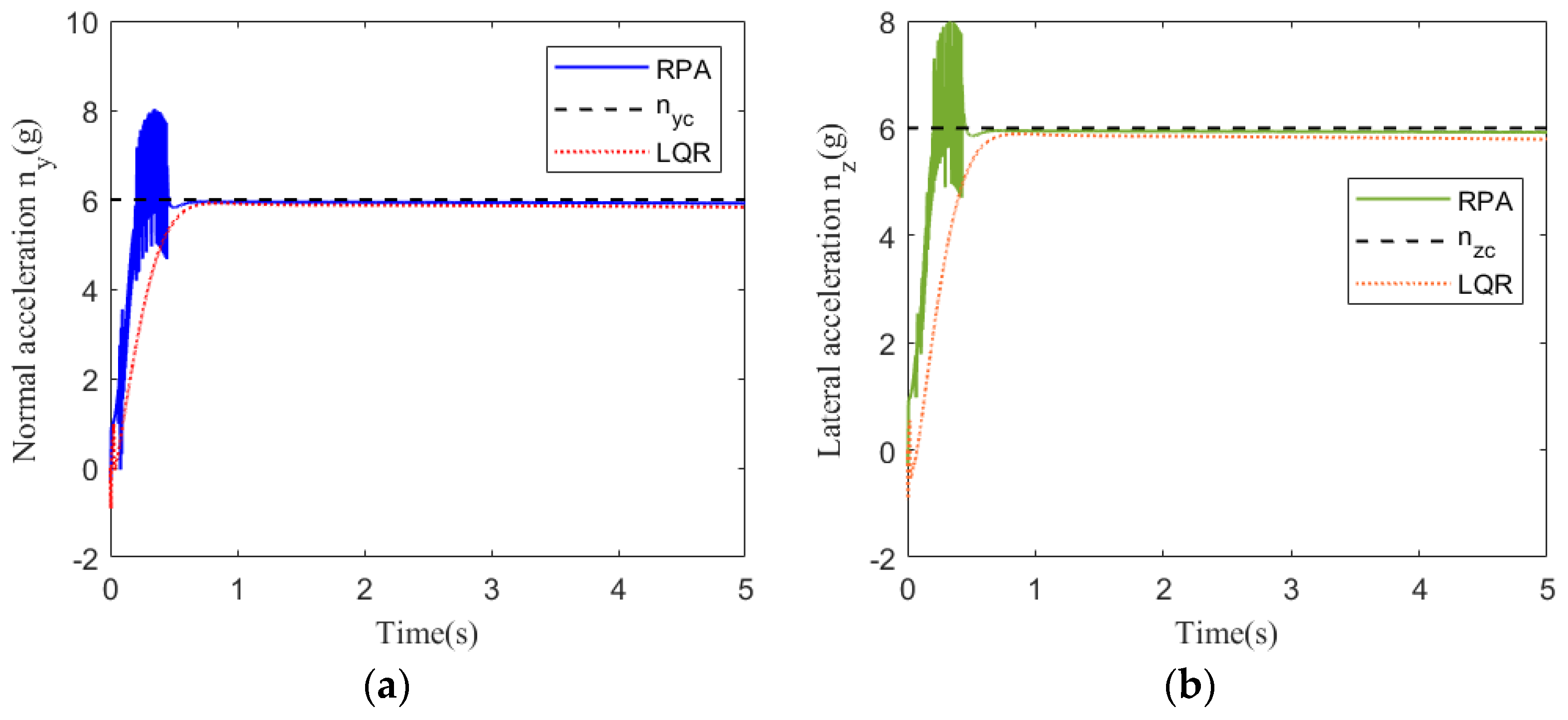
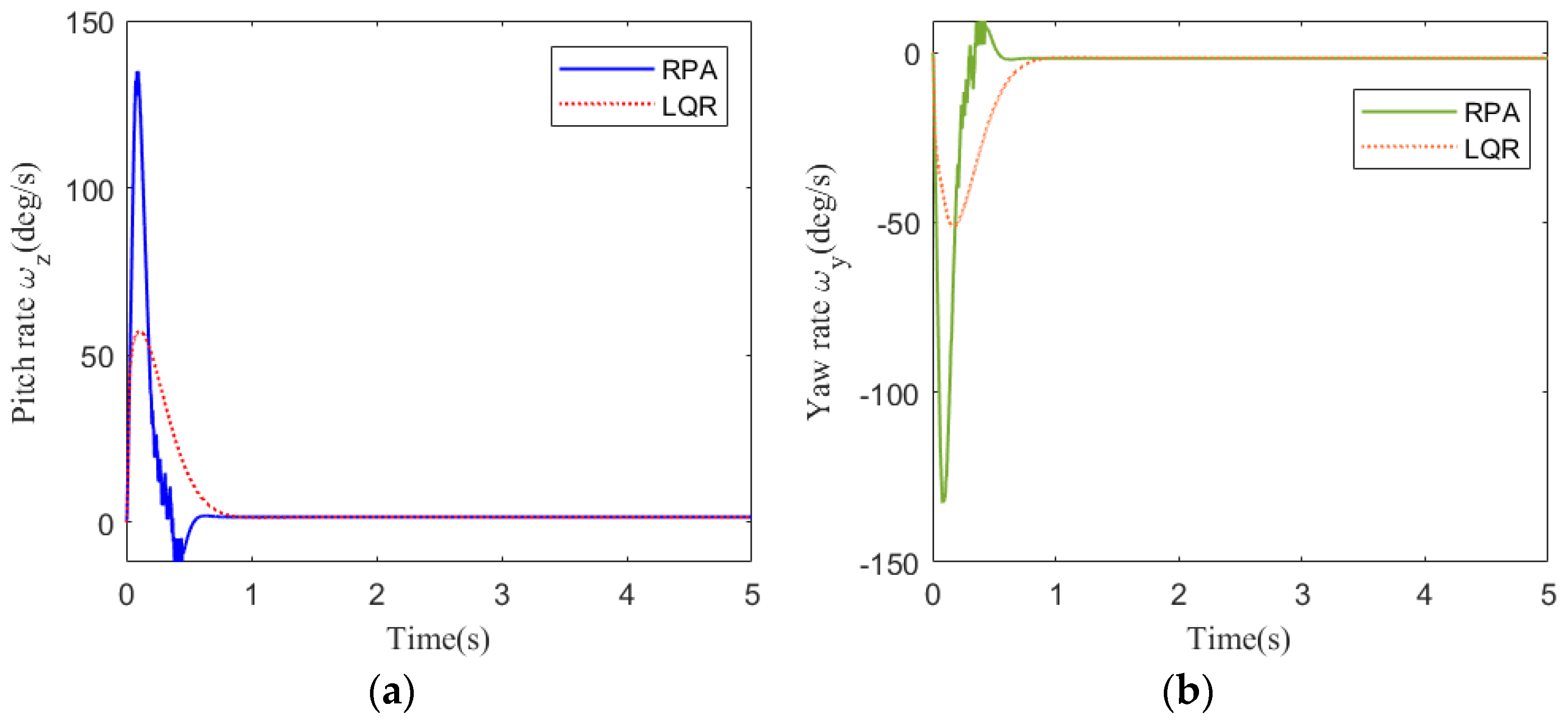
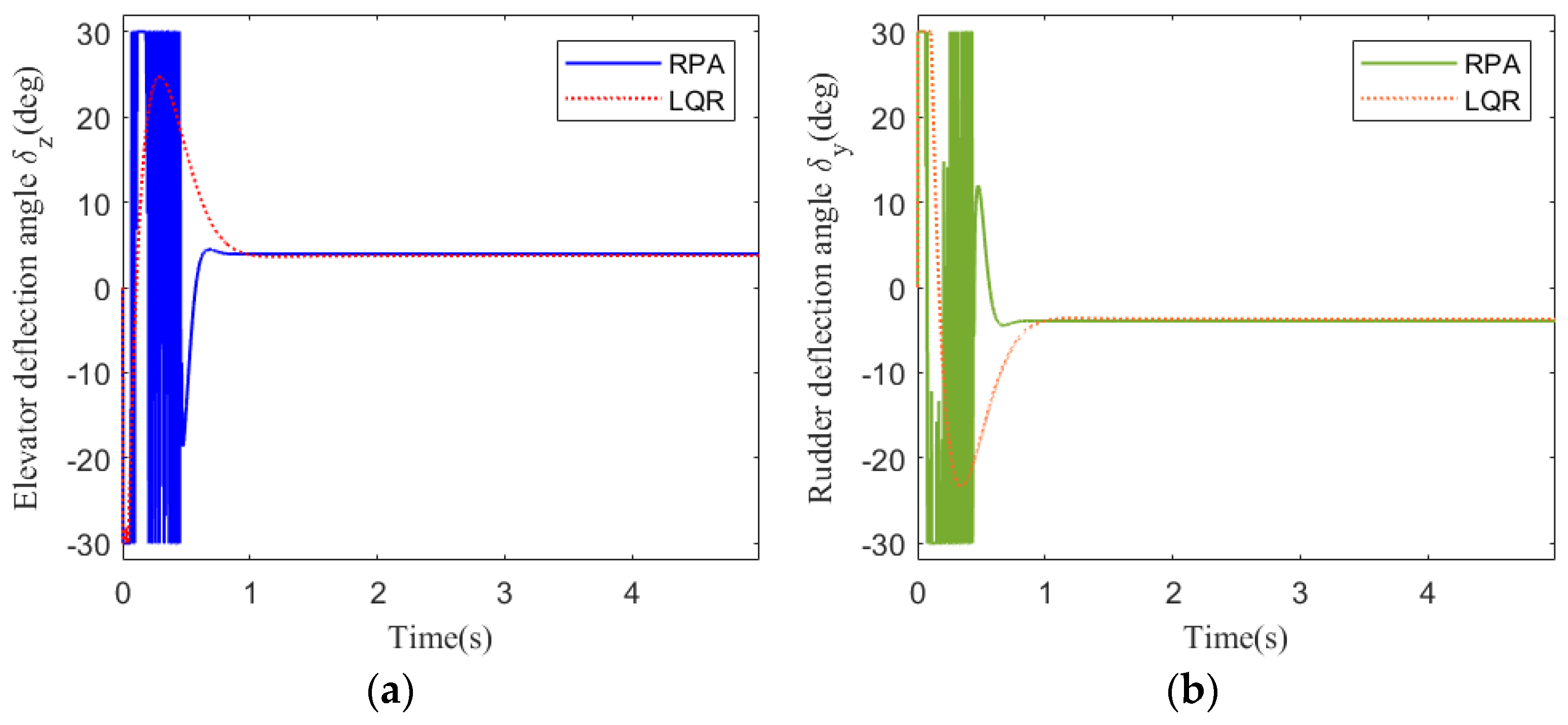
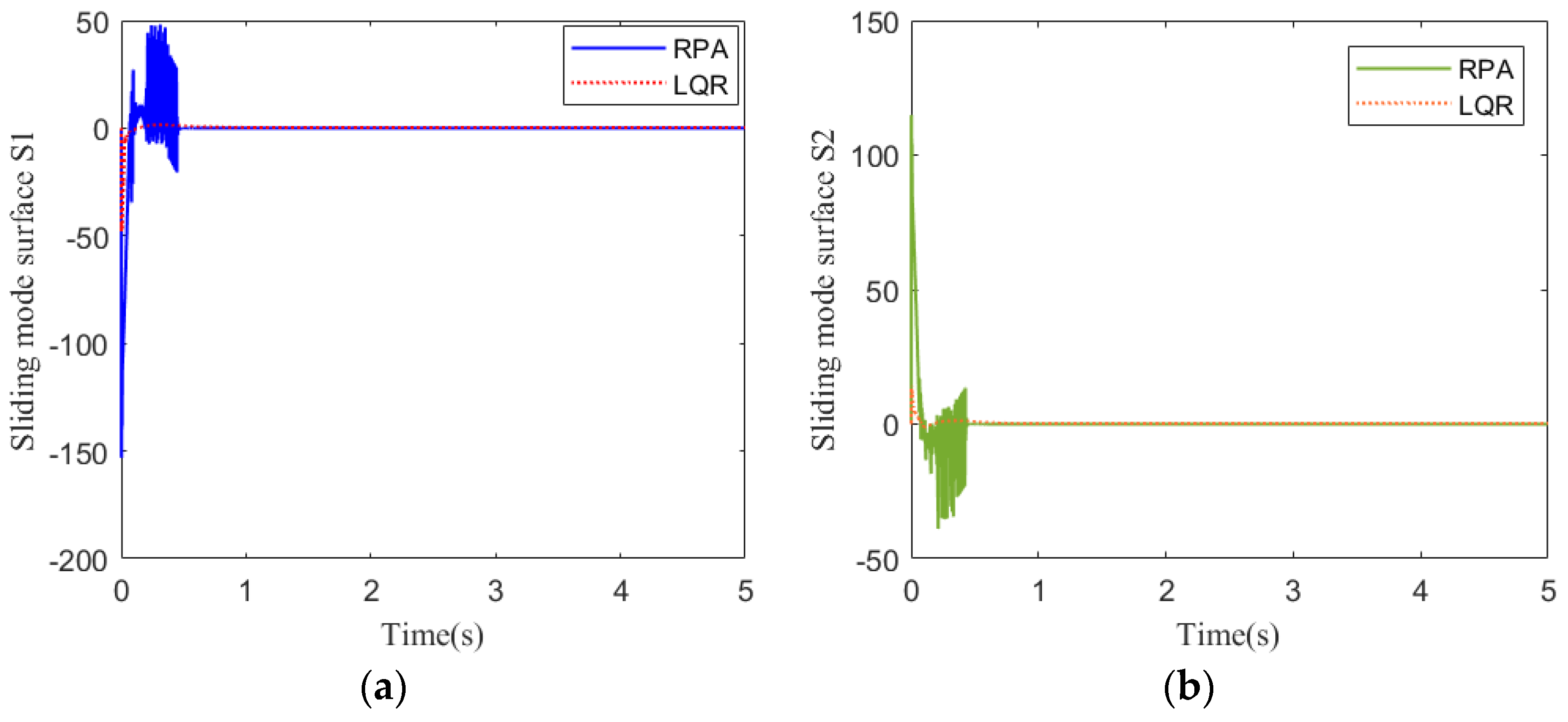
4. Simulation
4.1. Gain Scheduling Controller
4.2. Simulation Analysis
- (1)
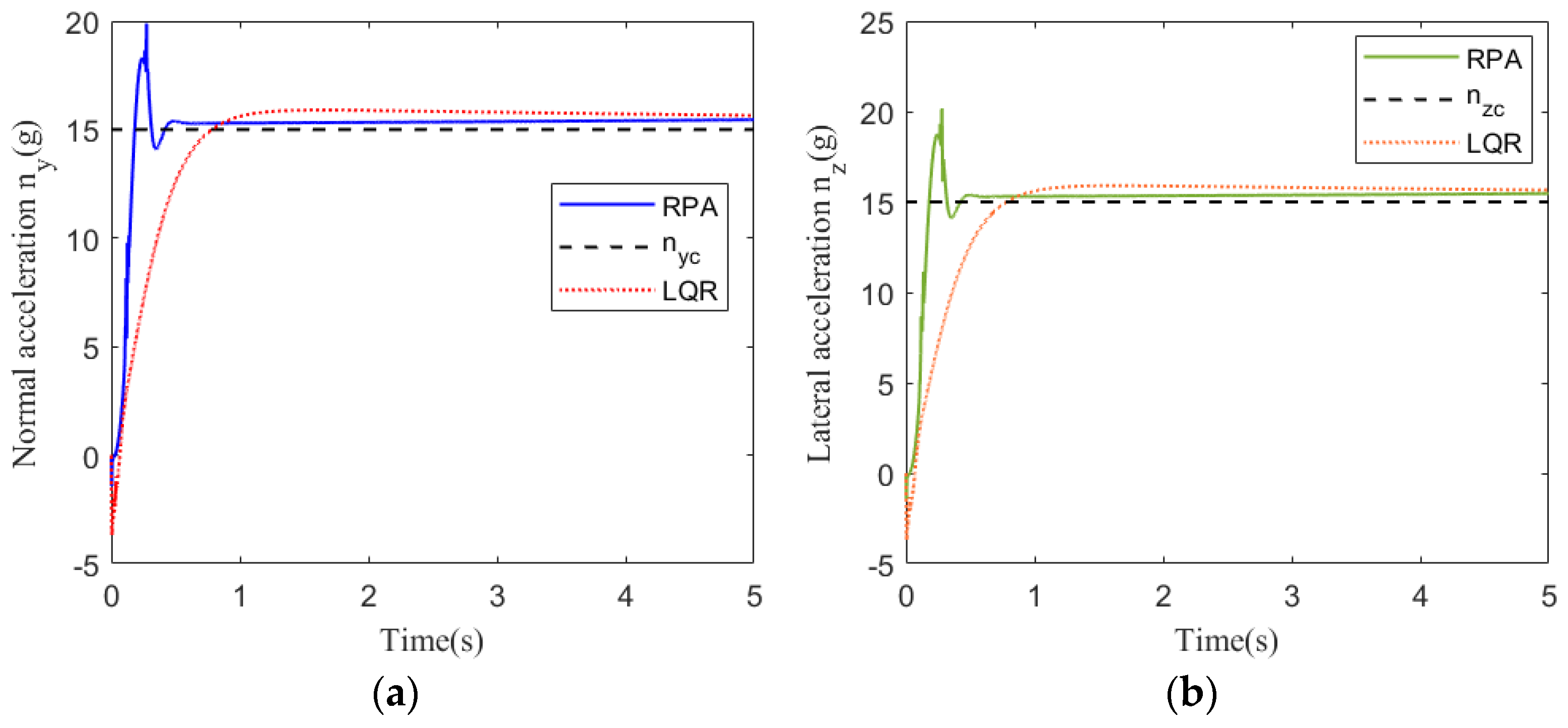
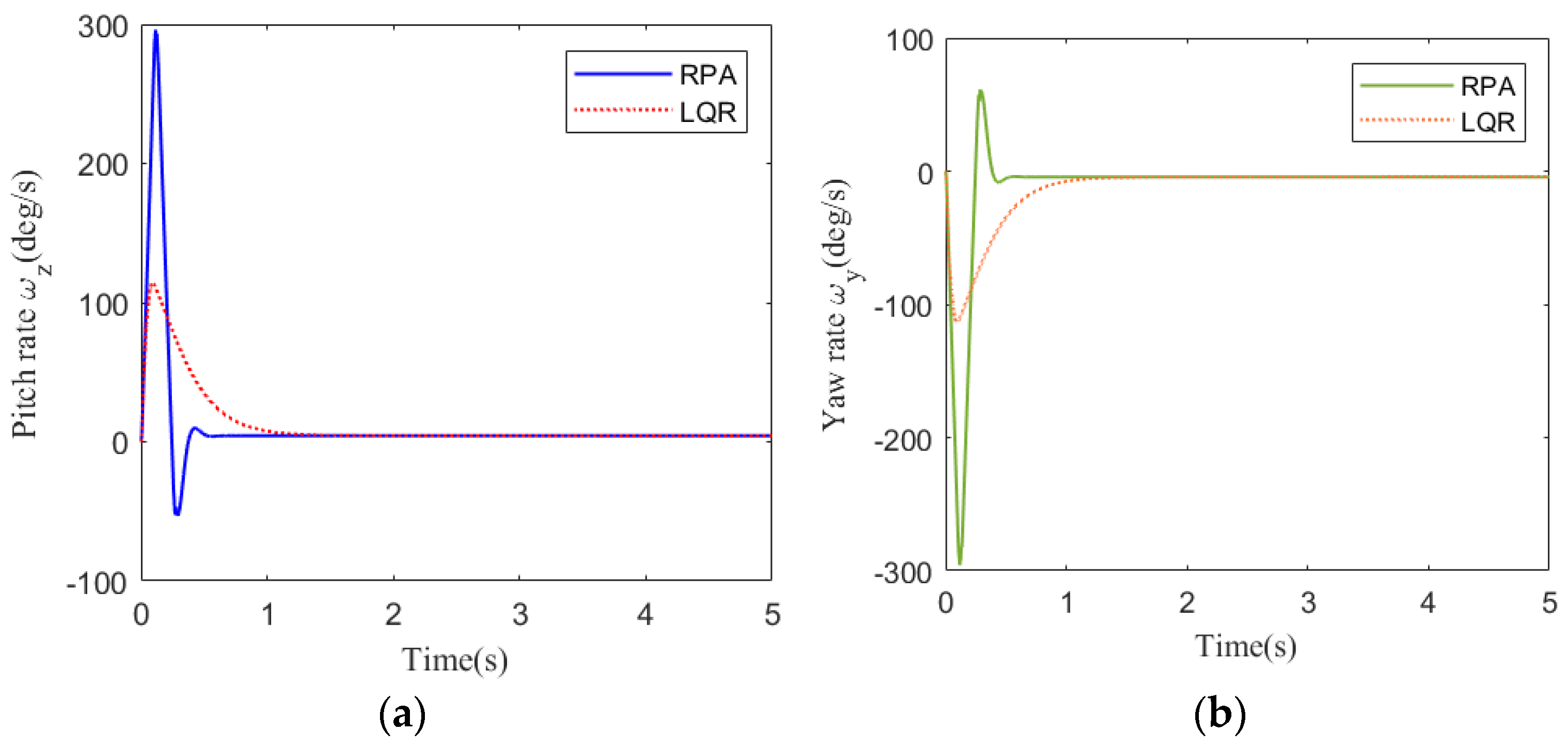
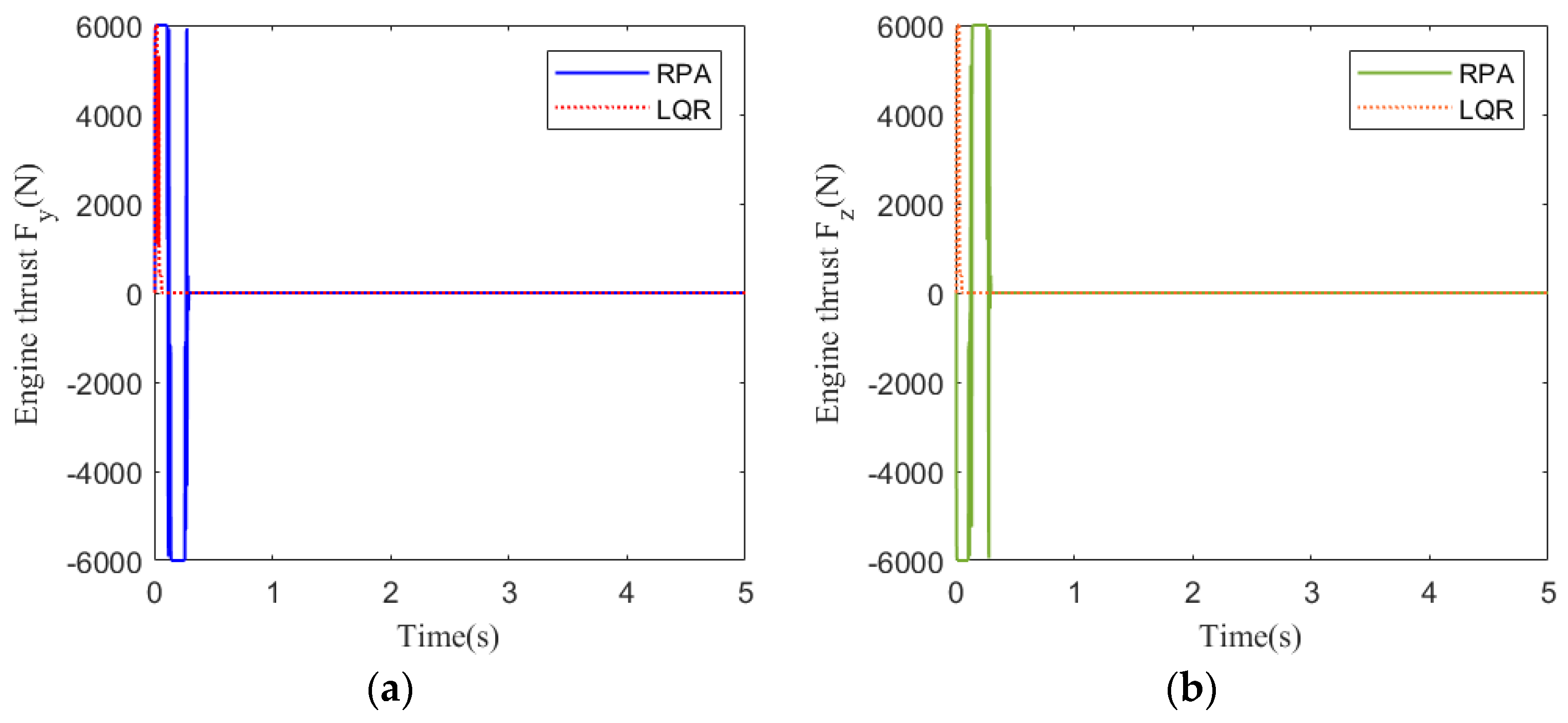
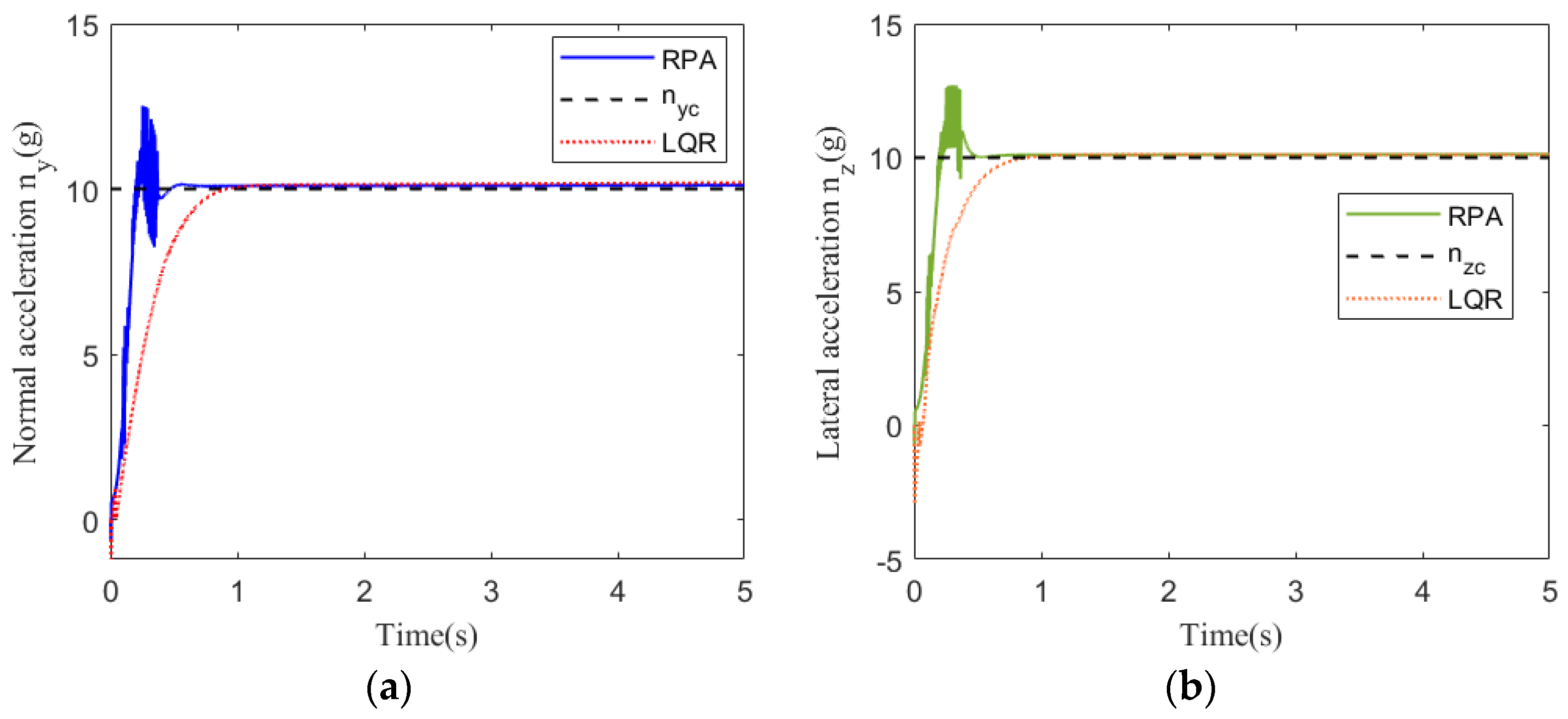
- Simulation at 25 km
- (2)
- Simulation at 30 km
- (3)
- Simulation at 35 km
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Boretti, A. Hydrogen hypersonic combined cycle propulsion: Advancements, challenges, and applications. Int. J. Hydrogen Energy 2024, 55, 394–399. [Google Scholar] [CrossRef]
- Zhu, C.; Xu, G.; Wei, C.; Cai, D.; Yu, Y. Impact-Time-Control Guidance Law for Hypersonic Missiles in Terminal Phase. IEEE Access 2020, 8, 44611–44621. [Google Scholar] [CrossRef]
- Bao, C.; Wang, P.; Tang, G. Integrated Guidance and Control for Hypersonic Morphing Missile Based on Variable Span Auxiliary Control. Int. J. Aerosp. Eng. 2019, 2019, 6413410. [Google Scholar] [CrossRef]
- Du, X.; Yang, Q.; Yang, H.; Bai, J.; Shi, Y. Infrared radiation characteristics of dagger-type hypersonic missile. Chin. J. Aeronaut. 2024, 37, 137–150. [Google Scholar] [CrossRef]
- Bai, C.; Lu, F.; Xu, X. Hybrid BTT/STT missile autopilot design. Aircr. Eng. Aerosp. Technol. 2017, 89, 809–814. [Google Scholar] [CrossRef]
- Ramteke, V.; Kothari, M. Accessibility Analysis and Robust Control Design of Dual-Spin Projectiles. J. Guid. Control Dyn. 2025, 48, 575–590. [Google Scholar] [CrossRef]
- Wang, Z.; Hao, Y. Reinforcement learning adaptive risk-sensitive fault-tolerant IGC method for a class of STT missile. Nonlinear Dyn. 2024, 112, 18195–18218. [Google Scholar] [CrossRef]
- Wang, Z.; Hao, Y.T.; Liu, J.L.; Bai, Y.F.; Yu, D.X. Reinforcement learning-adaptive fault-tolerant IGC method for a class of aircraft with non-affine characteristics and multiple uncertainties. Aeronaut. J. 2024, 129, 84–106. [Google Scholar] [CrossRef]
- Li, J.; Song, S.; Shi, X. Partial state-constrained integrated guidance and control for an STT missile with self-adjusting prescribed performance via non-barrier Lyapunov function method. Trans. Inst. Meas. Control, 2024; (early access). [Google Scholar] [CrossRef]
- Zou, Z.; Xu, P.; Chen, Y.; Yao, L.; Fu, C. Application of artificial intelligence in turbomachinery aerodynamics: Progresses and challenges. Artif. Intell. Rev. 2024, 57, 222. [Google Scholar] [CrossRef]
- Morlier, J.; Abu Salem, K.; Giuseppe, P.; Vittorio, C.; Vincenzo, B.; Davide, Z.; Mario, C.; Kokkolaras, M. Tools and methodologies for box-wing aircraft conceptual aerodynamic design and aeromechanic analysis. Mech. Ind. 2021, 22, 39. [Google Scholar] [CrossRef]
- Jiang, L.; Dong, C.; Pan, X.; Chen, G. Aerodynamic/control coupling optimization of reentry vehicle under wide speed range. Acta Mech. Sin. 2024, 41, 324259. [Google Scholar] [CrossRef]
- Rao, H.; Shi, Y.; Bai, J.; Chen, Y.; Yang, T.; Li, J. Aerodynamic Optimization Design of Supersonic Wing Based on Discrete Adjoint. Aerospace 2023, 10, 420. [Google Scholar] [CrossRef]
- Taufik, N.Z.B.; Zaidaan, S.; Mahmoud, E.; Abdallah, A.M.; Manaa, Z.; Abro, G.E.M.; Taib, M.N. Design and Analysis of the Effect of Trimmable Vertical Stabilizers for Enhanced Aircraft Maneuverability and Directional Stability. In Proceedings of the 2024 IEEE 12th Conference on Systems, Process & Control (ICSPC), Malacca, Malaysia, 7 December 2024; pp. 251–256. [Google Scholar]
- Yin, X.; Ma, H.; Zhang, W.; An, H.; Ke, Z.; Wang, L.; Nie, B. Analysis of the Controllability and Stability of a Hybrid High-Speed Compound Helicopter with a New Configuration. Int. J. Aeronaut. Space Sci. 2024, 25, 1205–1218. [Google Scholar] [CrossRef]
- Cockin, E.; Wang, X. Robust Sliding Mode Control for Air-to-Air Missile. arXiv 2024, arXiv:2411.06754. [Google Scholar]
- Hu, Y.; Yan, H.; Zhang, H.; Wang, M.; Zeng, L. Robust Adaptive Fixed-Time Sliding-Mode Control for Uncertain Robotic Systems With Input Saturation. IEEE Trans. Cybern. 2023, 53, 2636–2646. [Google Scholar] [CrossRef]
- Meng, Z.; Liu, L.; Duan, X.; Zhu, L.; Xu, Z. Compound control for electromagnetic linear load simulator. Trans. Inst. Meas. Control 2023, 45, 3020–3030. [Google Scholar] [CrossRef]
- Zhou, X.; Wang, W.; Shi, Y. Neural Network/PID Adaptive Compound Control Based on RBFNN Identification Modeling for an Aerial Inertially Stabilized Platform. IEEE Trans. Ind. Electron. 2024, 71, 16514–16522. [Google Scholar] [CrossRef]
- Lei, X.; Fu, F.; Wang, R. A High-Performance Compound Control Method for a Three-Axis Inertially Stabilized Platform under Multiple Disturbances. Symmetry 2022, 14, 1848. [Google Scholar] [CrossRef]
- Mu, Y.; Qi, L.; Sun, M.; Han, W. An Improved Deviation Coupling Control Method for Speed Synchronization of Multi-Motor Systems. Appl. Sci. 2024, 14, 5300. [Google Scholar] [CrossRef]
- Tang, Y.; Xu, W.; Liu, Y.; Dong, D. Dynamic Performance Enhancement Method Based on Improved Model Reference Adaptive System for SPMSM Sensorless Drives. IEEE Access 2021, 9, 135012–135023. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, H.; Li, M.; Liu, X. Speed Regulation and Optimization of Sensorless System of Permanent Magnet Synchronous Motor. Machines 2023, 11, 656. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Y.; Wang, M. Speed Fluctuation Suppression Strategy of Servo System with Flexible Load Based on Pole Assignment Fuzzy Adaptive PID. Mathematics 2022, 10, 3962. [Google Scholar] [CrossRef]
- Zhang, Z.; Wu, J.; Zheng, L.; Xie, D.; Tang, X. An Inductor Current Sensorless Control Strategy Based on Modified VSG Method for Single-Phase Microgrid Application With Seamless Transfer Capability. Front. Energy Res. 2021, 9, 752422. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, C.; Li, L.; Wang, Z.; Zong, X. Optimization of PID controller for stepper motor speed control system based on improved sparrow search algorithm. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2024, 238, 9397–9411. [Google Scholar] [CrossRef]
- He, C.; Zhou, D.; Sheng, W.; Xu, M.; Xi, Q.; Chen, Q. Fuzzy-PID controller design for RGV speed track based on improved PSO algorithm. J. Braz. Soc. Mech. Sci. Eng. 2024, 46, 669. [Google Scholar] [CrossRef]
- Zou, X.; Ding, H.; Li, J. Sliding mode speed control of permanent magnet synchronous motor based on improved reaching law. COMPEL—Int. J. Comput. Math. Electr. Electron. Eng. 2022, 42, 1335–1348. [Google Scholar] [CrossRef]
- Du, K.; Xiao, B.; Song, Z.; Xu, Y.; Tang, Z.; Xu, W.; Duan, H. A novel self-adaptation and sorting selection-based differential evolutionary algorithm applied to water distribution system optimization. J. Water Supply Res. Technol.-Aqua 2022, 71, 1068–1082. [Google Scholar] [CrossRef]
- Wang, M.; Wang, S.; Wang, H.; Li, C.; Qi, X.; Xu, J.; Xiao, Q. Functional waveform pulse variable speed stirring to improve mechanistic analysis and experimental study on the purification efficiency of zinc sulfate solution. Int. J. Chem. React. Eng. 2023, 21, 23–39. [Google Scholar] [CrossRef]
- Huang, C.-L.; Wu, C.-J.; Yang, S.-C. Full-Region Sensorless BLDC Drive for Permanent Magnet Motor Using Pulse Amplitude Modulation With DC Current Sensing. IEEE Trans. Ind. Electron. 2021, 68, 11234–11244. [Google Scholar] [CrossRef]
- Xu, W.; Zhang, J.; Wen, H. Robust anti-pitching control for high-speed multihull without velocity measurements. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2022, 237, 120–131. [Google Scholar] [CrossRef]
- Poureh, A.; Chamani, M.; Bahri, A. Nonlinear analysis of gain scheduled controllers for the NREL 5-MW turbine blade pitch control system. Int. J. Electr. Power Energy Syst. 2023, 145, 108578. [Google Scholar] [CrossRef]
- Li, P.; Li, P.; Zhao, J.; Zhang, B. Robust gain-scheduling static output-feedback H∞ control of vehicle lateral stability with heuristic approach. Inf. Sci. 2021, 546, 220–233. [Google Scholar] [CrossRef]
- Butkus, M.; Levišauskas, D.; Galvanauskas, V. Simple Gain-Scheduled Control System for Dissolved Oxygen Control in Bioreactors. Processes 2021, 9, 1493. [Google Scholar] [CrossRef]
- Liu, J.; Ma, Y.; Zhu, L.; Zhao, H.; Liu, H.; Yu, D. Improved Gain Scheduling Control and Its Application to Aero-Engine LPV Synthesis. Energies 2020, 13, 5967. [Google Scholar] [CrossRef]
- Bianchi, F.D.; Sánchez-Peña, R.S. A method for reducing implementation complexity in linear parameter-varying controllers. Automatica 2022, 146, 110588. [Google Scholar] [CrossRef]
- Zhang, K.; Zhou, B.; Jiang, H.; Duan, G. Event-triggered and self-triggered gain scheduling control of input constrained systems with applications to the spacecraft rendezvous. Int. J. Robust Nonlinear Control 2021, 31, 4629–4646. [Google Scholar] [CrossRef]
- Samia, C.; Houssem, J. The Use of a Heuristic Optimization Method to Improve the Design of a Discrete-time Gain Scheduling Control. Int. J. Control Autom. Syst. 2021, 19, 1836–1846. [Google Scholar] [CrossRef]
- Wang, Q.; Xue, A. Robust control for spacecraft rendezvous system with actuator unsymmetrical saturation: A gain scheduling approach. Int. J. Control 2017, 91, 1241–1250. [Google Scholar] [CrossRef]
- Li, Y.; Du, H.; He, Z.; Zhou, W. Robust Control for the IPT System With Parametric Uncertainty Using LMI Pole Constraints. IEEE Trans. Power Electron. 2020, 35, 1022–1035. [Google Scholar] [CrossRef]
- Xiong, S.; Chen, M.; Wei, Z. Tracking flight control of UAV based on multiple regions pole assignment method. Aerosp. Sci. Technol. 2022, 129, 107848. [Google Scholar] [CrossRef]





| Control Method | LQR (Linear Quadratic Regulator) | Sliding Mode Control (SMC) | Adaptive Control | Robust Control | Model Predictive Control (MPC) |
|---|---|---|---|---|---|
| Core Idea | Designs an optimal control law by minimizing a quadratic performance index (state error and control input). | Designs a sliding surface to drive the system state to the surface in finite time, with strong robustness to uncertainties and disturbances. | Estimates system parameters online and adjusts the control law to adapt to parameter variations and uncertainties. | Designs controllers by optimizing disturbance rejection performance (e.g., H∞ control) to enhance system robustness. | Predicts future system states based on the model and achieves control objectives by optimizing future control sequences. |
| Advantages |
|
|
|
|
|
| Disadvantages |
|
|
|
|
|
| Applicable Scenarios |
|
|
|
|
|
| Combination with Other Methods | Often combined with sliding mode control or robust control to enhance robustness. | Often combined with LQR to balance optimal performance and robustness. | Often combined with robust control to enhance robustness to unmodeled dynamics and disturbances. | Often combined with LQR to balance optimal performance and robustness. | Often combined with robust control to enhance robustness to uncertainties and disturbances. |
| Parameter | Value | Unit |
|---|---|---|
| 500 | kg | |
| 1963.4 | m/s | |
| 9.81 | m/s2 | |
| 0.0025 | s | |
| 0.001 | s | |
| 300 | Nms2 | |
| 300 | Nms2 | |
| 1.36 | m | |
| 6000 | N | |
| 400 | N | |
| 6000 | N | |
| 400 | N |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Zhou, D.; Zhang, Y. Design of Fast Response Compound Control System for Hypersonic Skid-to-Turn Missile. Symmetry 2025, 17, 504. https://doi.org/10.3390/sym17040504
Wang H, Zhou D, Zhang Y. Design of Fast Response Compound Control System for Hypersonic Skid-to-Turn Missile. Symmetry. 2025; 17(4):504. https://doi.org/10.3390/sym17040504
Chicago/Turabian StyleWang, Huan, Di Zhou, and Yiqun Zhang. 2025. "Design of Fast Response Compound Control System for Hypersonic Skid-to-Turn Missile" Symmetry 17, no. 4: 504. https://doi.org/10.3390/sym17040504
APA StyleWang, H., Zhou, D., & Zhang, Y. (2025). Design of Fast Response Compound Control System for Hypersonic Skid-to-Turn Missile. Symmetry, 17(4), 504. https://doi.org/10.3390/sym17040504






