Viscous Dissipation and Mixed Convection Effects on the Induced Magnetic Field for Peristaltic Flow of a Jeffrey Nanofluid
Abstract
1. Introduction
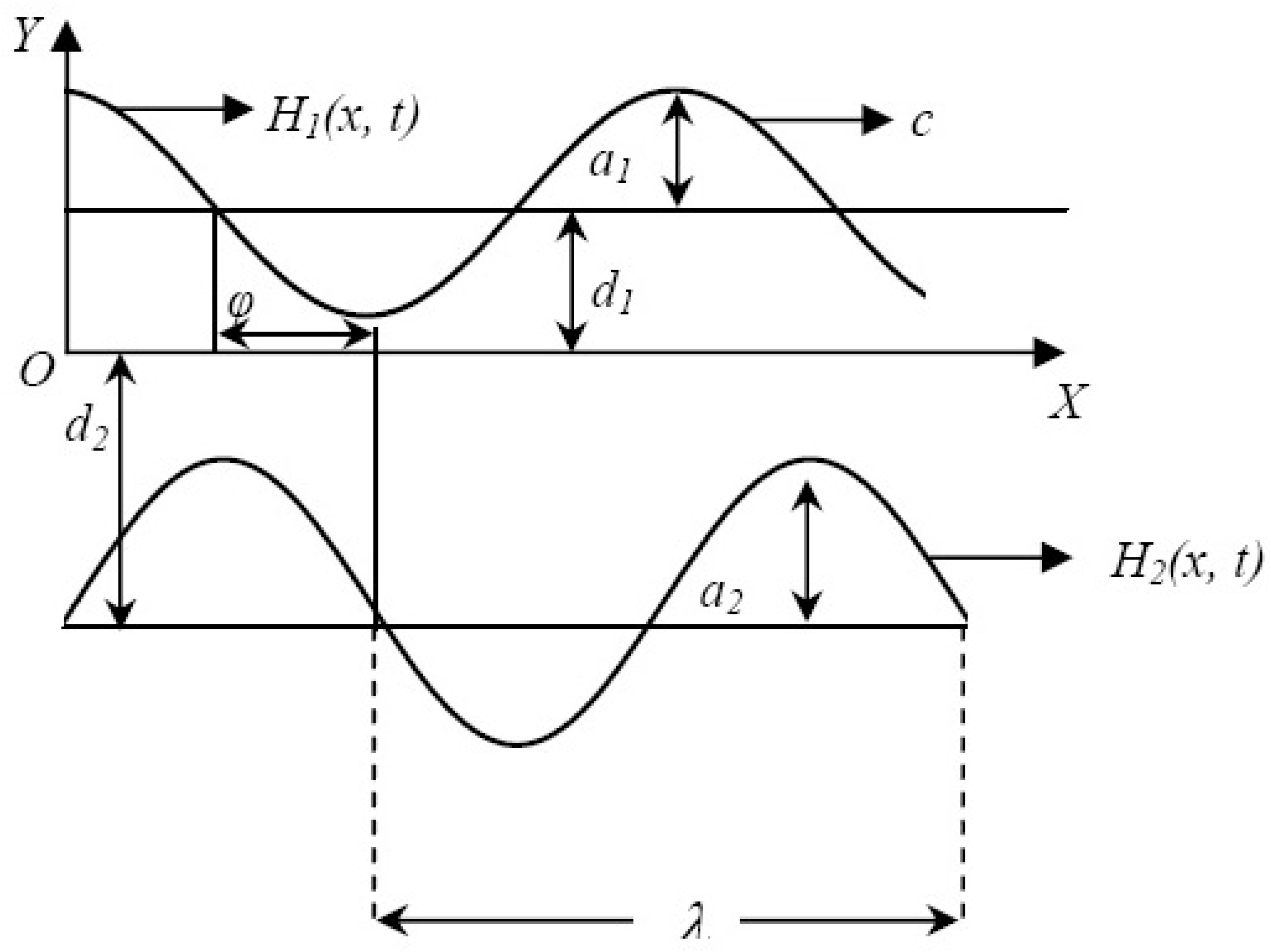
2. Problem Formulation
3. Numerical Solutions and Discussion
3.1. Validation of the Model
3.2. Axial Velocity Profile
3.3. Temperature Distribution Profile
3.4. Nanoparticle Concentration Distribution Profile
3.5. Axially Induced Magnetic Field Profile
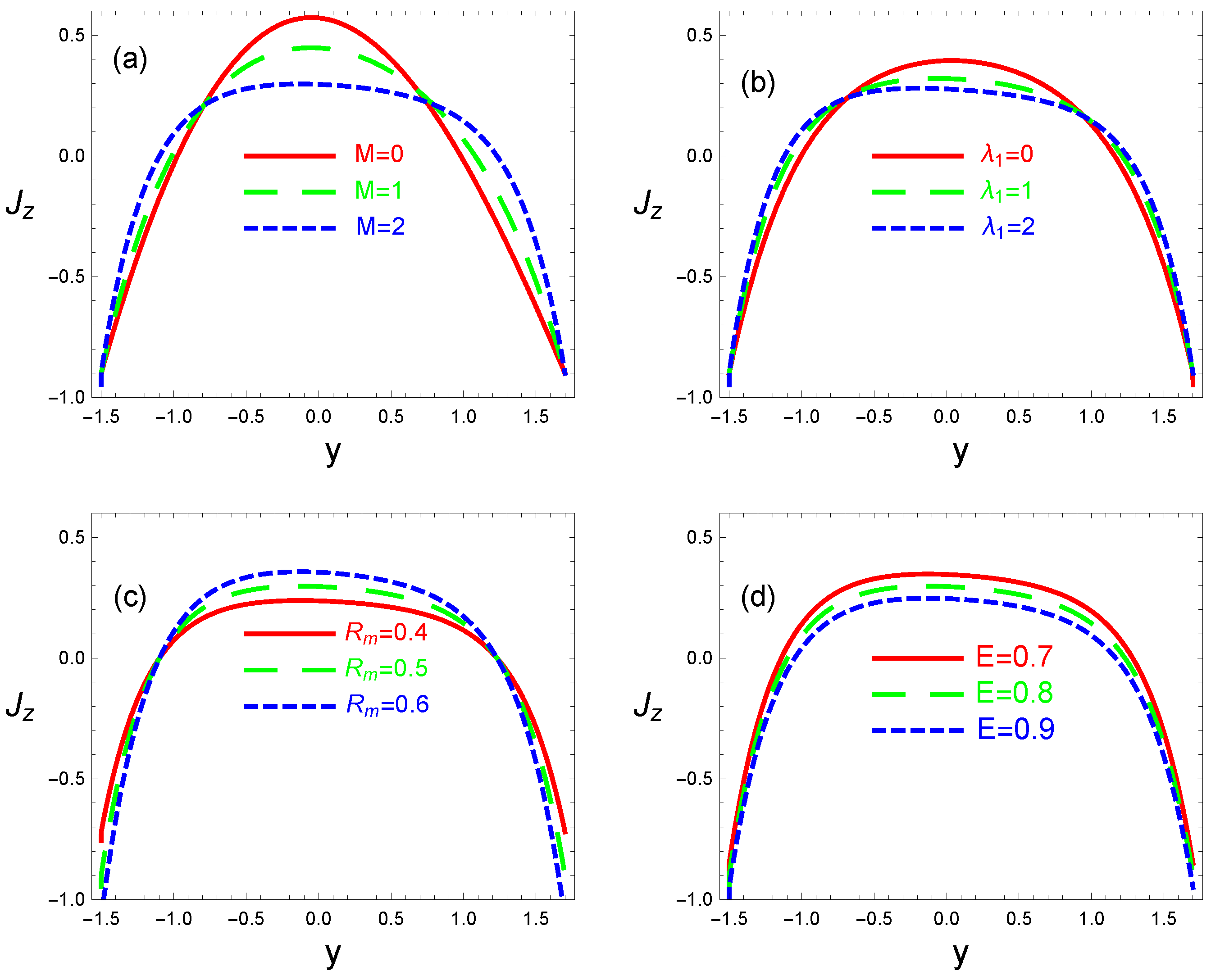
3.6. Current Density Distribution Profile
4. Conclusions
- (a)
- With an increase in M, the axial velocity increases close to the channel wall while decreasing toward the channel’s center.
- (b)
- The axial velocity magnitude increases toward the upper wall of the channel as increases, while it decreases close to the lower wall.
- (c)
- The effects of and on the temperature profile are opposite to each other.
- (d)
- When M is raised, the concentration of nanoparticles rises; when is raised, the concentration falls.
- (e)
- The axially induced magnetic field is increasingly influenced as increases.
- (f)
- The distribution of current density decreases as E increases.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a | traveling wave amplitude of upper wave | magnetic Reynolds number | |
| , | amplitudes of upper and lower waves | S | Stommer number |
| b | traveling wave amplitude of lower wave | extra-stress tensor | |
| Brinkman number | t | time | |
| c | speed of peristaltic wave | T | temperature of the fluid |
| volumetric volume expansion coefficient | temperatures at the upper and lower walls, respectively | ||
| C | volume fraction of nanoparticles | dimensionless velocities in the moving frame | |
| mass concentration at the upper and lower walls | velocity components in the fixed frame | ||
| specific heat under constant pressure | velocity vector | ||
| d | dimensionless width of the channel | coordinate system in the moving frame | |
| coefficient of Brownian motion parameter | coordinate system in the fixed frame | ||
| coefficient of thermophoretic diffusion parameter | thermal expansion parameter | ||
| electric field strength vector | wavelength | ||
| Eckert number | relaxation-to-retardation-times ratio | ||
| g | acceleration | retardation time | |
| local Grashof number | phase difference | ||
| dimensionless upper and lower walls, respectively | magnetic force function | ||
| components of induced magnetic field | density of the fluid | ||
| whole magnetic field vector | viscosity coefficient | ||
| induced magnetic field vector | permeability of magnetism | ||
| constant intensity of magnetic field | dynamic viscosity parameter | ||
| upper and lower walls, respectively | dimensionless wave number | ||
| identity tensor | dimensionless temperature | ||
| M | Hartmann number | shear rate | |
| Brownian motion parameter | Cauchy stress tensor for Jeffrey fluid | ||
| thermophoresis parameter | inverse of magnetic diffusivity | ||
| P | pressure | mass concentration | |
| Prandtl number | heat capacity of base fluid | ||
| local nanoparticle Grashof number | intrinsic heat capacity of particles | ||
| Reynolds number |
References
- Mekheimer, K.S. Effect of induced magnetic field on peristaltic flow of a couple stress fluid. Phys. Lett. A 2008, 372, 4271–4278. [Google Scholar] [CrossRef]
- Mekheimer, K.S. Peristaltic flow of a magneto-micropolar fluid: Effect of induced magnetic field. J. Appl. Math. 2008, 23, 570825. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, Y.; Mekheimer, M.S.; Ali, N. Effect of an induced magnetic field on the peristaltic flow of a third order fluid. Numer. Methods Partial. Differ. Equ. 2010, 26, 345–366. [Google Scholar] [CrossRef]
- Hayat, T.; Saleem, N.; Asghar, S.; Alhothuali, M.S.; Alhomaidan, A. Influence of induced magnetic field and heat transfer on peristaltic transport of a Carreau fluid. Commun. Nonlinear Sci. Numer. Simulat. 2011, 16, 3559–3577. [Google Scholar] [CrossRef]
- elmaboud, Y.A. Influence of induced magnetic field on peristaltic flow in an annulus. Commun. Nonlinear Sci. Numer. Simulat. 2012, 17, 685–698. [Google Scholar] [CrossRef]
- Akram, S.; Nadeem, S.; Hanif, M. Numerical and analytical treatment on peristaltic flow of Williams on fluid in the occurrence of induced magnetic field. J. Magn. Magn. Mater. 2013, 346, 142–151. [Google Scholar] [CrossRef]
- Akram, S.; Nadeem, S. Influence of induced magnetic field and heat transfer on the peristaltic motion of a Jeffrey fluid in an asymmetric channel: Closed form solutions. J. Magn. Magn. Mater. 2013, 328, 11–20. [Google Scholar] [CrossRef]
- Mustafa, M.; Hina, S.; Hayat, T.; Ahmad, B. Influence of induced magnetic field on the peristaltic flow of nanofluid. Meccanica 2014, 49, 521–534. [Google Scholar] [CrossRef]
- Hayat, T.; Nisar, Z.; Ahmad, B.; Yasmin, H. Simultaneous effects of slip and wall properties on MHD peristaltic motion of nanofluid with Joule heating. J. Magn. Magn. Mater. 2015, 395, 48–58. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Hayatb, T.; Alsaedi, A. Peristaltic transport of magneto-nanoparticles submerged in water: Model for drug delivery system. Phys. E 2015, 68, 123–132. [Google Scholar] [CrossRef]
- Kothandapani, M.; Prakash, J. Effect of radiation and magnetic field on peristaltic transport of nanofluids through a porous space in a tapered asymmetric channel. J. Magn. Magn. Mater. 2015, 378, 152–163. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Hayat, T.; Alsaedi, A. Numerical analysis for MHD peristaltic transport of Carreau-Yasuda fluid in a curved channel with Hall effects. J. Magn. Magn. Mater. 2015, 382, 104–110. [Google Scholar] [CrossRef]
- Hayat, T.; Bibi, S.; Rafiq, M.; Alsaedi, A.; Abbasi, F.M. Effect of an inclined magnetic field on peristaltic flow of Williams on fluid in an inclined channel with convective conditions. J. Magn. Magn. Mater. 2016, 401, 733–745. [Google Scholar] [CrossRef]
- Hayat, T.; Quratulain; Rafiq, M.; Alsaadi, F.; Ayub, M. Soret and Dufour effects on peristaltic transport in curved channel with radial magnetic field and convective conditions. J. Magn. Magn. Mater. 2016, 405, 358–369. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Ahmad, B.; Shehzad, S.A. Impact of magnetic field in three-dimensional flow of Sisko nanofluid with convective condition. J. Magn. Magn. Mater. 2016, 413, 1–8. [Google Scholar] [CrossRef]
- Hayat, T.; Shafique, M.; Tanveer, A.; Alsaedi, A. Hall and ion slip effects on peristaltic flow of Jeffrey nanofluid with Joule heating. J. Magn. Magn. Mater. 2016, 407, 51–59. [Google Scholar] [CrossRef]
- Nowar, K. Effect of Induced Magnetic Field on Peristaltic Transport of a nanofluid in an asymmetric channel: Closed form Solution. Appl. Math. Inf. Sci. 2017, 11, 373–381. [Google Scholar] [CrossRef]
- Kavya, S.; Nagendramma, V.; Ahammad, N.A.; Ahmad, S.; Raju, C.S.K.; Shah, N.A. Magnetic-hybrid nanoparticles with stretching/shrinking cylinder in a suspension of MoS4 and copper nanoparticles. Int. Commun. Heat Mass Transf. 2022, 136, 106150. [Google Scholar] [CrossRef]
- Matias, A.F.V.; Coelho, R.C.V.; Andrade, J.S.J.; Araújo, N.A.M. Flow through time–evolving porous media: Swelling and erosion. J. Comput. Sci. 2021, 53, 101360. [Google Scholar] [CrossRef]
- Matias, A.F.V.; Matias, D.F.V.; Neng, N.R.; Nogueira, J.M.F.; Andrade, J.S.J.; Coelho, R.C.V.; Araújo, N.A.M. Continuum model for extraction and retention in porous media. Phys. Fluids 2023, 35, 123611. [Google Scholar] [CrossRef]



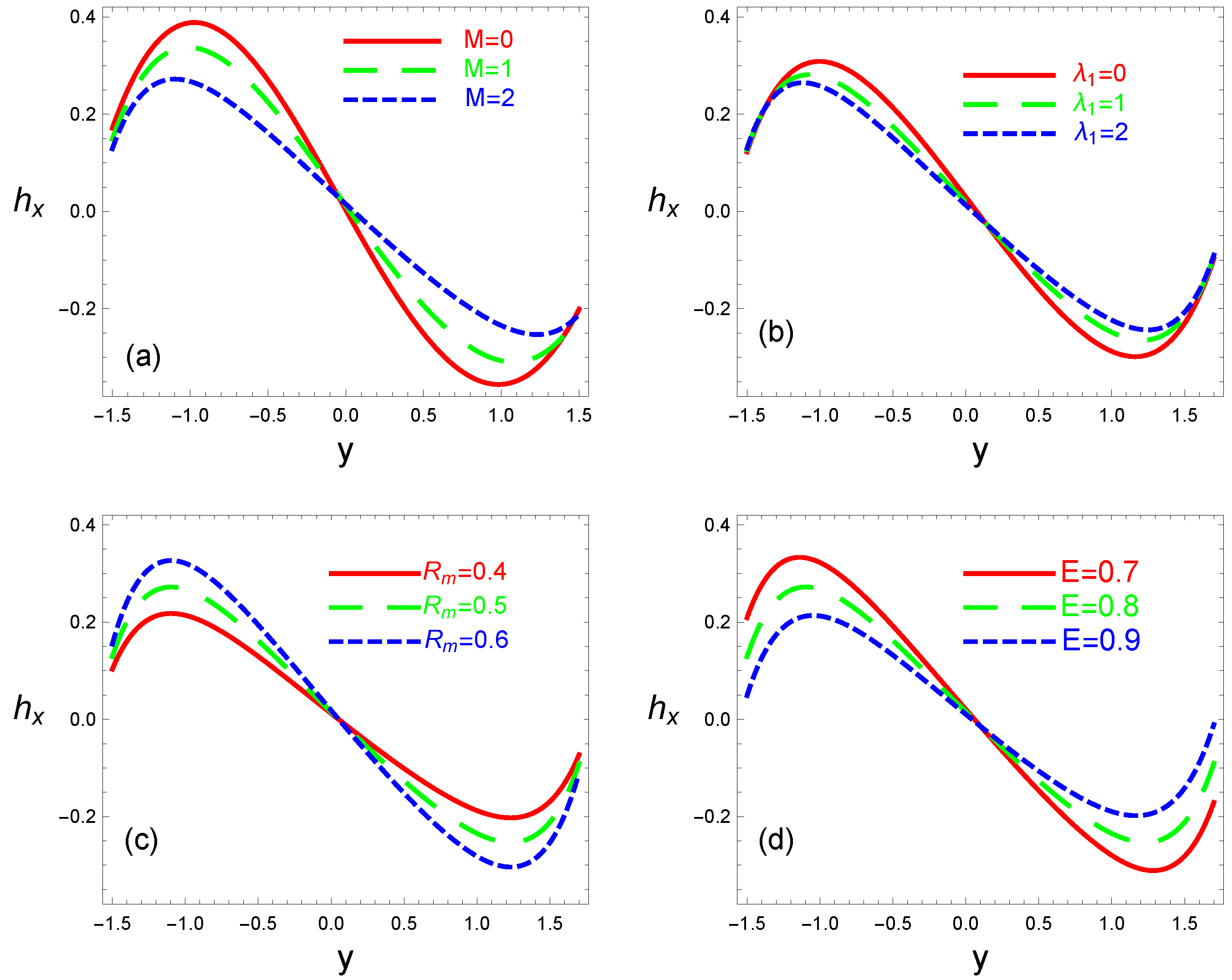
| y | Current Study | Akram et al. [7] |
|---|---|---|
| −1 | 0.48996 | 0.03 |
| −0.5 | 1.32663 | 0.6 |
| 0 | 1.49879 | 0.8 |
| 0.5 | 1.3595 | 0.82 |
| 1 | 0.842943 | 0.72 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Halouani, B.; Nowar, K. Viscous Dissipation and Mixed Convection Effects on the Induced Magnetic Field for Peristaltic Flow of a Jeffrey Nanofluid. Symmetry 2024, 16, 329. https://doi.org/10.3390/sym16030329
Halouani B, Nowar K. Viscous Dissipation and Mixed Convection Effects on the Induced Magnetic Field for Peristaltic Flow of a Jeffrey Nanofluid. Symmetry. 2024; 16(3):329. https://doi.org/10.3390/sym16030329
Chicago/Turabian StyleHalouani, Borhen, and Khalid Nowar. 2024. "Viscous Dissipation and Mixed Convection Effects on the Induced Magnetic Field for Peristaltic Flow of a Jeffrey Nanofluid" Symmetry 16, no. 3: 329. https://doi.org/10.3390/sym16030329
APA StyleHalouani, B., & Nowar, K. (2024). Viscous Dissipation and Mixed Convection Effects on the Induced Magnetic Field for Peristaltic Flow of a Jeffrey Nanofluid. Symmetry, 16(3), 329. https://doi.org/10.3390/sym16030329








