Dynamical Behavior of Small-Scale Buoyant Diffusion Flames in Externally Swirling Flows
Abstract
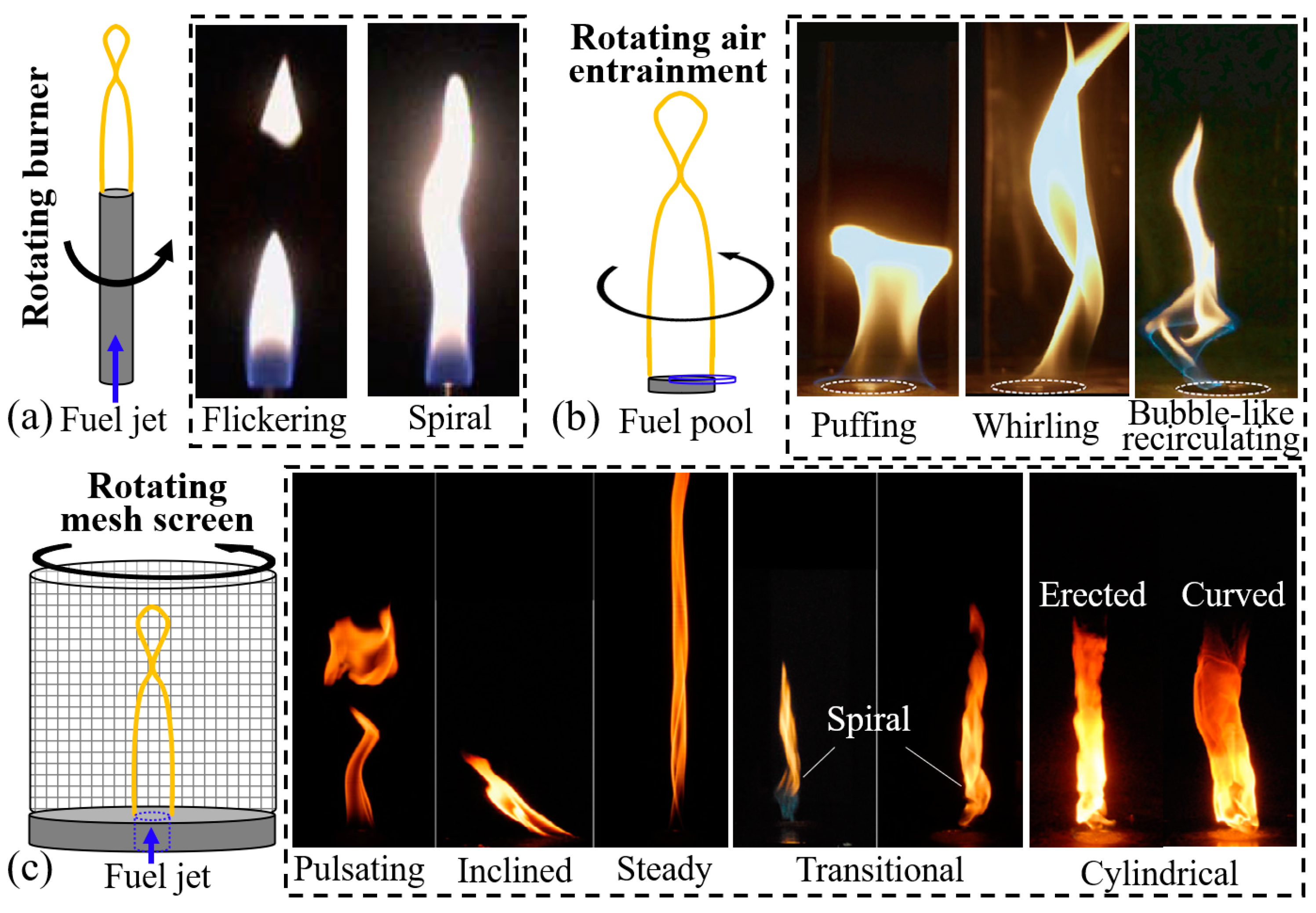
1. Introduction
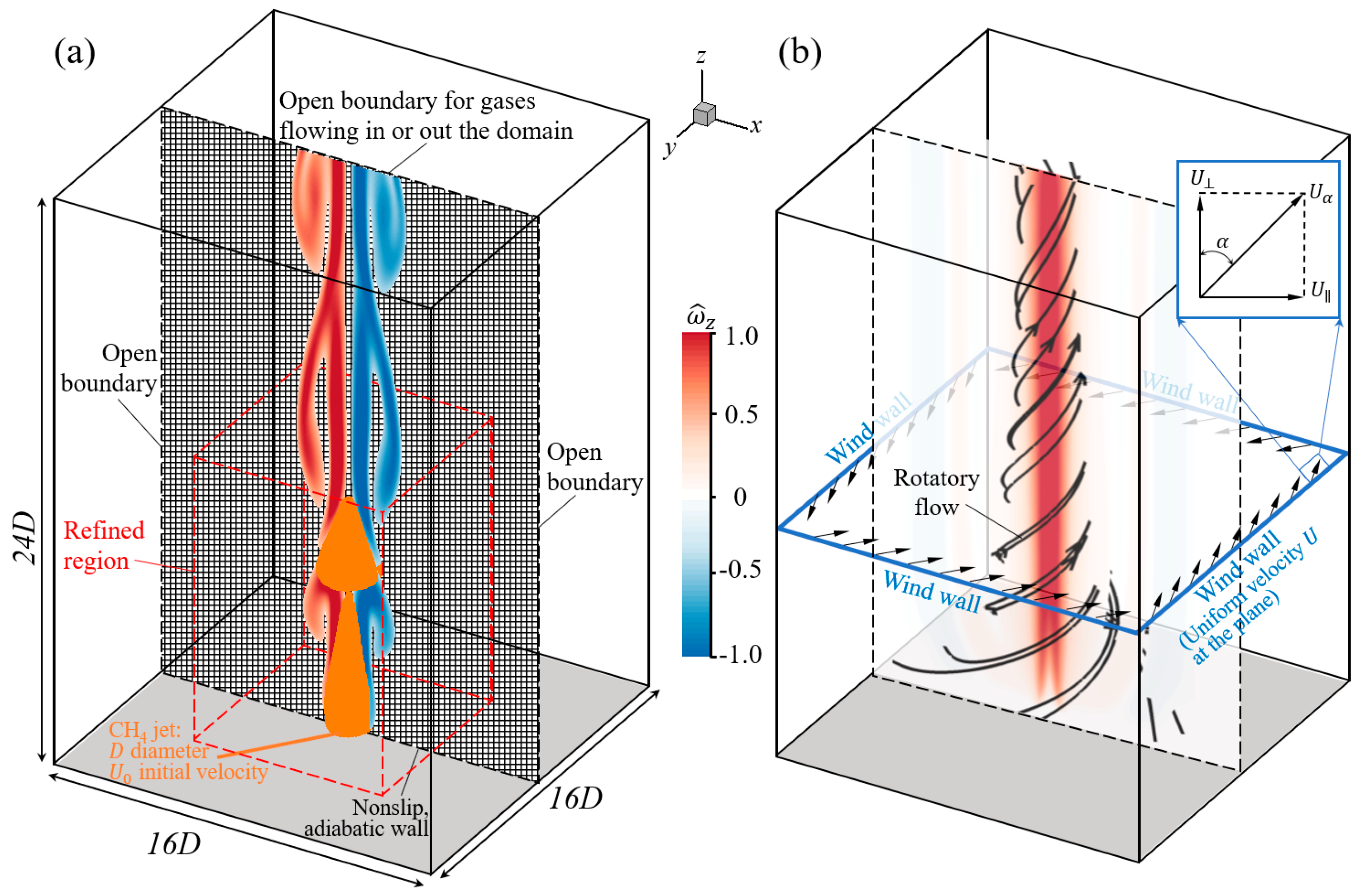
2. Computational Methodology
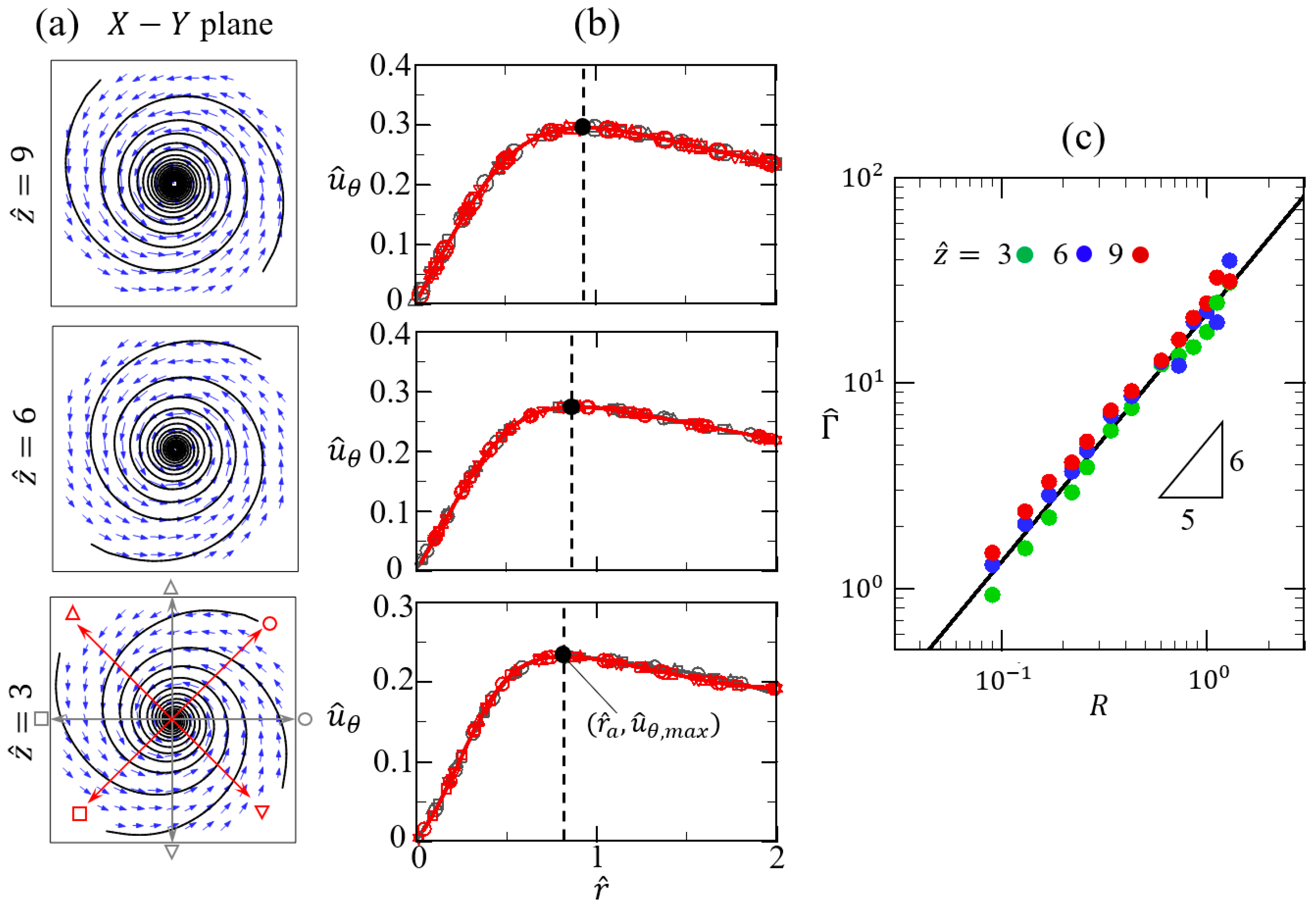
3. Results and Discussion
- How finite rate chemistry affects a flickering buoyant diffusion flame;
- How a flickering buoyant diffusion flame exhibits when the surrounding airflow is swirling;
- Why the flame flicker vanishes once the swirling intensity increases to a certain degree;
- What the vortex-dynamical interpretations for the flame variation under different swirling conditions are.
3.1. Finite Rate Chemistry Effects on Flame Flicker
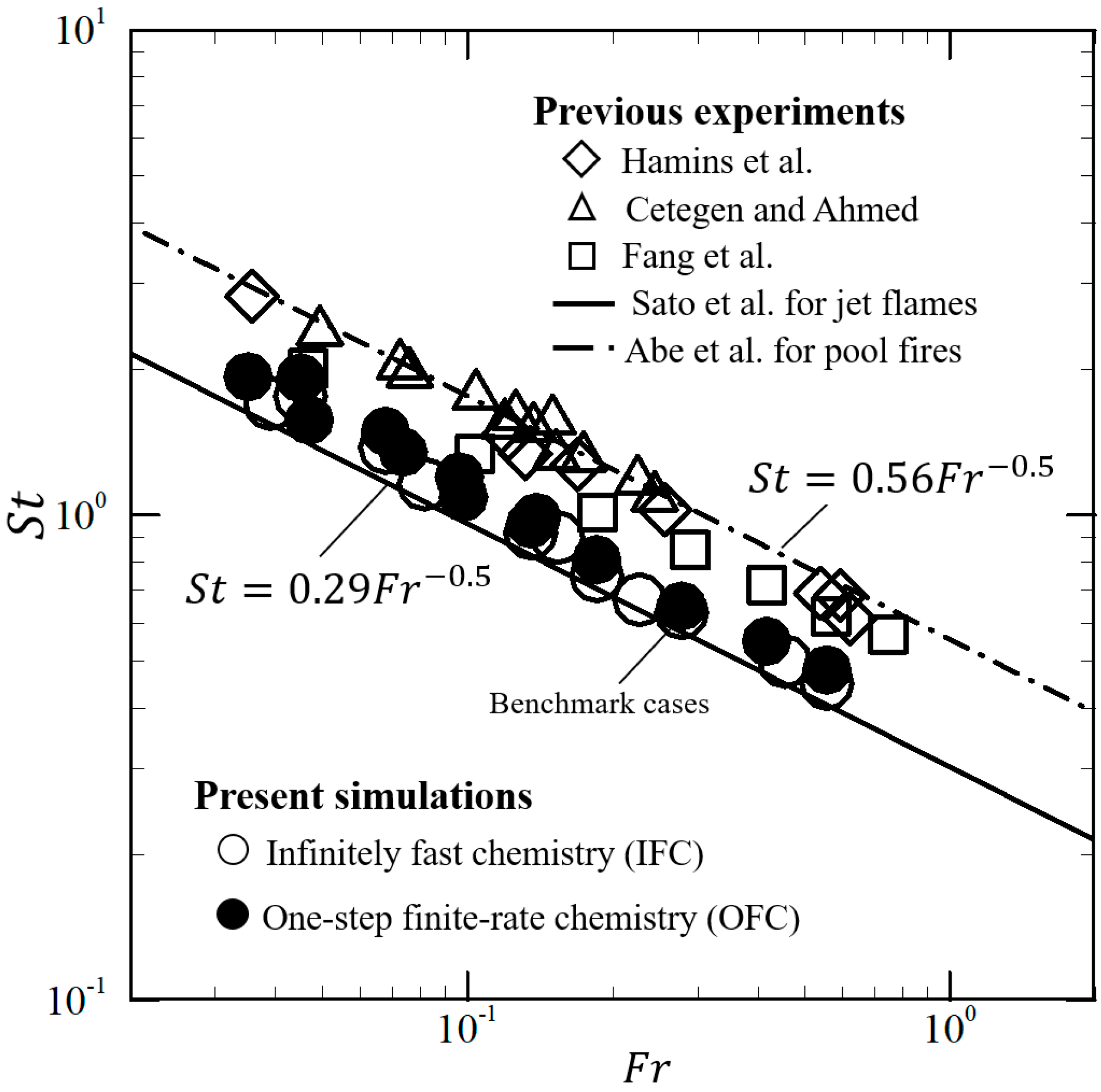
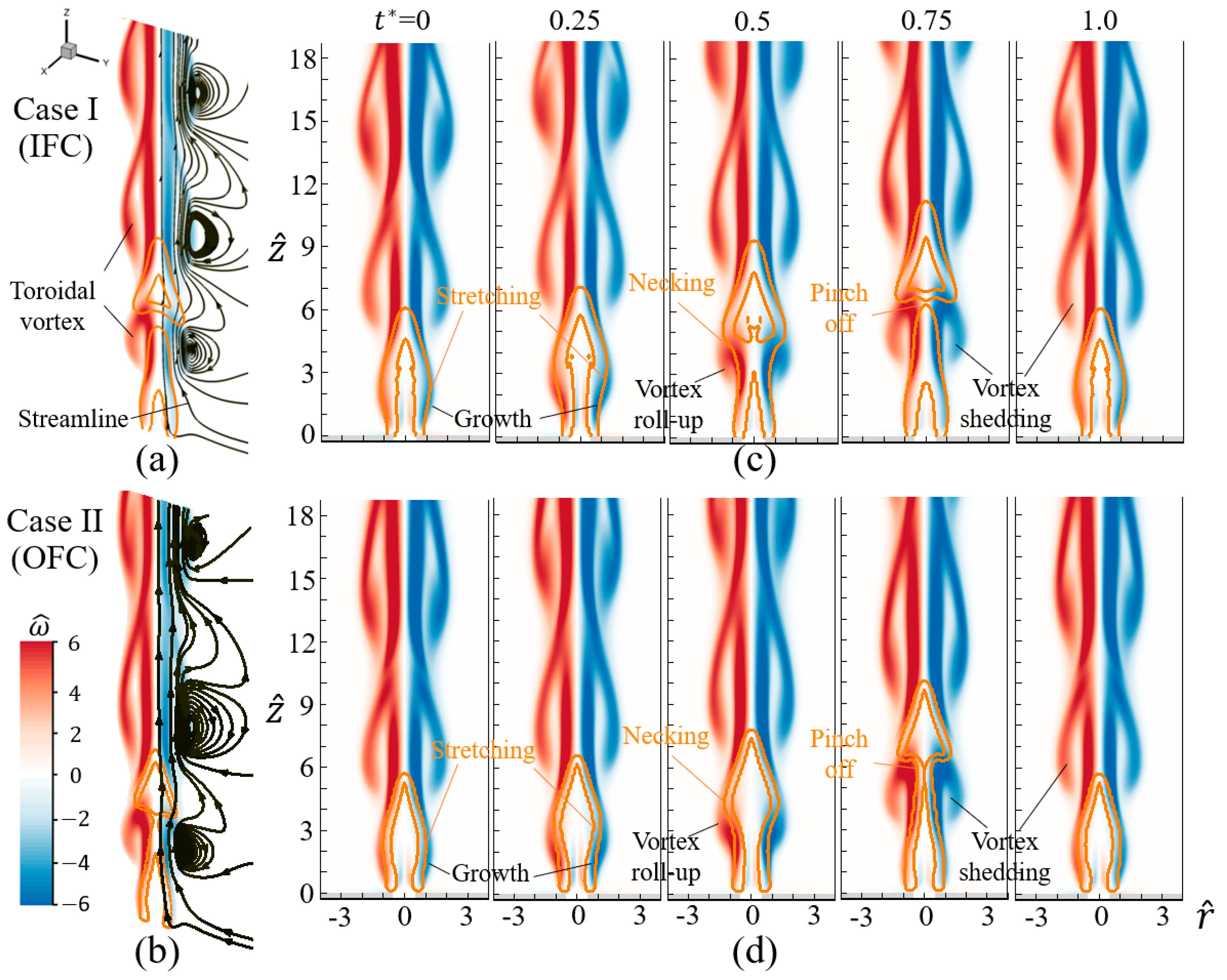
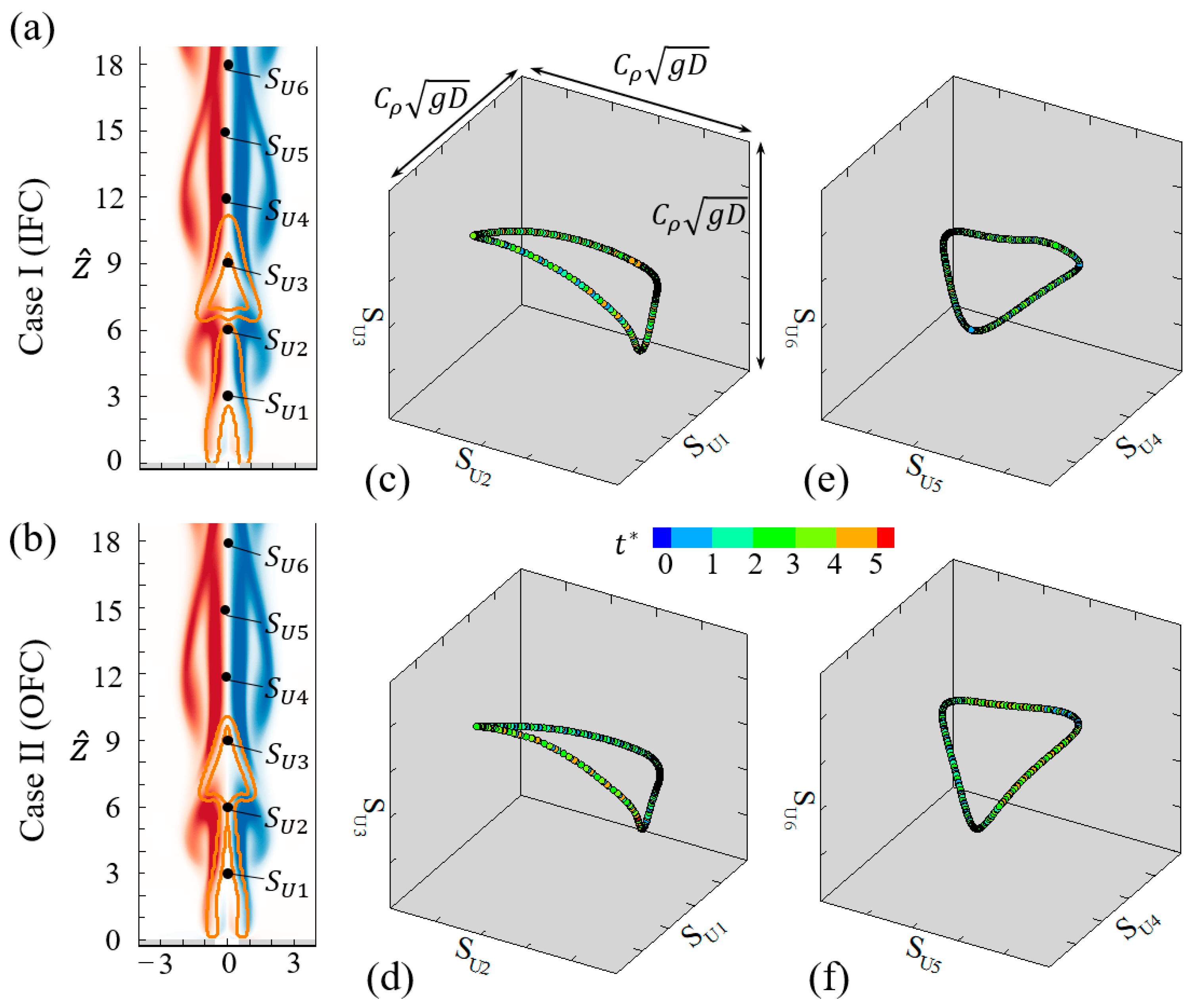
3.2. Flickering Flames: Benchmark Cases
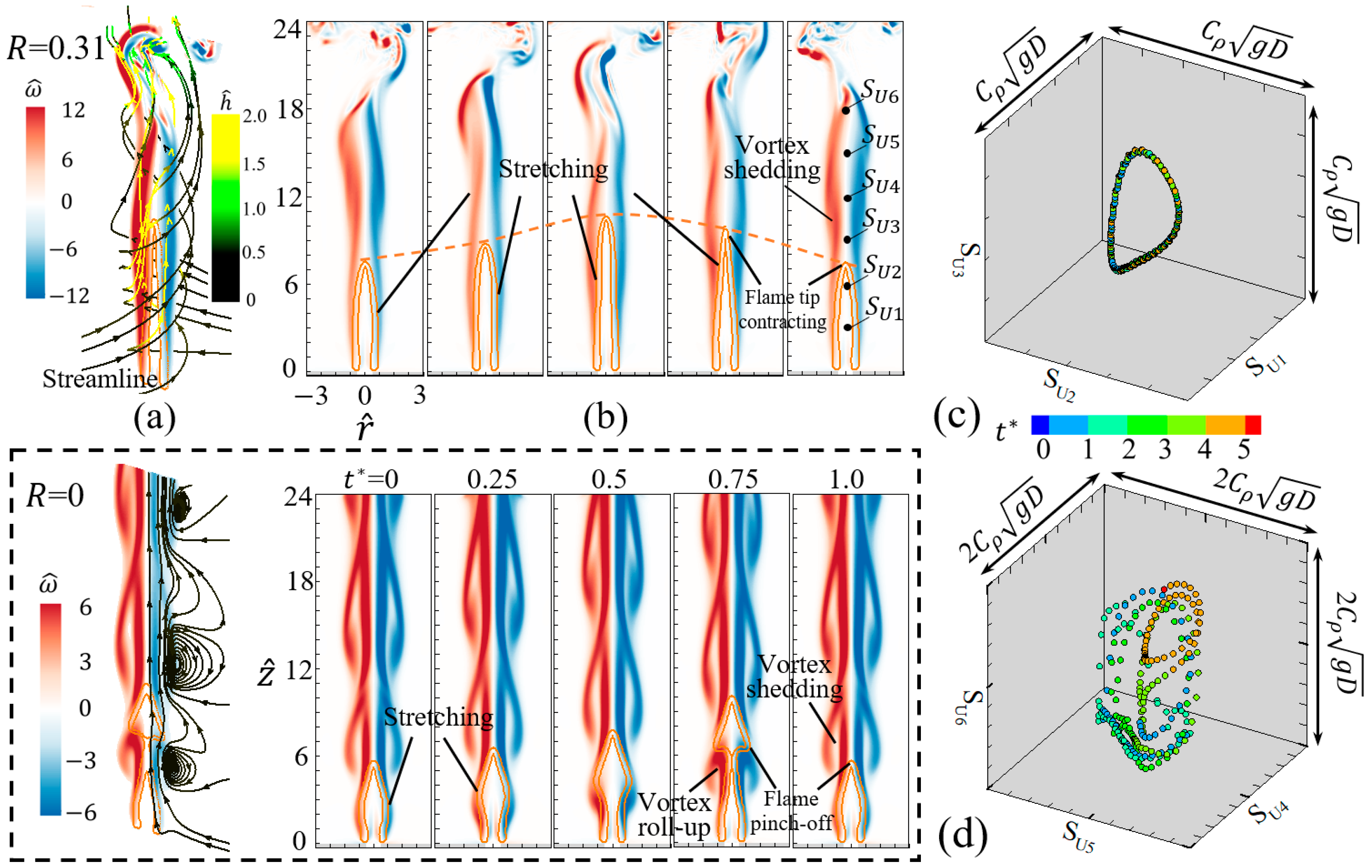
3.3. Faster Flickering Flames
3.4. Oscillating Flames
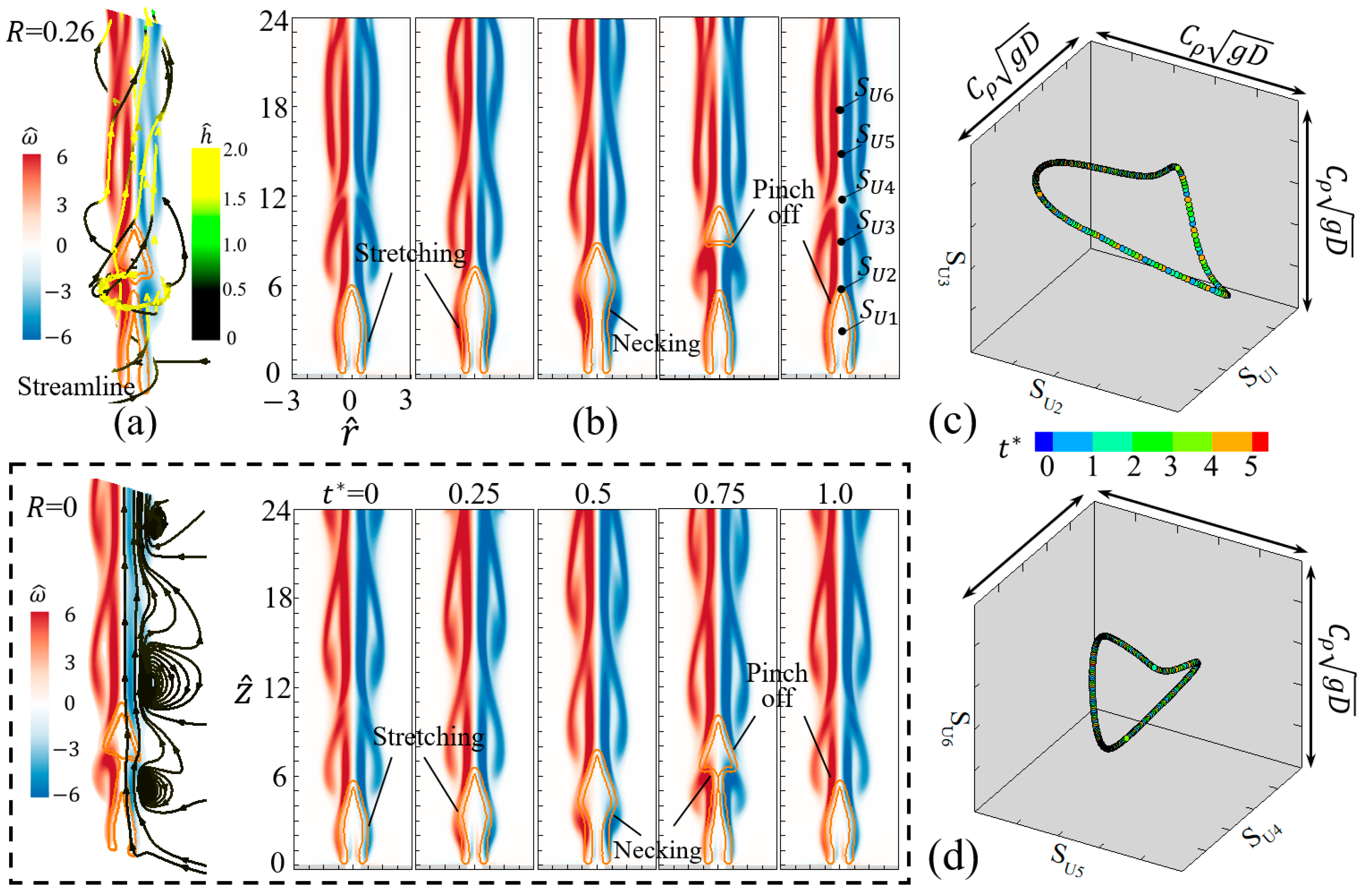
3.5. Steady Flames
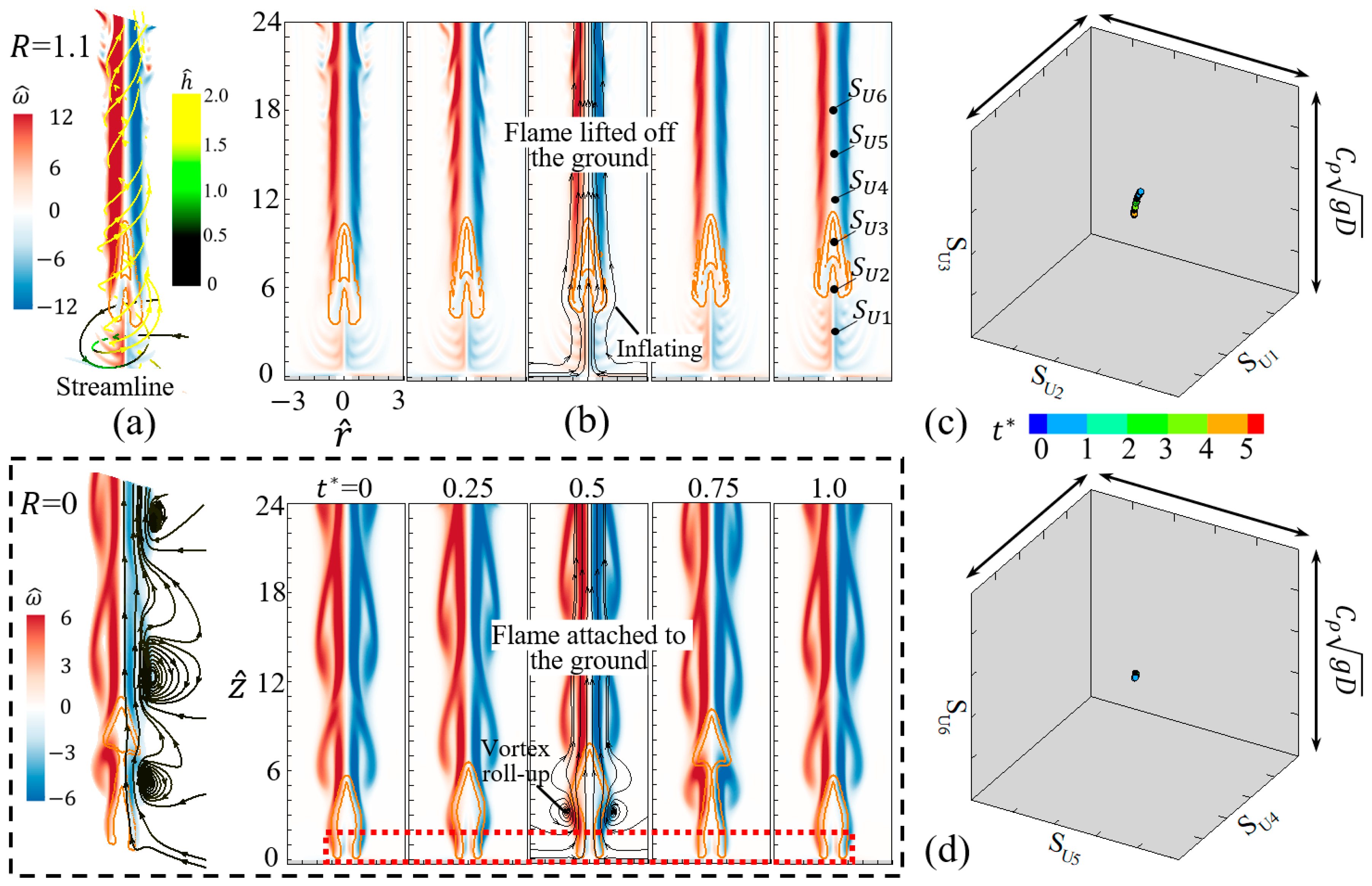
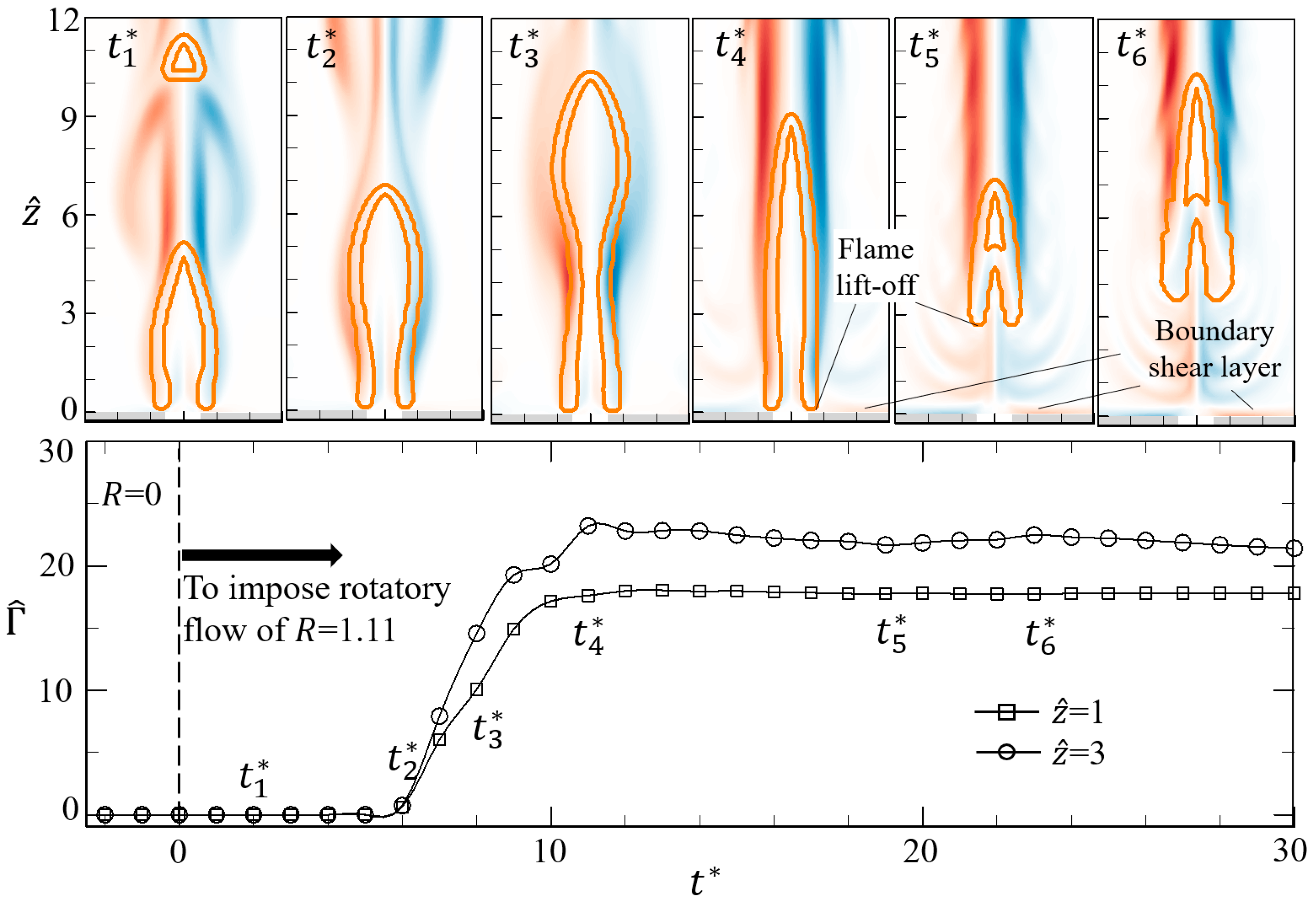
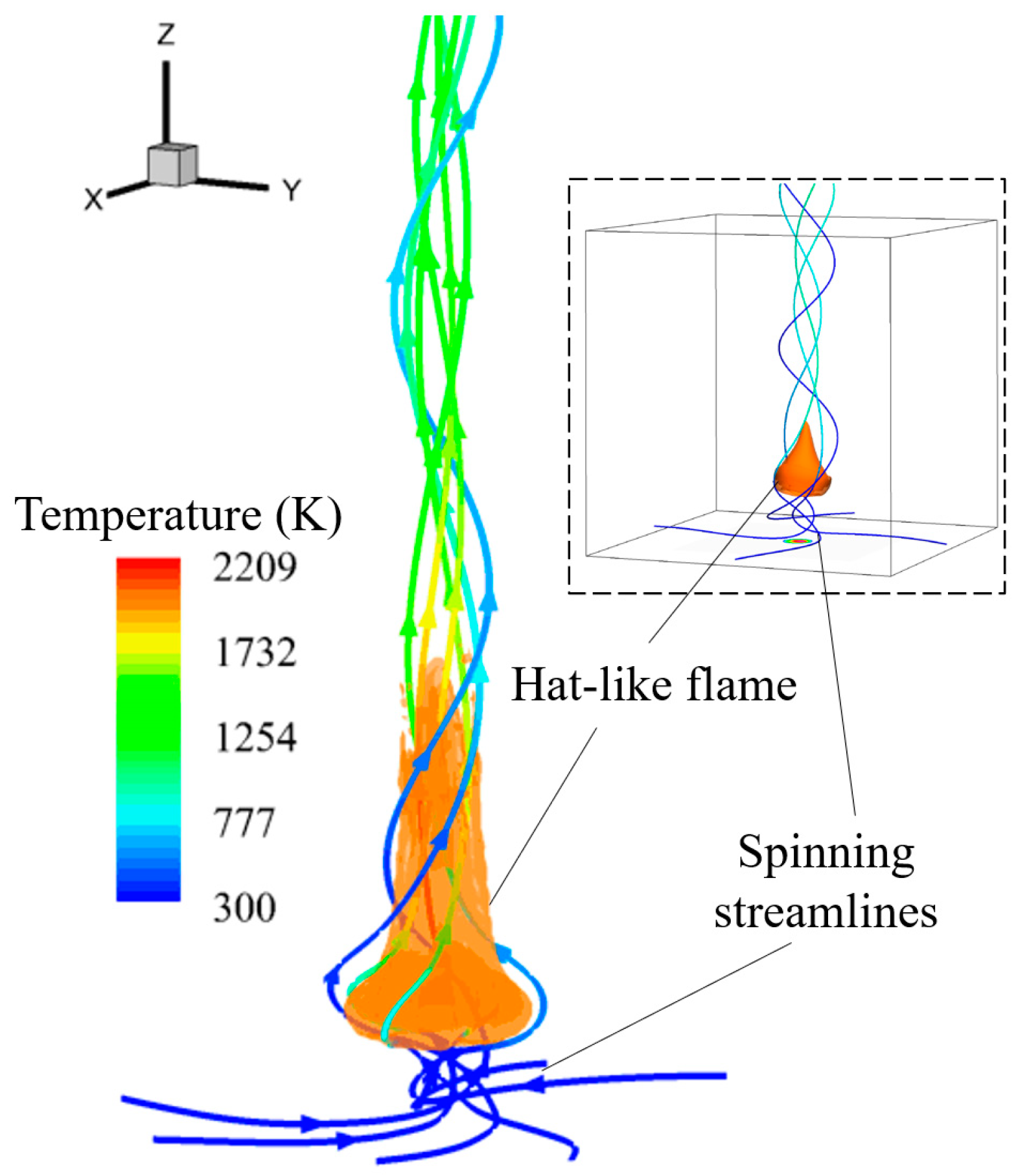
3.6. Lifted Flame
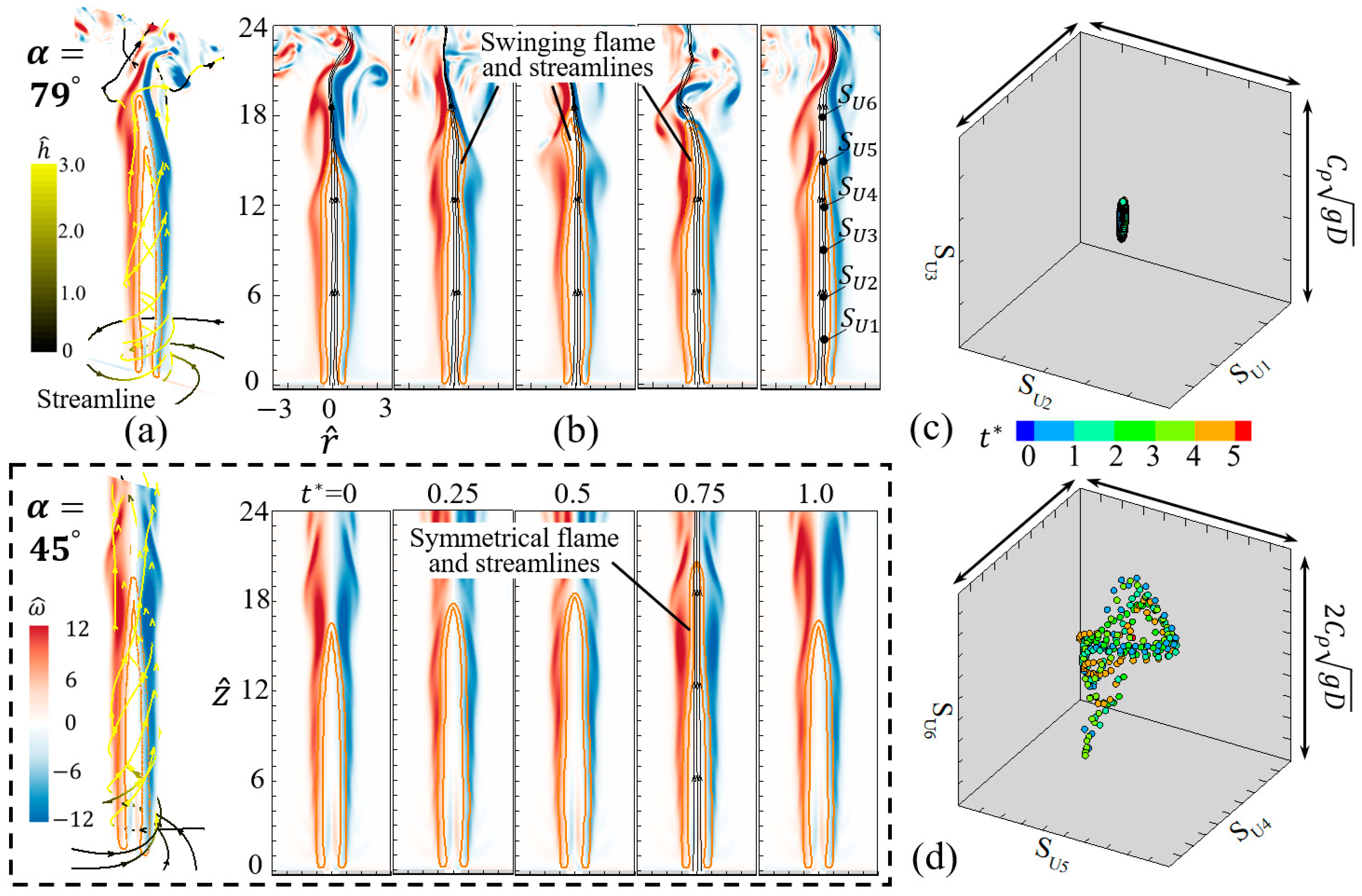
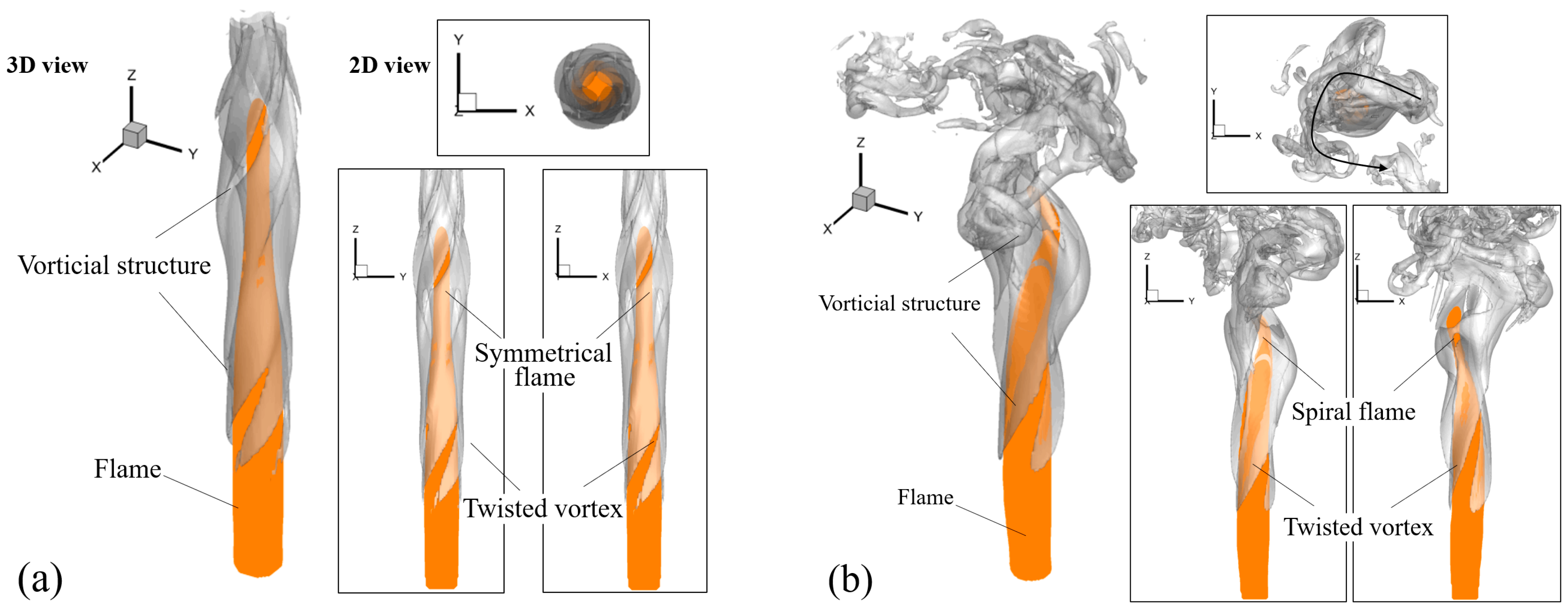
3.7. Spiral Flame
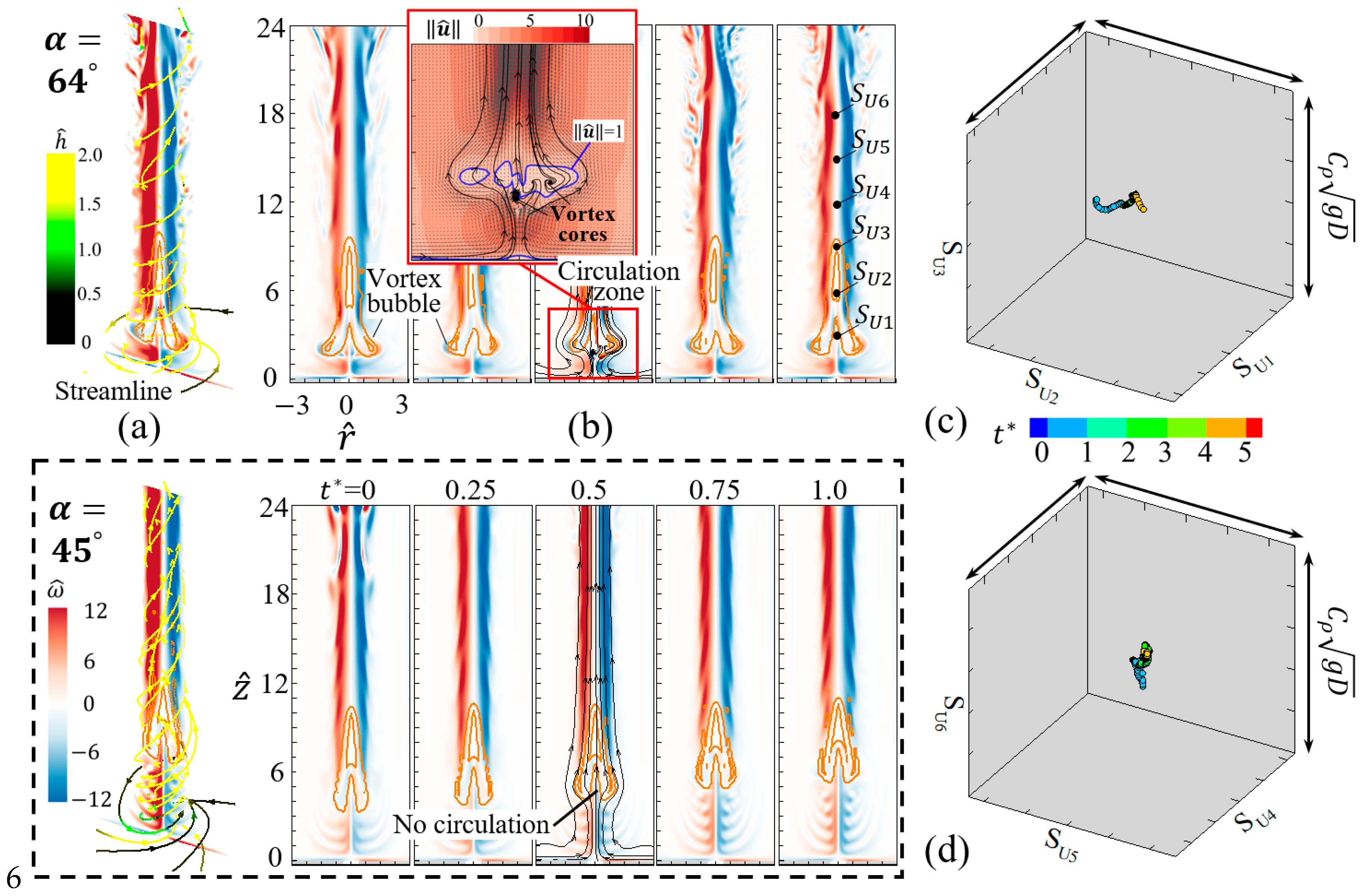
3.8. Vortex Bubble Flame
4. Concluding Remarks
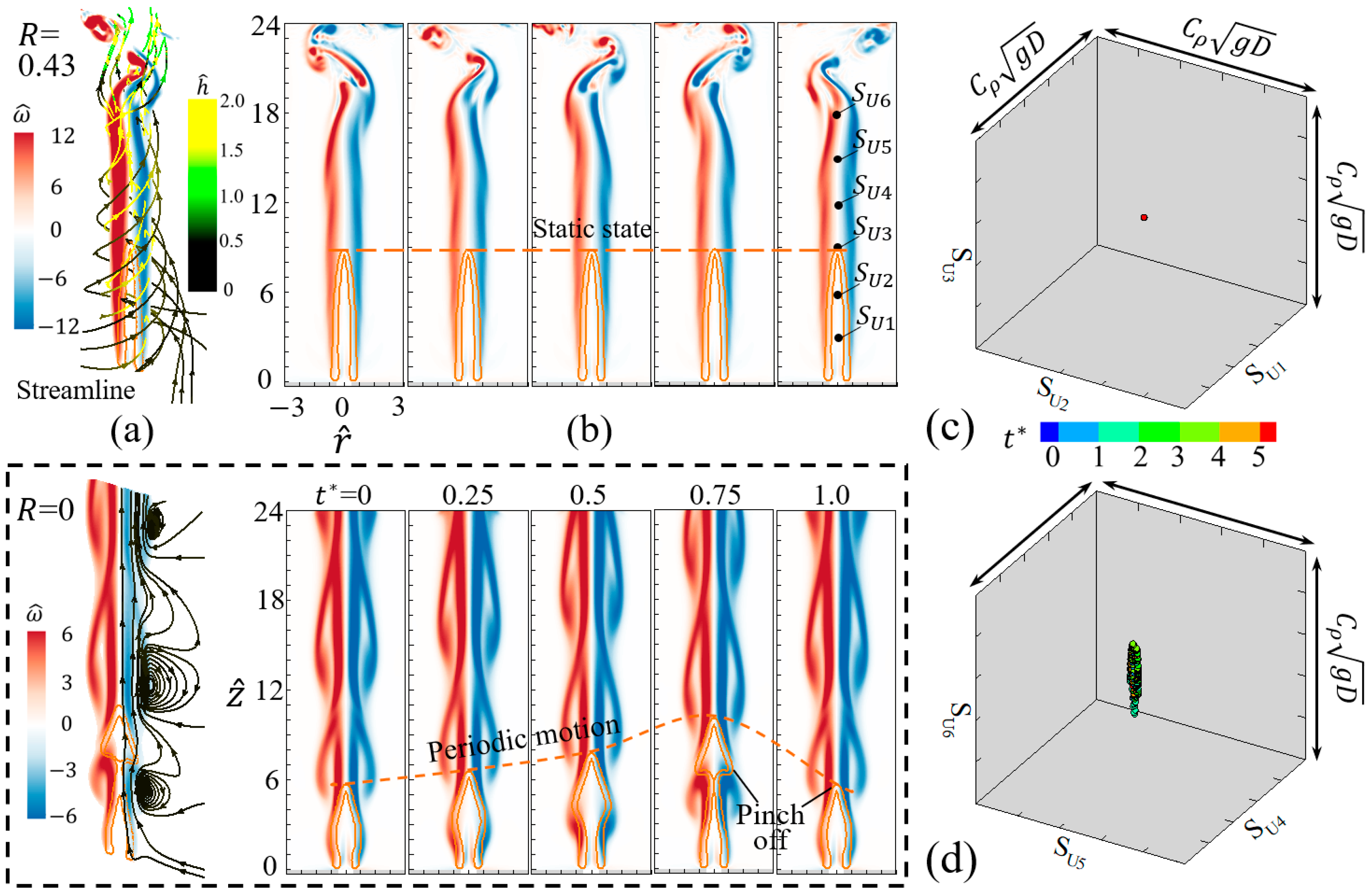
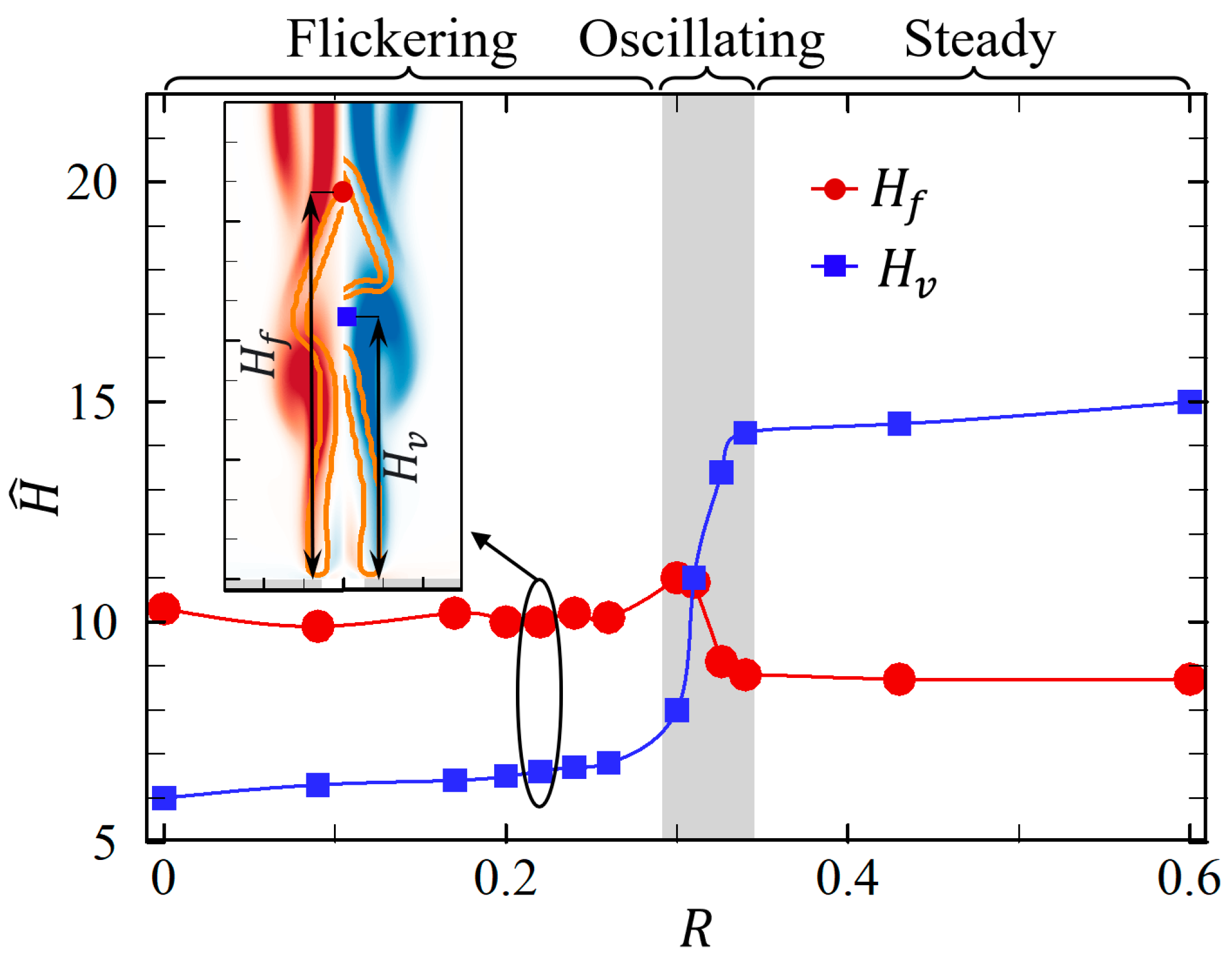
- The flickering flames have the distinct feature that the periodic shedding of the toroidal vortex around the flame. The portraits of these flames are the closed ring shape. Additionally, the topological structure of the flames is broken when the externally swirling flow is weak, for instance, the weak swirling conditions of 0.31 and in this study.
- The oscillating mode exhibits that the toroidal vortex sheds off behind the flame and occurs at the intermediate region (for instance, and in this study). The upstream portrait of these oscillating flames is the closed ring, while a big disturbance occurs in the downstream portrait.
- The steady mode hardly has the formation of a toroidal vortex around the flame, as the vortex shedding occurs far behind the flame. In the steady flames, the upstream phase portrait degenerates into a point, while the downstream portrait exhibits small oscillation. The formation of steady flames corresponds to the relevantly large region, for instance, and in this study.
- The lifted flames detach from the bottom wall due to the relatively small number. The phase portraits of the flames are nearly motionless. The present study shows that the large (>1.10) with the fixed causes a very small ratio of the residence time to the chemical time at the flame base, thereby leading to the lift-off of the flame.
- The spiral flames have a distinct feature in that the symmetry of shear layers around the flame is broken, compared with the four modes of flickering, oscillating, steady, and lifted flames. In these flames, the upstream phase portrait is a small ellipse, while the downstream portrait shows a big quasi-cycle. The asymmetric flames occur at a large , while is the same. For instance, = 0.60 and = 79° in this study.
- The vortex bubble flames show a different pattern in the occurrence of the vortex bubble for the vortex breakdown in the flame base, compared with the lifted flame. The phase portraits present a warping string within a relatively small range as the unstable bubble has time-varying barycenter and shape. These flames occur at the relatively large and ; for instance, = 1.30 and = 64° in this study.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Emmons, H.W.; Ying, S.-J. The fire whirl. Proc. Combust. Inst. 1967, 11, 475–488. [Google Scholar] [CrossRef]
- Bilger, R.W. Turbulent diffusion flames. Annu. Rev. Fluid Mech. 1989, 21, 101–135. [Google Scholar] [CrossRef]
- Tohidi, A.; Gollner, M.J.; Xiao, H. Fire whirls. Annu. Rev. Fluid Mech. 2018, 50, 187–213. [Google Scholar] [CrossRef]
- Sun, Y.; Zhao, D.; Ni, S.; David, T.; Zhang, Y. Entropy and flame transfer function analysis of a hydrogen-fueled diffusion flame in a longitudinal combustor. Energy 2020, 194, 116870. [Google Scholar] [CrossRef]
- Liu, N.; Lei, J.; Gao, W.; Chen, H.; Xie, X. Combustion dynamics of large-scale wildfires. Proc. Combust. Inst. 2021, 38, 157–198. [Google Scholar] [CrossRef]
- Ruoso, A.C.; Corrêa Bitencourt, L.; Urach Sudati, L.; Klunk, M.A.; Caetano, N.R. New parameters for the forest biomass waste ecofirewood manufacturing process optimization. Periódico Tchê Química 2019, 16, 560–571. [Google Scholar] [CrossRef]
- Yang, F.; Wang, T.; Deng, X.; Dang, J.; Huang, Z.; Hu, S.; Li, Y.; Ouyang, M. Review on hydrogen safety issues: Incident statistics, hydrogen diffusion, and detonation process. Int. J. Hydrogen Energy 2021, 46, 31467–31488. [Google Scholar] [CrossRef]
- Chamberlin, D.S.; Rose, A. The flicker of luminous flames. Proc. Combust. Inst. 1948, 1–2, 27–32. [Google Scholar] [CrossRef]
- Barr, J. Diffusion flames. Proc. Combust. Inst. 1953, 4, 765–771. [Google Scholar] [CrossRef]
- Chen, L.-D.; Seaba, J.; Roquemore, W.; Goss, L. Buoyant diffusion flames. Proc. Combust. Inst. 1989, 22, 677–684. [Google Scholar] [CrossRef]
- Sato, H.; Amagai, K.; Arai, M. Flickering frequencies of diffusion flames observed under various gravity fields. Proc. Combust. Inst. 2000, 28, 1981–1987. [Google Scholar] [CrossRef]
- Maxworthy, T. The flickering candle: Transition to a global oscillation in a thermal plume. J. Fluid Mech. 1999, 390, 297–323. [Google Scholar] [CrossRef]
- Jiang, X.; Luo, K. Combustion-induced buoyancy effects of an axisymmetric reactive plume. Proc. Combust. Inst. 2000, 28, 1989–1995. [Google Scholar] [CrossRef]
- Moreno-Boza, D.; Coenen, W.; Sevilla, A.; Carpio, J.; Sánchez, A.; Liñán, A. Diffusion-flame flickering as a hydrodynamic global mode. J. Fluid Mech. 2016, 798, 997–1014. [Google Scholar] [CrossRef]
- Moreno-Boza, D.; Coenen, W.; Carpio, J.; Sánchez, A.L.; Williams, F.A. On the critical conditions for pool-fire puffing. Combust. Flame 2018, 192, 426–438. [Google Scholar] [CrossRef]
- Wimer, N.T.; Lapointe, C.; Christopher, J.D.; Nigam, S.P.; Hayden, T.R.; Upadhye, A.; Strobel, M.; Rieker, G.B.; Hamlington, P.E. Scaling of the puffing Strouhal number for buoyant jets and plumes. J. Fluid Mech. 2020, 895, A26. [Google Scholar] [CrossRef]
- Gergely, A.; Paizs, C.; Tötös, R.; Néda, Z. Oscillations and collective behavior in convective flows. Phys. Fluids 2021, 33, 124104. [Google Scholar] [CrossRef]
- Patil, O.T.; Meehan, M.A.; Hamlington, P.E. Puffing frequency of interacting buoyant plumes. Phys. Rev. Fluids 2022, 7, L111501. [Google Scholar] [CrossRef]
- Pandey, K.; Basu, S.; Krishan, B.; Gautham, V. Dynamic self-tuning, flickering and shedding in buoyant droplet diffusion flames under acoustic excitation. Proc. Combust. Inst. 2021, 38, 3141–3149. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, Y.; Wei, Y.; Liu, S. Vortex shedding controlled combustion of the wake flame of an n-heptane wetted porous sphere. AIP Adv. 2022, 12, 105216. [Google Scholar] [CrossRef]
- Thirumalaikumaran, S.; Vadlamudi, G.; Basu, S. Insight into flickering/shedding in buoyant droplet-diffusion flame during interaction with vortex. Combust. Flame 2022, 240, 112002. [Google Scholar] [CrossRef]
- Durox, D.; Yuan, T.; Baillot, F.; Most, J. Premixed and diffusion flames in a centrifuge. Combust. Flame 1995, 102, 501–511. [Google Scholar] [CrossRef]
- Fujisawa, N.; Okuda, T. Effects of co-flow and equivalence ratio on flickering in partially premixed flame. Int. J. Heat Mass Transf. 2018, 121, 1089–1098. [Google Scholar] [CrossRef]
- Zukoski, E.; Cetegen, B.; Kubota, T. Visible structure of buoyant diffusion flames. Proc. Combust. Inst. 1985, 20, 361–366. [Google Scholar] [CrossRef]
- Buckmaster, J.; Peters, N. The infinite candle and its stability—A paradigm for flickering diffusion flames. Proc. Combust. Inst. 1988, 21, 1829–1836. [Google Scholar] [CrossRef]
- Cetegen, B.M.; Ahmed, T.A. Experiments on the periodic instability of buoyant plumes and pool fires. Combust. Flame 1993, 93, 157–184. [Google Scholar] [CrossRef]
- Sato, H.; Amagai, K.; Arai, M. Diffusion flames and their flickering motions related with Froude numbers under various gravity levels. Combust. Flame 2000, 123, 107–118. [Google Scholar] [CrossRef]
- Briones, A.M.; Aggarwal, S.K.; Katta, V.R. A numerical investigation of flame liftoff, stabilization, and blowout. Phys. Fluids 2006, 18, 043603. [Google Scholar] [CrossRef]
- Sitte, M.P.; Doan, N.A.K. Velocity reconstruction in puffing pool fires with physics-informed neural networks. Phys. Fluids 2022, 34, 087124. [Google Scholar] [CrossRef]
- Xia, X.; Zhang, P. A vortex-dynamical scaling theory for flickering buoyant diffusion flames. J. Fluid Mech. 2018, 855, 1156–1169. [Google Scholar] [CrossRef]
- Gharib, M.; Rambod, E.; Shariff, K. A universal time scale for vortex ring formation. J. Fluid Mech. 1998, 360, 121–140. [Google Scholar] [CrossRef]
- Yang, T.; Zhang, P. Faster flicker of buoyant diffusion flames by weakly rotatory flows. Theor. Comput. Fluid Dyn. 2023, 37, 781–798. [Google Scholar] [CrossRef]
- Kitahata, H.; Taguchi, J.; Nagayama, M.; Sakurai, T.; Ikura, Y.; Osa, A.; Sumino, Y.; Tanaka, M.; Yokoyama, E.; Miike, H. Oscillation and synchronization in the combustion of candles. J. Phys. Chem. A 2009, 113, 8164–8168. [Google Scholar] [CrossRef]
- Dange, S.; Pawar, S.A.; Manoj, K.; Sujith, R. Role of buoyancy-driven vortices in inducing different modes of coupled behaviour in candle-flame oscillators. AIP Adv. 2019, 9, 015119. [Google Scholar] [CrossRef]
- Bunkwang, A.; Matsuoka, T.; Nakamura, Y. Similarity of dynamic behavior of buoyant single and twin jet-flame (s). J. Therm. Sci. Technol. 2020, 15, JTST0028. [Google Scholar] [CrossRef]
- Tokami, T.; Toyoda, M.; Miyano, T.; Tokuda, I.T.; Gotoda, H. Effect of gravity on synchronization of two coupled buoyancy-induced turbulent flames. Phys. Rev. E 2021, 104, 024218. [Google Scholar] [CrossRef]
- Fujisawa, N.; Imaizumi, K.; Yamagata, T. Synchronization of dual diffusion flame in co-flow. Exp. Therm Fluid Sci. 2020, 110, 109924. [Google Scholar] [CrossRef]
- Liu, C.; Liu, X.; Ge, H.; Deng, J.; Zhou, S.; Wang, X.; Cheng, F. On the influence of distance between two jets on flickering diffusion flames. Combust. Flame 2019, 201, 23–30. [Google Scholar]
- Okamoto, K.; Kijima, A.; Umeno, Y.; Shima, H. Synchronization in flickering of three-coupled candle flames. Sci. Rep. 2016, 6, 36145. [Google Scholar] [CrossRef] [PubMed]
- Yang, T.; Chi, Y.; Zhang, P. Vortex interaction in triple flickering buoyant diffusion flames. Proc. Combust. Inst. 2022, 39, 1893–1903. [Google Scholar] [CrossRef]
- Chi, Y.; Yang, T.; Zhang, P. Dynamical mode recognition of triple flickering buoyant diffusion flames in Wasserstein space. Combust. Flame 2023, 248, 112526. [Google Scholar] [CrossRef]
- Forrester, D.M. Arrays of coupled chemical oscillators. Sci. Rep. 2015, 5, 16994. [Google Scholar] [CrossRef]
- Xia, X.; Fu, C.; Yang, Y.; Yang, X.; Gao, Y.; Qi, F. Vortex formation and frequency tuning of periodically-excited jet diffusion flames. Proc. Combust. Inst. 2021, 38, 2067–2074. [Google Scholar] [CrossRef]
- Wang, S.; Zheng, J.; Xu, L.; An, Q.; Han, X.; Zhang, C.; Li, L.; Xia, X.; Qi, F. Experimental investigation of the helical mode in a stratified swirling flame. Combust. Flame 2022, 244, 112268. [Google Scholar] [CrossRef]
- Battaglia, F.; Rehm, R.G.; Baum, H.R. The fluid mechanics of fire whirls: An inviscid model. Phys. Fluids 2000, 12, 2859–2867. [Google Scholar] [CrossRef]
- Lei, J.; Liu, N.; Satoh, K. Buoyant pool fires under imposed circulations before the formation of fire whirls. Proc. Combust. Inst. 2015, 35, 2503–2510. [Google Scholar] [CrossRef]
- Coenen, W.; Kolb, E.J.; Sánchez, A.L.; Williams, F.A. Observed dependence of characteristics of liquid-pool fires on swirl magnitude. Combust. Flame 2019, 205, 1–6. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, H.; Xia, X.; Zhang, P.; Qi, F. An experimental study of the blue whirl onset. Proc. Combust. Inst. 2022, 39, 3705–3714. [Google Scholar] [CrossRef]
- Ju, X.; Mizuno, M.; Matsuoka, T.; Yamazaki, T.; Kuwana, K.; Nakamura, Y. Effect of circulation on flame heights over liquid fuel pools. Combust. Flame 2022, 246, 112435. [Google Scholar] [CrossRef]
- Candel, S.; Durox, D.; Schuller, T.; Bourgouin, J.-F.; Moeck, J.P. Dynamics of swirling flames. Annu. Rev. Fluid Mech. 2014, 46, 147–173. [Google Scholar] [CrossRef]
- Chuah, K.H.; Kushida, G. The prediction of flame heights and flame shapes of small fire whirls. Proc. Combust. Inst. 2007, 31, 2599–2606. [Google Scholar] [CrossRef]
- Gotoda, H.; Ueda, T.; Shepherd, I.G.; Cheng, R.K. Flame flickering frequency on a rotating Bunsen burner. Chem. Eng. Sci. 2007, 62, 1753–1759. [Google Scholar] [CrossRef]
- Gotoda, H.; Asano, Y.; Chuah, K.H.; Kushida, G. Nonlinear analysis on dynamic behavior of buoyancy-induced flame oscillation under swirling flow. Int. J. Heat Mass Transf. 2009, 52, 5423–5432. [Google Scholar] [CrossRef]
- Lei, J.; Liu, N.; Jiao, Y.; Zhang, S. Experimental investigation on flame patterns of buoyant diffusion flame in a large range of imposed circulations. Proc. Combust. Inst. 2017, 36, 3149–3156. [Google Scholar] [CrossRef]
- McGrattan, K.; Hostikka, S.; McDermott, R.; Floyd, J.; Weinschenk, C.; Overholt, K. Fire dynamics simulator user’s guide. NIST Spec. Publ. 2013, 1019, 1–339. [Google Scholar]
- Yang, T.; Xia, X.; Zhang, P. Vortex-dynamical interpretation of anti-phase and in-phase flickering of dual buoyant diffusion flames. Phys. Rev. Fluids 2019, 4, 053202. [Google Scholar] [CrossRef]
- Linán, A.; Vera, M.; Sánchez, A.L. Ignition, liftoff, and extinction of gaseous diffusion flames. Annu. Rev. Fluid Mech. 2015, 47, 293–314. [Google Scholar] [CrossRef]
- Mullen, J.B.; Maxworthy, T. A laboratory model of dust devil vortices. Dyn. Atmos. Ocean. 1977, 1, 181–214. [Google Scholar] [CrossRef]
- Kolb, E.J. An Experimental Approach to the Blue Whirl; University of California: San Diego, CA, USA, 2018. [Google Scholar]
- Westbrook, C.K.; Dryer, F.L. Chemical kinetic modeling of hydrocarbon combustion. Prog. Energy Combust. Sci. 1984, 10, 1–57. [Google Scholar] [CrossRef]
- Fang, J.; Wang, J.-W.; Guan, J.-F.; Zhang, Y.-M.; Wang, J.-J. Momentum-and buoyancy-driven laminar methane diffusion flame shapes and radiation characteristics at sub-atmospheric pressures. Fuel 2016, 163, 295–303. [Google Scholar] [CrossRef]
- Hamins, A.; Yang, J.; Kashiwagi, T. An experimental investigation of the pulsation frequency of flames. Proc. Combust. Inst. 1992, 24, 1695–1702. [Google Scholar] [CrossRef]
- Abe, H.; Ito, A.; Torikai, H. Effect of gravity on puffing phenomenon of liquid pool fires. Proc. Combust. Inst. 2015, 35, 2581–2587. [Google Scholar] [CrossRef]
- Sahu, K.; Kundu, A.; Ganguly, R.; Datta, A. Effects of fuel type and equivalence ratios on the flickering of triple flames. Combust. Flame 2009, 156, 484–493. [Google Scholar] [CrossRef]
- Darabkhani, H.G.; Bassi, J.; Huang, H.; Zhang, Y. Fuel effects on diffusion flames at elevated pressures. Fuel 2009, 88, 264–271. [Google Scholar] [CrossRef]
- Bahadori, M.; Zhou, L.; Stocker, D.; Hegde, U. Functional dependence of flame flicker on gravitational level. AIAA J. 2001, 39, 1404–1406. [Google Scholar] [CrossRef]
- Zhang, H.; Xia, X.; Gao, Y. Instability transition of a jet diffusion flame in quiescent environment. Proc. Combust. Inst. 2021, 38, 4971–4978. [Google Scholar] [CrossRef]
- Cetegen, B.; Dong, Y. Experiments on the instability modes of buoyant diffusion flames and effects of ambient atmosphere on the instabilities. Exp. Fluids 2000, 28, 546–558. [Google Scholar] [CrossRef]
- Lawson, J.M.; Dawson, J.R. The formation of turbulent vortex rings by synthetic jets. Phys. Fluids 2013, 25, 105113. [Google Scholar] [CrossRef]
- Xia, X.; Mohseni, K. Far-field momentum flux of high-frequency axisymmetric synthetic jets. Phys. Fluids 2015, 27, 115101. [Google Scholar] [CrossRef]
- Hall, M. Vortex breakdown. Annu. Rev. Fluid Mech. 1972, 4, 195–218. [Google Scholar] [CrossRef]
- Lucca-Negro, O.; O’doherty, T. Vortex breakdown: A review. Prog. Energy Combust. Sci. 2001, 27, 431–481. [Google Scholar] [CrossRef]
- Chung, J.D.; Zhang, X.; Kaplan, C.R.; Oran, E.S. The structure of the blue whirl revealed. Sci. Adv. 2020, 6, eaba0827. [Google Scholar] [CrossRef]
- Xiao, H.; Gollner, M.J.; Oran, E.S. From fire whirls to blue whirls and combustion with reduced pollution. Proc. Natl. Acad. Sci. USA 2016, 113, 9457–9462. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y.; Hariharan, S.B.; Qi, H.; Gollner, M.J.; Oran, E.S. Conditions for formation of the blue whirl. Combust. Flame 2019, 205, 147–153. [Google Scholar] [CrossRef]
- Carpio, J.; Coenen, W.; Sánchez, A.; Oran, E.; Williams, F. Numerical description of axisymmetric blue whirls over liquid-fuel pools. Proc. Combust. Inst. 2021, 38, 2041–2048. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, T.; Ma, Y.; Zhang, P. Dynamical Behavior of Small-Scale Buoyant Diffusion Flames in Externally Swirling Flows. Symmetry 2024, 16, 292. https://doi.org/10.3390/sym16030292
Yang T, Ma Y, Zhang P. Dynamical Behavior of Small-Scale Buoyant Diffusion Flames in Externally Swirling Flows. Symmetry. 2024; 16(3):292. https://doi.org/10.3390/sym16030292
Chicago/Turabian StyleYang, Tao, Yuan Ma, and Peng Zhang. 2024. "Dynamical Behavior of Small-Scale Buoyant Diffusion Flames in Externally Swirling Flows" Symmetry 16, no. 3: 292. https://doi.org/10.3390/sym16030292
APA StyleYang, T., Ma, Y., & Zhang, P. (2024). Dynamical Behavior of Small-Scale Buoyant Diffusion Flames in Externally Swirling Flows. Symmetry, 16(3), 292. https://doi.org/10.3390/sym16030292




